Power Quality Improvement through a UPQC and a Resonant Observer-Based MIMO Control Strategy
Abstract
:1. Introduction
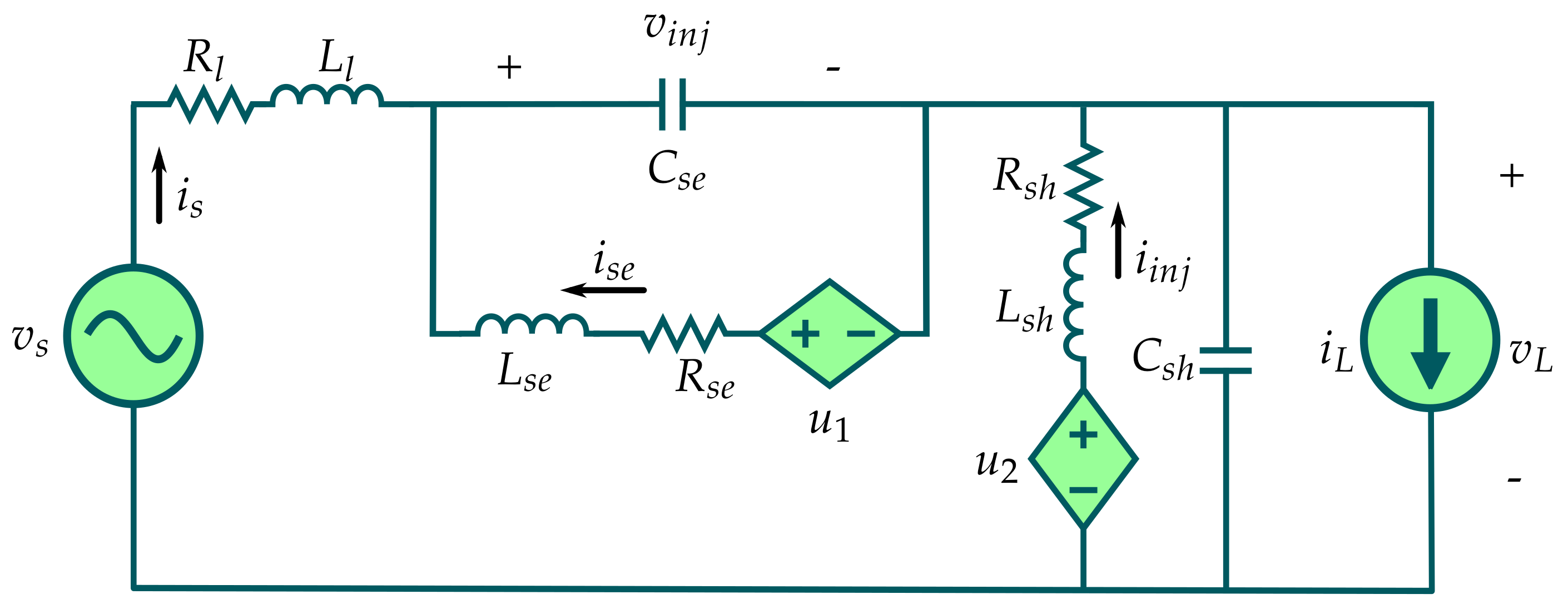
2. System Model Description
2.1. UPQC Stages and Continuous-Time Model
2.2. UPQC Discrete-Time System Model
2.3. DC-Link Discrete-Time Model
2.4. Sampling Period Selection
3. Control System Design
3.1. Control Objectives
- The feedback control must track desired pure sinusoidal reference signals so that the grid current and the load voltage can be free-distorted. Similarly, as the disturbance vector causes harmonic content on the controlled variables, the feedback control system must reject the grid voltage and the load current. According to the IEEE-519-2014 standard, the THD index for the voltage and the current (with low current consumption on the common connectionpPoint) must achieve the condition:where F depicts either a voltage or a current signal. is the magnitude for the ith harmonic and is the magnitude of the fundamental component.
- The power factor on the grid side must be 1. Therefore, the reference vector is defined with the variable as the grid voltage phase. The load voltage should have the same phase as to avoid phase jumps in loads. The current reference must have the same phase of to achieve the desired power factor correction. The parameter is the grid fundamental frequency. As a result, the reference signals are defined as
- The control system must achieve the steady state in a time less than a half sinusoidal cycle. This condition assures that the sags and swells will be imperceptible on the connected load according to the definition of a sag or swell in the IEEE-1159-2019 standard. Defining the settling time of the control system as , the transient time objective should beSimilarly, during a sag or swell event, the load voltage amplitude must be in the interval of following the same IEEE standard.
- To avoid saturation in the PWM interfaces, the control signals must not exceed the interval limit (Table 1 lists the numerical value of ). For the case of , the available magnitude is within .
- The DC-link voltage must remain at a constant voltage and the settling time for this stage should be 10 to 100 times lower than [28]. It implies that the DC-link variations due to transient events could be seen as static in the UPQC closed-loop dynamics.
3.2. Control Architecture
3.3. Resonant Extended State Observer Design
3.4. State Feedback Design
3.5. Control Law and Closed-Loop Dynamics
3.6. PI Control for DC Link
4. Experimental Setup and Results
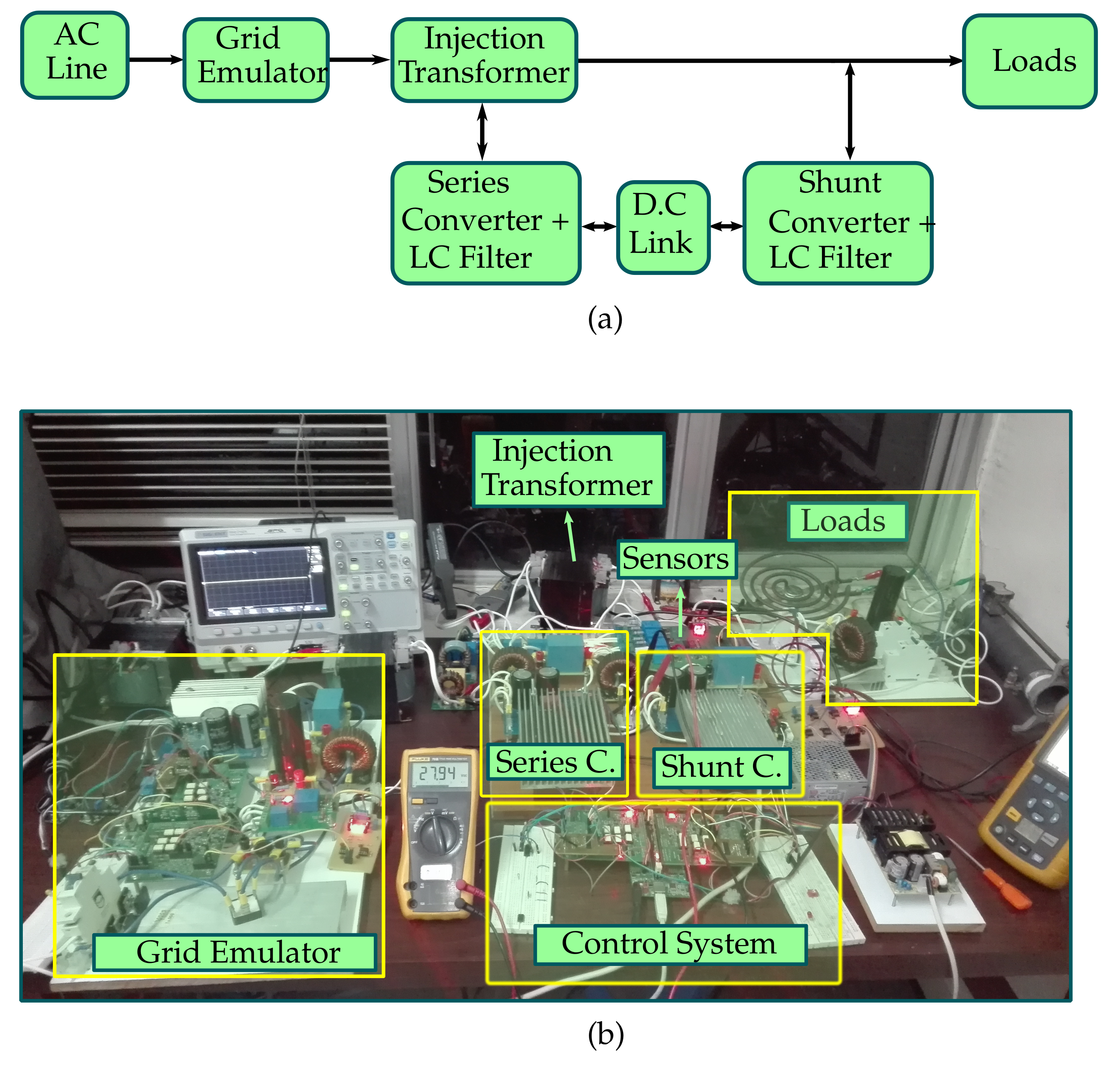
4.1. Experimental Setup Description
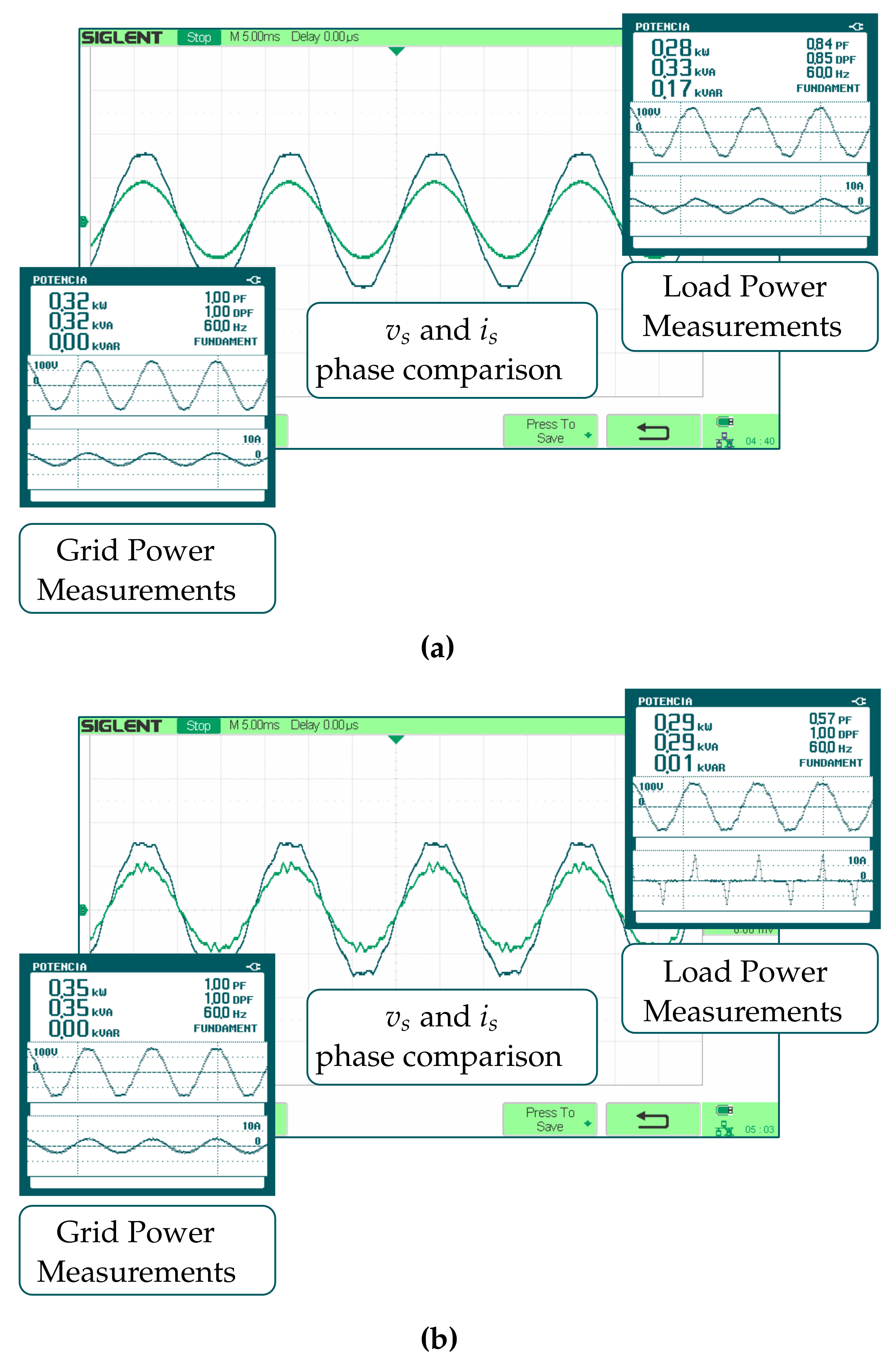
4.2. Harmonics Compensation
4.3. Power Factor Compensation
4.4. Sags and Swells Compensation
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- IEEE Standard 1159-2019—IEEE Recommended Practice for Monitoring Electric Power Quality; IEEE: New York, NY, USA, 2019; pp. 1–98.
- Chandrasekaran, K.; Ramachandaramurthy, V. An improved Dynamic Voltage Restorer for power quality improvement. Int. J. Electric. Power Energy Syst. 2016, 82, 354–362. [Google Scholar] [CrossRef]
- Moghassemi, A.; Padmanaban, S. Dynamic Voltage Restorer (DVR): A Comprehensive Review of Topologies, Power Converters, Control Methods, and Modified Configurations. Energies 2020, 13, 4152. [Google Scholar] [CrossRef]
- Jiang, S.; Cui, G.; Cao, L.; Li, C. Design of H∞ robust control for single-phase shunt Active Power Filters. In Proceedings of the 2008 IEEE 7th World Congress on Intelligent Control and Automation, Chongqing, China, 25–27 June 2008; pp. 4639–4642. [Google Scholar] [CrossRef]
- Wu, Z.; Zhang, G. Research on sliding mode control based on exact feedback linearization for single-phase shunt APF. In Proceedings of the 2016 IEEE 8th International Power Electronics and Motion Control Conference (IPEMC-ECCE Asia), Hefei, China, 22–26 May 2016; pp. 1350–1356. [Google Scholar] [CrossRef]
- Ghosh, A.; Ledwich, G. A unified power quality conditioner (UPQC) for simultaneous voltage and current compensation. Electr. Power Syst. Res. 2001, 59, 55–63. [Google Scholar] [CrossRef]
- Li, P.; Li, Y.; Yin, Z. Realization of UPQC H∞ coordinated control in Microgrid. Int. J. Electr. Power Energy Syst. 2015, 65, 443–452. [Google Scholar] [CrossRef]
- Trinh, Q.N.; Lee, H.H. Improvement of unified power quality conditioner performance with enhanced resonant control strategy. IET Gener. Transm. Distrib. 2014, 8, 2114–2123. [Google Scholar] [CrossRef]
- Trinh, Q.N.; Lee, H.H. Versatile UPQC Control System with a Modified Repetitive Controller under Nonlinear and Unbalanced Loads. J. Power Electron. 2015, 15, 1093–1104. [Google Scholar] [CrossRef] [Green Version]
- Tran, T.V.; Kim, K.H.; Lai, J.S. Optimized Active Disturbance Rejection Control with Resonant Extended State Observer for Grid Voltage Sensorless LCL -Filtered Inverter. IEEE Trans. Power Electron. 2021, 36, 13317–13331. [Google Scholar] [CrossRef]
- Errouissi, R.; Shareef, H.; Awwad, F. Disturbance Observer-Based Control for Three-Phase Grid-Tied Inverter with LCL Filter. IEEE Trans. Ind. Appl. 2021, 57, 5411–5424. [Google Scholar] [CrossRef]
- Errouissi, R.; Shareef, H.; Wahyudie, A. A Novel Design of PR Controller with Antiwindup Scheme for Single-Phase Interconnected PV Systems. IEEE Trans. Ind. Appl. 2021, 57, 5461–5475. [Google Scholar] [CrossRef]
- Xin, Q.; Wang, C.; Chen, C.Y.; Yang, G.; Chen, L. Robust Vibration Control Based on Rigid-Body State Observer for Modular Joints. Machines 2021, 9, 194. [Google Scholar] [CrossRef]
- Chandrakala Devi, S.; Singh, B.; Devassy, S. Modified generalised integrator based control strategy for solar PV fed UPQC enabling power quality improvement. IET Gener. Transm. Distrib. 2020, 14, 3127–3138. [Google Scholar] [CrossRef]
- Rajendran, M. Comparison of Various Control Strategies for UPQC to Mitigate PQ Issues. J. Inst. Eng. Ser. B 2021, 102, 19–29. [Google Scholar] [CrossRef]
- Alam, S.J.; Arya, S.R. Control of UPQC based on steady state linear Kalman filter for compensation of power quality problems. Chin. J. Electr. Eng. 2020, 6, 52–65. [Google Scholar] [CrossRef]
- IEEE Standard 519-2014—IEEE Recommended Practice and Requirements for Harmonic Control in Electric Power Systems; IEEE: New York, NY, USA, 2014; pp. 1–29.
- IEEE Std 1547-2018 (Revision of IEEE Standard 1547-2003). IEEE Standard for Interconnection and Interoperability of Distributed Energy Resources with Associated Electric Power Systems Interfaces; IEEE: New York, NY, USA, 2018; pp. 1–138. [Google Scholar] [CrossRef]
- International Electrotechnical Commission. Electromagnetic Compatibility (EMC)—Part 3-2: Limits—Limits for Harmonic Current Emissions (Equipment Input Current < 16 A per phase); International Standard IEC 61000-3-2:2018 + AMD1:2020; International Electrotechnical Commission: Geneva, Switzerland, 2020. [Google Scholar]
- Bueno-Contreras, H. Control system design of a quality power compensator. Master’s Thesis, Universidad Nacional de Colombia, Bogotá, Colombia, 2020. [Google Scholar]
- Kwan, K.H.; Chu, Y.C.; So, P.L. Model-Based H∞ Control of a Unified Power Quality Conditioner. IEEE Trans. Ind. Electron. 2009, 56, 2493–2504. [Google Scholar] [CrossRef]
- Patidar, R.D.; Singh, S.P. Harmonics estimation and modeling of residential and commercial loads. In Proceedings of the 2009 IEEE International Conference on Power Systems, Kharagpur, India, 27–29 December 2009; pp. 1–6. [Google Scholar] [CrossRef]
- Ruiz, J.; Ortiz, F. Metodologías para Identificar Fuentes Armónicas en Sistemas Eléctricos. Bachelor’s Thesis, Universidad Tecnológica de Pereira, Pereira, Colombia, 2007. [Google Scholar]
- Chen, C.T. Analog and Digital Control System Design: Transfer-Function, State-Space, and Algebraic Methods; Oxford Series in Electrical and Computer Engineering; Saunders College Pub.: Rochester, NY, USA, 1993. [Google Scholar]
- Lai, R.-S.; Ngo, K. A PWM method for reduction of switching loss in a full-bridge inverter. IEEE Trans. Power Electron. 1995, 10, 326–332. [Google Scholar] [CrossRef]
- Buso, S.; Mattavelli, P. Digital Control in Power Electronics; Morgan & Claypool: San Rafael, CA, USA, 2006; Volume 1, pp. 1–158. [Google Scholar] [CrossRef] [Green Version]
- Corradini, L.; Maksimovic, D.; Mattavelli, P.; Zane, R. Digital Control of High-Frequency Switched-Mode Power Converters; IEEE Press Series on Power Engineering; Wiley: Hoboken, NJ, USA, 2015. [Google Scholar]
- Melo, I. Diseño, Implementación y Evaluación de Diferentes Estrategias de Control Orientadas al Rechazo Activo de Perturbaciones para un Rectificador PFC que Permitan Obtener una alta Calidad de Energía Eléctrica Medida Desde los Parámetros de PF y THD de Corrient. Master’s Thesis, Universidad Nacional de Colombia, Bogotá, Colombia, 2015. [Google Scholar]
- Bueno-Contreras, H.; Ramos, G.A.; Costa-Castelló, R. Robust H∞ Design for Resonant Control in a CVCF Inverter Application over Load Uncertainties. Electronics 2020, 9, 66. [Google Scholar] [CrossRef] [Green Version]
- Ramos, G.A.; Ruget, R.I.; Costa-Castelló, R. Robust Repetitive Control of Power Inverters for Standalone Operation in DG Systems. IEEE Trans. Energy Convers. 2020, 35, 237–247. [Google Scholar] [CrossRef]
- Ramos, G.; Costa-Castelló, R. Comparison of Different Repetitive Control Architectures: Synthesis and Comparison. Application to VSI Converters. Electronics 2018, 7, 446. [Google Scholar] [CrossRef] [Green Version]
- McGrath, B.P.; Holmes, D.G. Accurate state space realisations of resonant filters for high performance inverter control applications. In Proceedings of the 2016 IEEE 2nd Annual Southern Power Electronics Conference (SPEC), Auckland, New Zealand, 5–8 December 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Ramos, G.A.; Costa-Castelló, R.; Cortés-Romero, J. LPV Observer-Based Strategy for Rejection of Periodic Disturbances with Time-Varying Frequency. Math. Probl. Eng. 2015, 2015, 380609. [Google Scholar] [CrossRef]
- Cortes, J.; Ramos, G.; Costa, R. Discrete-Time Resonant Observer Based Control for Periodic Signal Rejection. IEEE Lat. Am. Trans. 2015, 13, 1279–1285. [Google Scholar] [CrossRef] [Green Version]
- Mathworks Inc. Linear-Quadratic (LQ) State-Feedback Regulator for Discrete-Time State-Space System; The MathWorks, Inc.: Natick, MA, USA, 2021. [Google Scholar]
- Tien, D.; Gono, R.; Leonowicz, Z. A Multifunctional Dynamic Voltage Restorer for Power Quality Improvement. Energies 2018, 11, 1351. [Google Scholar] [CrossRef] [Green Version]
- ON Semiconductor. STK581U3C2D-E Application Note. Application Note, ON Semiconductor 2014. Available online: http://www.micro-semiconductor.sg/datasheet/8f-STK581U3C2D-E.pdf (accessed on 10 October 2021).
- Texas Instruments. TMS320x2833x, TMS320x2823x Technical Reference Manual. Tms320x2833x Datasheet. 2020. Available online: https://www.ti.com/lit/ug/sprui07/sprui07.pdf?ts=1634725545896 (accessed on 10 October 2021).

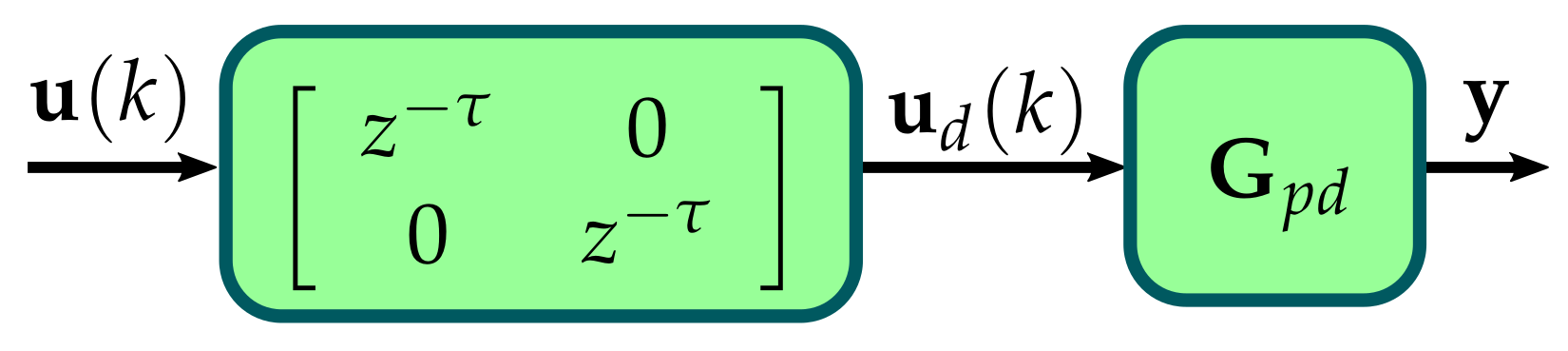
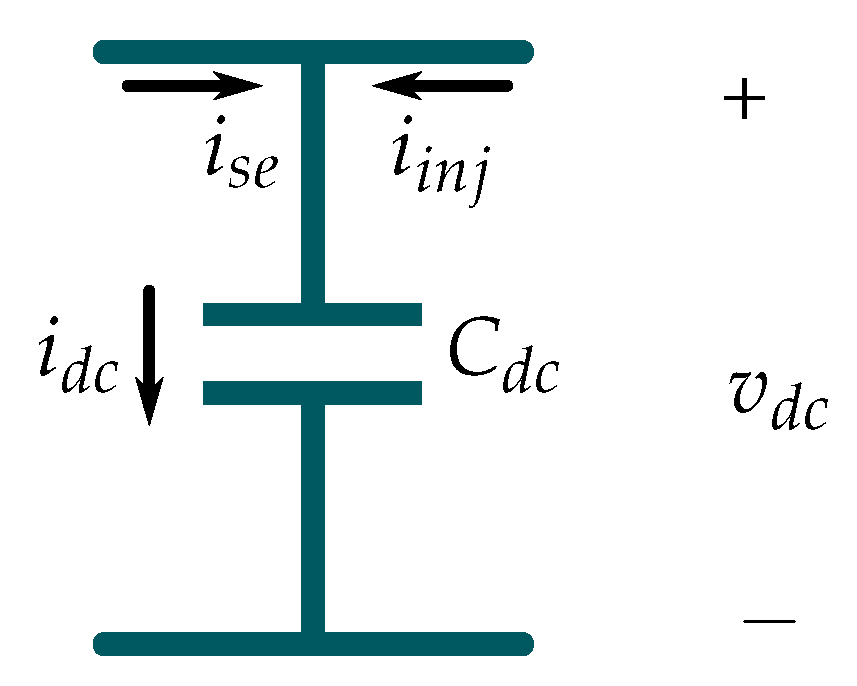




| Category | Parameter | Symbol | Value |
|---|---|---|---|
| UPQC parameters | Line Inductance | 700 H | |
| Line Resistance | 2 | ||
| Filters inductance | ; | 1.365 mH | |
| Filters resistances | ; | 0.85 | |
| Filters capacitance | ; | 40 F | |
| System parameters | DC link equivalent capacitance | 1.88 mF | |
| DC link desired voltage | 220 V | ||
| and voltage amplitude | ; | 110 | |
| Fundamental frequency | 60 Hz | ||
| Sampling Frequency | 10.2 KHz | ||
| Switching frequency | 18 KHz | ||
| Number of resonators | ; | 7 | |
| delay samples | 2 | ||
| Tuning values | tuning value | 0.0001 | |
| UPQC states weighing value | a | 10 | |
| UPQC delay states weighting value | b | 2 | |
| Weighting value for the resonators | 0.001 | ||
| error vector weighting value | 0.1 | ||
| state feedback states weighting value | 5 | ||
| Control signal weighting value | 10 | ||
| Proportional value for the PI control | P | 0.1184 | |
| Integral value for the PI control | I | 0.2239 |
| Item | Description | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| THD | RMS | THD | RMS | THD | RMS | THD | RMS | ||
| (%) | (V) | (%) | (A) | (%) | (V) | (%) | (A) | ||
| R_30 | Resistive load of 30 | 10.3 | 102 | 3.1 | 3.78 | 0.8 | 110.1 | 1.2 | 4.9 |
| R_50 | Resistive load of 50 | 2.4 | 112 | 2 | 2.08 | 0.8 | 110.1 | 3.5 | 2.24 |
| RL_30 | RL load of R = 30 L = 35 mH | 4.2 | 111.3 | 16.9 | 2.88 | 1.4 | 110.1 | 2.4 | 2.76 |
| RNL_50 | Nonlinear load with R = 50 | 5.4 | 105.4 | 78.7 | 6.56 | 2.6 | 110.1 | 3.7 | 5.24 |
| RNL_80 | Nonlinear load with R = 80 | 4.3 | 109.8 | 80.8 | 4.49 | 2.5 | 110.1 | 4.8 | 3.06 |
| Item | Description | Grid | Load | |||
|---|---|---|---|---|---|---|
| Power | PF | Power | PF | |||
| (W) | (W) | (VAR) | ||||
| R_30 | Resistive Load R = 30_ | 530 | 1 | 400 | 0 | 1 |
| R_50 | Resistive Load R = 50_ | 260 | 1 | 230 | 0 | 1 |
| RL_30 | RL Load R = 30 y L = 35 mH | 320 | 1 | 280 | 170 | 0.84 |
| RL_50 | RL Load R = 30 y L = 35 mH | 210 | 1 | 180 | 70 | 0.93 |
| RNL_50 | Nonlinear load R = 50 | 580 | 1 | 411 | 593 | 0.57 |
| RNL_80 | Carga No Lineal R = 80 | 350 | 1 | 282 | 407 | 0.57 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bueno-Contreras, H.; Ramos, G.A.; Costa-Castelló, R. Power Quality Improvement through a UPQC and a Resonant Observer-Based MIMO Control Strategy. Energies 2021, 14, 6938. https://doi.org/10.3390/en14216938
Bueno-Contreras H, Ramos GA, Costa-Castelló R. Power Quality Improvement through a UPQC and a Resonant Observer-Based MIMO Control Strategy. Energies. 2021; 14(21):6938. https://doi.org/10.3390/en14216938
Chicago/Turabian StyleBueno-Contreras, Holman, Germán Andrés Ramos, and Ramon Costa-Castelló. 2021. "Power Quality Improvement through a UPQC and a Resonant Observer-Based MIMO Control Strategy" Energies 14, no. 21: 6938. https://doi.org/10.3390/en14216938
APA StyleBueno-Contreras, H., Ramos, G. A., & Costa-Castelló, R. (2021). Power Quality Improvement through a UPQC and a Resonant Observer-Based MIMO Control Strategy. Energies, 14(21), 6938. https://doi.org/10.3390/en14216938







