Design of Nonlinear Backstepping Double-Integral Sliding Mode Controllers to Stabilize the DC-Bus Voltage for DC–DC Converters Feeding CPLs
Abstract
:1. Introduction
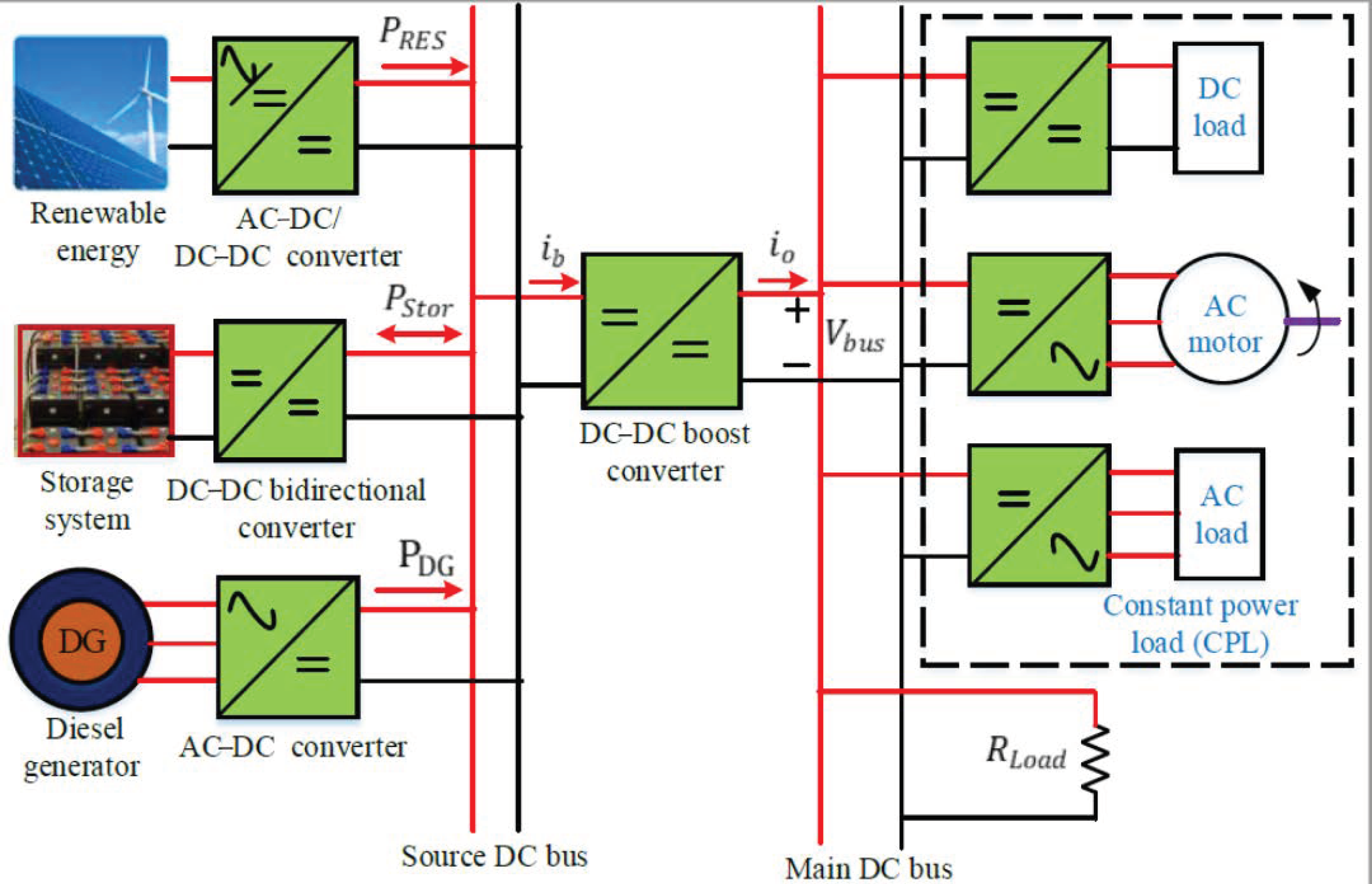
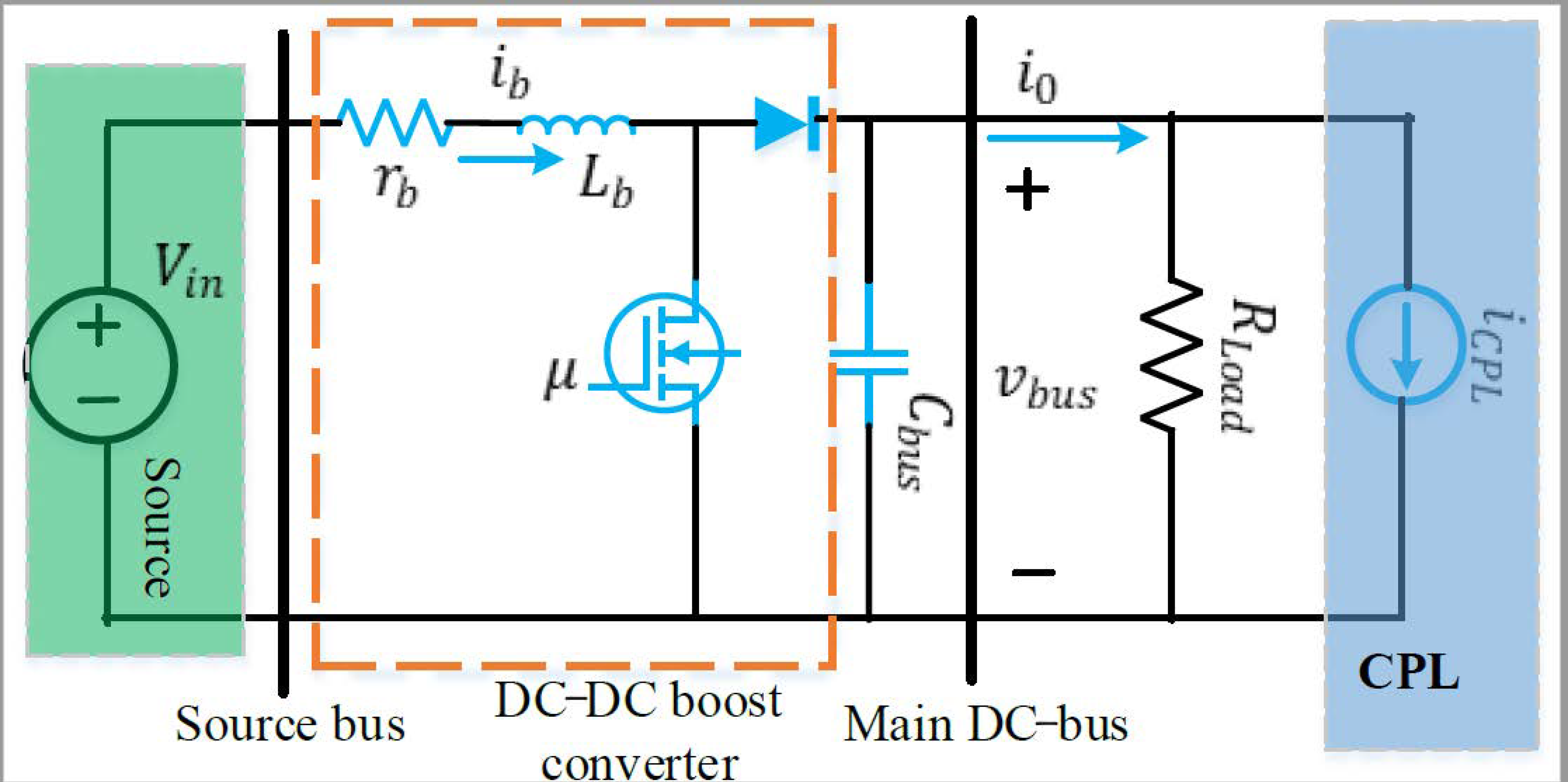
2. System Description and Its Dynamical Model
3. Proposed Controller Design Approach
- Transformation of the model into an exactly linearized system using an exact feedback linearization approach and making it suitable to apply the backstepping control approach;
- Elimination of the non-minimum phase problem of DDBCs by considering the total stored energy and its rate of change as two new state variables;
- Derivation of the control law using the proposed scheme that satisfies the desired tracking performance to track the DC-bus voltage; and
- Stability analysis of the whole system with the newly derived control input performed using the Lyapunov stability theory.
3.1. Transformation of the Model into an Exactly Linearized System Using the Exact Feedback Linearization
3.2. Controller Design
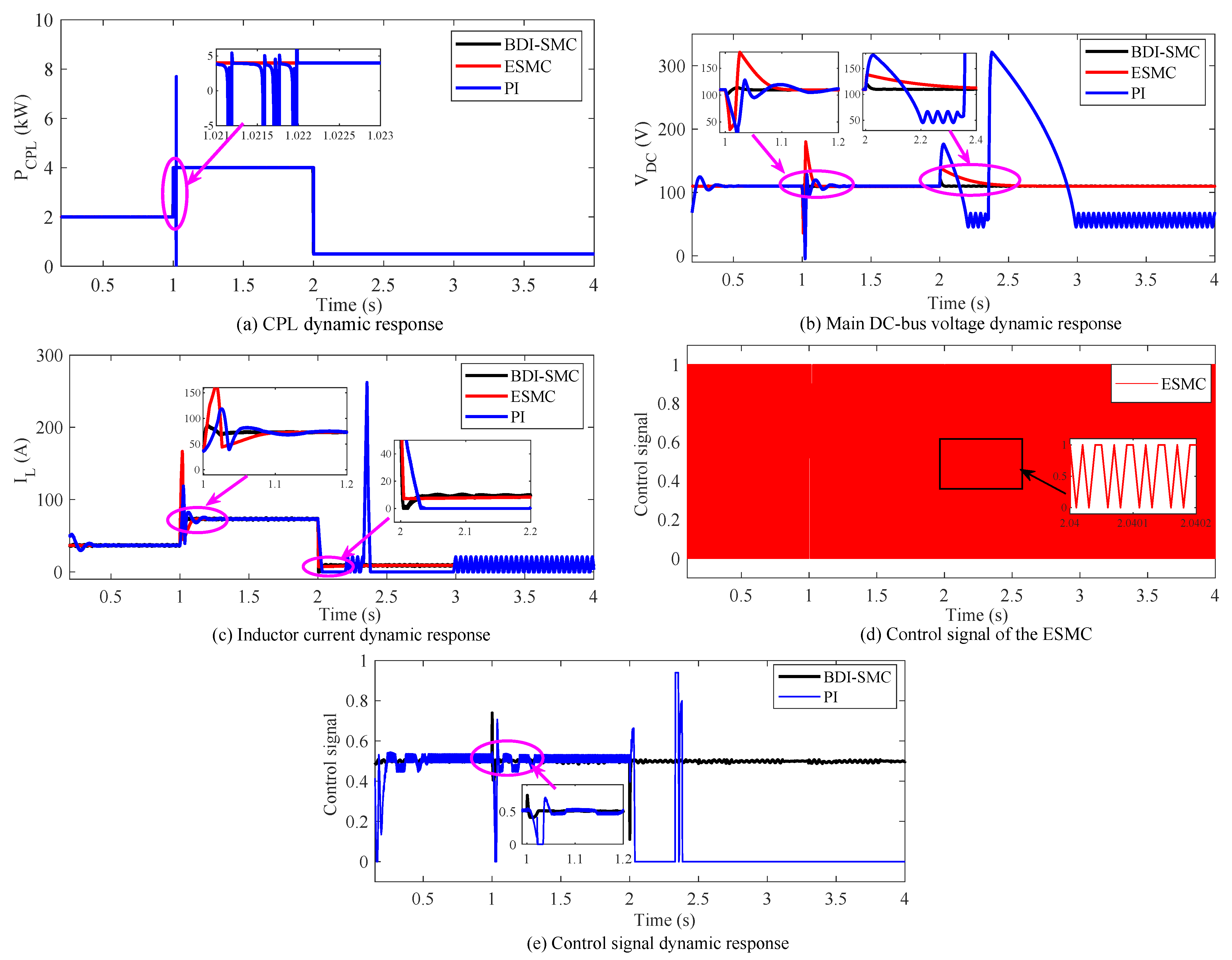
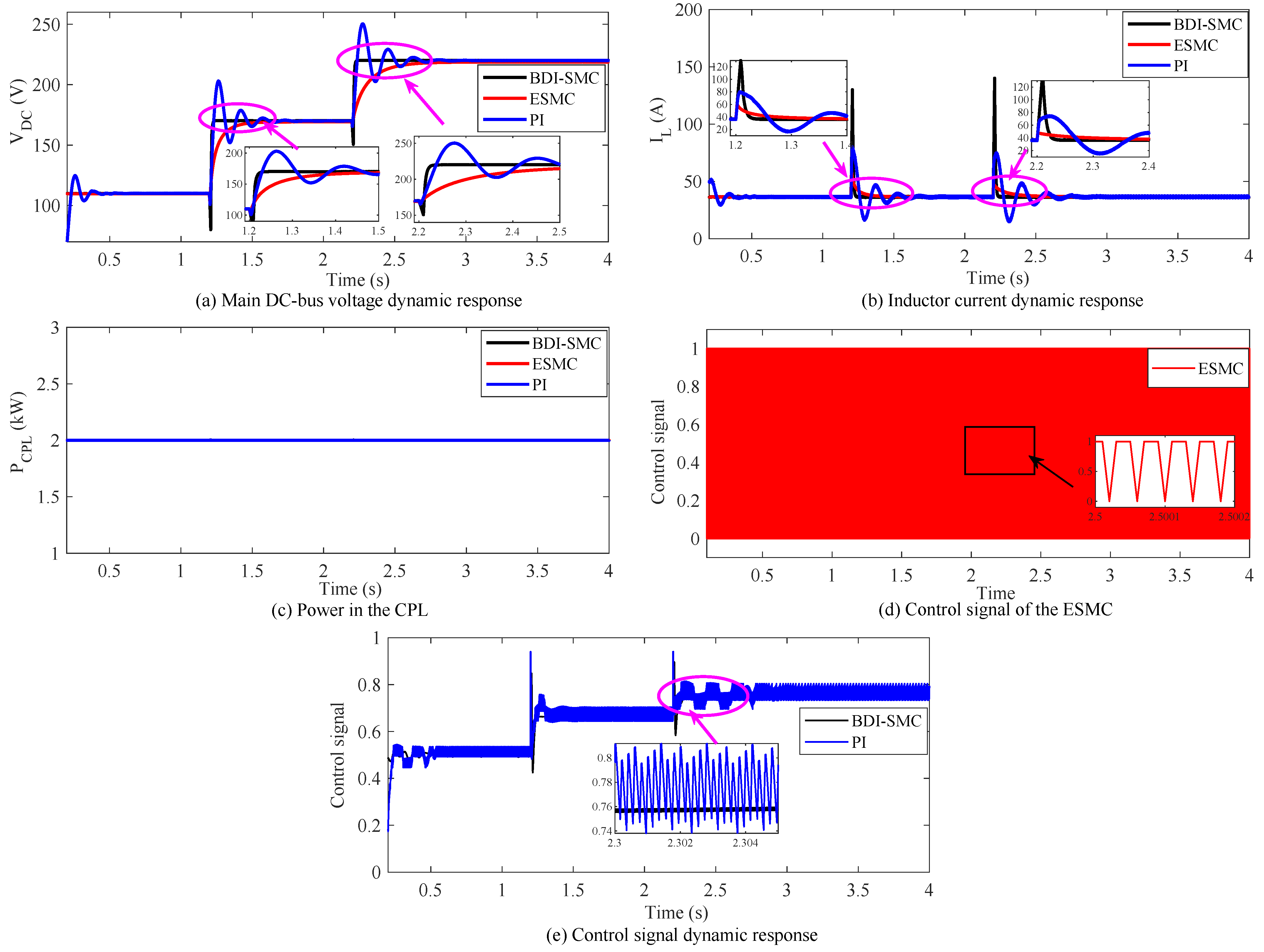
4. Simulation Results
- Case I: Controller performance investigation with variations in the reference power of the CPL;
- Case II: Controller performance investigation with variations in the reference voltage of the DC-bus; and
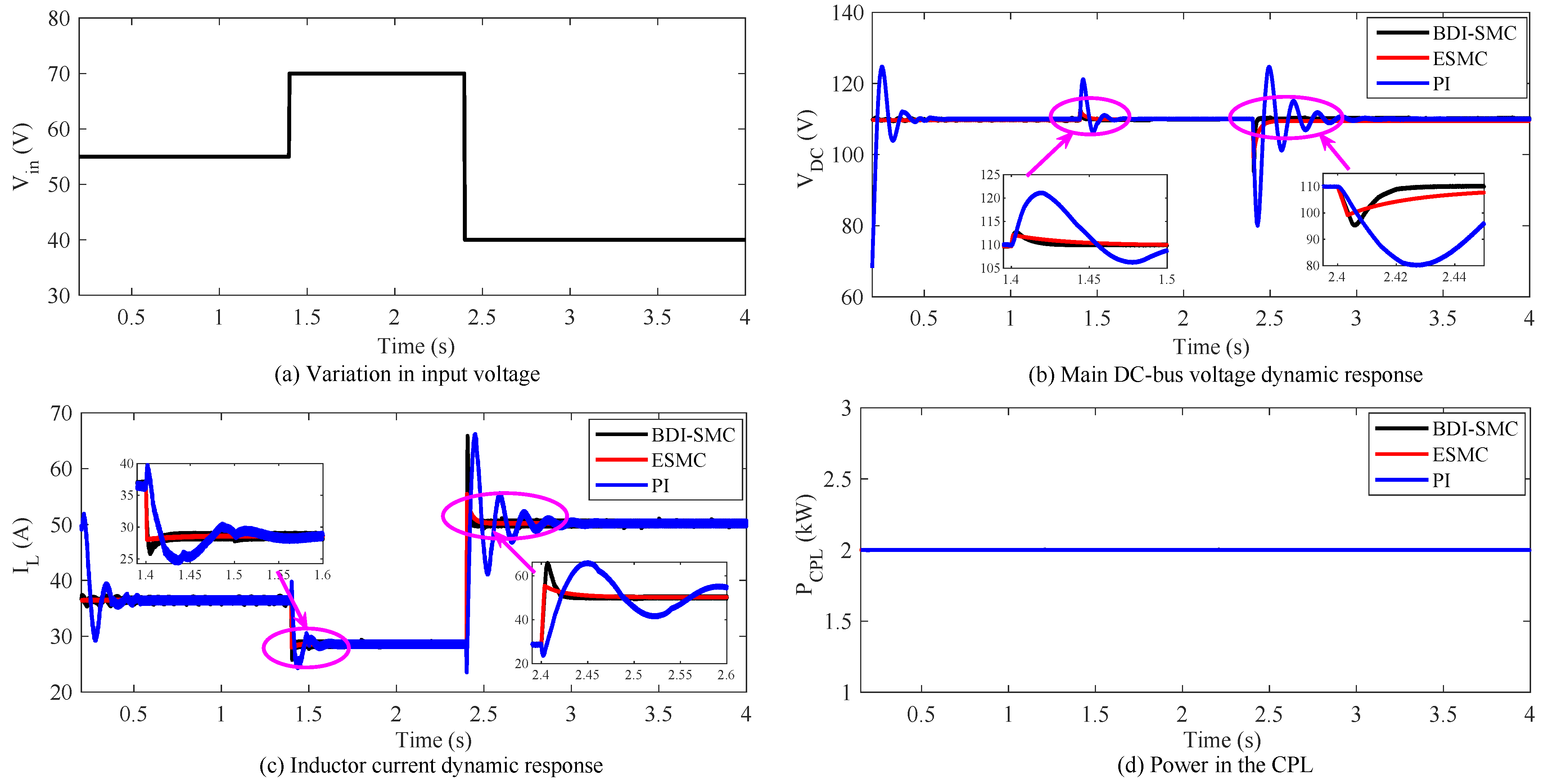
- Case III: Controller performance investigation with variations in the input voltage.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| BDI-SMC | Nonlinear Backstepping Double Integral-Sliding Mode Controller |
| BSC | Nonlinear Backstepping Controller |
| CPL | Constant Power Load |
| CCM | Continuous Conduction Mode |
| DGSs | Distributed Generation Systems |
| DDBC | DC–DC Boost Converter |
| DCDNs | DC Distribution Networks |
| ESMC | Existing Sliding Mode Controller |
| FBLCs | Nonlinear Feedback Linearizing Controllers |
| MPC | Model Predictive Controller |
| PV | Solar Photovoltaic |
| PECs | Power Electronic Converters |
| PI | Proportional-Integral Controller |
| PWM | Pulse Width Modulation |
| RESs | Renewable Energy Sources |
| SMC | Sliding Mode Controller |
| VPSs | Vehicular Power Systems |
References
- Roy, T.K.; Mahmud, M.A.; Oo, A.M.T.; Haque, M.E.; Muttaqi, K.M.; Mendis, N. Nonlinear adaptive backstepping controller design for islanded DC microgrids. IEEE Trans. Ind. Appl. 2018, 54, 2857–2873. [Google Scholar] [CrossRef]
- Orchi, T.F.; Mahmud, M.A.; Oo, A.M.T. Generalized dynamical modeling of multiple photovoltaic units in a grid-connected system for analyzing dynamic interactions. Energies 2018, 11, 296. [Google Scholar] [CrossRef] [Green Version]
- Roy, T.K.; Mahmud, M.A. Active power control of three-phase grid-connected solar PV systems using a robust nonlinear adaptive backstepping approach. Sol. Energy 2017, 153, 64–76. [Google Scholar] [CrossRef]
- Ghosh, S.; Roy, T.; Pramanik, M.; Mahmud, M.A. LMI-Based Optimal Linear Quadratic Controller Design for Multiple Solar PV Units Connected to Distribution Networks. In Proceedings of the 2021 IEEE Texas Power and Energy Conference (TPEC), College Station, TX, USA, 2–5 February 2021; pp. 1–6. [Google Scholar]
- Roy, T.K.; Mahmud, M.A.; Oo, A.M.T.; Bansal, R.; Haque, M.E. Nonlinear adaptive backstepping controller design for three-phase grid-connected solar photovoltaic systems. Electr. Power Compon. Syst. 2017, 45, 2275–2292. [Google Scholar] [CrossRef]
- Ghosh, S.K.; Roy, T.K.; Pramanik, M.A.H.; Sarkar, A.K.; Mahmud, M. An energy management system-based control strategy for DC microgrids with dual energy storage systems. Energies 2020, 13, 2992. [Google Scholar] [CrossRef]
- Xiao, J.; Wang, P.; Setyawan, L.; Xu, Q. Multi-level energy management system for real-time scheduling of DC microgrids with multiple slack terminals. IEEE Trans. Energy Convers. 2015, 31, 392–400. [Google Scholar] [CrossRef]
- Roy, T.K.; Mahmud, M.A. Dynamic stability analysis of hybrid islanded DC microgrids using a nonlinear backstepping approach. IEEE Syst. J. 2017, 12, 3120–3130. [Google Scholar] [CrossRef]
- Nasir, M.; Khan, H.A.; Hussain, A.; Mateen, L.; Zaffar, N.A. Solar PV-based scalable DC microgrid for rural electrification in developing regions. IEEE Trans. Sustain. Energy 2017, 9, 390–399. [Google Scholar] [CrossRef]
- Mendis, N.; Mahmud, M.A.; Roy, T.K.; Haque, M.E.; Muttaqi, K.M. Power management and control strategies for efficient operation of a solar power dominated hybrid DC microgrid for remote power applications. In Proceedings of the 2016 IEEE Industry Applications Society Annual Meeting, Portland, OR, USA, 2–6 October 2016; pp. 1–8. [Google Scholar]
- Roy, T.K.; Mahmud, M.A.; Oo, A.M.T.; Haque, M.E.; Muttaqi, K.M.; Mendis, N. Nonlinear adaptive backstepping controller design for controlling bidirectional power flow of BESSs in DC microgrids. In Proceedings of the 2016 IEEE Industry Applications Society Annual Meeting, Portland, OR, USA, 2–6 October 2016; pp. 1–8. [Google Scholar]
- Mahmud, M.A.; Roy, T.K.; Saha, S.; Haque, M.E.; Pota, H.R. Robust nonlinear adaptive feedback linearizing decentralized controller design for islanded DC microgrids. IEEE Trans. Ind. Appl. 2019, 55, 5343–5352. [Google Scholar] [CrossRef]
- Ravada, B.R.; Tummuru, N.R.; Ande, B.N.L. Photovoltaic-Wind and Hybrid Energy Storage Integrated Multi-Source Converter Configuration for DC Microgrid Applications. IEEE Trans. Sustain. Energy 2020, 12, 83–91. [Google Scholar] [CrossRef]
- Garg, A.; Tummuru, N.R.; Oruganti, R. Implementation of Energy Management Scenarios in a DC Microgrid using DC Bus Signaling. IEEE Trans. Ind. Appl. 2021, 57, 5306–5317. [Google Scholar] [CrossRef]
- Ghosh, S.K.; Roy, T.K.; Pramanik, M.A.H.; Ali, M.S. Energy management techniques to enhance DC-bus voltage transient stability and power balancing issues for islanded DC microgrids. In Advances in Clean Energy Technologies; Elsevier: Amsterdam, The Netherlands, 2021; pp. 349–375. [Google Scholar]
- Lucas, K.E.; Pagano, D.J.; Vaca-Benavides, D.A.; Garcia-Arcos, R.; Rocha, E.M.; Medeiros, R.L.; Rios, S.J. Robust Control of Interconnected Power Electronic Converters to Enhance Performance in dc distribution systems: A case of study. IEEE Trans. Power Electron. 2020, 36, 4851–4863. [Google Scholar] [CrossRef]
- Chang, F.; Cui, X.; Wang, M.; Su, W. Region of Attraction Estimation for DC Microgrids with Constant Power Loads Using Potential Theory. IEEE Trans. Smart Grid 2021, 12, 3793–3808. [Google Scholar] [CrossRef]
- Wang, M.; Tang, F.; Wu, X.; Niu, J.; Zhang, Y.; Wang, J. A Nonlinear Control Strategy for DC-DC Converter with Unknown Constant Power Load Using Damping and Interconnection Injecting. Energies 2021, 14, 3031. [Google Scholar] [CrossRef]
- AL-Nussairi, M.K.; Bayindir, R.; Padmanaban, S.; Mihet-Popa, L.; Siano, P. Constant Power Loads (CPL) with Microgrids: Problem Definition, Stability Analysis and Compensation Techniques. Energies 2017, 10, 1656. [Google Scholar] [CrossRef]
- Majstorovic, D.; Celanovic, I.; Teslic, N.D.; Celanovic, N.; Katic, V.A. Ultralow-latency hardware-in-the-loop platform for rapid validation of power electronics designs. IEEE Trans. Ind. Electron. 2011, 58, 4708–4716. [Google Scholar] [CrossRef]
- Lu, X.; Sun, K.; Guerrero, J.M.; Vasquez, J.C.; Huang, L.; Wang, J. Stability enhancement based on virtual impedance for DC microgrids with constant power loads. IEEE Trans. Smart Grid 2015, 6, 2770–2783. [Google Scholar] [CrossRef]
- Rahimi, A.M.; Emadi, A. Active damping in DC/DC power electronic converters: A novel method to overcome the problems of constant power loads. IEEE Trans. Ind. Electron. 2009, 56, 1428–1439. [Google Scholar] [CrossRef]
- Magne, P.; Marx, D.; Nahid-Mobarakeh, B.; Pierfederici, S. Large-signal stabilization of a DC-link supplying a constant power load using a virtual capacitor: Impact on the domain of attraction. IEEE Trans. Ind. Appl. 2012, 48, 878–887. [Google Scholar] [CrossRef]
- Gui, Y.; Han, R.; Guerrero, J.M.; Vasquez, J.C.; Wei, B.; Kim, W. Large-Signal Stability Improvement of DC-DC Converters in DC Microgrid. IEEE Trans. Energy Convers. 2021, 36, 2534–2544. [Google Scholar] [CrossRef]
- Augustine, S.; Mishra, M.K.; Lakshminarasamma, N. Adaptive droop control strategy for load sharing and circulating current minimization in low-voltage standalone DC microgrid. IEEE Trans. Sustain. Energy 2014, 6, 132–141. [Google Scholar] [CrossRef]
- Karami, Z.; Shafiee, Q.; Khayat, Y.; Yaribeygi, M.; Dragicevic, T.; Bevrani, H. Decentralized model predictive control of DC microgrids with constant power load. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 9, 451–460. [Google Scholar] [CrossRef]
- Karami, Z.; Shafiee, Q.; Sahoo, S.; Yaribeygi, M.; Bevrani, H.; Dragicevic, T. Hybrid Model Predictive Control of DC–DC Boost Converters With Constant Power Load. IEEE Trans. Energy Convers. 2020, 36, 1347–1356. [Google Scholar] [CrossRef]
- Vafamand, N.; Yousefizadeh, S.; Khooban, M.H.; Bendtsen, J.D.; Dragičević, T. Adaptive TS fuzzy-based MPC for DC microgrids with dynamic CPLs: Nonlinear power observer approach. IEEE Syst. J. 2018, 13, 3203–3210. [Google Scholar] [CrossRef] [Green Version]
- Zeng, J.; Zhang, Z.; Qiao, W. An interconnection and damping assignment passivity-based controller for a DC–DC boost converter with a constant power load. IEEE Trans. Ind. Appl. 2013, 50, 2314–2322. [Google Scholar] [CrossRef]
- Hassan, M.A.; Li, E.P.; Li, X.; Li, T.; Duan, C.; Chi, S. Adaptive passivity-based control of DC–DC buck power converter with constant power load in DC microgrid systems. IEEE J. Emerg. Sel. Top. Power Electron. 2018, 7, 2029–2040. [Google Scholar] [CrossRef]
- Mahmud, M.; Hossain, M.; Pota, H.; Oo, A.M. Robust partial feedback linearizing excitation controller design for multimachine power systems. IEEE Trans. Power Syst. 2016, 32, 3–16. [Google Scholar] [CrossRef]
- Roy, T.K.; Mahmud, M.A.; Shen, W.; Oo, A.M. An adaptive partial feedback linearizing control scheme: An application to a single machine infinite bus system. IEEE Trans. Circuits Syst. II Express Briefs 2019, 67, 2557–2561. [Google Scholar] [CrossRef]
- Roy, T.K.; Mahmud, M.A.; Shen, W.; Oo, A.M.T. A non-linear adaptive excitation control scheme for feedback linearized synchronous generations in multimachine power systems. IET Gener. Transm. Distrib. 2021, 15, 1501–1520. [Google Scholar] [CrossRef]
- Pramanik, M.; Roy, T.; Ghosh, S.; Anower, M.; Mahmud, M.A. Robust Partial Feedback Linearizing Excitation Controller Design for Higher-Order Synchronous Generator in SMIB Systems to Improve the Transient Stability. In Proceedings of the 2021 IEEE Texas Power and Energy Conference (TPEC), College Station, TX, USA, 2–5 February 2021; pp. 1–6. [Google Scholar]
- Rahimi, A.M.; Williamson, G.A.; Emadi, A. Loop-cancellation technique: A novel nonlinear feedback to overcome the destabilizing effect of constant-power loads. IEEE Trans. Veh. Technol. 2009, 59, 650–661. [Google Scholar] [CrossRef]
- Sulligoi, G.; Bosich, D.; Giadrossi, G.; Zhu, L.; Cupelli, M.; Monti, A. Multiconverter medium voltage DC power systems on ships: Constant-power loads instability solution using linearization via state feedback control. IEEE Trans. Smart Grid 2014, 5, 2543–2552. [Google Scholar] [CrossRef]
- Arora, S.; Balsara, P.; Bhatia, D. Input–output linearization of a boost converter with mixed load (constant voltage load and constant power load). IEEE Trans. Power Electron. 2018, 34, 815–825. [Google Scholar] [CrossRef]
- Roy, T.; Mahmud, M.A.; Ghosh, S.; Pramanik, M.; Kumar, R.; Oo, A.M. Design of an Adaptive Sliding Mode Controller for Rapid Earth Fault Current Limiters in Resonant Grounded Distribution Networks to Mitigate Powerline Bushfires. In Proceedings of the 2021 IEEE Texas Power and Energy Conference (TPEC), College Station, TX, USA, 2–5 February 2021; pp. 1–6. [Google Scholar]
- Roy, T.K.; Mahmud, M.A. Fault current compensations in resonant grounded distribution systems to mitigate powerline bushfires using a nonsingular terminal sliding model controller. IET Gener. Transm. Distrib. 2021, 1–14. [Google Scholar] [CrossRef]
- Singh, S.; Fulwani, D.; Kumar, V. Robust sliding-mode control of dc/dc boost converter feeding a constant power load. IET Power Electron. 2015, 8, 1230–1237. [Google Scholar] [CrossRef]
- Zhang, M.; Li, Y.; Liu, F.; Luo, L.; Cao, Y.; Shahidehpour, M. Voltage stability analysis and sliding-mode control method for rectifier in DC systems with constant power loads. IEEE J. Emerg. Sel. Top. Power Electron. 2017, 5, 1621–1630. [Google Scholar] [CrossRef]
- El Aroudi, A.; Martínez-Treviño, B.A.; Vidal-Idiarte, E.; Cid-Pastor, A. Fixed switching frequency digital sliding-mode control of DC-DC power supplies loaded by constant power loads with inrush current limitation capability. Energies 2019, 12, 1055. [Google Scholar] [CrossRef] [Green Version]
- Roy, T.; Mahmud, M.; Shen, W.; Oo, A.; Haque, M. Robust nonlinear adaptive backstepping excitation controller design for rejecting external disturbances in multimachine power systems. Int. J. Electr. Power Energy Syst. 2017, 84, 76–86. [Google Scholar] [CrossRef]
- Boutebba, O.; Semcheddine, S.; Krim, F.; Corti, F.; Reatti, A.; Grasso, F. A Nonlinear Back-stepping Controller of DC-DC Non Inverting Buck-Boost Converter for Maximizing Photovoltaic Power Extraction. In Proceedings of the 2020 IEEE International Conference on Environment and Electrical Engineering and 2020 IEEE Industrial and Commercial Power Systems Europe (EEEIC/I&CPS Europe), Madrid, Spain, 9–12 June 2020; pp. 1–6. [Google Scholar]
- Roy, T.; Morshed, M.; Tumpa, F.; Pervej, M. Robust adaptive backstepping speed controller design for a series DC motor. In Proceedings of the 2015 IEEE International WIE Conference on Electrical and Computer Engineering (WIECON-ECE), Dhaka, Banglades, 19–20 December 2015; pp. 243–246. [Google Scholar]
- Boutebba, O.; Laudani, A.; Lozito, G.M.; Corti, F.; Reatti, A.; Semcheddine, S. A Neural Adaptive Assisted Backstepping Controller for MPPT in Photovoltaic Applications. In Proceedings of the 2020 IEEE International Conference on Environment and Electrical Engineering and 2020 IEEE Industrial and Commercial Power Systems Europe (EEEIC/I&CPS Europe), Madrid, Spain, 9–12 June 2020; pp. 1–6. [Google Scholar]
- Xu, Q.; Jiang, W.; Blaabjerg, F.; Zhang, C.; Zhang, X.; Fernando, T. Backstepping control for large signal stability of high boost ratio interleaved converter interfaced DC microgrids with constant power loads. IEEE Trans. Power Electron. 2019, 35, 5397–5407. [Google Scholar] [CrossRef]
- Xu, Q.; Zhang, C.; Wen, C.; Wang, P.; Yeong, L.M. A novel adaptive backstepping controller for stabilization of DC/DC converter feeding constant power load. In Proceedings of the 2017 IEEE 26th International Symposium on Industrial Electronics (ISIE), Edinburgh, UK, 19–21 June 2017; pp. 570–575. [Google Scholar]
- Xu, Q.; Zhang, C.; Wen, C.; Wang, P. A novel composite nonlinear controller for stabilization of constant power load in DC microgrid. IEEE Trans. Smart Grid 2017, 10, 752–761. [Google Scholar] [CrossRef]
- Li, X.; Zhang, X.; Jiang, W.; Wang, J.; Wang, P.; Wu, X. A Novel Assorted Nonlinear Stabilizer for DC–DC Multilevel Boost Converter With Constant Power Load in DC Microgrid. IEEE Trans. Power Electron. 2020, 35, 11181–11192. [Google Scholar] [CrossRef]
- Wu, J.; Lu, Y. Adaptive Backstepping Sliding Mode Control for Boost Converter With Constant Power Load. IEEE Access 2019, 7, 50797–50807. [Google Scholar] [CrossRef]
| Parameters | Description | Value |
|---|---|---|
| Supply voltage | 55 V | |
| Main DC-bus voltage | 110 V | |
| Nominal power in constant power load | 2 kW | |
| Parasitic resistance of an inductor | 2 m | |
| Inductance of the converter | 5 mH | |
| Capacitance of the main DC-bus | 6 mF | |
| Resistive Load | ∞ |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghosh, S.K.; Roy, T.K.; Pramanik, M.A.H.; Mahmud, M.A. Design of Nonlinear Backstepping Double-Integral Sliding Mode Controllers to Stabilize the DC-Bus Voltage for DC–DC Converters Feeding CPLs. Energies 2021, 14, 6753. https://doi.org/10.3390/en14206753
Ghosh SK, Roy TK, Pramanik MAH, Mahmud MA. Design of Nonlinear Backstepping Double-Integral Sliding Mode Controllers to Stabilize the DC-Bus Voltage for DC–DC Converters Feeding CPLs. Energies. 2021; 14(20):6753. https://doi.org/10.3390/en14206753
Chicago/Turabian StyleGhosh, Subarto Kumar, Tushar Kanti Roy, Md. Abu Hanif Pramanik, and Md. Apel Mahmud. 2021. "Design of Nonlinear Backstepping Double-Integral Sliding Mode Controllers to Stabilize the DC-Bus Voltage for DC–DC Converters Feeding CPLs" Energies 14, no. 20: 6753. https://doi.org/10.3390/en14206753
APA StyleGhosh, S. K., Roy, T. K., Pramanik, M. A. H., & Mahmud, M. A. (2021). Design of Nonlinear Backstepping Double-Integral Sliding Mode Controllers to Stabilize the DC-Bus Voltage for DC–DC Converters Feeding CPLs. Energies, 14(20), 6753. https://doi.org/10.3390/en14206753







