Thermodynamic Evaluation of the Forced Convective Hybrid-Solar Dryer during Drying Process of Rosemary (Rosmarinus officinalis L.) Leaves
Abstract
:1. Introduction
2. Materials and Methods
2.1. Sample Preparation
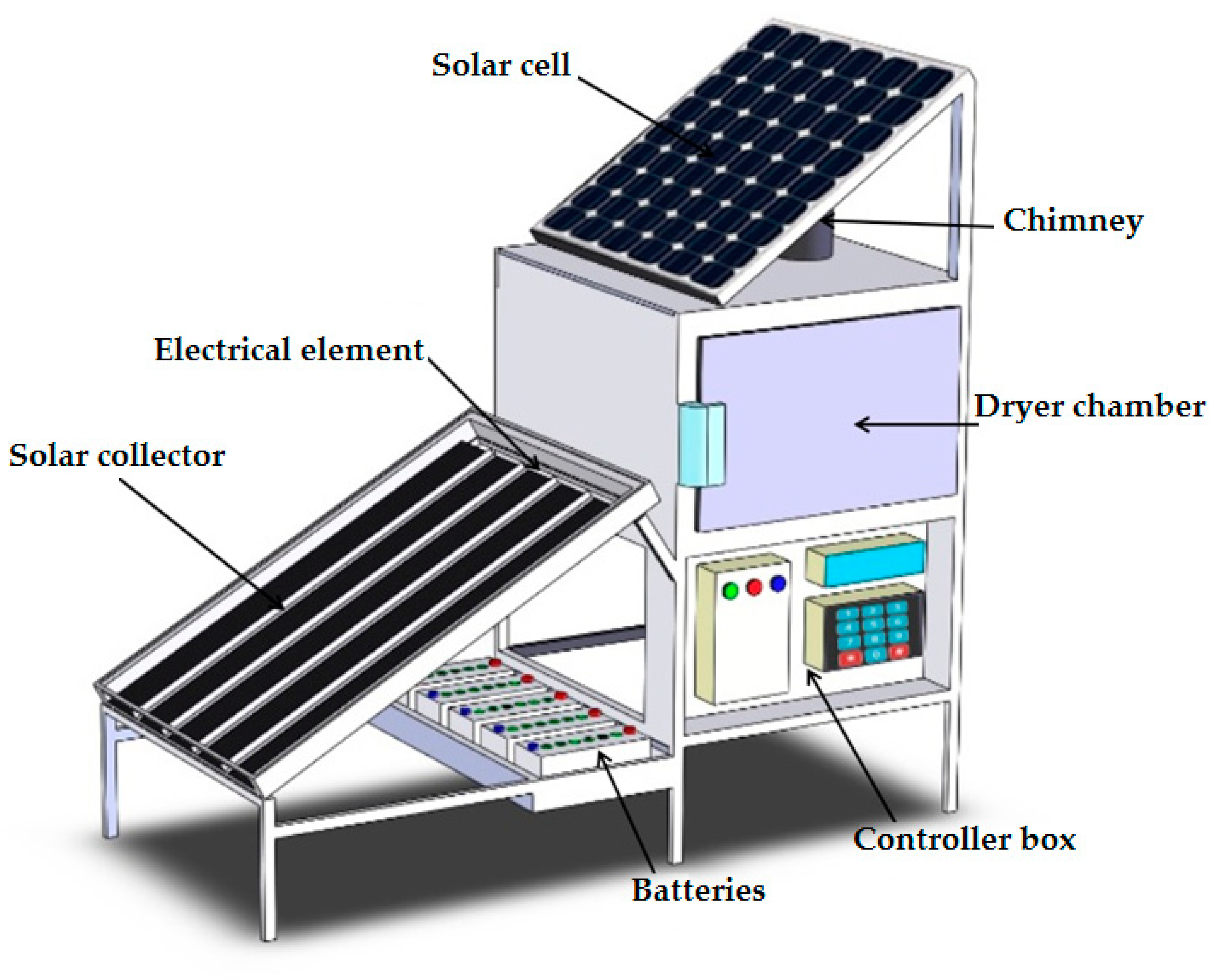
2.2. Dryer Equipment
2.3. Experimental Procedure
2.4. Data Analysis
2.4.1. Moisture Content Analysis
2.4.2. Effective Moisture Diffusivity Coefficient (Deff)
2.4.3. Activation Energy
2.4.4. Energy and Exergy Analysis
2.4.5. Specific Energy Consumption
3. Results
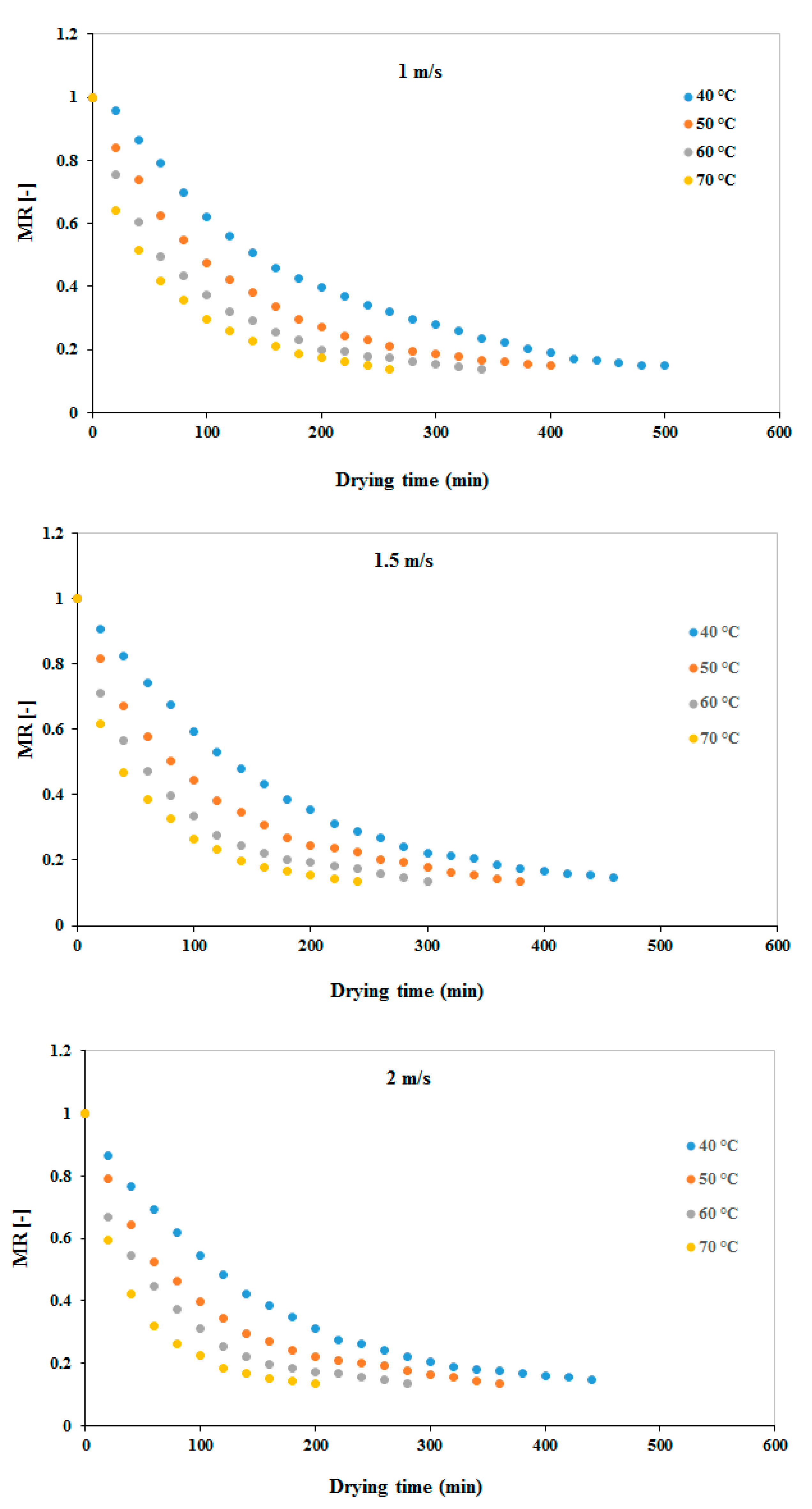
3.1. Moisture Content
3.2. Drying Rate
3.3. Determination of Deff
3.4. Activation Energy
3.5. Energy Utilization Ratio (EUR)
3.6. Energy Utilization (EU)
3.7. Input Exergy, Output Exergy, and Exergy Loss
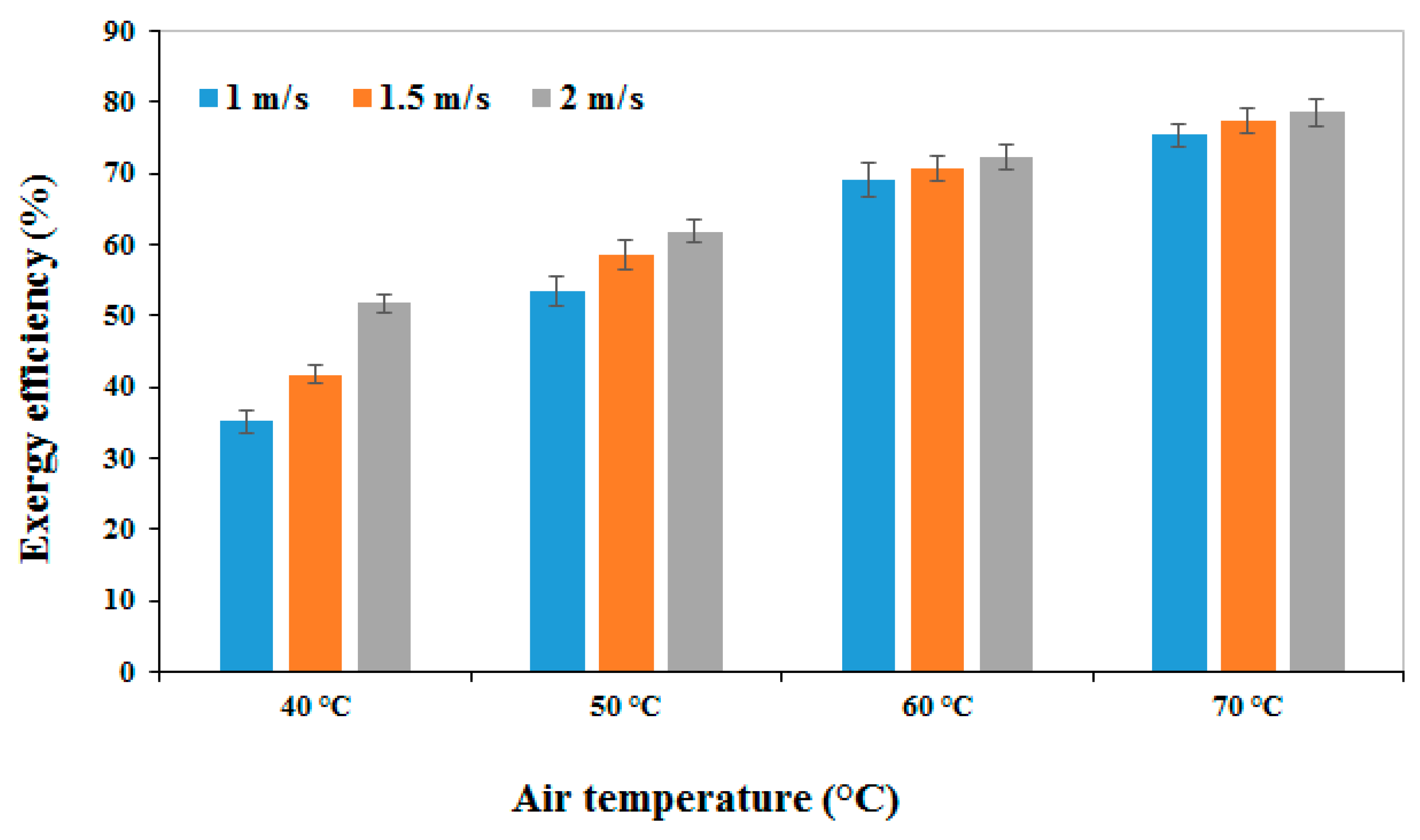
3.8. Exergy Efficiency
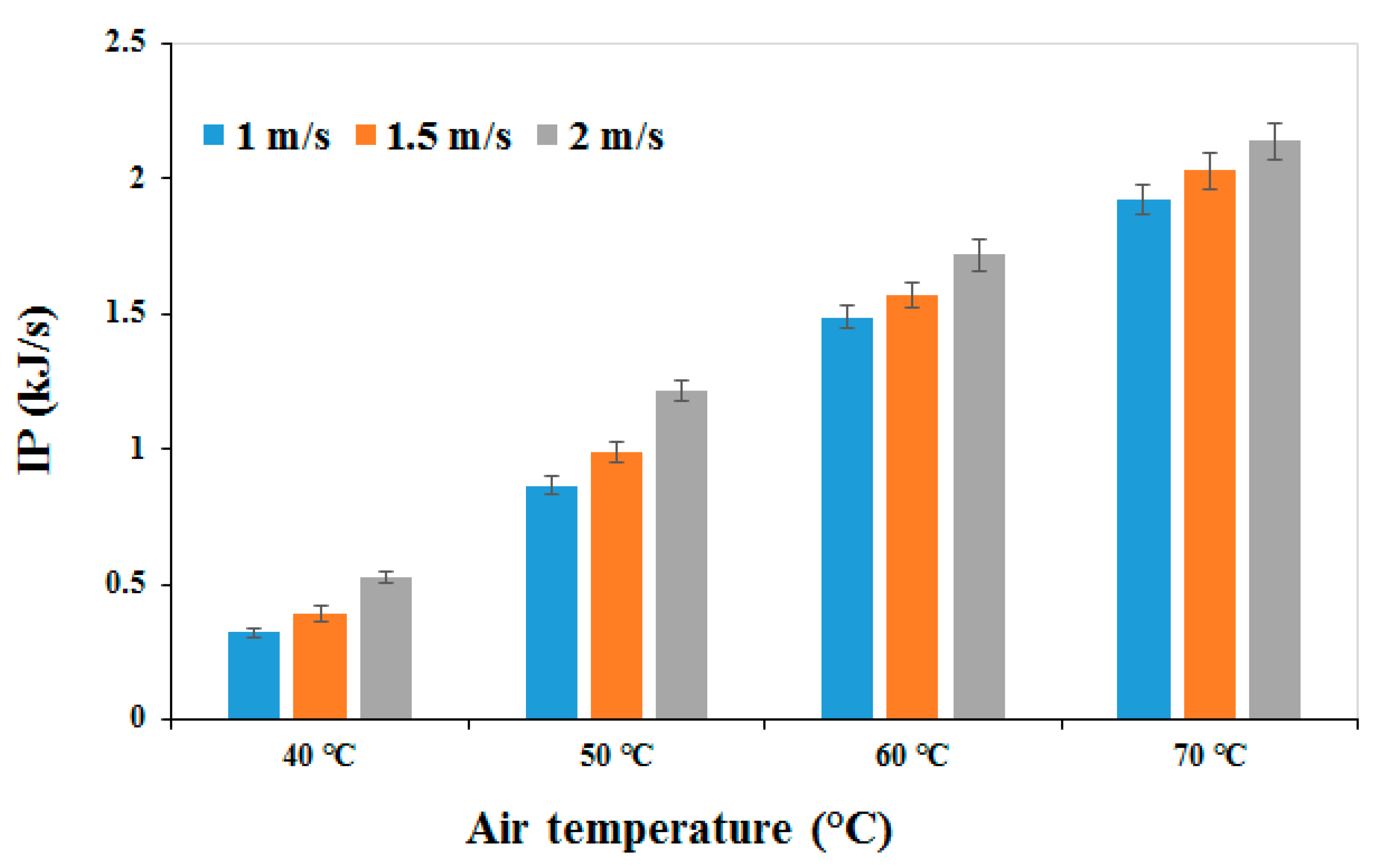
3.9. Exergetic Improvement Potential Rate (IP)
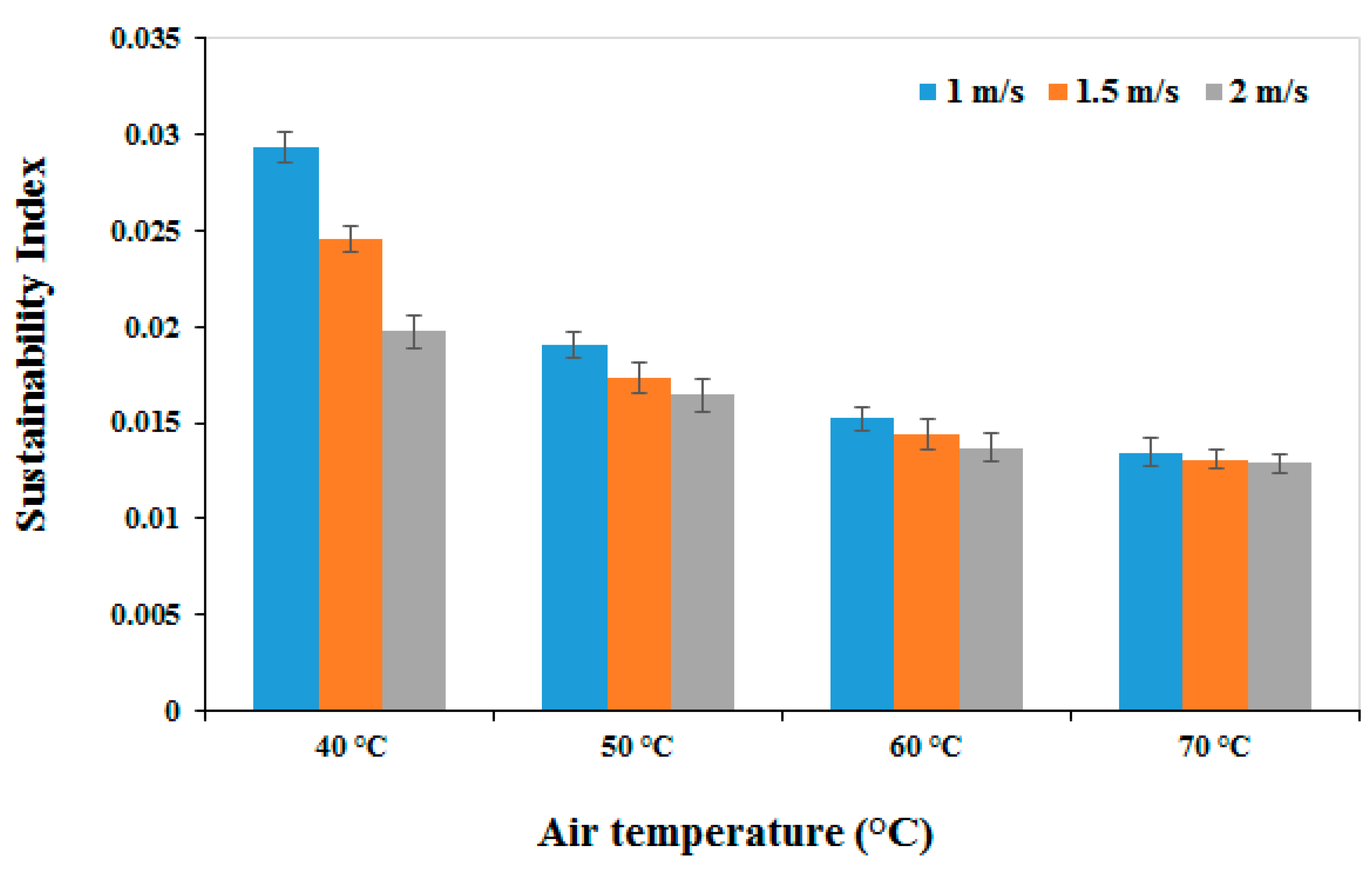
3.10. Sustainability Index (SI)
3.11. Specific Energy Consumption (SEC)
4. Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Karami, H.; Rasekh, M.; Darvishi, Y.; Khaledi, R. Effect of Drying Temperature and Air Velocity on the Essential Oil Content of Mentha aquatica L. J. Essent. Oil-Bear. Plants 2017, 20, 1131–1136. [Google Scholar] [CrossRef]
- de Macedo, L.M.; Santos, É.M.D.; Militão, L.; Tundisi, L.L.; Ataide, J.A.; Souto, E.B.; Mazzola, P.G. Rosemary (Rosmarinus officinalis L., syn Salvia rosmarinus Spenn.) and Its Topical Applications: A Review. Plants 2020, 9, 651. [Google Scholar] [CrossRef] [PubMed]
- Mohammed, H.A.; Al-Omar, M.S.; Mohammed, S.A.A.; Aly, M.S.A.; Alsuqub, A.N.A.; Khan, R.A. Drying Induced Impact on Composition and Oil Quality of Rosemary Herb, Rosmarinus Officinalis Linn. Molecules 2020, 25, 2830. [Google Scholar] [CrossRef]
- Kaveh, M.; Karami, H.; Jahanbakhshi, A. Investigation of mass transfer, thermodynamics, and greenhouse gases properties in pennyroyal drying. J. Food Process Eng. 2020, 43, e13446. [Google Scholar] [CrossRef]
- Karami, H.; Lorestani, A.N.; Tahvilian, R. Assessment of kinetics, effective moisture diffusivity, specific energy consumption, and percentage of thyme oil extracted in a hybrid solar-electric dryer. J. Food Process Eng. 2021, 44, e13588. [Google Scholar] [CrossRef]
- Karami, H.; Kaveh, M.; Mirzaee-Ghaleh, E.; Taghinezhad, E. Using PSO and GWO techniques for prediction some drying properties of tarragon (Artemisia dracunculus L.). J. Food Process Eng. 2018, 41, e12921. [Google Scholar] [CrossRef]
- Karami, H.; Rasekh, M.; Darvishi, Y. Effect of temperature and air velocity on drying kinetics and organo essential oil extraction efficiency in a hybrid dryer. Innov. Food Technol. 2017, 5, 65–75. [Google Scholar]
- Karami, H.; Rasekh, M. Kinetics mass transfer and modeling of tarragon drying (Artemisia dracunculus L.). Iran. J. Med. Arom. Plants Res. 2018, 34, 734–747. [Google Scholar]
- Suherman, S.; Susanto, E.E.; Zardani, A.W.; Dewi, N.H.R.; Hadiyanto, H. Energy–exergy analysis and mathematical modeling of cassava starch drying using a hybrid solar dryer. Cogent Eng. 2020, 7, 1771819. [Google Scholar] [CrossRef]
- Reyes, A.; Mahn, A.; Vásquez, F. Mushrooms dehydration in a hybrid-solar dryer, using a phase change material. Energy Convers. Manag. 2014, 83, 241–248. [Google Scholar] [CrossRef]
- Eltawil, M.A.; Azam, M.M.; Alghannam, A.O. Energy analysis of hybrid solar tunnel dryer with PV system and solar collector for drying mint (MenthaViridis). J. Clean. Prod. 2018, 181, 352–364. [Google Scholar] [CrossRef]
- Amer, B.M.A.; Gottschalk, K.; Hossain, M.A. Integrated hybrid solar drying system and its drying kinetics of chamomile. Renew. Energy 2018, 121, 539–547. [Google Scholar] [CrossRef]
- Bosomtwe, A.; Danso, J.K.; Osekre, E.A.; Opit, G.P.; Mbata, G.; Armstrong, P.; Arthur, F.H.; Campbell, J.; Manu, N.; McNeill, S.G.; et al. Effectiveness of the solar biomass hybrid dryer for drying and disinfestation of maize. J. Stored Prod. Res. 2019, 83, 66–72. [Google Scholar] [CrossRef]
- Aghbashlo, M.; Kianmehr, M.H.; Arabhosseini, A. Energy and Exergy Analyses of Thin-Layer Drying of Potato Slices in a Semi-Industrial Continuous Band Dryer. Dry. Technol. 2008, 26, 1501–1508. [Google Scholar] [CrossRef]
- Beigi, M.; Tohidi, M.; Torki-Harchegani, M. Exergetic analysis of deep-bed drying of rough rice in a convective dryer. Energy 2017, 140, 374–382. [Google Scholar] [CrossRef]
- Liu, Z.-L.; Bai, J.-W.; Wang, S.-X.; Meng, J.-S.; Wang, H.; Yu, X.-L.; Gao, Z.-J.; Xiao, H.-W. Prediction of energy and exergy of mushroom slices drying in hot air impingement dryer by artificial neural network. Dry. Technol. 2020, 38, 1959–1970. [Google Scholar] [CrossRef]
- Taskin, O.; Polat, A.; Etemoglu, A.B.; Izli, N. Energy and exergy analysis, drying kinetics, modeling, microstructure and thermal properties of convective-dried banana slices. J. Therm. Anal. Calorim. 2021. [Google Scholar] [CrossRef]
- Taheri-Garavand, A.; Karimi, F.; Karimi, M.; Lotfi, V.; Khoobbakht, G. Hybrid response surface methodology–artificial neural network optimization of drying process of banana slices in a forced convective dryer. Food Sci. Technol. Int. 2018, 24, 277–291. [Google Scholar] [CrossRef] [PubMed]
- Islam, M.A.; Mondal, M.H.T.; Akhtaruzzaman, M.; Sheikh, M.A.M.; Islam, M.M.; Haque, M.A.; Sarker, M.S.H. Energy, exergy, and milling performance of parboiled paddy: An industrial LSU dryer. Dry. Technol. 2021, 1–15. [Google Scholar] [CrossRef]
- Li, B.; Li, C.; Huang, J.; Li, C. Exergoeconomic Analysis of Corn Drying in a Novel Industrial Drying System. Entropy 2020, 22, 689. [Google Scholar] [CrossRef]
- Yu, X.-L.; Zielinska, M.; Ju, H.-Y.; Mujumdar, A.S.; Duan, X.; Gao, Z.-J.; Xiao, H.-W. Multistage relative humidity control strategy enhances energy and exergy efficiency of convective drying of carrot cubes. Int. J. Heat Mass Transf. 2020, 149, 119231. [Google Scholar] [CrossRef]
- Castro, M.; Román, C.; Echegaray, M.; Mazza, G.; Rodriguez, R. Exergy Analyses of Onion Drying by Convection: Influence of Dryer Parameters on Performance. Entropy 2018, 20, 310. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Karthikeyan, A.K.; Murugavelh, S. Thin layer drying kinetics and exergy analysis of turmeric (Curcuma longa) in a mixed mode forced convection solar tunnel dryer. Renew. Energy 2018, 128, 305–312. [Google Scholar] [CrossRef]
- Lakshmi, D.V.N.; Muthukumar, P.; Layek, A.; Nayak, P.K. Drying kinetics and quality analysis of black turmeric (Curcuma caesia) drying in a mixed mode forced convection solar dryer integrated with thermal energy storage. Renew. Energy 2018, 120, 23–34. [Google Scholar] [CrossRef]
- Tagnamas, Z.; Lamsyehe, H.; Moussaoui, H.; Bahammou, Y.; Kouhila, M.; Idlimam, A.; Lamharrar, A. Energy and exergy analyses of carob pulp drying system based on a solar collector. Renew. Energy 2021, 163, 495–503. [Google Scholar] [CrossRef]
- Kaveh, M.; Abbaspour-Gilandeh, Y.; Chen, G. Drying kinetic, quality, energy and exergy performance of hot air-rotary drum drying of green peas using adaptive neuro-fuzzy inference system. Food Bioprod. Process. 2020, 124, 168–183. [Google Scholar] [CrossRef]
- Vijayan, S.; Arjunan, T.V.; Kumar, A. Exergo-environmental analysis of an indirect forced convection solar dryer for drying bitter gourd slices. Renew. Energy 2020, 146, 2210–2223. [Google Scholar] [CrossRef]
- Suherman, S.; Hadiyanto, H.; Susanto, E.E.; Utami, I.A.P.; Ningrum, T. Hybrid solar dryer for sugar-palm vermicelli drying. J. Food Process Eng. 2020, 43, e13471. [Google Scholar] [CrossRef]
- Aghbashlo, M.; Mobli, H.; Rafiee, S.; Madadlou, A. Energy and exergy analyses of the spray drying process of fish oil microencapsulation. Biosyst. Eng. 2012, 111, 229–241. [Google Scholar] [CrossRef]
- Das, I.; Arora, A. Alternate microwave and convective hot air application for rapid mushroom drying. J. Food Eng. 2018, 223, 208–219. [Google Scholar] [CrossRef]
- Taghinezhad, E.; Kaveh, M.; Jahanbakhshi, A.; Golpour, I. Use of artificial intelligence for the estimation of effective moisture diffusivity, specific energy consumption, color and shrinkage in quince drying. J. Food Process Eng. 2020, 43, e13358. [Google Scholar] [CrossRef]
- Sehrawat, R.; Nema, P.K.; Kaur, B.P. Quality evaluation and drying characteristics of mango cubes dried using low-pressure superheated steam, vacuum and hot air drying methods. LWT 2018, 92, 548–555. [Google Scholar] [CrossRef]
- Kian-Pour, N.; Karatas, S. Impact of different geometric shapes on drying kinetics and textural characteristics of apples at temperatures above 100 °C. Heat Mass Transf. 2019, 55, 3721–3732. [Google Scholar] [CrossRef]
- Quispe-Fuentes, I.; Vega-Gálvez, A.; Vásquez, V.; Uribe, E.; Astudillo, S. Mathematical modeling and quality properties of a dehydrated native Chilean berry. J. Food Process Eng. 2017, 40, e12499. [Google Scholar] [CrossRef]
- Kaveh, M.; Chayjan, R.A.; Golpour, I.; Poncet, S.; Seirafi, F.; Khezri, B. Evaluation of exergy performance and onion drying properties in a multi-stage semi-industrial continuous dryer: Artificial neural networks (ANNs) and ANFIS models. Food Bioprod. Process. 2021, 127, 58–76. [Google Scholar] [CrossRef]
- Okunola, A.; Adekanye, T.; Idahosa, E. Energy and exergy analyses of okra drying process in a forced convection cabinet dryer. Res. Agric. Eng. 2021, 67, 8–16. [Google Scholar] [CrossRef]
- Liu, Z.-L.; Zielinska, M.; Yang, X.-H.; Yu, X.-L.; Chen, C.; Wang, H.; Wang, J.; Pan, Z.; Xiao, H.-W. Moisturizing strategy for enhanced convective drying of mushroom slices. Renew. Energy 2021, 172, 728–739. [Google Scholar] [CrossRef]
- Aviara, N.A.; Onuoha, L.N.; Falola, O.E.; Igbeka, J.C. Energy and exergy analyses of native cassava starch drying in a tray dryer. Energy 2014, 73, 809–817. [Google Scholar] [CrossRef]
- Mokhtarian, M.; Tavakolipour, H.; Kalbasi-Ashtari, A. Energy and exergy analysis in solar drying of pistachio with air recycling system. Dry. Technol. 2016, 34, 1484–1500. [Google Scholar] [CrossRef]
- Fudholi, A.; Sopian, K.; Yazdi, M.H.; Ruslan, M.H.; Gabbasa, M.; Kazem, H.A. Performance analysis of solar drying system for red chili. Sol. Energy 2014, 99, 47–54. [Google Scholar] [CrossRef]
- Prommas, R.; Keangin, P.; Rattanadecho, P. Energy and exergy analyses in convective drying process of multi-layered porous packed bed. Int. Commun. Heat Mass Transf. 2010, 37, 1106–1114. [Google Scholar] [CrossRef]
- Argo, B.D.; Ubaidillah, U. Thin-layer drying of cassava chips in multipurpose convective tray dryer: Energy and exergy analyses. J. Mech. Sci. Technol. 2020, 34, 435–442. [Google Scholar] [CrossRef]
- Yogendrasasidhar, D.; Pydi Setty, Y. Drying kinetics, exergy and energy analyses of Kodo millet grains and Fenugreek seeds using wall heated fluidized bed dryer. Energy 2018, 151, 799–811. [Google Scholar] [CrossRef]
- Ndukwu, M.C.; Bennamoun, L.; Abam, F.I.; Eke, A.B.; Ukoha, D. Energy and exergy analysis of a solar dryer integrated with sodium sulfate decahydrate and sodium chloride as thermal storage medium. Renew. Energy 2017, 113, 1182–1192. [Google Scholar] [CrossRef]
- Kavak Akpinar, E. The effects of some exergetic indicators on the performance of thin layer drying process of long green pepper in a solar dryer. Heat Mass Transf. 2019, 55, 299–308. [Google Scholar] [CrossRef]
- Mugi, V.R.; Chandramohan, V.P. Energy and exergy analysis of forced and natural convection indirect solar dryers: Estimation of exergy inflow, outflow, losses, exergy efficiencies and sustainability indicators from drying experiments. J. Clean. Prod. 2021, 282, 124421. [Google Scholar] [CrossRef]
- Erbay, Z.; Icier, F. Energy and exergy analyses on drying of olive leaves (olea europaea L.) In tray drier. J. Food Process Eng. 2011, 34, 2105–2123. [Google Scholar] [CrossRef]
- Amjad, W.; Ali Gilani, G.; Munir, A.; Asghar, F.; Ali, A.; Waseem, M. Energetic and exergetic thermal analysis of an inline-airflow solar hybrid dryer. Appl. Therm. Eng. 2020, 166, 114632. [Google Scholar] [CrossRef]
- Szeląg-Sikora, A.; Sikora, J.; Niemiec, M.; Gródek-Szostak, Z.; Suder, M.; Kuboń, M.; Borkowski, T.; Malik, G. Solar Power: Stellar Profit or Astronomic Cost? A Case Study of Photovoltaic Installations under Poland’s National Prosumer Policy in 2016–2020. Energies 2021, 14, 4233. [Google Scholar] [CrossRef]
- Kurpaska, S.; Knaga, J.; Latała, H.; Cupiał, M.; Konopacki, P.; Hołownicki, R. The Comparison of Different Types of Heat Accumulators and Benefits of Their Use in Horticulture. Sensors 2020, 20, 1417. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zadhossein, S.; Abbaspour-Gilandeh, Y.; Kaveh, M.; Szymanek, M.; Khalife, E.; D. Samuel, O.; Amiri, M.; Dziwulski, J. Exergy and Energy Analyses of Microwave Dryer for Cantaloupe Slice and Prediction of Thermodynamic Parameters Using ANN and ANFIS Algorithms. Energies 2021, 14, 4838. [Google Scholar] [CrossRef]
- Kaveh, M.; Abbaspour-Gilandeh, Y.; Nowacka, M. Optimisation of microwave-rotary drying process and quality parameters of terebinth. Biosyst. Eng. 2021, 208, 113–130. [Google Scholar] [CrossRef]

| Temperature (°C) | Air Velocity (m/s) | ||
|---|---|---|---|
| 1 | 1.5 | 2 | |
| 40 | 4.8046 × 10−10 | 6.28 × 10−10 | 7.43347 × 10−10 |
| 50 | 8.01047 × 10−10 | 8.40453 × 10−10 | 8.71977 × 10−10 |
| 60 | 9.24047 × 10−10 | 1.02903 × 10−9 | 1.11235 × 10−9 |
| 70 | 1.17427 × 10−9 | 1.2846 × 10−9 | 1.56832 × 10−9 |
| Temperature | EUR | EU | ||||
|---|---|---|---|---|---|---|
| 1 m/s | 1.5 m/s | 2 m/s | 1 m/s | 1.5 m/s | 2 m/s | |
| 40 (°C) | 0.246 | 0.274 | 0.323 | 0.017 | 0.019 | 0.021 |
| 50 (°C) | 0.293 | 0.316 | 0.363 | 0.025 | 0.028 | 0.032 |
| 60 (°C) | 0.340 | 0.367 | 0.432 | 0.034 | 0.039 | 0.042 |
| 70 (°C) | 0.375 | 0.414 | 0.502 | 0.043 | 0.048 | 0.060 |
| Temperature | Exergy Input | Exergy Output | Exergy Loss | ||||||
|---|---|---|---|---|---|---|---|---|---|
| 1 m/s | 1.5 m/s | 2 m/s | 1 m/s | 1.5 m/s | 2 m/s | 1 m/s | 1.5 m/s | 2 m/s | |
| 40 (°C) | 0.014 | 0.016 | 0.021 | 0.005 | 0.007 | 0.011 | 0.009 | 0.010 | 0.010 |
| 50 (°C) | 0.035 | 0.041 | 0.052 | 0.019 | 0.024 | 0.032 | 0.017 | 0.017 | 0.020 |
| 60 (°C) | 0.071 | 0.077 | 0.087 | 0.049 | 0.054 | 0.063 | 0.022 | 0.023 | 0.024 |
| 70 (°C) | 0.105 | 0.118 | 0.129 | 0.079 | 0.091 | 0.101 | 0.026 | 0.027 | 0.028 |
| Temperature (°C) | Air Velocity (m/s) | ||
|---|---|---|---|
| 1 | 1.5 | 2 | |
| 40 | 64.836 | 59.649 | 55.350 |
| 50 | 56.381 | 48.374 | 44.986 |
| 60 | 40.034 | 36.136 | 31.316 |
| 70 | 32.310 | 29.558 | 24.854 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karami, H.; Kaveh, M.; Golpour, I.; Khalife, E.; Rusinek, R.; Dobrzański, B., Jr.; Gancarz, M. Thermodynamic Evaluation of the Forced Convective Hybrid-Solar Dryer during Drying Process of Rosemary (Rosmarinus officinalis L.) Leaves. Energies 2021, 14, 5835. https://doi.org/10.3390/en14185835
Karami H, Kaveh M, Golpour I, Khalife E, Rusinek R, Dobrzański B Jr., Gancarz M. Thermodynamic Evaluation of the Forced Convective Hybrid-Solar Dryer during Drying Process of Rosemary (Rosmarinus officinalis L.) Leaves. Energies. 2021; 14(18):5835. https://doi.org/10.3390/en14185835
Chicago/Turabian StyleKarami, Hamed, Mohammad Kaveh, Iman Golpour, Esmail Khalife, Robert Rusinek, Bohdan Dobrzański, Jr., and Marek Gancarz. 2021. "Thermodynamic Evaluation of the Forced Convective Hybrid-Solar Dryer during Drying Process of Rosemary (Rosmarinus officinalis L.) Leaves" Energies 14, no. 18: 5835. https://doi.org/10.3390/en14185835
APA StyleKarami, H., Kaveh, M., Golpour, I., Khalife, E., Rusinek, R., Dobrzański, B., Jr., & Gancarz, M. (2021). Thermodynamic Evaluation of the Forced Convective Hybrid-Solar Dryer during Drying Process of Rosemary (Rosmarinus officinalis L.) Leaves. Energies, 14(18), 5835. https://doi.org/10.3390/en14185835










