Effect of Operating Conditions on the Performance of Gas–Liquid Mixture Roots Pumps
Abstract
Highlights
- The performance of the gas–liquid mixture Roots pump at different inlet CO2 volume conditions, rotational speeds, and pressure ratios is investigated;
- The influence of the pressure ratio and the gas volume fraction on the pump leakage is discussed;
- The outlet zone pressure and the working fluid properties are considered to be the main factors affecting the performance of the gas–liquid mixture Roots pump.
1. Introduction
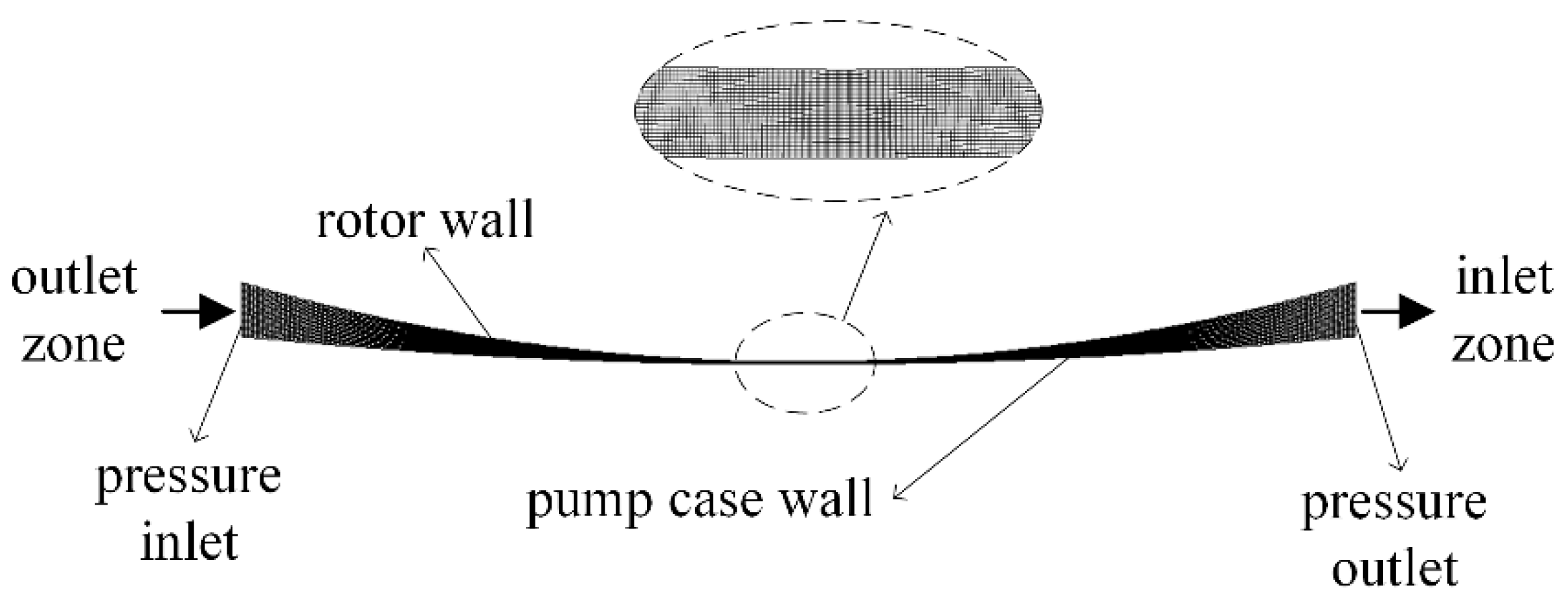
2. Problem Formulation
3. Computational Model and Validation
3.1. Computational Model
- The two-dimensional model is adopted in this paper to simplify the simulation, which ignores the axial flow within the Roots pump. By comparing the results from two-dimensional and three-dimensional models, [17] concluded that the two-dimensional model is consistent with experimental results in terms of the average parameters and pressure field, but not suitable for the calculation of the velocity field;
- The continuity equation is used in the simulations to describe the characteristics of the fluid, so the aggregation and breakage of the liquid phase cannot be included;
- Though CO2 and water are adopted as the working fluids, the dissolution of CO2 is not considered in the simulations. The CO2 solubility is low at high temperature; otherwise, the operation of the pump blades may cause the CO2 solubility to be less than the theoretical value, as described in [1]. Hence, this assumption is reasonable in the Roots pump simulations.
3.2. Experimental Validation
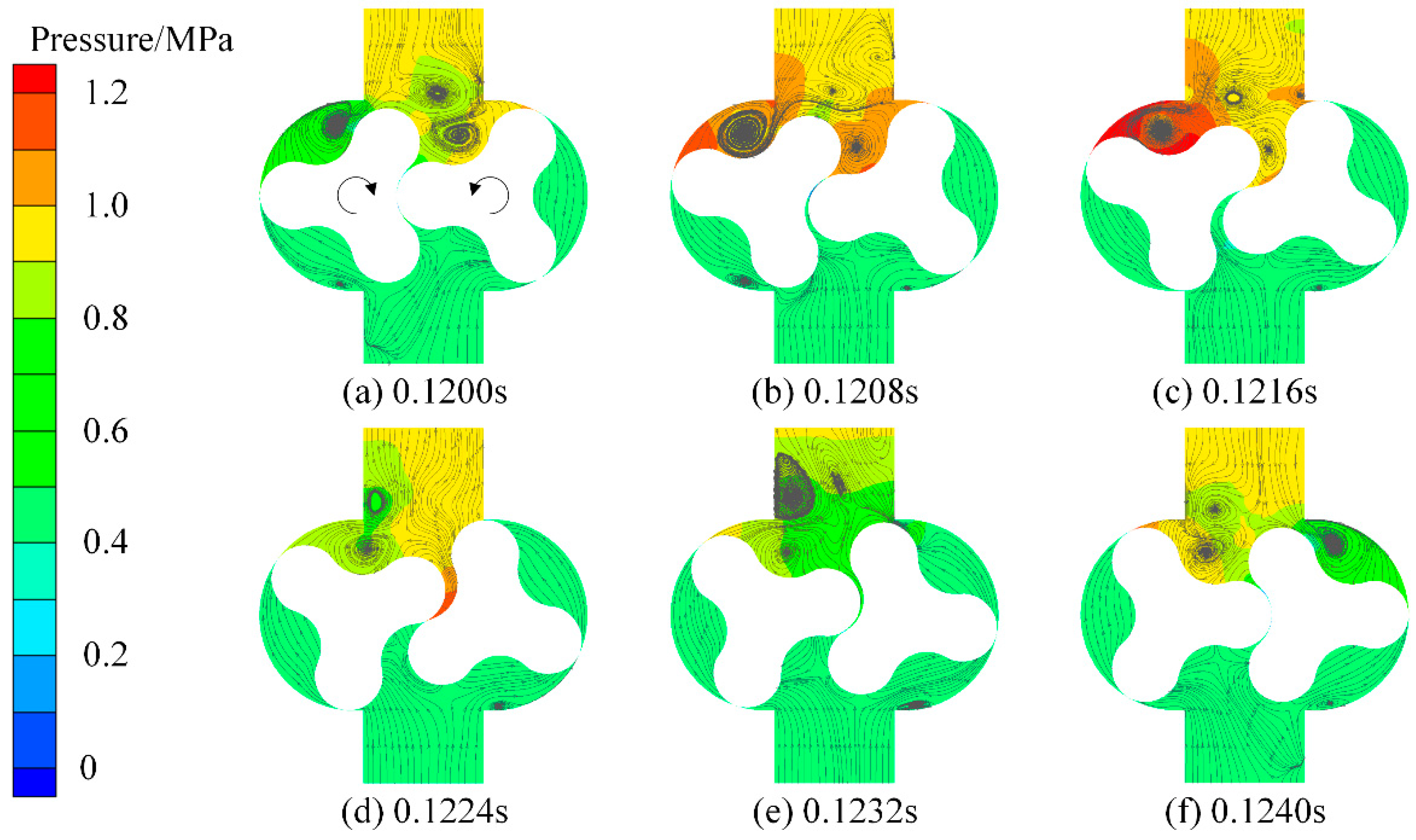
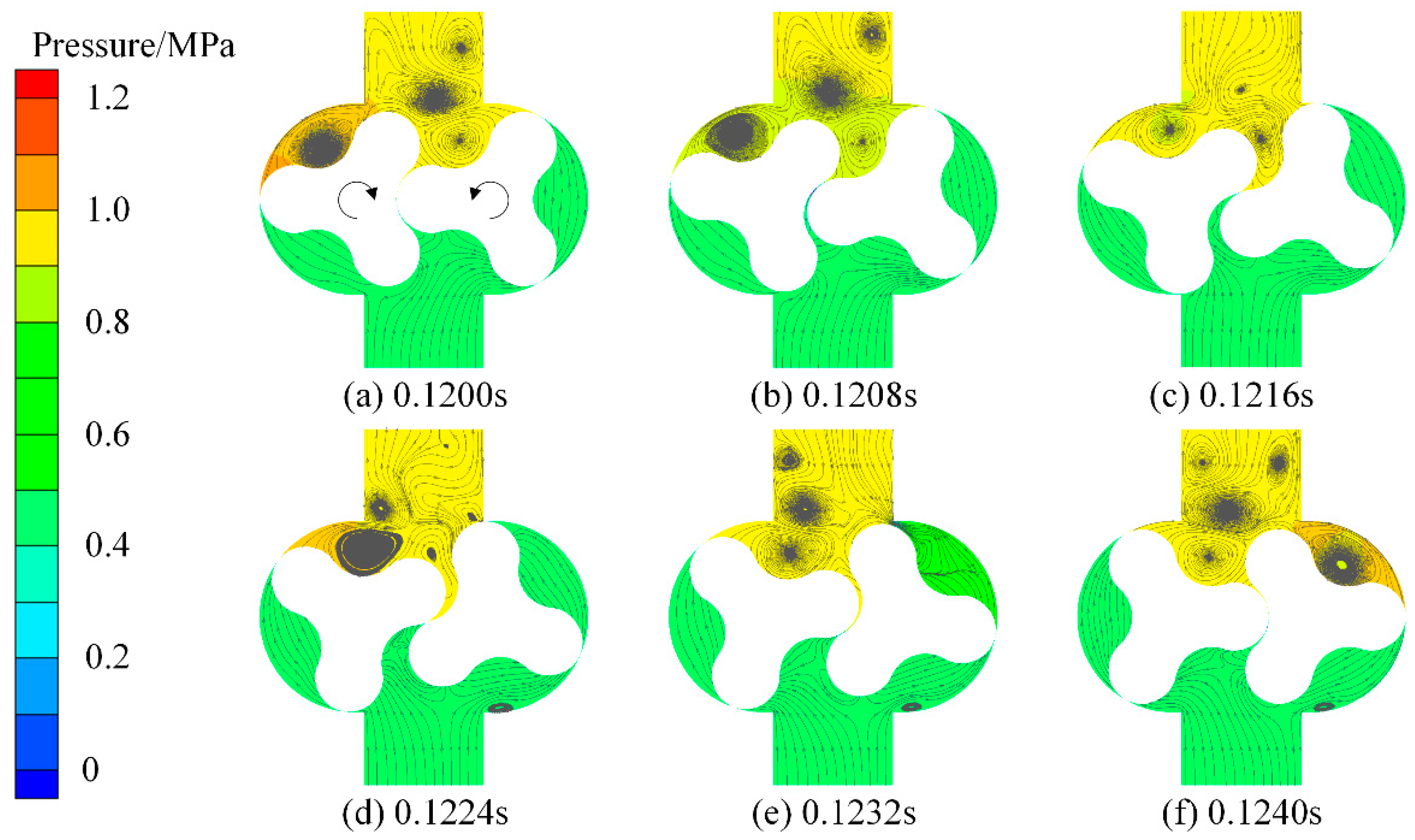
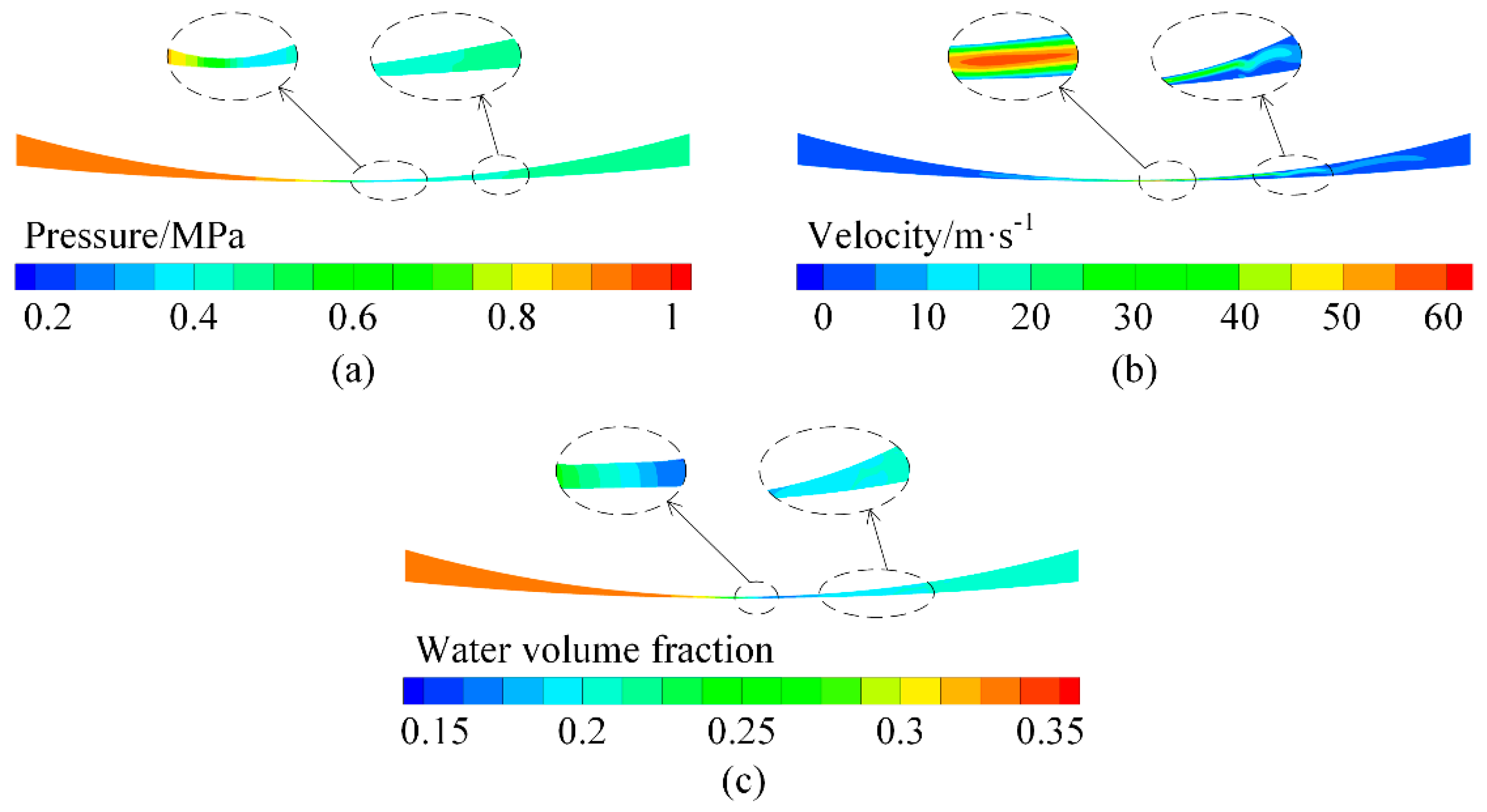
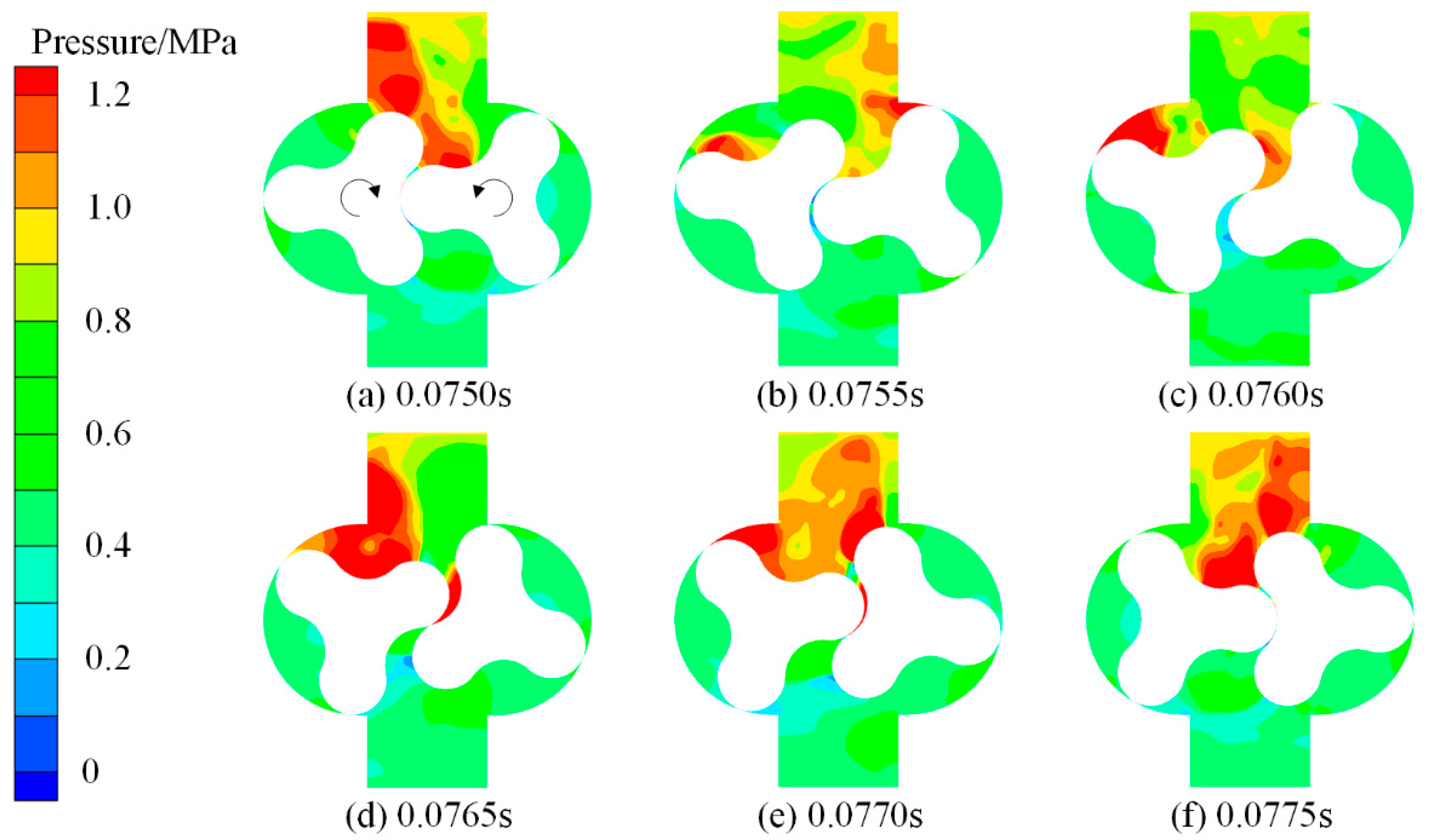
4. Results and Discussion
4.1. Effect of Inlet CO2 Volume Fraction
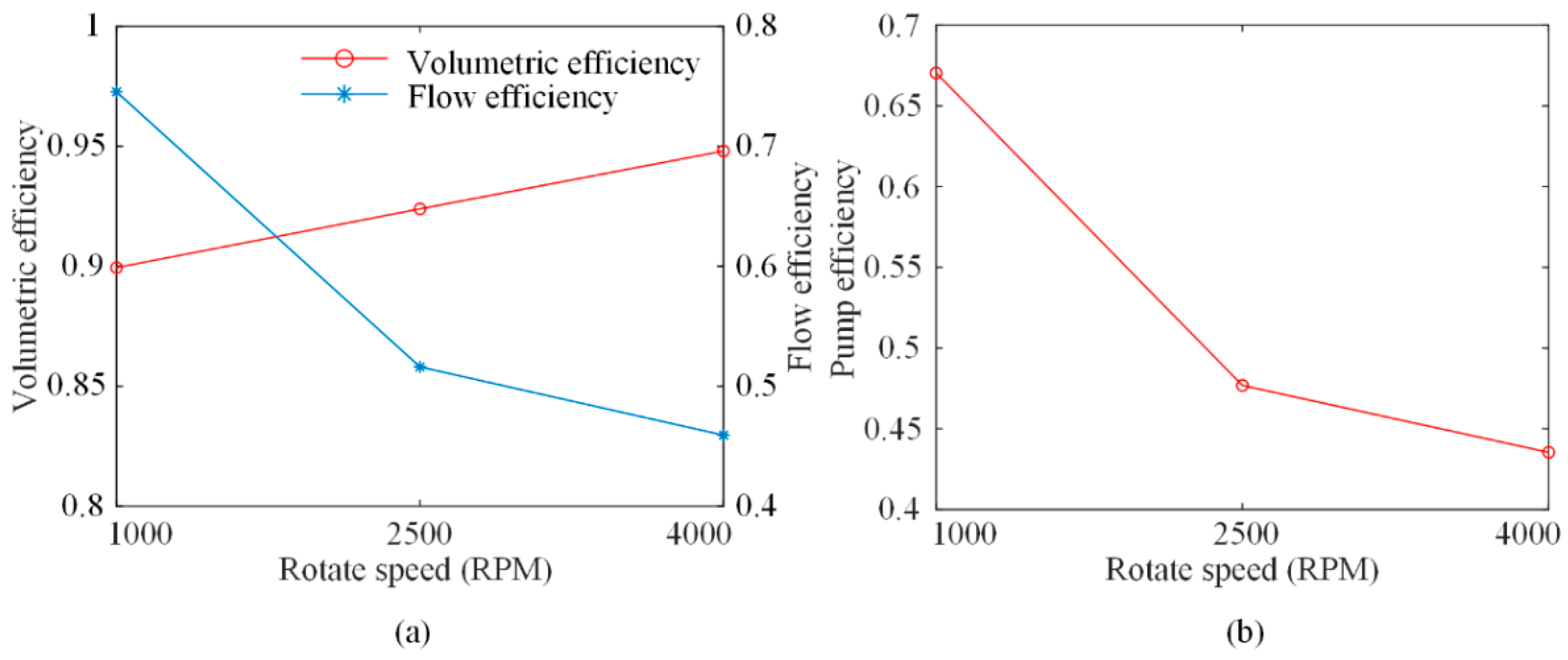
4.2. Effect of Rotational Speed
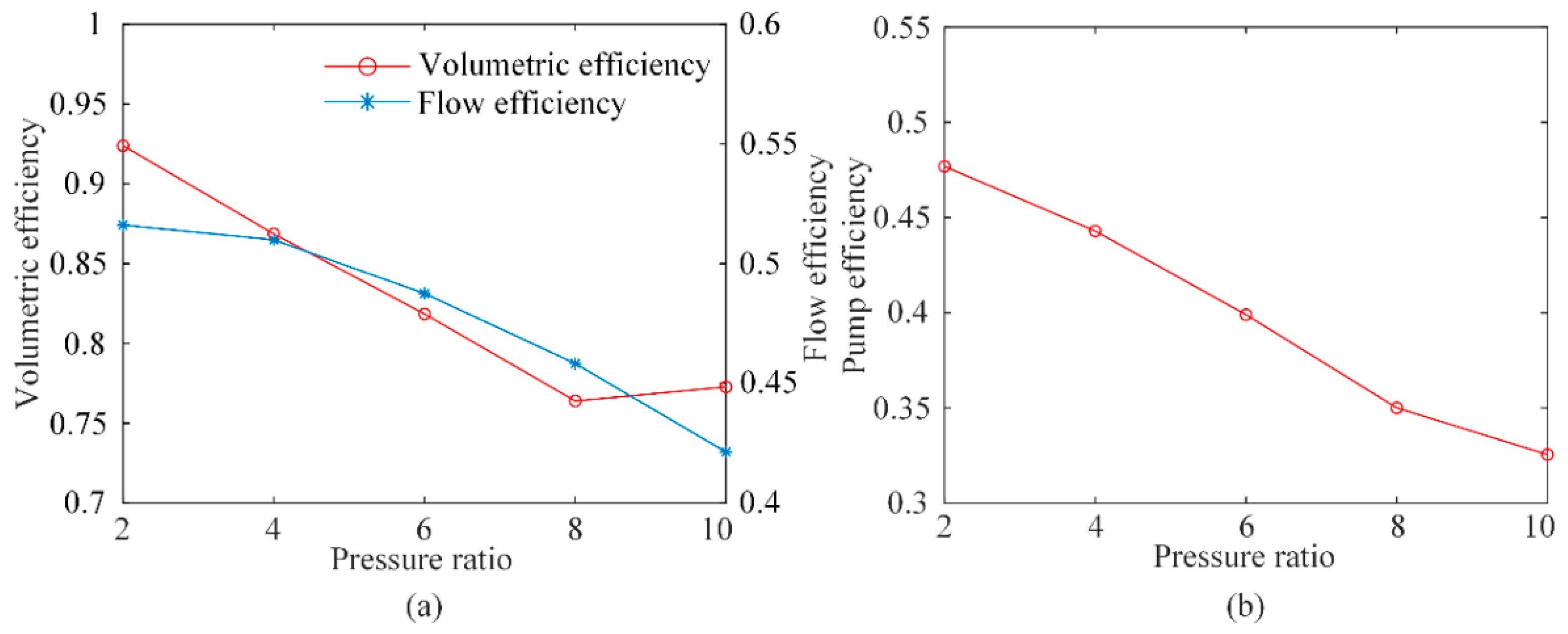
4.3. Effect of Pressure Ratio
5. Conclusions
- (1)
- When the inlet CO2 volume fraction increases from 0.8 to 1, the volumetric efficiency first increases from 92% to 97%, then decreases sharply to 80%. The flow efficiency increases from 52% to 73%, while the pump efficiency also increases from 48% to 64%, and then decreases to 59%. This is due to the differences in the properties of the gas and liquid phases. The decrease in the liquid phase volume flow leads to the deterioration of the sealing of the gaps and reduces the backflow’s impact in the outlet zone;
- (2)
- When the rotational speed increases from 1000 rpm to 4000 rpm, the volumetric efficiency increases from 90% to 95%, the flow efficiency decreases from 75% to 46%, and the pump efficiency decreases from 67% to 43%. The pressure difference between the inlet and outlet is considered to be the main factor governing the leakage in the Roots pump. The volumetric efficiency increases with the increase in the rotational speed, since the leakage remains unchanged while the theoretical volume flow rate increases. At high rotational speeds, the extrusion effect of the rotors on the fluid in the pump is more significant, leading to a decrease in flow efficiency;
- (3)
- As the pressure ratio increases from 2 to 10, the volumetric efficiency drops from 92% to 77% and then remains stable, while the flow efficiency drops from 52% to 42%, resulting in a decrease in the pump efficiency from 48% to 33%. The increase in the pressure ratio enhances the leakage, but it also reduces the gas volume fraction in the outlet zone, which is helpful in reducing the leakage. The combined effects make the volume efficiency first decrease, and then stabilize. Both of the effects can aggravate the impact of the fluid in the outlet zone and reduce the flow efficiency.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Half-center distance of rotors | |
| Distance of rotor and addendum arc center | |
| Rotor diameter | |
| Inlet and outlet diameter | |
| Internal energy of phase k | |
| Body force | |
| Gravitational acceleration | |
| Sensible enthalpy of phase k | |
| Number of standard uncertainty component | |
| Isentropic index; phase k | |
| Confidence factor | |
| Effective thermal conductivity | |
| Rotor length | |
| Liquid theoretical mass flow rate | |
| Mass exchange | |
| Moment of left rotor | |
| Moment of right rotor | |
| Number of standard uncertainty component | |
| Number of phases; number of measurements; rotational speed | |
| Computational domain pressure | |
| Inlet pressure | |
| Outlet pressure | |
| Theoretical volume flow rate | |
| Liquid phase theoretical volume flow rate | |
| Gas phase theoretical volume flow rate | |
| Liquid phase actual volume flow rate | |
| Gas phase actual volume flow rate | |
| Maximum rotor radius | |
| Minimum rotor radius; gas constant | |
| Radius of addendum arc | |
| Actual radius of addendum arc | |
| Actual radius of dedendum arc | |
| Standard deviation | |
| Volume heat source | |
| Computational domain temperature | |
| Time step | |
| Inlet temperature | |
| Extended uncertainty | |
| Multiphase mass average velocity | |
| Slip velocity of phase k | |
| Relative velocity of phase k | |
| X coordinate; direct measurement parameter | |
| Y coordinate; indirect measurement parameter | |
| Lobe number | |
| Angular coordinate; half-width of measure interval | |
| Rotors clearance | |
| Rotor area utilization coefficient | |
| Angular velocity of left rotor | |
| Angular velocity of right rotor | |
| Pump efficiency | |
| Flow efficiency | |
| Volume efficiency | |
| Density of gas–liquid mixture | |
| Density of phase k | |
| Volume fraction of phase k | |
| Type-A standard uncertainty | |
| Type-B standard uncertainty | |
| Combined standard uncertainty | |
| Dynamic viscosity of gas–liquid mixture | |
| Standard uncertainty component | |
| Dynamic viscosity of phase k |
References
- Kiely, D. Review of underwater thermal propulsion. In Proceedings of the 30th Joint Propulsion Conference and Exhibit, Indianapolis, IN, USA, 27–29 June 1994; Institute of Aeronautics and Astronautics: Reston, VA, USA, 1994; pp. 1–5. [Google Scholar]
- Si, Q.; Bois, G.; Liao, M.; Zhang, H.; Cui, Q.; Yuan, S. A Comparative Study on Centrifugal Pump Designs and Two-Phase Flow Characteristic under Inlet Gas Entrainment Conditions. Energies 2019, 13, 65. [Google Scholar] [CrossRef]
- Cao, F.; Gao, T.; Li, S.; Xing, Z.; Shu, P. Experimental analysis of pressure distribution in a twin screw compressor for multiphase duties. Exp. Therm. Fluid Sci. 2011, 35, 219–225. [Google Scholar] [CrossRef]
- He, Z.; Wang, T.; Wang, X.; Peng, X.; Xing, Z. Experimental Investigation into the Effect of Oil Injection on the Performance of a Variable Speed Twin-Screw Compressor. Energies 2018, 11, 1342. [Google Scholar] [CrossRef]
- Lobsinger, T.; Hieronymus, T.; Brenner, G. A CFD Investigation of a 2D Balanced Vane Pump Focusing on Leakage Flows and Multiphase Flow Characteristics. Energies 2020, 13, 3314. [Google Scholar] [CrossRef]
- Zhang, W.; Yu, Z.; Zahid, M.N.; Li, Y. Study of the Gas Distribution in a Multiphase Rotodynamic Pump Based on Interphase Force Analysis. Energies 2018, 11, 1069. [Google Scholar] [CrossRef]
- Guo, Q.; Qin, K.; Li, D.; Huang, C.; Luo, K. Numerical investigation and performance enhancement of roots pumps operating with gas-liquid mixtures. Vacuum 2020, 176, 109303. [Google Scholar] [CrossRef]
- Cai, Y.; Yao, L.; Wei, G. Generation of tooth profile for Roots rotor based on virtual linkage associated with Assur group. Adv. Mech. Eng. 2016, 8, 1–8. [Google Scholar] [CrossRef]
- Bhuyan, P.; Ghosh, S. Performance prediction of Roots blower based on shape of the rotor-profile using FVM with adaptive mesh redistribution technique and GA-tuned neural network. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 433. [Google Scholar] [CrossRef]
- Wang, J.; Liu, R.; Yang, S.; Li, H.; Wang, Z.; Li, Q. Geometric study and simulation of an elliptical rotor profile for Roots vacuum pumps. Vacuum 2018, 153, 168–175. [Google Scholar] [CrossRef]
- Laczik, B.; Zentay, P.; Horvath, R. A new approach for designing gear profiles using closed complex equations. Acta Polytech. Hung. 2014, 11, 159–172. [Google Scholar]
- Hsieh, C.-F. A new curve for application to the rotor profile of rotary lobe pumps. Mech. Mach. Theory 2015, 87, 70–81. [Google Scholar] [CrossRef]
- Maqsood, M.; Usman, A.; Bodla, M.F.; Ali, J. Evaluation of performance parameters of indigenously developed roots pumping system. IOP Conf. Ser. Mater. Sci. Eng. 2016, 146, 012047. [Google Scholar] [CrossRef]
- Jou, R.-Y.; Huang, W.-H. A Measurement System for the Performance Evaluation of Roots Blowers. In Proceedings of the 2018 IEEE International Conference on Advanced Manufacturing (ICAM), Yunlin, Taiwan, 16–18 November 2018; Institute of Electrical and Electronics Engineers (IEEE): Piscataway, NJ, USA, 2018; pp. 380–383. [Google Scholar]
- Singh, G.; Sun, S.; Kovacevic, A.; Li, Q.; Bruecker, C. Transient flow analysis in a Roots blower: Experimental and numerical investigations. Mech. Syst. Signal Process. 2019, 134, 106305. [Google Scholar] [CrossRef]
- Huang, S.; Guo, J.; Yang, F.X. Numerical simulation of 3D unsteady flow in a rotating pump by dynamic mesh technique. IOP Conf. Ser. Mater. Sci. Eng. 2013, 52, 022030. [Google Scholar] [CrossRef]
- Sun, S.; Zhao, B.; Jia, X. Three-dimensional numerical simulation and experimental validation of flows in working cham-bers and inlet/outlet pockets of Roots pump. Vacuum 2017, 137, 195–204. [Google Scholar] [CrossRef]
- Peng, X.; He, Z.; Shu, P. Design improvement on involute profile of Roots blower rotors. Compress. Blower Fan Technol. 2000, 3, 3–5. (In Chinese) [Google Scholar]
- Zhang, Q.; Wang, S.; Shi, J.; Wang, X.; Tomovic, M. Performance degradation model of roots pump in vacuum system based on leakage of rotor wear. In Proceedings of the 2016 IEEE 11th Conference on Industrial Electronics and Applications (ICIEA), Hefei, China, 5–7 June 2016; pp. 2174–2179. [Google Scholar] [CrossRef]
- Gordeeva, U.S.; Demikhov, K.E.; Ochkov, A.A. Specifics of the application of mechanical vacuum pumps in vacuum distillation units used in chemical and oil and gas industries. Nanosci. Nanotech. Nano-SciTech 2019, 2141, 030011. [Google Scholar] [CrossRef]
- Casari, N.; Fadiga, E.; Pinelli, M.; Suman, A.; Kovacevic, A.; Rane, S.; Ziviani, D. Numerical investigation of oil injection in a Roots blower operated as expander. IOP Conf. Ser. Mater. Sci. Eng. 2019, 604, 012075. [Google Scholar] [CrossRef]
- Hsieh, C.-F.; Deng, Y.-C. A design method for improving the flow characteristics of a multistage Roots pumps. Vacuum 2015, 121, 217–222. [Google Scholar] [CrossRef]
- Hsieh, C.-F.; Zhou, Q.-J. Fluid analysis of cylindrical and screw type Roots vacuum pumps. Vacuum 2015, 121, 274–282. [Google Scholar] [CrossRef]
- Liu, X.; Lu, J.; Gao, R. Numerical investigation of the aerodynamic performance affected by spiral inlet and outlet in a posi-tive displacement blower. Chin. J. Mech. Eng. 2013, 26, 957–966. [Google Scholar] [CrossRef]
- Sun, S.-K.; Jia, X.-H.; Xing, L.-F.; Peng, X.-Y. Numerical study and experimental validation of a Roots blower with backflow design. Eng. Appl. Comput. Fluid Mech. 2018, 12, 282–292. [Google Scholar] [CrossRef]
- Li, Y.-B.; Guo, D.-S.; Li, X.-B. Mitigation of radial exciting force of rotary lobe pump by gradually varied gap. Eng. Appl. Comput. Fluid Mech. 2018, 12, 711–723. [Google Scholar] [CrossRef]
- Cai, Y.; Yao, L. Roots Blower with Gradually Expanding Outlet Gap: Mathematical Modelling and Performance Simulation. In Proceedings of the 2nd International Conference on Advances in Mechanical Engineering and Industrial Informatics (AMEII 2016), Hangzhou, China, 9–10 April 2016; pp. 653–660. [Google Scholar] [CrossRef][Green Version]
- Ohtani, I.; Iwamoto, T. Reduction of Noise in Roots Blower. Bull. JSME 1981, 24, 547–554. [Google Scholar] [CrossRef]
- Vizgalov, S.V.; Volkov, M.V.; Chekushkin, G.N.; Khisameev, I.G. Enhancing the efficiency of a three-lobe roots blower by means of by-passing gas to the working cavity through an ejector adaptor. In Proceedings of the Oil and Gas Engineering (OGE-2017), Omsk, Russia, 24 April 2017; pp. 1–7. [Google Scholar] [CrossRef]
- Vizgalov, S.V.; Chekushkin, G.N.; Volkov, M.V. Investigation of three lobes roots blower with special ejector. IOP Conf. Ser. Mater. Sci. Eng. 2015, 90, 012082. [Google Scholar] [CrossRef]
- Xing, L.; Feng, J.; Chen, W.; Xing, Z.; Peng, X. Development and Testing of a Roots Pump for Hydrogen Recirculation in Fuel Cell System. Appl. Sci. 2020, 10, 8091. [Google Scholar] [CrossRef]
- Feng, J.; Xing, L.; Wang, B.; Wei, H.; Xing, Z. Effects of Working Fluids on the Performance of a Roots Pump for Hydrogen Recirculation in a PEM Fuel Cell System. Appl. Sci. 2020, 10, 8069. [Google Scholar] [CrossRef]
- De Graff, B.; Howell, M.; Kim, S. SNS cryogenic test facility Kinney vacuum pump commissioning and operation at 2K. In Proceedings of the Joint Cryogenic Engineering Conference/International Cryogenic Materials Conference, Madison, WI, USA, 9–13 July 2017; Iop Publishing Ltd.: Bristol, UK; Volume 278, p. 012161. [Google Scholar]
- He, Y.; Sun, D.; Liu, J.; Zhu, B. Optimization of a turbocharger and supercharger compound boosting system for a Miller cycle engine. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2017, 232, 238–253. [Google Scholar] [CrossRef]
- Su, C. Roots Blower and Application; Changsha Central South University of Technology Press: Changsha, China, 1999; pp. 29–37. (In Chinese) [Google Scholar]
- Ansys. Ansys Help, Ansys Fluent; Version 18.0; ANSYS Inc.: Canonsburg, PA, USA, 2016. [Google Scholar]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32. [Google Scholar] [CrossRef]
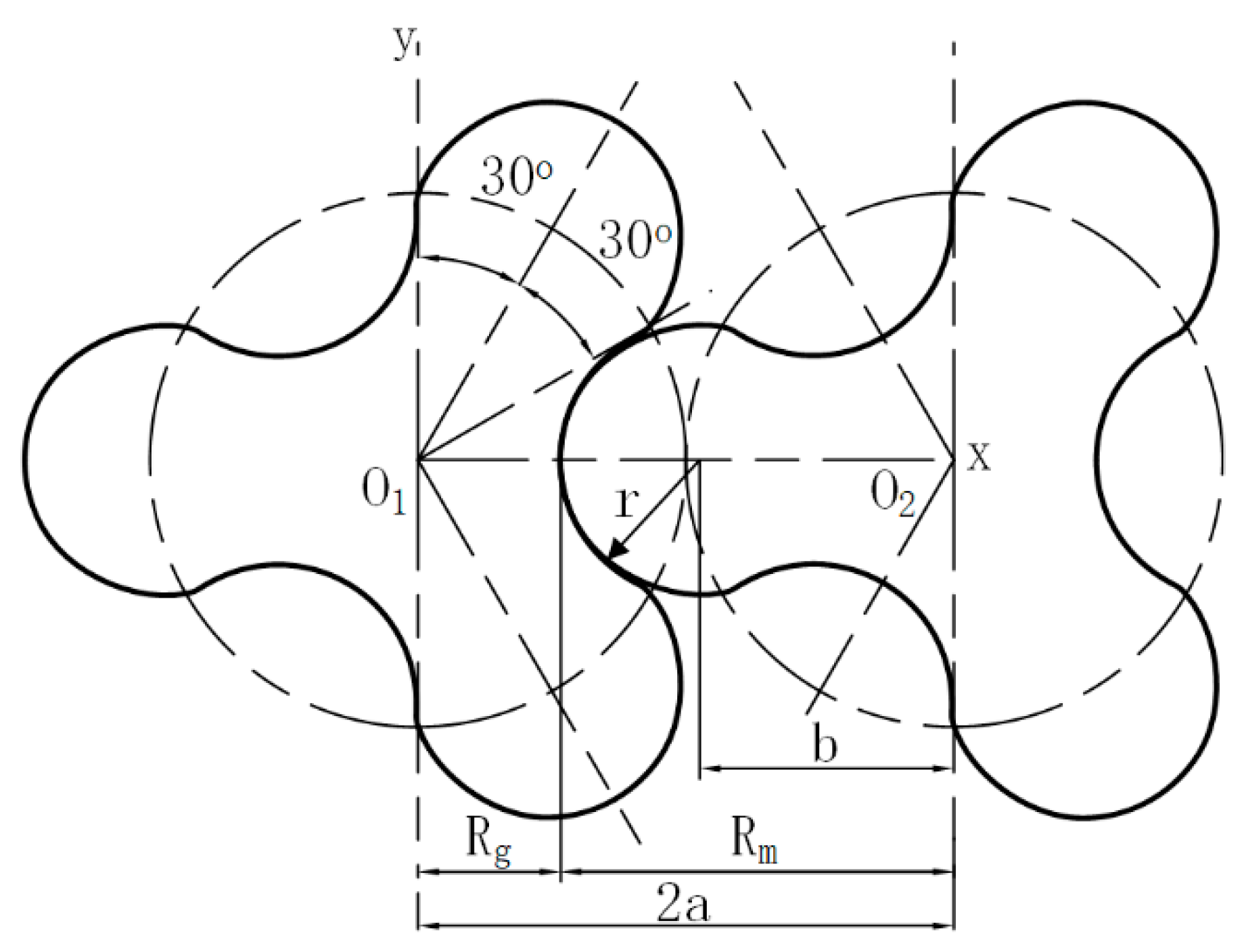


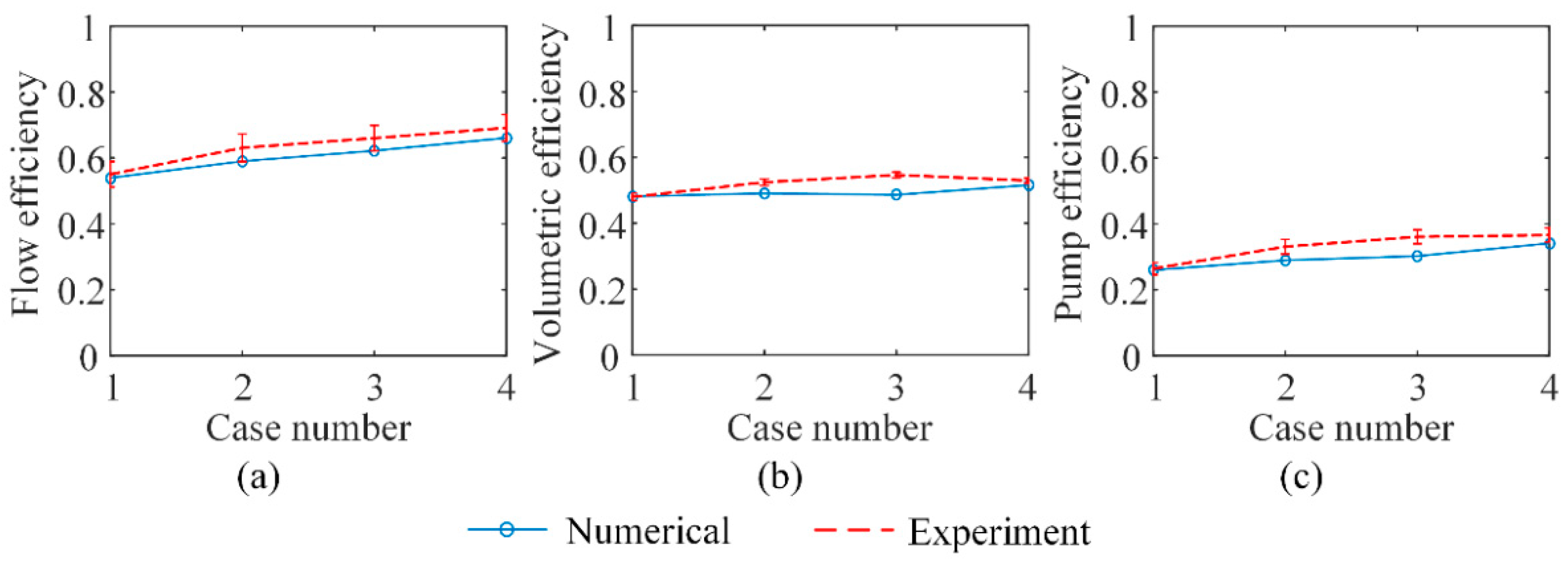
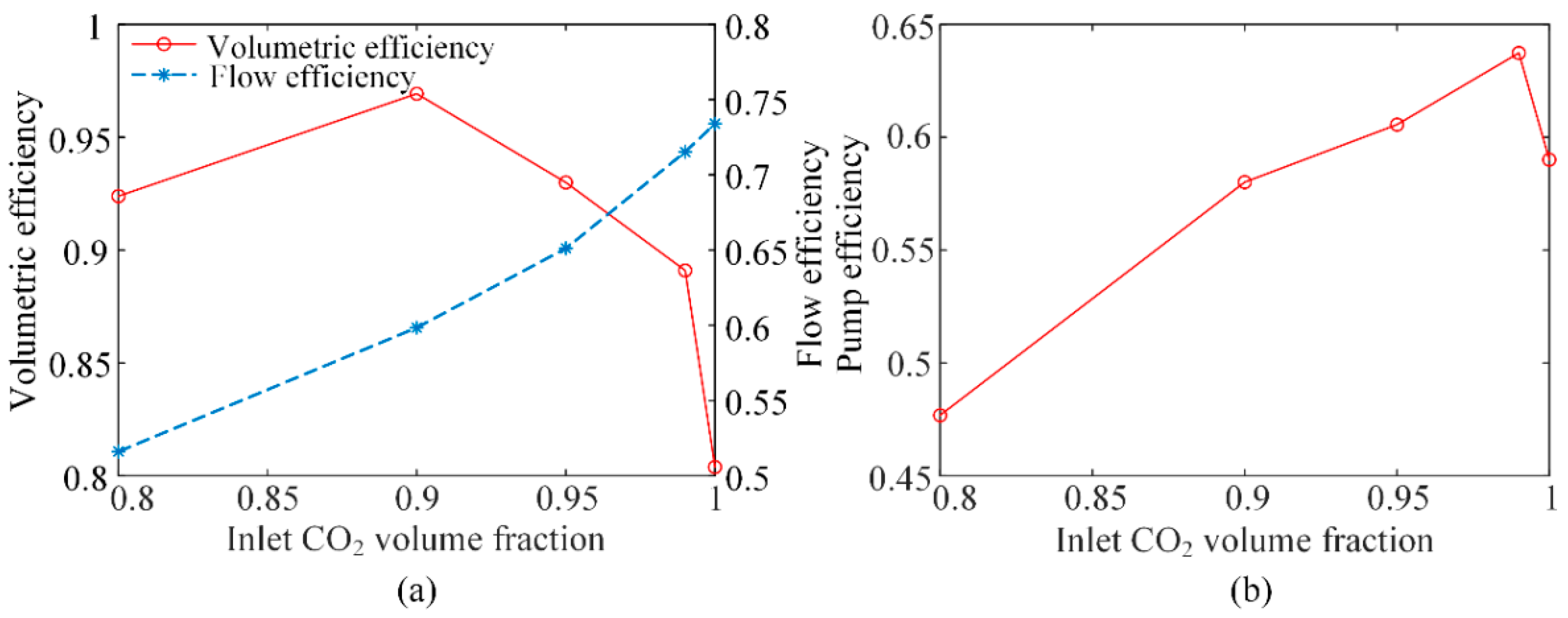

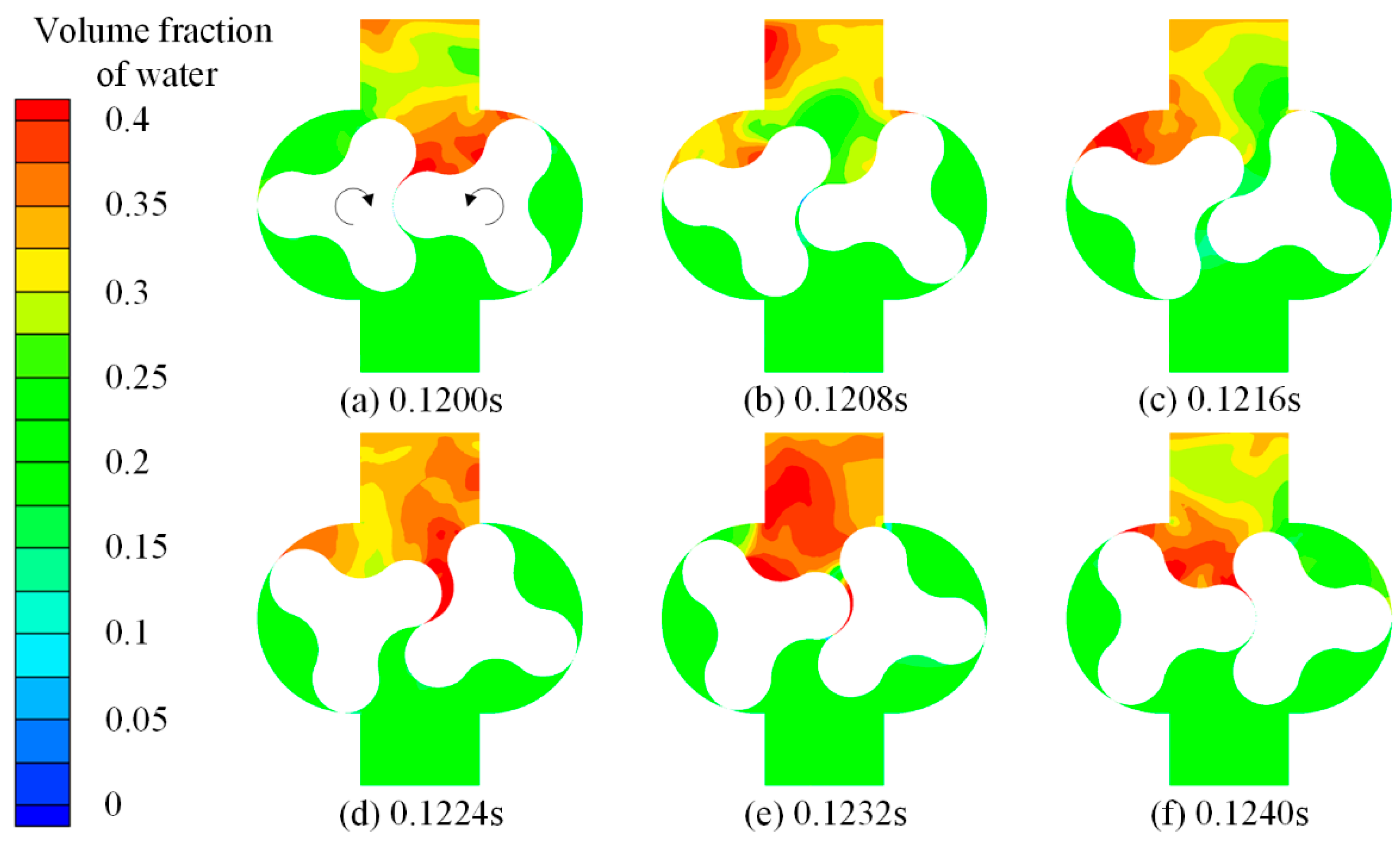








| Parameter | Value |
|---|---|
| D | 0.102 m |
| D0 | 0.064 m |
| Rm | 0.051 m |
| Rg | 0.022 m |
| a | 0.037 m |
| b | 0.033 m |
| r | 0.018 m |
| L | 0.134 m |
| δ | 0.02 mm |
| Instrument | Measuring Range | Accuracy |
|---|---|---|
| Gas flow meter | 0.8~8 m3/h | ±0.108 m3/h |
| Water flow meter | 63~630 L/h | ±8.505 L/h |
| Pressure transducer | 0~0.5 MPa | ±1250 Pa |
| Temperature transducer | 0~100 °C | ±0.2 °C |
| Tachograph | 2.5~999.9 PRM | ±0.5 RPM |
| Condition Number | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| Rotational speed (rpm) | 800 | 801 | 798 | 796 |
| Averaged gas mass flow rate (g/s) | 0.99 | 1.32 | 1.65 | 1.98 |
| Averaged water mass flow rate (kg/s) | 0.14 | 0.14 | 0.14 | 0.14 |
| Averaged inlet pressure (Pa) | 35,594 | 43,156 | 51,875 | 64,469 |
| Averaged outlet pressure (Pa) | 141,156 | 148,781 | 164,375 | 177,438 |
| Averaged inlet temperature (k) | 289.6 | 289.7 | 289.8 | 289.5 |
| Averaged outlet temperature (k) | 290.0 | 290.0 | 289.9 | 289.7 |
| Case Numbers | Inlet Pressure | Outlet Pressure | Rotational Speed | Inlet CO2 Volume Fraction |
|---|---|---|---|---|
| 1 | 0.46 MPa | 0.92 MPa | 2500 rpm | 0.80 |
| 2 | 0.90 | |||
| 3 | 0.95 | |||
| 4 | 0.99 | |||
| 5 | 1.00 |
| Case Numbers | Inlet Pressure | Outlet Pressure | Inlet CO2 Volume Fraction | Rotational Speed |
|---|---|---|---|---|
| 1 | 0.46 MPa | 0.92 MPa | 0.80 | 1000 rpm |
| 2 | 2500 rpm |
| Case Numbers | Inlet Pressure | Inlet CO2 Volume Fraction | Rotational Speed | Pressure Ratio |
|---|---|---|---|---|
| 1 | 0.46 MPa | 0.8 | 2500 rpm | 4 |
| 2 | 6 | |||
| 3 | 8 | |||
| 4 | 10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, Q.; Luo, K.; Li, D.; Huang, C.; Qin, K. Effect of Operating Conditions on the Performance of Gas–Liquid Mixture Roots Pumps. Energies 2021, 14, 5361. https://doi.org/10.3390/en14175361
Guo Q, Luo K, Li D, Huang C, Qin K. Effect of Operating Conditions on the Performance of Gas–Liquid Mixture Roots Pumps. Energies. 2021; 14(17):5361. https://doi.org/10.3390/en14175361
Chicago/Turabian StyleGuo, Qing, Kai Luo, Daijin Li, Chuang Huang, and Kan Qin. 2021. "Effect of Operating Conditions on the Performance of Gas–Liquid Mixture Roots Pumps" Energies 14, no. 17: 5361. https://doi.org/10.3390/en14175361
APA StyleGuo, Q., Luo, K., Li, D., Huang, C., & Qin, K. (2021). Effect of Operating Conditions on the Performance of Gas–Liquid Mixture Roots Pumps. Energies, 14(17), 5361. https://doi.org/10.3390/en14175361








