Comparison of 3D Solid and Beam–Spring FE Modeling Approaches in the Evaluation of Buried Pipeline Behavior at a Strike-Slip Fault Crossing
Abstract
:1. Introduction
2. Analytical Evaluation of Buried Pipeline Behavior
3. Modeling Procedure
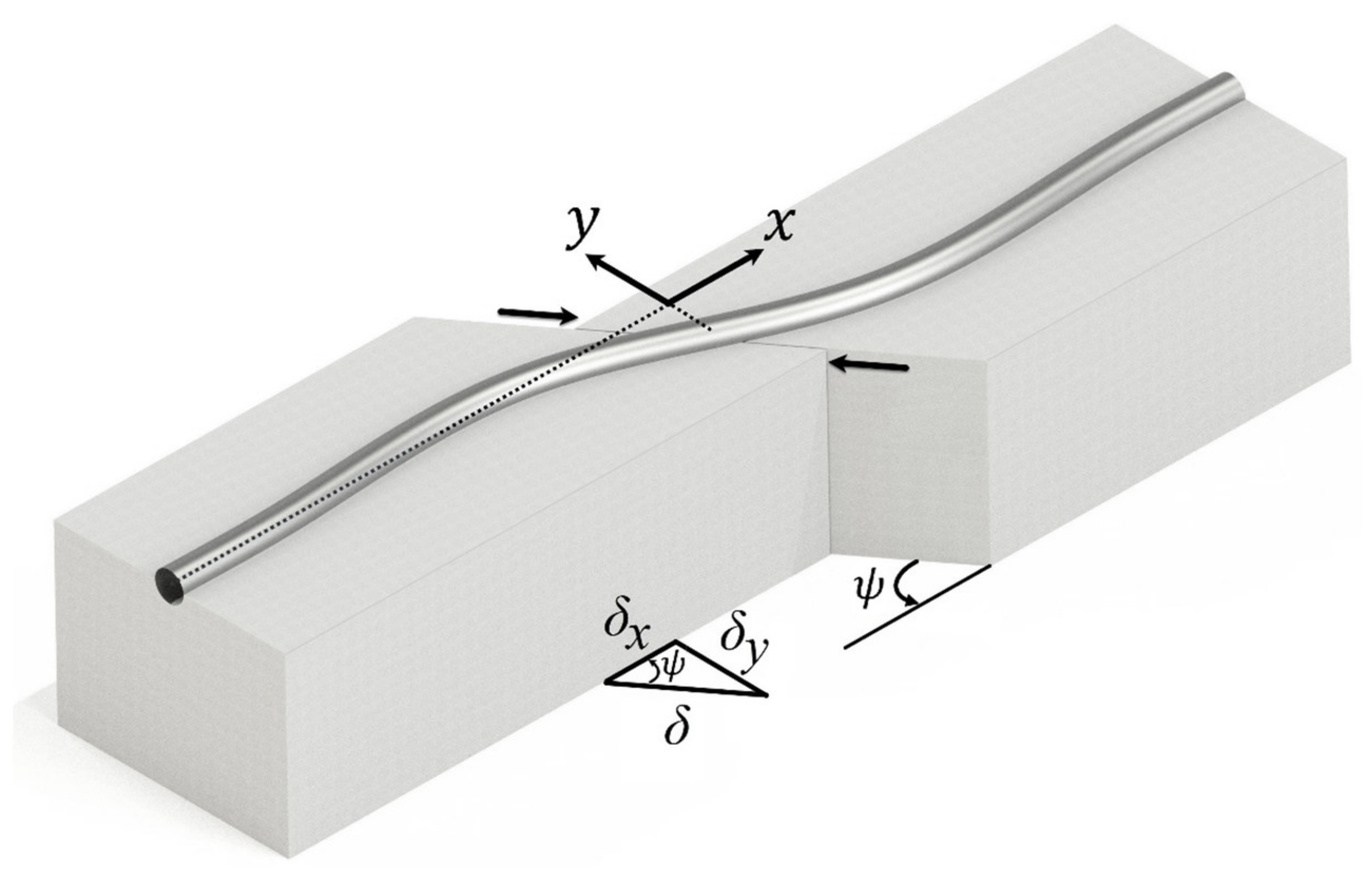
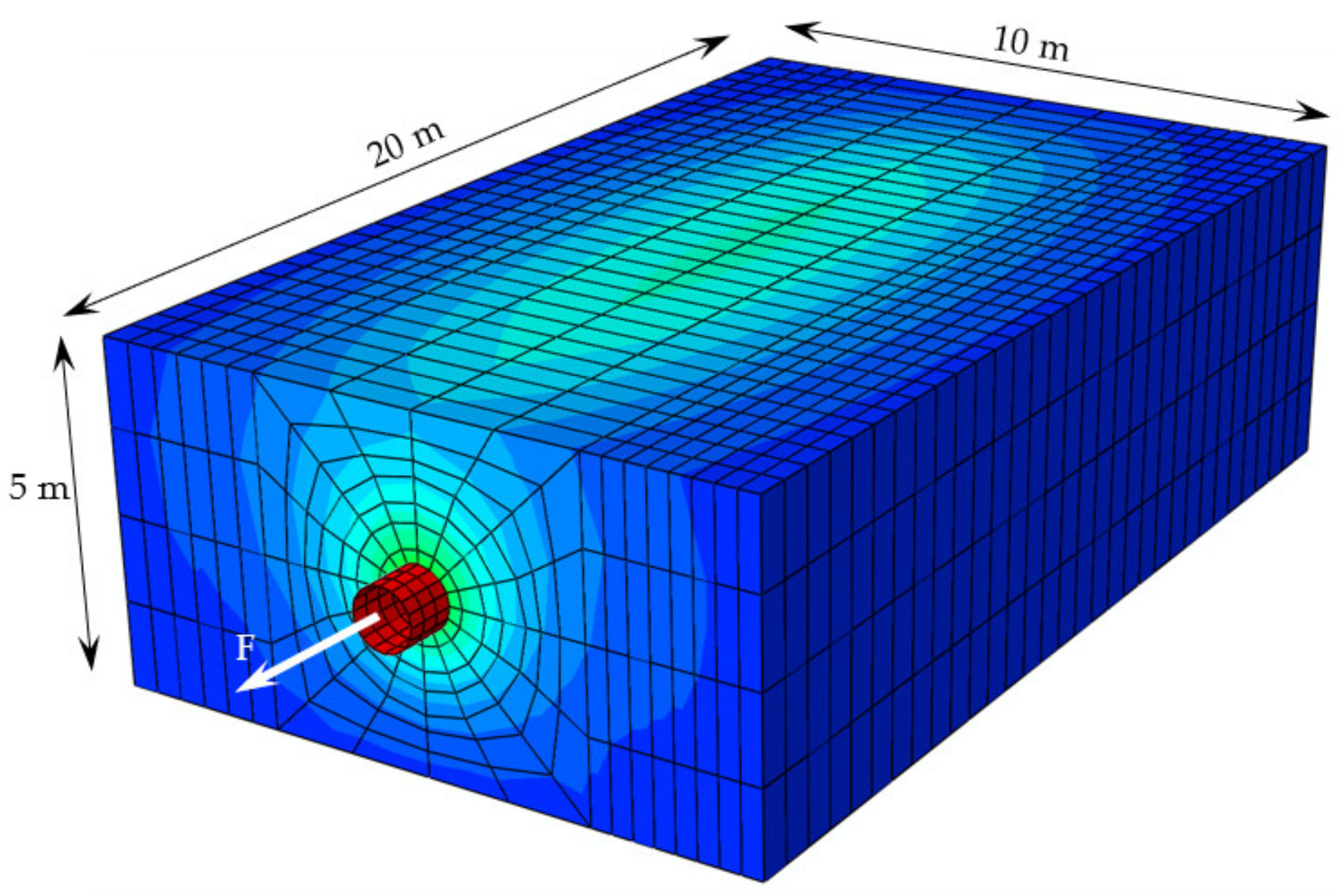
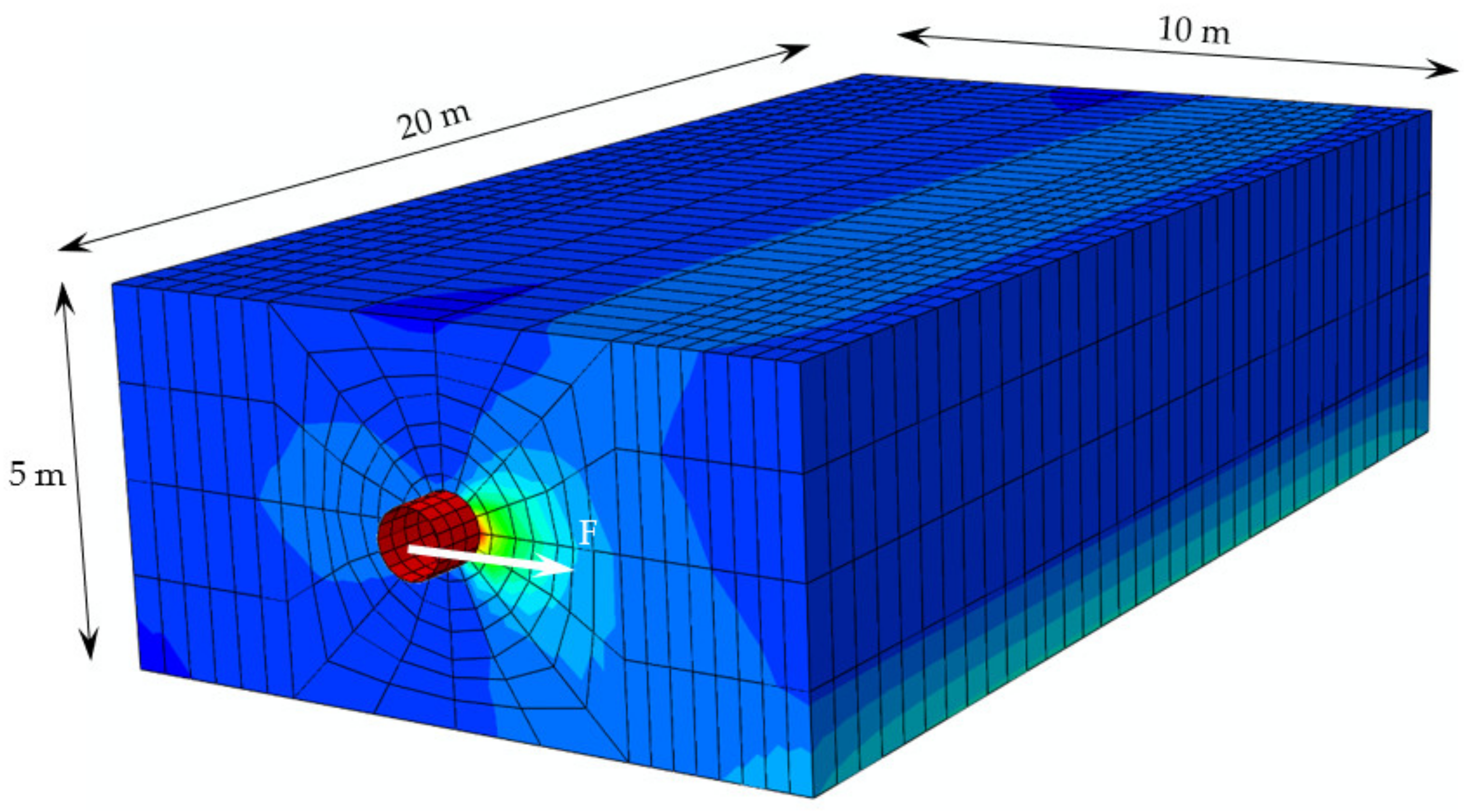
4. Longitudinal and Lateral Test Modeling
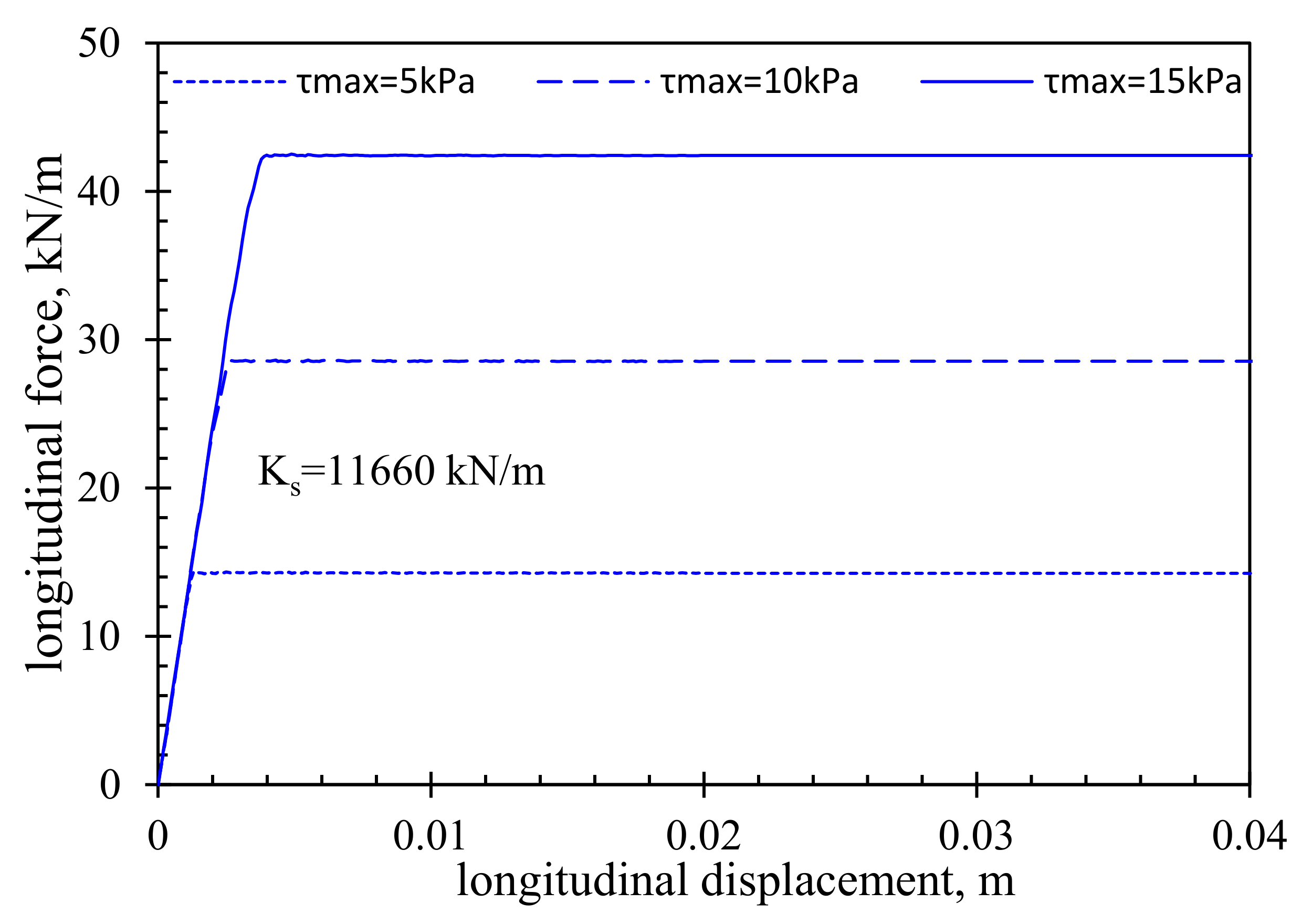
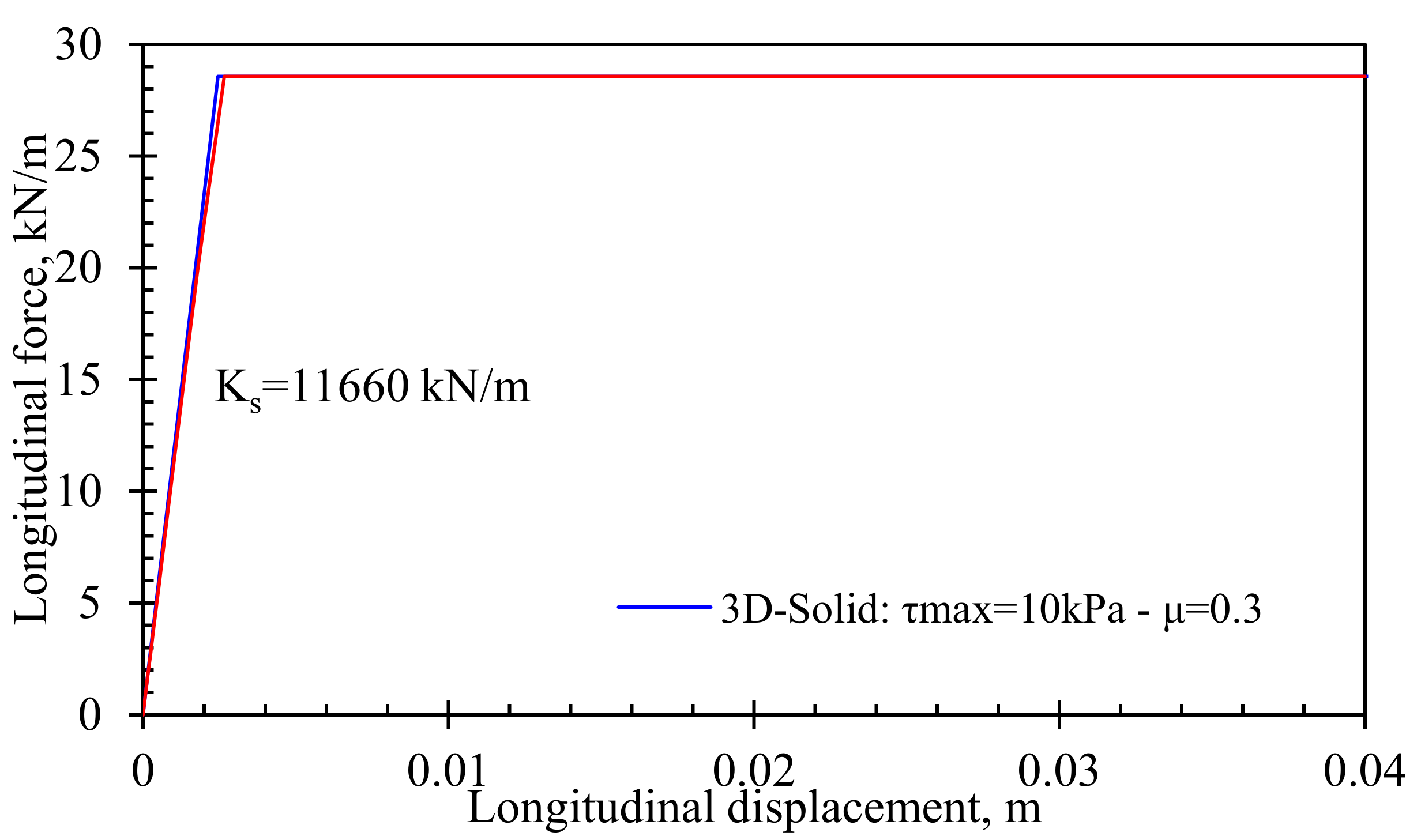
4.1. 3D FEM Analyses Results
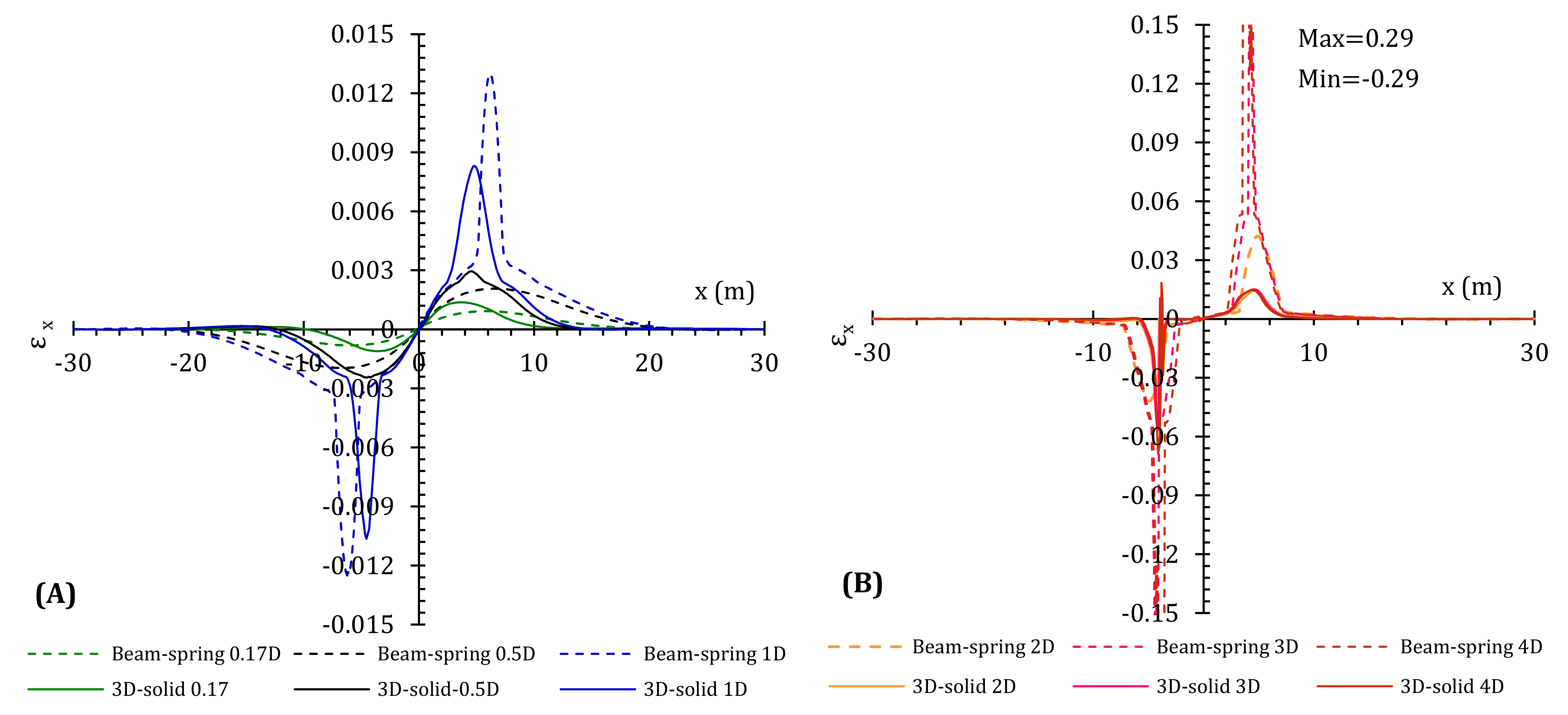
4.1.1. Longitudinal Pull-Out Test Analyses
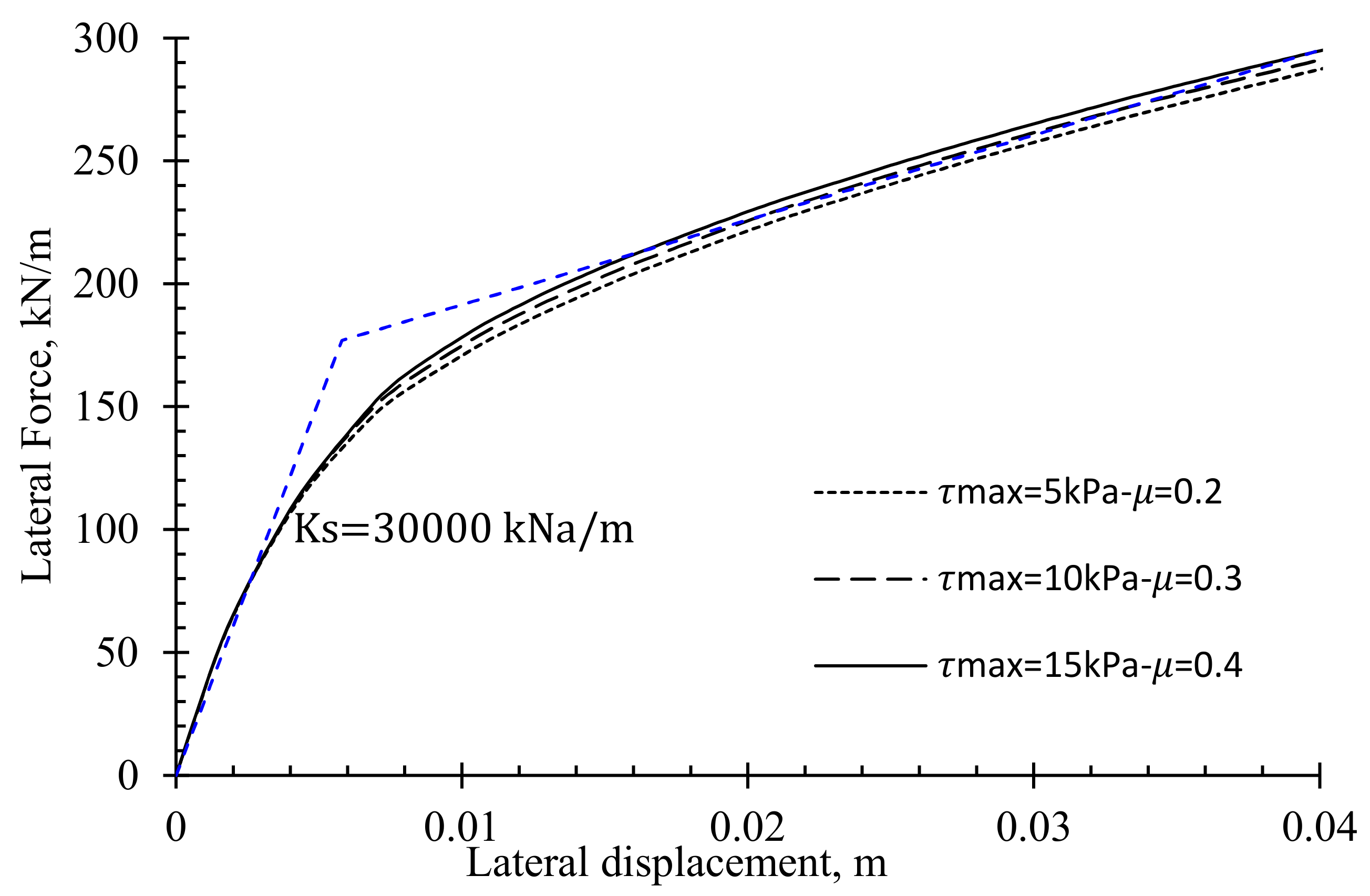
4.1.2. Lateral Sliding Test Analyses
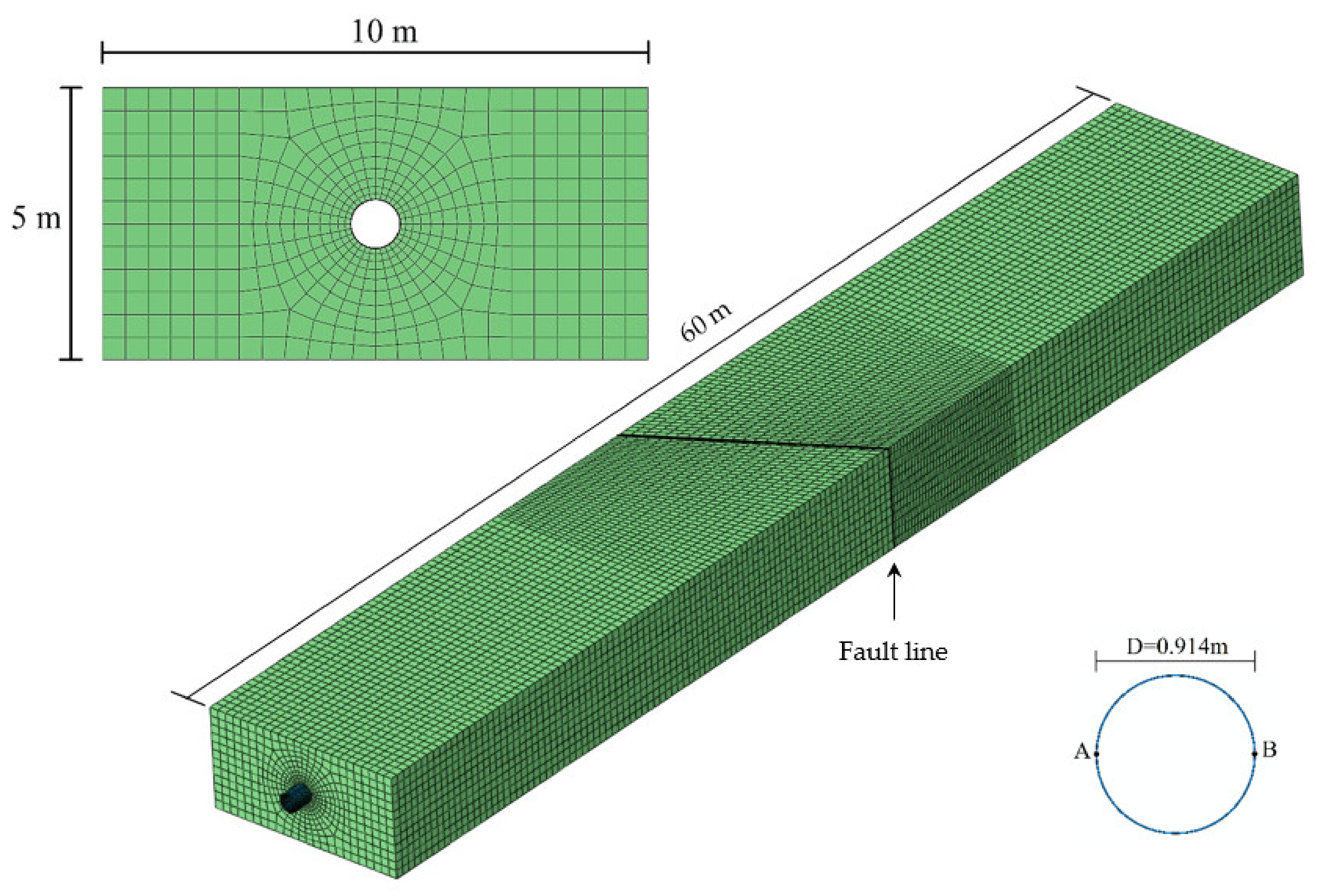
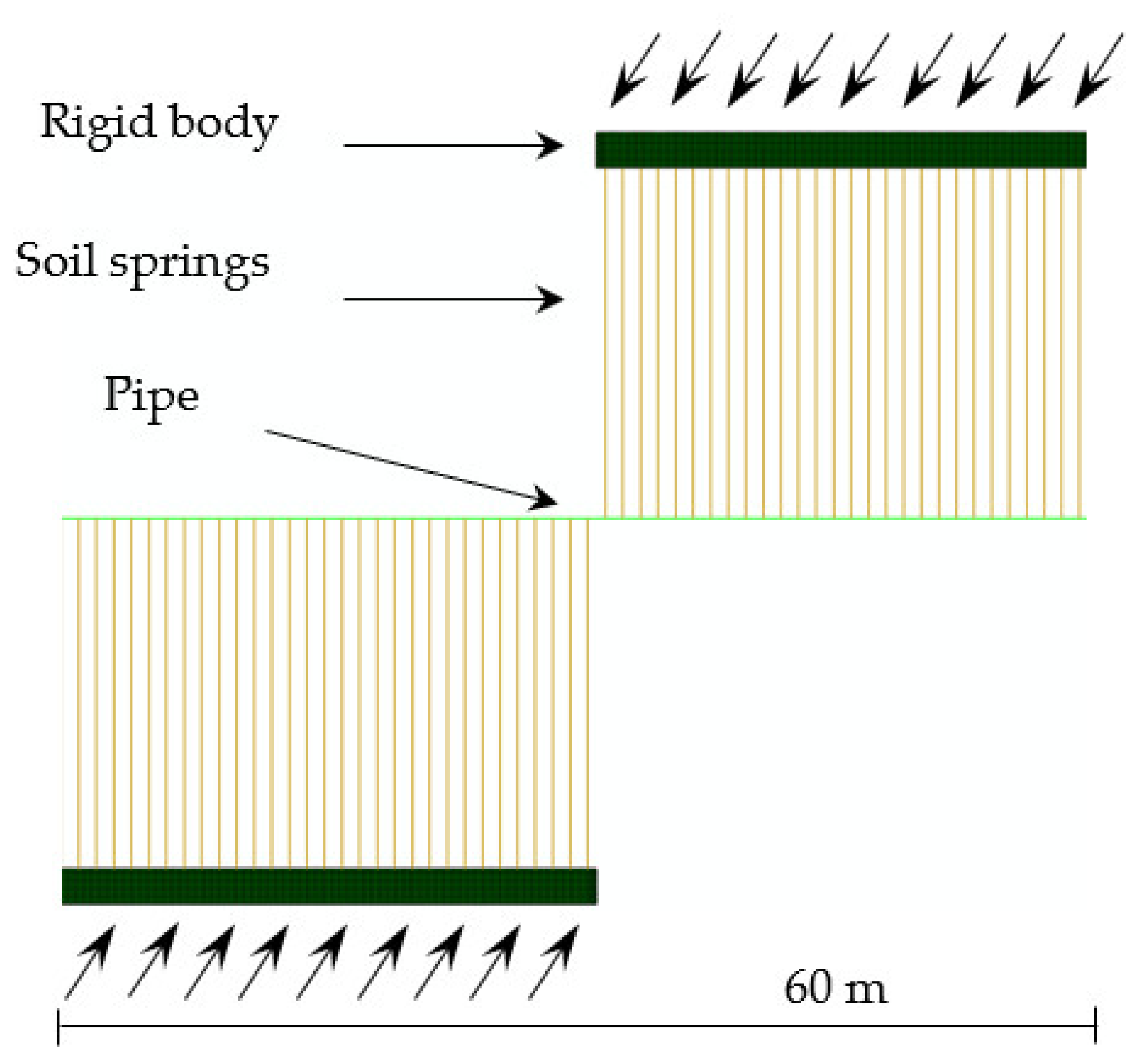
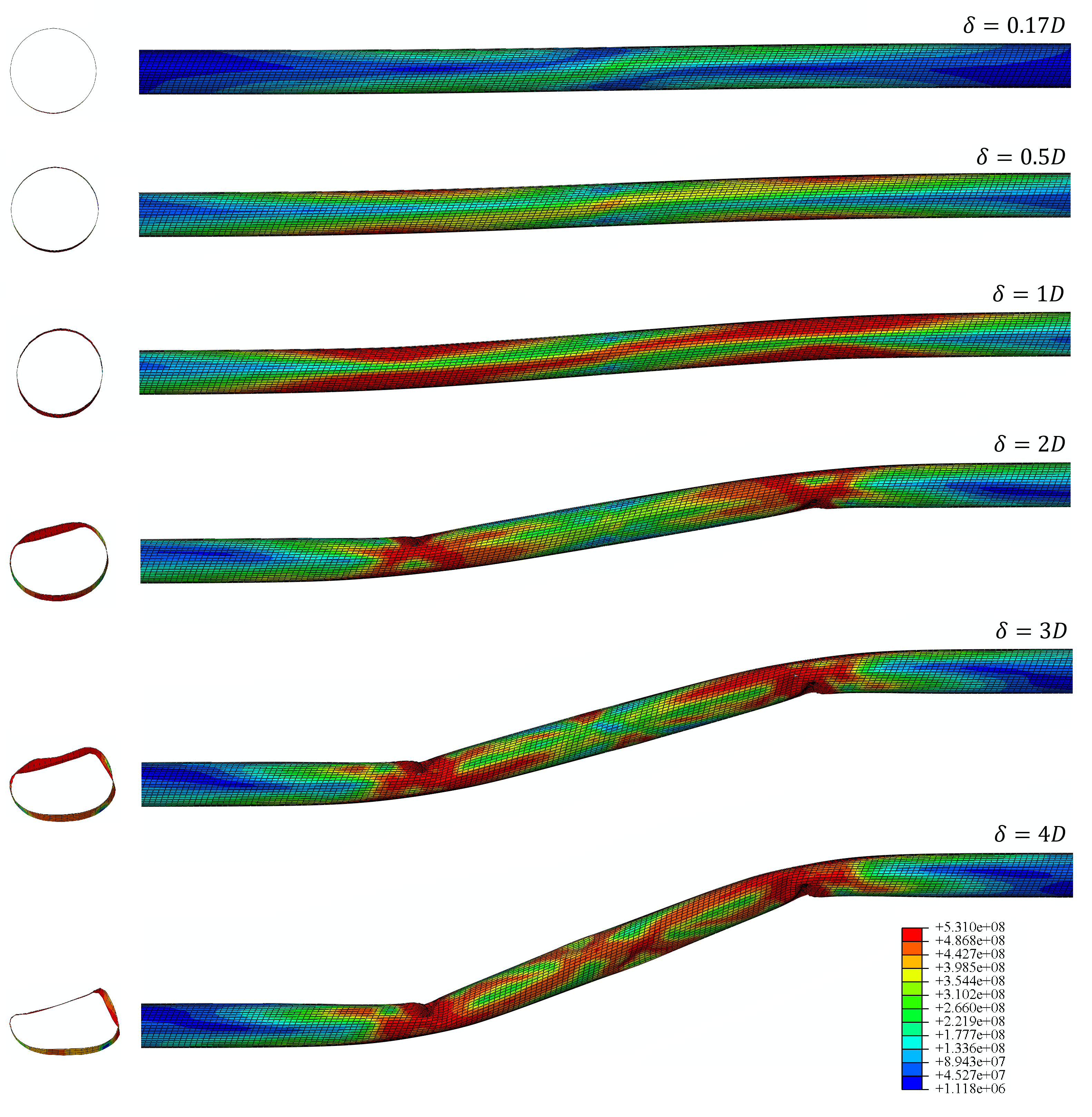
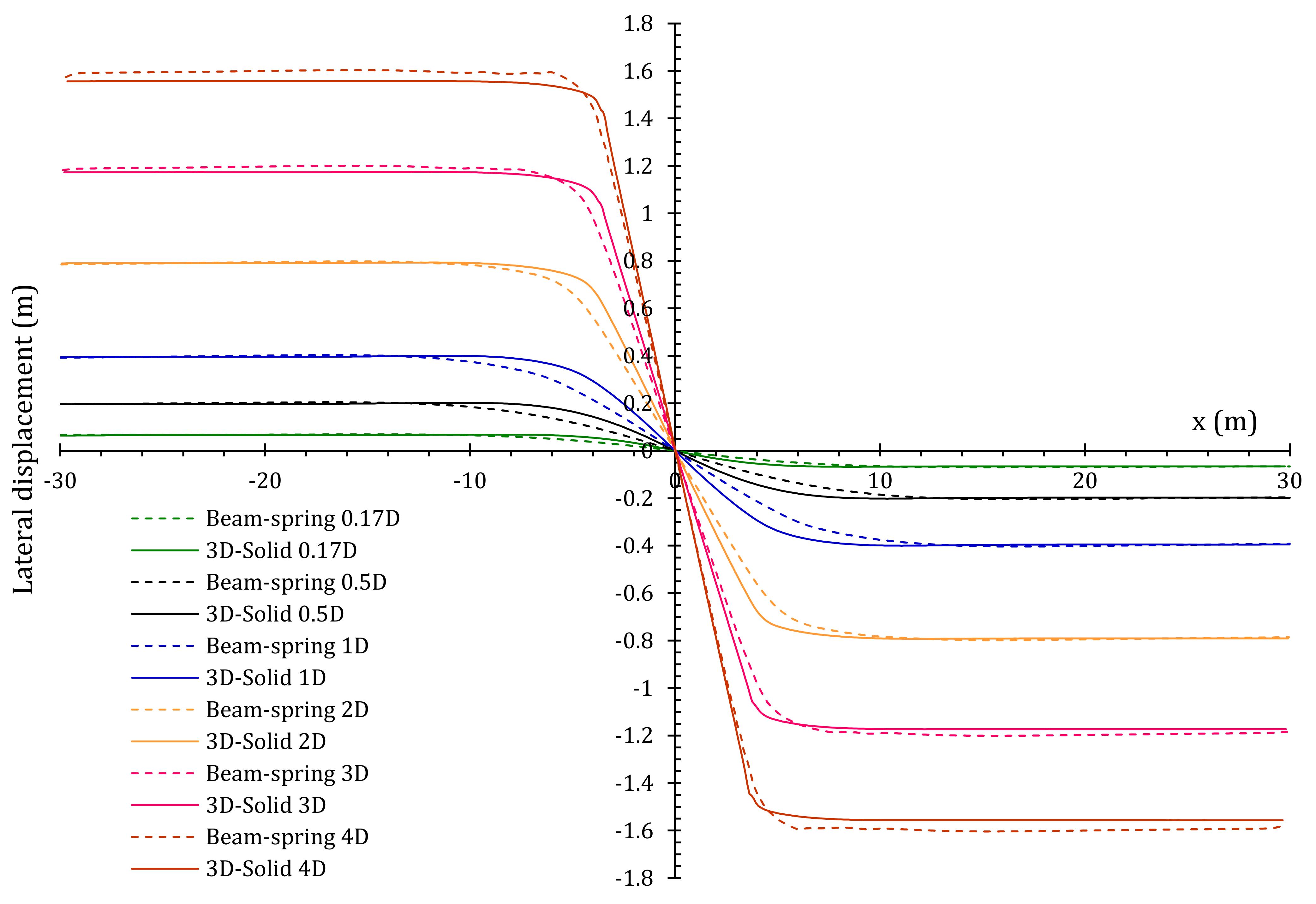
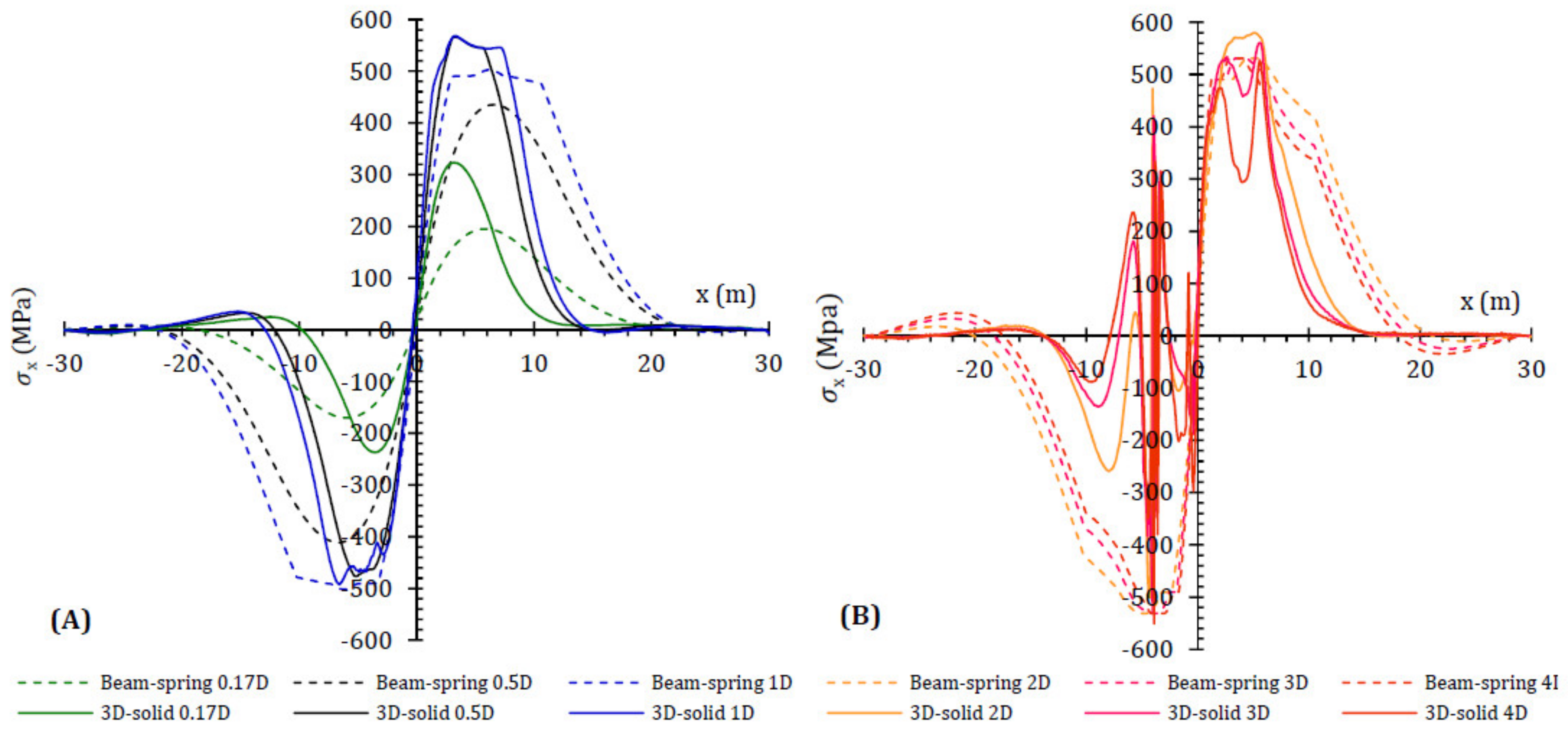
5. Modeling and Analysis Results of a Pipeline at a Strike-Slip Fault Crossing
5.1. FE Modeling
5.2. Analyses Results
6. Conclusions
- In the 3D solid model, because of the pressure and shear forces caused by the fault movement on the soil and pipeline around the high curvature zone, local confinement takes place, and soil stiffness surrounding the pipeline increases locally around the high curvature zone.
- Because of the locally stiffening of the soil and the local weakening of the inertia moment of the pipe at buckled zones in the 3D solid models, the high curvature zone length of the pipeline is shorter than in the beam–spring models.
- Due to the locally stiffening of the soil and the local weakening of the pipe cross-section moment of inertia in the 3D solid models, the soil–pipe interaction forces increase. Therefore, the buried pipeline in the 3D solid models before the occurrence of local buckling experiences higher stress and strain in comparison with the beam–spring models.
- In the 3D solid models, damage to the pipeline is visually detectable. Whereas, in the beam–spring models, observation of a local large strain on the pipeline is the best criterion for damage (e.g., buckling and ovalization) evaluation of the buried pipelines.
- In the 3D solid model, because of the local softening of the bending stiffness of the pipe cross-section, the pipe stress decreases at the buckled zone, which decreases the lateral deflection of the pipeline at further distances from the high curvature zone.
- Creating the 3D solid model is much more complex than the beam–spring model and it is easy to make mistakes in modeling for an amateur analyst. Moreover, modeling and analyzing take much time and cost. However, it can reproduce detailed and accurate results and cover all phenomena.
- Beam–spring models cannot include the effects of soil confinement at the high curvature zone and the local buckling of the buried pipe in the analysis, which are the main weaknesses of this modeling approach.
- Existing buried pipeline design guidelines have not included the effect of the local stiffening of the soil and the local softening of the pipe’s moment of inertia in the analysis recommendation. Therefore, to prevent the underestimation of the forces on the pipeline in the beam–spring modeling approach, it is recommended to assign stiffer nonlinear soil–pipe interaction spring properties to the high curvature length of the pipeline than the rest of the pipeline.
Author Contributions
Funding
Conflicts of Interest
References
- O’Rourke, M.J.; Liu, X. Response of Buried Pipelines Subject to Earthquake Effects; Monograph Series; Multidisciplinary Center for Earthquake Engineering Research (MCEER), USA: Buffalo, NY, USA, 1999. [Google Scholar]
- Aghabeigi, P.; Farahmand-Tabar, S. Seismic vulnerability assessment and retrofitting of historic masonry building of Malek Timche in Tabriz Grand Bazaar. Eng. Struct. 2021, 240, 112418. [Google Scholar] [CrossRef]
- Ariman, T.; Muleski, G.E. A review of the response of buried pipelines under seismic excitations. Earthq. Eng. Struct. Dyn. 1981, 9, 133–151. [Google Scholar] [CrossRef]
- Liang, J.; Sun, S. Site effects on seismic behavior of pipelines: A review. ASME J. Press. Vessel Technol. 2000, 122, 469–475. [Google Scholar] [CrossRef]
- O’Rourke, T.D.; Lane, P.A. Liquefaction Hazards and Their Effects on Buried Pipelines; Technical Report NCEER-89-0007; National Center for Earthquake Engineering Research: Buffalo, NY, USA, 1989. [Google Scholar]
- O’Rourke, T.D.; Palmer, M.C. Earthquake performance of gas transmission pipelines. Earthq. Spectra 1996, 20, 493–527. [Google Scholar] [CrossRef]
- Earthquake Engineering Research Institute. Kocaeli, Turkey Earthquake of August 17; EERI Special Earthquake Report; EERI: Oakland, CA, Canada, 17 August 1999. [Google Scholar]
- Uzarski, J.; Arnold, C. Chi-Chi, Taiwan, Earthquake of September 21, 1999 Reconnaissance Report; Earthquake Spectra 17 (Suppl. A); MCEER: Buffalo, NY, USA, 21 September 2001. [Google Scholar]
- Jennings, P.C. Engineering Features of the San Fernando Earthquake February 9, 1971; California Institute of Technology Report, CaltechEERL, California, 71–02; Caltech: Pasadena, CA, USA, 1971. [Google Scholar]
- MaCaffrey, M.A.; O’Rourke, T.D. Buried pipeline response to reverse faulting during the 1971 San Fernando Earthquake. ASME PVP 1983, 77, 151–159. [Google Scholar]
- Desmond, T.P.; Power, M.S.; Taylor, C.L.; Lau, R.W. Behavior of Large-Diameter Pipeline at Fault Crossings; ASCE: New York, NY, USA, 1995; pp. 296–303. [Google Scholar]
- Nakata, T.; Hasuda, K. Active fault I 1995 Hyogoken Nanbu earthquake. Kagaku 1995, 65, 127–142. [Google Scholar]
- Takada, S.; Nakayama, M.; Ueno, J.; Tajima, C. Report on Taiwan Earthquake; RCUSS, Earthquake Laboratory of Kobe University: Kobe, Japan, 1999; pp. 2–9. [Google Scholar]
- Kazama, M.; Noda, T. Damage statistics (Summary of the 2011 off the Pacific Coast of Tohoku Earthquake damage). J. Soils Found. 2012, 52, 780–792. [Google Scholar] [CrossRef] [Green Version]
- Wham, B.P.; Dashti, S.; Franke, K.; Kayen, R.; Oettle, N.K. Water supply damage caused by the 2016 Kumamoto Earthquake. J. Lowl. Technol. Int. 2017, 19, 165–174. [Google Scholar]
- Miyajima, M.; Fallahi, A.; Ikemoto, T.; Samaei, M.; Karimzadeh, S.; Setiawan, H.; Talebi, F.; Karashi, J. Site Investigation of the Sarpole-Zahab Earthquake, Mw 7.3 in SW Iran of November 12, 2017. JSCE J. Disaster FactSheets 2018. [Google Scholar]
- Bagriacik, A.; Davidson, R.A.; Hughes, M.W.; Bradley, B.A.; Cubrinovskic, M. Comparison of statistical and machine learning approaches to modeling earthquake damage to water pipelines. Soil Dyn. Earthq. Eng. 2018, 112, 76–88. [Google Scholar] [CrossRef]
- Manolis, G.D.; Beskos, D.E. Underground and Lifeline Structures. In Computer Analysis and Design of Earthquake Resistant Structures: A Handbook; Beskos, D.E., Anagnostopoulos, S.A., Eds.; CMP: Southampton, UK, 1997; pp. 775–837. [Google Scholar]
- Nourzadeh, D.; Mortazavi, P.; Ghalandarzadeh, A.; Takada, S.; Ahmadi, M. Performance assessment of the Greater Tehran Area buried gas distribution pipeline network under liquefaction. Soil Dyn. Earthq. Eng. 2019, 124, 16–34. [Google Scholar] [CrossRef]
- O’Rourke, M.J.; Gadicherla, V.; Abdoun, T. Centrifuge modeling of PGD response of buried pipe. Earthq. Eng. Eng. Vib. 2005, 4, 69–73. [Google Scholar] [CrossRef]
- Palmer, M.C.; O’Rourke, T.D.; Stewart, H.E.; O’Rourke, M.J.; Symans, M. Large displacement soil-structure interaction test facility for lifelines. In Proceedings of the 8th US National Conference Commemorating the 1906 San Francisco Earthquake, EERI, San Francisco, CA, USA, 18–22 April 2006. [Google Scholar]
- O’Rourke, T.D.; Bonneau, A. Lifeline performance under extreme loading during earthquakes. In Earthquake Geotechnical Engineering; Pitilakis, K.D., Ed.; Springer: Dordrecht, The Netherlands, 2007; pp. 407–432. [Google Scholar]
- Lin, T.J.; Liu, G.Y.; Chung, L.L.; Chou, C.H.; Huang, C.W. Verification of Numerical Modeling in Buried Pipelines under Large Fault Movements by Small-Scale Experiments. In Proceedings of the 15WCEE, Lisbon, Portugal, 24–28 September 2012; Volume 9, pp. 6685–6693. [Google Scholar]
- Xie, X.; Symans, M.D.; O’Rourke, M.J.; Abdoun, T.H.; O’Rourke, T.D.; Palmer, M.C.; Stewart, H.E. Numerical 47odelling of buried HDPE pipelines subjected to strike-slip faulting. J. Earthq. Eng. 2011, 15, 1273–1296. [Google Scholar] [CrossRef]
- Ha, D.; Abdoun, T.H.; O’Rourke, M.J.; Symans, M.D.; O’Rourke, T.D.; Palmer, M.C.; Stewart, H.E. Buried high-density polyethylene pipelines subjected to normal and strike–slip faulting—A centrifuge investigation. Can. Geotech. J. 2008, 45, 1733–1742. [Google Scholar] [CrossRef]
- Ha, D.; Abdoun, T.H.; O’Rourke, M.J.; Symans, M.D.; O’Rourke, T.D.; Palmer, M.C.; Stewart, H.E. Centrifuge modelling of earthquake effects on buried high-density polyethylene (HDPE) pipelines crossing high curvature zones. ASCE J. Geotech. Geoenviron. Eng. 2008, 134, 1501–1515. [Google Scholar] [CrossRef]
- Abdoun, T.H.; Ha, D.; O’Rourke, M.J.; Symans, M.D.; O’Rourke, T.D.; Palmer, M.C.; Stewart, H.E. Factors influencing the behavior of buried pipelines subjected to earthquake faulting. Soil Dyn. Earthq. Eng. 2009, 29, 415–427. [Google Scholar] [CrossRef]
- Newmark, N.M.; Hall, W.J. Pipeline design to resist large fault displacement. In Proceedings of the U.S. National Conference on Earthquake Engineering, University of Michigan, Ann Arbor, MI, USA, 18–20 June 1975; pp. 416–425. [Google Scholar]
- Kennedy, R.P.; Chow, A.M.; Williamson, R.A. Fault movement effects on buried oil pipeline. ASCE Transp. Eng. J. 1977, 103, 617–633. [Google Scholar] [CrossRef]
- Kennedy, R.P.; Kincaid, R.H. Fault crossing design for buried gas oil pipelines. ASME PVP 1983, 77, 1–9. [Google Scholar]
- Wang, L.R.L.; Yeh, Y.A. A refined seismic analysis and design of buried pipeline for fault movement. Earthq. Eng. Struct. Dyn. 1985, 13, 75–96. [Google Scholar] [CrossRef]
- Karamitros, D.; Bouckovalas, G.; Kouretzis, G. Stress analysis of buried steel pipelines at strike-slip fault crossings. Soil Dyn. Earthq. Eng. 2007, 27, 200–211. [Google Scholar] [CrossRef]
- Trifonov, O.V.; Cherniy, V.P. A semi-analytical approach to a nonlinear stress–strain analysis of buried steel pipelines crossing active faults. Soil Dyn. Earthq. Eng. 2010, 30, 1298–1308. [Google Scholar] [CrossRef]
- Karamitros, D.K.; Bouckovalas, G.D.; Kouretzis, G.P.; Gkesouli, V. An analytical method for strength verification of buried steel pipelines at normal fault crossings. Soil Dyn. Earthq. Eng. 2011, 31, 1452–1464. [Google Scholar] [CrossRef]
- Trifonov, O.V.; Cherniy, V.P. Elastoplastic stress-strain analysis of buried steel pipelines subjected to fault displacements with account for service loads. Soil Dyn. Earthq. Eng. 2012, 33, 54–62. [Google Scholar] [CrossRef]
- Talebi, F.; Kiyono, J. Introduction of the axial force terms to governing equation for buried pipeline subjected to strike-slip fault movements. Soil Dyn. Earthq. Eng. 2020, 133, 106125. [Google Scholar] [CrossRef]
- Talebi, F.; Kiyono, J. A refined nonlinear analytical method for buried pipelines crossing strike-slip faults. Earthq. Eng. Struct. Dyn. 2021, 1–24. [Google Scholar] [CrossRef]
- Talebi, F.; Kiyono, J. Evaluation of modeling approaches for buried pipelines subjected to strike–slip fault movements. In Proceedings of the 17th World Conference of Earthquake Engineering (17WCEE), Sendai, Japan, 13–18 September 2020. [Google Scholar]
- Vazouras, P.; Dakoulas, P.; Karamanos, S.A. Pipe–soil interaction and pipeline performance under strike–slip fault movements. Soil Dyn. Earthq. Eng. 2015, 72, 48–65. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, H.; Li, M.; Xia, M.; Zheng, W.; Wu, K.; Han, Y. Effects of steel properties on the local buckling response of high strength pipelines subjected to reverse faulting. J. Nat. Gas Sci. Eng. 2016, 33, 378–387. [Google Scholar] [CrossRef]
- Demirci, H.E.; Bhattacharya, S.; Karamitros, D.; Alexander, N. Experimental and numerical modelling of buried pipelines crossing reverse faults. Soil Dyn. Earthq. Eng. 2018, 114, 198–214. [Google Scholar] [CrossRef] [Green Version]
- Talebi, F.; Kiyono, J. Seismic response of buried pipelines to strong ground motion of strike-slip fault. In Landslides 5 Book, Understanding and Reducing Landslide Disaster Risk; Springer: Cham, Switzerland, 2020; pp. 55–60. Available online: https://link.springer.com/chapter/10.1007/978-3-030-60713-5_6.
- ABAQUS/CAE 2017. Dassault Systems Simulia Corp: Mayfield Heights, OH, USA, documentation of 2017 release.




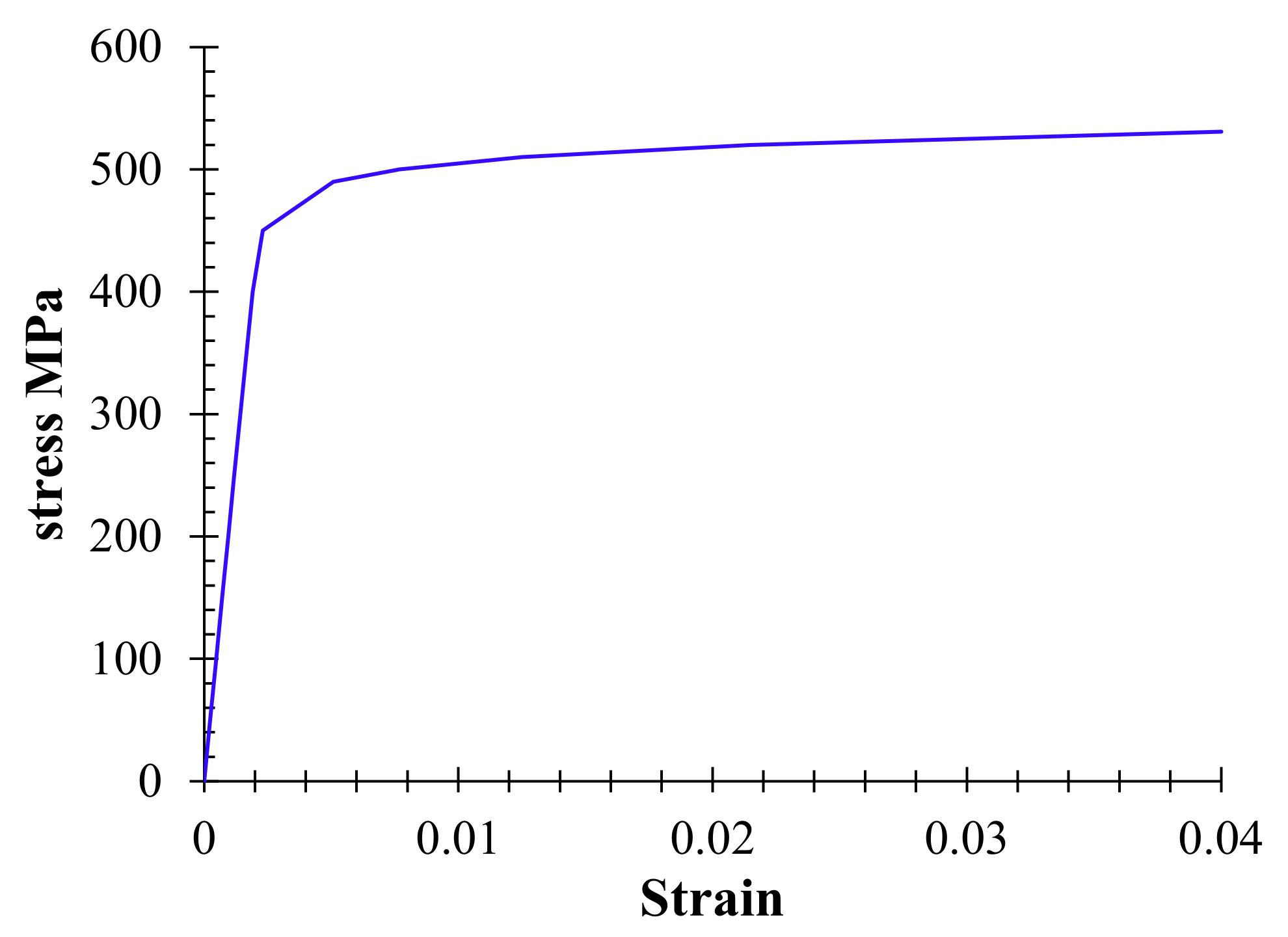
| Parameter | X65 Steel | Soil |
|---|---|---|
| Young’s Modulus (E) | 21 Tpa | 25 MPa |
| Poisson ratio (ν) | 0.3 | 0.5 |
| Density (ρ) | 7850 kg/m3 | 2000 kg/m3 |
| Cohesion (c) | - | 50 kPa |
| Friction Angle (Φ) | - | 0° |
| Yielding Stress ( | 490 MPa | - |
| Yielding Strain ( | 0.233% | - |
| Failure Stress ( | 531 MPa | - |
| Failure Strain ( | 4% | - |
| Initial Young’s modulus () | 210 GPa |
| Yielding stress | 490 MPa |
| a | 38.31 |
| r | 31.51 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Talebi, F.; Kiyono, J. Comparison of 3D Solid and Beam–Spring FE Modeling Approaches in the Evaluation of Buried Pipeline Behavior at a Strike-Slip Fault Crossing. Energies 2021, 14, 4539. https://doi.org/10.3390/en14154539
Talebi F, Kiyono J. Comparison of 3D Solid and Beam–Spring FE Modeling Approaches in the Evaluation of Buried Pipeline Behavior at a Strike-Slip Fault Crossing. Energies. 2021; 14(15):4539. https://doi.org/10.3390/en14154539
Chicago/Turabian StyleTalebi, Farzad, and Junji Kiyono. 2021. "Comparison of 3D Solid and Beam–Spring FE Modeling Approaches in the Evaluation of Buried Pipeline Behavior at a Strike-Slip Fault Crossing" Energies 14, no. 15: 4539. https://doi.org/10.3390/en14154539
APA StyleTalebi, F., & Kiyono, J. (2021). Comparison of 3D Solid and Beam–Spring FE Modeling Approaches in the Evaluation of Buried Pipeline Behavior at a Strike-Slip Fault Crossing. Energies, 14(15), 4539. https://doi.org/10.3390/en14154539





