Optimization of Modernization of a Single-Family Building in Poland Including Thermal Comfort
Abstract
1. Introduction
2. Materials and Methods
2.1. Building Model
2.2. Heating System Model
2.3. Ventilation System Model
2.4. Heat Gain Model
2.5. Cooling System Model
2.6. Thermal Comfort Model
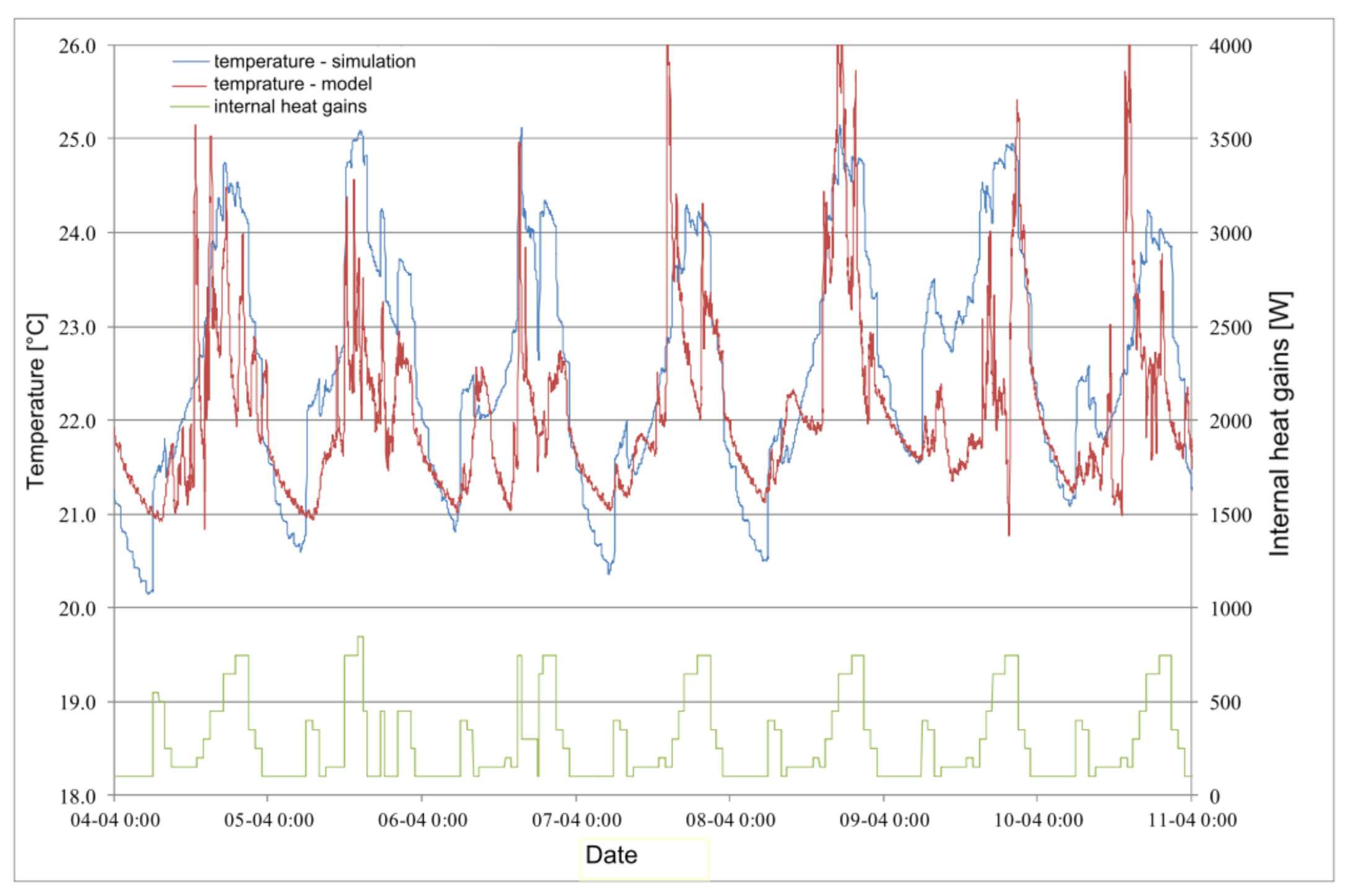
2.7. Model Verification
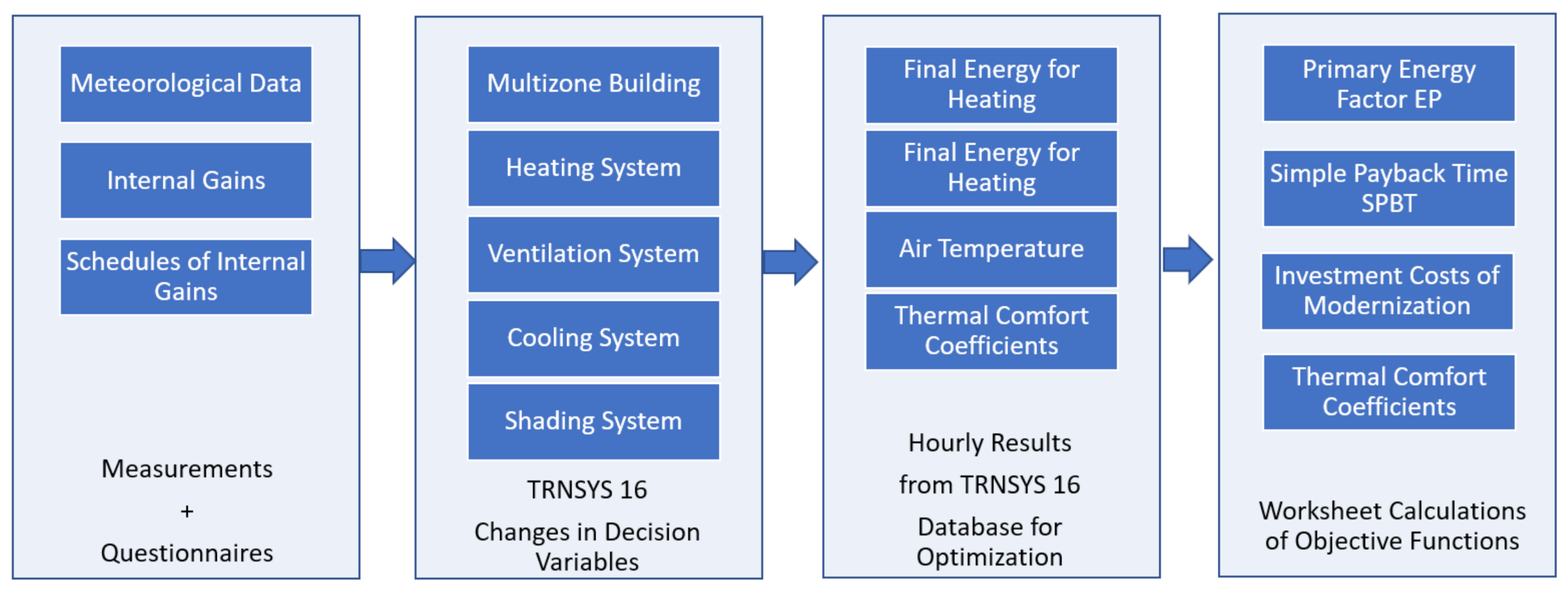
2.8. Research Procedure
3. Optimization
3.1. Decision Variables
- Building orientation;
- Insulation thickness of external walls and roof;
- Area of the windows in the garden façade of the building in zone 2;
- Type of windows (value of window heat transfer coefficient Uw and the total solar energy transmission coefficient gg);
- Type of ventilation system, cooling system, and shading system type;
- Night cooling.
- -
- S—Blinds on the windows limiting the heat gain from the sun; the blind is lowered if the internal temperature in the zone exceeds 22 °C, the shade coefficient of the blind was set to 0.5.
- -
- VN—Natural ventilation system, the air is removed by convection from the kitchen and toilets, the inflow of fresh air occurs by infiltration; the air change rate was determined during the experimental verification of the model and is 0.5 L/h for winter period, 0.72 for mid-period, and 1.06 for summer period.
- -
- VM—Mechanical ventilation system with heat recovery (thermal efficiency 60%) and constant ventilation air flows in zones (according to Table 11).
- -
- C—Cooling with individual air conditioners (with natural ventilation) or central cooling system (with mechanical ventilation); individual air conditioners are turned on if the temperature in the zone exceeds 24 °C, central cooling is turned on if the temperature in the living room exceeds 24 °C.
- -
- Wall type (thermal insulation of the building envelope) decision variable form 1 ÷ 12 according to Table 8; existing stage variant number 9.
- -
- System type (ventilation/cooling/shading type) decision variable values form 1 ÷ 8; existing stage variant number 4:
- 1
- (VN + S)—natural ventilation/no cooling system/external shading devices,
- 2
- (VM + S)—mechanical ventilation/no cooling system/external shading devices,
- 3
- (VM + C + S)—mechanical ventilation/central cooling system/external shading devices,
- 4
- (VN)—natural ventilation/no cooling system/without external shading devices,
- 5
- (VM)—mechanical ventilation/no cooling system/without external shading devices,
- 6
- (VM + C)—mechanical ventilation/central cooling system/without external shading devices,
- 7
- (VN + C)—natural ventilation/individual air conditioners/without external shading devices,
- 8
- (VN + C + S)—natural ventilation/individual air conditioners/external shading devices.
- -
- Window type values from 1 to 4 according to Table 10, existing stage variant number 4 (W_10):
- 1
- (W_2)—type of windows 2,
- 2
- (W_4)—type of windows 4,
- 3
- (W_7)—type of windows 7,
- 4
- (W_10)—type of windows 10.
3.2. Description of the Objective Function
- -
- Primary energy consumption factor EP for heating and cooling;
- -
- Simple payback time SPBT;
- -
- Investment costs of modernization KRIavg;
- -
- Thermal comfort coefficients PPDavg and DTavg.
3.3. Energy and Modernization Costs
4. Results
- -
- EP < 134.5 kWh/(m2a);
- -
- PPDavg < 26%;
- -
- SPBT < 1.1*60 years.
5. Discussion and Future Work
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- 2018/844. Directive (EU) 2018/844 of the European Parliament and of the Council of 30 May 2018 Amending Directive 2010/31/EU on the Energy Performance of Buildings and Directive 2012/27/EU on Energy Efficiency. In Official Journal of the European Union; EU: Brussels, Belgium, 2018; OJ L 156, 19.6.2018; pp. 75–91. [CrossRef]
- 2010/31/EU. Directive 2010/31/EU of the European Parliament and of the Council of 19 May 2010 on the Energy Performance of Buildings (Recast). In Official Journal of the European Union; EU: Brussels, Belgium, 2010; OJ L 153, 18.6.2010; pp. 13–35.
- Nowak-Dzieszko, K.; Rojewska-Warchał, M. Influence of the Balcony Glazing Construction on Thermal Comfort of Apartments in Retrofitted Large Panel Buildings. Procedia Eng. 2015, 108, 481–487. [Google Scholar] [CrossRef]
- Fanger, P.O. Thermal Comfort, Analysis and Applications in Environmental Engineering; McGraw-Hill: New York, NY, USA, 1970. [Google Scholar]
- ISO 7730 Ergonomics of the Thermal Environment—Analytical Determination and Interpretation of Thermal Comfort Using Calculation of the PMV and PPD Indices and Local Thermal Comfort Criteria. 2005. Available online: https://www.iso.org/standard/39155.html (accessed on 23 February 2021).
- EN 15251:2007 Indoor Environmental Input Parameters for Design and Assessment of Energy Performance of Buildings Addressing Indoor Air Quality, Thermal Environment, Lighting and Acoustics. 2007. Available online: http://www.cres.gr/greenbuilding/PDF/prend/set4/WI_31_Pre-FV_version_prEN_15251_Indoor_Environment.pdf (accessed on 23 February 2021).
- EN 16798-1:2019 Energy Performance of Buildings—Ventilation for Buildings—Part 1: Indoor Environmental Input Parameters for Design and Assessment of Energy Performance of Buildings Addressing Indoor air Quality, Thermal Environment, Lighting and Acous. 2019. Available online: https://standards.iteh.ai/catalog/standards/cen/b4f68755-2204-4796-854a-56643dfcfe89/en-16798-1-2019 (accessed on 23 February 2021).
- Cabanac, M. Physiological Role of Pleasure. Science 1971, 173, 1103–1107. [Google Scholar] [CrossRef] [PubMed]
- De Dear, R. Revisiting an old hypothesis of human thermal perception: Alliesthesia. Build. Res. Inf. 2011, 39, 108–117. [Google Scholar] [CrossRef]
- Xu, C.; Li, S.; Zhang, X.; Shao, S. Thermal comfort and thermal adaptive behaviours in traditional dwellings: A case study in Nanjing, China. Build. Environ. 2018, 142, 153–170. [Google Scholar] [CrossRef]
- Zhang, H.; Arens, E.; Pasut, W. Air temperature thresholds for indoor comfort and perceived air quality. Build. Res. Inf. 2011, 39, 134–144. [Google Scholar] [CrossRef]
- Zhai, Y.; Zhang, H.; Zhang, Y.; Pasut, W.; Arens, E.; Meng, Q. Comfort under personally controlled air movement in warm and humid environments. Build. Environ. 2013, 65, 109–117. [Google Scholar] [CrossRef]
- Huang, L.; Ouyang, Q.; Zhu, Y.; Jiang, L. A study about the demand for air movement in warm environment. Build. Environ. 2013, 61, 27–33. [Google Scholar] [CrossRef]
- Sun, W.; Cheong, K.; Melikov, A.K. Subjective study of thermal acceptability of novel enhanced displacement ventilation system and implication of occupants’ personal control. Build. Environ. 2012, 57, 49–57. [Google Scholar] [CrossRef]
- García, A.; Olivieri, F.; Larrumbide, E.; Ávila, P. Thermal comfort assessment in naturally ventilated offices located in a cold tropical climate, Bogotá. Build. Environ. 2019, 158, 237–247. [Google Scholar] [CrossRef]
- Lomas, K.J.; Kane, T. Summertime temperatures and thermal comfort in UK homes. Build. Res. Inf. 2013, 41, 259–280. [Google Scholar] [CrossRef]
- Petrova, S.; Gentile, M.; Mäkinen, I.H.; Bouzarovski, S. Perceptions of Thermal Comfort and Housing Quality: Exploring the Microgeographies of Energy Poverty in Stakhanov, Ukraine. Environ. Plan. A Econ. Space 2013, 45, 1240–1257. [Google Scholar] [CrossRef]
- Engvall, K.; Lampa, E.; Levin, P.; Wickman, P.; Öfverholm, E. Interaction between building design, management, household and individual factors in relation to energy use for space heating in apartment buildings. Energy Build. 2014, 81, 457–465. [Google Scholar] [CrossRef]
- Huang, L.; Zhu, Y.; Ouyang, Q.; Cao, B. Field survey of indoor thermal comfort in rural housing of northern China in heating season. J. Southeast Univ. 2010, 26, 169–172. [Google Scholar]
- Nematchoua, M.K.; Tchinda, R.; Orosa, J.A. Adaptation and comparative study of thermal comfort in naturally ventilated classrooms and buildings in the wet tropical zones. Energy Build. 2014, 85, 321–328. [Google Scholar] [CrossRef]
- Moujalled, B.; Cantin, R.; Guarracino, G. Comparison of thermal comfort algorithms in naturally ventilated office buildings. Energy Build. 2008, 40, 2215–2223. [Google Scholar] [CrossRef]
- Wagner, A.; Gossauer, E.; Moosmann, C.; Gropp, T.; Leonhart, R. Thermal comfort and workplace occupant satisfaction—Results of field studies in German low energy office buildings. Energy Build. 2007, 39, 758–769. [Google Scholar] [CrossRef]
- Akair, A.; Bánhidi, L. Thermal comfort investigation in Libya. Period. Polytech. Mech. Eng. 2007, 51, 45. [Google Scholar] [CrossRef][Green Version]
- Hameln, M.; Zmeureanu, R. Multi-objective life cycle optimization of a single-family house envelope. In Proceedings of the Canadian Conference on Building Simulation, Halifax, Canada, 1–4 May 2012; pp. 202–214. [Google Scholar]
- Chardon, S.; Brangeon, B.; Bozonnet, E.; Inard, C. Construction cost and energy performance of single family houses: From integrated design to automated optimization. Autom. Constr. 2016, 70, 1–13. [Google Scholar] [CrossRef]
- Grygierek, K.; Ferdyn-Grygierek, J.; Gumińska, A.; Baran, Ł.; Barwa, M.; Czerw, K.; Gowik, P.; Makselan, K.; Potyka, K.; Psikuta, A. Energy and Environmental Analysis of Single-Family Houses Located in Poland. Energies 2020, 13, 2740. [Google Scholar] [CrossRef]
- Hurnik, M.; Specjal, A.; Popiolek, Z.; Kierat, W. Assessment of single-family house thermal renovation based on comprehensive on-site diagnostics. Energy Build. 2018, 158, 162–171. [Google Scholar] [CrossRef]
- Fedorczak-Cisak, M.; Kotowicz, A.; Radziszewska-Zielina, E.; Sroka, B.; Tatara, T.; Barnaś, K. Multi-Criteria Optimisation of an Experimental Complex of Single-Family Nearly Zero-Energy Buildings. Energies 2020, 13, 1541. [Google Scholar] [CrossRef]
- Grygierek, K.; Ferdyn-Grygierek, J. Multi-Objective Optimization of the Envelope of Building with Natural Ventilation. Energies 2018, 11, 1383. [Google Scholar] [CrossRef]
- Petrovic, B.; Myhren, J.A.; Zhang, X.; Wallhagen, M.; Eriksson, O. Life cycle assessment of a wooden single-family house in Sweden. Appl. Energy 2019, 251, 113253. [Google Scholar] [CrossRef]
- Dara, C.; Hachem-Vermette, C.; Assefa, G. Life cycle assessment and life cycle costing of container-based single-family housing in Canada: A case study. Build. Environ. 2019, 163, 106332. [Google Scholar] [CrossRef]
- Becchio, C.; Dabbene, P.; Fabrizio, E.; Monetti, V.; Filippi, M. Cost optimality assessment of a single family house: Building and technical systems solutions for the nZEB target. Energy Build. 2015, 90, 173–187. [Google Scholar] [CrossRef]
- Ekström, T.; Bernardo, R.; Blomsterberg, Å. Cost-effective passive house renovation packages for Swedish single-family houses from the 1960s and 1970s. Energy Build. 2018, 161, 89–102. [Google Scholar] [CrossRef]
- Colli, C.; Bataille, A.; Antczak, E.; Buyle-Bodin, F. Life Cycle Assessment of a French Single-Family House Refurbishment: The “Bat-Eco2” Case Study. Procedia CIRP 2018, 69, 160–165. [Google Scholar] [CrossRef]
- Azizi, S.; Nair, G.; Olofsson, T. Adoption of Energy Efficiency Measures in Renovation of Single-Family Houses: A Comparative Approach. Energies 2020, 13, 6042. [Google Scholar] [CrossRef]
- Roberti, F.; Oberegger, U.F.; Lucchi, E.; Gasparella, A. Energy retrofit and conservation of built heritage using multi-objective optimization: Demonstration on a medieval building. Build. Simul. Appl. 2015, 2015, 189–197. [Google Scholar]
- Lucchi, E.; Tabak, M.; Troi, A. The “Cost Optimality” Approach for the Internal Insulation of Historic Buildings. Energy Procedia 2017, 133, 412–423. [Google Scholar] [CrossRef]
- Bjørneboe, M.G.; Svendsen, S.; Heller, A. Evaluation of the renovation of a Danish single-family house based on measurements. Energy Build. 2017, 150, 189–199. [Google Scholar] [CrossRef]
- Bjørneboe, M.G.; Svendsen, S.; Heller, A. Initiatives for the energy renovation of single-family houses in Denmark evaluated on the basis of barriers and motivators. Energy Build. 2018, 167, 347–358. [Google Scholar] [CrossRef]
- Dzikuć, M.; Piwowar, A.; Szufa, S.; Adamczyk, J.; Dzikuć, M. Potential and Scenarios of Variants of Thermo-Modernization of Single-Family Houses: An Example of the Lubuskie Voivodeship. Energies 2021, 14, 191. [Google Scholar] [CrossRef]
- Blazy, R.; Błachut, J.; Ciepiela, A.; Łabuz, R.; Papież, R. Thermal Modernization Cost and the Potential Ecological Effect—Scenario Analysis for Thermal Modernization in Southern Poland. Energies 2021, 14, 2033. [Google Scholar] [CrossRef]
- TRNSYS 16 Manual. 2018. Available online: http://web.mit.edu/parmstr/Public/Documentation/05-MathematicalReference.pdf (accessed on 10 October 2020).
- Rucińska, J. Optimization of Annual Energy Costs in a Single-Family House Including Thermal Comfort. Ph.D. Thesis, Warsaw University of Technology, Faculty of Environmental Engineering, Warszawa, Poland, 2013. [Google Scholar]
- PN-EN ISO 15927-4:2007 Hygrothermal Performance of Buildings—Calculation and Presentation of Climatic Data—Part 4: Hourly Data for Assessing the Annual Energy Use for Heating and Cooling. Polish Committee for Standardization 2007. Available online: https://www.iso.org/standard/41371.html (accessed on 23 February 2021).
- Tess Libraries. 2014. Available online: http://www.trnsys.com/tess-libraries/ (accessed on 10 October 2020).
- Skou, M.B.; Kragh, J. Proposal for an Energy Rating System of Windows in EU. 2009. Available online: https://www.buildup.eu/en/practices/publications/proposal-energy-rating-system-windows-eu (accessed on 10 October 2020).
- Rucińska, J.; Panek, A.; Trząski, A. Energy Certification of Windows in Poland. In Proceedings of the International World Sustainable Building Conferencei, Helsinki, Finlandia, 18–21 October 2011. [Google Scholar]
- 2002/91/EC. Directive 2002/91/EC of the European Parliament and of the Council 6 December 2002 on the Energy Performance of Buildings. In Official Journal of the European Union; EU: Brussels, Belgium, 2003; OJ L 1, 4.1.2003; pp. 65–71.
- Fabbri, M.; de Groote, M.; Rapf, O. Building Renovation Passports: Customised Roadmaps towards Deep Renovation and Better Homes; Buildings Performance Institute Europe: Brussels, Belgium, 2016; p. 46. [Google Scholar]
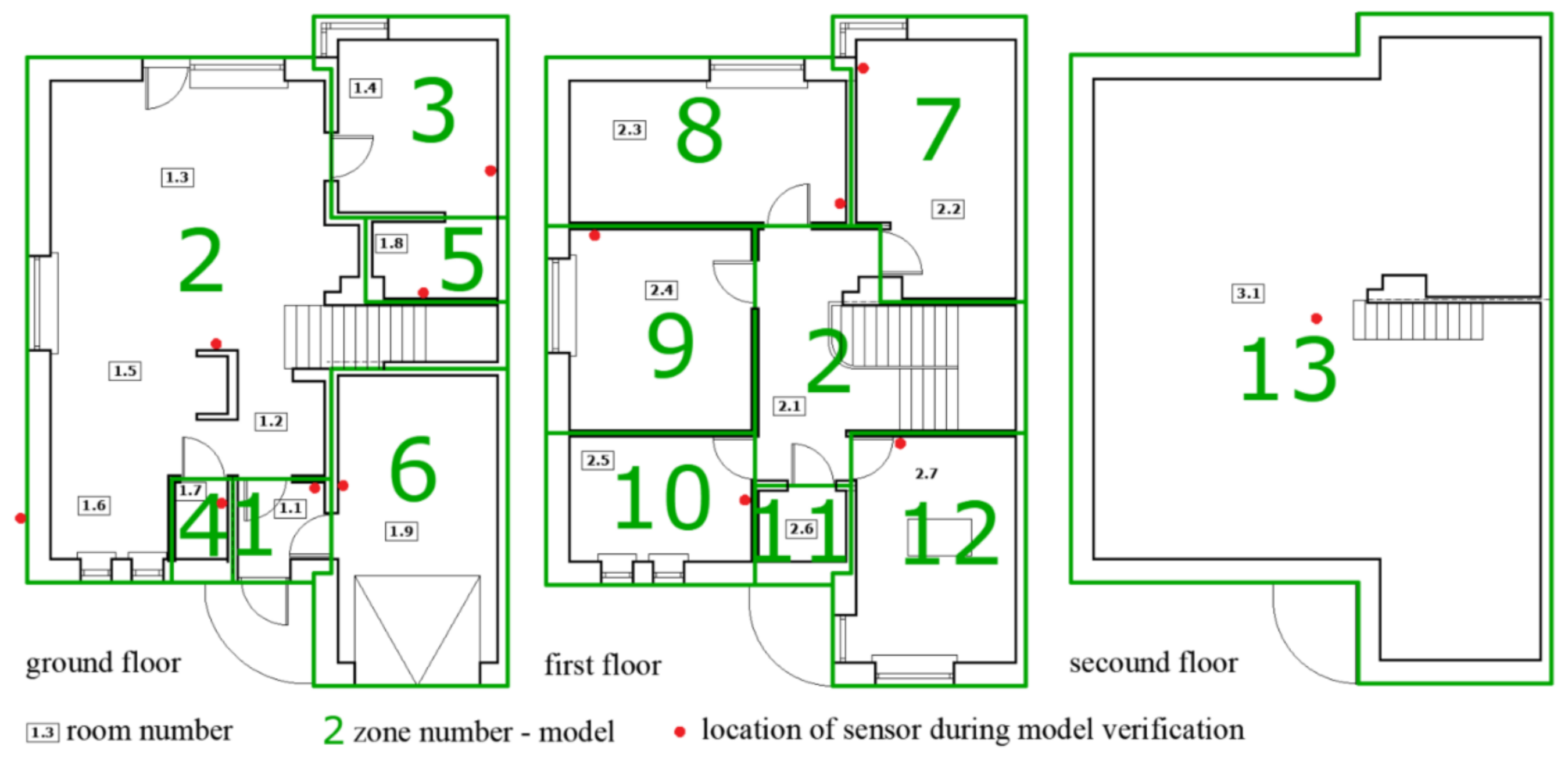

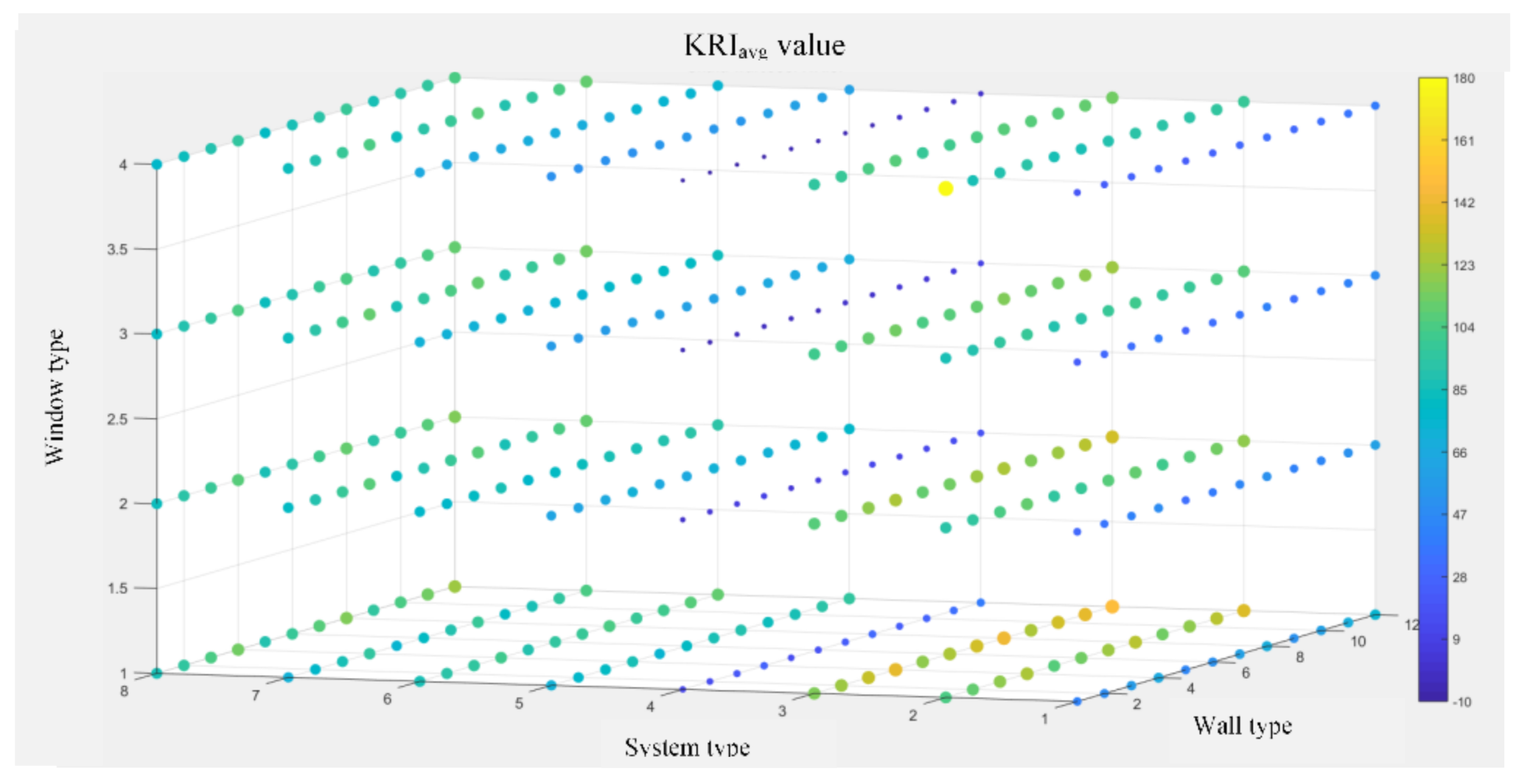
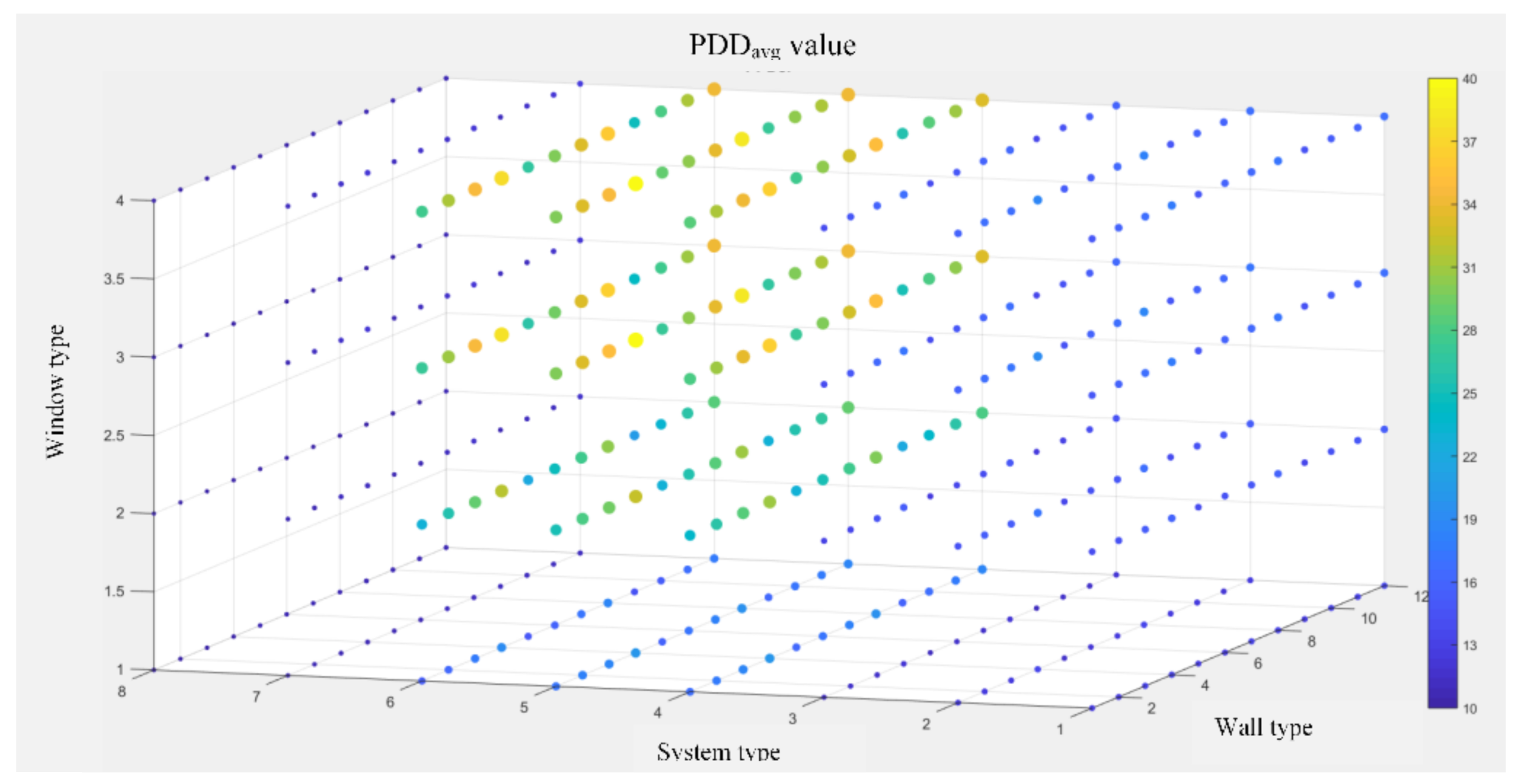
| Room Number | Room Type | Area (m2) | Zone Number | Room Number | Room Type | Area (m2) | Zone Number |
|---|---|---|---|---|---|---|---|
| 1.1 | Hall | 3.07 | 1 | 2.1 | Hall | 9.70 | 2 |
| 1.2 | Hall | 3.31 | 2 | 2.2 | Room | 14.33 | 7 |
| 1.3 | Living room | 28.99 | 2 | 2.3 | Room | 14.47 | 8 |
| 1.4 | Office room | 10.34 | 3 | 2.4 | Room | 13.54 | 9 |
| 1.5 | Kitchen | 11.68 | 2 | 2.5 | Bathroom | 8.43 | 10 |
| 1.7 | Toilet | 1.59 | 4 | 2.6 | Toilet | 2.29 | 11 |
| 1.8 | Boiler room | 3.52 | 5 | 2.7 | Room | 13.50 | 12 |
| 1.9 | Garage | 16.94 | 6 | 3.1 | Attic | 84.54 | 13 |
| Heat Transfer Coefficient U, (W/(m2K)) | |
|---|---|
| External wall | 0.283 |
| Roof | 0.208 |
| Window glazing | 1.4 |
| Doors | 1.6 |
| Garage doors | 1.6 |
| Windows g-value, (-) | 0.48 |
| Thermal bridges, Ψ-value, (W/(mK)) | |
| Corner | −0.11 |
| Floor—external wall | 0.04 |
| Roof—external wall | −0.15 |
| Window/doors—external wall | 0.08 |
| Balcony—external wall | 0.37 |
| Ground—external wall | −0.096 |
| Ground—internal wall | 0.65 |
| Room Number | Room Type | Radiator Type | Radiator Power (W) | Thermostatic Valve Flow Coefficient (m3/h) | Valve Authority |
|---|---|---|---|---|---|
| 1.1 | Hall | floor | 200 | 0.067 | 0.63 |
| 1.2 | Hall | - | - | - | - |
| 1.3 | Living room | compact | 1136 758 | 0.191 0.130 | 0.62 0.59 |
| 1.4 | Office Room | compact | 660 | 0.130 | 0.45 |
| 1.5 and 1.6 | Kitchen with dining room | rail | 258 | 0.070 | 0.24 |
| 1.7 | Toilet | rail | 251 | 0.059 | 0.32 |
| 1.8 | Boiler room | compact | 0 | 0.059 | 0.05 |
| 1.9 | Garage | compact | 630 | 0.130 | 0.41 |
| 2.1 | Hall | compact | 270 | 0.130 | 0.07 |
| 2.2 | Room | compact | 752 | 0.130 | 0.57 |
| 2.3 | Room | compact | 835 | 0.134 | 0.66 |
| 2.4 | Room | compact | 588 | 0.130 | 0.35 |
| 2.5 | Bathroom | floor rail | 443 295 | 0.180 0.054 | 0.41 0.51 |
| 2.6 | Toilet | rail | 232 | 0.070 | 0.19 |
| 2.7 | Room | compact | 877 | 0.176 | 0.42 |
| 3.1 | Attic | - | - | - | - |
| Zone | 1 | 2 | 3 | 4 | 5 | 6 | |
| 1.11–14.03 | 0.55 | 0.60 | 0.75 | 0.85 | 0.40 | 0.55 | |
| 15.03–31.05 15.09–31.10 | 0.95 | 0.95 | 0.75 | 0.85 | 0.40 | 0.95 | |
| 01.06–14.09 | 1.00 | 1.50 | 0.75 | 0.85 | 0.40 | 0.95 | |
| Zone | 7 | 8 | 9 | 10 | 11 | 12 | 13 |
| 1.11–14.03 | 0.40 | 0.40 | 0.40 | 0.40 | 0.40 | 0.50 | 0.40 |
| 15.03–31.05 15.09–31.10 | 0.95 | 0.40 | 0.95 | 0.40 | 0.40 | 0.50 | 0.40 |
| 01.06–14.09 | 1.00 | 1.00 | 1.00 | 0.50 | 1.00 | 1.00 | 0.40 |
| Zone Number | 2 | 3 | 7 | 8 | 9 | 12 |
|---|---|---|---|---|---|---|
| Supply air flow, (m3/h) | 820 | 410 | 410 | 410 | 410 | 410 |
| Nominal cooling power, (kW) | 2.0 | 2.0 | 2.0 | 2.0 | 2.0 | 2.0 |
| Period | Clothing Insulation (All Zones) (clo) | Metabolic Rate (Met) | ||||||
|---|---|---|---|---|---|---|---|---|
| 2 | 3 | 7 | 8 | 9 | 12 | |||
| 1.11–14.03 | 22-5 | 1.0 | 1.2 | 1.2 | 0.8 | 1.2 | 0.8 | 0.8 |
| 6-21 | 1.0 | 1.2 | 1.2 | 1.2 | 1.2 | 1.2 | 1.2 | |
| 15.03–31.05 15.09–31.10 | 22-5 | 0.7 | 1.2 | 1.2 | 0.8 | 1.2 | 0.8 | 0.8 |
| 6-21 | 0.7 | 1.2 | 1.2 | 1.2 | 1.2 | 1.2 | 1.2 | |
| 1.06–14.09 | 22-5 | 0.5 | 1.2 | 1.2 | 0.8 | 1.2 | 0.8 | 0.8 |
| 6-21 | 0.5 | 1.2 | 1.2 | 1.2 | 1.2 | 1.2 | 1.2 | |
| Period | External Air Temperature (°C) | Maximum/Minimum External Air Temperature (°C) | Solar Radiation, (W/m2) | ||||
|---|---|---|---|---|---|---|---|
| H | N | E | S | W | |||
| 26.01–6.02 | 1.00 | 13.83/−6.23 | 45.6 | 15.2 | 30.0 | 94.0 | 39.4 |
| 22.02–6.03 | 1.58 | 12.31/−15.67 | 66.0 | 24.9 | 40.0 | 81.3 | 52.9 |
| 04.04–11.04 | 11.78 | 26.64/0.26 | 131.6 | 43.4 | 75.1 | 111.2 | 86.1 |
| 18.04–24.04 | 10.12 | 28.88/−3.64 | 171.8 | 48.8 | 109.5 | 131.6 | 111.3 |
| 5.07–14.07 | 24.75 | 26.75/22.23 | 247.3 | 73.0 | 140.5 | 133.9 | 142.4 |
| Period | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Zone | 26.01–6.02. (te,avg * = 1.00 °C) | 22.02–6.03. (te,avg * = 1.58 °C) | 04.04–11.04. (te,avg * = 11.78 °C) | 18.04–24.04. (te,avg * = 10.12 °C) | 05.07–13.07. (te,avg * = 24.75 °C) | |||||
| σ K | ∆t K | σ K | ∆t K | σ K | ∆t K | σ K | ∆t K | σ K | ∆t K | |
| 1 | 1.03 | −0.30 | 0.56 | 0.45 | 0.66 | 0.07 | 0.78 | 0.52 | 0.75 | 0.53 |
| 2 | 0.60 | −0.42 | 0.75 | −0.27 | 1.36 | −0.45 | 1.20 | −0.26 | 1.25 | −0.52 |
| 3 | 0.69 | −0.36 | 0.71 | −0.48 | 1.18 | −0.54 | 0.96 | 0.15 | 0.92 | −0.14 |
| 4 | 1.87 | −0.54 | 1.32 | 0.04 | 1.92 | −0.27 | 1.71 | −0.17 | 1.48 | 0.26 |
| 5 | 0.76 | 0.56 | 1.09 | 1.00 | 0.75 | −0.32 | 0.74 | 0.36 | 0.69 | 0.16 |
| 6 | 0.88 | −0.59 | 0.85 | 0.88 | 0.88 | 0.50 | 0.73 | 0.09 | 1.52 | 1.05 |
| 7 | 0.55 | −0.12 | 0.53 | 0.01 | 0.71 | 0.10 | 0.90 | 0.52 | 1.41 | −0.99 |
| 8 | 0.66 | −0.48 | 0.48 | −0.19 | 0.65 | −0.32 | 0.70 | −0.21 | 1.19 | −0.32 |
| 9 | 0.76 | −0.25 | 0.81 | 0.50 | 1.07 | 0.46 | 1.03 | 0.15 | 1.80 | −1.26 |
| 10 | 0.65 | −0.45 | 0.42 | 0.03 | 0.45 | 0.24 | 0.48 | 0.17 | 0.70 | −0.05 |
| 11 | - | - | - | - | 0.45 | 0.00 | 0.58 | 0.00 | - | - |
| 12 | 0.82 | −0.37 | 0.72 | 0.18 | 0.75 | −0.13 | 1.10 | −0.23 | 1.26 | 0.33 |
| 13 | 1.48 | 0.54 | 1.03 | 0.85 | 1.73 | −0.67 | 1.53 | 0.03 | 1.41 | 0.72 |
| ΔQh,k% | −0.8 | 5.4 | 1.5 | −4.0 | - | |||||
| 0.88 | 0.62 | 1.13 | 1.03 | 1.28 | ||||||
| Δti ** | −0.21 | 0.23 | −0.10 | 0.09 | 0.10 | |||||
| Δti′ *** | −0.36 | 0.03 | −0.09 | 0.07 | −0.07 | |||||
| New Variable “Wall Type” | W_2 | W_4 | W_7 | W_10 | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Al | I | Uavg | Al | I | Uavg | Al | I | Uavg | Al | I | Uavg | |
| - | cm | - | cm | - | cm | - | cm | |||||
| 1 | 0.33 | 10 | 0.218 | 0.33 | 10 | 0.236 | 0.33 | 10 | 0.254 | 0.33 | 10 | 0.272 |
| 2 | 0.50 | 10 | 0.223 | 0.50 | 10 | 0.242 | 0.50 | 10 | 0.261 | 0.50 | 10 | 0.280 |
| 3 | 0.67 | 10 | 0.228 | 0.67 | 10 | 0.248 | 0.67 | 10 | 0.269 | 0.67 | 10 | 0.290 |
| 4 | 0.83 | 10 | 0.233 | 0.83 | 10 | 0.255 | 0.83 | 10 | 0.277 | 0.83 | 10 | 0.299 |
| 5 | 0.33 | 5 | 0.260 | 0.33 | 5 | 0.278 | 0.33 | 5 | 0.296 | 0.33 | 5 | 0.313 |
| 6 | 0.50 | 5 | 0.264 | 0.50 | 5 | 0.283 | 0.50 | 5 | 0.302 | 0.50 | 5 | 0.321 |
| 7 | 0.67 | 5 | 0.269 | 0.67 | 5 | 0.290 | 0.67 | 5 | 0.310 | 0.67 | 5 | 0.331 |
| 8 | 0.83 | 5 | 0.273 | 0.83 | 5 | 0.296 | 0.83 | 5 | 0.318 | 0.83 | 5 | 0.340 |
| 9 | 0.33 | 0 | 0.325 | 0.33 | 0 | 0.343 | 0.33 | 0 | 0.361 | 0.33 | 0 | 0.379 |
| 10 | 0.50 | 0 | 0.329 | 0.50 | 0 | 0.348 | 0.50 | 0 | 0.367 | 0.50 | 0 | 0.386 |
| 11 | 0.67 | 0 | 0.333 | 0.67 | 0 | 0.354 | 0.67 | 0 | 0.375 | 0.67 | 0 | 0.395 |
| 12 | 0.83 | 0 | 0.337 | 0.83 | 0 | 0.359 | 0.83 | 0 | 0.382 | 0.83 | 0 | 0.404 |
| Window Type | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Uw (W/(m2K)) | 0.8 | 0.8 | 1.0 | 1.0 | 1.2 | 1.2 | 1.2 | 1.4 | 1.4 | 1.4 | 1.6 | 1.6 |
| gg (–) | 0.21 | 0.28 | 0.28 | 0.35 | 0.32 | 0.35 | 0.42 | 0.35 | 0.42 | 0.48 | 0.35 | 0.42 |
| Zone | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | Σ |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Supply air (m3/h) | 0 | 100 | 50 | 0 | 0 | 0 | 50 | 50 | 50 | 0 | 0 | 50 | 0 | 350 |
| Exhaust air (m3/h) | 30 | 160 | 0 | 30 | 0 | 30 | 0 | 0 | 0 | 70 | 30 | 0 | 0 | 350 |
| Zone | 2 | 3 | 7 | 8 | 9 | 12 |
|---|---|---|---|---|---|---|
| zone area [m2] | 53.7 | 10.3 | 14.3 | 14.5 | 13.5 | 13.5 |
| wPPD_k | 0.45 | 0.09 | 0.12 | 0.12 | 0.11 | 0.11 |
| Type of Window | W_2 | W_4 | W_7 | W_10 | |
|---|---|---|---|---|---|
| Change of type of windows | Costs, EUR/m2 | 79.55 | 45.45 | 22.73 | 0.00 |
| Change of window area in the living room on the garden façade | Costs, EUR/m2 | 88.64 | 54.55 | 31.82 | 9.09 |
| System Type | VN + S | VN + C | VN + C + S | VM | VM + S | VM + C | VM + C + S |
|---|---|---|---|---|---|---|---|
| EUR | |||||||
| - | 2442 | 5814 | 6512 | ||||
| Al = 33% | 2244 | 4686 | 8058 | 8755 | |||
| Al = 50% | 2428 | 4870 | 8242 | 8940 | |||
| Al = 67% | 2662 | 5104 | 8476 | 9174 | |||
| Al = 83% | 2882 | 5324 | 8696 | 9393 | |||
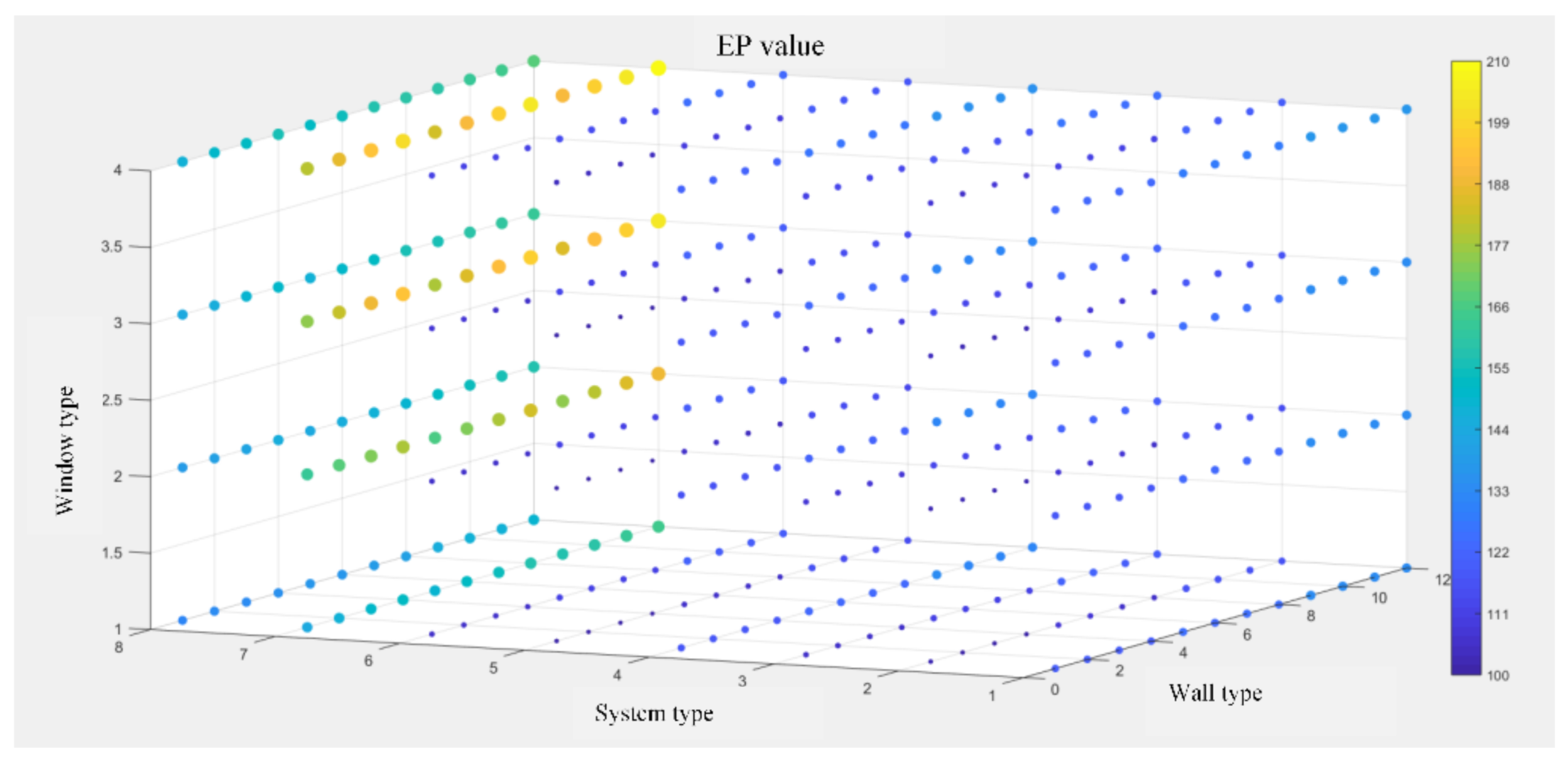
| Criterion | Variant Number | Value of Decision Variables 0 | EP | SPBT | PPDavg | DTavg | KRIavg | |
|---|---|---|---|---|---|---|---|---|
| - | - | - | - | years | % | K | % | |
| 1 | min EP | w270 w246 w269 | (4,2,5) (3,2,5) (4,1,5) | 103.2 103.3 103.3 | 57.3 56.0 66.2 | 32.10 29.62 20.57 | 4.30 4.04 2.92 | 97.4 94.5 113.6 |
| 2 | min SPBT | w2 w118 w 142 | (5,4,4) (6,4,4) (7,4,4) | 126.3 126.5 126.6 | 14.2 14.9 15.8 | 27.45 30.09 32.65 | 3.94 4.28 4.59 | 1.82 2.04 2.33 |
| 3 | min PPDavg | w197 w198 w218 | (1,2,8) (1,3,8) (2,2,8) | 137.4 141.6 139.4 | NN 1 NN 1 NN 1 | 10.86 10.91 10.93 | 0.58 0.61 0.60 | 75.0 68.1 79.7 |
| 4 | min KRIavg | w4 w5 w6 | (10,4,4) (11,4,4) (12,4,4) | 134.51 134.54 134.57 | NN 1 NN 1 NN 1 | 28.47 30.90 33.14 | 4.10 4.41 4.69 | 0.23 0.51 0.78 |
| 5 | min KRIEavg 3 | w3 w208 w232 | (1,4,4) (2,4,4) (3,4,4) | 121.1 121.8 122.0 | 18.3 18.9 19.6 | 28.44 31.17 33.81 | 4.05 4.38 4.70 | −9.5 2 −9.0 2 −8.5 2 |
| 6 | min PPDavgSPBT < 70 EP < 134,5 | w191 w210 w192 | (1,2,1) (2,2,1) (1,3,1) | 119.6 119.7 120.4 | 60.4 65.9 56.2 | 14.7 15.28 15.73 | 2.01 2.11 2.19 | 45.0 49.8 38.1 |
| 7 | min EP SPBT < 70 PPDavg < 20% | w245 w221 w199 | (3,1,5) (2,1,5) (1,1,5) | 103.5 103.6 103.8 | 63.8 61.3 59.4 | 19.33 18.88 17.58 | 2.76 2.58 2.40 | 108.6 103.3 99.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zawada, B.; Rucińska, J. Optimization of Modernization of a Single-Family Building in Poland Including Thermal Comfort. Energies 2021, 14, 2925. https://doi.org/10.3390/en14102925
Zawada B, Rucińska J. Optimization of Modernization of a Single-Family Building in Poland Including Thermal Comfort. Energies. 2021; 14(10):2925. https://doi.org/10.3390/en14102925
Chicago/Turabian StyleZawada, Bernard, and Joanna Rucińska. 2021. "Optimization of Modernization of a Single-Family Building in Poland Including Thermal Comfort" Energies 14, no. 10: 2925. https://doi.org/10.3390/en14102925
APA StyleZawada, B., & Rucińska, J. (2021). Optimization of Modernization of a Single-Family Building in Poland Including Thermal Comfort. Energies, 14(10), 2925. https://doi.org/10.3390/en14102925






