Experimental Investigation of the Spatial and Temporal Evolution of the Tangential and Normal E-Field Components along the Stress Grading System of a Real Stator Bar
Abstract
1. Introduction
2. Materials and Methods
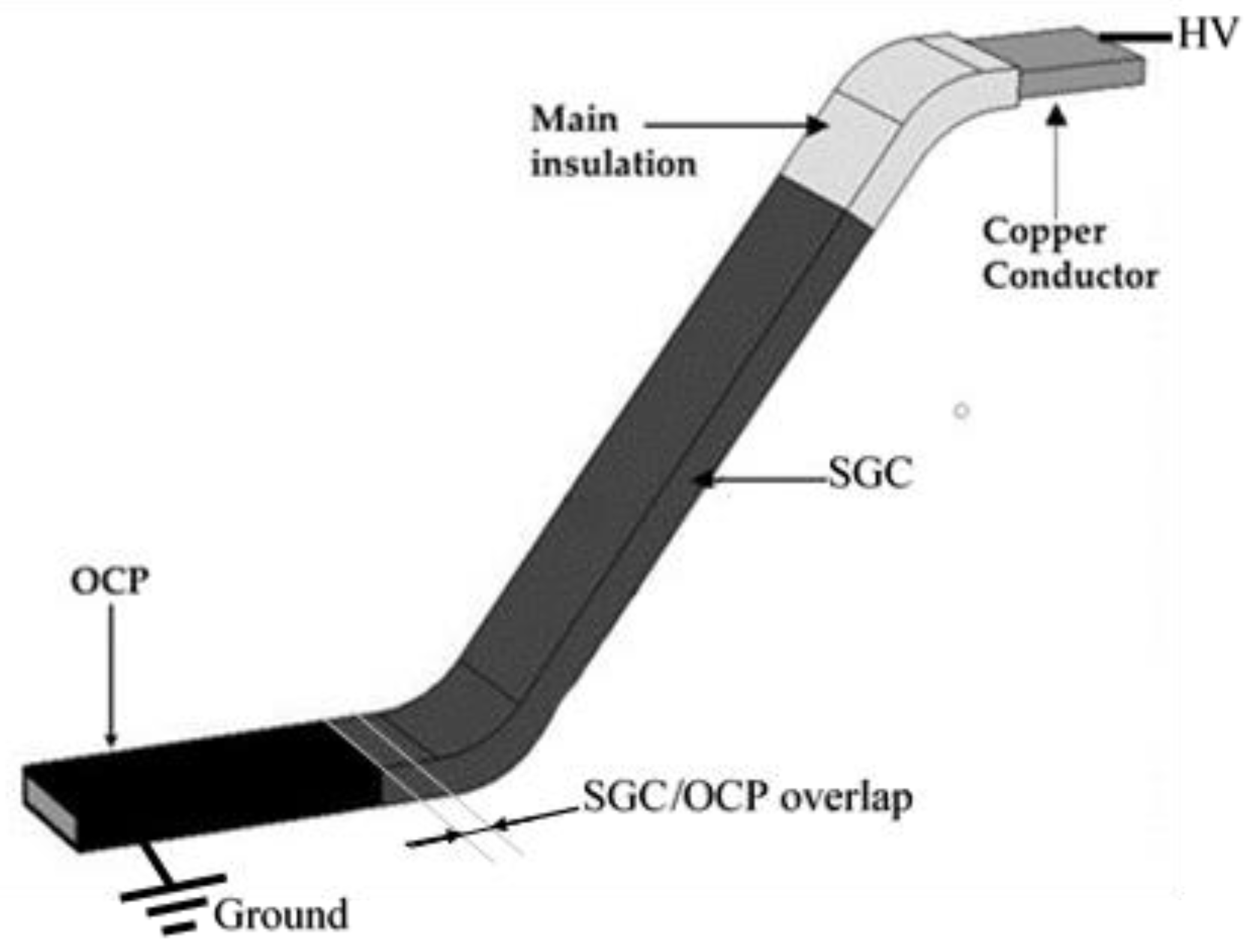
2.1. Model of the Stator Bar
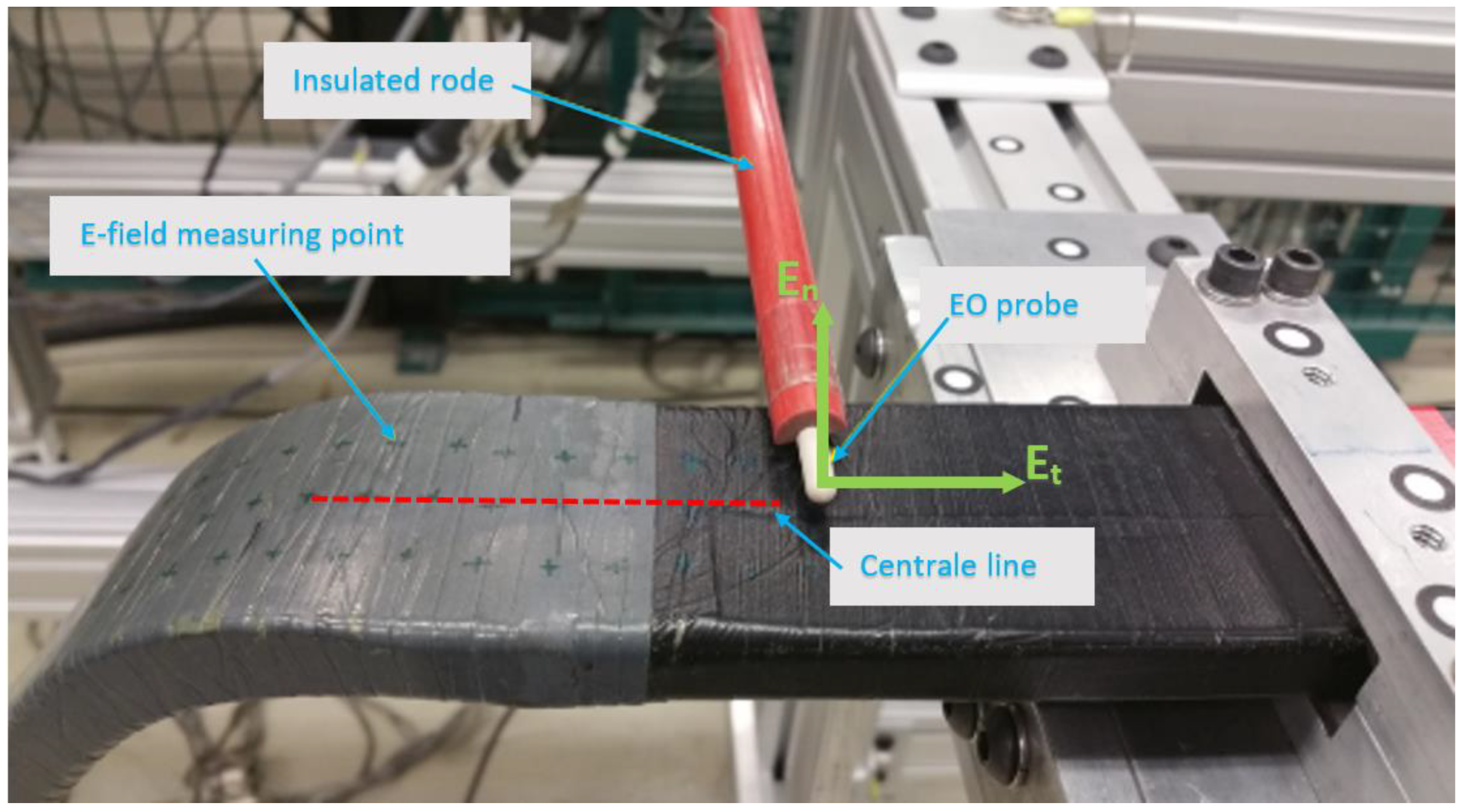
2.2. Electro-Optic E-Field System
2.3. Experimental Setup
3. Experimental Results
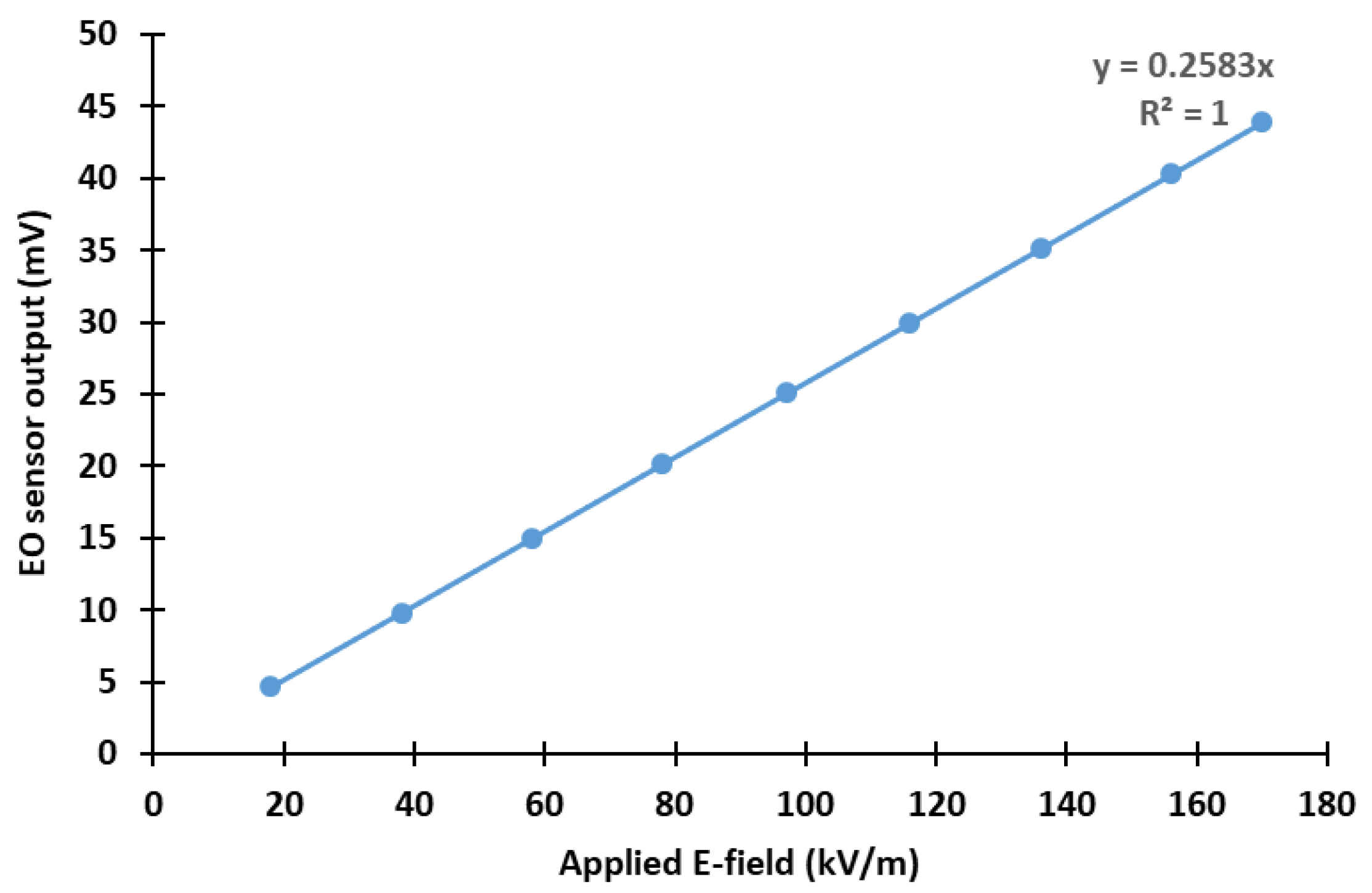
3.1. EO E-Field System Calibration
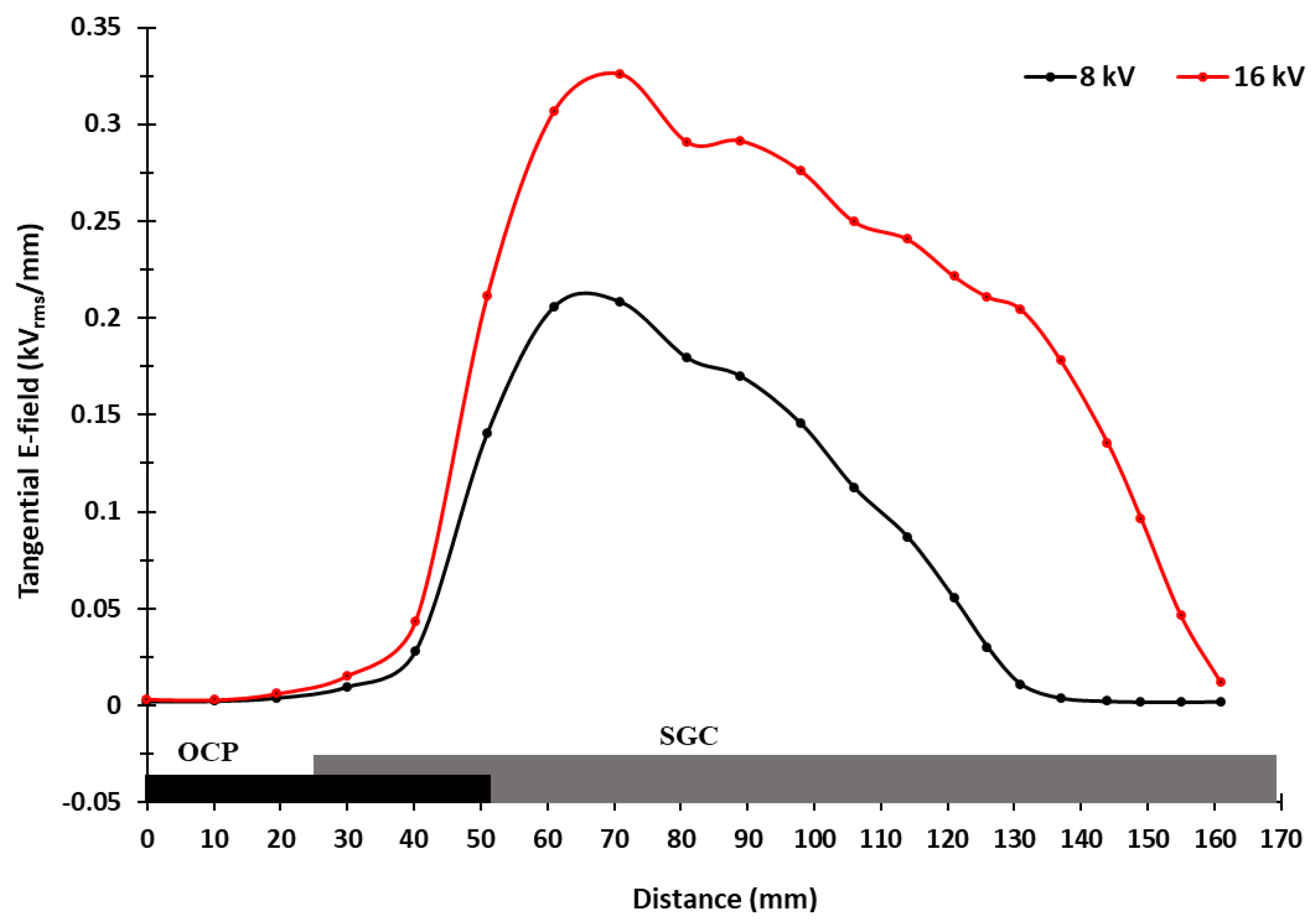
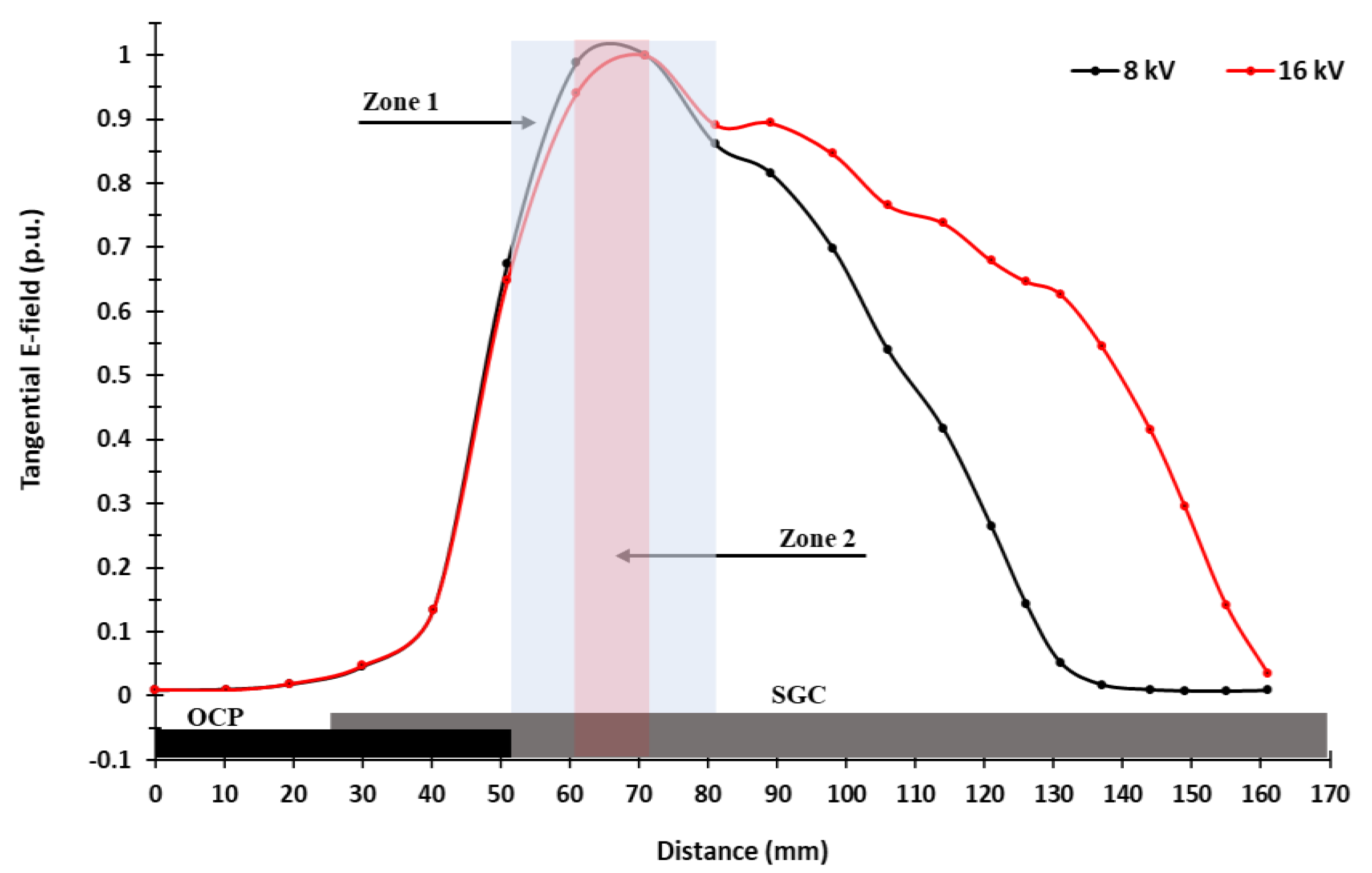
3.2. Distributrions of the Tangential E-Field Component
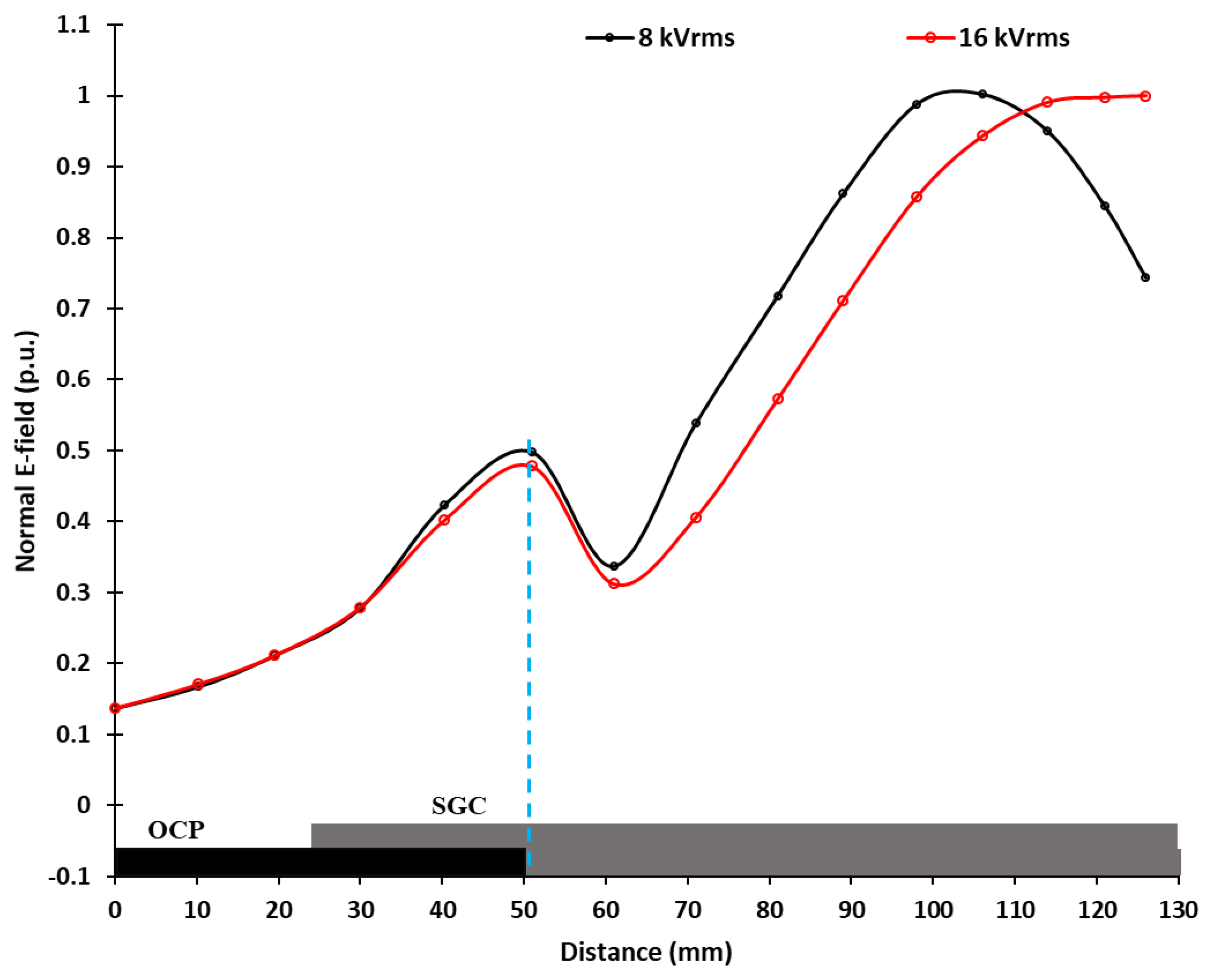
3.3. Distributrions of the Normal E-Field Component
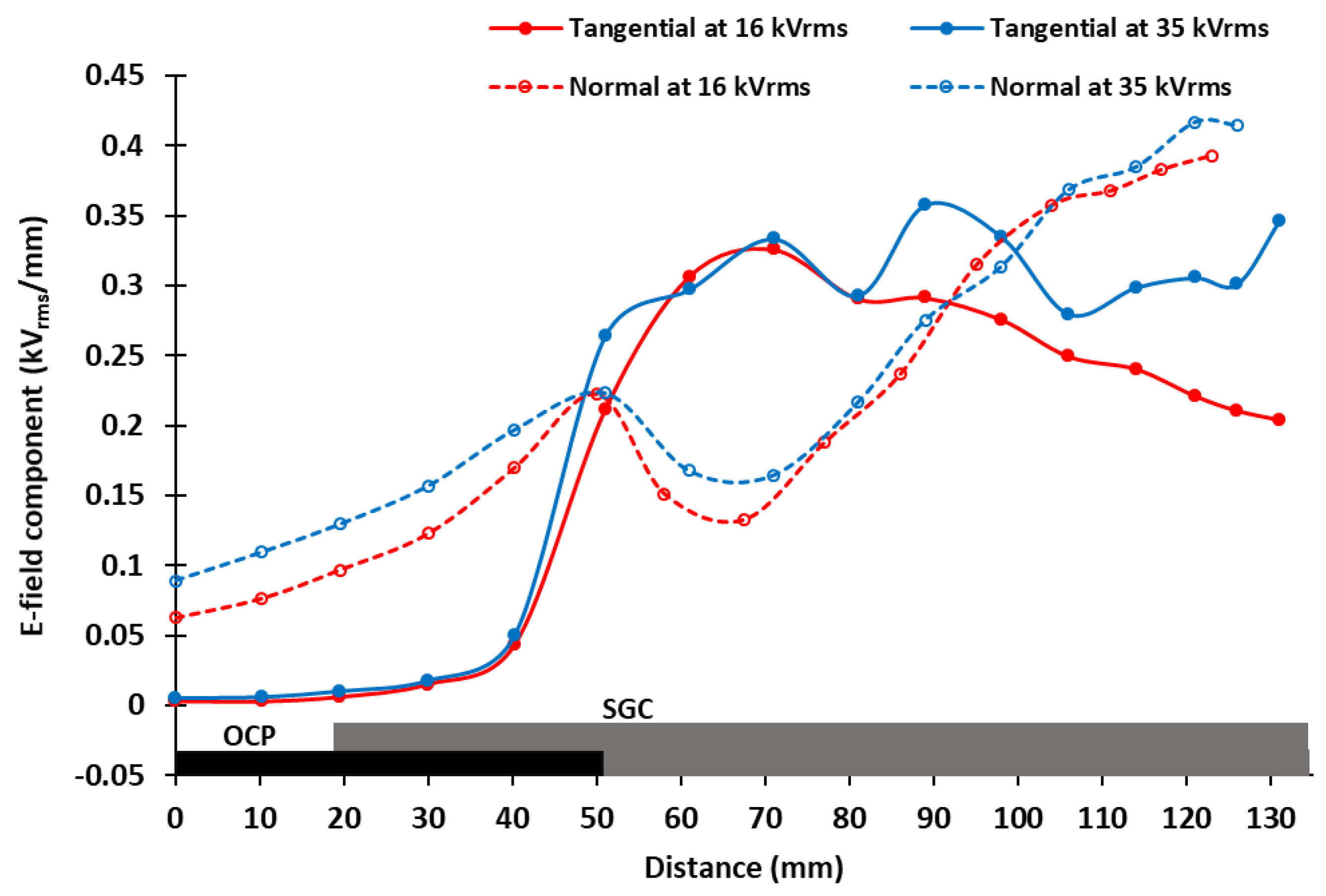
3.4. Distribution of the Tangential and Normal E-Field Components at 35 kVrms
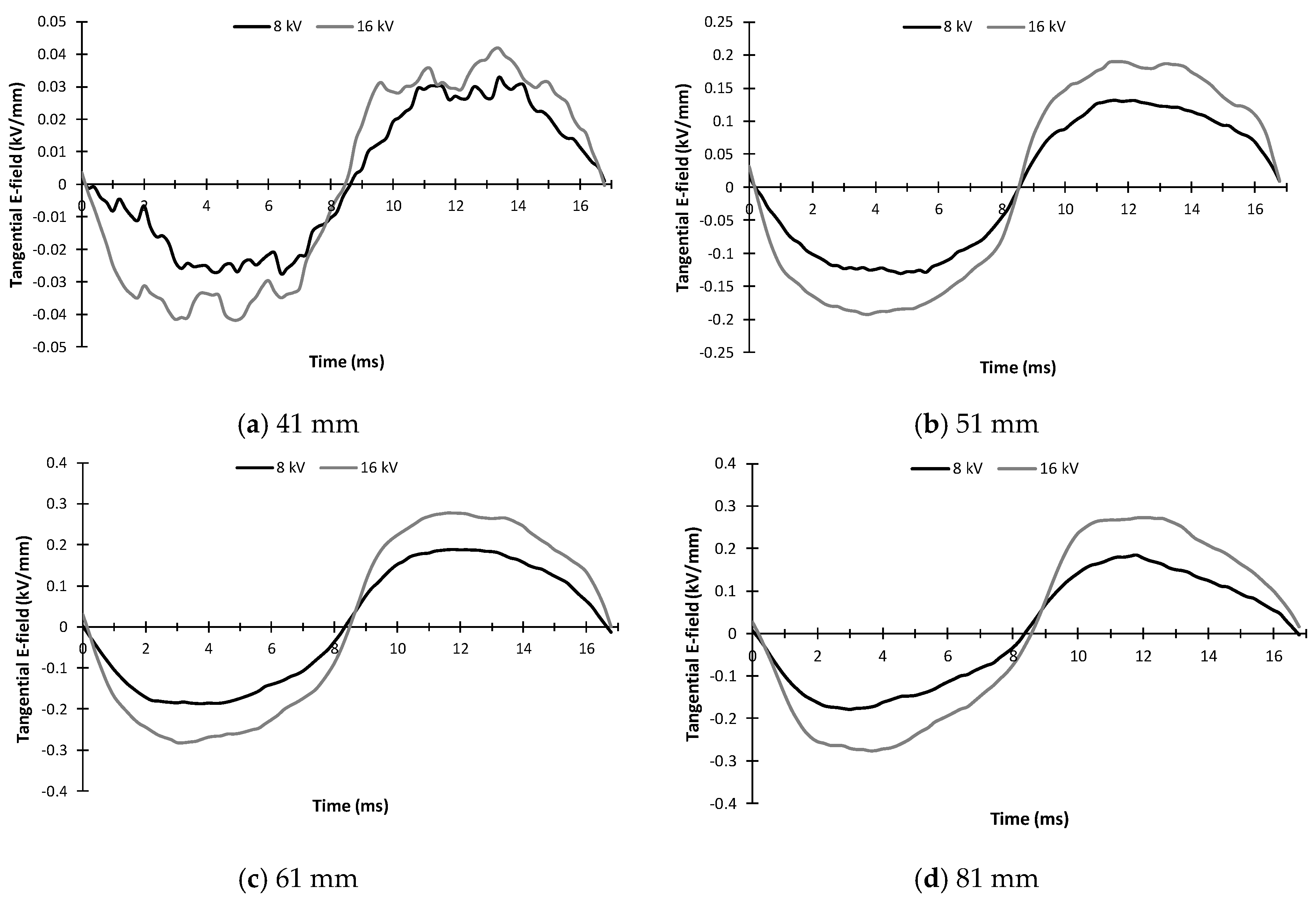
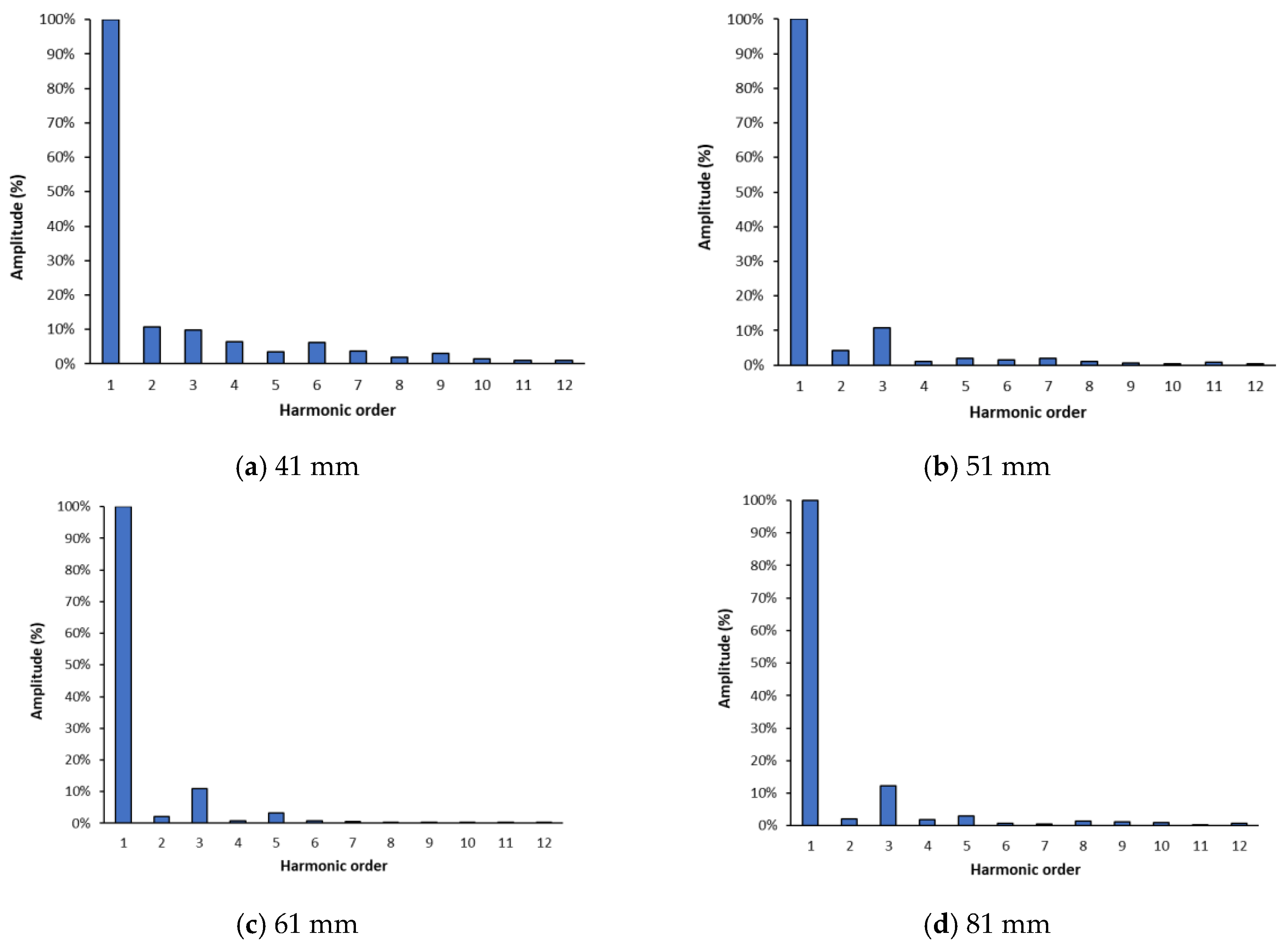
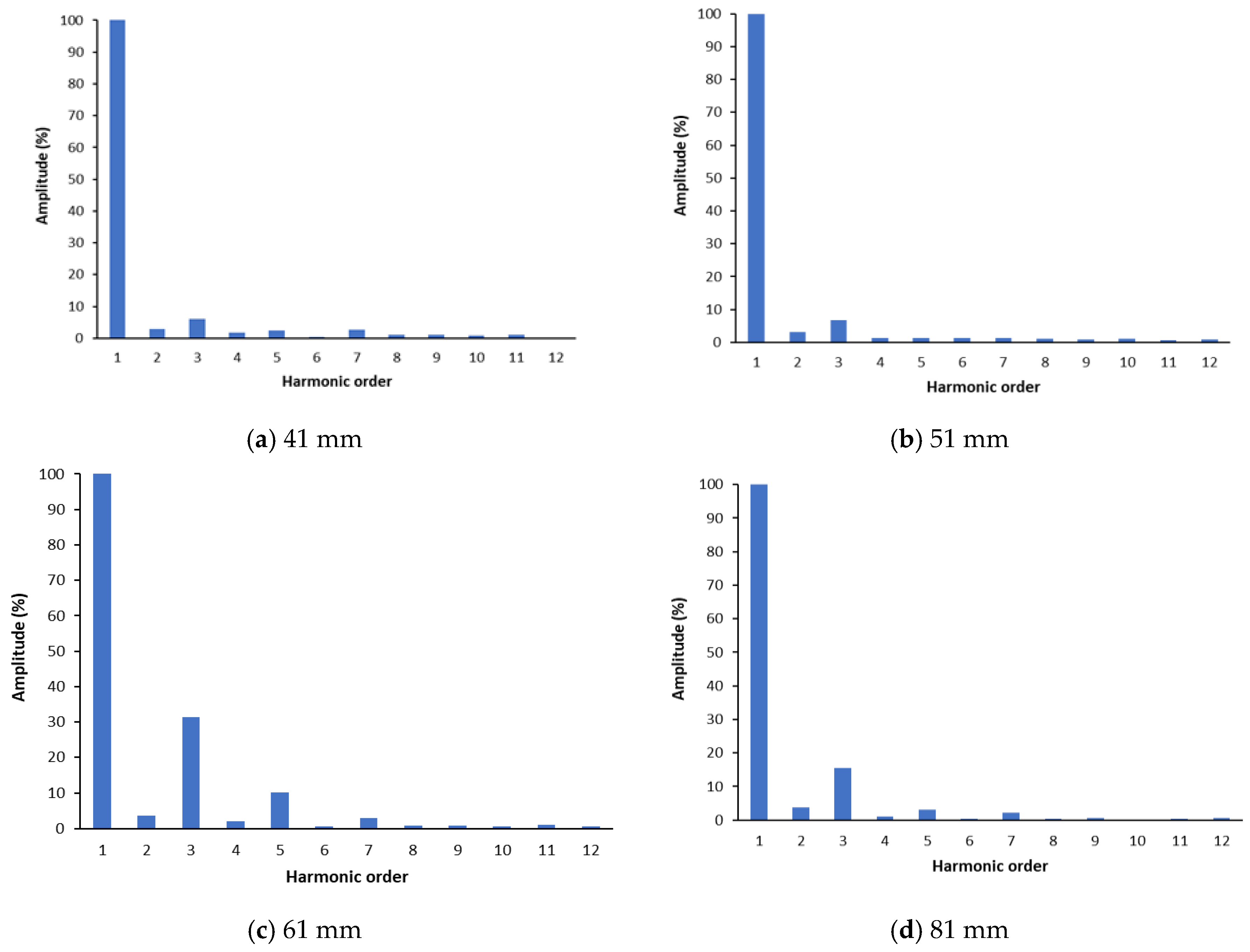
3.5. Waveforms of the Tangential and Normal E-Field Components
4. Discussion
4.1. Tangential E-Field Component
4.2. Normal E-Field Component
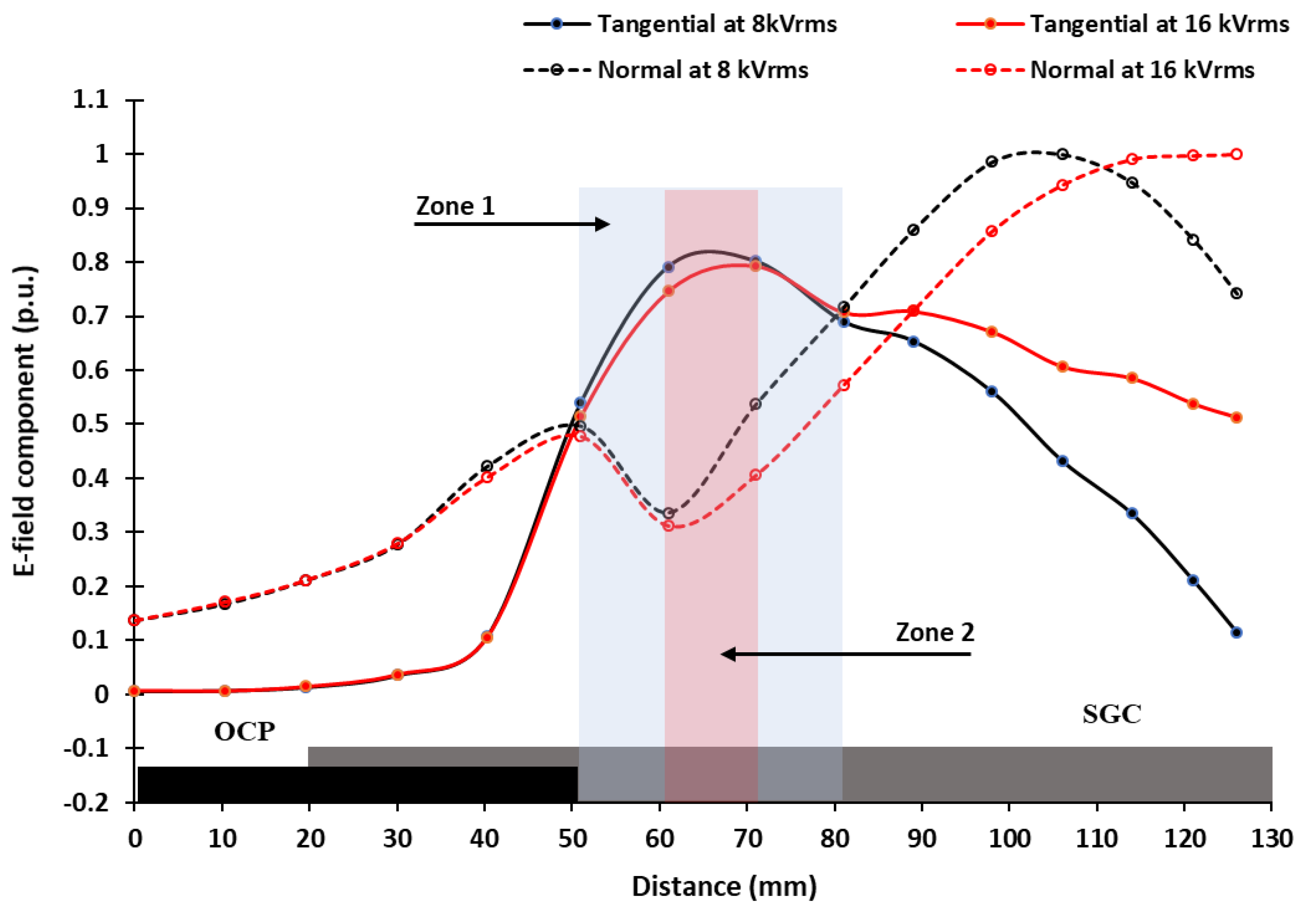
4.3. Dependency of the Tangential and Normal E-Field Components on the Applied Voltage
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Staubach, C.; Hildinger, T.; Staubach, A. Comprehensive Electrical and Thermal Analysis of the Stress Grading System of Large Hydro Generator. IEEE Electr. Insul. Mag. 2018, 34, 37–49. [Google Scholar] [CrossRef]
- Christen, T.; Donzel, L.; Greuter, F. Nonlinear Resistive Electric Field Grading Part 1: Theory and Simulation. IEEE Electr. Insul. Mag. 2010, 26, 47–59. [Google Scholar] [CrossRef]
- Qi, X.; Zheng, Z.; Boggs, S. Engineering with nonlinear dielectrics. IEEE Electr. Insul. Mag. 2004, 20, 27–34. [Google Scholar] [CrossRef]
- Tremblay, R.; Hudon, C. Improved Requirements for Stress-Grading Systems at Hydro-Québec. In Proceedings of the Iris Rotating Machine Conference (IRMC), San Antonio, TX, USA, 4–7 June 2007. [Google Scholar]
- Hudon, C.; Bélec, M. Partial discharge signal interpretation for generator diagnostics. IEEE Trans. Dielect. Ins. 2005, 12, 297–319. [Google Scholar] [CrossRef]
- Stone, G.C.; Boulter, E.A.; Culbert, I.; Dhirani, H. Electrical Insulation for Rotating Machines: Design, Evaluation, Aging, Testing and Repair; Wiley-IEEE Press: Hoboken, NJ, USA, 2014. [Google Scholar]
- Kempen, S.; Pohlmann, F.; Pinkert, K. Comparison of low-interaction methods of measurement for determining the distribution of surface potential on end corona protection configurations. In Proceedings of the Insucon Conference, Birmingham, UK, 25–28 May 2009. [Google Scholar]
- Shafiri, E. Analysis of Electrical and Thermal Stresses in the Stress Relief System of Inverter Fed Medium Voltage Induction Motors. Ph.D. Thesis, University of Waterloo, Waterloo, ON, Canada, 2010. [Google Scholar]
- Kumada, A.; Nakamura, T.; Hidaka, K.; Tsuboi, Y.; Yoshimitsu, T. Potential distribution on the stress grading system of high-voltage rotating machines—Part I: Measuring system. IEEE Trans. Dielectr. Electr. Insul. 2015, 22, 3163–3169. [Google Scholar] [CrossRef]
- Staubach, C.; Merte, R. Direct Electrical Field Strength Distribution Determination on Electrical Apparatus by Means of an Electro-Optical Miniature Field Sensor. In Proceedings of the 19th International Symposium on High Voltage Engineering, Pilsen, Czech Republic, 23–28 August 2015. [Google Scholar]
- Kainz, A.; Keplinger, F.; Hortschitz, W.; Kahr, M.; Steiner, H.; Stifter, M.; Hunt, J.R.; Resta-Lopez, J.; Rodin, V.; Welsch, C.P.; et al. Noninvasive 3D Field Mapping of Complex Static Electric Fields. Phys. Rev. Lett. 2019, 122, 244801. [Google Scholar] [CrossRef] [PubMed]
- Kainz, A.; Steiner, H.; Schalko, J.; Jachimowicz, A.; Kohl, F.; Stifter, M.; Beigelbeck, R.; Keplinger, F.; Hortschitz, W. Distorsion-free measurement of electric field strength with a MEMS sensor. Nat. Electron. 2018, 1, 68–73. [Google Scholar] [CrossRef] [PubMed]
- Gaborit, G.; Jarrige, P.; Lecoche, F.; Dahdah, J.; Duraz, E.; Volat, C.; Duvillaret, L. Single Shot and Vectorial Characterization of Intense Electric Field in Various Environments with Pigtailed Electrooptic Probe. IEEE Trans. Plasma Sci. 2014, 42, 1265–1273. [Google Scholar] [CrossRef]
- Volat, C.; Duvillaret, L.; Lecoche, F.; Dahdah, J.; Gaborit, G. Contactless optical sensors for in situ AC and DC electric field measurement and diagnostics. In Proceedings of the Electrical Insulation Conference (EIC), Ottawa, ON, Canada, 2–5 June 2013. [Google Scholar]
- ASTM D257-14. Standard Test Methods for DC Resistance or Conductance of Insulating Materials; ASTM International: West Conshohocken, PA, USA, 2014; p. 18. [Google Scholar]
- ASTM D4496-13. Standard Test Methods for DC Resistance or Conductance of Moderately Conductive Materials; ASTM International: West Conshohocken, PA, USA, 2013; p. 14. [Google Scholar]






| Applied Voltage (kVrms) | Normal E-field (kVrms/mm) | Tangential E-field (kVrms/mm) |
|---|---|---|
| 8 | 0.13 | 0.21 |
| 16 | 0.19 | 0.33 |
| 35 | 0.22 | 0.33 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Koné, G.; Volat, C.; Hudon, C.; Bernier, S. Experimental Investigation of the Spatial and Temporal Evolution of the Tangential and Normal E-Field Components along the Stress Grading System of a Real Stator Bar. Energies 2020, 13, 534. https://doi.org/10.3390/en13030534
Koné G, Volat C, Hudon C, Bernier S. Experimental Investigation of the Spatial and Temporal Evolution of the Tangential and Normal E-Field Components along the Stress Grading System of a Real Stator Bar. Energies. 2020; 13(3):534. https://doi.org/10.3390/en13030534
Chicago/Turabian StyleKoné, Gbah, Christophe Volat, Claude Hudon, and Simon Bernier. 2020. "Experimental Investigation of the Spatial and Temporal Evolution of the Tangential and Normal E-Field Components along the Stress Grading System of a Real Stator Bar" Energies 13, no. 3: 534. https://doi.org/10.3390/en13030534
APA StyleKoné, G., Volat, C., Hudon, C., & Bernier, S. (2020). Experimental Investigation of the Spatial and Temporal Evolution of the Tangential and Normal E-Field Components along the Stress Grading System of a Real Stator Bar. Energies, 13(3), 534. https://doi.org/10.3390/en13030534






