Speed Fluctuation Suppression for the Inverter Compressor Based on the Adaptive Revised Repetitive Controller
Abstract
1. Introduction
- It is convenient to activate or suspend RRC without affecting system normal operation.
- S–G filter implementation is simple and only consumes a small amount of multiplications and additions, which maintains the resonant frequencies corresponding with fundamental and harmonic disturbance.
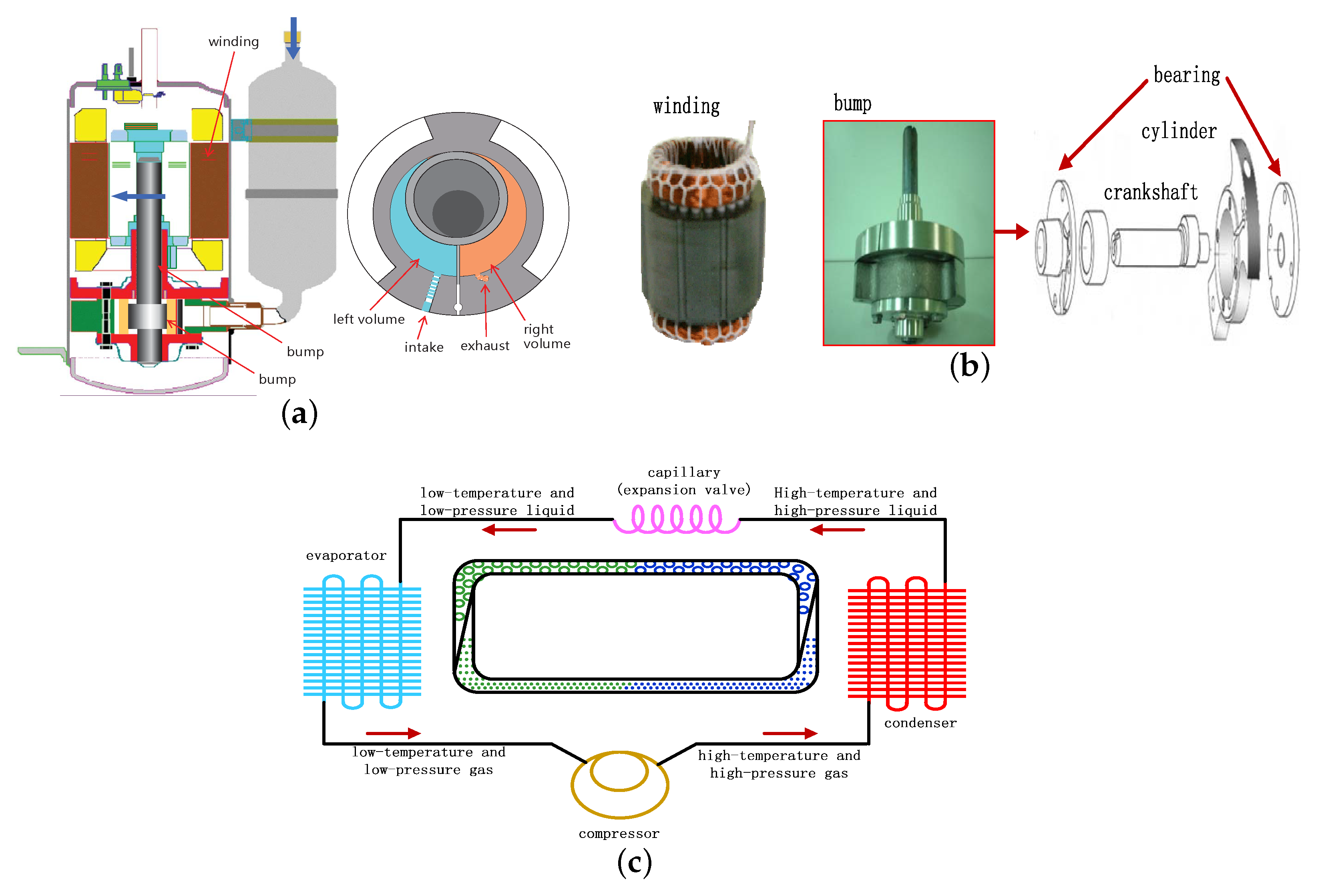
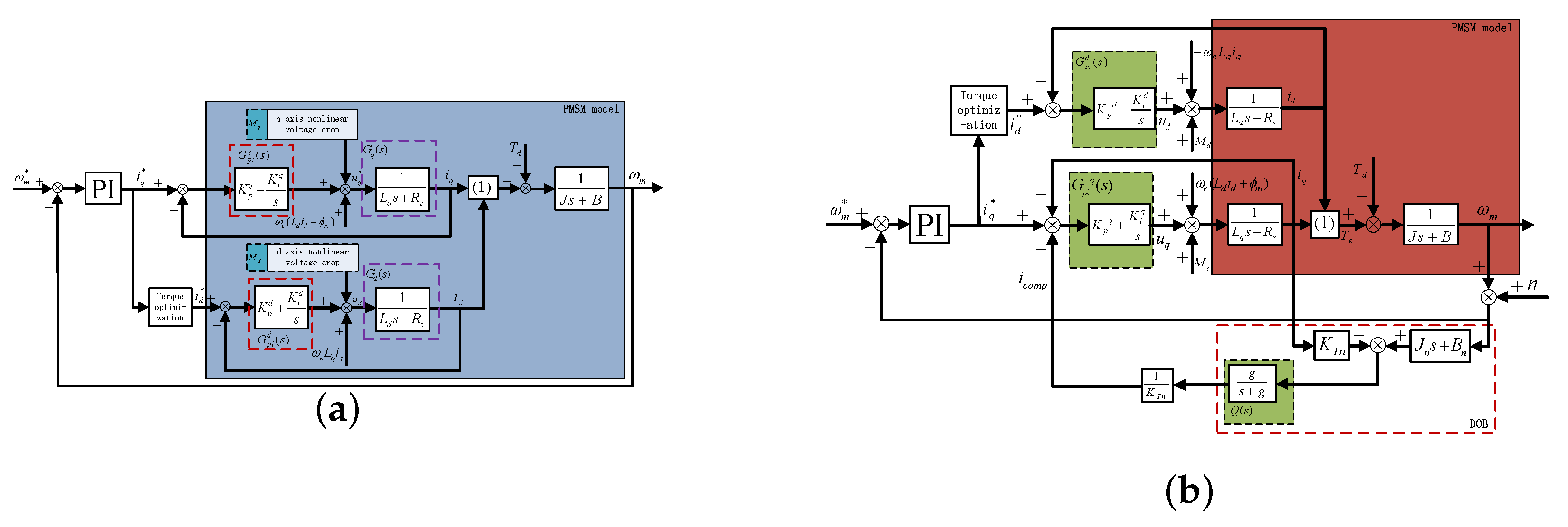
2. System Modeling and Analysis
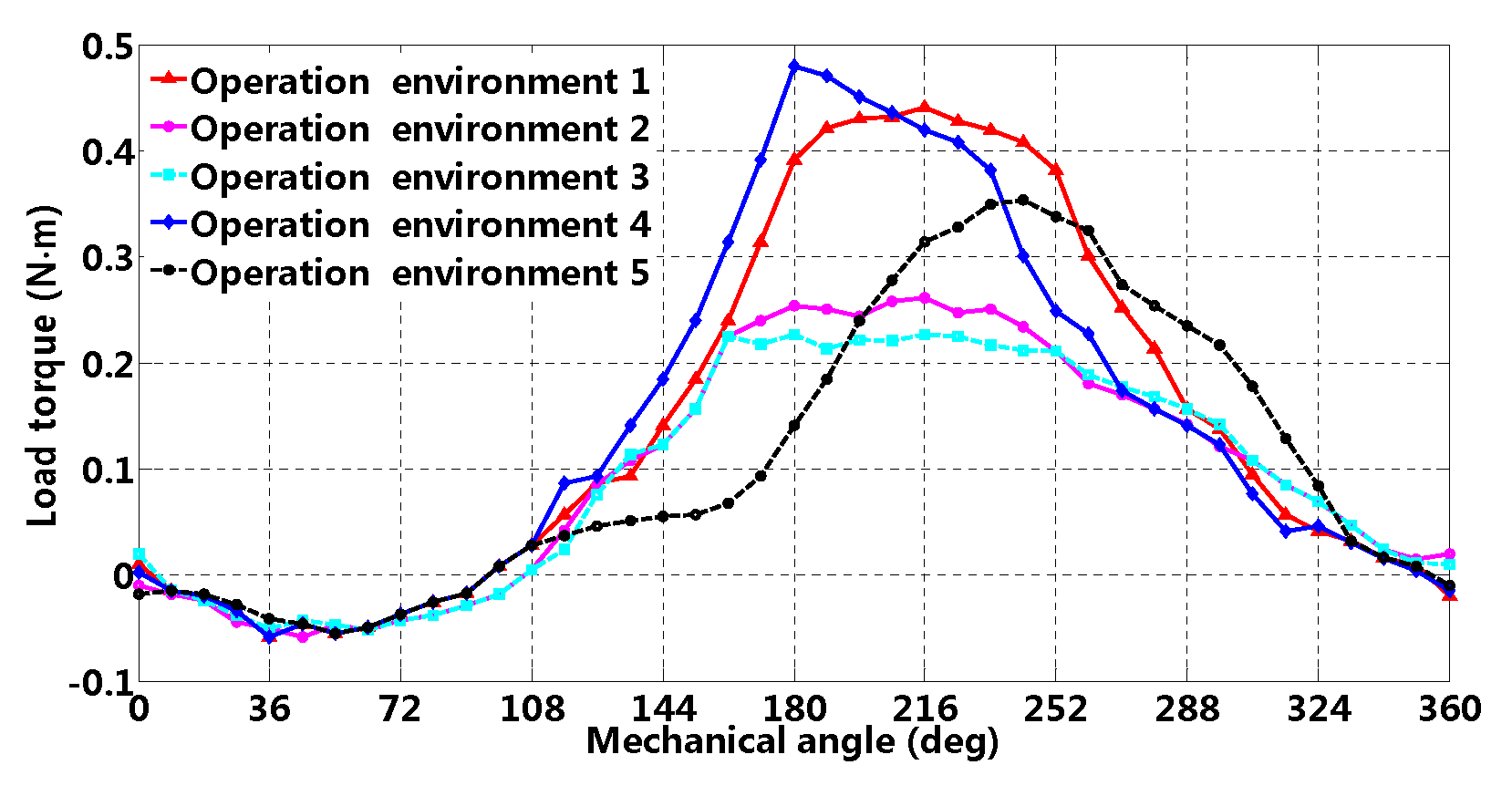
- Assumption 1: Load disturbance has identical periodicity and repetitiveness under almost the same operating condition.
- Assumption 2: The control objective aims to suppress speed fluctuation repetitively under almost the same operating condition.
- Assumption 3: Measurement noise mainly exists in the high frequency range, and the general low pass filter can filter off effectively.
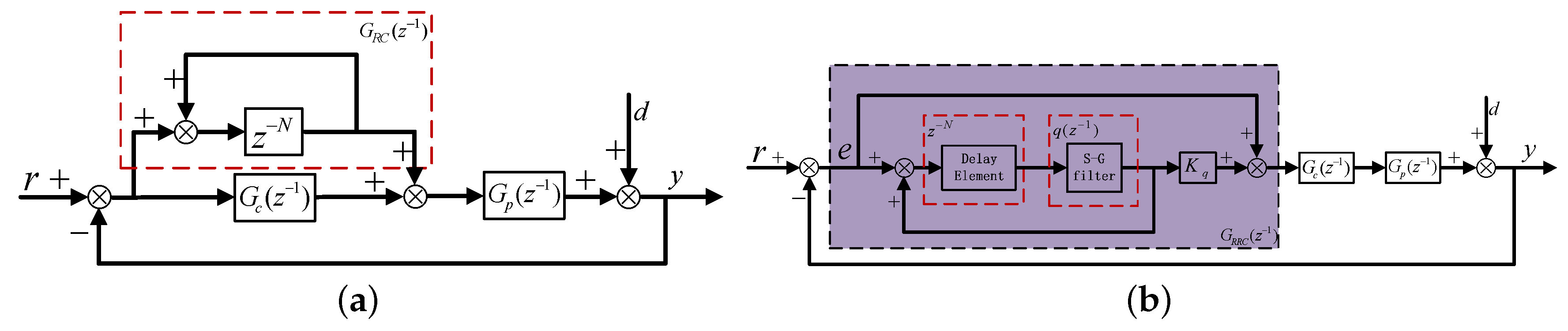
3. Revised Repetitive Controller Analysis and Design
3.1. Ideal Repetitive Controller
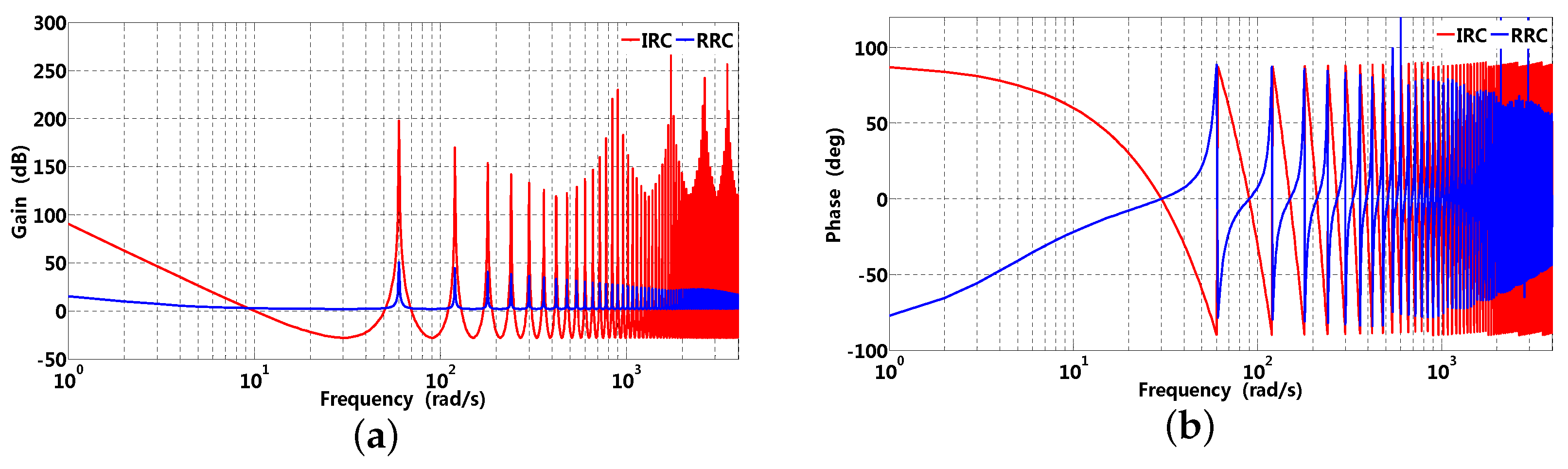
3.2. Revised Repetitive Controller
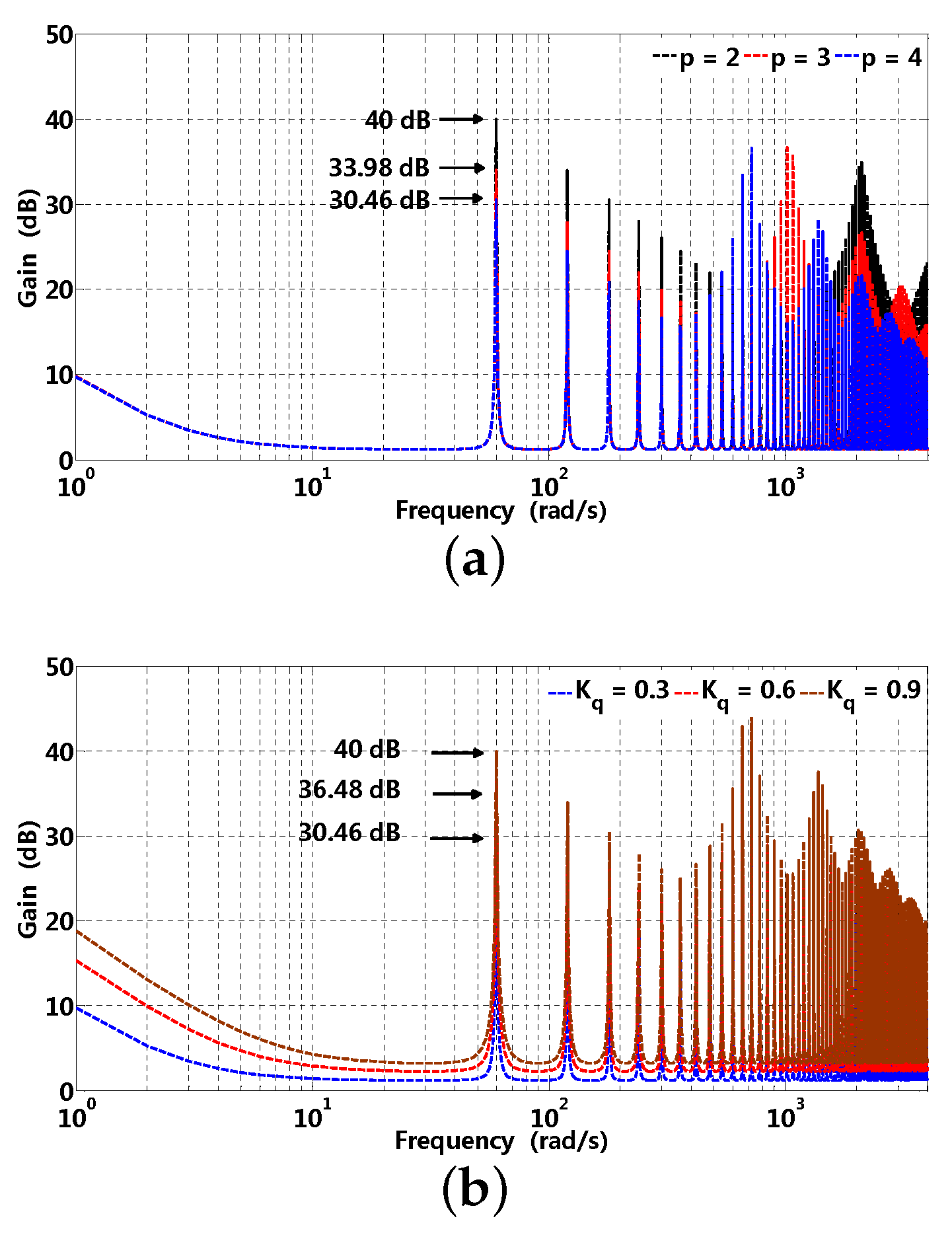
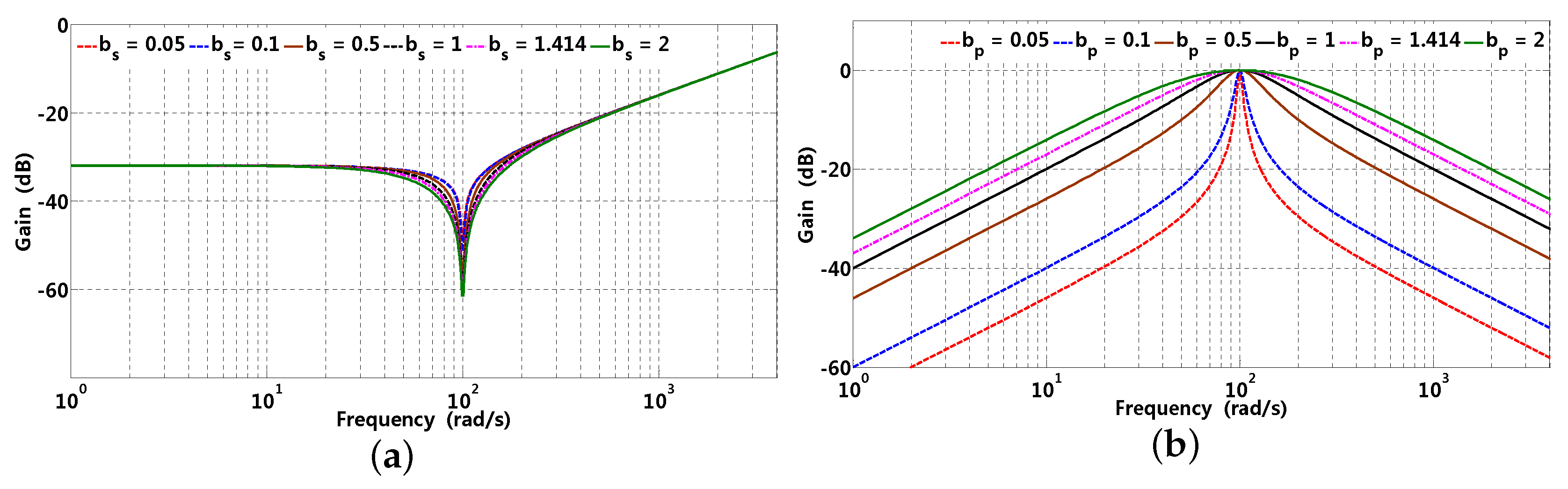
3.2.1. Coefficients of the S–G Filter
3.2.2. Phase Characteristics
3.2.3. Selection of D
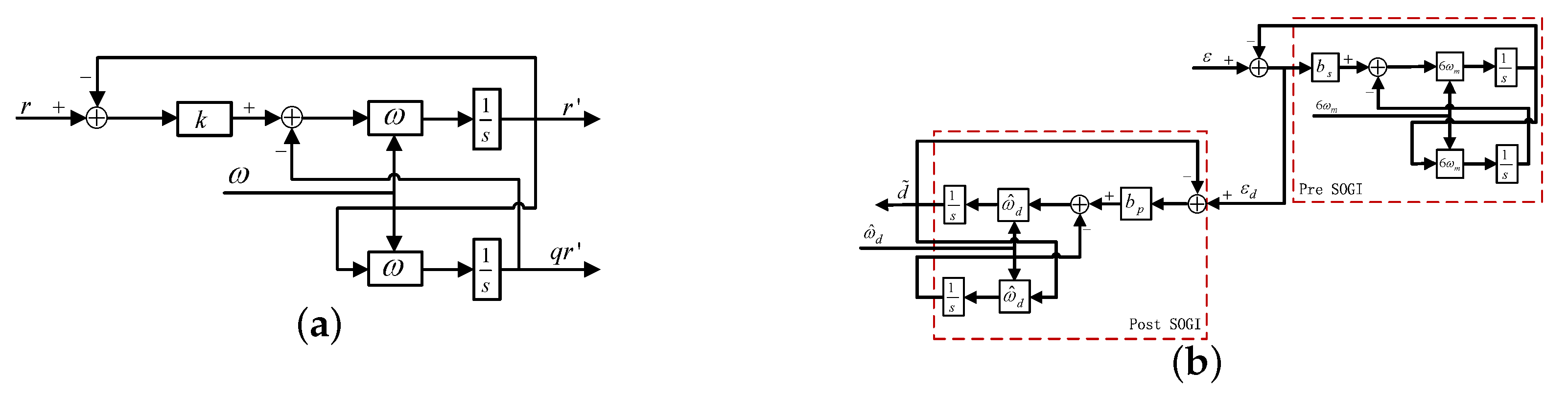
3.3. RRC Implementation
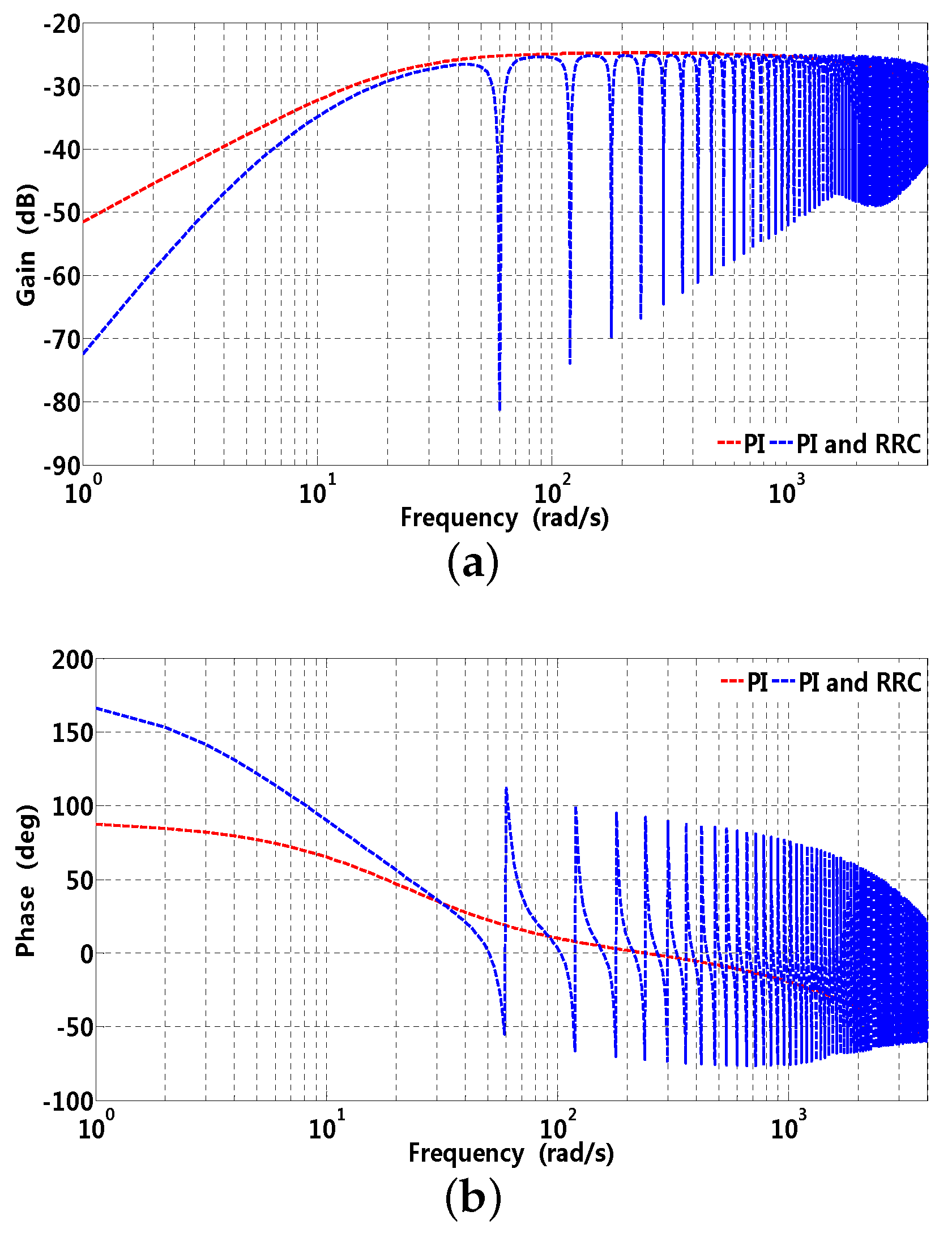
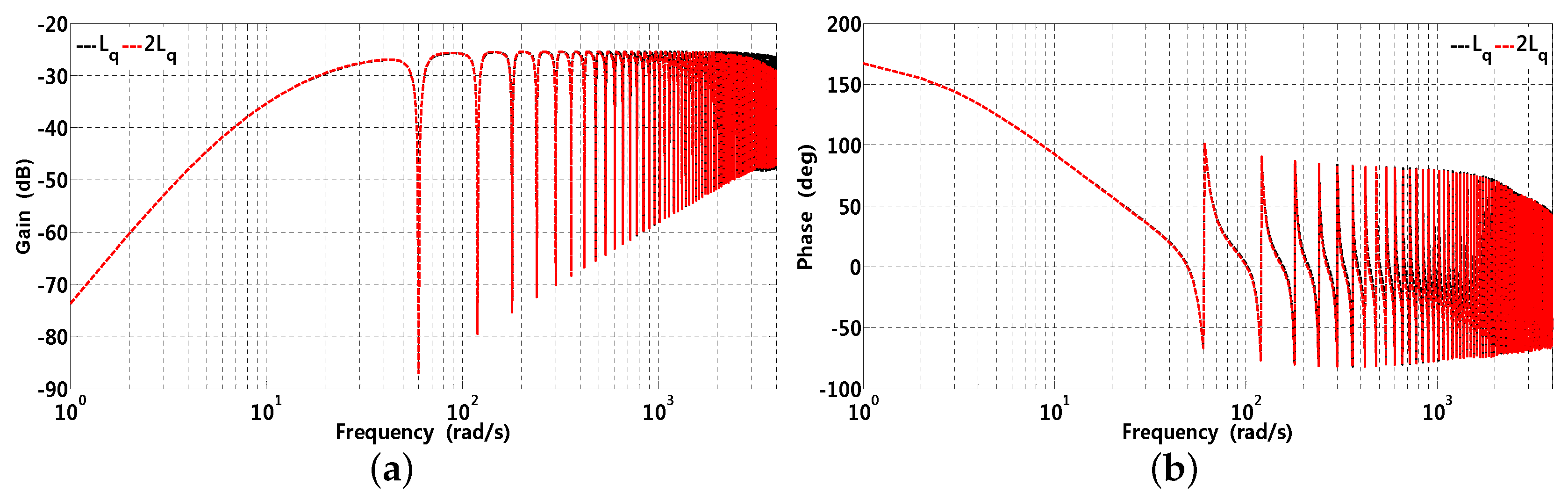
3.4. Robustness Analysis
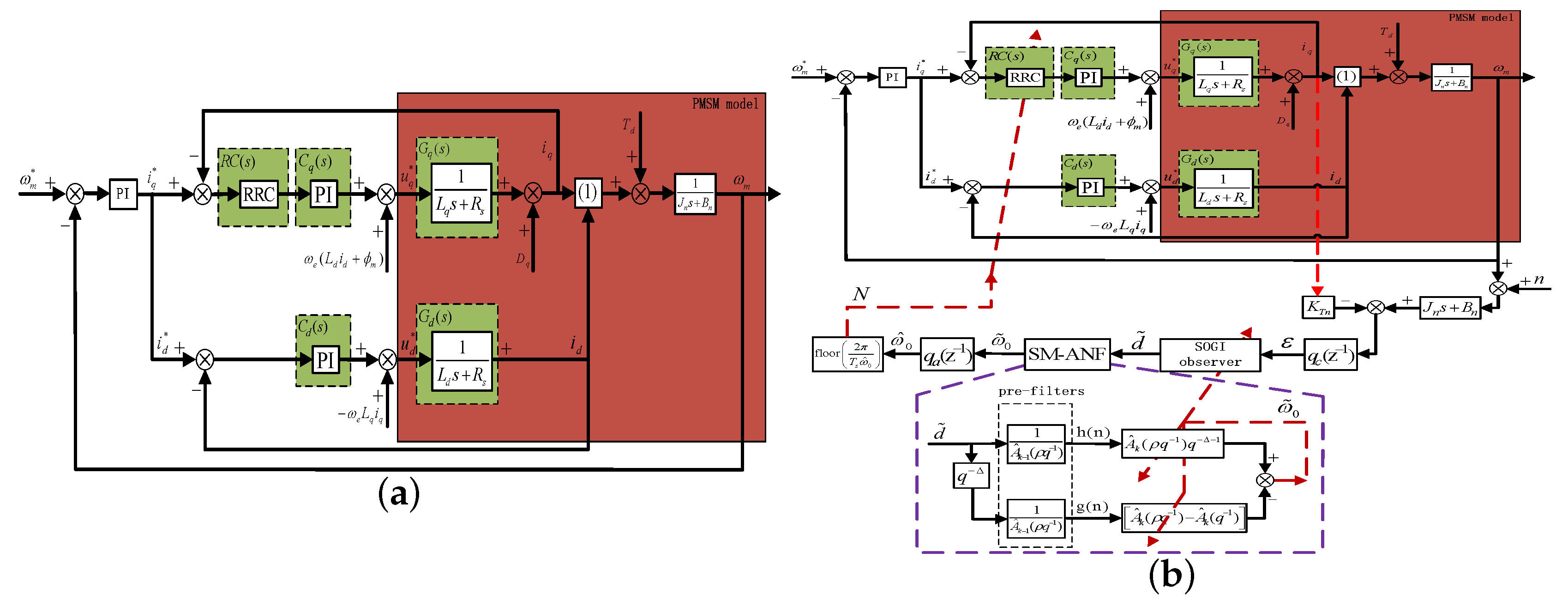
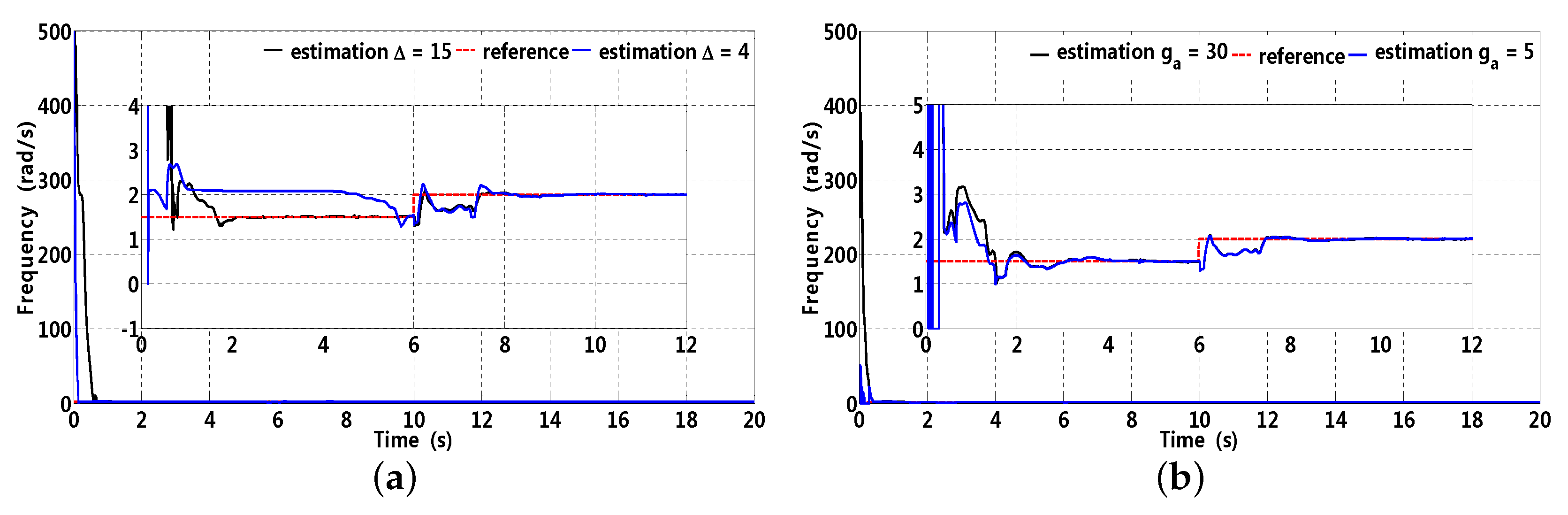
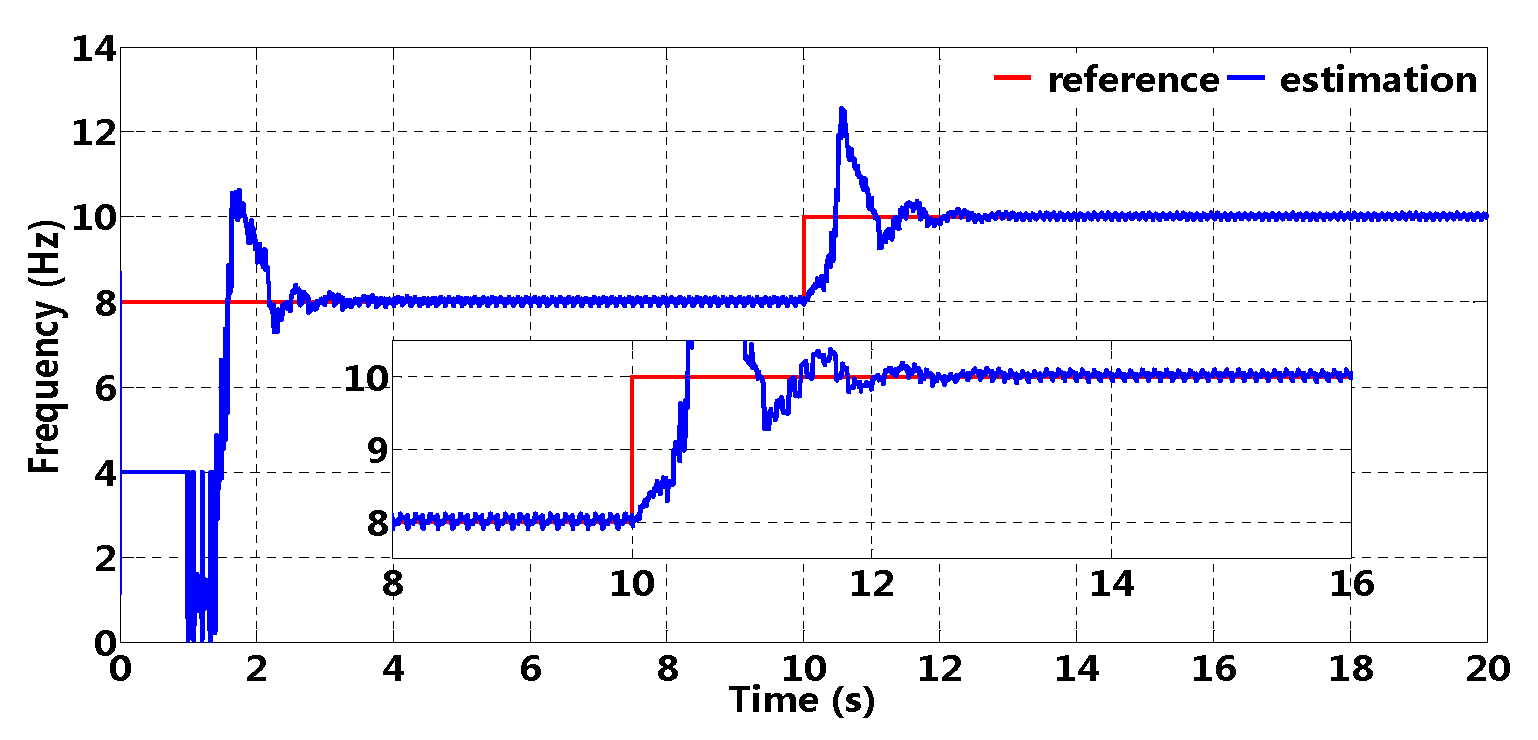
4. Fundamental Wave Frequency Estimation
4.1. SOGI Observer
4.2. SM-ANF Method
5. Simulations and Experiments
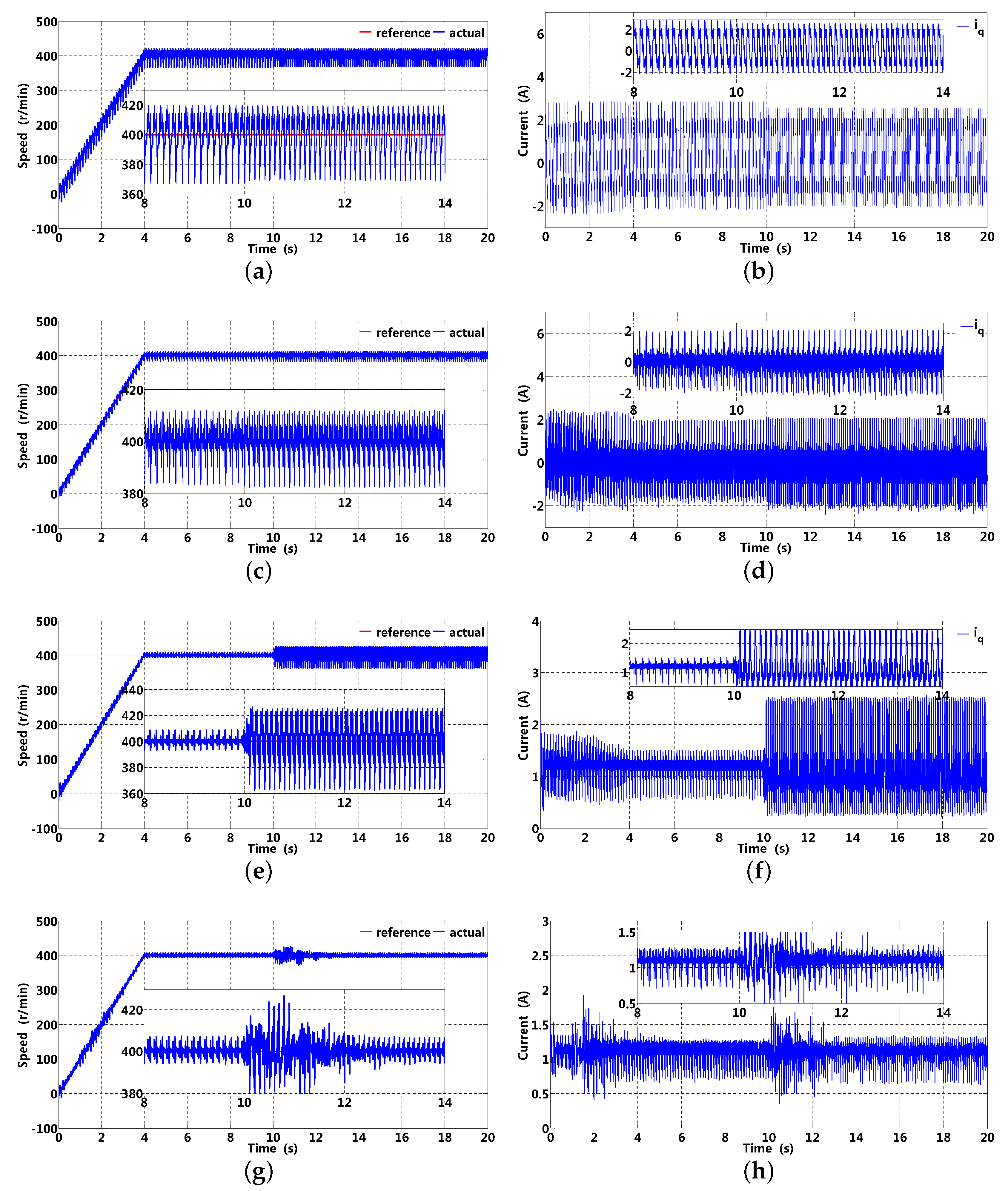
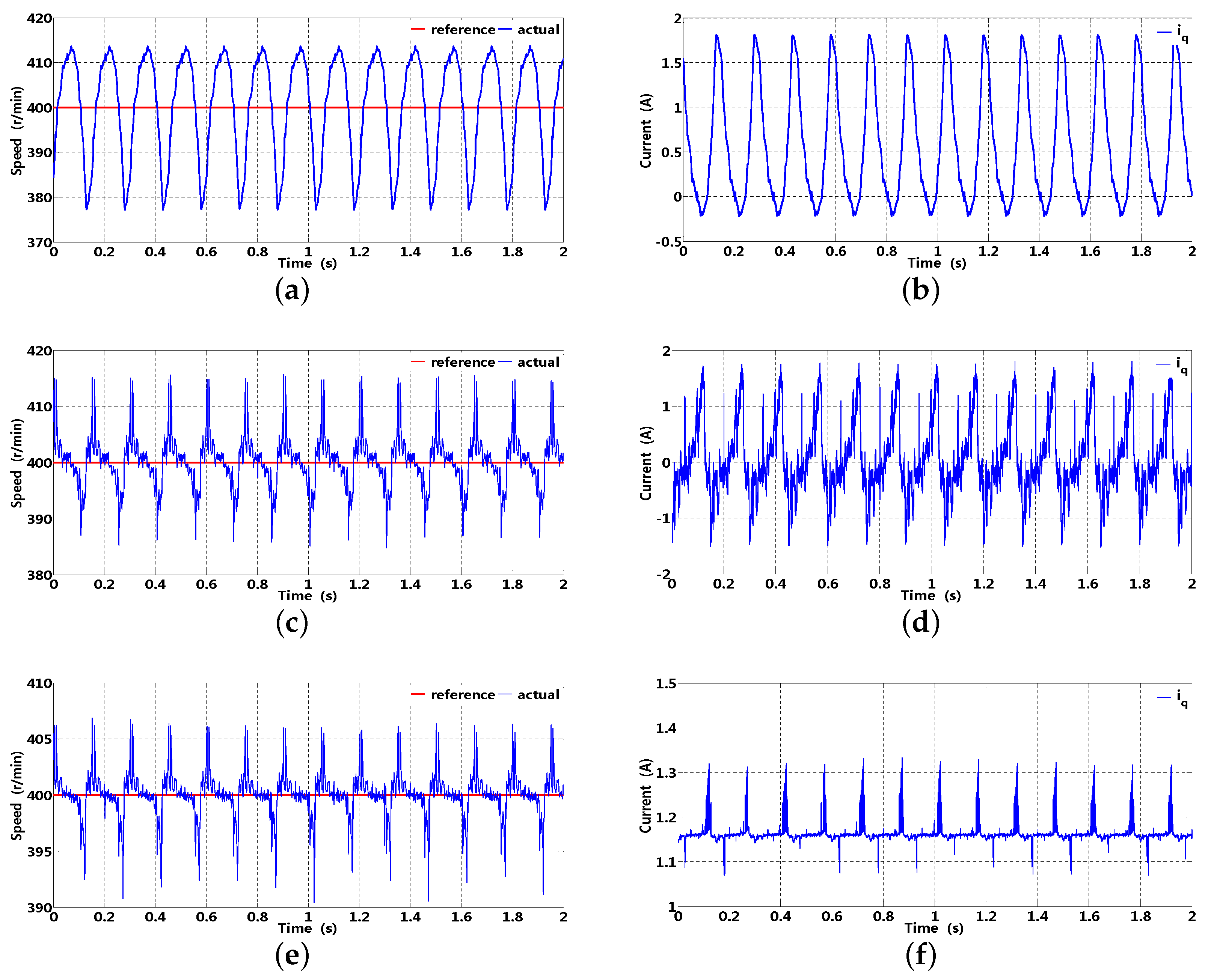
5.1. Simulation Analysis
5.2. Experiment Setup
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Zhang, G.Z. A Speed Fluctuation Method for Air Conditioner Compressor Based on Fourier Transformation. China Appl. 2013, S1, 491–496. [Google Scholar]
- Huang, H.; Ma, Y.J.; Zhang, L.Y.; Mi, X.T. Method to Reduce the Speed Ripple of Single Rotator Compressor of Air Conditioner in Low Frequency. Electr. Mach. Control 2011, 15, 98–102. [Google Scholar]
- Chen, W.H.; Yang, J.; Guo, L.; Li, S. Disturbance Observer-Based Control and Related Methods: An Overview. IEEE Trans. Ind. Electron. 2016, 63, 1083–1095. [Google Scholar] [CrossRef]
- Yang, J.; Chen, W.H.; Li, S.H.; Guo, L.; Yan, Y. Disturbance/Uncertainty Estimation and Attenuation Techniques in PMSM Drives—A Survey. IEEE Trans. Ind. Electron. 2016, 64, 3273–3285. [Google Scholar] [CrossRef]
- Ohnishi, K.; Shibata, M.; Murakami, T. Motion control for advanced mechatronics. IEEE-ASME Trans. Mechatron. 2002, 1, 56–67. [Google Scholar] [CrossRef]
- Huang, Y.; Xue, W.C. Active disturbance rejection control: Methodology, application and theoretical analysis. ISA Trans. 2014, 53, 963–976. [Google Scholar] [CrossRef]
- Fei, Q.; Deng, Y.T.; Li, H.W.; Liu, J.; Shao, M. Speed Ripple Minimization of Permanent Magnet Synchronous Motor Based on Model Predictive and Iterative Learning Controls. IEEE Access 2019, 34, 31791–31800. [Google Scholar] [CrossRef]
- Qian, W.Z.; Panda, S.K.; Xu, J.X. Torque ripple minimization in PM synchronous motors using iterative learning control. IEEE Trans. Power Electron. 2004, 19, 272–279. [Google Scholar] [CrossRef]
- Kuang, Z.; Du, B.C.; Cui, S.M.; Chan, C.C. Speed Control of Load Torque Feedforward Compensation Based on Linear Active Disturbance Rejection for Five-Phase PMSM. IEEE Access 2019, 7, 159787–159796. [Google Scholar] [CrossRef]
- Lu, E.; Li, W.; Yang, X.F.; Liu, Y. Anti-disturbance speed control of low-speed high-torque PMSM based on second-order non-singular terminal sliding mode load observer. ISA Trans. 2018, 88, 142–152. [Google Scholar] [CrossRef]
- Xu, W.; Junejo, A.K.; Liu, Y.; Rabiul Islam, M. Improved Continuous Fast Terminal Sliding Mode Control with Extended State Observer for Speed Regulation of PMSM Drive System. IEEE Trans. Veh. Technol. 2019, 68, 10465–10476. [Google Scholar] [CrossRef]
- Deng, Y.T.; Wang, J.L.; Li, H.W.; Liu, J.; Tian, D. Adaptive sliding mode current control with sliding mode disturbance observer for PMSM drives. ISA Trans. 2018, 82, 113–126. [Google Scholar] [CrossRef] [PubMed]
- Hara, S.; Yamamoto, Y.; Omata, M.; Nakano, M. Repetitive control system: A new type servo system for periodic exogenous signals. IEEE Trans. Autom. Control 1988, 33, 659–668. [Google Scholar] [CrossRef]
- Francis, B.A.; Wonham, W.M. The internal model principle for linear multivariable regulators. Appl. Math. Optim. 1975, 2, 170–194. [Google Scholar] [CrossRef]
- Chen, X.; Tomizuka, M. New Repetitive Control With Improved Steady-State Performance and Accelerated Transient. IEEE Trans. Control Syst. Technol. 2014, 22, 664–675. [Google Scholar] [CrossRef]
- Pipeleers, G.; Demeulenaere, B.; De Schutter, J.; Swevers, J. Robust high-order repetitive control: Optimal performance trade-offs. Automatica 2008, 44, 2628–2634. [Google Scholar] [CrossRef]
- Weiss, G.; Hafele, M. Repetitive control of MIMO systems using H∞ design. Automatic 1999, 35, 1185–1199. [Google Scholar] [CrossRef]
- Jiang, S.; Cao, D.; Peng, F.Z.; Li, Y.; Liu, J. Low THD, fast transient, and cost-effective synchronous-frame repetitive controller for three-phase UPS inverters. IEEE Trans. Power Electron. 2012, 27, 2819–2826. [Google Scholar] [CrossRef]
- Zou, Z.X.; Zhou, K.L.; Wang, Z.; Cheng, M. Frequency-Adaptive Fractional-Order Repetitive Control of Shunt Active Power Filters. IEEE Trans. Ind. Electron. 2015, 62, 1659–1668. [Google Scholar] [CrossRef]
- Lu, Y.S.; Lin, S.M.; Hauschild, M.; Hirzinger, G. A torque-ripple compensation scheme for harmonic drive systems. Electr. Eng. 2013, 95, 357–365. [Google Scholar] [CrossRef]
- Tang, Z.Y.; Akin, B. Suppression of Dead-Time Distortion Through Revised Repetitive Controller in PMSM Drives. IEEE Trans. Energy Convers. 2017, 32, 918–930. [Google Scholar] [CrossRef]
- Mattavelli, P.; Tubiana, L.; Zigliotto, M. Torque-ripple reduction in PM synchronous motor drives using repetitive current control. IEEE Trans. Power Electron. 2005, 20, 1423–1431. [Google Scholar] [CrossRef]
- Zhang, B.; Zhou, K.L.; Wang, Y.G.; Wang, D. Performance improvement of repetitive controlled PWM inverters: A phase-lead compensation solution. Int. J. Circuit Theory Appl. 2010, 38, 453–469. [Google Scholar] [CrossRef]
- Cui, P.L.; Li, S.; Zhao, G.Z.; Peng, C. Suppression of Harmonic Current in Active-Passive Magnetically Suspended CMG Using Improved Repetitive Controller. IEEE-ASME Trans. Mechatron. 2016, 21, 2132–2141. [Google Scholar] [CrossRef]
- Cao, Z.W.; Ledwich, G.F. Adaptive repetitive control to track variable periodic signals with fixed sampling rate. IEEE-ASME Trans. Mechatron. 2002, 7, 378–384. [Google Scholar]
- Weiss, G.; Zhong, Q.C.; Green, T.C.; Liang, J. H∞ repetitive control of DC-AC converters in microgrids. IEEE Trans. Power Electron. 2004, 19, 219–230. [Google Scholar] [CrossRef]
- Hornik, T.; Zhong, Q.C. H∞ repetitive voltage control of gridconnected inverters with a frequency adaptive mechanism. IET Power Electron. 2010, 3, 925–935. [Google Scholar] [CrossRef]
- Chen, D.; Zhang, J.M.; Qian, Z.M. An Improved Repetitive Control Scheme for Grid-Connected Inverter With Frequency-Adaptive Capability. IEEE Trans. Ind. Electron. 2013, 60, 814–823. [Google Scholar] [CrossRef]
- Chen, D.; Zhang, J.M.; Qian, Z.M. Research on fast transient and 6n ± 1 harmonics suppressing repetitive control scheme for three-phase grid-connected inverters. IET Power Electron. 2013, 6, 601–610. [Google Scholar] [CrossRef]
- Marino, R.; Tomei, P. Adaptive notch filters are local adaptive observers. Int. J. Adapt. Control Signal Process. 2015, 30, 128–146. [Google Scholar] [CrossRef]
- Li, G. A stable and efficient adaptive notch filter for direct frequency estimation. IEEE Trans. Signal Process. 1997, 45, 2001–2009. [Google Scholar]
- Cheng, M.H.; Tsai, J.L. A new IIR adaptive notch filter. Signal Process. 2006, 86, 1648–1655. [Google Scholar] [CrossRef]
- Sariyildiz, E.; Ohnishi, K. Stability and Robustness of Disturbance-Observer-Based Motion Control Systems. IEEE Trans. Ind. Electron. 2014, 62, 414–422. [Google Scholar] [CrossRef]
- Muramatsu, H.; Katsura, S. Adaptive Periodic-Disturbance Observer for Periodic-Disturbance Suppression. IEEE Trans. Ind. Inform. 2018, 14, 4446–4456. [Google Scholar] [CrossRef]
- William, A.B.; Taylor, F.J. Electronic Filter Design Handbook; McGraw-Hill: New York, NY, USA, 2006; pp. 566–570. [Google Scholar]
- Saeid, J.; Ioannou, P.A.; Ben, I.; Wang, Y. Robustness and Performance of Adaptive Suppression of Unknown Periodic Disturbances. IEEE Trans. Autom. Control 2015, 60, 2166–2171. [Google Scholar]
- Wu, X.H.; Panda, S.K.; Xu, J.X. Design of a Plug-In Repetitive Control Scheme for Eliminating Supply-Side Current Harmonics of Three-Phase PWM Boost Rectifiers Under Generalized Supply Voltage Conditions. IEEE Trans. Power Electron. 2010, 25, 1800–1810. [Google Scholar] [CrossRef]
- Sayed, A.H. Adaptive Filters; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2008; pp. 187–193. [Google Scholar]
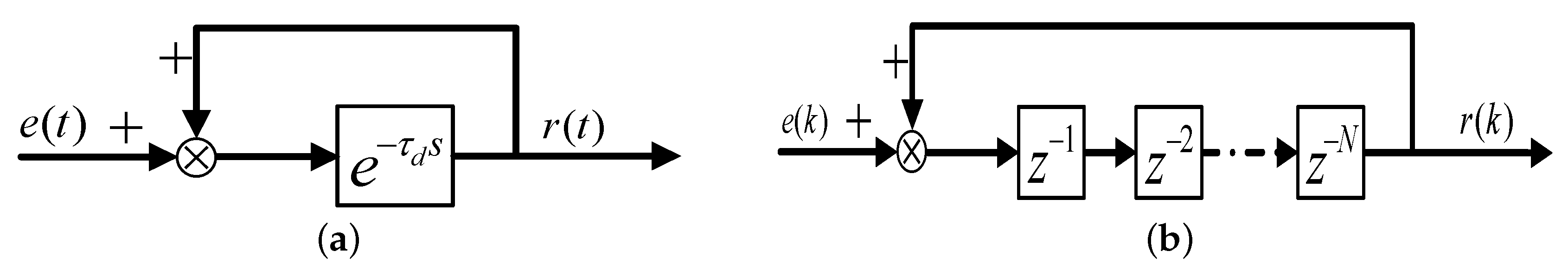





| Input: m, , , , , , , , , |
| Initialization: , , , |
| , , |
| for |
| Main loop: |
| Adaptive algorithm: |
| Fundamental frequency: |
| Parameter | Symbol | Value |
|---|---|---|
| Sampling time | 0.1 | |
| Cut-off frequency of general DOB | 800 | |
| Cut-off frequency of | 800 | |
| Cut-off frequency of | 10 | |
| Delay parameter | 15 | |
| Parameter of post SOGI | 0.1 | |
| Parameter of pre SOGI | 0.1 | |
| S-G filter order | p | 3 |
| Design parameter | D | 2.6 |
| Gain of RRC | 0.6 | |
| Step size of NLMS | 0.3 | |
| Stator winding resistance | 2.875 | |
| -axis inductance | 0.0085 | |
| -axis inductance | 0.0119 | |
| Rotor permanent magnetic flux | 0.175 | |
| Moment of inertia | 0.003 | |
| Pole pairs | 3 | |
| Electro-magnetic torque constant | 0.525 | |
| DC bus voltage | 310 | |
| Rated current | I | 7 A |
| Parameter | Symbol | Value |
|---|---|---|
| Pole pairs | 3 | |
| Stator winding resistance | R | 1.47 |
| -axis inductance | 6.87 mH | |
| -axis inductance | 9.31 mH | |
| Rotor permanent magnetic flux | 345 mWb | |
| Back electromotive force (BEMF) constant | 39.2 V/krpm | |
| Electro-magnetic torque constant | 0.46 Nm/A | |
| Moment of inertia | J | 310 Kg·mm |
| Rated current | 5.2 A | |
| Rated frequency | 60 Hz | |
| Cut-off frequency of general DOB | 800 | |
| Cut-off frequency of | 800 | |
| Cut-off frequency of | 10 | |
| Delay parameter | 15 | |
| Parameter of post SOGI | 0.1 | |
| Parameter of pre SOGI | 0.1 | |
| S–G filter order | p | 3 |
| Step size of NLMS | 0.3 | |
| Design parameter | D | 2.6 |
| Gain of RRC | 0.6 | |
| Bandwidth of ANF | , , | 0.8, 0.95, 0.99 |
| Forgetting factor | , , | 0.8, 0.95, 0.99 |
| Method | 20 Hz | 25 Hz |
|---|---|---|
| PI | 2.92% | 1.83% |
| 11.7 r/min | 9.2 r/min | |
| DOB | 1.35% | 0.94% |
| 5.4 r/min | 4.7 r/min | |
| ARRC | 0.46% | 0.34% |
| 1.8 r/min | 1.7 r/min |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, F.; Zhang, X.; Li, Z.; Wen, X.; You, L. Speed Fluctuation Suppression for the Inverter Compressor Based on the Adaptive Revised Repetitive Controller. Energies 2020, 13, 6342. https://doi.org/10.3390/en13236342
Meng F, Zhang X, Li Z, Wen X, You L. Speed Fluctuation Suppression for the Inverter Compressor Based on the Adaptive Revised Repetitive Controller. Energies. 2020; 13(23):6342. https://doi.org/10.3390/en13236342
Chicago/Turabian StyleMeng, Fankun, Xiaoning Zhang, Zhengguo Li, Xiaoqin Wen, and Linru You. 2020. "Speed Fluctuation Suppression for the Inverter Compressor Based on the Adaptive Revised Repetitive Controller" Energies 13, no. 23: 6342. https://doi.org/10.3390/en13236342
APA StyleMeng, F., Zhang, X., Li, Z., Wen, X., & You, L. (2020). Speed Fluctuation Suppression for the Inverter Compressor Based on the Adaptive Revised Repetitive Controller. Energies, 13(23), 6342. https://doi.org/10.3390/en13236342





