As discussed in the previous sections, a first challenge to address in OWFs is the inclusion of a unit able to BS. There is a growing research interest in GFM control strategies as, when applied to OWFs, this will allow island operation and BS service integration. GFM converters are defined as converters behaving as ideal voltage sources having voltage amplitude v* and angular frequency ω* controlled in closed loop. As presented in [
31], this could be suited to different inverter-based resources in the OWF, such as HVDC-connected OWFs, BESSs, and WTs. Only type 3 and type 4 WTs are considered due to their converter interface, where the GFM control can be applied. The motivation behind researching GFM converters in this area is to avoid the deployment of conventional units, i.e., SGs, which will emit CO
2 due to the use of fuels such as diesel or biomass. One of the first classifications of GFM control has been presented in [
33], which focuses on control structures for AC microgrids. In order to understand the most suitable option of a GFM control, an overview of different strategies is presented.
A GFM converter synchronization system can work on both island and grid-connected operations. Besides the purpose of BS and island operation, GFM control may also improve the stable operation of the converter independently of short-circuit level of the grid [
14]. Furthermore, GFM control can provide an inherent frequency response to system events. GFM converters can properly ride through cases of system split thanks to their capability to withstand a high rate of change of frequency (RoCoF) [
14]. Additionally, advanced control loops can be implemented on the basic of GFM structures in order to provide higher performance such as inertia emulation and short-circuit infeed [
14]. In this way, GFM converters may satisfy the BS requirements, as illustrated in [
31]; further research into this is necessary. Several different control topologies have been presented in the state-of-the-art of GFM converters. In the following, a review of the most relevant research with respect to popularity and applicability to OWF BS is presented.
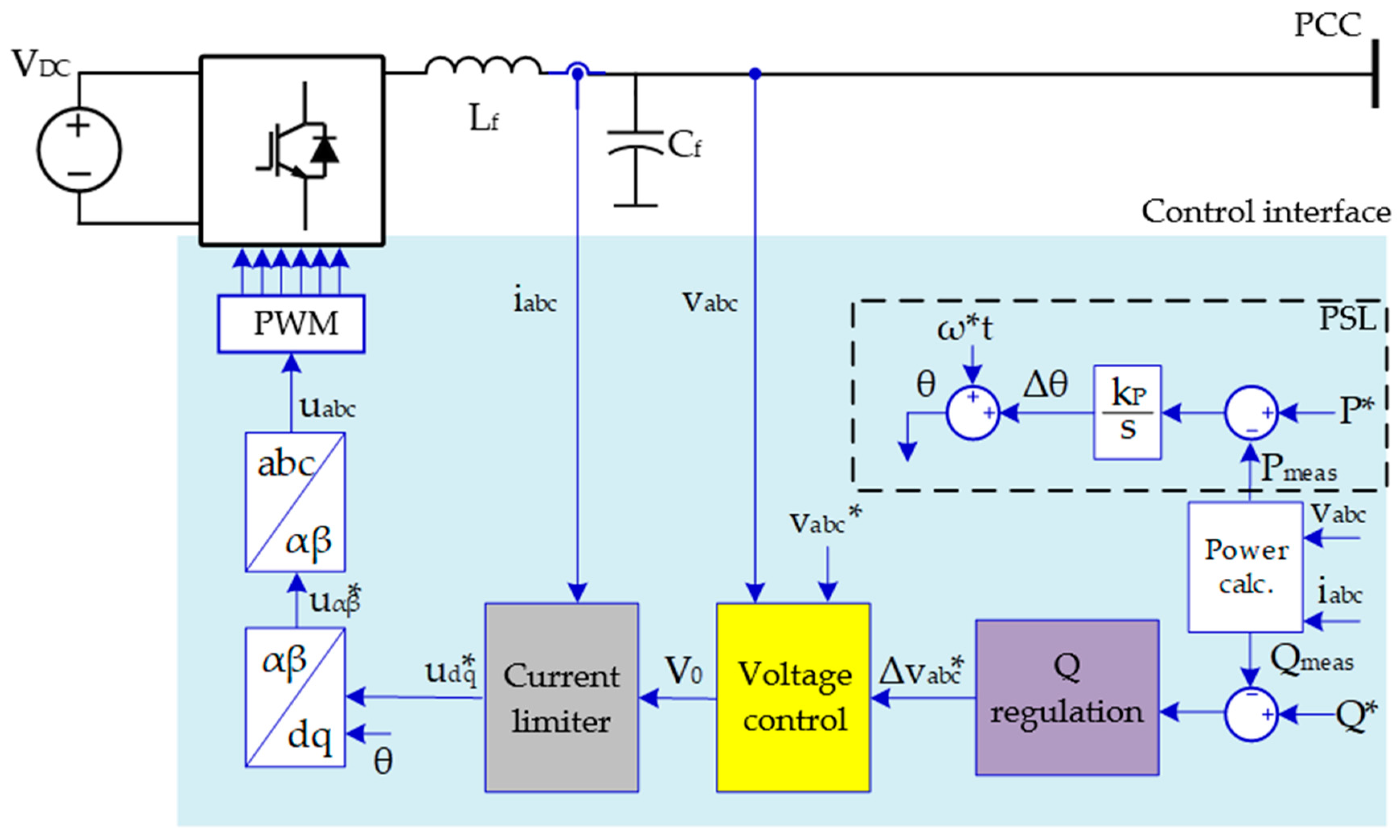
4.1. Basic Grid-Forming Control Method
The basic control structure of GFM control consists of two cascaded loops, as shown in
Figure 9 [
33].
Figure 9 shows an example where the controller applies two synchronous controllers in the dq frame. The control system inputs are the amplitude v* and the angular frequency ω* of the voltage that the converter forms at the point of common coupling (PCC). The reference values v* and ω* need to be calculated by a higher-level controller or via lookup tables [
33]. Voltage control is performed in the external loop, where the error between the measured voltage magnitude v
abc and the reference voltage v* is the input to the current controller, for which the output establishes the current reference i*. Subsequentially, the reference signal u
abc, is fed to the pulse-width modulation (PWM) block. Filters are commonly used, here, represented as a series filter inductor L
f and a shunt filter capacitor C
f. Usually, in industrial applications, these power converters are fed by stable DC voltage source V
DC, for example, driven by a BESS, fuel cells, or another source. In the following section, the source of V
DC is not discussed.
GFM converters can apply both the dq synchronous as well as the αβ stationary reference frame [
35,
36]. In
Figure 9, the transformation in dq frame is shown as an example.
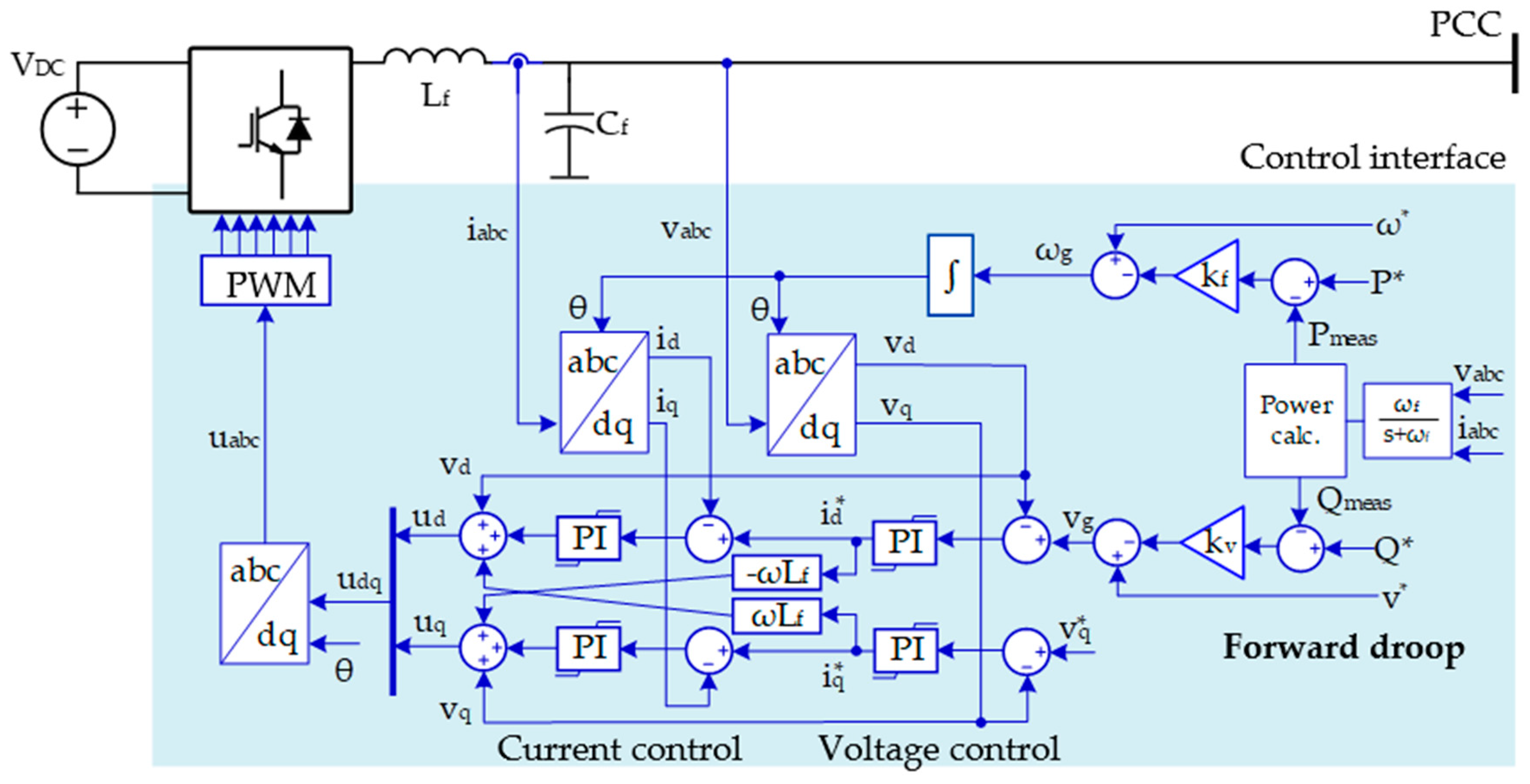
4.2. Droop-Based Control
To regulate the active P and reactive power Q provided to the grid, droop control is used for GFM strategies [
37]. These are also defined as voltage-source grid-supporting converters [
33]. The implementation shown in
Figure 10 is taken from [
33].
Droop control mimics the inherent regulation of SGs in grid-connection mode. Droop-based GFM control can be implemented both in islanded and grid-connected operations without any other type of GFM converter connected in the system [
33].
Droop control for the GFM strategy emerged as an alternative to different current sharing strategies for small-rated parallel converters, such as average-load sharing, centralised controllers, or master–slave [
38]. These types of strategies exploit high-bandwidth communication channels for control coordination. Communication requirements can be tricky for large systems such as OWFs in a situation such as BS. During BS, many devices in the system might be out of service. Therefore, fast and reliable communication might be challenging. Droop control is thus used to achieve power management in a decentralized manner. This implementation uses the forward droop equations as seen:
where k
v and k
f are the voltage and frequency droop coefficients, v
g and ω
g are the actual grid voltage grid angular frequency, and v* and ω* are the reference grid voltage and grid angular frequency, respectively. The parameters P* and Q* are the reference active and reactive power, while P
meas and Q
meas are the measured active and reactive power, respectively. A low-pass filter is applied to the measured active and reactive power before they are used in the control to avoid oscillations and disturbances in the measurements and to thus stabilise the control loops. The 1st-order low-pass filter with cutoff angular frequency ω
f is implemented before the power calculation stage.
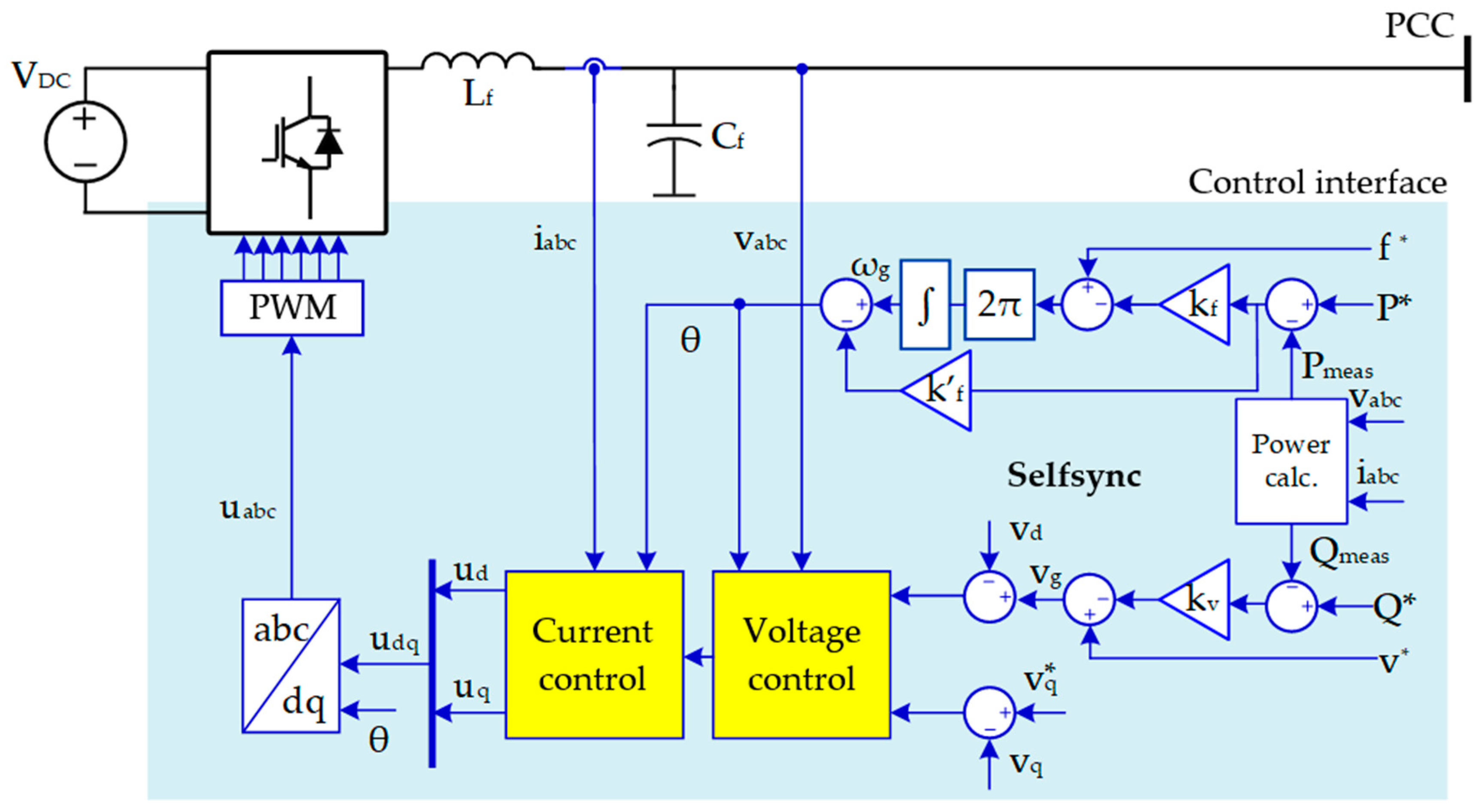
An alternative to this basic strategy is introduced having an angle feedforward proportional to the active power flow. This is shown in
Figure 11.
This was first proposed in [
39] and more recently in [
14] and is defined as Selfsync. In
Figure 5, the low-pass filter is not shown as considered included in the power calculation block. Furthermore, the voltage and current controllers are represented in single blocks and this style will also be adopted in the following figures.
This modification of the basic droop structure will improve the controller dynamics. The structure for V/Q droop remains untouched, while the P/f droop is a combination of the term k’
f (P* − P
meas), which is feed forward. According to the invention, active power oscillations between the inverters are avoided in this way [
28]. The output of the droop controls is then fed to a cascaded voltage and current controllers, as in previous implementations.
A disadvantage of droop structures is the fact that droop strategies can cause a steady-state error [
40]. This could be corrected by designing a supplementary control.
4.3. Virtual Synchronous Machine
With the intention of imitating the inertia provision by SGs, virtual synchronous machine (VSM) control has been introduced. This is a popular type of implementation which has been implemented in different applications and proven successful for inertia emulation [
41,
42]. Nevertheless, not all implementations of VSM are GFMs. The structures of VSM considered here are only GFMs for BS. There are several implementations of this type of control, and their main core is in the application of some form of the swing equation as shown in (3).
where ω is the angular frequency, T
m and T
e are the mechanical and electromagnetic torques respectively, k
d is the damping factor, and J is the moment of inertia, which will not influence the steady-state operation but has a strong impact on the dynamics. A large J implies more energy released or absorbed during the disturbance and a stronger ability for frequency support.
There are varying levels of model complexity, ranging from simple models which implement only the swing equation, to 9th-order models [
15]. High-order models can be directly implemented with the same parameters as a real SG. An advantage is the fact that these controller parameters can be tuned and updated according to a specific application by a software modification, as controllers do not have the same physical constraints as the SGs. However, as previously explained, power electronic converters may present other challenges.
Nevertheless, it has been seen that, mainly for high-order models, this kind of control might tend to be numerically instable [
43], the origin of which is not yet clear. In fact, it is not known whether this problem is intrinsic in the models or is merely due to numerical matters which can be solved by applying a proper integration method [
43].
In [
43], a classification of the main types of VSM implementations is made. These groups are distinct in current reference, voltage reference, and power reference as output reference from the VSM. The first two groups are GFM and will be presented further.
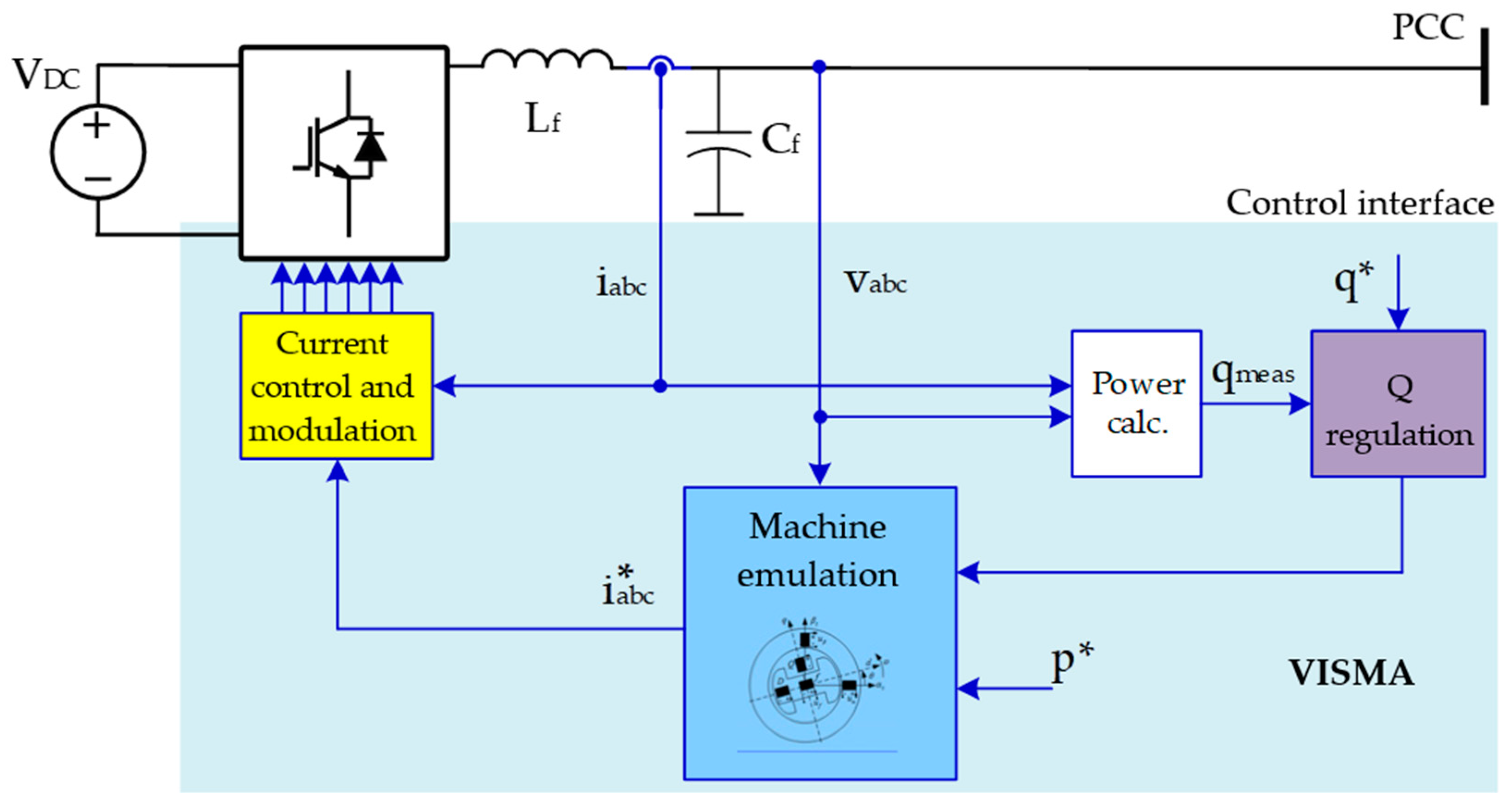
4.3.1. Current References from the SG Model
The current reference i
abc* can be the output of the full- or reduced-order SG model. As the measured voltage can be the input of a SG model to compute the resulting currents, this approach allows a rather simple configuration in high-order SG models. The first proposal of this type of VSM implementation is the VISMA [
44]. A simplified representation is shown in
Figure 12. To have the necessary performance, limitations and saturations can be embedded directly on the reference value.
4.3.2. Voltage References from the SG Model
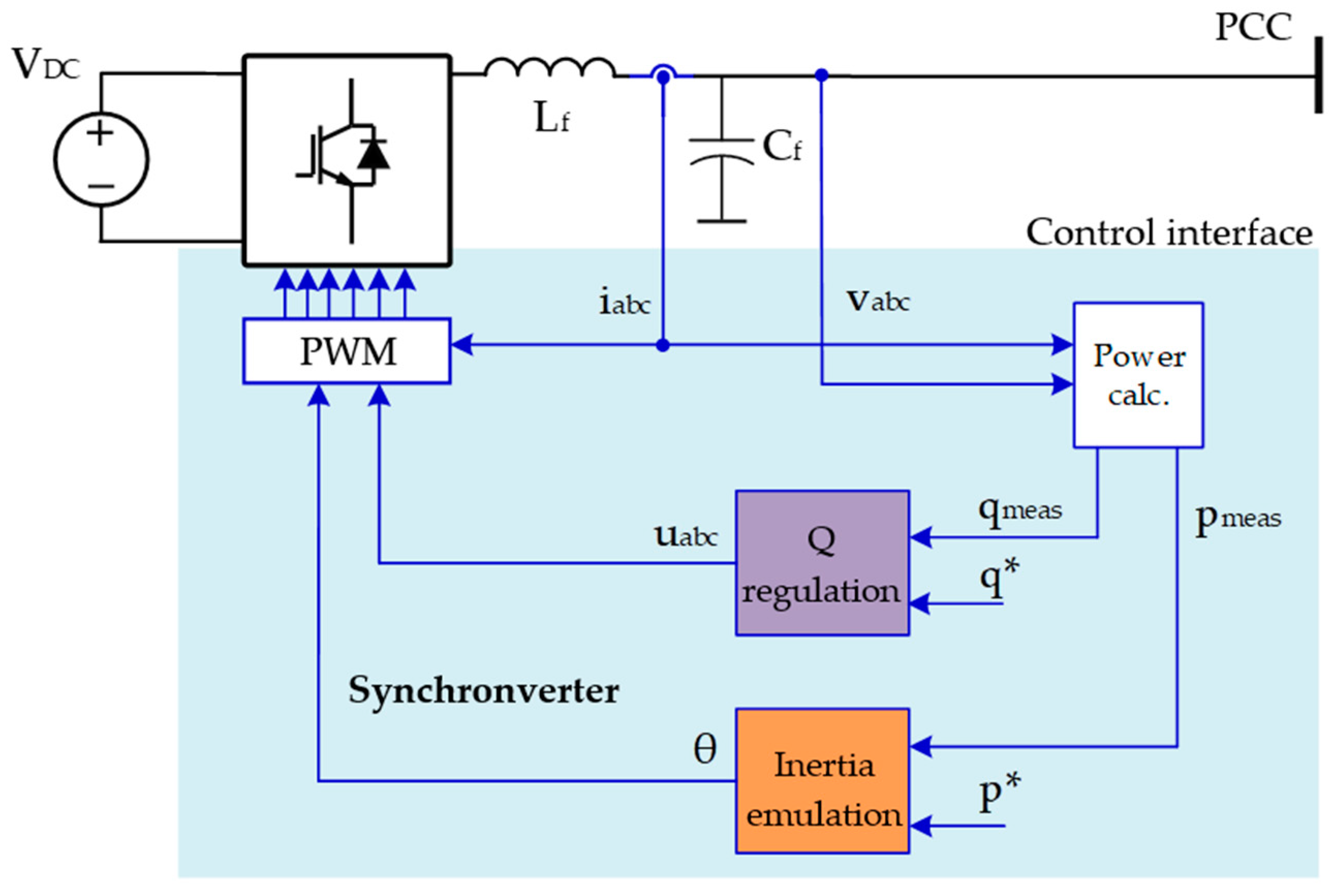
A different type of VSM implementation exploits a voltage reference output from the SG model. In [
45], these two VISMA concepts are compared. When applying reduced-order models, the active power depends both on the virtual inertia and the phase angle, which can be calculated from the swing equation. At the same time, the reactive power can be controlled separately. An example considers the 5th-order SG model of a round rotor machine which does not include damper windings for the rotor. As the voltage magnitude and phase angle are sent directly to the PWM block, this can be considered the most direct implementation of the VSM concept. The Synchronverter control discussed in [
46] and several other recent publications [
46,
47,
48,
49,
50,
51] apply this strategy, which is shown in
Figure 13.
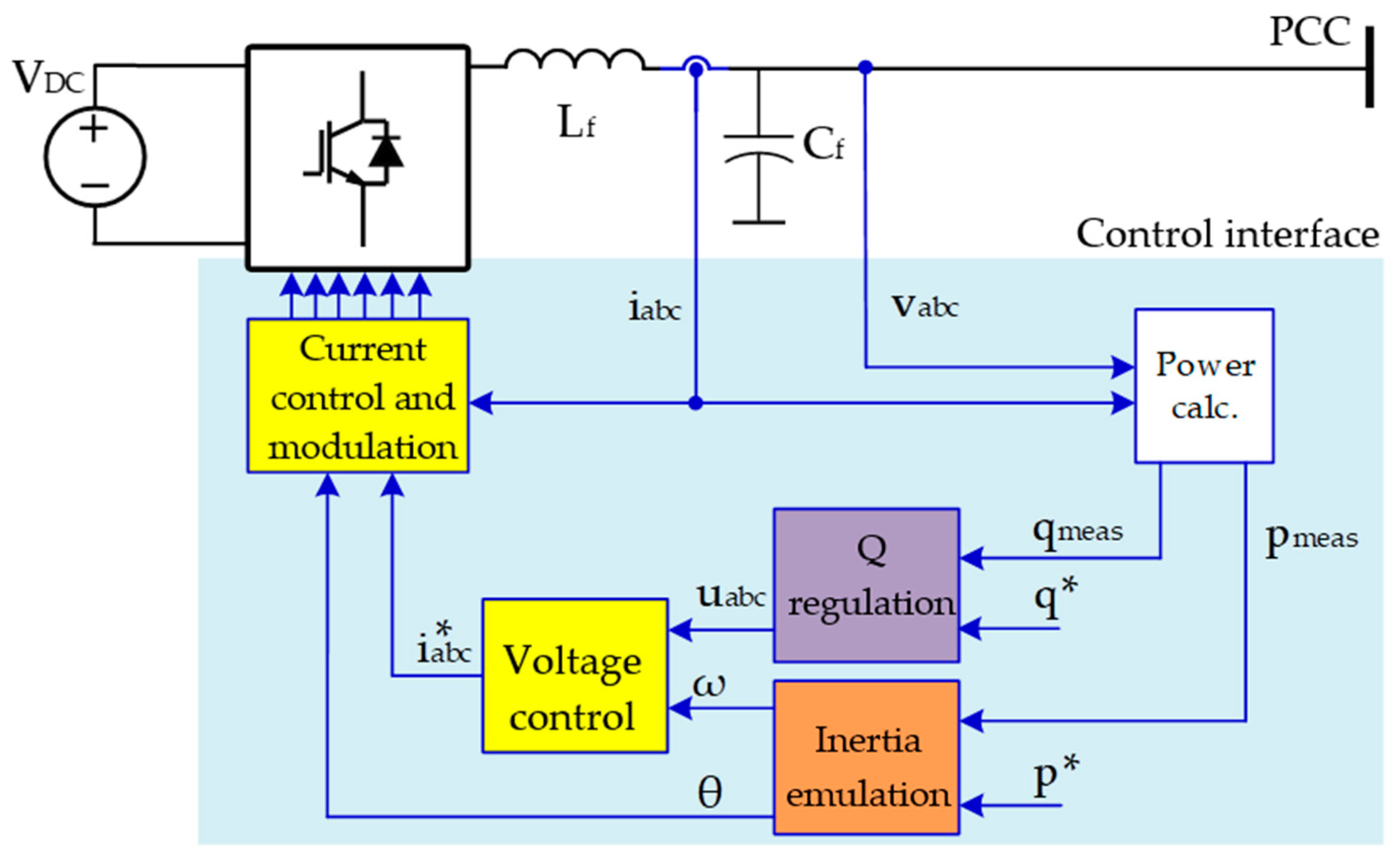
A disadvantage of this implementation is not having an explicit current signal in order to implement overcurrent protection. A similar approach with enhanced controllability is shown in
Figure 14. Higher flexibility for protection is obtained since both voltage and current can be kept within a defined range [
52,
53]. This is because a classical cascaded control is applied where voltage output is the reference for an external voltage loop with an internal current control. According to [
14], the optimal tuning of these controllers is necessary in this type of VSM control.
It should be noted that these control strategies of the VSM with voltage reference as an output of the SG model and droop-control show some similarity. In [
43], the equivalence between this implementation of VSM and droop control is demonstrated; droop gain k
P is the inverse of damping gain k
d in the swing equation. Furthermore, the 1st-order low-pass filter on the active power, as shown in
Figure 10, serves an analogous role as the emulated inertia. Consequentially, a droop controller can be tuned to replicate the SG small-signal characteristics.
An aspect common to all of SG emulation strategies is their short-circuit capability. In [
54], a virtual resistor is added to a VSM 3rd-order model to obtain an enhanced short-circuit capability. This implementation can be applied for the system to perform more similarly to a conventional BS unit. This may require further investigations, especially related to application to a power-electronic-dominated system such as OWFs.
4.4. Power-Synchronization Control
Another consolidated and popular GFM structure is the power synchronization control (PSC), shown in
Figure 15. The PSC control structure was initially presented in [
55] and refined in [
56]. It was developed originally for HVDC systems to cope especially with weak grids. In this approach, active power is used for synchronization as in the VSM. Nevertheless, the main difference is that the PSC loop (PSL) gets the phase angle directly by a single integration of the power difference. This differs from the swing equation in VSM, where the power difference drives the virtual rotor speed dynamics, which is then changed to an electrical angle by a double integration. Due to one less integrator, PSC has a higher stability margin. The angle θ is estimated and fed to the dq and αβ blocks in order to implement the transformation. On the other hand, the current at the fundamental frequency is not controlled, as the PSL contrasts it. Nevertheless, in power electronics, the current always needs to be limited to avoid component damage and a current limiter block is implemented, which receives a voltage signal and generates the u
dq* signal to be fed to the converter.
However, due to inherent steady-state droop, outer droops are needed for paralleling multiple GFM units. Moreover, neither virtual inertia nor damping are inherent in the design. Another disadvantage of PSC is represented by the fact that it needs a phase-locked loop (PLL)-based standard control as backup in case of grid-faults and initial phase synchronization.
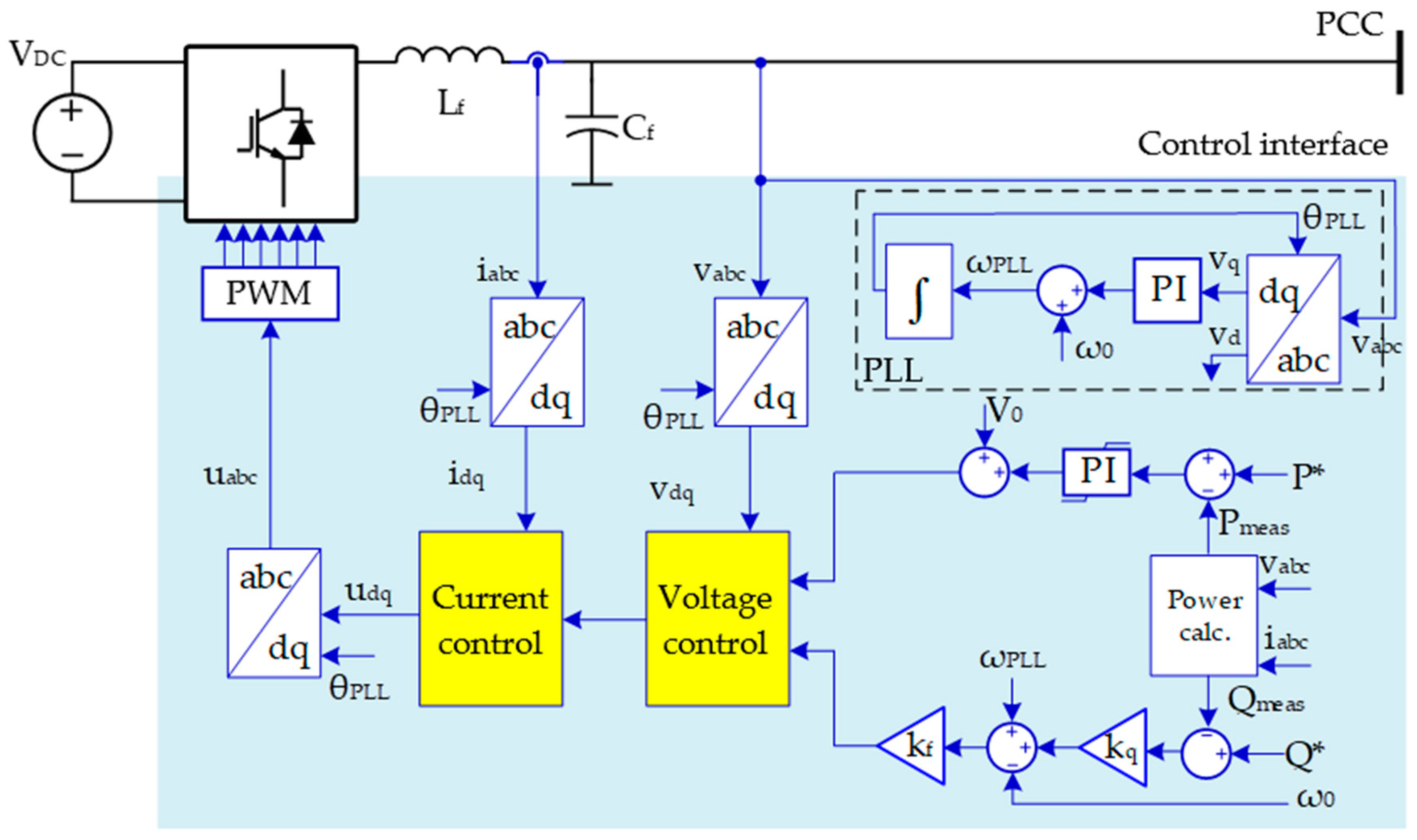
4.5. Distributed PLL
The distributed PLL (dPLL) control structure is based on [
57]. This was originally applied to GFM WTs and was developed for diode-rectifier (DR)-based HVDC-connected OWFs. Based on the voltage reference signal coming from the offshore converter, this type of controller generates the d-axis voltage reference from the active power flow from offshore. At the same time, based on the reactive power required by the DR unit, the frequency is regulated by a Q droop. Thus, dPLL can be said to be a voltage source controlled with Q-f, P-V coupling. This is shown in
Figure 16.
This approach exploits a PLL but does not set the q-axis voltage reference to 0 as usually done. Instead, a frequency control loop is embedded in the q-axis to use the output of the Q-f droop-controller. In this way, frequency deviations can be expressed by the output of the PLL.
In [
57], it is used to successfully provide GFM WT start-up in sequence and synchronization of offline WTs during connection automatically via the plug-and-play capability to implement frequency controllability. This is used to supply the reactive power required to energise the passive components of the system and to ramp up the voltage. In this way, active power can finally be delivered to an onshore grid. Nevertheless, the study includes only the application of dPLL to WTs in a DR-HVDC system in order to energise the offshore grid. Thus, it implements the self-start of the WT system and its synchronization to an energised onshore AC power system. Therefore, the energisation of an export cable and an onshore converter has not been investigated, which is an important operation for a BS unit.
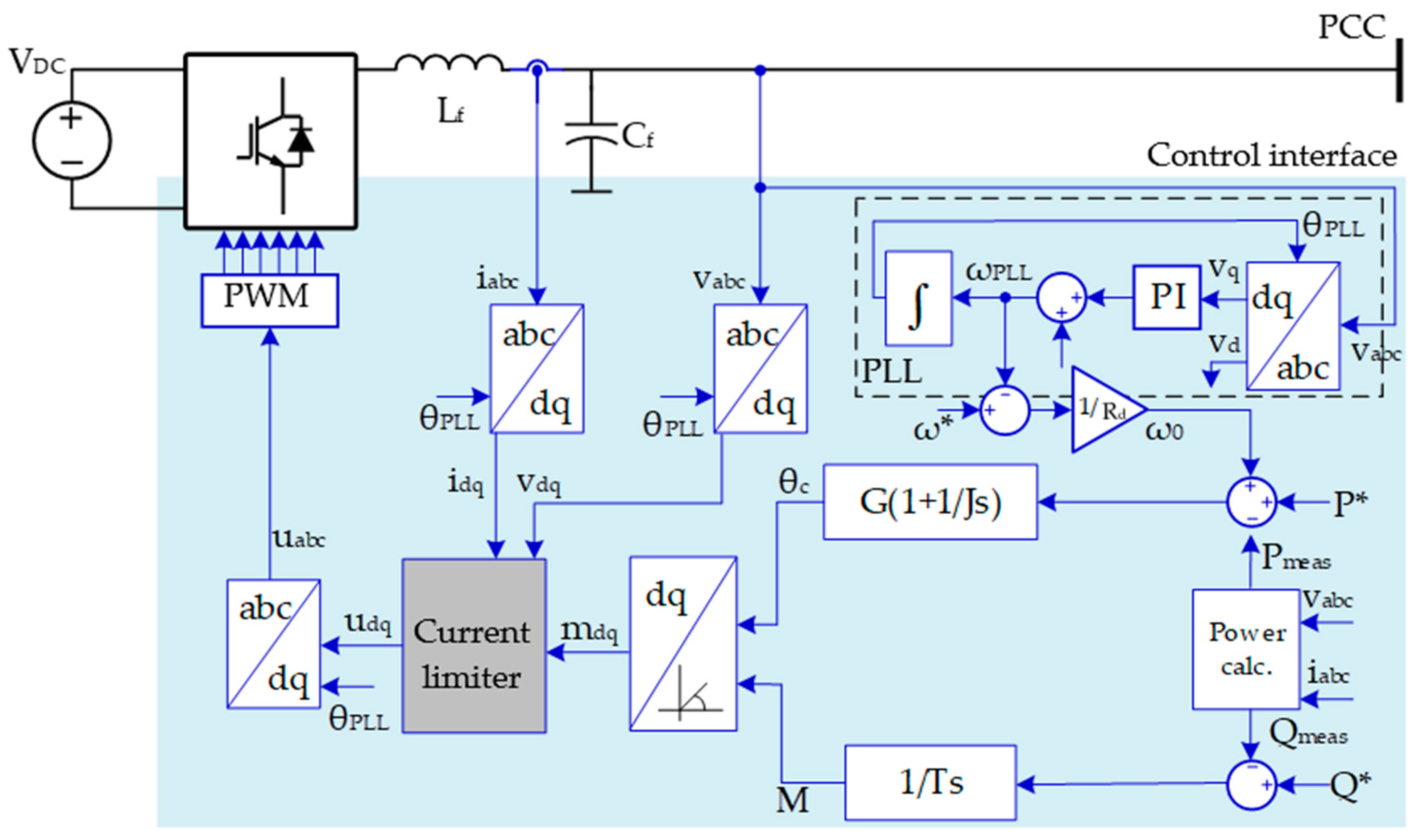
4.6. Direct Power Control
Direct power control (DPC) represents another relevant control for OWF BS. DPC was introduced by [
58], in which instantaneous P and Q are controlled without requiring AC voltage sensors, PLL, or an inner current controller via hysteresis comparators on the power errors and a look-up table to select the best converter switching time. Since then, it has undergone many enhancements to deliver improved performance like using space vector modulation for constant switching frequency, employing sliding mode control for robustness, modelling predictive control for the multivariable case, and employing grid voltage-modulated DPC for good transient-response and steady-state performance in nonlinear systems. This implementation of DPC is a type of GFL control as, even though a PLL is not used, estimation of the output voltage gives the reference for the phase angle. A GFM variation of the DPC can be found in [
56]. This is relevant as its main functionality is the ability to operate with very low short-circuit ratio (SCR), i.e., below 1.5. The control scheme is given in
Figure 17, which applies a PLL that synchronises the dq frame with the grid voltage vector.
The PLL continuously detects angle deviations in the case of islanding, where the converter is feeding passive loads. Two outer loops can be identified; the former is the reactive power control with integrator time constant T, linked to the internal voltage magnitude M of the converter. The latter is the active power loop in charge of controlling the internal voltage angle θ
c. The angle θ
c is related to the PLL angle θ
PLL as it is the phase angle shift between these two. A PI controller with gain G and time constant J is implemented in DPC. Moreover, the measured angular frequency of the PLL ω
PLL is used in order to apply a frequency droop control that adapts the active-power set point for given frequency variations. The scheme in [
59] has the main characteristics of a variation of DPC shown in [
60], which is applied to WTs.
4.7. Overview of Different Grid-Forming Strategies
Different types of GFM control structures have been explained. In the state-of-the-art literature, these have been applied to different devices, e.g., HVDC transmission, BESSs, WTs, and photovoltaic (PV) units, and with different purposes, such as weak grid applications and inertia emulation. Nevertheless, these all show potential to be applied to OWF for BS. In this section, a further overview is given to differentiate the controller in terms of comparison and results found in the state-of-the-art literature.
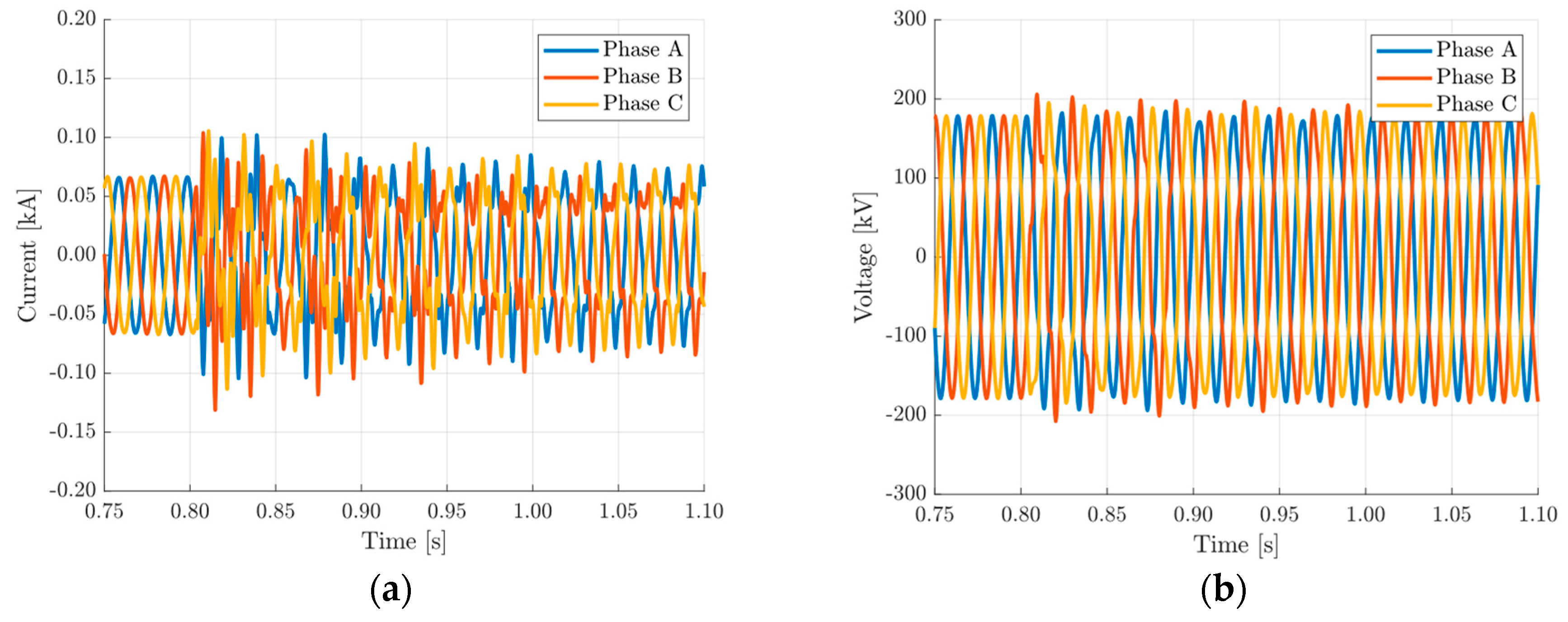
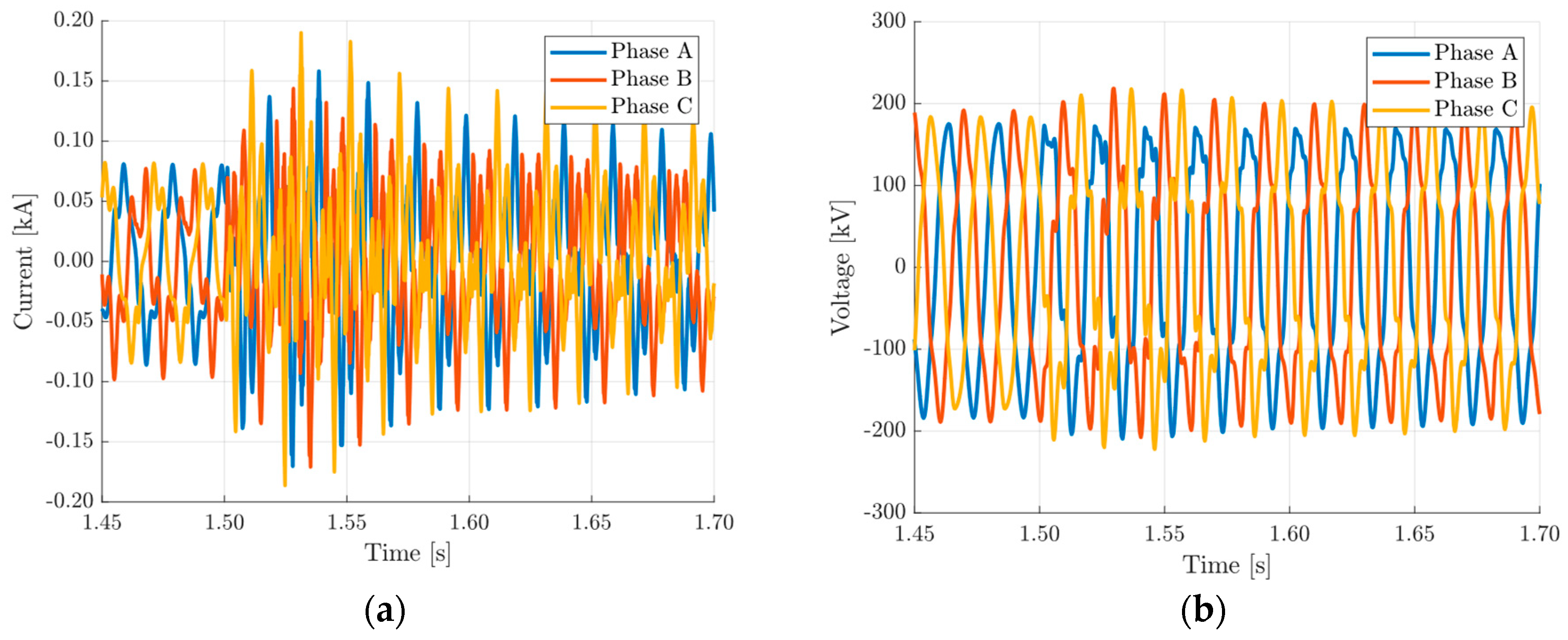
As stated, GFM control can be suitable to different devices in OWFs. The basic droop control structure has been used in [
61], where a 400-MW OWF consisting of 50 WTs with GFM capabilities is used for BS of the onshore grid. In this study, practical challenges in the energisation transient have been studied via electromagnetic transient (EMT) simulations. These challenges arise from the inrush phenomena due to the large reactive loads of the OWF export system. The simulation results showed that the OWF was able to BS and energise the onshore network and block loads also in the presence of wind fluctuations. The strategy shows promising results; however, considerable distortions in the voltage and current waveforms are seen during the BS performance. Therefore, more studies are relevant to reduce the magnitude of the inrush as well as the distortions to have a resilient system in real life. Similarly, in [
62], a 400-MW OWF connected by a 75-km long HVAC cable equipped with a mix of GFM and GFL WTs was applied for BS. It was shown that 25% of plant capacity droop controlled GFM was needed for stable operation during export cable energisation and block loading. This is interesting as an outstanding question regards the GFM capacity of the system. It is not clear if it is better to only use 100% GFM converters or if only a percentage of the system must be GFM. At the same time, research into the implication of performing a transition from GFM to GFL after the system is restored is needed. In [
63], droop control has been applied to an AC microgrid comprised of energy storage, for example, a BESS, and PVs in order to realize an autonomous control, i.e., power management in a decentralized manner. This could be used in large OWFs as it shows flexibility in the primary energy source. The proposed control is based on active power GFM droop for the BESS and GFL droop for the PVs. The algorithm uses the frequency from the BESS to control the production of PVs maintaining its state of charge (SoC) in a defined range. At the primary control level, the main power management is implemented locally. At the secondary and further levels, stricter frequency requirements can be achieved to mitigate the steady-state error. The control solution is validated with both EMT and real-time simulations, which proved that this strategy works by adjusting the power generation from the PVs to the supply load, keeping the BESS SoC in safe limits. In practical terms, this control setup could be adapted to OWFs, where a centralized storage behaves as a GFM unit while the WTs are in GFL mode.
Specifically, for VSM, a review of VSM applied to WTs is given in [
64]. For type-4 WTs with no additional energy storage, an analysis of their inertial response when controlled with VSM has been presented in [
65] and it showed that these WTs have worse performance in comparison to those with additional storage. Therefore, this paper emphasizes the advantage of storage for VSM control in WTS. In [
66], VSM control is implemented in type-4 WTs equipped with short-term energy storage in the DC link. The DC-link voltage is kept constant due to the additional storage unit which charges and discharges and is connected via a DC/DC converter. Similar to [
63] with PVs and droop control, the VSM control of the storage unit is made of three operation ranges based on the SoCs of the energy storage. The SoC is adjusted in the range of its setpoint during normal VSM operation. If the SoC falls below the setpoint range, the control will change its maximum power point tracking (MPPT) mode. Conversely, when SoC is above the setpoint range, the power intake will be reduced, enabling pitch control.
Field tests of GFM WTs with VSM control have been presented in [
41,
42]. A small-scale onshore WF with 23 WTs has been compared with WTs of the same type but with GFL current control. In island mode, these WTs have been subject to dynamic load changes in an islanded system. The WTs equipped with a VSM GFM converter showed appropriate inertial response. These results will point in the direction of having a 100% GFM WT system also in normal operation, a part of BS operation.
In [
15], a comprehensive overview of different VSM implementations is given. These are classified according to the order of the SG model. A comparison of the frequency characteristics is then implemented on a radial system, focusing on five different types of VSM implementation, comparing higher-order models, i.e., VISMA-based, lower-order models, and Synchronverter. The swing-equation-based model and the Synchronverter have similar frequency characteristics. When the synchronous impedance is introduced in the VSM, the system becomes more stable with a larger stability margin. For the 4th-order model, the transient impedance is active in the high-frequency domain, which leads to a smaller stability margin than that of the VSM of the 2nd-order with synchronous impedance.
The model of the VISMA-based method has the same characteristics as that of the VSM of the 2nd-order with synchronous impedance in the low-frequency domain. However, it introduces resonance at the synchronous frequency due to the differential term. A 180° phase lag is also induced at the resonant peak. The control strategies with low-order models have larger oscillations and longer settling times in response to the load and power steps as well as the grid frequency. In comparison, the other models have lower oscillations and/or lower settling times, which prove that the impedances of SG contribute to the oscillation damping.
In [
67], the application of PSC is proposed for integrating a type-3-based OWF connected via a HVDC link to a weak AC grid. The control strategy is proven feasible for stable operation during steady state and contingencies such as two fault cases, i.e., located offshore and onshore. Real-time simulations are used for the entire study. This provides a window of applicability of PSC in WTs to HVDC-connected OWFs. The WTs provided by PSC may BS the WF power island from offshore. BS of the onshore grid needs to be discussed.
In [
59], the control topology shown in
Figure 17 is applied to a 118-bus benchmark system. EMT simulations are performed, and the results show that the proposed control can provide active and reactive power regulation, fault-ride-through capability, and the capability to operate in a 100% inverter-based grid.
In [
12], a comparison of VSM, PSC, dPLL, and DPC has been applied to a 400-MW OWF connected via HVDC. It is shown that the OWF has different characteristics based on the control method used. As DPC is the most straightforward control technique with direct voltage and frequency control without any inner loops and does not have any electromechanical characteristics like inertia or damping, it results in the highest frequency swing along with the highest transient peaks. Comparatively, VSM has a lower frequency dip due to inertia emulation in its power controller, along with lower transient power peaks due to damping provided by virtual impedance. On the other hand, PSC has a higher frequency swing than VSM due to the absence of inertia emulation, but the damping provided by the voltage controller reduces the transient power peak compared to DPC. Contrary to the power-synchronization-based VSM and PSC methods, the frequency swing in dPLL is lowest, seen with no overshoot, due to the frequency controllability of the PLL-based frequency control loop, which also provides damping and reduces the transient power peak. According to this study, it is seen that all the four methods can deal with the energisation transients in a controlled manner while maintaining the stability of voltage and frequency at the offshore terminal. Therefore, these methods seem promising for future OWF BS. A comparison of these presented methods is shown in
Table 3, where the main advantages and disadvantages of each are outlined.