Prediction of Novel Humified Gas Turbine Cycle Parameters for Ammonia/Hydrogen Fuels
Abstract
1. Introduction
2. Materials and Methods
2.1. Combustion
2.2. Gas Turbine Cycle
3. Results
3.1. Numerical Analyses—Combustion
3.2. Numerical Analyses—Gas Turbine Cycle
3.3. Inlet Parameters Range Determination
3.3.1. Compressor Pressure Ratio
3.3.2. Compressor Polytropic Efficiency
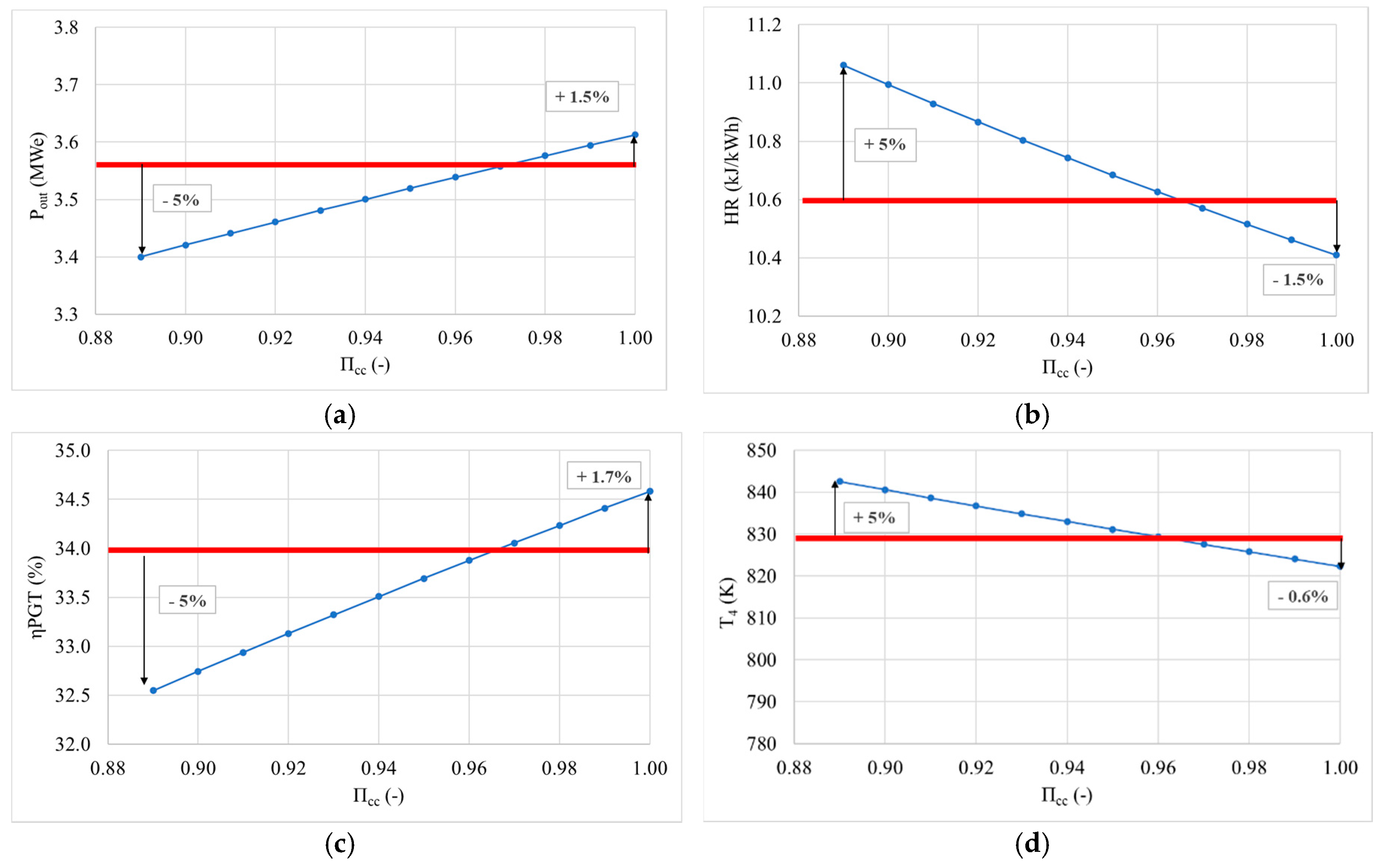
3.3.3. Combustion Chamber Pressure Ratio
3.3.4. Combustion Chamber Efficiency
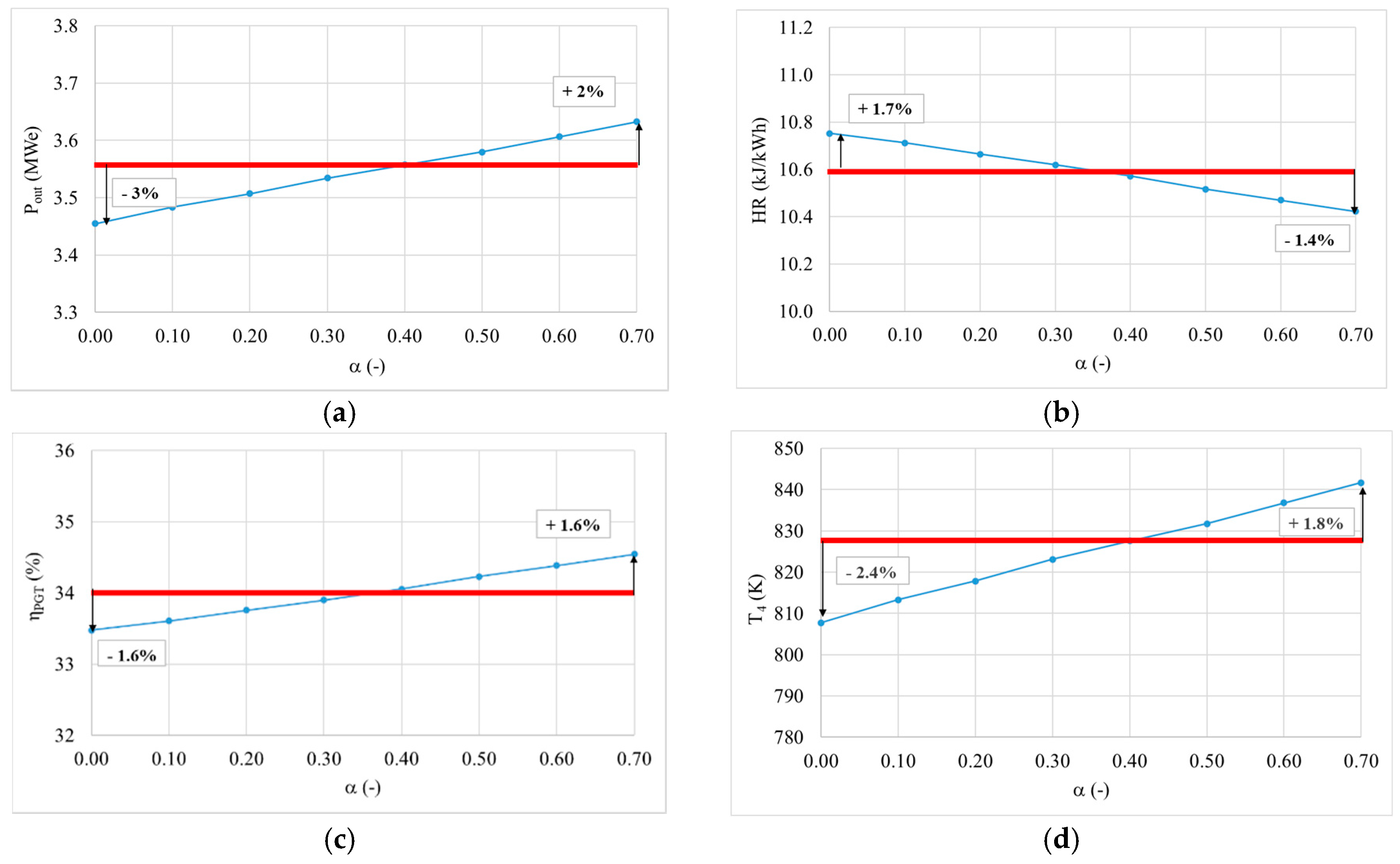
3.3.5. Steam/Fuel Ratio
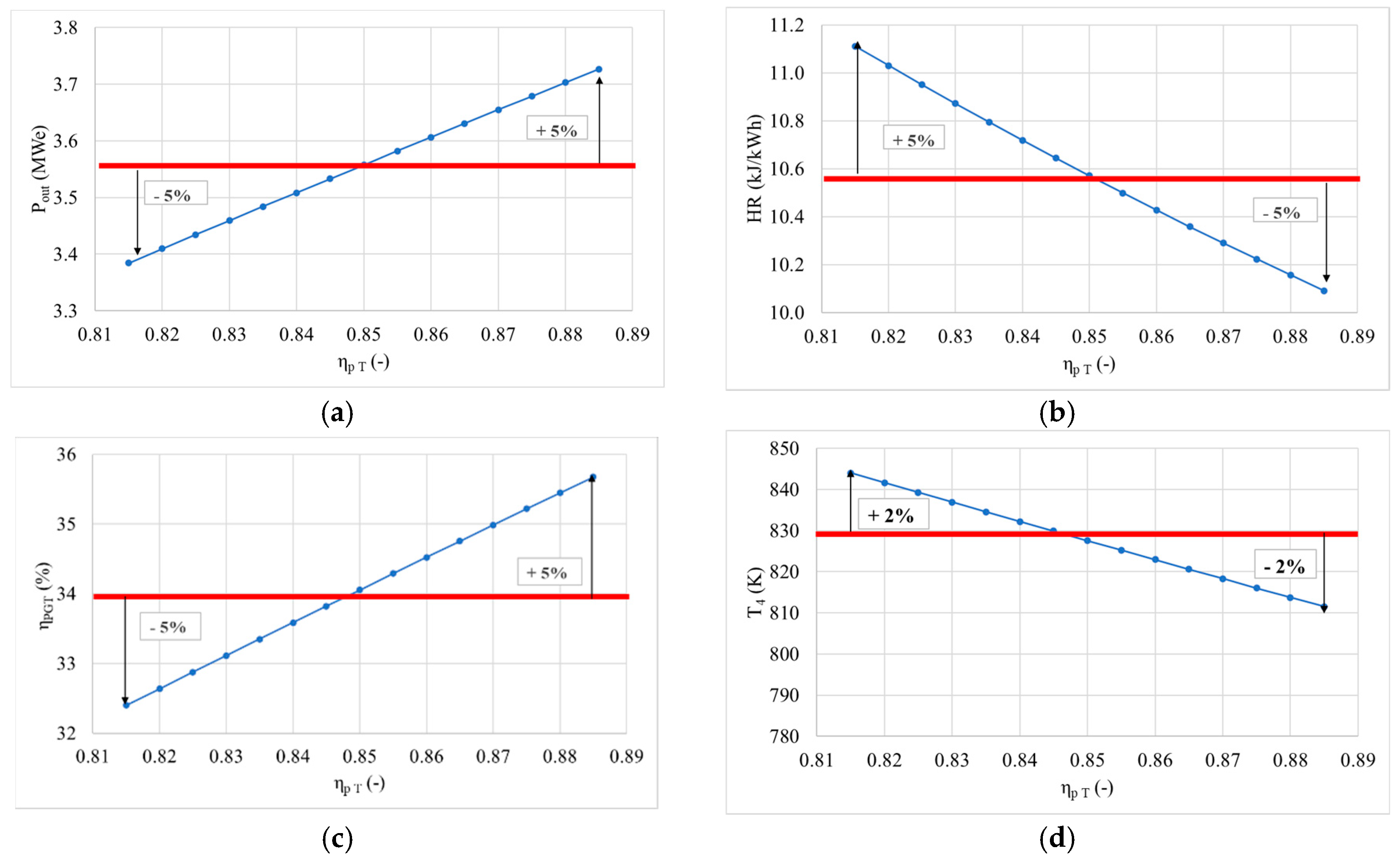
3.3.6. Gas Turbine Polytropic Efficiency
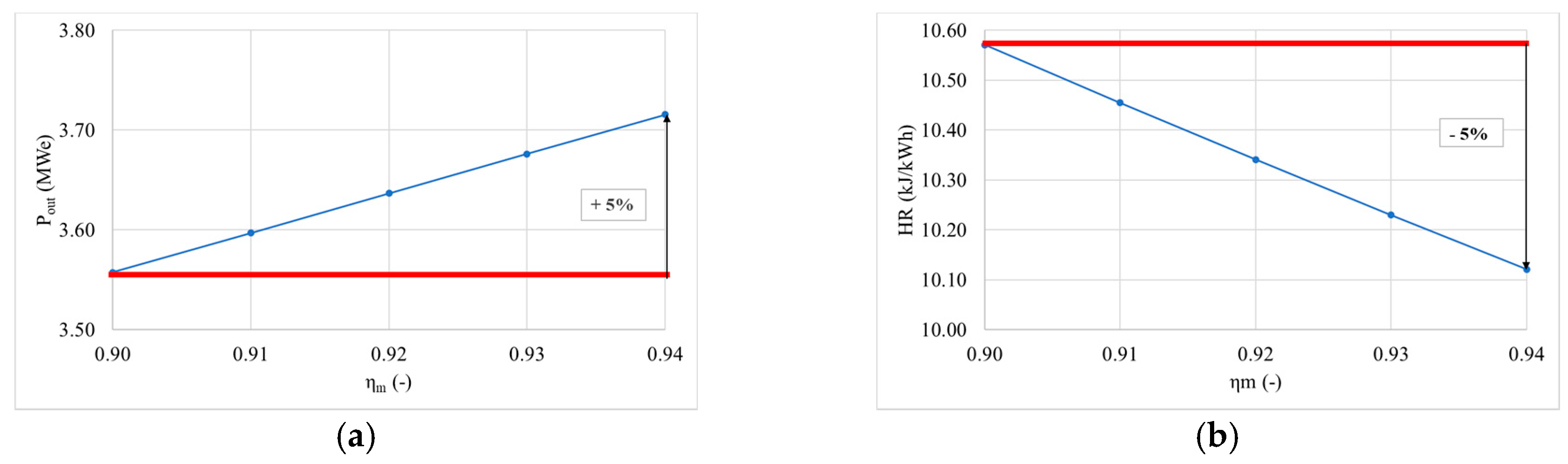
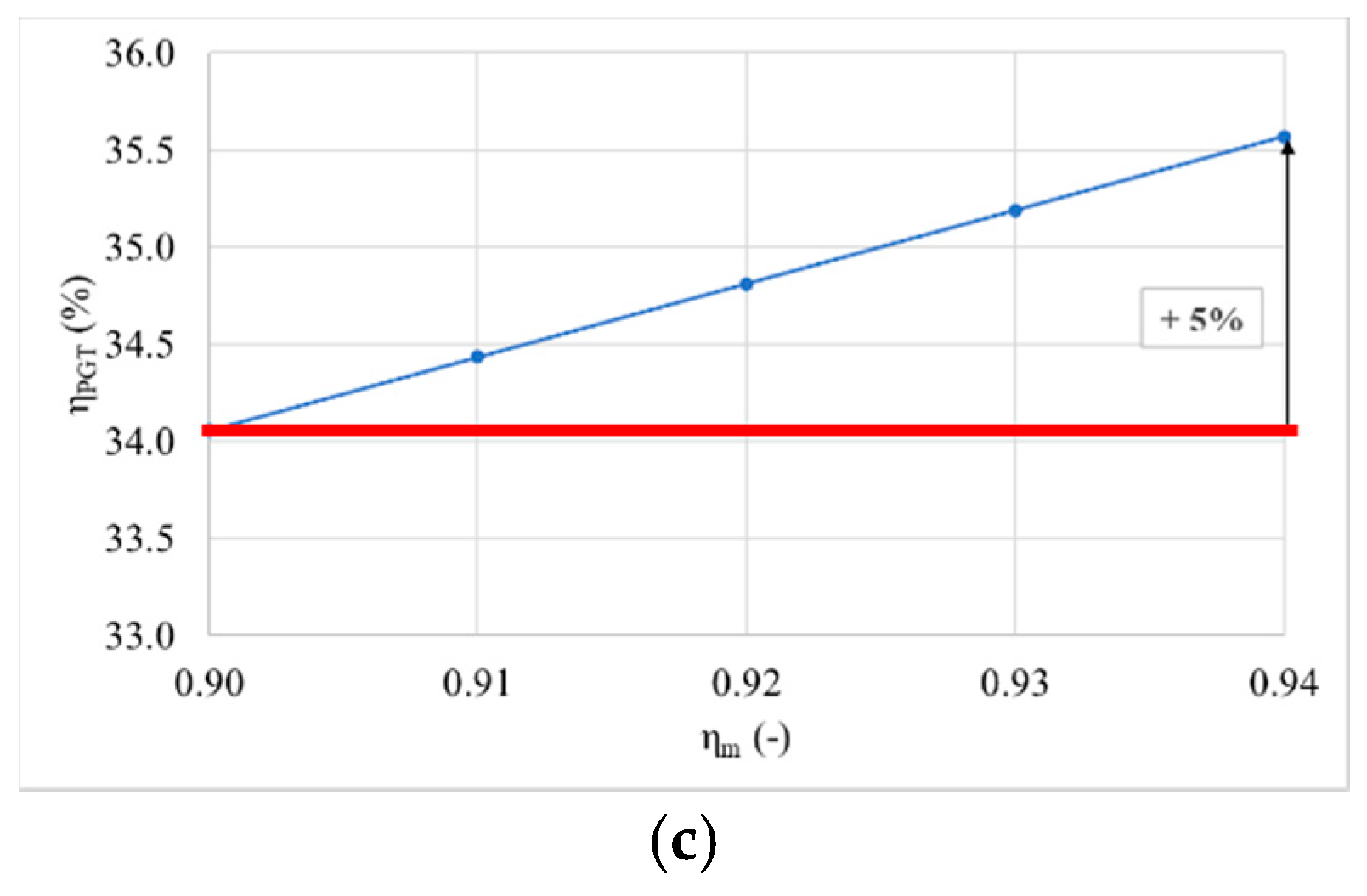
3.3.7. Mechanical Efficiency
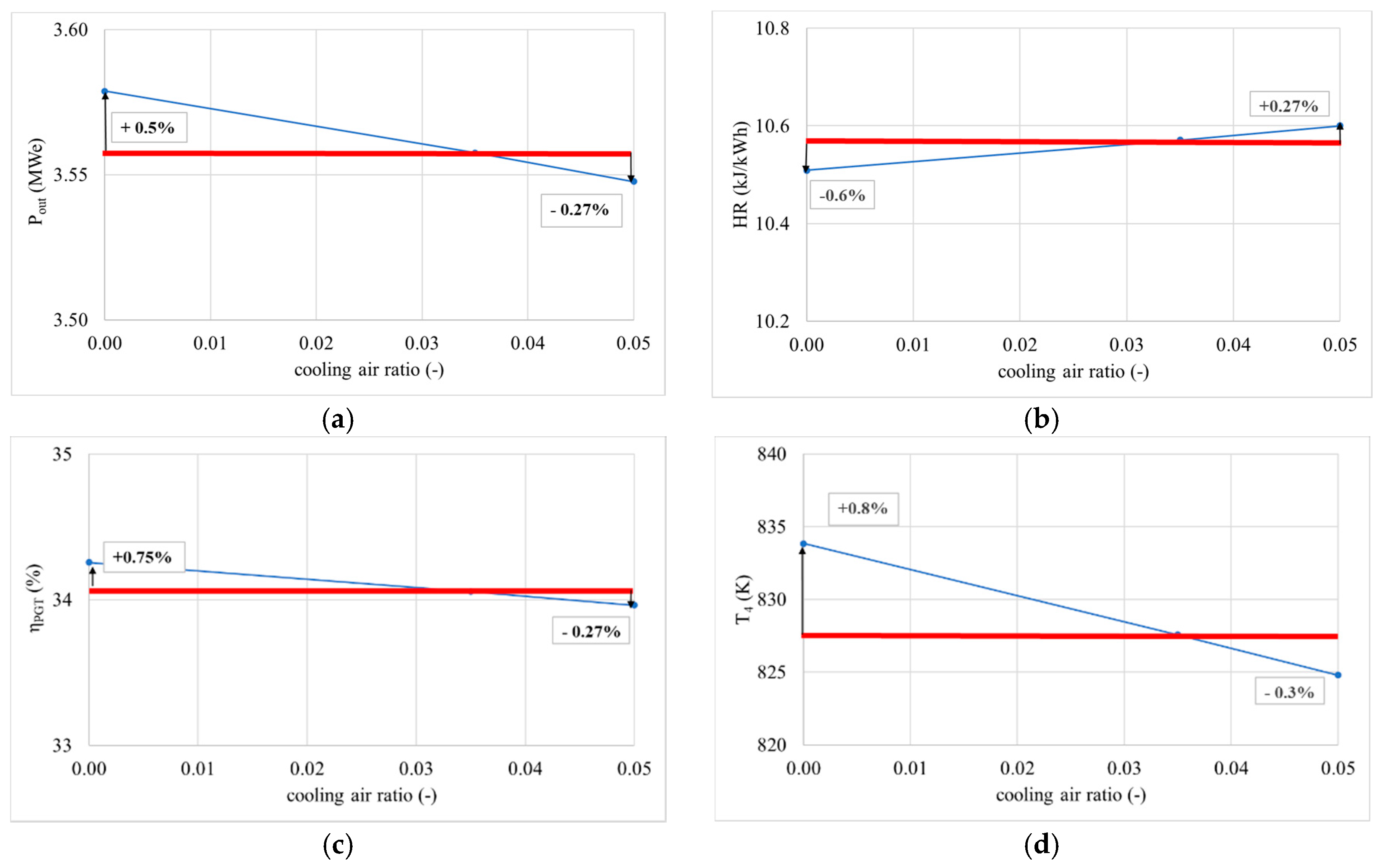
3.3.8. Cooling Air Ratio
3.4. Super-Efficient Gas Turbine Ammonia/Hydrogen Cycle
4. Conclusions
- High production of NOx and reactivity of ammonia occur at the central recirculation zone of the primary flame. However, the large pools of OH, H, O and N-based radicals are not entirely consumed in this region.
- Further recombination of these species with the already formed NOx and N2O species bring down the concentration of pollutant emissions at the end of the combustion chamber. An atmosphere comprised of 99.2% clean products (N2, H2O and H2) is obtained. However, it is clear that further use of ammonia (at 0.6% vol. concentration) can be accomplished using other post combustion techniques.
- The requirement of high-pressure ratios and low mechanical losses will increase power, thus making feasible the use of ammonia-based blends to produce power efficiently. Similarly, and as expected, the compressor polytropic efficiency and combustion efficiency also have a considerable impact on the final power output of the cycle.
- Also, it was observed that steam/fuel injection ratios were not critical for the improvement of efficiency or power, as the amount of steam is relatively small. Thus, further studies using humidified ammonia blends should focus on the chemistry and mitigation of NOx and emissions rather than the potential of this technique to raise efficiency or power outputs. Although the steam/fuel injection ratio is relatively low for conventional humidification purposes, the parameter produces limited impact to the process as a consequence of the high humidification increased caused by decomposition of ammonia (39.9% water concentration).
- Strong impacts were observed with an increase of the cooling air mass flow above 3.5%, with a decrease of the outlet power and overall efficiency up to 0.3% using cooling air mass flow values of 5%.
- Theoretical superefficient ammonia/hydrogen cycles could be obtained with the application of improved inlet parameters values (i.e., compressor, combustor, turbine and cooling), reaching theoretical maximum cycle efficiencies up to 43.4%. Thus, making highly competitive these cycles to fossil-based systems.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Ratio of the mass flowrate of the fuel and the air at the combustion chamber inlet (-) | T | temperature (K) | |
| cp CRN CRZ | Heat capacity at constant pressure (kJ/kgK) Chemical Reaction Network Central Recirculation Zone | To | the inlet compressor temperature (K) |
| is the combustion chamber inlet steam enthalpy (kJ/kg) | T2 | the outlet compressor temperature (K) | |
| is enthalpy of steam at the combustion chamber outlet (kJ/kg) | combustion products temperature at the turbine inlet (K) | ||
| hfuel | specific enthalpy of fuel at combustion chamber inlet (kJ/kg) | combustion products temperature at the end of the expansion (K) | |
| specific work of compression (kJ/kg) | the efficiency of a combustion chamber (-) | ||
| plant specific work (kJ/kg) | the efficiency of a plant (-) | ||
| LHV | the lower heating value (kJ/kg) | the mechanical efficiency (-) | |
| overall specific work of the expansion of the combustion products and cooling air mixture (kJ/kg) | is the polytropic efficiency of a compressor (-) | ||
| combustion products mass flow (kg/s) | is the polytropic efficiency of a turbine (-) | ||
| fuel mass flow at the combustion chamber inlet (kg/s) | the compressor pressure ratio (-) | ||
| air mass flow at the compressor inlet (kg/s) | is the cooling air distribution factor (-) | ||
| air mass flow at the combustion chamber inlet (kg/s) | is the amount of the amount of fuel added (kJ/kg) | ||
| is the cooling air distribution factor (-) | α | is the ratio of the vapour mass flow and fuel mass flow at the combustion chamber inlet (-) | |
| p PSR PFR | pressure (Pa) Perfectly Stirred Reactor Plug Flow Reactor | air mass flow for sealing relative to air mass flow at the compressor inlet (-) | |
| R | the universal gas constant (J/(mol.K)) | average relative error (%) | |
| cooling air mass flow specified to compressor inlet mass flow (-) |
References
- Birol, F. Hydrogen: Accelerating and Expanding Deployment; IEA: Paris, France, 2018. [Google Scholar]
- Posada, J.O.G.; Abdalla, A.H.; Oseghale, C.I.; Hall, P.J. Multiple regression analysis in the development of NiFe cells as energy storage solutions for intermittent power sources such as wind or solar. Int. J. Hydrog. Energy 2016, 41, 16330–16337. [Google Scholar] [CrossRef]
- Bowermaster, D. Renewable Ammonia Generation, Transport, and Utilization in the Transportation Sector; Technical Report for Electric Power Research Institute (EPRI): Palo Alto, CA, USA, February 2019. [Google Scholar]
- Kobayashi, H.; Hayakawa, A.; Somarathne, K.D.K.A.; Okafor, E.C. Science and Technology of Ammonia Combustion. Proc. Combust. Inst. 2018, 37, 109–133. [Google Scholar] [CrossRef]
- Guteša Božo, M.; Vigueras-Zuniga, M.O.; Buffi, M.; Seljak, T.; Valera-Medina, A. Fuel Rich Ammonia/Hydrogen Injection for Humidified Gas Turbines. Appl. Energy 2019, 251, 113334–1133346. [Google Scholar] [CrossRef]
- Valera-Medina, A.; Guteša, M.; Xiao, H.; Pugh, D.; Giles, A.; Goktepe, B.; Marsh, R.; Bowen, P. Premixed Ammonia/Hydrogen Swirl Combustion under Rich Fuel Conditions for Gas Turbines Operation. Int. J. Hydrog. Energy 2019, 44, 8615–8626. [Google Scholar] [CrossRef]
- Valera-Medina, A.; Syred, N.; Griffiths, A. Visualization of Isothermal Large Coherent Structures in a Swirl Burner. Combust. Flame 2009, 156, 1723–1734. [Google Scholar] [CrossRef]
- Kurata, O.; Iki, N.; Matsunuma, T.; Inoue, T.; Tsujimura, T.; Furutani, H.; Kobayashi, H.; Hayakawa, A. Performances and emission characteristics of NH3–air and NH3-CH4–air combustion gas-turbine power generations. Proc. Combust. Inst. 2017, 36, 3351–3359. [Google Scholar] [CrossRef]
- Hayakawa, A.; Goto, T.; Mimoto, R.; Arakawa, Y.; Kudo, T.; Kobayashi, H. Laminar burning velocity and Markstein length of ammonia/air premixed flames at various pressures. Fuel 2015, 159, 98–106. [Google Scholar] [CrossRef]
- Somarathne, K.D.K.A.; Hatakeyama, S.; Hayakawa, A.; Kobayashi, H. Numerical study of a low emission gas turbine like combustor for turbulent ammonia/air pre- mixed swirl flames with a secondary air injection at high pressure. Int. J. Hydrog. Energy 2017, 42, 27388–27399. [Google Scholar] [CrossRef]
- Xiao, H.; Howard, M.; Valera-Medina, A.; Dooley, S.; Bowen, P.J. Study on Reduced chemical mechanisms of ammonia/methane combustion under gas turbine conditions. Energy Fuels 2016, 30, 8701–8710. [Google Scholar] [CrossRef]
- Valera-Medina, A.; Marsh, R.; Runyon, J.; Pugh, D.; Beasley, P.; Hughes, T.; Bowen, P. Ammonia–methane combustion in tangential swirl burners for gas turbine power generation. Appl. Energy 2017, 185, 1362–1371. [Google Scholar] [CrossRef]
- Xiao, H.; Valera-Medina, A.; Marsh, R.; Bowen, P. Numerical study assessing various ammonia/methane reaction models for use under gas turbine conditions. Fuel 2017, 196, 344–351. [Google Scholar] [CrossRef]
- Valera-Medina, A.; Morris, S.; Runyon, J.; Pugh, D.; Marsh, R.; Beasley, P.; Hughes, T. Ammonia, methane and hydrogen for gas turbines. Energy Procedia 2015, 75, 118–123. [Google Scholar] [CrossRef]
- Valera-Medina, A.; Pugh, D.G.; Marsh, P.; Bulat, G.; Bowen, P. Preliminary study on lean premixed combustion of ammonia-hydrogen for swirling gas turbine combustors. Int. J. Hydrog. Energy 2017, 42, 24495–24503. [Google Scholar] [CrossRef]
- Xiao, H.; Valera-Medina, A. Chemical kinetic mechanism study on premixed combustion of ammonia/hydrogen fuels for gas turbine use. J. Eng. Gas Turbines Power 2017, 139, 8. [Google Scholar] [CrossRef]
- Chiesa, P. Combined Cycle Systems for Near-Zero Emission Power Generation, Chapter 5: Novel Cycles: Humid Air Cycle Systems; Woodhead Publishing (Elsevier): Cambridge, UK, 2012; pp. 162–185. [Google Scholar]
- Jonsson, M.; Yan, J. Humidified gas turbines—A review of proposed and implemented cycles. Energy 2005, 30, 1013–1078. [Google Scholar] [CrossRef]
- Taimoor, A.A.; Muhammad, A.; Saleem, W.; Zain-ul-Abdein, M. Humidified exhaust recirculation for efficient combined cycle gas turbines. Energy 2016, 106, 356–366. [Google Scholar] [CrossRef]
- Shaposhnikov, V.V.; Biryukov, B.V. On the Efficiency of Heat and Electric Power Plants Based on Combined-Cycle Plants with Overexpansion of the Working Fluid in the Gas Turbine and Injection of Steam into the Gas Path. Chem. Pet. Eng. 2018, 54, 94–99. [Google Scholar] [CrossRef]
- De Paepe, W.; Carrero, M.M.; Bram, S.; Parente, A.; Contino, F. Toward higher micro gas turbine efficiency and flexibility-humidified micro gas turbines: A review. J. Eng. Gas Turbines Power 2018, 140, 4038365. [Google Scholar] [CrossRef]
- Carapellucci, R.; Giordano, L. Upgrading existing gas-steam combined cycle power plants through steam injection and methane steam reforming. Energy 2019, 173, 229–243. [Google Scholar] [CrossRef]
- Fallah, M.; Siyahi, H.; Ghiasi, R.A.; Mahmoudi, S.M.S.; Yari, M.; Rosen, M.A. Comparison of different gas turbine cycles and advanced exergy analysis of the most effective. Energy 2016, 116, 701–715. [Google Scholar] [CrossRef]
- De Paepe, W.; Renzi, M.; Carrerro, M.M.; Caligiuri, C.; Contino, F. Micro gas turbine cycle humidification for increased flexibility: Numerical and experimental validation of different steam injection models. J. Eng. Gas Turbines Power 2019, 141, 021009. [Google Scholar] [CrossRef]
- Xi, D.; Wang, Y.; Liu, J.-Z.; Zhou, J.-H. Current status in the study of the humidified combustion. J. Eng. Therm. Energy Power 2014, 29, 1–6. [Google Scholar]
- Huang, Z.; Yang, C.; Yang, H.; Ma, X. Off-design heating/power flexibility for steam injected gas turbine based CCHP considering variable geometry operation. Energy 2018, 165, 1048–1060. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, X.; Yang, C.; Yang, Z. Control Strategies of Steam-injected Gas Turbine in CCHP System. Energy Procedia 2017, 105, 1520–1525. [Google Scholar] [CrossRef]
- De Paepe, W.; Sayad, P.; Bram, S.; Klingmann, J.; Contino, F. Experimental Investigation of the Effect of Steam Dilution on the Combustion of Methane for Humidified Micro Gas Turbine Applications. Combust. Sci. Technol. 2016, 188, 1199–1219. [Google Scholar] [CrossRef]
- De Paepe, W.; Carrerro, M.M.; Bram, S.; Parente, A.; Contino, F. Advanced Humidified Gas Turbine Cycle Concepts Applied to Micro Gas Turbine Applications for Optimal Waste Heat Recovery. Energy Procedia 2017, 105, 1712–1718. [Google Scholar] [CrossRef]
- De Paepe, W.; Montero Carrero, M.; Bram, S.; Contino, F.; Parente, A. Waste heat recovery optimization in micro gas turbine applications using advanced humidified gas turbine cycle concepts. Appl. Energy 2017, 207, 218–229. [Google Scholar] [CrossRef]
- Shahpouri, S.; Houshfar, E. Nitrogen oxides reduction and performance enhancement of combustor with direct water injection and humidification of inlet air. Clean Technol. Environ. Policy 2019, 21, 667–683. [Google Scholar] [CrossRef]
- Göke, S.; Paschereit, C.O. Influence of steam dilution on NOx formation in premixed natural gas and hydrogen flames. In Proceedings of the 50th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition 2012, Article number AIAA 2012-1272, 50th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, Nashville, TN, USA, 9–12 January 2012. [Google Scholar]
- Best, T.; Finney, K.N.; Ingham, D.B.; Pourkashanian, M. CO2-enhanced and humidified operation of a micro-gas turbine for carbon capture. J. Clean. Prod. 2018, 176, 370–381. [Google Scholar] [CrossRef]
- Shukla, A.K.; Singh, O. Performance evaluation of steam injected gas turbine-based power plant with inlet evaporative cooling. Appl. Therm. Eng. 2016, 102, 454–464. [Google Scholar] [CrossRef]
- Guteša, M.; Al-Doboon, A.; Valera-Medina, A.; Syred, N.; Bowen, P. CARSOXY (CO2-Argon-Steam-OxyFuel) Combustion in Gas Turbines for CCS Systems. In Proceedings of the 55th AIAA Aerospace Sciences Meeting, Grapevine, TX, USA, 9–13 January 2017. [Google Scholar] [CrossRef]
- Al-Doboon, A.; Guteša, M.; Valera-Medina, A.; Syred, N.; Ng, J.-H.; Chong, C.T. CO2-argon-steam oxy-fuel (CARSOXY) combustion for CCS inert gas atmospheres in gas turbines. Appl. Therm. Eng. 2017, 122, 350–358. [Google Scholar] [CrossRef]
- Guteša Božo, M.; Valera–Medina, A.; Syred, N.; Bowen, P. Fuel quality impact analysis for practical implementation of corn COB gasification gas in conventional gas turbine power plants. Biomass Bioenergy 2019, 122, 221–230. [Google Scholar] [CrossRef]
- Valera-Medina, A. Ammonia Gas Turbines. ETN Annu. Gen. Meet. Work; European Turbine Network: Amsterdam, The Netherlands, 2020; p. 59. [Google Scholar]
- Valera-Medina, A.; Syred, N.; Bowen, P. Central recirculation zone visualization in confined swirl combustors for terrestrial energy. J. Propuls. Power 2012, 29, 195–204. [Google Scholar] [CrossRef]
- Pugh, D.; Runyon, J.; Bowen, P.; Giles, A.; Valera-Medina, A.; Marsh, R.; Goktepe, B.; Hewlett, S. An Investigation of Ammonia Primary Flame Combustor Concepts for Emissions Reduction with OH*, NH2* and NH*Chemiluminescence at Elevated Conditions. Proc. Combust. Inst. 2020. [Google Scholar] [CrossRef]
- Mathieu, O.; Petersen, E.L. Experimental and modelling study on the high-temperature oxidation of Ammonia and related NOx chemistry. Combust. Flame 2015, 162, 554–570. [Google Scholar] [CrossRef]
- Marsh, R.; Runyon, J.; Giles, A.; Morris, S.; Pugh, D.; Valera-Medina, A.; Bowen, P. Premixed methane oxycombustion in nitrogen and carbon dioxide atmospheres: Measurement of operating limits, flame location and emissions. Proc. Combust. Inst. 2016, 36, 3949–3958. [Google Scholar] [CrossRef]
- Müller, K.J. Thermische Strömungsmaschinen: Auslegung und Berechnung; Springer-Verlag: Vienna, Austria, 1978; pp. 1–2. [Google Scholar] [CrossRef]
- Guteša, M. The Numerical Simulation Model of Gas Turbine Facility for Biomass Gasification Gas Application. Ph.D. Thesis, Faculty of technical sciences, University of Novi Sad, Novi Sad, Serbia, 2017. (In Serbian). [Google Scholar]
- Pugh, D.G.; Bowen, P.; Valera-Medina, A.; Runyon, J. Influence of pressure and humidity on NOx formation in a staged premixed swirling NH3/H2 flame at conditions of elevated temperature and pressure. Proc. Comb. Inst. 2019, 37, 5401–5409. [Google Scholar] [CrossRef]
- Grković, V.; Guteša, M. Lessons in Thermal Energy Plants, Equipment and Devices, Technical Sciences Textbooks; Faculty of Technical Sciences: Novi Sad, Serbia, 2015; (In English and in Serbian). [Google Scholar]

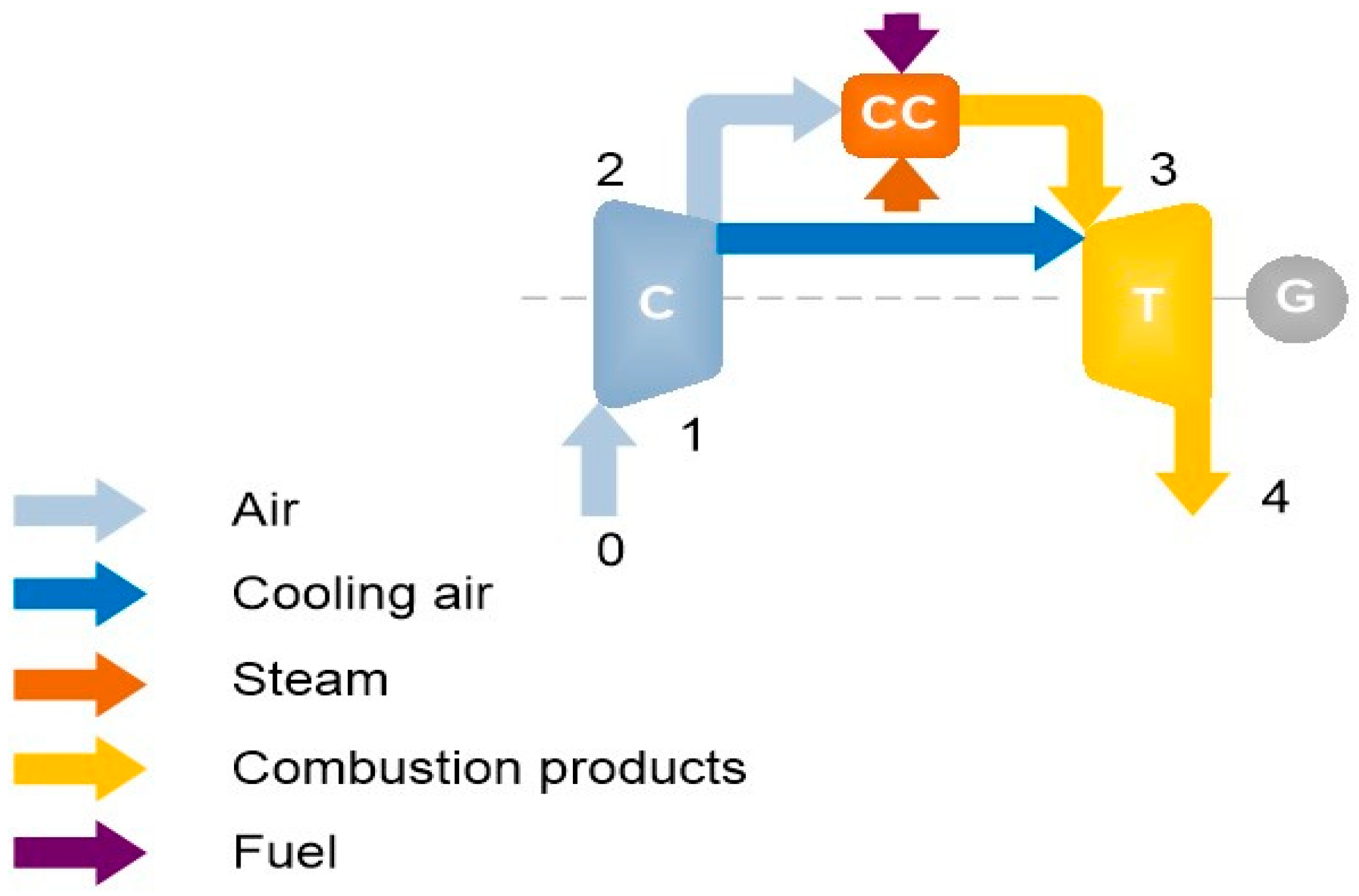
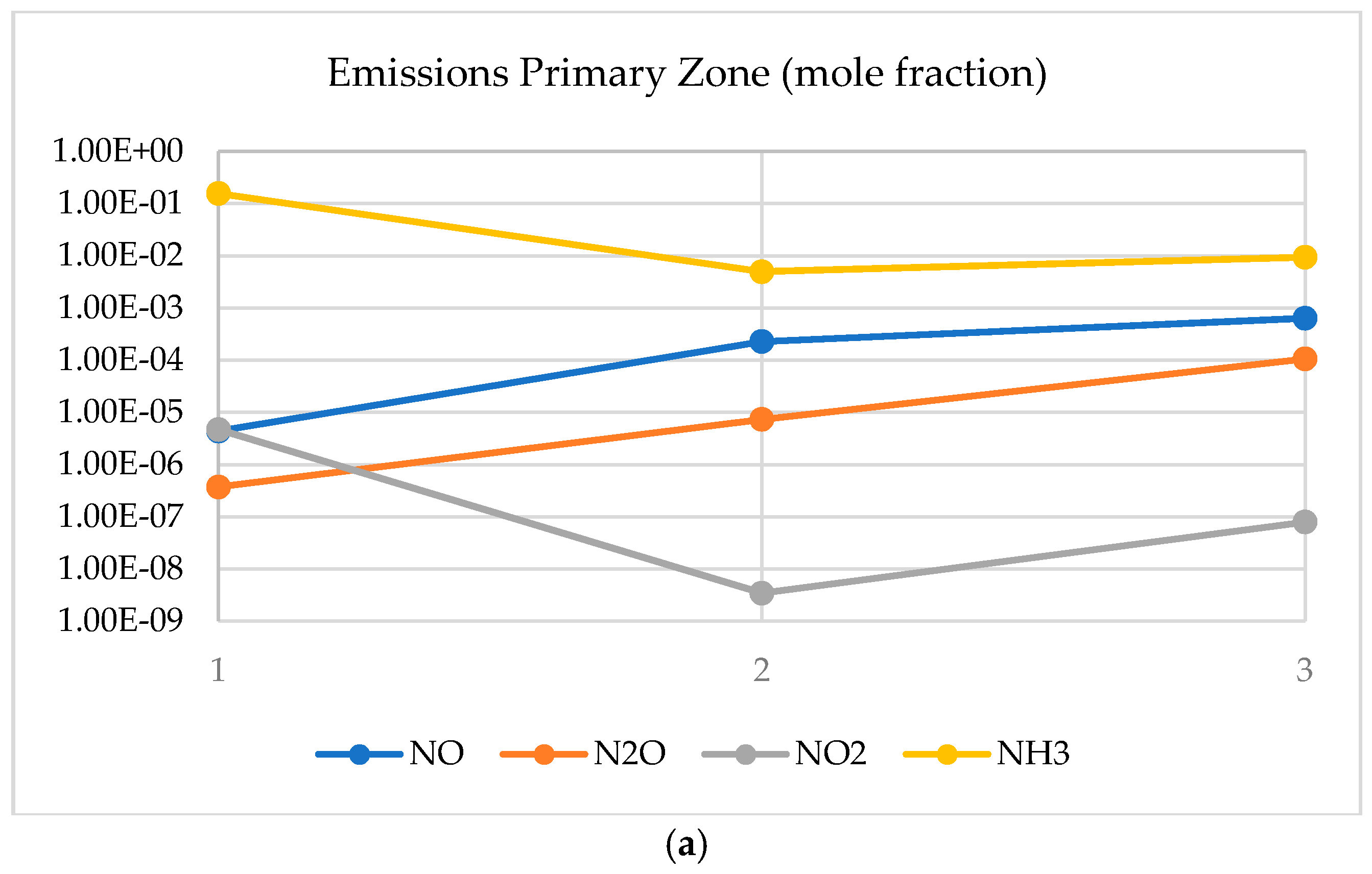
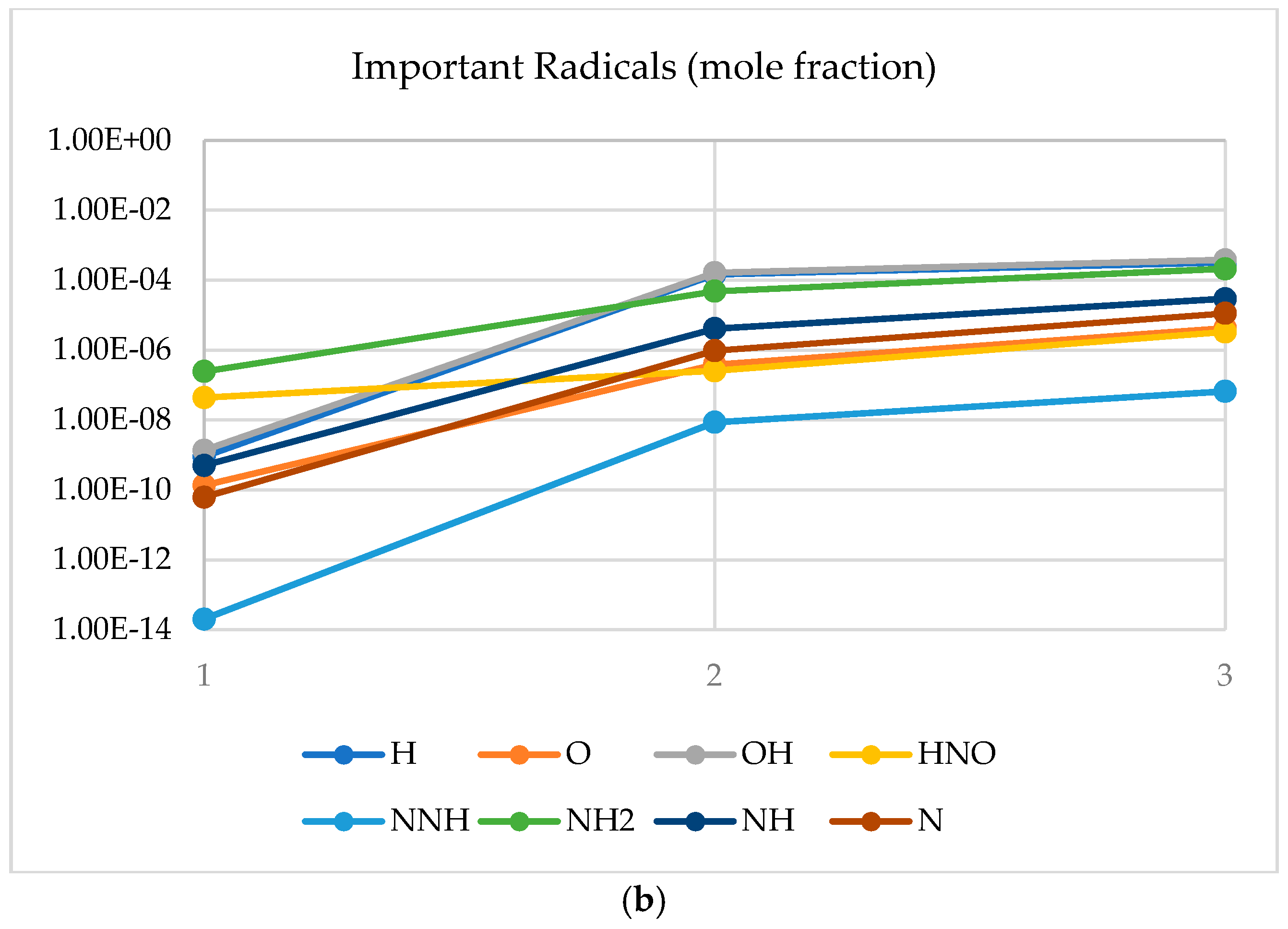

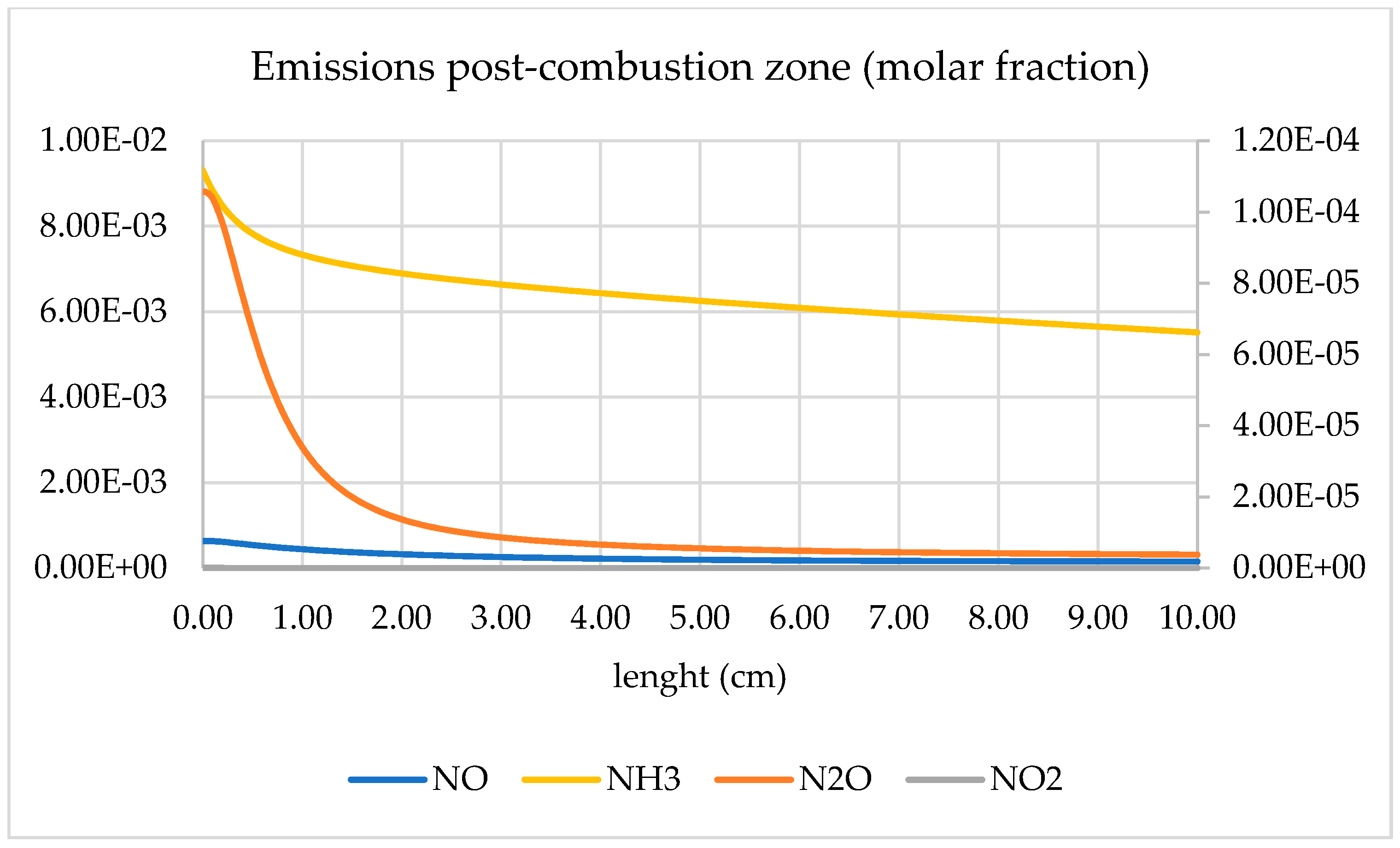



| Parameter | Symbol | Value | Units |
|---|---|---|---|
| Ambient pressure | po | 0.1 | MPa |
| Ambient temperature | T0 | 288 | K |
| Air mass flow for sealing relative to air mass flow at the compressor inlet | z | 0.01 | - |
| Compressor pressure ratio | Πc | 10.2 | - |
| Polytropic efficiency of a compressor | ηp C | 0.8 | - |
| Combustion chamber pressure ratio | ΠCC | 0.97 | - |
| Efficiency of a combustion chamber | ηCC | 0.9 | - |
| Gas turbine inlet temperature | T3 | 1260 | K |
| Polytropic efficiency of a turbine | ηp T | 0.85 | - |
| Mechanical efficiency | ηm | 0.9 | - |
| Cooling air mass flow specified to compressor inlet mass flow | rair | 0.035 | kg/kg |
| Cooling air distribution factor | M | 0.667 | - |
| Ratio of the vapour mass flow and fuel mass flow at the combustion chamber inlet | α | 0.4 | - |
| Air mass flow rate at the compressor inlet | m1 | 5.92 | kg/s |
| Air mass flow rate at the compressor outlet (combustion chamber inlet) | m2 | 5.65 | kg/s |
| Air mass flow rate for cooling gas turbine blades | mair | 0.21 | kg/s |
| Fuel mass flow rate | mfuel | 0.37 | kg/s |
| Steam mass flow rate (1.1 MPa, 459 K) | msteam | 0.15 | kg/s |
| Combustion products mass flow rate at the turbine inlet | m3 | 6.16 | kg/s |
| Combustion products mass flow rate at the turbine outlet | m4 | 6.43 | kg/s |
| Inlet Parameter | Value | ηPGT (%) | Pout (MWe) | HR (kJ/kWh) | T4 (K) | Optimal Range |
|---|---|---|---|---|---|---|
| Compressor Pressure Ratio | 9.00 | 32.52 | 3.47 | 11.07 | 848.37 | (8.5–11.5) |
| 11.50 | 35.52 | 3.63 | 10.14 | 808.34 | ||
| Compressor Polytropic Efficiency | 0.76 | 33.30 | 3.41 | 10.81 | 829.73 | (76–86%) |
| 0.86 | 35.01 | 3.74 | 10.28 | 824.61 | ||
| Combustion Chamber Pressure Ratio | 0.89 | 32.55 | 3.40 | 11.06 | 842.53 | (89–100%) |
| 1.00 | 34.58 | 3.61 | 10.41 | 822.34 | ||
| Combustion Chamber Efficiency | 0.87 | 32.76 | 3.56 | 10.99 | 827.56 | (87–94%) |
| 0.94 | 35.81 | 3.56 | 10.05 | 827.56 | ||
| Steam/Fuel Ratio | 0.00 | 33.48 | 3.45 | 10.75 | 807.78 | (0.0–0.7) |
| 0.70 | 34.54 | 3.63 | 10.42 | 841.72 | ||
| Gas Turbine Polytropic Efficiency | 0.82 | 32.40 | 3.38 | 11.11 | 843.99 | (82–89%) |
| 0.89 | 35.67 | 3.73 | 10.09 | 811.50 | ||
| Mechanical Efficiency | 0.90 | 34.06 | 3.56 | 10.6 | 828 | (90–94%) |
| 0.94 | 35.57 | 3.72 | 10.12 | 827.56 | ||
| Cooling Air Ratio | 0.00 | 34.26 | 3.58 | 10.51 | 833.84 | (0.00–0.05) |
| 0.05 | 33.96 | 3.55 | 10.60 | 824.79 |
| Parameter | Symbol | Value | Units |
|---|---|---|---|
| Ambient pressure | po | 0.1 | MPa |
| Ambient temperature | T0 | 288 | K |
| Air mass flow for sealing relative to air mass flow at the compressor inlet | z | 0.01 | - |
| Compressor pressure ratio | Πc | 11.5 | - |
| Polytropic efficiency of a compressor | ηp C | 0.86 | - |
| Combustion chamber pressure ratio | ΠCC | 0.99 | - |
| Efficiency of a combustion chamber | ηCC | 0.94 | - |
| Gas turbine inlet temperature | T3 | 1280 | K |
| Polytropic efficiency of a turbine | ηp T | 0.89 | - |
| Mechanical efficiency | ηm | 0.95 | - |
| Cooling air mass flow specified to compressor inlet mass flow | rair | 0.035 | kg/kg |
| Cooling air distribution factor | M | 0.667 | - |
| Ratio of the vapour mass flow and fuel mass flow at the combustion chamber inlet | α | 0.70 | - |
| Air mass flow rate at the compressor inlet | m1 | 5.92 | kg/s |
| Air mass flow rate at the compressor outlet (combustion chamber inlet) | m2 | 5.65 | kg/s |
| Air mass flow rate for cooling gas turbine blades | mair | 0.21 | kg/s |
| Fuel mass flow rate | mfuel | 0.37 | kg/s |
| Steam mass flow rate | msteam | 0.26 | kg/s |
| Combustion products mass flow rate at the turbine inlet | m3 | 6.27 | kg/s |
| Combustion products mass flow rate at the turbine outlet | m4 | 6.54 | kg/s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Božo, M.G.; Valera-Medina, A. Prediction of Novel Humified Gas Turbine Cycle Parameters for Ammonia/Hydrogen Fuels. Energies 2020, 13, 5749. https://doi.org/10.3390/en13215749
Božo MG, Valera-Medina A. Prediction of Novel Humified Gas Turbine Cycle Parameters for Ammonia/Hydrogen Fuels. Energies. 2020; 13(21):5749. https://doi.org/10.3390/en13215749
Chicago/Turabian StyleBožo, Milana Guteša, and Agustin Valera-Medina. 2020. "Prediction of Novel Humified Gas Turbine Cycle Parameters for Ammonia/Hydrogen Fuels" Energies 13, no. 21: 5749. https://doi.org/10.3390/en13215749
APA StyleBožo, M. G., & Valera-Medina, A. (2020). Prediction of Novel Humified Gas Turbine Cycle Parameters for Ammonia/Hydrogen Fuels. Energies, 13(21), 5749. https://doi.org/10.3390/en13215749






