Experimental Study of Harmonic Influence on Electrical Energy Metering
Abstract
1. Introduction
2. Research Methods
2.1. Influence of Harmonic Parameters on Power Measurement by Electronic Electricity Meters
2.2. Influence of Capacitor Banks with Different Levels of Reactive Power Compensation on the Measurement of Active and Reactive Power
2.3. Assessment of the Reactive Power Measurement under Realistic Distorted Voltages and Currents
3. Results and Discussion
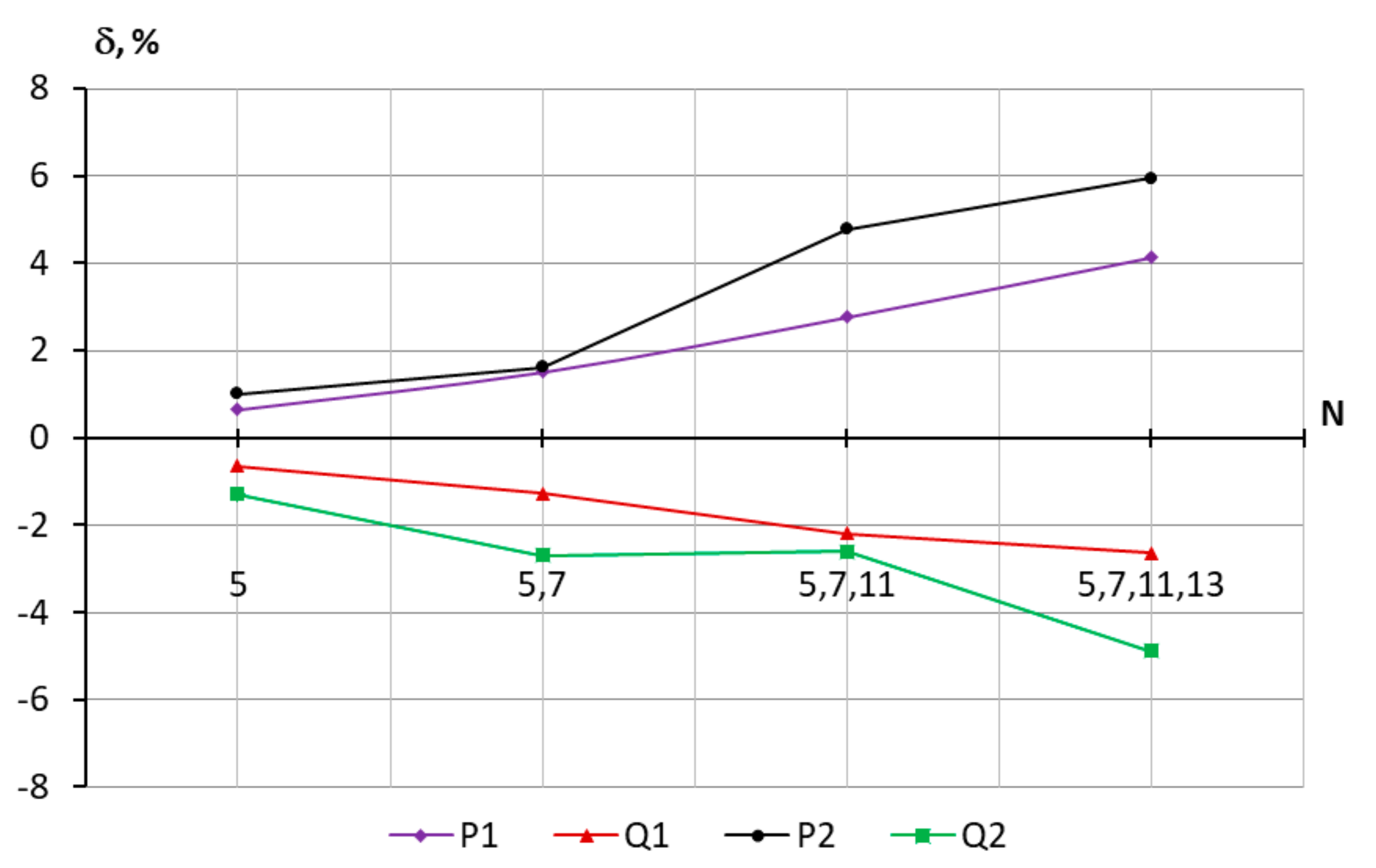
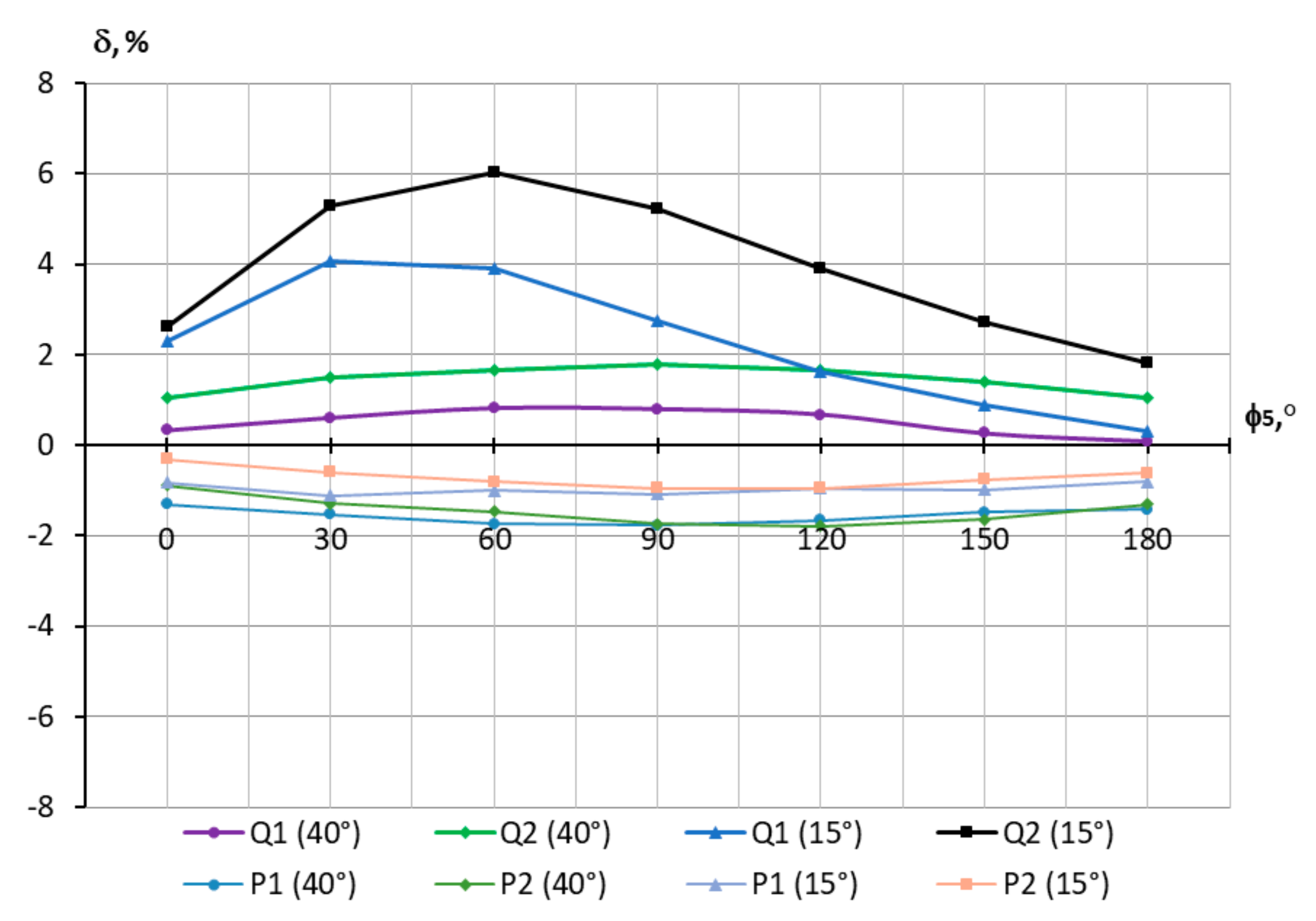
3.1. Influence of Harmonic Parameters on Power Measurement
3.2. Influence of Capacitor Banks on Power Measurement
3.3. Reactive Power Measurement under Realistic Distortions
4. Conclusions
- It has been revealed that, currently, virtually all enterprises can use electronic electricity meters, which give comparable readings for active and reactive energy in accordance with their accuracy class under sinusoidal conditions. Due to an increased distortion level in voltage and current, these energy meters are also used for measurements in the presence of harmonics. Under such conditions, the error of energy metering increases, wherein this error for active power depends on the number of harmonics taken into account and their amplitude, and for reactive power it depends further on the phase shift angle at the fundamental and high frequencies.
- In the presence of distortion, different types of meters can give different readings, especially when measuring reactive power. The studies performed have shown that reactive power can be measured using various mathematical models. With sinusoidal voltage and current, this approach will give comparable readings with respect to power consumption; however, in the presence of harmonics, the readings can vary significantly. On the basis of field measurements, it was revealed that the variation range of the phase shift angles of harmonics significantly increases when capacitor banks are connected. It was also found that for the conditions under consideration, the computational error of reactive power measurements according to various equations does not exceed 10%, provided that the THDU does not exceed 14%, and the THDI does not exceed 40%. If the voltage and current distortion values do not exceed the permissible values, the error can be estimated at, at most, 5–7%.
Author Contributions
Funding
Conflicts of Interest
References
- Aryshnskii, E.V.; Bazhin, V.Y.; Kawalla, R. Strategy of refining the structure of aluminum-magnesium alloys by complex microalloying with transition elements during casting and subsequent thermomechanical processing. Non Ferr. Met. 2019, 46, 28–32. [Google Scholar] [CrossRef]
- Vasiliev, B.Y.; Kozyaruk, A.E.; Mardashov, D.V. Increasing the utilization factor of an autonomous inverter under space vector control. Russ. Electr. Eng. 2020, 91, 247–254. [Google Scholar] [CrossRef]
- Zhukovskiy, Y.; Koteleva, N. Development of augmented reality system for servicing electromechanical equipment. J. Phys. Conf. Ser. 2018, 1015, 042068. [Google Scholar] [CrossRef]
- Abramovich, B.N.; Veprikov, A.A.; Sychev, Y.A.; Lyakh, D.A. Use of active power transducers in industrial dc power systems supplying electrolysis cells. Tsvetnye Met. 2020, 2, 95–100. [Google Scholar] [CrossRef]
- ElDeeb, A.B.; Brichkin, V.N.; Bertau, M.; Savinova, Y.A.; Kurtenkov, R.V. Solid state and phase transformation mechanism of kaolin sintered with limestone for alumina extraction. Appl. Clay Sci. 2020, 196, 105771. [Google Scholar] [CrossRef]
- Sizyakov, V.M.; Vlasov, A.A.; Bazhin, V.Y. Strategy tasks of the Russian metallurgical complex. Tsvetnye Met. 2016, 1, 32–37. [Google Scholar] [CrossRef]
- Munoz-Guijosa, J.M.; Kryltcov, S.B.; Solovev, S.V. Application of an active rectifier used to mitigate currents distortion in 6–10 KV distribution grids. J. Min. Inst. 2019, 236, 229–238. [Google Scholar]
- Sychev, Y.; Abramovich, B.; Prokhorova, V. The assesement of the shunt active filter efficiency under varied power supply source and load parameters. Int. J. Electr. Comput. Eng. 2020, 10, 5621–5630. [Google Scholar] [CrossRef]
- Belsky, A.; Dobush, V.S.; Haikal, S.F. Operation of a single-phase autonomous inverter as a part of a low-power wind complex. J. Min. Inst. 2019, 239, 564–569. [Google Scholar] [CrossRef]
- Femine, A.; Gallo, D.; Landi, C.; Luiso, M. Advanced instrument for field calibration of electrical energy meters. IEEE Trans. Instrum. Meas. 2009, 58, 618–625. [Google Scholar] [CrossRef]
- IEC. Electricity Metering Equipment—Particular Requirements—Part 21: Static Meters for AC Active Energy (Classes 0,5, 1 and 2); IEC 62053-21:2020; IEC: Geneva, Switzerland, 2020. [Google Scholar]
- IEC. Electricity Metering Equipment—Particular Requirements—Part 22: Static Meters for AC Active Energy (Classes 0,1S, 0,2S and 0,5S); IEC 62053-22:2020; IEC: Geneva, Switzerland, 2020. [Google Scholar]
- IEC. Electricity Metering Equipment—Particular Requirements—Part 23: Static Meters for Reactive Energy (Classes 2 and 3); IEC 62053-23:2020; IEC: Geneva, Switzerland, 2020. [Google Scholar]
- IEC. Electricity Metering Equipment—Particular Requirements—Part 24: Static Meters for Fundamental Component Reactive Energy (Classes 0,5S, 1S, 1, 2 and 3); IEC 62053-24:2020; IEC: Geneva, Switzerland, 2020. [Google Scholar]
- Barbaro, P.V.; Cataliotti, A.; Cosentino, V.; Nuccio, S. A novel approach based on nonactive power for the identification of disturbing loads in power systems. IEEE Trans. Power Deliv. 2007, 22, 1782–1789. [Google Scholar] [CrossRef]
- Cataliotti, A.; Cosentino, V.; Salvatore, N. The measurement of reactive energy in polluted distribution power systems: An analysis of the performance of commercial static meters. IEEE Trans. Power Deliv. 2008, 23, 1296–1301. [Google Scholar] [CrossRef]
- Hu, W.; Duan, X.; Li, Q.; Zhang, L. Impact analysis and testing of harmonic of electrified railway on energy metering. Phys. Procedia 2012, 24, 1024–1030. [Google Scholar] [CrossRef][Green Version]
- Driesen, J.; Van Craenenbroeck, T.; Van Dommelen, D. The registration of harmonic power by analog and digital power meters. IEEE Trans. Instrum. Meas. 1998, 47, 195–198. [Google Scholar] [CrossRef]
- Filipski, P.; Labaj, P.W. Evaluation of reactive power meters in the presence of high harmonic distortion. IEEE Trans. Power Deliv. 1992, 7, 1793–1799. [Google Scholar] [CrossRef]
- Ortiz, A.; Lehtonen, M.; Mañana, M.; Renedo, C.; Eguíluz, L.I. Energy meter behaviour under non-sinusoidal conditions. Renew. Energy Power Qual. J. 2004, 1, 296. [Google Scholar] [CrossRef]
- Bartolomei, L.; Cavaliere, D.; Mingotti, A.; Peretto, L.; Tinarelli, R. Testing of electrical energy meters subject to realistic distorted voltages and currents. Energies 2020, 13, 2023. [Google Scholar] [CrossRef]
- Ferrero, A. Definitions of electrical quantities commonly used in non-sinusoidal conditions. Eur. Trans. Electr. Power 2007, 8, 235–240. [Google Scholar] [CrossRef]
- Fiorucci, E. The measurement of actual apparent power and actual reactive power from the instantaneous power signals in single-phase and three-phase systems. Electr. Power Syst. Res. 2015, 121, 227–242. [Google Scholar] [CrossRef]
- IEEE Standard Definitions for the Measurement of Electric Power Quantities Under Sinusoidal, Nonsinusoidal, Balanced, or Unbalanced Conditions; IEEE 1459-2010; IEEE: New York, NY, USA, 2010.
- Gokhale, P.; Bakre, S. Impact of harmonics on accuracy of metering. In Proceedings of the International Conference on Information, Communication, Engineering and Technology (ICICET), Pune, India, 29–31 August 2018; pp. 1–3. [Google Scholar] [CrossRef]
- Hasanuzzaman Shawon, M.; Barczentewicz, S.; Bień, A.; Hanzelka, Z. Localization of Harmonic Sources in Power System—Simulation and Laboratory Study. Renew. Energy Power Qual. J. 2016, 1, 546–551. [Google Scholar] [CrossRef]
- Stevanovic, D.; Petkovic, P. A single-point method for identification sources of harmonic pollution applicable to standard power meters. Electr. Eng. 2019, 97, 165–174. [Google Scholar] [CrossRef]
- Lin, R.; Xu, L.; Zheng, X. A method for harmonic sources detection based on harmonic distortion power rate. IOP Conf. Ser. Mater. Sci. Eng. 2018, 322, 072038. [Google Scholar] [CrossRef]

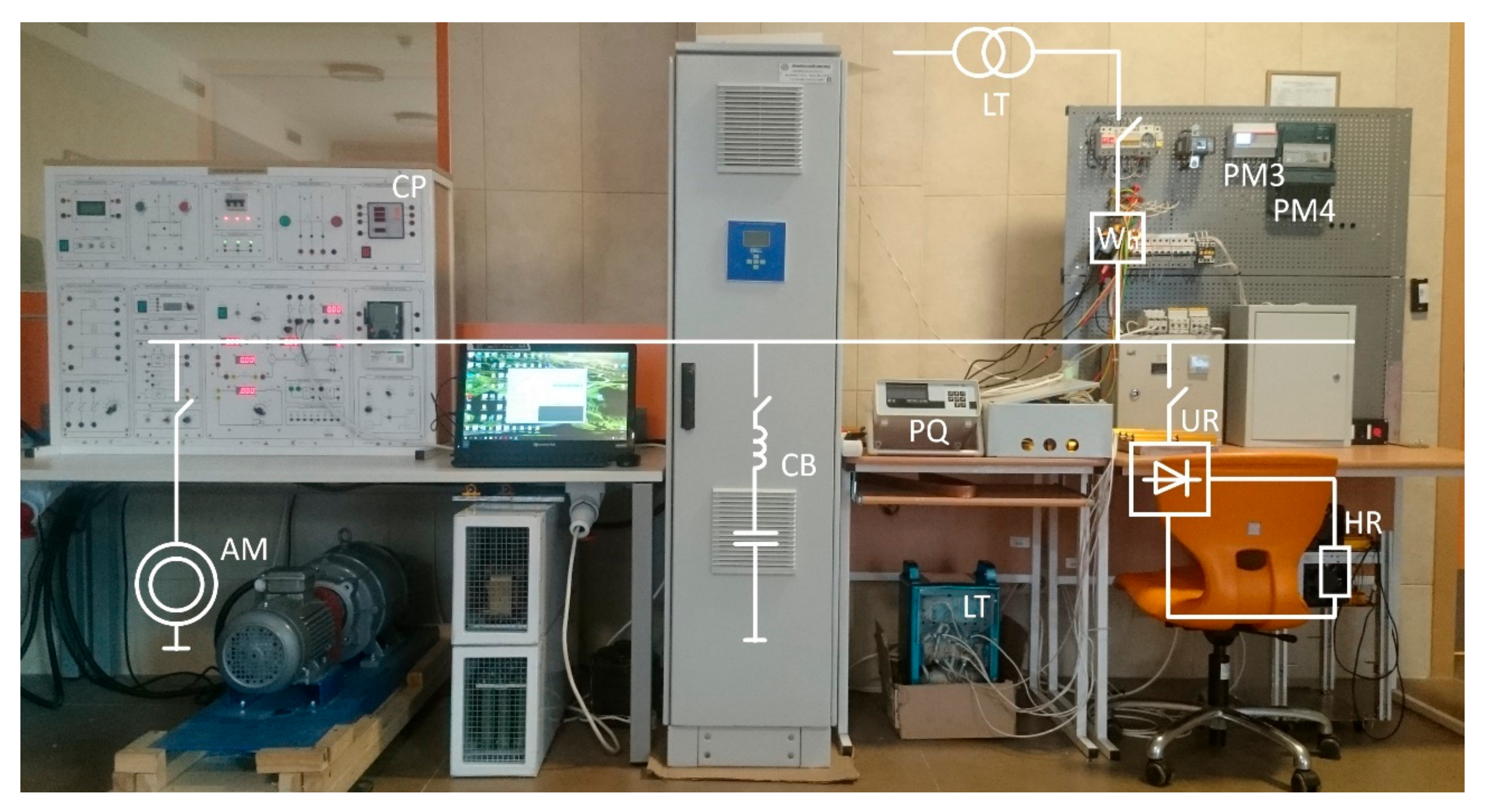



| Name of Equipment | Type | Parameters |
|---|---|---|
| PQ analyzer | Resurs UF2M | a.c. 0.2/0.5 for P/Q, THDU range 0.5–30% |
| AC source | Energoforma 3.3 | relative error 2% at ULN1/I1 range 20–220 V/0.05–7 A |
| PM1 | Elster A1800 | a.c. 0.5 s/1.0 for P/Q, Un = 230 V, In(max) = 5(10) A |
| PM2 | PM710MG | a.c. 1.0/2.0 for P/Q, Un = 230 V, In(max) = 5(6) A |
| № | ϕ1 | U5 | I5 | ϕ5 | U7 | I7 | ϕ7 | U11 | I11 | ϕ11 | U13 | I13 | ϕ13 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 40 | 30 | 40 | −100/17 | - | - | - | - | - | - | - | - | - |
| 2 | 40 | 30 | 40 | −100/17 | 20 | 25 | −100/13 | - | - | - | - | - | - |
| 3 | 40 | 30 | 40 | −100/17 | 20 | 25 | −100/13 | 21 | 17 | −95/10 | - | - | - |
| 4 | 40 | 30 | 40 | −100/17 | 20 | 25 | −100/13 | 21 | 17 | −95/10 | 18 | 15 | −95/10 |
| 5 | 20 | 40 | 60 | 260/−50 | - | - | - | - | - | - | - | - | - |
| 6 | 20 | 40 | 60 | 260/−50 | 25 | 40 | 260/−60 | - | - | - | - | - | - |
| 7 | 20 | 40 | 60 | 260/−50 | 25 | 40 | 260/−60 | 30 | 25 | 265/−70 | - | - | - |
| 8 | 20 | 40 | 60 | 260/−50 | 25 | 40 | 260/−60 | 30 | 25 | 265/−70 | 25 | 20 | 265/−70 |
| 9 | 15/40 | 15 | 25 | 0 | - | - | - | - | - | - | - | - | - |
| 10 | 15/40 | 15 | 25 | 30 | - | - | - | - | - | - | - | - | - |
| 11 | 15/40 | 15 | 25 | 60 | - | - | - | - | - | - | - | - | - |
| 12 | 15/40 | 15 | 25 | 90 | - | - | - | - | - | - | - | - | - |
| 13 | 15/40 | 15 | 25 | 120 | - | - | - | - | - | - | - | - | - |
| 14 | 15/40 | 15 | 25 | 150 | - | - | - | - | - | - | - | - | - |
| 15 | 15/40 | 15 | 25 | 180 | - | - | - | - | - | - | - | - | - |
| 16 | 15/40 | 15 | 15 | 0 | - | - | - | - | - | - | - | - | - |
| 17 | 15/40 | 15 | 30 | 0 | - | - | - | - | - | - | - | - | - |
| 18 | 15/40 | 15 | 45 | 0 | - | - | - | - | - | - | - | - | - |
| 19 | 15/40 | 15 | 60 | 0 | - | - | - | - | - | - | - | - | - |
| Equipment | Type | Parameters |
|---|---|---|
| LT | Suntek | Sn = 20 kVA, Un = 220/380 V, Imax = 80 A |
| AM | AIR 80 A | Pn = 1.5 kW, Un = 220/380 V, cosφ = 0.84 |
| CB | CB-134-0.4-3-0.5 | Qn = 2.5 kvar, Un = 220/380 V, ftun = 134 Hz |
| UR | Diode Rectifier | Pn = 1.0 kW, Un = 220/380 V, Imax = 60 A, m = 6 |
| PM3 | A43 412-200 | a.c. 1.0/2.0 for P/Q, Un = 3x230/400 V, In(max) = 5(80) A |
| PM4 | 230 AR | a.c. 1.0/2.0 for P/Q, Un = 3x230/400 V, In(max) = 5(60) A |
| Mode Number | AM with DC Generator | CB | UR with HR | Power Factor |
|---|---|---|---|---|
| Mode 1 | + | - | + | 0.42 (+L) |
| Mode 2 | + | + | + | 0.94 (+L) |
| Mode 3 | + | + | + | 0.92 (−C) |
| Mode 4 | + | - | + | 0.74 (+L) |
| Mode 5 | + | + | + | 0.94 (+L) |
| Mode 6 | + | + | + | 0.88 (−C) |
| N | PM3 | PM4 | PQ Analyser | Power Factor | δQ1, % | δQ2, % | |||
|---|---|---|---|---|---|---|---|---|---|
| P, W | Q, var | P, W | Q, var | P, W | Q, var | ||||
| 1 | 728 | 1554 | 733 | 1569 | 729 | 1550 | 0.42 (+L) | 0.3 | 1.2 |
| 2 | 772 | 183 | 771 | 260 | 773 | 240 | 0.94 (+L) | −23.8 | 8.3 |
| 3 | 785 | −285 | 787 | −322 | 787 | −340 | 0.92 (−C) | −16.2 | −5.3 |
| 4 | 1408 | 1293 | 1404 | 1332 | 1409 | 1330 | 0.74 (+L) | −2.8 | 0.2 |
| 5 | 1420 | 480 | 1420 | 522 | 1421 | 500 | 0.94 (+L) | −4.0 | 4.4 |
| 6 | 1442 | −776 | 1441 | −780 | 1444 | −790 | 0.88 (−C) | −1.8 | −1.3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shklyarskiy, Y.; Hanzelka, Z.; Skamyin, A. Experimental Study of Harmonic Influence on Electrical Energy Metering. Energies 2020, 13, 5536. https://doi.org/10.3390/en13215536
Shklyarskiy Y, Hanzelka Z, Skamyin A. Experimental Study of Harmonic Influence on Electrical Energy Metering. Energies. 2020; 13(21):5536. https://doi.org/10.3390/en13215536
Chicago/Turabian StyleShklyarskiy, Yaroslav, Zbigniew Hanzelka, and Aleksandr Skamyin. 2020. "Experimental Study of Harmonic Influence on Electrical Energy Metering" Energies 13, no. 21: 5536. https://doi.org/10.3390/en13215536
APA StyleShklyarskiy, Y., Hanzelka, Z., & Skamyin, A. (2020). Experimental Study of Harmonic Influence on Electrical Energy Metering. Energies, 13(21), 5536. https://doi.org/10.3390/en13215536







