Impedance Modeling and Stability Analysis of VSG Controlled Grid-Connected Converters with Cascaded Inner Control Loop
Abstract
1. Introduction
- (1)
- A novel SISO impedance model with simple mathematical expression is developed to analyze the impedance characteristics of the VSG controlled grid-connected converters with the cascaded inner control loop.
- (2)
- Based on the developed SISO impedance model, the analysis on how the impedance characteristics are shaped by controllers in the cascaded inner control loop is provided.
- (3)
- Impedance-based system stability of VSG controlled converters with different kinds of weak grid is analyzed, and the influence of cascaded inner control loop on possible system instabilities are quantified by differentiating the expression of developed SISO impedance model.
2. Small-Signal Modeling of VSG Controlled Converters with Cascaded Inner Control Loop
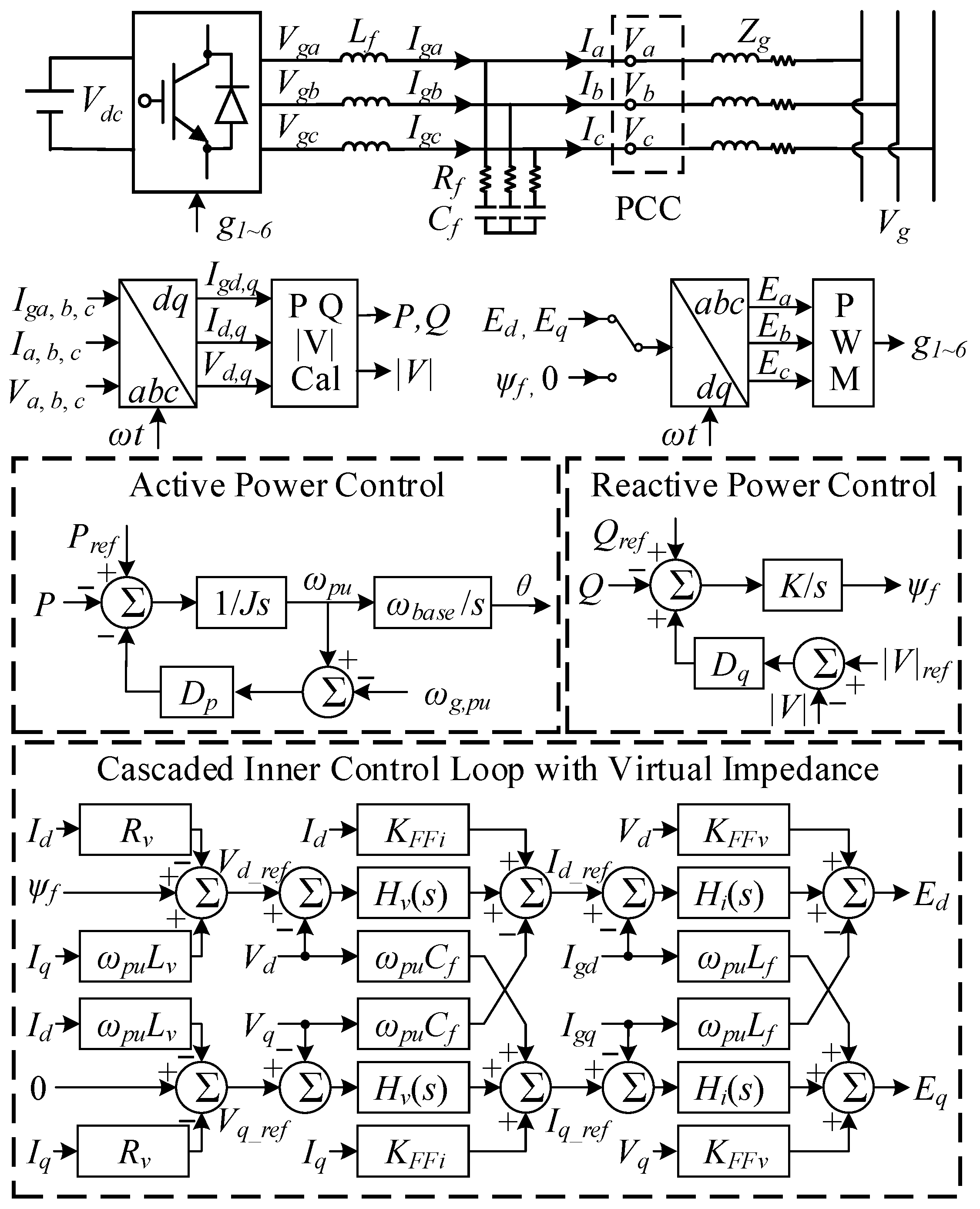
2.1. VSG Control Strategy with Cascaded Inner Control Loop
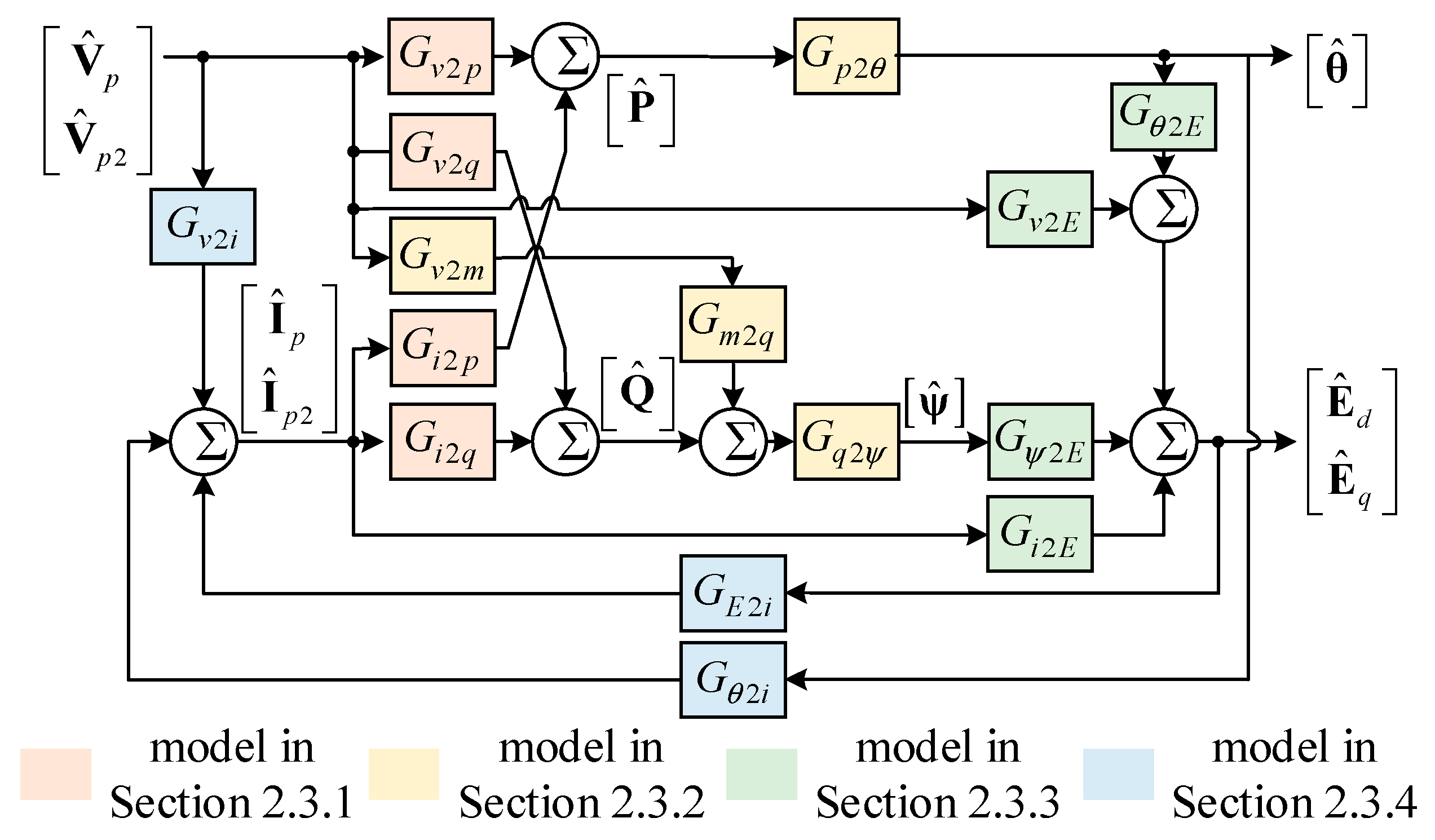
2.2. Definition of Small-Signal Models
2.3. Small-Signal Modeling of VSG Control Strategy
2.3.1. Modeling of Power Relationship
2.3.2. Modeling of Active and Reactive Power Control
2.3.3. Modeling of Cascaded Inner Control Loop with Virtual Impedance
2.3.4. Modeling of Modulation
2.4. Small-Signal Models of VSG Controlled Converter System
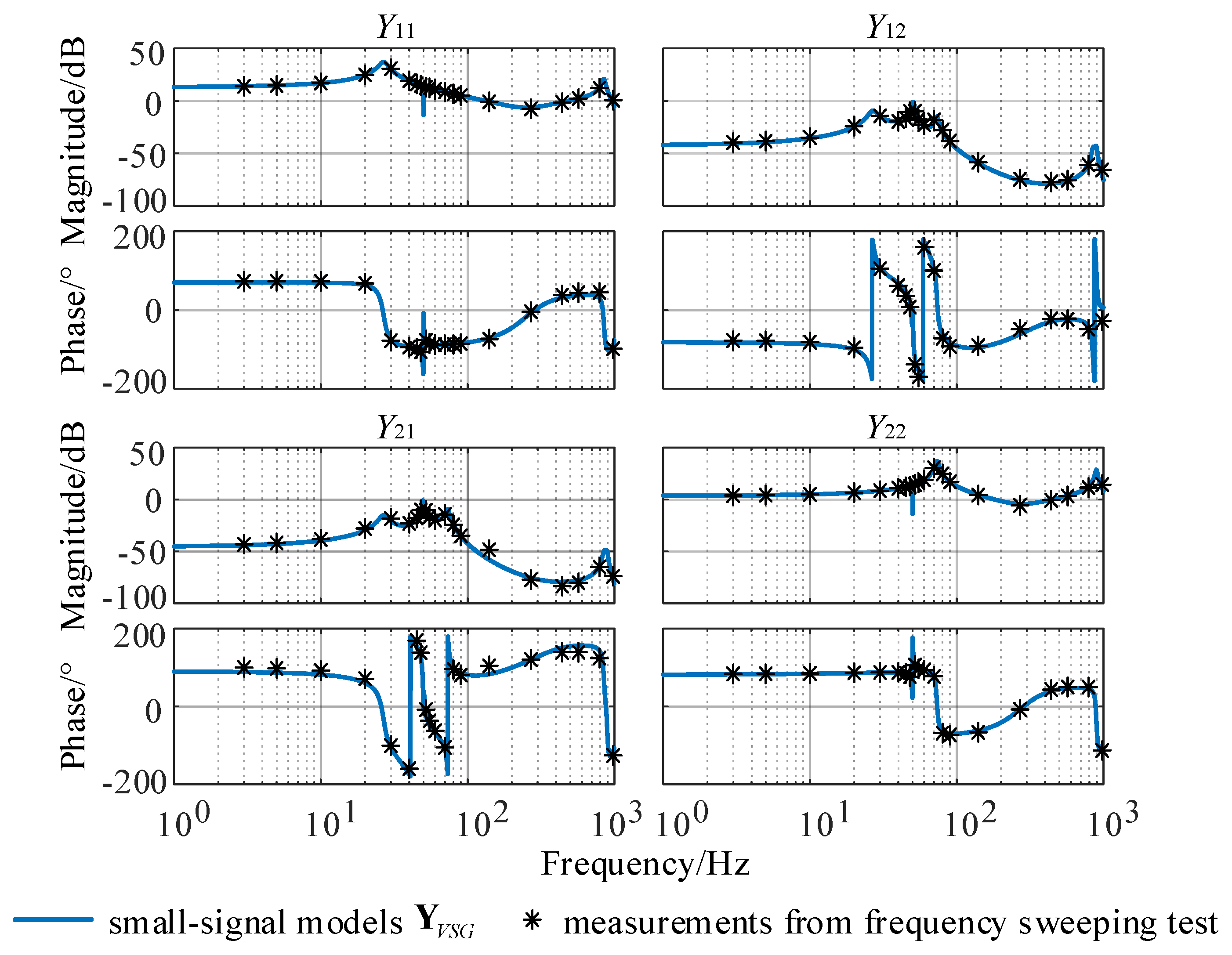
- (1)
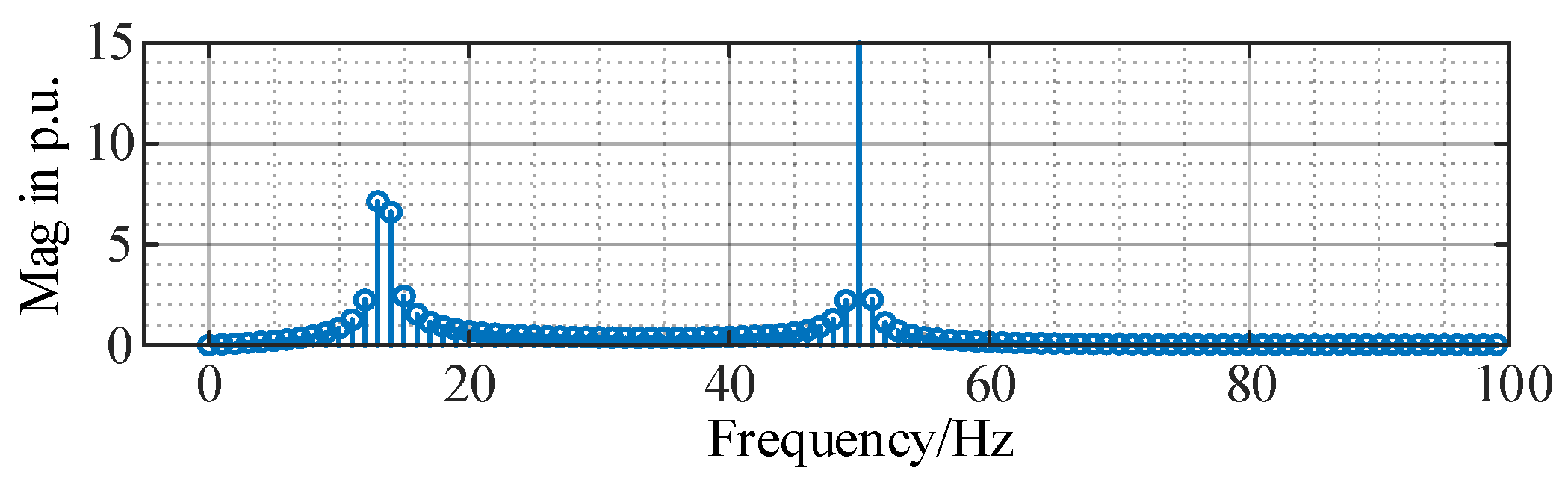
- The analytical small-signal models YVSG in (32) are in accordance with the measured results, which proves that the developed small-signal models of VSG controlled converters with cascaded inner control loop are correct.
- (2)
- The magnitude of off-diagonal elements in the developed admittance matrix YVSG is lower than the diagonal elements except around the fundamental frequency, which means the frequency coupling effect can be ignored except near the fundamental frequency.
3. Impedance Models and Impedance Characteristics of VSG Controlled Converter System
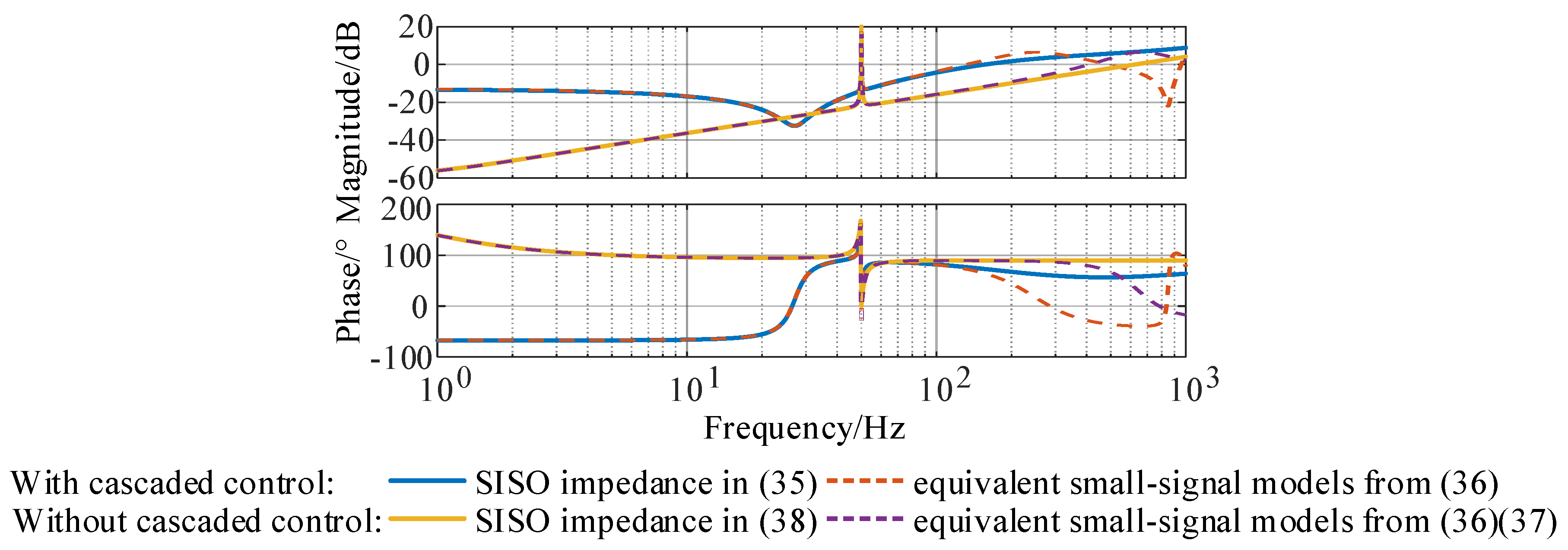
3.1. A Simplified SISO Impedance Model
3.2. Validation of Simplified SISO Impedance Model
3.3. Impedance Characteristics of VSG Controlled Converters with Cascaded Inner Control Loop
- (1)
- The magnitude of VSG controlled impedance increases in the wide-band frequency range with the application of cascaded inner control loop.
- (2)
- The impedance of VSG controlled converters can become capacitive below the fundamental frequency with the application of cascaded inner control loop, while the impedance without cascaded inner control loop is generally inductive.
4. Influence Analysis of the Cascaded Inner Control Loop on Impedance Characteristics
4.1. Causes of Capacitive Impedance Characteristics
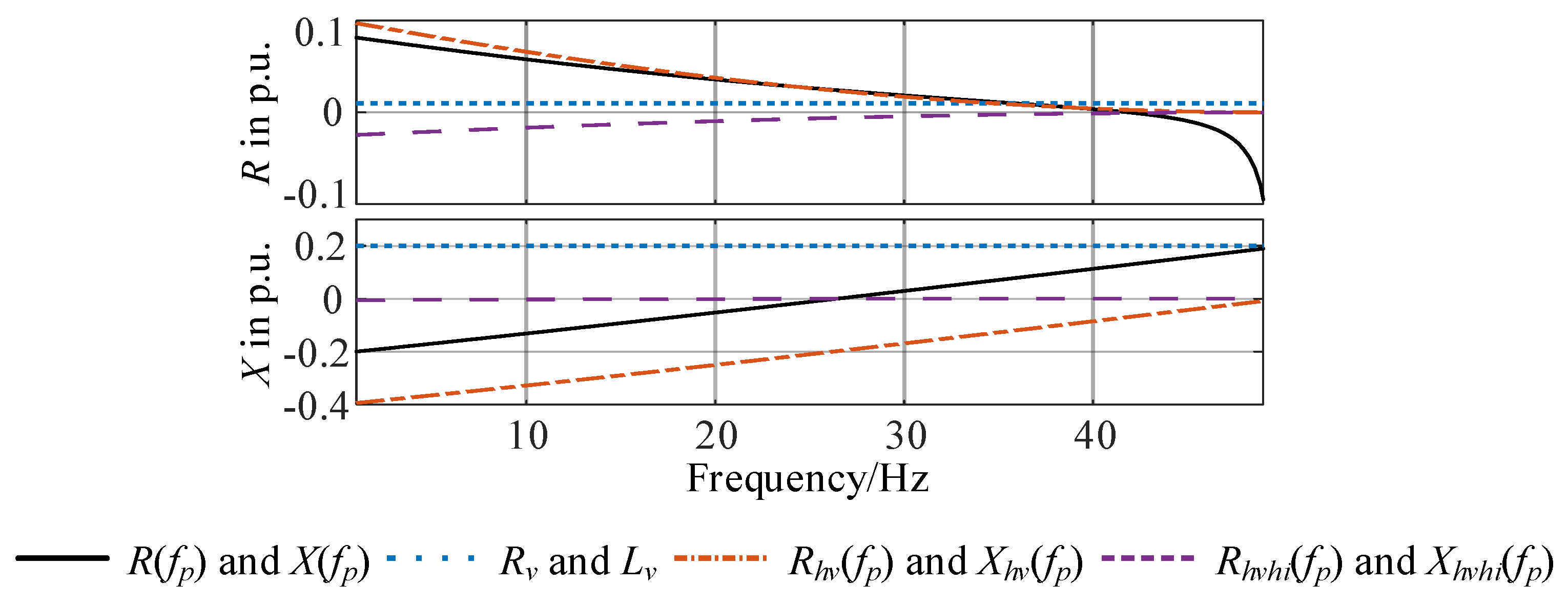
- (1)
- Since ω = 2π(fp − f1) < 0 when fp < f1, the reactance obtained from Xhv(fp) in (38) stays negative below the fundamental frequency, which means that the capacitive impedance characteristics are mainly caused by the effects of voltage controller in the cascaded control loop.
- (2)
- The resistance obtained from Rhvhi(fp) in (37) are negative below the fundamental frequency, which means the control effects of the cascaded voltage–current controller tend to cause negative resistance in this frequency range.
- (3)
- The resistance and reactance obtained from the Rv and Lv are both positive, which means the virtual impedance control can both reduce negative resistive and capacitive characteristics by choosing positive virtual resistance and virtual inductance.
4.2. Influence of Control Parameters in Cascaded Inner Control Loop
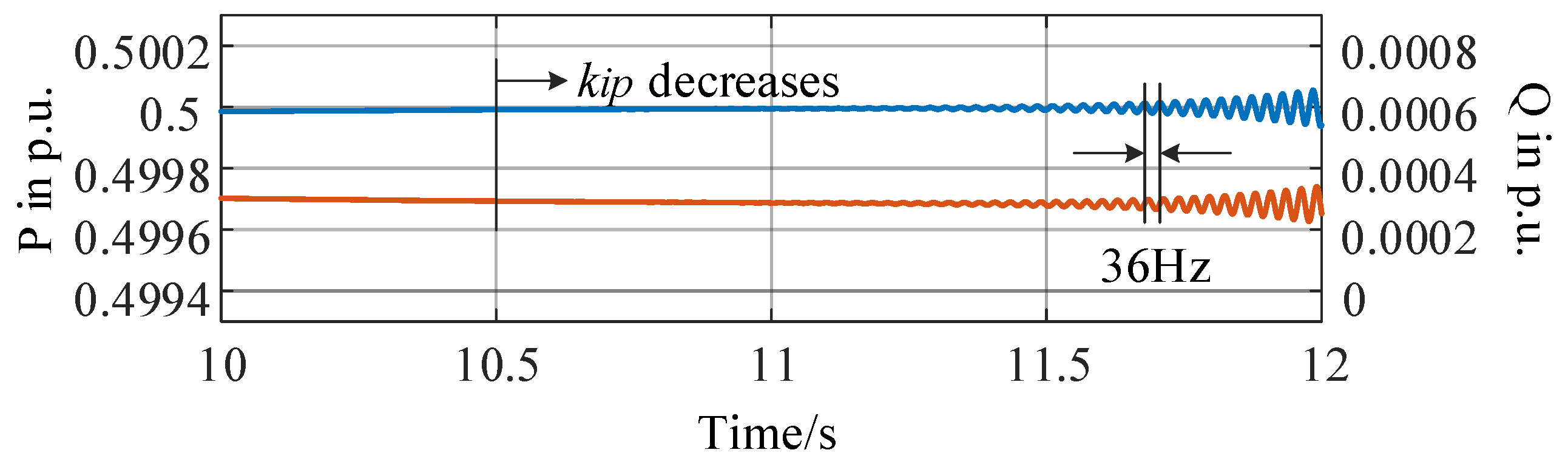
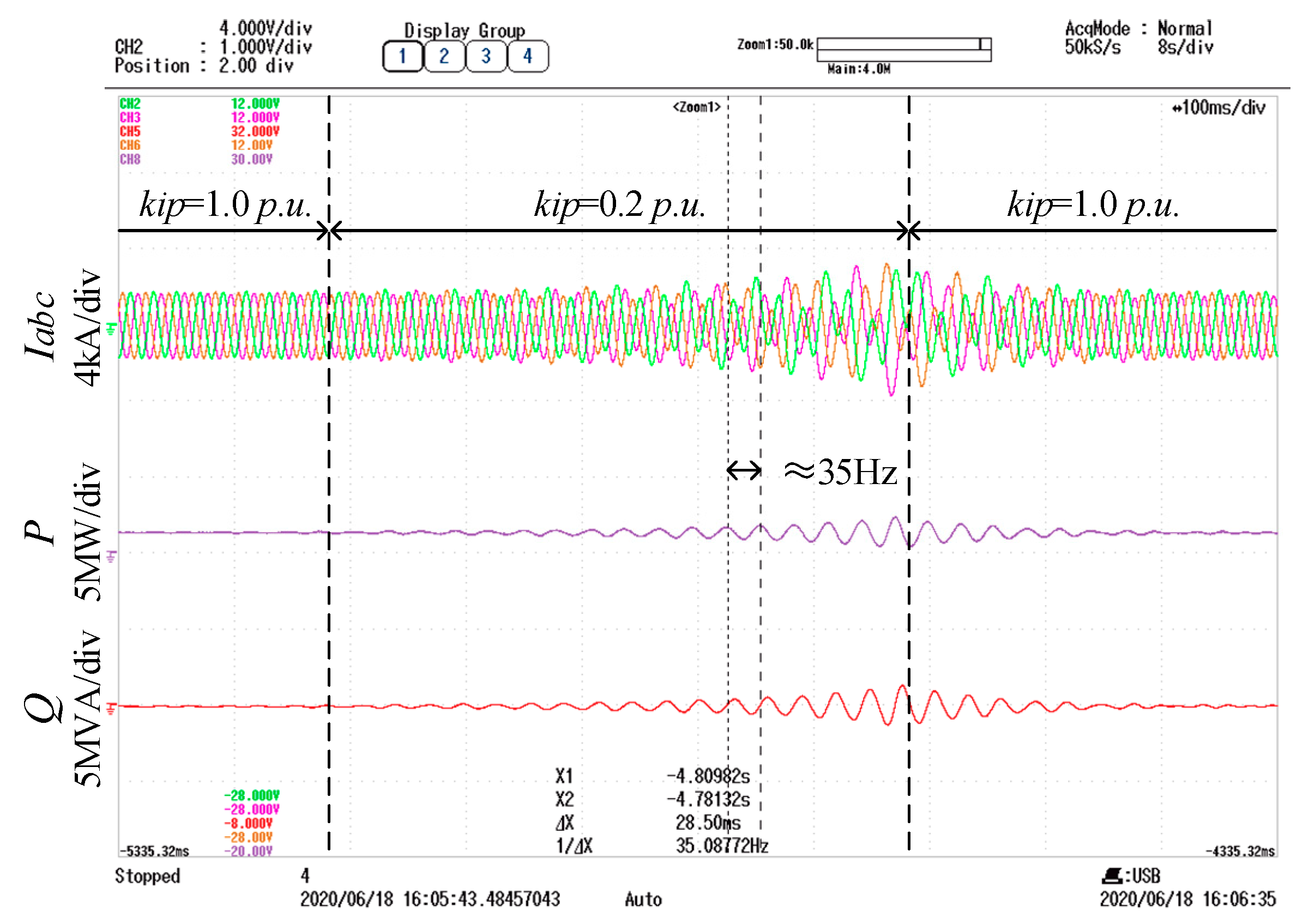
- (1)
- The negative resistive characteristics below the fundamental frequency can be reduced by increasing kip and kvp, or by decreasing kvi, and the influencing ability of kii is as not significant as the other control parameters in cascaded control loop.
- (2)
- The capacitive impedance characteristics below the fundamental frequency can be reduced by increasing kvp and kvi, and the influence ability of current controller is not as significant as the voltage controller.
5. Impedance-Based System Stability Analysis of VSG Controlled Converters with Weak Grid
5.1. Possible System Instability with Different Weak Grid
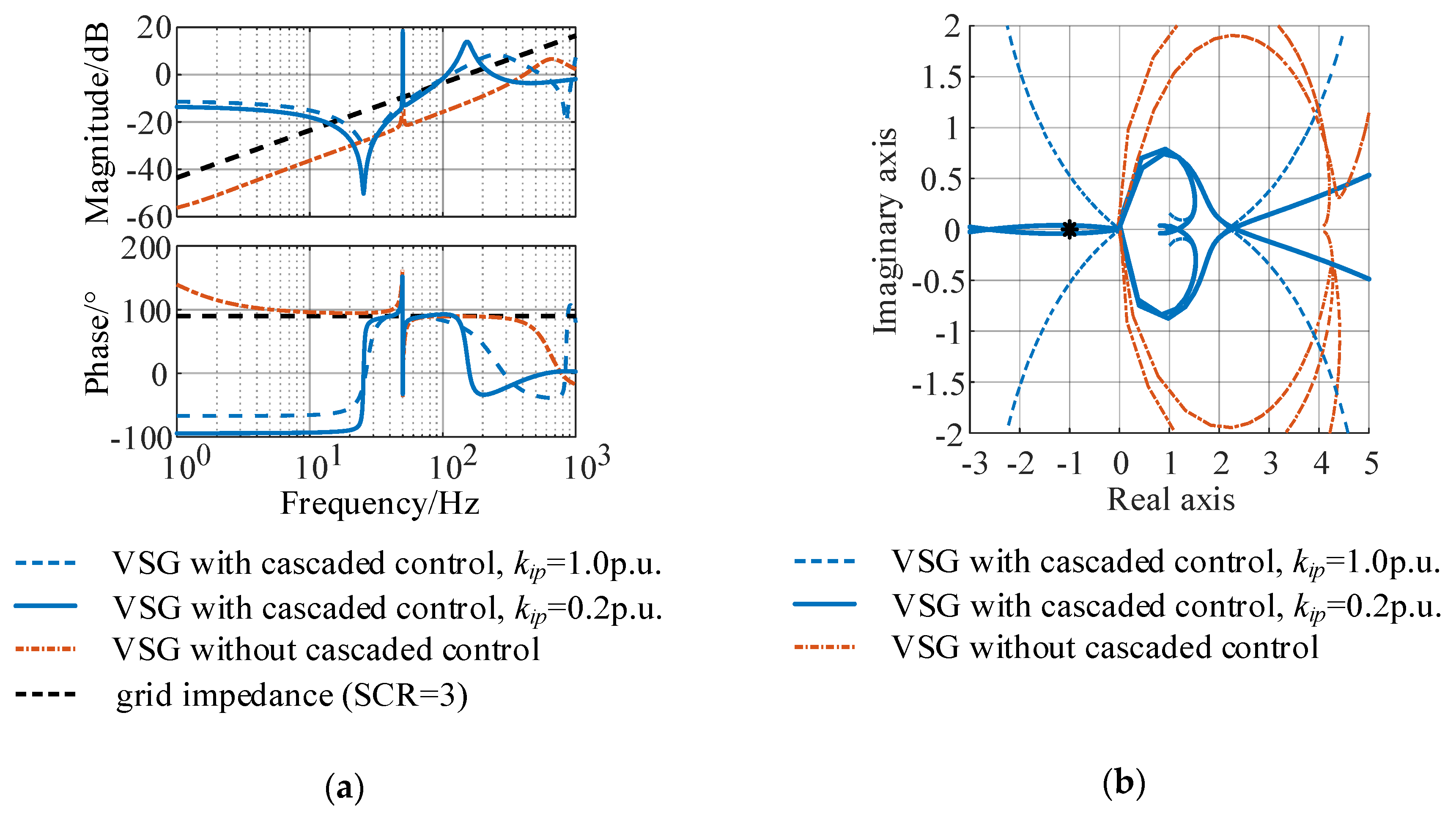
- (1)
- When connecting with the inductive weak grid, the capacitive impedances characteristics that shaped by the cascaded inner control loop may be incompatible with the inductive weak grid, especially when there is negative resistance in the capacitive frequency range.
- (2)
- When connecting with the series-compensated weak grid, the inductive impedance characteristics of converters may be unmatched with the capacitive grid, while the capacitive impedances characteristics shaped by the cascaded inner control loop can match the capacitive grid better.
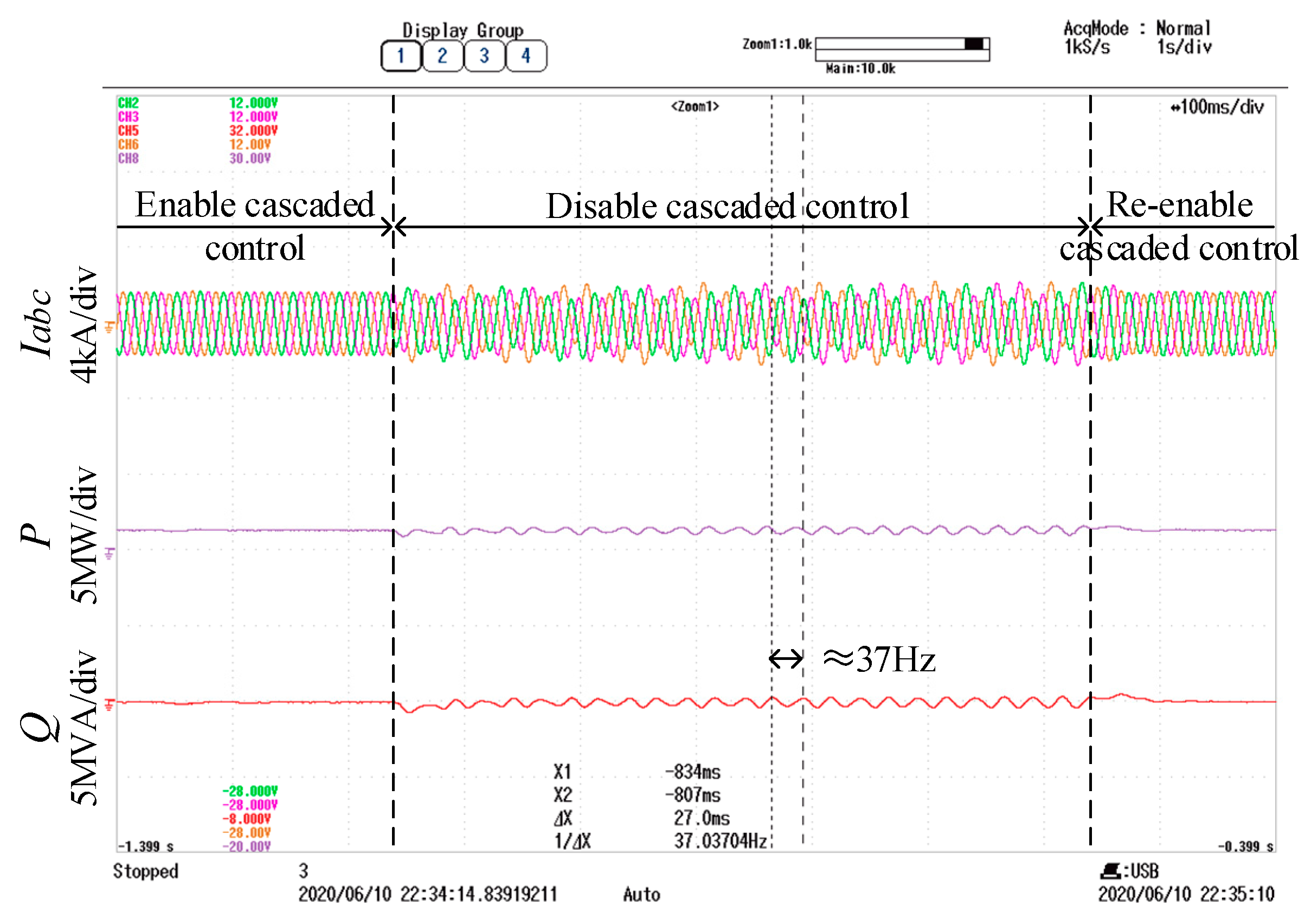
5.2. System Instability with Inductive Weak Grid—A Case Study
5.3. System Instability with Series-Compensated Weak Grid—A Case Study
6. Conclusions
- (1)
- The impedance characteristics of VSG controlled converters with cascaded inner control loop can become capacitive below the fundamental frequency, which is mainly caused by the voltage controller in the cascaded control loop. The cascaded voltage and current controller with the virtual impedance can also shape the capacitive and negative resistive characteristics in this frequency range.
- (2)
- The capacitive impedance characteristics can benefit the compatibility of converters operated with the series-compensated weak grid but may deteriorate the system stability with the inductive weak grid. Possible system instabilities can be avoided by increasing control parameter kvp, kip, and virtual resistance Rv to reduce negative resistance in the capacitive frequency range.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| V, I, E | voltage, current, and modulation signals |
| P, Q, |V| | active power, reactive power, and voltage magnitude |
| abc, dq, dc | subscripts indicating variables are in a/b/c-phase, on d/q-axis, or on dc side |
| ref | subscript indicating variables are reference variables |
| Lf, Cf, Rf | filter inductor, filter capacitor, and filter resistor of the converter |
| Vg, Zg | grid voltage and grid impedance |
| ψf | virtual back electrical magnetic field |
| θ | virtual rotor angular position |
| ωpu | per unit angular speed |
| ωg,pu | per unit angular frequency of the connected grid |
| J | inertial constant |
| Dp | damping coefficient of active power |
| Dq | voltage droop coefficient |
| K | integral coefficient of reactive power controller |
| Lv, Rv | virtual inductor and virtual resistor |
| KFFi, KFFv | feedforward gain of current and voltage |
| kvp, kvi | proportional and integral coefficient of cascaded voltage controller |
| kip, kii | proportional and integral coefficient of cascaded current controller |
| Hv(s), Hi(s) | transfer functions of the cascaded voltage and current controller |
| bold variables with symbol ^ above indicating variables are perturbed frequency components | |
| 1, p, p2 | subscripts indicating components at fundamental frequency, frequency fp, and frequency fp − 2f1 |
| Gx2y | small-signal blocks to describe the small-signal relationships between variables x and y |
| Tm,n | abbreviations of transfer matrices between variables m and n |
| YVSG | small-signal models of VSG controlled converters in 2 × 2 order admittance matrix |
| Ycf | 2 × 2 order admittance matrix model of the filter resistor Rf and filter capacitor Cf |
| / | SISO impedance model for VSG controlled converters with/without the cascaded control loop |
| / | equivalent admittance/impedance of the small-signal models |
| VSG | virtual synchronous generator |
| SISO | single-in-single-out |
| CHIL | control-hardware-in-loop |
| SG | synchronous generator |
| REGS | renewable energy generation system |
| PLL | phase-locked loop |
| EMF | virtual back electrical magnetic field |
| PCC | point of common coupling |
Appendix A
References
- Beck, H.-P.; Hesse, R. Virtual synchronous machine. In Proceedings of the 9th International Conference on Electrical Power Quality and Utilization, Barcelona, Spain, 9–11 October 2007; pp. 1–6. [Google Scholar]
- Subsynchronous Resonance Working Group of the System Dynamic Performance Subcommittee. Reader’s guide to subsynchronous resonance. IEEE Trans. Power Syst. 1992, 7, 150–157. [Google Scholar] [CrossRef]
- Liu, H.; Xie, X.; He, J.; Xu, T.; Yu, Z.; Wang, C.; Zhang, C. Subsynchronous interaction between direct-drive PMSG based wind farms and weak ac grid. IEEE Trans. Power Syst. 2017, 32, 4708–4720. [Google Scholar] [CrossRef]
- Shu, D.; Xie, X.; Rao, H.; Gao, X.; Jiang, Q.; Huang, Y. Sub- and super- synchronous interactions between STATCOMs and weak AC/DC transmissions with series compensations. IEEE Trans. Power Electron. 2018, 33, 7424–7437. [Google Scholar] [CrossRef]
- Chen, X.; Zhang, Y.; Wang, S.; Chen, J.; Gong, C. Impedance-phased dynamic control method for grid-connected inverters in a weak grid. IEEE Trans. Power Electron. 2017, 32, 274–283. [Google Scholar] [CrossRef]
- Zhang, B.; Du, X.; Zhao, J.; Zhou, J.; Zou, X. Impedance modeling and stability analysis of a three-phase three-level NPC inverter connected to the grid. CSEE J. Power Energy Syst. 2020, 6, 270–278. [Google Scholar]
- Sun, J. Impedance-based stability criterion for grid-connected inverters. IEEE Trans. Power Electron. 2011, 26, 3075–3078. [Google Scholar] [CrossRef]
- Wu, H.; Ruan, X.; Yang, D.; Chen, X.; Zhao, W.; Lv, Z.; Zhong, Q. Small-signal modeling and parameters design for virtual synchronous generators. IEEE Trans. Ind. Electron. 2016, 63, 4292–4303. [Google Scholar] [CrossRef]
- Mo, O.; D’Acro, S.; Suul, J.A. Evaluation of virtual synchronous machines with dynamic or quasi-stationary machine models. IEEE Trans. Ind. Electron. 2017, 64, 5952–5962. [Google Scholar] [CrossRef]
- Du, W.; Fu, Q.; Wang, H.F. Power system small-signal angular stability affected by virtual synchronous generators. IEEE Trans. Power Syst. 2019, 34, 3209–3219. [Google Scholar] [CrossRef]
- Li, G.; Chen, Y.; Luo, A.; He, Z.; Wang, H.; Zhu, Z.; Wu, W.; Zhou, L. Analysis and mitigation of subsynchronous resonance in series-compensated grid-connected system controlled by a virtual synchronous generator. IEEE Trans. Power Electron. 2020, 35, 11096–11107. [Google Scholar] [CrossRef]
- Yu, Y.; Zhang, M.; Antoine, M.; Li, H. Analysis of synchronous frequency resonance in VSG based on the sequence impedance models. In Proceedings of the 22nd International Conference on Electrical Machines and Systems, Harbin, China, 11–14 August 2019; pp. 1–6. [Google Scholar]
- Zhang, X.; Chen, J.; Chen, X. Impedance modeling and stability analysis of weak-grid interfaced single-phase VSG. In Proceedings of the 22nd International Conference on Electrical Machines and Systems, Harbin, China, 11–14 August 2019; pp. 1–6. [Google Scholar]
- Wu, W.; Zhou, L.; Chen, Y.; Luo, A.; Dong, Y. Sequence-impedance-based stability comparison between VSGs and traditional grid-connected inverters. IEEE Trans. Power Electron. 2019, 34, 46–52. [Google Scholar] [CrossRef]
- Zhong, Q.; Weiss, G. Synchronverters: Inverters that mimic synchronous generators. IEEE Trans. Ind. Electron. 2011, 58, 1259–1267. [Google Scholar] [CrossRef]
- Wang, X.; Chen, L.; Sun, D.; Zhang, L.; Nian, H. A modified self-synchronized synchronverter in unbalanced power grids with balanced currents and restrained power ripples. Energies 2019, 12, 923. [Google Scholar] [CrossRef]
- D’Arco, S.; Suul, J.A. Virtual synchronous machines—Classification of implementations and analysis of equivalence to droop controllers for microgrids. In Proceedings of the 2013 IEEE Grenoble Conference, Grenoble, France, 16–20 June 2013; pp. 1–7. [Google Scholar]
- D’Arco, S.; Suul, J.A.; Fosso, O.B. A virtual synchronous machine implementation for distributed control of power converters in smart grids. Electr. Power Syst. Res. 2015, 122, 180–197. [Google Scholar] [CrossRef]
- Hou, X.; Sun, Y.; Zhang, X.; Lu, J.; Wang, P.; Guerrero, J.M. Improvement of frequency regulation in VSG-based ac microgrid via adaptive virtual inertia. IEEE Trans. Power Electron. 2020, 35, 1589–1602. [Google Scholar] [CrossRef]
- Chen, J.; Liu, M.; Milano, F.; O’Donnell, T. Placement of virtual synchronous generator controlled electric storage combined with renewable generation. In Proceedings of the IEEE Milan PowerTech, Milan, Italy, 23–27 June 2019; pp. 1–6. [Google Scholar]
- Unamuno, E.; Rygg, A.; Amin, M.; Barrena, J.A. Impedance-based stability evaluation of virtual synchronous machine implementations in converter controllers. In Proceedings of the International Power Electronics Conference, Niigata, Japan, 20–24 May 2018; pp. 759–766. [Google Scholar]
- Li, C.; Liang, J.; Cipcigan, L.M.; Ming, W.; Colas, F.; Guillaud, X. DQ impedance stability analysis for the power-controlled grid-connected inverter. IEEE Trans. Energy Convers. 2020, 1. [Google Scholar] [CrossRef]
- Xu, Y.; Nian, H.; Xu, G.; Qiu, J. Cross-coupling over frequency and sequence in impedance modelling of grid-connected inverter. J. Eng. 2017, 2017, 990–995. [Google Scholar] [CrossRef]
- Xu, Y.; Nian, H.; Wang, T.; Chen, L.; Zheng, T. Frequency coupling characteristic modeling and stability analysis of doubly fed induction generator. IEEE Trans. Energy Convers. 2018, 33, 1475–1486. [Google Scholar] [CrossRef]
- Nian, H.; Xu, Y.; Chen, L.; Zhu, M. Modeling and analysis of dc-link dynamics in DFIG system with an indicator function. IEEE Access 2019, 7, 125401–125412. [Google Scholar] [CrossRef]
- Zou, X.; Du, X.; Tai, H. Two-variable admittance model for D-PMSG-based wind turbine and stability criterion based on magnitude and phase contour plot. IEEE Trans. Power Electron. 2020, 35, 1484–1498. [Google Scholar] [CrossRef]
- Liu, W.; Xie, X.; Zhang, X.; Li, X. Frequency-coupling admittance modeling of converter-based wind turbine generators and the control-hardware-in-the-loop validation. IEEE Trans. Energy Convers. 2020, 35, 425–433. [Google Scholar] [CrossRef]
- Li, G.; Sun, J. Control hardware-in-the-loop simulation for turbine impedance modeling and verification. In Proceedings of the 16th Wind Integration Workshop, Berlin, Germany, 16–18 October 2017; pp. 1–6. [Google Scholar]




| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Rated voltage, Us | 690 V | Damping coefficient, Dp | 400 |
| Rated power, Sb | 2.75 MW | Voltage reference, |V|ref | 1.0 p.u. |
| Rated frequency, f1 | 50 Hz | Reactive power reference, Qref | 0.0 p.u. |
| Power reference, Pref | 0.5 p.u. | Voltage droop coefficient, Dq | 0.02 |
| Inertial constant, J | 2 s | Integral coefficient, K | 0.5 |
| Filter resistor, Rf | 0.003 p.u. | Voltage controller, kvp, kvi | 0.59 736 |
| Filter inductor, Lf | 0.08 p.u. | Current controller, kip, kii | 1.27 14.3 |
| Filter capacitor, Cf | 0.074 p.u. | Virtual impedance, Lv, Rv | 0.2 0.01 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, Y.; Nian, H.; Wang, Y.; Sun, D. Impedance Modeling and Stability Analysis of VSG Controlled Grid-Connected Converters with Cascaded Inner Control Loop. Energies 2020, 13, 5114. https://doi.org/10.3390/en13195114
Xu Y, Nian H, Wang Y, Sun D. Impedance Modeling and Stability Analysis of VSG Controlled Grid-Connected Converters with Cascaded Inner Control Loop. Energies. 2020; 13(19):5114. https://doi.org/10.3390/en13195114
Chicago/Turabian StyleXu, Yunyang, Heng Nian, Yangming Wang, and Dan Sun. 2020. "Impedance Modeling and Stability Analysis of VSG Controlled Grid-Connected Converters with Cascaded Inner Control Loop" Energies 13, no. 19: 5114. https://doi.org/10.3390/en13195114
APA StyleXu, Y., Nian, H., Wang, Y., & Sun, D. (2020). Impedance Modeling and Stability Analysis of VSG Controlled Grid-Connected Converters with Cascaded Inner Control Loop. Energies, 13(19), 5114. https://doi.org/10.3390/en13195114







