Pore-Scale Analysis of Condensate Blockage Mitigation by Wettability Alteration
Abstract
:1. Introduction
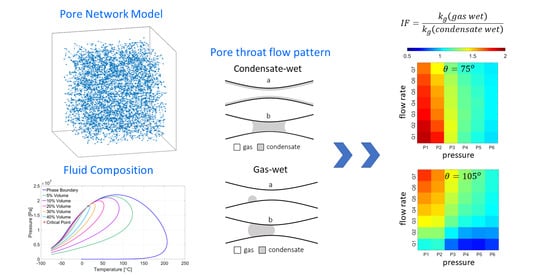
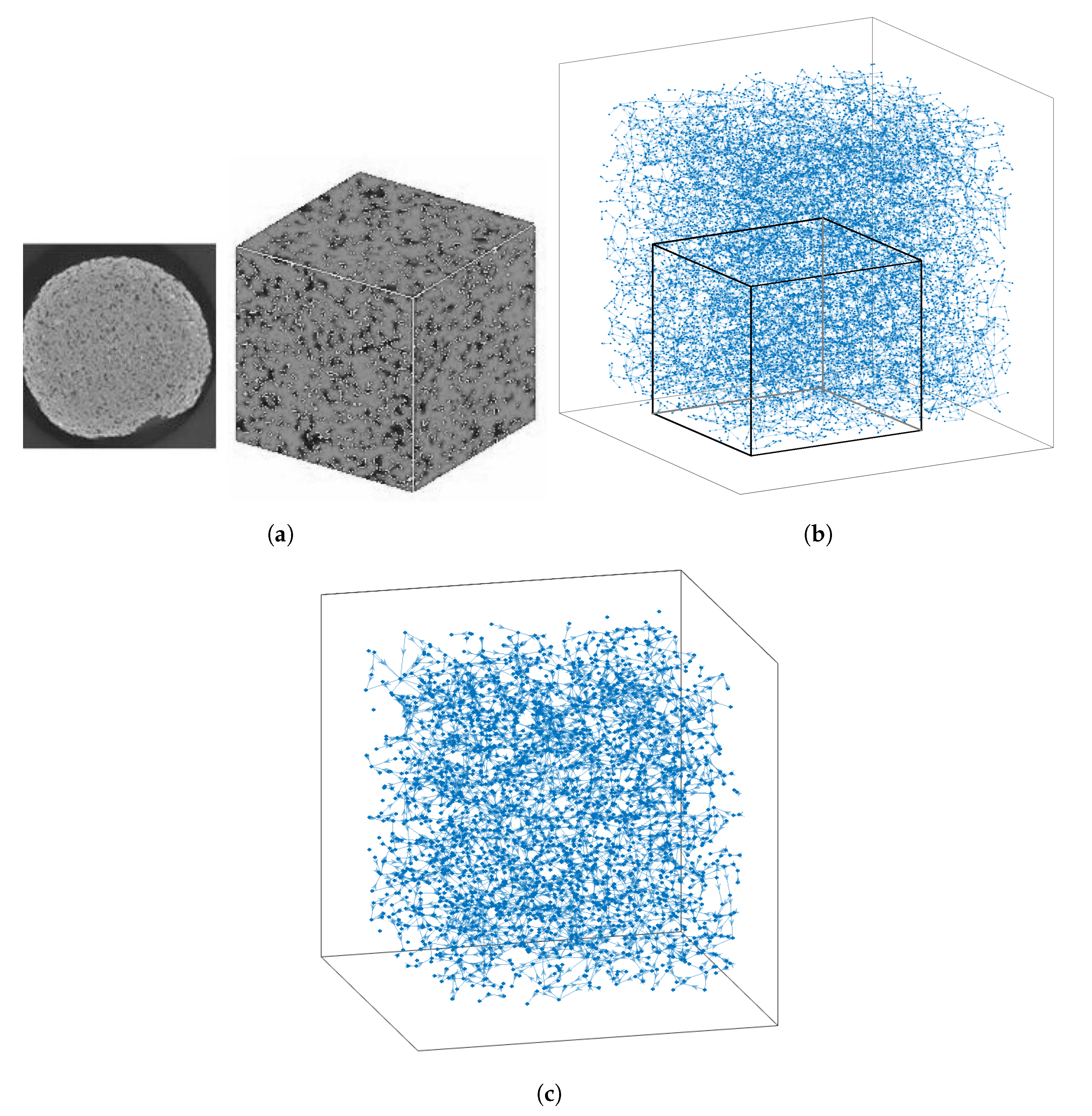
2. Pore-Network Model
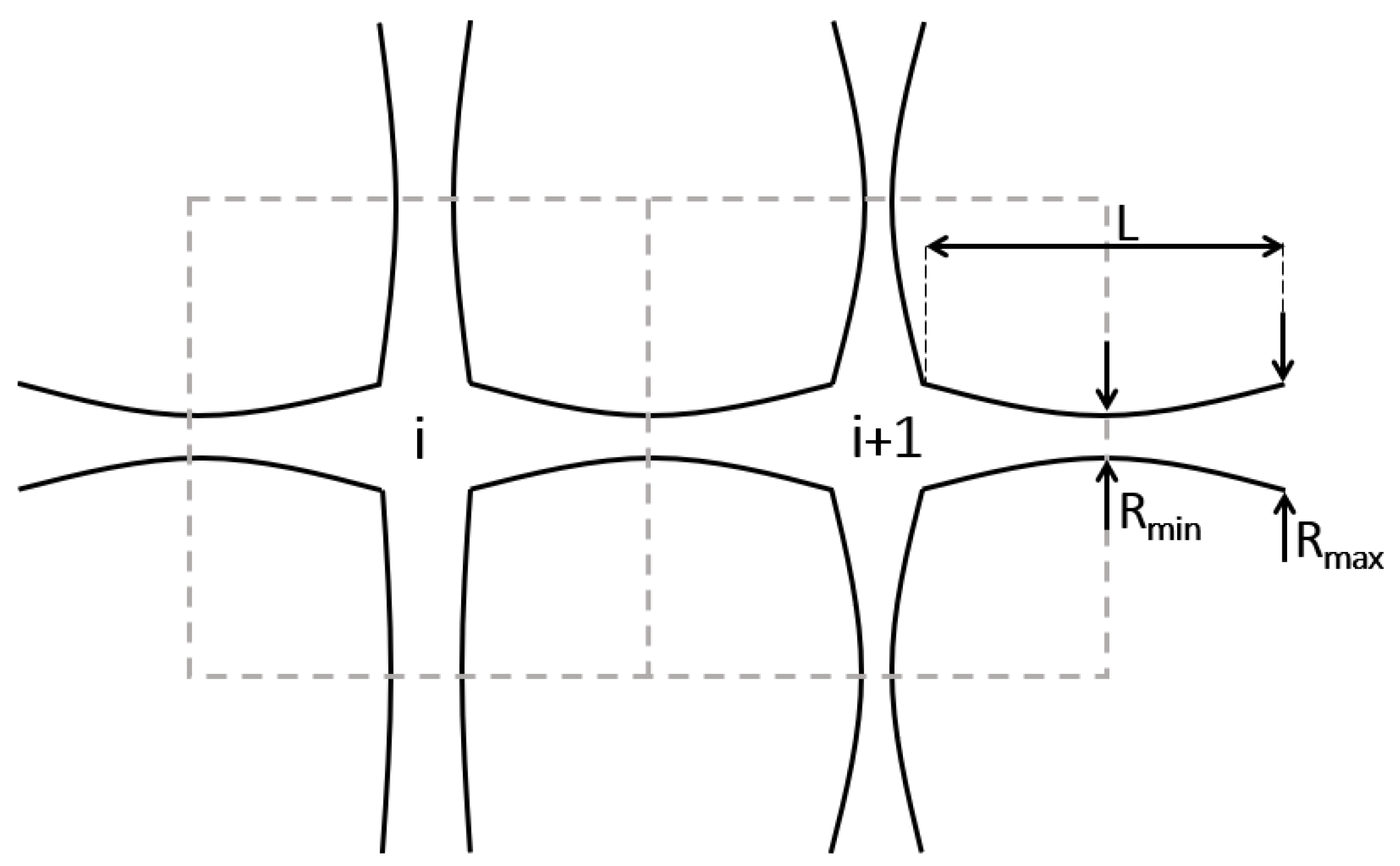
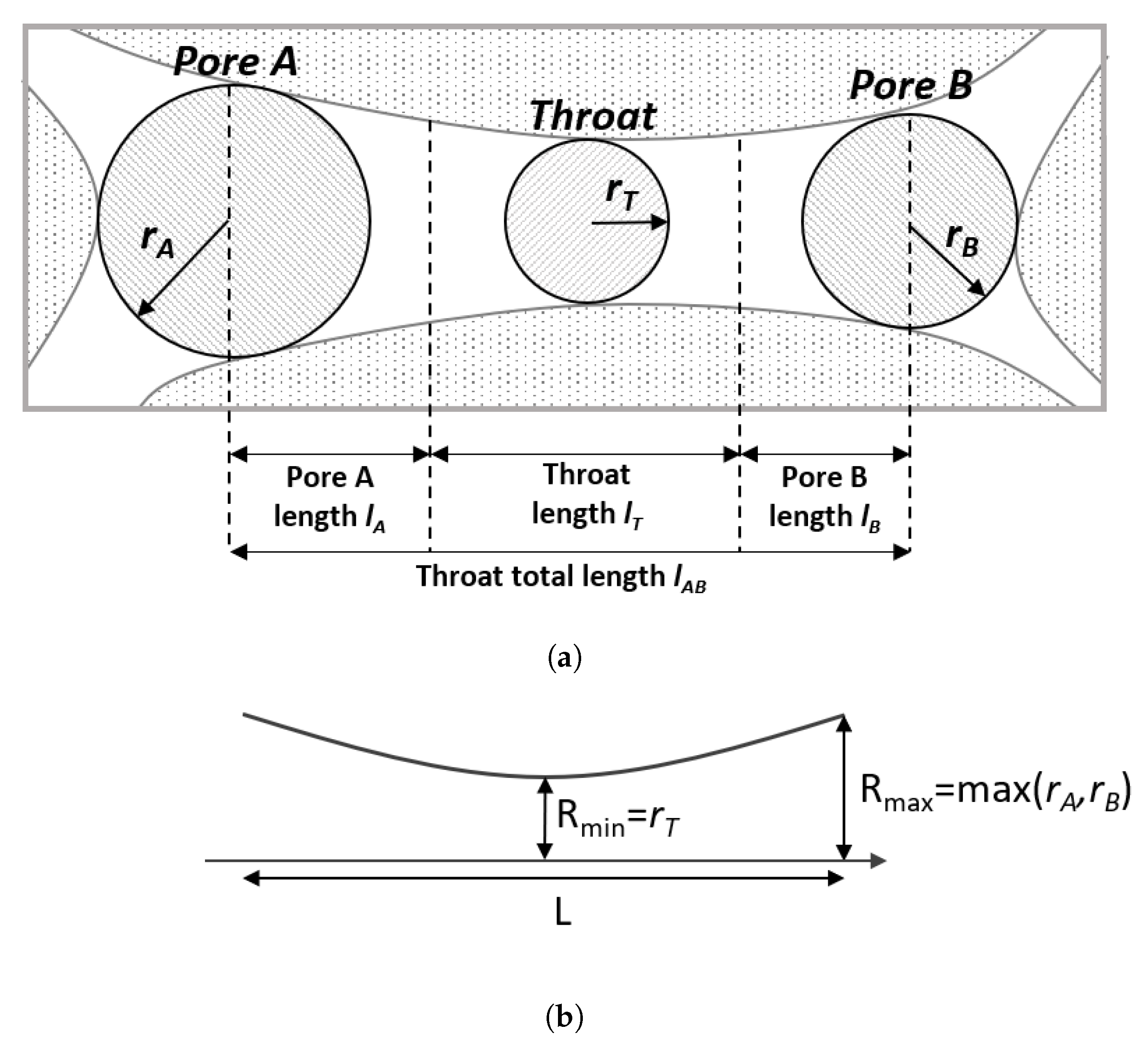
2.1. Pore-Network Geometry
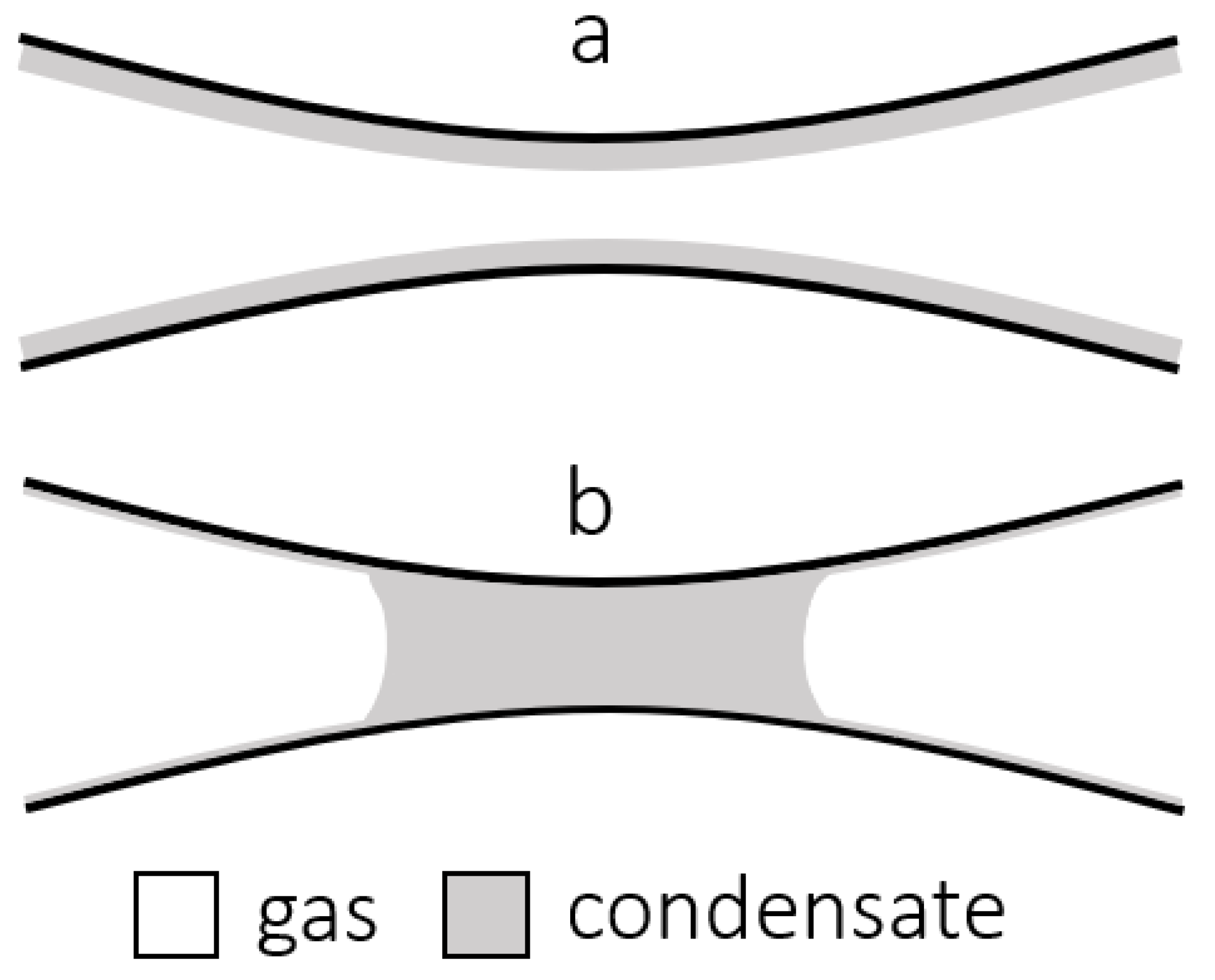
2.2. Two-Phase Flow Patterns
2.2.1. Conductances for Gas and Condensate Flow in Liquid-Wet Media
2.2.2. Conductances for Gas and Condensate Flow in Gas-Wet Media
2.3. Governing Equations
2.3.1. Molar Balance Equation
2.3.2. Volume Consistency Equation
2.3.3. Boundary Conditions
2.4. Model Validation
3. Results
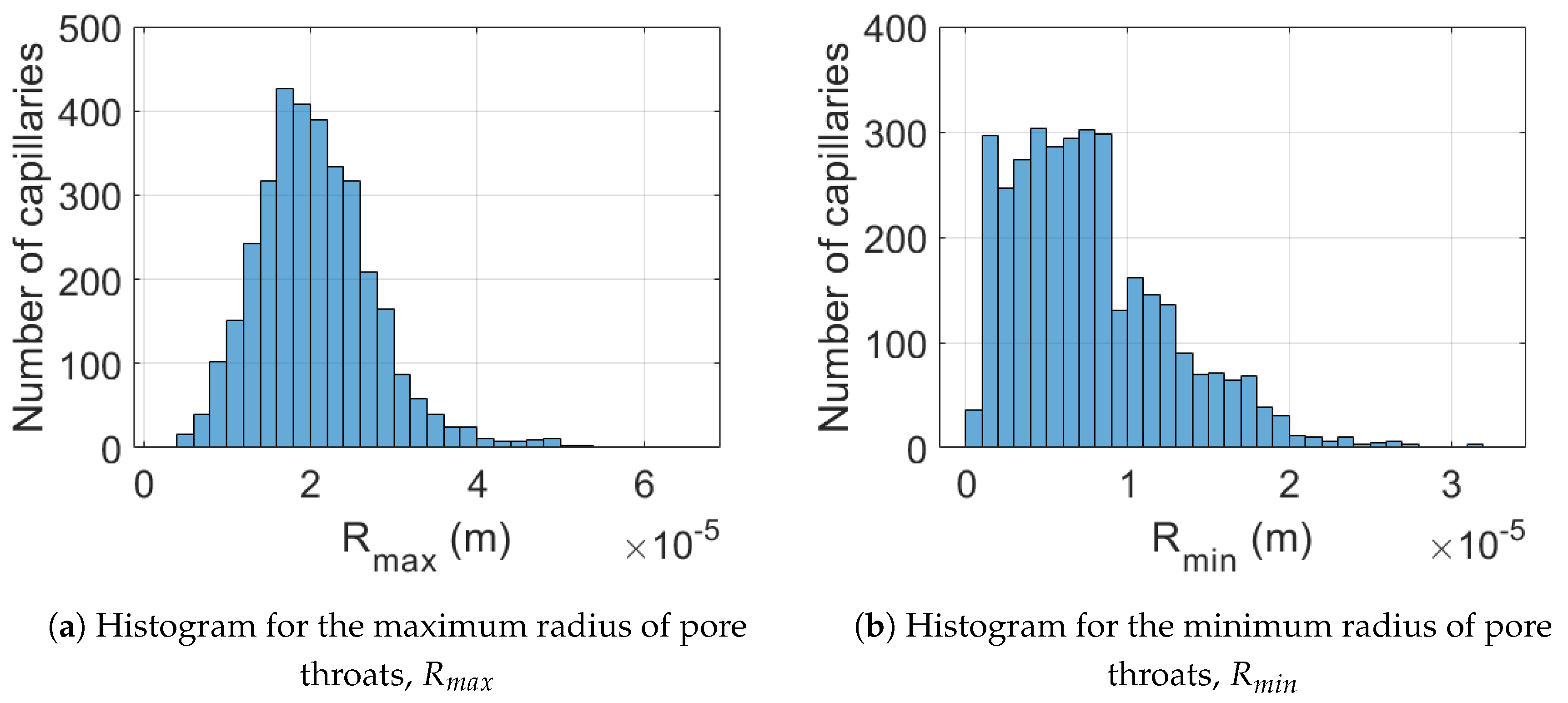
3.1. Pore-Network Description
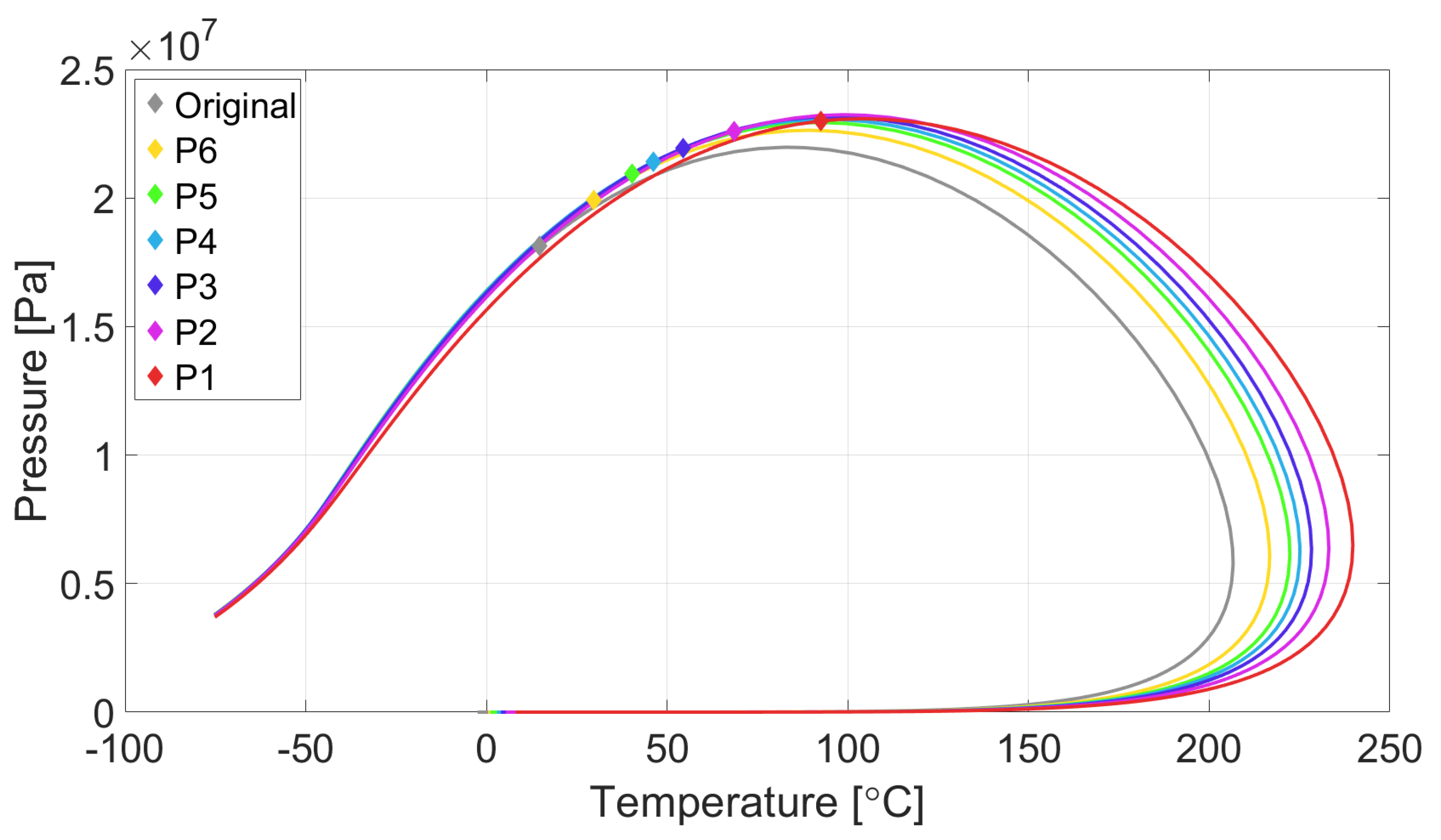
3.2. Fluid
3.3. Flow Conditions
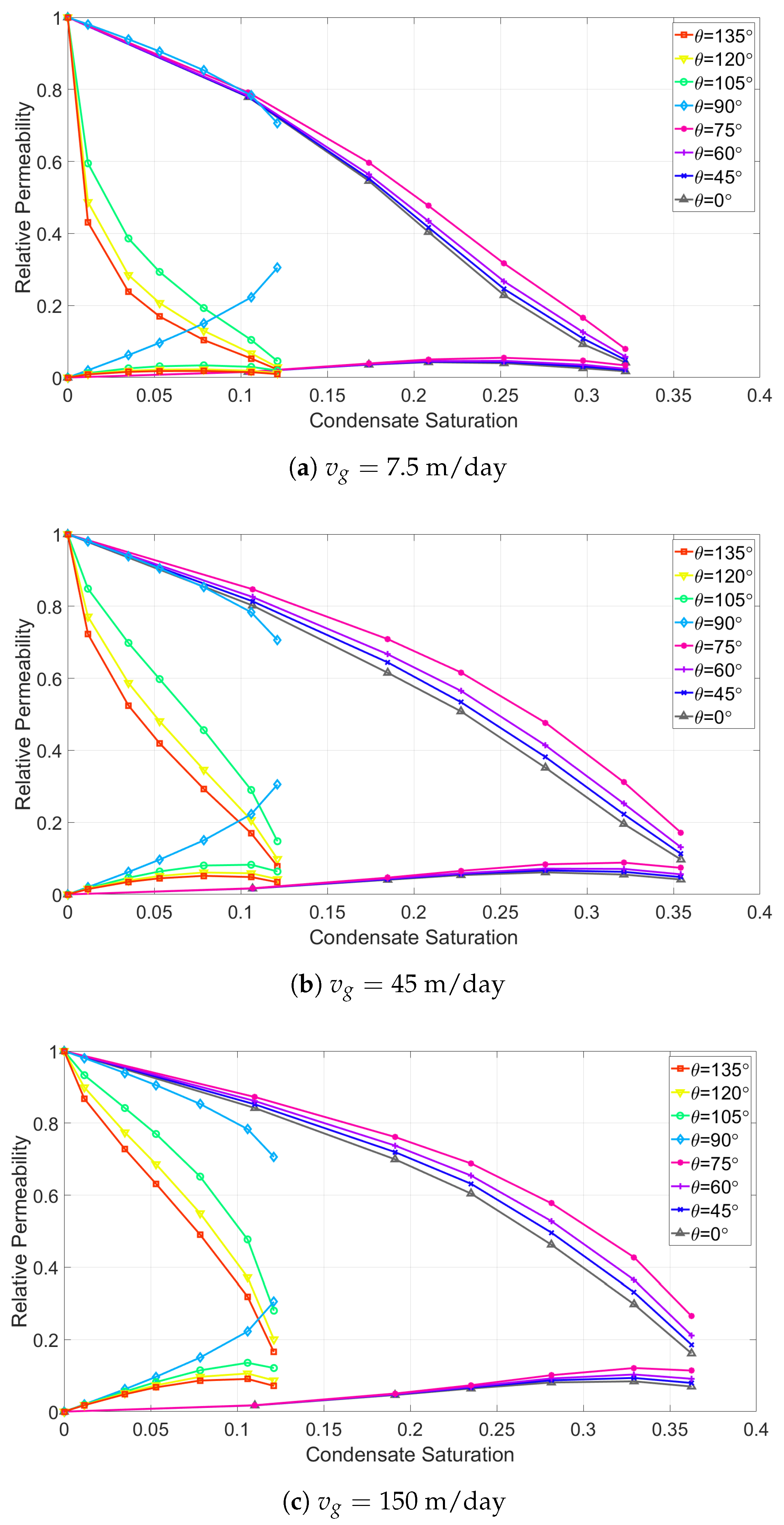
3.4. Relative Permeability Curves
3.5. Improvement Factor
3.6. Liquid Accumulation and Compositional Shift
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bang, V.S.S.; Pope, G.A.; Sharma, M.M.; Baran, J.R., Jr. Development of a successful chemical treatment for gas wells with liquid blocking. In SPE Annual Technical Conference and Exhibition; Society of Petroleum Engineers, 2009; Available online: https://doi.org/10.2118/124977-MS (accessed on 4 September 2020).
- Ahmadi, M.; Sharma, M.M.; Pope, G.; Torres, D.E.; McCulley, C.A.; Linnemeyer, H. Chemical treatment to mitigate condensate and water blocking in gas wells in carbonate reservoirs. SPE Prod. Oper. 2011, 26, 67–74. [Google Scholar] [CrossRef]
- Wu, S.; Firoozabadi, A. Permanent alteration of porous media wettability from liquid-wetting to intermediate gas-wetting. Transp. Porous Media 2010, 85, 189–213. [Google Scholar] [CrossRef]
- Al-Anazi, H.A.; Okasha, T.M.; Haas, M.D.; Ginest, N.H.; Al-Faifi, M. Impact of completion fluids on productivity in Gas/condensate reservoirs. In SPE Production Operations Symposium; Society of Petroleum Engineers, 2005; Available online: https://doi.org/10.2118/94256-MS (accessed on 4 September 2020).
- Fevang, Ø.; Whitson, C. Modeling gas-condensate well deliverability. SPE Reserv. Eng. 1996, 11, 221–230. [Google Scholar] [CrossRef]
- Ganie, K.; Idris, A.K.; Mohshim, D.F.; Sulaiman, W.R.W.; Saaid, I.M.; Malik, A.A. A review on the wettability alteration mechanism in condensate banking removal. J. Pet. Sci. Eng. 2019, 183, 106431. [Google Scholar] [CrossRef]
- Sharma, S.; Sheng, J.J. A comparative study of huff-n-puff gas and solvent injection in a shale gas condensate core. J. Nat. Gas Sci. Eng. 2017, 38, 549–565. [Google Scholar] [CrossRef] [Green Version]
- Hassan, A.; Mahmoud, M.; Al-Majed, A.; Alawi, M.B.; Elkatatny, S.; BaTaweel, M.; Al-Nakhli, A. Gas condensate treatment: A critical review of materials, methods, field applications, and new solutions. J. Pet. Sci. Eng. 2019, 177, 602–613. [Google Scholar] [CrossRef]
- Kewen, L.; Abbas, F. Experimental study of wettability alteration to preferential gas-wetting in porous media and its effects. SPE Reserv. Eval. Eng. 2000, 3, 139–149. [Google Scholar] [CrossRef]
- Ali, N.E.C.; Zoghbi, B.; Fahes, M.; Nasrabadi, H.; Retnanto, A. The impact of near-wellbore wettability on the production of gas and condensate: Insights from experiments and simulations. J. Pet. Sci. Eng. 2019, 175, 215–223. [Google Scholar]
- Franco-Aguirre, M.; Zabala, R.D.; Lopera, S.H.; Franco, C.A.; Cortés, F.B. Interaction of anionic surfactant-nanoparticles for gas-Wettability alteration of sandstone in tight gas-condensate reservoirs. J. Nat. Gas Sci. Eng. 2018, 51, 53–64. [Google Scholar] [CrossRef]
- Li, K.; Liu, Y.; Zheng, H.; Huang, G.; Li, G. Enhanced gas-condensate production by wettability alteration to gas wetness. J. Pet. Sci. Eng. 2011, 78, 505–509. [Google Scholar] [CrossRef]
- Gahrooei, H.R.E.; Ghazanfari, M.H. Toward a hydrocarbon-based chemical for wettability alteration of reservoir rocks to gas wetting condition: Implications to gas condensate reservoirs. J. Mol. Liq. 2017, 248, 100–111. [Google Scholar] [CrossRef]
- Hoseinpour, S.A.; Madhi, M.; Norouzi, H.; Soulgani, B.S.; Mohammadi, A.H. Condensate blockage alleviation around gas-condensate producing wells using wettability alteration. J. Nat. Gas Sci. Eng. 2019, 62, 214–223. [Google Scholar] [CrossRef]
- Noh, M.H.; Firoozabadi, A. Wettability alteration in gas-condensate reservoirs to mitigate well deliverability loss by water blocking. SPE Reserv. Eval. Eng. 2008, 11, 676–685. [Google Scholar] [CrossRef] [Green Version]
- Fahimpour, J.; Jamiolahmady, M. Optimization of fluorinated wettability modifiers for gas/condensate carbonate reservoirs. SPE J. 2015, 20, 729–742. [Google Scholar] [CrossRef]
- Tang, G.Q.; Firoozabadi, A. Wettability alteration to intermediate gas-wetting in porous media at elevated temperatures. Transp. Porous Media 2003, 52, 185–211. [Google Scholar] [CrossRef]
- Fahimpour, J.; Jamiolahmady, M. Impact of gas–condensate composition and interfacial tension on oil-repellency strength of wettability modifiers. Energy Fuels 2014, 28, 6714–6722. [Google Scholar] [CrossRef]
- Wang, Y.; Jin, J.; Ma, L.; Li, L.; Zhao, X. Influence of wettability alteration to preferential gas-wetting on displacement efficiency at elevated temperatures. J. Dispers. Sci. Technol. 2015, 36, 1274–1281. [Google Scholar] [CrossRef]
- Fahes, M.M.; Firoozabadi, A. Wettability alteration to intermediate gas-wetting in gas-condensate reservoirs at high temperatures. SPE J. 2007, 12, 397–407. [Google Scholar] [CrossRef]
- Fahimpour, J.; Jamiolahmady, M. An Improved Understanding of Performance of Wettability Alteration for Condensate Banking Removal Under Steady-State Flow Conditions. In Abu Dhabi International Petroleum Exhibition and Conference; Society of Petroleum Engineers, 2015; Available online: https://doi.org/10.2118/177901-MS (accessed on 4 September 2020).
- Jamiolahmady, M.; Danesh, A.; Tehrani, D.H.; Duncan, D.B. Positive effect of flow velocity on gas–condensate relative permeability: Network modelling and comparison with experimental results. Transp. Porous Media 2003, 52, 159–183. [Google Scholar] [CrossRef]
- Henderson, G.D.; Danesh, A.; Tehrani, D.; Peden, J. The effect of velocity and interfacial tension on relative permeability of gas condensate fluids in the wellbore region. J. Pet. Sci. Eng. 1997, 17, 265–273. [Google Scholar] [CrossRef]
- Henderson, G.D.; Danesh, A.; Al-Kharusi, B.; Tehrani, D. Generating reliable gas condensate relative permeability data used to develop a correlation with capillary number. J. Pet. Sci. Eng. 2000, 25, 79–91. [Google Scholar] [CrossRef]
- Whitson, C.H.; Fevang, Ø.; Sævareid, A. Gas condensate relative permeability for well calculations. Transp. Porous Media 2003, 52, 279–311. [Google Scholar] [CrossRef]
- Kumar, V.; Pope, G.A.; Sharma, M.M. Improving the gas and condensate relative permeability using chemical treatments. In SPE Gas Technology Symposium; Society of Petroleum Engineers, 2006; Available online: https://doi.org/10.2118/100529-MS (accessed on 4 September 2020).
- Bang, V.S.S.; Pope, G.; Sharma, M.M.; Baran, J.; Ahmadi, M. A new solution to restore productivity of gas wells with condensate and water blocks. SPE Reserv. Eval. Eng. 2010, 13, 323–331. [Google Scholar] [CrossRef]
- Li, K.; Firoozabadi, A. Phenomenological modeling of critical condensate saturation and relative permeabilities in gas/condensate systems. SPE J. 2000, 5, 138–147. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.; Mohanty, K.K. Pore-network model of flow in gas/condensate reservoirs. SPE J. 2000, 5, 426–434. [Google Scholar] [CrossRef]
- Bustos, C.I.; Toledo, P.G. Pore-level modeling of gas and condensate flow in two-and three-dimensional pore networks: Pore size distribution effects on the relative permeability of gas and condensate. Transp. Porous Media 2003, 53, 281–315. [Google Scholar] [CrossRef]
- Momeni, A.; Dadvar, M.; Hekmatzadeh, M.; Dabir, B. 3D pore network modeling and simulation for dynamic displacement of gas and condensate in wellbore region. Int. J. Multiph. Flow 2017, 97, 147–156. [Google Scholar] [CrossRef]
- Chalbaud, C.; Robin, M.; Bekri, S.; Egermann, P. Wettability impact on CO2 storage in aquifers: Visualisation and quantification using micromodel tests, pore network model and reservoir simulations. In Proceedings of the International Symposium of the Society of Core Analysts, Calgary, AB, Canada, 10–13 September 2007; pp. 10–12. [Google Scholar]
- Chapuis, O.; Prat, M. Influence of wettability conditions on slow evaporation in two-dimensional porous media. Phys. Rev. E 2007, 75, 046311. [Google Scholar] [CrossRef]
- Chraibi, H.; Prat, M.; Chapuis, O. Influence of contact angle on slow evaporation in two-dimensional porous media. Phys. Rev. E 2009, 79, 026313. [Google Scholar] [CrossRef] [Green Version]
- Sinha, P.K.; Mukherjee, P.P.; Wang, C.Y. Impact of GDL structure and wettability on water management in polymer electrolyte fuel cells. J. Mater. Chem. 2007, 17, 3089–3103. [Google Scholar] [CrossRef]
- Kuttanikkad, S.P.; Prat, M.; Pauchet, J. Pore-network simulations of two-phase flow in a thin porous layer of mixed wettability: Application to water transport in gas diffusion layers of proton exchange membrane fuel cells. J. Power Sources 2011, 196, 1145–1155. [Google Scholar] [CrossRef]
- Shao, C.; Yang, Z.; Zhou, G.; Lu, G. Pore network modeling of water block in low permeability reservoirs. Pet. Sci. 2010, 7, 362–366. [Google Scholar] [CrossRef] [Green Version]
- Huang, X.; Bandilla, K.W.; Celia, M.A. Multi-physics pore-network modeling of two-phase shale matrix flows. Transp. Porous Media 2016, 111, 123–141. [Google Scholar] [CrossRef]
- Fang, C.; Steinbrenner, J.E.; Wang, F.M.; Goodson, K.E. Impact of wall hydrophobicity on condensation flow and heat transfer in silicon microchannels. J. Micromech. Microeng. 2010, 20, 045018. [Google Scholar] [CrossRef]
- Chen, Y.; Cheng, P. Condensation of steam in silicon microchannels. Int. Commun. Heat Mass Transf. 2005, 32, 175–183. [Google Scholar] [CrossRef]
- Cubaud, T.; Ulmanella, U.; Ho, C.M. Two-phase flow in microchannels with surface modifications. Fluid Dyn. Res. 2006, 38, 772. [Google Scholar] [CrossRef]
- Wu, T.; Djilali, N. Experimental investigation of water droplet emergence in a model polymer electrolyte membrane fuel cell microchannel. J. Power Sources 2012, 208, 248–256. [Google Scholar] [CrossRef] [Green Version]
- Santos, R.M.; Kawaji, M. Developments on wetting effects in microfluidic slug flow. Chem. Eng. Commun. 2012, 199, 1626–1641. [Google Scholar] [CrossRef]
- Kawahara, A.; Chung, P.Y.; Kawaji, M. Investigation of two-phase flow pattern, void fraction and pressure drop in a microchannel. Int. J. Multiph. Flow 2002, 28, 1411–1435. [Google Scholar] [CrossRef]
- Chung, P.Y.; Kawaji, M. The effect of channel diameter on adiabatic two-phase flow characteristics in microchannels. Int. J. Multiph. Flow 2004, 30, 735–761. [Google Scholar] [CrossRef]
- Kawaji, M.; Chung, P.Y. Adiabatic gas–liquid flow in microchannels. Microscale Thermophys. Eng. 2004, 8, 239–257. [Google Scholar] [CrossRef]
- Reis, P.; Carvalho, M. Compositional Pore-Network Modeling of Gas-Condensate Flow: Effects of Interfacial Tension and Flow Velocity. arXiv 2020, arXiv:2007.12201. [Google Scholar]
- Dong, H. Micro-CT Imaging and Pore Network Extraction. Ph.D. Thesis, Department of Earth Science and Engineering, Imperial College London, London, UK, 2008. [Google Scholar]
- Fang, F.; Firoozabadi, A.; Abbaszadeh, M.; Radke, C. A Phenomenological modeling of critical condensate saturation. In SPE Annual Technical Conference and Exhibition; Society of Petroleum Engineers, 1996; Available online: https://doi.org/10.2118/36716-MS (accessed on 4 September 2020).
- Beresnev, I.A.; Li, W.; Vigil, R.D. Condition for break-up of non-wetting fluids in sinusoidally constricted capillary channels. Transp. Porous Media 2009, 80, 581. [Google Scholar] [CrossRef]
- Santos, M.; Carvalho, M. Pore network model for retrograde gas flow in porous media. J. Pet. Sci. Eng. 2019, 185, 106635. [Google Scholar] [CrossRef] [Green Version]
- Sochi, T. Newtonian Flow in Converging-Diverging Capillaries. Int. J. Model. Simul. Sci. Comput. 2013, 4, 1350011. [Google Scholar] [CrossRef]
- Kashid, M.N.; Agar, D.W. Hydrodynamics of liquid–liquid slug flow capillary microreactor: Flow regimes, slug size and pressure drop. Chem. Eng. J. 2007, 131, 1–13. [Google Scholar] [CrossRef]
- Peng, D.Y.; Robinson, D.B. A New Two-Constant Equation of State. Ind. Eng. Chem. Fundam. 1976, 15, 59–64. [Google Scholar] [CrossRef]
- Imperial College London. PERM—Petroleum Engineering & Rock Mechanics Group. Micro-CT Images and Networks: Sandstone S4. 2007. Available online: http://www.imperial.ac.uk/earth-science/research/research-groups/perm/research/pore-scale-modelling/micro-ct-images-and-networks/sandstone-s4/ (accessed on 25 August 2020).
- Jamiolahmady, M.; Danesh, A.; Henderson, G.; Tehrani, D. Variations of gas-condensate relative permeability with production rate at near wellbore conditions: A general correlation. In Offshore Europe; Society of Petroleum Engineers, 2003; Available online: https://doi.org/10.2118/120088-PA (accessed on 4 September 2020).
- Henderson, G.D.; Danesh, A.; Tehrani, D.H.; Al-Shaidi, S.; Peden, J.M. Measurement and correlation of gas condensate relative permeability by the steady-state method. SPE Reserv. Eval. Eng. 1998, 1, 134–140. [Google Scholar] [CrossRef]
- Lohrenz, J.; Bray, B.G.; Clark, C.R. Calculating viscosities of reservoir fluids from their compositions. J. Pet. Technol. 1964, 16, 1–171. [Google Scholar] [CrossRef]
- Weinaug, C.F.; Katz, D.L. Surface Tensions of Methane-Propane Mixtures. Ind. Eng. Chem. 1943, 35, 239–246. [Google Scholar] [CrossRef]
- Zhang, S.; Jiang, G.C.; Wang, L.; Qing, W.; Guo, H.T.; Tang, X.g.; Bai, D.G. Wettability alteration to intermediate gas-wetting in low-permeability gas-condensate reservoirs. J. Pet. Explor. Prod. Technol. 2014, 4, 301–308. [Google Scholar] [CrossRef] [Green Version]
- Al-Anazi, H.A.; Xiao, J.; Al-Eidan, A.A.; Buhidma, I.M.; Ahmed, M.S.; Al-Faifi, M.; Assiri, W.J. Gas productivity enhancement by wettability alteration of gas-condensate reservoirs. In European Formation Damage Conference; Society of Petroleum Engineers, 2007; Available online: https://doi.org/10.2118/107493-MS (accessed on 4 September 2020).
- Gilani, S.F.H.; Sharma, M.M.; Torres, D.E.; Ahmadi, M.; Pope, G.A.; Linnemeyer, H.C. Correlating Wettability Alteration with Changes in Gas Relative Permeability in Gas Condensate/Volatile Oil Reservoirs. In SPE International Symposium on Oilfield Chemistry; Society of Petroleum Engineers, 2011; Available online: https://doi.org/10.2118/141419-MS (accessed on 4 September 2020).
- Karandish, G.; Rahimpour, M.; Sharifzadeh, S.; Dadkhah, A. Wettability alteration in gas-condensate carbonate reservoir using anionic fluorinated treatment. Chem. Eng. Res. Des. 2015, 93, 554–564. [Google Scholar] [CrossRef]




| Component | Molar Fraction |
|---|---|
| CO | 0.07 |
| C1 | 0.65 |
| C2 | 0.13 |
| C3 | 0.07 |
| C6 | 0.05 |
| C10 | 0.025 |
| C16 | 0.005 |
| Label | Pressure [MPa] | Liquid Dropout [%] | [Pas] | [Pas] | [mN/m] |
|---|---|---|---|---|---|
| P6 | 21.57 | 1.17 | 0.0196 | ||
| P5 | 21.33 | 3.51 | 0.0271 | ||
| P4 | 21.84 | 5.31 | 0.0359 | ||
| P3 | 20.59 | 7.86 | 0.058 | ||
| P2 | 19.61 | 10.6 | 0.1221 | ||
| P1 | 17.5 | 12.12 | 0.3501 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Reis, P.K.P.; Carvalho, M.S. Pore-Scale Analysis of Condensate Blockage Mitigation by Wettability Alteration. Energies 2020, 13, 4673. https://doi.org/10.3390/en13184673
Reis PKP, Carvalho MS. Pore-Scale Analysis of Condensate Blockage Mitigation by Wettability Alteration. Energies. 2020; 13(18):4673. https://doi.org/10.3390/en13184673
Chicago/Turabian StyleReis, Paula K. P., and Marcio S. Carvalho. 2020. "Pore-Scale Analysis of Condensate Blockage Mitigation by Wettability Alteration" Energies 13, no. 18: 4673. https://doi.org/10.3390/en13184673
APA StyleReis, P. K. P., & Carvalho, M. S. (2020). Pore-Scale Analysis of Condensate Blockage Mitigation by Wettability Alteration. Energies, 13(18), 4673. https://doi.org/10.3390/en13184673