Study on the Collapse Process of Cavitation Bubbles Near the Concave Wall by Lattice Boltzmann Method Pseudo-Potential Model
Abstract
1. Introduction
2. Pseudo-Potential LBM-MRT
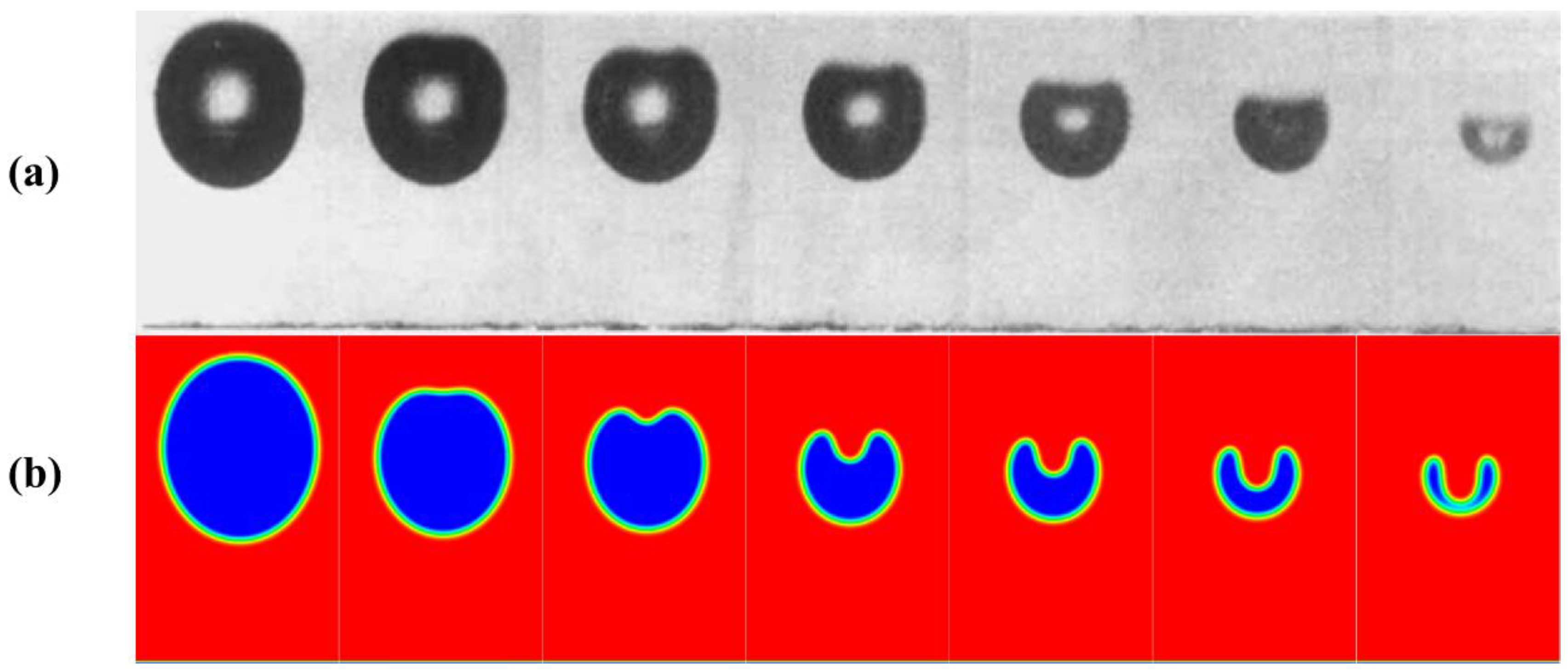
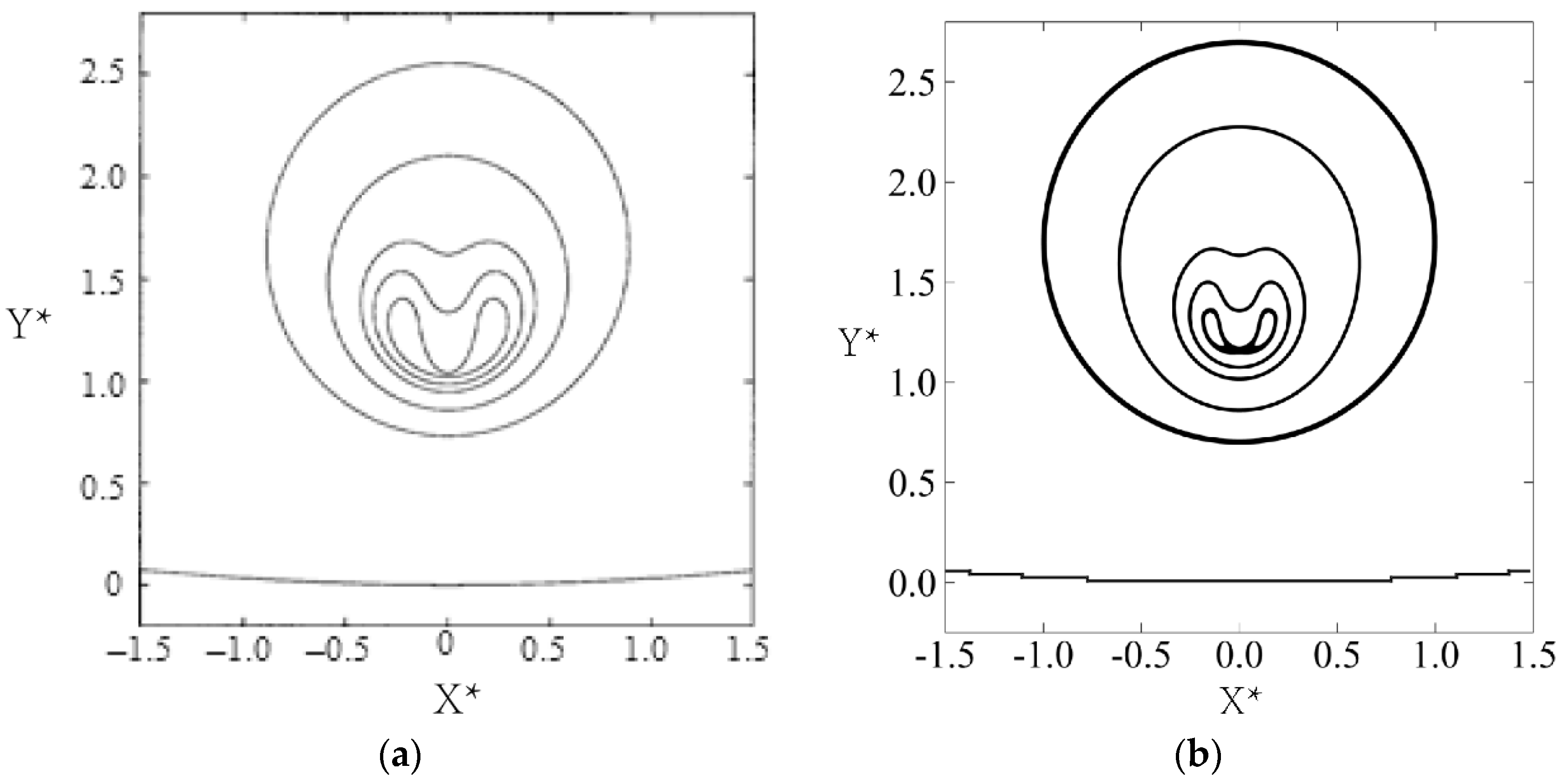
3. Model Validation
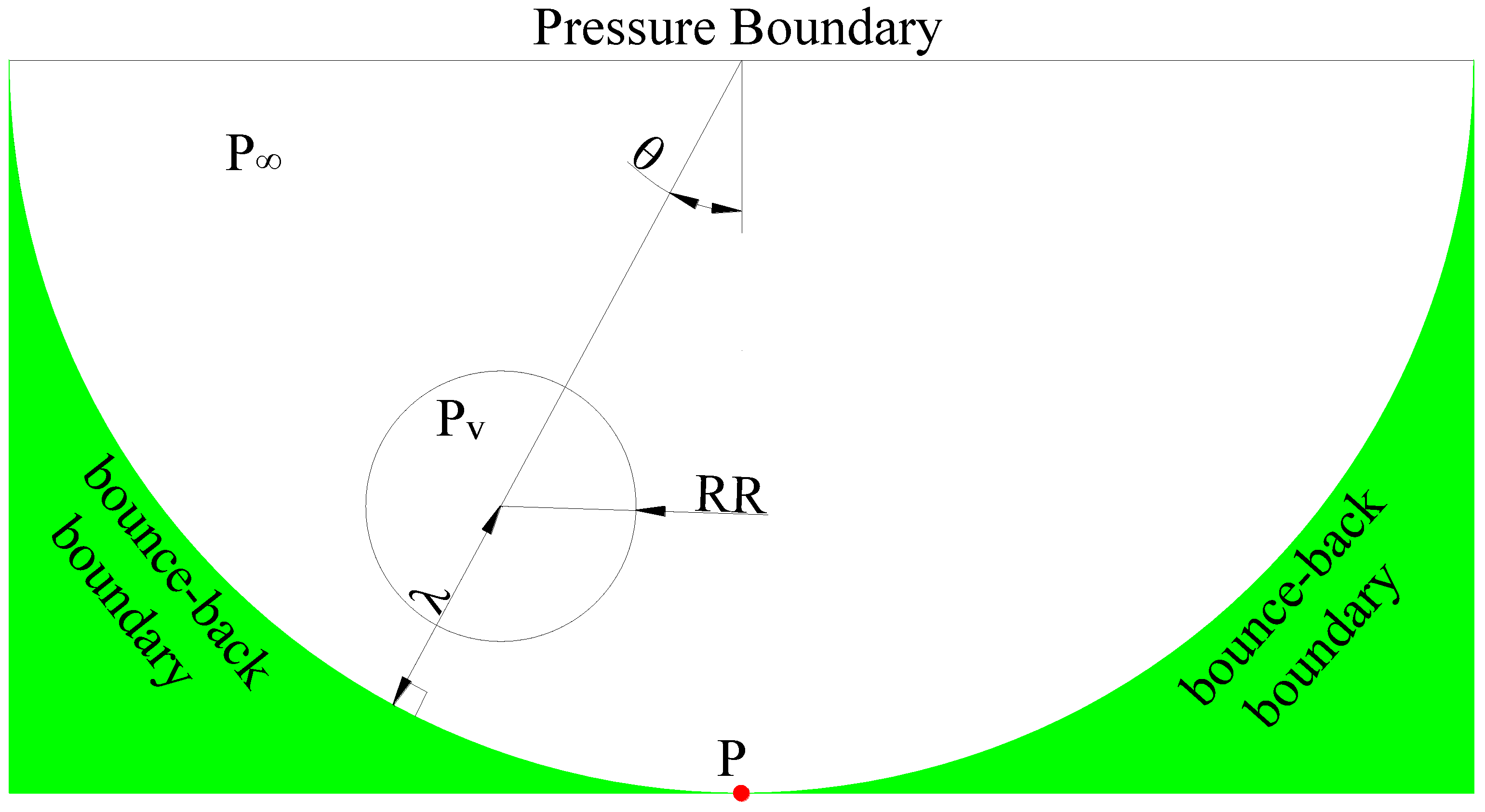
4. Collapse Process of the Cavitation Bubble Near the Concave Wall
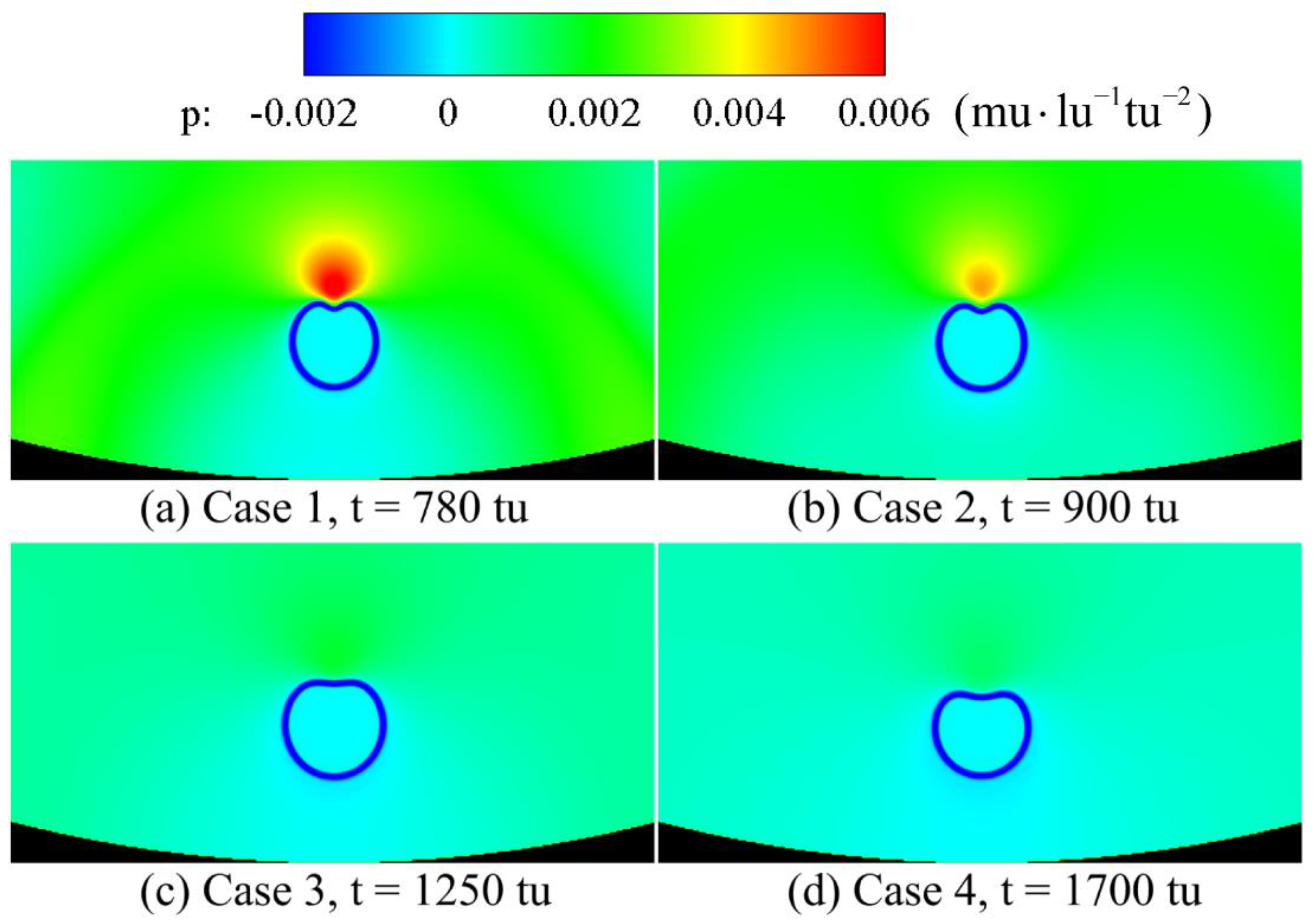
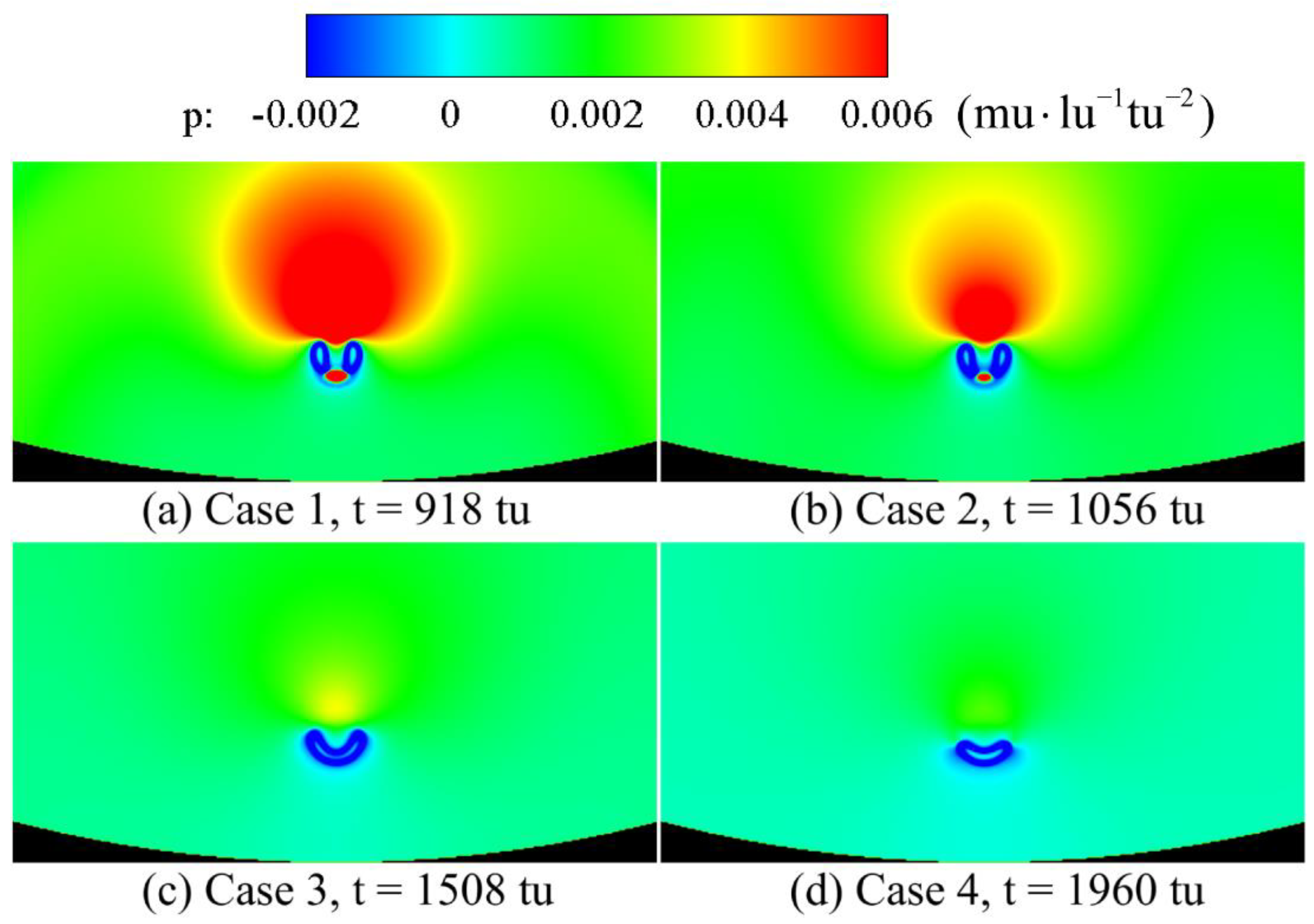
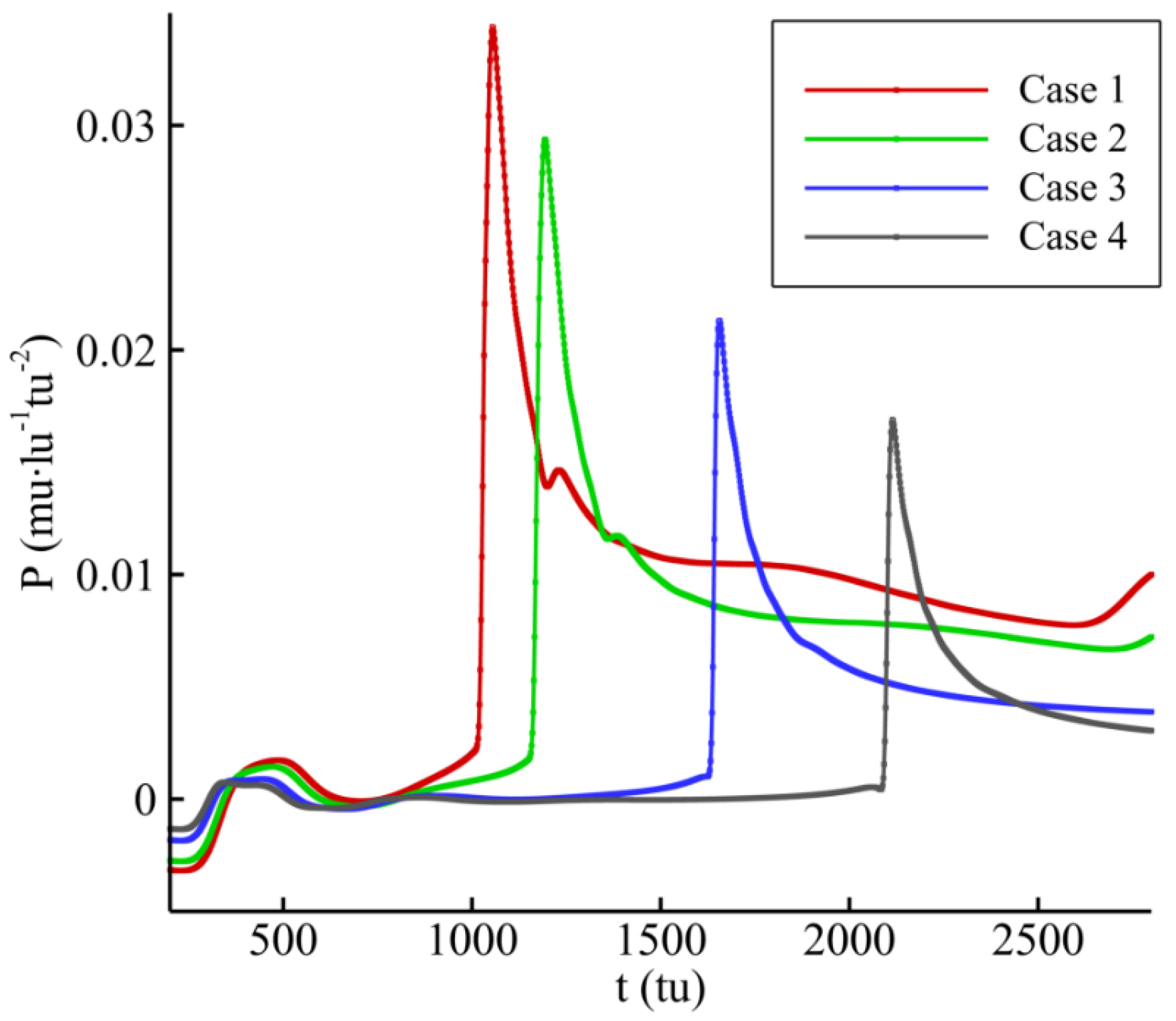
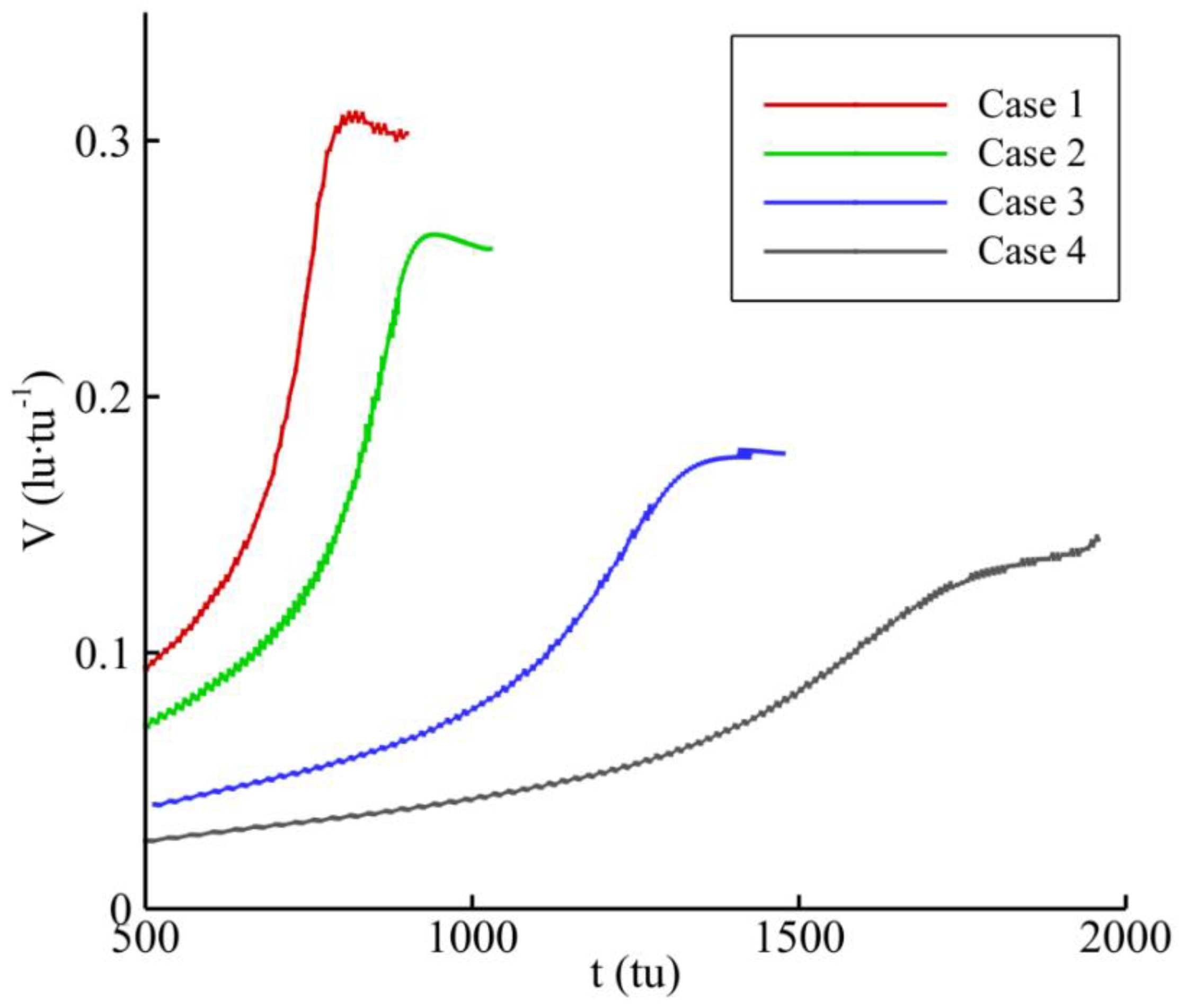
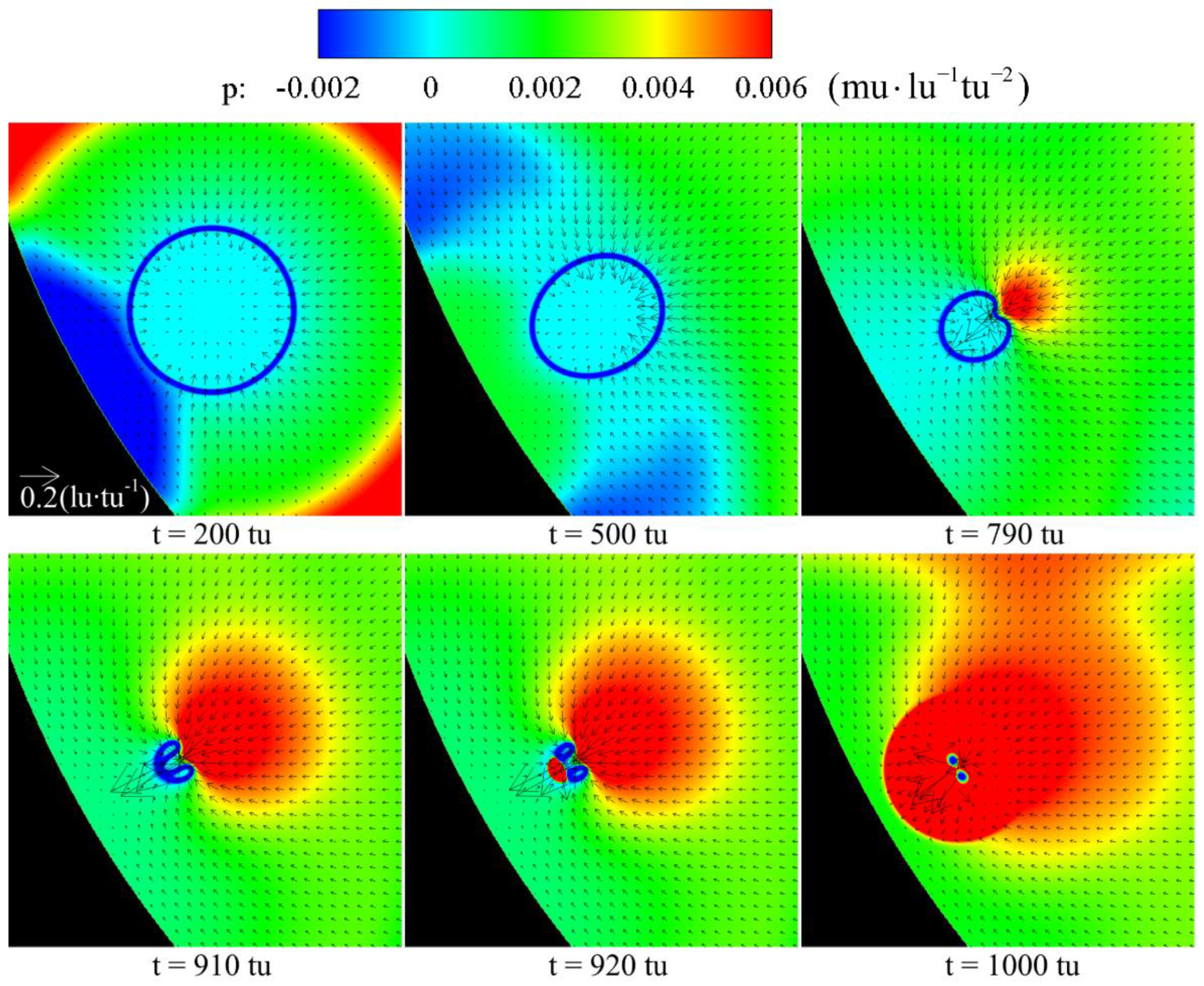
4.1. Evolution of the Single Bubble under Different Additional Pressures
4.2. Evolution of the Single Bubble in Different Angles with Vertical Direction
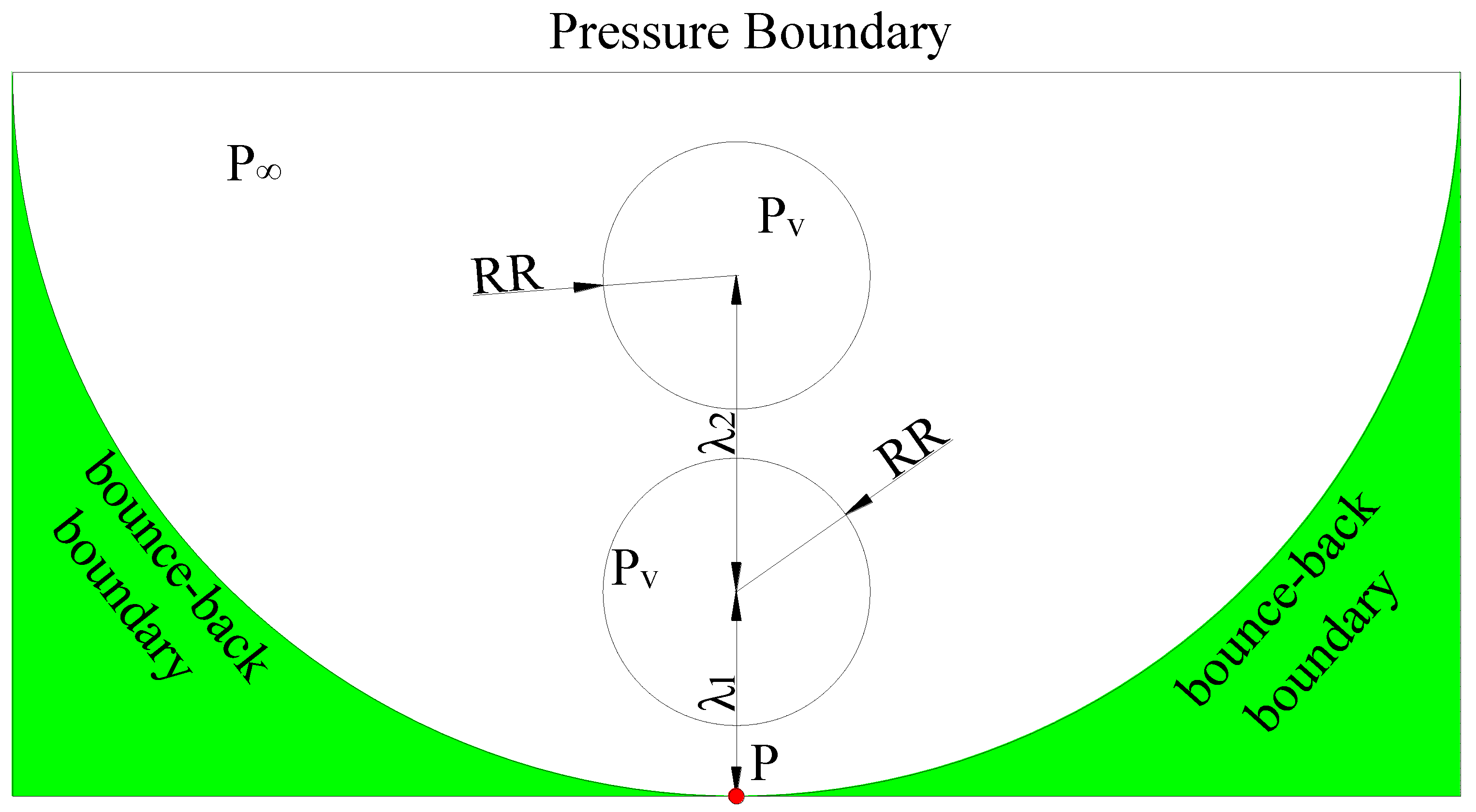
4.3. Evolution of the Double Bubbles with Vertical Arrangement
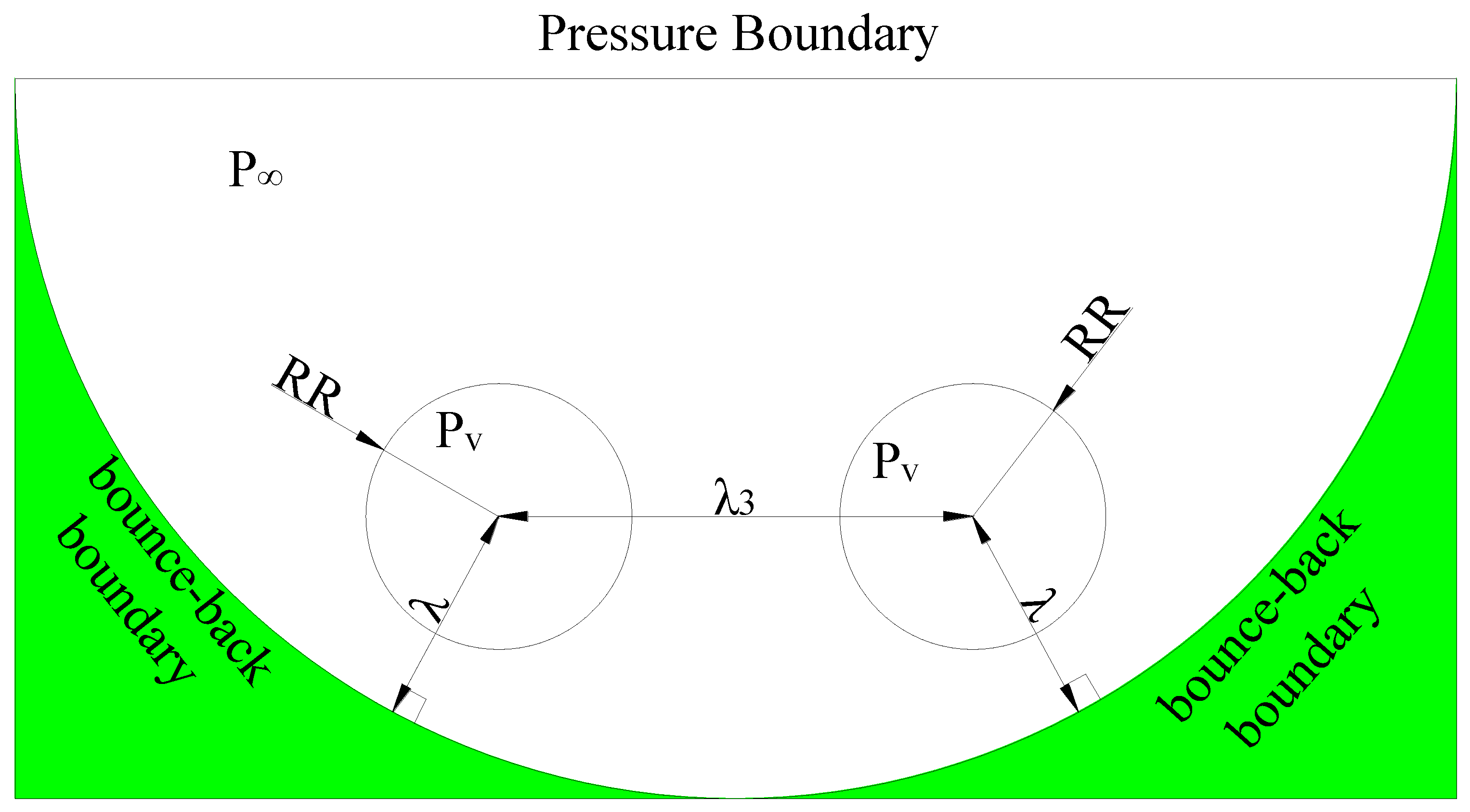
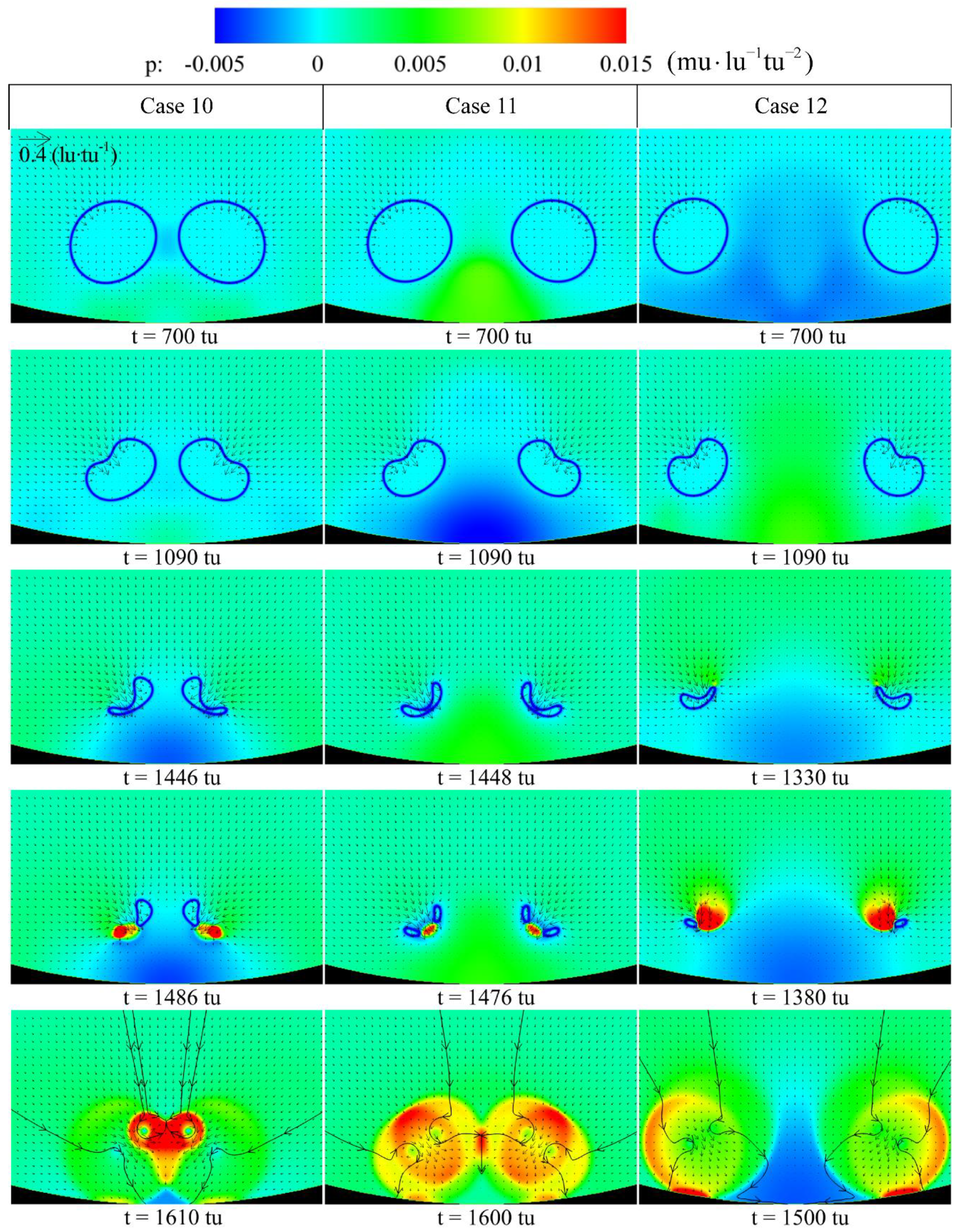
4.4. Evolution of the Double Bubbles with Horizontal Arrangement
5. Discussion
6. Conclusions
- The collapse process of cavitation bubble is affected by the pressure of the surrounding environment. When the additional pressure around the environment decreases, the velocity of cavitation-bubble collapse becomes slower, and the duration of the collapsing process increases accordingly. Moreover, no second collapse of cavitation bubble can be found when the additional pressure is lower than a critical value. When the angle of the cavitation bubble with vertical direction changes, the collapse process of cavitation bubble is similar, but the depression direction is perpendicular to the concave wall. After it collapses, the low-pressure zone is generated due to the vortex.
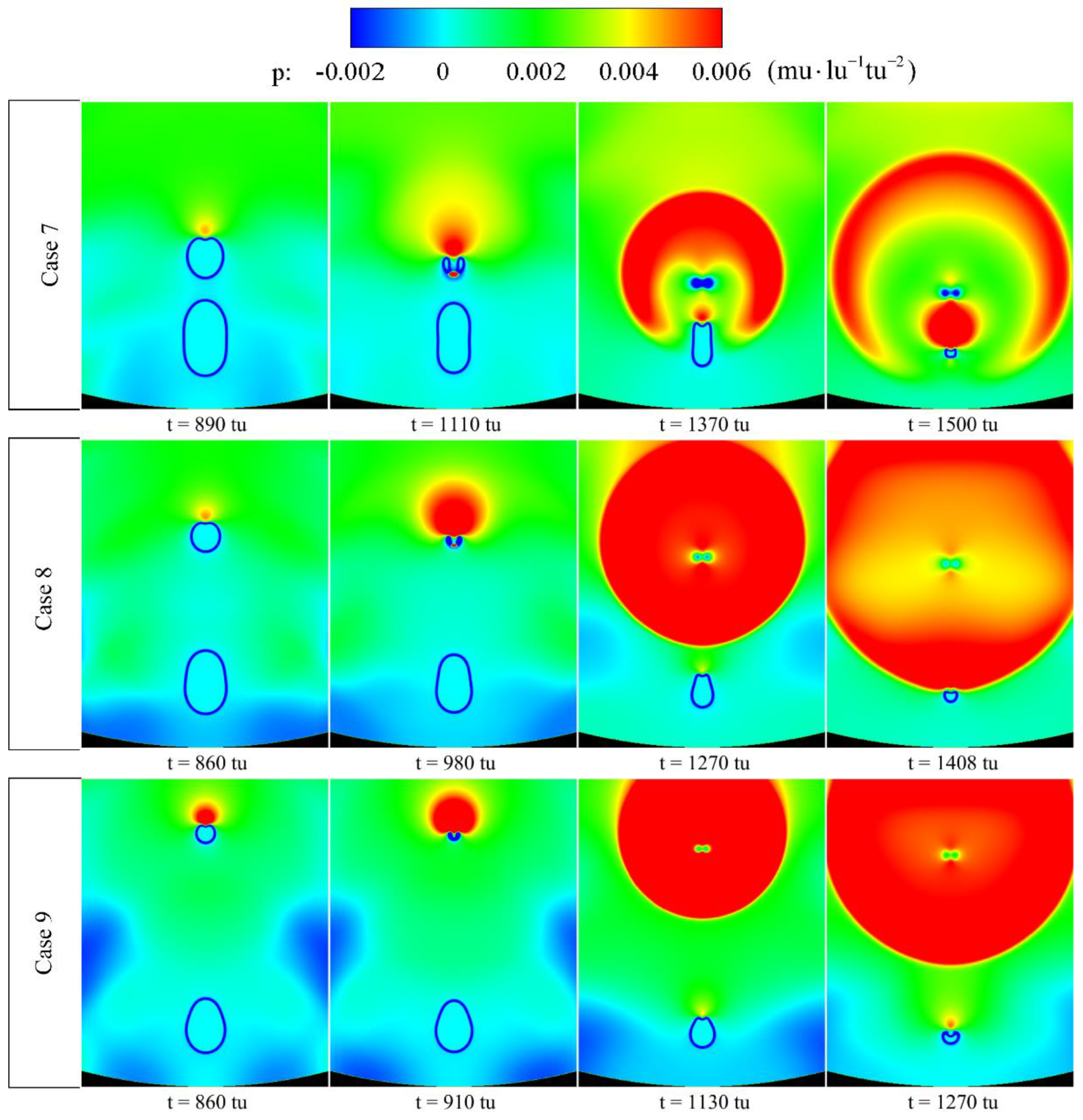
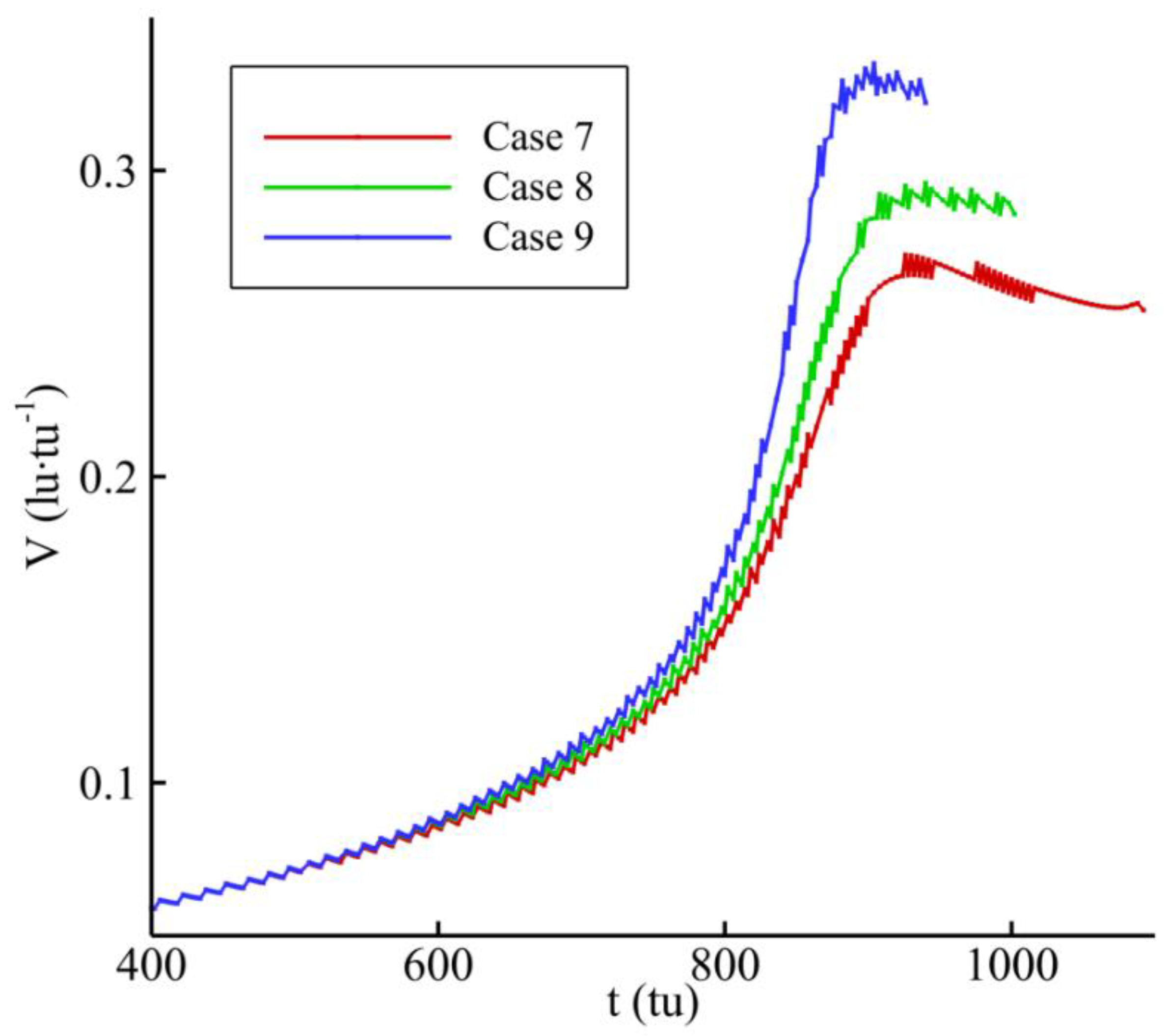
- When the double cavitation bubbles are arranged vertically, as the relative distance between cavitation bubbles increases, the pressure above the upper bubble increases, and the velocity of collapse also raises accordingly. With the increase in the relative distance, the interval between the first and the second collapses of the upper bubble becomes shorter; eventually there is no occurrence of the second collapse. After the upper bubble collapses, the collapse process of the lower one in each case is not the same. For example, the mutual effect between cavitation bubbles decreases as the relative distance increases. Besides, the shrinking center of the lower bubble shifts from its middle to the upper.
- When the double cavitation bubbles are arranged horizontally, the mutual effect between the cavitation bubbles gradually decreases, as the relative distance of the cavitation bubbles increases. The depression position of cavitation bubble gradually moves from the lower part to the upper part, as the relative distance increases. In Case 11, the cavitation-bubble collapse from the middle of the bubble under the interaction between cavitation bubbles and the influence of the wall on the bubbles. After the collapse, the pressure in the vortex zone increases accordingly, as the relative distance of the cavitation bubbles increases.
Author Contributions
Funding
Conflicts of Interest
References
- Brennen, C.; Acosta, A.J. The dynamic transfer function for a cavitating inducer. J. Fluids Eng. 1976, 98, 182–191. [Google Scholar] [CrossRef]
- Shutler, N.D.; Mesler, R.B. A photographic study of the dynamics and damage capabilities of bubbles collapsing near solid boundaries. J. Basic Eng. 1965, 87, 511–517. [Google Scholar] [CrossRef]
- Kornfeld, M.; Suvorov, L. On the destructive action of cavitation. J. Appli. Phys. 1944, 15, 495–506. [Google Scholar] [CrossRef]
- Naude’, C.F.; Ellis, A.T. On the mechanism of cavitation damage by nonhemispherical cavities collapsing in contact with a solid boundary. J. Basic Eng. 1961, 83, 648–656. [Google Scholar] [CrossRef]
- Kling, C.L.; Hammitt, F.G. A photographic study of spark-induced cavitation bubble collapse. J. Basic Eng. 1972, 94, 825–832. [Google Scholar] [CrossRef]
- Vogel, A.; Lauterborn, W.; Timm, R. Optical and acoustic investigations of the dynamics of laser-produced cavitation bubbles near a solid boundary. J. Fluid Mech. 1989, 206, 299–338. [Google Scholar] [CrossRef]
- Vogel, A.; Lauterborn, W. Acoustic transient generation by laser-produced cavitation bubbles near solid boundaries. J. Acoust. Soc. Am. 1988, 84, 719–731. [Google Scholar] [CrossRef]
- Tomita, Y.; Kodama, T. Interaction of laser-induced cavitation bubbles with composite surfaces. J. Appl. Phys. 2003, 94, 2809–2816. [Google Scholar] [CrossRef]
- Tomita, Y.; Robinson, P.B.; Tong, R.P.; Blake, J.R. Growth and collapse of cavitation bubbles near a curved rigid boundary. J. Fluid Mech. 2002, 466, 259–283. [Google Scholar] [CrossRef]
- Kim, D.; Kim, D. Underwater bubble collapse on a ridge-patterned structure. Phys. Fluids 2020, 32, 053312. [Google Scholar] [CrossRef]
- Howison, S.D.; Ockendon, J.R.; Peregrine, D.H.; Moore, D.W.; Blake, J.R.; Hooton, M.C.; Robinson, P.B.; Tong, R.P. Collapsing cavities, toroidal bubbles and jet impact. Math. Phys. Eng. Sci. 1997, 355, 537–550. [Google Scholar]
- Can, E.; Prosperetti, A. A level set method for vapor bubble dynamics. J. Comput. Phys. 2012, 231, 1533–1552. [Google Scholar] [CrossRef]
- Sagar, H.J.; Moctar, O. Numerical simulation of a laser-induced cavitation bubble near a solid boundary considering phase change. Ship Tech. Res. 2018, 65, 163–179. [Google Scholar] [CrossRef]
- Popinet, S.; Zaleski, S. Bubble collapse near a solid boundary: A numerical study of the influence of viscosity. J. Fluid Mech. 2002, 464, 137–163. [Google Scholar] [CrossRef]
- Shan, X.W.; Chen, H.D. Lattice Boltzmann model for simulating flows with multiple phases and components. Phys. Rev. E 1993, 47, 1815–1819. [Google Scholar] [CrossRef] [PubMed]
- d’Humières, D. Generalized lattice-boltzmann equations. In Rarefied Gas Dynamics: Theory and Simulations; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1994; pp. 450–458. [Google Scholar]
- Li, Q.; Luo, K.H.; Li, X.J. Lattice Boltzmann modeling of multiphase flows at large density ratio with an improved pseudopotential model. Phys. Rev. E 2013, 87, 053301. [Google Scholar] [CrossRef] [PubMed]
- Mao, Y.F.; Peng, Y.; Zhang, J.M. Study of cavitation bubble collapse near a wall by the modified lattice Boltzmann method. Water 2018, 10, 1439. [Google Scholar] [CrossRef]
- Peng, C.; Tian, S.C.; Li, G.S.; Sukop, M.C. Simulation of laser-produced single cavitation bubbles with hybrid thermal lattice Boltzmann method. Int. J. Heat Mass Transf. 2020, 149, 119136. [Google Scholar] [CrossRef]
- Shan, M.L.; Zhu, C.P.; Yao, C.; Yin, C.; Jiang, X.Y. Pseudopotential multi-relaxation-time lattice Boltzmann model for cavitation bubble collapse with high density ratio. Chin. Phys. B 2016, 25, 104701. [Google Scholar] [CrossRef]
- Shan, M.L.; Zhu, C.P.; Zhou, X.; Yin, C.; Han, Q.B. Investigation of cavitation bubble collapse near rigid boundary by lattice Boltzmann method. J. Hydrodyn. 2016, 28, 442–450. [Google Scholar] [CrossRef]
- Su, Y.W.; Tang, X.L.; Wang, F.J.; Li, X.Q.; Shi, X.Y. Three-dimensional cavitation bubble simulations based on lattice Boltzmann model coupled with carnahan-starling equation of state. Commun. Comput. Phys. 2017, 22, 473–493. [Google Scholar] [CrossRef]
- Yang, Y.; Shan, M.L.; Kan, X.F.; Shangguan, Y.Q.; Han, Q.B. Thermodynamic of collapsing cavitation bubble investigated by pseudopotential and thermal MRT-LBM. Ultrason. Sonochem. 2020, 62, 104873. [Google Scholar] [CrossRef] [PubMed]
- Mukherjee, S.; Abraham, J. A pressure-evolution-based multi-relaxation-time high-density-ratio two-phase lattice-Boltzmann model. Comput. Fluids 2007, 36, 1149–1158. [Google Scholar] [CrossRef]
- Lallemand, P.; Luo, L.S. Theory of the lattice Boltzmann method: Dispersion, dissipation, isotropy, Galilean invariance, and stability. Phys. Rev. E 2000, 61, 6546–6562. [Google Scholar] [CrossRef] [PubMed]
- Mohamad, A.A. Lattice Boltzmann Method: Fundamentals and Engineering Applications with Computer Codes; Springer: London, UK, 2017. [Google Scholar]
- Li, Q.; He, Y.L.; Tang, G.H.; Tao, W.Q. Improved axisymmetric lattice Boltzmann scheme. Phys. Rev. E 2010, 81, 056707. [Google Scholar] [CrossRef] [PubMed]
- Chen, S.Y.; Doolen, G.D. lattice Boltzmann method for fluid flows. Ann. Rev. Fluid Mech. 1998, 30, 329–364. [Google Scholar] [CrossRef]
- Sbragaglia, M.; Benzi, R.; Biferale, L.; Succi, S.; Sugiyama, K.; Toschi, F. Generalized lattice Boltzmann method with multirange pseudopotential. Phys. Rev. E 2007, 75, 026702. [Google Scholar] [CrossRef]
- Yuan, P.; Schaefer, L. Equations of state in a lattice Boltzmann model. Phys. Fluids 2006, 18, 042101. [Google Scholar] [CrossRef]
- Lauterborn, W.; Bolle, H. Experimental investigations of cavitation-bubble collapse in the neighbourhood of a solid boundary. J. Fluid Mech. 1975, 72, 391–399. [Google Scholar] [CrossRef]
- Zou, Q.S.; He, X.Y. On pressure and velocity boundary conditions for the lattice Boltzmann BGK model. Phys. Fluids 1997, 9, 1591–1598. [Google Scholar] [CrossRef]
- Krüger, T.; Kusumaatmaja, H.; Kuzmin, A.; Shardt, O.; Silva, G.; Viggen, E.M. The Lattice Boltzmann Method: Principles and Practice; Springer: New York, NY, USA, 2016. [Google Scholar]
- Plesset, M.S.; Chapman, R.B. Collapse of an initially spherical vapour cavity in the neighbourhood of a solid boundary. J. Fluid Mech. 1971, 47, 283–290. [Google Scholar] [CrossRef]
- Ishida, H.; Nuntadusit, C.; Kimoto, H.; Nakagawa, T.; Yamamoto, T. Cavitation bubble behavior near solid boundaries. In Proceedings of the 4th International Symposium on Cavitation, Pasadena, CA, USA, 20–23 June 2001. [Google Scholar]
- Shervani-Tabar, M.T.; Rouhollahi, R. Numerical study on the effect of the concave rigid boundaries on the cavitation intensity. Sci. Iran. 2017, 24, 1958–1965. [Google Scholar] [CrossRef][Green Version]
- Xue, H.H.; Shan, F.; Guo, X.S.; Tu, J.; Zhang, D. Cavitation bubble collapse near a curved wall by the multiple-relaxation-time shan–chen lattice Boltzmann model. Chin. Phys. Lett. 2017, 34, 084301. [Google Scholar] [CrossRef]




| (°) | (lu) | RR (lu) | Δp (mu·lu−1tu−2) | |
|---|---|---|---|---|
| Case 1 | 0 | 1.6 × RR | 70 | 0.0067 |
| Case 2 | 0 | 1.6 × RR | 70 | 0.0054 |
| Case 3 | 0 | 1.6 × RR | 70 | 0.0031 |
| Case 4 | 0 | 1.6 × RR | 70 | 0.0020 |
| (°) | (lu) | RR (lu) | Δp (mu·lu−1tu−2) | |
|---|---|---|---|---|
| Case 5 | 30 | 1.6 × RR | 70 | 0.0054 |
| Case 6 | 60 | 1.6 × RR | 70 | 0.0054 |
| (lu) | (lu) | RR (lu) | Δp (mu·lu−1tu−2) | |
|---|---|---|---|---|
| Case 7 | 1.6 × RR | 2.4 × RR | 70 | 0.0042 |
| Case 8 | 1.6 × RR | 3.2 × RR | 70 | 0.0042 |
| Case 9 | 1.6 × RR | 4.5 × RR | 70 | 0.0042 |
| (lu) | (lu) | RR (lu) | Δp (mu·lu−1tu−2) | |
|---|---|---|---|---|
| Case 10 | 2.4 × RR | 1.6 × RR | 70 | 0.0042 |
| Case 11 | 3.0 × RR | 1.6 × RR | 70 | 0.0042 |
| Case 12 | 4.5 × RR | 1.6 × RR | 70 | 0.0042 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Peng, Y. Study on the Collapse Process of Cavitation Bubbles Near the Concave Wall by Lattice Boltzmann Method Pseudo-Potential Model. Energies 2020, 13, 4398. https://doi.org/10.3390/en13174398
Liu Y, Peng Y. Study on the Collapse Process of Cavitation Bubbles Near the Concave Wall by Lattice Boltzmann Method Pseudo-Potential Model. Energies. 2020; 13(17):4398. https://doi.org/10.3390/en13174398
Chicago/Turabian StyleLiu, Yang, and Yong Peng. 2020. "Study on the Collapse Process of Cavitation Bubbles Near the Concave Wall by Lattice Boltzmann Method Pseudo-Potential Model" Energies 13, no. 17: 4398. https://doi.org/10.3390/en13174398
APA StyleLiu, Y., & Peng, Y. (2020). Study on the Collapse Process of Cavitation Bubbles Near the Concave Wall by Lattice Boltzmann Method Pseudo-Potential Model. Energies, 13(17), 4398. https://doi.org/10.3390/en13174398






