Effect of Principal Stress Field on the Development of Plastic Zone ahead of the Gateroad
Abstract
1. Introduction
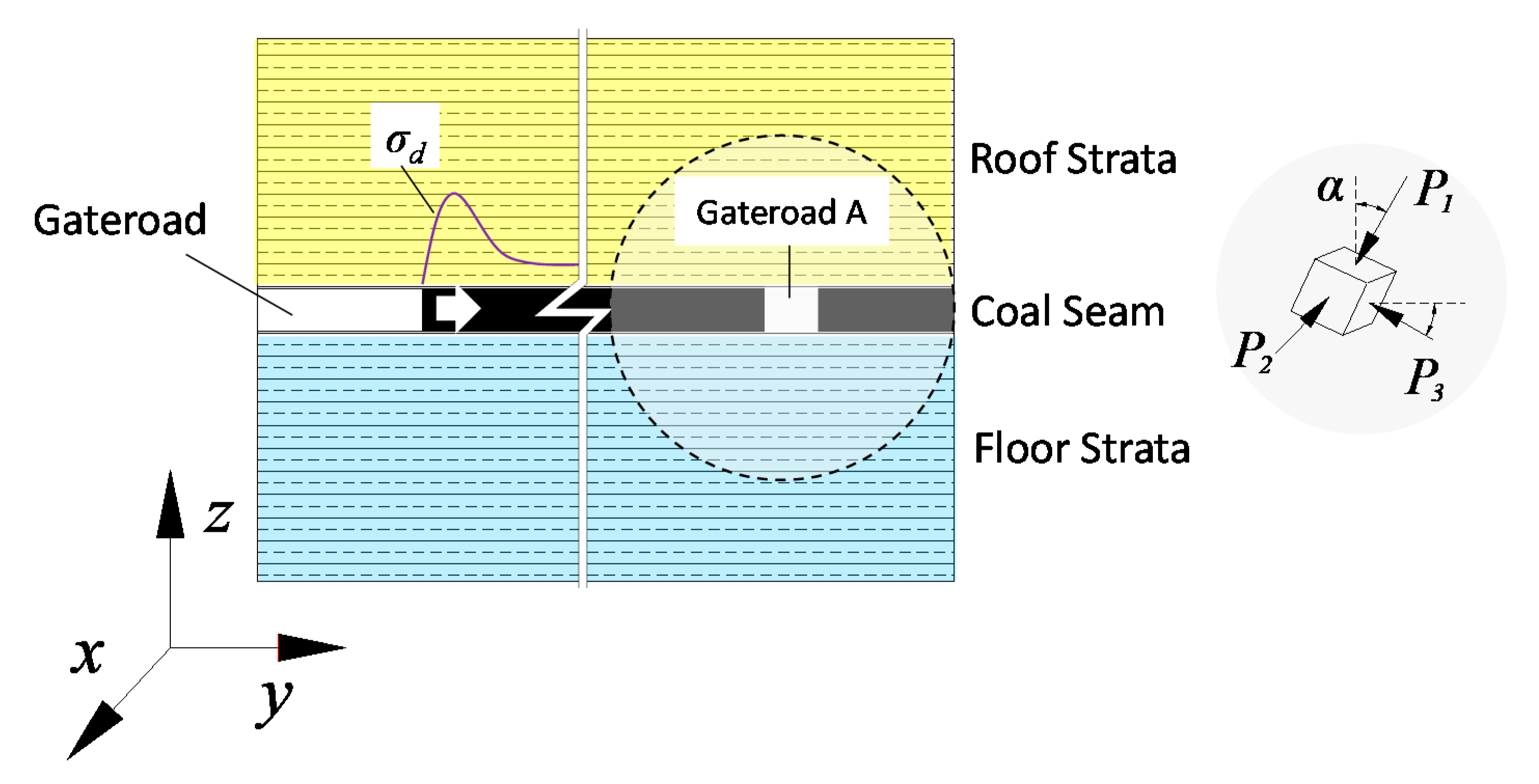
2. Numerical Model Setup
3. Modelling Plans
3.1. Plan 1
3.2. Plan 2
3.3. Plan 3
3.4. Plan 4
4. Numerical Modelling Results
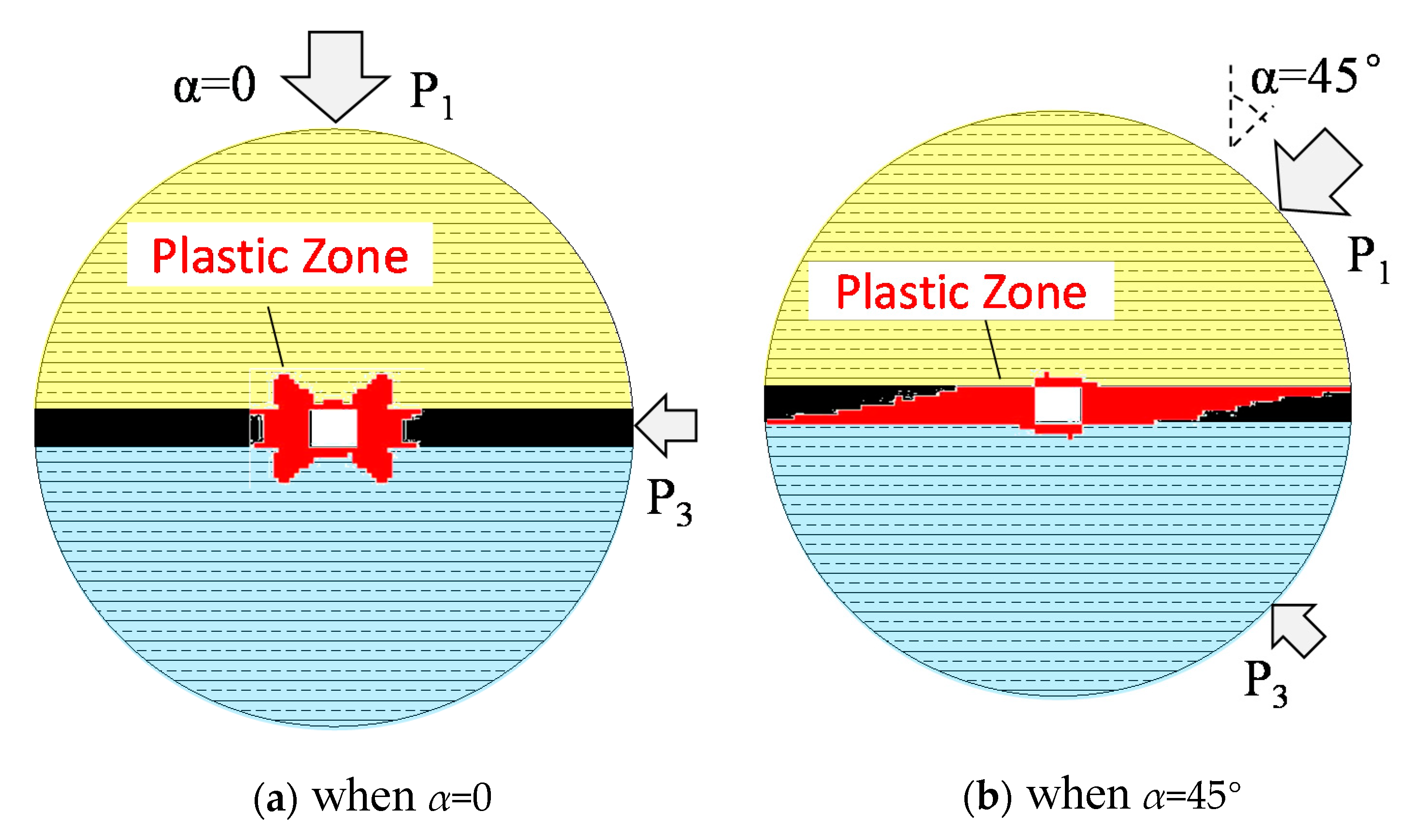
4.1. Influence of α (Angle between the P1 Direction and the Z-Axis)
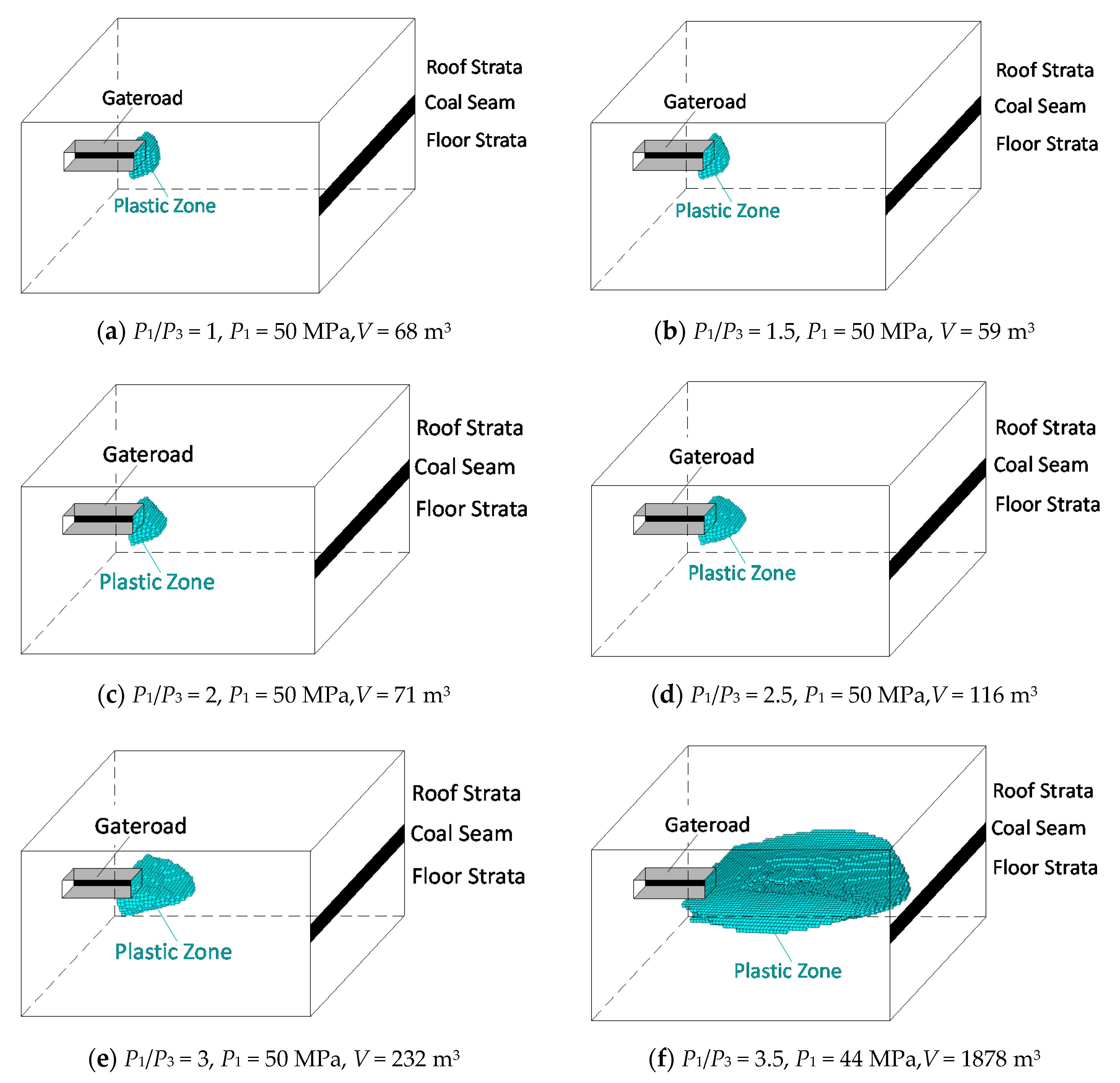
4.2. Influence of P1/P3 (Ratio of Maximum Principal Stress to Minimum Principal Stress)
- (1).
- When the magnitude of P1/P3 ranges from 1 to 3, the plastic zone volume ahead of gateroad increases linearly. The growth slope is approximately the same when P1/P3 is 1, 1.5, and 2. In these situations, the plastic zone volume is almost the same if P1 is constant. The shape of plastic zone in Figure 5 corroborates the above explanation. As shown in Figure 5a–c, the plastic zone volume is 68 m3, 59 m3, and 71 m3 when the P1/P3 is 1, 1.5, and 2.
- (2).
- The growth slope increases when P1/P3 is 2.5 and 3. In these situations, significant differences of plastic zone volume are observed when P1 is constant. The larger the value of P1/P3, the larger the plastic zone volume. In Figure 4, the plastic zone volume is 116 m3 and 232 m3 when the P1/P3 is 2.5 and 3, which is also shown as Figure 5d,e.
- (3).
- When the magnitude of P1/P3 is 3.5, the plastic zone volume ahead of gateroad presents approximately exponential growth along with the increasing of maximum principal stress. The magnitude reaches to 1878 m3 when the P1 equals to 44 MPa. Shown as Figure 5f, the distribution range of plastic zone is obviously increased ahead of gateroad. It should be noted that the dashed line in the Figure 4 refers to the critical value of maximum principal stress (P1) when the P1/P3 is 3.5. When the green line going asymptotically upwards to the dashed line, the plastic zone volume increases rapidly along with the growth of P1. Ultimately, it increases to infinity when the critical value of P1 is obtained.
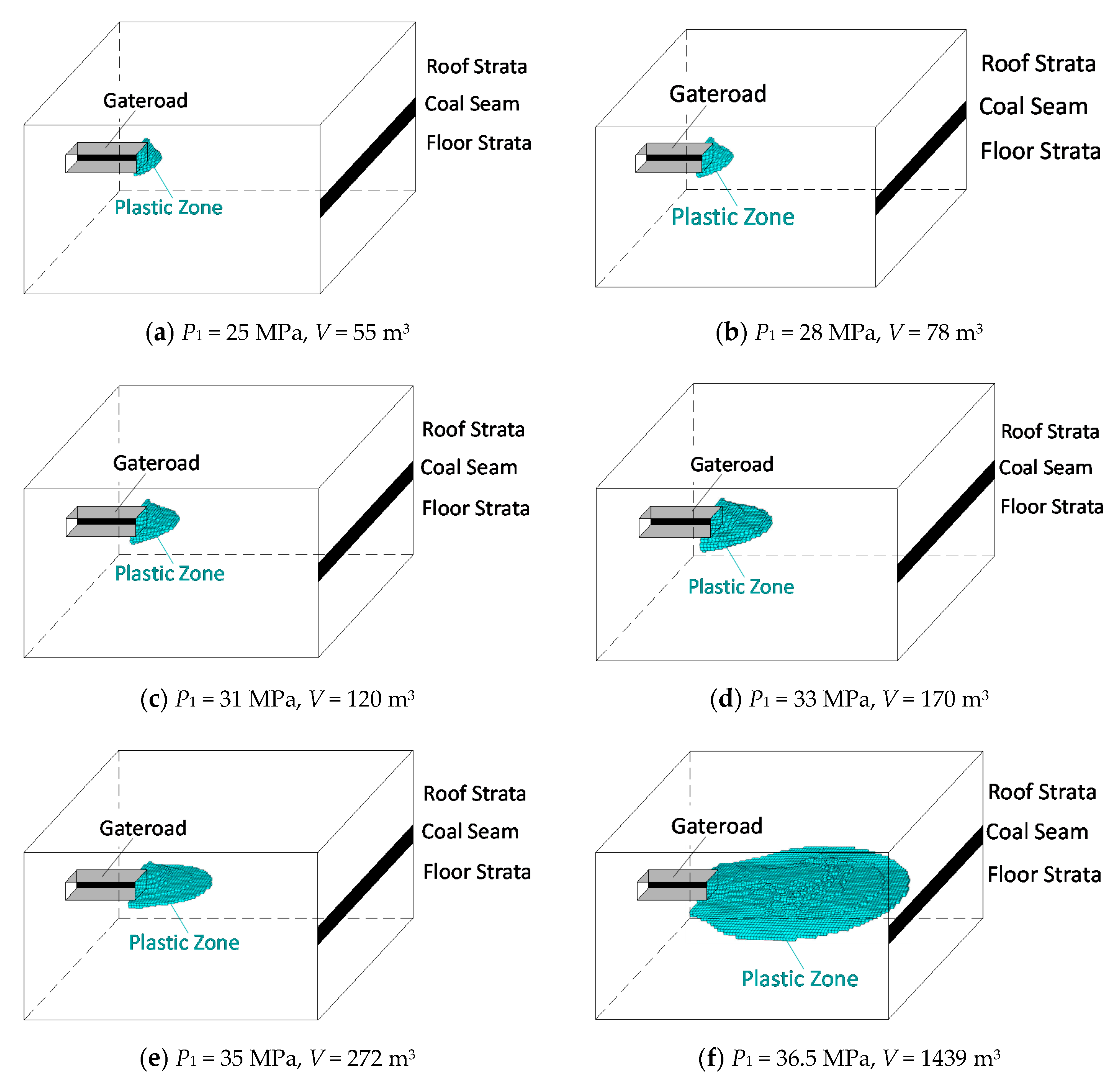
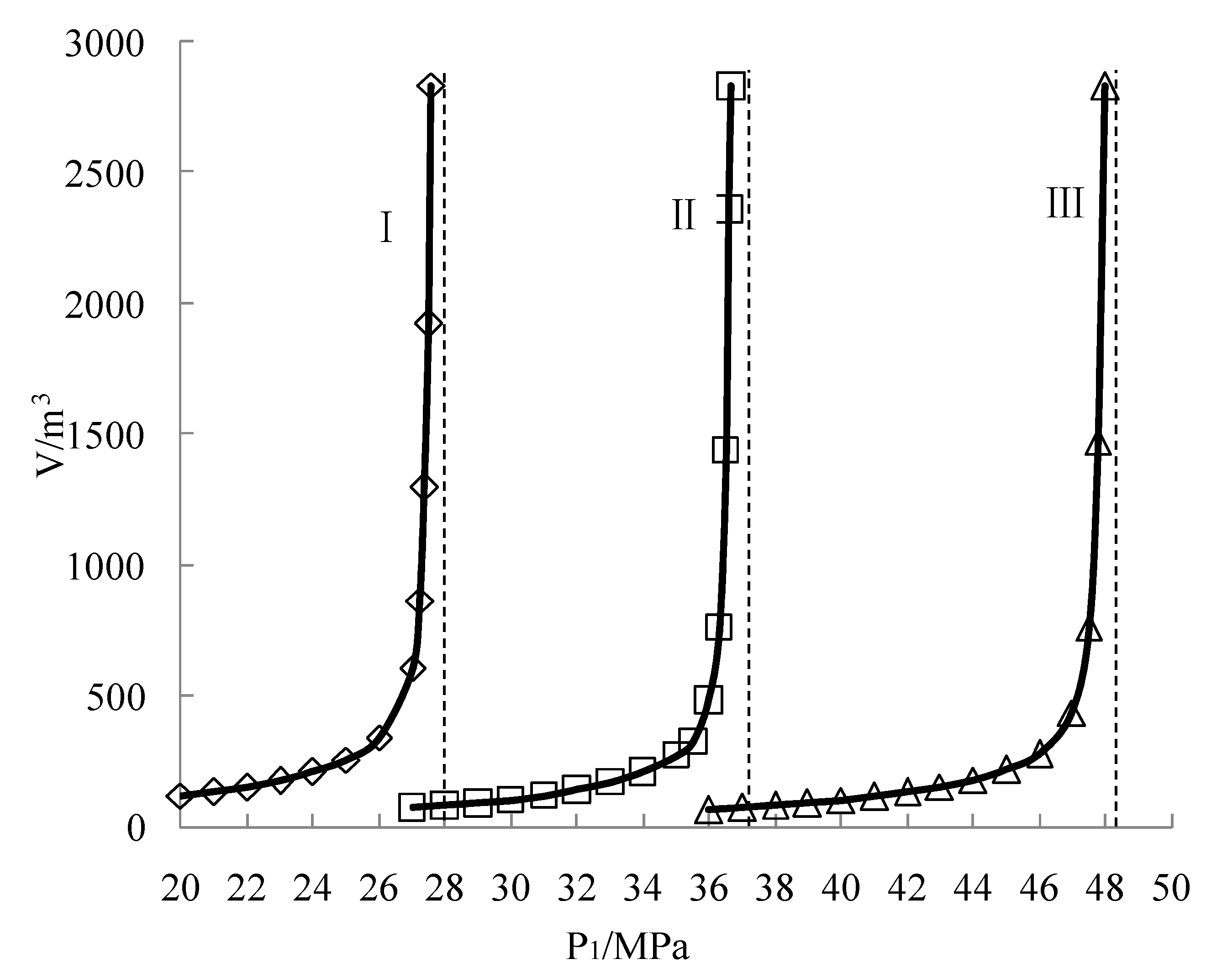
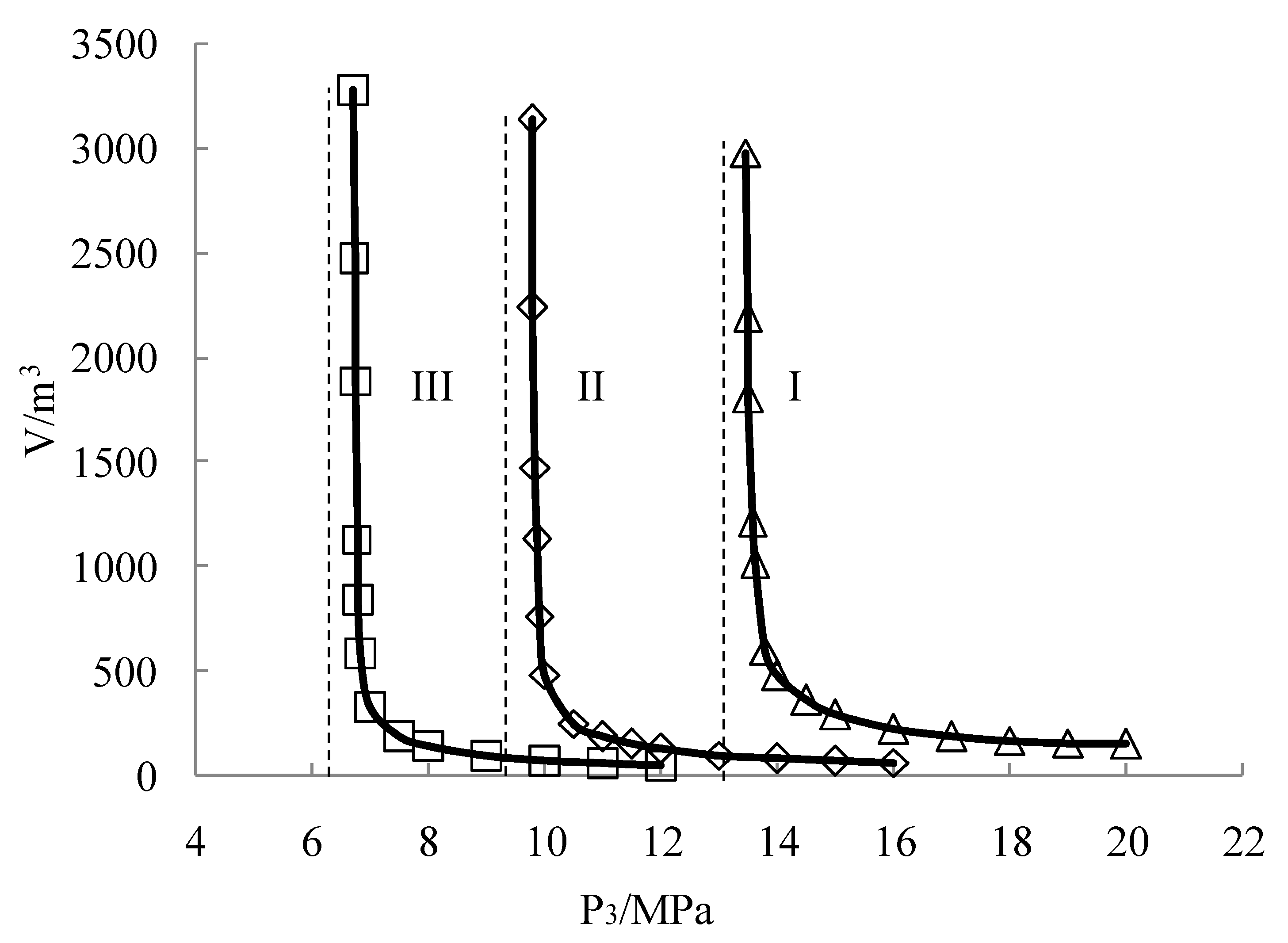
4.3. Influence of P1 (Maximum Principal Stress)
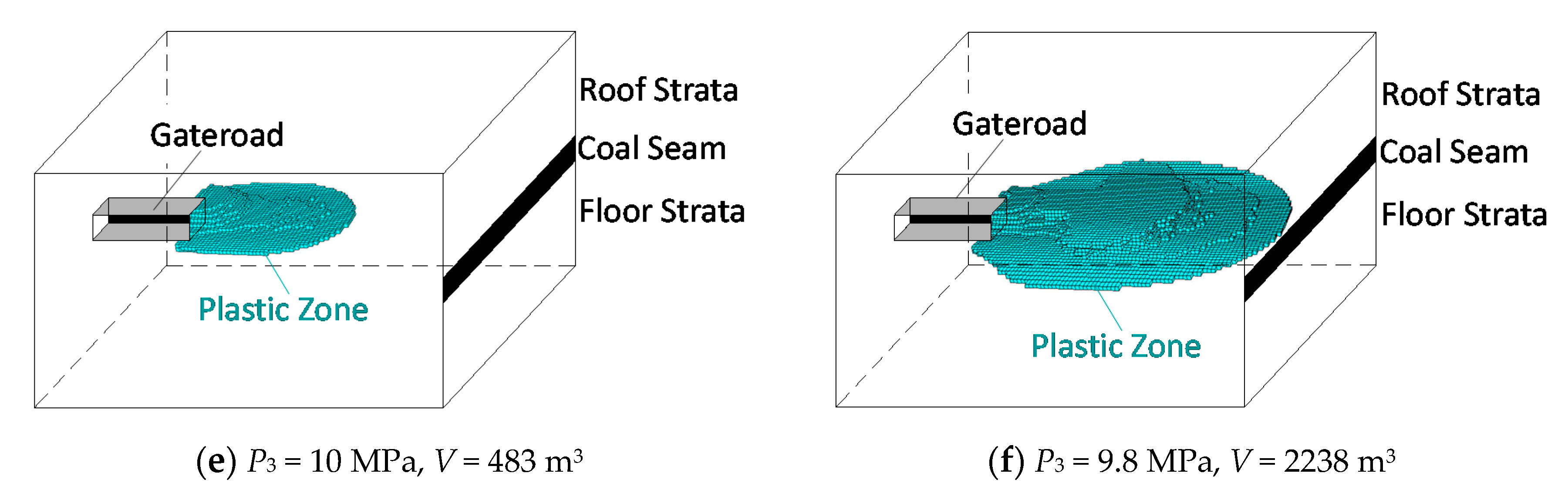
4.4. Influence of P3 (Minimum Principal Stress)
5. Discussion
5.1. Rotation of Butterfly-Shaped Plastic Zone’s Direction ahead of the Gateroad
5.2. Mutagenicity of Butterfly-Shaped Plastic Zone ahead of the Gateroad
5.3. Expansion of Butterfly-Shaped Plastic Zone ahead of the Gateroad
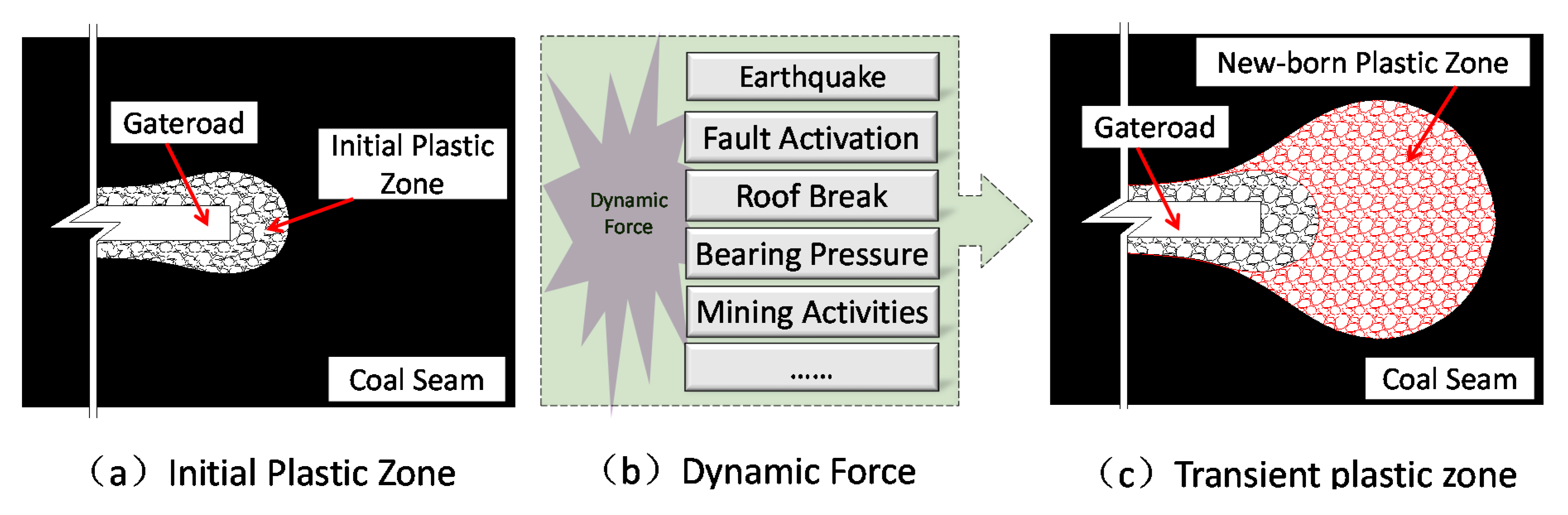
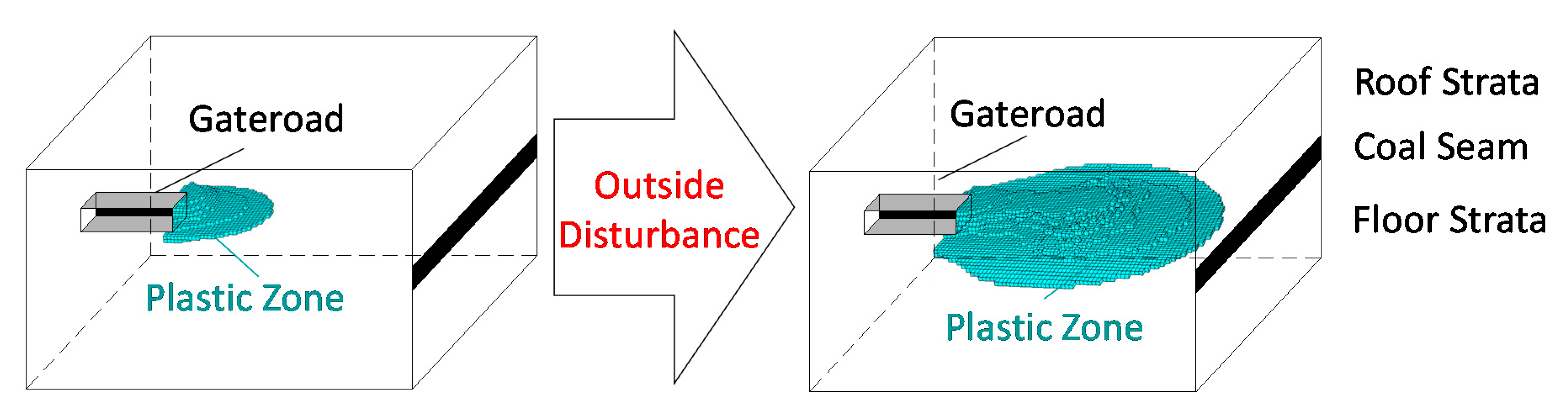
5.4. Implications for Coal and Gas Outburst Induced by the Expansion Plastic Zone ahead of Gateroad
6. Conclusions
- (1).
- The shape and range of plastic zone ahead of gateroad are determined by the maximum principal stress (P1) and the angle between the P1 direction and the Z-axis (α). It is also related closely to the minimum principal stress (P3) and the ratio of maximum principal stress to minimum principal stress (P1/P3). Under a certain stress state, a spheroid butterfly-shaped plastic zone will appear in the coal-rock mass ahead of the gateroad, which has a shape of more or less a flat-ball.
- (2).
- The plastic zone volume increases first and then decreases with the increase of α, and the direction of butterfly-shaped plastic zone ahead of gateroad is rotating with the evolution of α.
- (3).
- The plastic zone volume ahead of excavation face increases gradually with the increase of P1/P3. The mutagenicity of butterfly-shaped plastic zone is presented ahead of the gateroad under a certain value of P1/P3.
- (4).
- With the increase of P1 and decreasing of P3, the plastic zone volume is of exponential growth. The plastic zone volume approaches infinity when the critical value of maximum principal stress ([P1]) and the minimum principal stress ([P1]) is obtained.
- (5).
- The study of the effect of principal stress field on the expansion of plastic zone ahead of the gateroad is helpful for revealing the mechanisms of coal and gas outbursts. The critical stress state of butterfly-shaped plastic zone’s mutagenicity ahead of the gateroad can be used as an important indicator for assessing the risk of coal and gas outburst.
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, G.C.; He, F.L.; Jia, H.G.; Lai, Y.H. Analysis of gateroad atability in relation to yield pillar size: A case study. Rock Mech. Rock Eng. 2017, 50, 1263–1278. [Google Scholar] [CrossRef]
- Feng, G.R.; Wang, P.F.; Chugh, Y.P. Stability of gate roads next to an irregular yield pillar: A case study. Rock Mech. Rock Eng. 2019, 52, 2741–2760. [Google Scholar] [CrossRef]
- Zhang, G.C.; He, F.L.; Lai, Y.H.; Jia, H.G. Ground stability of underground gateroad with 1 km burial depth: A case study from Xingdong coal mine, China. J. Cent. South Univ. 2018, 25, 1386–1398. [Google Scholar] [CrossRef]
- Feng, G.R.; Wang, P.F.; Chugh, Y.P.; Zhao, J.L.; Wang, Z.Q.; Zhang, Z.P. A Coal Burst Mitigation Strategy for Tailgate during Deep Mining of Inclined Longwall Top Coal Caving Panels at Huafeng Coal Mine. Shock Vib. 2018, 2018. [Google Scholar] [CrossRef]
- Shen, B.; King, A.; Guo, H. Displacement, stress and seismicity in roadway roofs during mining-induced failure. Int. J. Rock Mech. Min. Sci. 2008, 45, 672–688. [Google Scholar] [CrossRef]
- Zhang, G.C.; Liang, S.J.; Tan, Y.L.; Xie, F.X.; Chen, S.J.; Jia, H.G. Numerical modelling for longwall pillar design: A case study from a typical longwall panel in China. J. Geophys. Eng. 2018, 15, 121–134. [Google Scholar] [CrossRef]
- Lawrence, W.J. A method for the design of longwall gateroad roof support. Int. J. Rock Mech. Min. 2009, 46, 789–795. [Google Scholar] [CrossRef]
- Trueman, R. The application of a numerical model to longwall coal mine roadway design. Min. Sci. Technol. 1990, 10, 157–165. [Google Scholar] [CrossRef]
- Kastner, H. Statik des Tunel und Stollenbauess; Springer: Berlin/Heidelberg, Germany, 1962. [Google Scholar]
- Abdel-Meguid, M.; Rowe, R.K.; Lo, K.Y. Three-dimensional analysis of unlined tunnels in rock subjected to high horizontal stress. Can. Geotech. J. 2003, 40, 1208–1224. [Google Scholar] [CrossRef]
- Leitman, M.J.; Villaggio, P. Plastic zone around circular holes. J. Eng. Mech. 2009, 135, 1467–1471. [Google Scholar] [CrossRef]
- Chen, L.W.; Peng, J.B.; Fan, W.; Sun, P. Analysis based on unified strength theory of non-uniform stress field circular roadway for plastic zone. J. China Coal Soc. 2007, 32, 20–23. [Google Scholar]
- Zhao, Z.Q. Study on Mechanism and Control Method of Deformation and Failure of Surrounding Rock in Large Deformation Mining Roadway; China University of Mining and Technology: Beijing, China, 2014. [Google Scholar]
- Meng, Q.B.; Han, L.J.; Xiao, Y.; Li, H.; Wen, S.Y.; Zhang, J. Numerical simulation study of the failure evolution process and failure mode of surrounding rock in deep soft rock roadways. Int. J. Min. Sci. Technol. 2016, 26, 209–221. [Google Scholar] [CrossRef]
- Guo, X.F.; Ma, N.J.; Zhao, X.D.; Zhao, Z.Q.; Li, Y.E. The general shapes and criterion for surrounding rock mass plastic zone of round roadway. J. China Coal Soc. 2016, 41, 1871–1877. [Google Scholar]
- Lu, Y.; Tu, S.H. Rules of distribution in a plastic zone of rocks surrounding a roadway affected by tectonic stress. Min. Sci. Tech. 2010, 20, 47–52. [Google Scholar] [CrossRef]
- Li, C.; Xu, J.H.; Pan, J.Z.; Ma, C. Plastic zone distribution laws and its types of surrounding rock in large-span roadway. Int. J. Min. Sci. Technol. 2012, 22, 23–28. [Google Scholar] [CrossRef]
- Shen, B. Coal mine roadway stability in soft rock: A case study. Rock Mech. Rock Eng. 2014, 47, 2225–2238. [Google Scholar] [CrossRef]
- Jiang, L.S.; Mitri, H.S.; Ma, N.J.; Zhao, X.D. Effect of foundation rigidity on stratified roadway roof stability in underground coal mines. Arab. J. Geosci. 2016, 9. [Google Scholar] [CrossRef]
- Jiang, L.S.; Sainoki, A.; Mitri, H.S.; Ma, N.J.; Liu, H.T.; Hao, Z. Influence of fracture-induced weakening on coal mine gateroad stability. Int. J. Rock Mech. Min. Sci. 2016, 88, 307–317. [Google Scholar] [CrossRef]
- Li, H.; Lin, B.Q.; Hong, Y.D.; Gao, Y.B.; Yang, W.; Liu, T.; Wang, R.; Huang, Z.B. Effects of in-situ stress on the stability of a roadway excavated through a coal seam. Int. J. Rock Mech. Min. Sci. 2017, 27, 917–927. [Google Scholar] [CrossRef]
- Jia, H.S.; Pan, K.; Liu, S.W.; Peng, B.; Fan, K. Evaluation of the mechanical instability of mining roadway overburden: Research and applications. Energies 2019, 12, 4265. [Google Scholar] [CrossRef]
- Yuan, Y.; Wang, W.J.; Li, S.Q.; Zhu, Y.J. Failure mechanism for surrounding rock of deep circular roadway in coal mine based on mining-induced plastic zone. Adv. Civ. Eng. 2018, 1–14. [Google Scholar] [CrossRef]
- Hou, C.; Zhang, Y.H.; Yan, Y. Effects of coal seam dip angle on the outburst in coal roadway excavation. Int. J. Min. Sci. Technol. 2019, 29, 757–764. [Google Scholar] [CrossRef]
- Kong, P.; Jiang, L.S.; Shu, J.M.; Sainoki, A. Effect of fracture heterogeneity on rock mass stability in a highly heterogeneous underground roadway. Rock Mech. Rock Eng. 2019, 52, 4547–4564. [Google Scholar] [CrossRef]
- Guo, X.F.; Zhao, Z.Q.; Gao, X.; Wu, X.Y.; Ma, N.J. Analytical solutions for characteristic radii of circular roadway surrounding rock plastic zone and their application. Int. J. Min. Sci. Technol. 2019, 29, 263–272. [Google Scholar] [CrossRef]
- Guo, X.F.; Zhao, Z.Q.; Gao, X.; Ma, Z.K. Directional sharp-point failure mechanism of rocks surrounding underground circular cavities subjected to large-scale failure. Math. Probl. Eng. 2019, 6, 1–19. [Google Scholar] [CrossRef]
- Guo, X.F.; Zhao, Z.Q.; Gao, X.; Ma, Z.K.; Ma, N.J. The criteria of underground rock structure failure and its implication on rockburst in roadway: A numerical method. Shock Vib. 2019, 1–12. [Google Scholar] [CrossRef]
- Zhao, Z.Q.; Jia, H.S.; Peng, B.; Dong, Y.Y. Tunnel surrounding rock deformation characteristics and control in deep coal mining. Geomaterials 2013, 3, 24–27. [Google Scholar] [CrossRef]
- Liu, H.T.; Qiao, B.Y.; Ma, N.J. Stability analysis and design of roadways in adjacent seams: A case study from Tashan coal mine in China. Arab. J. Geosci. 2020, 13, 308. [Google Scholar] [CrossRef]
- Kang, H. Study on characteristics of underground in-situ stress distribution in Shanxi coal mining fields. Chin. J. Geophys. 2009, 52, 1782–1792. [Google Scholar]
- Feng, G.R.; Wang, P.F. Simulation of recovery of upper remnant coal pillar while mining the ultra-close lower panel using longwall top coal caving. Int. J. Min. Sci. Technol. 2020, 30, 55–61. [Google Scholar] [CrossRef]
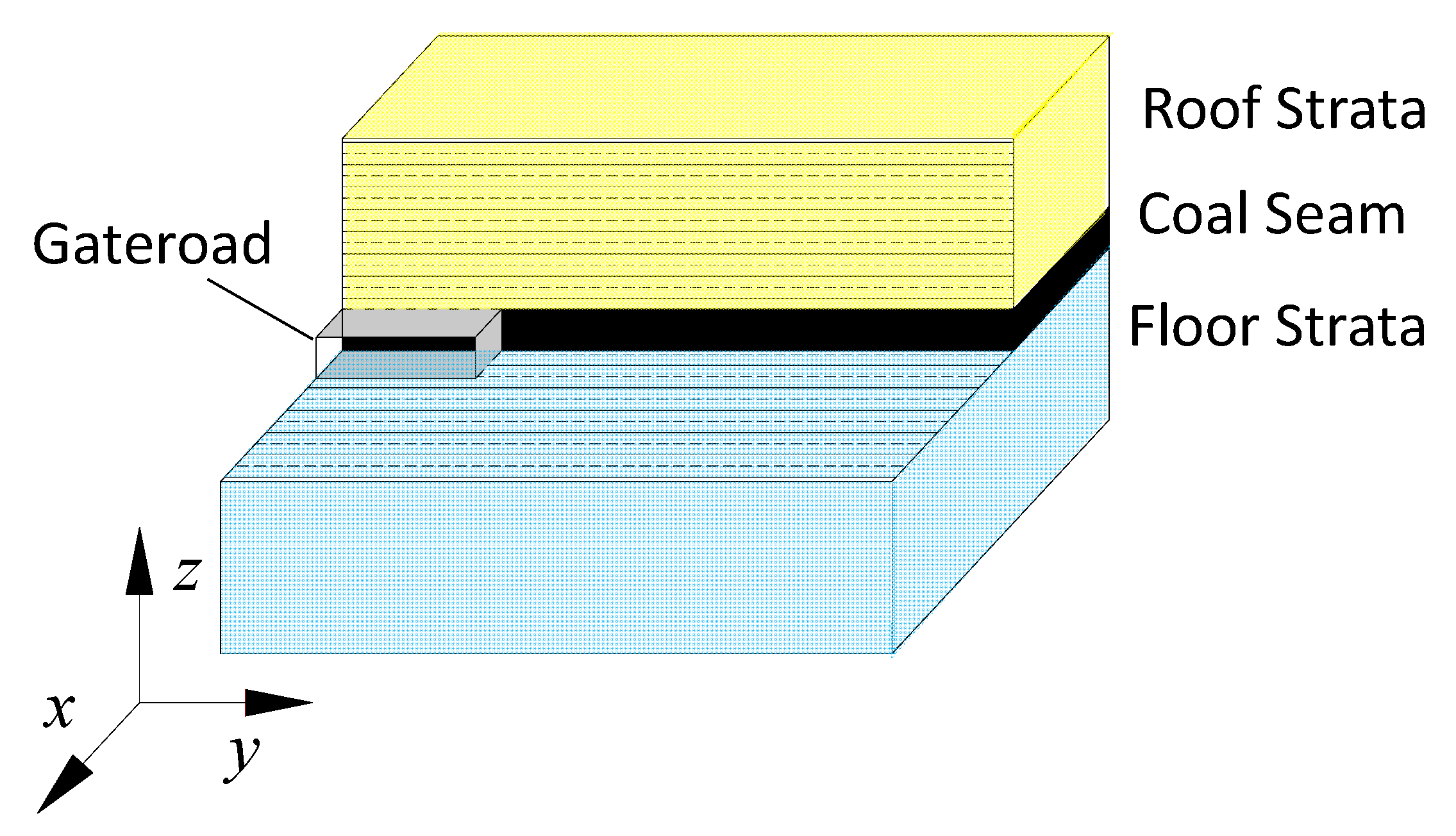
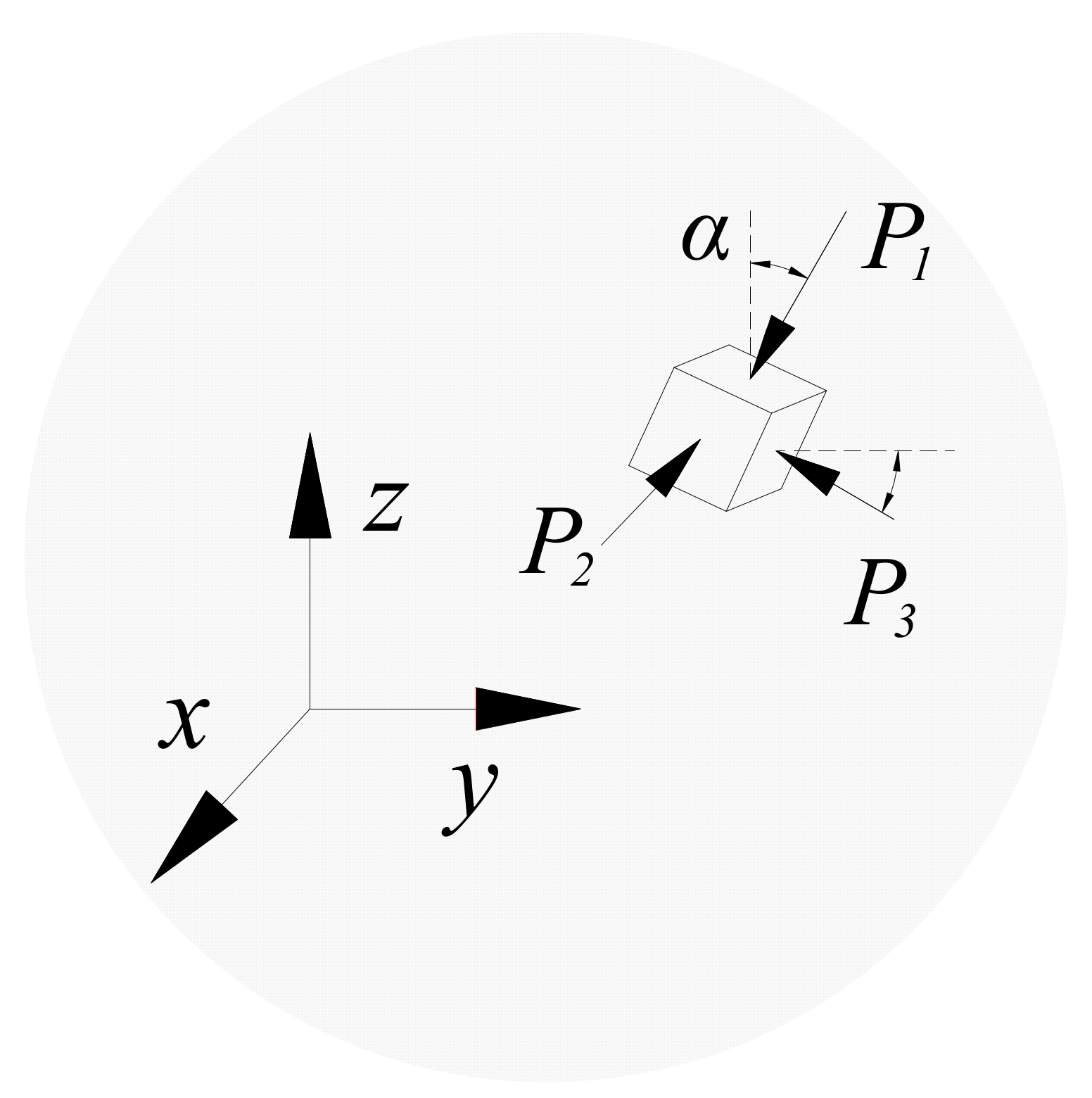




| Lithology | Thickness/m | Friction Angle/(°) | Cohesion/MPa | Density/(kg/m3) | Shear Modulus/GPa | Bulk Modulus/GPa | |
|---|---|---|---|---|---|---|---|
| Roof | 23 | 38 | 6 | 2600 | 8.0 | 8.2 | |
| Ⅰ | 4 | 25 | 1.0 | 1050 | 4.7 | 5.2 | |
| Coal | Ⅱ | 4 | 30 | 2 | 1100 | 4.9 | 5.7 |
| Ⅲ | 4 | 35 | 3 | 1300 | 5.2 | 6.1 | |
| Floor | 23 | 40 | 6.5 | 2700 | 8.0 | 8.5 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, H.; Guo, L.; Zhao, X.; Wang, P. Effect of Principal Stress Field on the Development of Plastic Zone ahead of the Gateroad. Energies 2020, 13, 4356. https://doi.org/10.3390/en13174356
Liu H, Guo L, Zhao X, Wang P. Effect of Principal Stress Field on the Development of Plastic Zone ahead of the Gateroad. Energies. 2020; 13(17):4356. https://doi.org/10.3390/en13174356
Chicago/Turabian StyleLiu, Hongtao, Linfeng Guo, Xidong Zhao, and Pengfei Wang. 2020. "Effect of Principal Stress Field on the Development of Plastic Zone ahead of the Gateroad" Energies 13, no. 17: 4356. https://doi.org/10.3390/en13174356
APA StyleLiu, H., Guo, L., Zhao, X., & Wang, P. (2020). Effect of Principal Stress Field on the Development of Plastic Zone ahead of the Gateroad. Energies, 13(17), 4356. https://doi.org/10.3390/en13174356




