An Integrated Three-Level Synergetic and Reliable Optimization Method Considering Heat Transfer Process, Component, and System
Abstract
1. Introduction
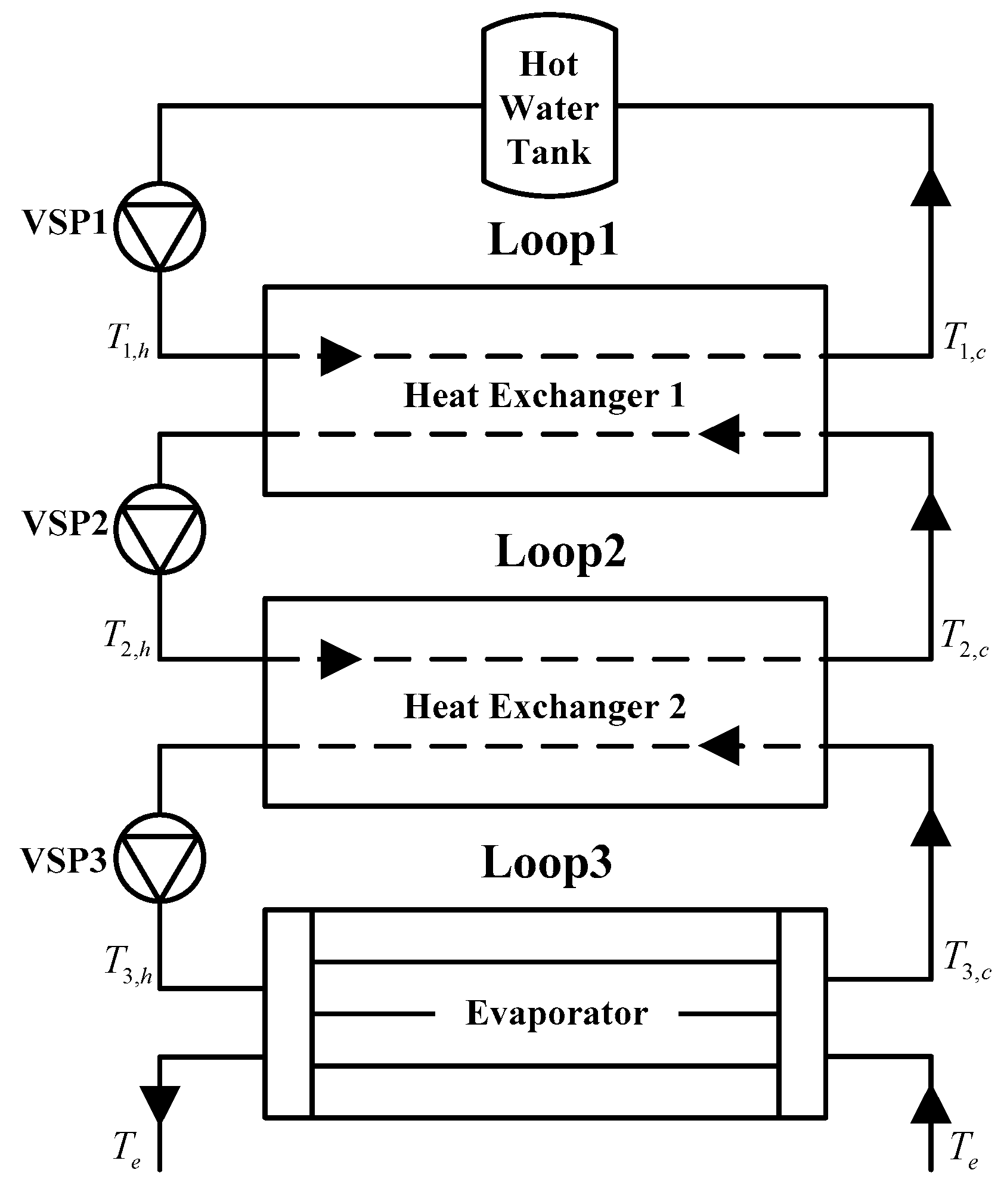
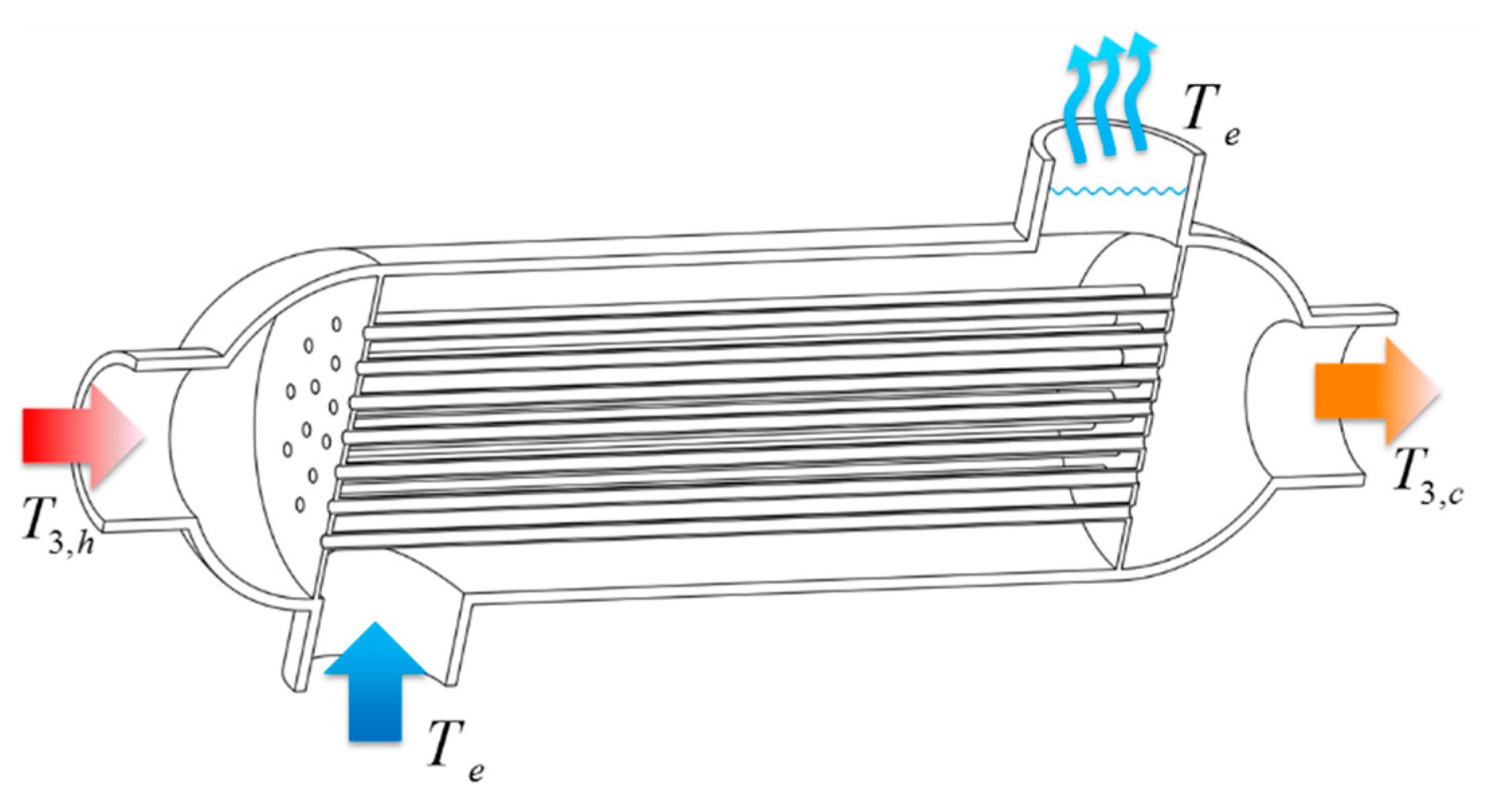
2. Physical Model and Optimization Problem of the HTS
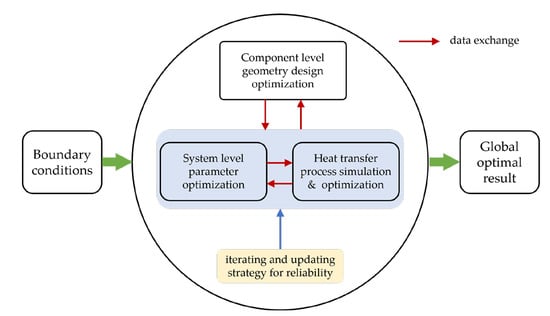
3. Synergetic Optimization Method
3.1. System Optimization Model
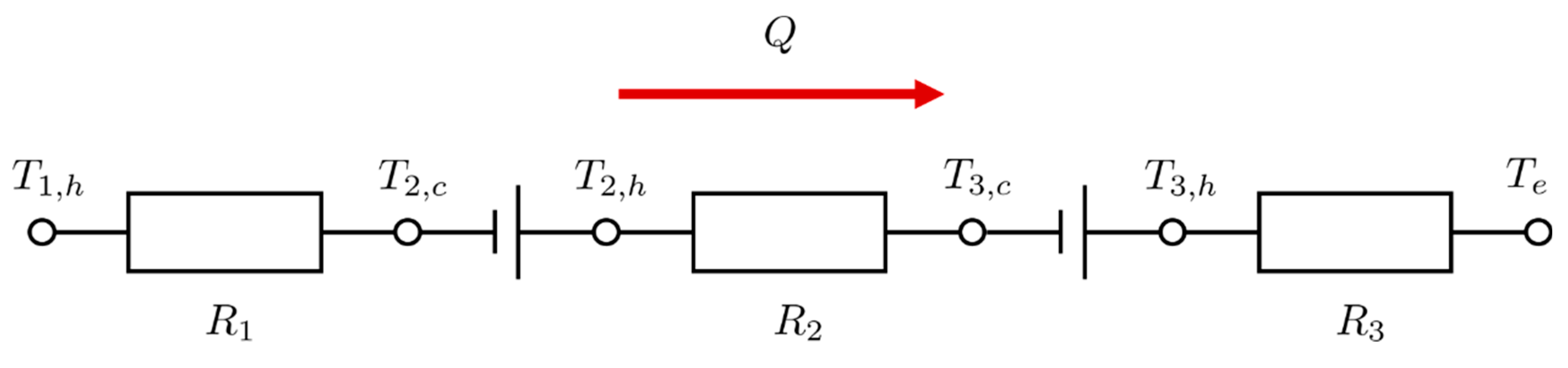
3.1.1. Heat Transfer and Fluid Flow Constraints of the HTS
3.1.2. Optimization Equations of the HTS
3.2. Simulation and Optimization of Heat Exchangers
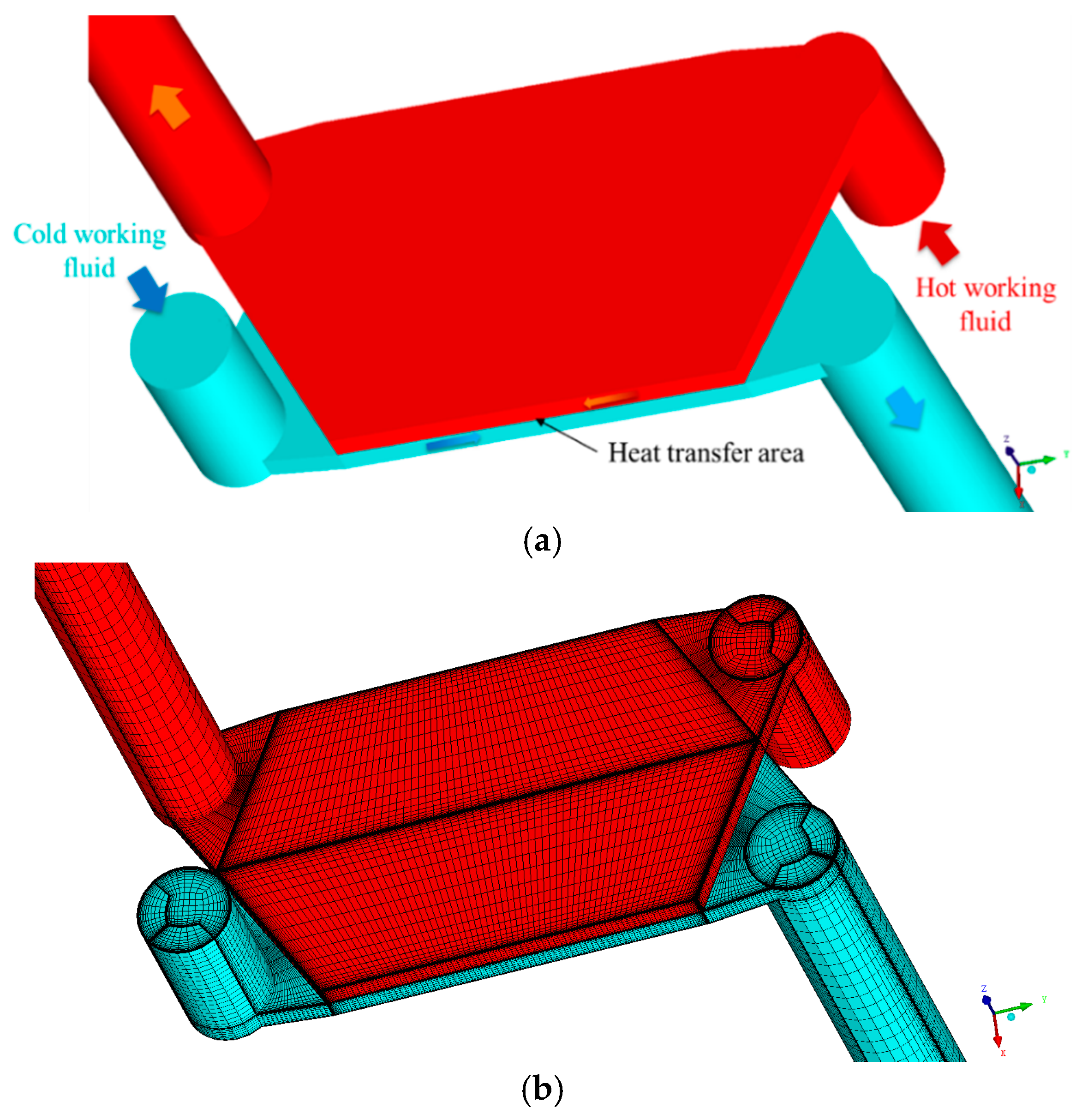
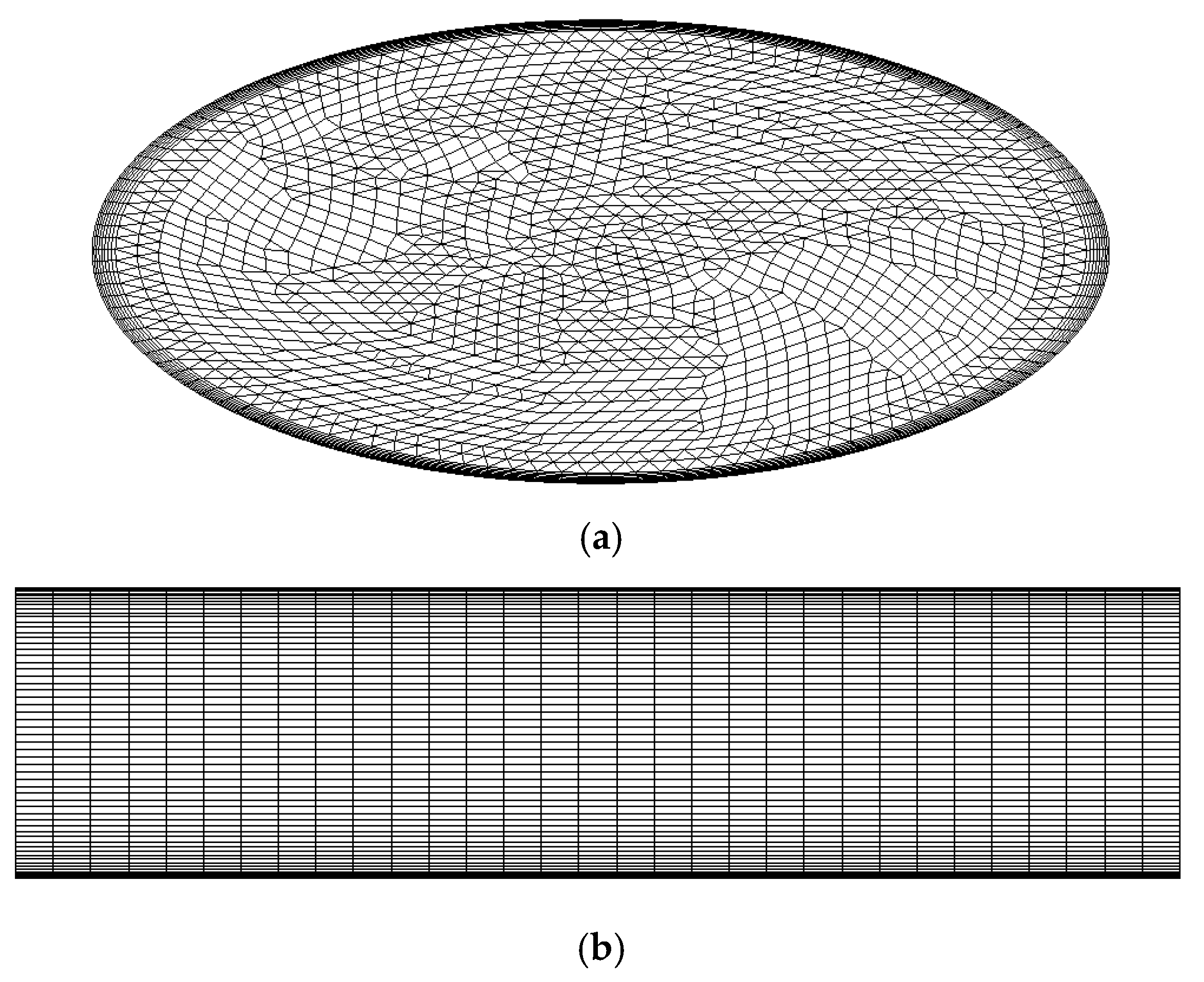
3.2.1. Simulation of Heat Exchangers HX1 and HX2
3.2.2. Convective Heat Transfer Optimization in the Evaporator
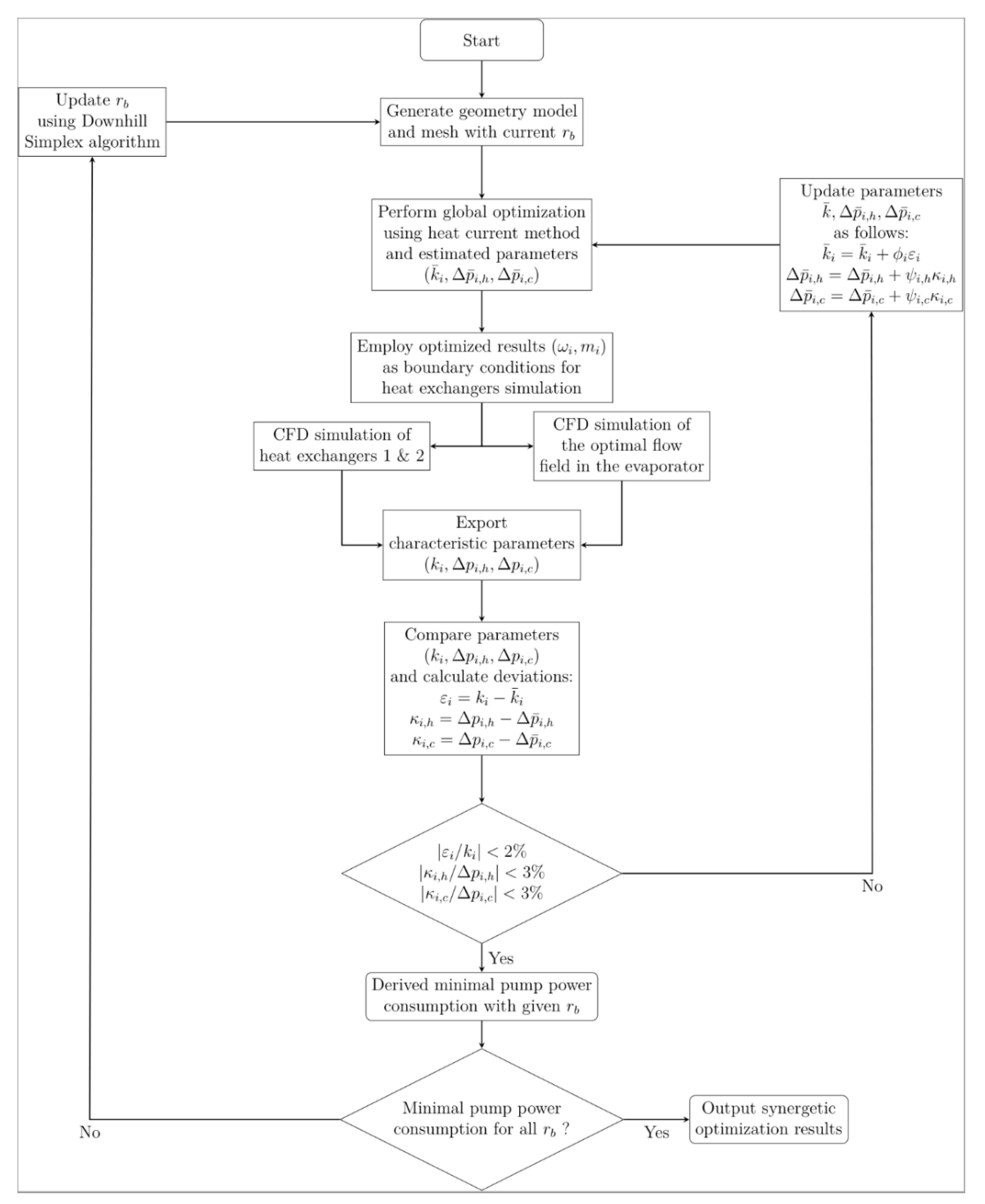
3.3. Synergetic Optimization Procedure
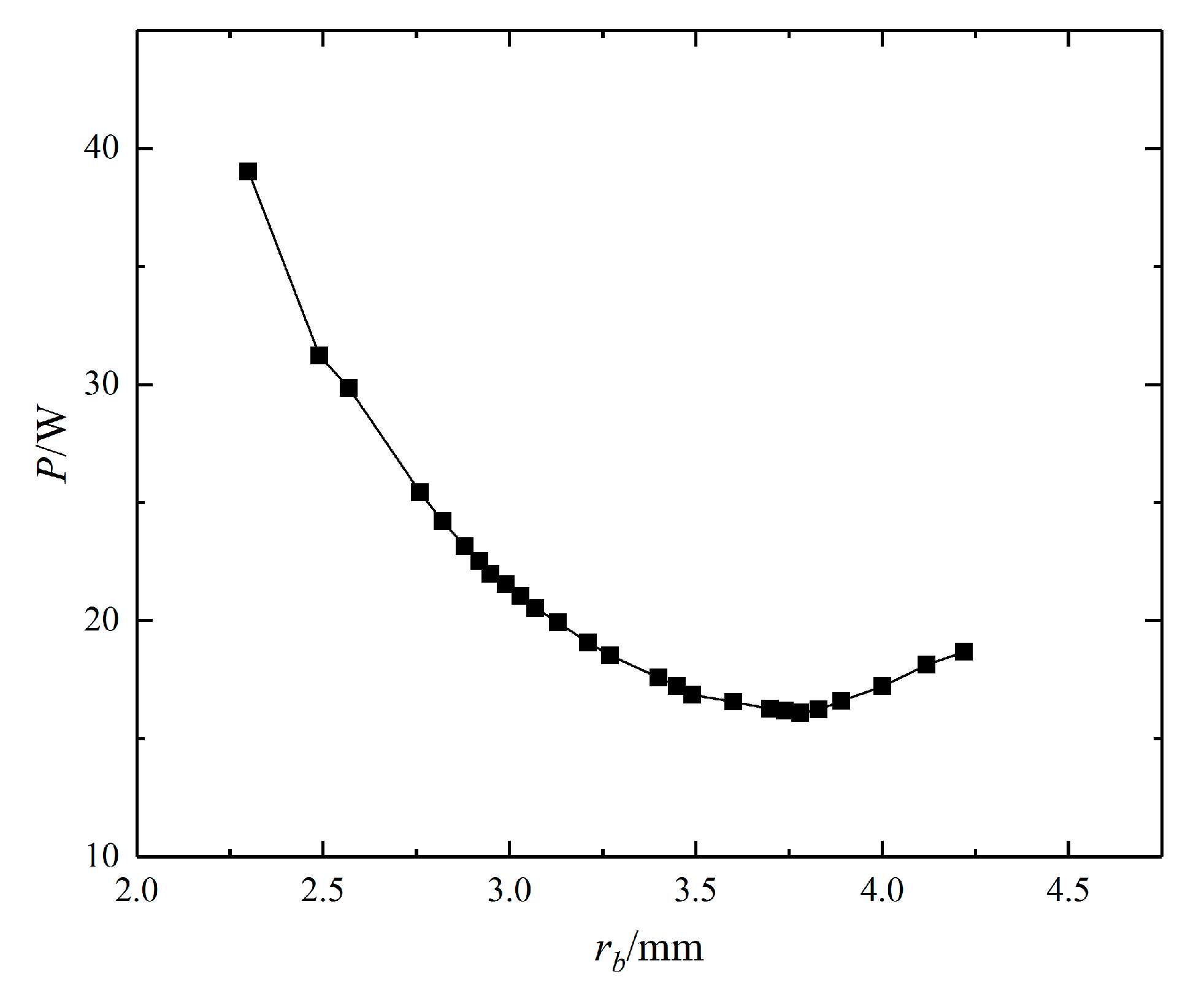
4. Optimization Results and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Partial Derivatives in Lagrange Equations
References
- Bordin, C.; Gordini, A.; Vigo, D. An optimization approach for district heating strategic network design. Eur. J. Oper. Res. 2016, 252, 296–307. [Google Scholar] [CrossRef]
- Ponce-Ortega, J.M.; Jimenez-Gutierrez, A.; Grossmann, I.E. Optimal synthesis of heat exchanger networks involving isothermal process streams. Comput. Chem. Eng. 2008, 32, 1918–1942. [Google Scholar] [CrossRef]
- Adjiman, C.S.; Androulakis, I.P.; Floudas, C.A. Global optimization of mixed-integer nonlinear problems. AIChE J. 2000, 46, 1769–1797. [Google Scholar] [CrossRef]
- Ravagnani, M.; Silva, A.; Arroyo, P.; Constantino, A. Heat exchanger network synthesis and optimisation using genetic algorithm. Appl. Therm. Eng. 2005, 25, 1003–1017. [Google Scholar] [CrossRef]
- Sameti, M.; Haghighat, F. Optimization approaches in district heating and cooling thermal network. Energy Build. 2017, 140, 121–130. [Google Scholar] [CrossRef]
- Bejan, A. Entropy generation minimization: The new thermodynamics of finite-size devices and finite-time processes. J. Appl. Phys. 1996, 79, 1191–1218. [Google Scholar] [CrossRef]
- Tsatsaronis, G.; Moran, M.J. Exergy-aided cost minimization. Energy Convers. Manag. 1997, 38, 1535–1542. [Google Scholar] [CrossRef]
- Lavric, V.; Baetens, D.; Pleşu, V.; De Ruyck, J. Entropy generation reduction through chemical pinch analysis. Appl. Therm. Eng. 2003, 23, 1837–1845. [Google Scholar] [CrossRef]
- Ahmadi, P.; Rosen, M.A.; Dincer, I. Multi-objective exergy-based optimization of a polygeneration energy system using an evolutionary algorithm. Energy 2012, 46, 21–31. [Google Scholar] [CrossRef]
- Kerdan, I.G.; Raslan, R.; Ruyssevelt, P. An exergy-based multi-objective optimisation model for energy retrofit strategies in non-domestic buildings. Energy 2016, 117, 506–522. [Google Scholar] [CrossRef]
- Song, C.; Tan, S.; Qu, F.; Liu, W.; Wu, Y. Optimization of mixed refrigerant system for LNG processes through graphically reducing exergy destruction of cryogenic heat exchangers. Energy 2019, 168, 200–206. [Google Scholar] [CrossRef]
- Marty, F.; Serra, S.; Sochard, S.; Reneaume, J.-M. Exergy Analysis and Optimization of a Combined Heat and Power Geothermal Plant. Energies 2019, 12, 1175. [Google Scholar] [CrossRef]
- Zhang, Y.; Wei, Z.; Wang, X. Inverse problem and variation method to optimize cascade heat exchange network in central heating system. J. Therm. Sci. 2017, 26, 545–551. [Google Scholar] [CrossRef]
- Chen, Q.; Hao, J.; Zhao, T. An alternative energy flow model for analysis and optimization of heat transfer systems. Int. J. Heat Mass Transf. 2017, 108, 712–720. [Google Scholar] [CrossRef]
- Chen, X.; Chen, Q.; Chen, H.; Xu, Y.-G.; Zhao, T.; Hu, K.; He, K.-L. Heat current method for analysis and optimization of heat recovery-based power generation systems. Energy 2019, 189, 116209. [Google Scholar] [CrossRef]
- Zhao, T.; Min, Y.; Chen, Q.; Hao, J. Electrical circuit analogy for analysis and optimization of absorption energy storage systems. Energy 2016, 104, 171–183. [Google Scholar] [CrossRef]
- Chen, Q.; Zhao, T. Heat recovery and storage installation in large-scale battery systems for effective integration of renewable energy sources into power systems. Appl. Therm. Eng. 2017, 122, 194–203. [Google Scholar] [CrossRef]
- Wei, H.; Ge, Z.; Yang, L.; Du, X. Entransy based optimal adjustment of louvers for anti-freezing of natural draft dry cooling system. Int. J. Heat Mass Transf. 2019, 134, 468–481. [Google Scholar] [CrossRef]
- Shao, W.; Chen, Q.; Zhang, M.-Q. Operation optimization of variable frequency pumps in compound series-parallel heat transfer systems based on the power flow method. Energy Sci. Eng. 2018, 6, 385–396. [Google Scholar] [CrossRef]
- Ahmadi, P.; Hajabdollahi, H.; Dincer, I. Cost and Entropy Generation Minimization of a Cross-Flow Plate Fin Heat Exchanger Using Multi-Objective Genetic Algorithm. J. Heat Transf. 2010, 133, 1–10. [Google Scholar] [CrossRef]
- Xie, G.; Song, Y.; Asadi, M.; Lorenzini, G. Optimization of Pin-Fins for a Heat Exchanger by Entropy Generation Minimization and Constructal Law. J. Heat Transf. 2015, 137, 1–9. [Google Scholar] [CrossRef]
- Misra, R.; Jakhar, S.; Agrawal, K.K.; Sharma, S.; Jamuwa, D.K.; Soni, M.S.; Das Agrawal, G. Field investigations to determine the thermal performance of earth air tunnel heat exchanger with dry and wet soil: Energy and exergetic analysis. Energy Build. 2018, 171, 107–115. [Google Scholar] [CrossRef]
- Valencia, G.; Núñez, J.; Duarte, J. Multiobjective Optimization of a Plate Heat Exchanger in a Waste Heat Recovery Organic Rankine Cycle System for Natural Gas Engines. Entropy 2019, 21, 655. [Google Scholar] [CrossRef]
- Li, K.; Wen, J.; Liu, Y.; Liu, H.; Wang, S.; Tu, J. Application of entransy theory on structure optimization of serrated fin in plate-fin heat exchanger. Appl. Therm. Eng. 2020, 173, 114809. [Google Scholar] [CrossRef]
- Wang, S.; Xiao, B.; Ge, Y.; He, L.; Li, X.; Liu, W.; Liu, Z. Optimization design of slotted fins based on exergy destruction minimization coupled with optimization algorithm. Int. J. Therm. Sci. 2020, 147, 106133. [Google Scholar] [CrossRef]
- Li, L.; Wan, H.; Gao, W.; Tong, F.; Li, H. Reliability based multidisciplinary design optimization of cooling turbine blade considering uncertainty data statistics. Struct. Multidiscip. Optim. 2018, 59, 659–673. [Google Scholar] [CrossRef]
- Sahin, A.Z. Second Law Analysis of Laminar Viscous Flow Through a Duct Subjected to Constant Wall Temperature. J. Heat Transf. 1998, 120, 76–83. [Google Scholar] [CrossRef]
- Sahin, A.Z. Thermodynamics of laminar viscous flow through a duct subjected to constant heat flux. Energy 1996, 21, 1179–1187. [Google Scholar] [CrossRef]
- Guo, Z.; Tao, W.; Shah, R. The field synergy (coordination) principle and its applications in enhancing single phase convective heat transfer. Int. J. Heat Mass Transf. 2005, 48, 1797–1807. [Google Scholar] [CrossRef]
- Tao, W.; Guo, Z.-Y.; Wang, B.-X. Field synergy principle for enhancing convective heat transfer––its extension and numerical verifications. Int. J. Heat Mass Transf. 2002, 45, 3849–3856. [Google Scholar] [CrossRef]
- Meng, J.-A.; Liang, X.-G.; Li, Z.-X. Field synergy optimization and enhanced heat transfer by multi-longitudinal vortexes flow in tube. Int. J. Heat Mass Transf. 2005, 48, 3331–3337. [Google Scholar] [CrossRef]
- Chen, Q.; Wang, M.; Pan, N.; Guo, Z.-Y. Optimization principles for convective heat transfer. Energy 2009, 34, 1199–1206. [Google Scholar] [CrossRef]
- Chen, X.; Zhao, T.; Zhang, M.-Q.; Chen, Q. Entropy and entransy in convective heat transfer optimization: A review and perspective. Int. J. Heat Mass Transf. 2019, 137, 1191–1220. [Google Scholar] [CrossRef]
- Rohsenow, W.M.; Hartnett, J.P.; Ganic, E.N.; Richardson, P.D. Handbook of Heat Transfer Fundamentals (Second Edition). J. Appl. Mech. 1986, 53, 232–233. [Google Scholar] [CrossRef]
- Gómez, A.; Montañés, C.; Camara, M.; Cubero, A.; Fueyo, N.; Muñoz, J.M. An OpenFOAM-based model for heat-exchanger design in the Cloud. Appl. Therm. Eng. 2018, 139, 239–255. [Google Scholar] [CrossRef]
- Dogan, S.; Darici, S.; Ozgoren, M. Numerical comparison of thermal and hydraulic performances for heat exchangers having circular and elliptic cross-section. Int. J. Heat Mass Transf. 2019, 145, 118731. [Google Scholar] [CrossRef]
- Zhao, T.; Liu, D.; Chen, Q. A collaborative optimization method for heat transfer systems based on the heat current method and entransy dissipation extremum principle. Appl. Therm. Eng. 2019, 146, 635–647. [Google Scholar] [CrossRef]
- Chung, T.J. Computational Fluid Dynamics, 2nd ed.; Cambridge University Press: Cambridge, UK, 2010; ISBN 978-0-511-78006-6. [Google Scholar]
- Reddy, J.N. Energy Principles and Variational Methods in Applied Mechanics, 3rd ed.; Wiley: Hoboken Chichester, NJ, USA, 2017; ISBN 978-1-119-08737-3. [Google Scholar]
- Barnard, R.W.; Pearce, K.; Schovanec, L. Inequalities for the Perimeter of an Ellipse. J. Math. Anal. Appl. 2001, 260, 295–306. [Google Scholar] [CrossRef]
- Chen, Q.; Liang, X.-G.; Guo, Z. Entransy theory for the optimization of heat transfer—A review and update. Int. J. Heat Mass Transf. 2013, 63, 65–81. [Google Scholar] [CrossRef]
- Liu, D.; Chen, Q.; He, K.-L.; Chen, X. An integrated system-level and component-level optimization of heat transfer systems based on the heat current method. Int. J. Heat Mass Transf. 2019, 131, 623–632. [Google Scholar] [CrossRef]
- Hamid, M.O.; Zhang, B.; Yang, L. Application of field synergy principle for optimization fluid flow and convective heat transfer in a tube bundle of a pre-heater. Energy 2014, 76, 241–253. [Google Scholar] [CrossRef]


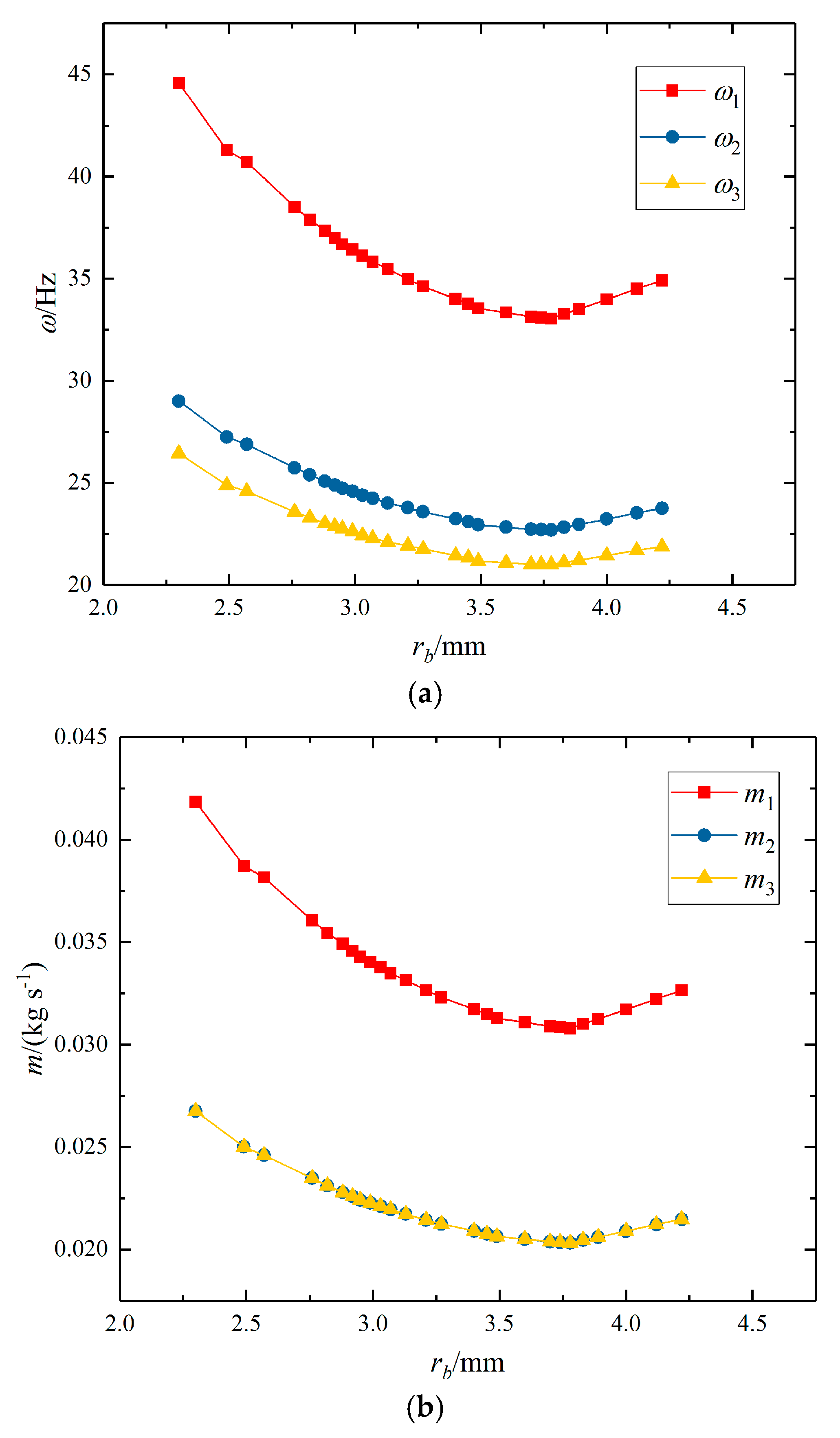
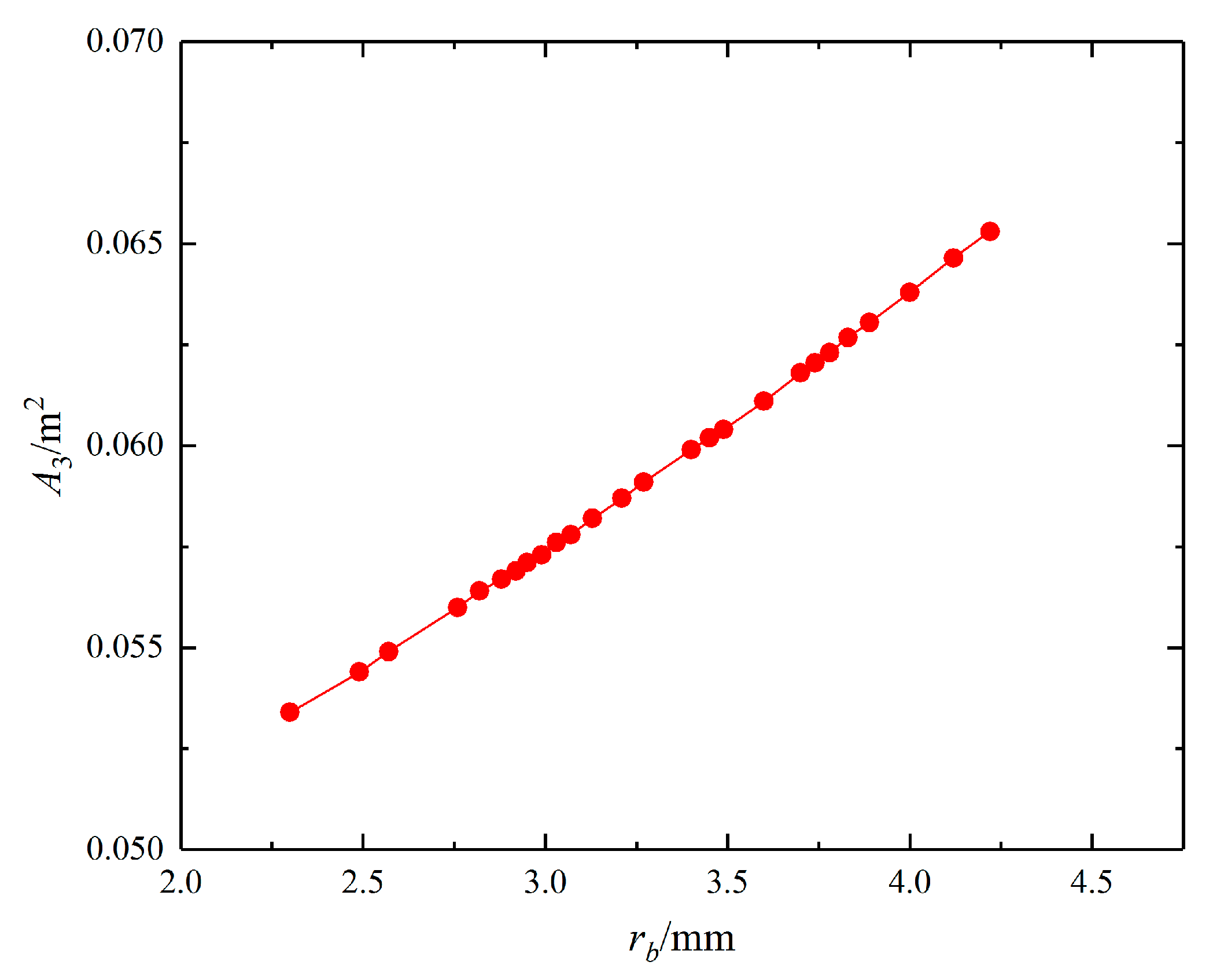

| Q (W) | T1,h (K) | Te (K) | A1 (m2) | A2 (m2) | ra (mm) |
|---|---|---|---|---|---|
| 1000 | 311.65 | 275.65 | 0.076 | 0.076 | 5.0 |
| Hs,1 (m) | Hs,2 (m) | Hs,3 (m) | b1 (m s2 kg−2) | b2 (m s2 kg−2) | b3 (m s2 kg−2) |
|---|---|---|---|---|---|
| 0.58 | 0.396 | 0.37 | 38,870 | 27,505 | 29,413 |
| A0,1 | a1,1 | a2,1 | a0,2 | a1,2 | a2,2 | a0,3 | a1,3 | a2,3 |
|---|---|---|---|---|---|---|---|---|
| 0.03591 | −1.256 | −267.4 | 0.0253 | −0.8877 | −662.2 | 0.03168 | −0.4897 | −241.5 |
| Geometry Optimization Included? | P (W) | rb (mm) | A3 (m2) | ω1 (Hz) | ω2 (Hz) | ω3 (Hz) | m1 (kg s−1) | m2 (kg s−1) | m3 (kg s−1) |
|---|---|---|---|---|---|---|---|---|---|
| Yes | 16.2 | 3.75 | 0.0623 | 31.1 | 22.8 | 21.1 | 0.0309 | 0.0204 | 0.0204 |
| No | 19.35 | 5.0 | 0.071 | 32.6 | 23.5 | 22.5 | 0.0324 | 0.0216 | 0.0227 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, T.; Liu, D.; He, K.-L.; Chen, X.; Chen, Q. An Integrated Three-Level Synergetic and Reliable Optimization Method Considering Heat Transfer Process, Component, and System. Energies 2020, 13, 4112. https://doi.org/10.3390/en13164112
Zhao T, Liu D, He K-L, Chen X, Chen Q. An Integrated Three-Level Synergetic and Reliable Optimization Method Considering Heat Transfer Process, Component, and System. Energies. 2020; 13(16):4112. https://doi.org/10.3390/en13164112
Chicago/Turabian StyleZhao, Tian, Di Liu, Ke-Lun He, Xi Chen, and Qun Chen. 2020. "An Integrated Three-Level Synergetic and Reliable Optimization Method Considering Heat Transfer Process, Component, and System" Energies 13, no. 16: 4112. https://doi.org/10.3390/en13164112
APA StyleZhao, T., Liu, D., He, K.-L., Chen, X., & Chen, Q. (2020). An Integrated Three-Level Synergetic and Reliable Optimization Method Considering Heat Transfer Process, Component, and System. Energies, 13(16), 4112. https://doi.org/10.3390/en13164112