Performance and Efficiency Analysis of an HT-PEMFC System with an Absorption Chiller for Tri-Generation Applications
Abstract
1. Introduction
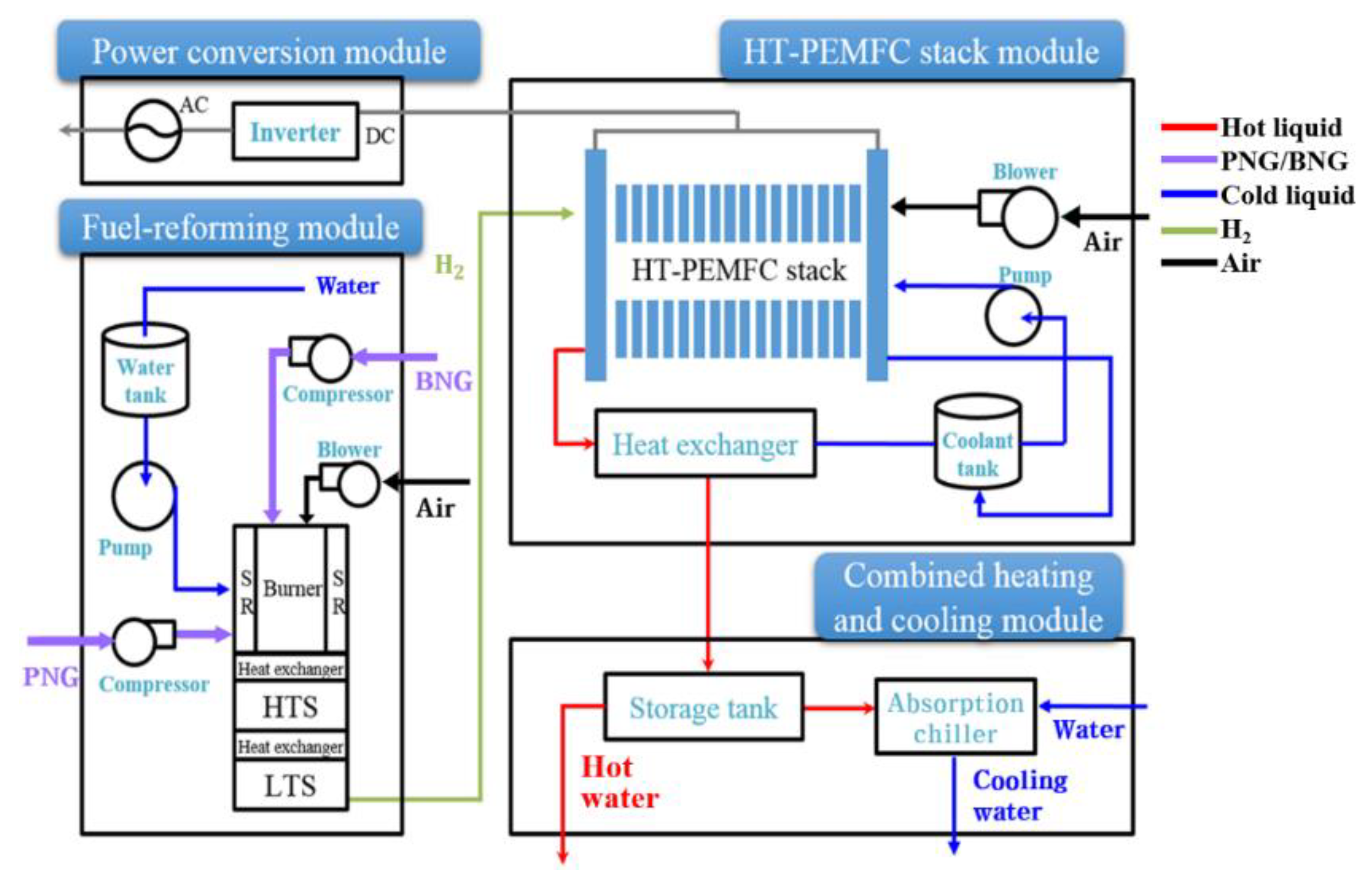
2. Analytical Model
2.1. Model Assumptions
- The natural gas constitutes 100% methane (CH4). All of the impurities in the natural gas are removed before utilization during the fuel pretreatment process. Hence, the minor components in the fuel and their properties are ignored.
- Peng–Robinson’s equation of state is followed by the individual gases and their mixtures to facilitate the relatively simple and accurate analysis of the natural gas reacting system.
- The operations of tri-generation system and its components are at the steady-state condition.
- The desulphurization component is included in the HT-PEMFC system to alleviate the sulfur poisoning of metal catalysts, such as Ni and Rh, inside the steam reforming (SR) reactor. Hence, the catalyst deactivation effect can be neglected.
- The LiBr solution in the absorber and generator, and any refrigerant (water) in the condenser and evaporator are under thermodynamic equilibrium corresponding to their temperatures and pressures. The temperature, pressure, and concentration within the each component of LiBr-H2O absorption chiller are assumed to be uniform.
- The water vapor that leaves the evaporator is assumed to be fully saturated, whereas the water leaves the condenser as saturated liquid.
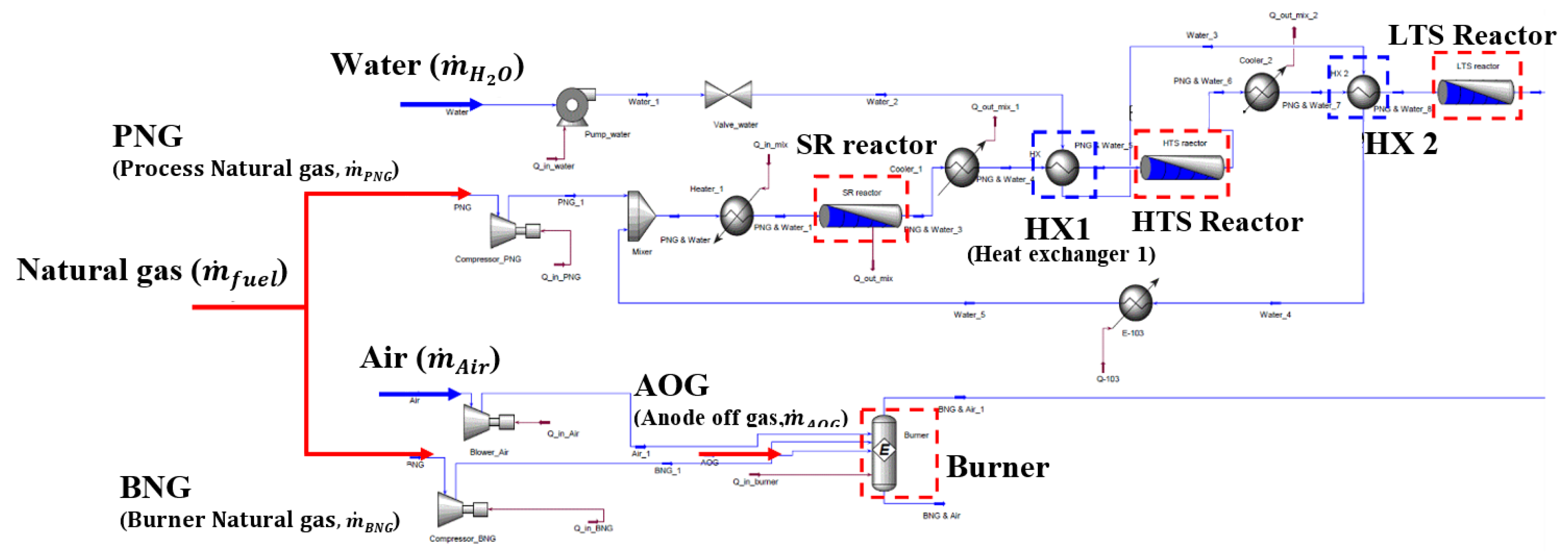
2.2. Fuel-Reforming Module
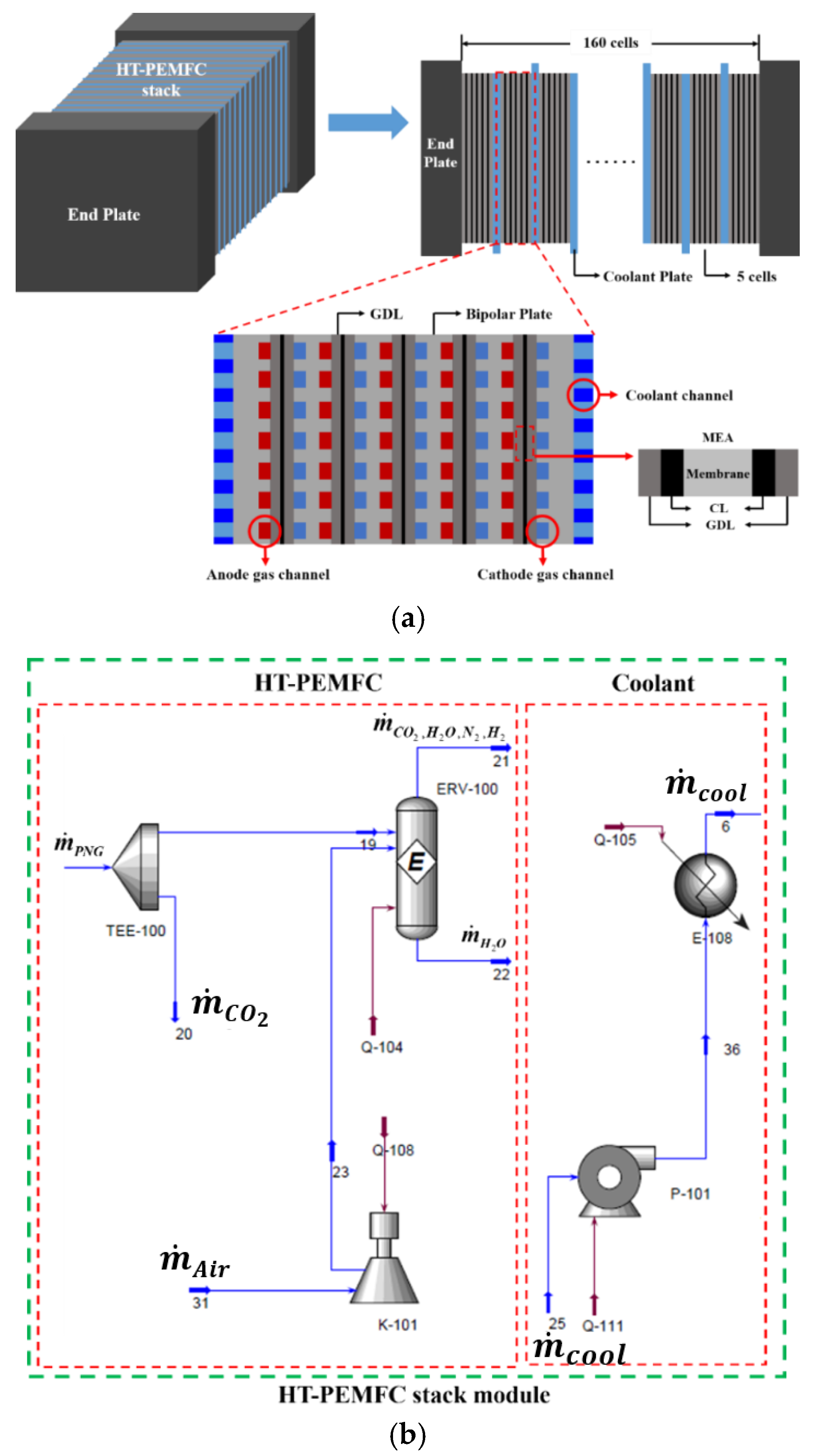
2.3. HT-PEMFC Stack Module
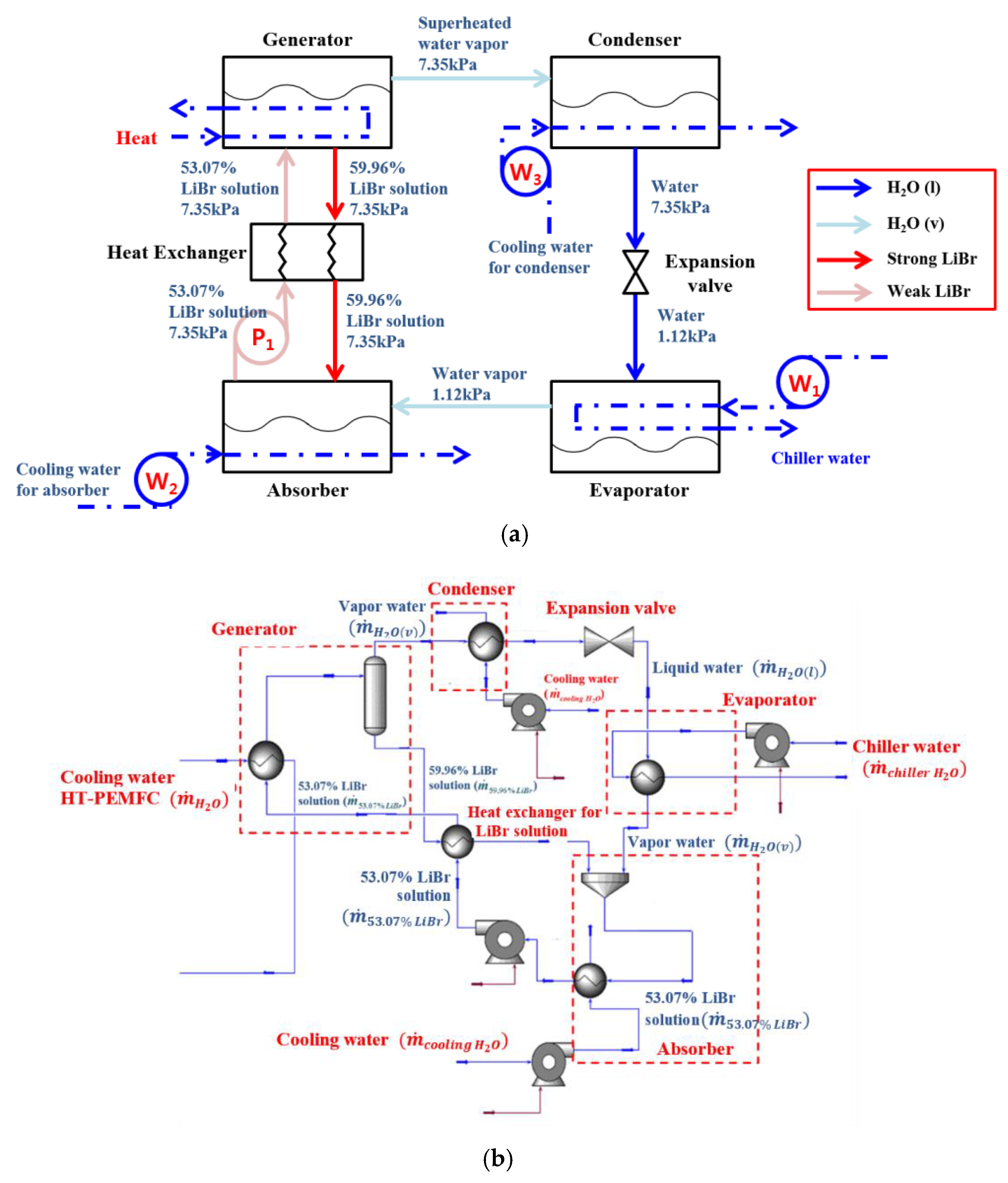
2.4. Single-Effect Absorption Chiller
2.5. Heat-Recovery Module
2.6. Operating Conditions
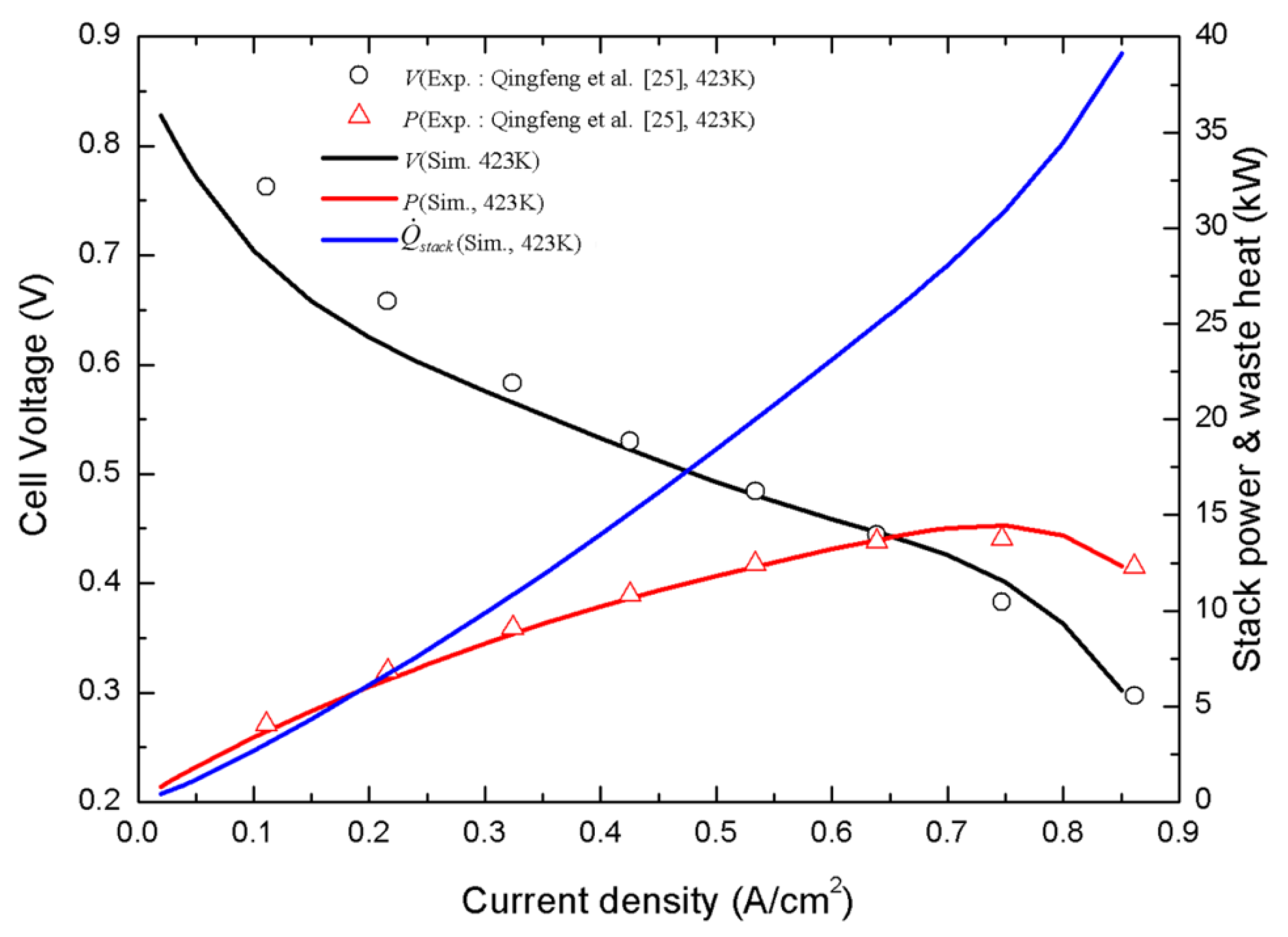
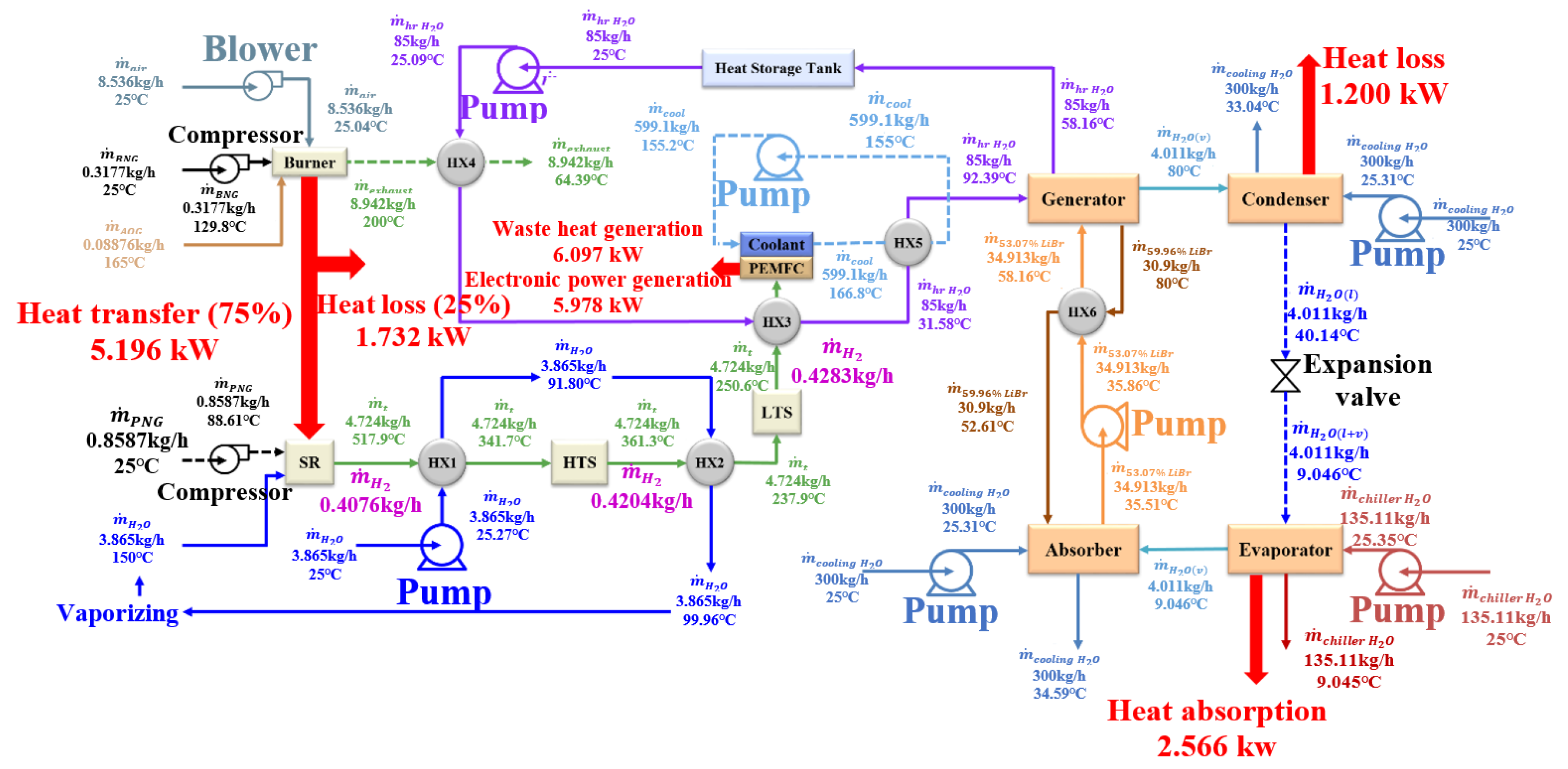
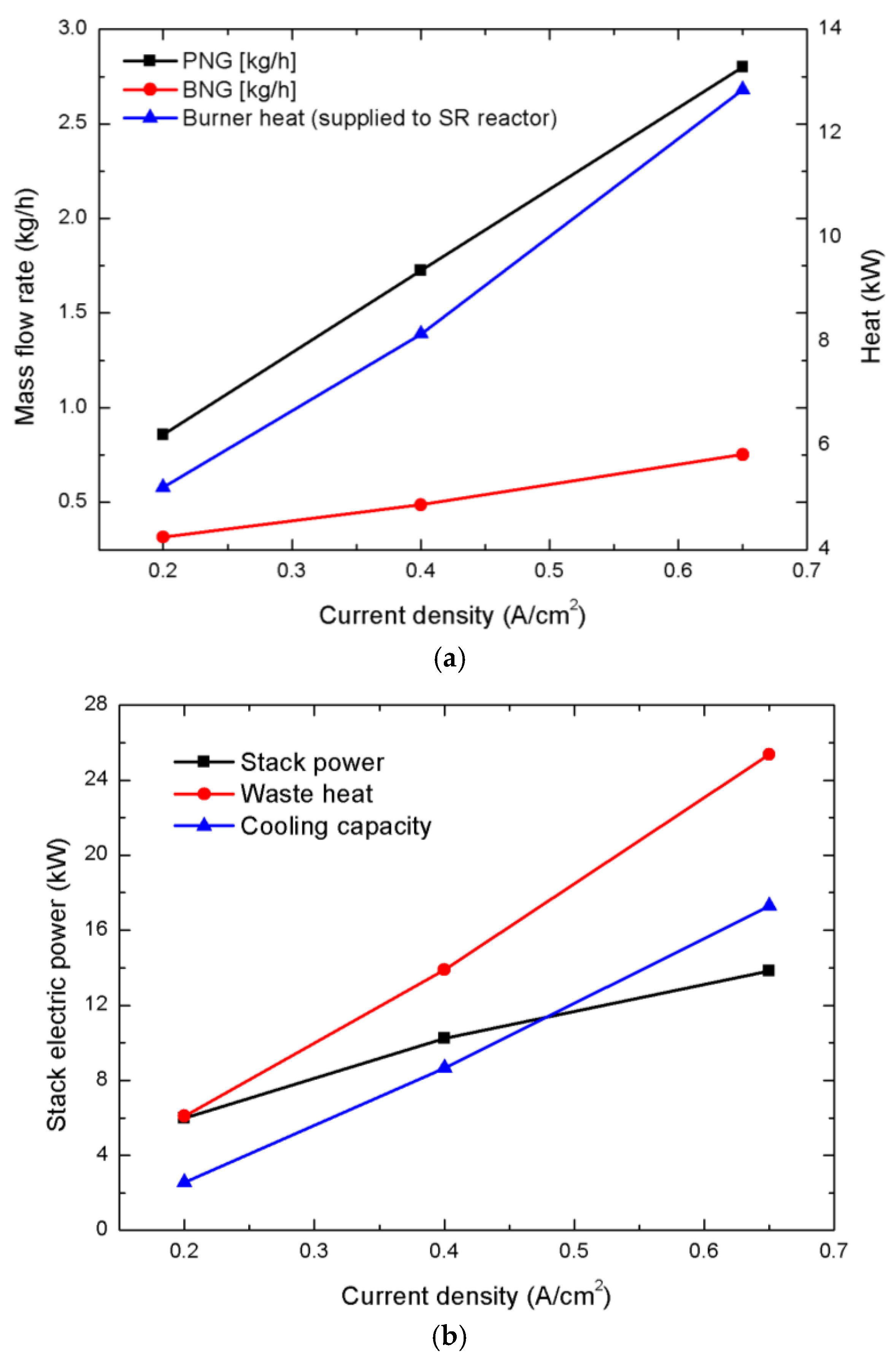
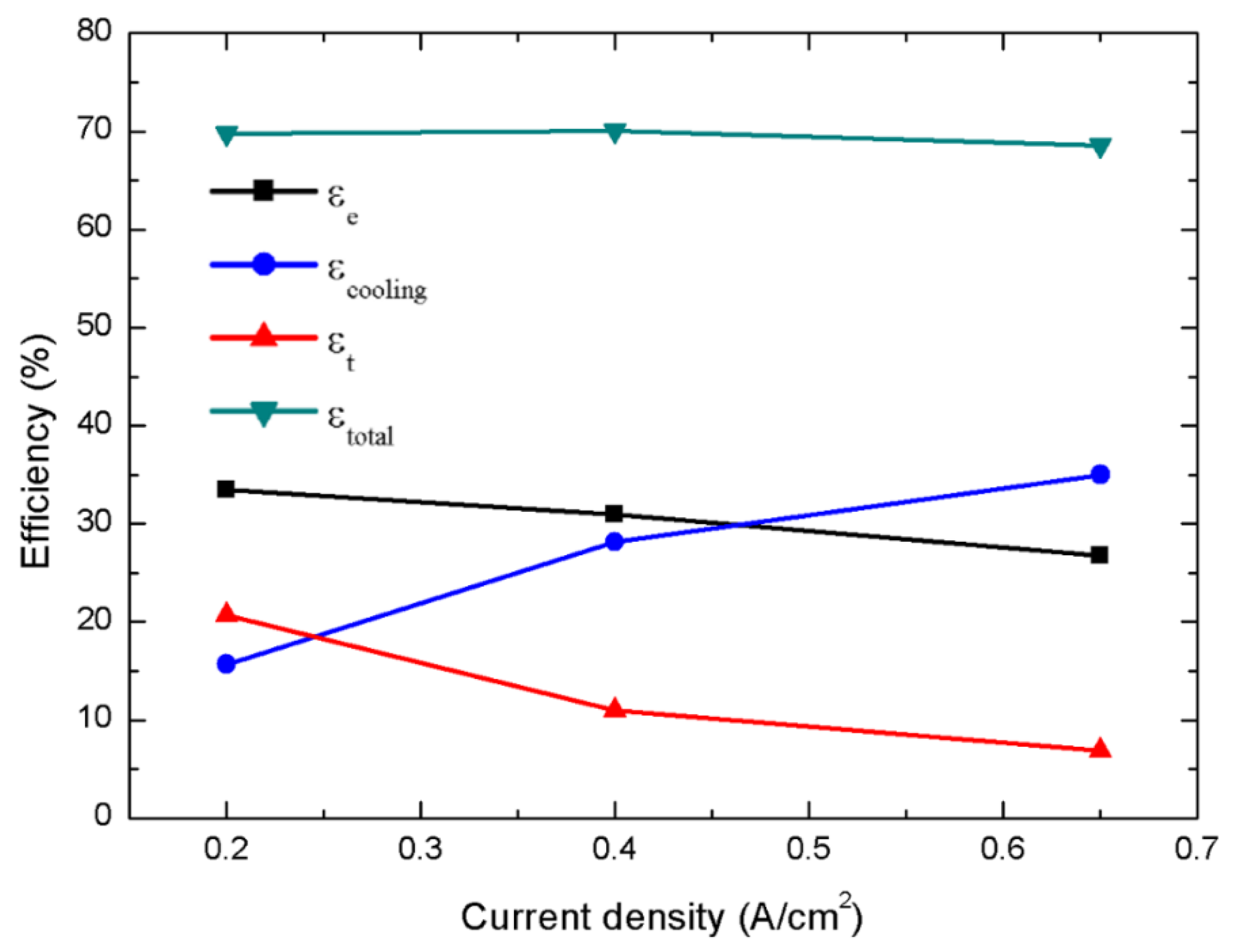
3. Results and Discussion
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Nomenclature
| area, m2 | |
| pre-exponential factor of reaction i | |
| pre-exponential factor of the adsorption constant for species j | |
| power consumption of an individual BOP component i, W | |
| concentration, mol·m−3 | |
| mass diffusivity of a species, m2∙s−1 | |
| activation energy of reaction i, kJ·mol−1 | |
| Faraday constant, C·mol−1 | |
| specific enthalpy of species j, kJ·mol−1 | |
| specific enthalpy change of reaction i, kJ·mol−1 | |
| adsorption specific enthalpy of species j, kJ·mol−1 | |
| current density, A/cm2 | |
| rate of reaction i | |
| adsorption constant for species j | |
| equilibrium constant of reaction i | |
| lower heating value, kJ·kg−1 | |
| volume flow rate, m3·s−1 | |
| molar weight, kg·kmol−1 | |
| mass flow rate, kg·h−1 | |
| number of the cell | |
| pressure, Pa | |
| stack power, W | |
| total heat generation from a stack, W | |
| reaction rate i, kmol∙(kg cat·h)−1 | |
| universal gas constant, 8.314 J∙(mol⋅K)−1 | |
| equivalent contact resistance to electron conduction, Ω | |
| area-specific resistance from proton transport, Ω | |
| temperature, K | |
| entire heat transfer coefficient, W∙m−2⋅K−1 | |
| thermodynamic equilibrium potential, V | |
| voltage, V | |
| number of electrons in the electrochemical reaction | |
| Greek letters | |
| transfer coefficient | |
| efficiency | |
| porosity | |
| density, kg⋅m−3 | |
| overpotential, V | |
| proton conductivity, S⋅m−1 | |
| stoichiometry flow ratio | |
| Subscripts & abbreviations | |
| anode | |
| activation | |
| anode off gas | |
| backward | |
| burner | |
| burner natural gas | |
| balance of plant | |
| Butler-Volmer | |
| cathode | |
| chiller | |
| concentration | |
| coolant | |
| cooling | |
| Coefficient of Performance | |
| combined heat, cooling and power | |
| Combined Heating and Cooling | |
| catalyst layer | |
| electric | |
| Equivalence Ratio | |
| forward | |
| Fuel Air Ratio | |
| gas diffusion layer | |
| gas turbine | |
| heat recovery | |
| water | |
| Heat Exchanger | |
| Hydrogen Oxidation Reaction | |
| High temperature | |
| High temperature-shifts | |
| reaction number | |
| in | |
| species | |
| BOP component | |
| limiting current density | |
| logarithmic mean temperature difference | |
| Low temperature-shifts | |
| Lithium bromide | |
| Low temperature | |
| logarithmic mean temperature | |
| membrane | |
| membrane electrode assembly | |
| standard condition | |
| ohmic | |
| optimum | |
| out | |
| oxygen | |
| Oxygen Reduction Reaction | |
| process natural gas | |
| Polybenzimidazole | |
| Phosphoric Acid | |
| Polymer electrolyte membrane fuel cell | |
| reference value | |
| supply | |
| Steam to carbon ratio | |
| Steam reforming | |
| Solid Oxide Fuel Cells | |
| thermal | |
| total | |
| tri-gen | tri-generation system |
| Water Gas Shift | |
References
- Hinaje, M.; Raël, S.; Noiying, P.; Nguyen, D.A.; Davat, B. An Equivalent Electrical Circuit Model of Proton Exchange Membrane Fuel Cells Based on Mathematical Modelling. Energies 2012, 5, 2724–2744. [Google Scholar] [CrossRef]
- Araya, S.S.; Andreasen, S.J.; Kær, S.K. Experimental Characterization of the Poisoning Effects of Methanol-Based Reformate Impurities on a PBI-Based High Temperature PEM Fuel Cell. Energies 2012, 5, 4251–4267. [Google Scholar] [CrossRef]
- Wang, F.C.; Lin, K.M. Impacts of Load Profiles on the Optimization of Power Management of a Green Building Employing Fuel Cells. Energies 2019, 12, 57. [Google Scholar] [CrossRef]
- Martin, I.S.; Ursua, A.; Sanchis, P. Modelling of PEM Fuel Cell Performance: Steady-State and Dynamic Experimental Validation. Energies 2014, 7, 670–700. [Google Scholar] [CrossRef]
- Wu, D.W.; Wang, R.Z. Combined cooling, heating and power: A review. Prog. Energy Combust. 2006, 32, 459–495. [Google Scholar] [CrossRef]
- Cozzolino, R. Thermodynamic Performance Assessment of a Novel Micro-CCHP System Based on a Low Temperature PEMFC Power Unit and a Half-Effect Li/Br Absorption Chiller. Energies 2018, 11, 315. [Google Scholar] [CrossRef]
- Gordon, J.M.; Ng, K.C. A general thermodynamic model for absorption chillers: Theory and experiment. Appl. Therm. Eng. 1995, 15, 73–83. [Google Scholar] [CrossRef]
- Xu, Y.; Zhang, S.; Xiao, Y. Modeling the dynamic simulation and control of a single effect LiBr-H2O Absorption chiller. Appl. Therm. Eng. 2016, 107, 1183–1191. [Google Scholar] [CrossRef]
- Martínez, J.C.; Martinez, P.J.; Bujedo, L.A. Development and experimental validation of a simulation model to reproduce the performance of a 17.6 kW LiBr-water absorption chiller. Renew. Energy 2016, 86, 473–482. [Google Scholar] [CrossRef]
- Ochoa, A.A.V.; Dutra, J.C.C.; Henríquez, J.R.G.; Dos Santos, C.A.C. Dynamic study of a single effect absorption chiller using the pair LiBr/H2O. Energy Convers. Manag. 2016, 108, 30–42. [Google Scholar] [CrossRef]
- Rubio-Maya, C.; Pacheco-Ibarra, J.J.; Belman-Flores, J.M.; Galván-González, S.R.; Mendoza-Covarrubias, C. NLP model of a LiBr-H2O absorption refrigeration system for the minimization of the annual operating cost. Appl. Therm. Eng. 2012, 37, 10–18. [Google Scholar] [CrossRef]
- Yin, H.; Qu, M.; Archer, D.H. Model based experimental performance analysis of a microscale LiBr-H2O steam-driven double-effect absorption Chiller. Appl. Therm. Eng. 2010, 30, 1741–1750. [Google Scholar] [CrossRef]
- Xu, Z.Y.; Wang, R.Z. Experimental verification of the variable effect absorption refrigeration cycle. Energy 2014, 77, 703–709. [Google Scholar] [CrossRef]
- Takezawa, S.; Wakahara, K.; Araki, T.; Onda, K.; Nagata, S. Cycle analysis using exhaust heat of SOFC and turbine combined cycle by absorption chiller. Electr. Eng. Jpn. 2009, 167, 49–55. [Google Scholar] [CrossRef]
- Malico, I.; Carvalhinho, A.P.; Tenreiro, J. Design of a trigeneration system using a high-temperature fuel cell. Int. J. Energy Res. 2009, 33, 144–151. [Google Scholar] [CrossRef]
- Yu, Z.; Han, J.; Cao, X. Investigation on performance of an integrated solid oxide fuel cell and absorption chiller tri-generation system. Int. J. Hydrog. Energy 2011, 36, 12561–12573. [Google Scholar] [CrossRef]
- Burer, M.; Tanaka, K.; Favrat, D.; Yamada, K. Multi-criteria optimization of a district cogeneration plant integrating a solid oxide fuel cell–gas turbine combined cycle, heat pumps and chillers. Energy 2003, 28, 497–518. [Google Scholar] [CrossRef]
- Tse, L.K.C.; Wilkins, S.; McGlashan, N.; Urban, B.; Martinez-Botas, R. Solid oxide fuel cell/gas turbine trigeneration system for marine applications. J. Power Sources 2011, 196, 3149–3162. [Google Scholar] [CrossRef]
- Margalef, P.; Samuelsen, S. Integration of a molten carbonate fuel cell with a direct exhaust absorption chiller. J. Power Sources 2010, 195, 5674–5685. [Google Scholar] [CrossRef]
- Intini, M.; De Antonellis, S.; Joppolo, C.M.; Casalegno, A. A trigeneration system based on polymer electrolyte fuel cell and desiccant wheel—Part B: Overall system design and energy performance analysis. Energy Convers. Manag. 2015, 106, 1460–1470. [Google Scholar] [CrossRef]
- Chen, X.; Gong, G.; Wan, Z.; Luo, L.; Wan, J. Performance analysis of 5 kW PEMFC-based residential micro-CCHP with absorption chiller. Int. J. Hydrog. Energy 2015, 40, 10647–10657. [Google Scholar] [CrossRef]
- Arsalis, A. Modeling and simulation of a 100 kWe HT-PEMFC subsystem integrated with an absorption chiller subsystem. Int. J. Hydrog. Energy 2012, 37, 13484–13490. [Google Scholar] [CrossRef]
- Ge, Y.; Tassou, S.; Chaer, L.; Suguartha, N. Performance evaluation of a tri-generation system with simulation and experiment. Appl. Energy 2009, 86, 2317–2326. [Google Scholar] [CrossRef]
- Najafi, B.; Antonellis, S.; Lntini, M.; Zago, M.; Rinaldi, F.; Casalegno, A. Atri-generation system based on plymer electrolyte fuel cell and desiccant whell—Part A: Fuel cell system modelling and parial load analysis. Energy Convers. Manag. 2015, 106, 1450–1459. [Google Scholar] [CrossRef]
- Al-Sulaiman, F.; Dincer, L.; Hamdullapur, F. Energy analysis of a trigeneration plant based on solid oxide fuel cell and organic Ranine cycle. Int. J. Hydrog. Energy 2010, 35, 5104–5113. [Google Scholar] [CrossRef]
- Tippawan, P.; Arpornwichanop, A.; Dincer, L. Energy and Exergy analyses of an ethanol-fueled solid oxide fuel cell for a trigeneration system. Energy 2015, 87, 228–339. [Google Scholar] [CrossRef]
- Authayanun, S.; Hacker, V. Energy and exergy analyses of a stand-alone HT-PEMFC based trigeneration system for residential applications. Energy Convers. Manag. 2018, 160, 230–242. [Google Scholar] [CrossRef]
- Jo, A.; Oh, K.; Lee, J.; Han, D.; Kim, D.; Kim, J.; Kim, B.; Kim, J.; Park, D.; Kim, M.; et al. Modeling and analysis of a 5 kWe HT-PEMFC system for residential heat and power generation. Int. J. Hydrog. Energy 2017, 42, 1698–1714. [Google Scholar] [CrossRef]
- Oh, K.; Ju, H. Temperature dependence of CO poisoning in high-temperature proton exchange membrane fuel cells with phosphoric acid-doped polybenzimidazole membranes. Int. J. Hydrog. Energy 2015, 40, 7743–7753. [Google Scholar] [CrossRef]
- Das, S.K.; Reis, A.; Berry, K.J. Experimental evaluation of CO poisoning on the performance of a high temperature proton exchange membrane fuel cell. J. Power Sources 2009, 193, 691–698. [Google Scholar] [CrossRef]
- Xu, J.; Froment, G.F. Methane steam reforming methanation and water-gas shift: I. Intrinsic kinetics. AIChE J. 1989, 35, 88–96. [Google Scholar]
- Oliveira, E.L.G.; Grande, C.A. Steam methane reforming in a Ni/Al2O3 catalyst: Kinetics and diffusional limitations in extrudates. Can. J. Chem. Eng. 2009, 87, 945–956. [Google Scholar] [CrossRef]
- Hou, K.; Hughes, R. The kinetics of methane steam reforming over a Ni/α-catalyst. Chem. Eng. J. 2001, 82, 311–328. [Google Scholar] [CrossRef]
- Chen, W.H.; Lin, M.R.; Jiang, T.L.; Chen, M.H. Modeling and simulation of hydrogen generation from high-temperature and low-temperature water gas shift reactions. Int. J. Hydrog. Energy 2008, 33, 6644–6656. [Google Scholar] [CrossRef]
- Chippar, P.; Oh, K.; Kim, W.G.; Ju, H. Numerical analysis of effects of gas crossover through membrane pinholes in high-temperature proton exchange membrane fuel cells. Int. J. Hydrog. Energy 2014, 39, 2863–2871. [Google Scholar] [CrossRef]
- Oh, K.; Jeong, G.; Cho, E.A.; Kim, W.; Ju, H. A CO poisoning model for high-temperature proton exchange membrane fuel cells comprising phosphoric acid-doped polybenzimidazole membranes. Int. J. Hydrog. Energy 2014, 39, 21915–21926. [Google Scholar] [CrossRef]
- Oh, K.; Chippar, P.; Ju, H. Numerical study of thermal stresses in high-temperature proton exchange membrane fuel cell (HT-PEMFC). Int. J. Hydrog. Energy 2014, 39, 2785–2794. [Google Scholar] [CrossRef]
- Won, S.; Oh, K.; Ju, H. Numerical degradation studies of high-temperature proton exchange membrane fuel cells with phosphoric acid-doped PBI membranes. Int. J. Hydrog. Energy 2016, 41, 8296–8306. [Google Scholar] [CrossRef]
- Qingfeng, L.; Hjuler, H.A.; Bjerrum, N.J. Phosphoric acid doped polybenzimidazole membranes: Physiochemical characterization and fuel cell applications. J. Appl. Electrochem. 2001, 31, 773–779. [Google Scholar] [CrossRef]
- Jiao, K.; Li, X. A three-dimensional non-isothermal model of high temperature proton exchange membrane fuel cells with phosphoric acid doped polybenizmidazole membranes. Fuel Cells 2010, 10, 351–362. [Google Scholar] [CrossRef]

| Arrhenius Expression | ||||||||||||
| Reaction | Catalyst Type | Activation Energy, Ei, kJ/mol | Ref. | Pre-Exponential Factor, Ai | Ref. | Equilibrium Constants, Kpi | Ref. | |||||
| 1 | Ni-Al2O3 | 217.01 | [32] | 2.084 × 1013 kmol∙bar0.5∙kgcat−1∙h−1 | [32] | 1.198 × 1013 exp(−26830/T) bar2 | [33] | |||||
| 2 | SR | Ni-Al2O3 | 68.20 | [32] | 3.359 × 107 kmol∙bar−1∙kgcat−1∙h−1 | [32] | 1.767 × 10−2 exp(4400/T) | [33] | ||||
| HTS | SHT-4 | 70 | [34] | 1.78 × 1022 × (1 + 0.0097∙SCR − 1.1364∙SCR2)T−8 | [34] | |||||||
| LTS | MDC-7 | 35 | [34] | 1.74 × 1017 × (1 − 0.1540∙SCR − 0.0008∙SCR2)T−8.5 | [34] | |||||||
| 3 | Ni-Al2O3 | 215.84 | [32] | 4.644 × 1013 kmol∙bar0.5∙kgcat−1∙h−1 | [32] | 2.117 × 1011 exp(−22430/T) bar2 | [33] | |||||
| Van’t Hoff Expression | ||||||||||||
| Parameter | Adsorption Specific Enthalpy, ∆hj, kJ/mol | Ref. | Pre-Exponential Factor, A(Kj) | Ref. | ||||||||
| CH4 | −38.28 | [31] | 6.65 × 10−4·bar−1 | [31] | ||||||||
| CO | −70.65 | [31] | 8.23 × 10−5·bar−1 | [31] | ||||||||
| H2 | −82.90 | [31] | 6.12 × 10−9·bar−1 | [31] | ||||||||
| H2O | 88.68 | [31] | 1.77 × 105·bar0 | [31] | ||||||||
| Specific Enthalpy of the LiBr Solution | |||
|---|---|---|---|
| 3.462023 | 162.81 | ||
| −2.679895 10−2 | −6.0418 | ||
| 1.3499 10−3 | 4.5348 10−3 | ||
| −6.55 10−6 | 1.2053 10−3 | ||
| Description | Value | Ref. |
|---|---|---|
| MEA area, | 300 cm2 | - |
| Operating temperature, | 165 °C | - |
| Anode/cathode stoichiometry, | 1.2/2.0 | - |
| Number of cells in a stack, | 160 | - |
| Thickness of anode/cathode CLs, GDLs, , | 0.015, 0.35 mm | - |
| Thickness of anode/cathode membrane, | 0.07 mm | - |
| Anode/Cathode inlet pressure | 1.0 atm | - |
| Reference hydrogen/oxygen molar concentration, | 40.88 mol/m3 | [40] |
| Electronic conductivity in BP, GDL, CL | 14000, 1250, 300 S/m | [40] |
| Phosphoric acid doping level | 18.7 | [40] |
| Anode/cathode transfer coefficient | 0.5, 0.65 | [40] |
| Reference exchange current density in anode/cathode, , | 1.0 × 109, 1.0 × 104 A/m2 | [35] |
| Volume fraction of ionomers in CLs | 0.3 | [40] |
| Porosity of GDL, CL | 0.6, 0.4 | [40] |
| Proton conductivity of the membrane, κ | 36.22 S/m | [39] |
| Operating Condition | ER | FAR | CO Fraction | H2 Fraction | H2 yield | COP | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| I = 0.2 A/cm2 | 1.33 (0.4236) | 5.052 | 0.5287 | 79.88 | 5.177 | 0.4284 | 358.3 | 248.6 | 5.980 | 2.558 | 0.494 | 30.679 | 14.351 | 18.950 | 63.981 |
| 1 (0.3177) | 4.610 | 0.5553 | 79.87 | 5.205 | 0.4283 | 362.7 | 251.6 | 5.978 | 2.566 | 0.493 | 33.429 | 15.692 | 20.656 | 69.777 | |
| 0.76 (0.2421) | 4.200 | 0.1197 | 79.95 | 4.428 | 0.4303 | 226.0 | 165.6 | 6.007 | 2.494 | 0.563 | 35.916 | 16.300 | 22.075 | 74.291 | |
| 0.4 A/cm2 | 1.33 (0.6503) | 4.867 | 0.5631 | 79.89 | 8.085 | 0.8608 | 304.3 | 250.8 | 10.228 | 8.729 | 1.080 | 28.870 | 26.439 | 10.230 | 65.538 |
| 1 (0.4877) | 4.507 | 0.6060 | 79.88 | 8.145 | 0.8604 | 310.4 | 255.9 | 10.223 | 8.657 | 1.062 | 30.974 | 28.147 | 10.982 | 70.103 | |
| 0.80 (0.3902) | 4.194 | 0.1581 | 79.97 | 7.135 | 0.8656 | 202.0 | 166.0 | 10.285 | 8.338 | 1.169 | 32.614 | 28.360 | 11.488 | 72.462 | |
| 0.65 A/cm2 | 1.33 (1.0035) | 4.780 | 0.6349 | 79.87 | 12.773 | 1.3967 | 302.7 | 265.2 | 13.819 | 17.270 | 1.352 | 25.009 | 32.658 | 6.387 | 64.054 |
| 1 (0.7526) | 4.439 | 0.6752 | 79.86 | 12.840 | 1.3960 | 307.1 | 269.2 | 13.812 | 17.292 | 1.347 | 26.761 | 35.008 | 6.838 | 68.606 | |
| 0.80 (0.6021) | 4.142 | 0.2389 | 79.97 | 11.280 | 1.4051 | 196.4 | 171.6 | 13.902 | 16.737 | 1.484 | 28.135 | 35.383 | 7.140 | 70.658 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gwak, G.; Kim, M.; Kim, D.; Faizan, M.; Oh, K.; Lee, J.; Choi, J.; Lee, N.; Lim, K.; Ju, H. Performance and Efficiency Analysis of an HT-PEMFC System with an Absorption Chiller for Tri-Generation Applications. Energies 2019, 12, 905. https://doi.org/10.3390/en12050905
Gwak G, Kim M, Kim D, Faizan M, Oh K, Lee J, Choi J, Lee N, Lim K, Ju H. Performance and Efficiency Analysis of an HT-PEMFC System with an Absorption Chiller for Tri-Generation Applications. Energies. 2019; 12(5):905. https://doi.org/10.3390/en12050905
Chicago/Turabian StyleGwak, Geonhui, Minwoo Kim, Dohwan Kim, Muhammad Faizan, Kyeongmin Oh, Jaeseung Lee, Jaeyoo Choi, Nammin Lee, Kisung Lim, and Hyunchul Ju. 2019. "Performance and Efficiency Analysis of an HT-PEMFC System with an Absorption Chiller for Tri-Generation Applications" Energies 12, no. 5: 905. https://doi.org/10.3390/en12050905
APA StyleGwak, G., Kim, M., Kim, D., Faizan, M., Oh, K., Lee, J., Choi, J., Lee, N., Lim, K., & Ju, H. (2019). Performance and Efficiency Analysis of an HT-PEMFC System with an Absorption Chiller for Tri-Generation Applications. Energies, 12(5), 905. https://doi.org/10.3390/en12050905




