Radiation Heat Transfer in a Complex Geometry Containing Anisotropically-Scattering Mie Particles
Abstract
:1. Introduction
2. Mathematical Formulations
2.1. Radiation Transfer Equation
2.2. Boundary Conditions
2.3. Scattering Phase Function
3. Blocked-Off-Region Procedure
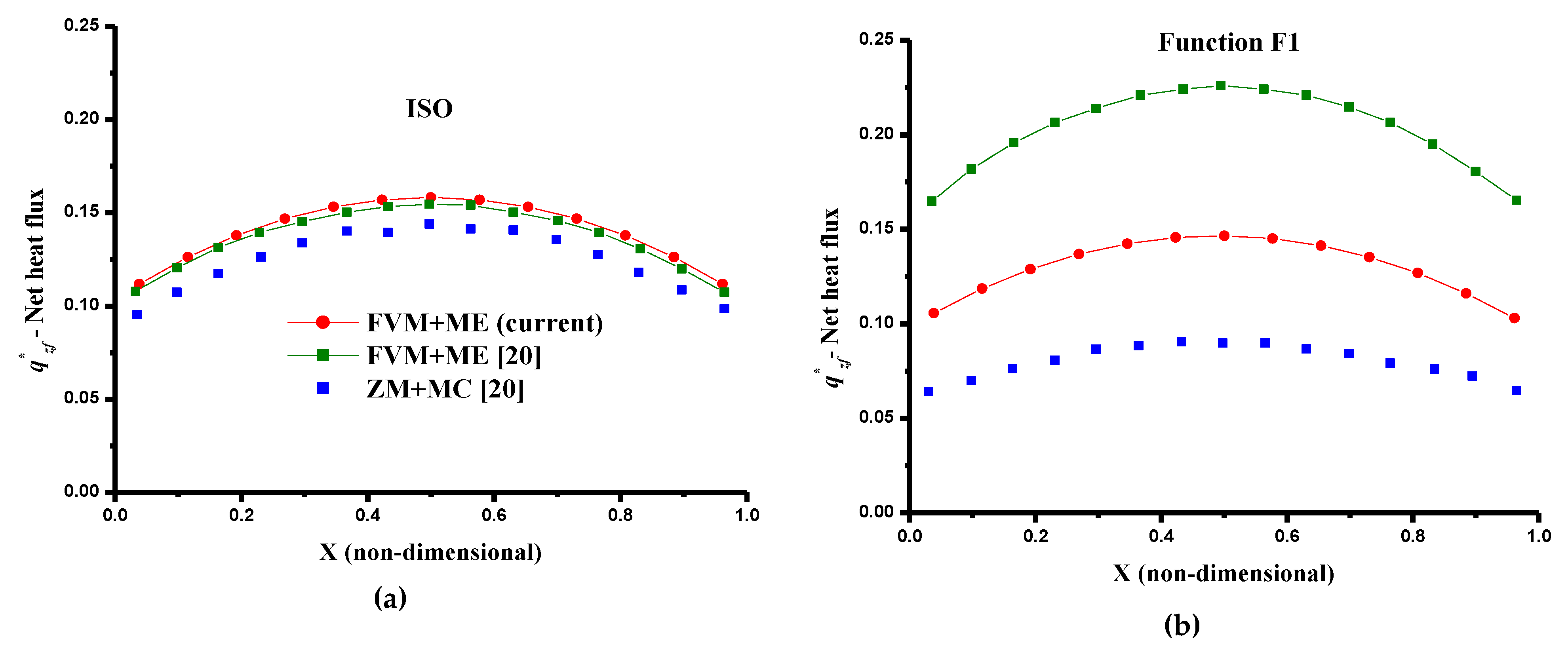
4. Validation
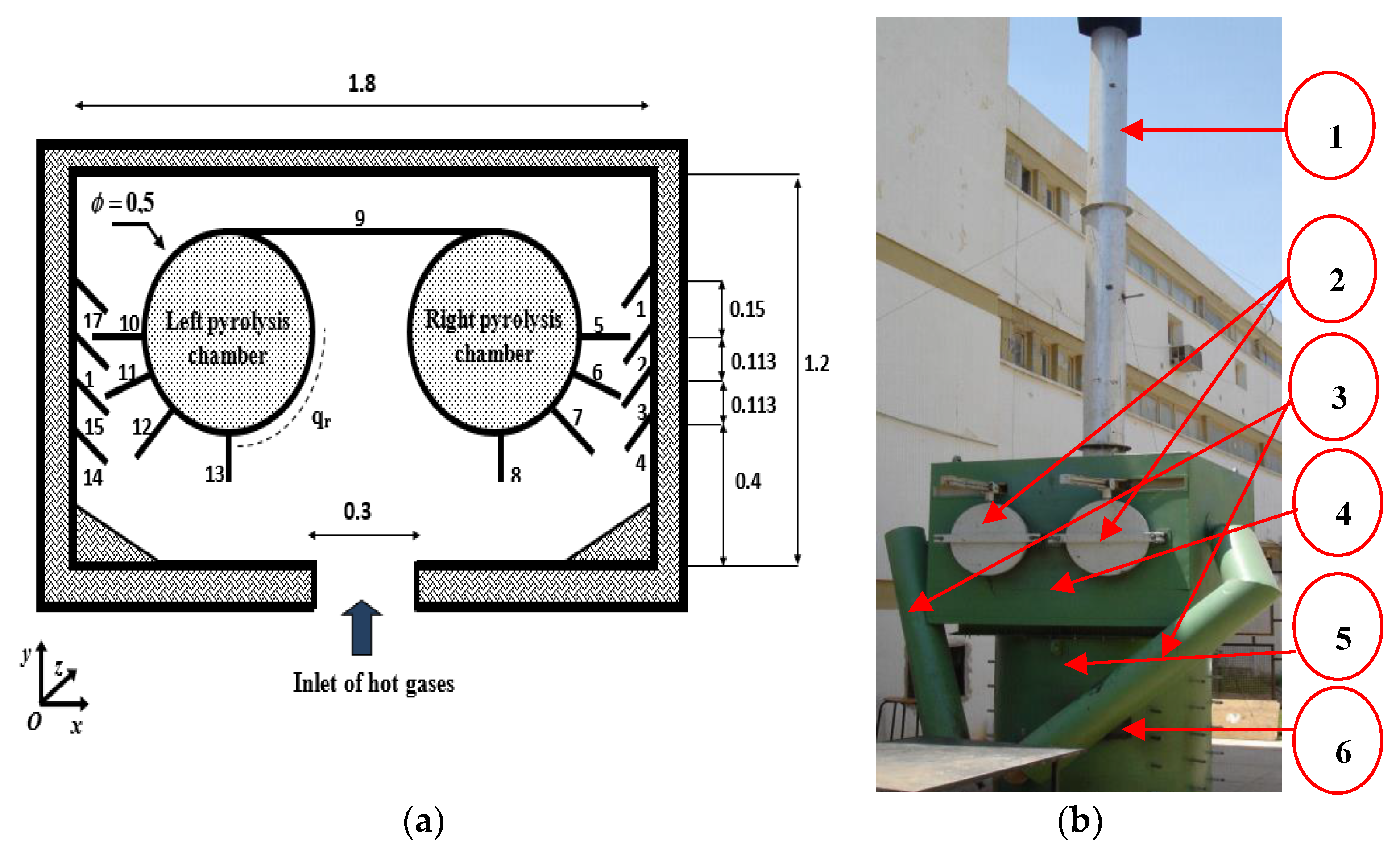
5. A 3D Complex Heat Recuperator of Biomass Pyrolysis Fumes
6. Result and Discussion
6.1. Grid Independence
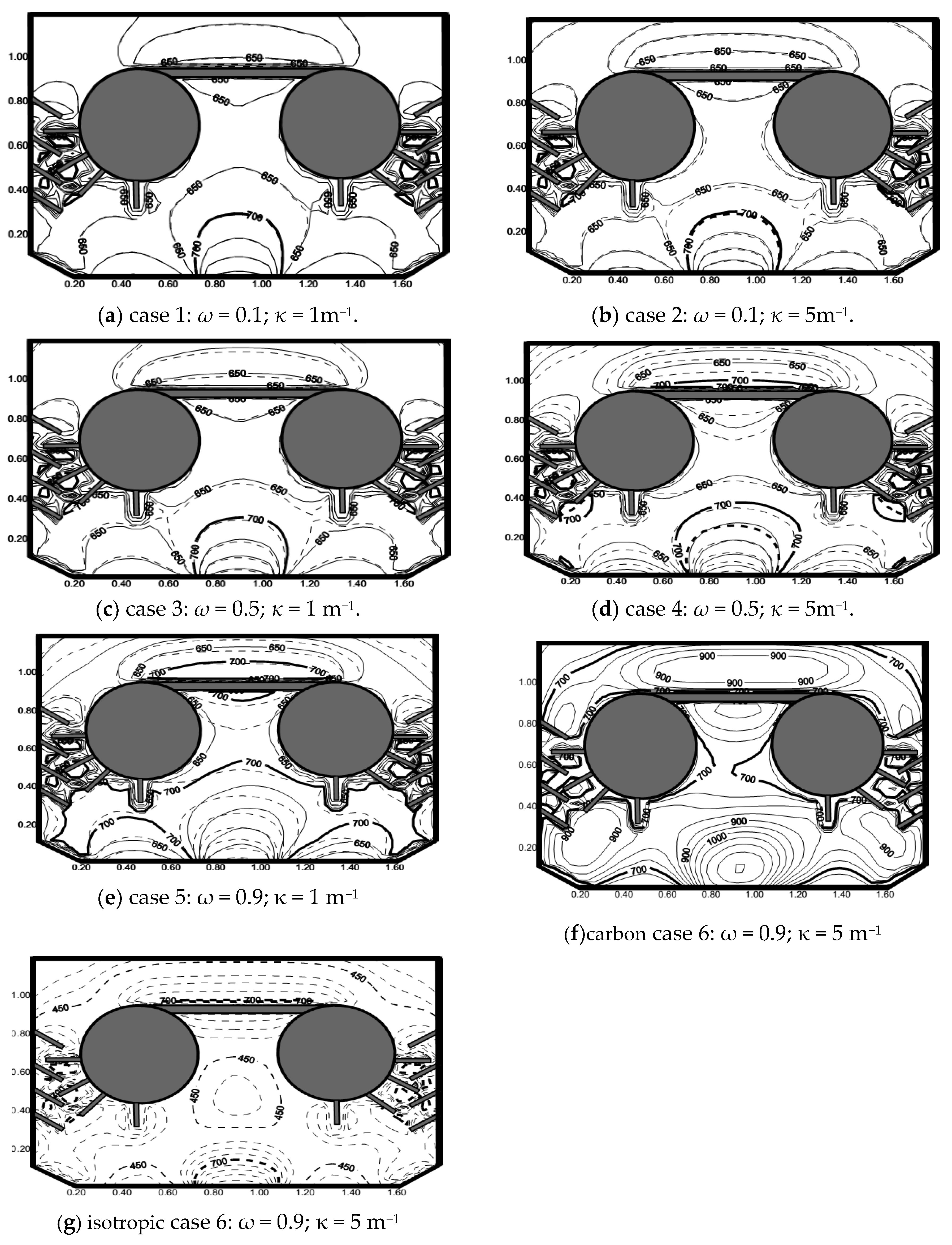
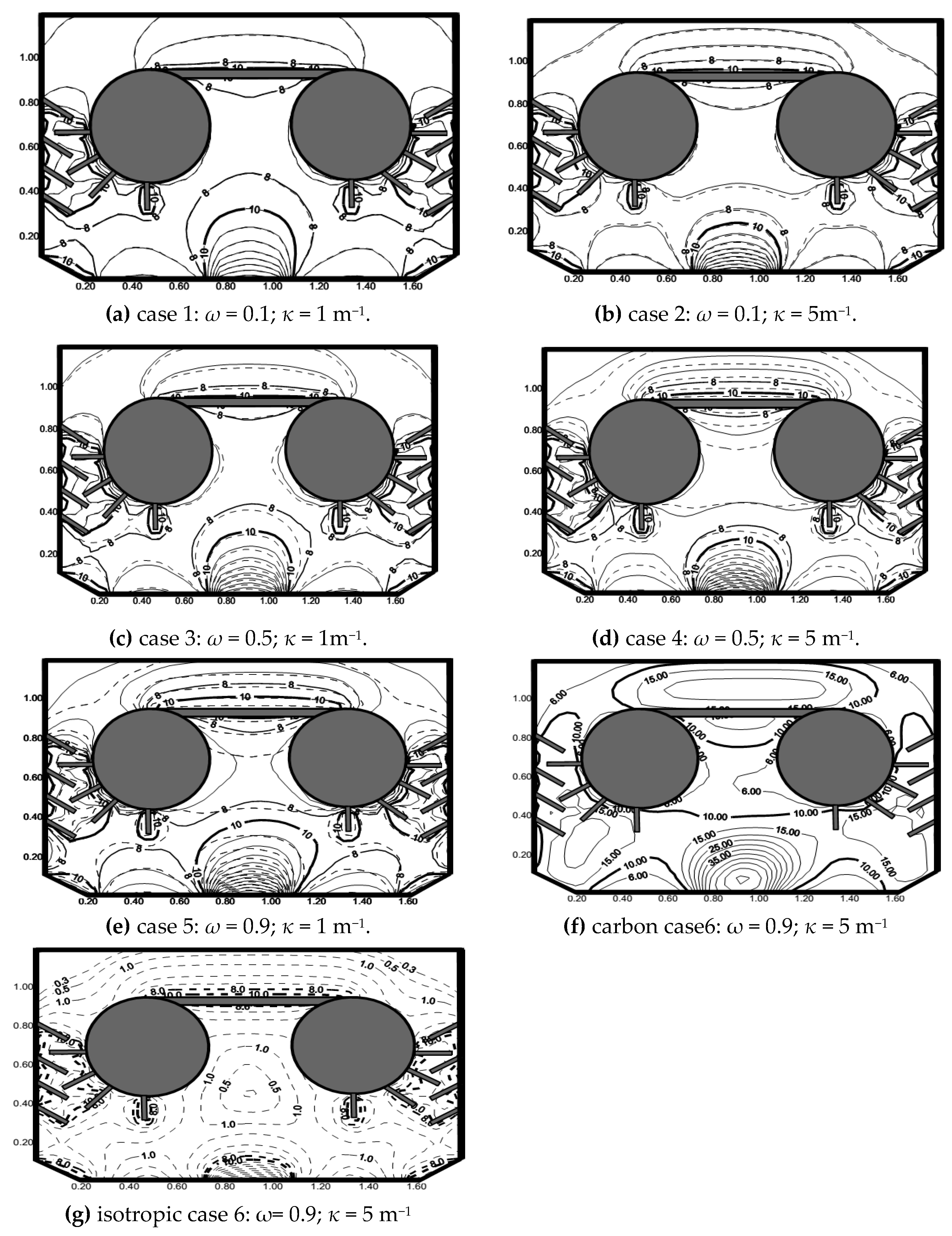
6.2. Absorption Coefficient and Scattering Albedo Effects
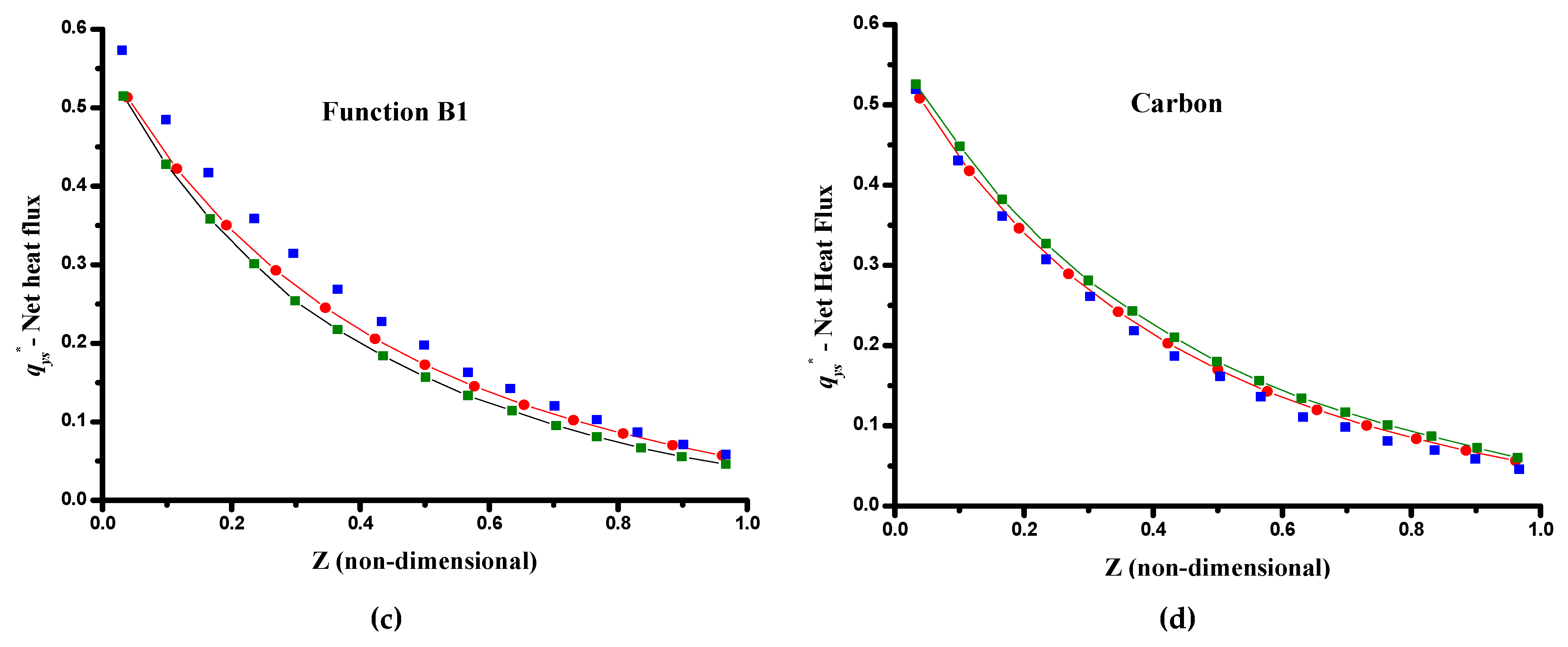
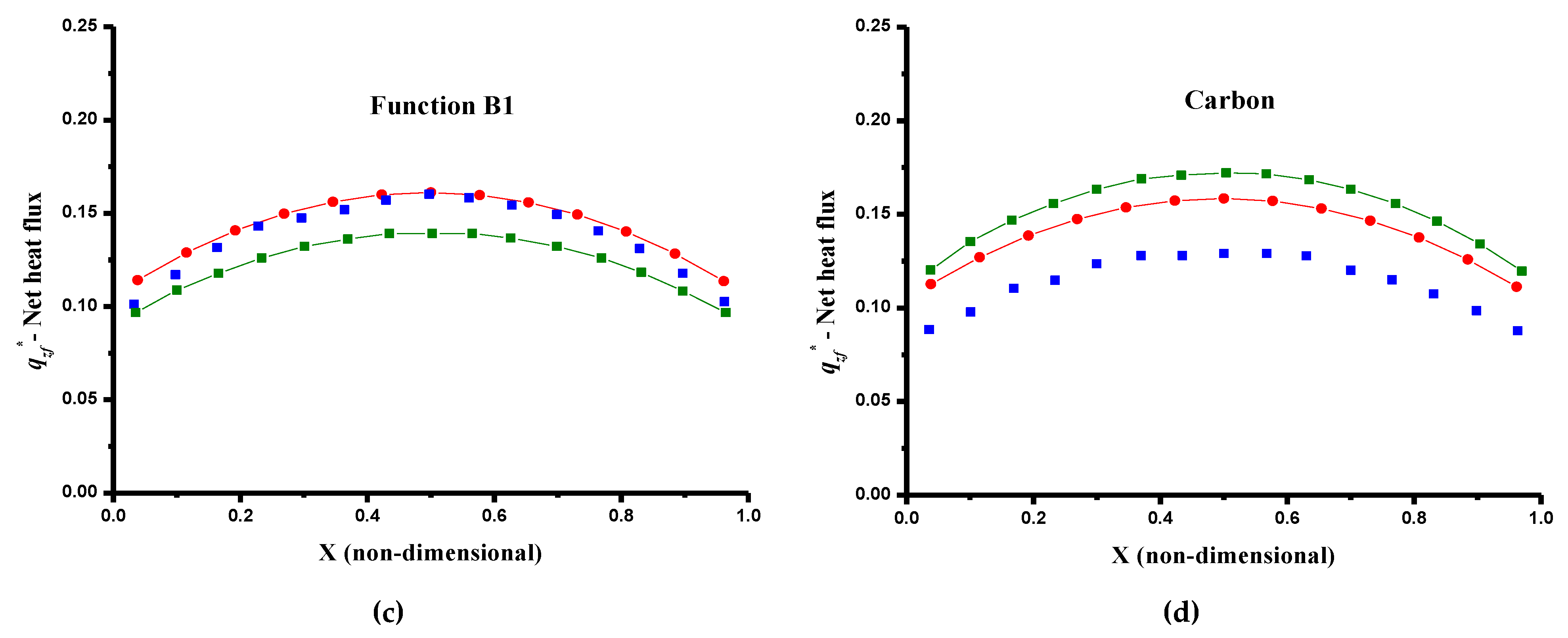
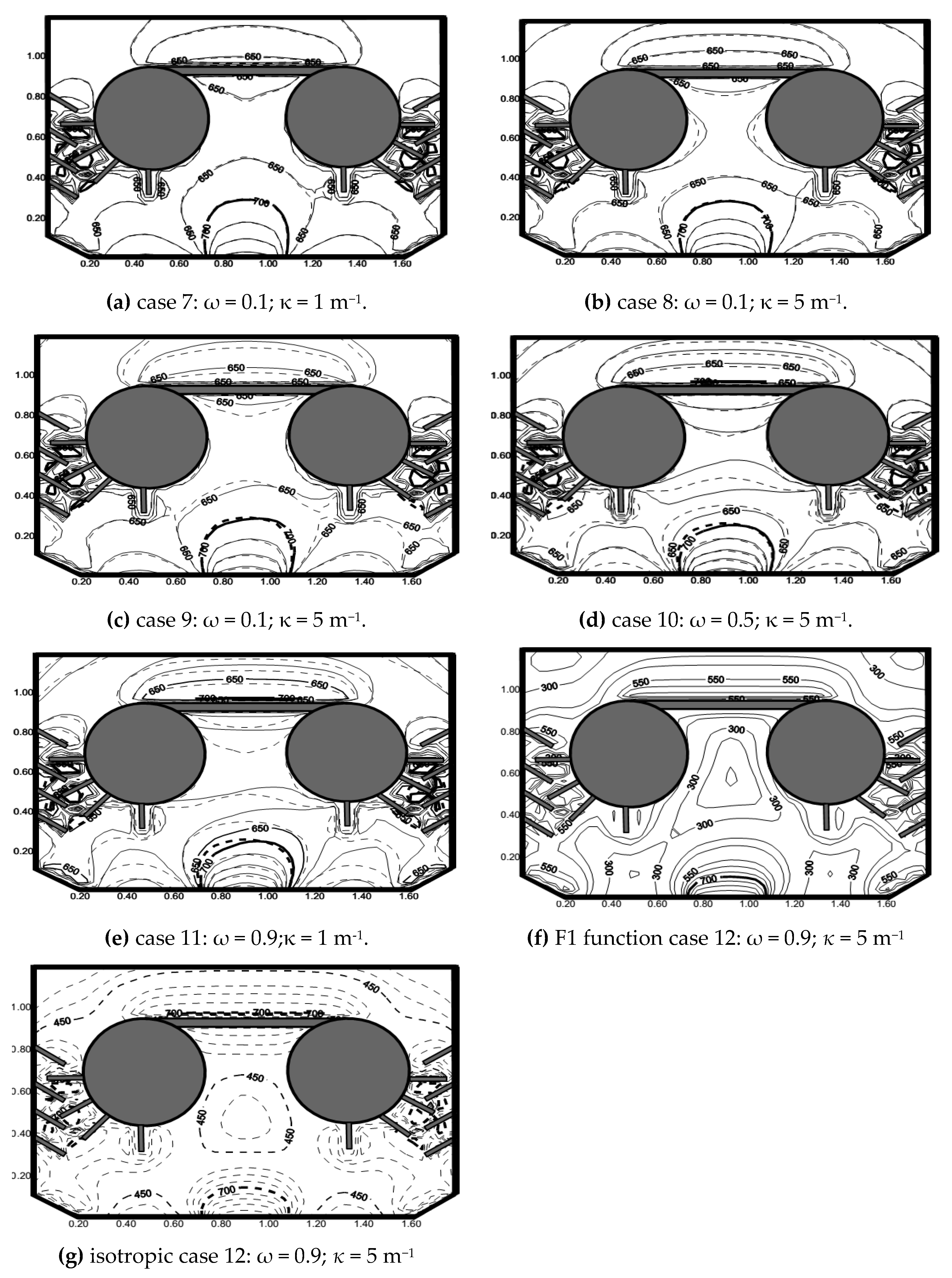
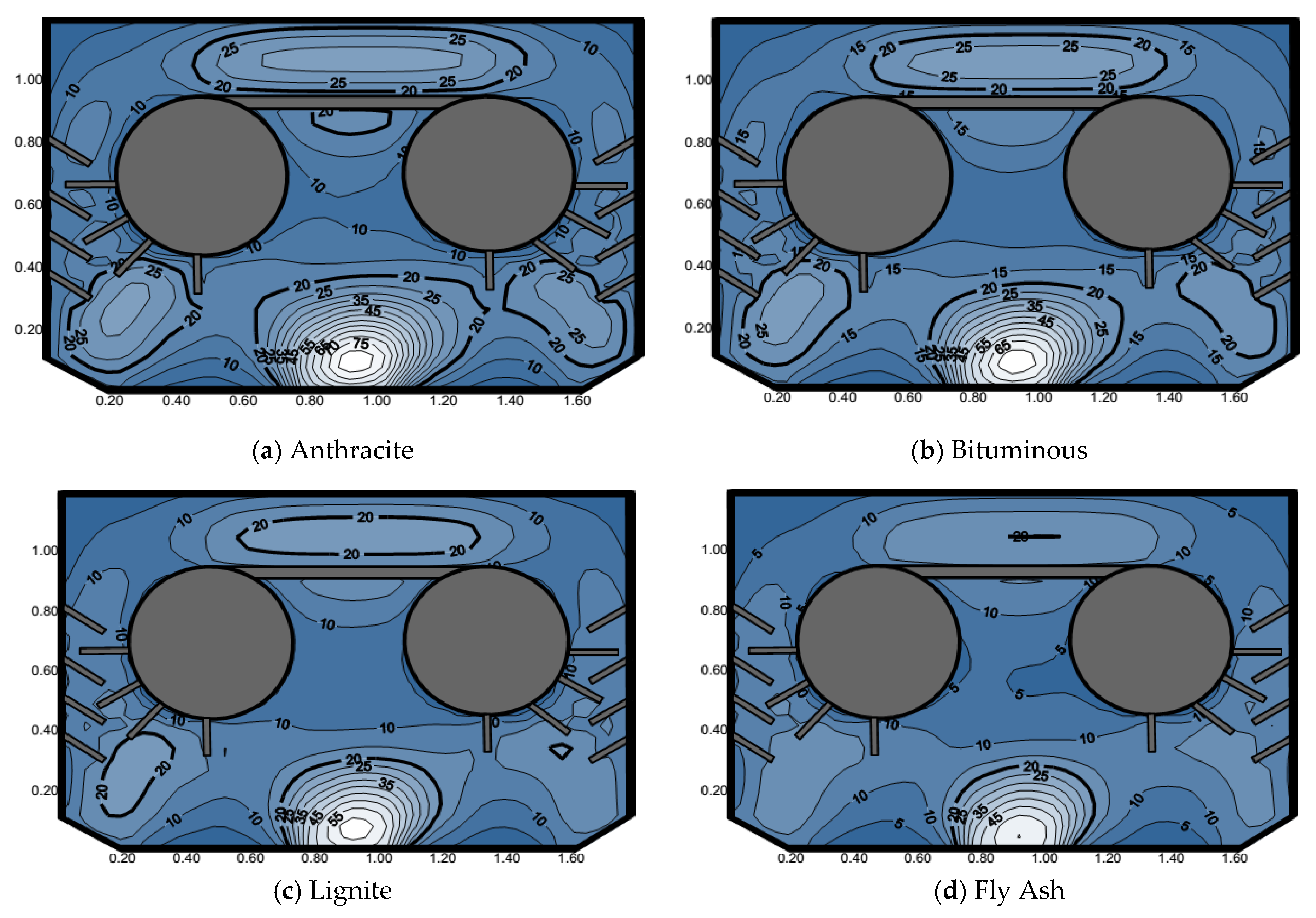
6.3. Particles Type Effect
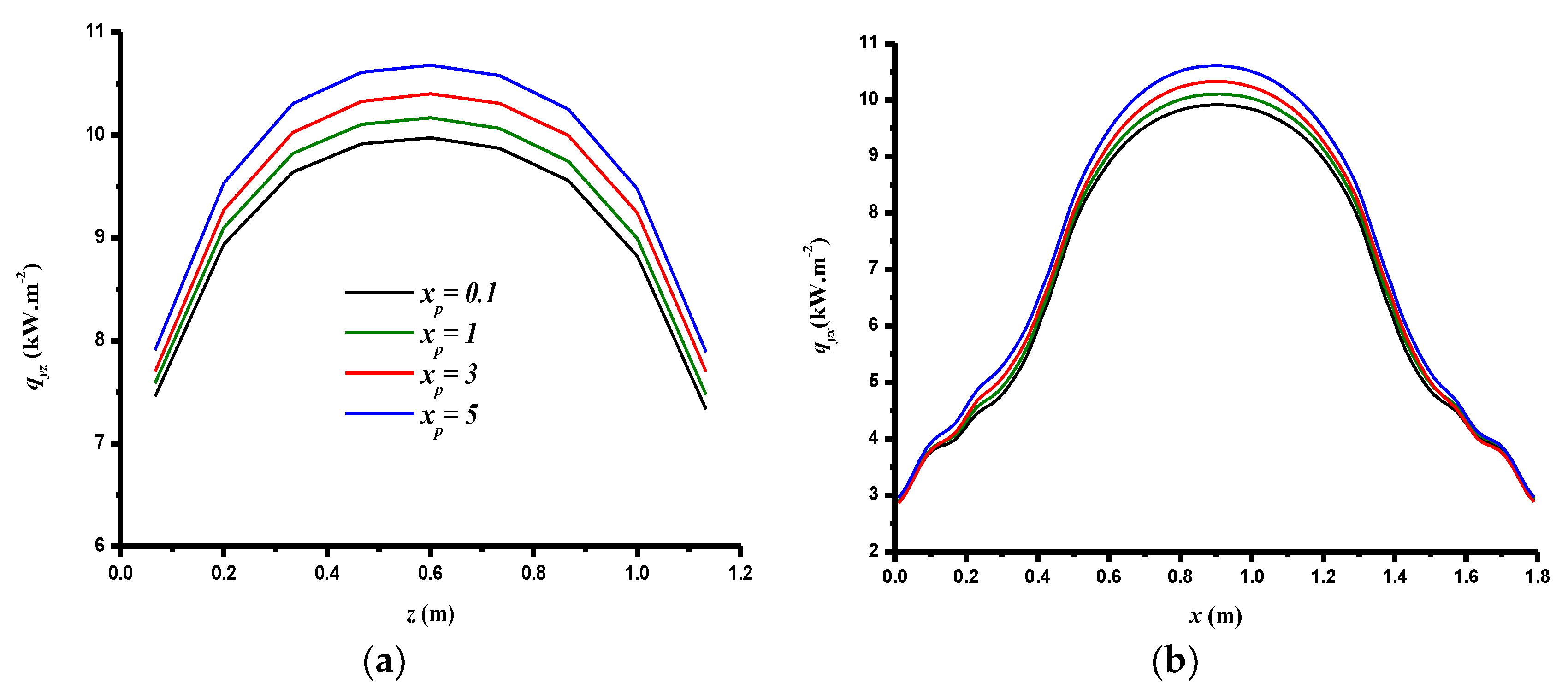
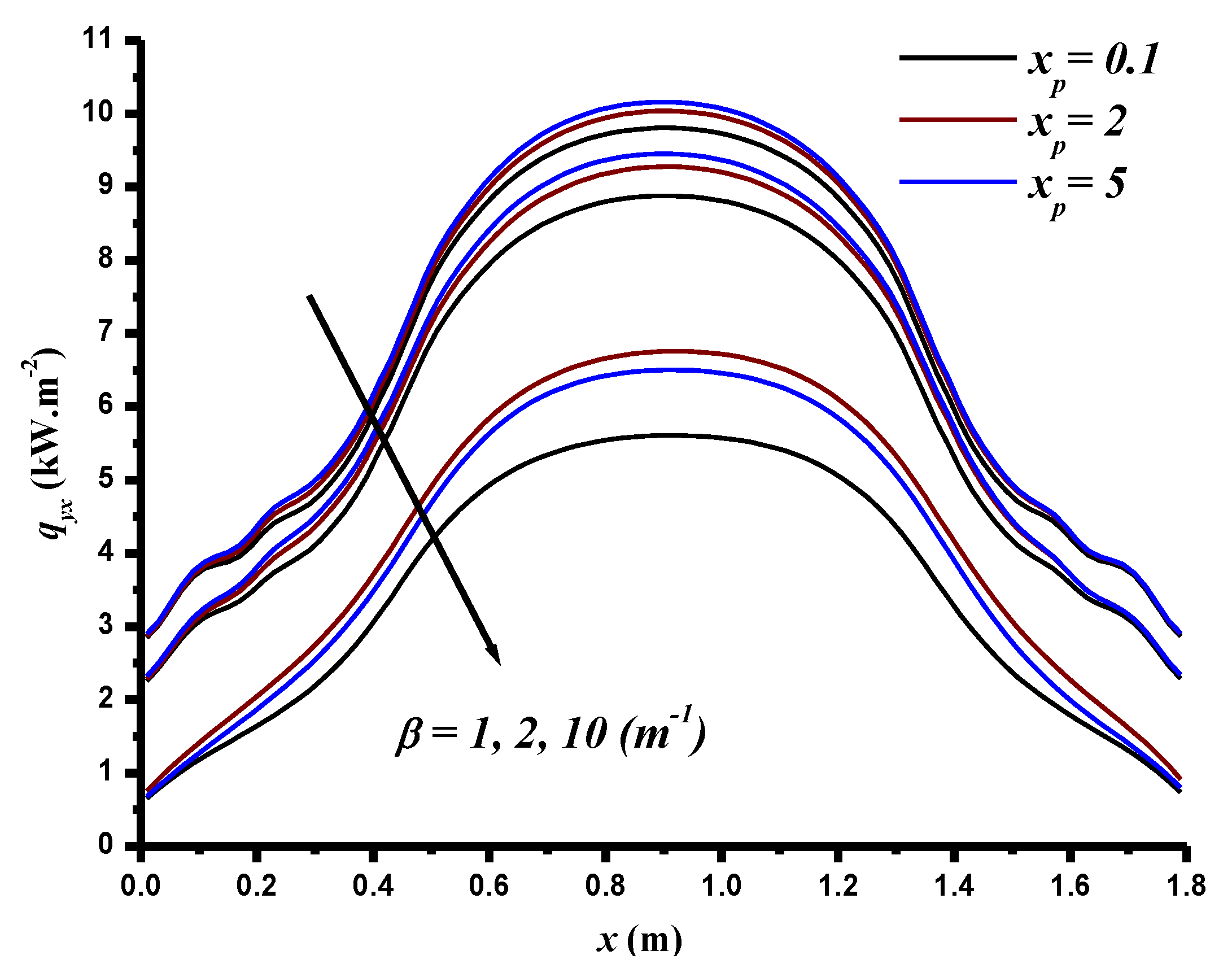
6.4. Particle Size Parameter Effect
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| coefficient of the discretized equations | |
| source term of the discretized equations | |
| D | direction cosines |
| E | radiation emissive power |
| I | radiant intensity |
| k | imaginarypart of the complex index |
| M | a large number (M = 1020) |
| m | complex index of refraction, face of control volume |
| number of angular discretization in the polar angle | |
| number of angular discretization in the azimuthal angle | |
| n | real part of the complex index of refraction or index in infinite series |
| Legendre Polynomial of order n | |
| q | radiation heat flux |
| efficiency factor | |
| position vector | |
| r | particle radius |
| S | source function |
| additional source terms for blocked-off region procedure | |
| s | distance in the direction of radiant intensity; curvilinear coordinate. |
| T | temperature |
| xp | particle size parameter |
| x, y, z | Cartesian coordinates |
| Greek Symbols | |
| extinction coefficient | |
| area of a control volume face | |
| control volume | |
| control solid angle | |
| emissivity | |
| absorption coefficient | |
| wavelength of incident radiation | |
| direction cosine in the x-direction | |
| reflectivity | |
| polar angle | |
| scattering angle | |
| scattering coefficient | |
| azimuthal angle | |
| phase function | |
| average scattering phase function | |
| scattering albedo coefficient | |
| angular direction | |
| Subscript | |
| l, l’ | angular directions |
| * | dimensionless |
| Av | average |
| b | blackbody |
| ext, sca, abs | extinction, scattering and absorption, respectively |
| e, w, n, s, r, f | east, west, north, south, rear and front neighbours of control volume P |
| E, W, N, S, B, T | nodes around the nodal point P |
| w | wall |
| Abbreviations | |
| RTE | radiation transfer equation |
| SPF | scattering phase function |
| WSGGM | weighted sum of gray gases model |
| ZM | zone method |
References
- Lockwood, F.C.; Shah, N.G. A new radiation solution method for incorporation in general combustion prediction procedures. Symp. Int. Combust. 1981, 18, 1405–1414. [Google Scholar] [CrossRef]
- Malalasekera, W.M.G.; Lockwood, F.C. Computer Simulation of the King’s Cross Fire: Effect of Radiative Heat Transfer on Fire. Proc. Inst. Mech. Eng. Part C 1991, 205, 201–208. [Google Scholar] [CrossRef]
- Malalasekera, W.M.G.; James, E.H. Radiative Heat Transfer Calculations in Three Dimensional Complex Geometries. J. Heat Transf. 1996, 118, 225–228. [Google Scholar] [CrossRef]
- Chai, J.C.; Lee, H.S.; Patankar, S.V. Treatment of Irregular Geometries Using a Cartesian-Coordinates-Based Discrete-Ordinates Method, Radiative Heat Transfer. Theory Appl. 1993, 244, 49–54. [Google Scholar]
- Fivel, W.A.; Jesse, J.P. Finite Element Formulation of the Discrete-Ordinates Method for Multidimensional Geometries, Radiative Heat Transfer. Curr. Res. 1994, 276, 49–57. [Google Scholar]
- Chai, J.C.; Parthasarathy, G.; Lee, H.S.; Patankar, S.V. Finite Volume Radiative Heat Transfer Procedure for Irregular Geometries. J. Heat Transf. 1995, 9, 410–415. [Google Scholar] [CrossRef]
- Chai, J.; Lee, H.S.; Patankar, S.V. Treatment of irregular geometries using a Cartesian coordinates finite volume radiative heat transfer procedure. Numer. Heat Transf. Part B 1994, 26, 225–235. [Google Scholar] [CrossRef]
- Borjini, M.N.; Farhat, H.; Radhouani, M.S. Analysis of radiative heat transfer in a partitioned idealized furnace. Numer. Heat Transf. Part A 2003, 35, 467–495. [Google Scholar] [CrossRef]
- Guedri, K.; Borjini, M.N.; Farhat, H. Modelization of Combined Radiative and Conductive Heat Transfer in Three-Dimensional Complex Enclosures. Int. J. Numer. Methods Heat Fluid Flow 2005, 15, 257–276. [Google Scholar] [CrossRef]
- Chai, J.C.; Moder, J.P. Spatial-Multiblock Procedure for Radiation Heat Transfer. Numer. Heat Transf. B 1997, 31, 277–293. [Google Scholar] [CrossRef]
- Coelho, P.J.; GonÇalves, J.M.; Carvalho, M.G.; Trivic, D.N. Modeling of Radiative Heat Transfer in Enclosures with Obstacles. Int. J. Heat Mass Transf. 1998, 41, 745–756. [Google Scholar] [CrossRef]
- Guedri, K.; Abbassi, M.A.; Borjini, M.N.; Halouani, K. Application of the finite-volume method to study the effects of baffles on radiative heat transfer in complex enclosures. Numer. Heat Transf. Part A 2009, 55, 1–27. [Google Scholar] [CrossRef]
- Abbassi, M.A.; Guedri, K.; Borjini, M.N.; Halouani, K.; Zeghmati, B. Modeling of radiative heat transfer in 2D complex heat recuperator of biomass pyrolysis furnace: A study of baffles shadow and soot volume fraction effects. Int. J. Math. Comput. Nat. Phys. Eng. 2014, 8, 450–460. [Google Scholar]
- Mengüç, M.P.; Viscanta, R. Radiative transfer in three-dimensional rectangular enclosures containing inhomogeneous, anisotropically scattering media. J. Quant. Spectrosc. Radiat. Transf. 1995, 33, 533–549. [Google Scholar] [CrossRef]
- Kim, T.K.; Lee, H.S. Effect of anisotropic scattering on radiative heat transfer in two-dimensional rectangular enclosures. Int. J. Heat Mass Transf. 1988, 31, 1711–1721. [Google Scholar] [CrossRef]
- Kim, T.K.; Lee, H.S. Radiative heat transfer in two dimensional anisotropic scattering media with collimated incidence. J. Quant. Spectrosc. Radiat. Transf. 1989, 42, 225–238. [Google Scholar] [CrossRef]
- Farmer, J.T.; Howell, J.R. Monte Carlo Prediction of Radiative Heat Transfer in Inhomogeneous, Anisotropic, Nongray Media. AIAA J. Thermophys. Heat Transf. 1994, 8, 133–139. [Google Scholar] [CrossRef]
- Guo, Z.; Maruyama, S. Scaling anisotropic scattering in radiative transfer in three-dimensional nonhomogeneous media. Int. Commun. Heat Mass Transf. 1999, 26, 997–1007. [Google Scholar] [CrossRef]
- Trivic, D.N.; O’Brien, T.J.; Amon, C.H. Modeling the radiation of anisotropically scattering media by coupling Mie theory with finite volume method. Int. J. Heat Mass Transf. 2004, 47, 5765–5780. [Google Scholar] [CrossRef]
- Trivic, D.N.; Amon, C.H. Modeling the 3-D radiation of anisotropically scattering media by two different methods. Int. J. Heat Mass Transf. 2008, 51, 2711–2732. [Google Scholar] [CrossRef]
- Trivic, D.N. 3-D radiation modeling of nongray gases–particles mixture by two different numerical methods. Int. J. Heat Mass Transf. 2014, 70, 298–312. [Google Scholar] [CrossRef]
- Hunter, B.; Guo, Z. Improved treatment of anisotropic scattering in radiation transfer analysis using the finite volume method. Heat Transf. Eng. 2015, 37, 341–350. [Google Scholar] [CrossRef]
- Guedri, K.; Al-Ghamdi, A.S.; Bouzid, A.; Abbassi, M.A.; Ghulman, H.A. Evaluation of the FTn finite volume method for transient radiative transfer in anisotropically scattering medium. Numer. Heat Transf. Part A 2015, 68, 1137–1154. [Google Scholar] [CrossRef]
- Chai, J.C.; Lee, H.S.; Patankar, S.V. Finite volume method for radiation heat transfer. J. Heat Transf. 1994, 8, 419–425. [Google Scholar] [CrossRef]
- Chai, J.C.; Patankar, S.V. Finite-volume method for radiation heat transfer. In Advances in Numerical Heat Transfer 2; Minkowycz, W.K., Sparrow, E.H., Eds.; Taylor & Francis: Boca Raton, FL, USA, 2000. [Google Scholar]
- Deirmendjian, D.; Clasen, R.; Viezee, V. Mie scattering with complex index of refraction. J. Opt. Soc. Am. 1961, 51, 620–633. [Google Scholar] [CrossRef]
- Abbassi, M.A.; Grioui, N.; Halouani, K.; Zoulalian, A.; Zeghmati, B. A practical approach for modelling and control of biomass pyrolysis pilot plant with heat recovery from combustion of pyrolysis products. Fuel Process. Technol. 2009, 90, 1278–1285. [Google Scholar] [CrossRef]
- Modest, M.F. Radiative Heat Transfer; Academic Press: New York, NY, USA, 2013. [Google Scholar]
- Togun, H.; Safaei, M.R.; Sadri, R.; Kazi, S.N.; Badarudin, A.; Hooman, K.; Sadeghinezhad, E. Numerical simulation of laminar to turbulent nanofluid flow and heat transfer over a backward-facing step. Appl. Math. Comput. 2014, 239, 153–170. [Google Scholar] [CrossRef]
- Hosseini, M.; Safaei, M.R.; Estellé, P.; Jafarnia, S.H. Heat transfer of water-based carbon nanotube nanofluids in the shell and tube cooling heat exchangers of the gasoline product of the residue fluid catalytic cracking unit. J. Therm. Anal. Calorim. 2019, 1–12. [Google Scholar] [CrossRef]
- Togun, H.; Ahmadi, G.; Abdulrazzaq, T.; Shkarah, A.J.; Kazi, S.N.; Badarudin, A.; Safaei, M.R. Thermal performance of nanofluid in ducts with double forward-facing steps. J. Taiwan Inst. Chem. Eng. 2015, 47, 28–42. [Google Scholar] [CrossRef]
- Safaei, M.R.; Togun, H.; Vafai, K.; Kazi, S.N.; Badarudin, A. Investigation of heat transfer enhancement in a forward-facing contracting channel using FMWCNT nanofluids. Numer. Heat Transf. Part A Appl. 2014, 66, 1321–1340. [Google Scholar] [CrossRef]
- Gholamalizadeh, E.; Pahlevanzadeh, F.; Ghani, K.; Karimipour, A.; Nguyen, T.K.; Safaei, M.R. Simulation of water/FMWCNT nanofluid forced convection in a microchannel filled with porous material under slip velocity and temperature jump boundary conditions. Int. J. Numer. Methods Heat Fluid Flow 2019. [Google Scholar] [CrossRef]




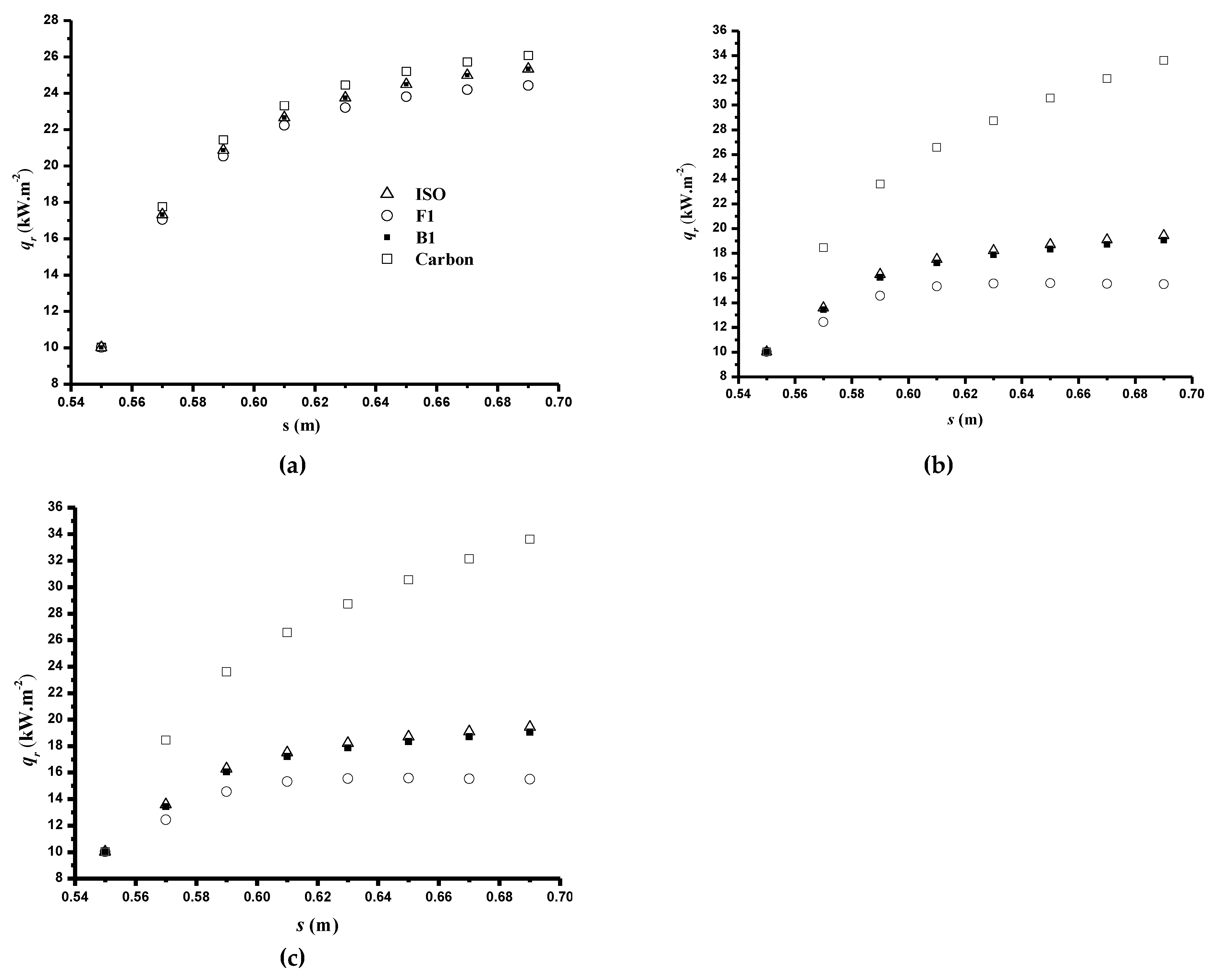

| Scattering Phase Function | F1 | B1 |
|---|---|---|
| Particle size parameter xp | 5 | 1 |
| Real part of complex refractive index n | 1.33 | very large, taken 108 |
| Imaginary part of complex refractive index k | 0 | 0 |
| Albedo | ω = 0.1 | ω = 0.5 | ω = 0.7 | ω = 0.9 | |
|---|---|---|---|---|---|
| ISO | TAv (K) | 585.10 | 585.01 | 584.29 | 577.00 |
| qAv (kW·m−2) | 6.60 | 6.43 | 6.21 | 5.47 | |
| B1 | TAv (K) | 585.12 | 585.16 | 584.37 | 575.19 |
| qAv (kW·m−2) | 6.60 | 6.46 | 6.25 | 5.51 | |
| F1 | TAv (K) | 585.25 | 585.39 | 583.12 | 558.34 |
| qAv (kW·m−2) | 6.60 | 6.45 | 6.19 | 5.07 | |
| Carbon | TAv (K) | 585.50 | 588.68 | 593.27 | 619.60 |
| qAv (kW·m−2) | 6.62 | 6.61 | 6.62 | 7.12 | |
| Albedo | ω = 0.1 | ω = 0.5 | ω = 0.7 | ω = 0.9 | |
|---|---|---|---|---|---|
| ISO | TAv (K) | 582.29 | 577.00 | 567.71 | 523.15 |
| qAv (kW·m−2) | 5.90 | 5.47 | 5.03 | 4.00 | |
| B1 | TAv (K) | 582.26 | 576.04 | 564.54 | 510.53 |
| qAv (kW·m−2) | 5.91 | 5.5 | 5.03 | 3.84 | |
| F1 | TAv (K) | 581.54 | 565.83 | 538.07 | 438.71 |
| qAv (kW·m−2) | 5.88 | 5.21 | 4.44 | 2.84 | |
| Carbon | TAv (K) | 584.47 | 598.97 | 623.96 | 724.18 |
| qAv (kW·m−2) | 5.99 | 6.25 | 6.92 | 11.75 | |
| Coal and Ash | Carbon | Anthracite | Bituminous | Lignite | Ash |
|---|---|---|---|---|---|
| Particle size parameter xp | 1 | 1 | 1 | 1 | 1 |
| Real part of complex refractive index n | 2.20 | 2.05 | 1.85 | 1.70 | 1.50 |
| Imaginary part of complex refractive index k | 1.120 | 0.540 | 0.220 | 0.066 | 0.020 |
| Albedo | ω = 0.1 | ω = 0.5 | ω = 0.7 | ω = 0.9 | |
|---|---|---|---|---|---|
| Anthracite | TAv (K) | 584.84 | 603.29 | 636.35 | 783.78 |
| qAv (kW·m−2) | 6.00 | 6.41 | 7.43 | 16.09 | |
| Bituminous | TAv (K) | 584.70 | 601.67 | 631.83 | 760.44 |
| qAv (kW·m−2) | 6.00 | 6.35 | 7.23 | 14.25 | |
| Lignite | TAv (K) | 584.51 | 599.4 | 625.18 | 729.51 |
| qAv (kW·m−2) | 5.99 | 6.27 | 6.97 | 12.09 | |
| Fly ash | TAv (K) | 584.33 | 597.41 | 619.45 | 703.79 |
| qAv (kW·m−2) | 5.98 | 6.20 | 6.75 | 10.53 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ettaleb, A.; Abbassi, M.A.; Farhat, H.; Guedri, K.; Omri, A.; Borjini, M.N.; Goodarzi, M.; Sarafraz, M.M. Radiation Heat Transfer in a Complex Geometry Containing Anisotropically-Scattering Mie Particles. Energies 2019, 12, 3986. https://doi.org/10.3390/en12203986
Ettaleb A, Abbassi MA, Farhat H, Guedri K, Omri A, Borjini MN, Goodarzi M, Sarafraz MM. Radiation Heat Transfer in a Complex Geometry Containing Anisotropically-Scattering Mie Particles. Energies. 2019; 12(20):3986. https://doi.org/10.3390/en12203986
Chicago/Turabian StyleEttaleb, Ali, Mohamed Ammar Abbassi, Habib Farhat, Kamel Guedri, Ahmed Omri, Mohamed Naceur Borjini, Marjan Goodarzi, and M. M. Sarafraz. 2019. "Radiation Heat Transfer in a Complex Geometry Containing Anisotropically-Scattering Mie Particles" Energies 12, no. 20: 3986. https://doi.org/10.3390/en12203986
APA StyleEttaleb, A., Abbassi, M. A., Farhat, H., Guedri, K., Omri, A., Borjini, M. N., Goodarzi, M., & Sarafraz, M. M. (2019). Radiation Heat Transfer in a Complex Geometry Containing Anisotropically-Scattering Mie Particles. Energies, 12(20), 3986. https://doi.org/10.3390/en12203986







