Nonlinear Viscoelastic–Plastic Creep Model Based on Coal Multistage Creep Tests and Experimental Validation
Abstract
:1. Introduction
2. Multistage Creep Tests
2.1. Specimen Preparation and Experimental Set-Up
- (a)
- The main engine adopts a framework structure with stiffness of more than 5000 kN/mm.
- (b)
- The maximum axial capacity is 500 kN, with measure resolution and precision less than 2.5 N and 1%, respectively. The maximum confining pressure is 50 MPa, with a measure resolution and precision of less than 0.001 MPa and ±1%, respectively.
- (c)
- Axial and radial extensometer deformation measuring ranges are 0–15 mm and 0–7 mm, respectively; and the measuring accuracy is ±0.5%.
- (d)
- Limit control system: When the parameters of axial deformation, radial deformation, or test time reach a preset value, or the specimen split, oil pipe blockage, and oil temperature too high, the device can be automatically stopped for self-protection.
2.2. Experimental Scheme
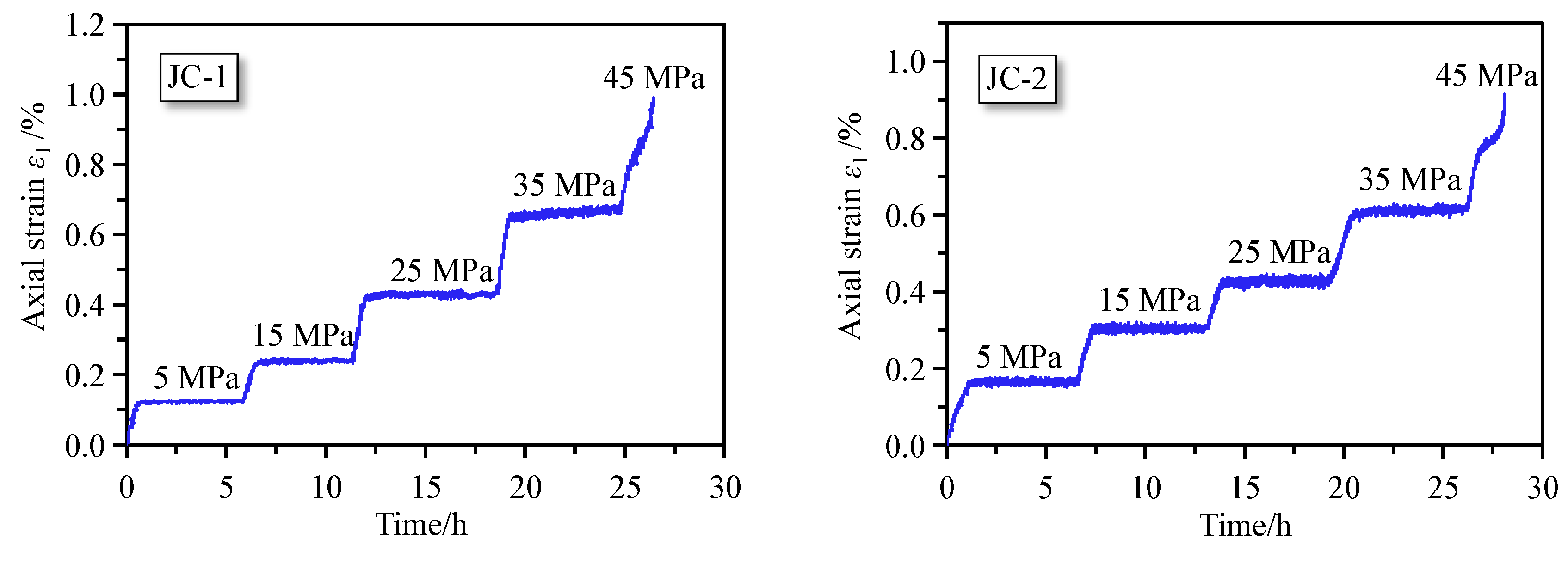
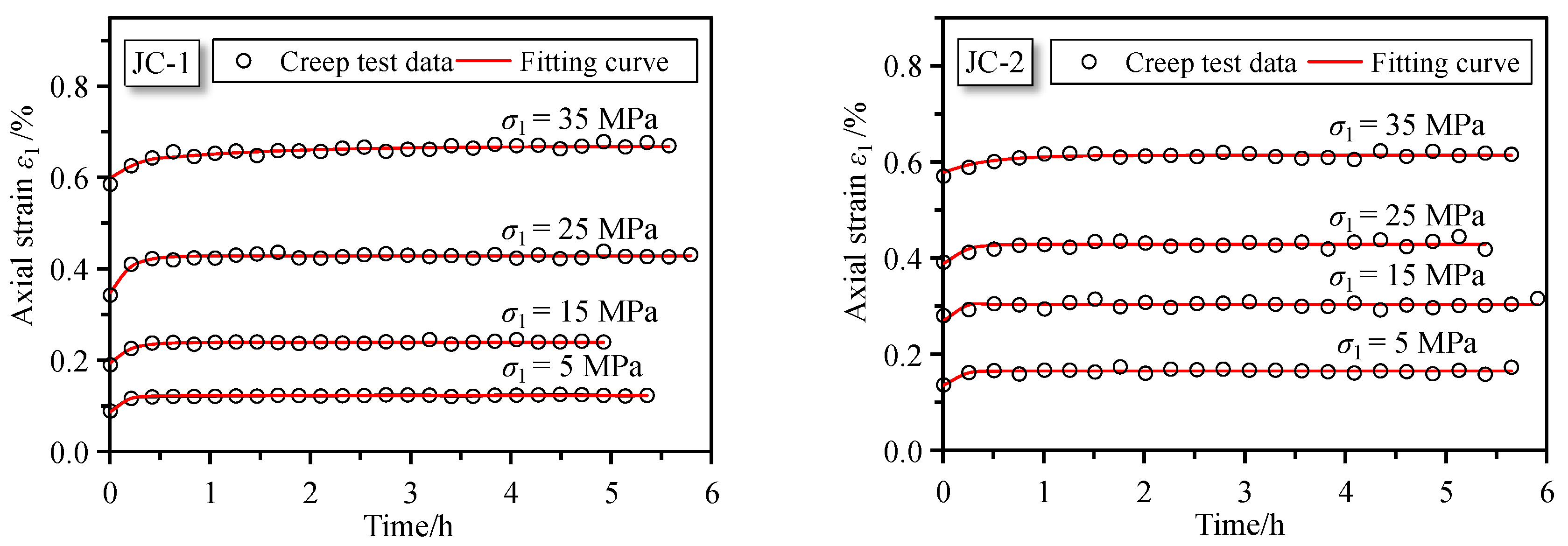
2.3. Experimental Results
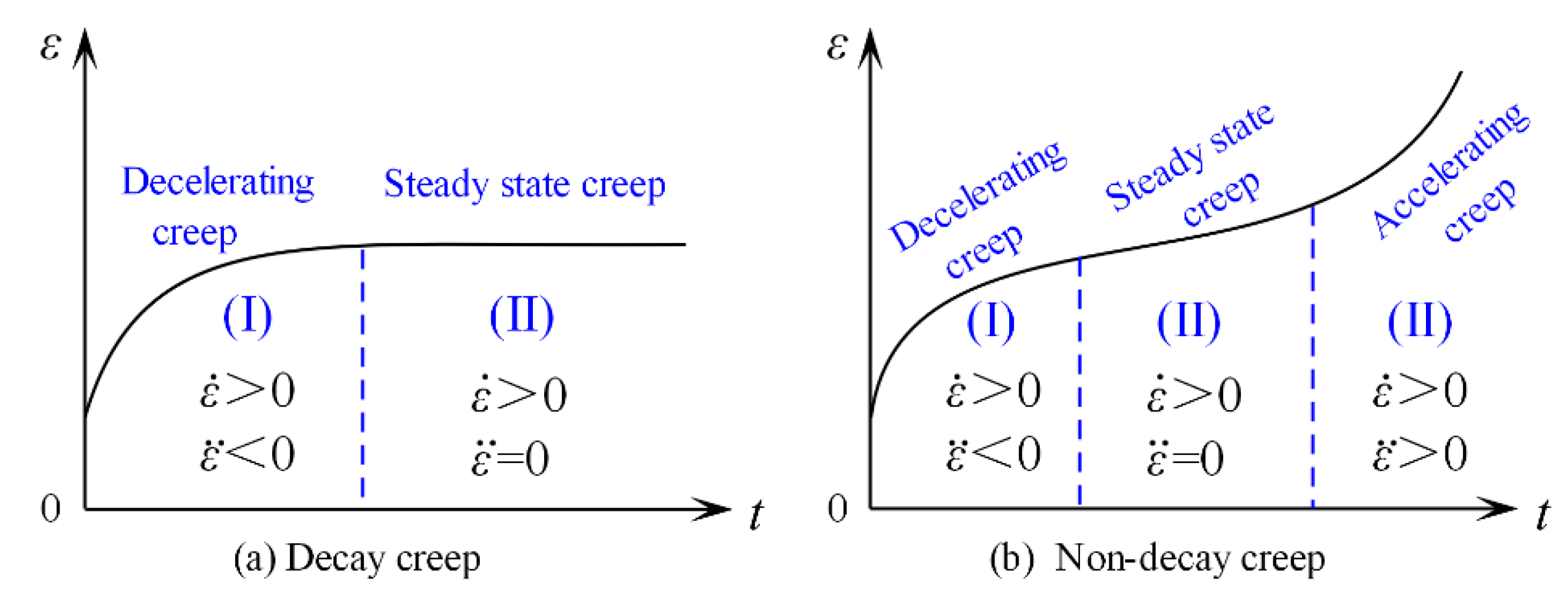
- (1)
- In decelerating creep, creep rate decreases with the increase in axial strain, that is, creep rate > 0 and creep acceleration < 0. The creep curve in this stage presents a convex shape.
- (2)
- In steady state creep, the coal strain tends to be constant along with time, that is, creep rate > 0 and creep acceleration = 0, with a linear change tendency of creep curve.
- (3)
- In accelerating creep, when the coal strain goes beyond the yield point, the axial strain and creep rate increase rapidly, that is, creep rate > 0 and creep acceleration > 0, and the creep curve is presented as a concave shape.
3. Analysis of Classical Nishihara Model
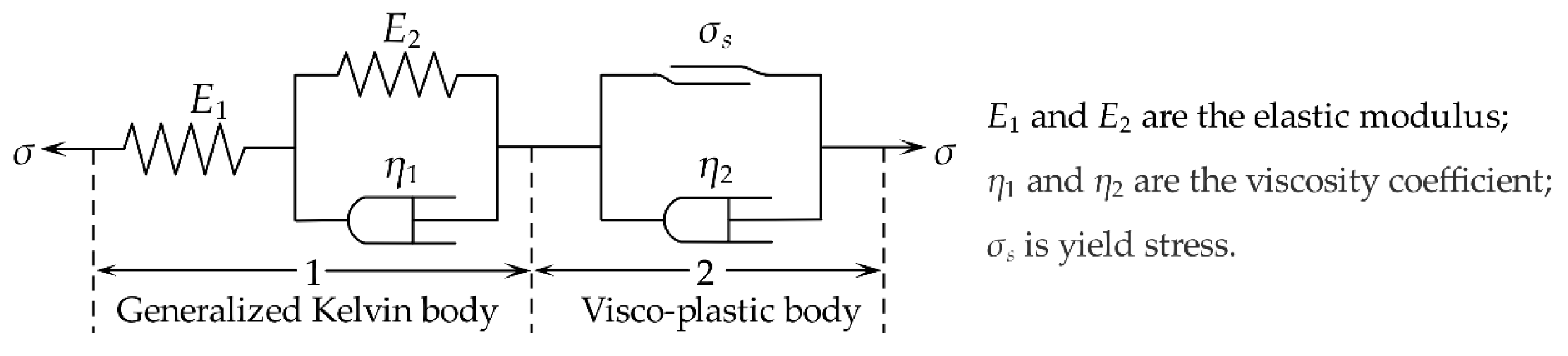
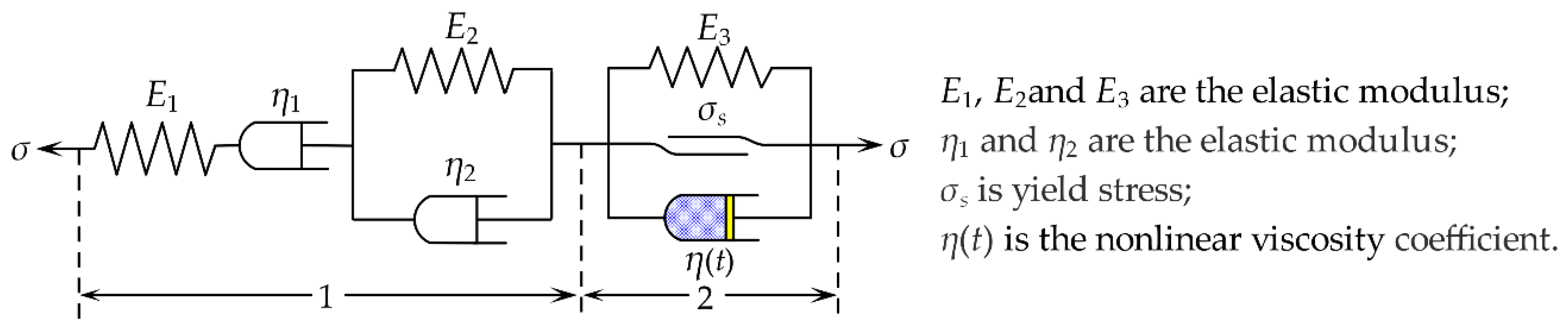
4. Establishment of Nonlinear Viscoelastic–Plastic Creep Model
4.1. Model Development
4.1.1. Improvement of Non-Newtonian Component
4.1.2. Introduction of Negative Elastic Modulus E3 (Shear Modulus G3)
4.2. Nonlinear Viscoelastic–Plastic Creep Model
5. Model Validation and Parametric Analysis
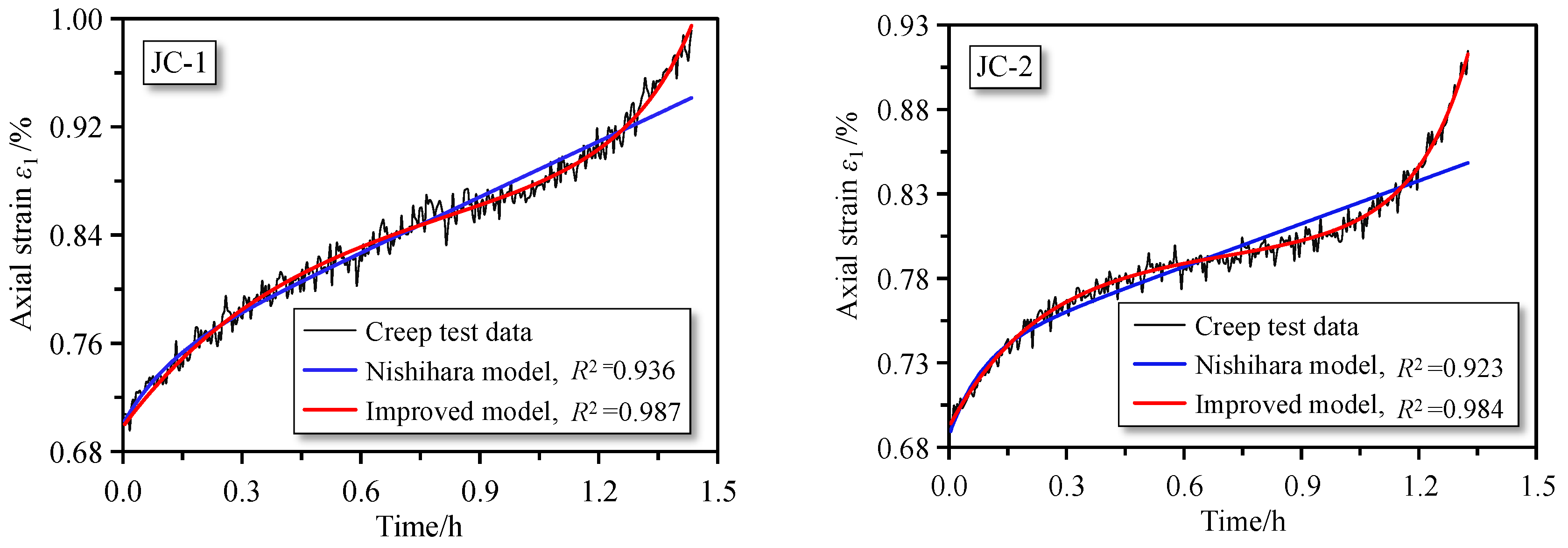
5.1. Model Validation
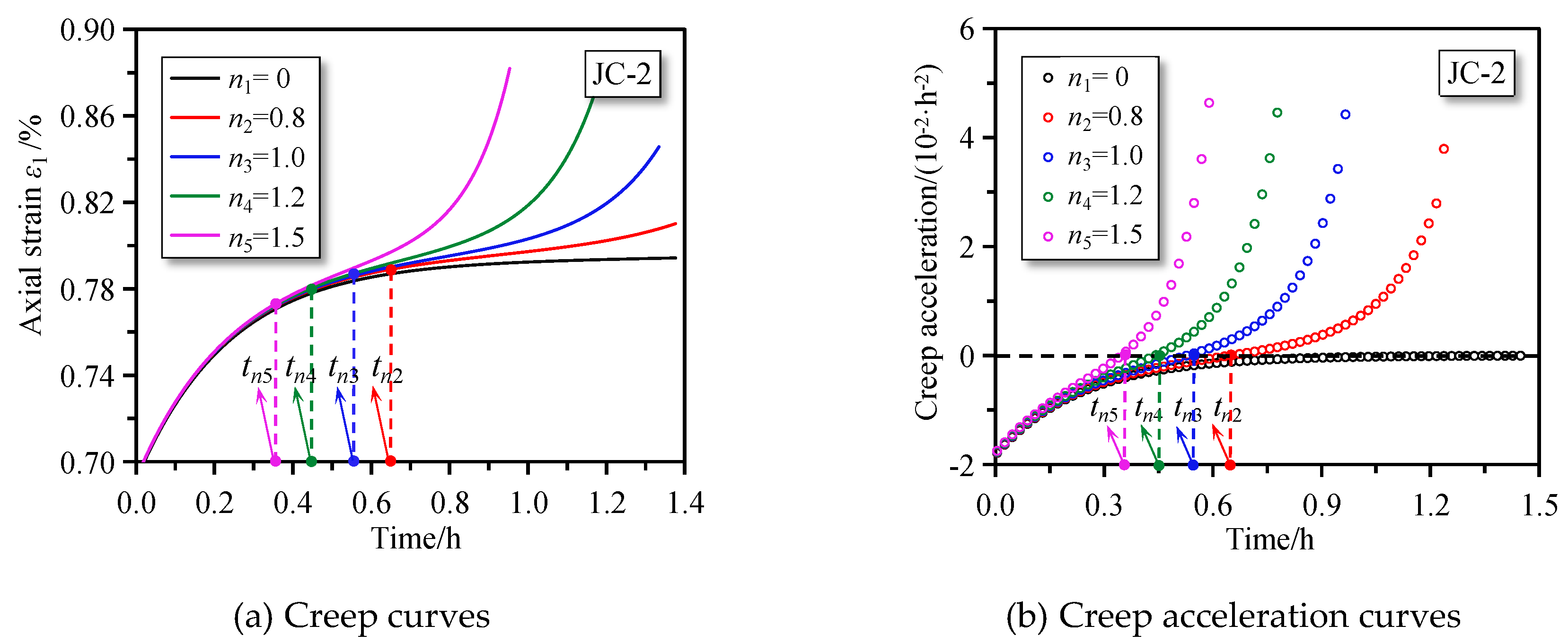
5.2. Parametric Sensitivity Analysis
6. Conclusions
- (1)
- A series of multistage creep tests was performed on coal specimens with different stress levels and found that the concavity–convexity in creep curves will be changed when coal enters accelerating creep stage. That is, a creep inflection point is found on the creep curve. If the occurrence time of the creep inflection point can be accurately predicted, then some effective protective measures can be taken in time to avoid the potential safety hazards caused by coal instability and failure.
- (2)
- The exponential function was used to fit the accelerating creep data of coal specimens and achieved the desired results. Thus, a non-Newtonian component was proposed to replace the ideal Newtonian component. Furthermore, a negative elastic modulus E3 (shear modulus G3) was introduced to establish a nonlinear viscoelastic–plastic body to describe the accelerating creep characteristics of coal. Therefore, a new nonlinear viscoelastic–plastic creep model that consists of a generalized Kelvin and improved viscoelastic–plastic body was proposed, and the creep equation of the improved model under constant confining pressure was also derived.
- (3)
- 1 stop analysis software was used to fit the experimental data of the non-decay creep characteristics in the coal multistage creep tests based on the proposed nonlinear viscoelastic–plastic creep model. The results show that the fitting correlation coefficients have R2 values above 0.98, and the fitting curves are highly consistent with the experimental data, which can accurately describe the non-decay creep characteristic of coal and verify the correctness and applicability of the model.
- (4)
- The analyses of creep rate and creep acceleration curves using different acceleration creep indexes n show that if n = 0, then the non-Newtonian component will degenerate into the ideal Newtonian body, and only the decelerating and steady-state creeps of coal will occur. If n > 0, then coal specimens will present non-decay creep characteristic. When the acceleration creep index n is large, the level of creep acceleration increases, and the occurrence of coal instability appears earlier.
Author Contributions
Funding
Conflicts of Interest
References
- Xie, H.P.; Gao, F.; Ju, Y. Research and development of rock mechanics in deep ground engineering. Chin. J. Rock Mech. Eng. 2015, 34, 2161–2178. [Google Scholar]
- Yang, S.Q.; Chen, M.; Jing, H.W.; Chen, K.F.; Meng, B. A case study on large deformation failure mechanism of deep soft rock roadway in Xin’An coal mine, China. Eng. Geol. 2017, 217, 89–101. [Google Scholar] [CrossRef]
- Wei, S.; Qi, Z.; Luan, Y.L.; Zhang, X.P. A study of surface subsidence and coal pillar safety for strip mining in a deep mine. Environ. Earth Sci. 2018, 77, 627. [Google Scholar]
- Xu, J.H.; Miao, X.X.; Zhang, X.C. Analysis of the time-dependence of the coal pillar stability. J. Chin. Coal Soc. 2005, 30, 433–437. [Google Scholar]
- Li, Q.; Shi, W.; Yang, R. Deformation mechanisms in a coal mine roadway in extremely swelling soft rock. SpringerPlus 2016, 5, 1310. [Google Scholar] [CrossRef]
- Liu, C.; Zhou, F.B.; Yang, K.K.; Xiang, X.; Liu, Y.K. Failure analysis of borehole liners in soft coal seam for gas drainage. Eng. Fail. Anal. 2014, 42, 274–283. [Google Scholar] [CrossRef]
- Chen, L.; Wang, E.Y.; Ou, J.C.; Fu, J.W. Coal and gas outburst hazards and factors of the No. B-1 Coalbed, Henan, China. Geosci. J. 2017, 22, 171–182. [Google Scholar] [CrossRef]
- Kim, D.K. Comparisons of overstress theory with an empirical model in creep prediction for cohesive soils. KSCE J. Civ. Eng. 2005, 9, 489–494. [Google Scholar] [CrossRef]
- Xia, C.C.; Jin, L.; Guo, R. Nonlinear theoretical rheological model for rock: a review and some problems. Chin. J. Rock Mech. Eng. 2011, 30, 454–463. [Google Scholar]
- Zhang, X.D.; Li, Y.J.; Zhang, S.G.; Huo, B.R. Creep study of soft rock and its engineering application. Chin. J. Rock Mech. Eng. 2004, 23, 1635–1639. [Google Scholar]
- Ma, K.; Wan, X.L.; Jia, W.F.; Wan, C.H. Advances in rock creep model research and discussion on some issues. Coal Geol. Chin. 2011, 23, 43–47. [Google Scholar] [CrossRef]
- Yang, Z.W.; Jin, A.B.; Zhou, Y.; Yan, Q.; Wang, K.; Gao, Y.J. Parametric analysis of Burgers model and creep properties of rock with particle flow code. Rock Soil Mech. 2015, 36, 240–248. [Google Scholar]
- Shao, J.F.; Zhu, Q.Z.; Su, K. Modeling of creep in rock materials in terms of material degradation. Comput. Geotech. 2003, 30, 549–555. [Google Scholar] [CrossRef]
- Shao, J.F.; Chau, K.T.; Feng, X.T. Modeling of anisotropic damage and creep deformation in brittle rocks. Int. J. Rock Mech. Min. Sci. 2006, 43, 582–592. [Google Scholar] [CrossRef]
- Ping, C.; Wen, Y.D.; Wang, Y.X.; Yuan, H.P.; Yuan, B.X. Study on nonlinear damage creep constitutive model for high-stress soft rock. Environ. Earth Sci. 2016, 75, 900. [Google Scholar]
- Kang, J.H.; Zhou, F.B.; Liu, C.; Liu, Y.K. A fractional nonlinear creep model for coal considering damage effect and experimental validation. Int. J. Nonlinear Mech. 2015, 76, 20–28. [Google Scholar] [CrossRef]
- Cai, T.; Feng, Z.; Jiang, Y. An improved hardening-damage creep model of lean coal: A theoretical and experimental study. Arab. J. Geosci. 2018, 11, 645. [Google Scholar] [CrossRef]
- Cai, T.T.; Feng, Z.C.; Zhao, D.; Jiang, Y.L. A creep model for lean coal based on hardening-damage mechanism. Rock Soil Mech. 2018, 39, 70–77. [Google Scholar]
- Wang, L.J.; Zhou, H.W.; Rong, T.L.; Ren, W.G. Research on experimental and nonlinear creep constitutive model of coal at depth. J. Chin. Coal Soc. 2018, 43, 110–116. [Google Scholar]
- Ömer, A.; Ito, T.; Özbay, U.; Kwasniewski, M.; Shariar, K.; Okuno, T.; Özgenoğlu, A.; Malan, D.F.; Okada, T. ISRM suggested methods for determining the creep characteristics of rock. Rock Mech. Rock Eng. 2014, 47, 275–290. [Google Scholar]
- The Professional Standards Compilation Group of Peoples Republic of China. DL/T5368-2007 Code for Rock Tests of Hydroelectric and Water Conservancy Engineering[S]; Water and Power Press: Beijing, China, 2007. [Google Scholar]
- Yang, Y.; Li, Y.; Zhou, X.K. Study on the coal creep test based on the improved Nishihara model. J. Chin. Coal Soc. 2014, 39, 2190–2194. [Google Scholar]
- Liu, H.Z.; Xie, H.Q.; He, J.D.; Xiao, M.L.; Zhuo, L. Nonlinear creep damage constitutive model for soft rocks. Mech. Time Depend. Mater. 2016, 21, 1–24. [Google Scholar] [CrossRef]
- Tao, B.; Wu, F.Q.; Guo, G.M.; Zhou, R.G. Flexibility of visco-elastoplastic model to rheological characteristics of rock and solution of rheological parameter. Chin. J. Rock Mech. Eng. 2005, 24, 3165–3171. [Google Scholar]
- Cao, S.G.; Bian, J.; Li, P. Rheologic constitutive relationship of rocks and a modifical model. Chin. J. Rock Mech. Eng. 2002, 21, 632–634. [Google Scholar]
- Jiang, Q.; Qi, Y.; Wang, Z.; Zhou, C. An extended Nishihara model for the description of three stages of sandstone creep. Geophys. J. Int. 2013, 193, 841–854. [Google Scholar] [CrossRef]
- Cao, L.; Lu, H.F.; Yao, D.X.; Zhou, L.; Xu, Z.D. Research summary of rock creep model and creep parameter identification. Open J. Nat. Sci. 2018, 6, 395–400. [Google Scholar] [CrossRef]
- Cheng, X. Optimization·Fitting·Modeling: Application Detailed; China Building Materials Press: Beijing, China, 2012; pp. 1–4. [Google Scholar]
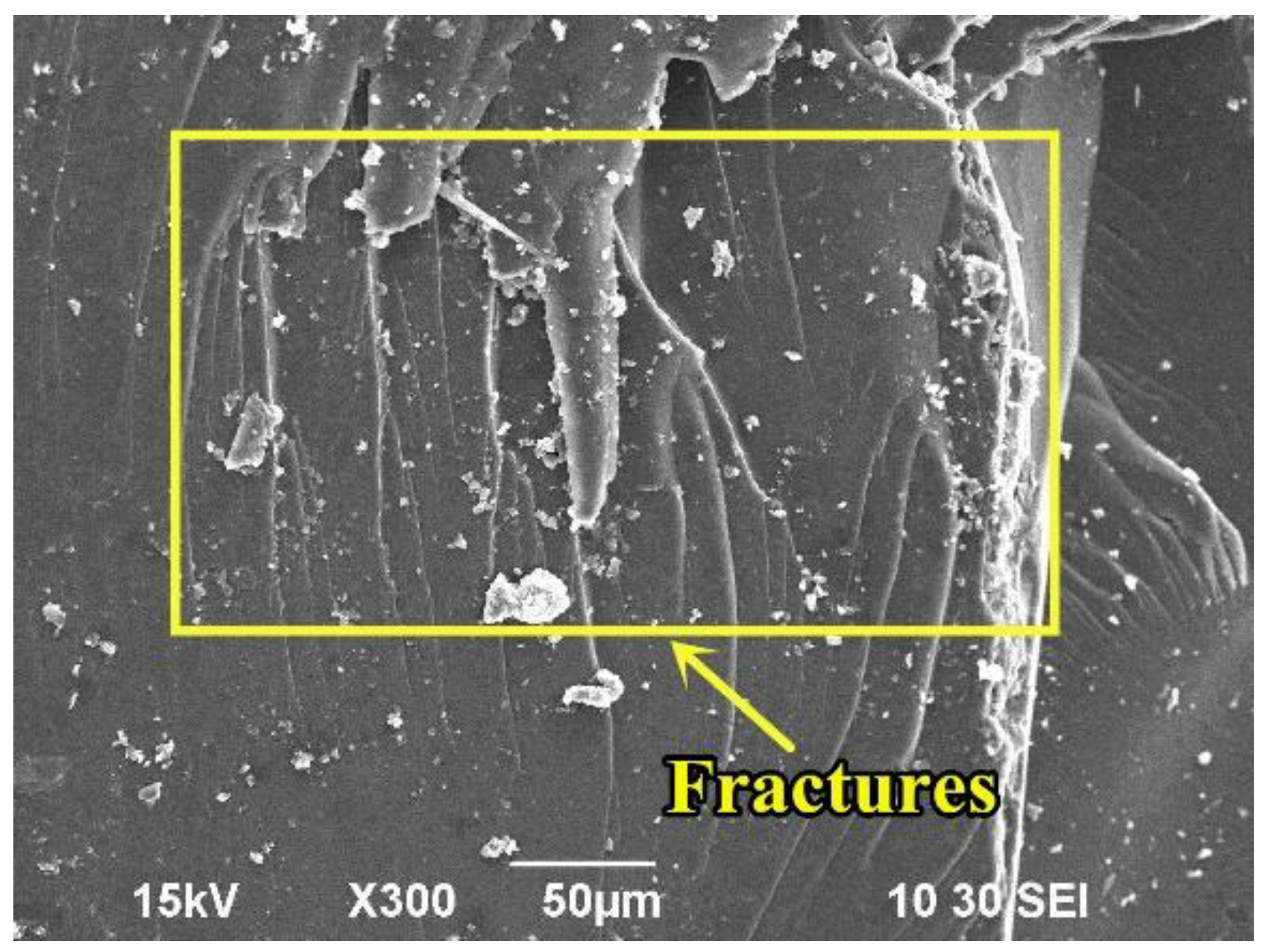





| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Adsorption constant a | 29.412 m3/t | Mad | 0.44% |
| Adsorption constant b | 2.252 MPa−1 | Ad | 8.12% |
| True density | 1.56 g·cm−3 | Vdaf | 10.58% |
| Apparent density | 1.49 g·cm−3 | FCad | 80.86% |
| Specimen | σ1/MPa | G1/GPa | G2/GPa | η1/(GPa·h) | R2 |
|---|---|---|---|---|---|
| JC-1 | 5 | 15.049 | 39.493 | 5.339 | 0.963 |
| 15 | 24.120 | 102.695 | 18.413 | 0.969 | |
| 25 | 23.091 | 97.723 | 16.608 | 0.956 | |
| 35 | 18.025 | 286.917 | 352.267 | 0.934 | |
| JC-2 | 5 | 9.814 | 44.575 | 6.066 | 0.937 |
| 15 | 17.452 | 128.684 | 9.252 | 0.925 | |
| 25 | 20.630 | 194.027 | 33.941 | 0.931 | |
| 35 | 19.599 | 317.160 | 136.092 | 0.953 |
| Specimen | ε0 | A | n | R2 |
|---|---|---|---|---|
| JC-1 | 0.8306 | 1.658×10−3 | 3.1724 | 0.9656 |
| JC-2 | 0.7946 | 2.24×10−5 | 6.4569 | 0.9765 |
| Model | Specimen | G1/GPa | G2/GPa | G3/GPa | η1/(GPa·h) | η0/(GPa·h) | η2/(GPa·h) | n | Sh/MPa | R2 |
|---|---|---|---|---|---|---|---|---|---|---|
| Improved model | JC-1 | 20.080 | 86.918 | −164.902 | 37.604 | 451.877 | - | 1.507 | 41.027 | 0.987 |
| JC-2 | 21.213 | 144.289 | −11.506 | 34.096 | 8.211 | - | 1.114 | 43.991 | 0.984 | |
| Nishihara model | JC-1 | 20.815 | 455.497 | - | 47.673 | - | 0.010 | - | 43.999 | 0.936 |
| JC-2 | 21.326 | 305.679 | - | 24.715 | - | 0.361 | - | 43.969 | 0.923 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Li, B.; Zhang, C.; Li, P. Nonlinear Viscoelastic–Plastic Creep Model Based on Coal Multistage Creep Tests and Experimental Validation. Energies 2019, 12, 3468. https://doi.org/10.3390/en12183468
Zhang J, Li B, Zhang C, Li P. Nonlinear Viscoelastic–Plastic Creep Model Based on Coal Multistage Creep Tests and Experimental Validation. Energies. 2019; 12(18):3468. https://doi.org/10.3390/en12183468
Chicago/Turabian StyleZhang, Junxiang, Bo Li, Conghui Zhang, and Peng Li. 2019. "Nonlinear Viscoelastic–Plastic Creep Model Based on Coal Multistage Creep Tests and Experimental Validation" Energies 12, no. 18: 3468. https://doi.org/10.3390/en12183468
APA StyleZhang, J., Li, B., Zhang, C., & Li, P. (2019). Nonlinear Viscoelastic–Plastic Creep Model Based on Coal Multistage Creep Tests and Experimental Validation. Energies, 12(18), 3468. https://doi.org/10.3390/en12183468







