Disturbance-Observer-Based Model Predictive Control for Battery Energy Storage System Modular Multilevel Converters
Abstract
:1. Introduction
- (1)
- A MPC method based on prediction accuracy improvement via two DOBs is designed for a grid-connected MMCs of battery energy storage systems, which has a simple structure and quite low cost of computation with the minimum order.
- (2)
- The accurate estimation and feedforward compensation for disturbances are achieved without sacrificing the original control performance.
- (3)
- The disturbance items which act on the cost functions during each sampling period assure the cost functions always maintain optimal performance.
2. MPC Strategy of the MMC
2.1. MPC Strategy for AC Current
2.2. MPC Strategy for Circulating Current
2.3. MPC Strategy for Capacitor Voltage Balancing
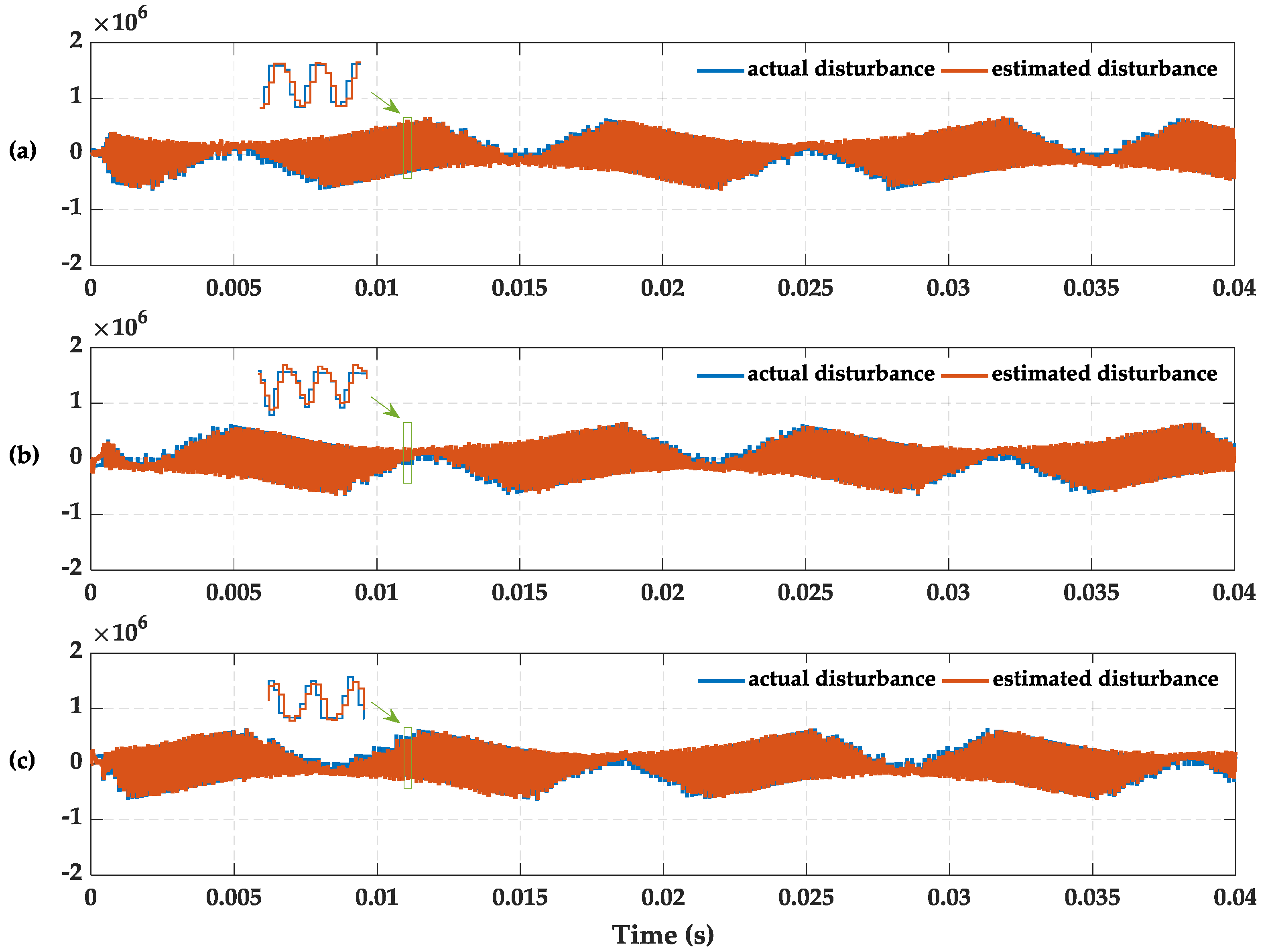
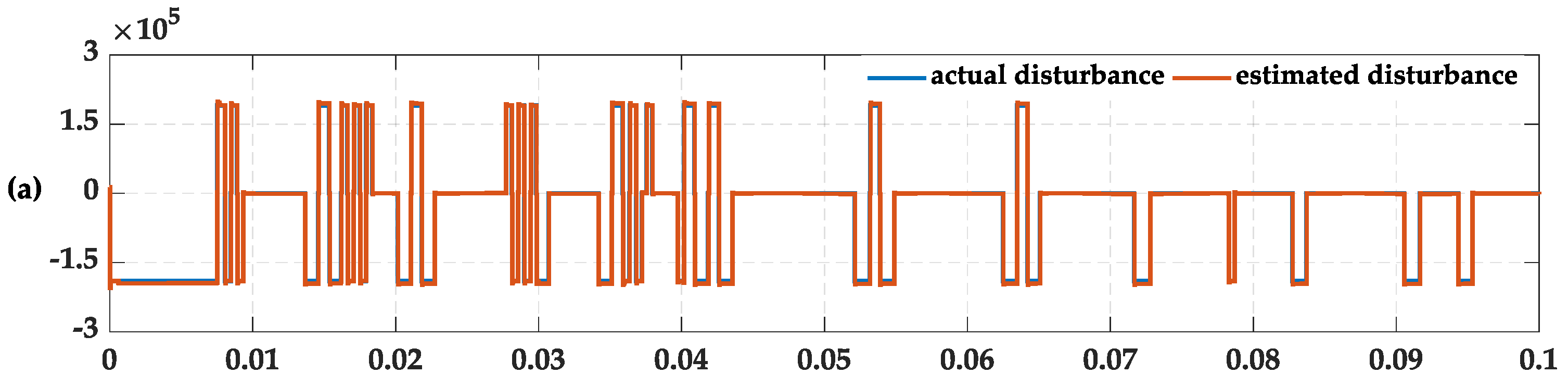
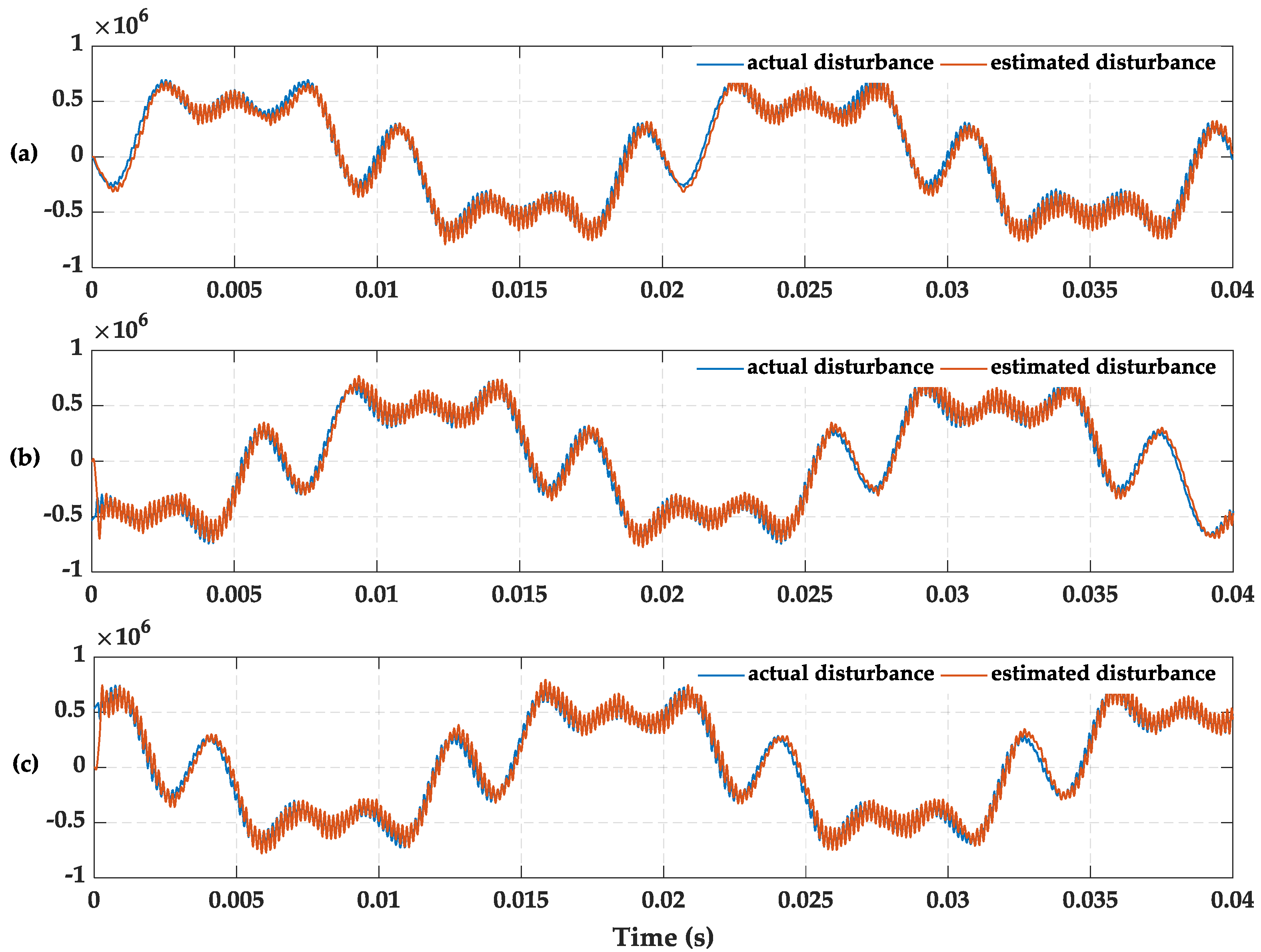
3. MPC Strategy of the MMC with Disturbance Observer
3.1. MPC Strategy for AC Current with DOB 1
3.2. MPC Strategy for Circulating Current with DOB 2
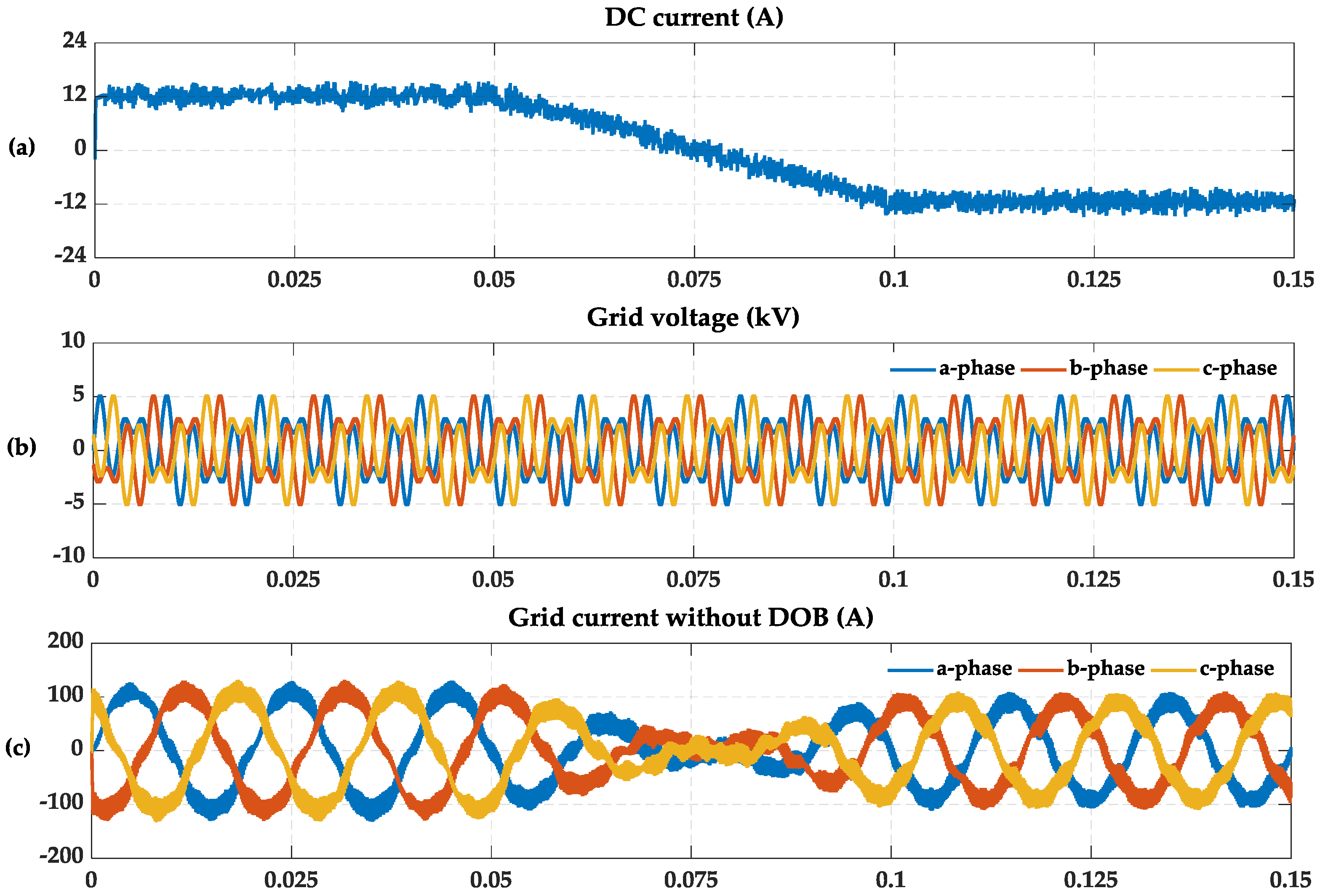
4. Simulation Results
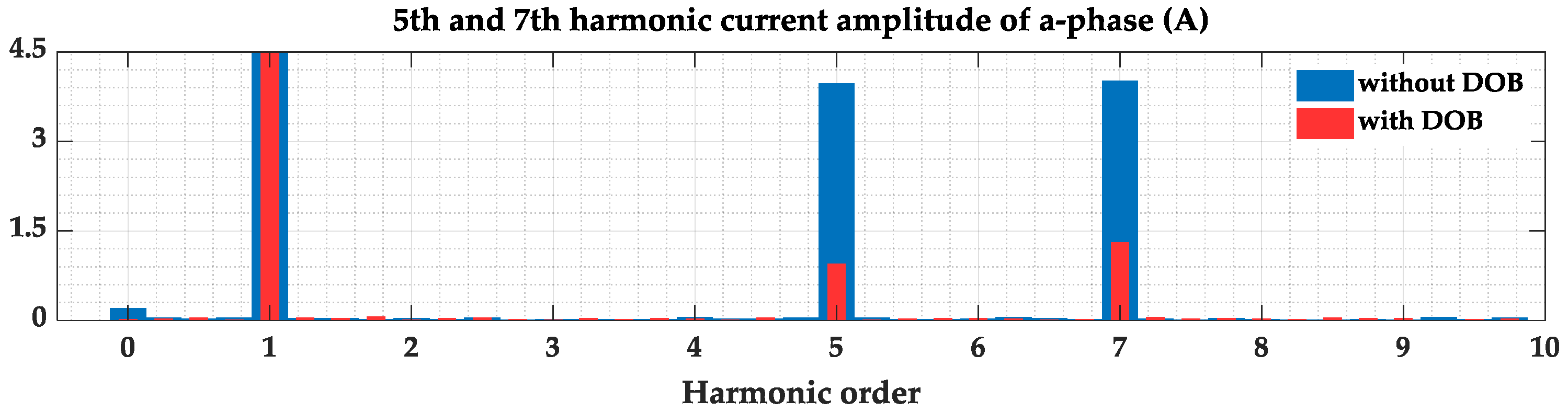
- Harmonic: 3-phase grid voltage with 30% of 5th and 7th harmonics.
- 3-phase voltage unbalance: a-phase line-to-ground fault.
- Voltage sag: 3-phase grid voltage with 80% of reduction in the period of 0.01 s–0.03 s.
- Parameter mismatches: actual inductance values change.
- Power reversal: the power flow reverses from 0.05 s to 0.1 s; the 3-phase grid voltage contain harmonics and voltage sag; and the actual inductance values decline during the entire simulation time.
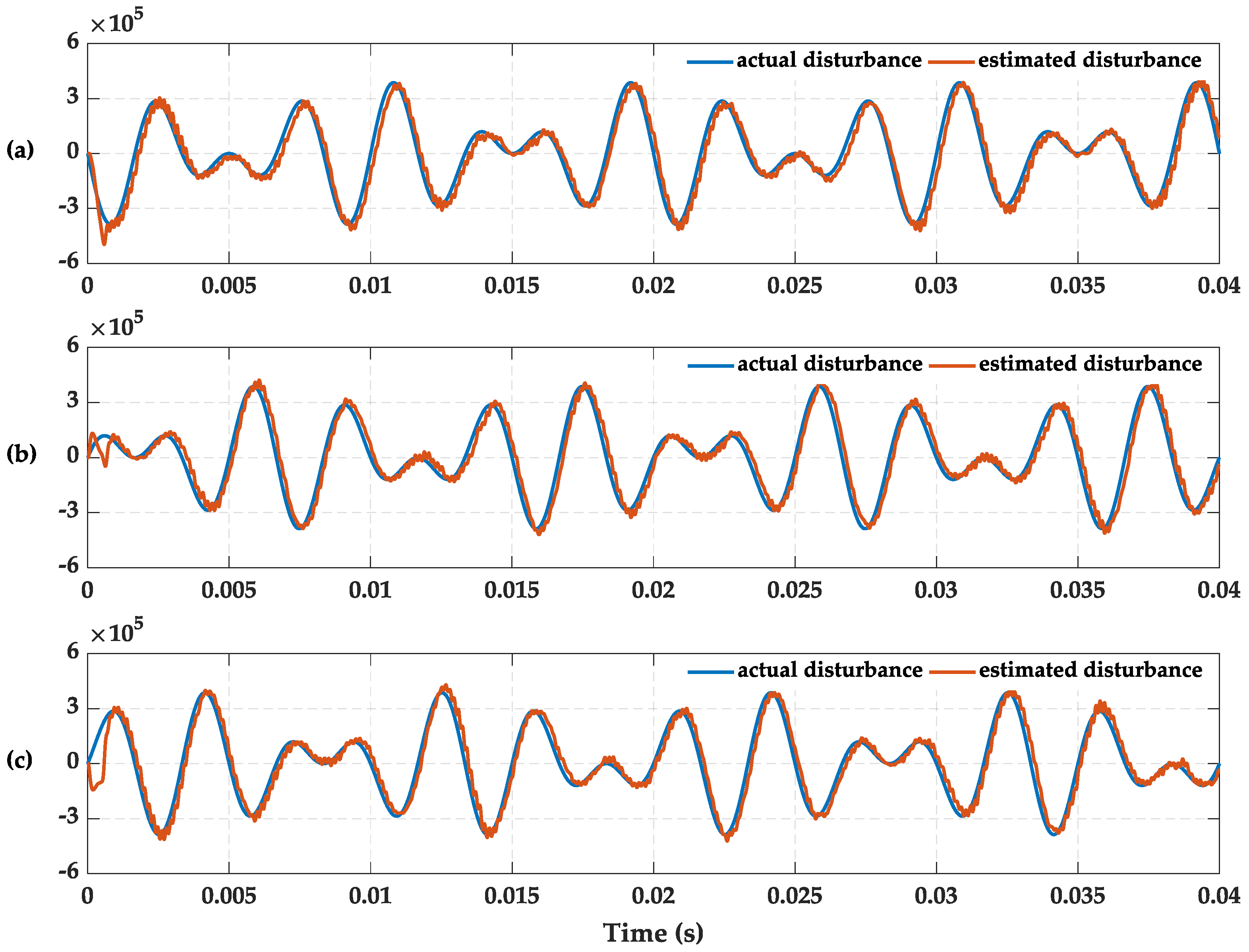
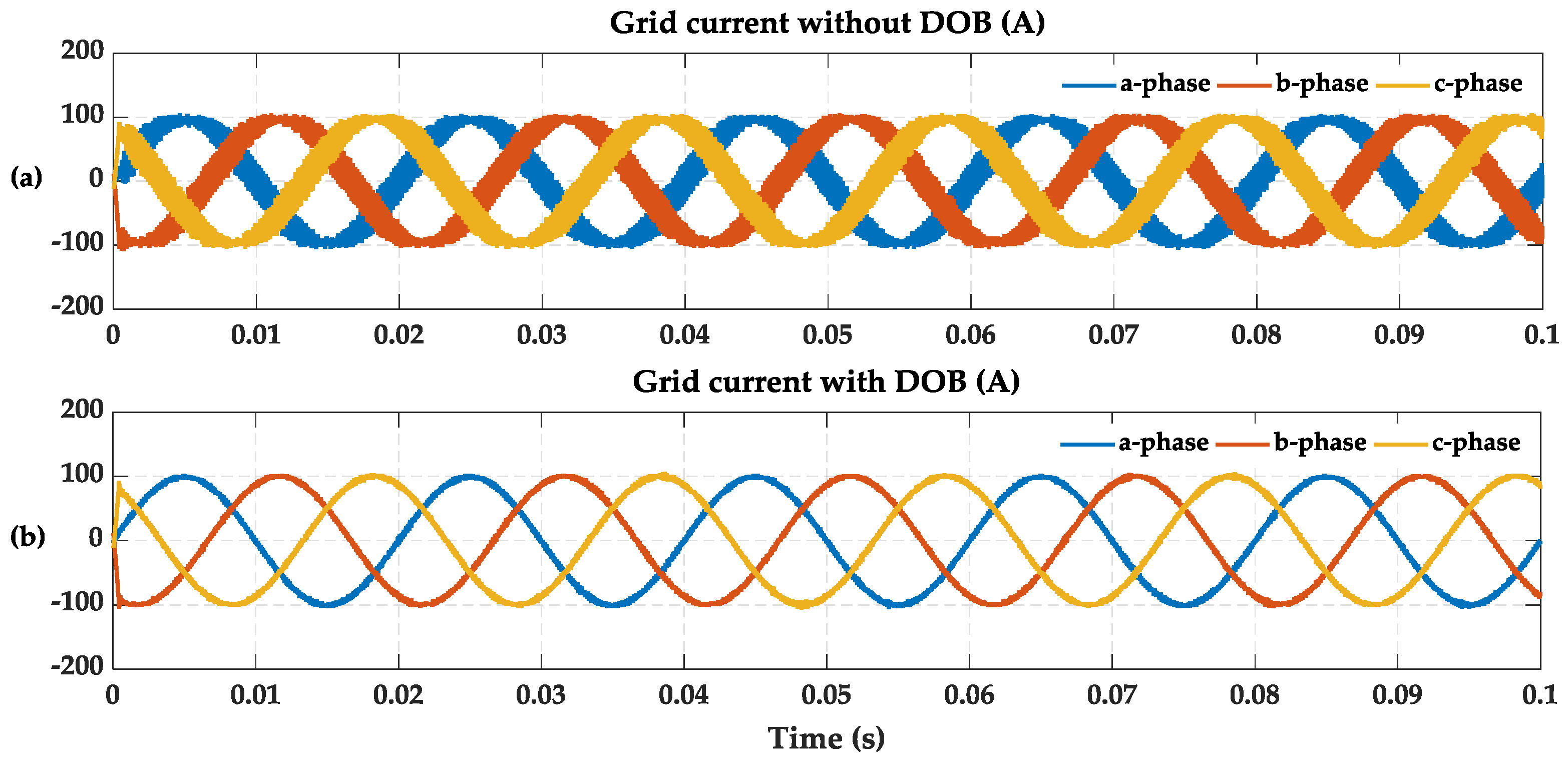
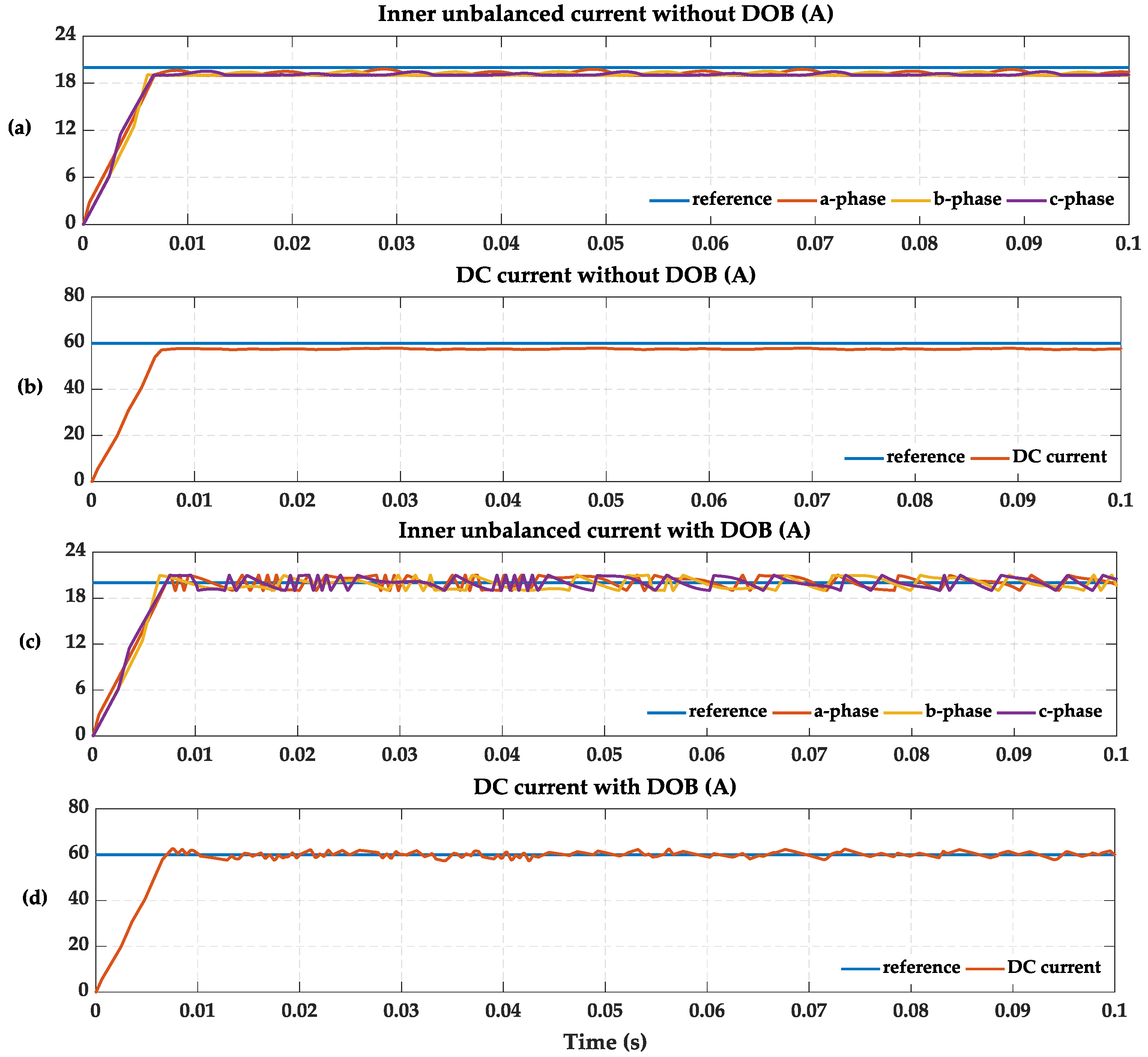
4.1. Simulations under Harmonic Condition
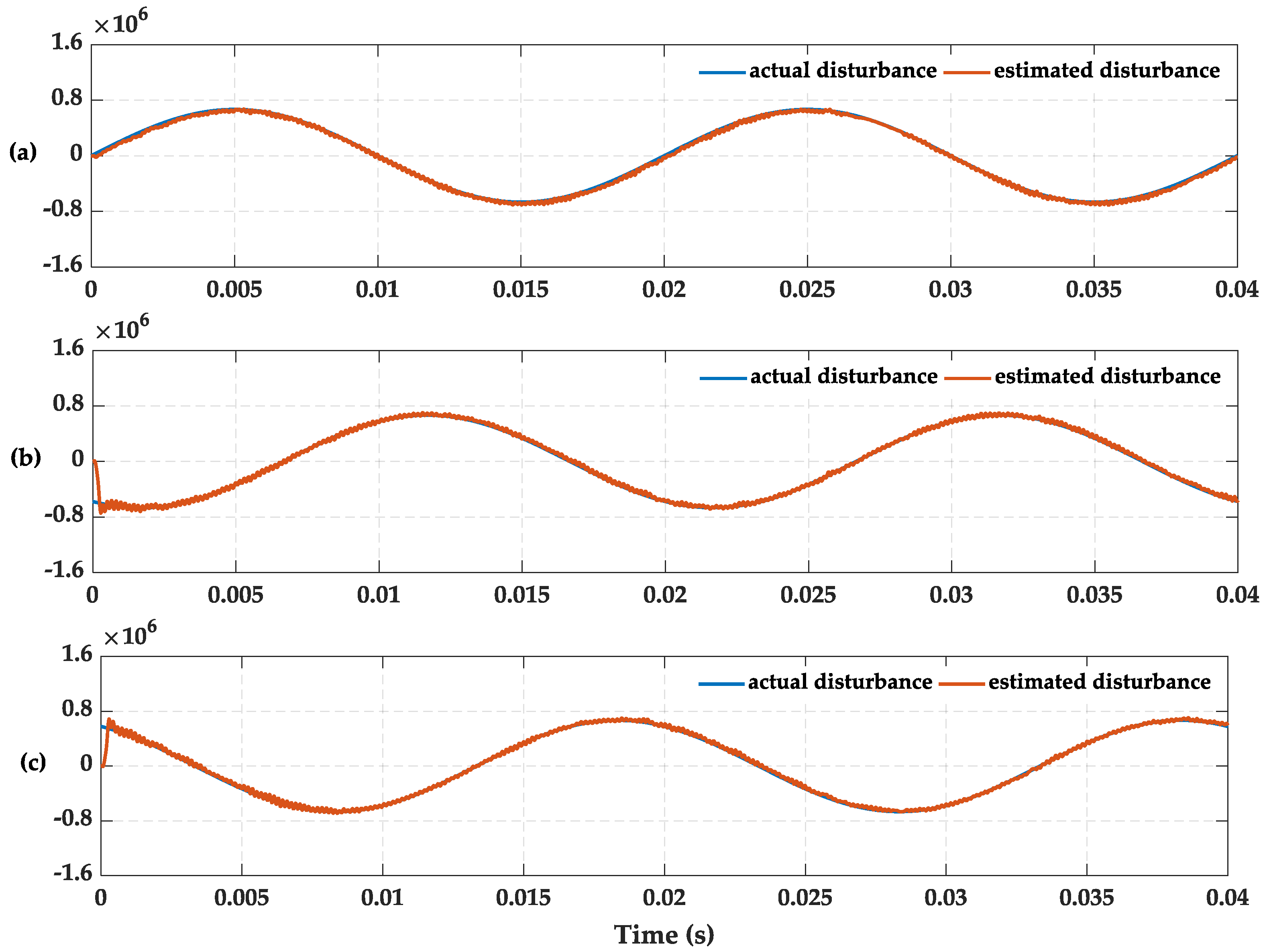
4.2. Simulations Under 3-Phase Voltage Unbalance Condition
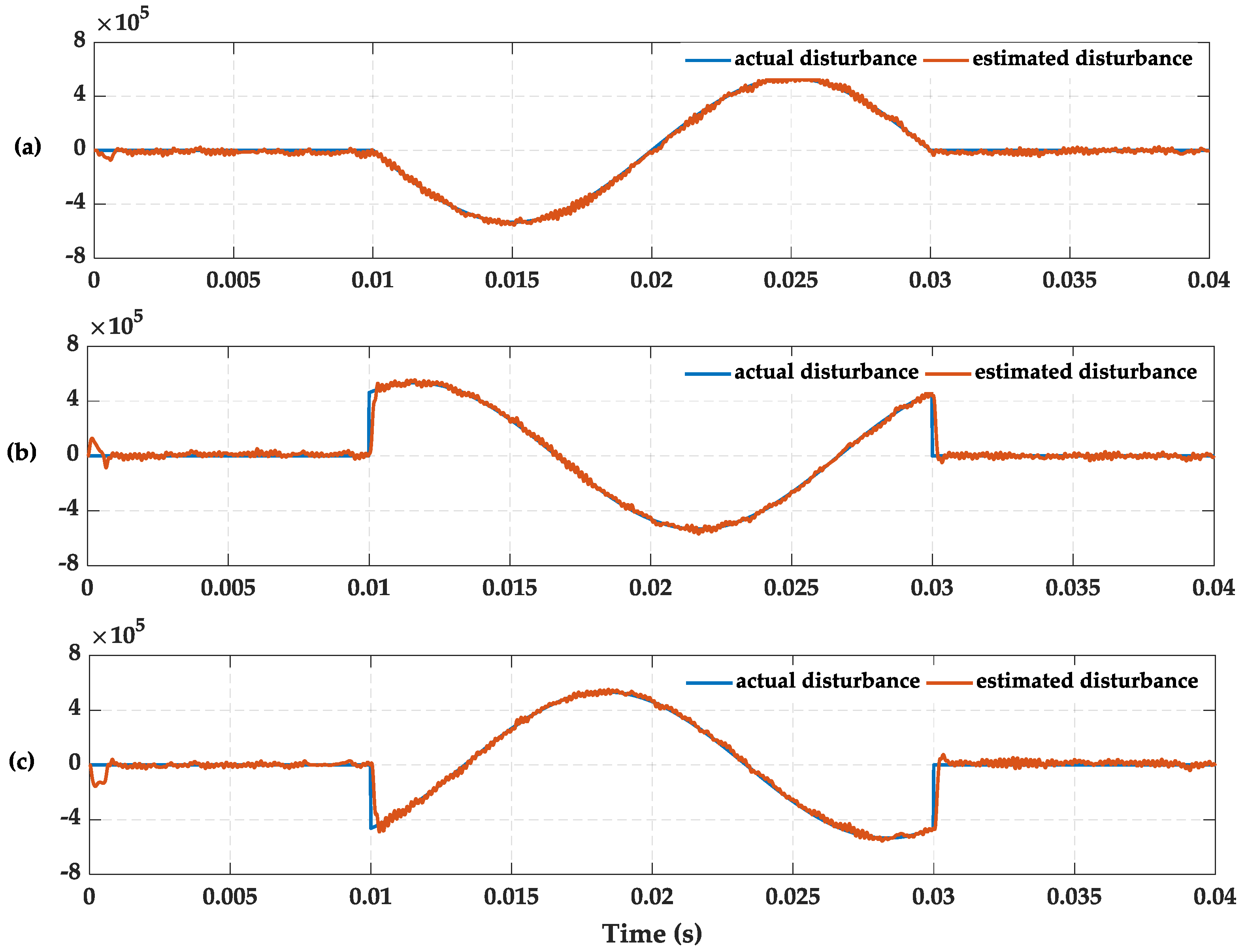
4.3. Simulations Under Voltage Sag Condition
4.4. Simulations under Parameter Mismatches Condition
4.4.1. Actual Inductance Value Reduction
4.4.2. Actual Inductance Values Increase
4.5. Power Reversal
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Yang, T.; Mok, K.T.; Tan, S.C.; Lee, C.K.; Hui, S.Y.R. Electric springs with coordinated battery management for reducing voltage and frequency fluctuations in microgrids. IEEE Trans. Smart Grid 2018, 9, 1943–1952. [Google Scholar] [CrossRef]
- Jiang, W.; Xue, S.; Zhang, L.; Xu, W.; Yu, K.; Chen, W.; Zhang, L. Flexible power distribution control in asymmetrical cascaded multilevel converter based hybrid energy storage system. IEEE Trans. Ind. Electron. 2018, 65, 6150–6159. [Google Scholar] [CrossRef]
- Yang, P.; Xia, Y.; Yu, M.; Wei, W.; Peng, Y. A decentralized coordination control method for parallel bidirectional power converters in a hybrid AC/DC microgrid. IEEE Trans. Ind. Electron. 2018, 65, 6217–6228. [Google Scholar] [CrossRef]
- Kwon, O.; Kim, J.S.; Kwon, J.M.; Kwon, B.H. Bidirectional grid-connected single-power-conversion converter with low input battery voltage. IEEE Trans. Ind. Electron. 2018, 65, 3136–3144. [Google Scholar] [CrossRef]
- Mehrasa, M.; Pouresmaeil, E.; Zabihi, S.; Vechiu, I.; Catalão, J.P.S. A multi-loop control technique for the stable operation of modular multilevel converters in HVDC transmission systems. Electr. Power Energy Syst. 2018, 96, 194–207. [Google Scholar] [CrossRef]
- Lee, K.J.; Park, B.G.; Kim, R.Y.; Hyun, D.S. Robust predictive current controller based on a disturbance estimator in a three-phase grid-connected inverter. IEEE Trans. Power Electron. 2012, 27, 276–283. [Google Scholar] [CrossRef]
- Xia, C.; Wang, M.; Song, Z.; Liu, T. Robust model predictive current control of three-phase voltage source PWM rectifier with online disturbance observation. IEEE Trans. Ind. Inform. 2012, 8, 459–471. [Google Scholar] [CrossRef]
- Mehrasa, M.; Pouresmaeil, E.; Akorede, M.F.; Zabihi, S.; Catalão, J.P.S. Function-based modulation control for modular multilevel converters under varying loading and parameters conditions. IET Gener. Trans. Distrib. 2017, 11, 3222–3230. [Google Scholar] [CrossRef]
- Hagiwara, M.; Akagi, H. Experiment and simulation of a modular push–pull PWM converter for a battery energy storage system. IEEE Trans. Ind. Appl. 2014, 50, 1131–1140. [Google Scholar] [CrossRef]
- Maharjan, L.; Yamagishi, T.; Akagi, H. Active-power control of individual converter cells for a battery energy storage system based on a multilevel cascade PWM converter. IEEE Trans. Power Electron. 2012, 27, 1099–1107. [Google Scholar] [CrossRef]
- Song, J.; Zhang, W.; Liang, H.; Jiang, J.; Yu, W. Fault-tolerant control for a flexible group battery energy storage system based on cascaded multilevel converters. Energies 2018, 11, 171. [Google Scholar] [CrossRef]
- Liang, H.; Guo, L.; Song, J.; Yang, Y.; Zhang, W.; Qi, H. State-of-charge balancing control of a modular multilevel converter with an integrated battery energy storage. Energies 2018, 11, 873. [Google Scholar] [CrossRef]
- Ota, J.I.Y.; Sato, T.; Akagi, H. Enhancement of performance, availability, and flexibility of a battery energy storage system based on a modular multilevel cascaded converter (MMCC-SSBC). IEEE Trans. Power Electron. 2016, 31, 2791–2799. [Google Scholar] [CrossRef]
- Zhang, M.; Huang, L.; Yao, W.; Lu, Z. Circulating harmonic current elimination of a CPS-PWM-based modular multilevel converter with a plug-in repetitive controller. IEEE Trans. Power Electron. 2014, 29, 2083–2097. [Google Scholar] [CrossRef]
- Yang, L.; Li, Y.; Li, Z.; Wang, P.; Xu, S.; Gou, R. Loss optimization of MMC by second-order harmonic circulating current injection. IEEE Trans. Power Electron. 2018, 33, 5739–5753. [Google Scholar] [CrossRef]
- Mehrasa, M.; Pouresmaeil, E.; Zabihi, S.; Catalao, J.P. Dynamic model, control and stability analysis of MMC in HVDC transmission systems. IEEE Trans. Power Deliv. 2017, 32, 1471–1482. [Google Scholar] [CrossRef]
- Mehrasa, M.; Pouresmaeil, E.; Taheri, S.; Vechiu, I.; Catalão, J.P. Novel control strategy for modular multilevel converters based on differential flatness theory. IEEE J. Emerg. Sel. Top. Power Electron. 2017, 6, 888–897. [Google Scholar] [CrossRef]
- Mehrasa, M.; Pouresmaeil, E.; Zabihi, S.; Caballero, J.T.; Catalão, J. A novel modulation function-based control of modular multilevel converters for high voltage direct current transmission systems. Energies 2016, 9, 867. [Google Scholar] [CrossRef]
- Qin, J.; Saeedifard, M. Predictive control of a modular multilevel converter for a back-to-back HVDC system. IEEE Trans. Power Deliv. 2012, 27, 1538–1547. [Google Scholar]
- Yang, J.; Li, S.; Chen, X.; Li, Q. Disturbance rejection of dead-time processes using disturbance observer and model predictive control. Chem. Eng. Res. Des. 2011, 89, 125–135. [Google Scholar] [CrossRef]
- Qin, S.J.; Badgwell, T.A. A survey of industrial model predictive control technology. Control Eng. Pract. 2003, 11, 733–764. [Google Scholar] [CrossRef] [Green Version]
- Liao, K.; Xu, Y. A robust load frequency control scheme for power systems based on second-order sliding mode and extended disturbance observer. IEEE Trans. Ind. Inform. 2018, 14, 3076–3086. [Google Scholar] [CrossRef]
- Wang, C.; Mi, Y.; Fu, Y.; Wang, P. Frequency control of an isolated micro-grid using double sliding mode controllers and disturbance observer. IEEE Trans. Smart Grid 2018, 9, 923–930. [Google Scholar] [CrossRef]
- Wang, B.; Dong, Z.; Yu, Y.; Wang, G.; Xu, D. Static-errorless deadbeat predictive current control using second-order sliding-mode disturbance observer for induction machine drives. IEEE Trans. Power Electron. 2018, 33, 2395–2403. [Google Scholar] [CrossRef]
- Yang, J.; Cui, H.; Li, S.; Zolotas, A. Optimized active disturbance rejection control for DC-DC buck converters with uncertainties using a reduced-order GPI observer. IEEE Trans. Circuits Syst. 2018, 65, 832–841. [Google Scholar] [CrossRef]
- Back, J.; Shim, H. Adding robustness to nominal output-feedback controllers for uncertain nonlinear systems: a nonlinear version of disturbance observer. Automatica 2008, 44, 2528–2537. [Google Scholar] [CrossRef]
- Liu, H.; Li, S. Speed control for PMSM servo system using predictive functional control and extended state observer. IEEE Trans. Ind. Electron. 2011, 59, 1171–1183. [Google Scholar] [CrossRef]
- Barros, J.D.; Silva, J.F.A.; Élvio, G.A.J. Fast-predictive optimal control of NPC multilevel converters. IEEE Trans. Ind. Electron. 2012, 60, 619–627. [Google Scholar] [CrossRef]
- Nguyen, H.T.; Jung, J.W. Disturbance-rejection-based model predictive control: flexible-mode design with a modulator for three-phase inverters. IEEE Trans. Ind. Electron. 2018, 65, 2893–2903. [Google Scholar] [CrossRef]
- Moon, J.W.; Gwon, J.S.; Park, J.W.; Kang, D.W.; Kim, J.M. Model predictive control with a reduced number of considered states in a modular multilevel converter for HVDC system. IEEE Trans. Power Deliv. 2015, 30, 608–617. [Google Scholar] [CrossRef]
- Tu, Q.; Xu, Z.; Xu, L. Reduced switching-frequency modulation and circulating current suppression for modular multilevel converters. IEEE Trans. Power Deliv. 2011, 26, 2009–2017. [Google Scholar]
- Moon, J.W.; Kim, C.S.; Park, J.W.; Kang, D.W.; Kim, J.M. Circulating current control in MMC under the unbalanced voltage. IEEE Trans. Power Deliv. 2013, 28, 1952–1959. [Google Scholar] [CrossRef]
- Kim, K.S.; Rew, K.H. Reduced order disturbance observer for discrete-time linear systems. Automatica 2013, 49, 968–975. [Google Scholar] [CrossRef]








| Parameters | Values | Units |
|---|---|---|
| Rated Power | 1.2 | MW |
| AC System Voltage | 9800 | V |
| Line Frequency | 50 | Hz |
| AC System Inductance | 2 | mH |
| DC Bus Voltage | 20 | kV |
| Number of SMs per arm | 10 | - |
| SM Capacitance | 0.002 | F |
| SM Capacitor Voltage | 2000 | V |
| Arm Inductance | 0.02 | H |
| Sampling and control period | 20 | μs |
| Parameters | DOB 1 | DOB 2 |
|---|---|---|
| Φ | 1 | 1 |
| Γ | 0.0017 | 0.0005 |
| G | 0.00002 | 0.00001 |
| C | 1 | 1 |
| K | 40,000 | 100,000 |
| λ | 0.2 | 1 |
| Phase | 5th/7th Harmonic (A) | THD (%) | ||
|---|---|---|---|---|
| Without DOB | With DOB | Without DOB | With DOB | |
| A | 3.99/4.04 | 0.95/1.30 | 6.97 | 2.86 |
| B | 4.02/4.01 | 0.90/1.31 | 6.75 | 2.76 |
| C | 4.03/4.01 | 0.97/1.31 | 6.68 | 2.97 |
| Phase | Fundamental Amplitude (A) | THD (%) | ||
|---|---|---|---|---|
| Without DOB | With DOB | Without DOB | With DOB | |
| A | 109 | 99.97 | 4.98 | 2.52 |
| B | 102.1 | 100.2 | 3.75 | 2.20 |
| C | 101.9 | 99.79 | 3.85 | 2.17 |
| Phase | Fundamental Amplitude (A) | THD (%) | ||
|---|---|---|---|---|
| Without DOB | With DOB | Without DOB | With DOB | |
| A | 95.66 | 100 | 21.56 | 2.12 |
| B | 95.68 | 99.96 | 21.44 | 2.06 |
| C | 95.62 | 100 | 21.56 | 2.13 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liao, Y.; You, J.; Yang, J.; Wang, Z.; Jin, L. Disturbance-Observer-Based Model Predictive Control for Battery Energy Storage System Modular Multilevel Converters. Energies 2018, 11, 2285. https://doi.org/10.3390/en11092285
Liao Y, You J, Yang J, Wang Z, Jin L. Disturbance-Observer-Based Model Predictive Control for Battery Energy Storage System Modular Multilevel Converters. Energies. 2018; 11(9):2285. https://doi.org/10.3390/en11092285
Chicago/Turabian StyleLiao, Yantao, Jun You, Jun Yang, Zuo Wang, and Long Jin. 2018. "Disturbance-Observer-Based Model Predictive Control for Battery Energy Storage System Modular Multilevel Converters" Energies 11, no. 9: 2285. https://doi.org/10.3390/en11092285
APA StyleLiao, Y., You, J., Yang, J., Wang, Z., & Jin, L. (2018). Disturbance-Observer-Based Model Predictive Control for Battery Energy Storage System Modular Multilevel Converters. Energies, 11(9), 2285. https://doi.org/10.3390/en11092285




