Hydraulic Fracturing Treatment Optimization for Low Permeability Reservoirs Based on Unified Fracture Design
Abstract
:1. Introduction
2. The Model
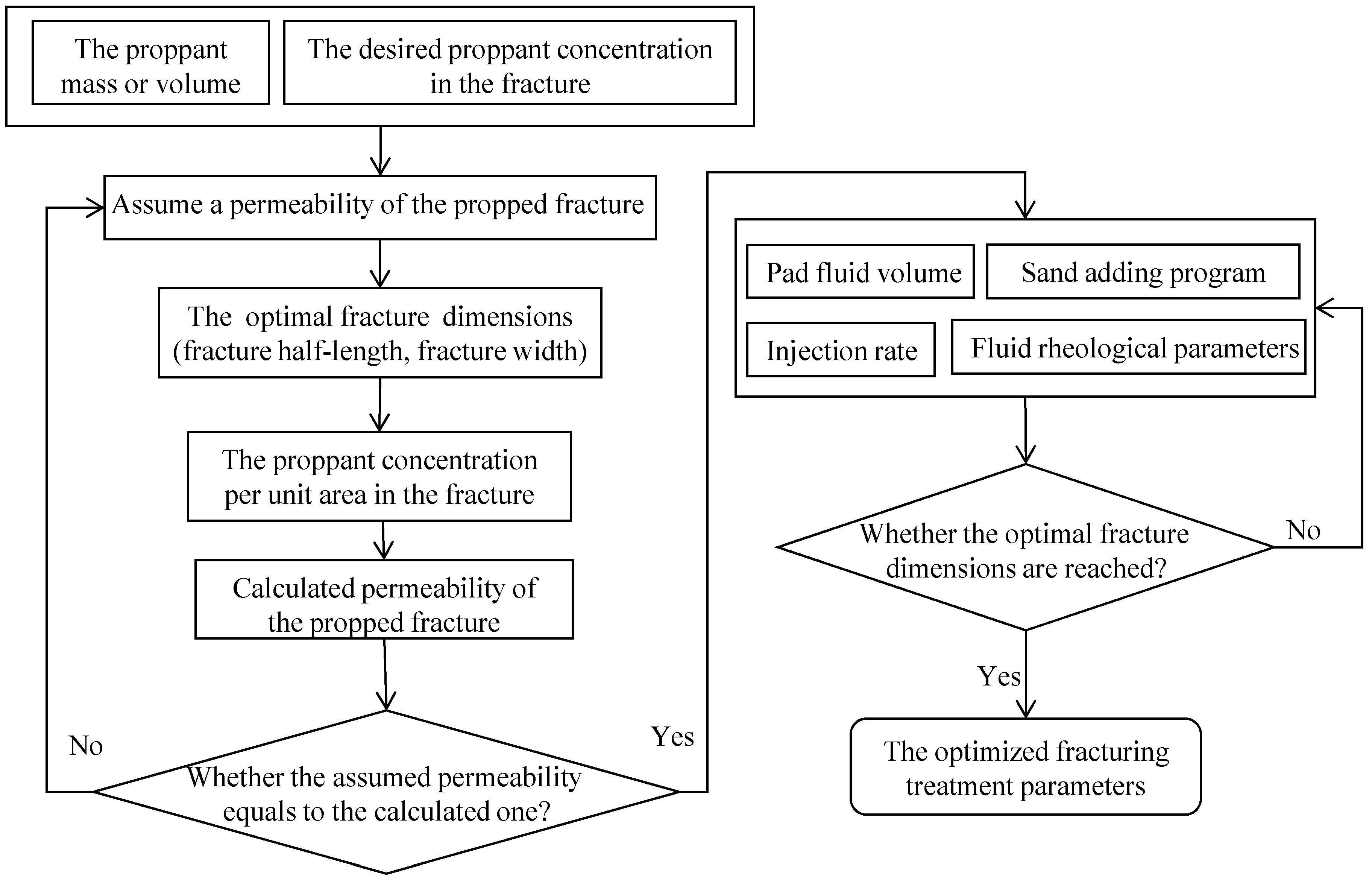
2.1. Fracture Geometry Optimization Based on UFD
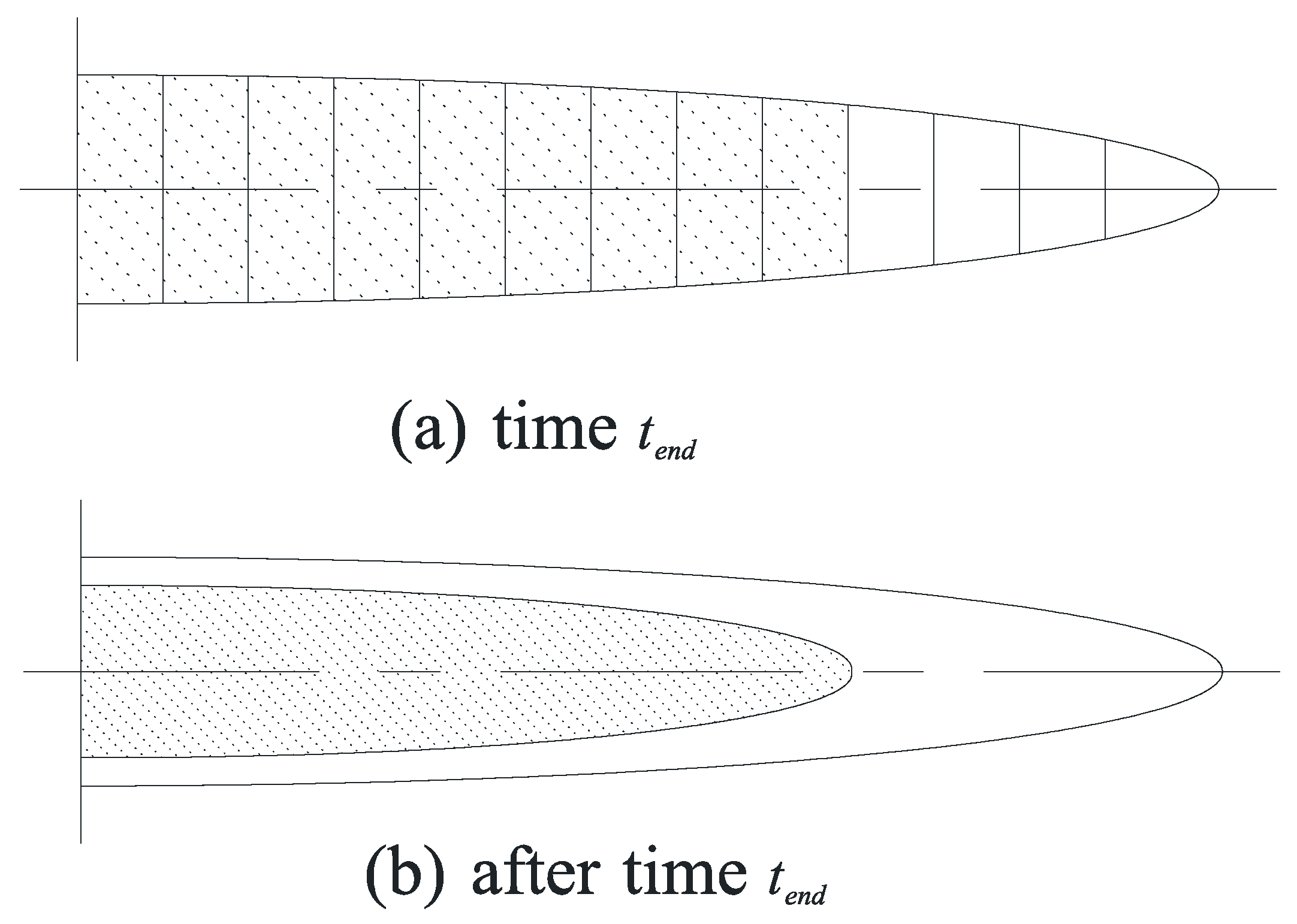
2.2. Treatment Optimization through a Fast Semi-Analytical Fracture Propagation Model
3. Results and Discussion
3.1. Basic Parameters
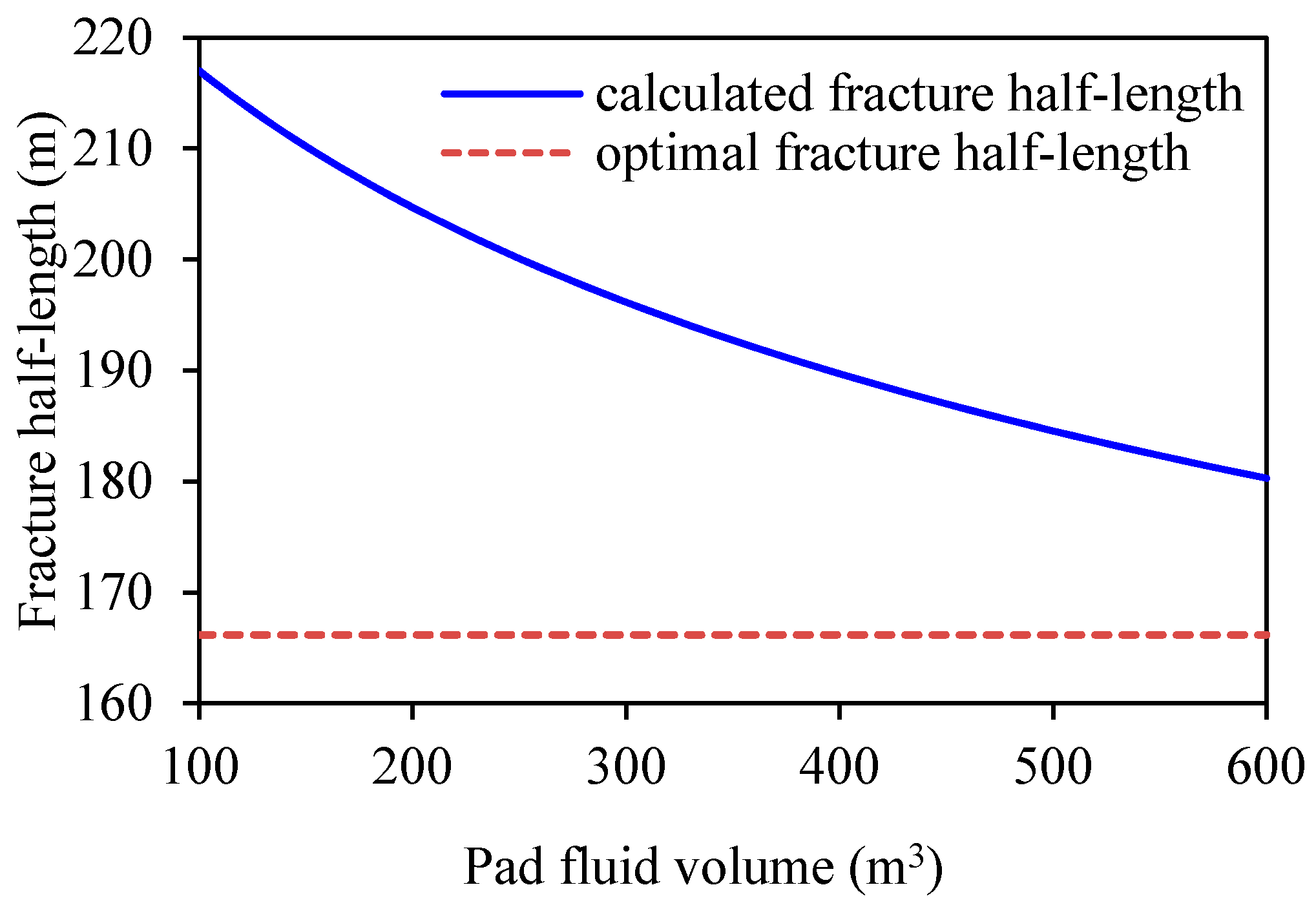
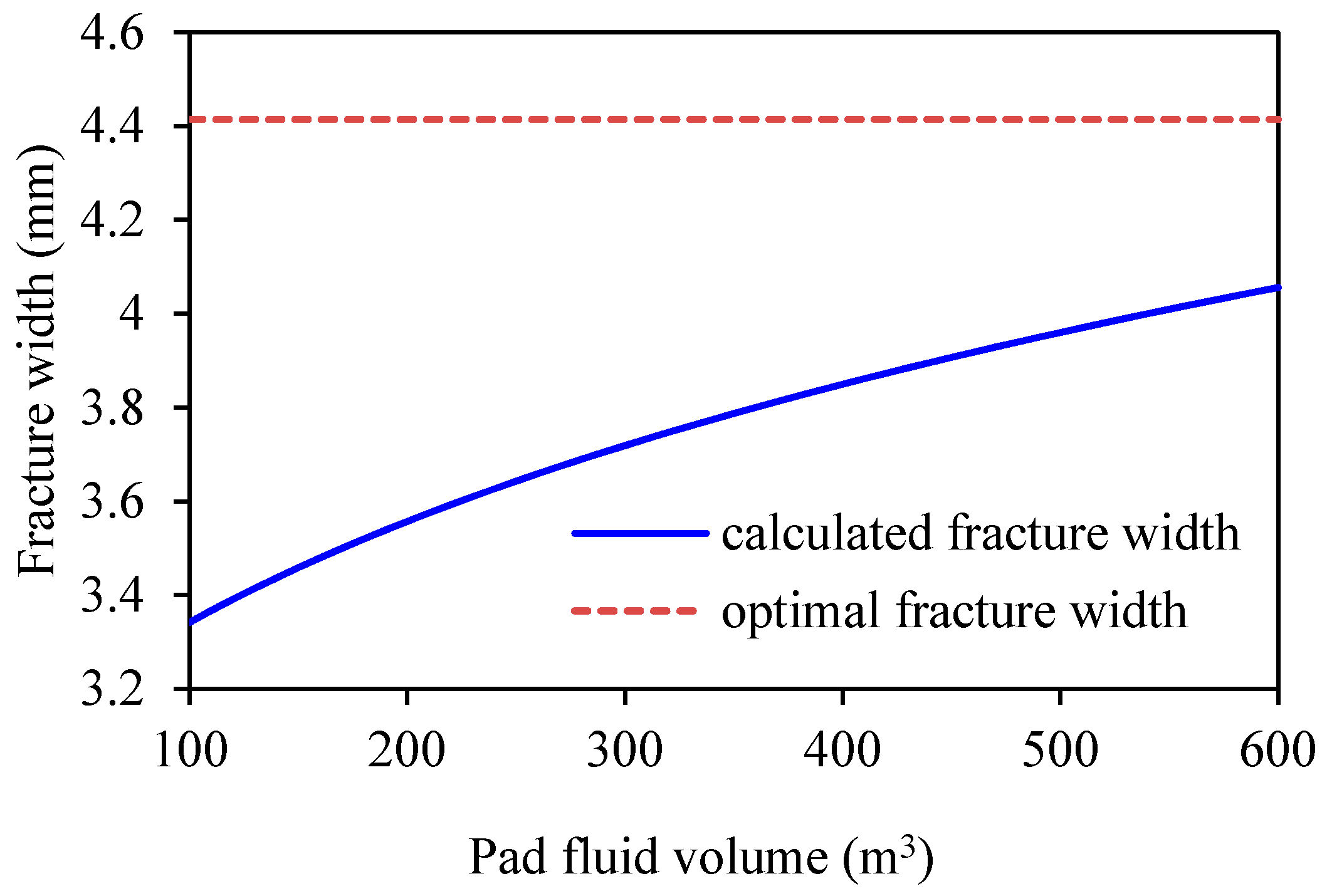
3.2. Optimization Method and Results
3.3. General Discussion
3.3.1. Injection Rate
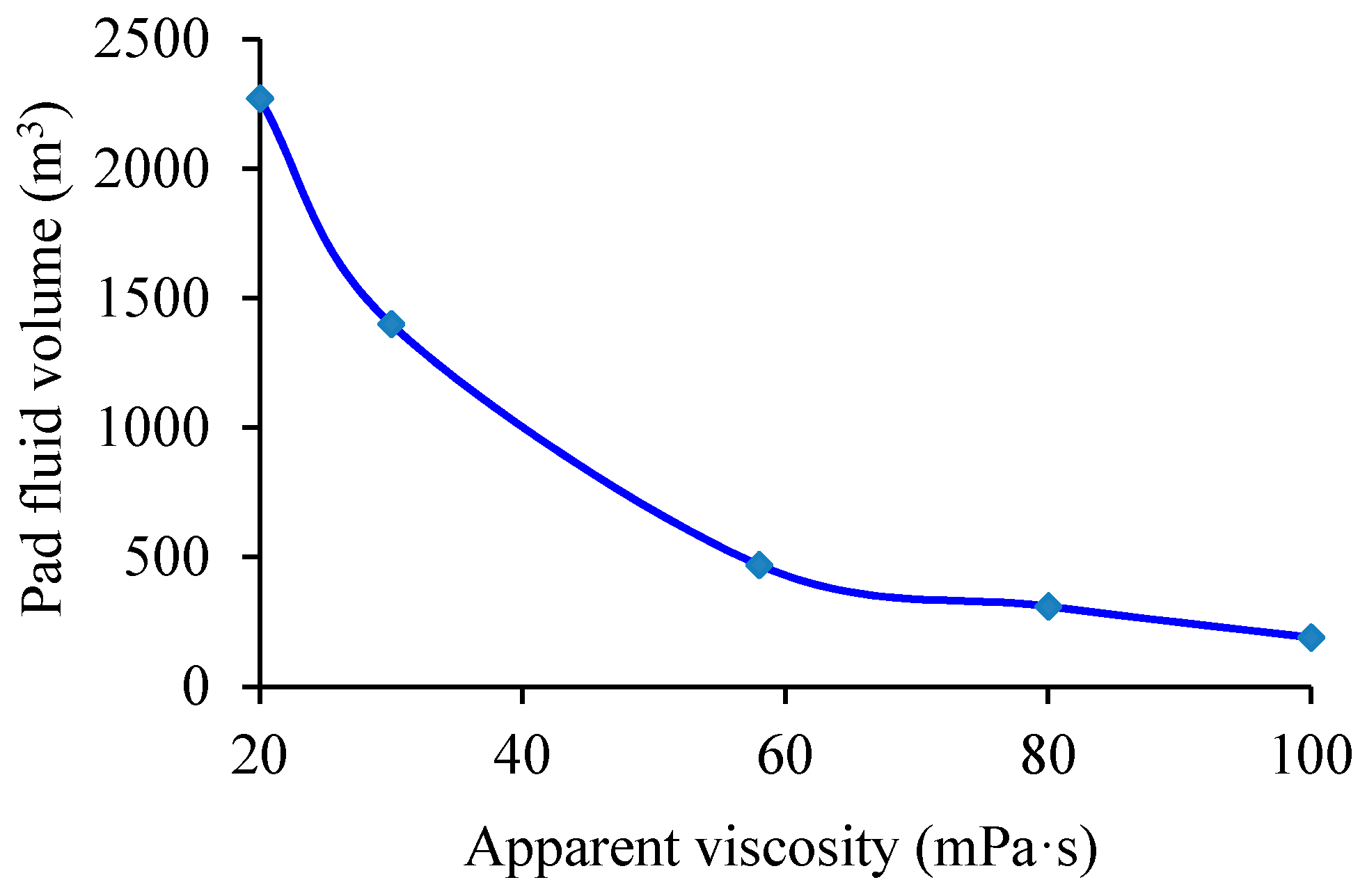
3.3.2. Fluid’s Apparent Viscosity
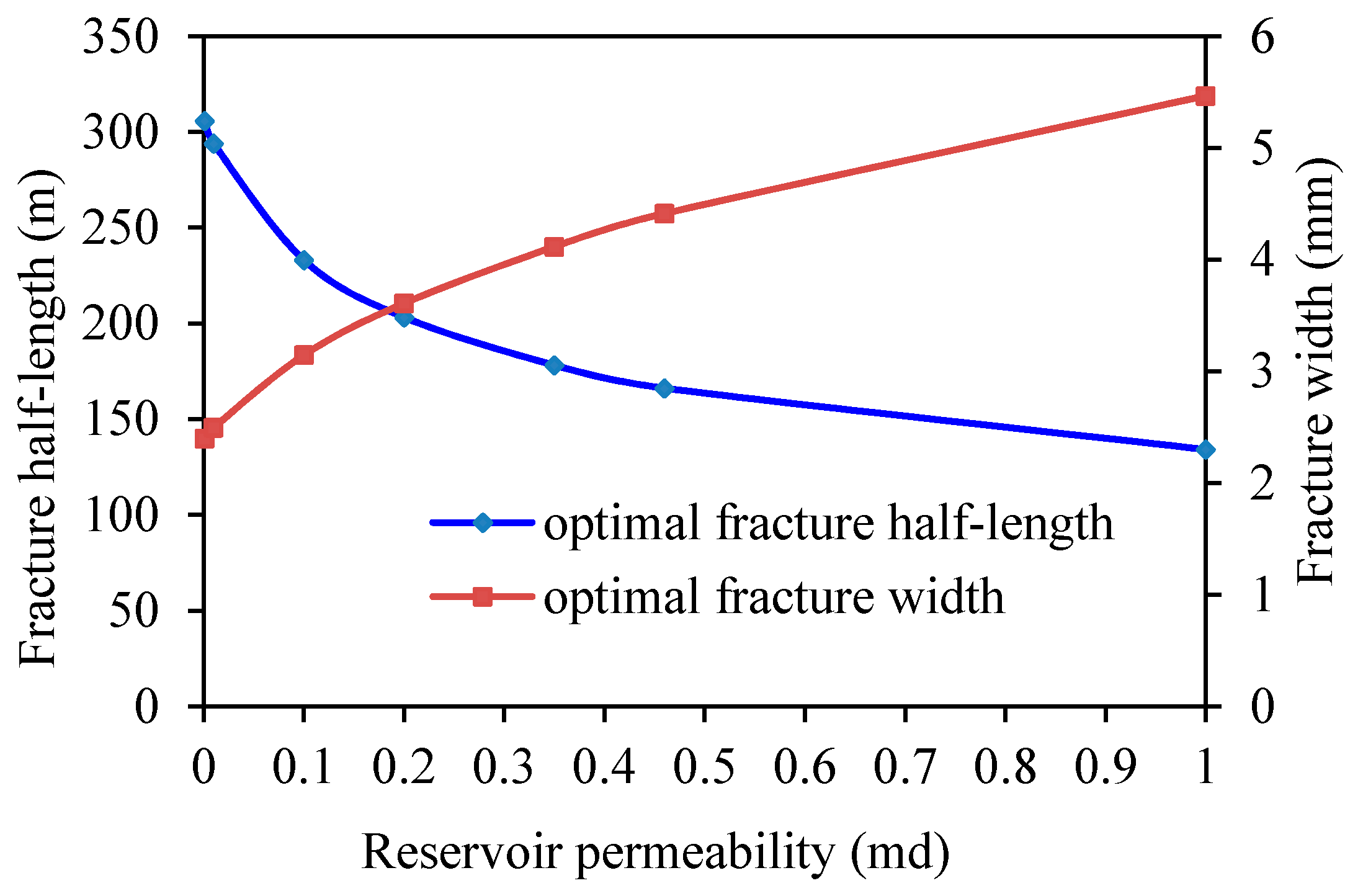
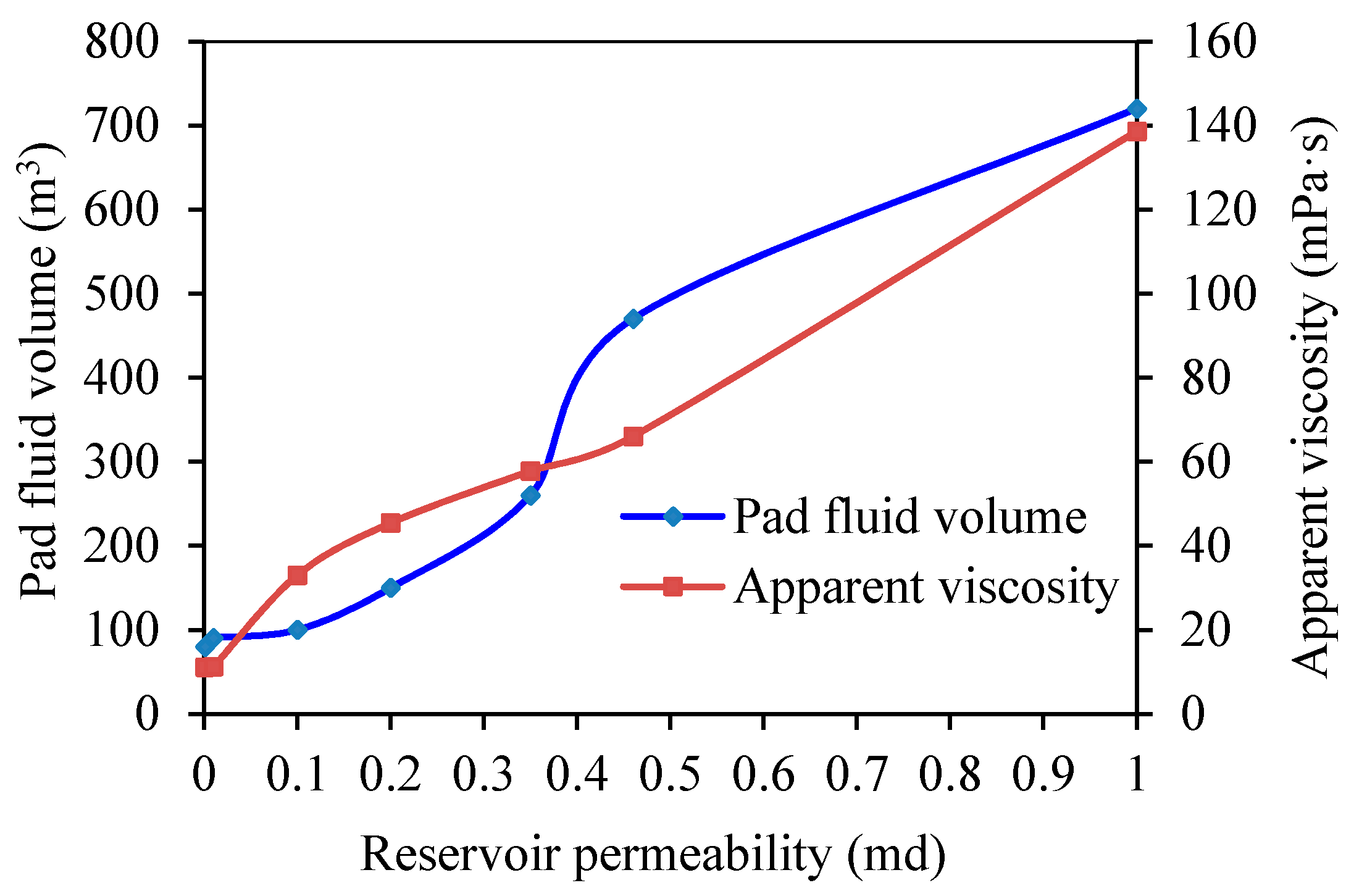
3.3.3. Reservoir Permeability
3.4. Special Discussion
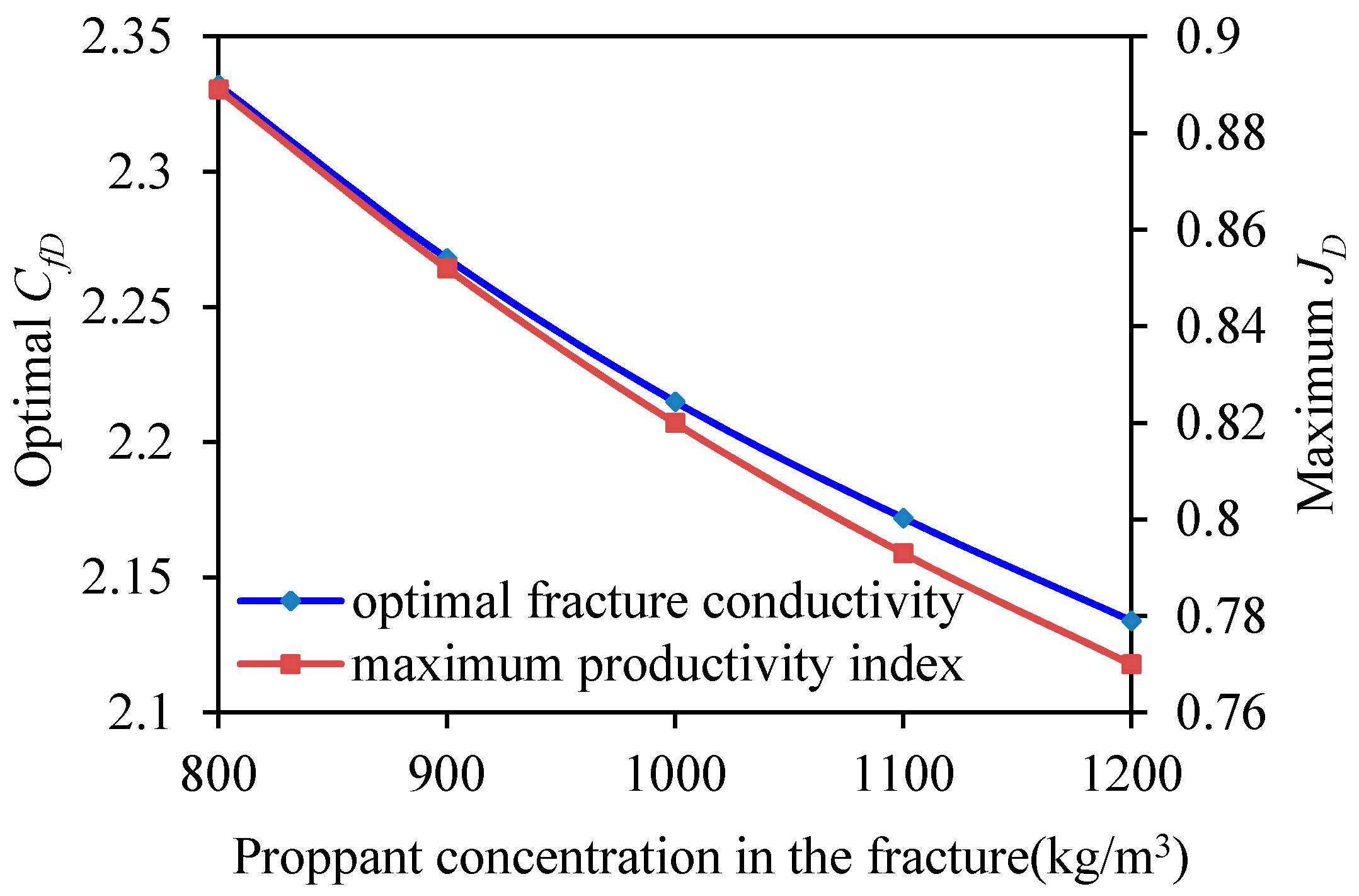
3.4.1. Desired Proppant Concentration in the Fracture
3.4.2. Injected Proppant Volume on the Ground
4. Conclusions
- (1)
- Through the semi-analytical fracture propagation model and the treatment optimization method, the desired proppant concentration in the fracture can be achieved by optimizing the proppant pumping curve index b. The optimal fracture half-length can be achieved by optimizing parameters such as pad fluid volume, injection rate, and fluid rheological parameters. Once both the desired proppant concentration and optimal fracture half-length are achieved, the optimal fracture width is also achieved naturally.
- (2)
- In order to obtain the optimal fracture dimensions, the treatment parameters are not unique. The optimal treatment parameters can be determined according to both the actual treatment conditions in the field and the optimization procedure. The prior empirical knowledge about the actual fracturing treatment can also make the optimization more efficiently.
- (3)
- For the sake of rapid calculation, a 2D fracture propagation model was used. This optimization method can provide complete and reasonable fracturing treatment parameters that meet the basic requirements of field designs and provide a reference for finer fracturing simulations and designs if necessary.
Author Contributions
Acknowledgments
Conflicts of Interest
Appendix A. Unified Fracture Design (UFD) Method
| 0.1 | 0.2 | 0.25 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1.0 | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.025 | 2.36 | 5.38 | 9.00 | 16.17 | 21.84 | 25.80 | 28.36 | 29.89 | 30.66 | 30.88 |
- (1)
- When : align
- (2)
- When :
| 1 | 0.7 | 0.5 | 0.25 | 0.2 | 0.1 | |
| a | 17.2 | 17.4 | 21.4 | 38.3 | 35 | 30.6 |
| b | 54.5 | 55.5 | 54.3 | 46 | 59 | 89.6 |
| c | 52.5 | 53.3 | 56.3 | 71.1 | 70 | 70.2 |
| d | 16.9 | 16.9 | 16.9 | 15.84 | 16.3 | 17.8 |
| a’ | 10 | |||||
| b’ | 36 | |||||
| c’ | 33 | |||||
References
- Hareland, G.; Rampersad, P.; Dharaphop, J.; Sasnanand, S. Hydraulic Fracturing Design Optimization. In Proceedings of the 1993 Eastern Regional Conference & Exhibition, Pittsburgh, PA, USA, 2–4 November 1993. [Google Scholar]
- Lynk, J.M.; Papandrea, R.; Collamore, A.; Quinn, T.; Cazeneuve, E.; Centurion, S. Hydraulic fracture completion optimization in Fayetteville shale: Case study. Int. J. Geomech. 2016, 17, 04016053. [Google Scholar] [CrossRef]
- Roussel, N.P.; Sharma, M.M. Optimizing Fracture Spacing and Sequencing in Horizontal-Well Fracturing. In Proceedings of the 2010 SPE International Symposium and Exhibition on Formation Damage Control, Lafayette, LA, USA, 10–12 February 2010. [Google Scholar]
- Jamiolahmady, M.; Sohrabi, M.; Mahdiyar, H. Optimization of Hydraulic Fracture Geometry. In Proceedings of the 2009 SPE Offshore Europe Oil & Gas Conference & Exhibition, Aberdeen, UK, 8–11 September 2009. [Google Scholar]
- Manrique, J.F.; Poe, B.D. Evaluation and Optimization of Low Conductivity Fractures. In Proceedings of the SPE Hydraulic Fracturing Technology Conference, College Station, TX, USA, 29–31 January 2007. [Google Scholar]
- Bruce, R.M.; Lucas, W.B.; Henry, R.J.; Michael, G.L. Optimization of Multiple Transverse Hydraulic Fractures in Horizontal Wellbores. In Proceedings of the SPE Unconventional Gas Conference, Pittsburgh, PA, USA, 23–25 February 2010. [Google Scholar]
- Zeng, F.; Zhao, G. The optimal hydraulic fracture geometry under non-Darcy flow effects. J. Petrol. Sci. Eng. 2010, 72, 143–157. [Google Scholar] [CrossRef]
- Siddhamshetty, P.; Yang, S.; Kwon, S.I. Modeling of hydraulic fracturing and designing of online pumping schedules to achieve uniform proppant concentration in conventional oil reservoirs. Comput. Chem. Eng. 2018, 114, 306–317. [Google Scholar] [CrossRef]
- Yang, S.; Siddhamshetty, P.; Kwon, S.I. Optimal pumping schedule design to achieve a uniform proppant concentration level in hydraulic fracturing. Comput. Chem. Eng. 2017, 101, 138–147. [Google Scholar] [CrossRef]
- Rahman, M.M.; Rahman, M.K.; Rahman, S.S. Optimizing treatment parameters for enhanced hydrocarbon production by hydraulic fracturing. J. Can. Pet. Technol. 2003, 42, 38–46. [Google Scholar] [CrossRef]
- Poulsen, D.K.; Soliman, M.Y. A Procedure for Optimal Hydraulic Fracturing Treatment Design. In Proceedings of the SPE Eastern Regional Meeting, Columbus, OH, USA, 12–14 November 1986. [Google Scholar]
- Soliman, M.Y.; Pongratz, R.; Rylance, M.; Prather, D. Fracture Treatment Optimization for Horizontal Well Completion. In Proceedings of the 2006 SPE Russian Oil and Gas Technical Conference and Exhibition, Moscow, Russia, 3–6 October 2006. [Google Scholar]
- Lopez-Hernandez, H.; Valkó, P.P.; Tai, T.P. Optimum Fracture Treatment Design Minimizes the Impact of Non-Darcy Flow Effects. In Proceedings of the SPE Annual Technical Conference and Exhibition, Houston, TX, USA, 26–29 September 2004. [Google Scholar]
- Kong, B.; Fathi, E.; Ameri, S. Coupled 3-D numerical simulation of proppant distribution and hydraulic fracturing performance optimization in Marcellus shale reservoirs. Int. J. Coal Geol. 2015, 147–148, 35–45. [Google Scholar] [CrossRef]
- Mahdiyar, H.; Jamiolahmady, M. Optimization of hydraulic fracture geometry in gas condensate reservoirs. Fuel 2014, 119, 27–37. [Google Scholar] [CrossRef]
- Wang, X.; Wang, Z.; Zeng, Q.; Yang, G.; Chen, T.; Guo, X. Non-Darcy effect on fracture parameters optimization in fractured CBM horizontal well. J. Nat. Gas Sci. Eng. 2015, 27, 1438–1445. [Google Scholar] [CrossRef]
- Masoomi, R.; Viktorovich, D.S. Simulation and optimization of the hydraulic fracturing operation in a heavy oil reservoir in southern Iran. J. Eng. Sci. Technol. 2017, 12, 241–255. [Google Scholar]
- Wang, J.; Jia, A. A general productivity model for optimization of multiple fractures with heterogeneous properties. J. Nat. Gas Sci. Eng. 2014, 21, 608–624. [Google Scholar] [CrossRef]
- Zeng, F.H.; Ke, Y.B.; Guo, J.C. An optimal fracture geometry design method of fractured horizontal wells in heterogeneous tight gas reservoirs. Sci. China Technol. Sci. 2016, 59, 241–251. [Google Scholar] [CrossRef]
- Atefeh, J.; Behnam, J. Optimization of hydraulic fracturing design under spatially variable shale fracability. J. Pet. Sci. Eng. 2016, 138, 174–188. [Google Scholar]
- Zhang, Z.; Li, X.; Yuan, W.; He, J.; Li, G.; Wu, Y. Numerical analysis on the optimization of hydraulic fracture networks. Energies 2015, 8, 12061–12079. [Google Scholar] [CrossRef]
- Zeng, F.H.; Guo, J.C. Optimized design and use of induced complex fractures in horizontal wellbores of tight gas reservoirs. Rock Mech. Rock Eng. 2016, 49, 1411–1423. [Google Scholar] [CrossRef]
- Chen, M.; Sun, Y.; Fu, P.; Charles, R.; Lu, Z.; Charles, H.T.; Thomas, A.B. Surrogate-based optimization of hydraulic fracturing in pre-existing fracture networks. Comput. Geosci. 2013, 58, 69–79. [Google Scholar] [CrossRef] [Green Version]
- Balen, R.M.; Mens, H.Z.; Economides, M.J. Applications of the Net Present Value (NPV) in the Optimization of Hydraulic Fractures. In Proceedings of the SPE Eastern Regional Meeting, Charleston, WV, USA, 1–4 November 1988. [Google Scholar]
- Kim, H.; Querin, O.M.; Steven, G.P. On the development of structural optimization and its relevance in engineering design. Des. Stud. 2002, 23, 85–102. [Google Scholar] [CrossRef]
- Hariharan, K.; Balaji, C. Material optimization: A case study using sheet metal-forming analysis. J. Mater. Process. Technol. 2009, 209, 324–331. [Google Scholar] [CrossRef]
- Chisari, C.; Bedon, C. Multi-objective optimization of FRP jackets for improving the seismic response of reinforced concrete frames. Am. J. Eng. Appl. Sci. 2016, 9, 669–679. [Google Scholar] [CrossRef]
- Rahman, M.M.; Rahman, M.K.; Rahman, S.S. An integrated model for multi-objective design optimization of hydraulic fracturing. J. Pet. Sci. Eng. 2001, 31, 41–62. [Google Scholar] [CrossRef]
- Yang, C.; Vyas, A.; Datta-Gupta, A.; Ley, S.B.; Biswas, P. Rapid multistage hydraulic fracture design and optimization in unconventional reservoirs using a novel Fast Marching Method. J. Pet. Sci. Eng. 2017, 156, 91–101. [Google Scholar] [CrossRef]
- Lee, S.; Min, B.; Wheeler, M.F. Optimal design of hydraulic fracturing in porous media using the phase field fracture model coupled with genetic algorithm. Comput. Geosci. 2018, 3, 833–849. [Google Scholar] [CrossRef]
- Mohaghegh, S.; Balanb, B.; Platon, V.; Ameri, S. Hydraulic fracture design and optimization of gas storage wells. J. Pet. Sci. Eng. 1999, 23, 161–171. [Google Scholar] [CrossRef]
- Shahab, M.; Andrei, P.; Sam, A. Intelligent Systems Can Design Optimum Fracturing Jobs. In Proceedings of the 1999 SPE Eastern Regional Conference and Exhibition, Charleston, WV, USA, 21–22 October 1999. [Google Scholar]
- Gorucu, S.E.; Ertekin, T. Optimization of the Design of Transverse Hydraulic Fractures in Horizontal Wells Placed in Dual Porosity Tight Gas Reservoirs. In Proceedings of the SPE Middle East Unconventional Gas Conference and Exhibition, Muscat, Oman, 31 January–2 February 2011. [Google Scholar]
- Rueda, J.I. Using a Mixed Integer Linear Programming Technique to Optimize a Fracture Treatment Design. In Proceedings of the 1994 SPE Eastern Regional Conference and Exhibition, Charleston, WV, USA, 8–10 November 1994. [Google Scholar]
- Li, J.C.; Gong, B.; Wang, H.G. Mixed integer simulation optimization for optimal hydraulic fracturing and production of shale gas fields. Eng. Optim. 2015, 48, 1378–1400. [Google Scholar] [CrossRef]
- Queipo, N.V.; Verde, A.J.; Canelón, J.; Pintos, S. Efficient global optimization for hydraulic fracturing treatment design. J. Pet. Sci. Eng. 2002, 35, 151–166. [Google Scholar] [CrossRef]
- Wangen, M. Finite element modeling of hydraulic fracturing in 3D. Comput. Geosci. 2013, 17, 647–659. [Google Scholar] [CrossRef] [Green Version]
- Gordeliy, E.; Peirce, A. Implicit level set schemes for modeling hydraulic fractures using the XFEM. Comput. Methods Appl. Mech. Eng. 2013, 266, 125–143. [Google Scholar] [CrossRef]
- Li, L.; Xia, Y.; Huang, B.; Zhang, L.; Li, M.; Li, A. The behaviour of fracture growth in Sedimentary rocks: A numerical study based on hydraulic fracturing processes. Energies 2016, 9, 169. [Google Scholar] [CrossRef]
- Wang, J.; Li, H.; Wang, Y.; Li, Y.; Jiang, B.; Luo, W. A new model to predict productivity of multiple-fractured horizontal well in naturally fractured reservoirs. Math. Probl. Eng. 2015, 2015, 892594. [Google Scholar] [CrossRef]
- Liu, Q.; Chen, Y.; Wang, W.; Liu, H.; Hu, X.; Xie, Y. A productivity prediction model for multiple fractured horizontal wells in shale gas reservoirs. J. Nat. Gas Sci. Eng. 2017, 42, 252–261. [Google Scholar] [CrossRef]
- Medeiros, F.; Ozkan, E.; Kazemi, H. Productivity and Drainage Area of Fractured Horizontal Wells in Tight Gas Reservoirs. In Proceedings of the 2007 SPE Rocky Mountain Oil & Gas Technology Symposium, Denver, CO, USA, 16–18 April 2007. [Google Scholar]
- Luo, W.; Wang, X.; Feng, Y.; Tang, C.; Zhou, Y. Productivity analysis for a vertically fractured well under non-Darcy flow condition. J. Pet. Sci. Eng. 2016, 146, 714–725. [Google Scholar] [CrossRef] [Green Version]
- Nordgren, R.P. Propagation of a vertical hydraulic fracture. Soc. Pet. Eng. J. 1972, 12, 306–314. [Google Scholar] [CrossRef]
- Valkó, P.P.; Economides, M.J. Hydraulic Fracture Mechanics; John Wiley & Sons: New York, NY, USA, 1995. [Google Scholar]
- Kovalyshen, Y.; Detournay, E. A reexamination of the classical PKN model of hydraulic fracture. Transp. Porous Media 2010, 81, 317–339. [Google Scholar] [CrossRef]
- Palmer, I.D.; Carroll, H.B. Numerical Solution for Height and Elongated Hydraulic Fractures. In Proceedings of the 1983 SPE/DOE Symposium on Low Permeability, Denver, CO, USA, 14–16 March 1983. [Google Scholar]
- Rahman, M.M.; Rahman, M.K. A Review of Hydraulic Fracture Models and Development of an Improved Pseudo-3D Model for Stimulating Tight Oil/Gas Sand. Energy Sources 2010, 32, 1416–1436. [Google Scholar] [CrossRef]
- Pitakbunkate, T.; Yang, M.; Valkó, P.P.; Economides, M.J. Hydraulic Fracture Optimization with a P-3D Model. In Proceedings of the SPE Production and Operations Symposium, Oklahoma City, OK, USA, 27–29 March 2011. [Google Scholar]
- Economides, M.J.; Demarchos, A.S. Benefits of a p-3D over a 2D Model for Unified Fracture Design. In Proceedings of the 2008 SPE International Symposium and Exhibition on Formation Damage Control, Lafayette, LA, USA, 13–15 February 2008. [Google Scholar]
- Meng, H.Z.; Brown, K.E. Coupling of Production Forecasting, Fracture Geometry Requirements and Treatment Scheduling in the Optimum Hydraulic Fracture Design. In Proceedings of the SPE/DOE Low Permeability Reservoirs Symposium, Denver, CO, USA, 18–19 May 1987. [Google Scholar]
- Rahman, M.M. Constrained hydraulic fracture optimization improves recovery from low permeable oil reservoirs. Energy Sources Part A 2008, 30, 536–551. [Google Scholar] [CrossRef]
- Valkó, P.P.; Economides, M.J. Heavy Crude Production from Shallow Formations: Long Horizontal Wells Versus Horizontal Fractures. In Proceedings of the SPE International Conference on Horizontal Well Technology, Calgary, AB, Canada, 1–4 November 1998. [Google Scholar]
- Economides, M.J.; Oligeny, R.E.; Valkó, P.P. Unified Fracture Design; Orsa Press: Houston, TX, USA, 2002. [Google Scholar]
- Demarchos, A.S.; Chomatas, A.S.; Economides, M.J. Pushing the Limits in Hydraulic Fracture Design. In Proceedings of the SPE International Symposium and Exhibition on Formation Damage Control, Lafayette, LA, USA, 18–20 February 2004. [Google Scholar]
- Romero, D.J.; Valkó, P.P.; Economides, M.J. Optimization of the Productivity Index and the Fracture Geometry of a Stimulated Well with Fracture Face and Choke Skins. In Proceedings of the 2002 SPE International Symposium and Exhibition on Formation Damage Control, Lafayette, LA, USA, 20–21 February 2003. [Google Scholar]
- Daal, J.A.; Economides, M.J. Optimization of Hydraulically Fractured Wells in Irregularly Shaped Drainage Areas. In Proceedings of the 2006 SPE International Symposium and Exhibition on Formation Damage Control, Lafayette, LA, USA, 15–17 February 2006. [Google Scholar]
- Martin, A.N.; Economides, M.J. Best Practices for Candidate Selection, Design and Evaluation of Hydraulic Fracture Treatments. In Proceedings of the SPE Production and Operations Conference and Exhibition, Tunis, Tunisia, 8–10 June 2010. [Google Scholar]
- Bhattacharya, S.; Nikolaou, M.; Economides, M.J. Unified Fracture Design for very low permeability reservoirs. J. Nat. Gas Sci. Eng. 2012, 9, 184–195. [Google Scholar] [CrossRef]
- Mukherjee, H.; Economides, M.J. A parametric comparison of horizontal and vertical well performance. SPE Form. Eval. 1991, 6, 209–216. [Google Scholar] [CrossRef]
- Wei, Y.; Economides, M.J. Transverse Hydraulic Fractures from a Horizontal Well. In Proceedings of the SPE Annual Technical Conference and Exhibition, Dallas, TX, USA, 9–12 October 2005. [Google Scholar]
- Rahman, M.M.; Sarma, H.K.; Yu, H. Transverse Fracturing of Horizontal Well-A Unified Fracture Design to Stimulate Tight Gas Sands. In Proceedings of the SPE Middle East Unconventional Gas Conference and Exhibition, Muscat, Oman, 28–30 January 2013. [Google Scholar]
- Marongiu-Porcu, M.; Economides, M.J.; Holditch, A.S. Economic and physical optimization of hydraulic fracturing. J. Nat. Gas Sci. Eng. 2013, 14, 91–107. [Google Scholar] [CrossRef]
- Zhang, T.; Pang, W.; Du, J.; He, Y.; He, Q.; Liu, H.; Feng, X.; Song, B.; Ehlig-Economides, C.A. Actual and Optimal Hydraulic Fracture Design in a Tight Gas Reservoir. In Proceedings of the SPE Hydraulic Fracturing Technology Conference, Woodlands, TX, USA, 4–6 February 2014. [Google Scholar]
- Bhattacharya, S.; Nikolaou, M. Comprehensive optimization methodology for stimulation design of low-permeability unconventional gas reservoirs. SPE J. 2016, 21, 947–964. [Google Scholar] [CrossRef]

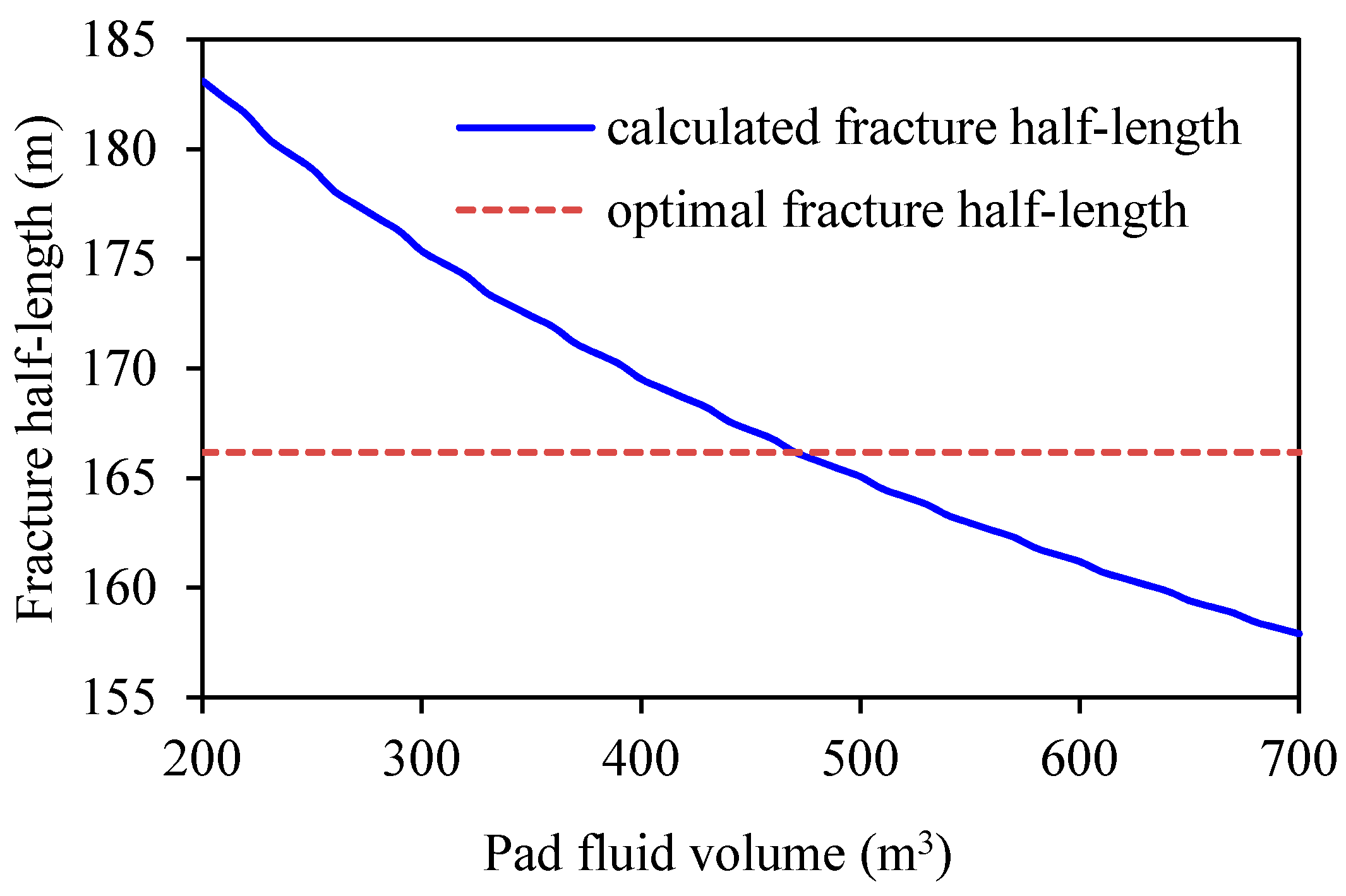

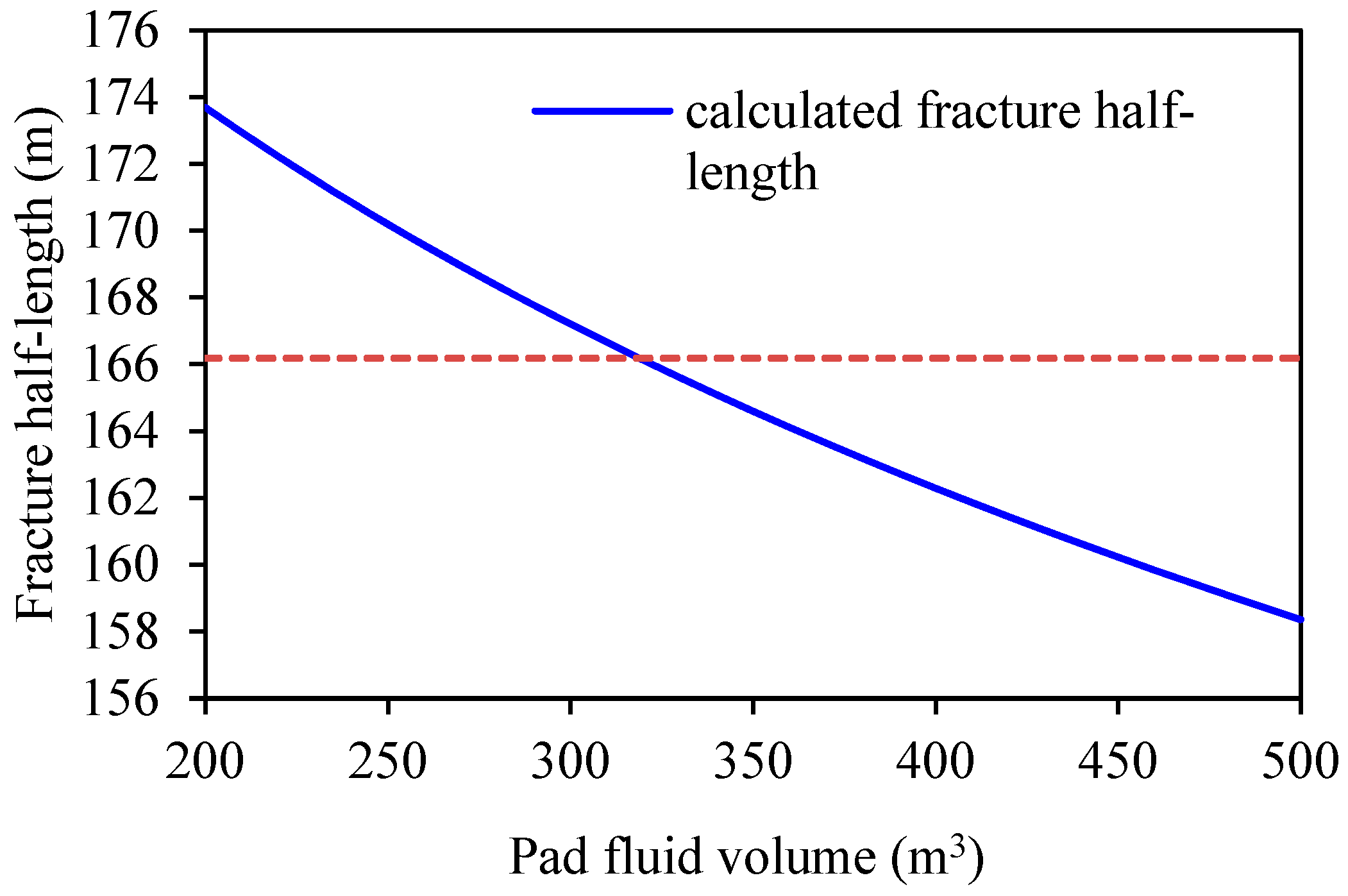
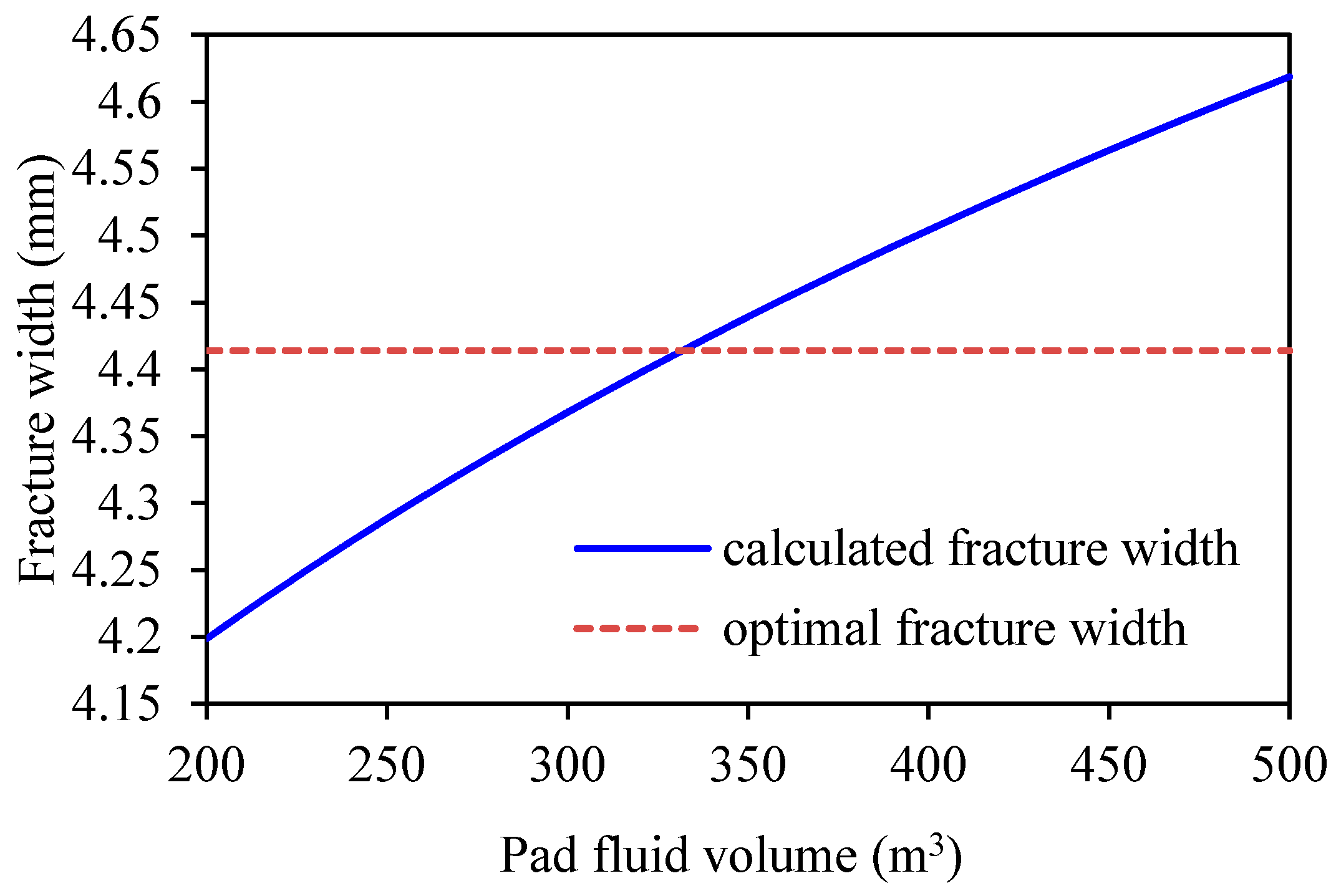
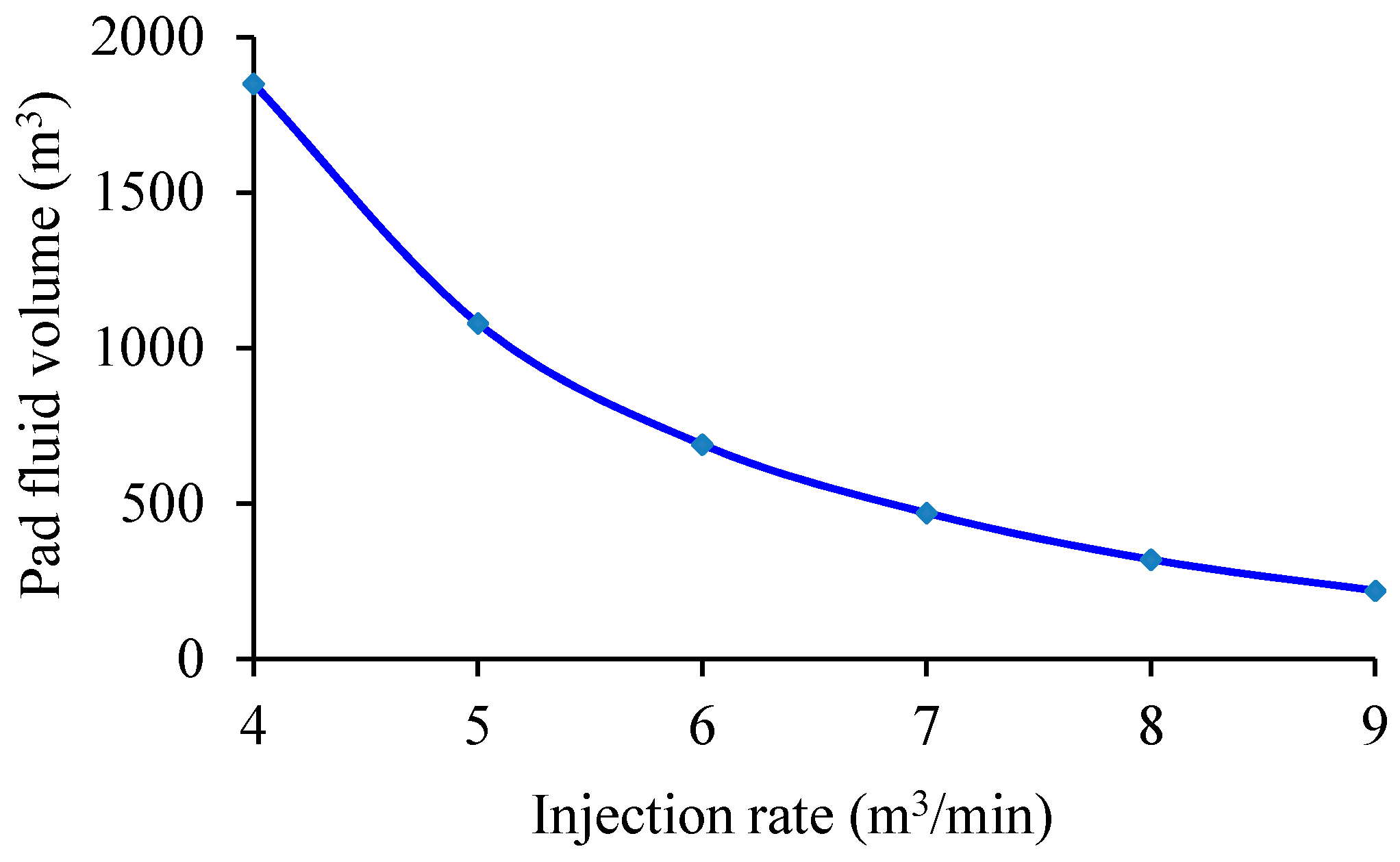




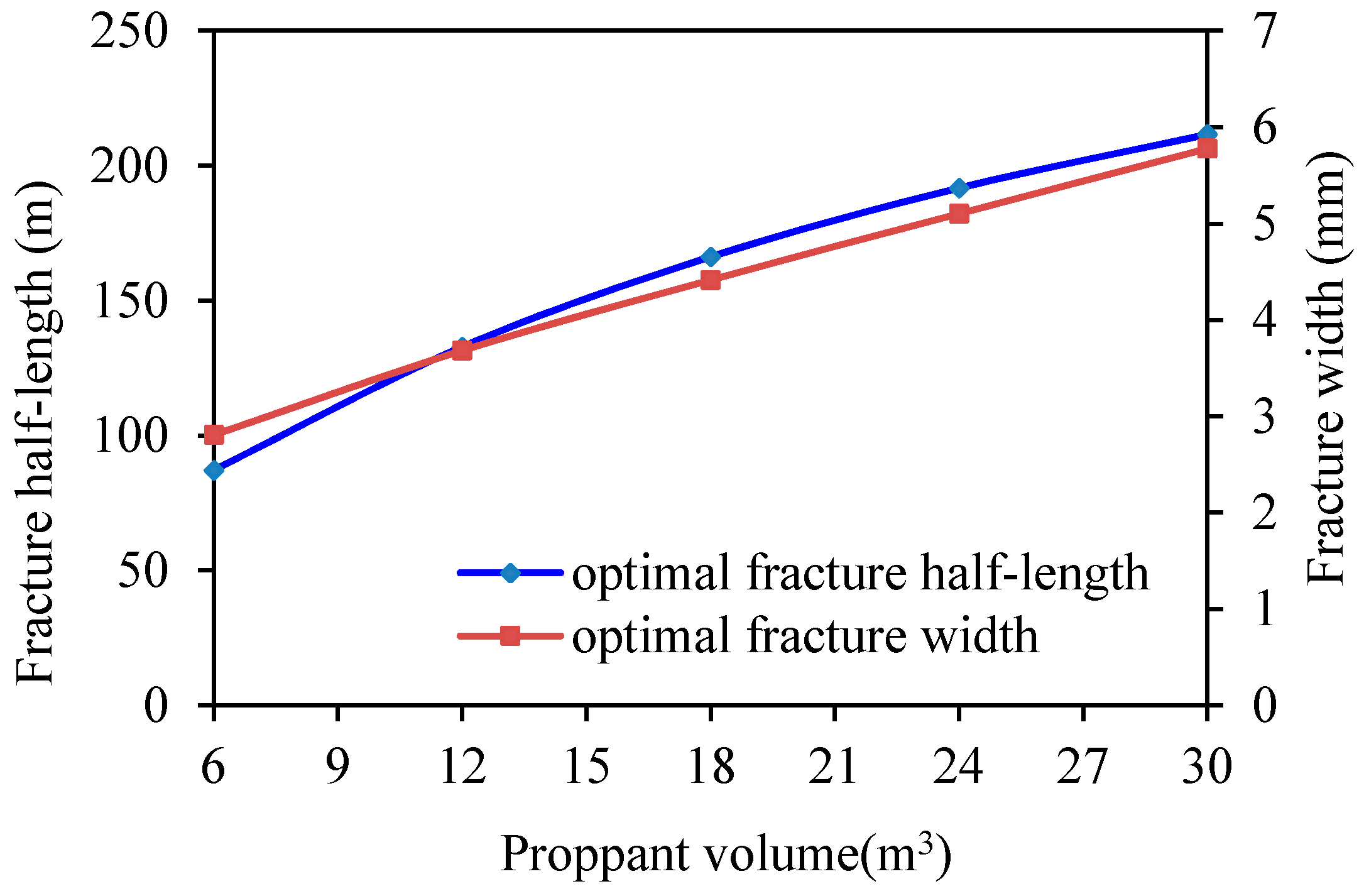


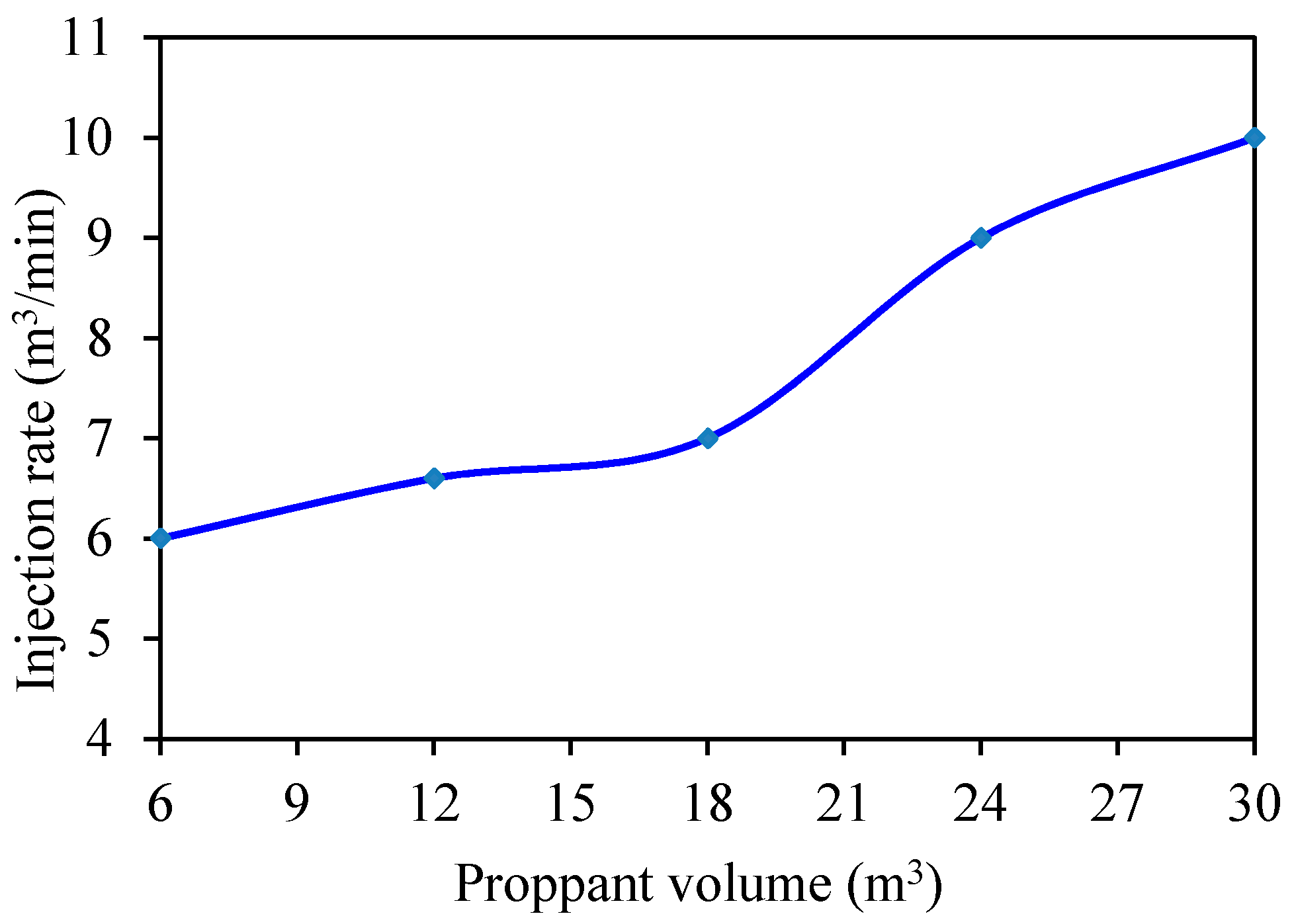
| Reservoir depth | 2611 m | Rock elastic modulus | 35 GPa |
| Horizontal segment length | 1000 m | Rock Poisson’s ratio | 0.3 |
| Reservoir net pay thickness | 20 m | Proppant apparent density | 1630 kg/m3 |
| Reservoir porosity | 1.39% | Proppant porosity | 38.7% |
| Reservoir average permeability | 0.46 md | Fluid-loss coefficient | 0.05 mm/min0.5 |
| Drainage area length | 1200 m | Fracturing segment number | 6 |
| Drainage area width | 600 m | - | - |
| Variable | Pad Fluid Volume (m3) | Proppant Pumping Curve Index b | Fluid Consistency Coefficient K (Pa·sn) | Fluid Flow Index n |
|---|---|---|---|---|
| Range | 100–800 | 0.5–0.8 | 0.1–0.7 | 0.1–0.6 |
| Search step length | 50 (10) | 0.01 | 0.05 | 0.05 |
| Preset Parameters | Fracture Optimization Results | Treatment Optimization Results | |||
|---|---|---|---|---|---|
| Proppant volume on the ground (m3) | 18 | Proppant number | 2.039 | Injection rate (m3/min) | 7 |
| Proppant mass on the ground (kg) | 29,340 | Maximum for single fracture | 0.82 | Pad fluid volume (m3) | 470 |
| Desired proppant concentration (kg/m3) | 1000.000 | Optimal | 2.215 | Sand adding curve index b | 0.63 |
| Calculated | 2.213 | Consistency coefficient K (Pa·sn) | 0.7 | ||
| Calculated proppant concentration (kg/m3) | 1001.062 | Optimal fracture half-length (m) | 166.180 | ||
| Calculated fracture half-length (m) | 166.184 | Flow index n | 0.6 | ||
| Fracture height (m) | 20 | Optimal fracture width (mm) | 4.414 | Apparent viscosity (mPa·s) | 58 |
| - | - | Calculated fracture width (mm) | 4.409 | - | - |
| - | - | Total error (%) | 0.109 | - | - |
| Pumping Stages | Desired Proppant Concentration (kg/m3) | ||||
|---|---|---|---|---|---|
| 800 | 900 | 1000 | 1100 | 1200 | |
| 1st stage sand ratio (%) | 5.976 | 7.67 | 9.443 | 11.626 | 14.313 |
| 2nd stage sand ratio (%) | 10.773 | 12.722 | 14.614 | 16.787 | 19.283 |
| 3rd stage sand ratio (%) | 15.205 | 17.105 | 18.867 | 20.812 | 22.956 |
| 4th stage sand ratio (%) | 19.417 | 21.102 | 22.616 | 24.239 | 25.979 |
| 5th stage sand ratio (%) | 23.473 | 24.835 | 26.03 | 27.283 | 28.595 |
| 6th stage sand ratio (%) | 27.408 | 28.37 | 29.198 | 30.050 | 30.927 |
| 7th stage sand ratio (%) | 31.245 | 31.749 | 32.176 | 32.609 | 33.047 |
| 8th stage sand ratio (%) | 35 | 35 | 35 | 35 | 35 |
| Proppant pumping curve coefficient a | 5.976 | 7.670 | 9.443 | 11.626 | 14.313 |
| Proppant pumping curve index b | 0.85 | 0.73 | 0.63 | 0.53 | 0.43 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ai, K.; Duan, L.; Gao, H.; Jia, G. Hydraulic Fracturing Treatment Optimization for Low Permeability Reservoirs Based on Unified Fracture Design. Energies 2018, 11, 1720. https://doi.org/10.3390/en11071720
Ai K, Duan L, Gao H, Jia G. Hydraulic Fracturing Treatment Optimization for Low Permeability Reservoirs Based on Unified Fracture Design. Energies. 2018; 11(7):1720. https://doi.org/10.3390/en11071720
Chicago/Turabian StyleAi, Kun, Longchen Duan, Hui Gao, and Guangliang Jia. 2018. "Hydraulic Fracturing Treatment Optimization for Low Permeability Reservoirs Based on Unified Fracture Design" Energies 11, no. 7: 1720. https://doi.org/10.3390/en11071720
APA StyleAi, K., Duan, L., Gao, H., & Jia, G. (2018). Hydraulic Fracturing Treatment Optimization for Low Permeability Reservoirs Based on Unified Fracture Design. Energies, 11(7), 1720. https://doi.org/10.3390/en11071720





