Economic Model Predictive Control with Nonlinear Constraint Relaxation for the Operational Management of Water Distribution Networks
Abstract
1. Introduction
2. Economic Model Predictive Control of WDNs
2.1. Control-Oriented Model of WDNs
2.2. Economic MPC of WDNs
- Economic: To minimize the operational costs while supplying enough water to satisfy all the demands with suitable pressure;
- Safety: To reserve suitable volumes of water in storage tanks for satisfying all the underlying uncertain demands.
3. Economic Model Predictive Control of WDNs with Nonlinear Constraint Relaxation
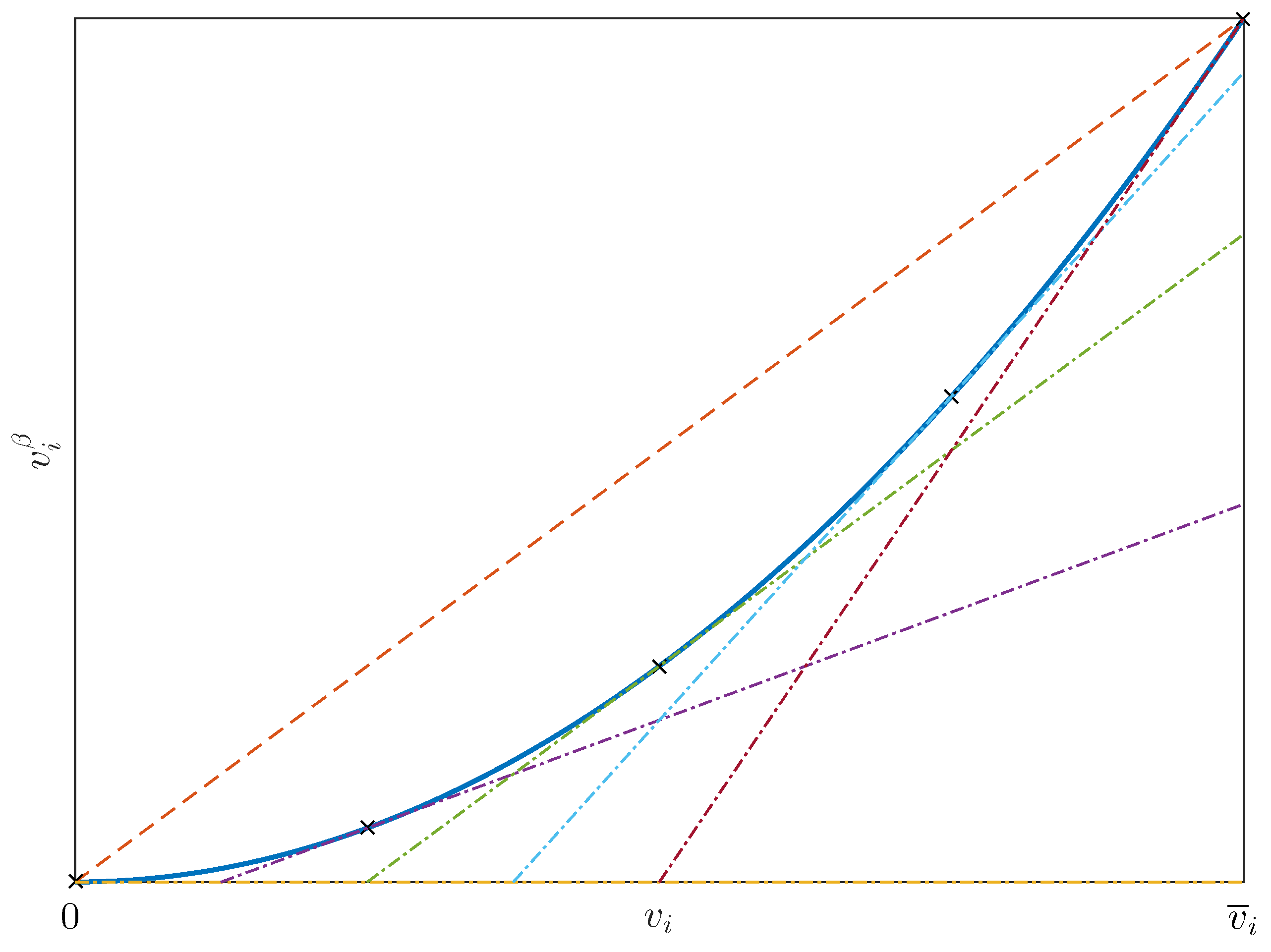
3.1. Nonlinear Constraint Relaxation for Unidirectional Pipes
3.2. Nonlinear Constraint Relaxation for Bidirectional Pipes
3.3. Economic MPC with Nonlinear Constraint Relaxation
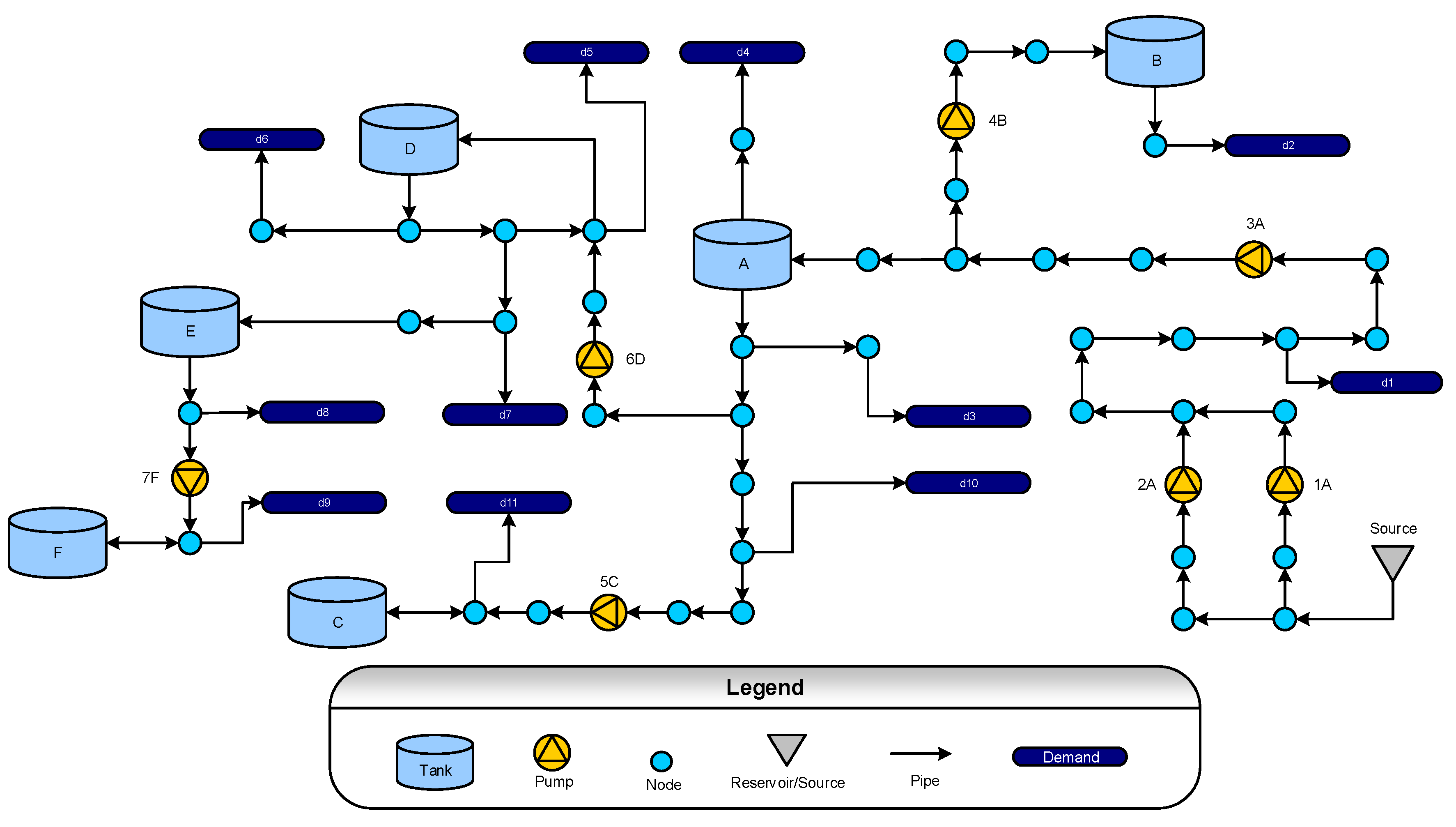
4. Application: the Richmond Water Distribution Network
4.1. System Description
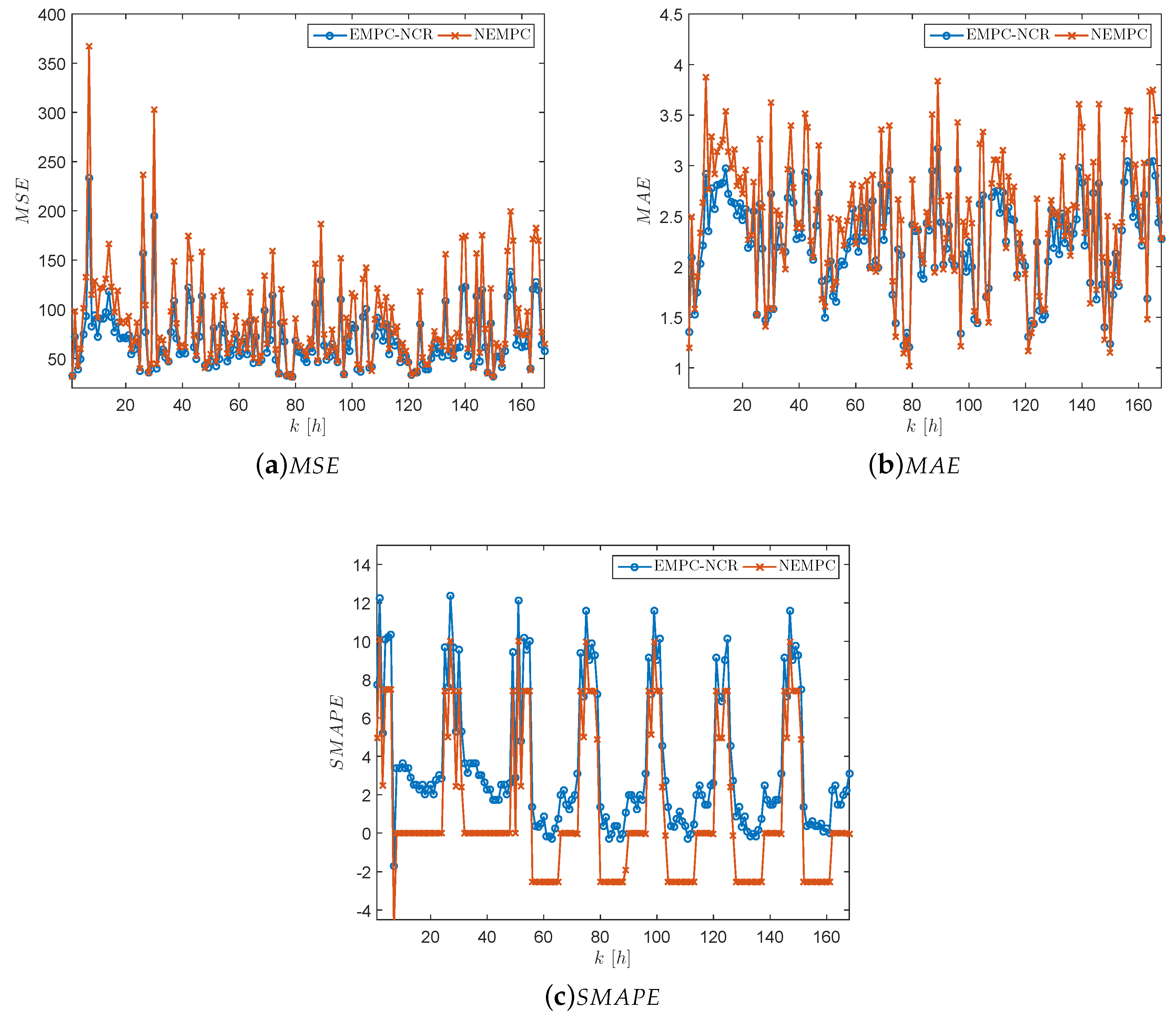
4.2. Key Performance Indicators
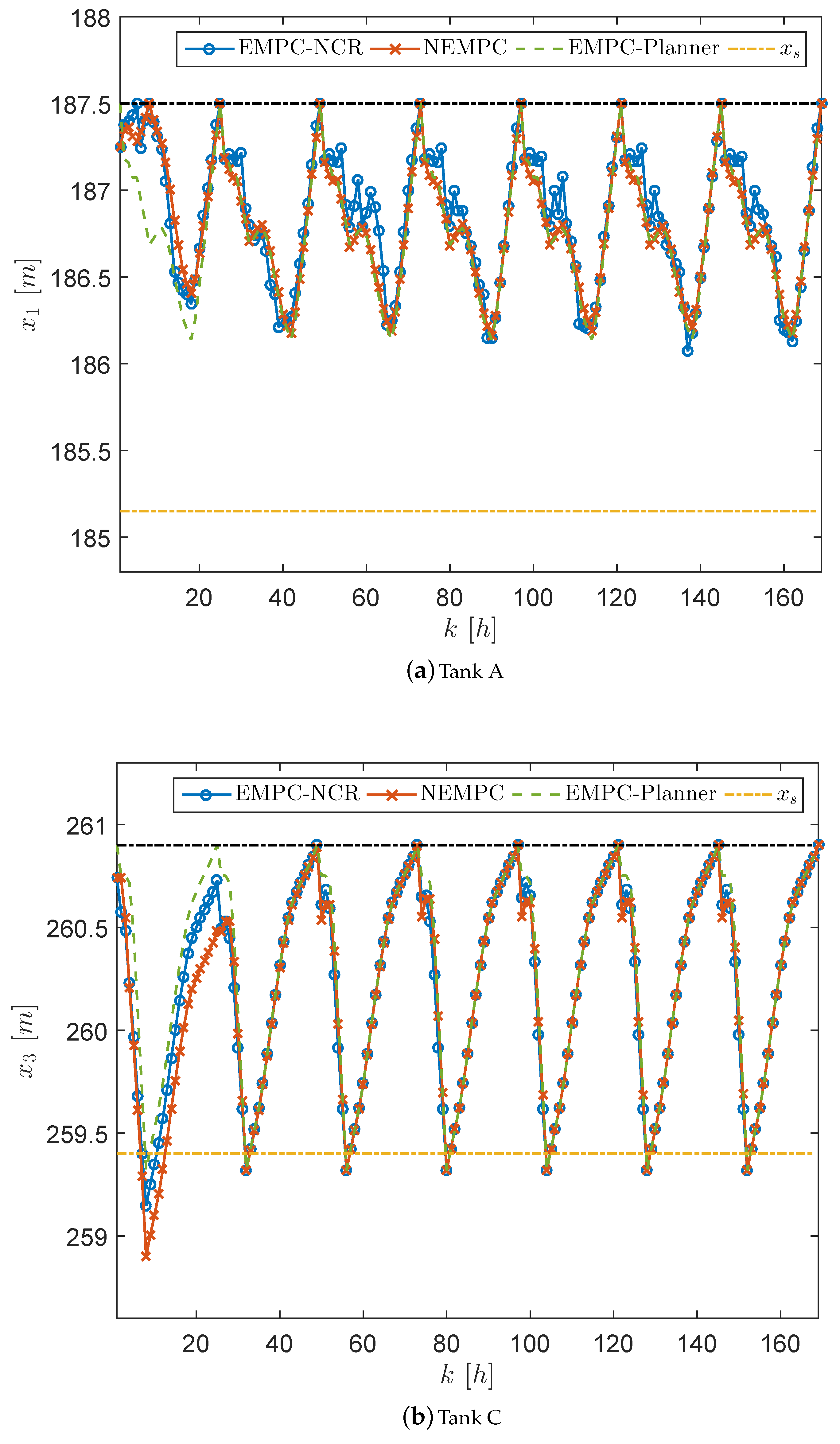
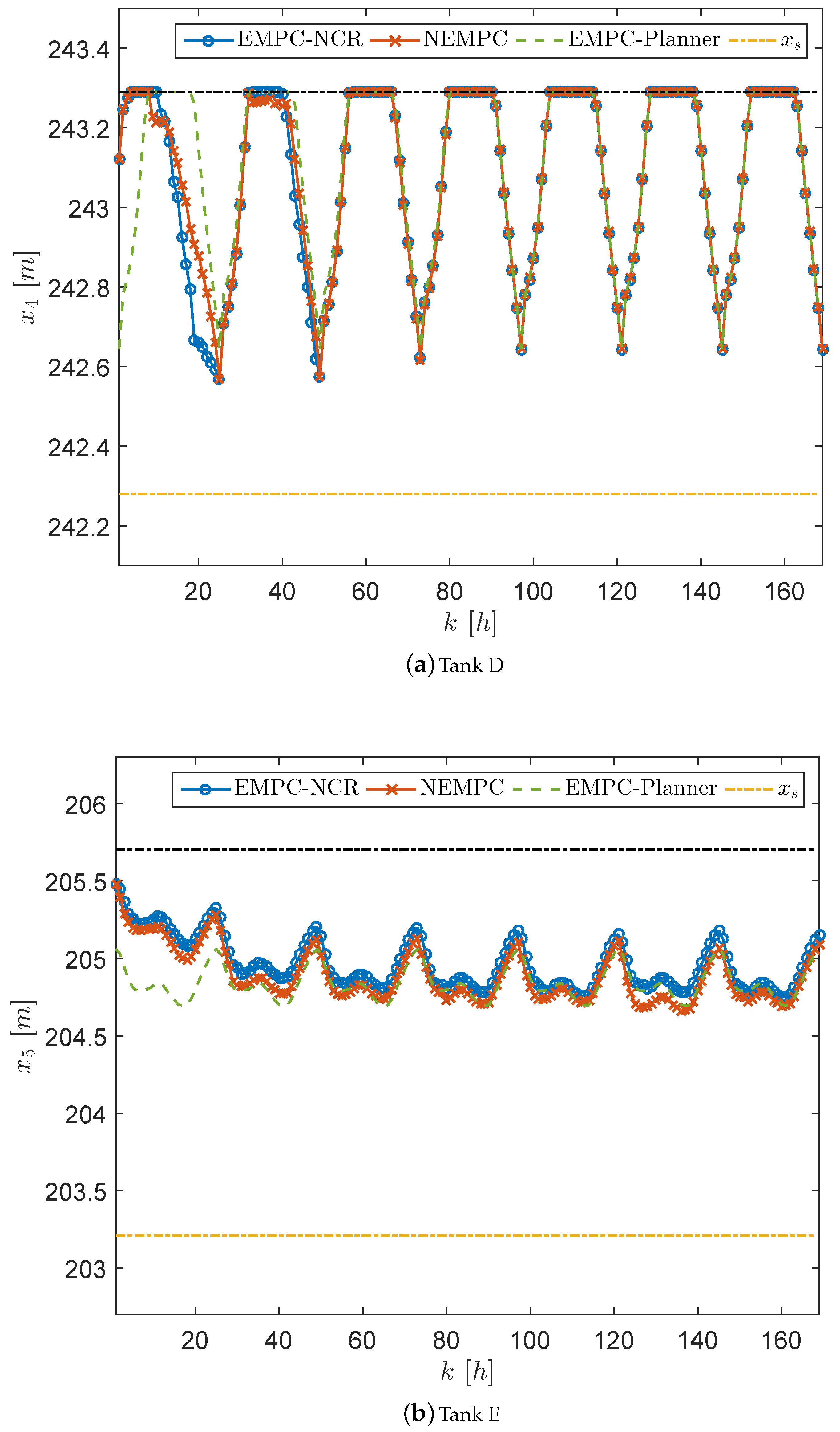
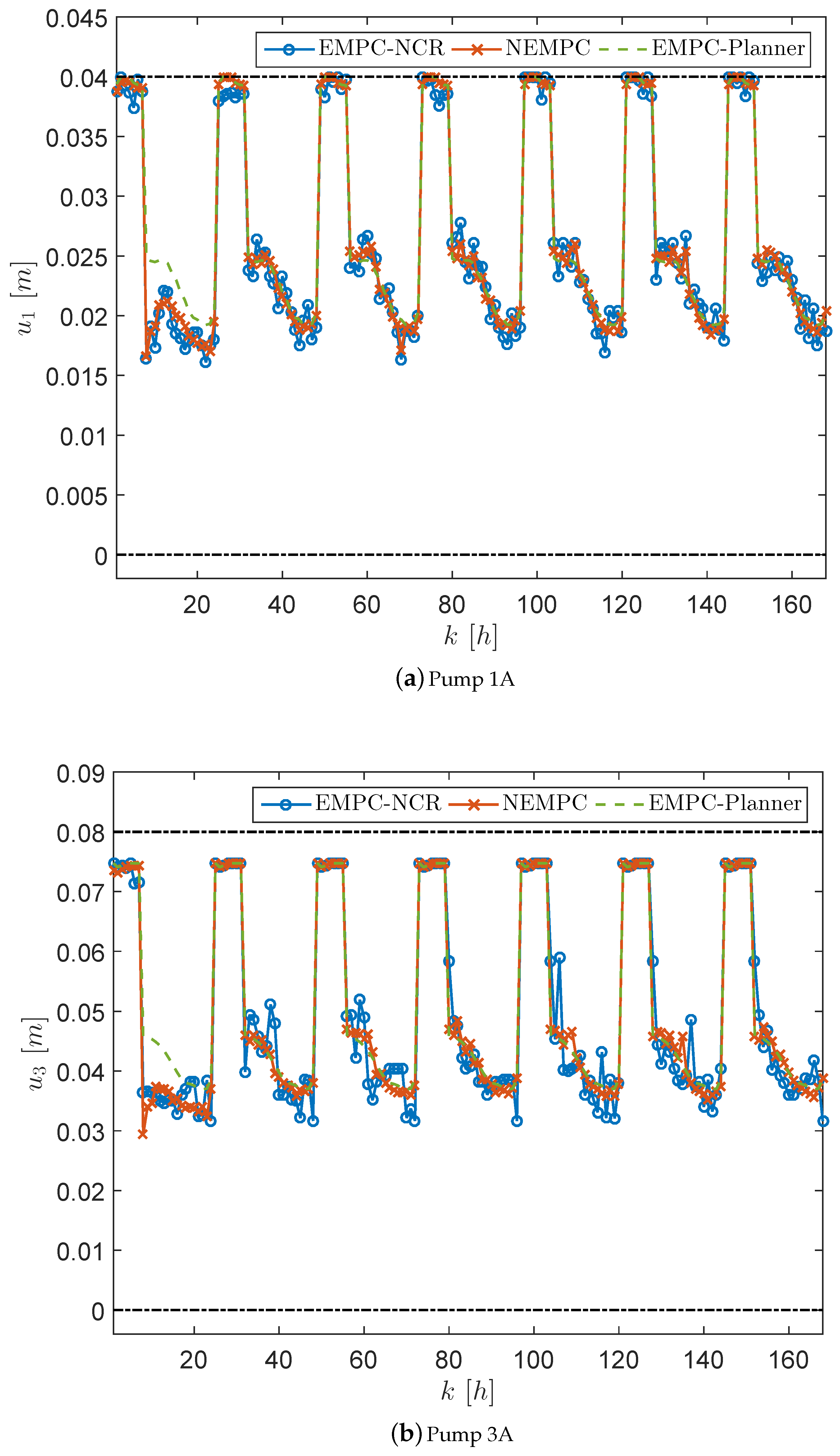
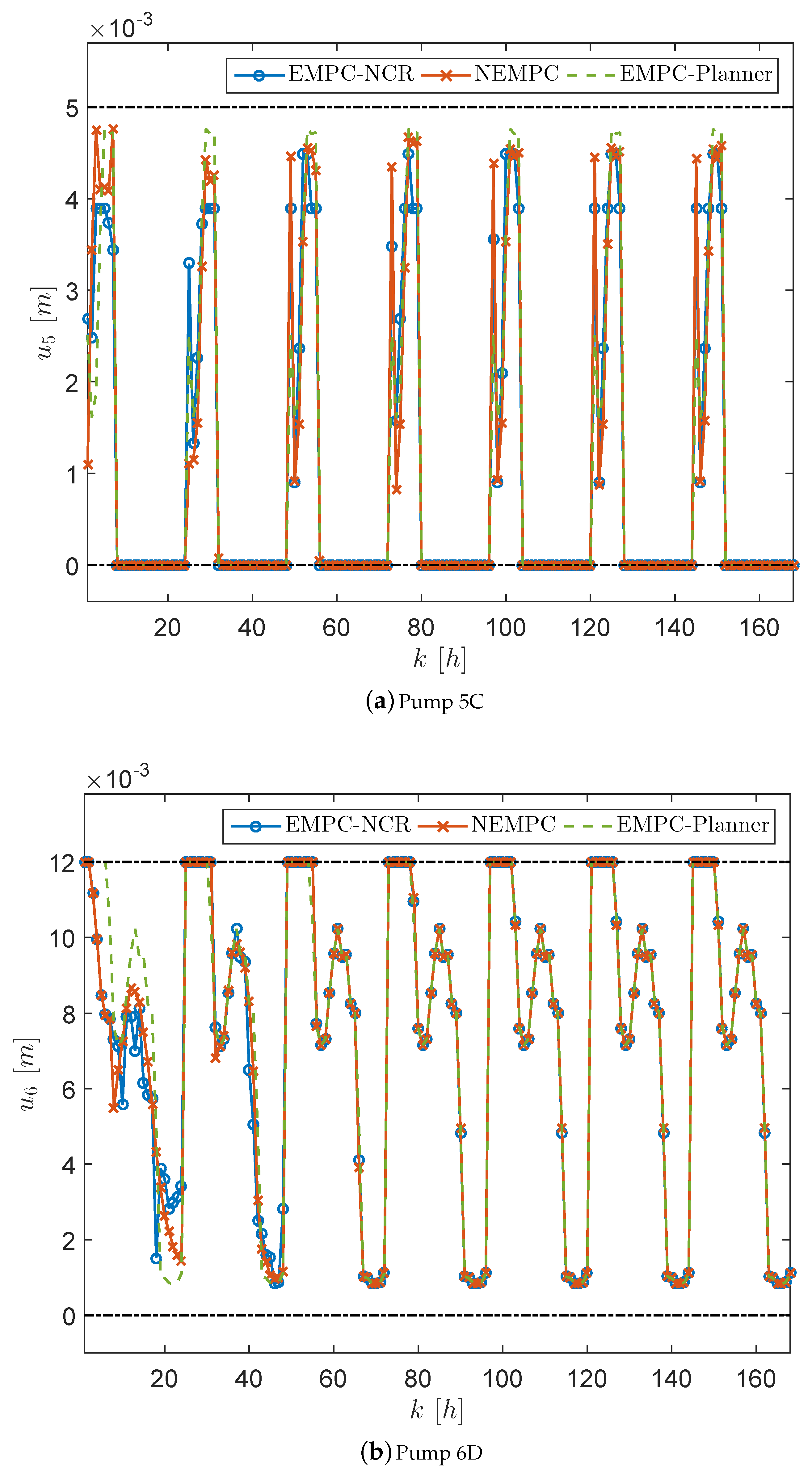
4.3. Simulation Results
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Mays, L. Water Distribution Systems Handbook; McGraw-Hill: New York, NY, USA, 2000. [Google Scholar]
- Yazdani, A.; Jeffrey, P. A complex network approach to robustness and vulnerability of spatially organized water distribution networks. Phys. Soc. 2010, 15, 1–18. [Google Scholar]
- Di Nardo, A.; Natale, M.D.; Giudicianni, C.; Musmarra, D.; Varela, J.R.; Santonastaso, G.; Simone, A.; Tzatchkov, V. Redundancy Features of Water Distribution Systems. In Proceedings of the International Conference on Water Distribution Systems, Cartagena, DC, USA, 24–28 July 2016; pp. 412–419. [Google Scholar]
- Brown, C.; Lund, J.; Cai, X.; Reed, P.; Zagona, E.; Ostfeld, A.; Hall, J.; Characklis, G.; Yu, W.; Brekke, L. The future of water resources systems analysis: Toward a scientific framework for sustainable water management. Water Resour. Res. 2015, 51, 6110–6124. [Google Scholar] [CrossRef]
- Puig, V.; Ocampo-Martinez, C.; Perez, R.; Cembrano, G.; Quevedo, J.; Escobet, T. Real-Time Monitoring and Operational Control of Drinking-Water Systems; Springer: Cham, Switzerland, 2017. [Google Scholar]
- Wang, Y.; Puig, V.; Cembrano, G. Non-linear Economic Model Predictive Control of Water Distribution Networks. J. Process Control 2017, 56, 23–34. [Google Scholar] [CrossRef]
- Todini, E.; Pilati, S. A gradient method for the analysis of pipe networks. In Proceedings of the International Conference on Computer Applications for Water Supply and Distribution, Leicester, UK, 8–10 September 1987; pp. 1–20. [Google Scholar]
- Rawlings, J.; Mayne, D. Model Predictive Control: Theory and Design; Nob Hill Publishing: Madison, WI, USA, 2009. [Google Scholar]
- Mayne, D. Model predictive control: Recent developments and future promise. Automatica 2014, 50, 2967–2986. [Google Scholar] [CrossRef]
- Heidarinejad, M.; Liu, J.; Christofides, P. Economic model predictive control of nonlinear process systems using Lyapunov techniques. AIChE J. 2012, 58, 855–870. [Google Scholar] [CrossRef]
- Han, H.; Qiao, J. Nonlinear Model-Predictive Control for Industrial Processes: An Application to Wastewater Treatment Process. IEEE Trans. Ind. Electron. 2014, 61, 1970–1982. [Google Scholar] [CrossRef]
- Liu, S.; Zhang, J.; Liu, J. Economic MPC with terminal cost and application to an oilsand primary separation vessel. Chem. Eng. Sci. 2015, 136, 27–37. [Google Scholar] [CrossRef]
- Zeng, J.; Liu, J. Economic Model Predictive Control of Wastewater Treatment Processes. Ind. Eng. Chem. Res. 2015, 54, 5710–5721. [Google Scholar] [CrossRef]
- Pereira, M.; Limon, D.; Muñoz de la Peña, D.; Valverde, L.; Alamo, T. Periodic Economic Control of a Nonisolated Microgrid. IEEE Trans. Ind. Electron. 2015, 62, 5247–5255. [Google Scholar] [CrossRef]
- Ellis, M.; Liu, J.; Christofides, P. Economic Model Predictive Control: Theory, Formulations and Chemical Process Applications; Springer: Cham, Switzerland, 2017. [Google Scholar]
- Ohar, Z.; Ostfeld, A. Optimal design and operation of booster chlorination stations layout in water distribution systems. Water Res. 2014, 58, 209–220. [Google Scholar] [CrossRef] [PubMed]
- Creaco, E.; Alvisi, S.; Franchini, M. A Multi-step Approach for Optimal Design and Management of the C-Town Pipe Network Model. In Proceedings of the 16th Water Distribution System Analysis Conference, Bari, Italy, 14–17 July 2014; pp. 37–44. [Google Scholar]
- Schwartz, R.; Housh, M.; Ostfeld, A. Least-Cost Robust Design Optimization of Water Distribution Systems under Multiple Loading. J. Water Resour. Plan. Manag. 2016, 142, 04016031. [Google Scholar] [CrossRef]
- Price, E.; Ostfeld, A. Graph Theory Modeling Approach for Optimal Operation of Water Distribution Systems. J. Hydraul. Eng. 2016, 142, 04015061. [Google Scholar] [CrossRef]
- Price, E.; Ostfeld, A. Optimal Pump Scheduling in Water Distribution Systems Using Graph Theory under Hydraulic and Chlorine Constraints. J. Water Resour. Plan. Manag. 2016, 142, 04016037. [Google Scholar] [CrossRef]
- Di Nardo, A.; Di Natale, M.; Giudicianni, C.; Santonastaso, G.; Savic, D. Simplified Approach to Water Distribution System Management via Identification of a Primary Network. J. Water Resour. Plan. Manag. 2018, 144, 1–9. [Google Scholar] [CrossRef]
- Cembrano, G.; Wells, G.; Quevedo, J.; Pérez, R.; Argelaguet, R. Optimal control of a water distribution network in a supervisory control system. Control Eng. Pract. 2000, 8, 1177–1188. [Google Scholar] [CrossRef]
- Ocampo-Martinez, C.; Puig, V.; Cembrano, G.; Quevedo, J. Application of MPC strategies to the management of complex networks of the urban water cycle. IEEE Control Syst. Mag. 2013, 33, 15–41. [Google Scholar] [CrossRef]
- Wang, Y.; Ocampo-Martinez, C.; Puig, V. Stochastic Model Predictive Control based on Gaussian Processes applied to Drinking Water Networks. IET Control Theory Appl. 2016, 10, 947–955. [Google Scholar] [CrossRef]
- Pereira, M.; Muñoz de la Peña, D.; Limon, D.; Alvarado, I.; Alamo, T. Application to a drinking water network of robust periodic MPC. Control Eng. Pract. 2016, 57, 50–60. [Google Scholar] [CrossRef]
- Wang, Y.; Ramón Salvador, J.; Muñoz de la Peña, D.; Puig, V.; Cembrano, G. Periodic Nonlinear Economic Model Predictive Control with Changing Horizon for Water Distribution Networks. In Proceedings of the 20th IFAC World Congress, Toulouse, France, 9–14 July 2017; pp. 6588–6593. [Google Scholar]
- Wang, Y.; Mũnoz de la Peña, D.; Puig, V.; Cembrano, G. A novel formulation of economic model predictive control for periodic operations. In Proceedings of the European Control Conference, Limassol, Cyprus, 12–15 June 2018. [Google Scholar]
- Limon, D.; Pereira, M.; Muñoz de la Peña, D.; Alamo, T.; Grosso, J. Single-layer economic model predictive control for periodic operation. J. Process Control 2014, 24, 1207–1224. [Google Scholar] [CrossRef]
- Wang, Y.; Ocampo-Martinez, C.; Puig, V.; Quevedo, J. Gaussian-process-based demand forecasting for predictive control of drinking water networks. In Critical Information Infrastructures Security; Springer: Cham, Switzerland, 2016; pp. 69–80. [Google Scholar]
- Rossman, L.A. EPANET2 Users Manual. National Risk Management Research Laboratory: Cincinnati, OH, USA, 2000. [Google Scholar]
- Toro, R.; Ocampo-Martinez, C.; Logist, F.; Van Impe, J.; Puig, V. Tuning of Predictive Controllers for Drinking Water Networked Systems. In Proceedings of the 18th IFAC World Congress, Milan, Italy, 28 August–2 September 2011; pp. 14507–14512. [Google Scholar]
- Löfberg, J. YALMIP: A Toolbox for Modeling and Optimization in MATLAB. In Proceedings of the IEEE International Symposium on Computer Aided Control Systems Design, New Orleans, LA, USA, 2–4 September 2004. [Google Scholar]
- MOSEK ApS. The MOSEK Optimization Toolbox for MATLAB 8.1.0.49. Available online: https://docs.mosek.com/8.1/toolbox/index.html (accessed on 12 April 2018).
- Currie, J.; Wilson, D. OPTI: Lowering the Barrier Between Open Source Optimizers and the Industrial MATLAB User. In Proceedings of the Foundations of Computer-Aided Process Operations, Savannah, Georgia, 8–11 January 2012. [Google Scholar]

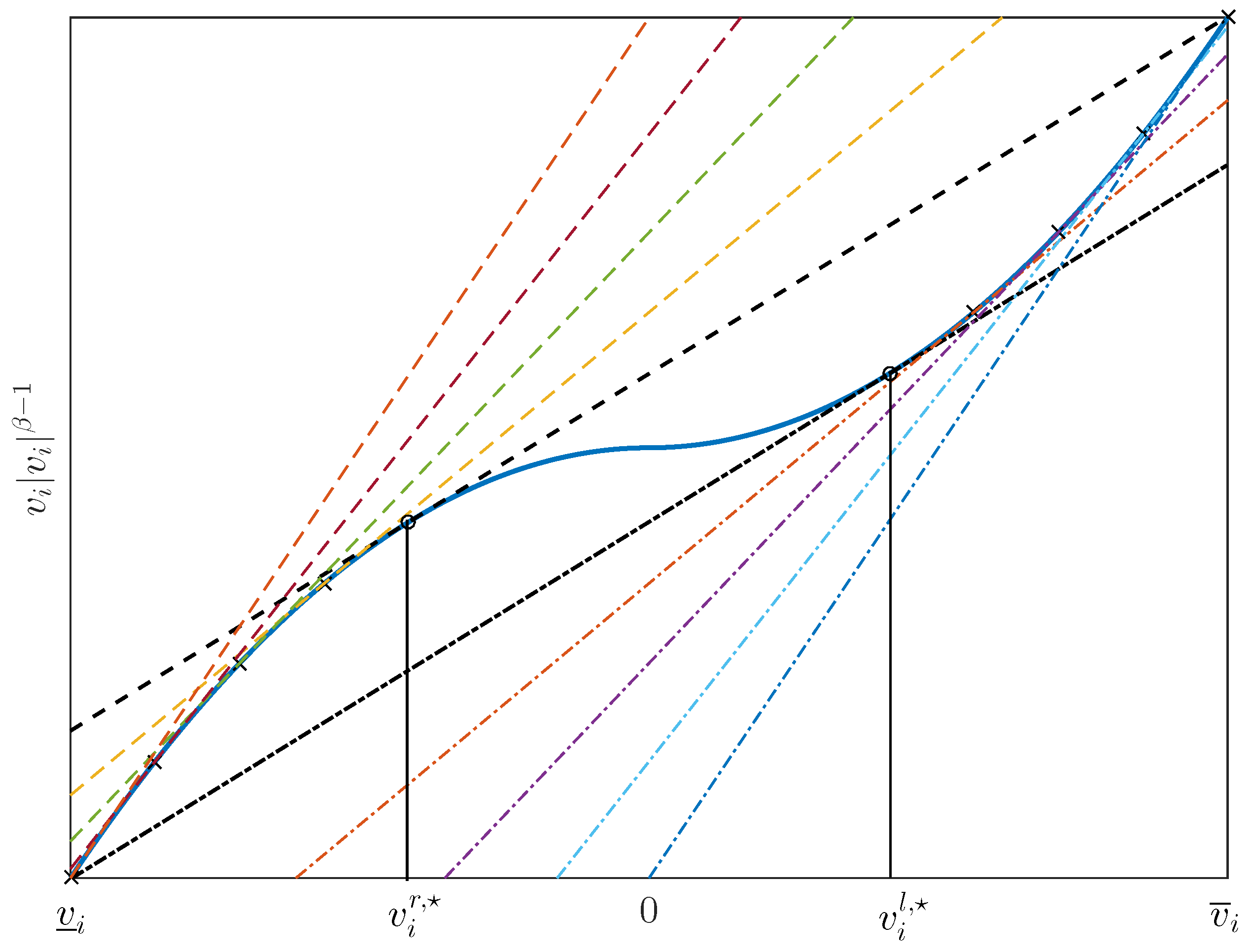
| Pipe | Coeffi. | Pipe | Coeffi. | Pipe | Coeffi. | Pipe | Coeffi. |
|---|---|---|---|---|---|---|---|
| 788 | 0.0098 | 1085 | 0.0132 | 1304 | 0.0156 | 1844 | 0.0092 |
| 790 | 0.0112 | 1107 | 0.0175 | 1638 | 0.0118 | 1848 | 0.0092 |
| 793 | 0.0103 | 1153 | 0.0101 | 1645 | 0.0099 | 1849 | 0.0114 |
| 794 | 0.0098 | 1154 | 0.0130 | 1653 | 0.0107 | 1879 | 0.0098 |
| 841 | 0.0094 | 1178 | 0.0165 | 1740 | 0.0100 | 1913 | 0.0113 |
| 911 | 0.0115 | 1196 | 0.0367 | 1752 | 0.0103 | 1964 | 0.0119 |
| 912 | 0.0118 | 1208 | 0.0131 | 1753 | 0.0130 | 1978 | 0.0111 |
| 993 | 0.0101 | 1209 | 0.0137 | 1783 | 0.0168 | 2010 | 0.0120 |
| 1020 | 0.0094 | 1210 | 0.0143 | 1793 | 0.0189 | - | - |
| 1033 | 0.0102 | 1278 | 0.0101 | 1832 | 0.0145 | - | - |
| 1036 | 0.0101 | 1301 | 0.0172 | 1842 | 0.0097 | - | - |
| Tank | Elevation (m) | Volume (m) | Hydraulic Head (m) |
|---|---|---|---|
| A | 184.13 | 1.02 | 185.15 |
| B | 216 | 2.03 | 218.03 |
| C | 258.9 | 0.5 | 259.40 |
| D | 241.18 | 1.1 | 242.28 |
| E | 203.01 | 0.01 | 203.03 |
| F | 235.71 | 0.19 | 235.90 |
| MPC Strategy | |||
|---|---|---|---|
| EMPC-NCR | 0.6992 | 0.2604 | 6.7078 |
| NEMPC | 0.7028 | 0.1914 | 6.5249 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Alamo, T.; Puig, V.; Cembrano, G. Economic Model Predictive Control with Nonlinear Constraint Relaxation for the Operational Management of Water Distribution Networks. Energies 2018, 11, 991. https://doi.org/10.3390/en11040991
Wang Y, Alamo T, Puig V, Cembrano G. Economic Model Predictive Control with Nonlinear Constraint Relaxation for the Operational Management of Water Distribution Networks. Energies. 2018; 11(4):991. https://doi.org/10.3390/en11040991
Chicago/Turabian StyleWang, Ye, Teodoro Alamo, Vicenç Puig, and Gabriela Cembrano. 2018. "Economic Model Predictive Control with Nonlinear Constraint Relaxation for the Operational Management of Water Distribution Networks" Energies 11, no. 4: 991. https://doi.org/10.3390/en11040991
APA StyleWang, Y., Alamo, T., Puig, V., & Cembrano, G. (2018). Economic Model Predictive Control with Nonlinear Constraint Relaxation for the Operational Management of Water Distribution Networks. Energies, 11(4), 991. https://doi.org/10.3390/en11040991







