A Robust Digital Control Strategy Using Error Correction Based on the Discrete Lyapunov Theorem
Abstract
:1. Introduction
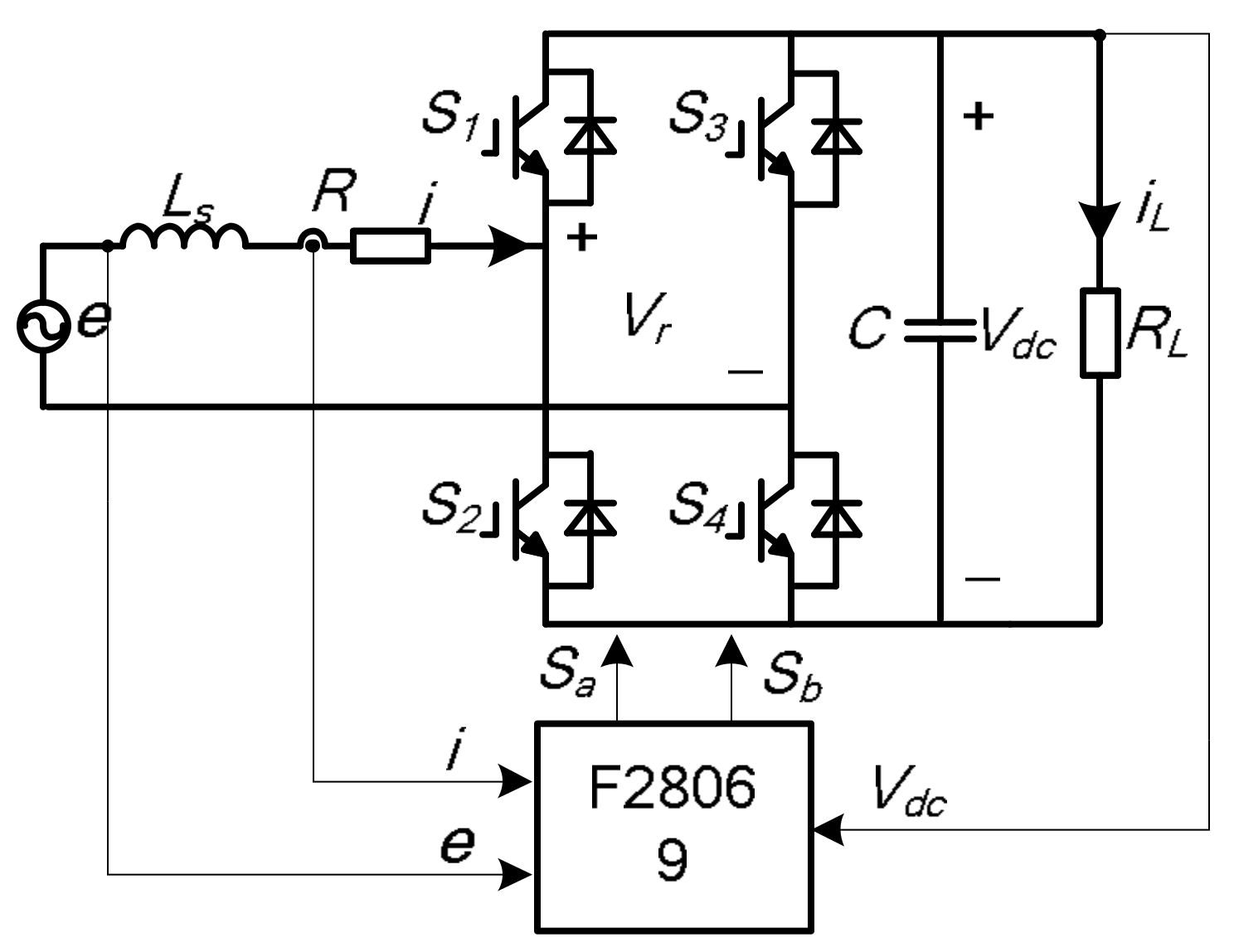
2. Modeling of the Converter
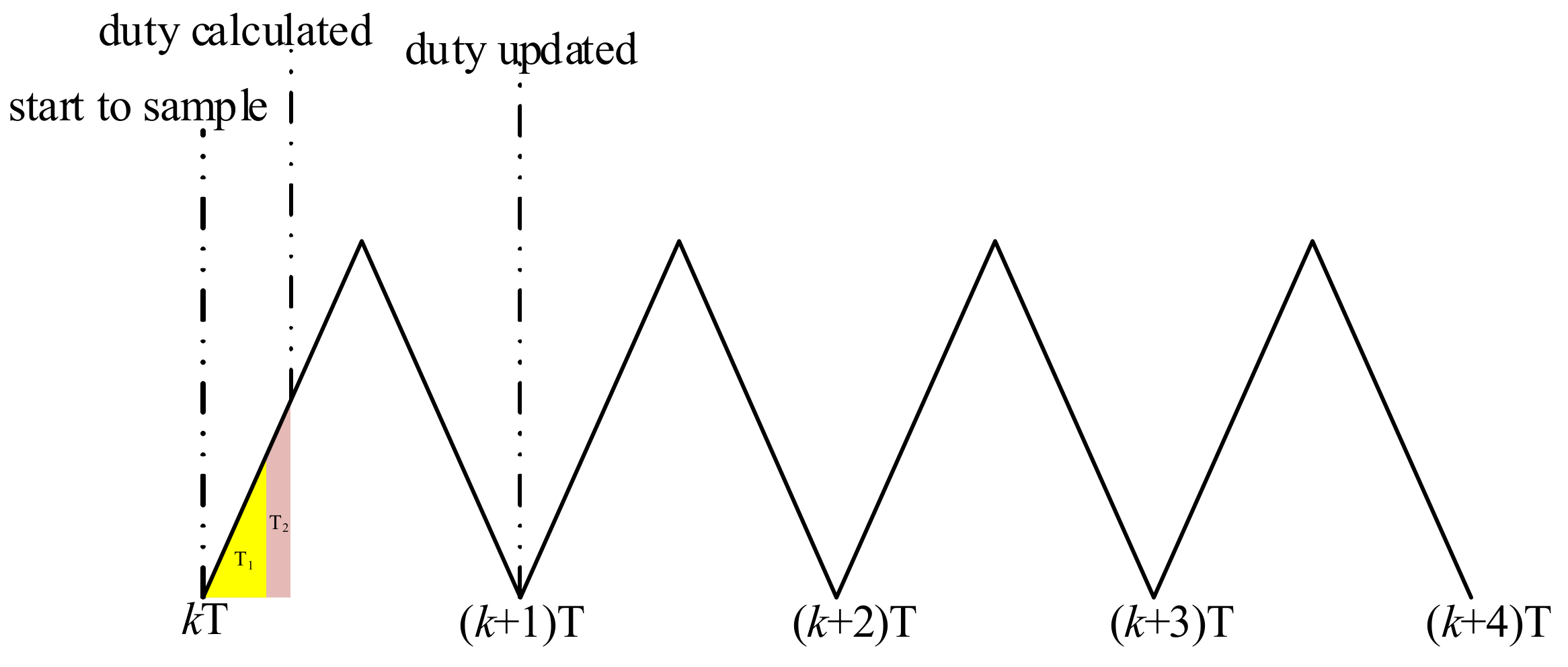
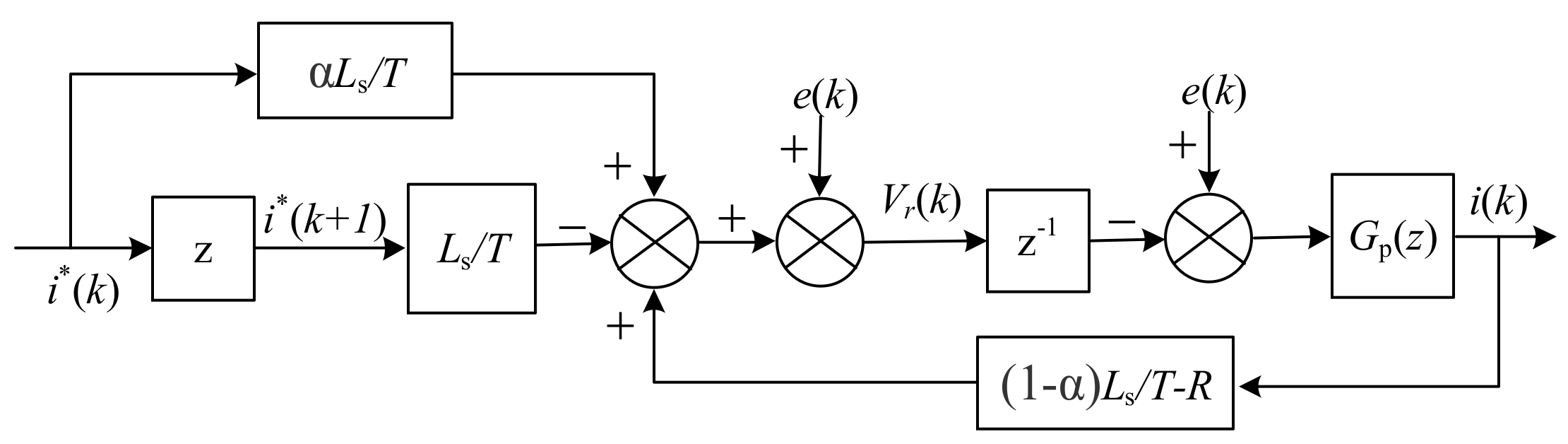
3. The Principle of the Proposed Method
- (1)
- L(0) = 0;
- (2)
- L(x(k)) > 0 for all x(k) ≠ 0;
- (3)
- L(x(k)) → ∞ as ‖x(k)‖ → ∞;
- (4)
- ΔL(x(k)) < 0 for all x(k) ≠ 0.
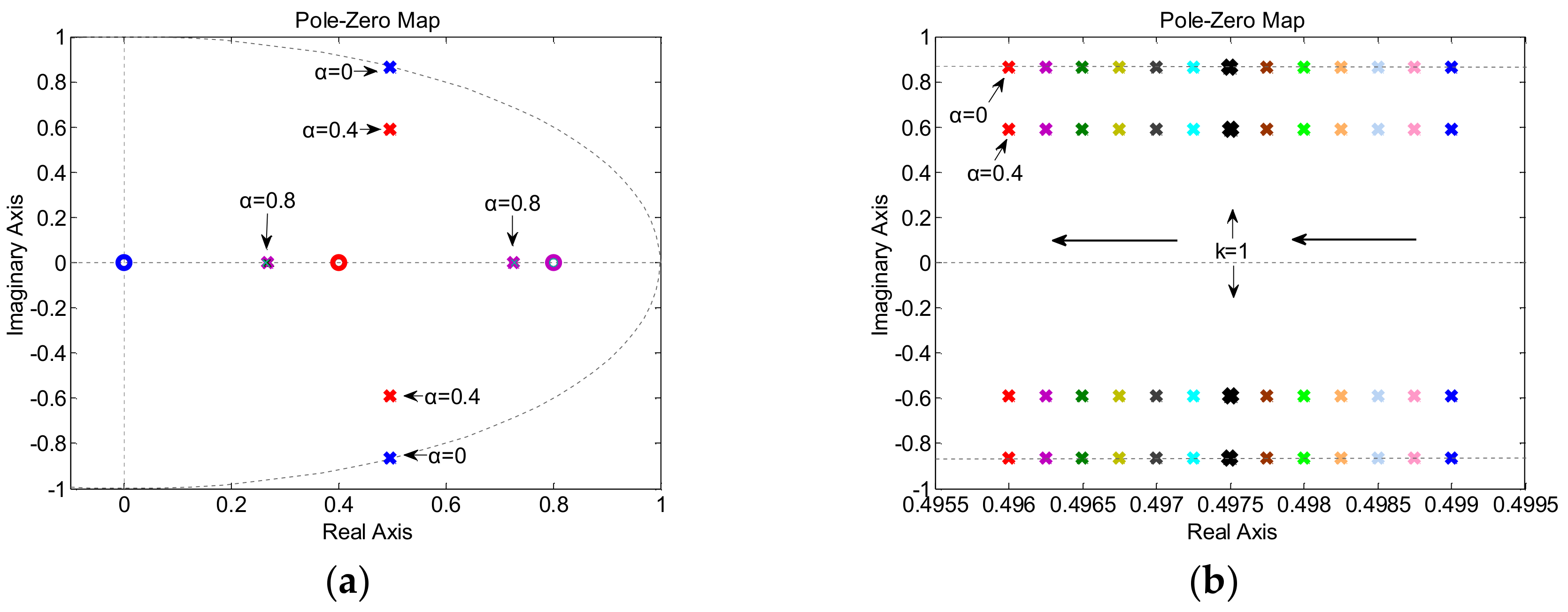
4. Selection and Analysis of Control Coefficient
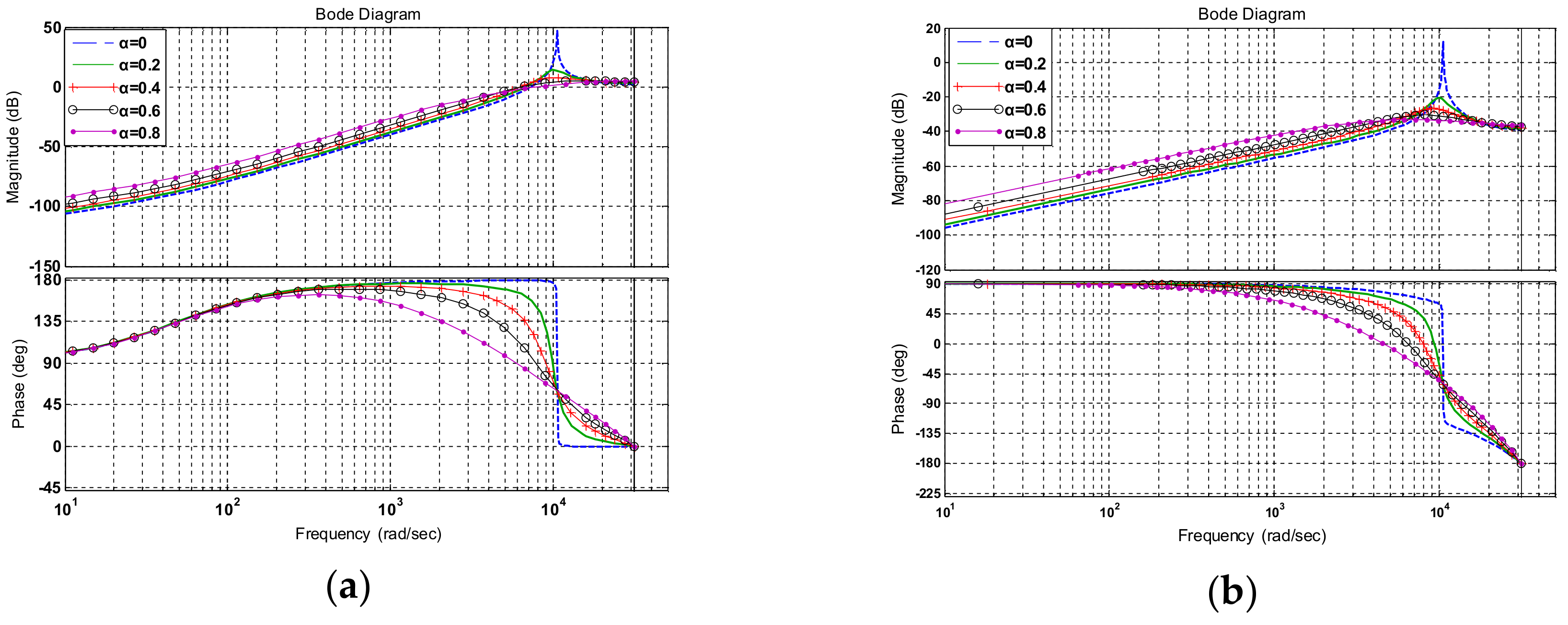
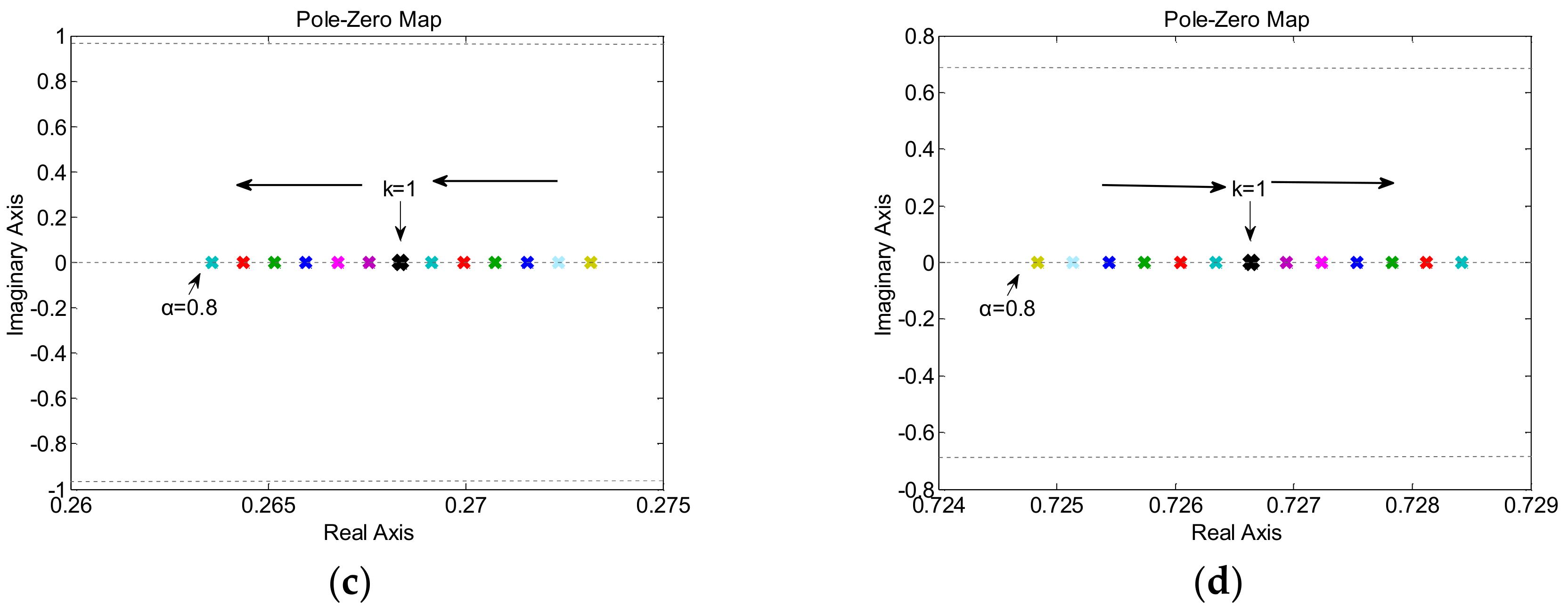
4.1. Influence of Stability
4.2. Influence of Steady-State Error
4.3. Influence of Robustness
4.4. Selection of α
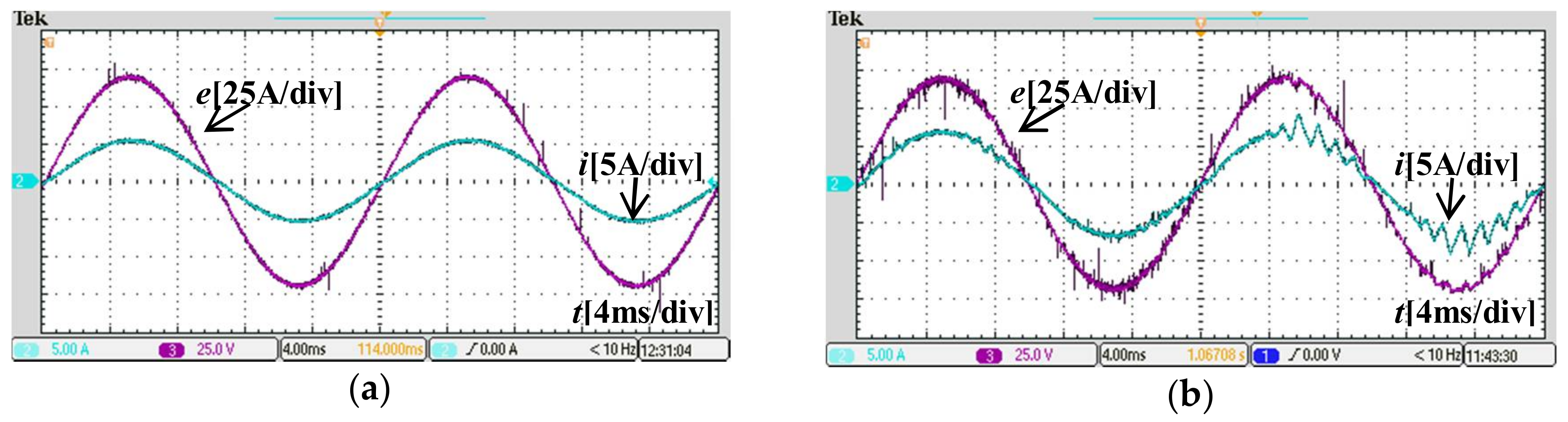
5. Experimental Results
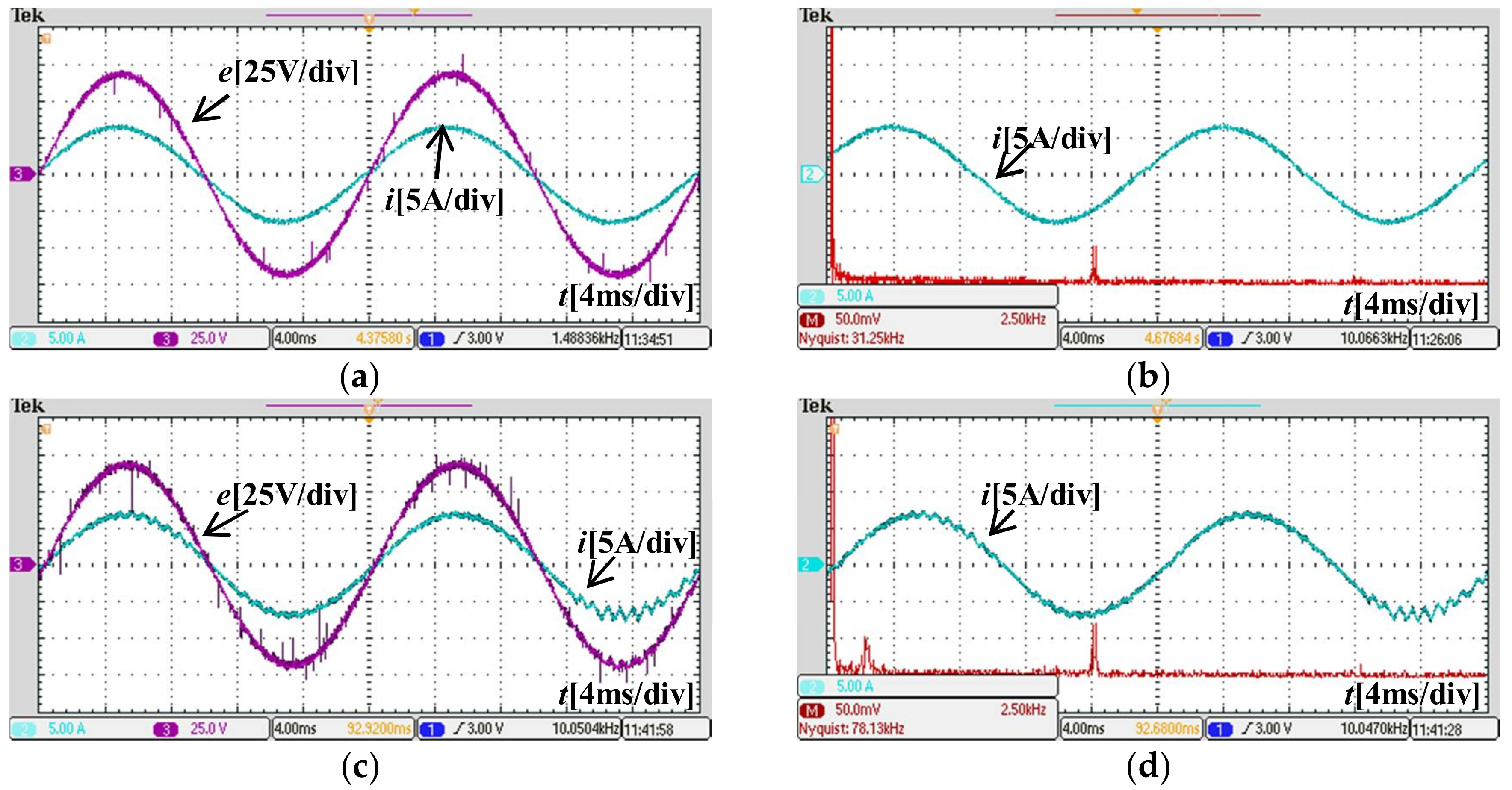
5.1. Steady-State Performance Tests
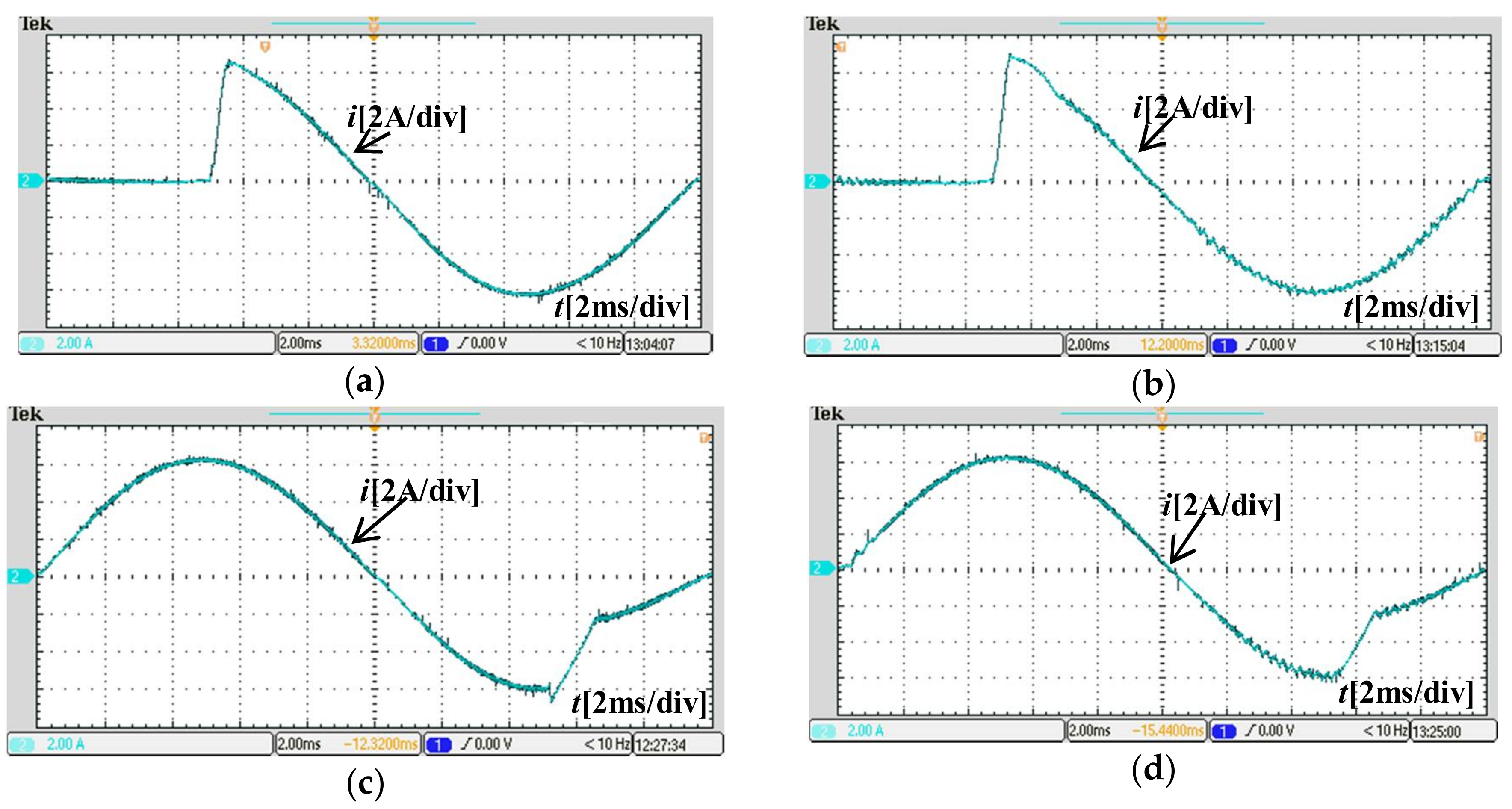
5.2. Dynamic Response Tests
5.3. Investigation of Robustness
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Shahid, A. An overview of control architecture for next generation smart grids. In Proceedings of the 2017 19th International Conference on Intelligent System Application to Power Systems (ISAP), San Antonio, TX, USA, 17–20 September 2017; pp. 1–5. [Google Scholar]
- Gai, W.; Chen, D.; Yin, J.; Chen, L. High-speed maglev parallel control and management system-overview and framework. In Proceedings of the 2014 International Conference on Informative and Cybernetics for Computational Social Systems (ICCSS), Qingdao, China, 9–10 October 2014; pp. 24–28. [Google Scholar]
- Li, P.; Song, Y.D.; Li, D.Y.; Cai, W.C.; Zhang, K. Control and Monitoring for Grid-Friendly Wind Turbines: Research Overview and Suggested Approach. IEEE Trans. Power Electron. 2015, 30, 1979–1986. [Google Scholar] [CrossRef]
- Calderon-Lopez, G.; Villarruel-Parra, A.; Kakosimos, P.; Ki, S.K.; Todd, R.; Forsyth, A.J. Comparison of digital PWM control strategies for high-power interleaved DC–DC converters. IET Power Electron. 2018, 11, 391–398. [Google Scholar] [CrossRef]
- Chattopadhyay, S.; Das, S. A Digital Current-Mode Control Technique for DC–DC Converters. IEEE Trans. Power Electron. 2006, 21, 1718–1726. [Google Scholar] [CrossRef]
- Pan, S.Z.; Jain, P.K. A Low-Complexity Dual-Voltage-Loop Digital Control Architecture with Dynamically Varying Voltage and Current References. IEEE Trans. Power Electron. 2014, 29, 2049–2060. [Google Scholar] [CrossRef]
- Qi, C.; Chen, X.; Tu, P.; Wang, P. Deadbeat control for a single-phase cascaded H-bridge rectifier with voltage balancing modulation. IET Power Electron. 2018, 11, 610–617. [Google Scholar] [CrossRef]
- Xueguang, Z.; Wenjie, Z.; Jiaming, C.; Dianguo, X. Deadbeat Control Strategy of Circulating Currents in Parallel Connection System of Three-Phase PWM Converter. IEEE Trans. Energy Convers. 2014, 29, 406–417. [Google Scholar]
- Hung, G.K.; Chang, C.-C.; Chen, C.-L. Analysis and implementation of a delay compensated deadbeat current controller for solar inverters. IEE Proc. Circuits Devices Syst. 2001, 148, 279–286. [Google Scholar] [CrossRef]
- Stumper, J.F.; Hagenmeyer, V.; Kuehl, S.; Kennel, R. Deadbeat Control for Electrical Drives: A Robust and Performant Design Based on Differential Flatness. IEEE Trans. Power Electron. 2015, 30, 4585–4596. [Google Scholar] [CrossRef]
- SLarrinaga, A.; Vidal, M.A.R.; Oyarbide, E.; Apraiz, J.R.T. Predictive control strategy for dc/ac converters based on direct power control. IEEE Trans. Ind. Electron. 2007, 54, 1261–1271. [Google Scholar] [CrossRef]
- Rodriguez, J.; Kazmierkowski, M.P.; Espinoza, J.R.; Zanchetta, P.; Abu-Rub, H.; Young, H.A.; Rojas, C.A. State of the art of finite control set model predictive control in power electronics. IEEE Trans. Ind. Inform. 2013, 9, 1003–1016. [Google Scholar] [CrossRef]
- Bibian, S.; Jin, H. High Performance Predictive Dead-Beat Digital Controller for DC Power Supplies. IEEE Trans. Power Electron. 2002, 17, 420–427. [Google Scholar] [CrossRef]
- Bode, G.H.; Loh, P.C.; Newman, M.J.; Holmes, D.G. An improved robust predictive current regulation algorithm. IEEE Trans. Ind. Appl. 2005, 41, 1720–1733. [Google Scholar] [CrossRef]
- Siami, M.; Abbaszadeh, A.; Khaburi, D.A.; Rodriguez, J. Robustness improvement of predictive current control using prediction error correction for permanent magnet synchronous machines. IEEE Trans. Ind. Electron. 2016, 63, 3458–3466. [Google Scholar] [CrossRef]
- Di Cairano, S.; Heemels, W.P.M.H.; Lazar, M.; Bemporad, A. Stabilizing Dynamic Controllers for Hybrid Systems: A Hybrid Control Lyapunov Function Approach. IEEE Trans. Autom. Control 2014, 59, 2629–2643. [Google Scholar] [CrossRef]
- Wang, J.W.; Wu, H.N.; Li, H.X. Fuzzy Control Design for Nonlinear ODE-Hyperbolic PDE-Cascaded Systems: A Fuzzy and Entropy-Like Lyapunov Function Approach. IEEE Trans. Fuzzy Syst. 2014, 22, 1313–1324. [Google Scholar] [CrossRef]
- Furqon, R.; Chen, Y.J.; Tanaka, M.; Tanaka, K.; Wang, H.O. An SOS-Based Control Lyapunov Function Design for Polynomial Fuzzy Control of Nonlinear Systems. IEEE Trans. Fuzzy Syst. 2017, 25, 775–787. [Google Scholar] [CrossRef]
- Sefa, I.; Ozdemir, S.; Komurcugil, H.; Altin, N. Comparative study on Lyapunov-function-based control schemes for single-phase grid-connected voltage-source inverter with LCL filter. IET Renew. Power Gener. 2017, 11, 1473–1482. [Google Scholar] [CrossRef]
- Kwak, S.; Yoo, S.J.; Park, J. Finite control set predictive control based on Lyapunov function for three-phase voltage source inverters. IET Power Electron. 2014, 7, 2726–2732. [Google Scholar] [CrossRef]
- Parvez, M.; Mekhilef, S.; Tan, N.M.L.; Akagi, H. A robust modified model predictive control (MMPC) based on Lyapunov function for three-phase active-front-end (AFE) rectifier. In Proceedings of the 2016 IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 18–24 March 2016; pp. 1163–1168. [Google Scholar]
- Akter, M.P.; Mekhilef, S.; Tan, N.M.L.; Akagi, H. Modified model predictive control of a bidirectional AC-DC converter based on Lyapunov function for energy storage systems. IEEE Trans. Ind. Electron. 2016, 63, 704–715. [Google Scholar] [CrossRef]
- Du, G.; Liu, Z.; Du, F.; Li, J. Performance Improvement of Model Predictive Control Using Control Error Compensation for Power Electronic Converters Based on the Lyapunov Function. J. Power Electron. 2017, 17, 983–990. [Google Scholar]




| Varible | Symbol | Value |
|---|---|---|
| The grid voltage (RMS) | e | 50 V/50 Hz |
| Filter inductance | Ls | 3.1 mH |
| Equivalent series resistance | R | 0.3 Ω |
| DC side capacitor | C | 1000 μF |
| Sampling period | T | 1 × e−4 s |
| Switching frequency | f | 10 kHz |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, G.; Li, J.; Du, F.; Liu, Z. A Robust Digital Control Strategy Using Error Correction Based on the Discrete Lyapunov Theorem. Energies 2018, 11, 848. https://doi.org/10.3390/en11040848
Du G, Li J, Du F, Liu Z. A Robust Digital Control Strategy Using Error Correction Based on the Discrete Lyapunov Theorem. Energies. 2018; 11(4):848. https://doi.org/10.3390/en11040848
Chicago/Turabian StyleDu, Guiping, Jiajian Li, Fada Du, and Zhifei Liu. 2018. "A Robust Digital Control Strategy Using Error Correction Based on the Discrete Lyapunov Theorem" Energies 11, no. 4: 848. https://doi.org/10.3390/en11040848
APA StyleDu, G., Li, J., Du, F., & Liu, Z. (2018). A Robust Digital Control Strategy Using Error Correction Based on the Discrete Lyapunov Theorem. Energies, 11(4), 848. https://doi.org/10.3390/en11040848




