Simulating Engineering Flows through Complex Porous Media via the Lattice Boltzmann Method
Abstract
:1. Introduction
2. Methodology
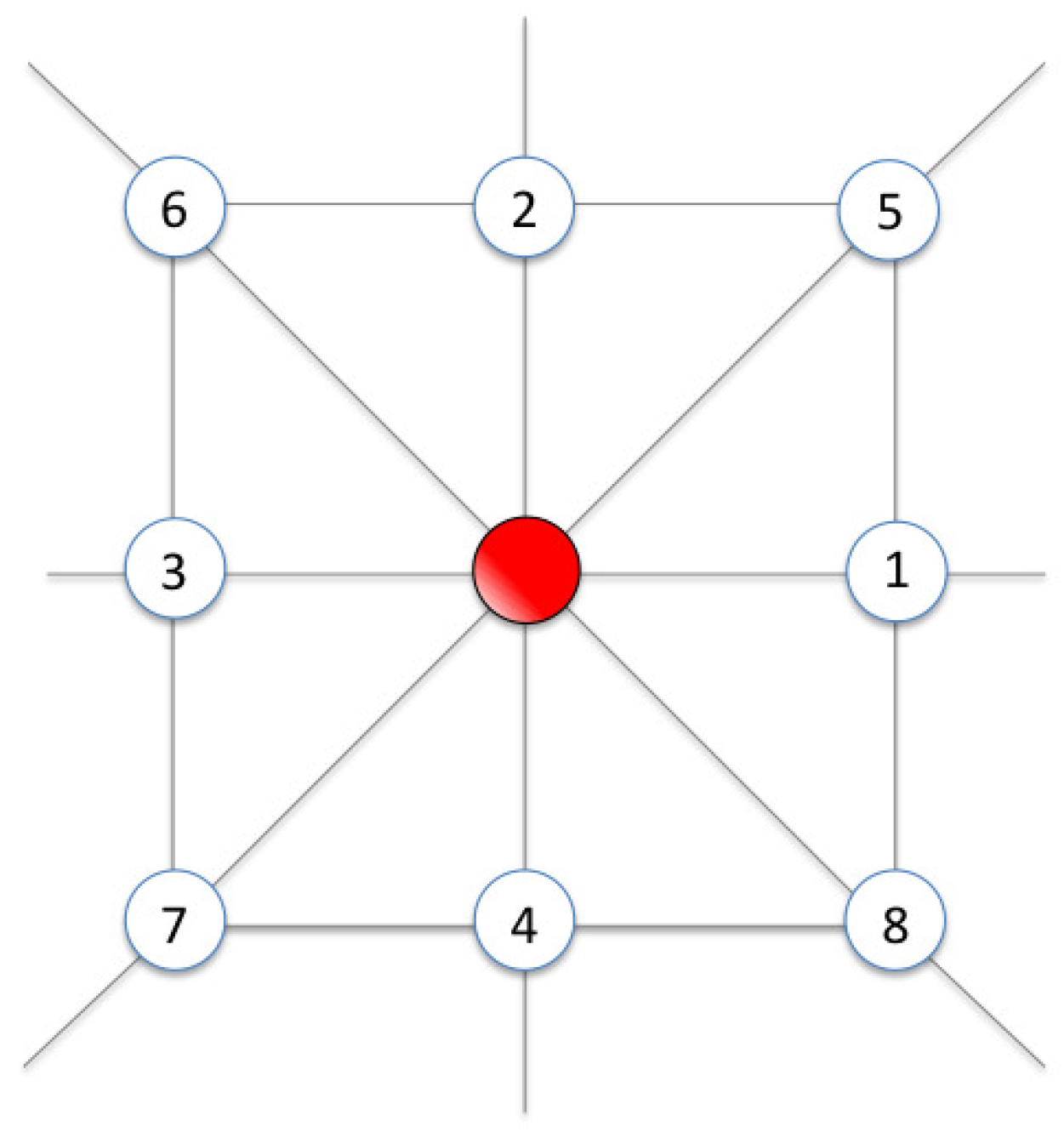
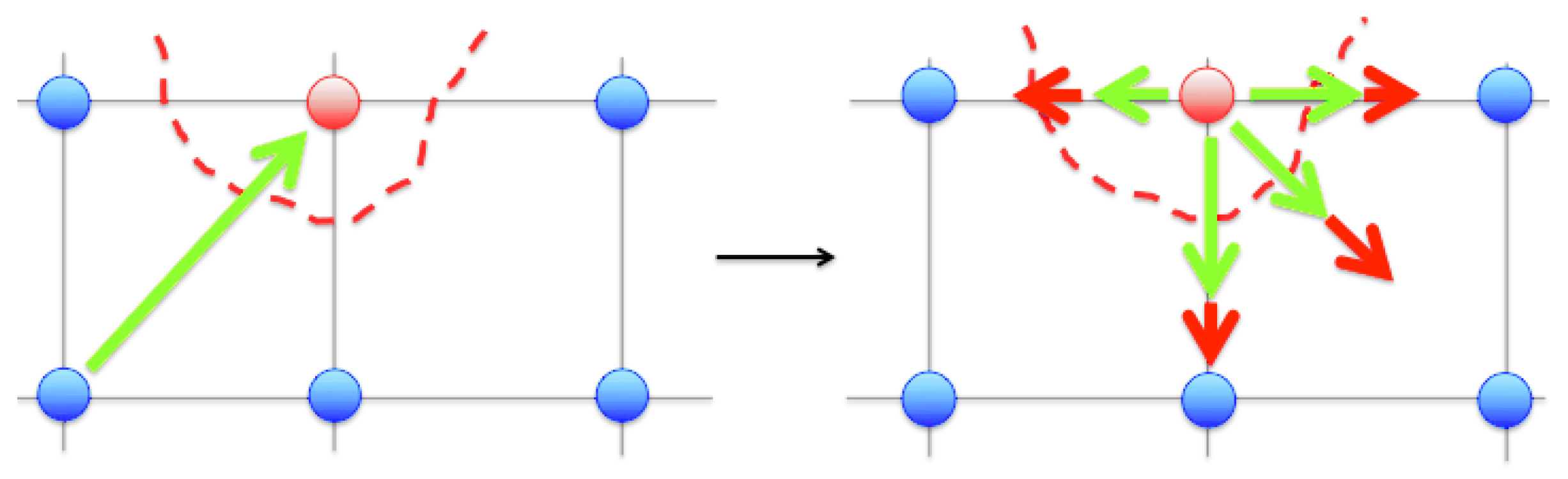
2.1. The Lattice Boltzmann Method
- the adoption of a simplified formulation of the collision operator, that is the so-called Bhatnagar-Gross-Krook (BGK) approximation [32]: , with the relaxation time towards local equilibrium;
2.2. Reactive Boundary Condition
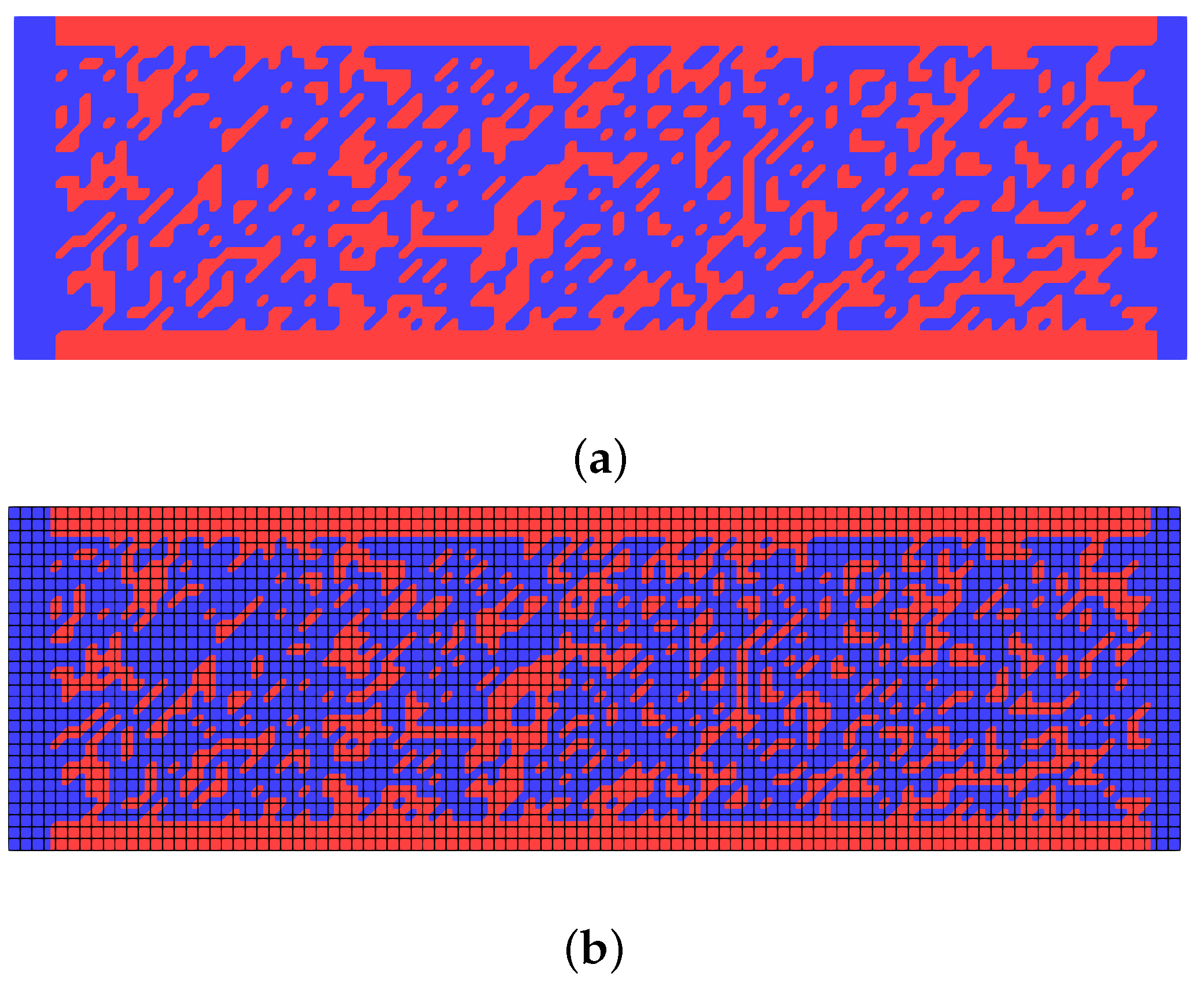
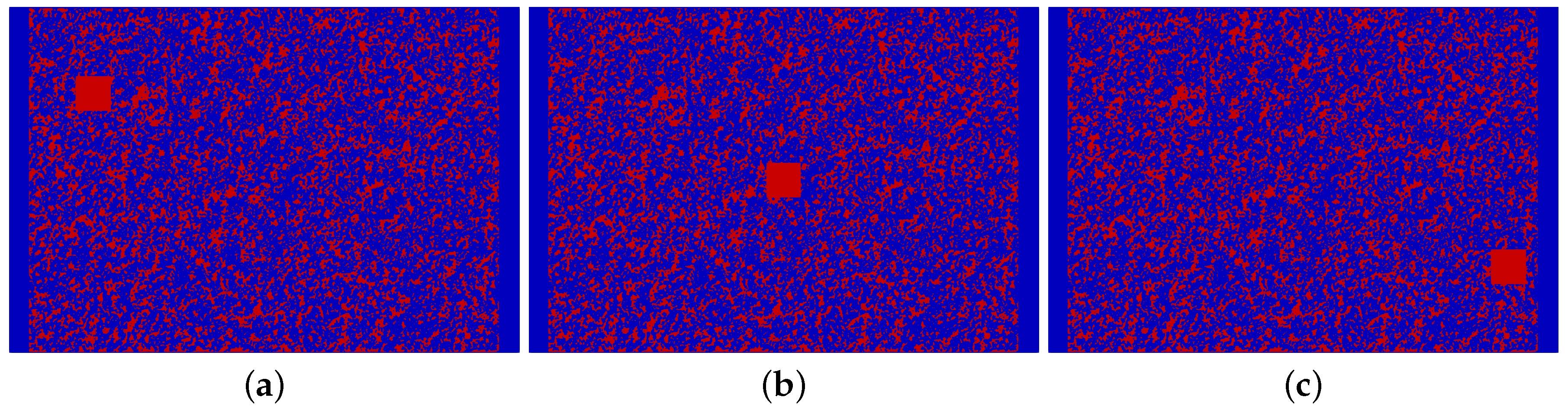
2.3. Porous Domain Reconstruction
3. Results and Discussion
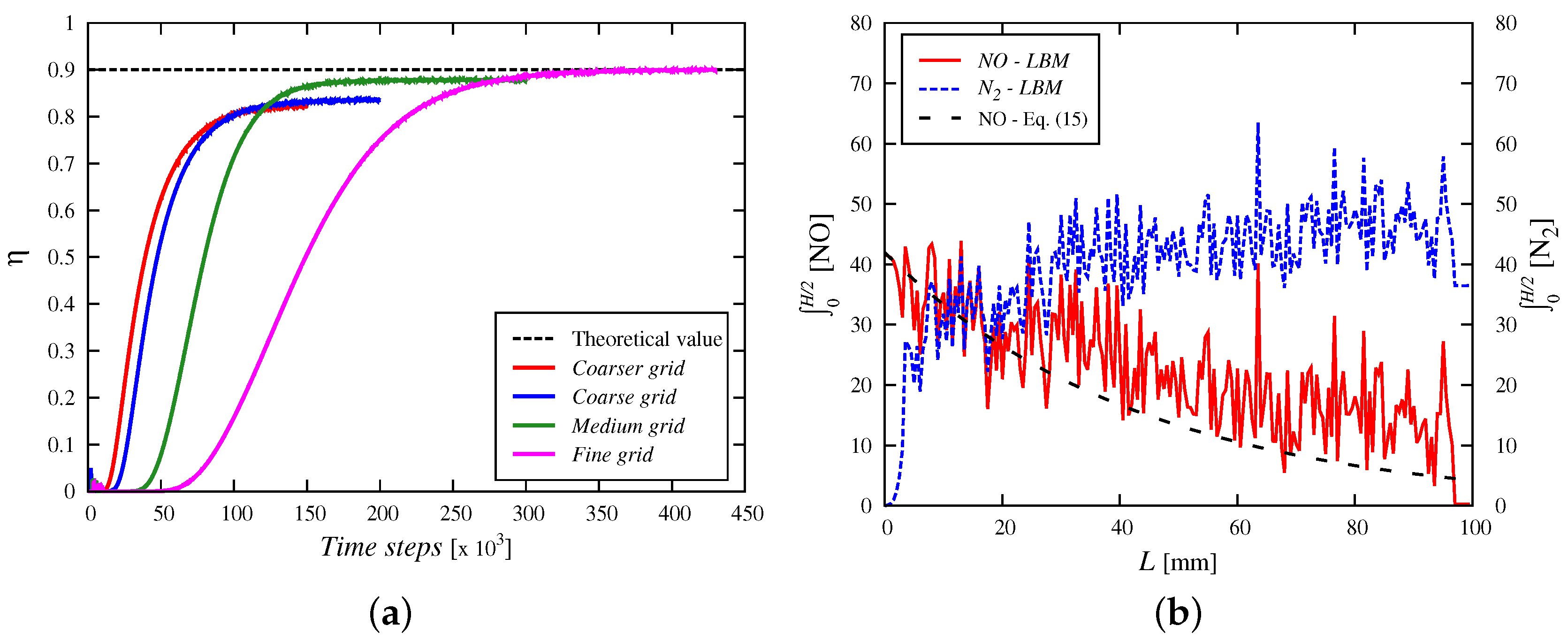
3.1. Selective Catalytic Reduction of NO Emissions
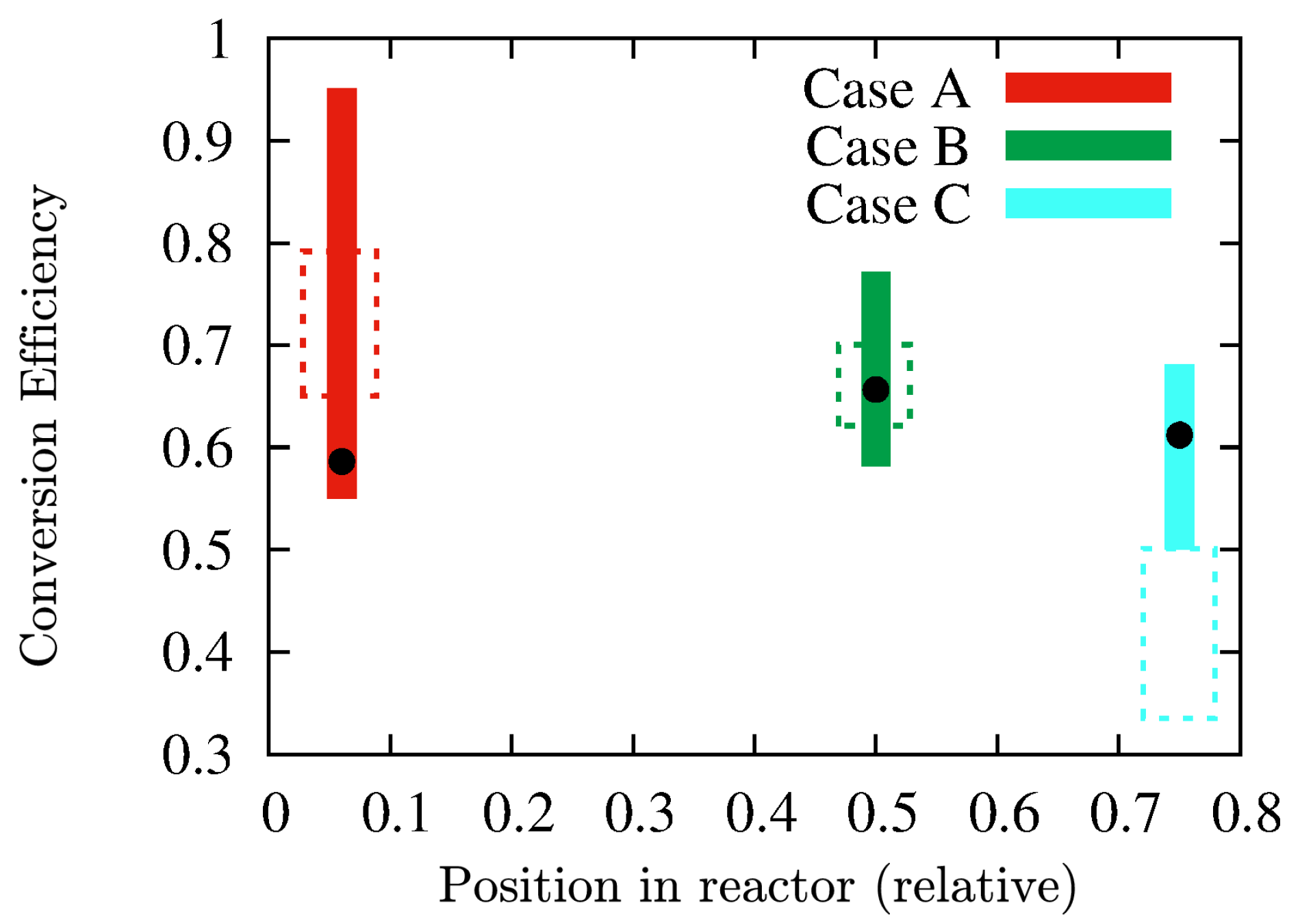
3.2. Heterogeneous Catalysis in Pulsed-Flow Reactors
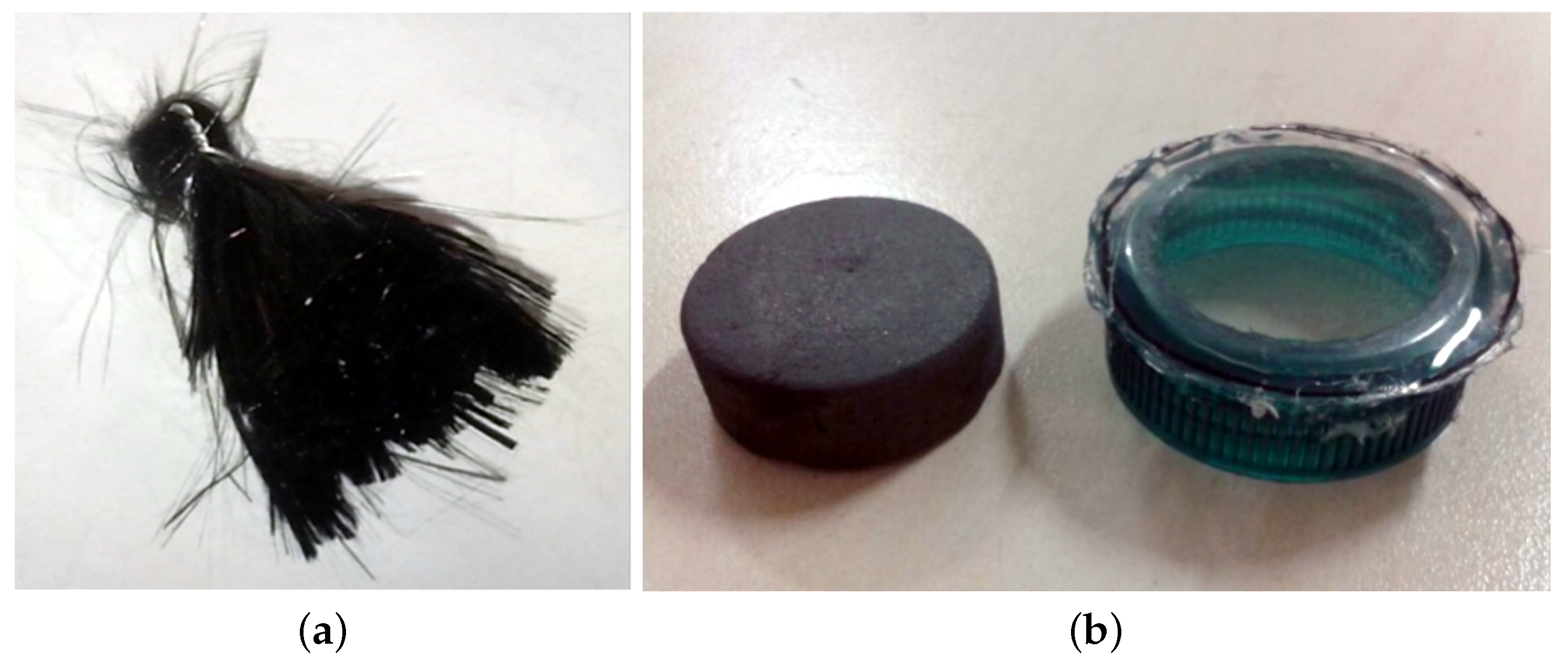
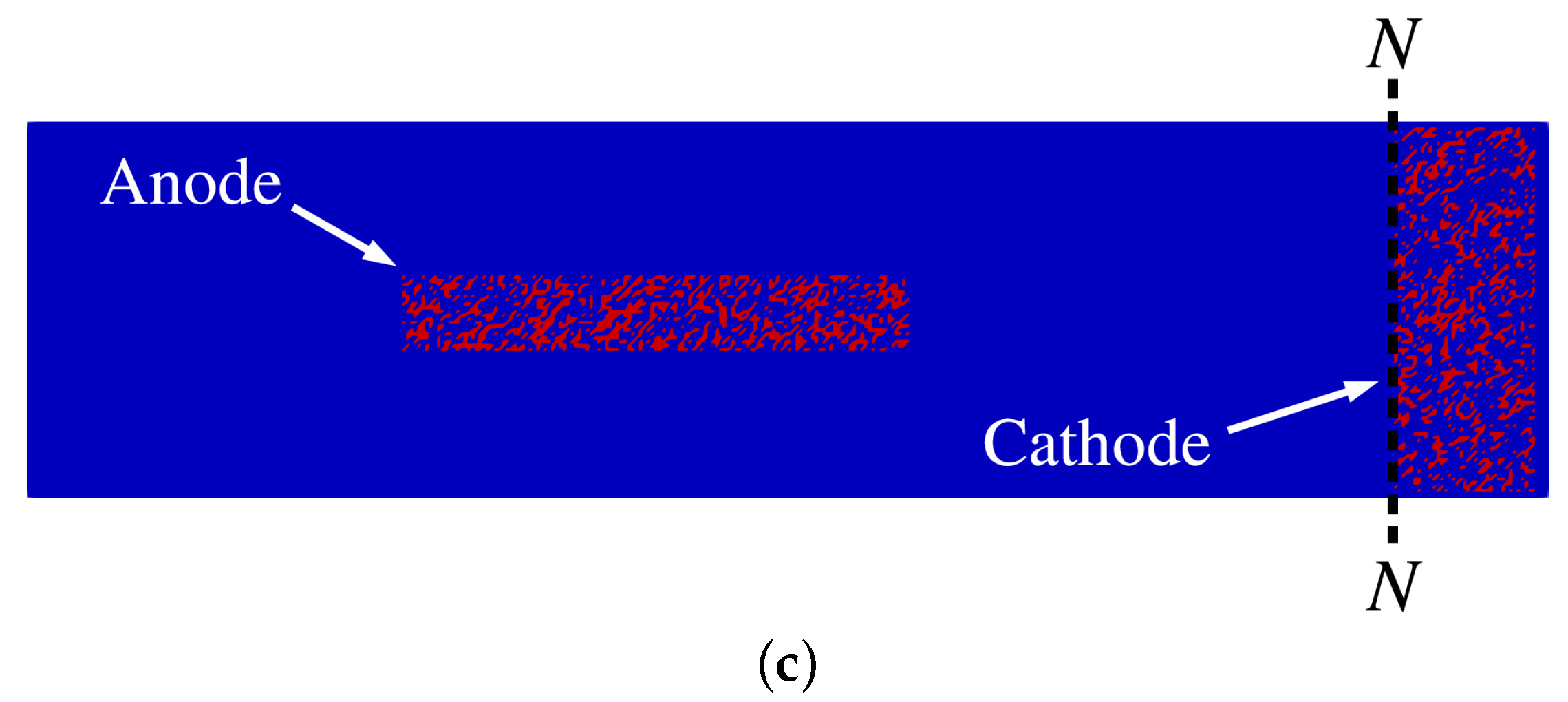
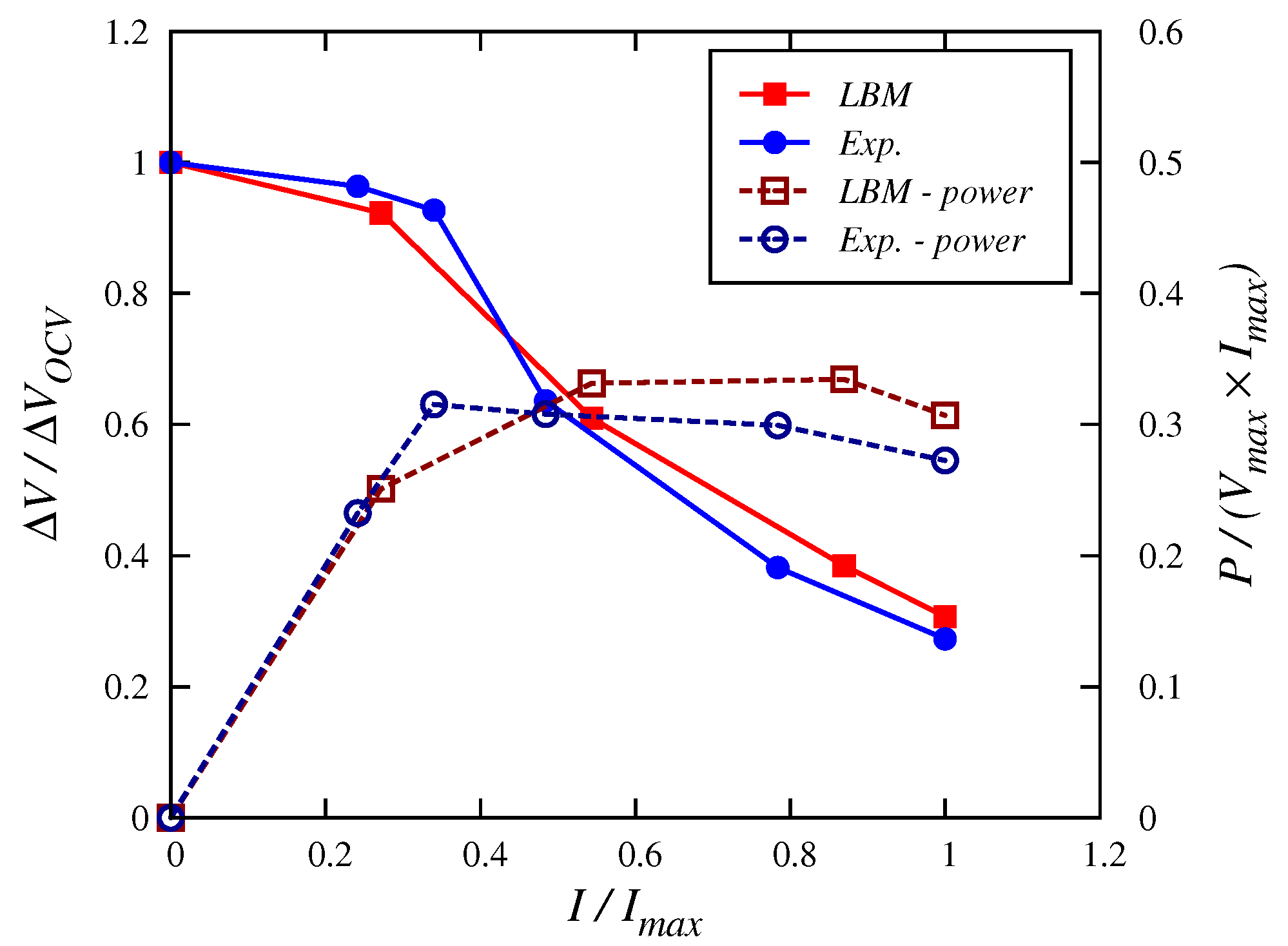
3.3. Microbial Fuel Cells Characterization
- negligible activation losses in the small currents region, due to the slow start-up time of bacterial activity (typically 3–5 days);
- quasi-linear Ohmic-losses region, which represents the majority of the MFC operational range;
- no appreciable concentration-loss phase.
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| BTE | Boltzmann Transport Equation |
| CFD | Computational Fluid Dynamics |
| DPF | Diesel Particulate Filter |
| LBM | Lattice Boltzmann Method |
| LU | Lattice Units |
| MFC | Microbial Fuel Cell |
| MLUPS | Milions Lattice site Updates per Second |
| OCV | Open-Circuit Voltage |
| SCR | Selective Catalytic Reduction |
| TAP | Temporal Analysis of Products |
| USPC | Unsteady-State Processes in Catalysis |
References
- Succi, S. The Lattice Boltzmann Equation for Fluid Dynamics and Beyond; Oxford University Press: Oxford, UK, 2004. [Google Scholar]
- Succi, S.; Vergassola, M.; Benzi, R. Lattice Boltzmann scheme for two-dimensional magnetohydrodynamics. Phys. Rev. A 1991, 43, 4521. [Google Scholar] [CrossRef] [PubMed]
- Benzi, R.; Succi, S.; Vergassola, M. The Lattice Boltzmann equation: Theory and applications. Phys. Rep. 1992, 222, 145–197. [Google Scholar] [CrossRef]
- Calí, A.; Succi, S.; Cancelliere, A.; Benzi, R.; Gramignani, M. Diffusion and hydrodynamic dispersion with the Lattice Boltzmann method. Phys. Rev. A 1992, 45, 5771. [Google Scholar] [CrossRef] [PubMed]
- Chen, S.; Doolen, G.D. Lattice Boltzmann Method for fluid flows. Annu. Rev. Fluid Mech. 1998, 30, 329–364. [Google Scholar] [CrossRef]
- Sukop, M.C.; Thorne, D.T. Lattice Boltzmann Modeling: An Introduction for Geoscientists and Engineers; Springer Verlag: Heidelberg, Germany, 2006. [Google Scholar]
- Aidun, C.K.; Clausen, J.R. Lattice-Boltzmann method for complex flows. Annu. Rev. Fluid Mech. 2010, 42, 439–472. [Google Scholar] [CrossRef]
- Montessori, A.; Falcucci, G. Lattice Boltzmann Modeling of Complex Flows for Engineering Applications; Morgan & Claypool Publishers: San Rafael, CA, USA, 2018. [Google Scholar]
- Calmidi, V.V.; Mahajan, R.L. Forced convection in high porosity metal foams. J. Heat Transf. 2000, 122, 557–565. [Google Scholar] [CrossRef]
- Kim, S.Y.; Kang, B.H.; Kim, J.H. Forced convection from aluminum foam materials in an asymmetrically heated channel. Int. J. Heat Mass Transf. 2001, 44, 1451–1454. [Google Scholar] [CrossRef]
- Mancin, S.; Zilio, C.; Cavallini, A.; Rossetto, L. Heat transfer during air flow in aluminum foams. Int. J. Heat Mass Transf. 2010, 53, 4976–4984. [Google Scholar] [CrossRef]
- Bai, M.; Chung, J.N. Analytical and numerical prediction of heat transfer and pressure drop in open-cell metal foams. Int. J. Therm. Sci. 2011, 50, 869–880. [Google Scholar] [CrossRef]
- Williams, J.L. Monolith structures, materials, properties and uses. Catal. Today 2001, 69, 3–9. [Google Scholar] [CrossRef]
- Kee, R.J.; Coltrin, M.E.; Glarborg, P.; Zhu, H. Chemically Reacting Flow: Theory, Modeling, and Simulation, 2nd ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2018. [Google Scholar]
- Kirubakaran, A.; Jain, S.; Nema, R. A review on fuel cell technologies and power electronic interface. Renew. Sustain. Energy Rev. 2009, 13, 2430–2440. [Google Scholar] [CrossRef]
- Logan, B.E. Exoelectrogenic bacteria that power microbial fuel cells. Nat. Rev. Microbiol. 2009, 7, 375–381. [Google Scholar] [CrossRef] [PubMed]
- Ingham, D.; Pop, I. Transport Phenomena in Porous Media, 1st ed.; Elsevier Science: Oxford, UK, 1998. [Google Scholar]
- Vafai, K. Handbook of Porous Media, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Nield, D.A.; Bejan, A. Convection in Porous Media; Springer International Publishing: Cham, Switzerland, 2017. [Google Scholar]
- Chiappini, D.; Bella, G.; Festuccia, A.; Simoncini, A. Direct numerical simulation of an open-cell metallic foam through lattice Boltzmann method. Commun. Comput. Phys. 2015, 18, 707–722. [Google Scholar] [CrossRef]
- Zarghami, A.; Di Francesco, S.; Biscarini, C. Porous substrate effects on thermal flows through a REV-scale finite volume lattice Boltzmann model. Int. J. Mod. Phys. C 2014, 25, 1350086. [Google Scholar] [CrossRef]
- Chiappini, D. Numerical simulation of natural convection in open-cells metal foams. Int. J. Heat Mass Transf. 2018, 117, 527–537. [Google Scholar] [CrossRef]
- Gray, F.; Cen, J.; Boek, E.S. Simulation of dissolution in porous media in three dimensions with lattice Boltzmann, finite-volume, and surface-rescaling methods. Phys. Rev. E 2016, 94, 043320. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Cai, J.; Xin, F.; Huai, X.; Guo, J. Lattice Boltzmann simulation of endothermal catalytic reaction in catalyst porous media. Appl. Therm. Eng. 2013, 50, 1194–1200. [Google Scholar] [CrossRef]
- Li, X.; Chen, J.; Xu, M.; Huai, X.; Xin, F.; Cai, J. Lattice Boltzmann simulation of catalytic reaction in porous media with buoyancy. Appl. Therm. Eng. 2014, 70, 586–592. [Google Scholar] [CrossRef]
- Safi, M.A.; Prasianakis, N.I.; Mantzaras, J.; Lamibrac, A.; Büchi, F.N. Experimental and pore-level numerical investigation of water evaporation in gas diffusion layers of polymer electrolyte fuel cells. Int. J. Heat Mass Transf. 2017, 115, 238–249. [Google Scholar] [CrossRef]
- Zhou, L.; Ku, Z.; Chen, L.; Tao, W. Lattice Boltzmann simulation of gas-solid adsorption processes at pore-scale level. J. Comput. Phys. 2015, 300, 800–813. [Google Scholar] [CrossRef]
- Montessori, A.; Prestininzi, P.; Rocca, M.L.; Falcucci, G.; Succi, S.; Kaxiras, E. Effects of Knudsen diffusivity on the effective reactivity of nanoporous catalyst media. J. Comput. Sci. 2015, 17, 377–383. [Google Scholar] [CrossRef]
- Falcucci, G.; Montessori, A.; Prestininzi, P.; Succi, S.; Barroo, C.; Bell, D.; Biener, M.M.; Biener, J.; Zugic, B.; Kaxiras, E. Mapping reactive flow patters in monolithic nanoporous catalysts. Microfluid. Nanofluid. 2016, 20, 1–13. [Google Scholar] [CrossRef]
- Falcucci, G.; Amati, G.; Krastev, V.K.; Montessori, A.; Yablonsky, G.S.; Succi, S. Heterogeneous catalysis in pulsed-flow reactors with nanoporous gold hollow spheres. Chem. Eng. Sci. 2017, 166, 274–282. [Google Scholar] [CrossRef]
- Montemore, M.M.; Montessori, A.; Succi, S.; Barroo, C.; Falcucci, G.; Bell, D.C.; Kaxiras, E. Effect of nanoscale flows on the surface structure of nanoporous catalysts. J. Chem. Phys. 2017, 146, 214703. [Google Scholar] [CrossRef] [PubMed]
- Bhatnagar, P.; Gross, E.; Krook, M. A model for collision processes in gases. I. Small amplitude processes in charged and neutral one-component systems. Phys. Rev. 1954, 94, 511. [Google Scholar] [CrossRef]
- Qian, Y.H.; d’Humiéres, D.; Lallemand, P. Lattice BGK models for Navier-Stokes equation. EPL (Eur. Lett.) 1992, 17, 479. [Google Scholar] [CrossRef]
- Gunstensen, A.K.; Rothman, D.H.; Zaleski, S.; Zanetti, G. Lattice Boltzmann model of immiscible fluids. Phys. Rev. A 1991, 43, 4320. [Google Scholar] [CrossRef] [PubMed]
- Shan, X.; Doolen, G. Diffusion in a multicomponent lattice Boltzmann equation model. Phys. Rev. E 1996, 54, 3614. [Google Scholar] [CrossRef]
- Yang, J.; Boek, E.S. A comparison study of multi-component Lattice Boltzmann models for flow in porous media applications. Comput. Math. Appl. 2013, 65, 882–890. [Google Scholar] [CrossRef]
- Arcidiacono, S.; Mantzaras, J.; Ansumali, S.; Karlin, I.V.; Frouzakis, C.; Boulouchos, K.B. Simulation of binary mixtures with the lattice Boltzman method. Phys. Rev. E 2006, 74, 056707. [Google Scholar] [CrossRef] [PubMed]
- Arcidiacono, S.; Karlin, I.V.; Mantzaras, J.; Frouzakis, C.E. Lattice Boltzmann model for the simulation of multicomponent mixtures. Phys. Rev. E 2007, 76, 046703. [Google Scholar] [CrossRef] [PubMed]
- Gorban, A.N.; Karlin, I.V. General approach to constructing models of the Boltzmann equation. Phys. A 1994, 206, 401–420. [Google Scholar] [CrossRef]
- Arcidiacono, S.; Mantzaras, J.; Karlin, I.V. Lattice Boltzmann simulation of catalytic reactions. Phys. Rev. E 2008, 78, 046711. [Google Scholar] [CrossRef] [PubMed]
- Kang, J.; Prasianakis, N.I.; Mantzaras, J. Thermal multicomponent lattice Boltzmann model for catalytic reactive flows. Phys. Rev. E 2014, 89, 063310. [Google Scholar] [CrossRef] [PubMed]
- Asinari, P. Semi-implicit-linearized multiple-relaxation-time formulation of lattice Boltzmann schemes for mixture modeling. Phys. Rev. E 2006, 73, 056705. [Google Scholar] [CrossRef] [PubMed]
- Asinari, P.; Luo, L.S. A consistent lattice Boltzmann equation with baroclinic coupling for mixtures. J. Comput. Phys. 2008, 227, 3878–3895. [Google Scholar] [CrossRef]
- Walsh, S.D.C.; Saar, M.O. Interpolated lattice Boltzmann boundary conditions for surface reaction kinetics. Phys. Rev. E 2010, 82, 066703. [Google Scholar] [CrossRef] [PubMed]
- Krastev, V.; Amati, G.; Jannelli, E.; Falcucci, G. Direct Numerical Simulation of SCR Reactors through Kinetic Approach. SAE Tech. Pap. 2016. [Google Scholar] [CrossRef]
- Benjamin, S.F.; Gall, M.; Roberts, C.A. Tuning the standard SCR reaction kinetics to model NO conversion in a diesel engine exhaust SCR catalyst system under steady state conditions in 1D and 3D geometries using ammonia gas as the reductant. SAE Tech. Pap. 2012. [Google Scholar] [CrossRef]
- Madia, G.S. Measures to enhance the NOx conversion in urea-SCR systems for automotive applications. Ph.D. Thesis, Swiss Federal Institute of Technology Zurich, Zurich, Switzerland, 2002. [Google Scholar]
- Adler, J. Ceramic diesel particulate filters. Int. J. Appl. Ceram. Technol. 2005, 2, 429–439. [Google Scholar] [CrossRef]
- Shekhtman, S.O.; Yablonsky, G.S.; Chen, S.; Gleaves, J.T. Thin-zone TAP-reactor—Theory and application. Chem. Eng. Sci. 1999, 54, 4371–4378. [Google Scholar] [CrossRef]
- Shekhtman, S.O.; Yablonsky, G.S.; Gleaves, J.T.; Fushimi, R. “State defining experiment” in chemical kinetics—Primary characterization of catalyst activity in a TAP experiment. Chem. Eng. Sci. 2003, 58, 4843–4859. [Google Scholar] [CrossRef]
- Feres, R.; Yablonsky, G.S.; Mueller, A.; Baernstein, A.; Zheng, X.; Gleaves, J.T. Probabilistic analysis of transport-reaction processes over catalytic particles: Theory and experimental testing. Chem. Eng. Sci. 2009, 64, 568–581. [Google Scholar] [CrossRef]
- Marin, G.B.; Yablonsky, G.S. Kinetics of Chemical Reactions. Decoding Complexity; Wiley-VCH: Weinheim, Germany, 2011. [Google Scholar]
- Suzuki, T.; Tagawa, T.; Aida, T. Fifth unsteady-state processes in catalysis; a special issue of Chemical Engineering Science. Chem. Eng. Sci. 2008, 63, 4889–4890. [Google Scholar] [CrossRef]
- Bird, G.A. Molecular Gas Dynamics and the Direct Simulation of Gas Flows; Clarendon Press: Wotton-under-Edge, UK, 1994. [Google Scholar]
- Nastro, R.A.; Falcucci, G.; Minutillo, M.; Jannelli, E. Microbial Fuel Cells in Solid Waste Valorization: Trends and Applications. In Modelling Trends in Solid and Hazardous Waste Management; Sengupta, D., Agrahari, S., Eds.; Springer: Singapore, 2017. [Google Scholar]
- Nastro, R.A.; Jannelli, N.; Minutillo, M.; Guida, M.; Trifuoggi, M.; Andreassi, L.; Facci, A.L.; Krastev, V.K.; Falcucci, G. Performance Evaluation of Microbial Fuel Cells Fed by Solid Organic Waste: Parametric Comparison between Three Generations. Energy Proc. 2017, 105, 1102–1108. [Google Scholar] [CrossRef]
- Jannelli, N.; Nastro, R.A.; Cigolotti, V.; Minutillo, M.; Falcucci, G. Low pH, high salinity: Too much for microbial fuel cells? Appl. Energy 2017, 192, 543–550. [Google Scholar] [CrossRef]
- Corning Inc. Falcon Tubes and Pipets. Available online: https://www.corning.com/worldwide/en/products/life-sciences/resources/brands/falcon-brand-products/falcon-liquid-handling.html (accessed on 18 January 2018).
- Krastev, V.K.; Falcucci, G. Numerical Simulation of MFC Performance: A Lattice Boltzmann Study. In Proceedings of the 6th European Fuel Cell Technology Applications Conference, Naples, Italy, 12–15 December 2017. [Google Scholar]
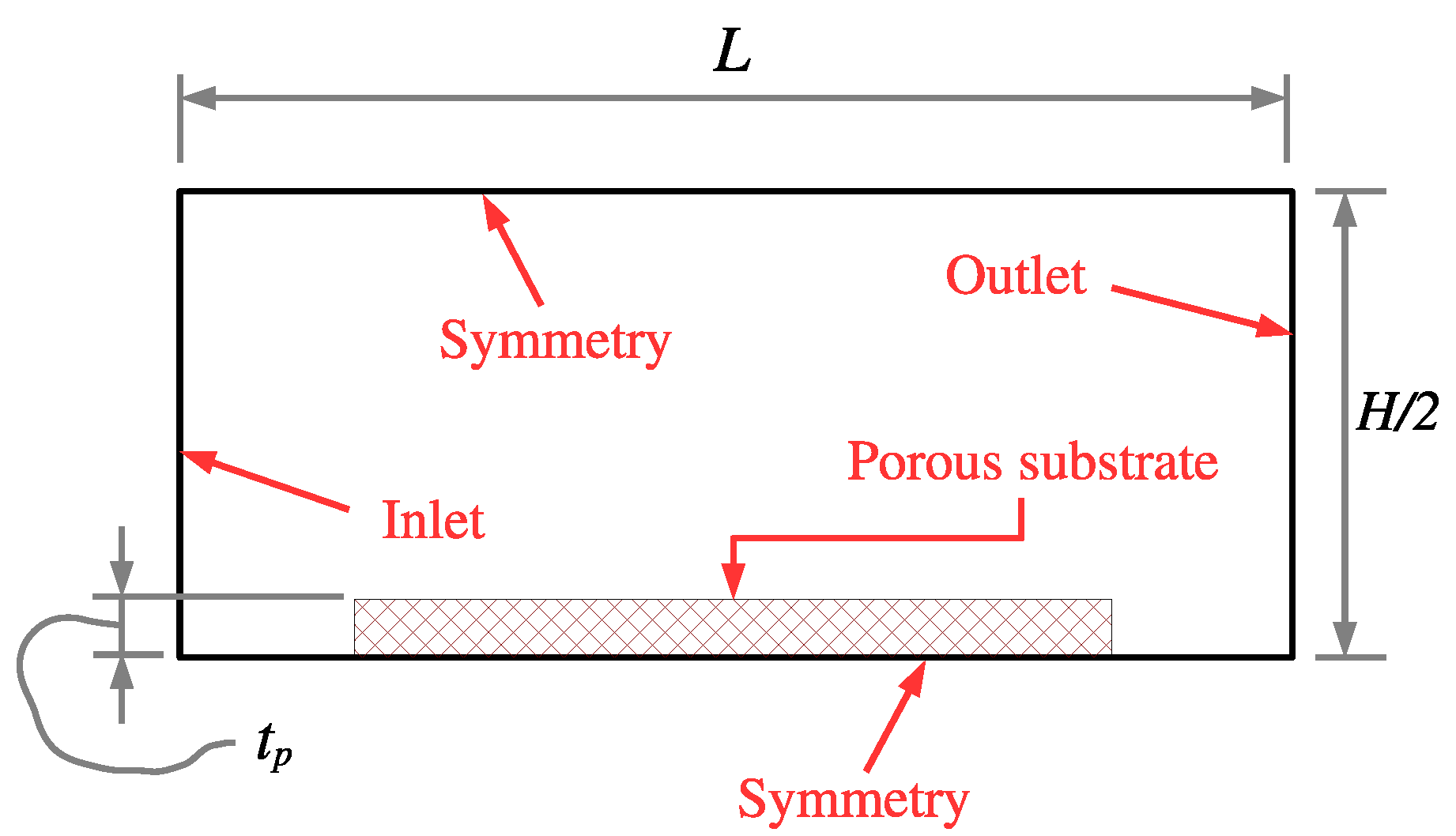
| Parameter | Value |
|---|---|
| Channel length L (mm) | 100 |
| Half-channel height (mm) | 6 |
| Substrate thickness (mm) | |
| Chemical species | 3 |
| Gas mixture viscosity (m/s) | |
| (%) | ∼70 |
| ∼100 | |
| Reaction parameter p | |
| Exhaust gas reference temperature (K) | 623 |
| Parameter | [LU] | |
|---|---|---|
| Length | (m) | 400 |
| Kinematic viscosity | (m/s) | 1.6667 |
| Density | (kg/m) | 1 |
| H concentration | (mol/L) | |
| pH | ||
| Open-Circuit Voltage (OCV) | (V) |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Krastev, V.K.; Falcucci, G. Simulating Engineering Flows through Complex Porous Media via the Lattice Boltzmann Method. Energies 2018, 11, 715. https://doi.org/10.3390/en11040715
Krastev VK, Falcucci G. Simulating Engineering Flows through Complex Porous Media via the Lattice Boltzmann Method. Energies. 2018; 11(4):715. https://doi.org/10.3390/en11040715
Chicago/Turabian StyleKrastev, Vesselin Krassimirov, and Giacomo Falcucci. 2018. "Simulating Engineering Flows through Complex Porous Media via the Lattice Boltzmann Method" Energies 11, no. 4: 715. https://doi.org/10.3390/en11040715
APA StyleKrastev, V. K., & Falcucci, G. (2018). Simulating Engineering Flows through Complex Porous Media via the Lattice Boltzmann Method. Energies, 11(4), 715. https://doi.org/10.3390/en11040715






