Availability of Ambient RF Energy in d-Dimensional Wireless Networks
Abstract
:1. Introduction
1.1. Related Works
1.2. Contributions
- By modeling transmitters as a d-dimensional large-scale network, we derive the mean and the CDF of the harvested-energy under BPL and Rayleigh fading model using tools from stochastic geometry. The networks with and without interference control are separately considered. Our unified framework is general and the derived results can be applied to 1-D, 2-D and 3-D networks.
- Considering the practical constraint imposed by the RF harvesting circuit, we propose two metrics: the EEHP and the SMHE to measure the availability of the ambient RF energy. Other works like [11] have proposed metric such as power outage probability. However, the threshold they refer to in their work is the circuit power consumption, not the suggested turn-on power of the harvester (considered in this paper).
- We derive compact expressions for the CDF and mean of the RF harvested energy including some closed-form expressions for a special case (which is practical in the real environment). The numerical method to calculate the RF energy distribution is given. To gain insight into the results, we derive and analyze the lower bound of distribution function and the upper bound of the mean. These results can be readily used to evaluate the communication capacity of wireless powered nodes.
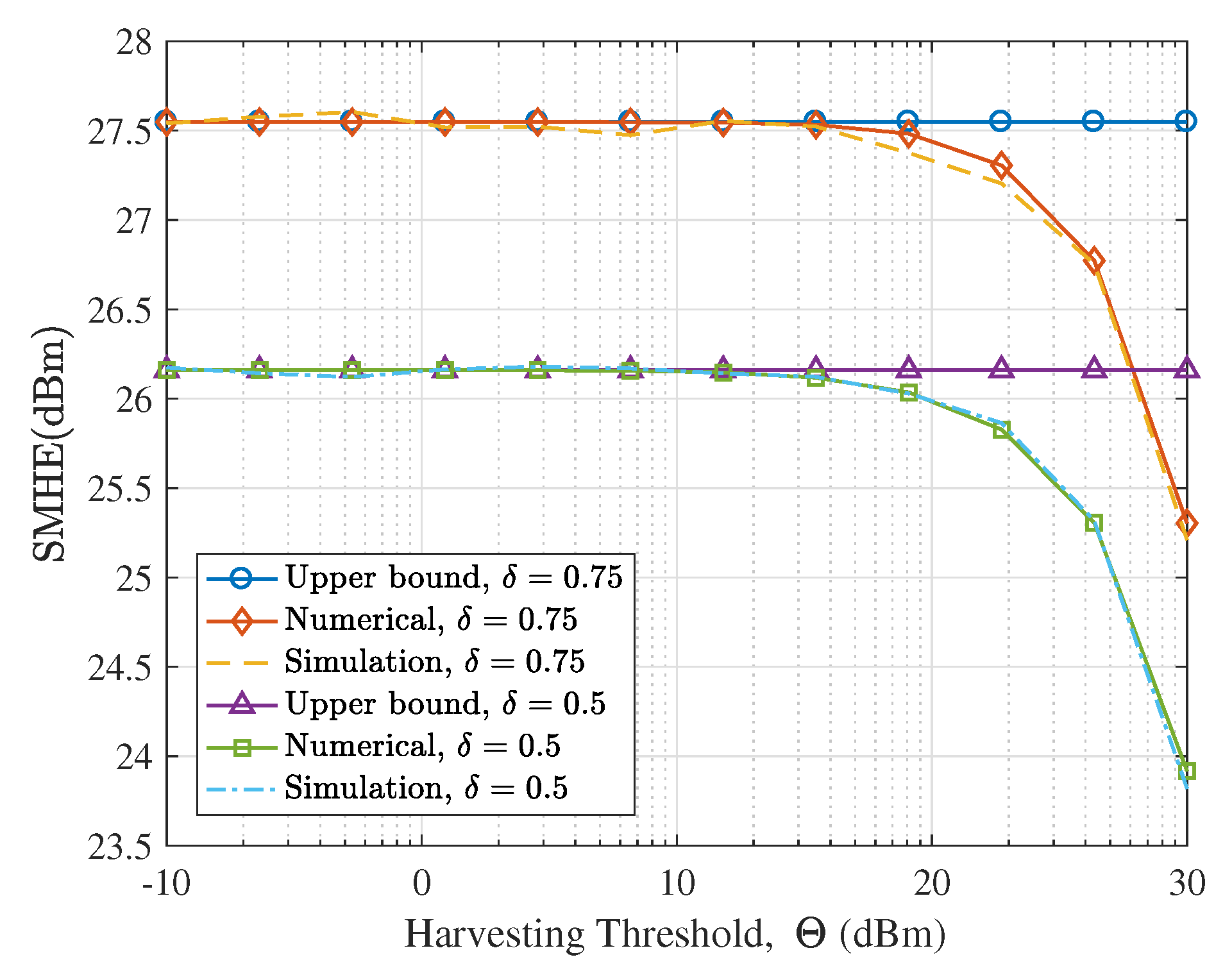
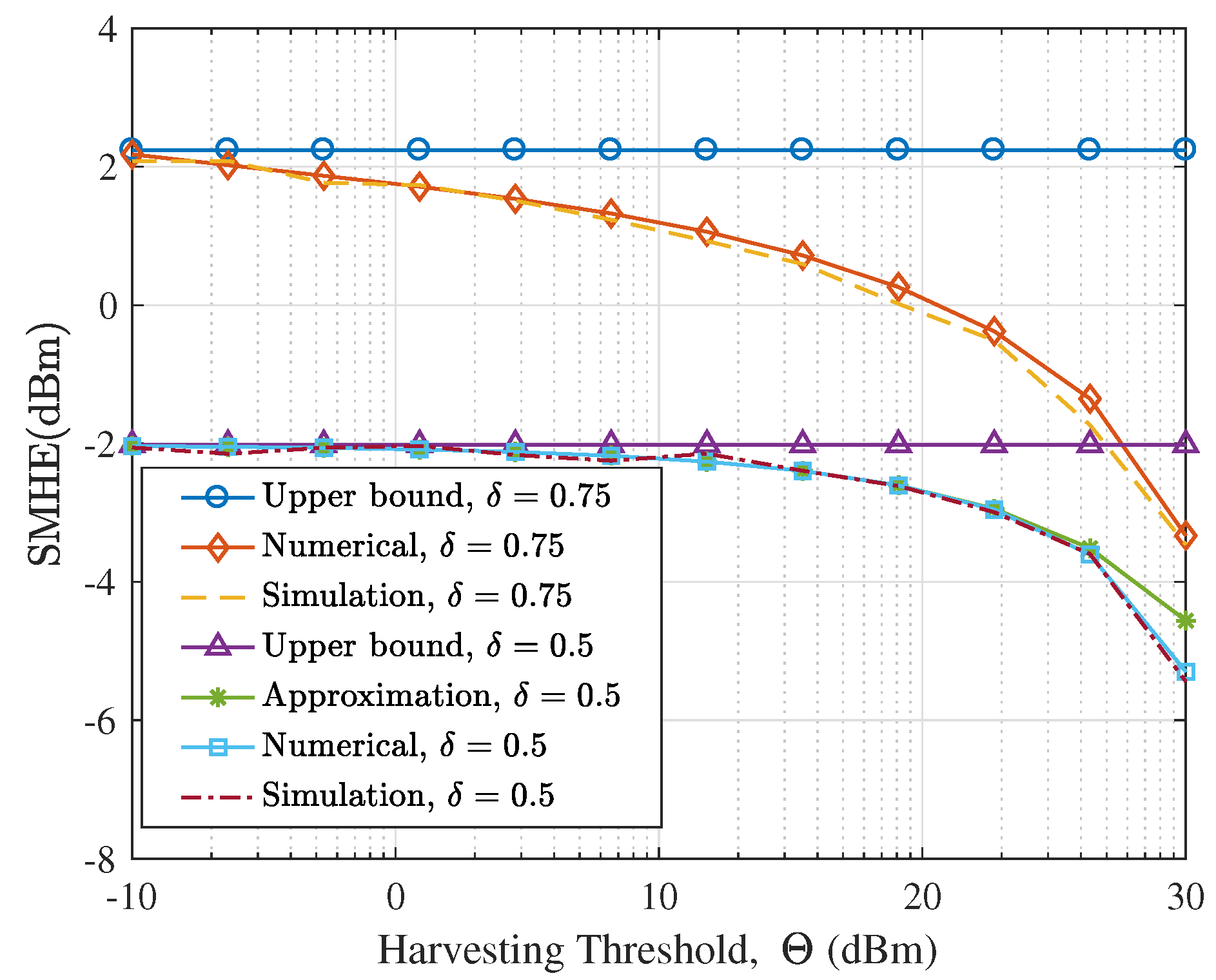
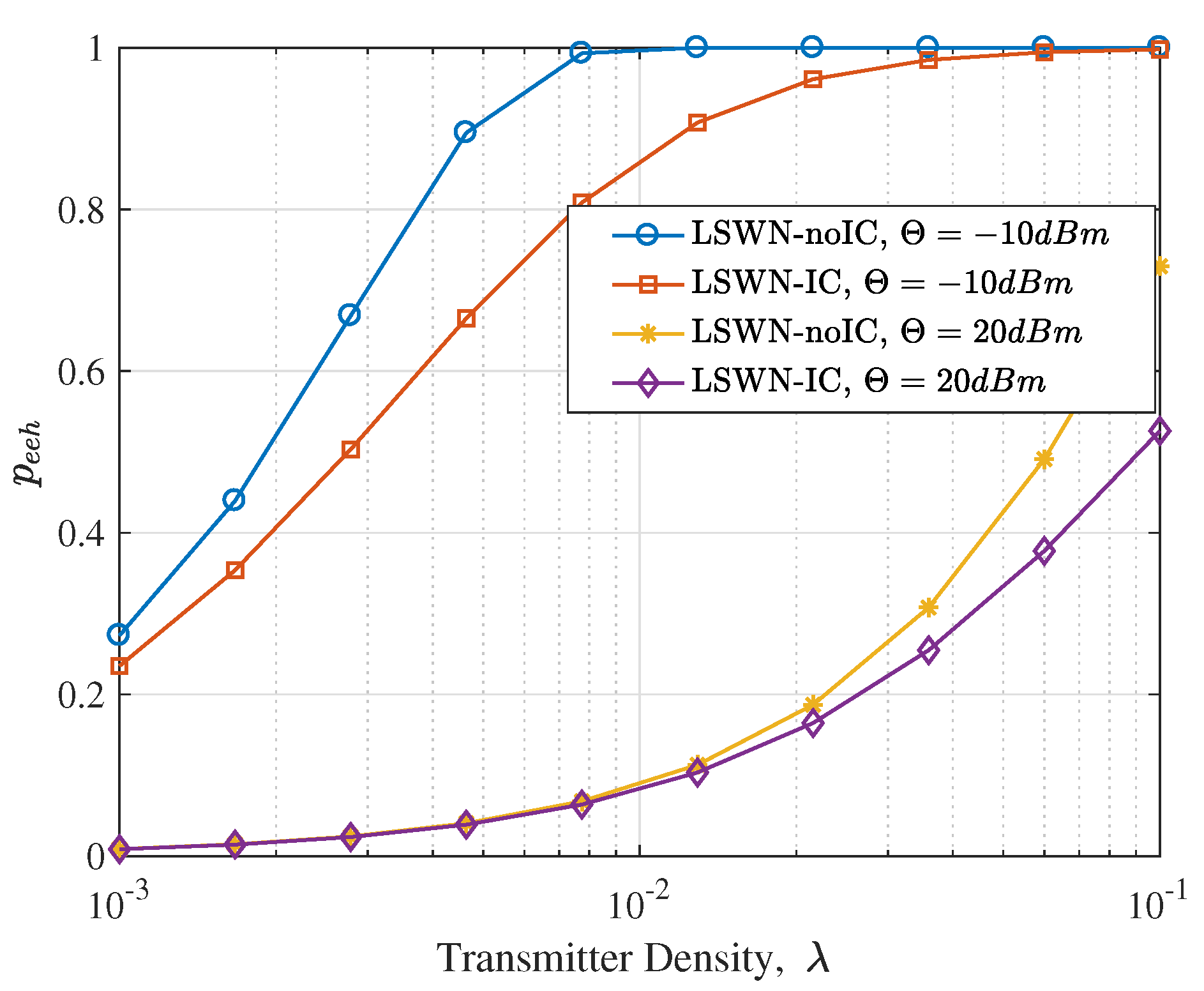
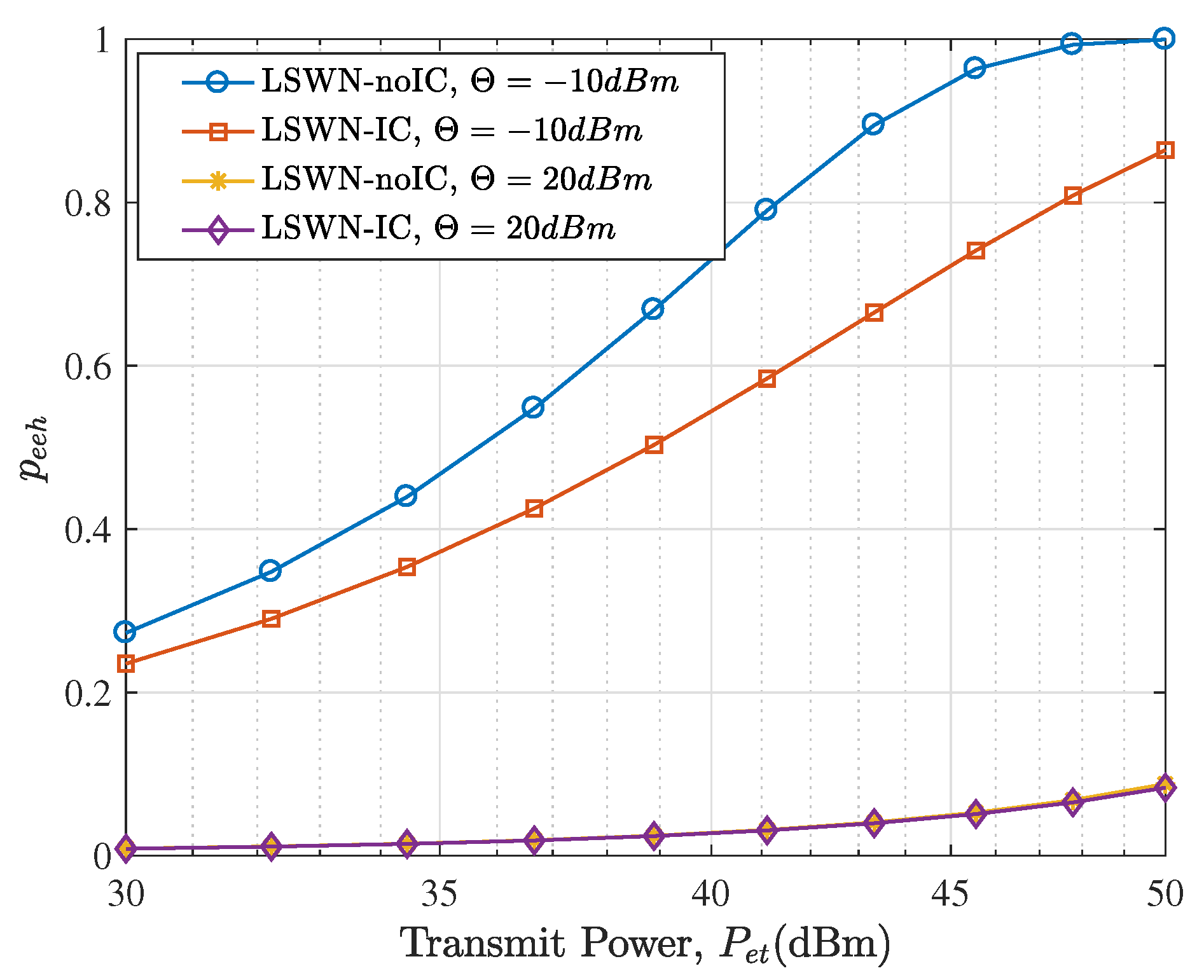
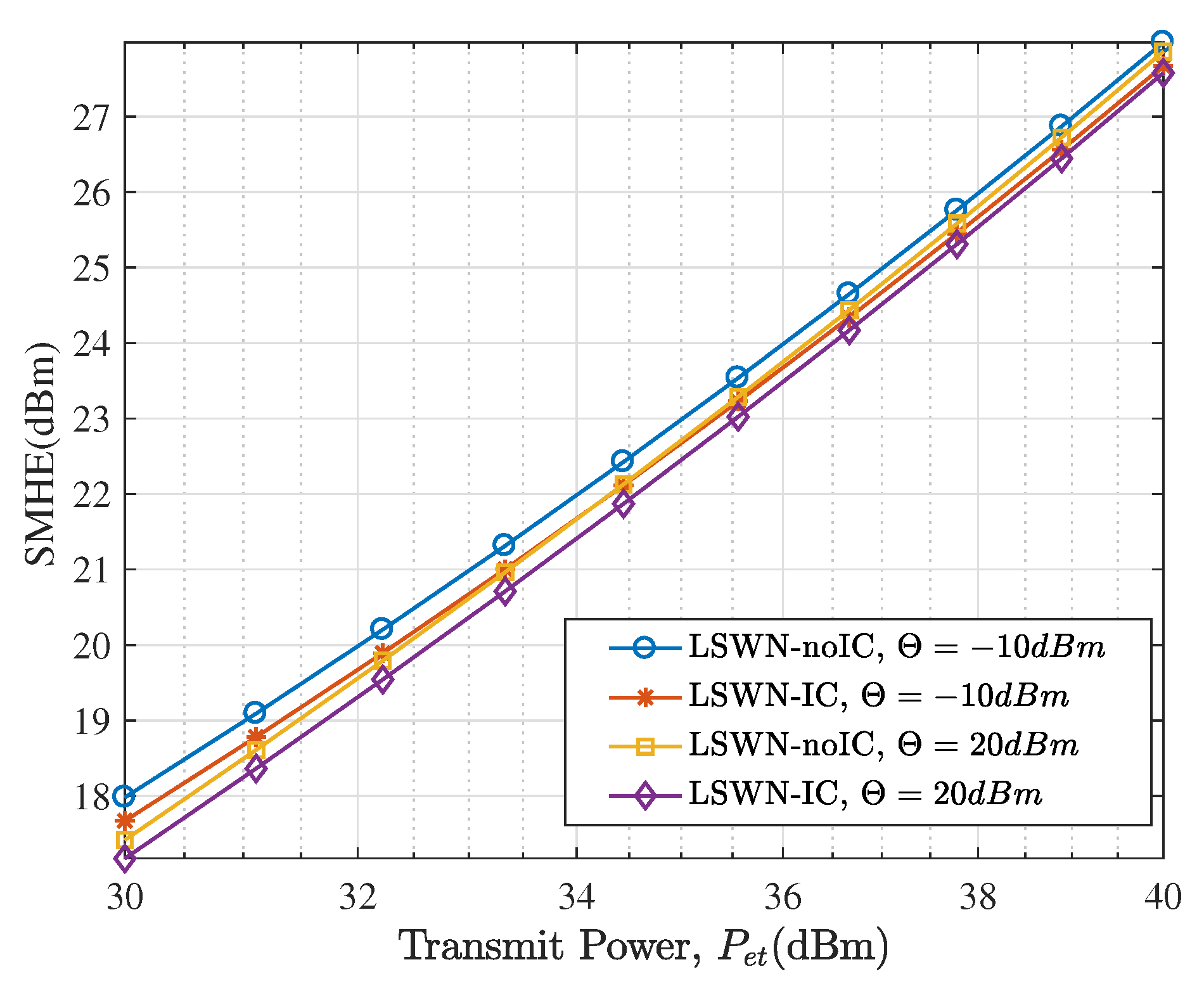
- We validate the theoretical analysis with Monte Carlo simulations. The proposed bounds of the EEHP and the SMHE for different settings are verified. We show that while the harvesting threshold has a significant effect on the EEHP, it has a negligible impact on the SMHE, especially for dense networks. Also, we illustrate that in terms of improving the SMHE, the increase of transmitter density is more efficient than increasing transmit power. Last but not the least, we show that interference control has a trivial effect on RF energy harvesting performance for a sparse network; Since the performance of LSWN-IC and LSWN-noIC is comparable in sparse networks, the mathematically tractable expressions for SMHE and EEHP for LSWN-IC can serve as surrogate metrics to analyze LSWN-noIC.
2. System Model and Performance Metrics
2.1. System Model
2.2. Metrics of Availability
3. RF Energy Harvesting in LSWN-IC
3.1. Distribution of Received Power
3.2. Spatial Mean Harvestable Energy
4. RF Energy Harvesting in LSWN-noIC
4.1. Laplace Transform of the Received Power
4.2. Distribution of Received Power
4.3. Spatial Mean Harvestable Energy
5. Simulation Results and Discussion
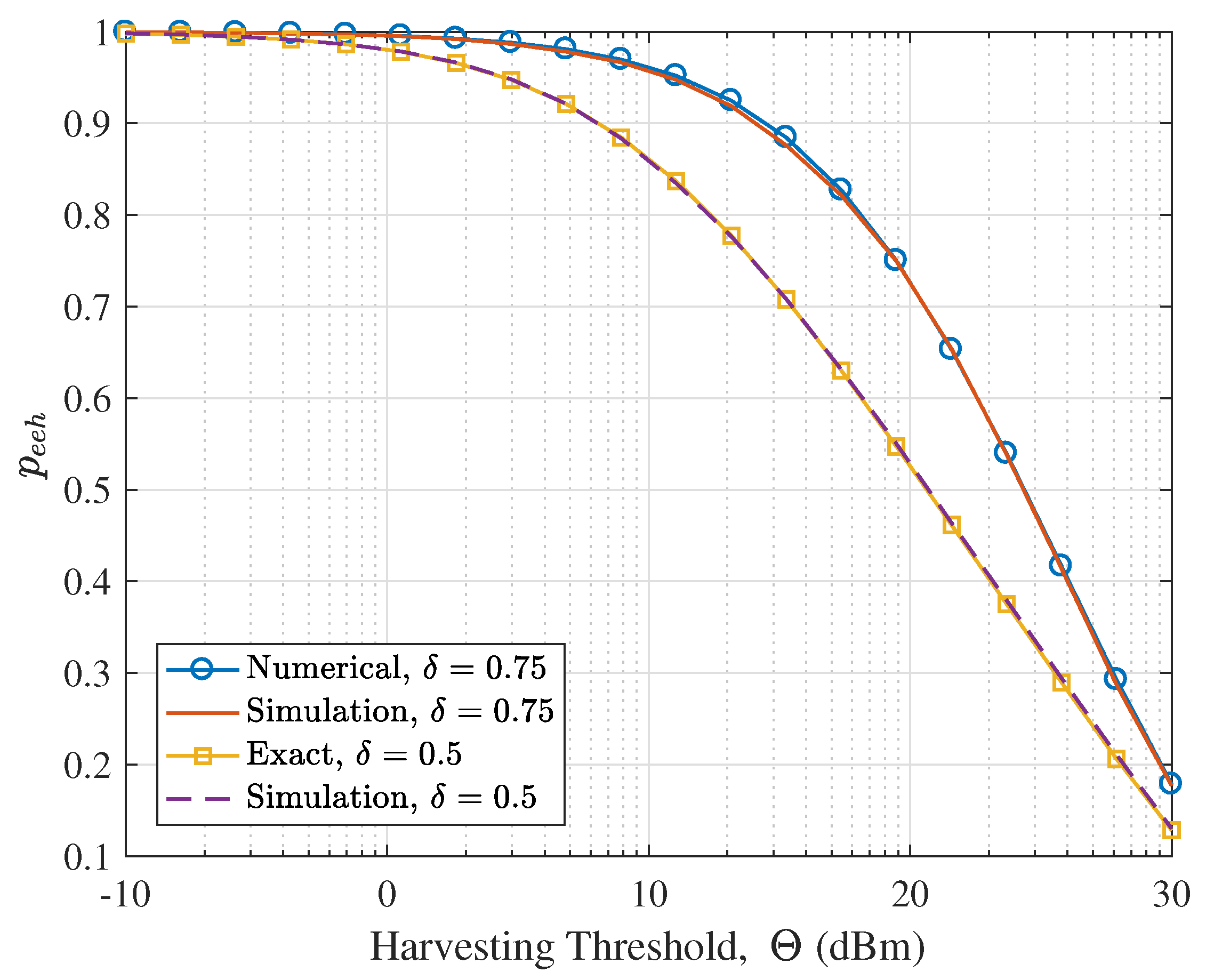
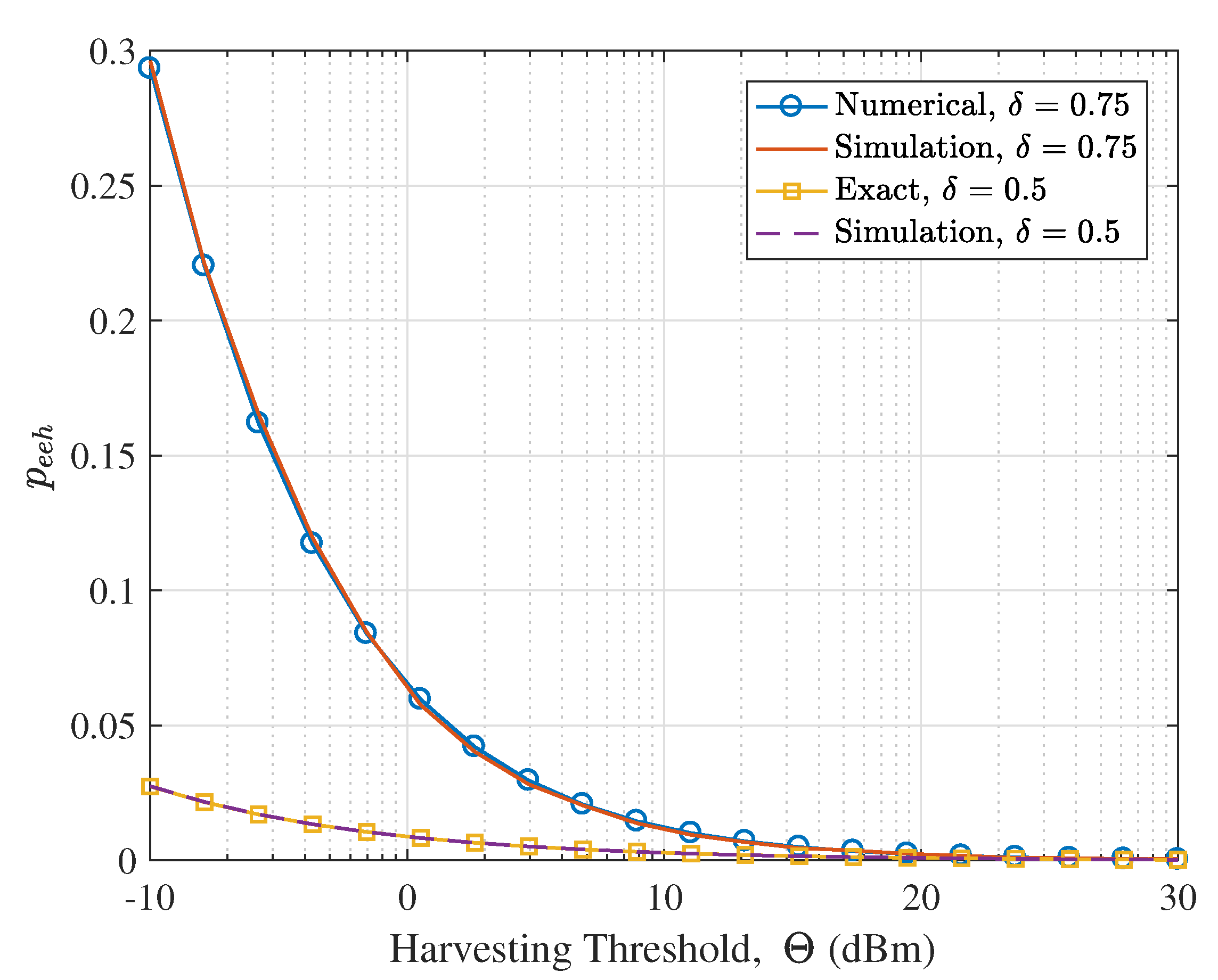
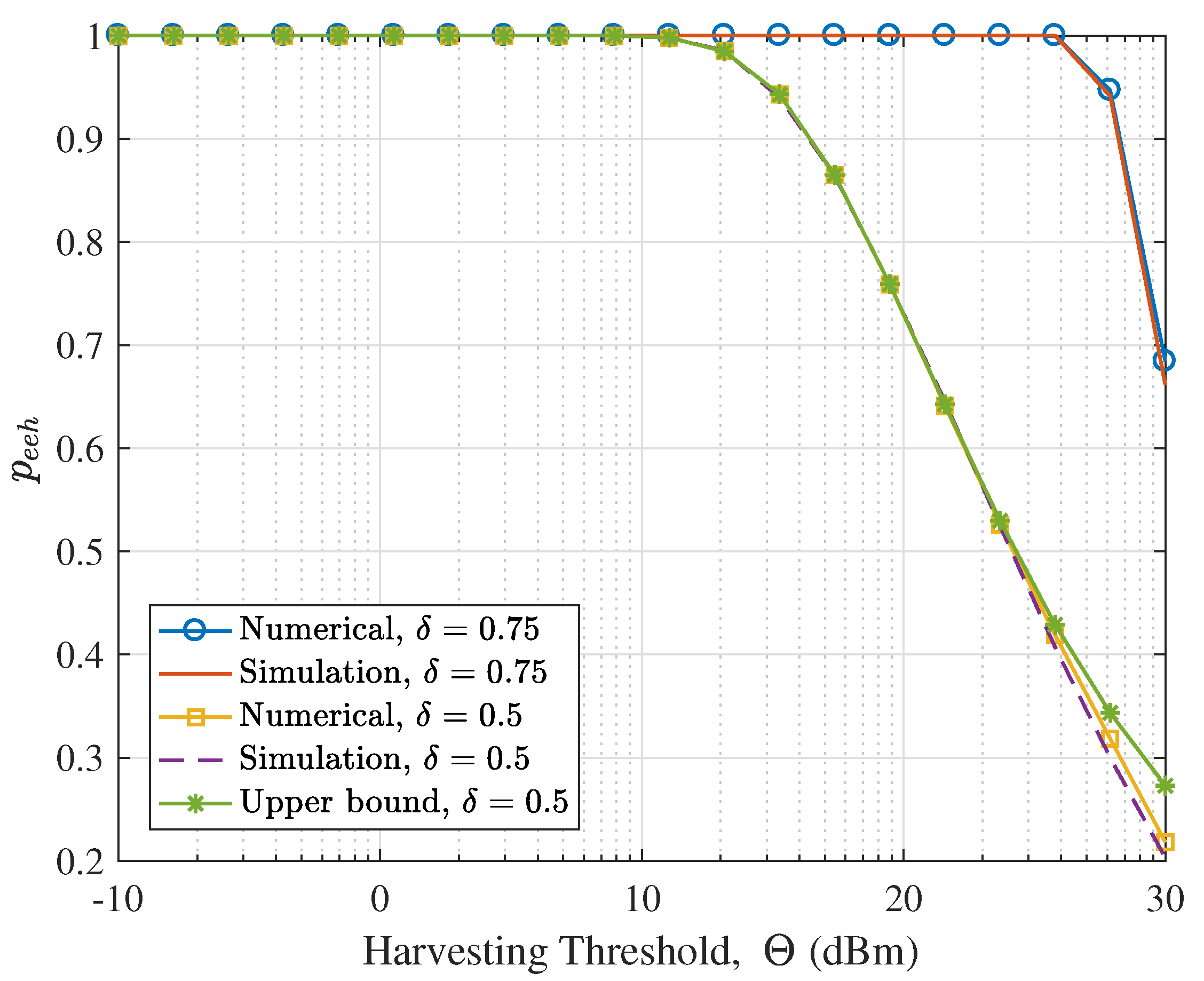
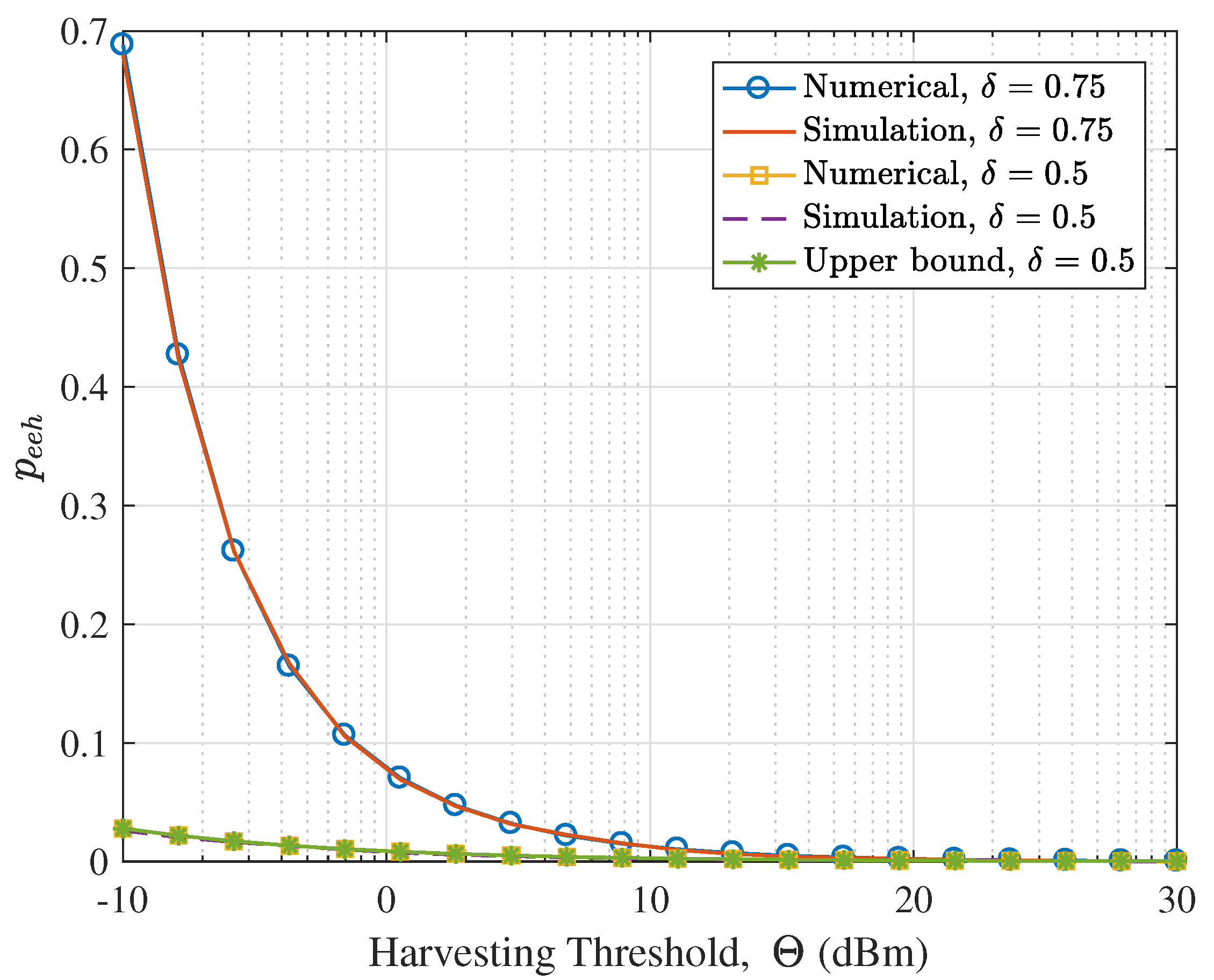
5.1. Verifying the Proposed Analysis Framework
5.2. Availability Analysis of Ambient RF Energy
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Mekikis, P.V.; Kartsakli, E.; Antonopoulos, A.; Alonso, L.; Verikoukis, C. Connectivity Analysis in Clustered Wireless Sensor Networks Powered by Solar Energy. IEEE Trans. Wireless Commun. 2018, PP, 1. [Google Scholar] [CrossRef]
- Bi, S.; Ho, C.K.; Zhang, R. Wireless powered communication: Opportunities and challenges. IEEE Commun. Mag. 2015, 53, 117–125. [Google Scholar] [CrossRef]
- Huang, K.; Zhong, C.; Zhu, G. Some new research trends in wirelessly powered communications. IEEE Wirel. Commun. 2016, 23, 19–27. [Google Scholar] [CrossRef]
- Lee, S.; Zhang, R.; Huang, K. Opportunistic wireless energy harvesting in cognitive radio networks. IEEE Trans. Wirel. Commun. 2013, 12, 4788–4799. [Google Scholar] [CrossRef]
- Krikidis, I. Simultaneous information and energy transfer in large-scale networks with/without relaying. IEEE Trans. Commun. 2014, 62, 900–912. [Google Scholar] [CrossRef]
- Xia, H.; Natarajan, B.; Liu, C. Feasibility of simultaneous information and energy transfer in LTE-A small cell networks. In Proceedings of the 2014 IEEE 11th Consumer Communications and Networking Conference (CCNC), Las Vegas, NV, USA, 10–13 January 2014; pp. 20–25. [Google Scholar]
- Che, Y.L.; Duan, L.; Zhang, R. Spatial Throughput Maximization of Wireless Powered Communication Networks. IEEE J. Sel. Areas Commun. 2015, 33, 1534–1548. [Google Scholar] [CrossRef]
- Mekikis, P.V.; Lalos, A.S.; Antonopoulos, A.; Alonso, L.; Verikoukis, C. Wireless Energy Harvesting in Two-Way Network Coded Cooperative Communications: A Stochastic Approach for Large Scale Networks. IEEE Commun. Lett. 2014, 18, 1011–1014. [Google Scholar] [CrossRef]
- Inaltekin, H.; Wicker, S.B. The behavior of unbounded path-loss models and the effect of singularity on computed network interference. In Proceedings of the 4th Annual IEEE Communications Society Conference on Sensor, Mesh and Ad Hoc Communications and Networks (SECON’07), San Diego, CA, USA, 18–21 June 2007; pp. 431–440. [Google Scholar]
- Zhang, R.; Ho, C.K. MIMO Broadcasting for Simultaneous Wireless Information and Power Transfer. IEEE Trans. Wirel. Commun. 2013, 12, 1989–2001. [Google Scholar] [CrossRef]
- Flint, I.; Lu, X.; Privault, N.; Niyato, D.; Wang, P. Performance analysis of ambient RF energy harvesting: A stochastic geometry approach. In Proceedings of the 2014 IEEE Global Communications Conference, Austin, TX, USA, 8–12 December 2014; pp. 1448–1453. [Google Scholar]
- Sakr, A.H.; Hossain, E. Analysis of K-Tier Uplink Cellular Networks With Ambient RF Energy Harvesting. IEEE J. Sel. Areas Commun. 2015, 33, 2226–2238. [Google Scholar] [CrossRef]
- Oliveira, D.; Oliveira, R. Modeling energy availability in RF Energy Harvesting Networks. In Proceedings of the 2016 International Symposium on Wireless Communication Systems (ISWCS), Poznań, Poland, 20–23 September 2016; pp. 383–387. [Google Scholar]
- Wang, L.; Wong, K.K.; Heath, R.W.; Yuan, J. Wireless Powered Dense Cellular Networks: How Many Small Cells Do We Need? IEEE J. Sel. Areas Commun. 2017, 35, 2010–2024. [Google Scholar] [CrossRef]
- Khan, T.A.; Heath, R.W. Wireless Power Transfer in Millimeter Wave Tactical Networks. IEEE Signal Process. Lett. 2017, 24, 1284–1287. [Google Scholar] [CrossRef]
- Zewde, T.A.; Gursoy, M.C. Energy efficiency analysis for wireless-powered cellular networks. In Proceedings of the 2017 51st Annual Conference on Information Sciences and Systems (CISS), Baltimore, MD, USA, 22–24 March 2017; pp. 1–6. [Google Scholar]
- Ma, X.; Zhang, J.; Yin, X.; Trivedi, K.S. Design and Analysis of a Robust Broadcast Scheme for VANET Safety-Related Services. IEEE Trans. Veh. Technol. 2012, 61, 46–61. [Google Scholar] [CrossRef]
- Guo, J.; Zhang, Y.; Chen, X.; Yousefi, S.; Guo, C.; Wang, Y. Spatial Stochastic Vehicle Traffic Modeling for VANETs. IEEE Trans. Intell. Transp. Syst. 2018, 19, 416–425. [Google Scholar] [CrossRef]
- Gupta, P.; Kumar, P.R. Internets in the sky: Capacity of 3D wireless networks. In Proceedings of the 39th IEEE Conference on Decision and Control, Sydney, Australia, 12–15 Decemver 2000; Volume 3, pp. 2290–2295. [Google Scholar]
- Ng, S.C.; Mao, G.; Anderson, B.D. Critical density for connectivity in 2D and 3D wireless multi-hop networks. IEEE Tran. Wirel. Commun. 2013, 12, 1512–1523. [Google Scholar] [CrossRef]
- Omri, A.; Hasna, M.O. Modeling and Performance Analysis of 3-D Heterogeneous Networks With Interference Management. IEEE Commun. Lett. 2017, 21, 1787–1790. [Google Scholar] [CrossRef]
- Zander, J. Distributed cochannel interference control in cellular radio systems. IEEE Trans. Veh. Technol. 1992, 41, 305–311. [Google Scholar] [CrossRef]
- Chiu, S.N.; Stoyan, D.; Kendall, W.S.; Mecke, J. Stochastic Geometry and Its Applications; John Wiley & Sons, Ltd: Chichester, UK, 2013. [Google Scholar]
- Andrews, J.G.; Baccelli, F.; Ganti, R.K. A Tractable Approach to Coverage and Rate in Cellular Networks. IEEE Trans. Commun. 2011, 59, 3122–3134. [Google Scholar] [CrossRef]
- Rappaport, T.S. Wireless Communications: Principles and Practice, 2nd ed.; Pearson Education: Delhi, India, 2010. [Google Scholar]
- Haenggi, M.; Ganti, R.K. Interference in Large Wireless Networks; Now Publishers Inc.: Breda, The Netherlands, 2009. [Google Scholar]
- Abeywickrama, S.; Samarasinghe, T.; Ho, C.K.; Yuen, C. Wireless Energy Beamforming Using Received Signal Strength Indicator Feedback. IEEE Trans. Signal Process. 2018, 66, 224–235. [Google Scholar] [CrossRef]
- Valenta, C.R.; Durgin, G.D. Harvesting wireless power: Survey of energy-harvester conversion efficiency in far-field, wireless power transfer systems. IEEE Microw. Mag. 2014, 15, 108–120. [Google Scholar]
- Nintanavongsa, P.; Muncuk, U.; Lewis, D.R.; Chowdhury, K.R. Design optimization and implementation for RF energy harvesting circuits. IEEE J. Emerg. Sel. Top. Circuits Syst. 2012, 2, 24–33. [Google Scholar] [CrossRef]
- Haenggi, M. On distances in uniformly random networks. IEEE Trans. Inf. Theory 2005, 51, 3584–3586. [Google Scholar] [CrossRef]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products (Seventh Edition). Table Integrals 2007, 103, 1161–1171. [Google Scholar]
- Lowen, S.B.; Teich, M.C. Power-law shot noise. IEEE Trans. Inf. Theory 1990, 36, 1302–1318. [Google Scholar] [CrossRef]
- Srinivasa, S.; Haenggi, M. Modeling interference in finite uniformly random networks. In Proceedings of the International Workshop on Information Theory for Sensor Networks (WITS’07), Santa Fe, NM, USA, 16–21 June 2007; pp. 1–12. [Google Scholar]
- Feller, W. An Introduction to Probability Theory and Its Applications; Wiley: New York, NY, USA, 1968; Volume 2, pp. 435–436. [Google Scholar]
- Abate, J.; Whitt, W. A unified framework for numerically inverting Laplace transforms. INFORMS J. Comput. 2006, 18, 408–421. [Google Scholar] [CrossRef]
- Kamel, M.; Hamouda, W.; Youssef, A. Ultra-Dense Networks: A Survey. IEEE Commun. Surv. Tutor. 2016, 18, 2522–2545. [Google Scholar] [CrossRef]
- Lu, W.; Di Renzo, M. Stochastic Geometry Modeling of Cellular Networks: Analysis, Simulation and Experimental Validation. In Proceedings of the 18th ACM International Conference on Modeling, Analysis and Simulation of Wireless and Mobile Systems (MSWiM’15), Cancun, Mexico, 2–6 November 2015; ACM: New York, NY, USA, 2015; pp. 179–188. [Google Scholar]
- Haenggi, M. Stochastic Geometry for Wireless Networks; Cambridge University Press: New York, NY, USA, 2012. [Google Scholar]




| Literature | Network Model | Large Scale Fading Model | Small Scale Fading Model | RF Energy Harvesting Performance |
|---|---|---|---|---|
| Flint et al. [11] | Ginibre -DPP in | BPL | Not considered | Exact mean; Upper bound for CCDF |
| Sakr and Hossain [12] | K-tier HPPP in | UBPL | Rayleigh | CDF in integration form; closed-form for |
| Oliveira and Oliveira [13] | finite HPPP in | BPL | Rayleigh | Approximated CDF in infinite series, modeled by generalized Gamma distribution and Normal distribution |
| Wang et al. [14] | 2-tier HPPP in | Sub-6GHz: UBPL/ mmWave:Blockage path loss model | Sub-6GHz: Nakagami; /mmWave: not considered | sub-6GHz:CDF in integral form; exact form for infinite antennas number/ mmWave: CDF in integral form |
| Khan and Heath [15] | HPPP in | BPL | Nakagami | CDF in integral of Gamma function |
| Zewde and Gursoy [16] | HPPP in | BPL | Rayleigh | Mean in closed-form |
| Our work | HPPP in | BPL | Rayleigh | LSWN-IC: CDF in integral form; closed-form for . Mean in integral form; exact upper bound / LSWN-noIC: CDF in inverse Laplace function; lower bound for . Mean in inverse Laplace function; exact upper bound and compact approximation for . |
| Network Type | EEHP | SMHE |
|---|---|---|
| LSWN-IC | Integral form in (18), exact form in (19) for | Integral form in (20); Upper bound in (24) |
| LSWN-noIC | ILT of (35); Compact upper bound in (40) for | Compound expression of ILT in (46); Upper bound in (54) and accurate approximation in (55) for |
| Density | ||
|---|---|---|
| = 0.1 | L = 1000, N = | L = 200, N = |
| = 0.0001 | L = 1000, N = | L = 400, N = |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xia, H.; Li, Y.; Zhang, H.; Natarajan, B. Availability of Ambient RF Energy in d-Dimensional Wireless Networks. Energies 2018, 11, 668. https://doi.org/10.3390/en11030668
Xia H, Li Y, Zhang H, Natarajan B. Availability of Ambient RF Energy in d-Dimensional Wireless Networks. Energies. 2018; 11(3):668. https://doi.org/10.3390/en11030668
Chicago/Turabian StyleXia, Hongxing, Yongzhao Li, Hailin Zhang, and Balasubramaniam Natarajan. 2018. "Availability of Ambient RF Energy in d-Dimensional Wireless Networks" Energies 11, no. 3: 668. https://doi.org/10.3390/en11030668
APA StyleXia, H., Li, Y., Zhang, H., & Natarajan, B. (2018). Availability of Ambient RF Energy in d-Dimensional Wireless Networks. Energies, 11(3), 668. https://doi.org/10.3390/en11030668




