A Semi-Analytical Method for Simulating Two-Phase Flow Performance of Horizontal Volatile Oil Wells in Fractured Carbonate Reservoirs
Abstract
:1. Introduction
2. Methodology
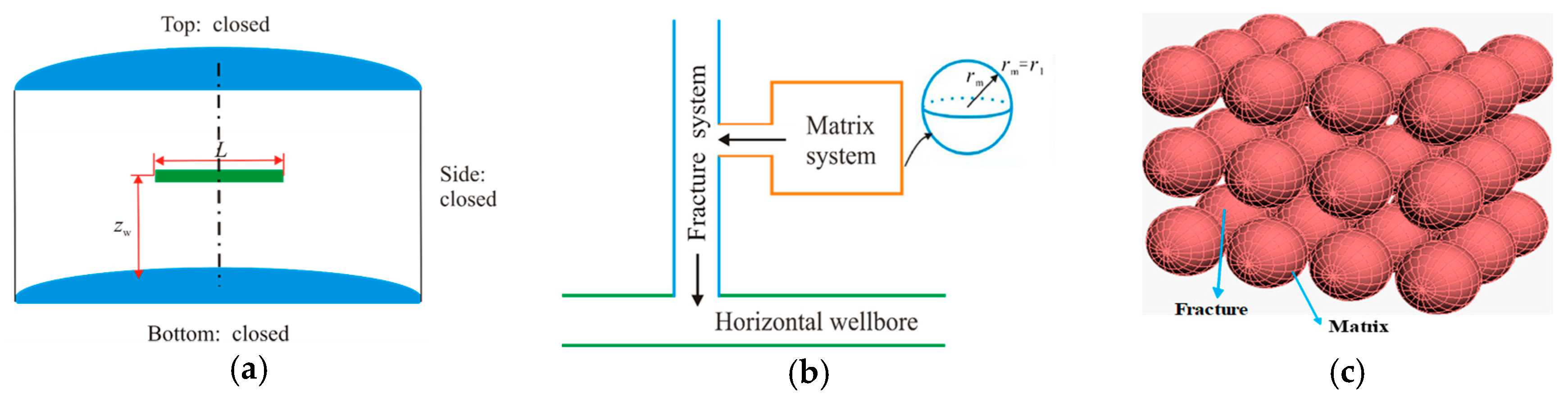
2.1. Model Assumption
2.2. Mathematical Model
2.3. Solution to Mathematical Model
2.3.1. Linearization of Flow Equation
2.3.2. Solution of Equations
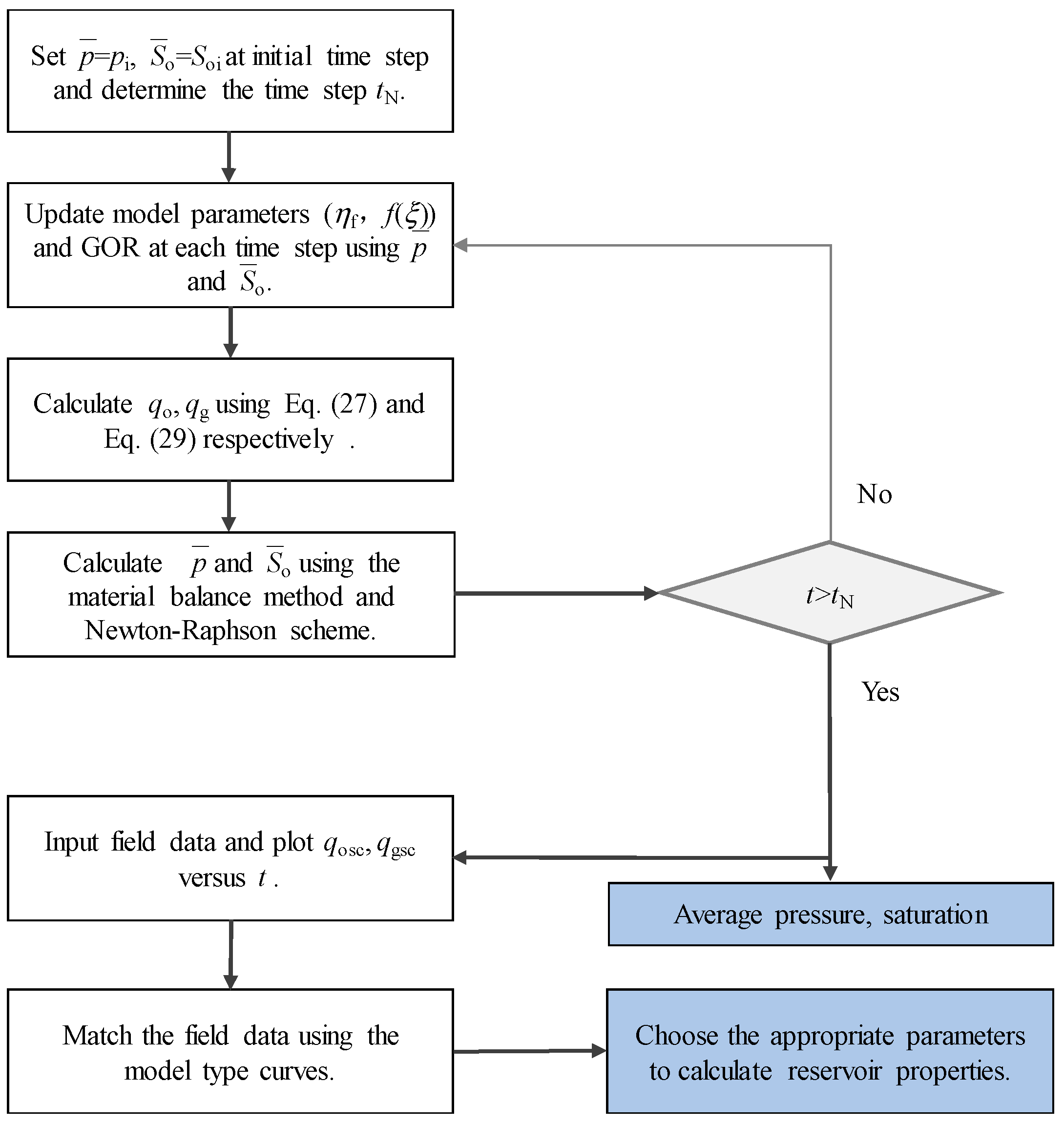
2.4. Work Flow of Two-Phase Flow Analysis
2.4.1. Produced GOR Calculation
2.4.2. Material-Balance Equations
2.4.3. History Matching and Production Prediction
3. Results and Discussion
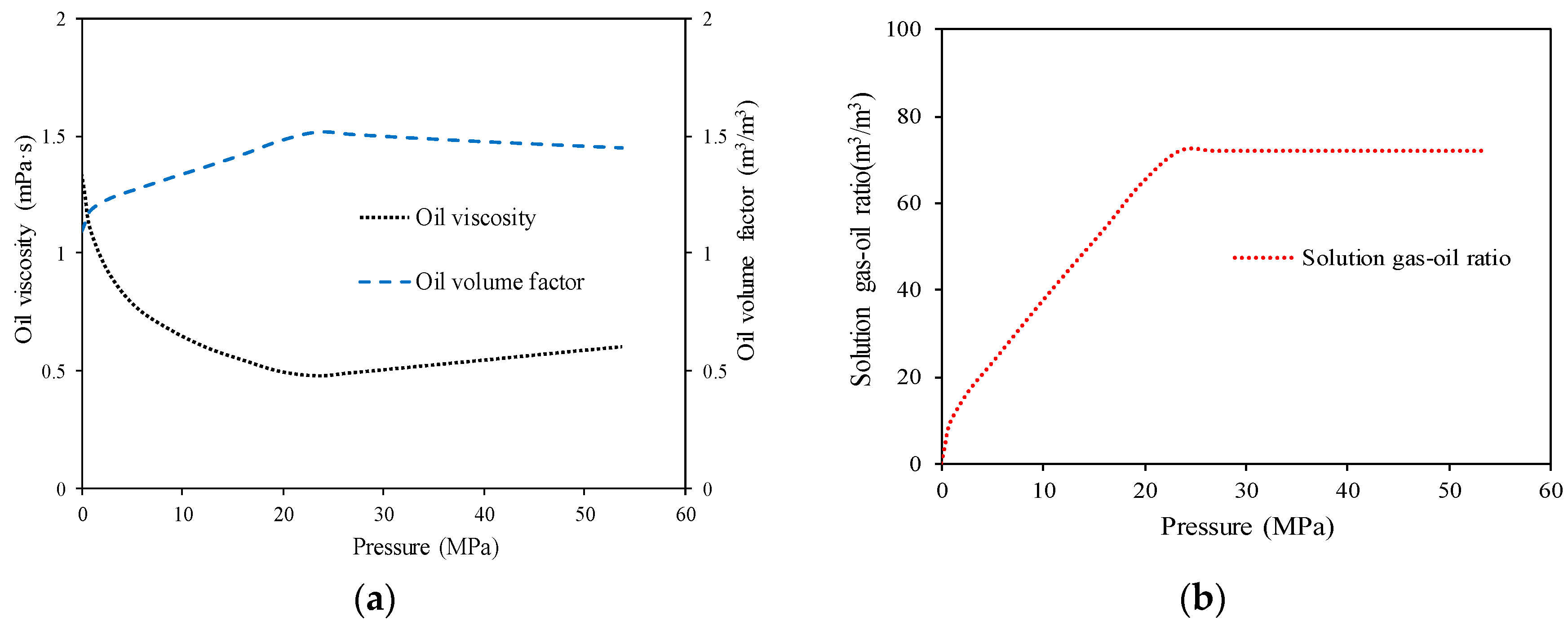
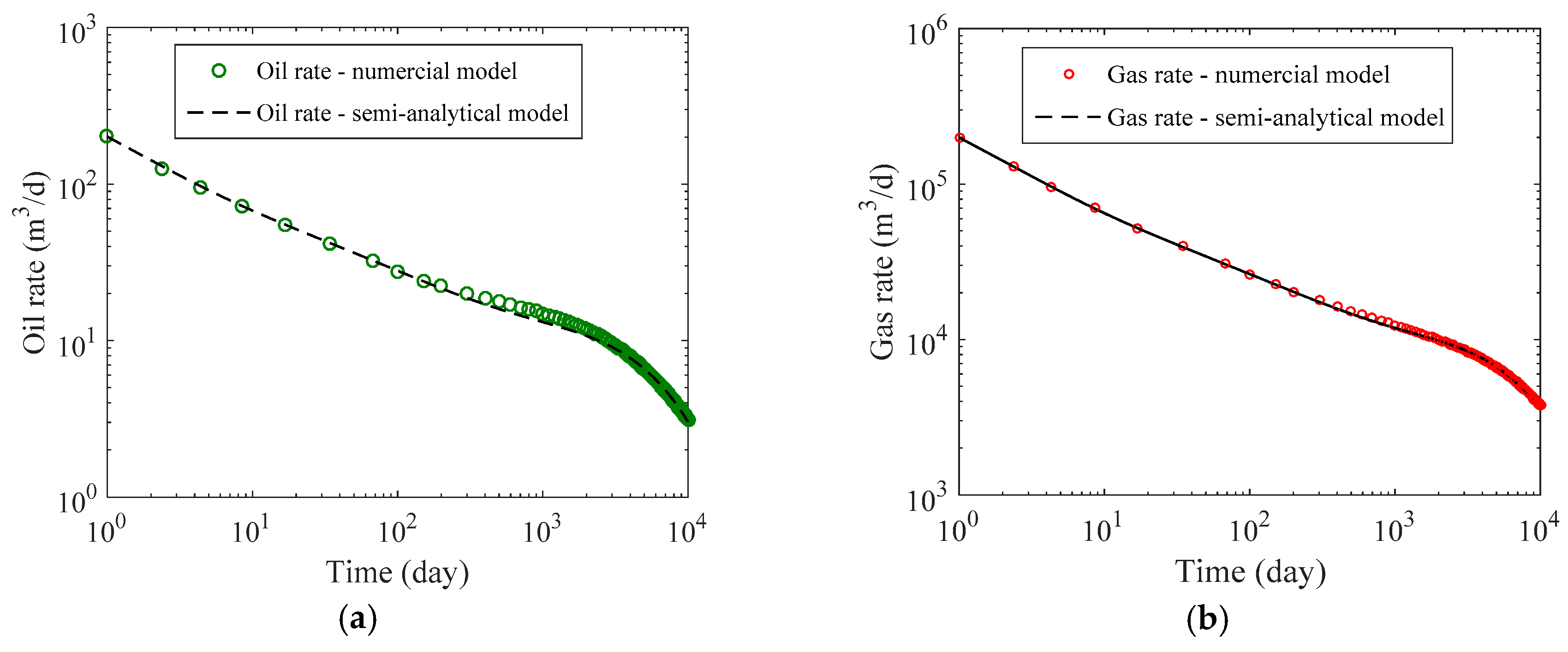
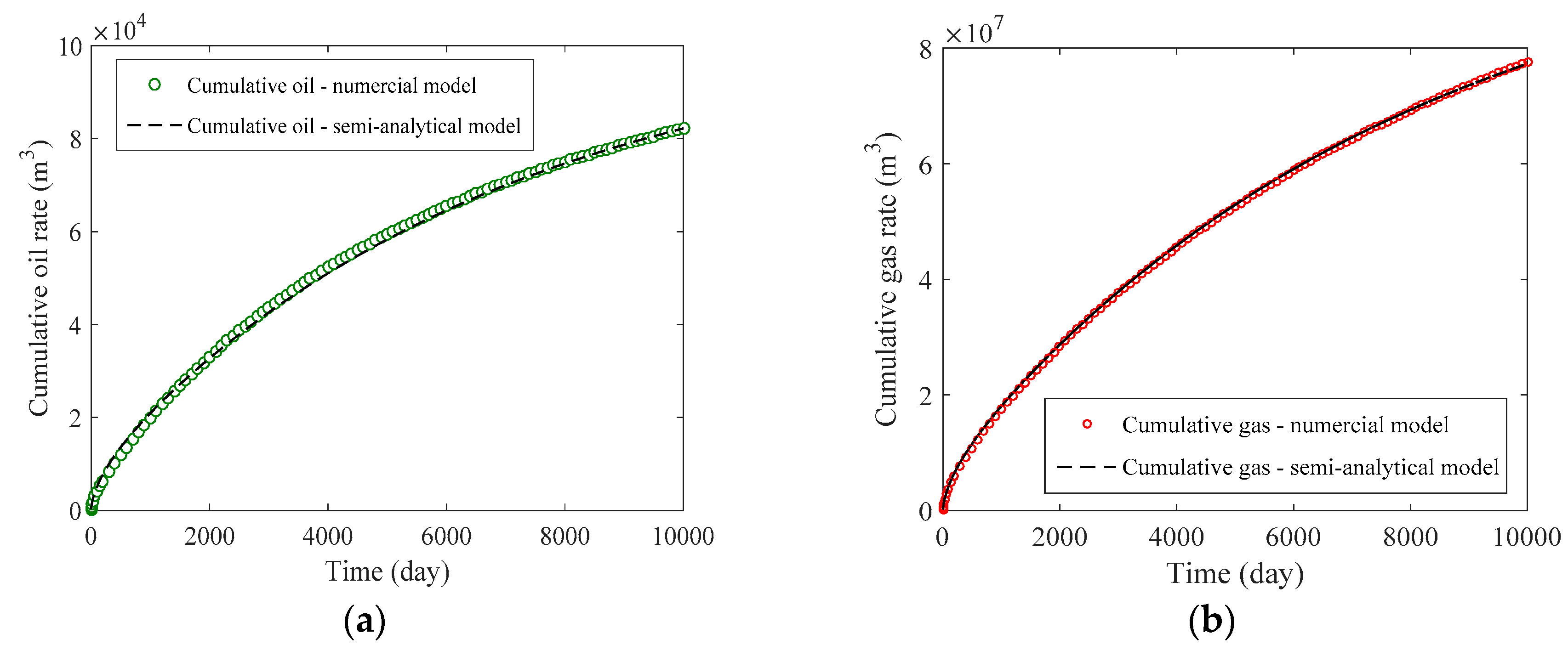
3.1. Model Verification
3.2. Sensitivity Analysis
3.2.1. Permeability Stress Sensitivity of Fracture
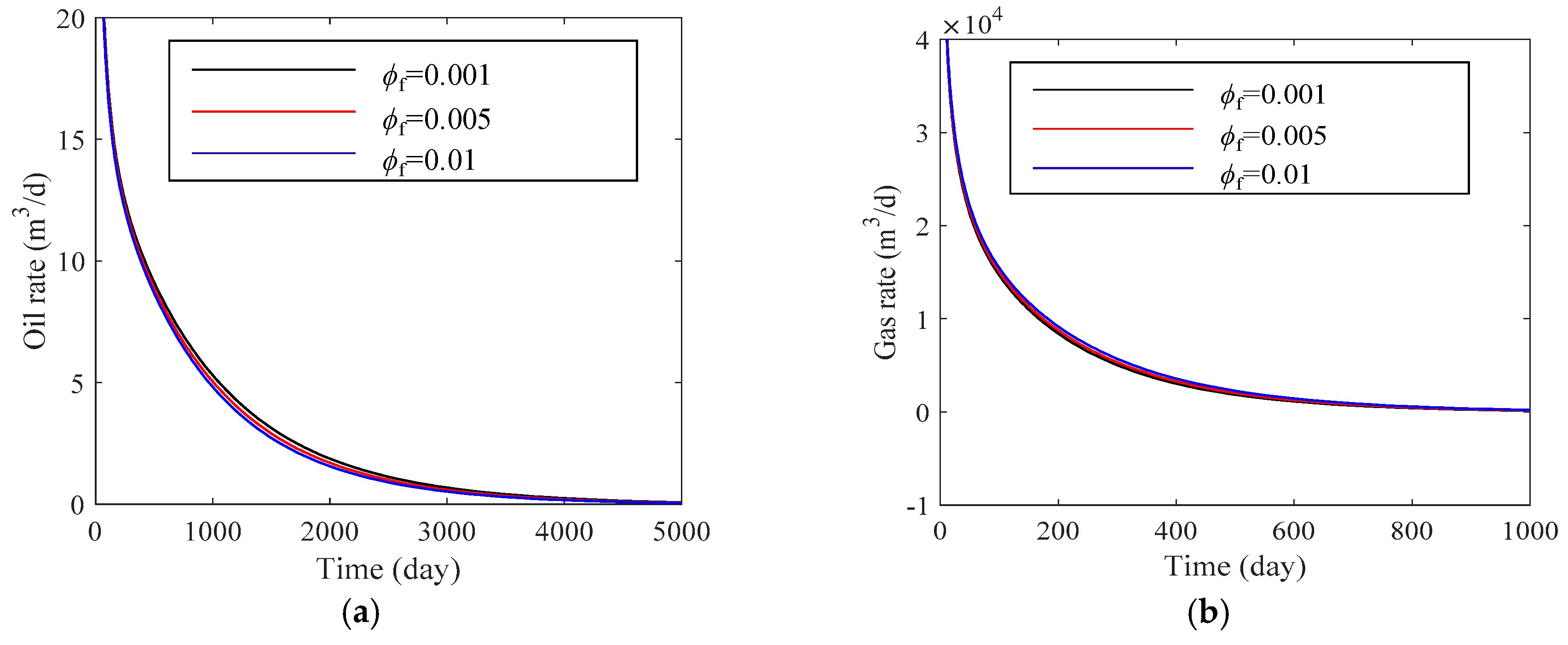
3.2.2. Fracture Porosity
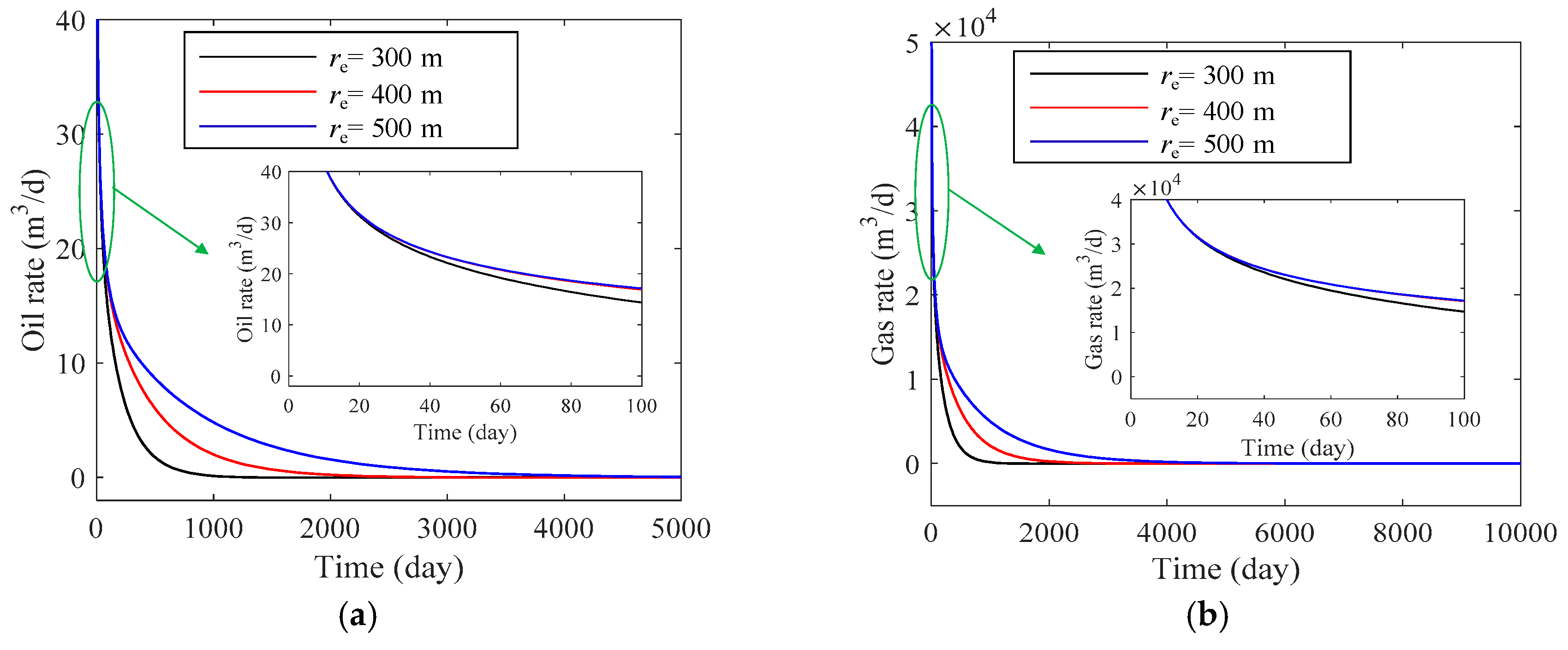
3.2.3. Radial Distance of External Boundary
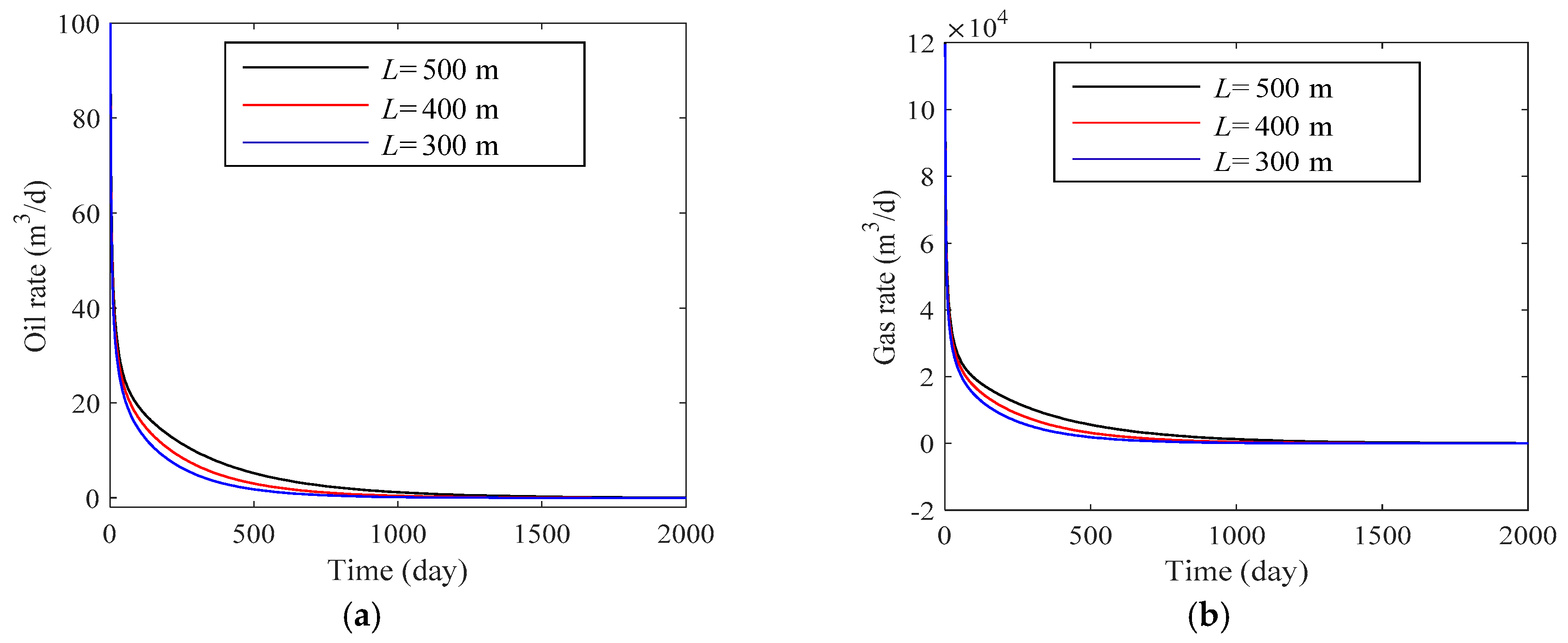
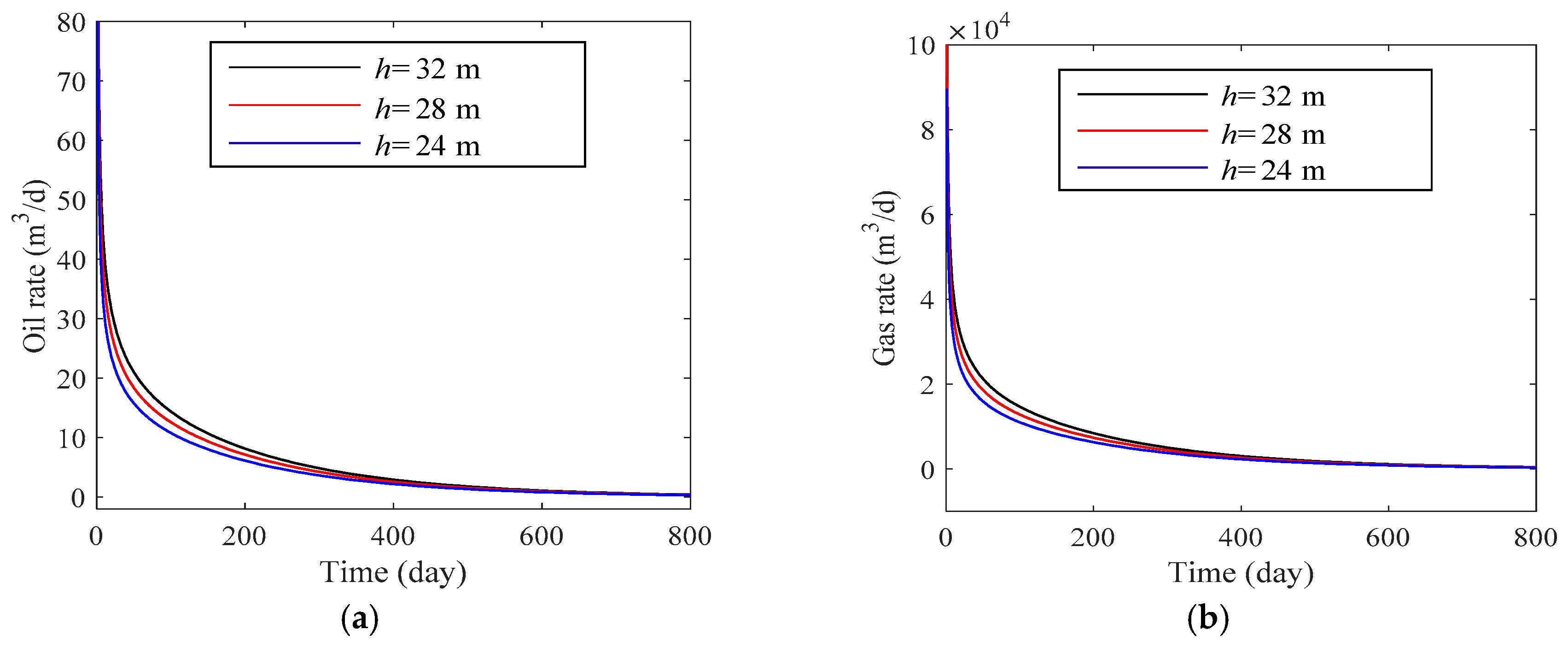
3.2.4. Horizontal Wellbore Length and Reservoir Thickness
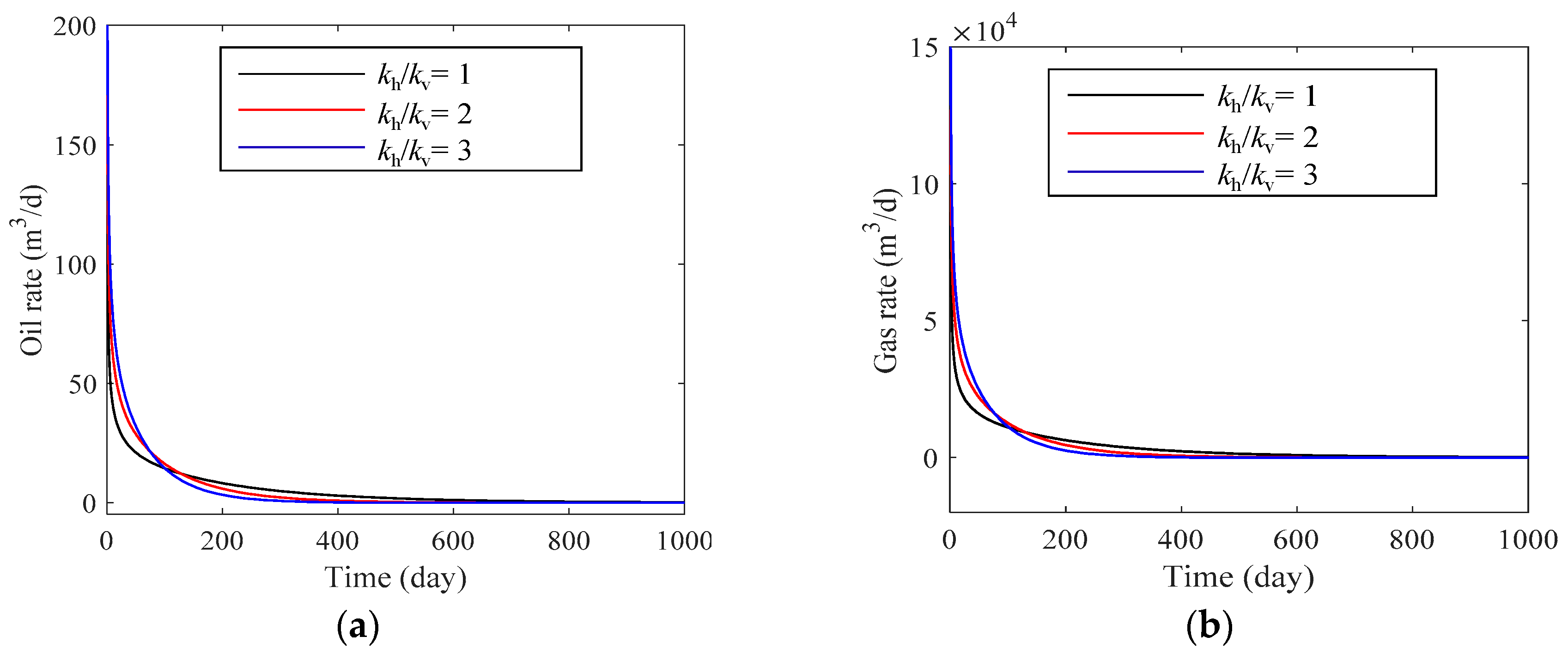
3.2.5. Ratio of Horizontal Permeability to Perpendicular Permeability
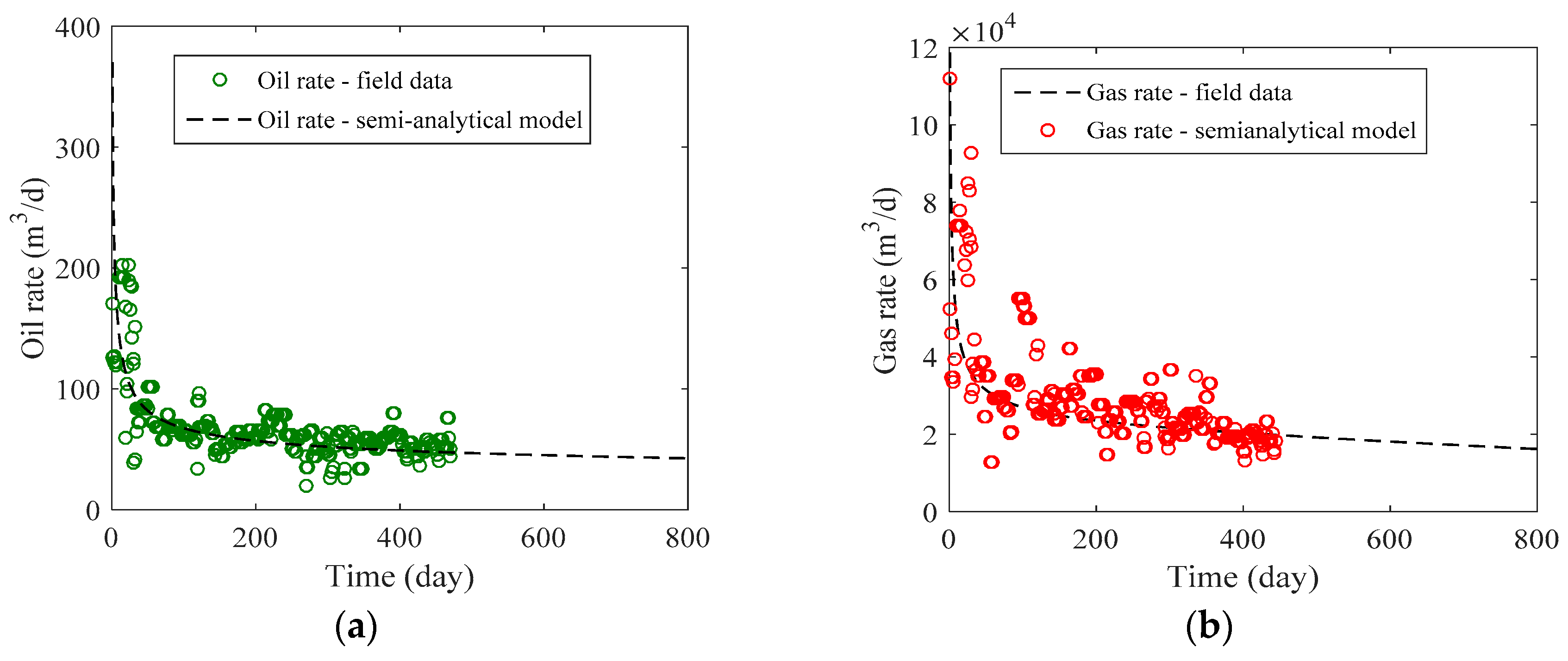
3.3. Methodology Application: A Case Study
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Gas formation volume factor, m3/m3 | Pressure of fracture, MPa | ||
| Initial gas formation volume factor, m3/m3 | Pressure of matrix, MPa | ||
| Oil formation volume factor, m3/m3 | Bottom-hole pressure, MPa | ||
| Initial oil formation volume factor, m3/m3 | q | Production rate of horizontal well at wellhead dominated by a line source production, m3/d | |
| Cumulative oil production rate, m3/d | Production rate from point source dominated by a point source production, m3/d | ||
| Formation thickness, m | Surface gas production rate, m3/d | ||
| I0() | Modified Bessel function of the first kind, zero order, dimensionless | Cross flow rate from matrix to fracture, m3/d | |
| I1() | Modified Bessel function of the first kind, first order, dimensionless | Surface gas production rate, m3/d | |
| IGIP | Initial gas in place, m3/d | Radial distance in reservoir formation, m | |
| IOIP | Initial oil in place, m3/d | re | Radial distance of side external boundary, m |
| Horizontal permeability of fracture, mD | rinv | Investigation radius, m | |
| Perpendicular permeability of fracture, mD | rM | Radial distance in a spherical matrix block, m | |
| Permeability of matrix, mD | rw | Wellbore radial, m | |
| Relative permeability to oil of fracture system, dimensionless | r1 | Radius of a spherical matrix block, m | |
| Relative permeability to oil of matrix system, dimensionless | RGIP | Remaining gas in place, m3/d | |
| K0() | Modified Bessel function of the second kind, zero order, dimensionless | ROIP | Remaining oil in place, m3/d |
| K1() | Modified Bessel function of the second kind, first order, dimensionless | Dissolved GOR, m3/m3 | |
| L | Horizontal wellbore length, m | Initial dissolved GOR, m3/m3 | |
| Pseudo pressure, MPa2/(mPa·s) | Laplace transform variable, dimensionless | ||
| Pseudo pressure of fracture, MPa2/(mPa·s) | Gas saturation, dimensionless | ||
| Pseudo pressure of matrix, MPa2/(mPa·s) | Oil saturation, dimensionless | ||
| Initial pseudo pressure, MPa2/(mPa·s) | time, day | ||
| Pseudo pressure of bottom-hole, MPa2/(mPa·s) | Velocity of oil flow, m/h | ||
| Cumulative gas production rate, m3/d | z | Perpendicular distance from bottom, m | |
| Pressure, MPa | zw | Perpendicular distance of horizontal Well from bottom, m | |
| Initial formation pressure, MPa | |||
| Greeks Symbols | |||
| Porosity, m3/m3 | Fluid viscosity, mPa·s | ||
| Density, kg/m3 | Differential operator | ||
| Conversion factor | Difference calculation | ||
| Conversion factor | Variable in z direction, m | ||
| Superscripts | |||
| Laplace transform | Average parameter | ||
| Flow rate per unit area | |||
| Subscripts | |||
| e | External | Oil | |
| Fracture | Production | ||
| Gas | Relative | ||
| h | Horizontal | Surface condition | |
| Initial | v | Vertical | |
| Matrix | Wellbore | ||
References
- Thiebot, B.M.; Sakthikumar, S.S. Cycling fractured reservoirs containing volatile oil: Laboratory investigation of the performance of lean gas or nitrogen injection. In Proceedings of the Middle East Oil Show, Manama, Bahrain, 16–19 November 1991. [Google Scholar]
- Ghorbani, D.; Kharrat, R. Fluid characterization of an Iranian carbonate oil reservoir using different PVT packages. In Proceedings of the Asia Pacific Oil and Gas Conference and Exhibition, Jakarta, Indonesia, 17–19 April 2000. [Google Scholar]
- Kang, Z.; Wu, Y.-S.; Li, J.; Zhang, J.; Wang, G. Modeling multiphase flow in naturally fractured vuggy petroleum reservoirs. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 24–27 September 2006. [Google Scholar] [CrossRef]
- Gringarten, A.C.; Ogunrewo, O.; Uxukbayev, G. Assessment of individual skin factors in gas condensate and volatile oil wells. In Proceedings of the SPE EUROPEC/EAGE Annual Conference and Exhibition, Vienna, Austria, 23–26 May 2011. [Google Scholar] [CrossRef]
- Poon, D.C. Decline curves for predicting production performance from horizontal wells. J. Can. Petrol. Technol. 1991, 30, 77–81. [Google Scholar] [CrossRef]
- Ozkan, E. Analysis of horizontal-well responses: Contemporary vs. conventional. SPE Reserv. Eval. Eng. 2001, 4, 260–269. [Google Scholar] [CrossRef]
- Hashemi, A.; Nicolas, L.M.; France, G.D.; Gringarten, A.C. Well test analysis of horizontal wells in gas-condensate reservoirs. SPE Reserv. Eval. Eng. 2006, 9, 86–99. [Google Scholar] [CrossRef]
- Hagoort, J. A simplified analytical method for estimating the productivity of a horizontal well producing at constant rate or constant pressure. J. Petrol. Sci. Eng. 2009, 64, 77–87. [Google Scholar] [CrossRef]
- Nie, R.-S.; Meng, Y.-F.; Jia, Y.-L.; Zhang, F.-X.; Yang, X.-T.; Niu, X.-J. Dual porosity and dual permeability modeling of horizontal well in naturally fractured reservoir. Transp. Porous Med. 2012, 92, 213–235. [Google Scholar] [CrossRef]
- Nie, R.-S.; Jia, Y.-L.; Meng, Y.-F.; Wang, Y.; Yuan, J.; Xu, W. New type curves for modeling productivity of horizontal well with negative skin factors. SPE Reserv. Eval. Eng. 2012, 15, 486–497. [Google Scholar] [CrossRef]
- Wu, Y.-H.; Cheng, L.-S.; Huang, S.-J.; Jia, P.; Zhang, J.; Lan, X.; Huang, H.-L. A practical method for production data analysis from multistage fractured horizontal wells in shale gas reservoirs. Fuel 2016, 186, 821–829. [Google Scholar] [CrossRef]
- Jia, P.; Cheng, L.-S.; Clarkson, C.R. A Laplace-domain hybrid model for representing flow behavior of multifractured horizontal wells communicating through secondary fractures in unconventional reservoirs. SPE J. 2017, 22, 1856–1876. [Google Scholar] [CrossRef]
- Warren, J.E.; Root, P.J. The behavior of naturally fractured reservoirs. SPE J. 1963, 228, 245–255. [Google Scholar] [CrossRef]
- Kazemi, H. Pressure transient analysis of naturally fractured reservoirs with uniform fracture distribution. SPE J. 1969, 11, 451–462. [Google Scholar] [CrossRef]
- De Swaan, O.A. Analytical solutions for determining naturally fractured reservoir properties by well testing. SPE J. 1976, 16, 117–122. [Google Scholar] [CrossRef]
- Ozkan, E.; Ohaeri, U. Unsteady flow to a well produced at a constant pressure in a fractured reservoir. SPE Form. Eval. 1987, 2, 186–200. [Google Scholar] [CrossRef]
- Al-Ghamdi, A.; Ershaghi, I. Pressure transient analysis of dually fractured reservoirs. SPE J. 1996, 1, 93–100. [Google Scholar] [CrossRef]
- Arps, J.J. Analysis of decline curves. Trans. AIME 1945, 160, 228–247. [Google Scholar] [CrossRef]
- Shea, G.B.; Higgins, R.V.; Lechtenberg, H.J. Decline and forecast studies based on performances of selected California oilfields. J. Pet. Technol. 1964, 16, 959–965. [Google Scholar] [CrossRef]
- Masoner, L.O. A decline analysis technique incorporating corrections for total fluid rate changes. SPE Reserv. Eval. Eng. 1996, 2, 533–541. [Google Scholar] [CrossRef]
- Hsieh, F.; Vega, C.; Vega, L. Reserves estimation using a PC decline analysis program. J. Can. Petrol. Technol. 2001, 40, 13–15. [Google Scholar] [CrossRef]
- Gaskari, R.; Mohaghegh, S.; Jalali, J. An integrated technique for production data analysis with application to mature fields. SPE Prod. Oper. 2007, 22, 403–416. [Google Scholar] [CrossRef]
- Ilk, D.; Rushing, J.A.; Perego, A.D.; Blasingame, T.A. Exponential vs. hyperbolic decline in tight gas sands: Understanding the origin and implications for reserve estimates using Arps’ decline curves. In Proceedings of the SPE Annual Technical Conference and Exhibition, Denver, CO, USA, 21–24 September 2008. [Google Scholar]
- Wilson, A. Comparison of empirical and analytical methods for production forecasting. J. Pet. Technol. 2015, 67, 133–136. [Google Scholar] [CrossRef]
- Wu, Y.-S. Numerical simulation of single-phase and multiphase non-Darcy flow in porous and fractured reservoirs. Transp. Porous Med. 2002, 49, 209–240. [Google Scholar] [CrossRef]
- Gill, H.; Issaka, M.B. Pressure transient behavior of horizontal and slant wells intersecting a high-permeability layer. In Proceedings of the SPE Middle East Oil and Gas Show and Conference, Manama, Bahrain, 11–14 March 2007. [Google Scholar]
- Mahmoud, M.A.; Nasr-El-Din, H.A. Challenges during shallow and deep carbonate reservoirs stimulation. J. Energy Resour. Technol. 2012, 137, 8. [Google Scholar] [CrossRef]
- Rao, X.; Cheng, L.-S.; Cao, R.-Y.; Jiang, J.-M.; Fang, S.-D.; Jia, P.; Wang, L. Engineering analysis with boundary elements a novel green element method based on two sets of nodes. Eng. Anal. Bound. Elem. 2018, 91, 124–131. [Google Scholar] [CrossRef]
- Rao, X.; Cheng, L.-S.; Cao, R.-Y.; Jiang, J.-M.; Li, N.; Fang, S.-D.; Wang, L. A novel green element method by mixing the idea of the finite difference method. Eng. Anal. Bound. Elem. 2018, 95, 238–247. [Google Scholar] [CrossRef]
- Perrine, R.L. Analysis of pressure-buildup curves. In Proceedings of the Drilling and Production Practice, New York, NY, USA, 1 January 1956. [Google Scholar]
- Martin, J.C. Simplified equations of flow in gas drive reservoirs and the theoretical foundation of two-phase pressure buildup analyses. AIME 1959, 216, 309–311. [Google Scholar]
- Fetkovich, M.J. The isochronal testing of oil wells. In Proceedings of the Fall Meeting of the Society of Petroleum Engineers of AIME, Las Vegas, NV, USA, 30 September–3 October 1973. [Google Scholar]
- Raghavan, R. Well test analysis: Wells producing by solution gas drive. SPE J. 1976, 16, 196–208. [Google Scholar] [CrossRef]
- Camacho-V, R.G.; Raghavan, R. Performance of wells in solution-gas-drive reservoirs. SPE Form. Eval. 1989, 4, 611–620. [Google Scholar] [CrossRef]
- Ozkan, E.; Raghavan, R. New solutions for well-test-analysis problems: Part 1—Analytical considerations. SPE Form. Eval. 1991, 6, 359–368. [Google Scholar] [CrossRef]
- Ozkan, E.; Raghavan, R. New solutions for well-test-analysis problems: Part 2—Computational considerations and applications. SPE Form. Eval. 1991, 6, 369–378. [Google Scholar] [CrossRef]
- Ng, M.G.; Aguilera, R. Well test analysis of horizontal wells in bounded naturally fractured reservoirs. J. Can. Petrol. Technol. 1999, 38, 20–24. [Google Scholar] [CrossRef]
- O’Sullivan, M.J. A similarity method for geothermal well test analysis. Water Resour. Res. 1981, 17, 390–398. [Google Scholar] [CrossRef]
- Bøe, A.; Skjaeveland, S.; Whitson, C. Two-phase pressure test analysis. SPE Form. Eval. 1989, 4, 604–610. [Google Scholar] [CrossRef]
- Kissling, W.; McGuinness, M.; McNabb, A. Analysis of one-dimensional horizontal two-phase flow in geothermal reservoirs. Transp. Porous Med. 1992, 7, 223–253. [Google Scholar] [CrossRef]
- Ayala, L.F.; Kouassi, J.P. The similarity theory applied to the analysis of two-phase flow in gas-condensate reservoirs. Energy Fuel 2007, 21, 1592–1600. [Google Scholar] [CrossRef]
- Zhang, M.; Ayala, L.F. Analytical study of constant gas–oil-ratio behavior as an infinite-acting effect in unconventional two-phase reservoir systems. SPE J. 2016, 22, 1–11. [Google Scholar] [CrossRef]
- Jia, P.; Cheng, L.-S.; Huang, S.-J. A semi-analytical model for the flow behavior of naturally fractured formations with multi-scale fracture networks. J. Hydrol. 2016, 537, 208–220. [Google Scholar] [CrossRef]
- Jia, P.; Cheng, L.-S.; Clarkson, C.R. Flow behavior analysis of two-phase flowback and early-time production from hydraulically-fractured shale gas wells using a hybrid numerical/analytical model. Int. J. Coal. Geol. 2017, 180, 14–31. [Google Scholar] [CrossRef]
- Li, Y.; Zuo, W.; Yu, W.; Chen, Y. A fully three dimensional semi-analytical model for shale gas reservoirs with hydraulic fractures. Energies 2018, 11, 436. [Google Scholar] [CrossRef]
- Clarkson, C.R.; Qanbari, F. A semi-analytical forecasting method for unconventional gas and light oil wells: A hybrid approach for addressing the limitations of existing empirical and analytical methods. SPE Reserv. Eval. Eng. 2014, 18, 260–263. [Google Scholar] [CrossRef]
- Clarkson, C.R.; Qanbari, F. An approximate semi-analytical two-phase forecasting method for multifractured tight light-oil wells with complex fracture geometry. J. Can. Petrol. Technol. 2015, 54, 489–508. [Google Scholar] [CrossRef]
- Guo, J.-C.; Nie, R.-S.; Jia, Y.-L. Unsteady-state diffusion modeling of gas in coal matrix for horizontal well production. AAPG Bull. 2014, 98, 1669–1697. [Google Scholar] [CrossRef]
- Stehfest, H. Numerical inversion of Laplace transforms. ACM Commun. 1970, 13, 47–49. [Google Scholar] [CrossRef]
- Kuchuk, F.-J. Radius of investigation for reserve estimation from pressure transient well tests. In Proceedings of the SPE Middle East Oil and Gas Show and Conference, Manama, Bahrain, 15–18 March 2009. [Google Scholar]
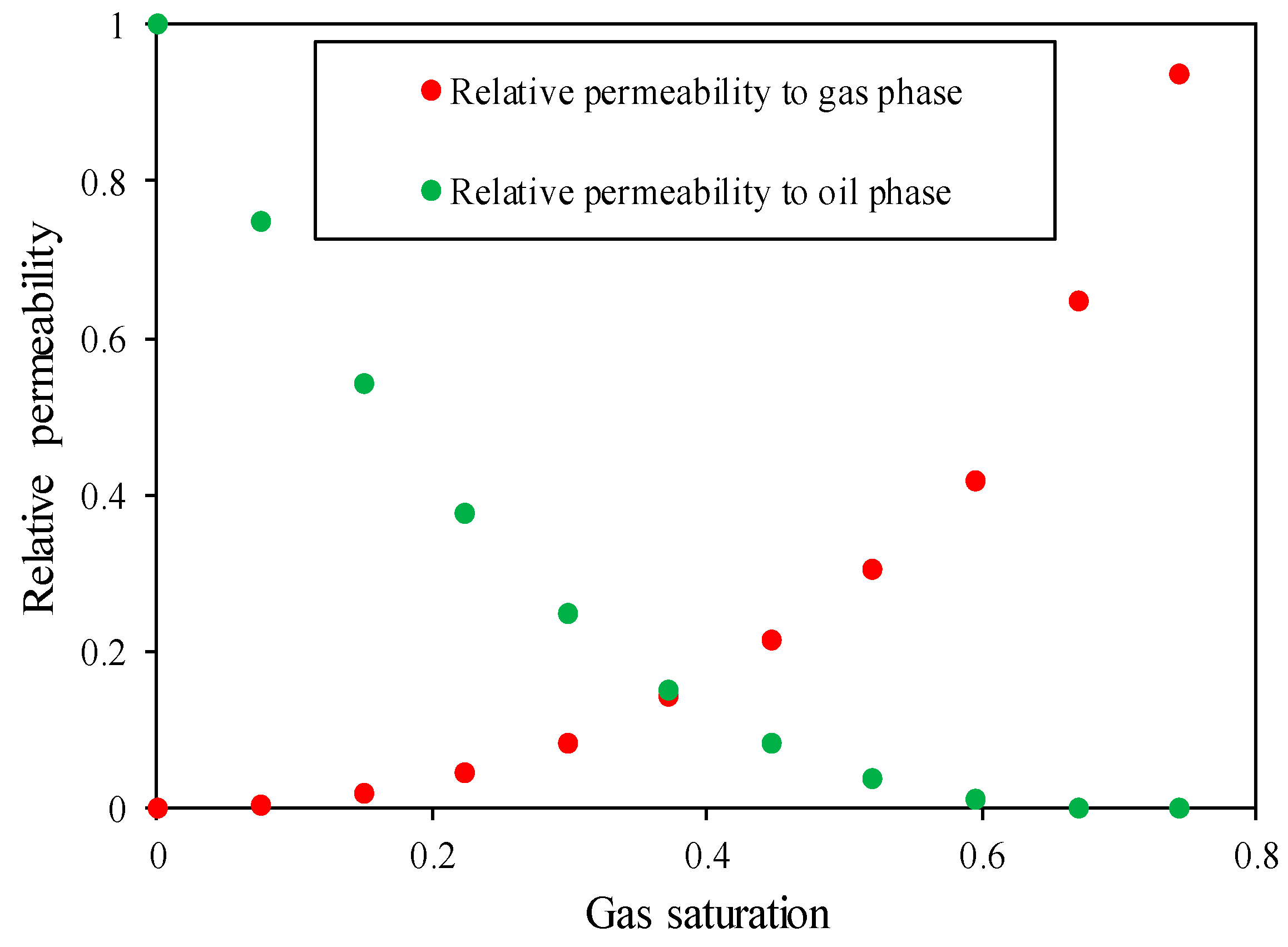

| Parameters | Symbol | Unit | Value |
|---|---|---|---|
| Wellbore radius | r | m | 0.07 |
| Formation thickness | h | m | 38 |
| Matrix porosity | ϕM | Dimensionless | 0.1 |
| Horizontal permeability | kh | mD | 0.25 |
| Perpendicular permeability | kv | mD | 0.25 |
| Permeability modulus | γ | MPa−1 | 2.2 × 10−3 |
| Rock compressibility | Ct | MPa−1 | 1.0 × 10−4 |
| Horizontal wellbore length | L | m | 500 |
| Perpendicular distance of horizontal well | zw | m | 19 |
| Radial distance of external boundary | re | m | 880 |
| Initial water saturation | Swi | Dimensionless | 0.15 |
| Oil API gravity | / | Dimensionless | 40 |
| Reservoir temperature | T | K | 323.15 |
| Initial reservoir pressure | pi | MPa | 24.48 |
| Bottom-hole pressure | pwf | MPa | 6 |
| Bubble point pressure | pb | MPa | 22.34 |
| Parameters | Symbol | Unit | Value |
|---|---|---|---|
| Wellbore radius | r | m | 0.07 |
| Matrix porosity | ϕM | Dimensionless | 0.108 |
| Rock compressibility | Ct | MPa−1 | 1.0 × 10−4 |
| Perpendicular distance of horizontal well | zw | m | 16 |
| Initial water saturation | Swi | Dimensionless | 15 |
| Oil API gravity | / | Dimensionless | 40 |
| Reservoir temperature | T | K | 323.15 |
| Initial reservoir pressure | pi | MPa | 26.48 |
| Bottom-hole pressure | pwf | MPa | 6 |
| Bubble point pressure | pb | MPa | 22.34 |
| Perpendicular permeability | kv | mD | 0.25 |
| Parameters | Symbol | Unit | Value |
|---|---|---|---|
| Wellbore radius | r | m | 0.07 |
| Formation thickness | h | m | 24 |
| Matrix porosity | ϕM | Dimensionless | 0.148 |
| Rock compressibility | Ct | MPa−1 | 1.0 × 10−4 |
| Perpendicular distance of horizontal well | zw | m | 12 |
| Initial water saturation | Swi | Dimensionless | 0.15 |
| Oil API gravity | / | Dimensionless | 40 |
| Reservoir temperature | T | K | 358 |
| Initial reservoir pressure | pi | MPa | 24.8 |
| Bottom-hole pressure | pwf | MPa | 6 |
| Bubble point pressure | pb | MPa | 22.34 |
| Interpreted Parameters | Symbol | Unit | Value |
|---|---|---|---|
| Fracture permeability | kh | mD | 2.6 |
| Permeability modulus | γ | MPa−1 | 0.014 |
| Fracture porosity | ϕf | Dimensionless | 0.005 |
| Horizontal wellbore length | L | m | 580 |
| Radial distance of external boundary | re | m | 850 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; Cheng, L.; Xue, Y.; Huang, S.; Wu, Y.; Jia, P.; Sun, Z. A Semi-Analytical Method for Simulating Two-Phase Flow Performance of Horizontal Volatile Oil Wells in Fractured Carbonate Reservoirs. Energies 2018, 11, 2700. https://doi.org/10.3390/en11102700
Wang S, Cheng L, Xue Y, Huang S, Wu Y, Jia P, Sun Z. A Semi-Analytical Method for Simulating Two-Phase Flow Performance of Horizontal Volatile Oil Wells in Fractured Carbonate Reservoirs. Energies. 2018; 11(10):2700. https://doi.org/10.3390/en11102700
Chicago/Turabian StyleWang, Suran, Linsong Cheng, Yongchao Xue, Shijun Huang, Yonghui Wu, Pin Jia, and Zheng Sun. 2018. "A Semi-Analytical Method for Simulating Two-Phase Flow Performance of Horizontal Volatile Oil Wells in Fractured Carbonate Reservoirs" Energies 11, no. 10: 2700. https://doi.org/10.3390/en11102700
APA StyleWang, S., Cheng, L., Xue, Y., Huang, S., Wu, Y., Jia, P., & Sun, Z. (2018). A Semi-Analytical Method for Simulating Two-Phase Flow Performance of Horizontal Volatile Oil Wells in Fractured Carbonate Reservoirs. Energies, 11(10), 2700. https://doi.org/10.3390/en11102700




