Study on the Evaluation Index System and Evaluation Method of Voltage Stability of Distribution Network with High DG Penetration
Abstract
:1. Introduction
2. Voltage Stability Evaluation Index (VSE)
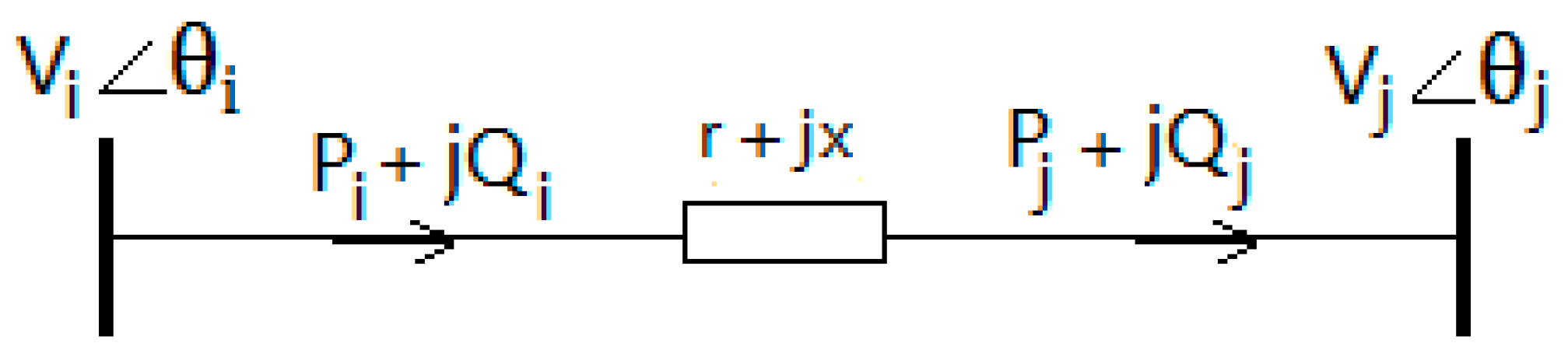
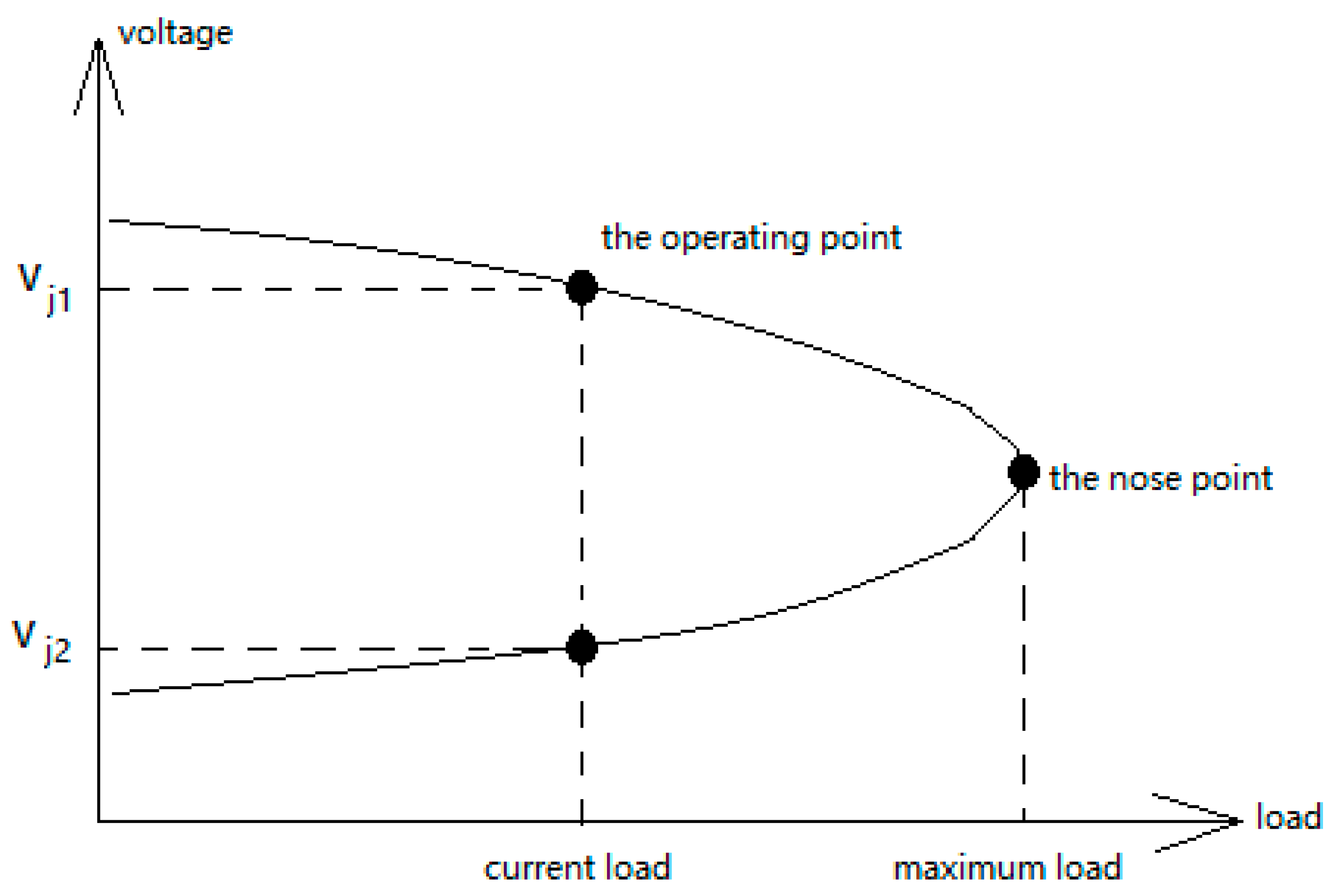
2.1. Traditional VSE
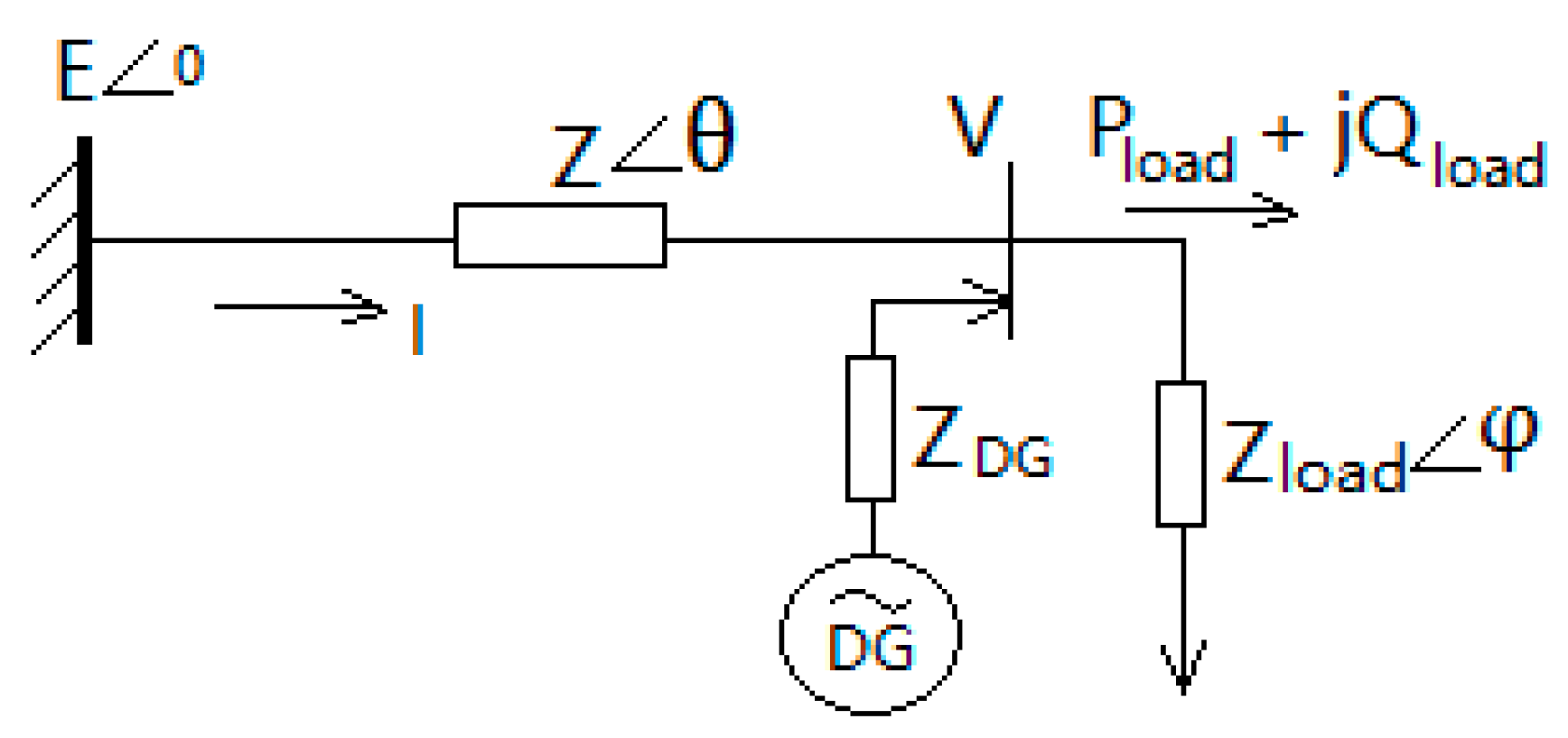
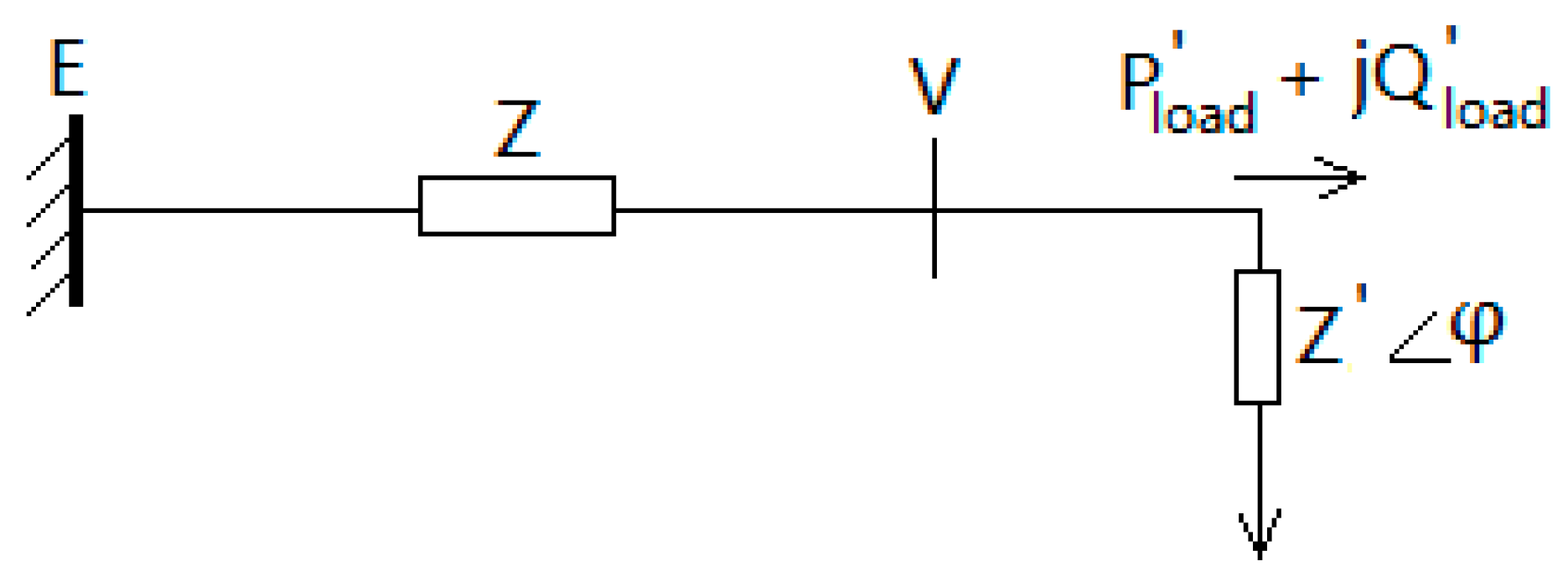
2.2. Static Voltage Stability Analysis Model with High Penetration DG
3. Simulation and Analysis
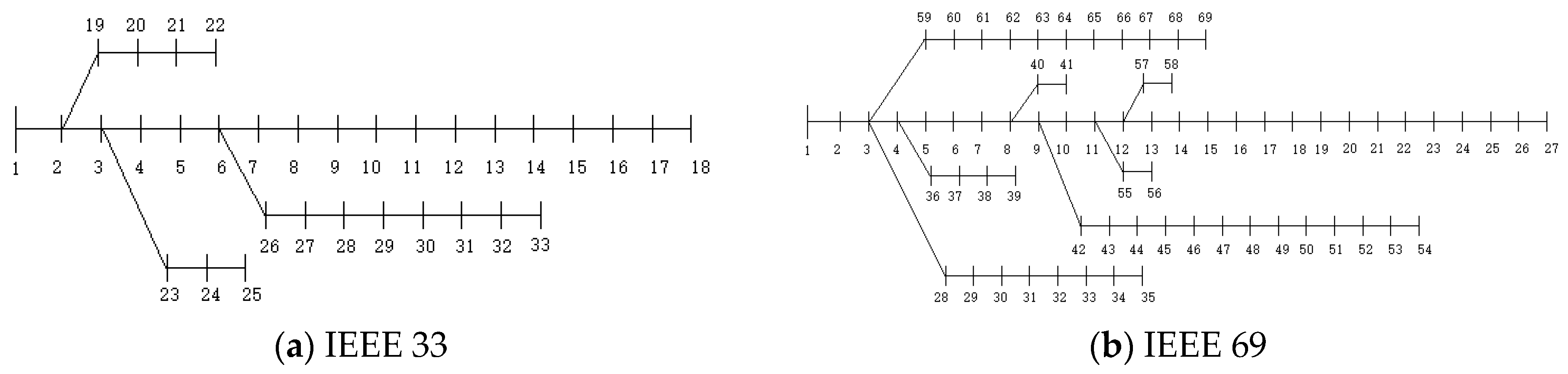
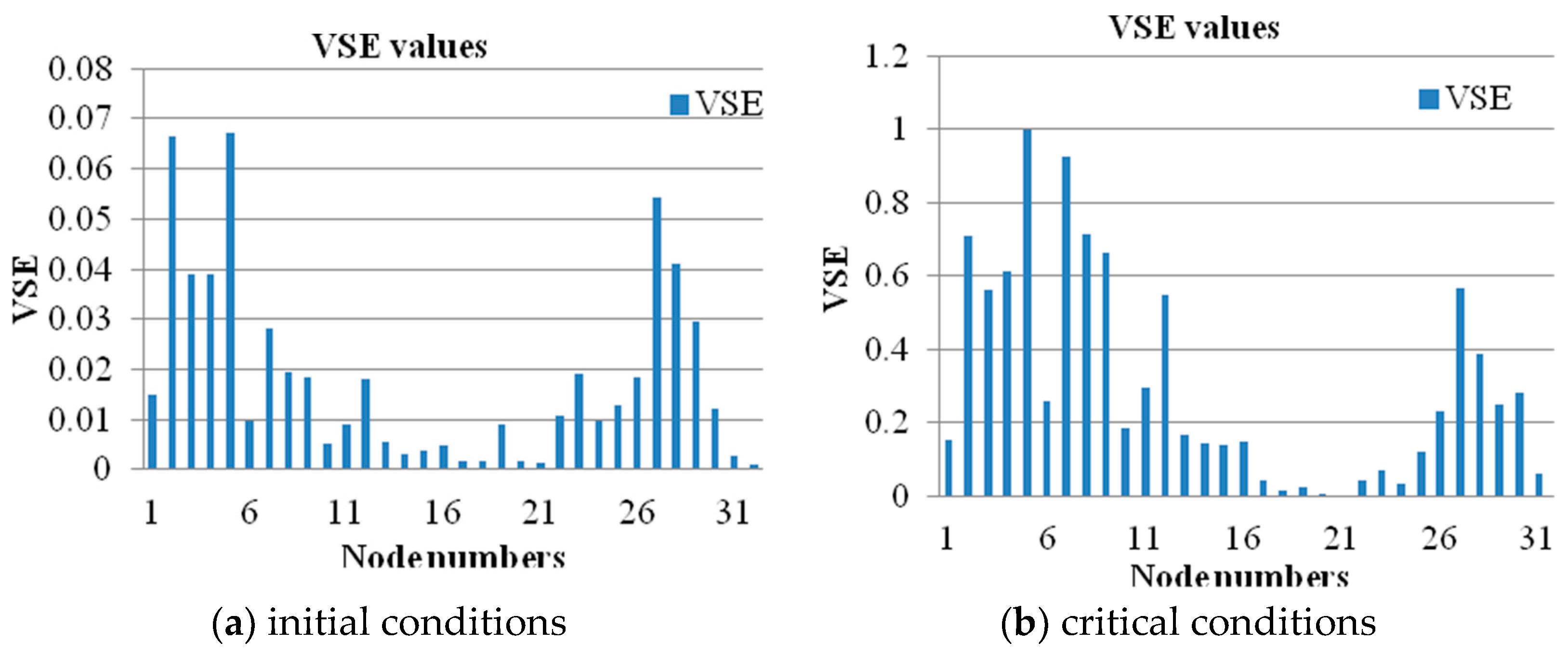
3.1. Simulations
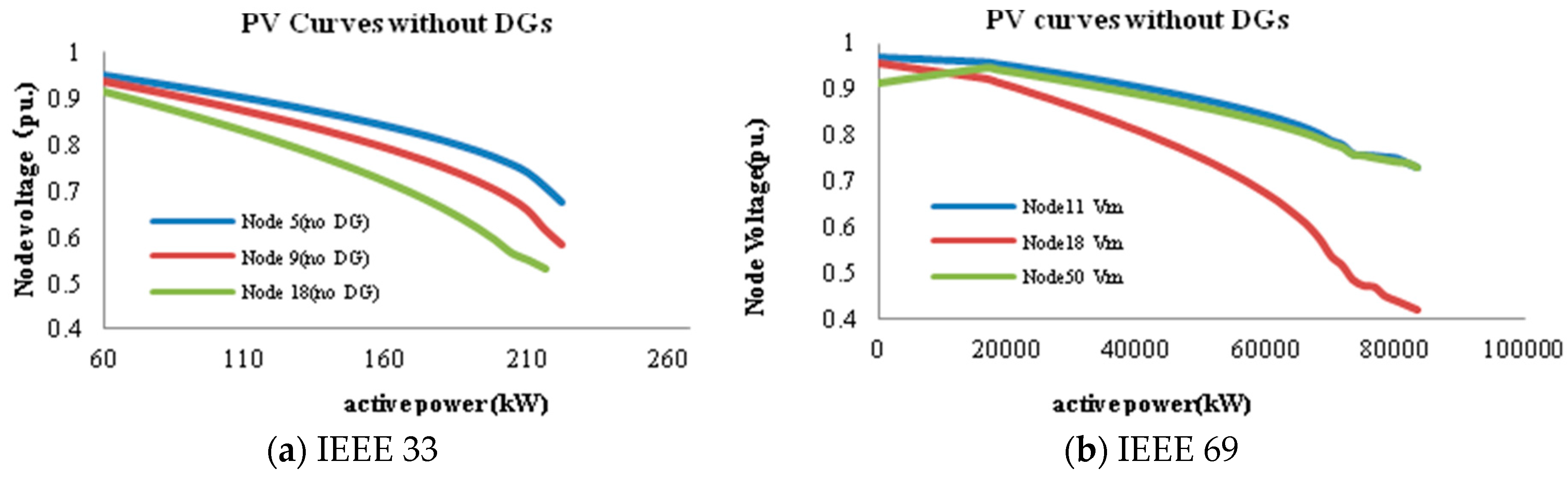
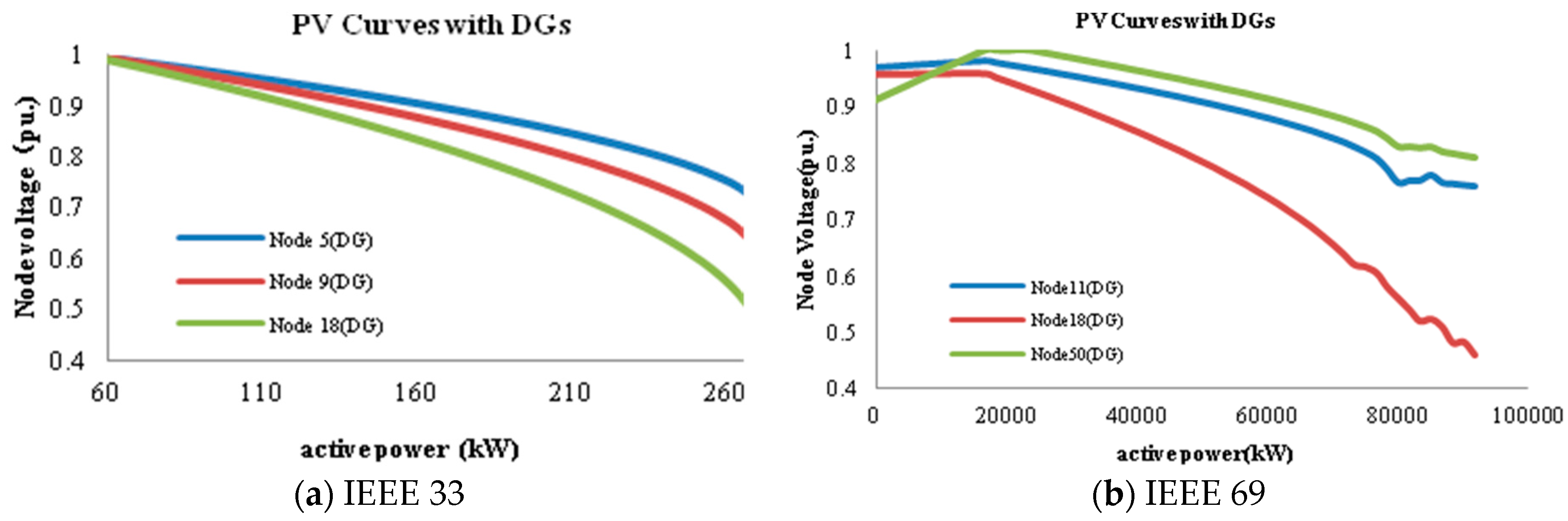
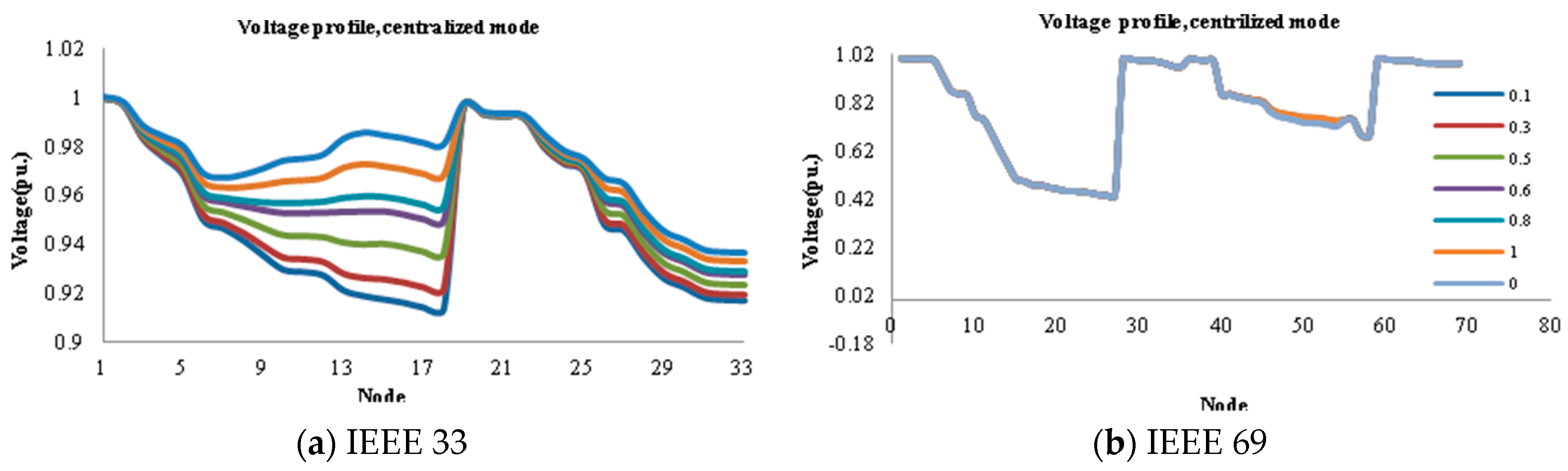
3.2. Centralized and Decentralized Access
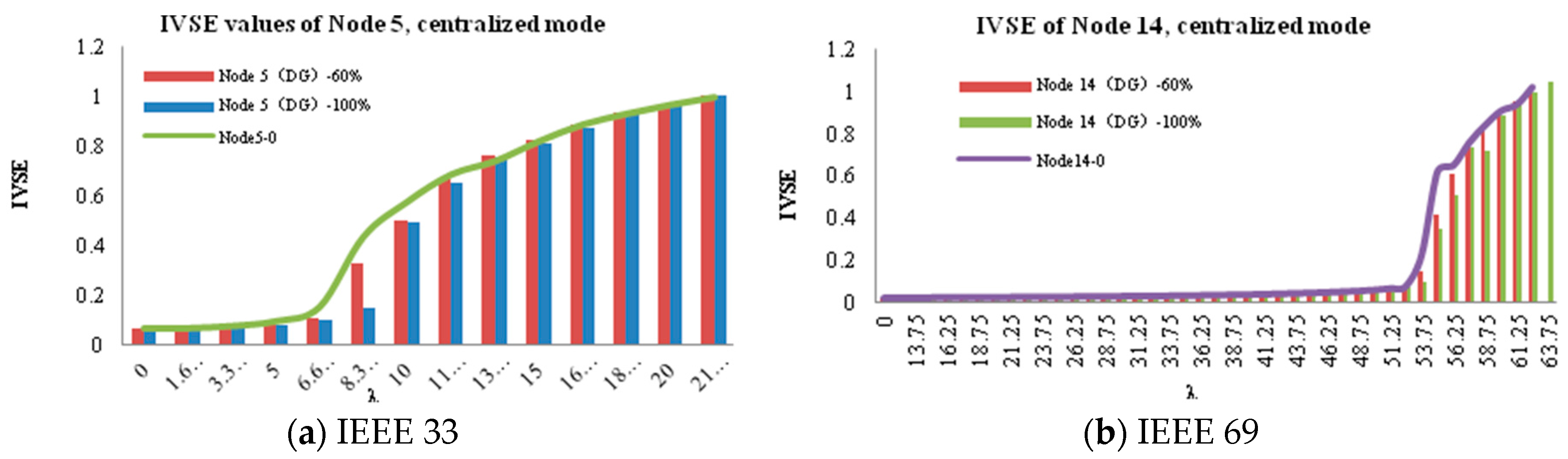
3.2.1. Centralized Access
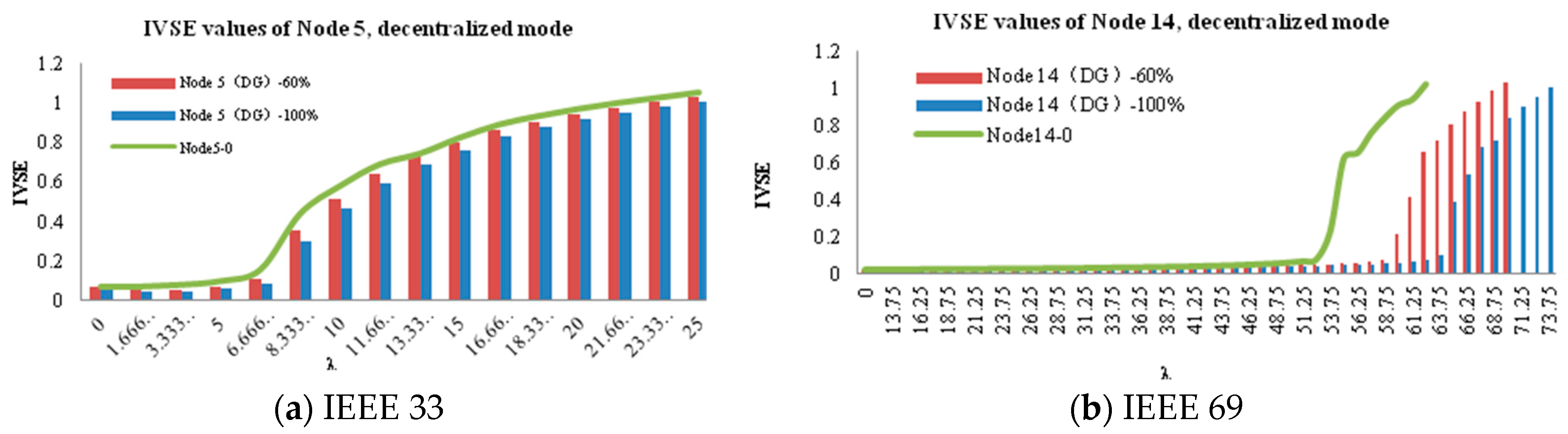
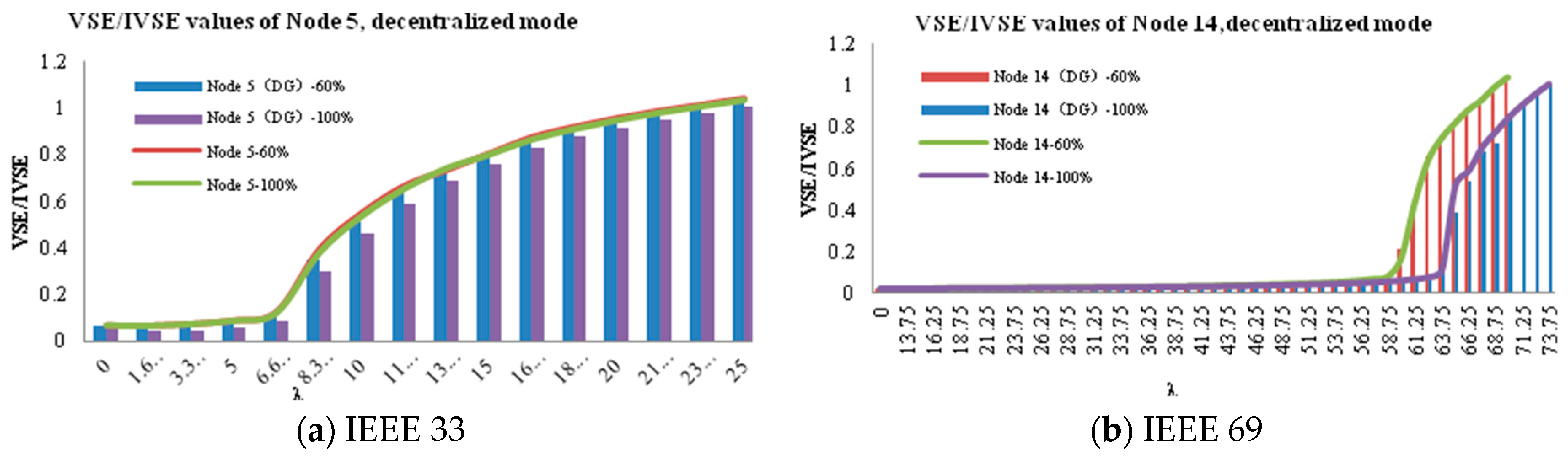
3.2.2. Decentralized Access
4. Comparison of Results
5. Discussion
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Shu, Y.; Zhang, Z.; Guo, J.; Zhang, Z. Study on Key Factors and Solution of Renewable Energy Accommodation. Proc. CSEE 2017, 37, 1–8. [Google Scholar]
- Ackerman, T.; Anderson, G.; Seder, L. Distributed generation: A definition. Electr. Power Syst. Res. 2001, 57, 195–204. [Google Scholar] [CrossRef]
- Ma, Z.; An, T.; Shang, Y. State of the Art and Development Trends of Power Distribution Technologies. Proc. CSEE 2015, 36, 1552–1567. [Google Scholar]
- Chen, G.; Li, M.; Xu, T.; Liu, M. Study on Technical Bottleneck of New Energy Development. Proc. CSEE 2017, 37, 20–26. [Google Scholar]
- Lin, S.; Li, X.; Liu, Y. Present Investigation of Voltage Stability and Composite Load’s Influence on It. Proc. CSU-EPSA 2008, 20, 66–74. [Google Scholar]
- Zhong, W.; Tang, Y. Transient Voltage Stability Analysis of Differential-algebra Equation in Power System. Proc. CSEE 2010, 30, 10–16. [Google Scholar]
- Chen, N.; Zhu, L.; Wang, W. Strategy for Reactive Power Control of Wind Farm for Improving Voltage Stability in Wind Power Integrated Region. Proc. CSEE 2009, 29, 102–108. [Google Scholar]
- Chen, Q.; Li, L.; Wang, Q.; Zeng, Y. Simulation Model of Photovoltaic Generation Grid-Connected System and Its Impacts on Voltage Stability in Distribution Grid. Trans. China Electr. Tech. Soc. 2013, 28, 241–247. [Google Scholar]
- Liu, X.-D.; Guo, R.; Zhang, J.-F.; Zeng, R.-M. Voltage stability assessment frame for distribution network with wind farms. Power Syst. Prot. Control 2013, 41, 77–81. [Google Scholar]
- Qin, W.; Ren, C.; Han, X.; Wang, P.; Liu, Z. Power System Voltage Stability Risk Assessment Considering the Limit of Load Fluctuation. Proc. CSEE 2015, 35, 4102–4111. [Google Scholar]
- Bai, Y.; Wang, P.; Han, X.; Qin, W. Risk Assessment of Static Voltage Stability Based on Load Uncertainty Modeling. Proc. CSEE 2016, 36, 3470–3478. [Google Scholar]
- Vita, V. Development of a decision-making algorithm for the optimum size and placement of distributed generation units in distribution networks. Energies 2017, 10, 1433. [Google Scholar] [CrossRef]
- Vita, V.; Alimardan, T.; Ekonomou, L. The impact of distributed generation in the distribution networks’ voltage profile and energy losses. In Proceedings of the 9th IEEE European Modeling Symposium on Mathematical Modeling and Computer Simulation, Madrid, Spain, 6–8 October 2015; pp. 260–265. [Google Scholar]
- Zhang, Y.; Zhou, S.; Wang, L.; Huang, Y.; Zhang, G. The Theoretical Foundations of Static Voltage Stability Analysis Model. Proc. CSEE 1999, 19, 55–63. [Google Scholar]
- Paramasivam, M.; Salloum, A.; Ajjarapu, V.; Vittal, V.; Bhatt, B.; Liu, N. Dynamic optimization based reactive power planning to mitigate slow voltage recovery and short term voltage instability. IEEE Trans Power Syst. 2013, 28, 3865–3873. [Google Scholar] [CrossRef]
- Amrane, Y.; Boudour, M.; Belazzoug, M. A new optimal reactive power planning based on differential search algorithm. Int. J. Electr. Power Energy Syst. 2015, 64, 551–561. [Google Scholar] [CrossRef]
- Lu, Z.; Li, H.; Qiao, Y. Flexibility Evaluation and Supply/Demand Balance Principle of Power System with High-penetration Renewable Electricity. Proc. CSEE 2017, 37, 9–19. [Google Scholar]
- Jiang, T.; Ai, L.; Yang, Y. On-ling voltage stability index based on load margin. Electr. Power Autom. Equip. 2009, 29, 39–42. [Google Scholar]
- Sheng, W.; Liu, K.Y.; Liu, Y.; Meng, X.; Li, Y. Optimal placement and sizing of distributed generation via an improved non-dominated sorting genetic algorithm II. IEEE Trans. Power Deliv. 2015, 30, 569–578. [Google Scholar] [CrossRef]
- Zeinalzadeh, A.; Mohammadi, Y.; Morad, M.H. Optimal multi objective placement and sizing of multiple DGs and shunt capacitor banks simultaneously considering load uncertainty via MOPSO approach. Int. J. Electr. Power Energy Syst. 2015, 67, 336–349. [Google Scholar] [CrossRef]
- Tan, Y.; Li, X.; Cai, Y.; Wang, C. Modeling Cascading Failures in Power Grid Based on Dynamic Power Flow and Vulnerable Line Identification. Proc. CSEE 2015, 35, 615–622. [Google Scholar]
- Zheng, Y.; Sun, J.W.; Zhang, C.; Lin, X.N. Study of Voltage Stability Margin for the Distribution Network with Electric Vehicle Integration. Trans. China Electr. Tech. Soc. 2014, 29, 20–26. [Google Scholar]
- Prabha, K. Power System Stability and Control; McGraw-Hill Education: New York, NY, UAS, 1994. [Google Scholar]
- Wang, X. Modern Power System Analysis. Sci. Press 2003, 1, 66–68. [Google Scholar]
- He, J.; Chen, G.Y.; Tao, Z.D.; Wang, Y.J.; Xu, G.J. Reactive Power Optimization in Distribution System with Distributed Generators Considering Voltage Stability Index. Shanxi Electr. Power 2015, 43, 30–31. [Google Scholar]
- Hu, H.; Wu, S.; Xia, X.; Gan, D. Computing the Maximum Penetration Level of Multiple Distributed Generators in Distribution Network Taking into Account Voltage Regulation Constraints. Proc. CSEE 2006, 26, 13–19. [Google Scholar]
- Kim, T.E.; Kim, J.E. Considerations for the feasible operating range of distributed generation interconnected to power distribution system. In Proceedings of the IEEE PES Summer Meeting, Chicago, IL, USA, 21–25 July 2002. [Google Scholar]
- Hung, D.Q.; Mithulananthan, N.; Lee, K.Y. Optimal placement of dispatchable and nondispatchable renewable DG units in distribution networks for minimizing energy loss. Electr. Power Energy Syst. 2014, 55, 179–186. [Google Scholar] [CrossRef]
- Gozel, T.; Hocaoglu, M.H. An analytical method for the sizing and sitting of distributed generators in radial systems. Electr. Power Syst. Res. 2009, 79, 912–918. [Google Scholar] [CrossRef]
- Moradi, M.H.; Tousi, S.M.R.; Abedini, M. Multi-objective PFDE algorithm for solving the optimal sitting and sizing problem of multiple DG sources. Int. J. Electr. Power Energy Syst. 2014, 56, 117–126. [Google Scholar] [CrossRef]

| DG Access Centralized | ||||||
|---|---|---|---|---|---|---|
| After DG Access | Initial Load Condition (Unit: MW) | |||||
| DG Access Ratio | Limit Power | Active Power Loss | Reactive Power Loss | |||
| 33-Node | 69-Node | 33-Node | 69-Node | 33-Node | 69-Node | |
| 0% | 1.31 | 0.5 | 0.202 | 0.226 | 0.134 | 0.102 |
| 10% | 1.308 | 0.5 | 0.186 | 0.197 | 0.122 | 0.089 |
| 30% | 1.308 | 0.51 | 0.159 | 0.149 | 0.105 | 0.068 |
| 50% | 1.308 | 0.51 | 0.142 | 0.115 | 0.092 | 0.053 |
| 60% | 1.296 | 0.51 | 0.135 | 0.103 | 0.088 | 0.047 |
| 80% | 1.296 | 0.51 | 0.128 | 0.087 | 0.081 | 0.04 |
| 100% | 1.296 | 0.52 | 0.128 | 0.081 | 0.077 | 0.036 |
| DG Access Decentralized | ||||||
|---|---|---|---|---|---|---|
| After DG Access | Initial Load Condition (Unit: MW) | |||||
| DG Access Ratio | Limit Power | Active Power Loss | Reactive Power Loss | |||
| 33-Node | 69-Node | 33-Node | 69-Node | 33-Node | 69-Node | |
| 0% | 1.31 | 0.5 | 0.202 | 0.226 | 0.134 | 0.102 |
| 10% | 1.332 | 0.51 | 0.182 | 0.194 | 0.122 | 0.088 |
| 30% | 1.368 | 0.52 | 0.165 | 0.142 | 0.11 | 0.064 |
| 50% | 1.38 | 0.53 | 0.149 | 0.105 | 0.098 | 0.048 |
| 60% | 1.404 | 0.53 | 0.139 | 0.091 | 0.091 | 0.042 |
| 80% | 1.464 | 0.54 | 0.131 | 0.074 | 0.084 | 0.034 |
| 100% | 1.5 | 0.55 | 0.122 | 0.068 | 0.078 | 0.031 |
| References | Methods Used | Access Mode | Size of DG/DGs (MW) | Active Loss (MW) |
|---|---|---|---|---|
| Ref. [29] | Analytical method | centralized | 2.5 | 0.1112 |
| Ref. [30] | GA | decentralized | 1.5/0.423/1.0714 | 0.1063 |
| PSO | 1.177/0.982/0.83 | 0.1053 | ||
| Proposed method | Evaluation method | centralized | 2.8 | 0.1054 |
| decentralized | 0.754/1.099/1.071 | 0.101 |
| References | Methods Used | Access Mode | Size of DG/DGs (MW) | Active Loss (MW) |
|---|---|---|---|---|
| Ref. [29] | Analytical method | centralized | 1.8078 | 0.0834 |
| Ref. [30] | GA | decentralized | 0.9297/1.0752/0.985 | 0.089 |
| PSO | 0.993/1.1998/0.796 | 0.0832 | ||
| Proposed method | Evaluation method | centralized | 1.873 | 0.0839 |
| decentralized | 0.6267/0.5805/1.719 | 0.0699 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tu, J.; Yin, Z.; Xu, Y. Study on the Evaluation Index System and Evaluation Method of Voltage Stability of Distribution Network with High DG Penetration. Energies 2018, 11, 79. https://doi.org/10.3390/en11010079
Tu J, Yin Z, Xu Y. Study on the Evaluation Index System and Evaluation Method of Voltage Stability of Distribution Network with High DG Penetration. Energies. 2018; 11(1):79. https://doi.org/10.3390/en11010079
Chicago/Turabian StyleTu, Jingjing, Zhongdong Yin, and Yonghai Xu. 2018. "Study on the Evaluation Index System and Evaluation Method of Voltage Stability of Distribution Network with High DG Penetration" Energies 11, no. 1: 79. https://doi.org/10.3390/en11010079
APA StyleTu, J., Yin, Z., & Xu, Y. (2018). Study on the Evaluation Index System and Evaluation Method of Voltage Stability of Distribution Network with High DG Penetration. Energies, 11(1), 79. https://doi.org/10.3390/en11010079





