A Transient Analytical Model for Predicting Wellbore/Reservoir Temperature and Stresses during Drilling with Fluid Circulation
Abstract
:1. Introduction
2. Problem Formulation
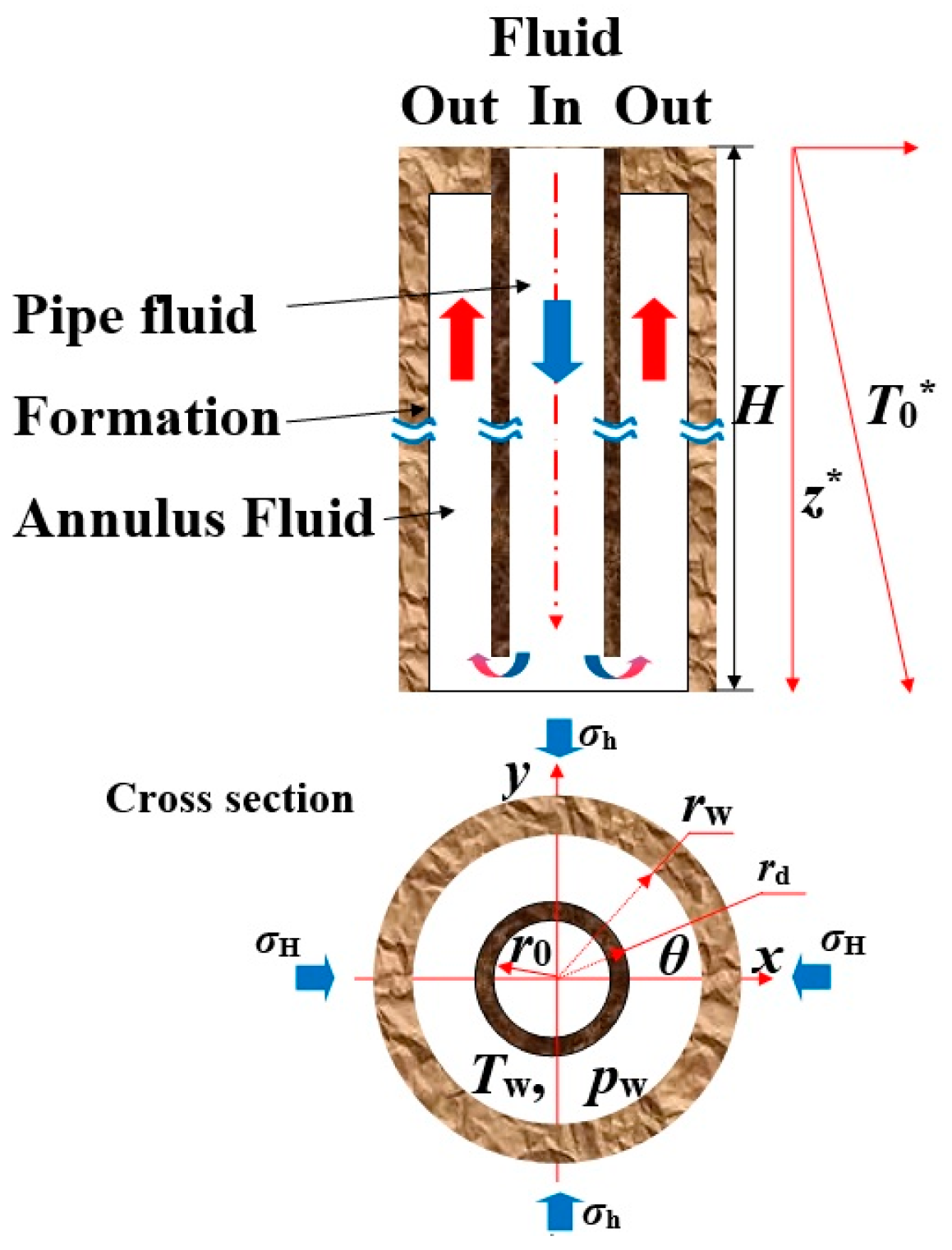
2.1. Problem Description
2.2. Governing Equations
2.2.1. Wellbore Heat Transfer
2.2.2. Heat Conduction in Rock
2.2.3. Rock Deformation
- Equations of equilibrium
- Strain-displacement relations
- Linear TM constitutive equation for isotropic medium
2.3. Boundary Conditions
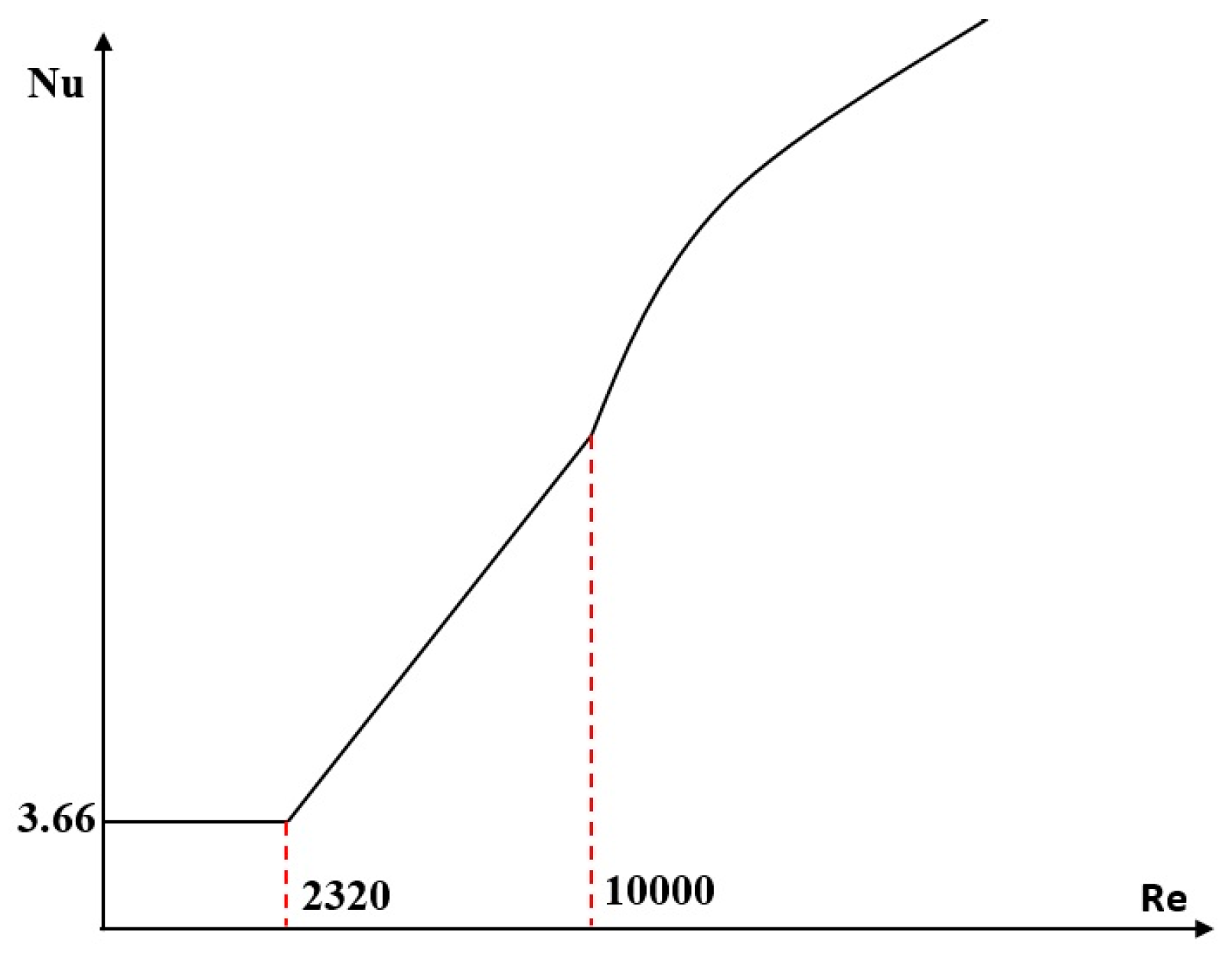
2.4. Heat Transfer Coefficients (HTCs)
3. Dimensional Analysis
4. Solution Method
5. Results and Discussions
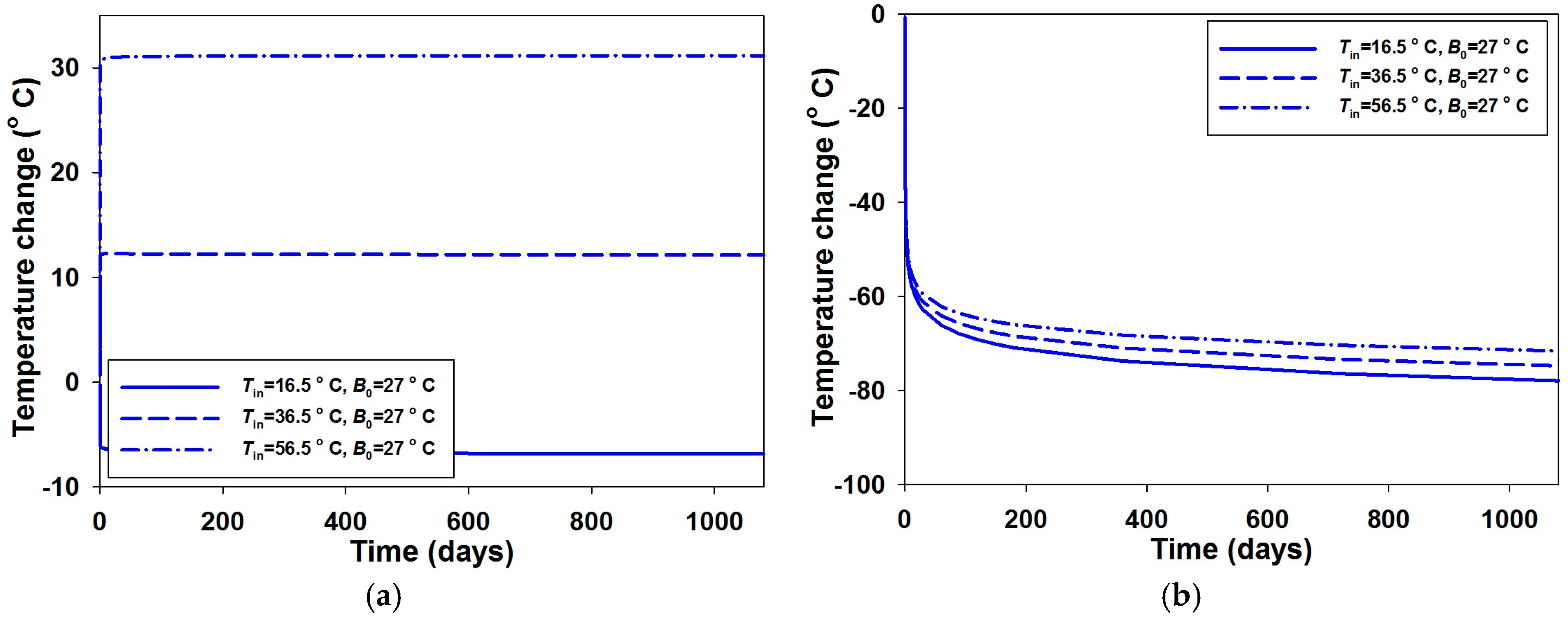
5.1. Wellbore Temperature Responses
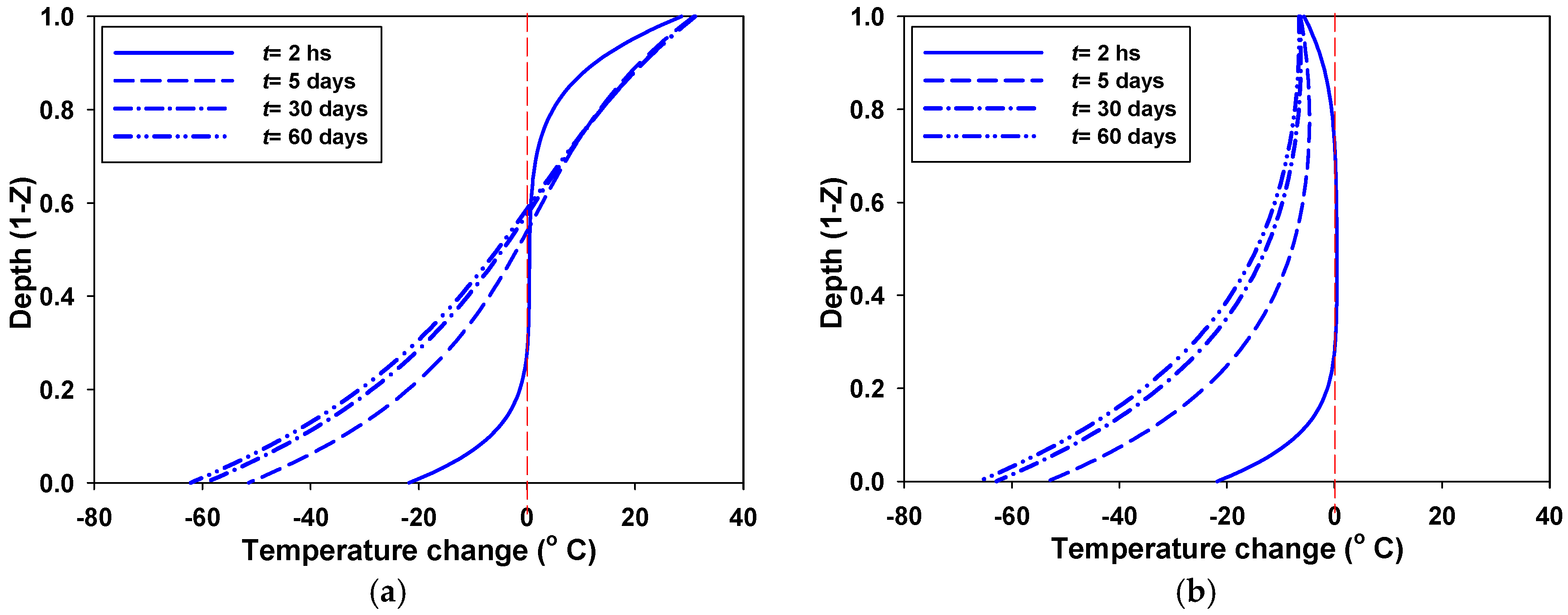
5.2. Temperature Change Near the Wellbore in the Rock
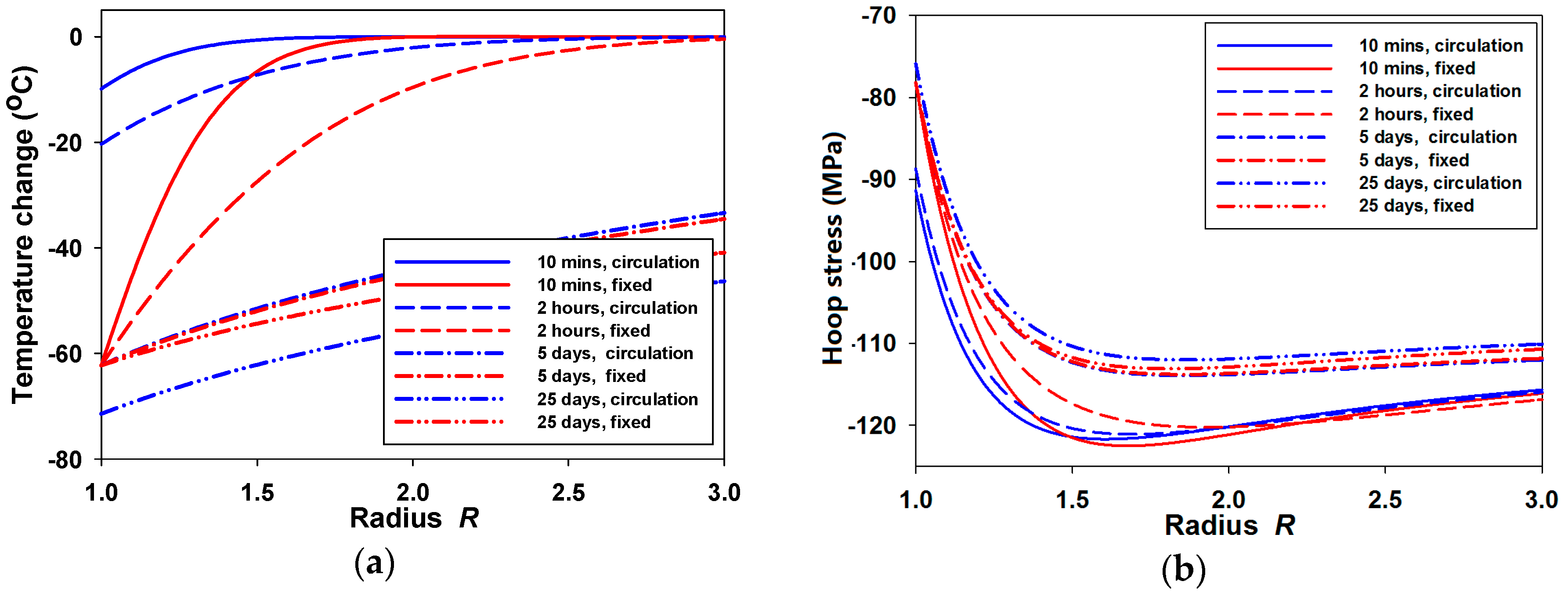
5.3. Comparisons of Near-Well Temperature and Stresses for a System Subject to Fixed and Variable Wellbore Temperature
6. Conclusions
- The fluid circulation rate plays a dominant role in the temperature evolution of the W/R system. The higher circulation rates (say cooling), the larger bottom-hole temperature change and thus the larger induced tensile stresses around the wellbore.
- The effect of the injection fluid temperature on the outlet rock temperature change occurs rapidly. The outlet rock temperature change reaches a value quickly and is almost unchanged after that time.
- The rock temperature of the upper part of the wellbore is determined mainly by the injection condition. It is possible for the upper open-hole section to develop breakouts due to the thermal stresses induced by the heating. Therefore, both wellbore cooling and heating should be taken into account during wellbore stability analysis.
- This work provides a more consistent prediction on the temperature evolution and stress distribution along the wellbore resulting from the variable well temperature profiles associated with fluid circulation, thus making possible a more accurate wellbore stability analysis. The analysis for fixed boundary condition may over- or under-estimate the stress conditions around the wellbore, thus leading to inaccurate prediction of the mud weight density required to maintain a stable well.
- Based on the first two points, varying circulation rates may be a more efficient way to manage bottom-hole temperature and bottom-hole stress conditions, rather than changing injection fluid temperature.
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| (Note: The variables with symbol “^” denote the Laplace transform of the corresponding variables.) | |||
| A0 | Geothermal gradient (°C/m) | Td* | Fluid temperature in the pipe (°C) |
| Aa | Annulus cross sectional area (m2) | Tin* | Injection fluid temperature (°C) |
| Ad | Tubing cross sectional area (m2) | Tr* | Formation temperature (°C) |
| ai | Coefficients defined by Equation (15) (i = 1 or 2) | Tr | Formation temperature change (°C) |
| B0 | Surface soil temperature (°C) | Tw* | Wellbore wall temperature (°C) |
| Bi | Biot number defined by Equation (15) | T0* | Initial formation temperature (°C) |
| b | Coefficient defined by Equation (17) | ur | Radial displacement (m) |
| cl | Fluid specific heat (J/(kg∙K)) | uθ | Hoop displacement (m) |
| cr | Rock specific heat (J/(kg∙K)) | v | Poisson’s ratio |
| cd | Pipe specific heat (J/(kg∙K)) | va | Fluid velocity in the annulus (m/s) |
| c | Coefficient defined by Equation (15) | vd | Fluid velocity in the tubing (m/s) |
| Ci | Coefficients in Equations (25) and (30) (i = 1 or 2) | wa | Annulus width wa = rw − rd (m) |
| D | Hydraulic diameter | z* | Coordinate in the z direction (m) |
| d | Coefficient defined by Equation (17) | Z | Dimensionless coordinate in the z direction |
| dr | Thermal diffusivity for the rock (m2/s) | ||
| e | Coefficient defined by Equation (17) | Greek symbols | |
| f, h | Expressions defined by Equation (29) | α | Volumetric thermal expansion coefficient (1/K) |
| g | Gravitational acceleration (m/s2) | β | Elastic constant defined by Equation (15) |
| G | Shear modulus (Pa) | γ | =αK (Pa/K) |
| had | Overall heat transfer coefficient (W/m2K) | λi | Expressions defined by Equation (31) (i = 1 or 2) |
| ha | HTC between the fluid and formation (W/m2K) | δ0 | Pipe thickness δ0 = rd − r0 (m) |
| hd | HTC between the fluid and inner tubing (W/m2K) | δij | Kronecker’s delta |
| H | Wellbore depth (m) | ρl | Fluid mass density ρl (Kg/m3) |
| Hi | Expressions defined by Equation (46) | ρd | Rock mass density ρr (Kg/m3) |
| kl | Fluid thermal conductivity (W/(m∙K)) | ρr | Pipe mass density ρd (Kg/m3) |
| kr | Rock thermal conductivity (W/(m∙K)) | μ | Fluid viscosity μ (Pa∙s) |
| kd | Pipe thermal conductivity (W/(m∙K)) | ω | Dimensionless rotation displacement |
| K | Bulk modulus (Pa) | σH | Maximum horizontal principal stress (Pa) |
| mi | Constants in Equations (42) and (44) (i = 1, 2 or 4) | σh | Minimum horizontal principal stress (Pa) |
| Na | Nusselt number between fluid and formation | σv | Vertical principal stress (Pa) |
| Nad | Overall Nusselt number | σij | Stress change tensor (Pa) |
| Nd | Nusselt number between fluid and inner tubing | σij* | Total stress σij* (Pa) |
| Nu | Nusselt number | σijR | Initial stress (Pa) |
| Prd | Prandtl numbers for the pipe | Ξij | Dimensionless strain tensor |
| Prl | Prandtl numbers for the fluid | Σij | Dimensionless stress change tensor |
| Prr | Prandtl numbers for the formation | Пw | Dimensionless wellbore pressure |
| pw | wellbore pressure (Pa) | Λ | Expressions defined by Equation (28) |
| p0 | Isotropic far-field stress (Pa) | Ωi | Dimensionless displacement (i = R or θ) |
| P0 | Dimensionless isotropic far-field stress | Θa | Dimensionless annulus fluid temperature change |
| Qd | Injection rate Qd (Kg/s) | Θd | Dimensionless pipe fluid temperature change |
| Qa | Pump out rate Qa (Kg/s) | Θin | Dimensionless injection temperature change |
| Qi | Expressions defined by Equation (31) (i = 1 or 2) | Θr | Dimensionless formation temperature change |
| r | Coordinate in the radial direction (m) | τ | Dimensionless time |
| R | Dimensionless coordinate in the radial direction | χa | Coefficient defined by Equation (17) |
| rd | Pipe outer radius (m) | χd | Coefficient defined by Equation (17) |
| r0 | Pipe inner radius (m) | ζH | Ratio of wellbore depth to the wellbore radius |
| rw | Wellbore radius rw (m) | ζ0 | Ratio of inner pipe radius to wellbore radius |
| Rea | Reynolds number for fluid flow in the annulus | ζd | Ratio of outer pipe radius to wellbore radius |
| Red | Reynolds number for fluid flow in the tubing | εd | Ratio of thermal conductivity of pipe to fluid. |
| R | Dimensionless coordinate in the radial direction | εr | Ratio of thermal conductivity of rock to fluid |
| s | Complex number in Laplace transformation | εij | Strain tensor |
| s0 | Deviatoric far-field stress (Pa) | εv | Volumetric strain |
| S0 | Dimensionless deviatoric far-field stress | Φ | Ratio of production rate to injection rate |
| t* | Time (s) | ||
| Ta* | Fluid temperature in the annulus (°C) | ||
References
- Khaksar, A.; Jalalifar, M.H.; Aslannejad, M. Analysis of vertical, horizontal and deviated wellbores stability by analytical and numerical methods. J. Pet. Explor. Prod. Technol. 2014, 4, 359–369. [Google Scholar]
- Caenn, D.R.; Darley, H.C.H.; Gray, G.R. Composition and Properties of Drilling and Completion Fluids, 7th ed.; Elsevier: Amsterdam, The Netherlands; Boston, MA, USA, 2017. [Google Scholar]
- Rahimi, R.; Asaba, M.; Nygaard, R. Analysis of analytical fracture models for wellbore strengthening applications: An experimental approach. J. Nat. Gas Sci. Eng. 2016, 36 Pt A, 865–874. [Google Scholar] [CrossRef]
- Lee, H.; Moon, T.; Haimson, B.C. Borehole breakouts induced in Arkosic sandstones and a discrete element analysis. Rock Mech. Rock Eng. 2016, 49, 1369–1388. [Google Scholar] [CrossRef]
- Sadeghalvaad, M.; Sabbaghi, S. The effect of the TiO2/polyacrylamide nanocomposite on water-based drilling fluid properties. Powder Technol. 2015, 272, 113–119. [Google Scholar] [CrossRef]
- Khaled, M.S.; Shokir, E.M. Effect of drillstring vibration cyclic loads on wellbore stability. In Proceedings of the SPE Middle East Oil & Gas Show and Conference, Manama, Bahrain, 6–9 March 2017. [Google Scholar]
- Kanfar, M.F.; Chen, Z.; Rahman, S.S. Effect of material anisotropy on time-dependent wellbore stability. Int. J. Rock Mech. Min. Sci. 2015, 78, 36–45. [Google Scholar] [CrossRef]
- Ma, T.; Wu, B.; Fu, J.; Zhang, Q.; Chen, P. Fracture pressure prediction for layered formations with anisotropic rock strengths. J. Nat. Gas Sci. Eng. 2017, 38, 485–503. [Google Scholar] [CrossRef]
- Llanos, E.M.; Zarrouk, S.; Hogarth, R.A. Numerical model of the Habanero geothermal reservoir, Australia. Geothermics 2015, 33, 308–319. [Google Scholar] [CrossRef]
- Bullard, E.C. The time necessary for a bore hole to attain temperature equilibrium. Geophys. J. Int. 1947, 5, 27–130. [Google Scholar] [CrossRef]
- Moss, J.T.; White, P.D. How to calculate temperature profiles in a water injection well. Oil Gas J. 1959, 57, 174–177. [Google Scholar]
- Edwardson, M.J.; Girner, H.M.; Parkison, H.R.; Williams, C.D.; Matthews, C.S. Calculation of formation temperature disturbances caused by mud circulation. J. Pet. Technol. 1962, 14, 415–426. [Google Scholar] [CrossRef]
- Tragesser, A.F.; Crawford, P.B.; Crawford, H.R. A method for calculating circulating temperature. J. Pet. Technol. 1967, 19, 1507–1512. [Google Scholar] [CrossRef]
- Ramey, H.J. Wellbore heat transmission. J. Pet. Technol. 1962, 14, 427–435. [Google Scholar] [CrossRef]
- Raymond, L.R. Temperature distribution in a circulating drilling fluid. J. Pet. Technol. 1969, 21, 333–342. [Google Scholar] [CrossRef]
- Holmes, C.S.; Swift, S.C. Calculation of circulating mud temperatures. J. Pet. Technol. 1970, 22, 670–674. [Google Scholar] [CrossRef]
- Keller, H.H.; Couch, E.J.; Berry, P.M. Temperature distribution in circulating mud columns. Old SPE J. 1973, 13, 23–30. [Google Scholar] [CrossRef]
- Sump, G.D.; Williams, B.B. Prediction of wellbore temperature during mud circulation and cementing operations. J. Eng. Ind. 1973, 95, 1083–1092. [Google Scholar] [CrossRef]
- Arnold, F.C. Temperature variation in a circulating wellbore fluid. ASME J. Energy Res. Technol. 1990, 112, 79–83. [Google Scholar] [CrossRef]
- Kabir, C.S.; Hasan, A.R.; Kouba, G.E. Determining circulating fluid temperature in drilling, workover, and well-control operations. SPE Drill. Complet. 1996, 11, 74–79. [Google Scholar] [CrossRef]
- Fomin, S.; Hashida, H.; Chugunov, V.; Kuznetsov, A.V. A borehole temperature during drilling in a fractured rock formation. Int. J. Heat Mass Transf. 2005, 48, 385–394. [Google Scholar] [CrossRef]
- Wu, B.; Zhang, X.; Jeffrey, R.G. A model for downhole fluid and rock temperature prediction during circulation. Geothermics 2014, 50, 202–212. [Google Scholar] [CrossRef]
- Kirsch, G. Die Theorie der Elastizität und die Bedürfnisse der Festigkeitslehre. Zeitschrift des Vereines deutscher Ingenieure 1898, 42, 797–807. [Google Scholar]
- Hiramatsu, Y.; Oka, Y. Stress around a shaft or level excavated in ground with a three-dimensional stress state. Mem. Fac. Eng. Kyotu Univ. 1962, 24, 56–76. [Google Scholar]
- Biot, M.A. General solutions of the equations of elasticity and consolidation for a porous material. J. Appl. Mech. 1956, 28, 91–96. [Google Scholar]
- Carter, J.P.; Booker, J.R. Elastic consolidation around a deep circular tunnel. Int. J. Solids Struct. 1982, 18, 1059–1074. [Google Scholar] [CrossRef]
- Detournay, E.; Cheng, A.H.D. Poroelastic response of a wellbore in a non-hydrostatic stress field. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1988, 25, 171–182. [Google Scholar] [CrossRef]
- Rajapakse, R.D. Stress analysis of wellbore in poroelastic medium. J. Eng. Mech. 1993, 119, 1205–1227. [Google Scholar] [CrossRef]
- Ekbote, S.; Abousleiman, Y.; Cui, L.; Zaman, M. Analyses of inclined wellbores in poroelastic media. Int. J. Geomech. 2004, 4, 178–190. [Google Scholar] [CrossRef]
- Chen, G.; Yu, L. Consolidation around a tunnel in a general poroelastic medium under anisotropic initial stress conditions. Comput. Geotech. 2015, 66, 39–52. [Google Scholar] [CrossRef]
- Abousleiman, Y.; Cui, L. Poroelastic solutions in transversely isotropic media for wellbore and cylinder. Int. J. Solids Struct. 1998, 35, 4905–4929. [Google Scholar] [CrossRef]
- McTigue, D.F. Flow to a heated wellbore in porous, thermoelastic rock: Analysis. Water Resour. Res. 1990, 26, 1763–1774. [Google Scholar] [CrossRef]
- Kurashige, M. A thermoelastic theory of fluid-filled porous materials. Int. J. Solids Struct. 1989, 25, 1039–1052. [Google Scholar] [CrossRef]
- Charlez, P.A. Rock Mechanics. Volume 2. Petroleum Applications; Éditions Technip: Paris, France, 1997. [Google Scholar]
- Zhou, Y.; Rajapakse, R.K.; Graham, J. Coupled consolidation of a porous medium with a cylindrical or a spherical cavity. Int. J. Numer. Anal. Meth. Geomech. 1998, 22, 449–475. [Google Scholar] [CrossRef]
- Ghassemi, A.; Diek, A. Effects of thermal osmosis on shale instability. In Proceedings of the 4th North American Rock Mechanics Symposium, Seattle, WA, USA, 31 July–3 August 2000. Paper ARMA-2000-0231. [Google Scholar]
- Wang, Y.; Dusseault, M. A coupled conductive-convective thermo-poroelastic solution and implications for wellbore stability. J. Pet. Sci. 2003, 38, 187–198. [Google Scholar] [CrossRef]
- Choi, S.K.; Tan, C.P.; Freij-Ayoub, R. A coupled mechanical-thermal-physico-chemical model for the study of time-dependent wellbore stability in shales. Elsevier Geo-Eng. Book Ser. 2004, 2, 581–586. [Google Scholar]
- Wu, B.; Zhang, X.; Jeffrey, R.G.; Wu, B. A semi-analytic analysis of a wellbore in a non-isothermal low-permeable porous medium under non-hydrostatic stresses. Int. J. Solids Struct. 2012, 49, 1472–1484. [Google Scholar] [CrossRef]
- Wu, B.; Zhang, X.; Jeffrey, R.G. An extended overcore stress measurement method based on a thermo-poro-elastic analysis of the stresses in three-dimensional states. Int. J. Rock Mech. Min. Sci. 2016, 89, 75–93. [Google Scholar]
- Li, X.; Cui, L.; Roegiers, J.C. Thermo-poro-elastic analyses of inclined wellbores. In Proceedings of the SPE/ISRM Rock Mechanics in Petroleum Engineering, Trondheim, Norway, 8–10 July 1998; pp. 443–451. [Google Scholar]
- Abousleiman, Y.; Ekbote, S. Solutions for the inclined borehole in a porothermoelastic transversely isotropic medium. J. Appl. Mech. 2005, 72, 102–114. [Google Scholar] [CrossRef]
- Gao, J.; Deng, J.; Lan, K.; Song, Z.; Feng, Y.; Chang, L. A porothermoelastic solution for the inclined borehole in a transversely isotropic medium subjected to thermal osmosis and thermal filtration effects. Geothermics 2017, 67, 114–134. [Google Scholar] [CrossRef]
- Coussy, O. Poromechanics; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2004. [Google Scholar]
- Mikheyev, M. Fundamentals of Heat Transfer; Peace Publishes: Moscow, Russia, 1960. [Google Scholar]
- Isachenko, V.P.; Osipova, V.A.; Sukomel, A.S. Heat Transfer; Energiya: Moscow, Russia, 1975. [Google Scholar]
- Whillhite, G.P. Over-all heat transfer coefficients in steam and hot water injection wells. J. Pet. Technol. 1967, 19, 607–617. [Google Scholar] [CrossRef]
- Stehfest, H. Algorithm 368: Numerical inversion of Laplace transforms. Commun. ACM 1970, 13, 47–49. [Google Scholar] [CrossRef]


| Parameters | Value |
|---|---|
| Pipe internal radius r0 and thickness δ0 (m) | 0.0462, 0.01 |
| Wellbore radius rw and height H (m) | 0.0762, 4131 |
| Injection rate Qd and pump out rate Qa (Kg/s) | 23.0, 23.0 |
| Injection and surface temperature Tin*, B0 (°C) | 36.5, 27 |
| Formation geothermal gradient A0 (°C/m) | 0.047 |
| Initial temperature T0* (°C) | A0z + B0 |
| Fluid and rock specific heat cl, cr (J/(kg∙K)) | 4200, 790 |
| Pipe specific heat cd (J/(kg∙K)) | 460 |
| Fluid, rock ther. conductivity kl, kr (W/(m∙K)) | 0.68, 2.2 |
| pipe thermal conductivity kd (W/(m∙K)) | 50 |
| Fluid and rock mass density ρl, ρr (Kg/m3) | 900, 2700 |
| Pipe mass density ρd (Kg/m3) | 7800 |
| Fluid viscosity μ (Pa∙s) | 0.0004 |
| Thermal expansion coefficient α (1/K) | 5.0 × 10−6 |
| Max horizontal, principal stress σH (MPa) | −170 |
| Min horizontal, principal stress σh (MPa) | −130 |
| Vertical, principal stress σv (MPa) | −110 |
| Shear modulus G (Pa) | 1.5 × 1010 |
| Poisson ratio v | 0.25 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, B.; Liu, T.; Zhang, X.; Wu, B.; Jeffrey, R.G.; Bunger, A.P. A Transient Analytical Model for Predicting Wellbore/Reservoir Temperature and Stresses during Drilling with Fluid Circulation. Energies 2018, 11, 42. https://doi.org/10.3390/en11010042
Wu B, Liu T, Zhang X, Wu B, Jeffrey RG, Bunger AP. A Transient Analytical Model for Predicting Wellbore/Reservoir Temperature and Stresses during Drilling with Fluid Circulation. Energies. 2018; 11(1):42. https://doi.org/10.3390/en11010042
Chicago/Turabian StyleWu, Bisheng, Tianle Liu, Xi Zhang, Bailin Wu, Robert G. Jeffrey, and Andrew P. Bunger. 2018. "A Transient Analytical Model for Predicting Wellbore/Reservoir Temperature and Stresses during Drilling with Fluid Circulation" Energies 11, no. 1: 42. https://doi.org/10.3390/en11010042
APA StyleWu, B., Liu, T., Zhang, X., Wu, B., Jeffrey, R. G., & Bunger, A. P. (2018). A Transient Analytical Model for Predicting Wellbore/Reservoir Temperature and Stresses during Drilling with Fluid Circulation. Energies, 11(1), 42. https://doi.org/10.3390/en11010042





