Small Scale Organic Rankine Cycle (ORC): A Techno-Economic Review

Abstract

The Organic Rankine Cycle (ORC) is widely considered as a promising technology to produce electrical power output from low-grade thermal sources. In the last decade, several power plants have been installed worldwide in the MW range. However, despite its market potential, the commercialization of ORC power plants in the kW range did not reach a high level of maturity, for several reasons. Firstly, the specific price is still too high to offer an attractive payback period, and secondly, potential costumers for small-scale ORCs are typically SMEs (Small-Medium Enterprises), generally less aware of the potential savings this technology could lead to. When it comes to small-scale plants, additional design issues arise that still limit the widespread availability of the technology. This review paper presents the state of the art of the technology, from a technical and economic perspective. Working fluid selection and expander design are illustrated in detail, as they represent the bottleneck of the ORC technology for small-scale power production. In addition, a European market analysis is presented, which constitutes a useful instrument to understand the future evolution of the technology.

1. Introduction

The continuous increase in energy demand is posing questions on how the network of energy production should evolve in future years. Recent policy decisions [1] set the reduction of pollutant emissions and the improvement in the efficiency of conversion systems as energetic targets. To this end, experts agree that a key approach to reach this goal is the on-site generation of electricity [2]. This is based on the growth of several small power plants that produce electricity directly used in the vicinity of production sites, avoiding transmission losses and lowering carbon emissions. Furthermore, the usage of wasted heat is of importance to achieve the objectives posed by government regulators. In the EU, 800 TWh of thermal energy are discharged every year to the environment as wasted heat from industrial processes [3]. The described scenario has led the scientific community to investigate new technologies capable of producing efficiently localized power. The Organic Rankine Cycle (ORC) received attention since it is capable of producing electrical energy when it is coupled with a renewable energy source and hence converts wasted thermal energy into electricity. These capabilities make this technology suitable for different applications, such as biomass, geothermal, solar and waste heat recovery.

At present, ORC is a mature technology in the MW power range [4]. However, the downsizing of this technology poses challenges that make small-scale ORC still unattractive at the commercial level. In fact, the plant specific cost (€/kW) for small-scale applications is still too high to guarantee a reasonable return on investment. In this work, the specific cost for competitive ORC plants will be quantified as the ratio of ORC unit cost and power output.

The ORC market capacity in the range of 1–100 kW is small, with an approximated installed capacity worldwide of 4.95 MW [5]. Despite the large market potential for small-scale ORC, the high specific cost of the technology makes it currently uncompetitive compared to other existing technologies (e.g., wind, solar, etc.). To this end, the efficiency of the ORC components should be improved to maximize the power production of such power plants, whilst keeping their cost as low as possible. A compromise between these two parameters is essential for the future development of ORC for decentralized power production.

Several ORC architectures have been presented in the literature with added components to the basic thermodynamic processes with the aim of increasing the performance of the system. However, in small-scale ORCs, a simpler plant schematic is usually preferred, which is mainly driven by its capability of a lower specific cost. The cost of the power plant needs to be low enough to guarantee a decent payback period to the end user. Small power plants are intended to be installed in industrial or civil facilities in which specialized technicians are not available to face system breakdowns. Therefore, low pressure levels, limited turbine rotational speed and non-toxic organic fluids need to be used, thus enforcing the limits to the options generally available in ORC design. The aforementioned limitation on thermodynamic and technical parameters lowers the optimal performance of the cycle.

Thermo-economic analysis has been implemented to minimize the cost of ORC systems. However, it is important to mention that the cost engineering techniques often lead to high discrepancy compared to the realistic cost. Lemmens [6] applied cost engineering techniques to estimate the price of an ORC and concluded that the results may diverge by up to +30%. Lecompte et al. [7] proposed a novel framework for the multi-objective thermo-economic optimization of ORC systems. Quoilin et al. [8] show how the optimal working fluid and thermodynamic conditions change when considering as the objective function the minimization of the specific cost of the system rather than the cycle performance. Cavazzini and Dal Toso [9] studied a commercial ORC for small-scale applications to retrofit an internal combustion engine. They stated that the system under investigation was not feasible due to high costs. Whiye and Sayma [10] proposed a method to improve the economies of scale of small-scale ORC systems. They demonstrated that ORC systems can be fitted with a single radial inflow turbine when in the 2–30-kW range of power output.

The design of ORC components is widely discussed in the literature. The need for competitive €/kW systems has led to the investigation of low-cost heat exchanger solutions. Lazova et al. [11] proposed an innovative helical coil heat exchanger for supercritical ORC systems that improved the heat transfer coefficient and hence the cycle efficiency. Bari and Hossain [12] adapted a commercial shell and tube heat exchanger to recover heat from the exhaust gas of an internal combustion engine. From the experimental results, the power output of the engine was increased by up to 23.7%. Longo [13] ran experiments on a Brazed Plate Heat Exchanger (BPHE) using R134aas the working fluid. He claimed that the heat transfer coefficient of the super-heated vapour is from 3–8% higher than the one of saturated vapour. Different experimental setups have installed BPHEs because of their wide availability, low cost and size. BPHEs have been the prevailing choice in small-scale ORC systems that are commercially available. The pump absorbs a percentage of the power produced by the expander, which varies depending on the organic fluid considered. Quoilin et al. [14], in their ORC survey, observed that the power consumption of the pump has a non-negligible impact on the net power the system can deliver as opposed to what occurs in the Rankine cycle. They show that the percentage of the power absorption of the pump may exceed 10% of the power produced by the turbine, when considering fluids such as R 1234yf and R 134a.

Arguably, heat exchangers and pumps are available off-the-shelf in a wide range of specifications and applications. On the contrary, the turbo-generator and the working fluid do not present the same market maturity. The former requires an ad hoc design for each specific application. The latter affects the selection of the proper thermodynamic parameters and, hence, the performance of the system.

Literature studies show that there is no single working fluid that is optimal for every ORC application. Drescher and Brüggemann [15], in their work on fluid selection for the ORC in biomass applications, found out that the family of alkylbenzenes offers the highest cycle efficiencies. Tchanche et al. [16] investigated working fluids for solar applications. They found out that the R134a outperforms the other fluids analysed.

Expanders have a strong impact on the system performance and can be categorized as turbomachines or volumetric. Turbo expanders generally offer higher efficiencies at the cost of a more complex technology, while volumetric expanders are less expensive, but also have reduced performance. Imran et al. [17] provided a comprehensive review of volumetric expanders for low-grade heat recovery. Manfrida and Fiaschi [18] compared the performance of volumetric and turbo expanders suitable for small-scale ORC.

The aim of this paper is two-fold. Firstly, the maximum specific cost of production is quantified. This is defined as the production cost that ORC companies should not exceed to become competitive in the energy market. Furthermore, the mathematical models employed in the literature for the design of the thermodynamic cycle and expanders of ORCs are listed with particular emphasis to articles that combine technical and economical aspects in the design process. In conclusion, the authors suggest a direction to take in the development of ORC for small-scale applications to make this technology successful at a commercial level.

2. State of The Art of The Technology

This section aims at determining the specific cost of production at which ORC technologies become competitive with respect to alternative power producing technologies. The incentive scenario in European countries and supporting case studies are presented to investigate whether it is economical to invest in ORC in different fields of application.

The competitive specific cost for ORC technologies has been estimated from the comparison with the technologies currently available in the market to produce electric power. The specific cost of installed plants that are based on wind [19], solar PVs [20], Internal Combustion Engines (ICEs) [21,22], gas turbines [23] and hydro [24] are shown in Figure 1.

The trend line in Figure 1 is representative of the average specific cost among all of the technologies considered. It is a good approximation to consider ORC competitive whenever the specific cost of the plant falls below this trend line. Based on the results shown in Figure 1, the specific cost of ORC units should not exceed the value of 3500 €/kW and 2500 €/kW, respectively, in the power range of 5–10 kW and 10–100 kW. Notice that the technologies that have a specific cost that is lower than the average value are ICEs, gas turbines and solar. Arguably, all of them present some weaknesses with respect to the ORC technologies, for example: gas turbines and ICEs burn fuel to produce electricity, emitting CO${}_2$. The purchase of the fuel represents an additional cost to operate such plants, while the CO${}_2$ emissions deny access to incentives and increase the emissions of green house gases. On the other hand, PVs do not guarantee a continuous production of electricity during the day and throughout the year.

Generally, an important catalyst for the widespread dissemination of the ORC technology is the price of electricity, i.e., the price at which industries buy electricity from the grid. In fact, those countries in which the specific price of the electrical energy is higher guarantee a more attractive payback period. Figure 2 displays the cost of electricity in European countries for the period 2014–2016 [25].

Italy, Malta, Germany and the United Kingdom are among the countries in which electricity costs are highest, whilst Sweden and Finland are listed among those countries in which the cost of electricity is lowest. Additionally, it is evident from Figure 2 that the fluctuation of the price of electricity has not been high in the last three years. Given the situation depicted with respect to the energy market, the incentive to invest in ORC technology can be related to the actual incentive scenario in European countries, presented in Section 2.1.

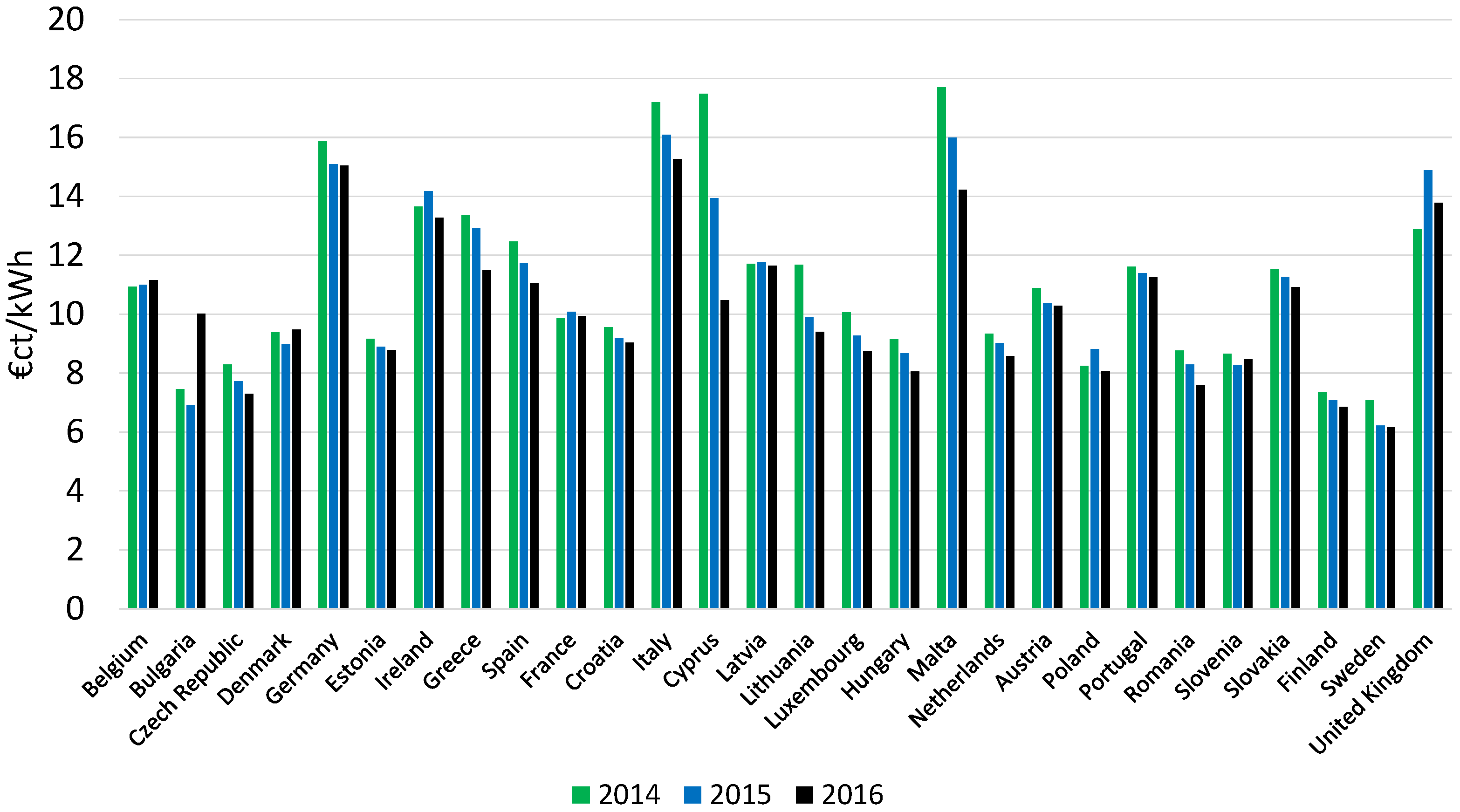
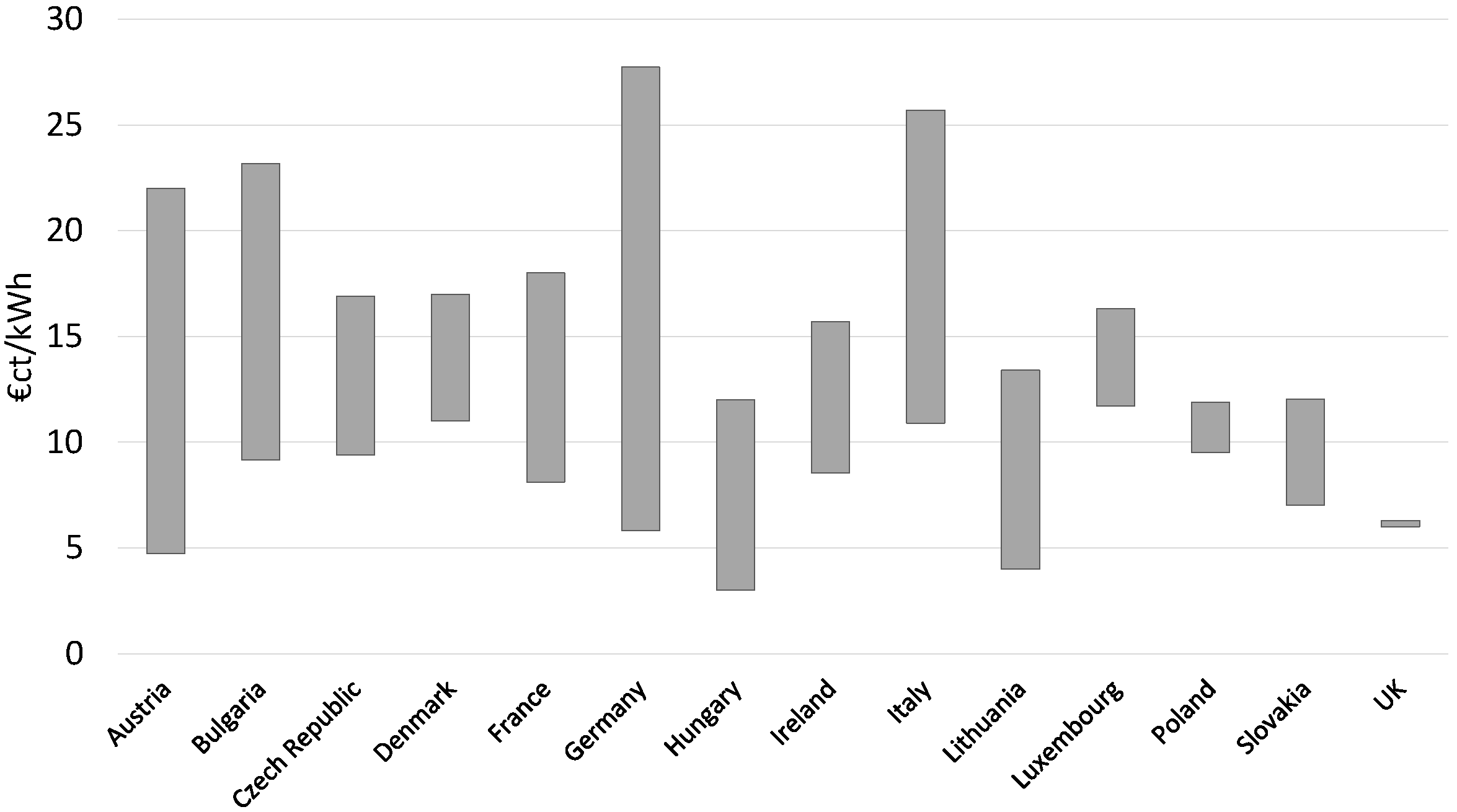
2.1. European Incentives Scenario

The incentives for the ORC market fall under two main categories. On tone side, ORC power plants based on renewable energy sources (such as biomass, biogas, solar thermal or geothermal) may benefit from incentives and subsidies to promote the transition towards an increased share of renewable energy in the overall energy mix. On the other side, ORC power plants based on waste heat sources receive incentives under energy efficiency programs. The former is regulated by guidelines and recommendations as described in Directive 2009/28/EC of the European Parliament and of the Council. The latter is regulated by Directive 2012/27/EU of the European Parliament and of the Council, which promotes energy efficiency.

The European Union is devoted to complying with the Kyoto Protocol in order to control the energy consumption, to increase the share of renewable energy and to improve energy efficiency.

According to the 2009/28/EC directive, it is favourable to support the demonstration and commercialization phase of decentralized renewable energy production due to multiple benefits occurring with such an investment. The European Council set a binding target of 20% final energy consumption from renewable sources by 2020. To this end, each of the Member States is required to develop national action plans (for example, the National Renewable Energy Action Plan, the National Energy Efficiency Action Plan) tailored to their own resources.

The European incentive scheme [26] provides the following incentive systems:

• Feed-in Tariffs (FiTs)
• Premium Tariffs (FiP)
• Green certificates/quota obligations
• Investment incentives
• Auctions/tenders
• Net metering

A Feed-in Tariff (FiT) is an energy supply policy to encourage the spreading of renewable energy technologies. FiT ensures a pre-defined sale agreement for the electricity produced and fed to the grid for a defined contract period (typically 10–25 years), which compensates for the extra costs incurred in regards to investments in renewable technologies. FiTs are independent of market price and ensure a revenue stream to those who install power plants based on the production of energy from renewable sources. The financial support provided is differentiated by technology type, project size and country. For instance, incentives for biogas and biomass are granted based on several factors (e.g., quality, composition, installed capacity). Figure 3 provides the spectrum of FiTs for different European countries for the year 2016 [27].

Figure 3 shows bars that represent the range of incentives provided by different European countries for those who install plants based on technologies eligible for FiTs. Taller bars refer to those countries that provide incentives sorted by renewable technology adopted and plant size. Smaller bars refer to those countries in which the incentives are not differentiated. Notice that the taller bars correspond to the European countries in which the support from FiTs is higher. As can be observed from Figure 3, some countries present more complex subsidies plans (e.g., Germany and Italy) than others (e.g., UK and Poland). The overall amount dedicated to feed-in tariff schemes is recalculated annually.

Premium tariffs offer a premium on top of the market price to those who produce electricity from renewable sources. As opposed to FiT, which guarantee a predictable return on investment regardless of fluctuations in electricity prices, premium tariffs are still susceptible to price variation and consequently stimulate the production of electricity when demand and consequently price is highest.

Green certificates are issued to eligible renewable energy producers based on the energy produced and supplied to the grid. Such energy is sold to the grid at a price that is the sum of the green certificate value and of the current price of the electricity. Energy suppliers are obliged to include a specific amount of renewable electricity (quotas) in their portfolio. By purchasing green certificates from renewable energy generators, they can fulfill this requirement.

Investment incentives include subsidies, as well as low-interest, long-term loans paid up front for renewable projects.

Table 1. Incentives on the investment cost [28].

Country	Cost Covered (%)	Amount Awarded (k€)	Note
Belgium	5–50	80–1500	Min invest. 7.5–25 k€
Bulgaria	15–70	57	-
Czech Republic	50–70	3600	-
Finland	30–40	-	-
France	20–40	1000–5000	-
Germany	30	-	Min invest. 30 k€
Italy	25	-	-
Latvia	-	2000	-
Lithuania	80	200–1500	-
Luxembourg	40–45	-	-
Poland	100	-	Min invest. 45.53–113.826 k€, 40 kW
Romania	50	2000	Own consumption only
Slovenia	50	200	-

reports the investment incentives awarded by different European countries [28].

Auctions represent an alternative to FiTs. New power plants that are based on renewable technologies (e.g., biomass, solar, geothermal, etc.) can benefit from FiTs. Existing power plants can receive subsidies via auctions. Operators simultaneously submit sealed bids to obtain subsidies for a certain allocated capacity. The lowest bidder wins, ensuring an extra reward, which sums up with the price at which the grid pays its electricity. This way, the most cost-effective projects are awarded.

Net-metering [29] is a support scheme for which the generated electricity is fed back to the grid and offsets the consumption. In the case of excess production, there is no reimbursement, but it might be carried forward to the next billing period.

Figure 4 shows the cost of electricity and the price at which the owner of a power plant can sell the electricity generated to the grid in absence of incentives in the year 2016 in several European countries [25].

The benefits of installing ORC technology can be two-fold. There is the option for those factories that consume electricity to produce it through an ORC plant as opposed to buying it from the grid at its market price. Alternatively, there exists the opportunity to install an ORC plant with the objective to obtain an income from selling the electricity produced back to the grid. In the former case, the investor can calculate the return on investment based on the savings on the energy bill plus the incentives from feed-in tariffs (if any). In the latter case, the investor covers his/her expenses through the earnings of selling the energy and the subsidies resulting in a reduced investment cost (see Table 1).

The ORC technology attractiveness depends on the Payback Period (PBP) offered to the end user. In this study, it has been assumed that a three-year PBP guarantees the diffusion of a technology.

Different possible commercial scenarios are analysed to provide an overview of the convenience for ORC companies to run businesses in this sector, assuming that the production cost lies on the trend line presented in Figure 1. For the sake of simplicity, it has been assumed that the ORC plant is produced and sold from an ORC company directly to an end user (i.e., an industrial company, an engine operator, etc.). The revenue that an ORC company can achieve has been evaluated, considering a three-year PBP for the end user. As a worst case scenario, the absence of investment incentives has been considered. Specifically, three different business cases are investigated:

• Case 1: An industrial company invests in the installation of an ORC system to produce the electrical energy demand of its factory. The ORC plant is coupled to process waste heat. The investment is paid back from the savings on the energy bill since the electricity is not purchased any more at the market price from the grid.
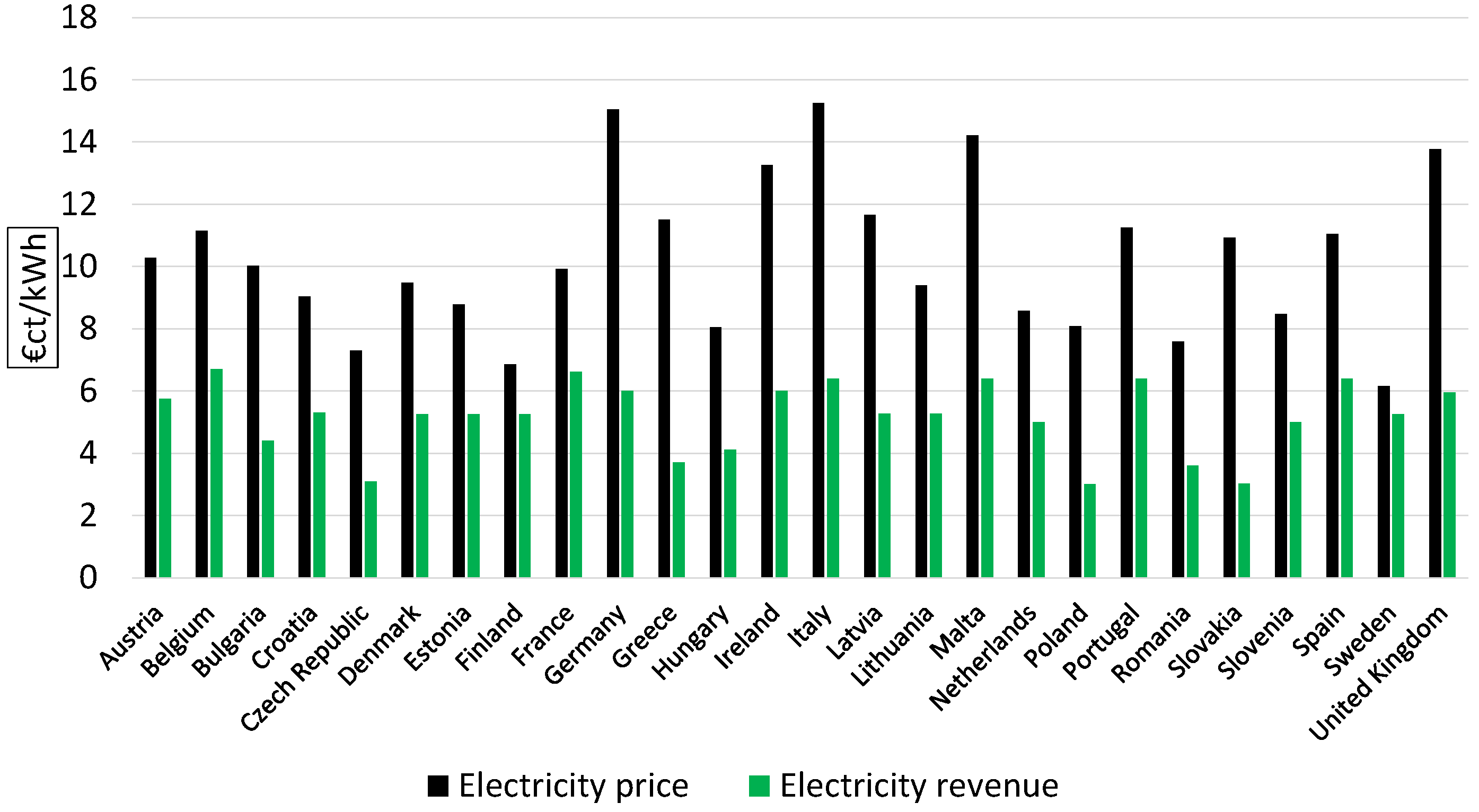
• Case 2: A stationary engine operator installs an ORC unit to generate additional power by recovering the thermal energy in the exhaust gasses to sell it to the grid at the actual market price. In this case, the income depends on the price at which the grid purchases the energy produced by the ORC plant (see Figure 4).
• Case 3: An ORC plant is installed to produce electrical energy from a renewable energy source, such as biomass, solar or geothermal energy. Such a plant is eligible for the feed-in tariff.

Notice that Cases 1 and 2 refer to the ORC technology applied to waste heat recovery systems, which is not considered as a renewable energy. In this circumstance, incentives are not provided. Case 3, instead, takes advantage of the incentives for the production of electricity from a renewable source.

Italy, Germany, the United Kingdom and France have been investigated in this work. However, the reasoning can be easily extended to different countries. As concerns case 3, an average value for the feed in tariffs has been considered for each country analysed among those presented in Figure 3. Specifically, 20 €ct/kWh, 17 €ct/kWh, 7.92 €ct/kWh and 9.745 €ct/kWh have been selected respectively for Italy, Germany, the United Kingdom and France. In addition, the United Kingdom provides an extra 5.78 €ct/kWh benefit to the end users who export energy to the grid, which has been included in the calculations. The ORC plant has been assumed to operate 85% of the time, which corresponds to 7446 h/year. The operation and maintenance (O&M) costs have been considered equal to 3 €ct/kW. The time value of the money and the opportunity cost have been evaluated applying a 4% discount rate [30]. Furthermore, a 2% inflation rate has been considered. Therefore, the calculated nominal discount rate is 6.08%. Lemmens [6], in his cost analysis for a 375-kW ORC system, considers that the integration costs are 11% of the total cost of the plant. The impact of the installation costs decreases with the size of the plant. It must be noted that the integration costs have not been included in this study, primarily because they depend highly on the plant and heat source whereat the ORC will be coupled. ORC companies are aiming at creating semi-independent ORC systems for low power outputs, effectively minimizing installation costs. A high level of commercial maturity for small-scale ORC systems is likely to come from applications that require low customization and enable high volume sales. Such applications include waste heat recovery from ICEs and gas turbines, where integration costs are likely to be lower. Instead, typical large-scale ORC systems have been applied to applications that require high levels of customization such as geothermal and industrial wasted heat, where integration costs are inevitably considerably higher. For the aforementioned reasons, although such costs for commercial systems can be as high as 10%, installation costs are not considered in the current analysis.

It has been considered that the ORC companies install the plant at the specific cost identified in Figure 1 and that they sell it at a price that guarantees a payback period of three years to the end user. Therefore, the revenue for the ORC companies is calculated as the difference between the price at which they sell the ORC plant to the end user and the cost of production determined using the data in Figure 1.

Figure 5a–d reports the results of the analysis.

As expected, the revenue for the ORC companies is greater in those countries where the price of the electricity and the incentives are higher. Figure 5 highlights the minimum plant size at which an income is guaranteed to the ORC company, in case it succeeds in producing the system at the specific cost identified by the trend line of Figure 1. Each case will now be analysed independently: in Case 1, the revenue obtained by the ORC company is calculated considering an initial investment cost, based on the data depicted in Figure 1. Assuming that the ORC plant operates 7446 h/year and that the end-user saves an amount of money that depends on the cost of the electricity in the country of interest (see Figure 4), the revenue is calculated as the difference between the actualized savings in the energy bill in the first three years of operation of the plant and the initial investment cost. For example, in Italy, Germany and the United Kingdom, an ORC company would benefit from producing ORC plants with a power output above 10 kW (see Figure 5a–c), while in France, an ORC company would make no profit for the installation of ORC plants of power output below 100 kW (see Figure 5d). In fact, the cost of electricity in France is lower with respect to that of the other countries under investigation (see Figure 4), which leads to lower savings on the energy bill.

Case 2 considers the installation of an ORC plant to produce electricity from the exhaust gas of a stationary engine. It has been assumed that the electricity produced is sold back to the grid at the price indicated in Figure 4. Considering that the ORC plant operates 7446 h/year during the three-year pay-back period, the revenue for the ORC company is calculated as the difference between the actualized income generated by selling the electricity produced to the grid and the initial investment cost. None of the analysed countries allow the ORC companies to obtain a revenue from the investment scheme as described in Case 2, when the power output of the ORC is below 200 kW (see Figure 5a–d). It can be concluded that the current scheme of incentives needs to be improved to push ORC companies to invest in the recovery of wasted heat for the production of energy to sell to the grid. For example, the revenue from selling the electricity to the grid could be augmented with respect to the current values, shown in Figure 4.

Case 3 investigates the installation of ORC systems coupled to renewable energy sources. When an ORC system is used to exploit the energy produced from a renewable source, the end-user benefits from the FiTs. Therefore, it is possible to calculate the revenue for the ORC company as the difference between the income obtained in the first three years and the initial investment cost. As illustrated in Figure 5a–d, the installation of ORC systems for applications that make them eligible for FiTs results in the highest revenue for the ORC companies, among the options analysed. In Italy and Germany, where the cost of electricity is high (see Figure 4), the installation of ORC systems guarantees the company an income when the power output is above 5 kW. In the United Kingdom, it is convenient to install an ORC, under the incentive scheme of FiTs, if the power production of the plant is above 20 kW. Finally, in France, the option is not cost effective for a power production below 100 kW.

2.2. ORC Market Analysis

Section 2.1 reported the relative advantage of investing in ORC technology depending on location and pay-back scheme. Section 2.2 reports the companies currently involved in the design and commercialization of ORC systems for the production of power in the small-scale range, i.e., below 100 kW.

Table 2. Non-exhaustive list of small-scale ORC system producers.

Company Name	Country	Power (kW)	Expander Type	Heat source T (°C)	Notes
Exergy [33]	IT	100–240,000	Radial	-	Commercial
Triogen [34]	NLD	160	Axial	200–300	Patent on expander coupled with the pump
Enogia [35]	FR	10–20–40–100	Radial	90–160–400	Commercial, turbine coupled with high speed generator
Rainbow [36]	FR	100	Axial	-	Expander 12–15 krpm, efficiency > 80%
Entropea Labs [37]	UK	20–300	Radial	400–500	Prototype
ElectraTherm [38]	USA	35–65–110	Screw	77–122	Commercial, induction asynchronous generator brushless
Zuccato Energia [39]	IT	30–40–50	Radial	Water T > 94	Commercial, synchronous generator 15 krpm, ceramic bearings
GE clean energy [40]	USA	125	Radial	143	Commercial, R 245fa, rotational speed 26.5 krpm, magnetic bearings
Infinity turbine [41]	USA	5–50–100	Radial	<110	fluid R 245fa, magnetic bearings
Pratt and Whitney [42]	USA	80–260	Radial	91–149	Fluid R 245fa, 2 pole induction machine
Termo 2 Power [43]	PL	<300	Rotary lobe	-	Prototype, 1.5–3 krpm, self exciting synchronous generator
Calnetix [44]	USA	125	Axial	Low	24.5 krpm, magnetic bearings
Mattei [45]	IT	3	Vane	80–150	-
Rank [46]	SP	50–100	Radial	85–140	2–5 years payback period
EXA [47]	IT	15–150	Piston/screw	70–350	Fluids R 134, R 245fa, Toluene, Induction generator
NewComen [48]	IT	3–120	-	-	-
Orcan [49]	GER	20	Radial	550	-
ConPower [50]	GER	13–75	-	-	Prototype
Clean power [51]	USA	77	Scroll	270	Expander speed 1.5–1.8 krpm, fluid R 245fa
ZE [52]	UK	95–130	Multi stage radial	-	Permanent magnet generator
ICENOVA [53]	IT	10–30	Eneftech scroll	150	R 245fa, Regenerated cycle
Climeon [54]	SWE	150	Turbine	70–120	-
Exoes [55]	FR	15	Piston swashplate	-	Transport applications
E-rational [56]	BEL	<500	Single screw	105–150	Asynchronous generator
Opcon [57]	SWE	<800	SRM Turbine	250	-

shows a list of existing ORC companies in the small-scale range. It has to be noted that all companies to the authors’ knowledge have been included; however, it can be expected that this list may not be all-inclusive.

To date, companies that develop ORC plants in the MW range, such as Turboden, Ormat and Enertime, have not been trying to expand their business towards the small-scale market. This constitutes an additional proof that scaling down this technology is not straight forward.

Table 2 reveals that companies are trying to gain competitive advantage designing ad hoc expanders. In fact, the expander design together with the working fluid selection represent the main unsolved problems in this field. Most of the companies, active in the market, opted for a turbo expander. However, it can be noticed that volumetric expanders are preferred when the power output reaches values as low as 30 kW. Moreover, the temperature of the heat source varies over a wide range depending on which market sector companies are trying to reach. Generally, most of the low temperature heat sources (i.e., below 300 °C) refer to geothermal or solar applications and low temperature wasted heat (e.g., in the engine cooling). High temperature heat sources (i.e., above 300 °C) are typical of waste heat recovery from high-grade sources and biomass applications.

Despite of the relatively large number of companies outlined in Table 2, most of them are still developing prototypes. The reason being that the specific cost depicted in Figure 1 represents a difficult target to reach.

Scientists are putting much effort into the development of new methods to improve the performance of small-scale ORCs. The research in the ORC field is not recent. In 1964, Tabor and Bronicki [31] published a work in which they investigated suitable working fluids for small vapour turbines. In 1984, Angelino et al. [32] presented a review of the Italian activity in the research field of ORC. Section 3 and Section 4 outline the methods proposed in the literature for the selection of the optimal working fluid and expander. The analysis that follows does not aim at being conclusive, nor at presenting original results. The purpose of Section 3 and Section 4 is to provide the reader with the state of the art of the ORC technology in small-scale applications.

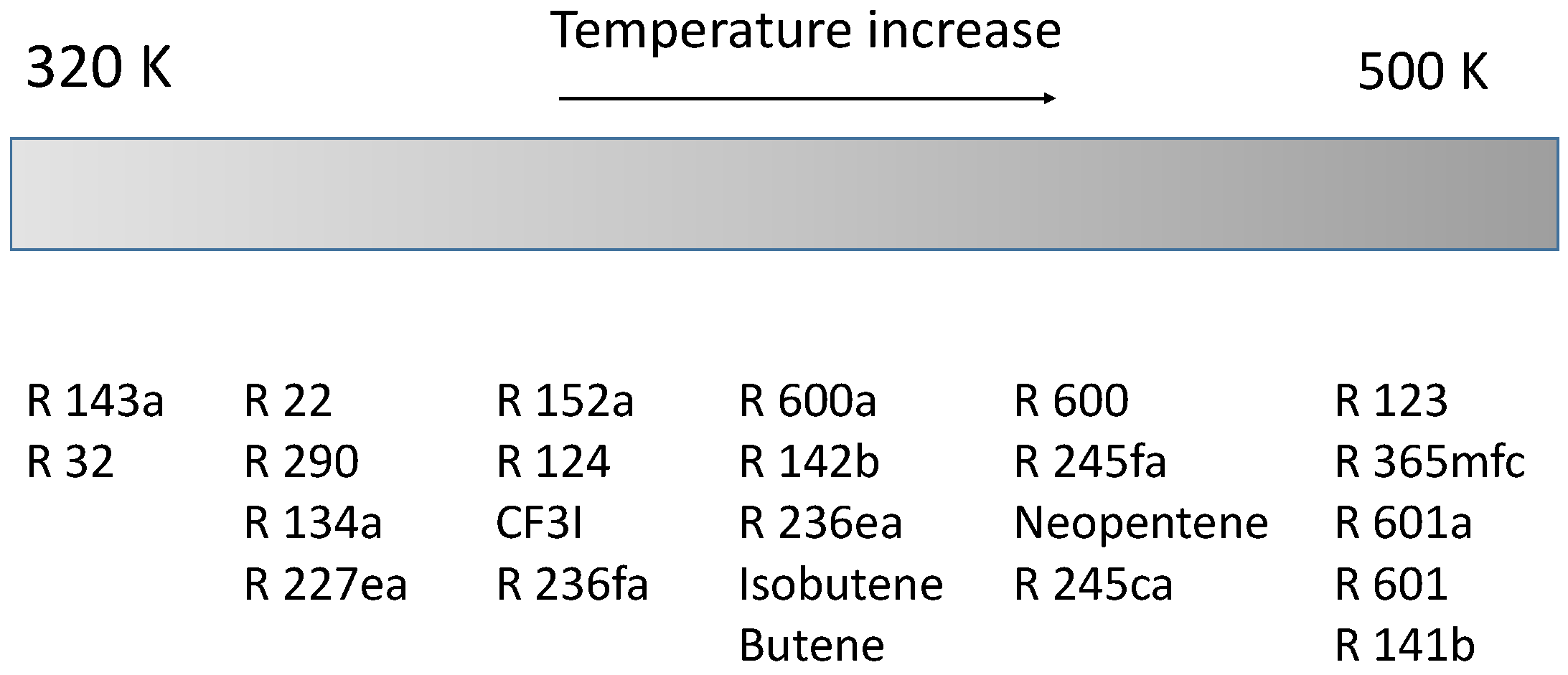
3. Working Fluid Selection

The selection of the working fluid is a key aspect in the design of ORC systems. The literature reports hundreds of studies on this topic. However, the authors did not identify a single fluid that is suitable for all applications.

For example, Figure 6 reports a work performed by Wang et al. [58] that clearly highlights that the optimal working fluid is strongly dependent on the temperature of the heat source. The working fluid selection has an impact on the thermodynamic performance, size and cost of the system. Therefore, its correct selection is crucial at the design phase of an ORC plant.

The aim of this section is two-fold. Firstly, the most common methodologies used by ORC experts to tackle the problem of working fluid selection are outlined. Subsequently, the effect of the fluid thermo physical parameters on the design of the ORC components is discussed.

The optimal organic fluid has to be selected among dozens of different options available. As a first step, it is useful to categorize fluids into different groups. A first categorization can be based on chemical composition. Organic compounds can be classified as alkanes, fluorinated alkanes, ethers, fluorinated ethers, aromatics, linear siloxanes, PFCs, HFOs, HFCs, etc. Another major classification is based on the slope of the saturated vapour curve, whereby fluids are categorized as wet, isentropic and dry. Wet fluids, isentropic fluids and dry fluids present, respectively, a negative, an infinite and a positive slope of the saturated vapour curve.

According to Bao and Zhao [59], dry and isentropic working fluids are to be preferred in ORC applications. This is for multiple reasons. As opposed to wet fluids, dry and isentropic fluids can remain in the vapour phase throughout the expansion process, thus avoiding the erosion of the blades. Furthermore, dry and isentropic fluids allow one to minimize the level of de-superheating, hence reducing the overall heat transfer surface, which in turn lowers the cost of the system. Bao and Zhao [59] underline that the use of an extremely dry working fluid implies the need for a regenerated cycle to improve the performance of the system, which might increase the size and cost of the plant. It can be concluded that there exists a trade-off between performance and cost that strongly depends on the selection of the type of organic fluid.

An additional categorization of organic fluids is dictated by government regulators. The increase in popularity of organic compounds in several applications led to the phasing-out of fluids that are dangerous for people and the environment. GWP (Global Warming Potential) and ODP (Ozone Depletion Potential) measure the impact of fluids to the environment [60]. The National Fire Protection Association (NFPA) developed a system to quantify the equivalent health, flammability and reactivity for chemicals commonly used in industry [61]. ASHRAE (American Society of Heating, Refrigerating and Air-Conditioning Engineers) published several handbooks offering guidelines for the selection of proper engineering fluids [62].

The first step in the process of fluid selection is to pinpoint a limited number of fluids that meet the requirements dictated by government regulators. Then, thermo-physical properties, such as autoignition temperature, deterioration temperature and freezing point, are typically checked to shortlist a few fluids that could be suitable for the thermodynamic conditions of the heat source. In particular, the deterioration temperature plays a crucial role in the determination of the reliability of the system. In fact, when deterioration occurs, the organic fluid needs to be replaced in the ORC plants, which constitutes a non-negligible cost. Erhart et al. [63] pointed out that the recovery of the used working fluid that did not experience deterioration can significantly reduce the operating cost of ORC systems. The application sets the maximum temperatures that the working fluid will see and the thermal capacity of the heat source. Following a preliminary screening of the fluids based on the aforementioned properties, the remaining candidates are studied in detail to make a final decision on the fluid that best suits the specific requirements of an application. Tchanche et al. [16] compared the performance of twenty working fluids in solar applications, considering thermodynamic performance and environmental properties. They highlighted that flammability is a key factor in the selection process of the working fluid. Papadopoulos et al. [64] introduced the CAMD (Computer-Aided Molecular Design) technique to select the optimal working fluid for ORC applications. They took into account technical, economic and safety aspects in ranking conventional and non-conventional fluids.

The performance of the working fluid in an ORC system is evaluated by means of the thermodynamic analysis. Different methods have been proposed to perform the thermodynamic analysis of the ORC. Those are based on steady or unsteady (dynamic) models. The reader is referred to the work of Ziviani et al. [65], who presented an extended review of the modelling tools employed in the design of ORC systems. Linke et al. [66] reviewed the approaches developed by researchers for the systematic selection of working fluids and the design, integration and control of ORCs. Mago et al. [67] investigated four different dry fluids in basic and regenerative ORC configurations. They ranked the fluids combining first and second law efficiency. Saleh et al. [68] investigated 31 different organic fluids for low temperature ORCs. Drescher et al. [15] discussed ORC fluid selection in biomass applications, based on the efficiency of the system. Rayegan and Tao [69] proposed a procedure to compare ORC working fluids. He screened them in terms of thermal efficiency, net power generated, vapour expansion ratio and exergy efficiency. Dai et al. [70] used a genetic algorithm to compare organic fluids using exergy efficiency as the objective function. They demonstrated that organic fluids perform better than water in exploiting energy from waste heat sources at low temperature. In particular, R236ea resulted in being the best performing among the ten fluids considered. Qiu [71] introduced the bucket effect and the spinal point method to select the proper working fluid for ORC applications. Some authors investigated zeotropic mixtures through steady state analysis to understand if they can offer any advantage. Angelino and Di Paliano [72] investigated fluid mixtures for ORC applications. They pointed out that the use of mixtures complicates the design of components in that fluid fractionation (i.e., the separation of a chemical compound into components) should be avoided in the heat exchangers during phase change. It is important to note that the state of the art in the modelling of ORC systems is based on steady state conditions.

Dynamic models characterize the behaviour of the system under transient conditions. This type of analysis is extremely important for applications in which the duty cycle of the heat source presents fluctuating behaviour such as vehicle or solar applications. The dynamic behaviour of ORC systems depends on the working fluid considered. In particular, the heat transfer properties of the organic fluid play a crucial role in the definition of the dynamics of the system. In fact, the heat transfer properties of the working fluid affect the design of the heat exchangers, which are the components with the highest time constant in an ORC system. The working fluids with good heat transfer properties allow for the design of more compact heat exchangers, which in turn enhances the ability of ORC to both reduce cost/weight and to react faster to varying conditions of the heat source. Fast reacting systems are crucial in applications where the heat source is subject to a duty cycle and therefore does not have constant thermodynamic conditions (e.g., temperature and/or mass flow rate). Several works aim at defining a proper control strategy to maximize the performance of the ORC over the whole duty cycle of the heat source. Quoilin et al. [73] proposed a dynamic model to study the behaviour of a small-scale ORC for waste heat recovery applications. Particular emphasis was given to the transient behaviour of the heat exchangers to design a proper control system. Desideri et al. [74] compared the moving boundary and finite volume techniques in the design of heat exchangers for ORC applications. Zhang et al. [75] studied a multi-variable control strategy for a 100-kW ORC system.

Some works consider a “black box” analysis, in which the ORC system is studied at the process level. Maizza and Maizza [76] considered fixed values for the efficiency of the components. Sciubba et al. [77] used a black box analysis to simulate a dual loop ORC for marine applications. This implies that the thermodynamic parameters selected might affect negatively the design of the components. For this reason, some authors have proposed methods in which the selection of the thermodynamic parameters is bound by constraints that arise from practical limitations in the component design [78,79]. Furthermore, some works considered thermo-economic optimization models [80,81]. In such models, researchers identify which components are more responsible for the high specific cost of small-scale ORC systems. Only a few papers deal with fluid selection procedures based on simultaneous optimization of thermodynamic performance and component design. Quoilin et al. [82] compared screening methods and operating map methods as the most common ones considered in the literature. They state that screening methods are the most commonly used in the literature and that they can be misleading in the process of fluid selection. The operating map methods deal with the interaction between working fluid and expander type. He considered a radial inflow turbine, a screw and a scroll expander. Franchetti et al. [83] considered the operating map method to select the proper working fluid for an ORC in which a radial inflow turbine is selected as the expander.

Based on the literature survey, the authors provide general guidelines to underline those properties of the working fluid that have an impact on the specific cost of small-scale ORCs:

• Price: Since organic fluids are expensive (20–30 €/kg), it is important to find the correct trade-off between cost and performance [71]. Generally, fluids used in operating ORC plants are extensively used in other fields, which lowers their price.
• Density: Chen et al. [84] underline that low density leads to a high volumetric flow rate of the working fluid. This has an impact on different components of the system. The higher the volumetric flow rate, the bigger the size of the components and, in turn, their cost. However, a high volumetric flow rate allows for the reduction of the rotational speed of the expander, which has a positive impact on the reliability of turbo-expanders.
• Condensation pressure: Ideally, the condensation pressure should be as close as possible to atmospheric. In fact, a high condensing pressure leads to an increase in the overall system pressure, which requires more resistant and therefore more expensive materials. Condensing pressures below 0.5 bar leads to an increase in the sealing costs to prevent air from entering the system. Moreover, lower pressures increase the size of the condenser. [14].
• Freezing point: The freezing point needs to be well below the minimum ambient temperature in the site in which the system is installed, to avoid solidification of the working fluid during periods of inactivity of the ORC plant [85].
• Cycle top pressure: It is important to keep the cycle top pressure well below the fluid critical pressure [86]. This is necessary to prevent the formation of liquid droplets during the expansion process and to overcome instability during vaporization. Low pressure allows for the use of less expensive materials, which has a positive impact on system costs. High pressure implies high fluid density, which in turns lowers the system size. A trade-off has to be found through a techno-economic analysis.
• Heat transfer coefficient: The heat transfer coefficient plays a crucial role in the definition of the size and cost of the heat exchangers [87]. The selection of an organic compound with good heat transfer properties would lead to the reduction of the heat transfer surface, which, in turn, lowers the overall size, weight and cost of the ORC system [88].
• Fluid decomposition temperature: Organic fluids suffer from chemical decomposition at high temperature [89]. For this reason, it is important that the temperature of the heat source in the evaporator does not overcome the decomposition temperature of the working fluid.
• Molecular weight: Fluids with high molecular weight allow for a smaller rotational speed of the expander [90], which in turn affects positively its efficiency and typically diminishes the cost of the generator.

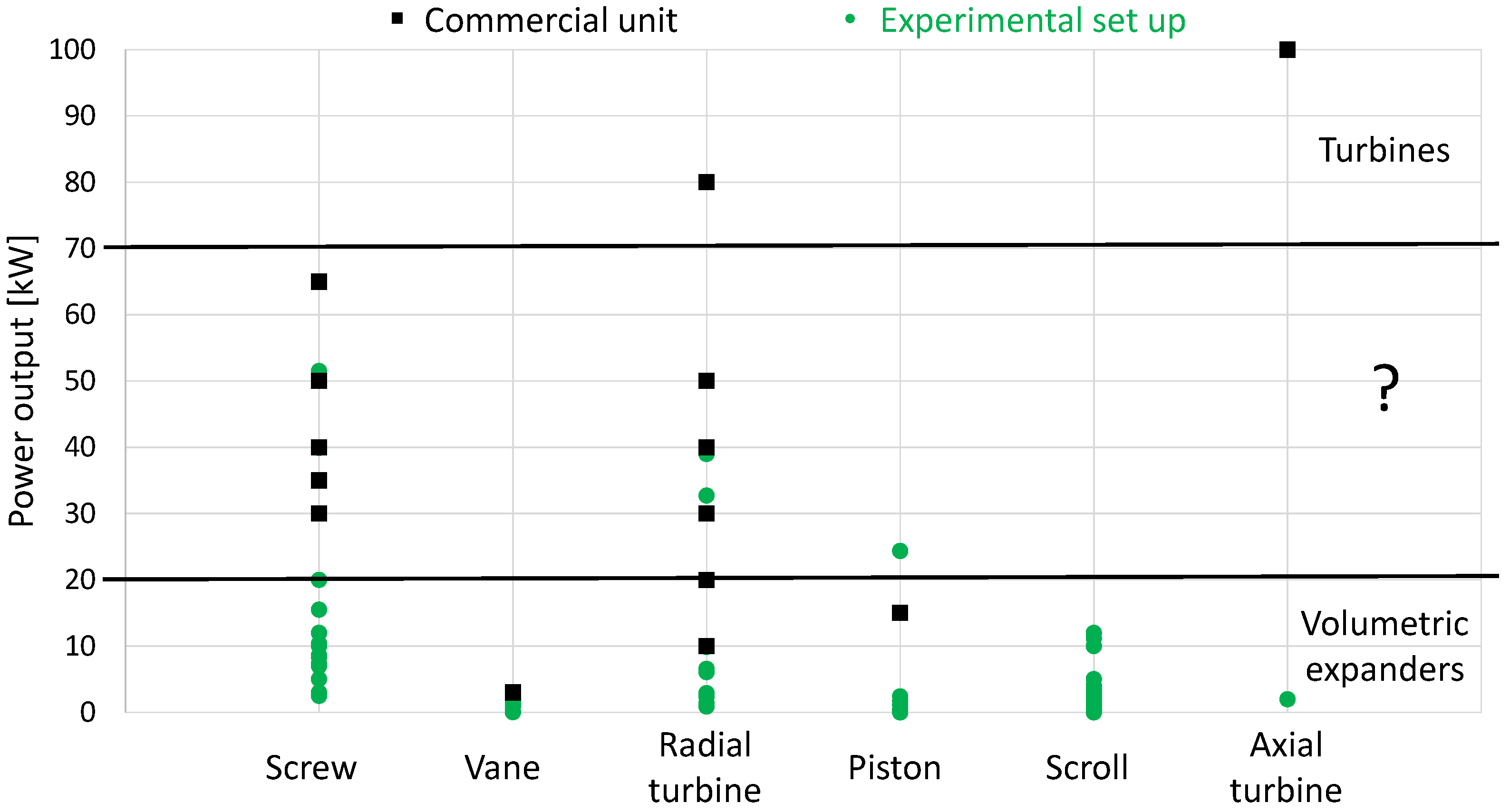
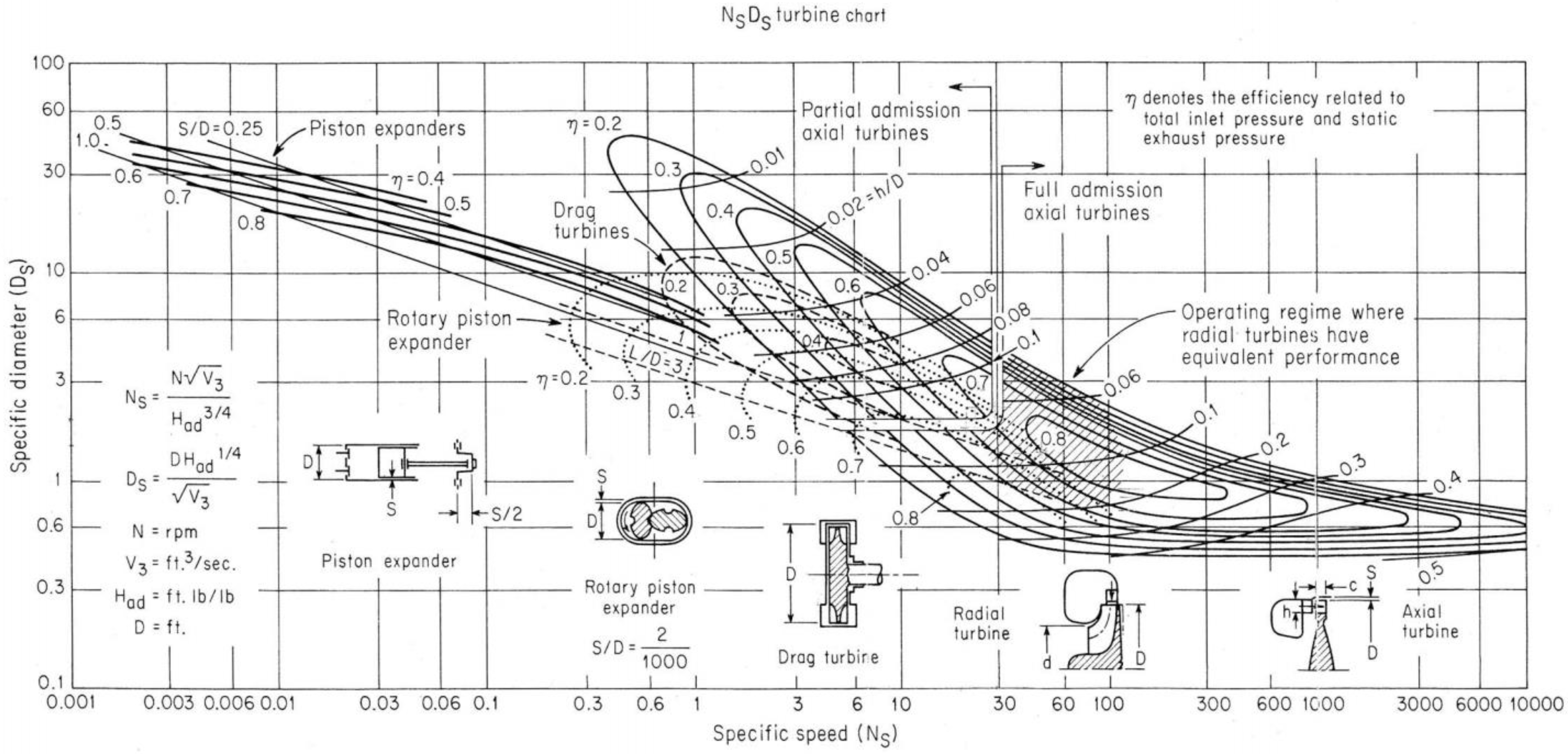
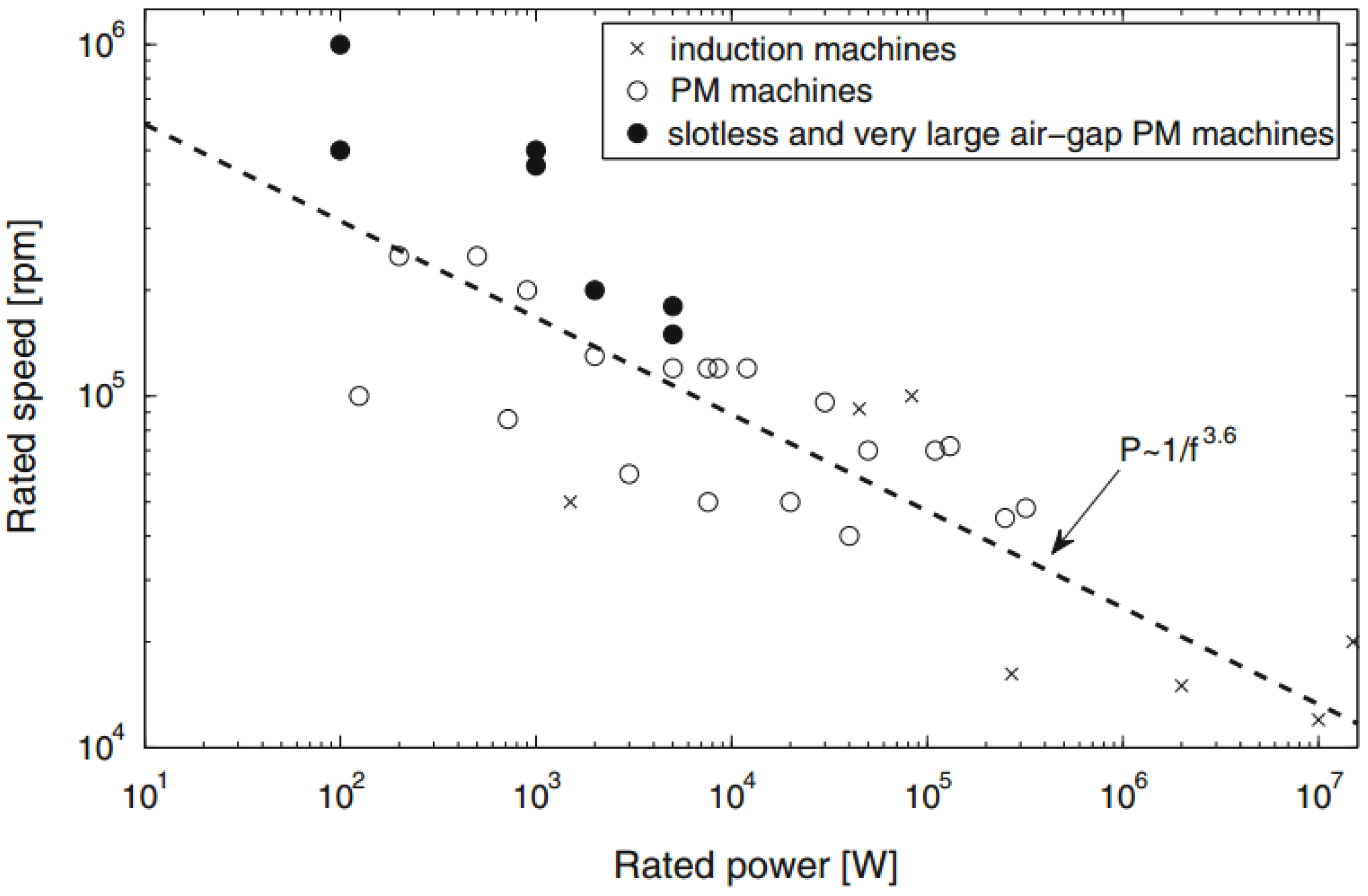
4. Expander Selection

The choice of the appropriate expander for small power ratings represents an unresolved problem. Literature reviews on small-scale expanders propose different configurations [86]. The options available present both pros and cons that complicate the selection of a single “ideal” expander.

As is clear from Table 2, ORC companies are trying to develop their expanders in house. As testified by Qiu et al. [86], the commercial market lacks appropriate expanders for small-scale applications.

The expander is certainly the ORC component for which the highest drop in performance arises when downsizing ORC plants from the MW to the kW power range. Efficiencies reported in the literature [17] show values below 70%.

Expanders can be categorized into two main groups: volumetric and turbo expanders. Scroll, screw and vane expanders are the most common machines among the volumetric ones [91,92,93]. Li et al. [94] recently proposed a piston expander with the aim of reducing the component complexity and cost. A detailed explanation of the available mathematical models goes beyond the scope of this work. For an in-depth analysis, the interested readers can refer to the work of Imran et al. [17] who reviewed the published mathematical models for the design of volumetric expanders.

Radial inflow, radial outflow and axial turbines are the most common turbo-machines in the literature [95,96,97]. As opposed to volumetric machines, turbo-machines are convenient in the high power output range, while they become inefficient for low power production. The reason for this is mainly related to very high rotational speeds leading to bearing failures. Imran et al. [17] suggest that their high rotational speed and their cost are the reasons for which radial inflow turbines are not suitable for power ratings below 50 kW. Qiu et al. [86] state that turbine expanders are used for power outputs greater than 50 kW, because the efficiency substantially drops below this value. Models for the design of turbines are available in the literature. Rahbar et al. [98] and Fiaschi et al. [99] proposed detailed models for radial turbines. Palumbo et al. [100] published the mathematical model of a radial outflow turbine. Jubori et al. [101] and Lazzaretto and Manente [102] derived a model for the design of axial turbines in ORC applications. However, the aforementioned studies have not been validated against experimental data. Kang [103] presented a model coupled to an experiment of a radial turbine for ORC applications using R 245fa as the working fluid. The maximum efficiency achieved during the experimental campaign was 78.7%, and the maximum power output was 32.7 kW.

Generally, volumetric expanders are considered when the power output is low. In fact, when the power output exceeds a certain level, the performance lowers and the size increases exponentially, increasing its cost and reducing its practicality. Leibowitz et al. [104] in their analysis on cost-effective small-scale ORC systems identify that in the range of 20–50 kW, twin screw expanders are the most promising. They also state that this kind of volumetric expander offers efficiencies of 70% during low speed operation. Such small-scale ORC systems can be installed at a cost of about 1500–2000 €/kW.

Kenneth and Nichols [105] presented a graph that provides a guideline to select the proper expander based on the specific speed ($N_s$) and the specific diameter ($D_s$); see Figure 7.

In Figure 7, the parameters $N_s$ and $D_s$ are calculated as a function of the volumetric flow rate ($V_3$) and the adiabatic expander enthalpy drop ($H_{ad}$). Different types of expanders present optimal operation performance in certain ranges of the non-dimensional parameters $N_s$ and $D_s$. For instance, piston expanders perform well when designed for low values of a specific speed (0.01–0.1), while the optimal $N_s$ for radial turbines is in the range of 30–300.

Notice that the graph presented in Figure 7 has been derived using experimental data of existing machines, which adopt steam or air as the working fluids. Therefore, the values reported may differ when considering the use of organic compounds. However, it can be generally concluded that piston expanders are characterized by big specific diameters and low rotational speeds, while radial turbines by high rotational speeds and small specific diameters.

Several works include an experimental investigation of small-scale ORCs. Figure 8 shows the characteristics of the experimental setups installed worldwide.

Figure 8a–c underline that the majority of the test rigs are in the 1–10-kW range. In accordance with Figure 7, most of the experimental setups have volumetric expanders. The top pressure of the cycle and the volumetric expansion ratio are low in most of the cases. The results reported testify that the efficiency of volumetric machines lies in the 60%–80% range.

Figure 9 classifies the experimental test rigs of Figure 8 and the commercial units of Table 2 based on the type of expander. Note that the data have been drawn by the publications referenced in the equivalent captions. The interested reader is referred to the equivalent publications for further details on the data collection.

The choice of the expander, as described in Figure 9, is a strong function of the power output of the system. Based on the data analysed, it can be concluded that volumetric expanders tend to cover the power range of 0–20 kW, while turbo machines are chosen when the power output exceeds 70 kW (see Figure 9). At power outputs exceeding 70 kW, volumetric machines are bulky, and their performance is strongly affected by leakage losses. In the power range of 20–70 kW, the performance of the two types of expanders (i.e., turbo and volumetric) is comparable. Therefore, the experience of the designer plays a crucial role in the selection of the machine. It can also be noticed that there is discordance between the power output of experimental setups and commercial units. Generally, in academia, the trend is to investigate smaller scale units (see Figure 8) and then extrapolate the results to higher power outputs, while ORC companies are keen to look into the development of power units above 30 kW (see Table 2).

Section 4.1, Section 4.2 and Section 4.3 will describe the advantages and drawbacks of the selection of different expanders respectively in ORC systems of power output in the ranges of 1–20 kW, 20–70 kW and 70–100 kW. Section 4.4 will clarify the results depicted in Figure 9.

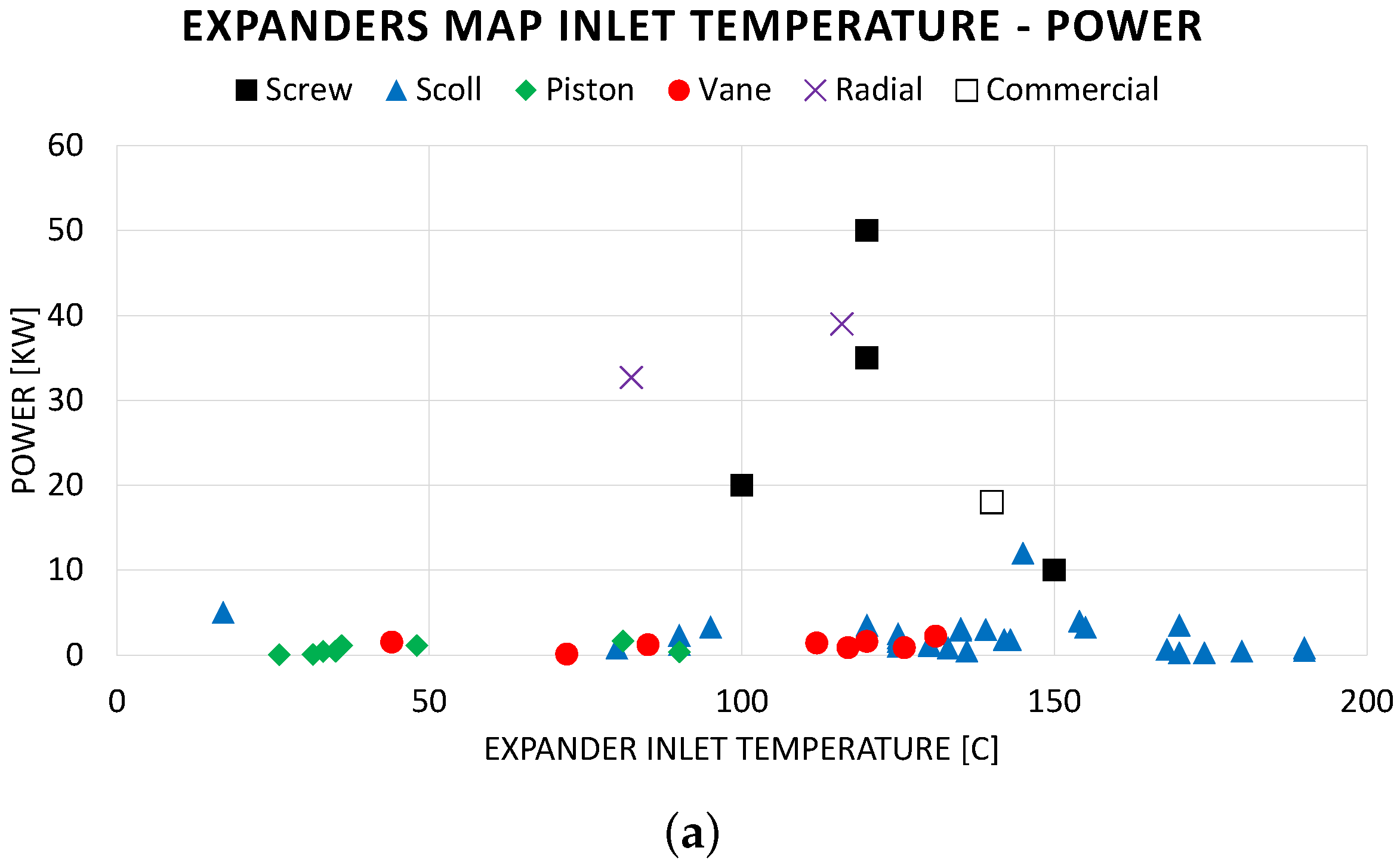
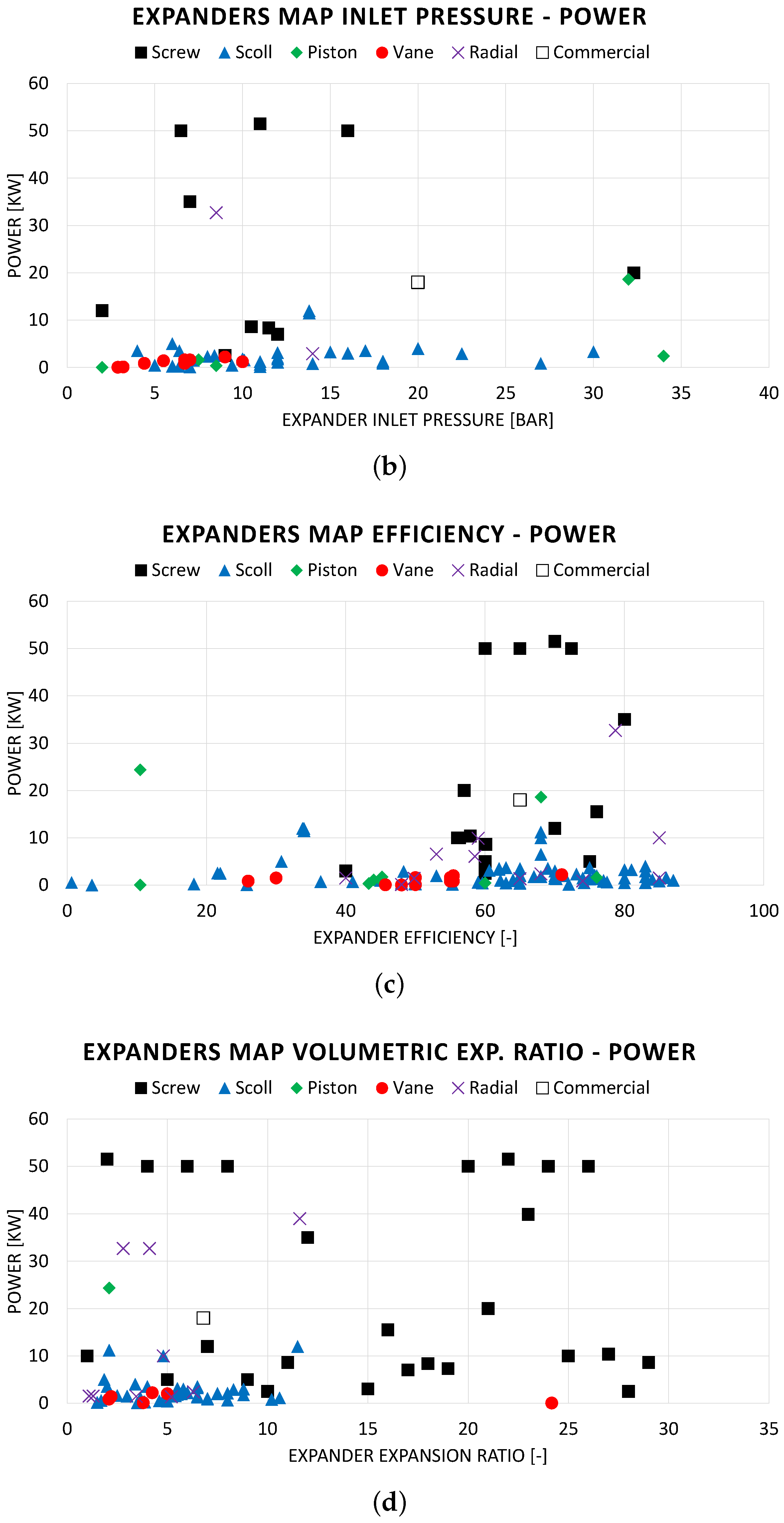
4.1. ORC Plants of 1–20 kW

ORC systems in the power range of 1–20 kW are widely investigated as a feasible solution to produce power in transport and stationary applications. Vehicles [107] and micro-solar plants [108] are the most promising fields in which such a system could be applied in the near future. In this power range, numerous experimental studies are found in the literature offering a thorough understanding of the performance of different expander types. As Figure 7 and Figure 8 depict, researchers demonstrated that volumetric expanders outperform turbo machines in this power range. Specifically, scroll and rotary vane expanders offer the highest performance.

Scroll expanders are receiving interests in the 1–10-kW range because of the absence of valves and their relatively low cost [86]. Lemort et al. [109] proposed a model of a 2-kW scroll expander, which they validated experimentally, achieving a maximum efficiency of 68%. Yun et al. [110] tested an ORC based on two scroll expanders working in parallel. The purpose of their work was to improve the performance of the system under transient conditions. The maximum power output measured on dual mode was below 3.5 kW. Chang et al. [111] tested an ORC system with a scroll-type expander. They obtained a maximum power output of 2.3 kW and a maximum efficiency of 73%.

According to Imran et al. [17], scroll expanders represent the best choice when the power output of the system is in the range of 1–25 kW. These expanders show higher performance when the expansion is slightly wet and the volumetric ratio between the inlet and the outlet sections of the machine is limited (see Figure 8).

In the 1–10-kW power range, rotary vane expanders represent another suitable solution for ORC applications. Some recently-published papers present experimental measurements and evaluations of rotary vane machines [112,113]. Vane expanders can work with fluids at low temperature and pressure, e.g., Qiu et al. [86] proposed a vane expander that can handle 120 °C and 7 bar. Rotary vane expanders result in low rotational speeds, the ability to work in the presence of liquid and minimal maintenance cost [59]. The interested reader may refer to the following studies, among others, for details on the design of rotary vane expanders: [114,115,116].

Lemort et al. [117], in their work on the comparison of piston, screw and scroll expanders, state that screw expanders cover the power range above 20 kW. Qiu et al. [86] in their work explain that screw expanders in the power range below 10 kW are hard to find on the market, due to difficulties in their sealing.

At a commercial level, EXA, Enogia and Mattei developed ORC systems in the range of 1–10 kW (see Table 2). Qiu et al. [86] provide an overview of the prices of different types of ORC expanders.

4.2. ORC Plants of 20–70 kW

The 20–70-kW power range represents a grey area in the expander selection. The weight and size of volumetric expanders increase exponentially when the power output of the system exceeds 20 kW. Turbo expanders require a high rotational speed that may lead to a failure of the bearings. Furthermore, the high rotational speed of turbo machines complicates the coupling between the turbo expander and an off-the-shelf electric generator.

Peterson et al. [118] state that radial turbines are considered for power outputs above 50 kW. Franchetti et al. [83] poses a limit of 70 krpm for the rotational speed of radial turbines. Fiaschi et al. [119] proposed a radial turbine mean line model, showing that a rotational speed of up to 44 krpm is needed for the production of 50 kW power (the total to static efficiency being about 70%). Kang [103] performed an experimental study on a radial expander in the 30-kW range. He experimentally achieved an efficiency up to 78.7%. Hsieh et al. [106] performed an experimental investigation of a 20-kW screw expander, reaching an efficiency of 57% and producing 19.7 kW.

Leibowitz et al. [104], in their analysis on cost effective small-scale ORC systems in the 20–50-kW range, identify that twin screw expanders are the most promising. He states that twin screw expanders can reach efficiencies of 70% during low speed operation and that their cost of installation is approximately 1500–2000 $/kW.

Several ORC companies are developing prototypes in this power range (see Figure 9 and Table 2). Among the expanders available, radial and screw represent the most recurrent choices. The major drawback of radial turbines is represented by their high rotational speeds in the power range of 20–70 kW, while that of screw expanders is represented by their high leakage losses (which are proportional to the size of the machine) [17].

4.3. ORC Plants of 70–100 kW

The power range of 70–100 kW represents an attractive market for ORC companies. As depicted in Table 2, different companies are developing ORC systems in this power range. Despite a large commercial interest, the number of studies that report experimental results of ORC systems in the 70–100-kW power range is limited (see Figure 9). Fu et al. [120] presented a literature review in which they highlighted the lack of experimental studies on ORC systems with a power output exceeding 50 kW.

Figure 9 and Table 2 show that turbo expanders are favoured over volumetric machines in the power range of 70–100 kW. The rotational speed of turbo machines decreases exponentially when the power output exceeds 70 kW. This prevents the bearings from failure and allows the coupling of the expander with an off-the-shelf electric generator. The size and weight of volumetric expanders increase exponentially for power outputs above 70 kW. This implies an increase in leakages, which in turn lowers the efficiency of the machine. Fiaschi et al. [119] stated that radial turbines and screw expanders represent the only options in the power range of 70–100 kW. The majority of the ORC companies involved in the development of ORC units in the power range of 70–100 kW offer radial turbines. Finally, a few companies developed volumetric expanders (see Table 2).

4.4. Comparative Analysis

Volumetric expanders present characteristics that make them a strong candidate in exploiting power in small-scale ORCs and in those applications in which the duty cycle of the heat source is variable. In fact, volumetric machines are able to operate smoothly even when the thermodynamic conditions at the inlet of the machine vary significantly. Another major advantage of volumetric machines is that they operate at low rotational speeds (typically below 3000 rpm) allowing for direct connection with low-speed electric generators. This leads to two advantages. First, low speed electric generators are inexpensive and widely available on the market. Low rotational speeds, which are typical of volumetric machines, allow for the use of common bearings, which increases the longevity of the rotating parts and reduces the frequency of maintenance periods. Second, the low cost of the electric generator helps in reducing the return on investment and in making the technology competitive.

The main drawbacks of volumetric expanders derive from the process of lubrication and from leakages, which cause volumetric and fluid dynamic losses. The former decrease volumetric efficiency due to a reduction of the flow rate that contributes to the production of work. Volumetric losses cannot be eliminated, because they occur from the required expansion gap to safeguard moving parts that are subjected to thermal and mechanical stresses. Fluid dynamic losses are classified into concentrated and distributed losses. Concentrated losses are due to the interaction between the fluid and the edges of the moving parts of the machine. Distributed losses are generated by the interaction between the working fluid and the shroud.

Turbomachines, as reported in Section 4.2, are generally not suitable for power production below 10 kW, mainly because of their rotational speeds. This imposes a severe challenge when coupling the turbine with the electric generator. Two different options are available to couple the turbo machine to the electric generator. One possibility is to use a gear-box to decouple the rotational speed of the turbine from that of the electric generator. However, this would introduce high mechanical losses [121]. The other option is to adopt a high speed electric generator directly coupled to the expander. The high cost of such machines increases the total cost of the ORC.

Figure 10 reports a map of existing high speed electric generators [122].

As can be observed from Figure 10, the options available in the power range of interest are limited to permanent magnet machines. In addition, the balancing of the machine with the turbine implies low tolerances during the manufacturing process. Overall, high speed turbines represent a more efficient alternative to volumetric expanders when the power output is greater than 20 kW. However, the higher cost of turbo expanders (with respect to volumetric machines) renders them cost effective only in applications wherein they considerably outperform volumetric machines; as for example, when the thermodynamic conditions of the heat source are stable over time.

5. Conclusions

This paper provides an overview of the state of the art in ORC technology for low power applications. The objective of the analysis is two-fold. Firstly, an economic investigation has been developed to determine the specific cost that would make ORC technology competitive in small-scale power production. Secondly, a technical overview on the methodologies employed in the literature for the selection of the expander and of the working fluid has been presented.

The conclusions drawn from this study are:

• The specific cost of ORC units should not exceed the value of 3500 €/kW and 2500 €/kW in the power range of 5–10 kW and 10–100 kW, respectively.
• The main reason why ORC technology is not widely available at a commercial level in small-scale power production is its high specific cost. Therefore, efforts should tend towards the optimization of the system to reduce the specific cost, which in turn lowers the payback period.
• With the objective of reducing the specific cost of the technology for small-scale applications, it is important that research pursues the goal of performing a more holistic analysis, in which all indicators that influence the specific costs are optimized at the same time. Thermodynamic, economic and technical aspects should be optimized together to guarantee the dissemination of small-scale ORC plants.
• The literature lacks experimental data of ORC systems in the power range of 10–100 kW, which are required to facilitate the diffusion of the ORC technology in the small-scale power range.
• The expander plays a crucial role in the definition of the system’s performance. Much effort has been put into the investigation of volumetric expanders. However, it is important to highlight that the efficiency, compactness and power-to-weight ratio of turbo expanders overcome those of volumetric machines, particularly when the power output is above 10 kW. The reduction in cost of high speed electric generators together with the use of working fluids that do not require an unreasonably high turbine rotational speed could rapidly make small-scale ORC units cost-effective.

ORC systems for the production of power outputs in the range of 1–100 kW still experience little market absorption. The advances in technology together with further government incentives on investments are essential to fill in the existing market gap.