Thermal and Electromagnetic Combined Optimization Design of Dry Type Air Core Reactor
Abstract
:1. Introduction
2. Methods
2.1. Thermal and Electromagnetic Optimization
2.1.1. The Thermal Efficiency Optimization
2.1.2. The Electromagnetic Efficiency Optimization
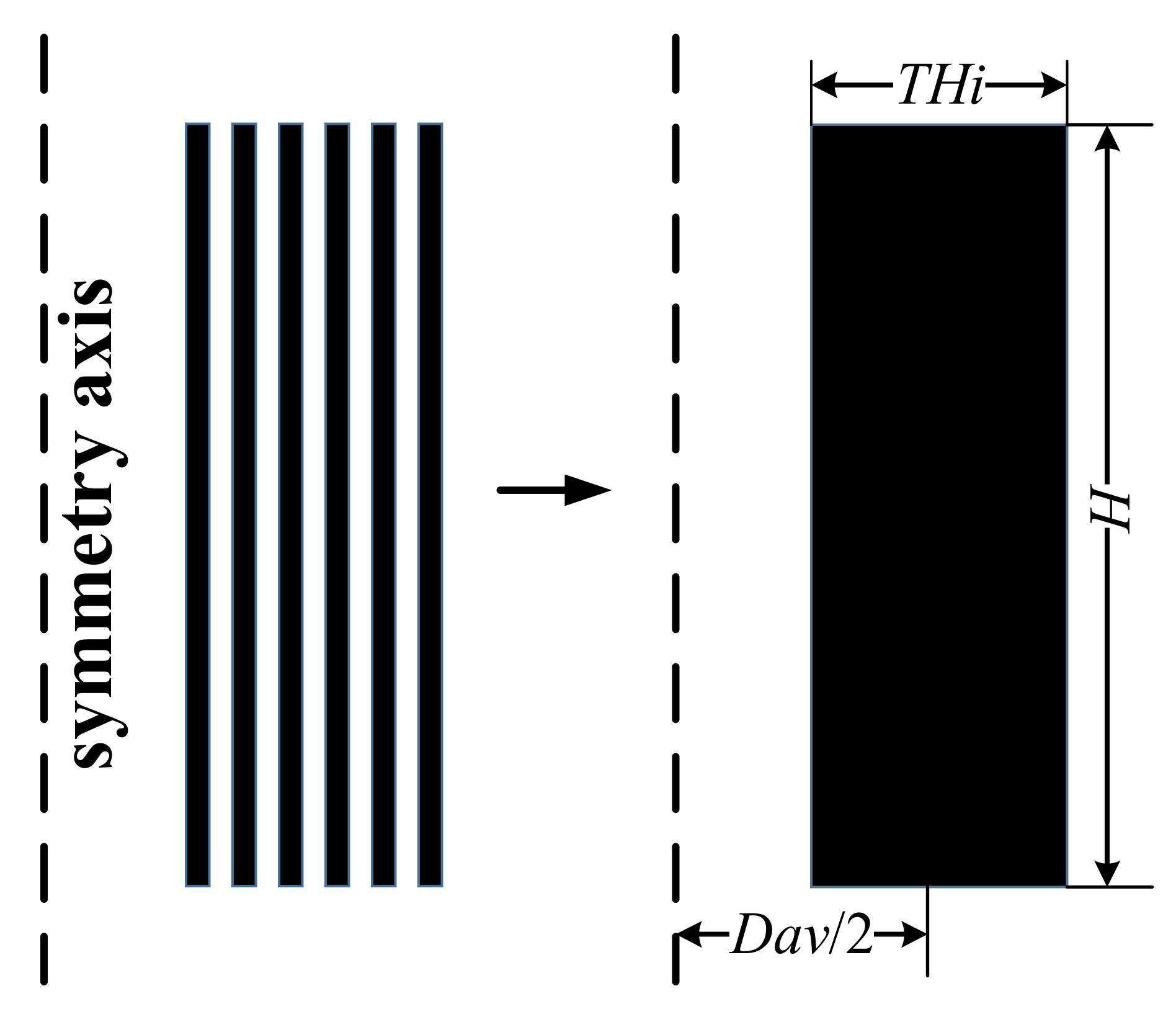
2.2. The Comprehensive Optimization Method
2.2.1. Equality Constraint Conditions
2.2.2. The Realization of the Optimization Method
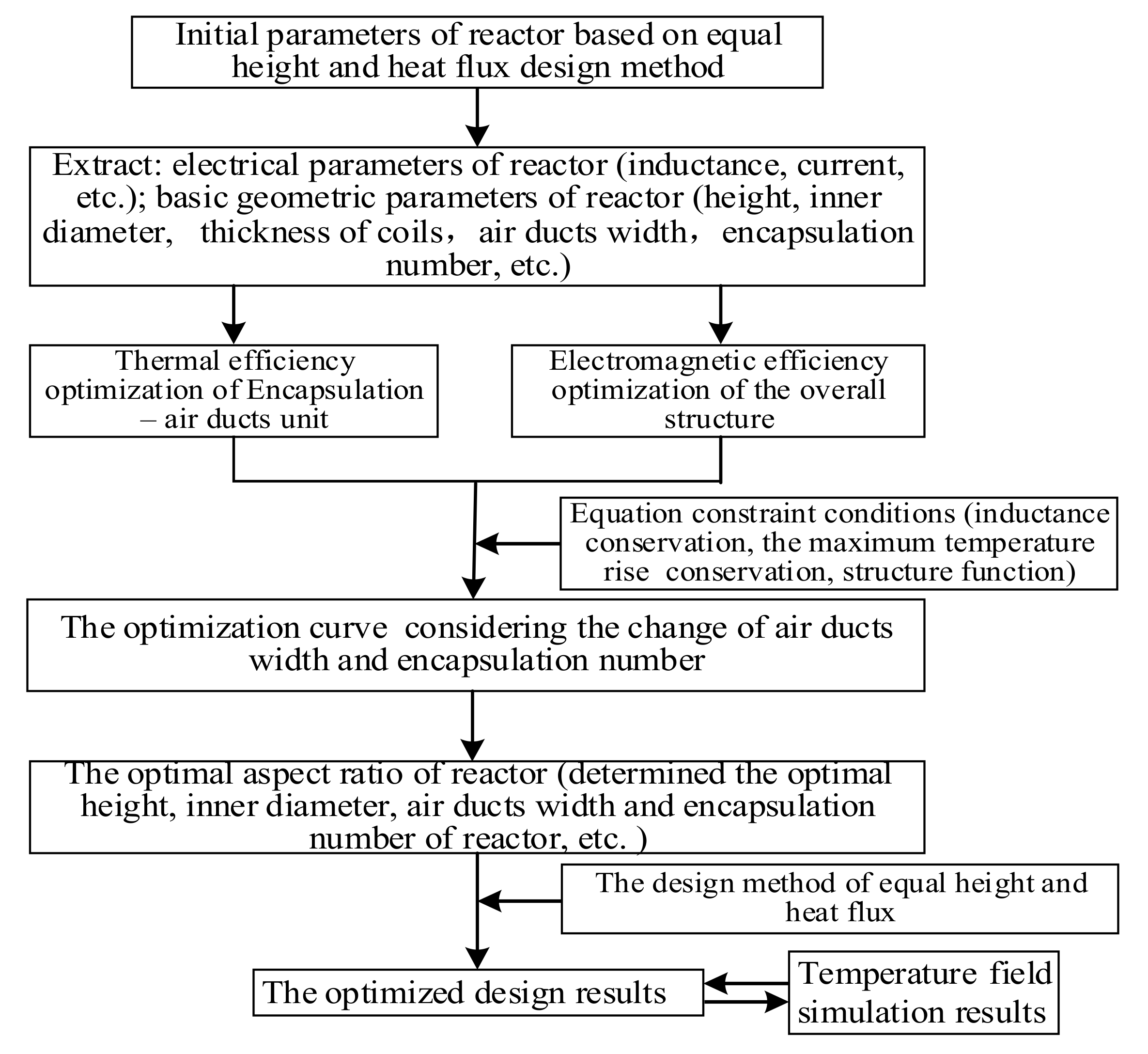
2.2.3. The Overall Optimization Process
3. Results
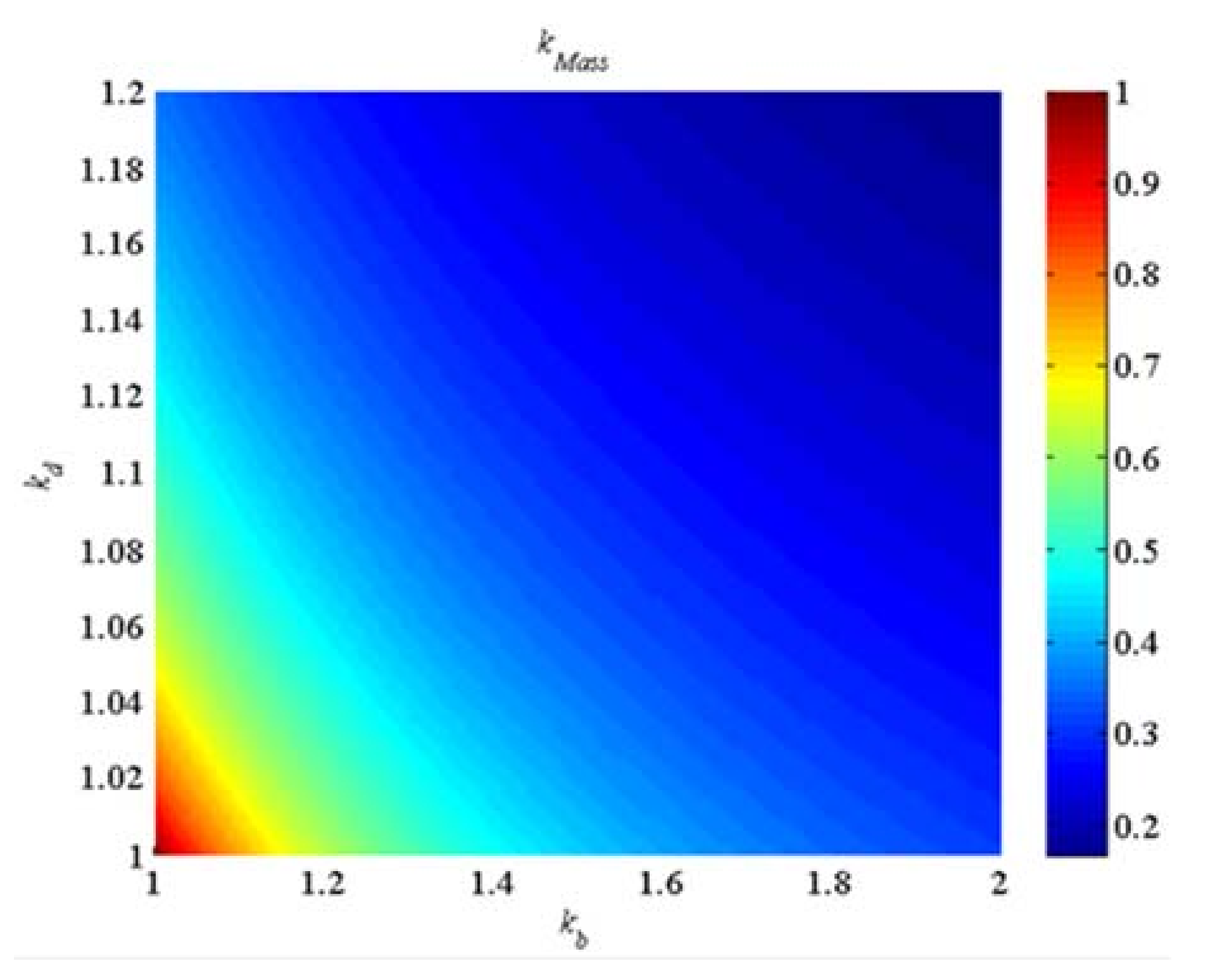
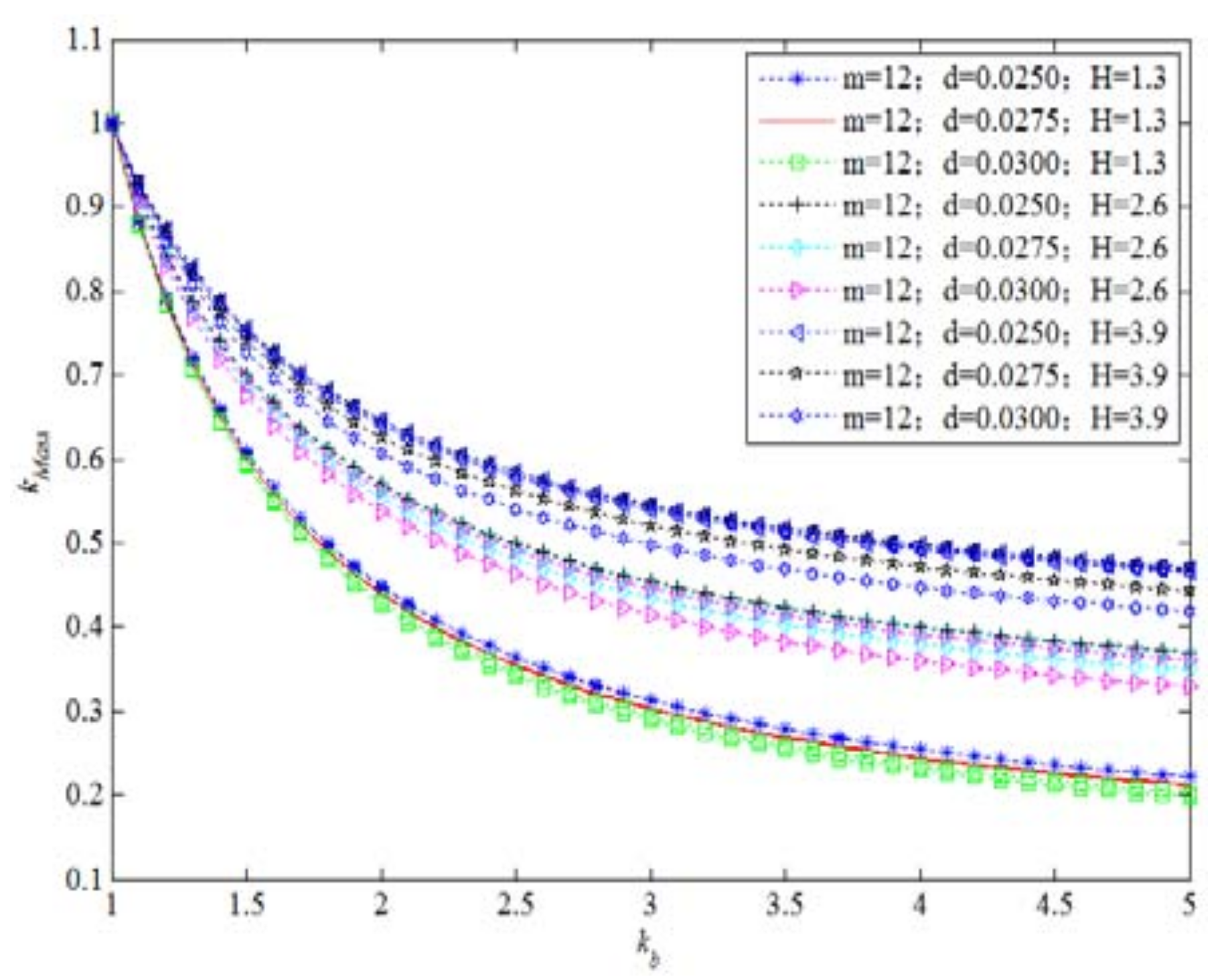
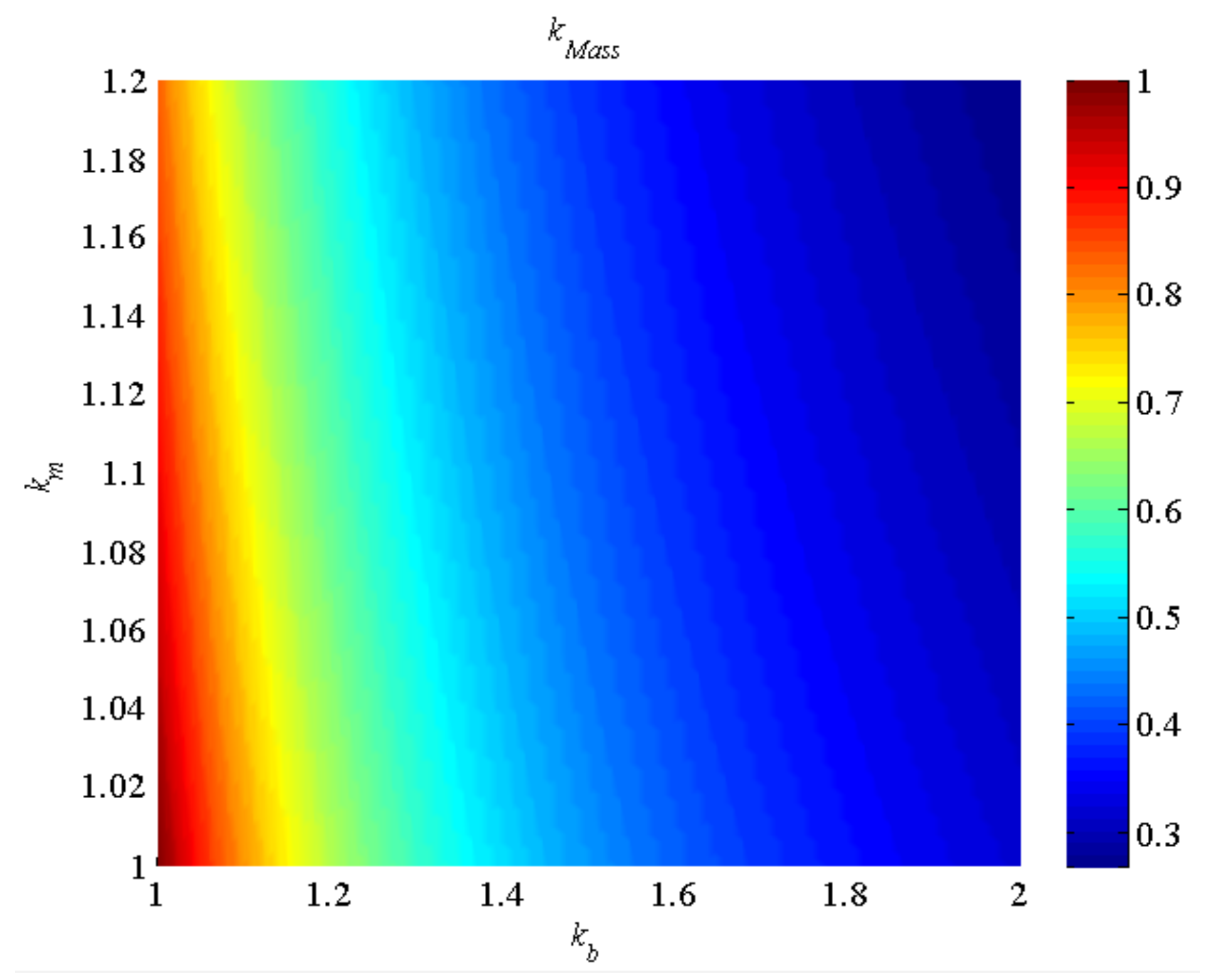
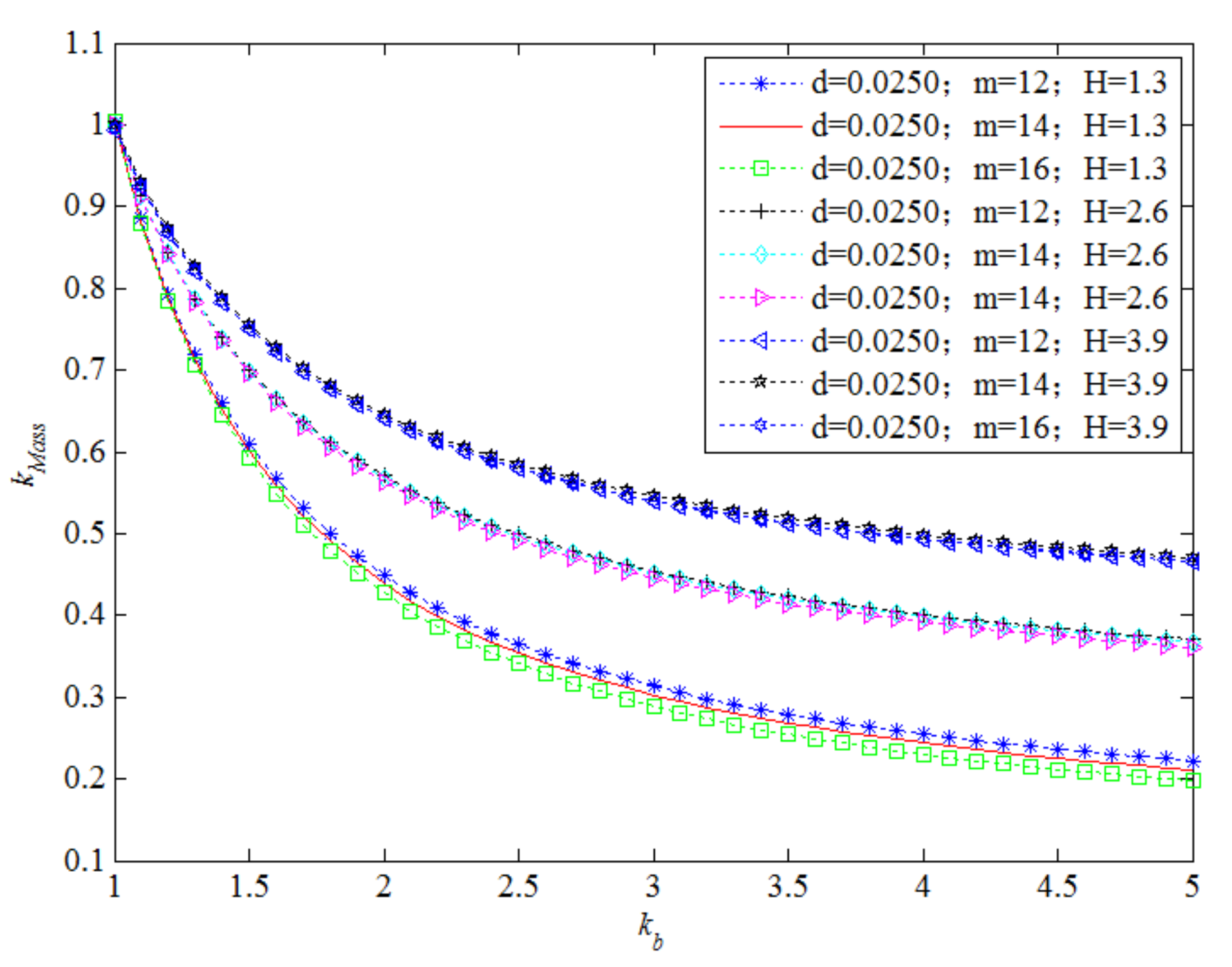
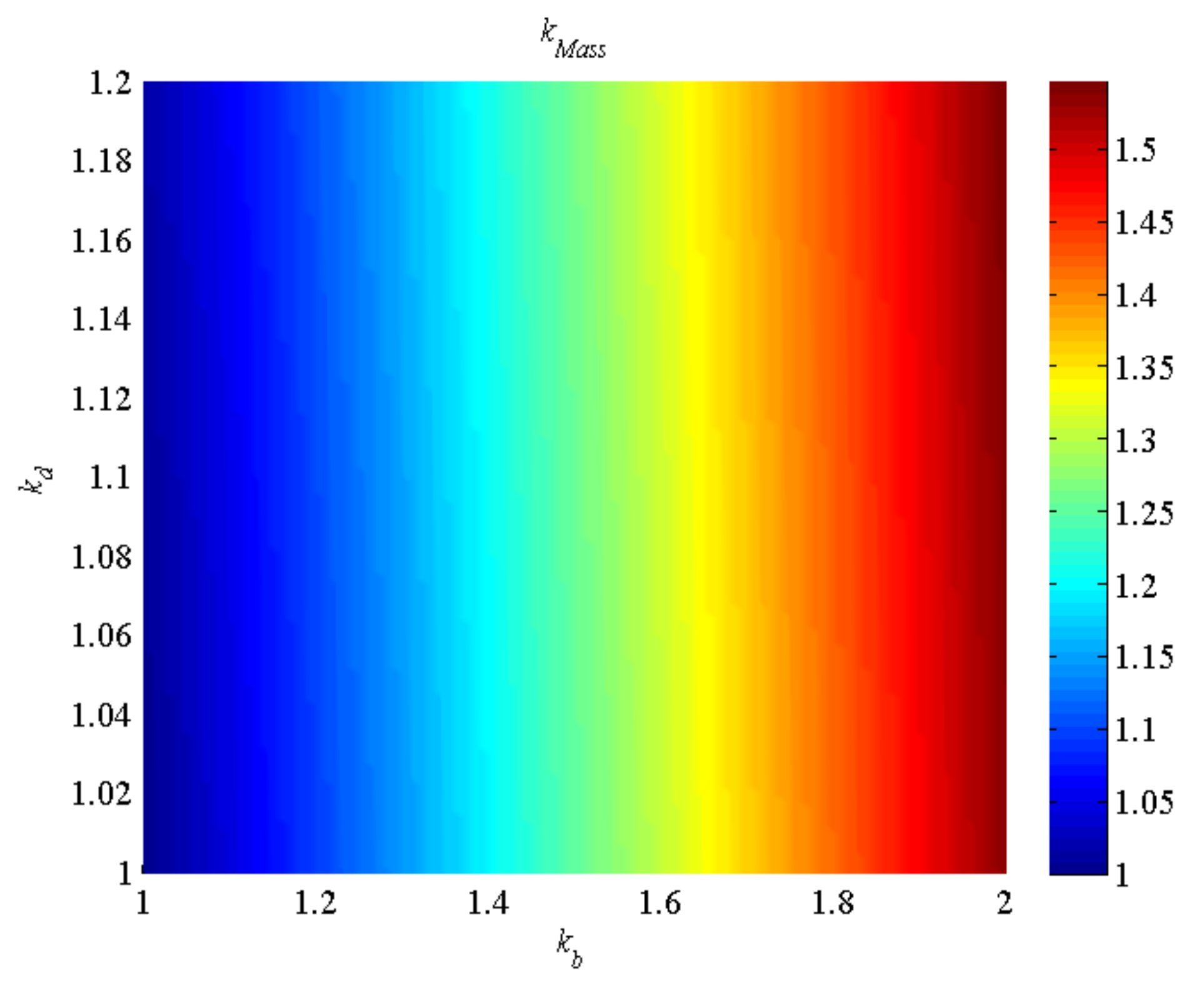
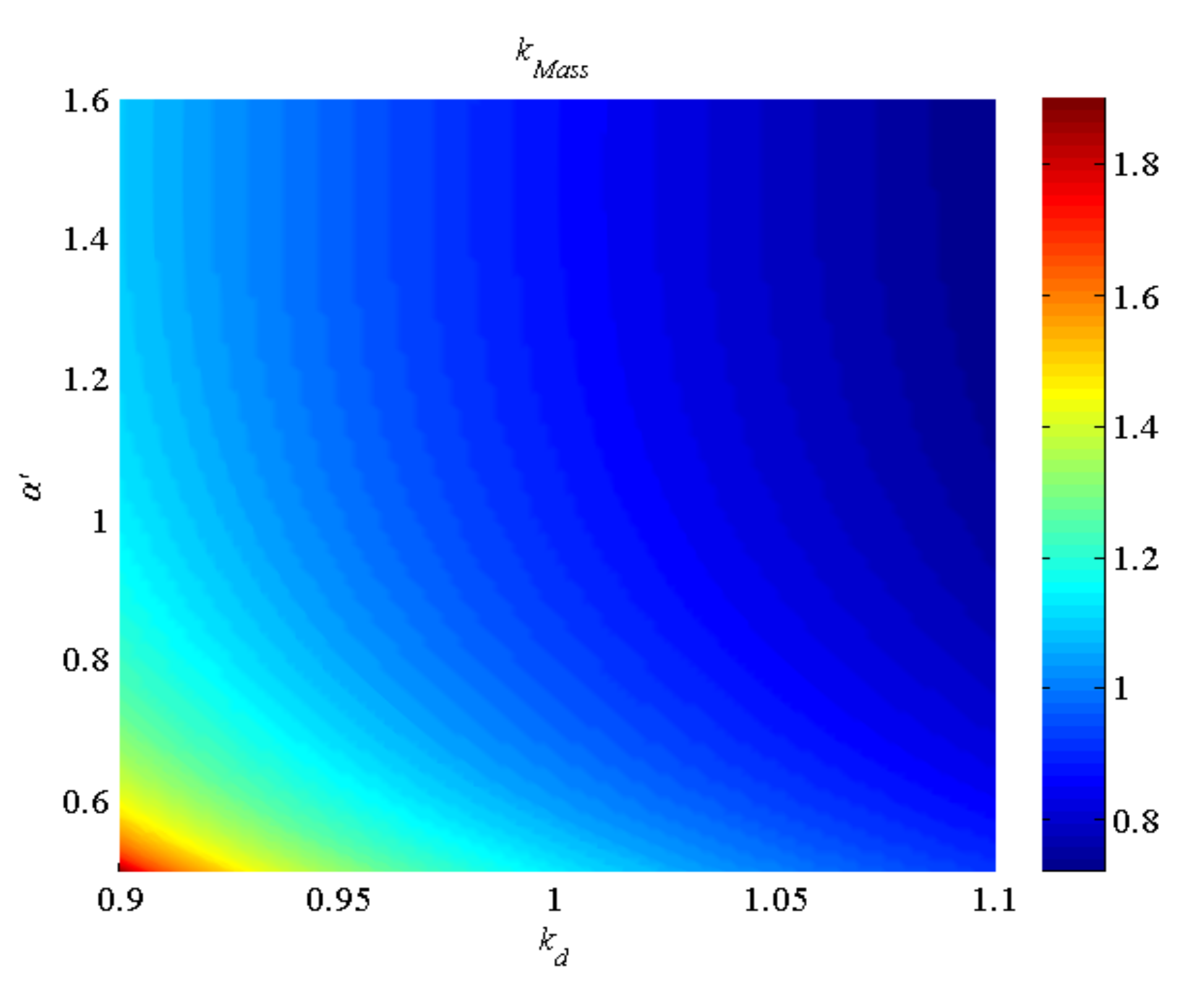
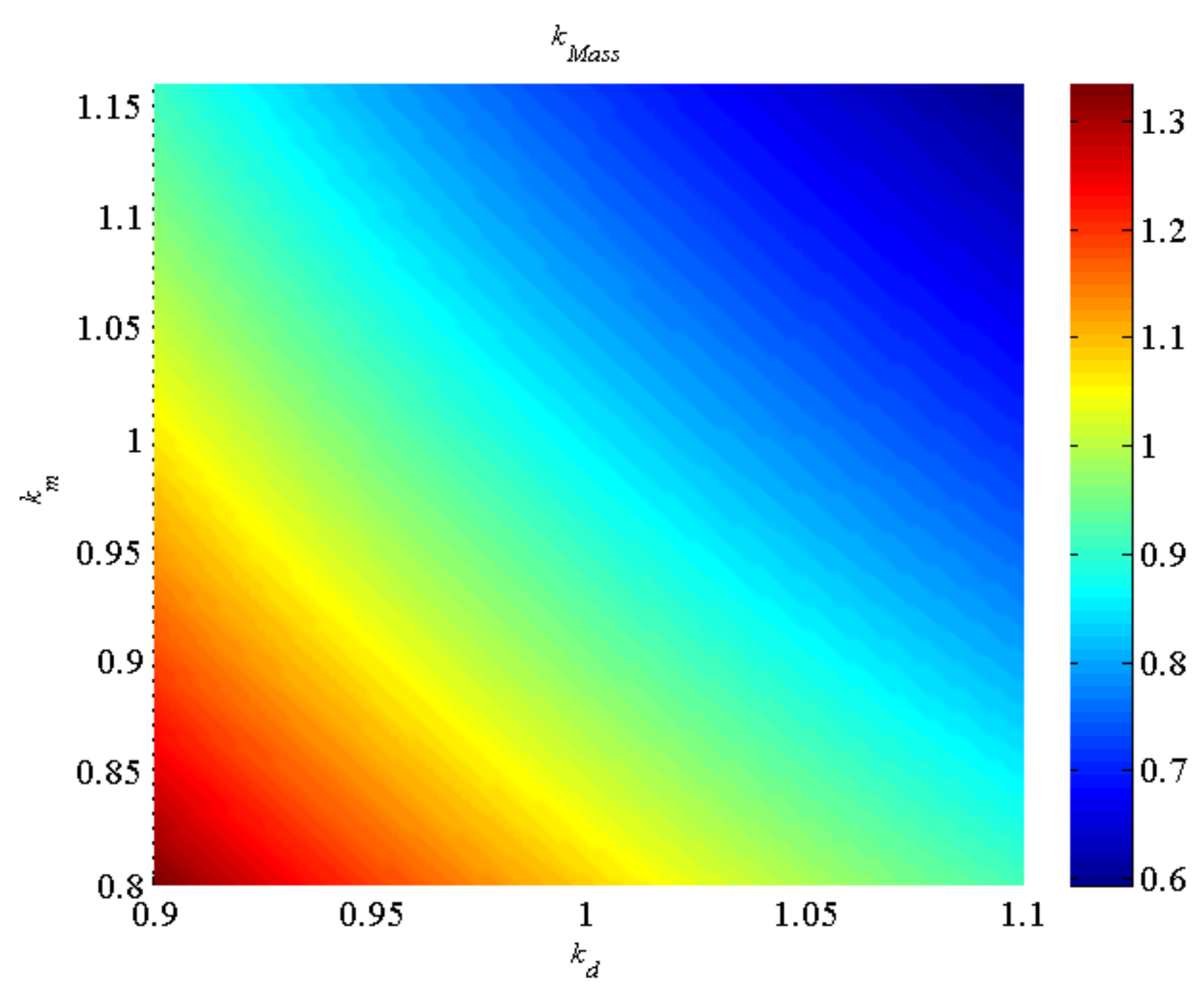
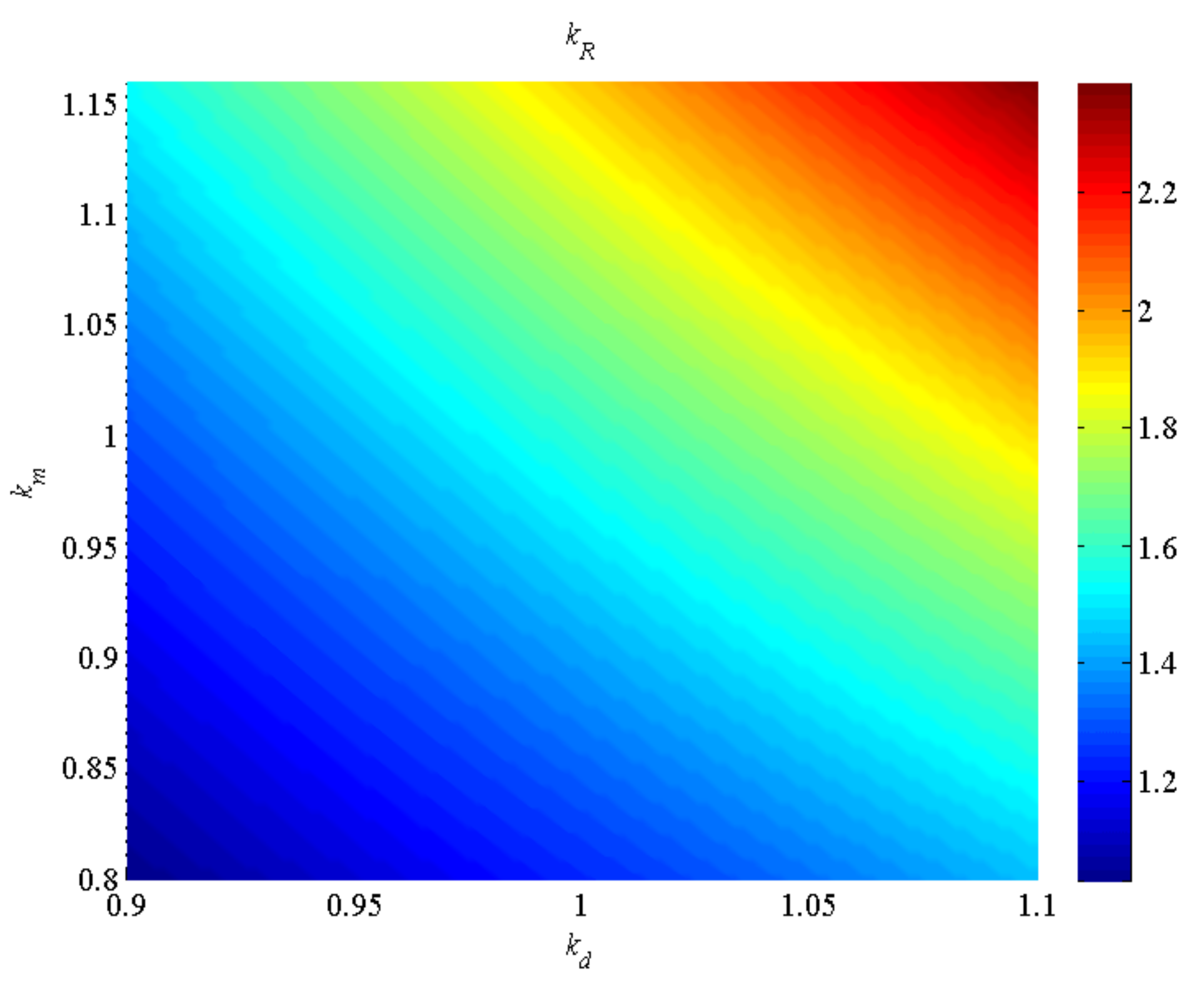
3.1. Optimization Curves
3.1.1. Considering Only the Change of Air Duct Width
3.1.2. Considering Both the Change of Air Duct Width and Encapsulation Number
3.2. Design Results
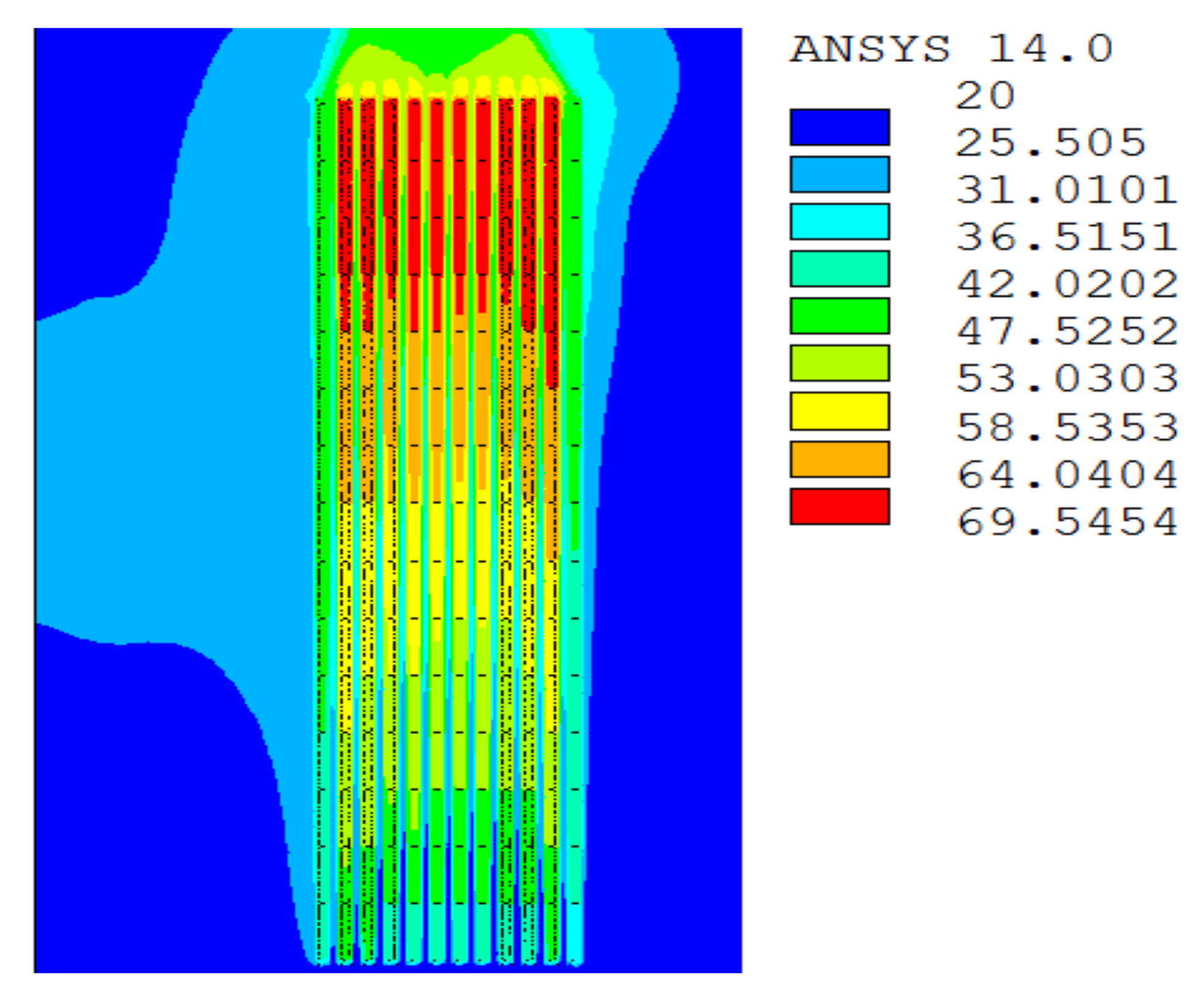
3.3. Simulation Verification
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Symbols and Abbreviations
| a | The radial width of encapsulation |
| b | The single turn axial height of encapsulation |
| Cp | The specific heat at constant pressure |
| d | The width of air ducts |
| D | The diameter of encapsulation |
| Dav | The average diameter of thick wall coils |
| H | The height of thick wall coils |
| I | The current of encapsulation |
| kad | The ratio value of radial width of encapsulation to air duct width |
| kc | The ratio of total losses to resistance losses |
| kx | The proportionality factor of |
| L | The inductance value of the reactor |
| m | The encapsulation number of the reactor |
| The mass flow rate of fluid in air ducts | |
| Mass | The metal conductor usage of reactor |
| Nu | The Nusselt number in air ducts |
| qw | The heat flux conducted from encapsulation surface to air ducts |
| R | The resistance of the reactor |
| S | The area of the single turn conductor |
| T | The temperature of encapsulation surface |
| The temperature of fluid in air ducts | |
| THi | The thickness of thick wall coils |
| Tmax | The maximum temperature rise of encapsulation |
| The average velocity of fluid | |
| The average turn number | |
| ρ | The fluid density of fluid |
| λ | The heat conductivity coefficient of fluid |
| g | The acceleration of gravity |
| β | The thermal expansion coefficient of fluid |
| ρ1 | The mass density of metal conductor |
| μf | The dynamic viscosity corresponds to the fluid temperature |
| μw | The dynamic viscosity corresponds to the encapsulation surface temperature |
| γ | The conductivity of metal conductor |
| ν | The kinematic viscosity of fluid |
Appendix A
References
- Yu, Z.; Wang, S. Optimum design of dry-type air-core reactor based on coupled multi-physics of reconstructed finite element model. Trans. China Electrotech. Soc. 2015, 30, 71–78. [Google Scholar]
- Lin, Q.; Liu, Q.; Liang, Y.; Liu, T.; Li, X. Research of monitoring method for interturn short circuit fault of air-core reactor. Power Capacit. React. Power Compens. 2015, 36, 96–100. [Google Scholar]
- Huang, J.; Xiao, Z.; Chen, G. The calculation and design of air core reactor. Electr. Drive 1991, 5, 46–55. [Google Scholar]
- Luo, L.; Zhao, S.; Xu, J.; Wei, L.; Lou, Y. Design of Large Inductance Power Cable. High Volt. Eng. 2015, 41, 2635–2642. [Google Scholar]
- Li, Y.; Zhang, Z.; Zhu, L.; Jiang, M. Calculation and design of dry-type air-core reactor. Transformer 2013, 50, 1–6. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, Z.; Li, L.; Li, G.; Jiang, M. Calculation and design of dry-type air-core reactor. Energy Power Eng. 2013, 5, 1101–1104. [Google Scholar] [CrossRef]
- Zhang, C.; Zhao, Y.; Ma, X. Optimization design of separated dry-type air-core reactor based on modified differential evolution algorithm. In Proceedings of the Third International Conference on Information Science and Technology, Yangzhou, China, 23–25 March 2013. [Google Scholar]
- Liu, Z.; Ouyang, S.; Geng, Y.; Wang, J. Study of genetic algorithm in the optimum design of air-core reactor. Adv. Technol. Electr. Eng. Energy 2003, 22, 45–49. [Google Scholar]
- Ouyang, S.; Liu, Z.; Geng, Y.; Wang, J. A new improved genetic algorithm and its application in the optimum design of the reactor. Comput. Eng. 2003, 29, 16–17. [Google Scholar]
- Zhao, Y.; Chen, F.; Kang, B.; Ma, X. Optimum design of dry-type air-core reactor based on the additional constraints balance and hybrid genetic algorithm. Int. J. Appl. Electromagn. 2010, 33, 279–284. [Google Scholar]
- Lu, S.; Sun, C.; Lu, Z. An improved quantum-behaved particle swarm optimization method for short-term combined economic emission hydrothermal scheduling. Energy Convers. Manag. 2010, 51, 561–571. [Google Scholar] [CrossRef]
- Smolka, J.; Nowak, A.J. Shape optimization of coils and cooling ducts in dry-type transformers using computational fluid dynamics and genetic algorithm. IEEE Trans. Magn. 2011, 47, 1726–1731. [Google Scholar] [CrossRef]
- Yan, X.; Dai, Z.; Yu, C.; Qi, Y. Research on magnetic field and temperature field of air core power reactor. In Proceedings of the International Electrical Machines and Systems (ICEMS2011), Beijing, China, 20–23 August 2011. [Google Scholar]
- Yuan, Z.; He, J.; Pan, Y.; Yin, X.; Luo, C.; Chen, S. Research on electromagnetic efficiency optimization in the design of air-core coils. Int. Trans. Electr. Energy Syst. 2015, 25, 789–798. [Google Scholar] [CrossRef]
- Yuan, F.; Yuan, Z.; Wang, Y.; Liu, J.; Su, H.; He, J. Research of Electromagnetic and Thermal Optimization Design on Air Core Reactor. IEEJ Trans. Electr. Electron. Eng. 2017. [Google Scholar]
- Liu, Q.F.; Dang, H.G.; Liang, Y.C.; Miao, H.T. Optimization algorithm of the reactor design. Power Capacit. React. Power Compens. 2011, 32, 47–60. [Google Scholar]
- Chen, F.; Zhao, Y.; Ma, X. Optimum design of dry-type air-core reactor based on design variable reconstruction. Proc. Chin. Soc. Electr. Eng. 2009, 29, 99–106. [Google Scholar]
- Wu, T.; Wang, X.; Xu, G.; Pan, Y.; Chen, W. Engineering Heat Transfer; Huazhong University of Science and Technology Press: Wuhan, China, 2011; pp. 120–130. [Google Scholar]
- Gnielinski, V. New equations for heat and mass transfer in the turbulent flow in pipes and channels. NASA STI/Recon Tech. Rep. A 1975, 41, 8–16. [Google Scholar]
- Gnielinski, V. New equations for heat and mass transfer in turbulent pipe and channel flows. NASA STI/Recon Tech. Rep. A 1976, 16, 359–368. [Google Scholar]
- Sun, X. The design of air core reactor. Transformer 1988, 11, 7–10. [Google Scholar]
- Cao, J.; Cheng, T.; Jiang, Z.; Wen, X.; Zhang, M. Coupling calculation of temperature field for dry-type smoothing reactor. In Proceedings of the International Conference on Electrical Machines & Systems ICEMS 2014, Hangzhou, China, 22–25 October 2014; pp. 3259–3263. [Google Scholar]
- Wu, D. Temperature field distribution and infrared temperature measurement method research of 35 kV dry-type reactor. Transformer 2013, 50, 62–65. [Google Scholar]
- Chen, F.; Zhao, Y.; Ma, X. An efficient calculation for the temperature of dry air-core reactor based on coupled multi-physics model. In Proceedings of the 2012 Sixth International Conference on Electromagnetic Field Problems and Applications, Dalian, China, 19–21 June 2012. [Google Scholar]
- Zhang, Y.J.; Qin, W.N.; Liang, G.; Ruan, J.J.; Huang, T. Analysis of temperature rise in reactors using coupled multi-physics simulations. In Proceedings of the IEEE International Conference on Applied Superconductivity & Electromagnetic Devices, Beijing, China, 25–27 October 2013. [Google Scholar]
- Yuan, F.; Yuan, Z.; Liu, J.; Wang, Y.; Mo, W. Research on Temperature Field Simulation of Dry Type Air-Core Reactor. In Proceedings of the 20th International Conference on Electrical Machines and Systems (ICEMS 2017), Sydney, NSW, Australia, 11–14 August 2017. [Google Scholar]
- Yuan, F.; Yuan, Z.; Wang, Y.; Liu, J.; Su, H.; He, J. Thermal Optimization for Nature Convection Cooling Performance of Air Core Reactor with the Rain Cover. IEEJ Trans. Electr. Electron. Eng. 2017. [Google Scholar]
- Yuan, Z.; He, J.; Pan, Y.; Yin, X.; Ding, C.; Ning, S.; Li, H. Thermal analysis of air-core power reactors. ISRN Mech. Eng. 2013, 2013, 1–6. [Google Scholar] [CrossRef]
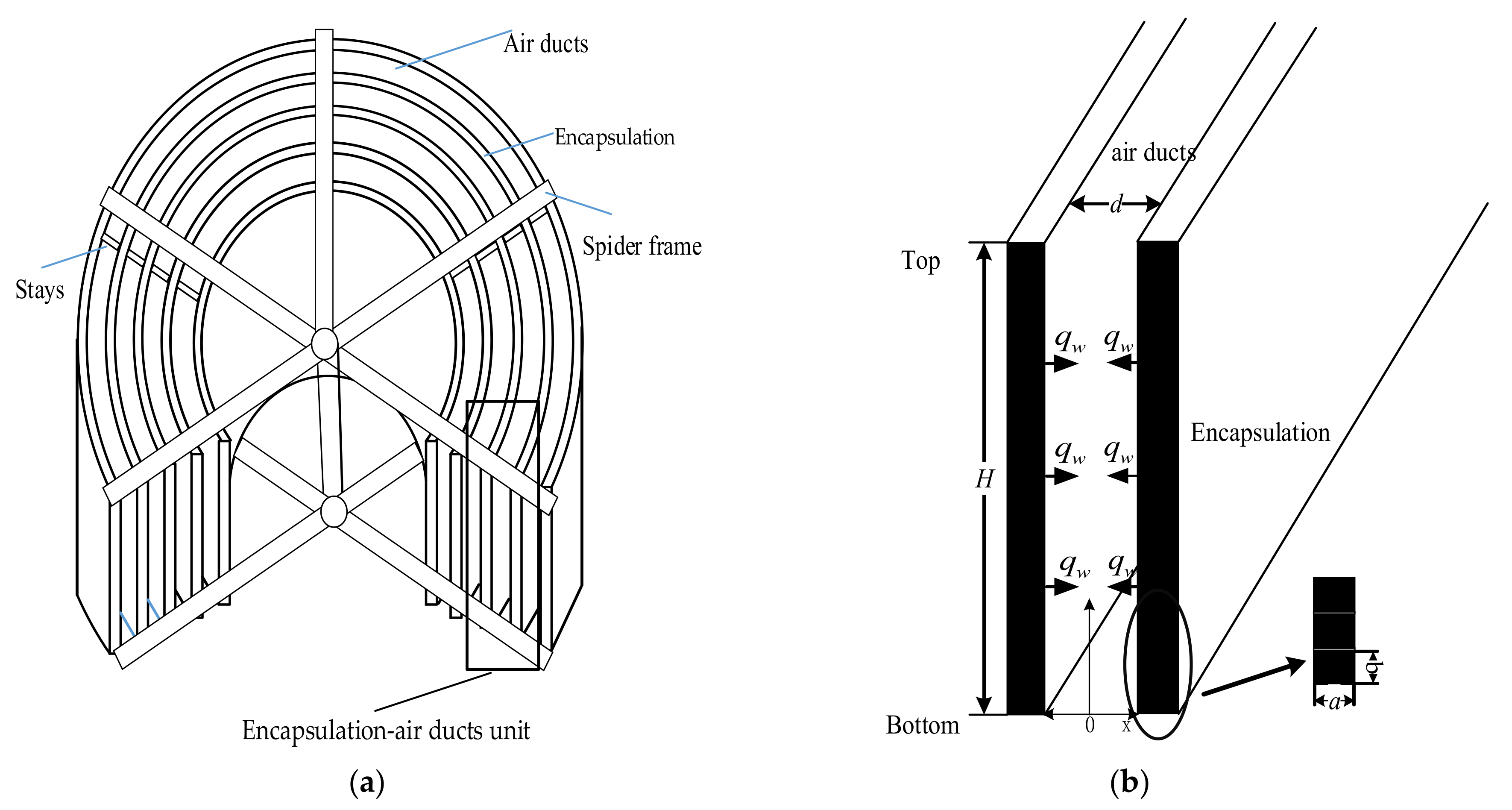


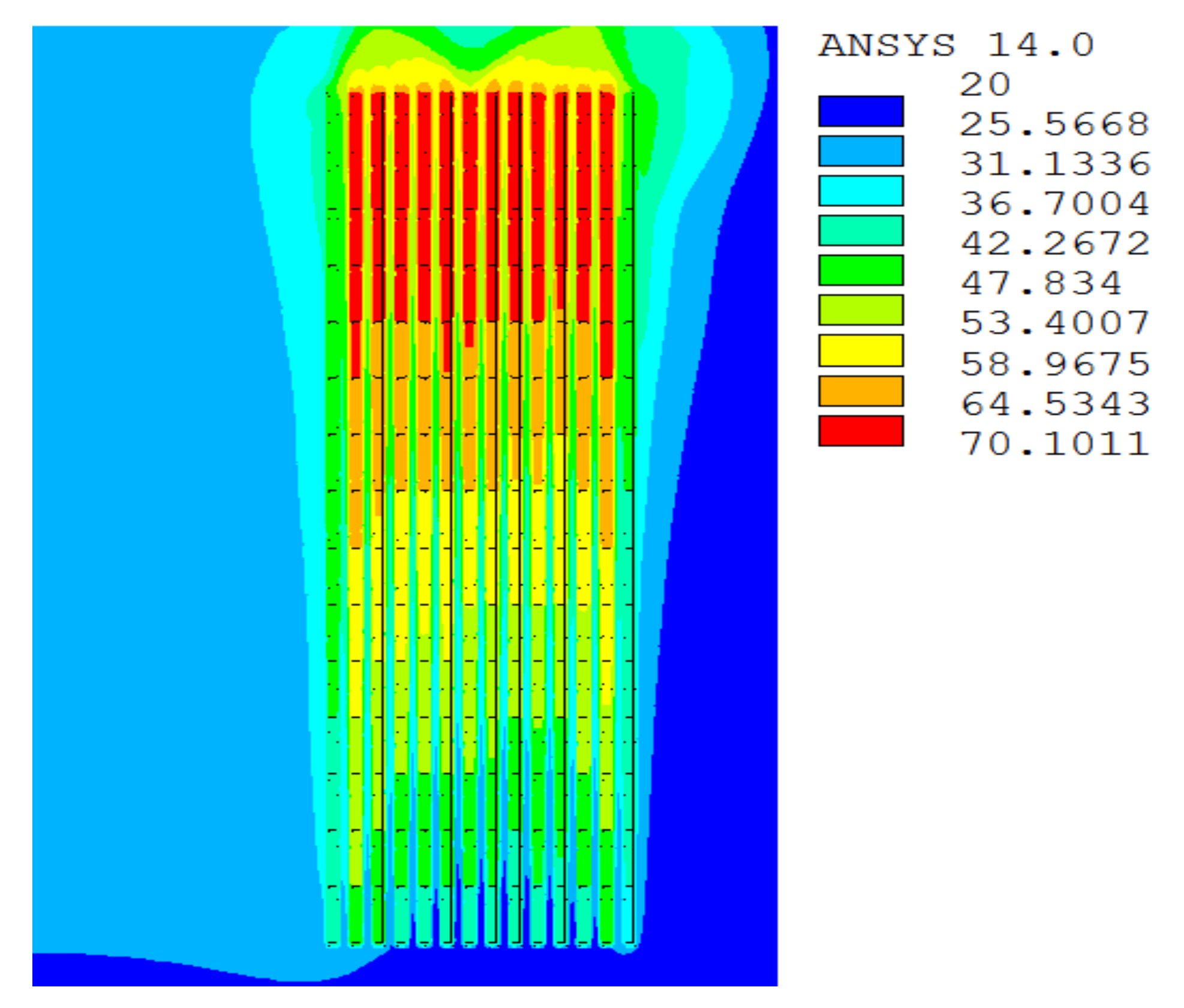
| Reactor | Parameters |
|---|---|
| Rated inductance (mH) | 20.9 |
| Rated current (A) | 875.5 |
| Height of the reactor (m) | 1.3 |
| Inside diameter (m) | 1.6 |
| Outside diameter (m) | 2.4 |
| Width of air ducts (m) | 0.025 |
| Average turn number | 122.5 |
| Number of encapsulation | 12 |
| Maximum temperature rise (°C) | 48 |
| Method | 1 | 2 | 3 |
|---|---|---|---|
| H (m) | 1.3 | 2.58 | 2.48 |
| THi (m) | 0.390 | 0.397 | 0.42 |
| Dav (m) | 1.99 | 1.72 | 1.77 |
| H/Dav | 0.65 | 1.5 | 1.4 |
| THi/Dav | 0.2 | 0.231 | 0.24 |
| 122.5 | 175 | 169 | |
| d (m) | 0.025 | 0.0275 | 0.0275 |
| m | 12 | 12 | 14 |
| Mass (t) | 1.62 | 1.18 | 0.99 |
| KMass | 1 | 0.73 | 0.61 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, F.; Yuan, Z.; Chen, L.; Wang, Y.; Liu, J.; He, J.; Pan, Y. Thermal and Electromagnetic Combined Optimization Design of Dry Type Air Core Reactor. Energies 2017, 10, 1989. https://doi.org/10.3390/en10121989
Yuan F, Yuan Z, Chen L, Wang Y, Liu J, He J, Pan Y. Thermal and Electromagnetic Combined Optimization Design of Dry Type Air Core Reactor. Energies. 2017; 10(12):1989. https://doi.org/10.3390/en10121989
Chicago/Turabian StyleYuan, Fating, Zhao Yuan, Lixue Chen, Yong Wang, Junxiang Liu, Junjia He, and Yuan Pan. 2017. "Thermal and Electromagnetic Combined Optimization Design of Dry Type Air Core Reactor" Energies 10, no. 12: 1989. https://doi.org/10.3390/en10121989
APA StyleYuan, F., Yuan, Z., Chen, L., Wang, Y., Liu, J., He, J., & Pan, Y. (2017). Thermal and Electromagnetic Combined Optimization Design of Dry Type Air Core Reactor. Energies, 10(12), 1989. https://doi.org/10.3390/en10121989





