Analysis of Analytical Models Developed under the Uniaxial Strain Condition for Predicting Coal Permeability during Primary Depletion
Abstract
:1. Introduction
- To derive a new permeability model by taking mean effective stress as the fundamental cause of permeability variation during gas depletion;
- To investigate the influence of matrix shrinkage on cleat deformation in the proposed permeability model by incorporating an effective deformation coefficient of coal matrix;
- To compare four commonly-used analytical permeability models, the P&M, Improve P&M, S&D, and C&B models.
2. Review of Sorption-Induced Strain and Permeability Models
2.1. Modeling of Sorption-Induced Strain
2.2. Summary of Sorption-Induced Strain
- Application of collection of matchsticks geometry. The four models listed above are based on matchstick representation. Besides, all four use a geo-mechanics approach, making them more transparent and easy to understand.
- Boundary conditions: uniaxial strain and constant vertical stress. The original P&M, improved P&M, S&D, and C&B models were developed under the uniaxial strain condition. Another assumption employed by all models is that the vertical stress is constant over the life of producing wells because the burial depth of CBM reservoirs does not change with depletion.
- Strain-/stress-based models. Gu and Chalaturnyk [15] offered a distinction based on whether a model is strain-based or stress-based. The original P&M model and improved P&M model are based on the change in volumetric strain due to the desorption of methane, thus resulting in changes in cleat porosity and permeability. The other two are based on the variation of effective stress resulting from desorption-induced volumetric strain, and then permeability.
- Cleat volume compressibility, Cf. This input parameter in stress-based models is the bridge connecting effective stress and permeability. However, the definition of cleat volume compressibility differs from each other. In the S&D model, Cf is defined with respect to the change in effective horizontal stress [37]; however, in the C&B model, it is defined with respect to the mean effective stress. Moreover, a poor history match was obtained using the S&D model due to the constant Cf value applied, so the molders modified the model for a variable value of Cf [38]. However, this change is still purely based on history matching, without scientific backing.
- Matrix volumetric strain. All four models estimate the matrix shrinkage as a result of desorption in a manner similar to thermal contraction of a material, which is based on an empirical approach without a theoretical explanation to clarify the relationship between volumetric strain and the effect of adsorption; hence, our fundamental understanding on desorption-induced matrix strain should be improved.
- Cleat aperture. In previous studies, the decrease in the dimension of the coal matrix due to shrinkage was regarded as equal to the increase in the dimension of the cleat aperture. However, matrix strain measured in the laboratory under the unconfined condition neglects the restriction of filling material between two adjacent matrix blocks on the matrix deformation. All four models do not take the interaction between adjacent matrixes into account, meaning that a larger matrix strain is applied in analytical models.
3. Formulation of Stress-Dependent Permeability
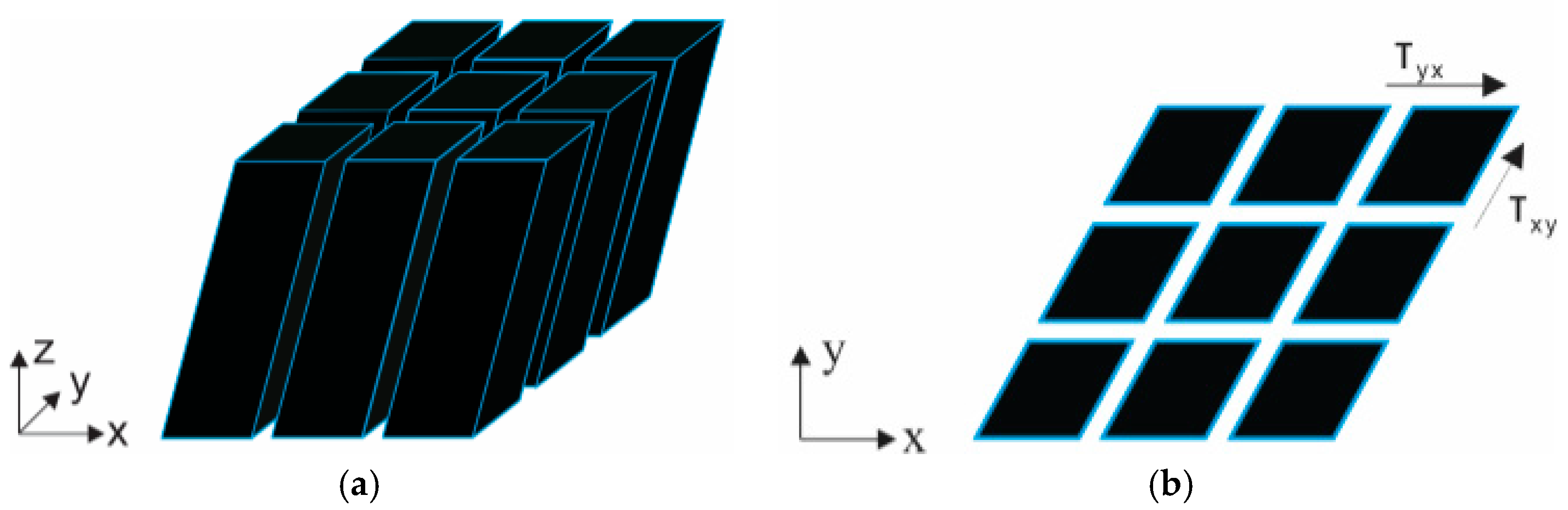
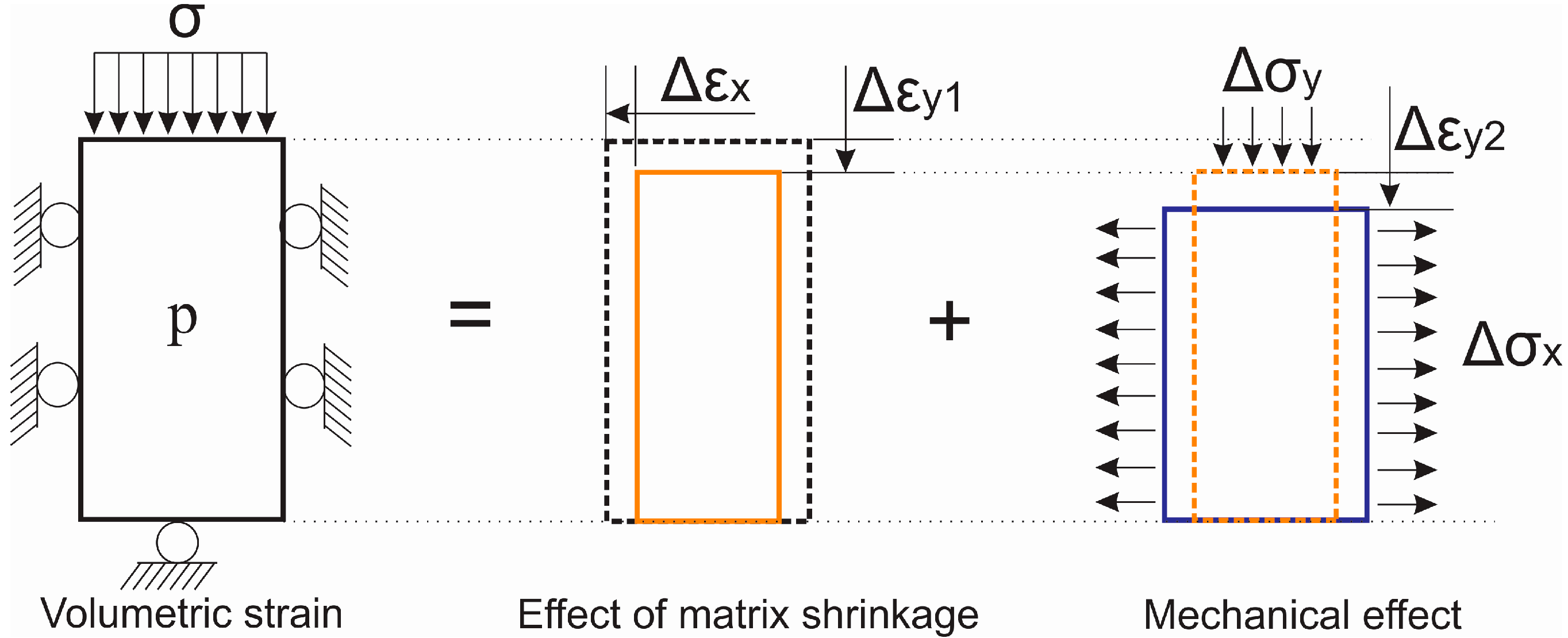
3.1. In-Situ Stress Decomposition
3.2. Development of a New Permeability Model
3.2.1. Strain Induced by Mean Effective Stress
3.2.2. Coal Matrix Strain Induced by Gas Sorption
3.2.3. Establishment of Permeability Model
4. Model Validation and Comparison
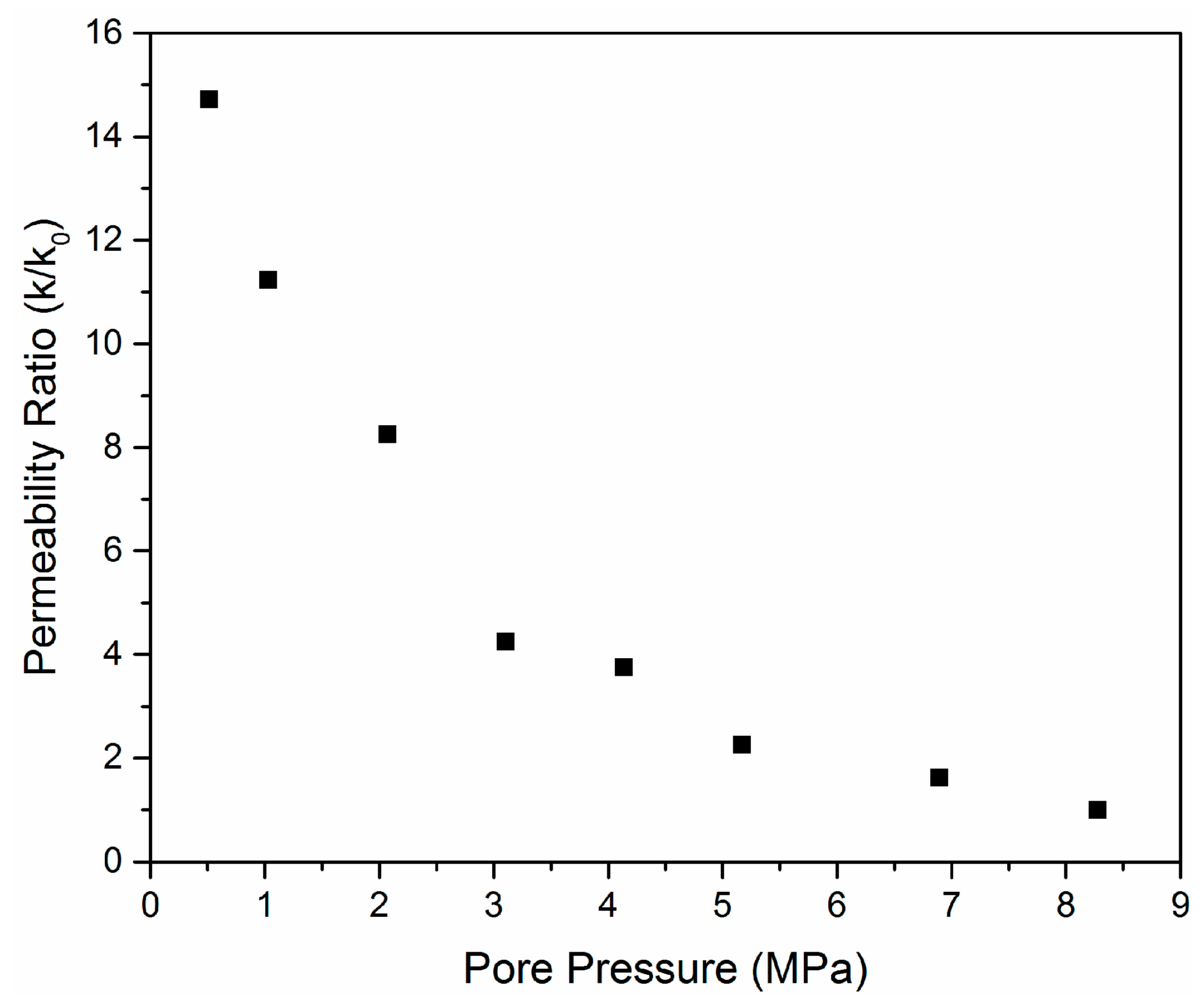
4.1. Experimental Results
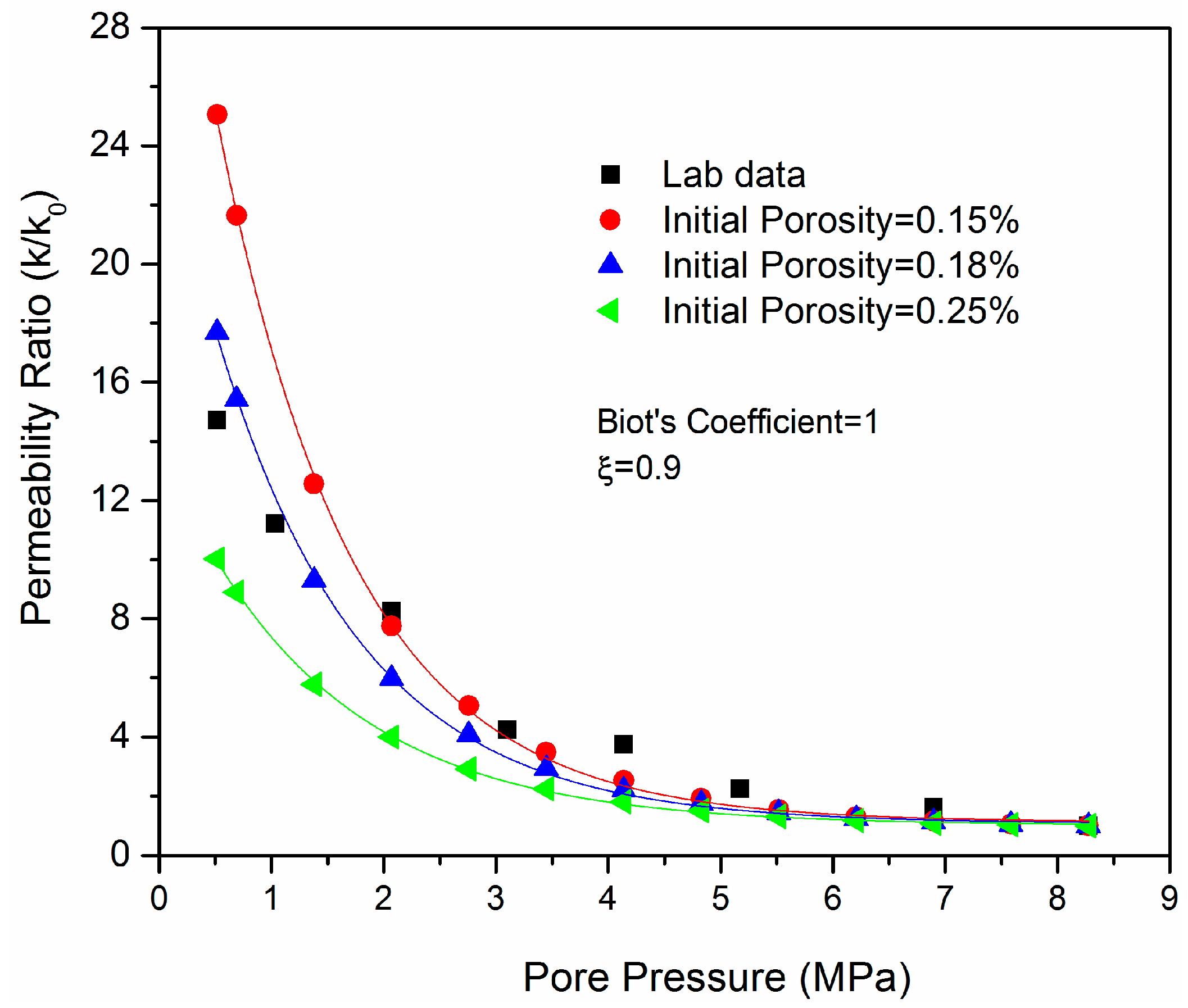
4.2. Model Validation
4.3. Model Comparison
4.4. Model Performance and Evaluation
5. Summary and Conclusions
- The proposed model decreases the effect of coal matrix shrinkage on cleat/fracture deformation and coal permeability by incorporating an effective deformation coefficient in the shrinkage term of the model. This effect, as expected, becomes significant in the low-pressure range.
- The proposed model is capable of accurately capturing the overall rising trend of coal permeability with gas depletion. Initial cleat porosity and cleat compressibility, being the critical input parameters, are the bridges connecting those two competing terms and permeability variation.
- For stress-based analytical models, the permeability predicted by the S&D model is always larger than the C&B model if the same input values are substituted when the same cleat compressibility factor is used.
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A. Analytical Permeability Models
Appendix A.1. Original and Improved Palmer and Mansoori model
Appendix A.2. Shi and Durucan Model
Appendix A.3. Cui and Bustin Model
References
- Harpalani, S.; Schraufnagel, R.A. Shrinkage of coal matrix with release of gas and its impact on permeability of coal. Fuel 1990, 69, 551–556. [Google Scholar] [CrossRef]
- Feng, R.; Harpalani, S.; Pandey, R. Laboratory measurement of stress-dependent coal permeability using pulse-decay technique and flow modeling with gas depletion. Fuel 2016, 177, 76–86. [Google Scholar] [CrossRef]
- Laubach, S.; Marrett, R.; Olson, J.; Scott, A. Characteristics and origins of coal cleat: A review. Int. J. Coal Geol. 1998, 35, 175–207. [Google Scholar] [CrossRef]
- Liu, S.; Wang, Y.; Harpalani, S. Anisotropy characteristics of coal shrinkage/swelling and its impact on coal permeability evolution with CO2 injection. Greenh. Gases Sci. Technol. 2016, 6, 615–632. [Google Scholar] [CrossRef]
- Seidle, J.; Jeansonne, M.; Erickson, D. Application of matchstick geometry to stress dependent permeability in coals. In Proceedings of the SPE Rocky Mountain Regional Meeting, Casper, WY, USA, 18–21 May 1992. [Google Scholar]
- Ma, Q.; Harpalani, S.; Liu, S. A simplified permeability model for coalbed methane reservoirs based on matchstick strain and constant volume theory. Int. J. Coal Geol. 2011, 85, 43–48. [Google Scholar] [CrossRef]
- Mitra, A.; Harpalani, S.; Liu, S. Laboratory measurement and modeling of coal permeability with continued methane production: Part 1—Laboratory results. Fuel 2012, 94, 110–116. [Google Scholar] [CrossRef]
- Zimmerman, R.W. Pore volume and porosity changes under uniaxial strain conditions. Transp. Porous Media 2017, 119, 481–498. [Google Scholar] [CrossRef]
- Feng, R. An optimized transient technique and flow modeling for laboratory permeability measurements of unconventional gas reservoirs with tight structure. J. Nat. Gas Sci. Eng. 2017, 46, 603–614. [Google Scholar] [CrossRef]
- Feng, R.; Harpalani, S.; Liu, J. Optimized pressure pulse-decay method for laboratory estimation of gas permeability of sorptive reservoirs: Part 2—Experimental study. Fuel 2017, 191, 565–573. [Google Scholar] [CrossRef]
- Feng, R.; Liu, J.; Harpalani, S. Optimized pressure pulse-decay method for laboratory estimation of gas permeability of sorptive reservoirs: Part 1—Background and numerical analysis. Fuel 2017, 191, 555–564. [Google Scholar] [CrossRef]
- Palmer, I.; Mansoori, J. How permeability depends on stress and pore pressure in coalbeds: A new model. In Proceedings of the SPE Annual Technical Conference and Exhibition, Denvor, CO, USA, 6–9 October 1996. [Google Scholar]
- Shi, J.Q.; Durucan, S. A model for changes in coalbed permeability during primary and enhanced methane recovery. SPE Reserv. Eval. Eng. 2005, 8, 291–299. [Google Scholar] [CrossRef]
- Cui, X.; Bustin, R. Volumetric strain associated with methane desorption and its impact on coalbed gas production from deep coal seams. AAPE Bull. 2005, 89, 1181–1202. [Google Scholar] [CrossRef]
- Gu, F.; Chalaturnyk, R. Numerical simulation of stress and strain due to gas sorption/desorption and their effects on in situ permeability of coalbeds. J. Can. Pet. Technol. 2006, 45. [Google Scholar] [CrossRef]
- Palmer, I. Permeability changes in coal: Analytical modeling. Int. J. Coal Geol. 2009, 77, 119–126. [Google Scholar] [CrossRef]
- Patching, T. Retention and release of gas in coal—A review. Can. Min. Met. Bull. 1970, 63, 1302–1308. [Google Scholar]
- Somerton, W.; Söylemezoḡlu, I.; Dudley, R. Effect of stress on permeability of coal. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1975, 12, 129–145. [Google Scholar] [CrossRef]
- Robertson, E.P.; Christiansen, R.L. A permeability model for coal and other fractured, sorptive-elastic media. SPE J. 2008, 13, 314–324. [Google Scholar] [CrossRef]
- Feng, R.; Harpalani, S.; Pandey, R. Evaluation of various pulse-decay laboratory permeability measurement techniques for highly stressed coals. Rock Mech. Rock Eng. 2017, 50, 297–308. [Google Scholar] [CrossRef]
- Liu, H.-H.; Rutqvist, J. A new coal-permeability model: Internal swelling stress and fracture—Matrix interaction. Transp. Porous Media 2010, 82, 157–171. [Google Scholar] [CrossRef]
- Liu, J.; Chen, Z.; Elsworth, D.; Miao, X.; Mao, X. Evolution of coal permeability from stress-controlled to displacement-controlled swelling conditions. Fuel 2011, 90, 2987–2997. [Google Scholar] [CrossRef]
- Harpalani, S.; Mitra, A. Impact of CO2 injection on flow behavior of coalbed methane reservoirs. Transp. Porous Media 2010, 82, 141–156. [Google Scholar] [CrossRef]
- Levine, J. Model study of the influence of matrix shrinkage on absolute permeability of coal bed reservoirs. Geol. Soc. Lond. Spec. Publ. 1996, 109, 197–212. [Google Scholar] [CrossRef]
- Robertson, E.P. Modeling permeability in coal using sorption-induced strain data. In Proceedings of the SPE Annual Technical Conference and Exhibition, Dallas, TX, USA, 9–12 October 2005. [Google Scholar]
- Seidle, J.R.; Huitt, L. Experimental measurement of coal matrix shrinkage due to gas desorption and implications for cleat permeability increases. In Proceedings of the International Meeting on Petroleum Engineering, Beijing, China, 14–17 November 1995. [Google Scholar]
- Wang, G.; Wei, X.; Wang, K.; Massarotto, P.; Rudolph, V. Sorption-induced swelling/shrinkage and permeability of coal under stressed adsorption/desorption conditions. Int. J. Coal Geol. 2010, 83, 46–54. [Google Scholar] [CrossRef]
- Liu, S. Estimation of Different Coal Compressibilities of Coalbed Methane Reservoirs under Replicated In Situ Condition; Southern Illinous University Carbondale: Carbondale, IL, USA, 2012. [Google Scholar]
- Fan, J.; Feng, R.; Wang, J.; Wang, Y. Laboratory investigation of coal deformation behavior and its influence on permeability evolution during methane displacement by CO2. Rock Mech. Rock Eng. 2017, 50, 1725–1737. [Google Scholar] [CrossRef]
- Chikatamarla, L.; Cui, X.; Bustin, R.M. Implications of volumetric swelling/shrinkage of coal in sequestration of acid gases. In Proceedings of the International Coalbed Methane Symposium, Tuscaloosa, AL, USA, 3–7 May 2004. [Google Scholar]
- Palmer, I.; Mavor, M.; Gunter, B. Permeability changes in coal seams during production and injection. In Proceedings of the International Coalbed Methane Symposium, University of Alabama, Tuscaloosa, AL, USA, 23–24 May 2007. [Google Scholar]
- Shi, J.; Durucan, S. Drawdown induced changes in permeability of coalbeds: A new interpretation of the reservoir response to primary recovery. Transp. Porous Media 2004, 56, 1–16. [Google Scholar] [CrossRef]
- Wang, S.; Elsworth, D.; Liu, J. A mechanistic model for permeability evolution in fractured sorbing media. J. Geophys. Res. Solid Earth 2012, 117. [Google Scholar] [CrossRef]
- Pan, Z.; Connell, L.D. A theoretical model for gas adsorption-induced coal swelling. Int. J. Coal Geol. 2007, 69, 243–252. [Google Scholar] [CrossRef]
- Liu, S.; Harpalani, S. A new theoretical approach to model sorption-induced coal shrinkage or swelling. AAPG Bull. 2013, 97, 1033–1049. [Google Scholar] [CrossRef]
- Pan, Z.; Connell, L. Modelling permeability for coal reservoirs: A review of analytical models and testing data. Int. J. Coal Geol. 2012, 92, 1–44. [Google Scholar] [CrossRef]
- Shi, J.-Q.; Pan, Z.; Durucan, S. Analytical models for coal permeability changes during coalbed methane recovery: Model comparison and performance evaluation. Int. J. Coal Geol. 2014, 136, 17–24. [Google Scholar] [CrossRef]
- Shi, J.Q.; Durucan, S. Exponential growth in san juan basin fruitland coalbed permeability with reservoir drawdown: Model match and new insights. SPE Reserv. Eval. Eng. 2010, 13, 914–925. [Google Scholar] [CrossRef]
- Walder, J.; Nur, A. Porosity reduction and crustal pore pressure development. J. Geophys. Res. Solid Earth 1984, 89, 11539–11548. [Google Scholar] [CrossRef]
- Cui, X.; Bustin, R.M.; Chikatamarla, L. Adsorption-induced coal swelling and stress: Implications for methane production and acid gas sequestration into coal seams. J. Geophys. Res. Solid Earth 2007, 112. [Google Scholar] [CrossRef]
- Clarkson, C.R.; Pan, Z.; Palmer, I.; Harpalani, S. Predicting sorption-induced strain and permeability increase with depletion for coalbed-methane reservoirs. SPE J. 2010, 15, 152–159. [Google Scholar] [CrossRef]
- Zhang, H.; Liu, J.; Elsworth, D. How sorption-induced matrix deformation affects gas flow in coal seams: A new fe model. Int. J. Rock Mech. Min. Sci. 2008, 45, 1226–1236. [Google Scholar] [CrossRef]
- Zahner, B. Application of material balance to determine ultimate recovery of a san juan fruitland coal well. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 5–8 October 1997. [Google Scholar]
- Liu, S.; Harpalani, S.; Pillalamarry, M. Laboratory measurement and modeling of coal permeability with continued methane production: Part 2—Modeling results. Fuel 2012, 94, 117–124. [Google Scholar] [CrossRef]

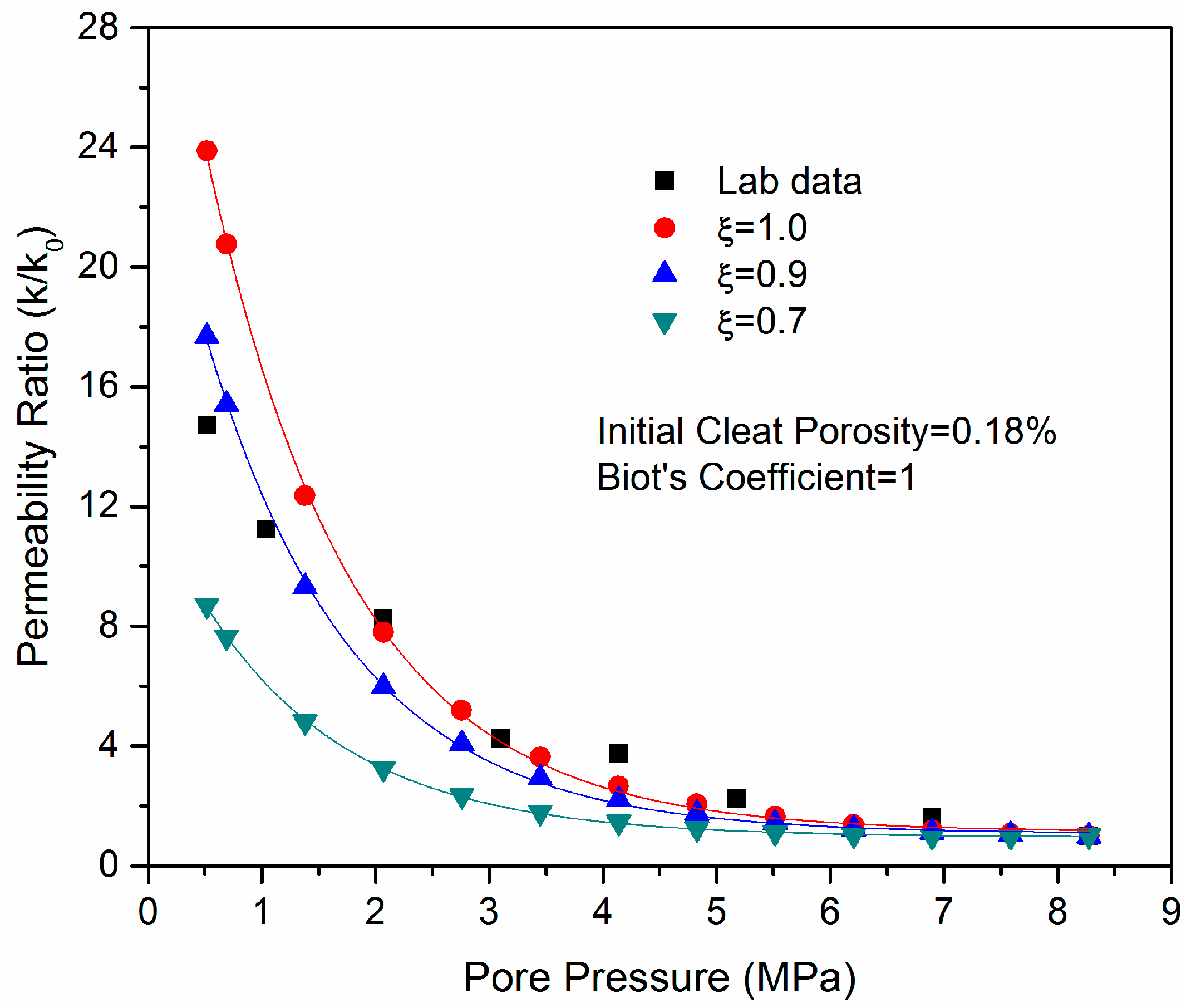
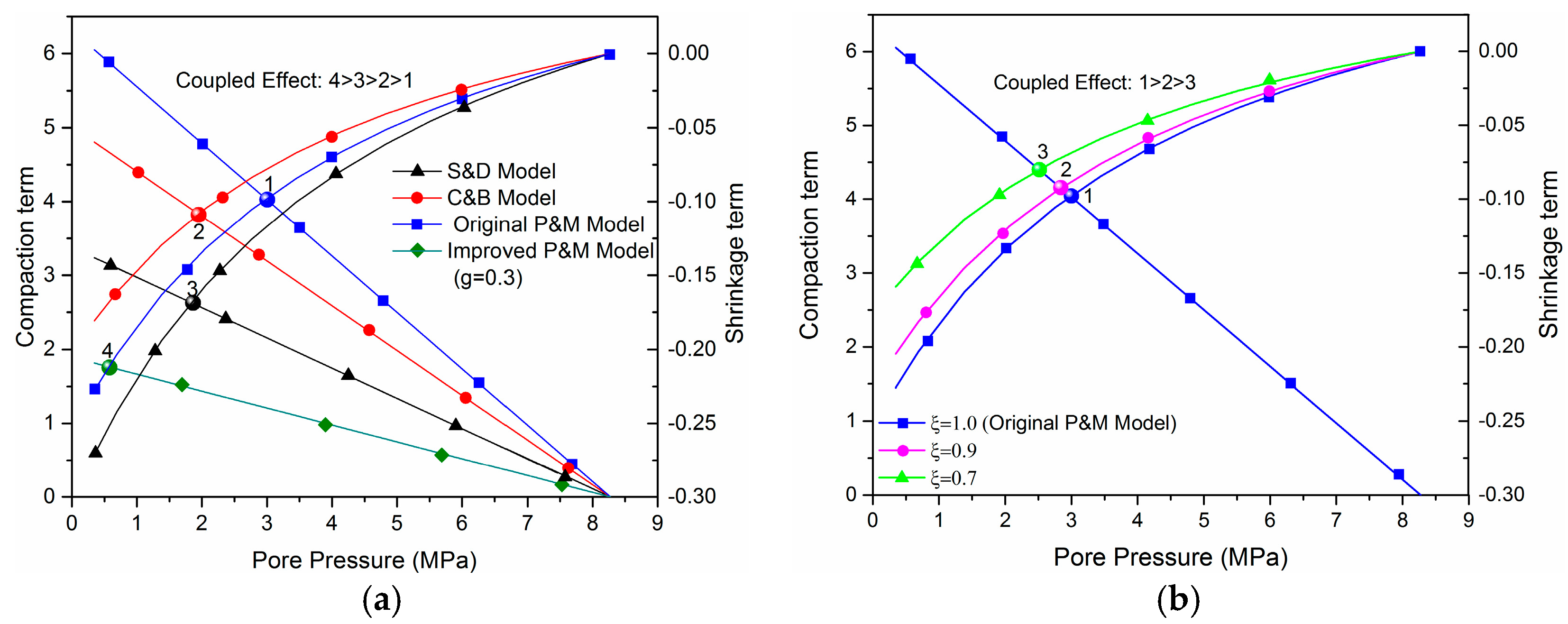
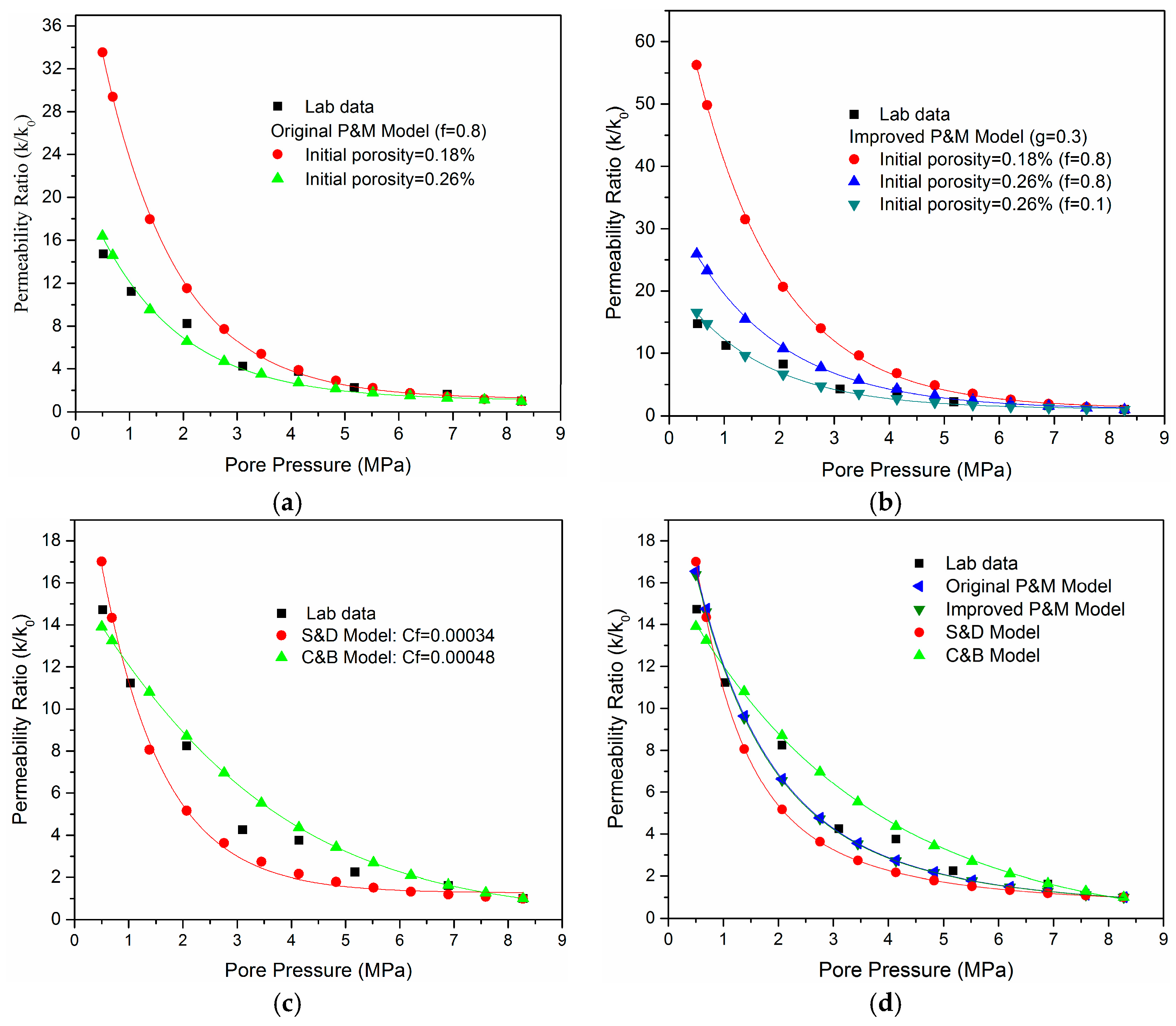
| Input Parameters | Selected Value | Sources |
|---|---|---|
| Young’s Modulus E, MPa | 3793 | Liu [28]; Palmer and Mansoori [12] |
| Poisson’s Ratio ν, Dimensionless | 0.29 | Liu [28] |
| Initial Porosity ϕ0, Dimensionless | 0.1–0.5% | Palmer and Mansoori [12] |
| ɛl, Dimensionless | 0.023 | Zahner [43] |
| pɛ, MPa | 4.5 | Zahner [43] |
| Model | Analytical Equation of Primary Variable | Coef. Δp | Coef. Δɛ |
|---|---|---|---|
| Shi and Durucan (2004) | |||
| Cui and Bustin (2005) | |||
| Palmer and Mansoori (1996) | |||
| Improved Palmer et al. (2007) | |||
| Proposed model |
| Pore Pressure, MPa | 8.3 | 6.9 | 5.2 | 4.1 | 3.1 | 2.1 | 1.0 | 0.5 | Average Deviation (%) | |
| Lab data, k/k0 | 1.0 | 1.6 | 2.3 | 3.8 | 4.3 | 8.3 | 11.2 | 14.7 | ||
| P&M model | k/k0 | 1.0 | 1.3 | 1.9 | 2.7 | 4.1 | 6.6 | 11.6 | 16.5 | 12.6 |
| Deviation, % | 0 | 21.2 | 14.1 | 26.7 | 4.5 | 19.6 | 3.1 | 11.7 | ||
| C&B model | k/k0 | 1.0 | 1.2 | 1.6 | 2.1 | 3.1 | 5.2 | 10.6 | 13.9 | 21.6 |
| Deviation, % | 0 | 26.7 | 28.4 | 42.7 | 26.4 | 37.4 | 5.5 | 5.7 | ||
| S&D model | k/k0 | 1.0 | 1.6 | 3.1 | 4.4 | 6.2 | 8.7 | 11.9 | 17.1 | 15.9 |
| Deviation, % | 0 | 1.4 | 36.5 | 17.4 | 44.9 | 5.6 | 5.6 | 15.9 | ||
| Proposed model | k/k0 | 1.0 | 1.7 | 2.1 | 2.7 | 3.8 | 6.5 | 11.5 | 16.1 | 9.8 |
| Deviation, % | 0 | 2.8 | 5.8 | 27.7 | 9.9 | 21.0 | 2.0 | 9.1 | ||
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Wang, Z.; Shi, L.; Feng, R. Analysis of Analytical Models Developed under the Uniaxial Strain Condition for Predicting Coal Permeability during Primary Depletion. Energies 2017, 10, 1849. https://doi.org/10.3390/en10111849
Li C, Wang Z, Shi L, Feng R. Analysis of Analytical Models Developed under the Uniaxial Strain Condition for Predicting Coal Permeability during Primary Depletion. Energies. 2017; 10(11):1849. https://doi.org/10.3390/en10111849
Chicago/Turabian StyleLi, Chuanming, Zhiqiang Wang, Lei Shi, and Ruimin Feng. 2017. "Analysis of Analytical Models Developed under the Uniaxial Strain Condition for Predicting Coal Permeability during Primary Depletion" Energies 10, no. 11: 1849. https://doi.org/10.3390/en10111849
APA StyleLi, C., Wang, Z., Shi, L., & Feng, R. (2017). Analysis of Analytical Models Developed under the Uniaxial Strain Condition for Predicting Coal Permeability during Primary Depletion. Energies, 10(11), 1849. https://doi.org/10.3390/en10111849





