Quantifying the Dosimetric Impact of Proton Range Uncertainties on RBE-Weighted Dose Distributions in Intensity-Modulated Proton Therapy for Bilateral Head and Neck Cancer
Abstract
1. Introduction
2. Materials and Methods
2.1. Contouring and Treatment Planning
2.2. Range Uncertainties
2.3. LETd and RWD Calculations
2.4. Dosimetric Analysis
3. Results
3.1. Mean Dose (Dmean) Evaluation for OARs
3.2. Maximum Dose (Dmax) Evaluation for OARs
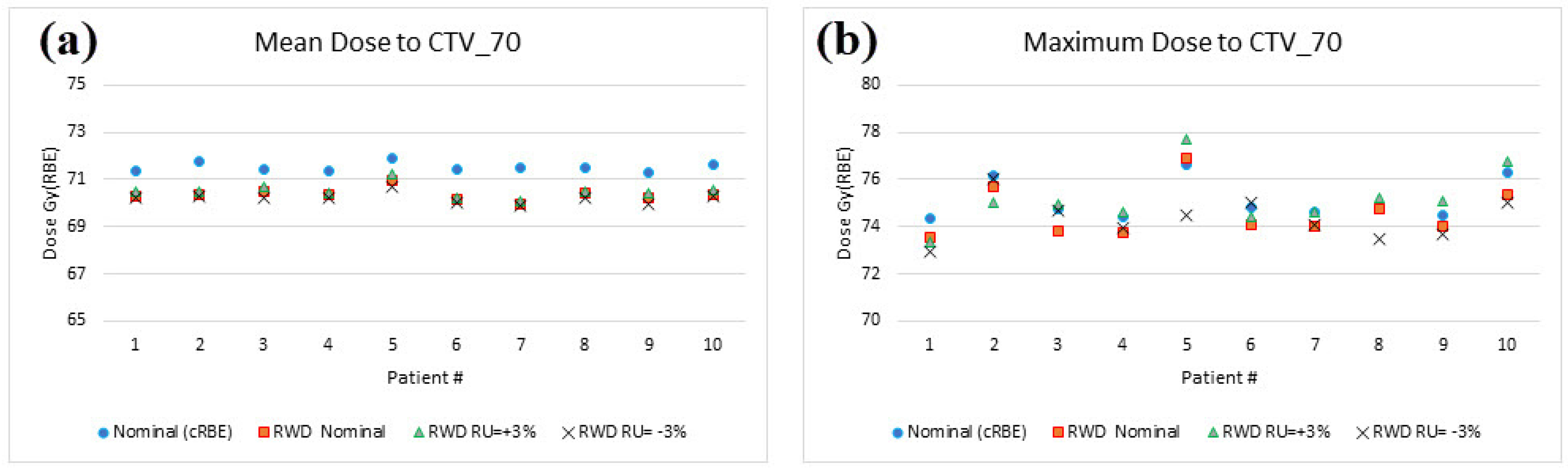
3.3. Dmean and Dmax Evaluation for Target Volumes
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Moreno, A.C.; Frank, S.J.; Garden, A.S.; Rosenthal, D.I.; Fuller, C.D.; Gunn, G.B.; Reddy, J.P.; Morrison, W.H.; Williamson, T.D.; Holliday, E.B.; et al. Intensity modulated proton therapy (IMPT)—The future of IMRT for head and neck cancer. Oral Oncol. 2019, 88, 66–74. [Google Scholar] [CrossRef] [PubMed]
- Padannayil, N.M.; Sharma, D.S.; Nangia, S.; Patro, K.C.; Gaikwad, U.; Burela, N. IMPT of head and neck cancer: Unsupervised machine learning treatment planning strategy for reducing radiation dermatitis. Radiat. Oncol. 2023, 18, 11. [Google Scholar] [CrossRef] [PubMed]
- Rana, S.; Storey, M.; Manthala Padannayil, N.; Shamurailatpam, D.S.; Bennouna, J.; George, J.; Chang, J. Investigating the utilization of beam-specific apertures for the intensity-modulated proton therapy (IMPT) head and neck cancer plans. Med. Dosim. 2021, 46, e7–e11. [Google Scholar] [CrossRef] [PubMed]
- Vai, A.; Molinelli, S.; Rossi, E.; Iacovelli, N.A.; Magro, G.; Cavallo, A.; Pignoli, E.; Rancati, T.; Mirandola, A.; Russo, S.; et al. Proton Radiation Therapy for Nasopharyngeal Cancer Patients: Dosimetric and NTCP Evaluation Supporting Clinical Decision. Cancers 2022, 14, 1109. [Google Scholar] [CrossRef] [PubMed]
- Paganetti, H.; Blakely, E.; Carabe-Fernandez, A.; Carlson, D.J.; Das, I.J.; Dong, L.; Grosshans, D.; Held, K.D.; Mohan, R.; Moiseenko, V.; et al. Report of the AAPM TG-256 on the relative biological effectiveness of proton beams in radiation therapy. Med. Phys. 2019, 46, e53–e78. [Google Scholar] [CrossRef] [PubMed]
- Paganetti, H. Relative biological effectiveness (RBE) values for proton beam therapy. Variations as a function of biological endpoint, dose, and linear energy transfer. Phys. Med. Biol. 2014, 59, R419–R472. [Google Scholar] [CrossRef] [PubMed]
- Rørvik, E.; Fjæra, L.F.; Dahle, T.J.; Dale, J.E.; Engeseth, G.M.; Stokkevåg, C.H.; Thörnqvist, S.; Ytre-Hauge, K.S. Exploration and application of phenomenological RBE models for proton therapy. Phys. Med. Biol. 2018, 63, 185013. [Google Scholar] [CrossRef] [PubMed]
- McNamara, A.; Willers, H.; Paganetti, H. Modelling variable proton relative biological effectiveness for treatment planning. Br. J. Radiol. 2020, 93, 20190334. [Google Scholar] [CrossRef] [PubMed]
- McNamara, A.L.; Schuemann, J.; Paganetti, H. A phenomenological relative biological effectiveness (RBE) model for proton therapy based on all published in vitro cell survival data. Phys. Med. Biol. 2015, 60, 8399–8416. [Google Scholar] [CrossRef]
- Unkelbach, J.; Botas, P.; Giantsoudi, D.; Gorissen, B.L.; Paganetti, H. Reoptimization of Intensity Modulated Proton Therapy Plans Based on Linear Energy Transfer. Int. J. Radiat. Oncol. Biol. Phys. 2016, 96, 1097–1106. [Google Scholar] [CrossRef]
- Haas-Kogan, D.; Indelicato, D.; Paganetti, H.; Esiashvili, N.; Mahajan, A.; Yock, T.; Flampouri, S.; MacDonald, S.; Fouladi, M.; Stephen, K.; et al. National Cancer Institute Workshop on Proton Therapy for Children: Considerations Regarding Brainstem Injury. Int. J. Radiat. Oncol. Biol. Phys. 2018, 101, 152–168. [Google Scholar] [CrossRef] [PubMed]
- Underwood, T.S.A.; McNamara, A.L.; Appelt, A.; Haviland, J.S.; Sørensen, B.S.; Troost, E.G.C. A systematic review of clinical studies on variable proton Relative Biological Effectiveness (RBE). Radiother. Oncol. 2022, 175, 79–92. [Google Scholar] [CrossRef] [PubMed]
- Carabe, A.; España, S.; Grassberger, C.; Paganetti, H. Clinical consequences of relative biological effectiveness variations in proton radiotherapy of the prostate, brain and liver. Phys. Med. Biol. 2013, 58, 2103–2117. [Google Scholar] [CrossRef] [PubMed]
- Giantsoudi, D.; Sethi, R.V.; Yeap, B.Y.; Eaton, B.R.; Ebb, D.H.; Caruso, P.A.; Rapalino, O.; Chen, Y.-L.E.; Adams, J.A.; Yock, T.I.; et al. Incidence of CNS Injury for a Cohort of 111 Patients Treated with Proton Therapy for Medulloblastoma: LET and RBE Associations for Areas of Injury. Int. J. Radiat. Oncol. Biol. Phys. 2016, 95, 287–296. [Google Scholar] [CrossRef] [PubMed]
- Giovannini, G.; Böhlen, T.; Cabal, G.; Bauer, J.; Tessonnier, T.; Frey, K.; Debus, J.; Mairani, A.; Parodi, K. Variable RBE in proton therapy: Comparison of different model predictions and their influence on clinical-like scenarios. Radiat. Oncol. 2016, 11, 68. [Google Scholar] [CrossRef] [PubMed]
- Hirayama, S.; Matsuura, T.; Yasuda, K.; Takao, S.; Fujii, T.; Miyamoto, N.; Umegaki, K.; Shimizu, S. Difference in LET-based biological doses between IMPT optimization techniques: Robust and PTV-based optimizations. J. Appl. Clin. Med. Phys. 2020, 21, 42–50. [Google Scholar] [CrossRef] [PubMed]
- Ödén, J.; Eriksson, K.; Toma-Dasu, I. Incorporation of relative biological effectiveness uncertainties into proton plan robustness evaluation. Acta Oncol. 2017, 56, 769–778. [Google Scholar] [CrossRef]
- Ödén, J.; Eriksson, K.; Toma-Dasu, I. Inclusion of a variable RBE into proton and photon plan comparison for various fractionation schedules in prostate radiation therapy. Med. Phys. 2017, 44, 810–822. [Google Scholar] [CrossRef]
- Yepes, P.; Adair, A.; Frank, S.J.; Grosshans, D.R.; Liao, Z.; Liu, A.; Mirkovic, D.; Poenisch, F.; Titt, U.; Wang, Q.; et al. Fixed- versus Variable-RBE Computations for Intensity Modulated Proton Therapy. Adv. Radiat. Oncol. 2019, 4, 156–167. [Google Scholar] [CrossRef]
- Bahn, E.; Bauer, J.; Harrabi, S.; Herfarth, K.; Debus, J.; Alber, M. Late Contrast Enhancing Brain Lesions in Proton-Treated Patients With Low-Grade Glioma: Clinical Evidence for Increased Periventricular Sensitivity and Variable RBE. Int. J. Radiat. Oncol. Biol. Phys. 2020, 107, 571–578. [Google Scholar] [CrossRef]
- Hahn, C.; Eulitz, J.; Peters, N.; Wohlfahrt, P.; Enghardt, W.; Richter, C.; Luhr, A. Impact of range uncertainty on clinical distributions of linear energy transfer and biological effectiveness in proton therapy. Med. Phys. 2020, 47, 6151–6162. [Google Scholar] [CrossRef] [PubMed]
- Rana, S.; Traneus, E.; Jackson, M.; Tran, L.; Rosenfeld, A.B. Quantitative analysis of dose-averaged linear energy transfer (LETd) robustness in pencil beam scanning proton lung plans. Med. Phys. 2022, 49, 3444–3456. [Google Scholar] [CrossRef] [PubMed]
- Traneus, E.; Oden, J. Introducing Proton Track-End Objectives in Intensity Modulated Proton Therapy Optimization to Reduce Linear Energy Transfer and Relative Biological Effectiveness in Critical Structures. Int. J. Radiat. Oncol. Biol. Phys. 2019, 103, 747–757. [Google Scholar] [CrossRef] [PubMed]
- Beetz, I.; Schilstra, C.; van der Schaaf, A.; van den Heuvel, E.R.; Doornaert, P.; van Luijk, P.; Vissink, A.; van der Laan, B.F.A.M.; Leemans, C.R.; Bijl, H.P.; et al. NTCP models for patient-rated xerostomia and sticky saliva after treatment with intensity modulated radiotherapy for head and neck cancer: The role of dosimetric and clinical factors. Radiother. Oncol. 2012, 105, 101–106. [Google Scholar] [CrossRef] [PubMed]
- Christianen, M.E.M.C.; Schilstra, C.; Beetz, I.; Muijs, C.T.; Chouvalova, O.; Burlage, F.R.; Doornaert, P.; Koken, P.W.; Leemans, C.R.; Rinkel, R.N.P.M.; et al. Predictive modelling for swallowing dysfunction after primary (chemo)radiation: Results of a prospective observational study. Radiother. Oncol. 2012, 105, 107–114. [Google Scholar] [CrossRef] [PubMed]
- Wagenaar, D.; Schuit, E.; van der Schaaf, A.; Langendijk, J.A.; Both, S. Can the mean linear energy transfer of organs be directly related to patient toxicities for current head and neck cancer intensity-modulated proton therapy practice? Radiother. Oncol. 2021, 165, 159–165. [Google Scholar] [CrossRef]
- Garbacz, M.; Gajewski, J.; Durante, M.; Kisielewicz, K.; Krah, N.; Kopeć, R.; Olko, P.; Patera, V.; Rinaldi, I.; Rydygier, M.; et al. Quantification of biological range uncertainties in patients treated at the Krakow proton therapy centre. Radiat. Oncol. 2022, 17, 50. [Google Scholar] [CrossRef] [PubMed]
- Gutierrez, A.; Rompokos, V.; Li, K.; Gillies, C.; D’Souza, D.; Solda, F.; Fersht, N.; Chang, Y.C.; Royle, G.; Amos, R.A.; et al. The impact of proton LET/RBE modeling and robustness analysis on base-of-skull and pediatric craniopharyngioma proton plans relative to VMAT. Acta Oncol. 2019, 58, 1765–1774. [Google Scholar] [CrossRef] [PubMed]
- Guan, F.; Geng, C.; Ma, D.; Bronk, L.; Kerr, M.; Li, Y.; Gates, D.; Kroger, B.; Sahoo, N.; Titt, U.; et al. RBE Model-Based Biological Dose Optimization for Proton Radiobiology Studies. Int. J. Part. Ther. 2018, 5, 160–171. [Google Scholar] [CrossRef] [PubMed]
- Marshall, T.I.; Chaudhary, P.; Michaelidesová, A.; Vachelová, J.; Davídková, M.; Vondráček, V.; Schettino, G.; Prise, K.M. Investigating the Implications of a Variable RBE on Proton Dose Fractionation Across a Clinical Pencil Beam Scanned Spread-Out Bragg Peak. Int. J. Radiat. Oncol. Biol. Phys. 2016, 95, 70–77. [Google Scholar] [CrossRef]
- Resch, A.F.; Landry, G.; Kamp, F.; Cabal, G.; Belka, C.; Wilkens, J.J.; Parodi, K.; Dedes, G. Quantification of the uncertainties of a biological model and their impact on variable RBE proton treatment plan optimization. Phys. Med. 2017, 36, 91–102. [Google Scholar] [CrossRef] [PubMed]
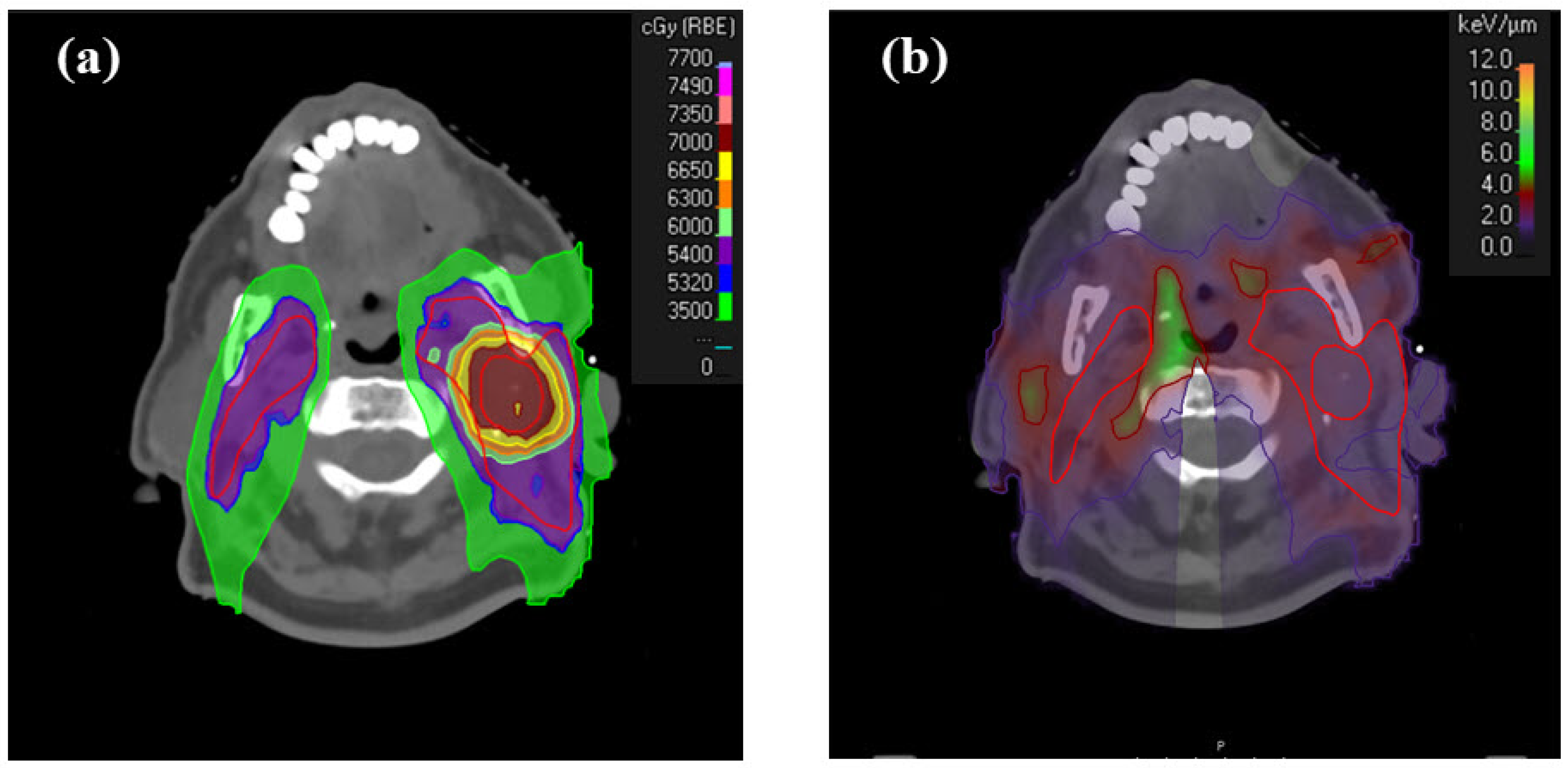
| Δavg | |||||||
|---|---|---|---|---|---|---|---|
| Dmean [Gy(RBE)] | Dmax [Gy(RBE)] | ||||||
| OARs | Nominal | RU = +3% | RU = −3% | Nominal | RU = +3% | RU = −3% | |
| Brachail Plexus_L | - | - | - | 10.44 | 10.18 | 10.75 | |
| Brachical Plexus_R | - | - | - | 9.86 | 9.65 | 10.36 | |
| Brain | - | - | - | 5.80 | 4.93 | 6.59 | |
| Brainstem | - | - | - | 3.14 | 2.78 | 3.86 | |
| Constrictor Muscles | 6.75 | 5.59 | 7.85 | - | - | - | |
| Esophagus | 2.76 | 2.09 | 3.40 | - | - | - | |
| Larynx | 6.06 | 5.18 | 6.91 | - | - | - | |
| Mandible | - | - | - | 7.17 | 7.13 | 7.10 | |
| Oral Cavity | 3.33 | 2.60 | 4.00 | - | - | - | |
| Parotid_L | 4.59 | 4.11 | 5.02 | - | - | - | |
| Parotid_R | 4.02 | 3.72 | 4.27 | - | - | - | |
| Spinal Cord | - | - | - | 3.53 | 2.13 | 5.19 | |
| Thyroid | 6.72 | 6.54 | 6.90 | - | - | - | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rana, S.; Manthala Padannayil, N.; Tran, L.; Rosenfeld, A.B.; Saeed, H.; Kasper, M. Quantifying the Dosimetric Impact of Proton Range Uncertainties on RBE-Weighted Dose Distributions in Intensity-Modulated Proton Therapy for Bilateral Head and Neck Cancer. Curr. Oncol. 2024, 31, 3690-3697. https://doi.org/10.3390/curroncol31070272
Rana S, Manthala Padannayil N, Tran L, Rosenfeld AB, Saeed H, Kasper M. Quantifying the Dosimetric Impact of Proton Range Uncertainties on RBE-Weighted Dose Distributions in Intensity-Modulated Proton Therapy for Bilateral Head and Neck Cancer. Current Oncology. 2024; 31(7):3690-3697. https://doi.org/10.3390/curroncol31070272
Chicago/Turabian StyleRana, Suresh, Noufal Manthala Padannayil, Linh Tran, Anatoly B. Rosenfeld, Hina Saeed, and Michael Kasper. 2024. "Quantifying the Dosimetric Impact of Proton Range Uncertainties on RBE-Weighted Dose Distributions in Intensity-Modulated Proton Therapy for Bilateral Head and Neck Cancer" Current Oncology 31, no. 7: 3690-3697. https://doi.org/10.3390/curroncol31070272
APA StyleRana, S., Manthala Padannayil, N., Tran, L., Rosenfeld, A. B., Saeed, H., & Kasper, M. (2024). Quantifying the Dosimetric Impact of Proton Range Uncertainties on RBE-Weighted Dose Distributions in Intensity-Modulated Proton Therapy for Bilateral Head and Neck Cancer. Current Oncology, 31(7), 3690-3697. https://doi.org/10.3390/curroncol31070272





