Learning Multiple Movements in Parallel—Accurately and in Random Order, or Each with Added Noise?
Abstract
1. Introduction
2. Materials and Methods
2.1. Participants
2.2. Design
2.2.1. Intervention
2.2.2. Test Design
2.3. Data Analysis
3. Results
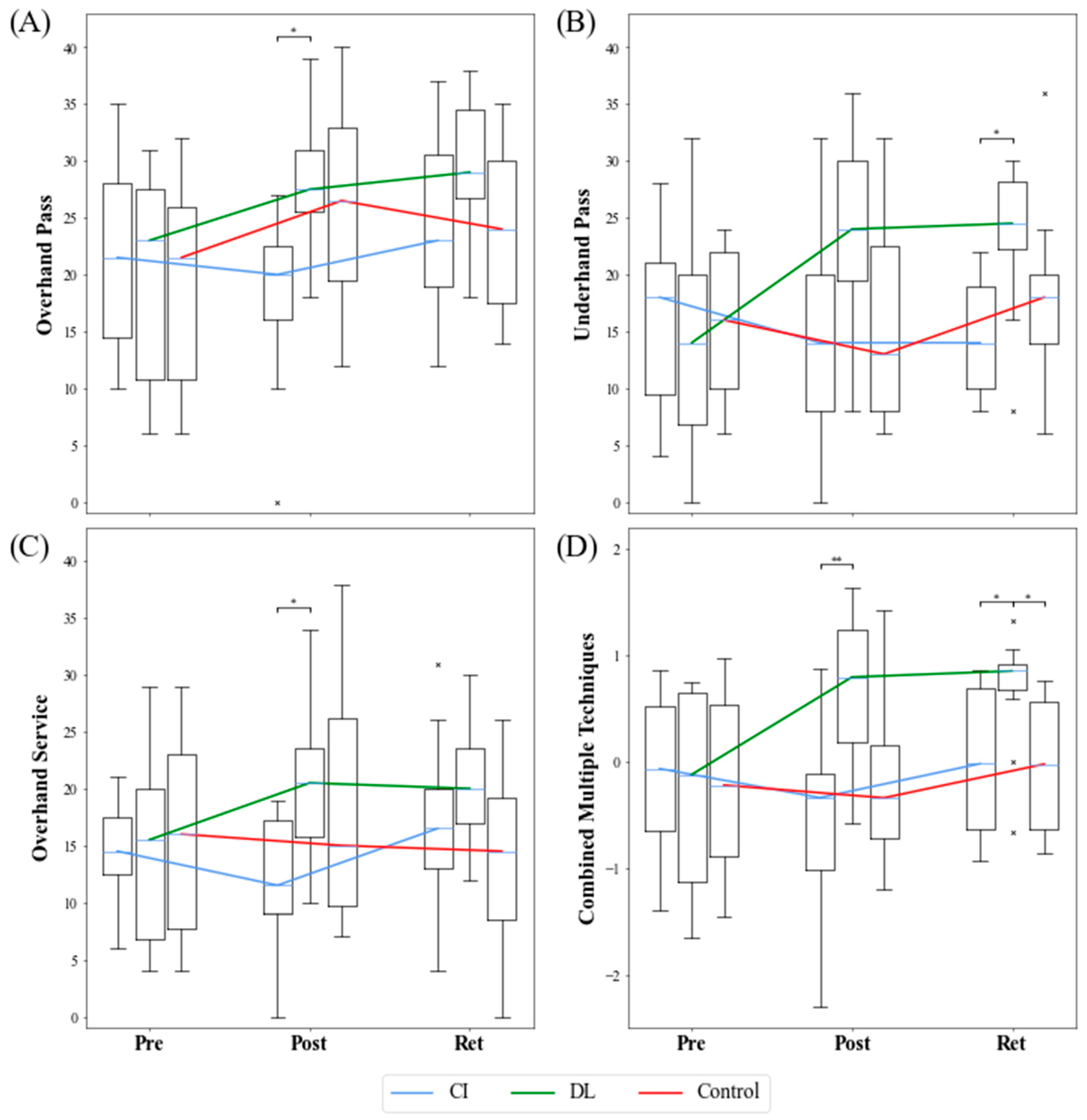
3.1. Development within the Groups throughout the Measurement Times
3.2. Development between Groups in the Individual Skills across Measurement Period
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| A Standing Position |
|---|
| 1 Stand with one leg forward, change leg position while performing. |
| 2 Stand on one leg, change leg while executing. 3 Both legs parallel. |
| 4 Both knees bent, but parallel. |
| 5 Bend knees while performing. |
| 6 Both knees bent, but one leg in front, change leg position while executing. |
| 7 Legs slightly apart. |
| 8 Spread legs slightly while performing. |
| 9 Extend one leg forward. |
| 10 Leg raised, knee bent to chest height, switch with other leg. |
| 11 As in Task 10, but change angle of legs. |
| 12 Stand on the balls of the feet. |
| 13 Stand on the heels of the feet. |
| B Trunk Movement |
| 14 Forward movement during the execution. |
| 15 Sideways movement during execution. |
| 16 Backward movement during execution. |
| 17 Rounding in during execution. |
| 18 Straighten during the execution. |
| C Head Movement |
| 19 Look up. |
| 20 Look down. |
| 21 Head circling. |
| 22 One eye closed. |
| 23 Both eyes blinking. |
| D Hand/Arm Movement |
| 24 Arms higher. |
| 25 Arms (more) forward. |
| 26 Arms sideways. |
| 27 Elbow position slightly back to the side. |
| 28 Elbow position slightly forward and inward. |
| 29 Elbows bent. |
| 30 Elbows extended. |
| 31 Arms crossed. |
| 32 Hands on top of each other. |
| 33 Hands parallel |
| 34 Hands supinated. |
| 35 Hands pronated. |
| 36 Hands as fists. |
| 37 Hands wide open. |
| E Mass Movement (Velocity Change) |
| 38 One step forward during the execution. |
| 39 Two steps forward during the execution. |
| 40 One step backward during execution. |
| 41 Two steps backward during the execution. |
| 42 Side steps to the left and right during execution. |
| 43 Moving one leg forward, backward, left, right during the execution. |
| 44 During execution one quick step forward. |
| 45 During execution one quick step backward. |
| 46 During the execution two quick steps to the front. |
| 47 During execution two quick steps backward. |
| 48 During the execution quick lateral steps to the left and right. |
| 49 Quick one-legged movement forward, backward, left, right during execution. |
| F Jumps/Hops |
| 50 During the execution single-leg hops to the front, back, left, right. |
| 51 While performing two-legged hop forward, backward, left, right. |
| 52 Jump with execution of the skill before landing. |
| 53 Jump with execution of the skill exactly at the landing. |
| G Running |
| 54 Fast runs to the execution position. |
| 55 Fast and slow runs during the performance. |
| 56 Half turn left/right on command immediately before execution. |
| 57 Execution with hip position 60,120,180,240,300 to the strike direction. |
| H Position Changes |
| 58 Execution in seated position with legs crossed, legs forward, hurdle seat position, legs wide apart, one leg bent. |
| 59 Execution in a seated position with legs crossed, legs forward, hurdle seat position, legs wide apart, one leg bent. |
| 60 Execution lying bent, stretched, on the side. |
| 61 but faster. |
| 62 but slower. |
| 63 Execution backwards |
| I Combine at Least two of all above |
| 64 Jump and turn left while performing |
| 65 27 and 46 while execution |
| 66 21 and 32 while execution |
| 67 Different type of balls. |
| 68 Different terrains (e.g., on sand, grass, soft floor mat, ...) |
References
- Bock, O.; Schneider, S.; Bloomberg, J. Conditions for interference versus facilitation during sequential sensorimotor adaptation. Exp. Brain Res. 2001, 138, 359–365. [Google Scholar] [CrossRef]
- Battig, W.F. The flexibility of human memory. In Levels of Processing in Human Memory; Cermak, L.S., Craik, F.I., Eds.; Erlbaum: Hillsdale, NJ, USA, 1979; pp. 23–44. [Google Scholar]
- Shea, J.B.; Morgan, R.L. Contextual interference effects on the acquisition, retention, and transfer of a motor skill. J. Exp. Psychol. Hum. Learn. Mem. 1979, 5, 179–187. [Google Scholar] [CrossRef]
- Schöllhorn, W.I. Individualität—Ein vernachlässigter Parameter? [Individuality—A neglected parameter?]. Leistungssport 1999, 29, 7–11. [Google Scholar]
- Schöllhorn, W.I. Practical concequences of systems dynamic approach to skill and strength training. Acta Acad. Olymp. Est. 2000, 8, 25–37. [Google Scholar]
- Battig, W.F. Facilitation and interference. In Acquisition of Skill; Bilodeau, E.A., Ed.; Academic Press: New York, NY, USA, 1966; pp. 215–244. [Google Scholar]
- Shea, J.B.; Zimny, S.T. Context effects in memory and learning movement information. In Memory and Control of Action; Magill, R.A., Ed.; North-Holland: New York, NY, USA, 1983; pp. 345–366. [Google Scholar]
- Magill, R.A.; Hall, K.G. A review of the contextual interference effect in motor skill acquisition. Hum. Mov. Sci. 1990, 9, 241–289. [Google Scholar] [CrossRef]
- Brady, F. Contextual Interference: A Meta-Analytic Study. Percept. Mot. Skills 2004, 99, 116–126. [Google Scholar] [CrossRef]
- Barreiros, J.; Figueiredo, T.; Godinho, M. The contextual interference effect in applied settings. Eur. Phys. Educ. Rev. 2007, 13, 195–208. [Google Scholar]
- Pollock, B.J.; Lee, T.D. Dissociated contextual interference effects in children and adults. Percept. Mot. Ski. 1997, 84, 851–858. [Google Scholar]
- Guadagnoli, M.A.; Holcomb, W.R.; Weber, T.J. The relationship between contextual interference effects and performer expertise on the learning of a putting task. J. Hum. Mov. Stud. 1999, 37, 19–36. [Google Scholar]
- Hall, K.G.; Domingues, D.A.; Cavazos, R. Contextual Interference Effects with Skilled Baseball Players. Percept. Mot. Ski. 1994, 78, 835–841. [Google Scholar] [CrossRef]
- Schöllhorn, W.I.; Rizzi, N.; Slapšinskaitė-Dackevičienė, A.; Leite, N. Always Pay Attention to Which Model of Motor Learning You Are Using. Int. J. Environ. Res. Public Health 2022, 19, 711. [Google Scholar] [CrossRef]
- Bortoli, L.; Robazza, C.; Durigon, V.; Carra, C. Effects of contextual interference on learning technical sports skills. Percept. Mot. Ski. 1992, 75, 555–562. [Google Scholar] [CrossRef] [PubMed]
- Fialho, J.V.A.P.; Benda, R.N.; Ugrinowitsch, H. The contextual interference effect in a serve skill acquisition with experienced volleyball players. J. Hum. Mov. Stud. 2006, 50, 65–77. [Google Scholar]
- French, K.E.; Rink, J.E.; Werner, P.H. Effects of contextual interference on retention of three volleyball skills. Percept. Mot. Ski. 1990, 71, 179–186. [Google Scholar] [CrossRef]
- Jones, L.L.; French, K.E. Effects of contextual interference on acquisition and retention of three volleyball skills. Percept. Mot. Ski. 2007, 105 Pt 1, 883–890. [Google Scholar] [CrossRef]
- Kalkhoran, J.F.; Shariati, A. The Effects of Contextual Interference on Learning Volleyball Motor Skills. J. Sport Sci. 2015, 6, 12–20. [Google Scholar]
- Meira, J.R.; Tani, G. Contextual interference effects assessed by extended transfer trials in the acquisition of the volleyball serve. J. Hum. Mov. Stud. 2003, 45, 449–468. [Google Scholar]
- Pasand, F.; Heydar, F.; Gholamhossien, N. The Effect of Gradual Increase in Contextual Interference on Acquisition, Retention and Transfer of Volleyball Skills. Int. J. Kinesiol. Sports Sci. 2016, 4, 72–77. [Google Scholar]
- Travlos, A.K. Specificity and variability of practice, and contextual interference in acquisition and transfer of an underhand volleyball serve. Percept. Mot. Ski. 2010, 110, 298–312. [Google Scholar] [CrossRef]
- Zetou, E.; Michalopoulou, M.; Giazitzi, K.; Kioumourtzoglou, E. Contextual interference effects in learning volleyball skills. Percept. Mot. Ski. 2007, 104, 995–1004. [Google Scholar] [CrossRef]
- Moxley, S.E. Schema: The variability of practice hypothesis. J. Mot. Behav. 1979, 11, 65–70. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, R.A. A schema theory of discrete motor skill learning. Psychol. Rev. 1975, 82, 225–260. [Google Scholar] [CrossRef]
- Wulf, G.; Lee, T.D. Contextual interference in movements of the same class: Differential effects on program and parameter learning. J. Mot. Behav. 1993, 25, 254–263. [Google Scholar] [CrossRef] [PubMed]
- Schöllhorn, W.I. Invited commentary: Differential learning is different from contextual interference learning. Hum. Mov. Sci. 2016, 47, 240–245. [Google Scholar] [CrossRef]
- Postman, L.; Underwood, B.J. Critical issues in interference theory. Mem. Cogn. 1973, 1, 19–40. [Google Scholar] [CrossRef]
- Briggs, G.E. Retroactive inhibition as a function of the degree of original and interpolated learning. J. Exp. Psychol. 1957, 53, 60–67. [Google Scholar] [CrossRef]
- Duncan, C.P. Transfer in motor learning as a function of degree of first-task learning and inter-task similarity. J. Exp. Psychol. 1953, 45, 1–11. [Google Scholar] [CrossRef]
- Melton, A.W.; von Lackum, W.J. Retroactive and proactive inhibition in retention: Evidence for a two-factor theory of retroactive inhibition. Am. J. Psychol. 1941, 44, 157–174. [Google Scholar] [CrossRef]
- Wright, D.L.; Shea, C.H. Contextual Dependencies in Motor Skills. Mem. Cogn. 1991, 19, 361–370. [Google Scholar] [CrossRef]
- Broadbent, D.P.; Causer, J.; Williams, A.M.; Ford, P.R. The role of error processing in the contextual interference effect during the training of perceptual-cognitive skills. J. Exp. Psychol. Hum. Percept. Perform. 2017, 43, 1329. [Google Scholar] [CrossRef]
- Maxwell, J.P.; Masters, R.S.; Kerr, E.; Weedon, E. The implicit benefit of learning without errors. Q. J. Exp. Psychol. Sect. A 2001, 54, 1049–1068. [Google Scholar] [CrossRef] [PubMed]
- Feghhi, I.; Abdoli, B.; Valizadeh, R. Compare contextual interference effect and practice specificity in learning basketball free throw. Procedia Soc. Behav. Sci. 2011, 15, 2176–2180. [Google Scholar] [CrossRef]
- Baddeley, A.D. Exploring Working Memory: Selected Works of Alan Baddeley, 1st ed.; Routledge: London, UK, 2018. [Google Scholar]
- Bjork, R.A. Forgetting as a friend of learning. In Remembering; Psychology Press: London, UK, 2014; pp. 39–52. [Google Scholar]
- Lee, T.D.; Magill, R.A.; Weeks, D.J. Influence of practice schedule on testing schema theory predictions in adults. J. Mot. Behav. 1985, 17, 282–289. [Google Scholar] [CrossRef] [PubMed]
- Lee, T.D.; Magill, R.A. The locus of contextual interference in motor-skill acquisition. J. Exp. Psychol. Learn. Mem. Cogn. 1983, 9, 730–746. [Google Scholar] [CrossRef]
- Bernstein, N.A. The Co-Ordination and Regulation of Movements; Pergamon Press: New York, NY, USA, 1967. [Google Scholar]
- Haykin, S. Neural Networks: A Comprehensive Foundation, 1st ed; McMaster University: Toronto, ON, Canada, 1994. [Google Scholar]
- Lage, G.M.; Ugrinowitsch, H.; Apolinário-Souza, T.; Vieira, M.M.; Albuquerque, M.R.; Benda, R.N. Repetition and variation in motor practice: A review of neural correlates. Neurosci. Biobehav. Rev. 2015, 57, 132–141. [Google Scholar] [CrossRef]
- Baddeley, A. A new component of working memory? Trends in Cognitive Sciences. Episodic Buffer 2000, 4, 417–423. [Google Scholar]
- Schöner, G.; Haken, H.; Kelso, J.A.S. A stochastic theory of phase transitions in human hand movement. Biol. Cybern. 1986, 53, 247–257. [Google Scholar] [CrossRef]
- Schöllhorn, W.I. Differenzielles Lehren und Lernen von Bewegung—Durch veränderte Annahmen zu neuen Konsequenzen [Differential teaching and learning of movement—Through changed assumptions to new consequences]. In Zur Vernetzung von Forschung und Lehre in Biomechanik; Gabler, H., Göhner, U., Schiebl, F., Eds.; Sportmotorik und Trainingswissenschaft; Czwalina: Hamburg, Germany, 2005; pp. 125–135. [Google Scholar]
- Schöllhorn, W.I.; Beckmann, H.; Michelbrink, M.; Sechelmann, M.; Trockel, M.; Davids, K. Does noise provide a basis for the unification of motor learning theories? Int. J. Sport Psychol. 2006, 37, 186–206. [Google Scholar]
- Janssen, D.; Schöllhorn, W.I.; Lubienetzki, J.; Fölling, K.; Kokenge, H.; Davids, K. Recognition of emotions in gait patterns by means of artificial neural nets. J. Nonverbal Behav. 2008, 32, 79–92. [Google Scholar] [CrossRef]
- Janssen, D.; Schöllhorn, W.I.; Newell, K.M.; Jäger, J.M.; Rost, F.; Vehof, K. Diagnosing fatigue in gait patterns by support vector machines and self-organizing maps. Hum. Mov. Sci. 2011, 30, 966–975. [Google Scholar] [CrossRef] [PubMed]
- Burdack, J.; Horst, F.; Aragonés, D.; Eekhoff, A.; Schöllhorn, W.I. Fatigue-related and timescale-dependent changes in individual movement patterns identified using support vector machine. Front. Psychol. 2020, 11, 551548. [Google Scholar] [CrossRef]
- Horst, F.; Kramer, F.; Schäfer, B.; Eekhoff, A.; Hegen, P.; Nigg, B.M.; Schöllhorn, W.I. Daily changes of individual gait patterns identified by means of support vector machines. Gait Posture 2016, 49, 309–314. [Google Scholar] [CrossRef]
- Horst, F.; Eekhoff, A.; Newell, K.M.; Schöllhorn, W.I. Intra-individual gait patterns across different time-scales as revealed by means of a supervised learning model using kernel-based discriminant regression. PLoS ONE 2017, 12, e0179738. [Google Scholar] [CrossRef] [PubMed]
- Horst, F.; Mildner, M.; Schöllhorn, W.I. One-year persistence of individual gait patterns identified in a follow-up study—A call for individualised diagnose and therapy. Gait Posture 2017, 58, 476–480. [Google Scholar] [CrossRef] [PubMed]
- Römer, J.; Schöllhorn, W.I.; Jaitner, T.; Preiss, R. Differenzielles Lernen bei der Aufschlagannahme im Volleyball. In Messplätze, Messplatztraining, motorisches Lernen: Ausgewählte Beiträge; Krug, J., Müller, T., Eds.; Academia Verlag: Sankt Augustin, Germany, 2003; pp. 129–133. [Google Scholar]
- Schönherr, T.; Schöllhorn, W.I. Differencial Learning in Basketball. In Book of Abstracts, Proceedings of the EWOMS-European Workshop on Movement Science, Mechanics-Physiology-Psychology, Münster, Germany, 22–24 May 2003; Schöllhorn, W.I., Bohn, C., Jäger, J.M., Schaper, H., Alichman, M., Eds.; Sport und Buch Strauss: Köln, Germany, 2003; pp. 58–59. [Google Scholar]
- Trockel, M.; Schöllhorn, W.I. Differential Learning in Football. In Book of Abstracts, Proceedings of the EWOMS-European Workshop on Movement Science, Mechanics-Physiology-Psychology, Münster, Germany, 22–24 May 2003; Schöllhorn, W.I., Bohn, C., Jäger, J.M., Schaper, H., Alichman, M., Eds.; Sport und Buch Strauss: Köln, Germany, 2003; pp. 64–65. [Google Scholar]
- Schöllhorn, W.; Röber, F.; Jaitner, T.; Hellstern, W.; Käubler, W. Discrete and continuous effects of traditional and differential sprint training. In Proceedings of the 6th Annual Congress of the European College of Sport Sciences, Colonia, Germany, 24–28 July 2001; pp. 24–28. [Google Scholar]
- Albrecht, S.; Janssen, D.; Quarz, E.; Newell, K.M.; Schöllhorn, W.I. Individuality of Movements in music-finger and body movements during playing of the flute. Hum. Mov. Sci. 2014, 35, 131–144. [Google Scholar] [CrossRef]
- Pabel, S.-O.; Freitag, F.; Hrasky, V.; Zapf, A.; Wiegand, A. Randomised controlled trial on differential learning of toothbrushing in 6- to 9-year-old children. Clin. Oral Investig. 2018, 22, 2219–2228. [Google Scholar] [CrossRef]
- Serrien, B.; Tassignon, B.; Verschueren, J.; Meeusen, R.; Baeyens, J.P. Short-term effects of differential learning and contextual interference in a goalkeeper-like task: Visuomotor response time and motor control. Eur. J. Sport Sci. 2020, 20, 1061–1071. [Google Scholar] [CrossRef]
- Hegen, P.; Schöllhorn, W.I. Gleichzeitig in verschiedenen Bereichen besser werden, ohne zu wiederholen? Paralleles differenzielles Training von zwei Techniken im Fußball. Leistungssport 2012, 42, 17–23. [Google Scholar]
- Schöllhorn, W.I.; Paschke, M.; Beckmann, H. Differenzielles Training im Volleyball beim Erlernen von zwei Techniken. In Volleyball 2005; Beach-WM, Artikel; Langolf, K., Roth, R., Eds.; Czwalina: Hamburg, Germany, 2006; pp. 97–105. [Google Scholar]
- Apidogo, J.B.; Burdack, J.; Schöllhorn, W.I. Repetition without Repetition or Differential Learning of Multiple Techniques in Volleyball? Int. J. Environ. Res. Public Health 2021, 18, 10499. [Google Scholar] [CrossRef] [PubMed]
- Klimesch, W.; Doppelmayr, M.; Russegger, H.; Pachinger, T.; Schwaiger, J. Induced alpha band power changes in the human EEG and attention. Neurosci. Lett. 1998, 244, 73–76. [Google Scholar] [CrossRef]
- Minguillon, J.; Lopez-Gordo, M.A.; Pelayo, F. Stress Assessment by Prefrontal Relative Gamma. Front. Comput. Neurosci. 2016, 10, 101. [Google Scholar] [CrossRef] [PubMed]
- Henz, D.; Schöllhorn, W.I. Differential training facilitates early consolidation in motor learning. Front. Behav. Neurosci. 2016, 10, 199. [Google Scholar] [CrossRef] [PubMed]
- Henz, D.; John, A.; Merz, C.; Schöllhorn, W.I. Post-task effects on EEG brain activity differ for various differential learning and contextual interference protocols. Front. Hum. Neurosci. 2018, 12, 19. [Google Scholar] [CrossRef] [PubMed]
- Schöllhorn, W.; Horst, F. Effects of complex movements on the brain as a result of increased decision-making. J. Complexity Health Sci. 2020, 2, 40–45. [Google Scholar] [CrossRef]
- Reynoso, S.R.; Sabido Solana, R.; Reina Vaíllo, R.; Moreno Hernández, F.J. Differential learning applied to volleyball serves in novice athletes. Apunt. Educ. Fis. Esports 2013, 114, 45–52. [Google Scholar]
- Cohen, J. The Concepts of Power Analysis BT—Statistical Power Analysis for the Behavioral Sciences (Revised Edition); Hillsdale, N.J., Ed.; Lawrence Erlbaum Associates Inc.: Mahwah, NJ, USA, 1988; ISBN 9780805802832. [Google Scholar]
- Fisher, R.A. On the Mathematical Foundations of Theoretical Statistics. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 1922, 222, 309–368. [Google Scholar]
- Oftadeh, S.; Bahram, A.; Yaali, R.; Ghadiri, F.; Schöllhorn, W.I. External Focus or Differential Learning: Is There an Additive Effect on Learning a Futsal Goal Kick? Int. J. Environ. Res. Public Health 2021, 19, 317. [Google Scholar] [CrossRef]
- Beckmann, H.; Schöllhorn, W. Differential learning in shot put. In European Workshop on Movement Sciences; Schöllhorn, W.I., Bohn, C., Jäger, J.M., Schaper, H., Alichmann, M., Eds.; Sport & Buch Strauß: Cologne, Germany, 2003; p. 68. [Google Scholar]
- Touchette, P.; Howard, J. Errorless learning contingencies and stimululs control transfer in delayed prompting. J. Appl. Behav. Anal. 1984, 17, 175–188. [Google Scholar] [CrossRef]
- Metcalfe, J. Learning from Errors. Annu. Rev. Psychol. 2017, 68, 465–489. [Google Scholar] [CrossRef]
- Hodges, N.J.; Lhose, K.R. Difficulty is a real challenge: A Perspective on the Role of Cognitive Effort in Motor Skill Learning. J. Appl. Res. Mem. Cogn. 2020, 9, 455–460. [Google Scholar] [CrossRef]
- Schöllhorn, W.I. Process-oriented analysis of movement patterns. In Proceedings of the International Society of Biomechanics XIVth Congress, Paris, France, 4–8 July 1993; pp. 1212–1213. [Google Scholar]
- Schöllhorn, W.I. Time course-oriented analysis of biomechanical movement patterns by means of orthogonal reference functions. In Proceedings of the XVth Congress of the International Society of Biomechanics (ISB), Jyväskylä, Finland, 2–6 July 1995; pp. 2–6. [Google Scholar]
- Schöllhorn, W.I.; Bauer, H.U. Identifying individual movement styles in high performance sports by means of self-organizing Kohonen maps. In Proceedings of the ISBS-Conference Proceedings Archive, Konstanz, Germany, 21–25 July 1998. [Google Scholar]
- Schöllhorn, W.I.; Nigg, B.M.; Stefanyshyn, D.J.; Liu, W. Identification of individual walking patterns using time discrete and time-continuous data sets. Gait Posture 2002, 15, 180–186. [Google Scholar] [CrossRef]
- Horst, F.; Janssen, D.; Beckmann, H.; Schöllhorn, W.I. Can individual movement characteristics across different throwing disciplines be identified in high-performance decathletes? Front. Psychol. 2020, 11, 2262. [Google Scholar] [CrossRef] [PubMed]
- Jäger, J.M.; Schöllhorn, W.I. Situation-orientated recognition of tactical patterns in volleyball. J. Sports Sci. 2007, 25, 1345–1353. [Google Scholar] [CrossRef]
- Jäger, J.M.; Schöllhorn, W.I. Identifying individuality and variability in team tactics by means of statistical shape analysis and multilayer perceptrons. Hum. Mov. Sci. 2012, 31, 303–317. [Google Scholar] [CrossRef]
- Schöllhorn, W.I.; Jäger, J.M.; Janssen, D. Artificial neural network models of sports motions. In Routledge Handbook of Biomechanics and Human Movement Science; Routledge: Abingdon, UK, 2008; pp. 50–64. [Google Scholar]
- Kunz, H. Leistungsbestimmende Faktoren im Zehnkampf—Eine Längsschnittstudie an Schweizer Spitzenathleten. Ph.D. Thesis, Universität Heidelberg: Heidelberg, Germany, 1980. [Google Scholar]
- Janssen, D.; Gebkenjans, F.; Beckmann, H.; Schöllhorn, W.I. Analyzing learning approaches by means of complex movement pattern analysis. Int. J. Sport Psychol. 2010, 41, 18. [Google Scholar]
- Rendell, M.A.; Farrow, D.; Masters, R.; Plummer, N. Implicit practice for skill adaptation in expert performers. Int. J. Sports Sci. Coach. 2011, 6, 553–566. [Google Scholar] [CrossRef]
- Orgo, L.; Bachmann, M.; Lass, J.; Hinrikus, H. Effect of negative and positive emotions on EEG spectral asymmetry. In Proceedings of the 2015, 37th Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Milan, Italy, 25–29 August 2015; pp. 8107–8110. [Google Scholar]
- Dietrich, A. Transient hypo-frontality as a mechanism for the psychological effects of exercise. Psychiatry Res. 2006, 145, 79–83. [Google Scholar] [CrossRef]
- John, Y.J.; Sawyer, K.S.; Srinivasan, K.; Müller, E.J.; Munn, B.R.; Shine, J.M. It’s about time: Linking dynamical systems with human neuroimaging to understand the brain. Netw. Neurosci. 2022, 1–54. [Google Scholar] [CrossRef]
- Henz, D.; Schöllhorn, W.I. EEG brain activity in dynamic health qigong training: Same effects for mental practice and physical training? Front. Psychol. 2017, 8, 154. [Google Scholar] [CrossRef][Green Version]
- Ricci, S.; Tatti, E.; Nelson, A.B.; Panday, P.; Chen, H.; Tononi, G.; Cirelli, C.; Ghilardi, M.F. Extended visual sequence learning leaves a local trace in the spontaneous EEG. Front. Neurosci. 2021, 15, 707828. [Google Scholar] [CrossRef]
- Wiener, N. Cybernetics or Control and Communication in the Animal and the Machine; The Technology Press: Cambridge, MA, USA, 1948. [Google Scholar]
- Wiener, N. The Human Use of Human Beings: Cybernetics and Society; Houghton Mifflin Company: Boston, MA, USA, 1950. [Google Scholar]
- Shannon, C.E. Claude Elwood Shannon. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Pask, G.; Scott, B.C.E. Learning strategies and individual competence. Int. J. Man. Mach. Stud. 1972, 4, 217–253. [Google Scholar] [CrossRef]
- Cube, F.V. Kybernetische Grundlagen des Lernens und Lehrens; Huber: Stuttgart, Germany, 1965. [Google Scholar]
- Schöllhorn, W.I. Coordination dynamics and its consequences on sports. Int. J. Comput. Sci. Sport 2003, 2, 40–46. [Google Scholar]
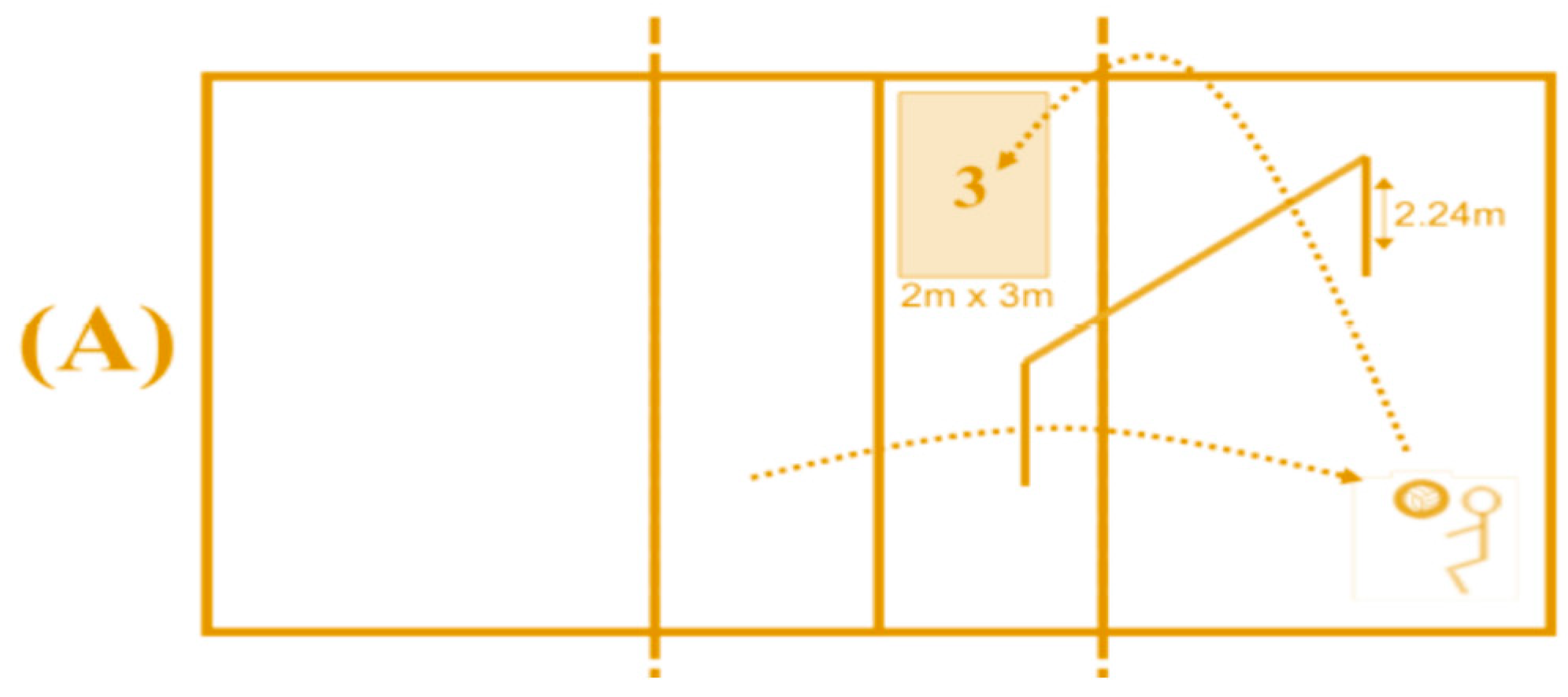
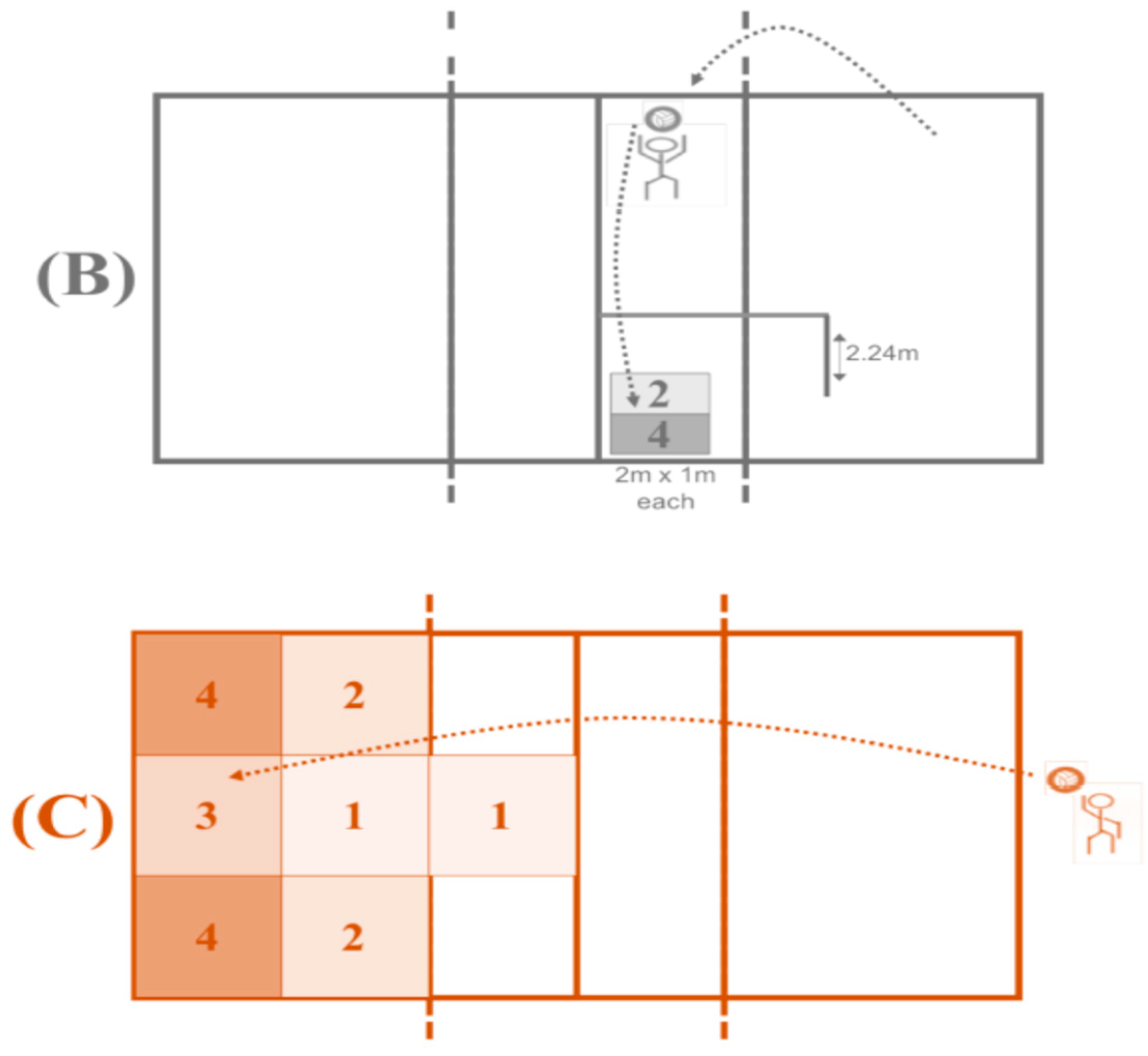
| Authors. | Number of Skills to Be Stabilized | Groups | Average Age [Years] | Number of Subjects | Overall Trials | Number of Sessions | Over All Duration [Days] | Post Test [Yes/No] | Retention Test [Hours after Post Test] | Transfer Test [Yes/No] | Post Test: Blocked > Rndm [Yes/No] | Ret./Transf Test: Rndm > Blocked [Yes/No] |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Bortolli et al., 1992 [15] | Three/underhand serve/underarm pass/overhand pass | Blocked/random/serial/hi-ser | 14.6 | 53 | 576 | 8 | 60 | n | 1 | y | n | non-systematic |
| Fialho et al., 2006 [16] | Three serves (tennis, float, Asian) | Blocked/random | 16.3 | 15 | 184 | 4 | 5 | y | 24 | y | n | y |
| French et al., 1990 [17] | Three/overhead serve/overhand pass/overhand pass | Blocked/random/random-blocked | <14 | 139 | 270 | 9 | 11 | y | 48 | y | n | n |
| Jones et al., 2007 [18] | Three/underhand serve/underarm pass/overhand pass | Blocked/random/random-blocked | 14 | 51 | 270 | 9 | 9 | y | 48 | n | n | n |
| Kalkhoran et al., 2015 [19] | Three/overhead serve/underarm pass/overhand pass | Blocked/random/serial | 21.5 | 60 | 378 | 9 | 9 | y | 48/72 | y | y | y |
| Meira et al., 2003 [20] | Three serves (underhand, overhead, Asian) | Blocked/random | 12.7 | 36 | 288 | 11 | 11 | n | n | y | n | n |
| Pasand et al., 2016 [21] | Three/underhand serve/underarm pass/overhand pass | Blocked/random/increasing random | 22.5 | 45 | 1215 | 9 | 21 | y | 48 | y | y | y |
| Travlos 2010 [22] | One serve (underhand–5 directions) | Blocked/random/serial/constant/specific | 14.1 | 72 | 120 | 3 | 21 | n | 72 | y | n | y |
| Zetou et al., 2007 [23] | Three/?? serve/underarm pass/overhand pass | Blocked/random | 12.4 | 26 | 270 | 10 | 70 | y | 336 | n | n | n |
| Group | Age [Years] | Weight [kg] | Height [m] | BMI [kg/m2] | Experience [Years] |
|---|---|---|---|---|---|
| CI | 25.08 (1.62) | 68.25 (4.37) | 1.68 (0.06) | 24.65 (1.77) | 7.58 (2.06) |
| DL | 25.83 (2.62) | 71.52 (6.78) | 1.69 (0.07) | 25.15 (2.20) | 8.25 (1.76) |
| CO | 23.50 (2.81) | 69.08 (3.87) | 1.66 (0.07) | 25.28 (2.17) | 6.83 (2.12) |
| All | 24.81 (2.54) | 70.08 (5.18) | 1.68 (0.07) | 25.03 (2.01) | 7.56 (2.02) |
| Comparison | Friedman Test and Kruskal–Wallis Test | Post-Hoc Dunn–Bonferroni Tests |
|---|---|---|
| OVERHAND PASS | ||
| CO | p = 0.004 ** | Pre vs. Post: p = 0.005 **, r = 0.913 +++ |
| Pre-Post-Ret | (Pre: 19.5000, Post: 26.4167, Ret: 23.8333 ) | |
| DL | p = 0.001 ** | Pre vs. Post: p = 0.018 *, r = 0.795 +++ |
| Pre-Post-Ret | (Pre: 20.3333, Post: 28.0000, Ret: 29.5833) | Pre vs. Ret: p = 0.002 **, r = 0.972 +++ |
| CI | p = 0.020 * | Post vs. Ret: p = 0.024 *, r = 0.766 +++ |
| Pre-Post-Ret | (Pre: 21.3333 Post: 18.0833, Ret: 24.4167) | |
| Pre | 𝜒2 (2) = 0.286, p = 0.867 | |
| CO-DL-CI | (CO: 17.38 DL: 18.46, CI: 19.67) | |
| Post | p = 0.013 * | CI vs. DL: p = 0.016 *, r = 0.571 ++ |
| CO-DL-CI | (CO: 20.83, DL: 23.33, CI: 11.33) | |
| Ret | p = 0.164 | |
| CO-DL-CI | (CO: 15.63: DL: 23.17, CI: 16.71) | |
| OVERHAND SERVE | ||
| CO | p = 0.049 * | Post vs. Ret: p = 0.057 *, r = 0.677 ++ |
| Pre-Post-Ret | (Pre:15.9167, Post: 18.2500, Ret: 13.7500) | |
| DL | p = 0.013 * | Pre vs. Post: p = 0.052 *, r = 0.766 +++ |
| Pre-Post-Ret | (Pre: 15.0833, Post: 20.7500, Ret: 20.3333) | Pre vs. Ret: p = 0.008 **, r = 0.559 ++ |
| CI | p = 0.015 * | Pre vs. Ret: p = 0.014 *, r = 0.707 +++ |
| Pre-Post-Ret | (Pre: 14.6667, Post: 11.6667, Ret: 16.9167) | Post vs. Ret: p = 0.014 *, r = 0.707 +++ |
| Pre | p = 0.926 | |
| CO-DL-CI | (CO: 19.46, DL: 18.17, CI: 17.88) | |
| Post | p = 0.028 * | CI vs. DL: p = 0.023 *, r = 0.543 ++ |
| CO-DL-CI | (CO: 19.00, DL: 23.96, CI: 12.54) | |
| Ret | p = 0.114 | CO vs. DL: p = 0.023 *, r = 0.235 + |
| CO-DL-CI | (CO: 14.58, DL: 23.38, CI: 17.54) | |
| UNDERHAND PASS | ||
| CO | p = 0.843 | |
| Pre-Post-Ret | (Pre: 15.7500 Post: 17.9444, Ret: 18.6667) | |
| DL | p = 0.005 ** | Pre vs. Post: p = 0.003 **, r = 0.854 +++ |
| Pre-Post-Ret | (Pre: 14.7500 Post: 23.6667, Ret: 23.3333) | Pre vs. Ret: p = 0.011 *, r = 0.736 +++ |
| CI | p = 0.933 | |
| Pre-Post-Ret | (Pre: 16.6667, Post: 14.5000, Ret: 14.8333) | |
| Pre | p = 0.768 | |
| CO-DL-CI | (CO: 18.75, DL: 16.83, CI: 19.92) | |
| Post | p = 0.041 * | CI vs. DL: p = 0.021 *, r = 0.470 + |
| CO-DL-CI | (CO: 15.96, DL: 24.71, CI: 14.83) | DL vs. CO: p = 0.041 *, r = 0.417 + |
| Ret | p = 0.009 ** | CI vs. DL: p = 0.003 **, r = 0.601 ++ |
| CO-DL-CI | (CO: 16.46, DL: 25.83, CI: 13.21) | CO vs. DL: p = 0.029 *, r = 0.446 + |
| COMBINED | ||
| CO Pre-post ret | p = 0.779 | |
| (PRE: 1.83, POST: 2.08, RET: 2.08) | ||
| DL Pre-post-ret | p = 0.000 *** | Pre vs. post: p = 0.000 ***, r = 1.178 +++ |
| (PRE: 1.00, POST: 2.67, RET: 2.33) | Pre vs. ret: p = 0.001 **, r = 0.942 +++ | |
| CI Pre-post-ret | p = 0.076 * (PRE: 1.92, POST: 1.58 RET: 2.50) | |
| Pre CO-DL-CI | χ2 (2) = 0.047, p = 0.977 (CO: 18.58, DL: 18.00, CI: 18.92) | |
| Post CO-DL-CI | χ2 (2) = 9.664, p = 0.008 ** (CO: 16.33; DL: 26.00 CI: 13.17) | CI vs. DL: p = 0.009 **, r = 0.609 ++ |
| Ret CO-DL-CI | χ2 (2) = 10.392, p = 0.006 ** (CO:14.25; DL: 26.50, CI: 14.75) | CO vs. DL: p = 0.013 *, r = 0.581 ++ CI vs. DL: p = 0.019 *, r = 0.557 ++ |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Apidogo, J.B.; Burdack, J.; Schöllhorn, W.I. Learning Multiple Movements in Parallel—Accurately and in Random Order, or Each with Added Noise? Int. J. Environ. Res. Public Health 2022, 19, 10960. https://doi.org/10.3390/ijerph191710960
Apidogo JB, Burdack J, Schöllhorn WI. Learning Multiple Movements in Parallel—Accurately and in Random Order, or Each with Added Noise? International Journal of Environmental Research and Public Health. 2022; 19(17):10960. https://doi.org/10.3390/ijerph191710960
Chicago/Turabian StyleApidogo, Julius B., Johannes Burdack, and Wolfgang I. Schöllhorn. 2022. "Learning Multiple Movements in Parallel—Accurately and in Random Order, or Each with Added Noise?" International Journal of Environmental Research and Public Health 19, no. 17: 10960. https://doi.org/10.3390/ijerph191710960
APA StyleApidogo, J. B., Burdack, J., & Schöllhorn, W. I. (2022). Learning Multiple Movements in Parallel—Accurately and in Random Order, or Each with Added Noise? International Journal of Environmental Research and Public Health, 19(17), 10960. https://doi.org/10.3390/ijerph191710960








