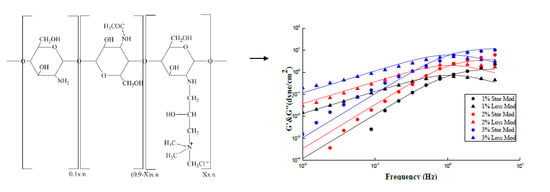
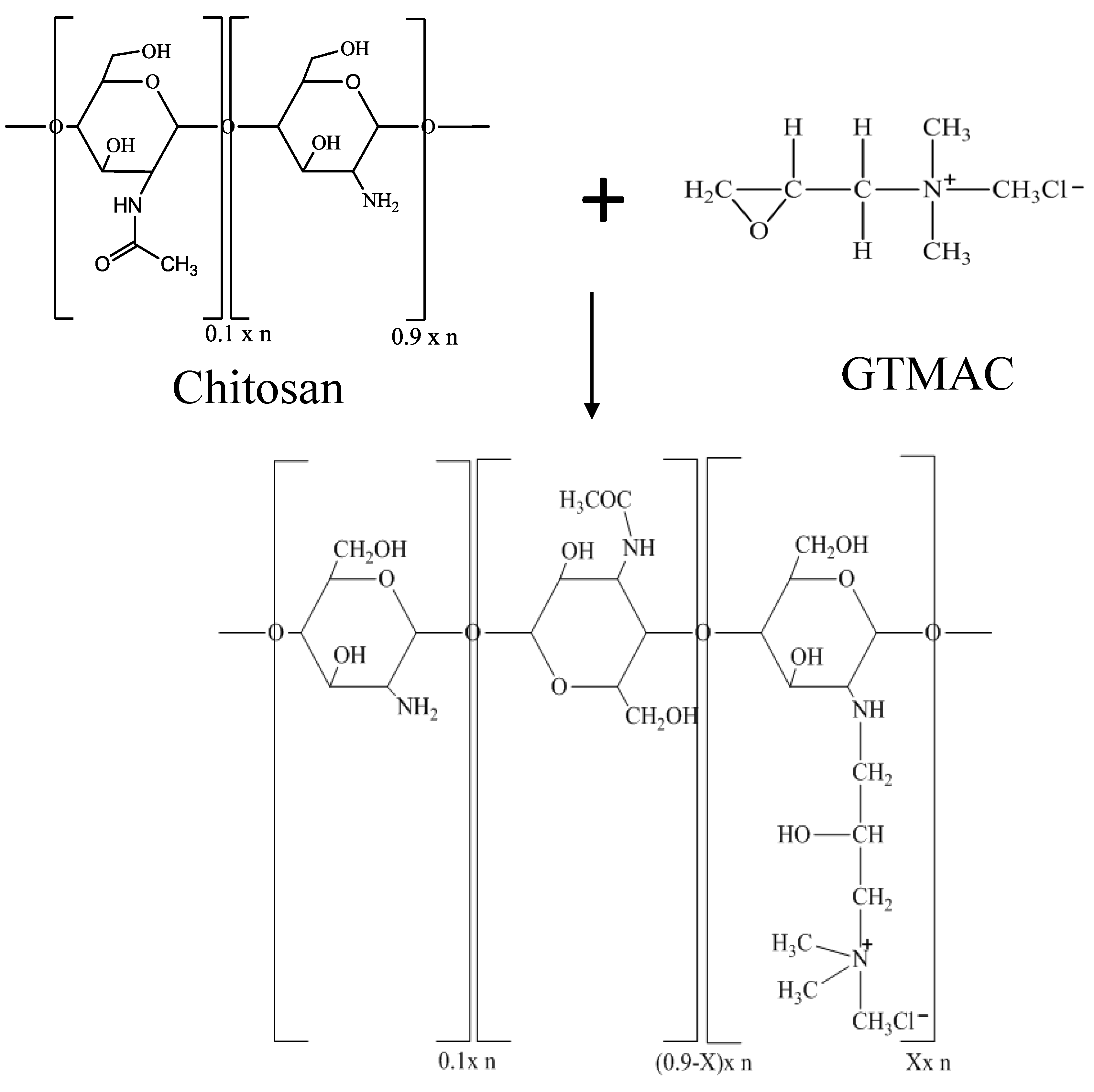
Synthesis and Rheological Characterization of Water-Soluble Glycidyltrimethylammonium-Chitosan
Abstract
:1. Introduction
2. Experimental Method
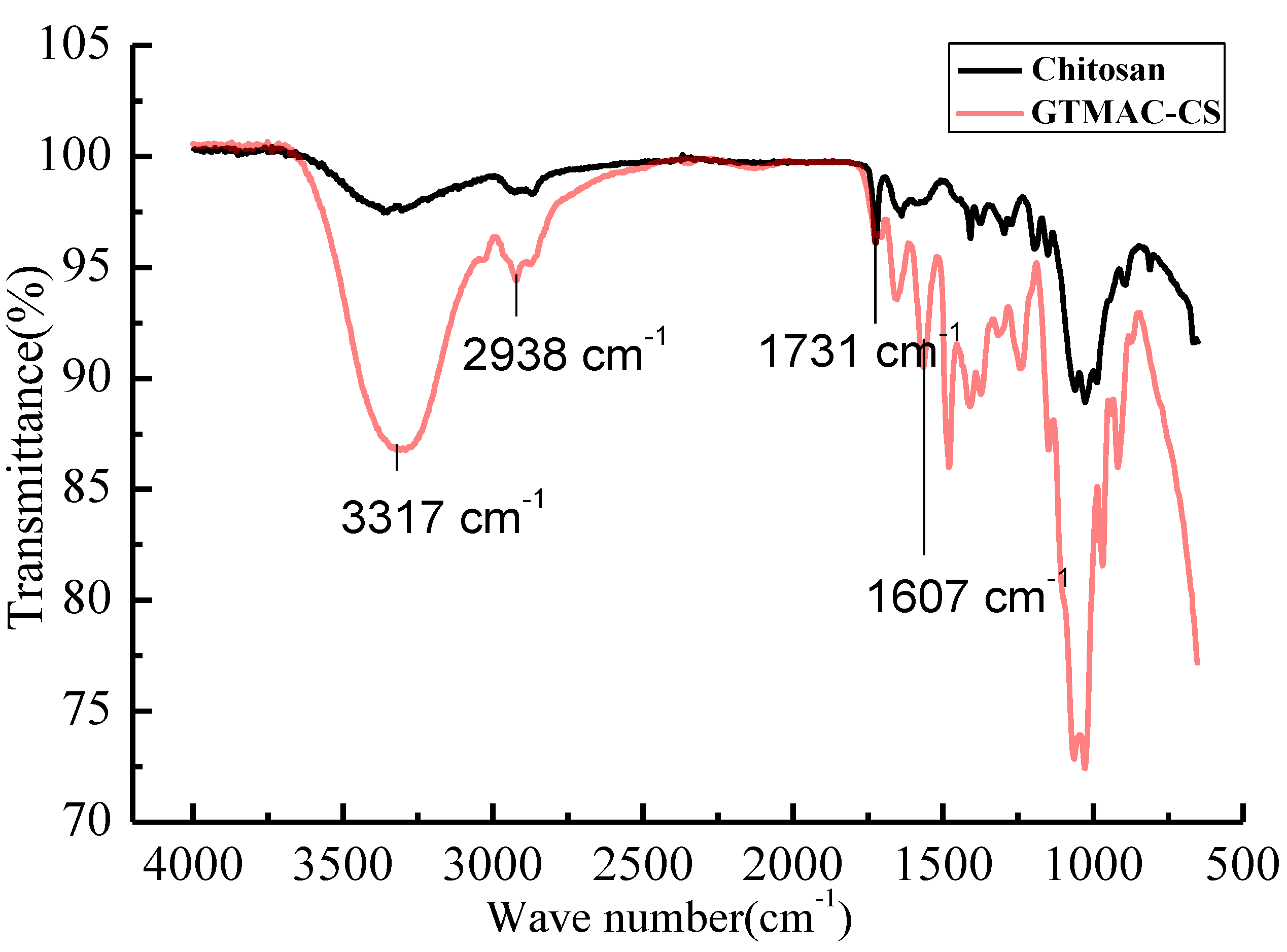
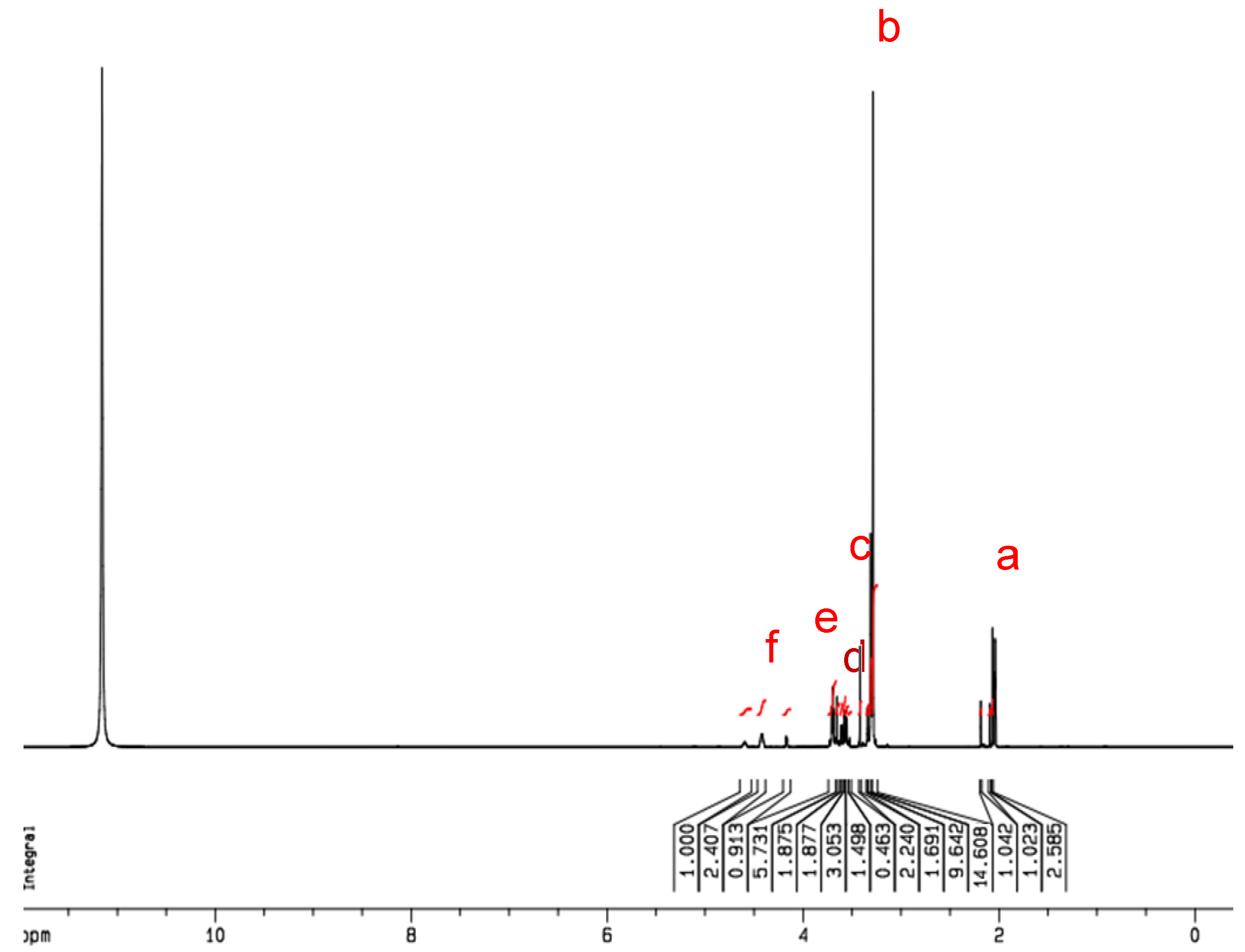
3. Results and Discussion

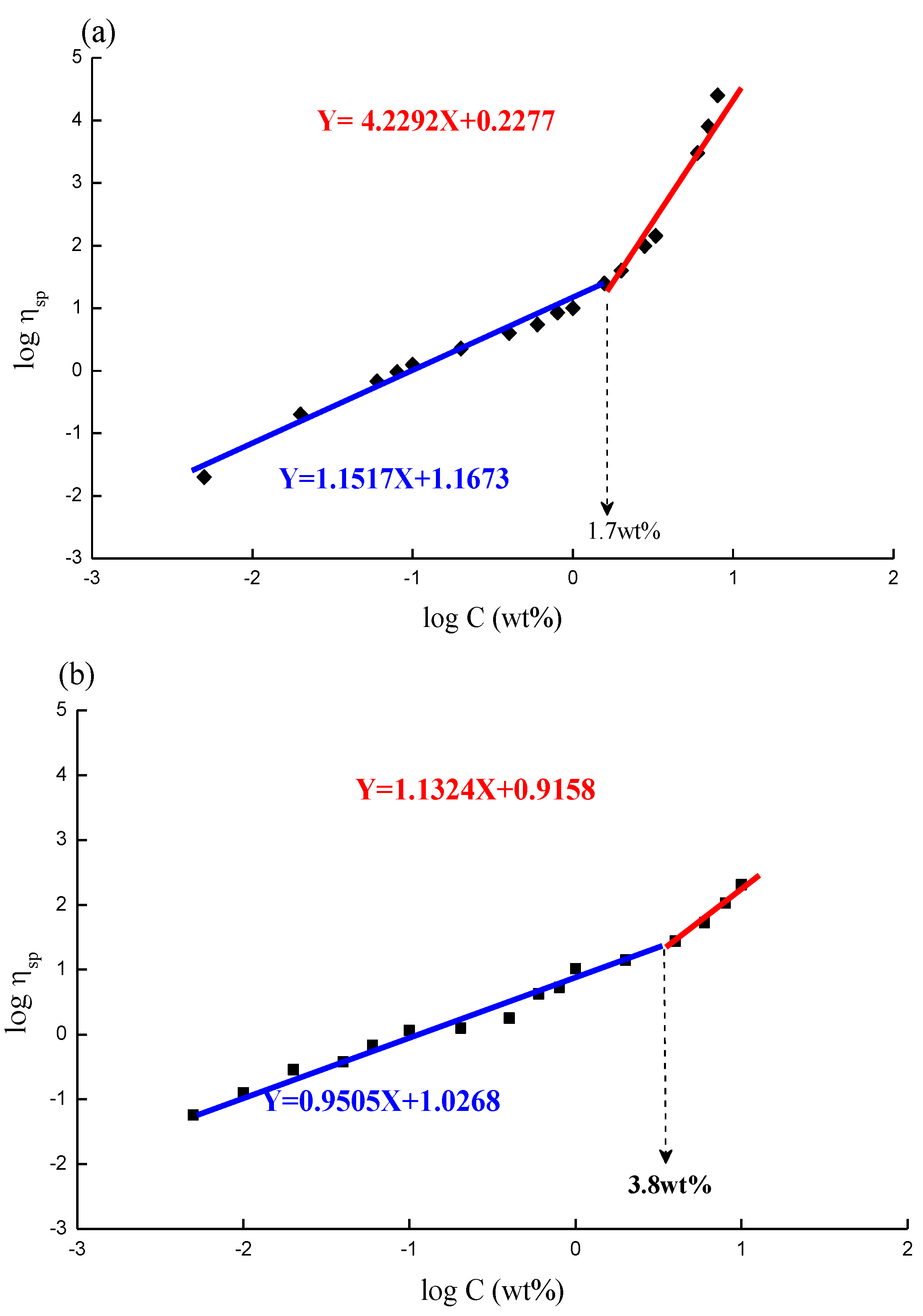
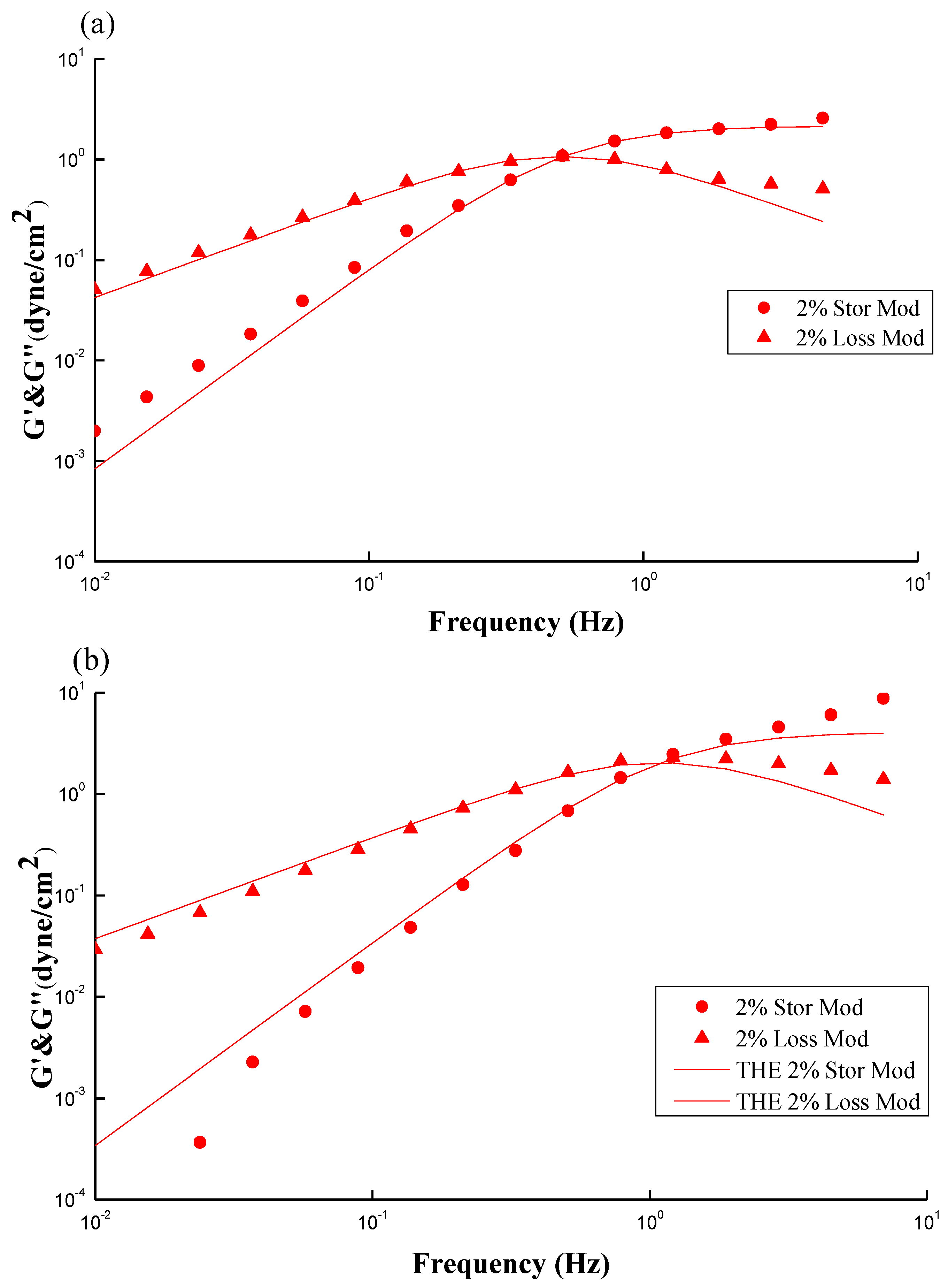

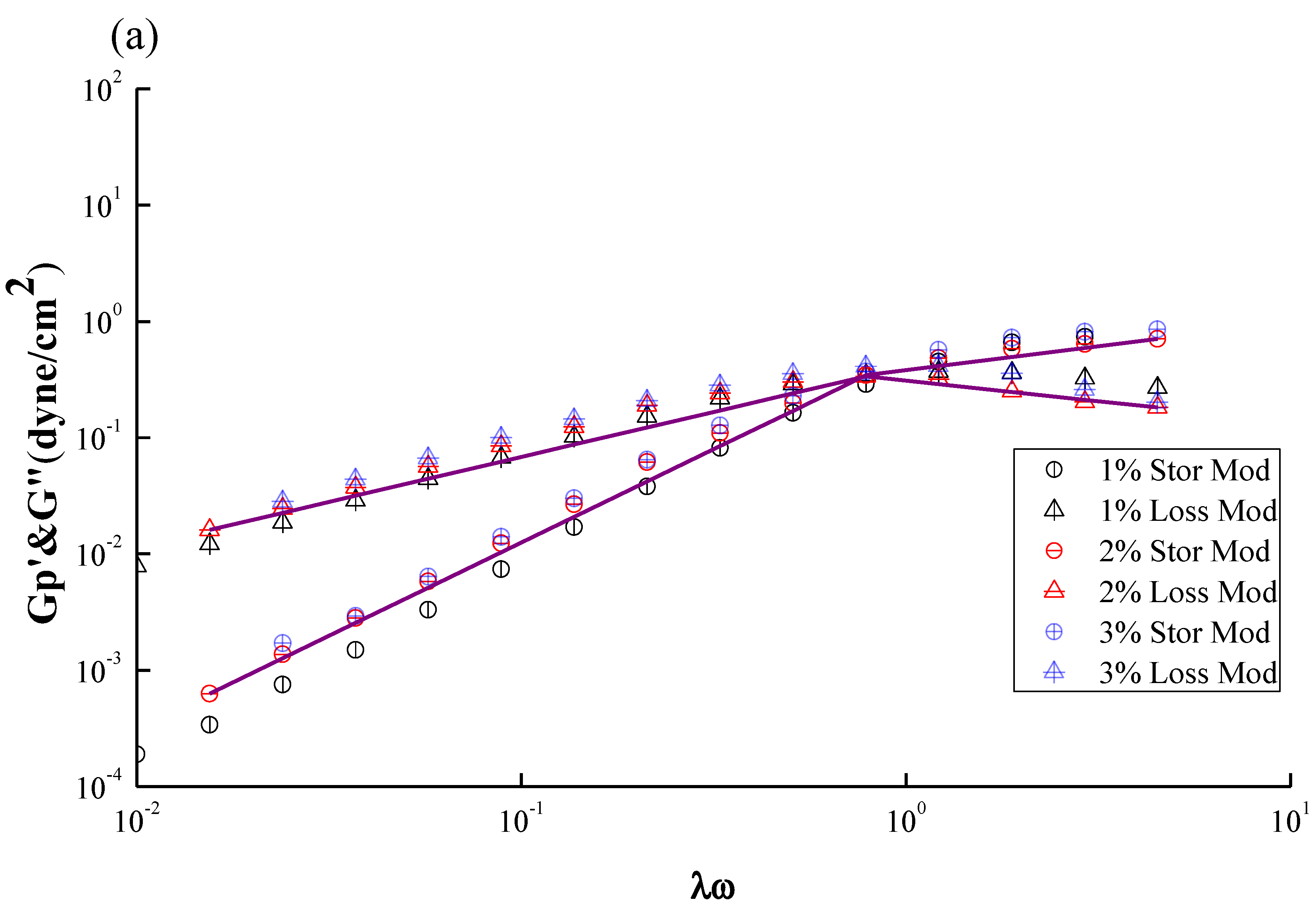
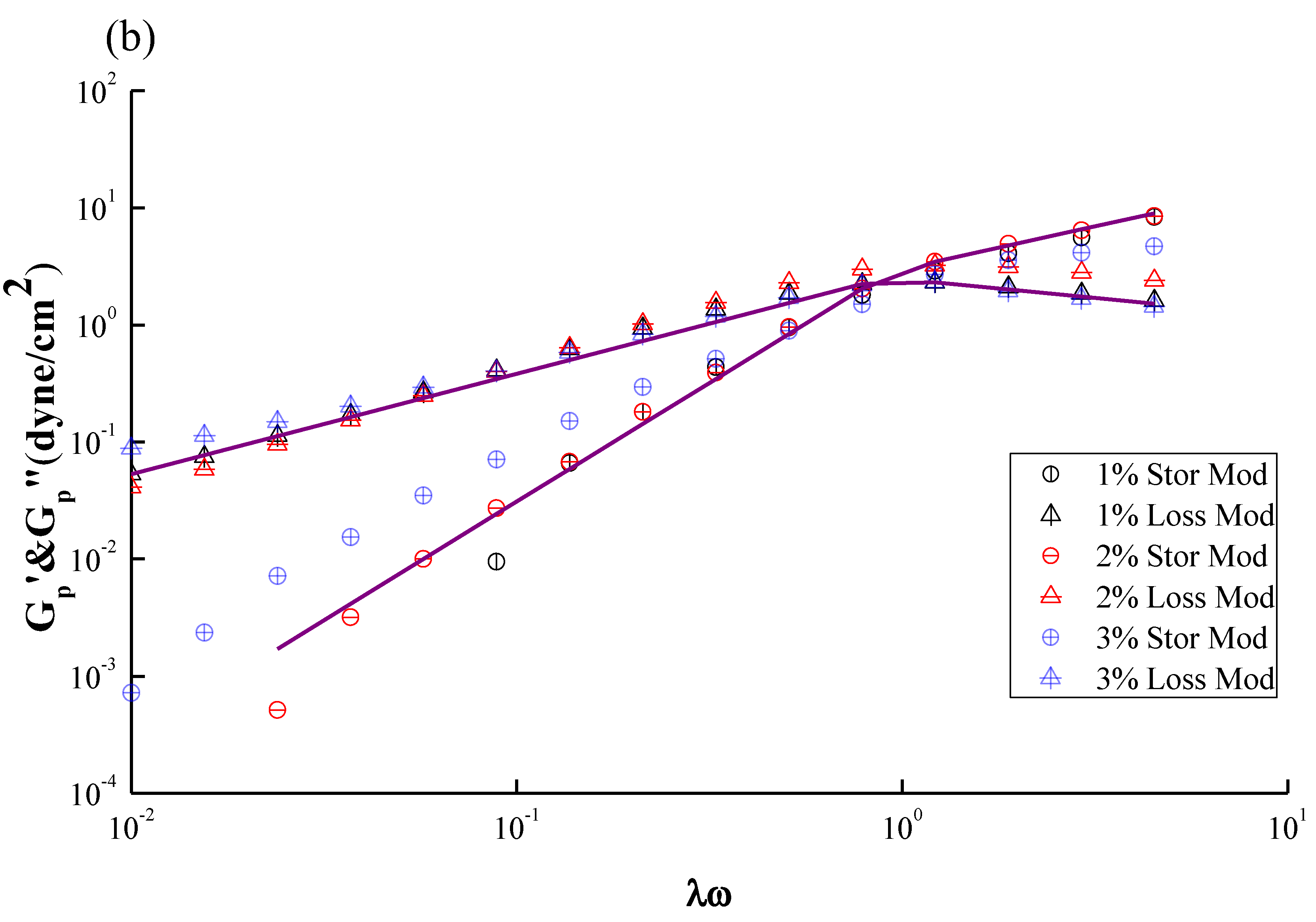
| Concentration | ωc (Hz) | λ(s) | G∞(dyne/cm2) | Dev.G′ # | Dev.Gʺ # | |
|---|---|---|---|---|---|---|
| CS | 1 wt% | 2.43 | 0.41 | 0.31 | 0.20 | 0.02 |
| 2 wt% | 0.51 | 1.96 | 2.20 | 0.06 | 0.03 | |
| 3 wt% | 0.33 | 3.03 | 4.52 | 0.04 | 0.02 | |
| GTMAC-CS | 1 wt% | 1.02 | 0.98 | 0.66 | 0.02 | 0.09 |
| 2 wt% | 1.09 | 0.91 | 2.45 | 0.04 | 0.00 | |
| 3 wt% | 1.08 | 0.92 | 6.35 | 0.10 | 0.19 |
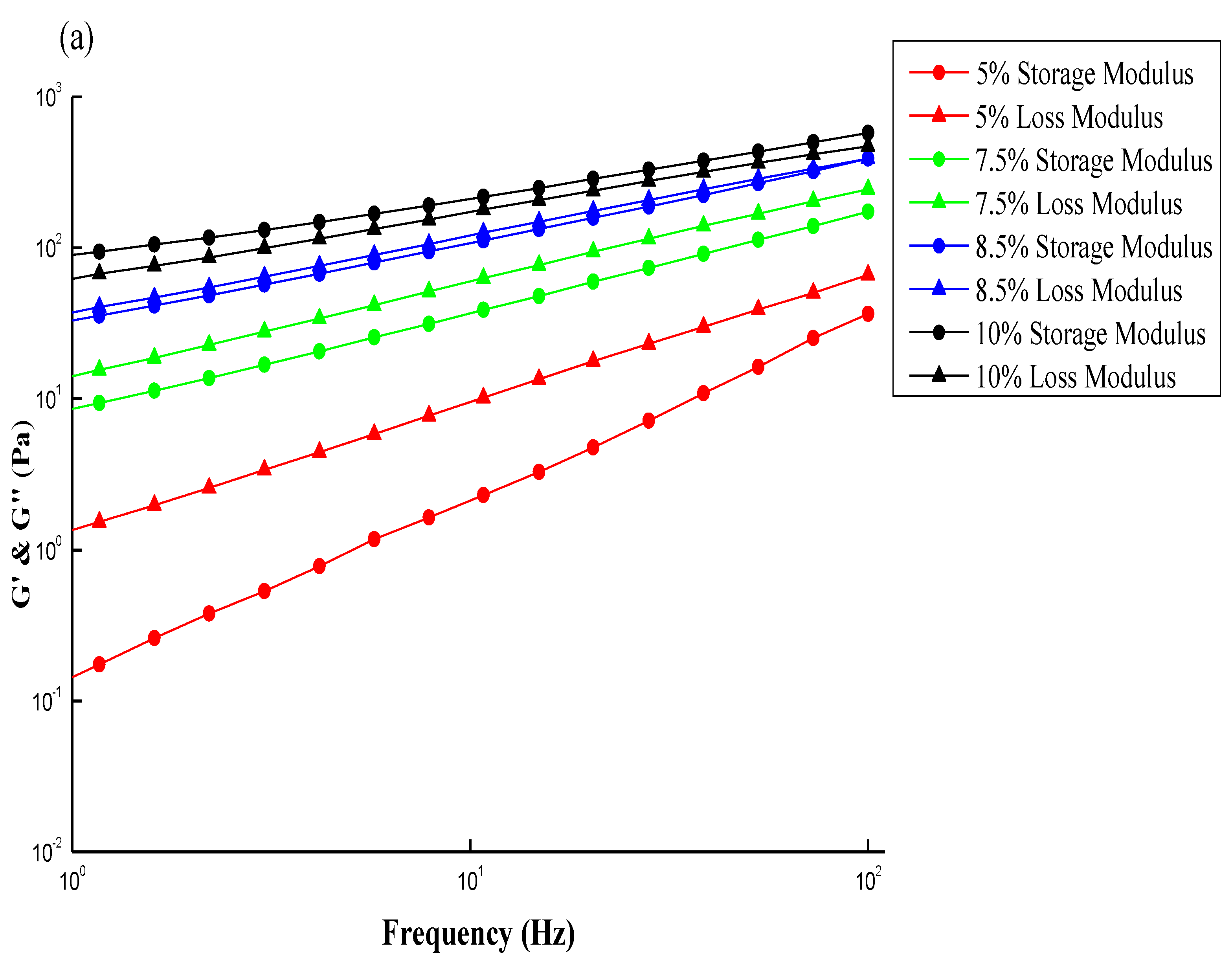

4. Conclusions
Acknowledgment
Author Contributions
Conflicts of Interest
References
- Ehrlich, H. Chitin and collagen as universal and alternative templates in biomineralization. Int. Geol. Rev. 2010, 52, 661–699. [Google Scholar] [CrossRef]
- Lee, S.Y.; Kim, J.H.; Kuon, I.C.; Jeong, S.Y. Self-aggregates of deoxycholic acid-modified chitosan as a novel carrier of adriamycin. Colloid Polym. Sci. 2000, 278, 1216–1219. [Google Scholar] [CrossRef]
- Ávila, A.; Bierbrauer, K.; Pucci, G.; López-González, M.; Strumia, M. Study of optimization of the synthesis and properties of biocomposite films based on grafted chitosan. J. Food Eng. 2012, 109, 752–761. [Google Scholar] [CrossRef]
- Bhattarai, N.; Gunn, J.; Zhang, M. Chitosan-based hydrogels for controlled, localized drug delivery. Adv. Drug Deliv. Rev. 2010, 62, 83–99. [Google Scholar] [CrossRef]
- Casettari, L.; Vllasaliu, D.; Lam, J.K.W.; Soliman, M.; Illum, L. Biomedical applications of amino acid-modified chitosans: A review. Biomaterials 2012, 33, 7565–7583. [Google Scholar] [CrossRef]
- Cho, J.; Heuzey, M.C.; Bégin, A.; Carreau, P.J. Viscoelastic properties of chitosan solutions: Effect of concentration and ionic strength. J. Food Eng. 2006, 74, 500–515. [Google Scholar] [CrossRef]
- Charles, W.P.; Jonathan, J.W.; Gudrun, S. A review on tough and sticky hydrogels. Colloid Polym. Sci. 2013, 291, 2031–2047. [Google Scholar] [CrossRef]
- Hamdine, M.; Heuzey, M.C.; Bégin, A. Viscoelastic properties of phosphoric and oxalic acid-based chitosan hydrogels. Rheol. Acta 2006, 45, 659–675. [Google Scholar] [CrossRef]
- Seetapan, N.; Maingam, K.; Plucktaveesak, N.; Sirivat, A. Linear viscoelasticity of thermoassociative chitosan-g-poly(N-isopropylacrylamide) copolymer. Rheol. Acta 2006, 45, 1011–1018. [Google Scholar] [CrossRef]
- Rwei, S.P.; Lien, C.C. Synthesis and Rheological Characterization of Sulfonated Chitosan Solutions. Colloid Polym. Sci. 2014, 292, 785–795. [Google Scholar] [CrossRef]
- Hermann, M.B.; Klaus, G. 1,3-Propane sultone, an extremely potent experimental carcinogen: What should be expected in humans. Toxicol. Lett. 2004, 151, 251–254. [Google Scholar] [CrossRef] [PubMed]
- Bendoraitiene, J.; Kavaliauskaite, R.; Klimaviciute, R.; Zemaitaitis, A. Peculiarities of Starch Cationization with Glycidyltrimethylammonium Chloride. Starch/Starke 2006, 58, 623–631. [Google Scholar] [CrossRef]
- Pedone, E.; Cavallaro, G.; Richardson, S.C.W.; Duncan, R.; Giammona, G. α,β-poly (asparthylhydrazide)–glycidyltrimethylammonium chloride copolymers (PAHy–GTA): Novel polymers with potential for DNA delivery. J. Controll. Release 2001, 77, 139–153. [Google Scholar] [CrossRef]
- Xiao, B.; Wan, Y.; Wang, X.Y.; Zha, Q.C.; Liu, H.M.; Qiu, Z.Y.; Zhang, S.M. Synthesis and characterization of N-(2-hydroxy)propyl-3-trimethyl ammonium chitosan chloride for potential application in gene delivery. Colloids Surf. B 2012, 91, 168–174. [Google Scholar] [CrossRef]
- Lim, S.H.; Hudson, S.M. Synthesis and antimicrobial activity of a watersoluble chitosan derivative with a fiber-reactive group. Carbohydr. Res. 2004, 339, 313–319. [Google Scholar] [CrossRef] [PubMed]
- Zhao, S.H.; Wu, X.-T.; Guo, W.-C.; Du, Y.-M.; Yu, L.; Tang, J. N-(2-hydroxyl) propyl-3-trimethyl ammonium chitosan chloride nanoparticle as a novel delivery system for Parathyroid Hormone-Related Protein 1–34. Int. J. Pharm. 2010, 393, 268–272. [Google Scholar] [CrossRef] [PubMed]
- Xu, Y.M.; Du, Y.M.; Huang, R.H.; Gao, L.P. Preparation and modification of N-(2-hydroxyl) propyl-3-trimethyl ammonium chitosan chloride nanoparticle as a protein carrier. Biomaterials 2003, 24, 5015–5022. [Google Scholar] [CrossRef] [PubMed]
- Sonia, T.A.; Sharma, C.P. In vitro evaluation of N-(2-hydroxy)propyl-3-trimethyl ammonium chitosan for oral insulin delivery. Carbohydr. Polym. 2011, 84, 103–109. [Google Scholar] [CrossRef]
- Cheng, Y.; Cai, H.; Yin, B.; Yao, P. Cholic acid modified N-(2-hydroxy)-propyl-3-trimethylammonium chitosan chloride for superoxide dismutase delivery. Int. J. Pharm. 2013, 454, 425–434. [Google Scholar]
- Van Vlierberghe, S.; Dubruel, P.; Schacht, E. Biopolymer-Based Hydrogels As Scaffolds for Tissue Engineering Applications: A Review. Biomacromolecules 2011, 12, 1387–1408. [Google Scholar] [CrossRef] [PubMed]
- Rwei, S.P.; Chen, S.W.; Mao, C.F.; Fang, H.W. Viscoelasticity and wearability of hyaluronate solutions. Biochem. Eng. J. 2008, 40, 211–217. [Google Scholar] [CrossRef]
- Rwei, S.P.; Lee, H.Y.; Yoo, S.D.; Wang, L.Y.; Lin, J.G. Magnetorheological characteristics of aqueous suspensions that contain Fe3O4 nanoparticles. Colloid Polym. Sci. 2005, 283, 1253–1258. [Google Scholar] [CrossRef]
- Morris, E.R.; Cutler, A.N.; Ross-Murphy, S.B.; Rees, D.A.; Price, J. Concentration and shear rate dependence of viscosity in random coil polysaccharide solutions. Carbohydr. Polym. 1981, 1, 5–21. [Google Scholar] [CrossRef]
- Pereira, M.C.; Wyn-Jones, E.; Morris, E.R.; Ross-Murphy, S.B. Characterisation of interchain association in polysaccharide solutions by ultrasonic relaxation and velocity. Carbohydr. Polym. 1982, 2, 103–113. [Google Scholar] [CrossRef]
- Seale, R.; Morris, E.R.; Rees, D.A. Interactions of alginates with univalent cations. Carbohydr. Res. 1982, 110, 101–112. [Google Scholar] [CrossRef]
- Calero, N; Muñoz, J.; Ramírez, P.; Guerrero, A. Flow behaviour, linear viscoelasticity and surface properties of chitosan aqueous solutions. Food Hydrocoll. 2010, 24, 659–666. [Google Scholar]
- Annable, T.; Buscall, R.; Ettelaie, R. Network formation and its consequences for the physical behaviour of associating polymers in solution. Colloids Surf. A 1996, 112, 97–116. [Google Scholar] [CrossRef]
- Pottier, N. Relaxation time distributions for an anomalously diffusing particle. Phys. A 2011, 390, 2863–2879. [Google Scholar] [CrossRef]
- Baig, C.; Mavrantzas, V.G.; Öttinger, H.C. On Maxwell’s relations of thermodynamics for polymeric liquids away from equilibrium. Macromolecules 2011, 44, 640–646. [Google Scholar] [CrossRef]
- Molchanov, V.S.; Philippova, O.E. Effects of concentration and temperature on viscoelastic properties of aqueous potassium oleate solutions. Colloid J. 2009, 71, 239–245. [Google Scholar] [CrossRef]
- Papoulia, K.D.; Panoskaltsis, V.P.; Kurup, N.V.; Korovajchuk, I. Rheological representation of fractional order viscoelastic material models. Rheol. Acta 2010, 49, 381–400. [Google Scholar] [CrossRef]
- Van de Manakker, F.; Vermonden, T.; Morabit, N.; van Nostrum, C.F.; Hennink, W.E. Rheological Behavior of Self-Assembling PEG-β-Cyclodextrin/PEG-Cholesterol Hydrogels. Langmuir 2008, 24, 12559–12567. [Google Scholar] [CrossRef] [PubMed]
- Steffe, J.F. Rheological Methods in Food Process Engineering, 2nd ed.; Freeman Press: East Lansing, MI, USA, 1996; Chapter 5. [Google Scholar]
- Likhtman, A.E.; McLeish, T.C.B. Quantitative theory for linear dynamics of linear entangled polymers. Macromolecules 2002, 35, 6332–6343. [Google Scholar] [CrossRef]
- Likthman, A.E.; Marques, G.M. First-passage problem for the Rouse polymer chain: An exact solution. Europhys. Lett. 2006, 75, 971–977. [Google Scholar] [CrossRef]
- Larson, R.G. Constitutive Equations for Polymer Melts and Solutions, 1st ed.; Butterworths: Boston, MA, USA, 1988; Chapter 5. [Google Scholar]
- Goodwin, J.W.; Hughes, R.W.; Lam, C.K.; Miles, J.A.; Warren, B.C.H. The rheological properties of a hydrophobically modified cellulose. In Polymers in Aqueous Media: Performance through Association. Advances in Chemistry; Glass, J.E., Ed.; American Chemical Society: Washington, DC, USA, 1989; Volume 223, pp. 365–378. [Google Scholar]
- Vermonden, T.; van Steenbergen, M.J.; Besseling, N.A.M.; Marcelis, A.T.M.; Hennink, W.E.; Sudhölter, E.J.R.; Cohen Stuart, M.A. Linear rheology of water-soluble reversible neodymium(III) coordination polymers. J. Am. Chem. Soc. 2004, 126, 15802–15808. [Google Scholar] [CrossRef] [PubMed]
- Gan, W.; Xiong, W.; Yu, Y.; Li, S. Effects of the molecular weight of poly(ether imide) on the viscoelastic phase separation of poly(ether imide)/epoxy blends. J. Appl. Polym. Sci. 2009, 114, 3158–3167. [Google Scholar]
- Kohga, M. Viscoelastic behavior of hydroxyl terminated polybutadiene containing glycerin. J. Appl. Polym. Sci. 2011, 122, 706–713. [Google Scholar] [CrossRef]
- Lelli, G.; Terenzi, A.; Kenny, J.M.; Torre, L. Modelling of the chemo-rheological behavior of thermosetting polymer nanocomposites. Polym. Compos. 2009, 30, 1–12. [Google Scholar] [CrossRef]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rwei, S.-P.; Chen, Y.-M.; Lin, W.-Y.; Chiang, W.-Y. Synthesis and Rheological Characterization of Water-Soluble Glycidyltrimethylammonium-Chitosan. Mar. Drugs 2014, 12, 5547-5562. https://doi.org/10.3390/md12115547
Rwei S-P, Chen Y-M, Lin W-Y, Chiang W-Y. Synthesis and Rheological Characterization of Water-Soluble Glycidyltrimethylammonium-Chitosan. Marine Drugs. 2014; 12(11):5547-5562. https://doi.org/10.3390/md12115547
Chicago/Turabian StyleRwei, Syang-Peng, Yu-Ming Chen, Wen-Yan Lin, and Whe-Yi Chiang. 2014. "Synthesis and Rheological Characterization of Water-Soluble Glycidyltrimethylammonium-Chitosan" Marine Drugs 12, no. 11: 5547-5562. https://doi.org/10.3390/md12115547
APA StyleRwei, S.-P., Chen, Y.-M., Lin, W.-Y., & Chiang, W.-Y. (2014). Synthesis and Rheological Characterization of Water-Soluble Glycidyltrimethylammonium-Chitosan. Marine Drugs, 12(11), 5547-5562. https://doi.org/10.3390/md12115547