Development of Analytical Quality by Design Compliant Chaotropic Chromatography Method for Ziprasidone and Its Five Impurities Determination
Abstract
1. Introduction
2. Results and Discussion
2.1. Development of AQbD Compliant Chaotropic Chromatography Method
2.2. Computation of 3D-DS and 2D-DS for the Selection of Working Point
2.3. Quantitative Robustness Testing
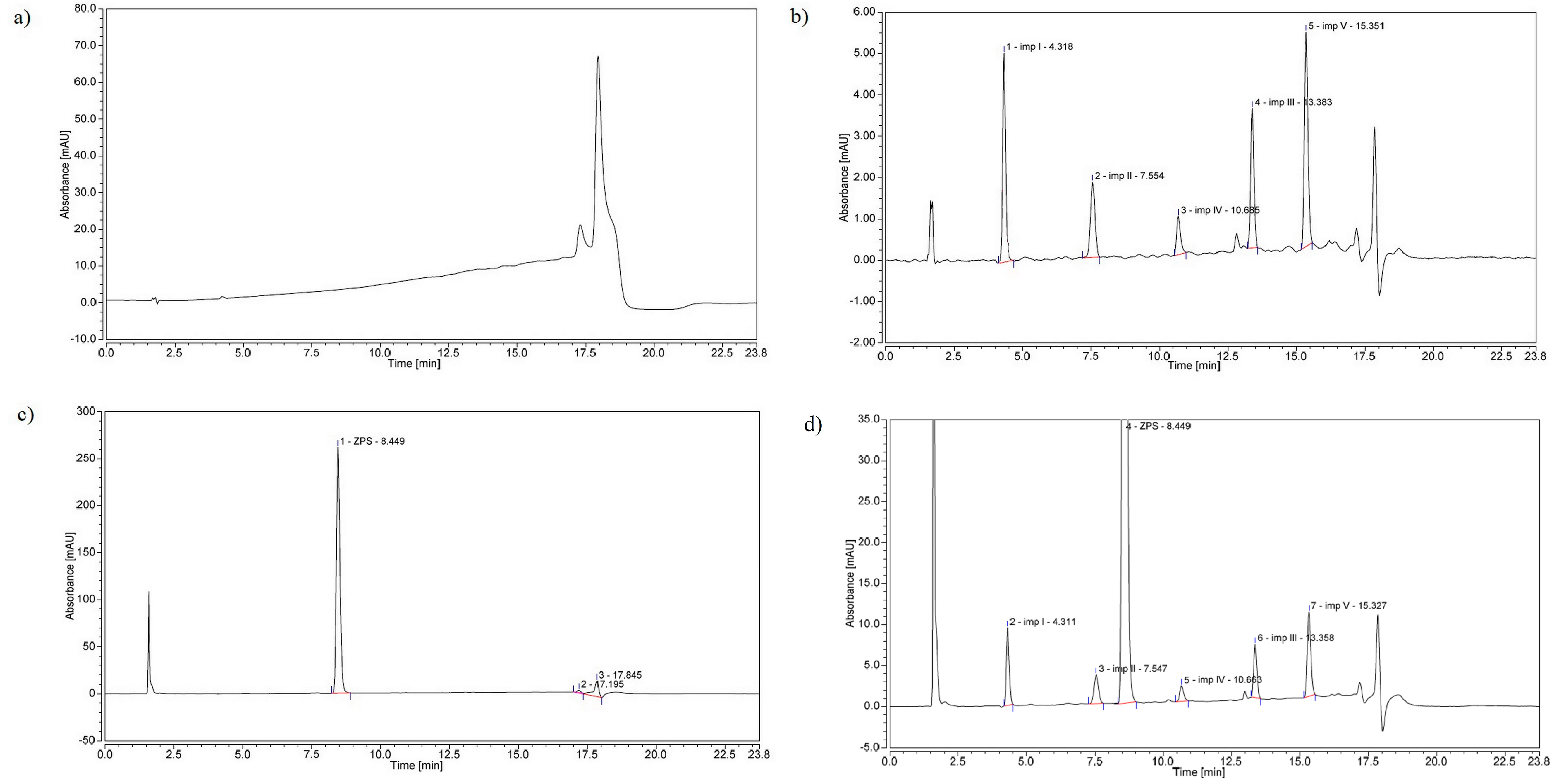
2.4. Method Validation
3. Materials and Methods
3.1. Reagents and Chemicals
3.2. Chromatographic Conditions
3.3. Standard Solutions for Method Development and Robustness Testing
3.4. Standard Solutions for Method Validation
3.4.1. Solutions for Selectivity Estimation
3.4.2. Solutions for Linearity Estimation
3.4.3. Solutions for Accuracy Estimation
3.4.4. Solutions for Precision Estimation
3.5. Software
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Caley, C.F.; Cooper, C.K. Ziprasidone: The fifth atypical antipsychotic. Ann. Pharmacother. 2002, 36, 839–851. [Google Scholar] [CrossRef] [PubMed]
- Zakowiecki, D.; Cal, K. Development of rapid and robust stability-indicating method for analysis of ziprasidone (hydrochloride and freebase) as drug substance and in medicines by UPLC. Acta Pol. Pharm. 2012, 69, 809–819. [Google Scholar] [PubMed]
- Chemicalize—Instant Cheminformatics Solutions. Available online: https://chemicalize.com/ (accessed on 10 May 2023).
- European Pharmacopoeia, 11th ed.; Council of Europe: Strasbourg, France, 2023.
- United States Pharmacopoeia, 47NF; US Pharmacopeia Convention: Rockville, MD, USA, 2022.
- Obradović, D.; Filipić, S.; Nikolić, K.; Čarapić, M.; Agbaba, D. Optimization of TLC method for separation and determination of ziprasidone and its impurities. J. Liq. Chromatogr. Relat. Technol. 2016, 39, 271–276. [Google Scholar] [CrossRef][Green Version]
- Priya, G.R.; Karikalan, M.; Asadulla, S.; Rajesh, S.; Krishna, J.S.R.; Sridhar, S.V. Development and Method Validation Using HPLC for Assay of Ziprasidone Capsule. Int. J. Pharm. Sci. Res. 2011, 2, 2325–2327. [Google Scholar] [CrossRef]
- Čarapić, M.; Nikolić, K.; Marković, B.; Petković, M.; Pavlović, M.; Agbaba, D. Ultra-performance liquid chromatography tandem mass-spectrometry (UHPLC-MS /MS) for the rapid, simultaneous analysis of ziprasidone and its impurities. Biomed. Chromatogr. 2019, 33, e4384. [Google Scholar] [CrossRef] [PubMed]
- Čarapić, M.; Marković, B.; Pavlović, M.; Agbaba, D.; Nikolić, K. Comparative study of performances of UHPLC-MS/MS and HPLC/UV methods for analysis of ziprasidone and its main impurities. Acta Chromatogr. 2022, 35, 260–271. [Google Scholar] [CrossRef]
- Pan, L.; LoBrutto, R.; Kazakevich, Y.V.; Thompson, R. Influence of inorganic mobile phase additives on the retention, efficiency and peak symmetry of protonated basic compounds in reversed-phase liquid chromatography. J. Chromatogr. A 2004, 1049, 63–73. [Google Scholar] [CrossRef]
- Cecchi, T. Ion-Pair Chromatography and Related Techniques; CRC Press: Boca Raton, FL, USA, 2009. [Google Scholar] [CrossRef]
- Pilorz, K.; Choma, I. Isocratic reversed-phase high-performance liquid chromatographic separation of tetracyclines and flumequine controlled by a chaotropic effect. J. Chromatogr. A 2004, 1031, 303–305. [Google Scholar] [CrossRef]
- Shulyak, N.; Piponski, M.; Kovalenko, S.; Stoimenova, T.B.; Drapak, I.; Piponska, M.; Rezk, M.R.; Abbeyquaye, A.D.; Oleshchuk, O.; Logoyda, L. Chaotropic salts impact in HPLC approaches for simultaneous analysis of hydrophilic and lipophilic drugs. J. Sep. Sci. 2021, 44, 2908–2916. [Google Scholar] [CrossRef]
- Flieger, J.; Czajkowska-Żelazko, A. Comparison of chaotropic salt and ionic liquid as mobile phase additives in reversed phase high-performance liquid chromatography of biogenic amines. J. Sep. Sci. 2011, 34, 733–739. [Google Scholar] [CrossRef]
- Dobričić, V.; Vukadinović, D.; Jančić-Stojanović, B.; Vladimirov, S.; Čudina, O. AQbD-Oriented Development of a New LC Method for Simultaneous Determination of Telmisartan and Its Impurities. Chromatographia 2017, 80, 1199–1209. [Google Scholar] [CrossRef]
- Prajapati, P.B.; Patel, A.S.; Shah, S.A. DoE-Based Analytical-FMCEA for Enhanced AQbD Approach to MEER-RP-HPLC Method for Synchronous Estimation of 15 Anti-Hypertensive Pharmaceutical Dosage Forms. J. AOAC Int. 2022, 105, 34–45. [Google Scholar] [CrossRef] [PubMed]
- Tomić, J.; Đajić, N.; Agbaba, D.; Otašević, B.; Malenović, A.; Protić, A. Robust optimization of gradient RP HPLC method for simultaneous determination of ivabradine and its eleven related substances by AQbD approach. Acta Chromatogr. 2021, 34, 1–11. [Google Scholar] [CrossRef]
- Čolović, J.; Rmandić, M.; Malenović, A. Robust Optimization of Chaotropic Chromatography Assay for Lamotrigine and its Two Impurities in Tablets. Chromatographia 2018, 82, 565–577. [Google Scholar] [CrossRef]
- ICH Harmonized Guideline: Validation of Analytical Procedures: Text and Methodology Q2 (R1); ICH: Geneva, Switzerland, 2005.
- Vemić, A.; Kalinić, M.; Čolović, J.; Erić, S.; Malenović, A. Chapter 1: Recent Progress in Fundamental Understanding and Practice of Chaotropic Chromatography: Rationalizing the Effects of Analytes’ Structure with Pharmaceutical Applications. In Advances in Chromatography; Grushka, E., Grinberg, N., Eds.; CRC Press Taylor & Francis Group: Boca Raton, FL, USA, 2017; Volume 54, pp. 1–42. [Google Scholar] [CrossRef]
- Gkountanas, K.; Malenović, A.; Dotsikas, Y. Determination of Bupropion and Its Impurities via a Chaotropic Chromatography Method Following Analytical Quality-by-Design Principles for Method Development. Pharmaceuticals 2022, 15, 1196. [Google Scholar] [CrossRef] [PubMed]
- Dewe, W.; Marini, R.D.; Chiap, P.; Hubert, P.; Crommen, J.; Boulanger, B. Development of response models for optimising HPLC methods. Chemom. Intell. Lab. Syst. 2004, 74, 263–268. [Google Scholar] [CrossRef]
- Dispas, A.; Avohou, H.; Lebrun, P.; Hubert, P.; Hubert, C. ‘Quality by Design’ approach for the analysis if impurities in pharmaceutical drug products and drug substances. Trends Anal. Chem. 2018, 101, 24–33. [Google Scholar] [CrossRef]
- Crowther, J.B. Chapter 12: Validation of Pharmaceutical Test Methods. In Handbook of Modern Pharmaceutical Analysis; Ahuja, S., Scypinski, S., Eds.; Academic Press: New York, NY, USA, 2001; Volume 3, pp. 415–443. [Google Scholar]


| Gradient Elution Mode | |
|---|---|
| ATP | The efficient baseline separation and accurate determination of ziprasidone and its five impurities assay in capsules. Probability: π ≥ 80% Separation factors: S ≥ 0 min Retention time of the last eluting peak: t_imp. V < 15.5 min Mean value of peak widths according to the USP: <WUSP> ≤ 0.235 min The time elapsed from the elution of the first to the elution of the last peak: t_imp. V − t_imp. I < 12 min Recovery values: 98.0–102.0% for ziprasidone, 70.0–130.0% for impurities. Limit of detection: not less than 0.05% |
| CMP | x1—initial content of methanol in the mobile phase (%, v/v) x2—the final content of methanol in the mobile phase (%, v/v) x3—the duration of the gradient (min) |
| CMA | S (min) t_imp. V (min) <WUSP> (min) t_imp. V − t_imp. I (min) |
| № | x1 | x2 | x3 | te_imp. x | tb_imp. III | t_imp. V | <WUSP> | t_imp. V − t_imp. I |
|---|---|---|---|---|---|---|---|---|
| 1 | 35 | 75 | 17.5 | 15.00 | 15.03 | 17.21 | 0.24 | 12.405 |
| 2 | 40 | 75 | 17.5 | 13.66 | 13.73 | 16.14 | 0.25 | 12.302 |
| 3 | 35 | 85 | 17.5 | 13.11 | 13.11 | 15.00 | 0.21 | 10.248 |
| 4 | 40 | 85 | 17.5 | 12.04 | 12.04 | 14.04 | 0.22 | 10.192 |
| 5 | 35 | 80 | 15.0 | 12.81 | 12.81 | 14.62 | 0.21 | 9.860 |
| 6 | 40 | 80 | 15.0 | 11.84 | 11.84 | 13.77 | 0.22 | 9.915 |
| 7 | 35 | 80 | 20.0 | 15.24 | 15.24 | 17.43 | 0.24 | 12.589 |
| 8 | 40 | 80 | 20.0 | 13.84 | 13.84 | 16.23 | 0.25 | 12.358 |
| 9 | 37.5 | 75 | 15.0 | 13.24 | 13.24 | 15.31 | 0.23 | 11.012 |
| 10 | 37.5 | 85 | 15.0 | 11.63 | 11.63 | 13.35 | 0.20 | 9.063 |
| 11 | 37.5 | 75 | 20.0 | 15.62 | 15.63 | 18.14 | 0.26 | 13.832 |
| 12 | 37.5 | 85 | 20.0 | 13.72 | 13.73 | 15.82 | 0.23 | 11.515 |
| 13 | 37.5 | 80 | 17.5 | 13.38 | 13.42 | 15.53 | 0.23 | 11.257 |
| 14 | 37.5 | 80 | 17.5 | 13.39 | 13.40 | 15.52 | 0.23 | 11.242 |
| 15 | 37.5 | 80 | 17.5 | 13.50 | 13.51 | 15.59 | 0.23 | 11.298 |
| 16 | 37.5 | 80 | 17.5 | 13.50 | 13.50 | 15.58 | 0.23 | 11.289 |
| t_imp. V | te_imp x | tb_imp III | <WUSP> | t_imp V − t_imp I | |
|---|---|---|---|---|---|
| b0 | 15.554 * | 13.443 * | 13.458 * | 0.2289 * | 11.272 * |
| b1 | −0.511 * | −0.598 * | −0.593 * | 0.0041 * | −0.042 * |
| b2 | −1.076 * | −0.878 * | −0.890 * | −0.0132 * | −1.067 * |
| b3 | 1.321 * | 1.113 * | 1.115 * | 0.0152 * | 1.306 * |
| b12 | 0.029 | 0.068 | 0.057 * | / | 0.012 |
| b13 | −0.087 * | −0.108 * | −0.108 * | / | −0.072 * |
| b23 | −0.090 * | −0.073 * | −0.073 * | / | −0.092 * |
| B11 | −0.050 * | −0.055 | −0.053 * | / | −0.080 * |
| b22 | 0.094 * | 0.065 * | 0.072 * | / | 0.095 * |
| b33 | 0.006 | 0.045 | 0.028 | / | −0.011 |
| R2 | 0.9998 | 0.9993 | 0.9994 | 0.9838 | 0.9999 |
| adj. R2 | 0.9996 | 0.9982 | 0.9986 | 0.9797 | 0.9996 |
| pred. R2 | 0.9995 | 0.9984 | 0.9977 | 0.9711 | 0.9989 |
| № | A | B | C | D | E | F | G | H | J | K | L | P_imp I | P_imp II | P_API | P_imp III | P_imp IV | P_imp V |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 39.5 | 1 | 76.5 | 16.5 | 1 | 10 | −1 | 0.9 | −1 | 35 | 229 | 1417.35 | 353.84 | 9069.79 | 418.65 | 281.58 | 1378.96 |
| 2 | 37.5 | 1 | 78.5 | 16 | 1 | 10 | 1 | 0.9 | −1 | 25 | 231 | 1640.374 | 369.832 | 10,040.28 | 405.841 | 238.698 | 1451.263 |
| 3 | 39.5 | −1 | 78.5 | 16.5 | −1 | 10 | 1 | 1.1 | −1 | 25 | 229 | 1095.628 | 344.924 | 7130.304 | 254.497 | 212.253 | 1177.324 |
| 4 | 37.5 | 1 | 76.5 | 16.5 | 1 | 5 | 1 | 1.1 | 1 | 25 | 229 | 1051.206 | 264.087 | 6899.781 | 254.641 | 224.772 | 1053.161 |
| 5 | 37.5 | −1 | 78.5 | 16 | 1 | 10 | −1 | 1.1 | 1 | 35 | 229 | 1640.114 | 243.709 | 10624.03 | 408.023 | 283.986 | 1522.191 |
| 6 | 37.5 | −1 | 76.5 | 16.5 | −1 | 10 | 1 | 0.9 | 1 | 35 | 231 | 1301.494 | 361.236 | 8404.344 | 246.173 | 258.071 | 1176.329 |
| 7 | 39.5 | −1 | 76.5 | 16 | 1 | 5 | 1 | 1.1 | −1 | 35 | 231 | 1376.018 | 215.475 | 9289.761 | 203.823 | 221.739 | 1327.625 |
| 8 | 39.5 | 1 | 76.5 | 16 | −1 | 10 | −1 | 1.1 | 1 | 25 | 231 | 1084.411 | 325.384 | 6888.604 | 258.061 | 195.279 | 1093.397 |
| 9 | 39.5 | 1 | 78.5 | 16 | −1 | 5 | 1 | 0.9 | 1 | 35 | 229 | 1768.829 | 306.912 | 10,205.74 | 531.56 | 297.964 | 1532.409 |
| 10 | 37.5 | 1 | 78.5 | 16.5 | −1 | 5 | −1 | 1.1 | −1 | 35 | 231 | 2001.457 | 289.047 | 9661.038 | 371.323 | 272.7 | 1340.322 |
| 11 | 39.5 | −1 | 78.5 | 16.5 | 1 | 5 | −1 | 0.9 | 1 | 25 | 231 | 1202.518 | 270.312 | 7721.945 | 269.349 | 229.951 | 1201.462 |
| 12 | 37.5 | −1 | 76.5 | 16 | −1 | 5 | −1 | 0.9 | −1 | 25 | 229 | 1625.379 | 400.925 | 10207.9 | 254.525 | 320.083 | 1531.502 |
| Substance | LOD (μg mL−1) | Linearity | |||||
|---|---|---|---|---|---|---|---|
| Concentration Range (µg mL–1) | a | b | r | Concentration Level (µg mL–1) | Recovery ** (%) | ||
| Ziprasidone | - | 25–75 | 0.7832 | −0.032 | 0.9999 | 40 | 98.65 (0.76%) *** |
| 50 | 98.56 (0.88%) | ||||||
| 60 | 98.57 (0.34%) | ||||||
| Impurity I | 0.15 | 0.5 *–1.2 | 1.0216 | 0.0945 | 0.9989 | 0.5 | 97.01 (0.61%) *** |
| 1.0 | 102.80 (0.24%) | ||||||
| 1.2 | 105.90 (0.16%) | ||||||
| Impurity II | 0.15 | 0.5 *–1.2 | 0.6459 | 0.0167 | 0.9965 | 0.5 | 105.37 (0.78%) *** |
| 1.0 | 93.77 (3.99%) | ||||||
| 1.2 | 95.77 (0.13%) | ||||||
| Impurity III | 0.15 | 0.5 *–1.2 | 0.82092 | 0.0675 | 0.9989 | 0.5 | 97.82 (0.66%) *** |
| 1.0 | 99.36 (0.39%) | ||||||
| 1.2 | 104.65 (1.24%) | ||||||
| Impurity IV | 0.15 | 0.5 *–1.2 | 0.3345 | −0.0313 | 0.9958 | 0.5 | 107.57 (3.26%) *** |
| 1.0 | 98.42 (2.90%) | ||||||
| 1.2 | 106.35 (8.15%) | ||||||
| Impurity V | 0.15 | 0.5 *–1.2 | 1.5028 | 0.0173 | 0.9949 | 0.5 | 101.95 (1.06%) *** |
| 1.0 | 85.69 (1.07%) | ||||||
| 1.2 | 89.02 (0.31%) | ||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rmandić, M.; Vasilić, Đ.; Rašević, M.; Zečević, M.; Otašević, B.; Protić, A.; Malenović, A. Development of Analytical Quality by Design Compliant Chaotropic Chromatography Method for Ziprasidone and Its Five Impurities Determination. Pharmaceuticals 2023, 16, 1296. https://doi.org/10.3390/ph16091296
Rmandić M, Vasilić Đ, Rašević M, Zečević M, Otašević B, Protić A, Malenović A. Development of Analytical Quality by Design Compliant Chaotropic Chromatography Method for Ziprasidone and Its Five Impurities Determination. Pharmaceuticals. 2023; 16(9):1296. https://doi.org/10.3390/ph16091296
Chicago/Turabian StyleRmandić, Milena, Đorđe Vasilić, Marija Rašević, Mira Zečević, Biljana Otašević, Ana Protić, and Anđelija Malenović. 2023. "Development of Analytical Quality by Design Compliant Chaotropic Chromatography Method for Ziprasidone and Its Five Impurities Determination" Pharmaceuticals 16, no. 9: 1296. https://doi.org/10.3390/ph16091296
APA StyleRmandić, M., Vasilić, Đ., Rašević, M., Zečević, M., Otašević, B., Protić, A., & Malenović, A. (2023). Development of Analytical Quality by Design Compliant Chaotropic Chromatography Method for Ziprasidone and Its Five Impurities Determination. Pharmaceuticals, 16(9), 1296. https://doi.org/10.3390/ph16091296






