A Study on Repositioning Nalidixic Acid via Lanthanide Complexation: Synthesis, Characterization, Cytotoxicity and DNA/Protein Binding Studies
Abstract
1. Introduction
2. Results
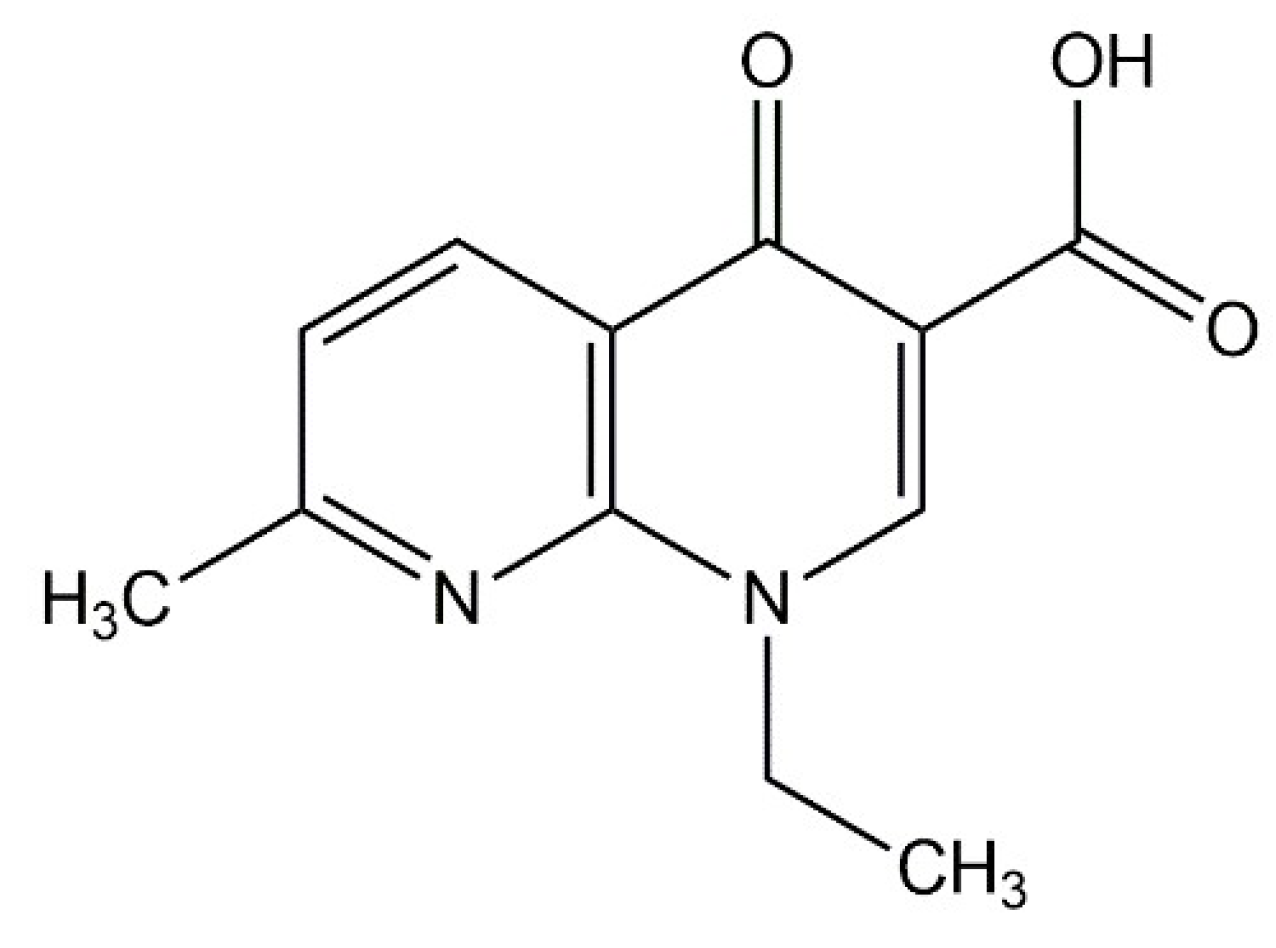
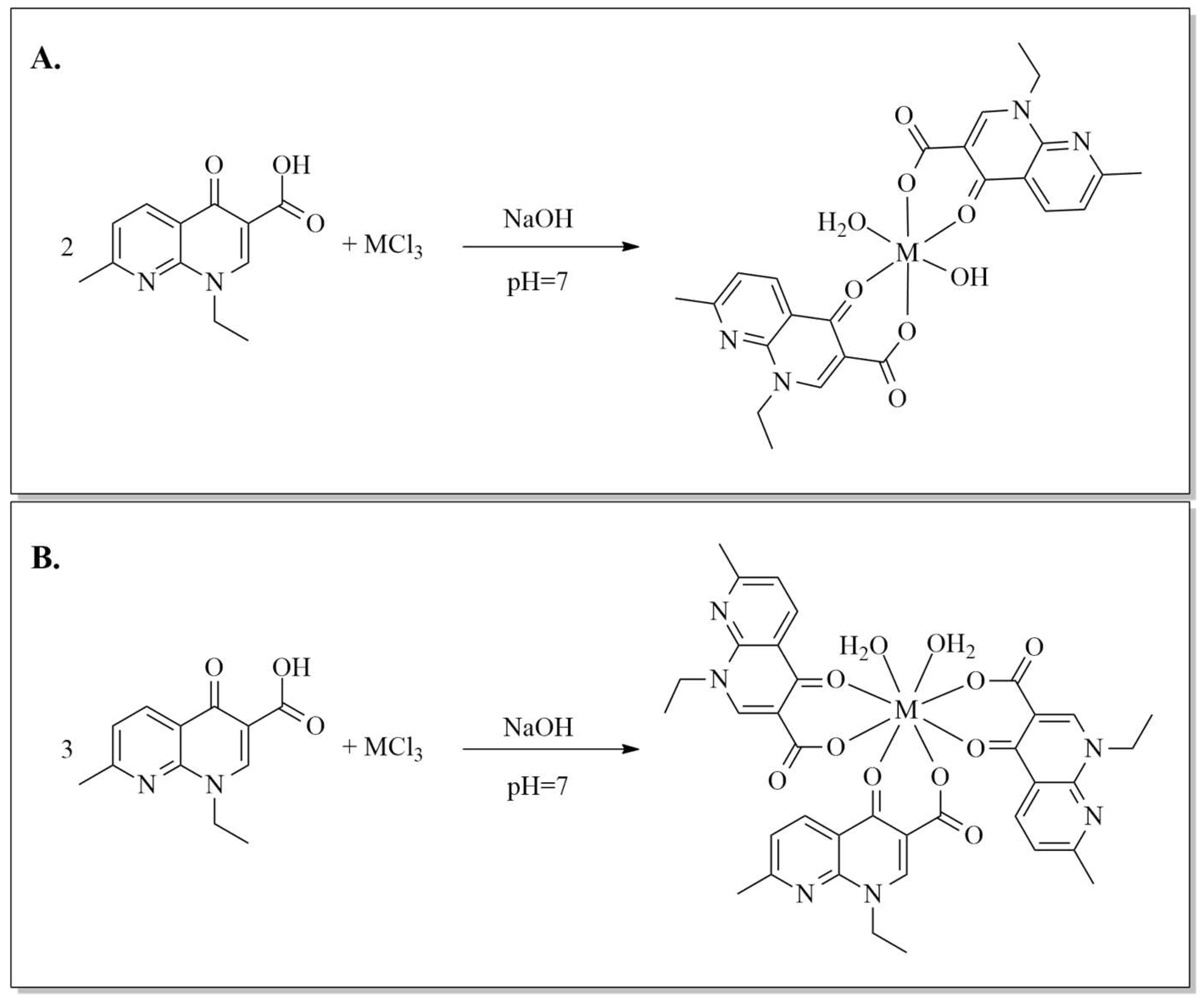
2.1. Synthesis
2.2. Structural Characterization
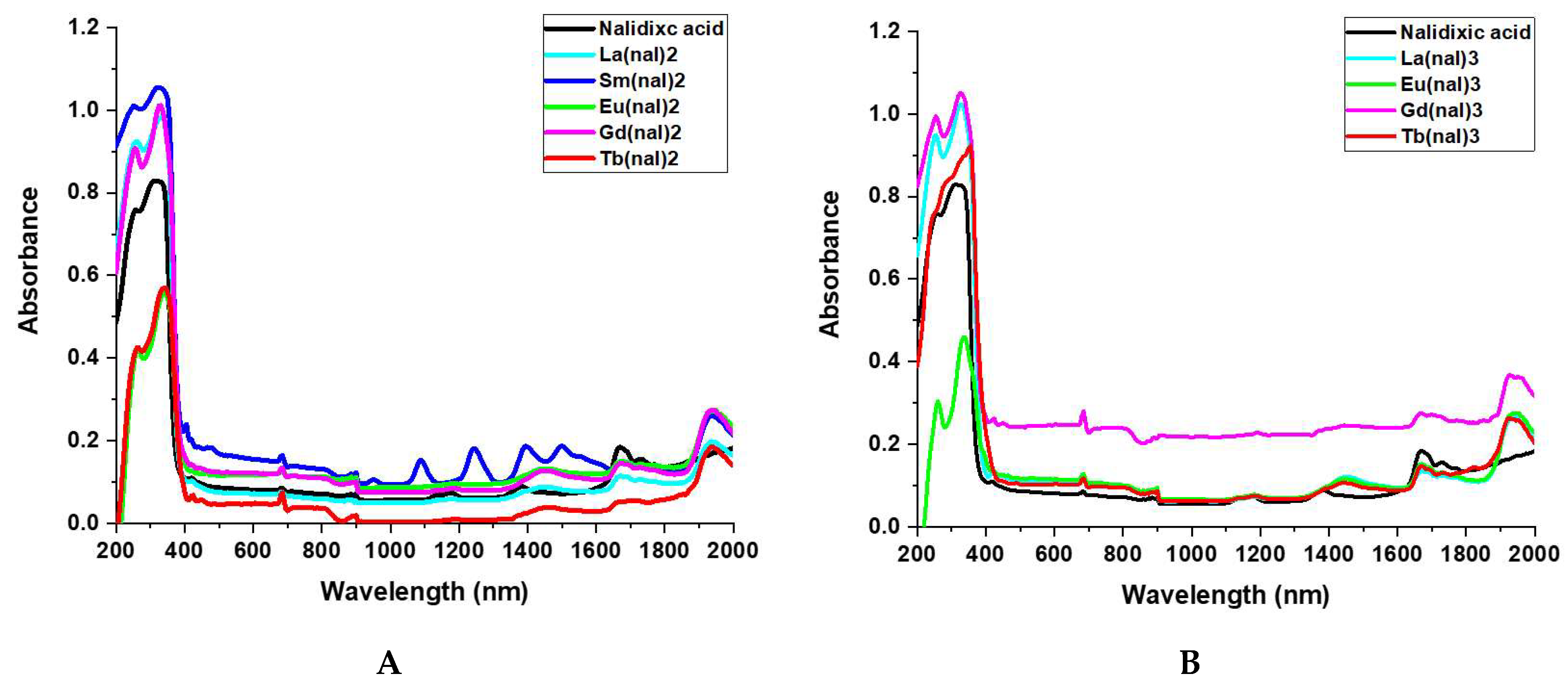
2.2.1. UV–Vis–NIR Spectra
2.2.2. FT-IR Spectra
2.2.3. Mass Spectra
2.2.4. Thermal Behavior
H NMR Spectra
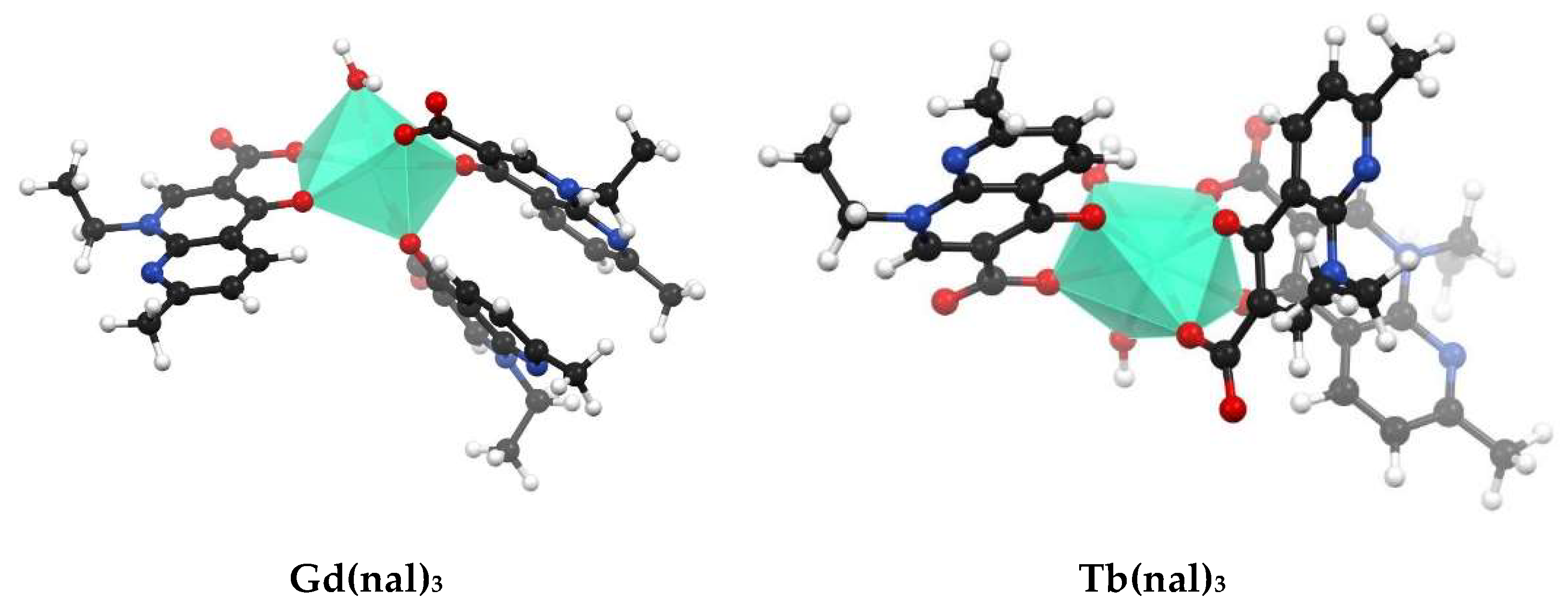
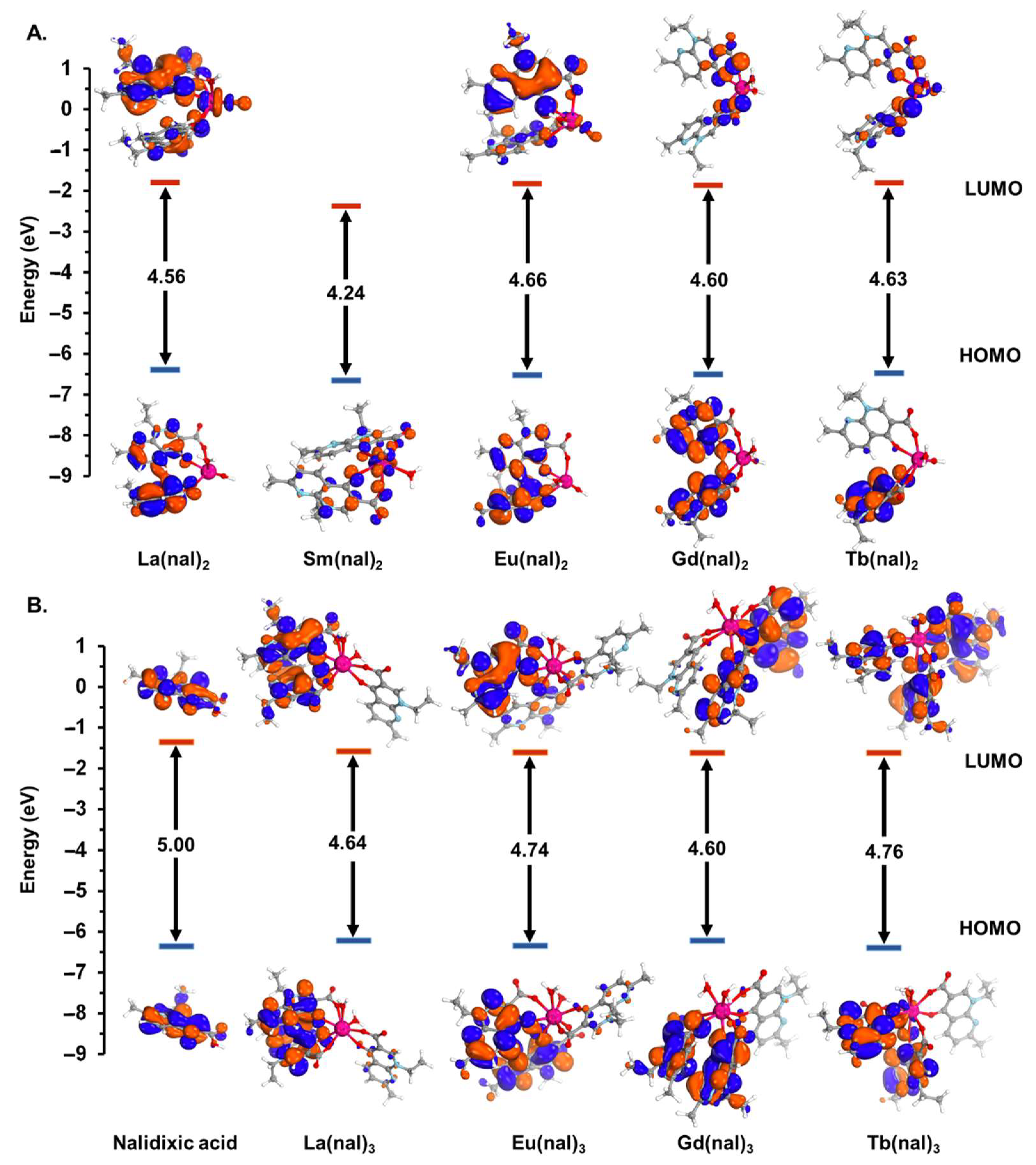
2.3. Computational Studies
2.4. Cytotoxicity Studies
2.5. DNA Binding Studies
2.5.1. Stability Studies
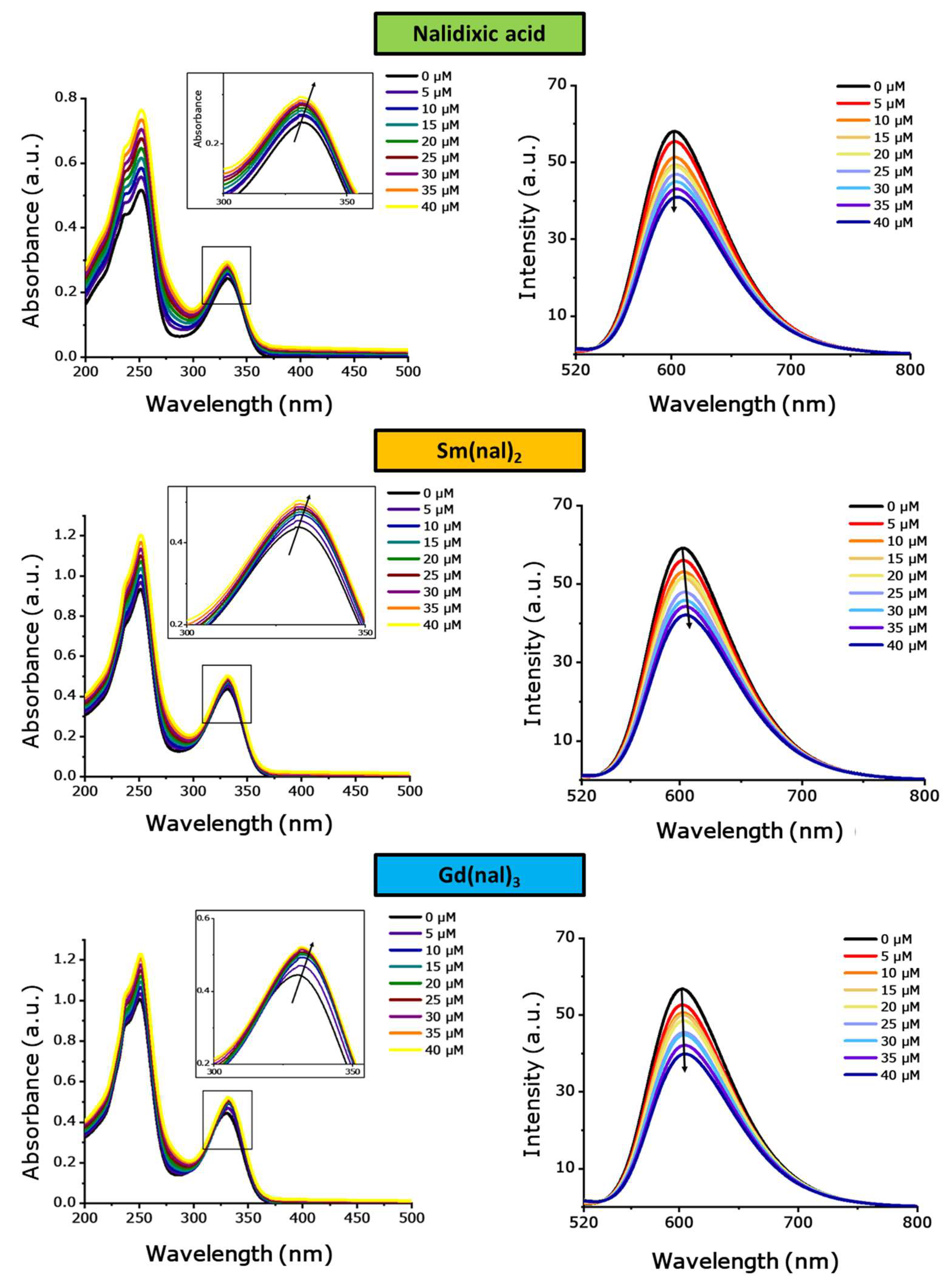
2.5.2. UV–Vis Spectroscopy
2.5.3. Fluorescence Spectroscopy
2.6. HSA and Apo-Tf Binding Studies
2.6.1. Studies Regarding the Fluorescence Quenching Mechanism
2.6.2. Studies on Conformational Changes of HSA and apo-Tf Due to Interaction with the Tested Compounds
3. Discussion
3.1. Structural Characterization
3.2. Computational Studies
3.3. Cytotoxicity Studies
3.4. DNA Binding Studies
3.5. HSA and Apo-Tf Binding Studies
4. Materials and Methods
4.1. Synthesis
4.2. Physicochemical Characterization of the Complexes
4.3. Computational Studies
4.4. Cytotoxicity Studies
4.5. Studies on DNA Binding
4.5.1. Stability Studies
4.5.2. UV–Vis Spectra
4.5.3. Competitive Binding Assay with Ethidium Bromide (EB) through Fluorescence Spectroscopy
4.6. HSA and Apo-Tf Binding Studies
4.6.1. Studies on fluorescence Quenching Mechanism
4.6.2. Studies on Conformational Changes of HSA and Apo-Tf Due to the Interaction with the Tested Compounds
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dalhoff, A.; Shalit, I. Immunomodulatory effects of quinolones. Lancet Infect. Dis. 2003, 3, 359–371. [Google Scholar] [CrossRef]
- Mukherjee, P.; Mandal, E.R.; Das, S.K. Evaluation of Antiproliferative Activity of Enoxacin on a Human Breast Cancer Cell Line. Int. J. Hum. Genet. 2005, 5, 57–63. [Google Scholar] [CrossRef]
- Yu, M.; Li, R.; Zhang, J. Repositioning of antibiotic levofloxacin as a mitochondrial biogenesis inhibitor to target breast cancer. Biochem. Biophys. Res. Commun. 2016, 471, 639–645. [Google Scholar] [CrossRef] [PubMed]
- Perucca, P.; Savio, M.; Cazzalini, O.; Mocchi, R.; Maccario, C.; Sommatis, S.; Ferraro, D.; Pizzala, R.; Pretali, L.; Fasani, E.; et al. Structure–activity relationship and role of oxygen in the potential antitumour activity of fluoroquinolones in human epithelial cancer cells. J. Photochem. Photobiol. B Biol. 2014, 140, 57–68. [Google Scholar] [CrossRef] [PubMed]
- Sousa, E.J.; Graça, I.; Baptista, T.; Vieira, F.Q.; Palmeira, C.; Henrique, R.; Jerónimo, C. Enoxacin inhibits growth of prostate cancer cells and effectively restores microRNA processing. Epigenetics 2013, 8, 548–558. [Google Scholar] [CrossRef]
- Aranha, O.; Grignon, R.; Fernandes, N.; McDonnell, T.J.; Wood, D.P.; Sarkar, F.H. Suppression of human prostate cancer cell growth by ciprofloxacin is associated with cell cycle arrest and apoptosis. Int. J. Oncol. 2003, 22, 787–794. [Google Scholar] [CrossRef]
- Aranha, O.; Wood, D.P.; Sarkar, F.H. Ciprofloxacin mediated cell growth inhibition, S/G2-M cell cycle arrest, and apoptosis in a human transitional cell carcinoma of the bladder cell line. Clin. Cancer Res. 2000, 6, 891–900. [Google Scholar]
- Chen, T.-C.; Hsu, Y.-L.; Tsai, Y.-C.; Chang, Y.-W.; Kuo, P.-L.; Chen, Y.-H. Gemifloxacin inhibits migration and invasion and induces mesenchymal–epithelial transition in human breast adenocarcinoma cells. J. Mol. Med. 2014, 92, 53–64. [Google Scholar] [CrossRef]
- Kan, J.-Y.; Hsu, Y.-L.; Chen, Y.-H.; Chen, T.-C.; Wang, J.-Y.; Kuo, P.-L. Gemifloxacin, a Fluoroquinolone Antimicrobial Drug, Inhibits Migration and Invasion of Human Colon Cancer Cells. BioMed Res. Int. 2013, 2013, 159786. [Google Scholar] [CrossRef]
- Fief, C.A.; Hoang, K.G.; Phipps, S.D.; Wallace, J.; Deweese, J.E. Examining the Impact of Antimicrobial Fluoroquinolones on Human DNA Topoisomerase IIα and IIβ. ACS Omega 2019, 4, 4049–4055. [Google Scholar] [CrossRef]
- Yadav, V.; Talwar, P. Repositioning of fluoroquinolones from antibiotic to anti-cancer agents: An underestimated truth. Biomed. Pharmacother. 2019, 111, 934–946. [Google Scholar] [CrossRef] [PubMed]
- Ashburn, T.T.; Thor, K.B. Drug repositioning: Identifying and developing new uses for existing drugs. Nat. Rev. Drug Discov. 2004, 3, 673–683. [Google Scholar] [CrossRef] [PubMed]
- Yella, J.K.; Yaddanapudi, S.; Wang, Y.; Jegga, A.G. Changing Trends in Computational Drug Repositioning. Pharmaceuticals 2018, 11, 57. [Google Scholar] [CrossRef] [PubMed]
- Kumara, A.; Saxena, N.; Mehrotrab, A.; Srivastava, N. Review: Studies On The Synthesis Of Quinolone Derivatives with Their Antibacterial Activity (Part1). Curr. Org. Chem. 2020, 24, 817–854. [Google Scholar] [CrossRef]
- Mihaila, M.; Hotnog, C.M.; Bostan, M.; Munteanu, A.C.; Vacaroiu, I.A.; Brasoveanu, L.I.; Uivarosi, V. Anticancer Activity of Some Ruthenium(III) Complexes with Quinolone Antibiotics: In Vitro Cytotoxicity, Cell Cycle Modulation, and Apoptosis-Inducing Properties in LoVo Colon Cancer Cell Line. Appl. Sci. 2021, 11, 8594. [Google Scholar] [CrossRef]
- Song, G.; Yan, Q.; He, Y. Studies on Interaction of Norfloxacin, Cu2+, and DNA by Spectral Methods. J. Fluoresc. 2005, 15, 673–678. [Google Scholar] [CrossRef]
- Drevenšek, P.; Turel, I.; Ulrih, N.P. Influence of copper(II) and magnesium(II) ions on the ciprofloxacin binding to DNA. J. Inorg. Biochem. 2003, 96, 407–415. [Google Scholar] [CrossRef]
- Guo, D.-S.; Jing, B.-Y.; Yuan, X.-Y. Influence of Mg+2 and Cu+2 on the interaction between quinolone and calf thymus DNA. J. Fluoresc. 2011, 21, 113–118. [Google Scholar]
- Robles, J.; Martín-Polo, J.; Alvarez-Valtierra, L.; Hinojosa, L.; Díaz, G.M. A Theoretical-Experimental Study on the Structure and Activity of Certain Quinolones and the Interaction of Their Cu(II)-Complexes on a DNA Model. Met. Drugs 2000, 7, 301–311. [Google Scholar] [CrossRef]
- Saha, D.K.; Padhye, S.; Anson, C.E.; Powell, A.K. Hydrothermal synthesis, crystal structure, spectroscopy, electrochemistry and antimycobacterial evaluation of the copper (II) ciprofloxacin complex: [Cu(cf)2(BF4)2]·6H2O. Inorg. Chem. Commun. 2002, 5, 1022–1027. [Google Scholar] [CrossRef]
- El-Halim, H.F.A.; Mohamed, G.G.; El-Dessouky, M.M.; Mahmoud, W.H. Ligational behaviour of lomefloxacin drug towards Cr(III), Mn(II), Fe(III), Co(II), Ni(II), Cu(II), Zn(II), Th(IV) and UO2(VI) ions: Synthesis, structural characterization and biological activity studies. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2011, 82, 8–19. [Google Scholar] [CrossRef] [PubMed]
- Patitungkho, S.; Adsule, S.; Dandawate, P.; Padhye, S.; Ahmad, A.; Sarkar, F.H. Synthesis, characterization and anti-tumor activity of moxifloxacin–Copper complexes against breast cancer cell lines. Bioorganic Med. Chem. Lett. 2011, 21, 1802–1806. [Google Scholar] [CrossRef] [PubMed]
- Batista, D.D.G.; da Silva, P.B.; Stivanin, L.; Lachter, D.R.; Silva, R.S.; Felcman, J.; Louro, S.R.; Teixeira, L.R.; Soeiro, M.D.N.C. Co(II), Mn(II) and Cu(II) complexes of fluoroquinolones: Synthesis, spectroscopical studies and biological evaluation against Trypanosoma cruzi. Polyhedron 2011, 30, 1718–1725. [Google Scholar] [CrossRef]
- Regiel-Futyra, A.; Dąbrowski, J.M.; Mazuryk, O.; Śpiewak, K.; Kyzioł, A.; Pucelik, B.; Brindell, M.; Stochel, G. Bioinorganic antimicrobial strategies in the resistance era. Coord. Chem. Rev. 2017, 351, 76–117. [Google Scholar] [CrossRef]
- Gouvea, L.R.; Garcia, L.S.; Lachter, D.R.; Nunes, P.R.; Pereira, F.D.C.; Silveira-Lacerda, E.P.; Louro, S.R.; Barbeira, P.J.S.; Teixeira, L.R. Atypical fluoroquinolone gold(III) chelates as potential anticancer agents: Relevance of DNA and protein interactions for their mechanism of action. Eur. J. Med. Chem. 2012, 55, 67–73. [Google Scholar] [CrossRef]
- Efthimiadou, E.K.; Thomadaki, H.; Sanakis, Y.; Raptopoulou, C.; Katsaros, N.; Scorilas, A.; Karaliota, A.; Psomas, G. Structure and biological properties of the copper(II) complex with the quinolone antibacterial drug N-propyl-norfloxacin and 2,2′-bipyridine. J. Inorg. Biochem. 2007, 101, 64–73. [Google Scholar] [CrossRef]
- Shingnapurkar, D.; Butcher, R.; Afrasiabi, Z.; Sinn, E.; Ahmed, F.; Sarkar, F.; Padhye, S. Neutral dimeric copper–sparfloxacin conjugate having butterfly motif with antiproliferative effects against hormone independent BT20 breast cancer cell line. Inorg. Chem. Commun. 2007, 10, 459–462. [Google Scholar] [CrossRef]
- Lesher, G.Y.; Froelich, E.J.; Gruett, M.D.; Bailey, J.H.; Brundage, R.P. 1,8-Naphthyridine Derivatives. A New Class of Chemotherapeutic Agents. J. Med. Pharm. Chem. 1962, 91, 1063–1065. [Google Scholar] [CrossRef]
- Gleckman, R.; Alvarez, S.; Joubert, D.W.; Matthews, S.J. Drug therapy reviews: Nalidixic acid. Am. J. Hosp. Pharm. 1979, 36, 1071–1076. [Google Scholar] [CrossRef]
- Crumplin, G.C.; Smith, J.T. Nalidixic Acid: An Antibacterial Paradox. Antimicrob. Agents Chemother. 1975, 8, 251–261. [Google Scholar] [CrossRef]
- Ahmed, M.; Kelley, S.O. Enhancing the Potency of Nalidixic Acid toward a Bacterial DNA Gyrase with Conjugated Peptides. ACS Chem. Biol. 2017, 12, 2563–2569. [Google Scholar] [CrossRef] [PubMed]
- de Carvalho, A.B.; de Souza, P.; de Andrade, L.M.; Binatti, I.; Pedroso, E.F.; Krambrock, K.; Oliveira, W.X.; Pereira-Maia, E.C.; Silva-Caldeira, P.P. Novel copper(II) coordination polymer containing the drugs nalidixic acid and 8-hydroxyquinoline: Evaluation of the structural, magnetic, electronic, and antitumor properties. Polyhedron 2018, 156, 312–319. [Google Scholar] [CrossRef]
- Al-Khodir, F.A.; Refat, M.S. Synthesis, structural characterization and biological studies of some nalidixic acid–metal complexes: Metalloantibiotic complexes of some divalent and trivalent metal ions. J. Mol. Struct. 2015, 1094, 22–35. [Google Scholar] [CrossRef]
- Hudej, R.; Kljun, J.; Kandioller, W.; Repnik, U.; Turk, B.; Hartinger, C.; Keppler, B.; Miklavčič, D.; Turel, I. Synthesis and Biological Evaluation of the Thionated Antibacterial Agent Nalidixic Acid and Its Organoruthenium(II) Complex. Organometallics 2012, 31, 5867–5874. [Google Scholar] [CrossRef]
- Kljun, J.; Bytzek, A.K.; Kandioller, W.; Bartel, C.; Jakupec, M.A.; Hartinger, C.G.; Keppler, B.K.; Turel, I. Physicochemical Studies and Anticancer Potency of Ruthenium η6-p-Cymene Complexes Containing Antibacterial Quinolones. Organometallics 2011, 30, 2506–2512. [Google Scholar] [CrossRef]
- Lee, S.S.; Jung, O.-S.; Lee, C.O.; Choi, S.U.; Jun, M.-J.; Sohn, Y.S. Cationic diamineplatinum (II) complexes of nalidixic acid. Inorg. Chim. Acta 1995, 239, 133–138. [Google Scholar] [CrossRef]
- Loganathan, R.; Ganeshpandian, M.; Bhuvanesh, N.S.; Palaniandavar, M.; Muruganantham, A.; Ghosh, S.K.; Riyasdeen, A.; Akbarsha, M.A. DNA and protein binding, double-strand DNA cleavage and cytotoxicity of mixed ligand copper(II) complexes of the antibacterial drug nalidixic acid. J. Inorg. Biochem. 2017, 174, 1–13. [Google Scholar] [CrossRef]
- Kumar, M.; Kumar, G.; Mogha, N.K.; Jain, R.; Hussain, F.; Masram, D.T. Structure, DNA/proteins binding, docking and cytotoxicity studies of copper(II) complexes with the first quinolone drug nalidixic acid and 2,2′-dipyridylamine. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2019, 212, 94–104. [Google Scholar] [CrossRef]
- Bueloni, B.; Sanna, D.; Garribba, E.; Castro, G.R.; León, I.E.; Islan, G.A. Design of nalidixic acid-vanadium complex loaded into chitosan hybrid nanoparticles as smart strategy to inhibit bacterial growth and quorum sensing. Int. J. Biol. Macromol. 2020, 161, 1568–1580. [Google Scholar] [CrossRef]
- Măciucă, A.-M.; Munteanu, A.-C.; Uivarosi, V. Quinolone Complexes with Lanthanide Ions: An Insight into their Analytical Applications and Biological Activity. Molecules 2020, 25, 1347. [Google Scholar] [CrossRef]
- Munguba, G.H.L.; Urquiza-Carvalho, G.A.; Silva, F.T.; Simas, A.M. The complex build algorithm to set up starting structures of lanthanoid complexes with stereochemical control for molecular modeling. Sci. Rep. 2021, 11, 21493. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Chang, H.; Xie, J.; Zhao, B.; Liu, B.; Xu, S.; Pei, W.; Ren, N.; Huang, L.; Huang, W. Recent developments in lanthanide-based luminescent probes. Coord. Chem. Rev. 2014, 273–274, 201–212. [Google Scholar] [CrossRef]
- Parker, D.; Fradgley, J.D.; Wong, K.-L. The design of responsive luminescent lanthanide probes and sensors. Chem. Soc. Rev. 2021, 50, 8193–8213. [Google Scholar] [CrossRef]
- Lacerda, S.; Tóth, É. Lanthanide Complexes in Molecular Magnetic Resonance Imaging and Theranostics. ChemMedChem 2017, 12, 883–894. [Google Scholar] [CrossRef] [PubMed]
- Zaldo, C. 10-Lanthanide-based luminescent thermosensors: From bulk to nanoscale. In Advanced Nanomaterials; Martín-Ramos, P., Silva, R., Eds.; Elsevier: Amsterdam, The Netherlands, 2018; pp. 335–379. ISBN 978-0-12-813840-3. [Google Scholar]
- Bao, G. Lanthanide complexes for drug delivery and therapeutics. J. Lumin. 2020, 228, 117622. [Google Scholar] [CrossRef]
- Munteanu, A.; Musat, M.G.; Mihaila, M.; Badea, M.; Olar, R.; Nitulescu, G.M.; Rădulescu, F.; Brasoveanu, L.I.; Uivarosi, V. New heteroleptic lanthanide complexes as multimodal drugs: Cytotoxicity studies, apoptosis, cell cycle analysis, DNA interactions, and protein binding. Appl. Organomet. Chem. 2021, 35, e6062. [Google Scholar] [CrossRef]
- Franklin, S.J. Lanthanide-mediated DNA hydrolysis. Curr. Opin. Chem. Biol. 2001, 5, 201–208. [Google Scholar] [CrossRef]
- Komiyama, M.; Takeda, N.; Shigekawa, H. Hydrolysis of DNA and RNA by lanthanide ions: Mechanistic studies leading to new applications. Chem. Commun. 1999, 16, 1443–1451. [Google Scholar] [CrossRef]
- Hussain, A.; Chakravarty, A.R. Photocytotoxic lanthanide complexes. J. Chem. Sci. 2012, 124, 1327–1342. [Google Scholar] [CrossRef]
- Liu, C.; Wang, M.; Zhang, T.; Sun, H. DNA hydrolysis promoted by di- and multi-nuclear metal complexes. Coord. Chem. Rev. 2004, 248, 147–168. [Google Scholar] [CrossRef]
- Sreedhara, A.; Cowan, J.A. Catalytic hydrolysis of DNA by metal ions and complexes. JBIC J. Biol. Inorg. Chem. 2001, 6, 337–347. [Google Scholar] [CrossRef] [PubMed]
- Návia, M.V.D.; Gálico, D.A.; Caires, F.J.; Luiz, J.M.; Nunes, R.S. Synthesis, thermal behavior, and spectroscopic study of the solid nalidixate of selected light trivalent lanthanides. J. Therm. Anal. 2018, 132, 1717–1727. [Google Scholar] [CrossRef]
- El-Shenawy, A.; Atta, A.; Refat, M. Complexation of Gadolinium(III) and terbium(III) with nalidixic acid (NDX): Molar conductivity, thermal and spectral investigation. Int. J. Electrochem. Sci. 2014, 9, 5187–5203. [Google Scholar]
- Uivarosi, V.; Badea, M.; Olar, R.; Marinescu, D.; Nicolescu, T.O.; Nitulescu, G.M. Thermal degradation behavior of some ruthenium complexes with fluoroquinolone derivatives as potential antitumor agents. J. Therm. Anal. 2011, 105, 645–650. [Google Scholar] [CrossRef]
- Zaky, M.; El-Sayed, M.Y.; El-Megharbel, S.M.; Taleb, S.A.; Refat, M.S. Complexes of nalidixic acid with some vital metal ions: Synthesis, chemical structure elucidation, and antimicrobial evaluation. Russ. J. Gen. Chem. 2013, 83, 2488–2501. [Google Scholar] [CrossRef]
- Rozanska, X.; Stewart, J.J.P.; Ungerer, P.; Leblanc, B.; Freeman, C.; Saxe, P.; Wimmer, E. High-Throughput Calculations of Molecular Properties in the MedeA Environment: Accuracy of PM7 in Predicting Vibrational Frequencies, Ideal Gas Entropies, Heat Capacities, and Gibbs Free Energies of Organic Molecules. J. Chem. Eng. Data 2014, 59, 3136–3143. [Google Scholar] [CrossRef]
- Świsłocka, R.; Regulska, E.; Karpińska, J.; Świderski, G.; Lewandowski, W. Molecular Structure and Antioxidant Properties of Alkali Metal Salts of Rosmarinic Acid. Experimental and DFT Studies. Molecules 2019, 24, 2645. [Google Scholar] [CrossRef] [PubMed]
- Weiss, J.N. The Hill equation revisited: Uses and misuses. FASEB J. 1997, 11, 835–841. [Google Scholar] [CrossRef]
- Neugebauer, U.; Szeghalmi, A.; Schmitt, M.; Kiefer, W.; Popp, J.; Holzgrabe, U. Vibrational spectroscopic characterization of fluoroquinolones. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2005, 61, 1505–1517. [Google Scholar] [CrossRef]
- Munteanu, A.-C.; Badea, M.; Olar, R.; Silvestro, L.; Dulea, C.; Negut, C.-D.; Uivarosi, V. Synthesis and Structural Investigation of New Bio-Relevant Complexes of Lanthanides with 5-Hydroxyflavone: DNA Binding and Protein Interaction Studies. Molecules 2016, 21, 1737. [Google Scholar] [CrossRef]
- Sastri, V.R.; Perumareddi, J.R.; Rao, V.R.; Rayudu, G.V.S.; Bünzli, J.-C. Modern Aspects of Rare Earths and Their Complexes; Elsevier: Amsterdam, The Netherlands, 2003; ISBN 0080536689. [Google Scholar]
- Takeuchi, M.; Martra, G.; Coluccia, S.; Anpo, M. Investigations of the Structure of H2O Clusters Adsorbed on TiO2 Surfaces by Near-Infrared Absorption Spectroscopy. J. Phys. Chem. B 2005, 109, 7387–7391. [Google Scholar] [CrossRef] [PubMed]
- Nakamoto, K. Infrared and Raman Spectra of Inorganic and Coordination Compounds, Part B: Applications in Coordination, Organometallic, and Bioinorganic Chemistry; Wiley-Interscience: New York, NY, USA, 2009; ISBN 047174493X. [Google Scholar]
- Joshi, R.; Pandey, N.; Tilak, R.; Yadav, S.K.; Mishra, H.; Pokharia, S. New triorganotin(IV) complexes of quinolone antibacterial drug sparfloxacin: Synthesis, structural characterization, DFT studies and biological activity. Appl. Organomet. Chem. 2018, 32, e4324. [Google Scholar] [CrossRef]
- Emelina, T.B.; Freidzon, A.Y.; Bagaturyants, A.A.; Karasev, V.E. Electronic Structure and Energy Transfer in Europium(III)–Ciprofloxacin Complexes: A Theoretical Study. J. Phys. Chem. A 2016, 120, 7529–7537. [Google Scholar] [CrossRef] [PubMed]
- Polishchuk, A.V.; Karaseva, E.T.; Emelina, T.; Karasev, V.E. Spectroscopic and Photophysical Properties of Protonated Forms of Some Fluoroquinolones in Solutions. J. Fluoresc. 2012, 22, 373–379. [Google Scholar] [CrossRef] [PubMed]
- Efthimiadou, E.K.; Karaliota, A.; Psomas, G. Mononuclear metal complexes of the second-generation quinolone antibacterial agent enrofloxacin: Synthesis, structure, antibacterial activity and interaction with DNA. Polyhedron 2008, 27, 1729–1738. [Google Scholar] [CrossRef]
- Schinzel, S.; Bindl, M.; Visseaux, M.; Chermette, H. Structural and Electronic Analysis of Lanthanide Complexes: Reactivity May Not Necessarily Be Independent of the Identity of the Lanthanide Atom—A DFT Study. J. Phys. Chem. A 2006, 110, 11324–11331. [Google Scholar] [CrossRef][Green Version]
- Sharma, M.; Ganeshpandian, M.; Sanjeev, A.; Tamilarasan, A.; Mattaparthi, V.S.K.; Islam, N.S.; Palaniandavar, M. Bis- and mixed-ligand copper(II) complexes of nalidixic acid the antibacterial drug: Mode of nalidixate coordination determines DNA binding and cleavage and cytotoxicity. Inorg. Chim. Acta 2020, 504, 119450. [Google Scholar] [CrossRef]
- Behzadi, H.; Roonasi, P.; Assle taghipour, K.; van der Spoel, D.; Manzetti, S. Relationship between electronic properties and drug activity of seven quinoxaline compounds: A DFT study. J. Mol. Struct. 2015, 1091, 196–202. [Google Scholar] [CrossRef]
- Helal, M.; El-Awdan, S.; Salem, M.; Abd-Elaziz, T.; Moahamed, Y.; El-Sherif, A.; Mohamed, G. Synthesis, biological evaluation and molecular modeling of novel series of pyridine derivatives as anticancer, anti-inflammatory and analgesic agents. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2015, 135, 764–773. [Google Scholar] [CrossRef]
- Oyewole, R.O.; Oyebamiji, A.K.; Semire, B. Theoretical calculations of molecular descriptors for anticancer activities of 1, 2, 3-triazole-pyrimidine derivatives against gastric cancer cell line (MGC-803): DFT, QSAR and docking approaches. Heliyon 2020, 6, e03926. [Google Scholar] [CrossRef]
- Nagaraja, V.; Godbole, A.A.; Henderson, S.R.; Maxwell, A. DNA topoisomerase I and DNA gyrase as targets for TB therapy. Drug Discov. Today 2017, 22, 510–518. [Google Scholar] [CrossRef] [PubMed]
- Charrier, C.; Salisbury, A.-M.; Savage, V.J.; Duffy, T.; Moyo, E.; Chaffer-Malam, N.; Ooi, N.; Newman, R.; Cheung, J.; Metzger, R.; et al. Novel Bacterial Topoisomerase Inhibitors with Potent Broad-Spectrum Activity against Drug-Resistant Bacteria. Antimicrob. Agents Chemother. 2017, 61, e02100-16. [Google Scholar] [CrossRef] [PubMed]
- Kato, J.-I.; Nishimura, Y.; Imamura, R.; Niki, H.; Hiraga, S.; Suzuki, H. New topoisomerase essential for chromosome segregation in E. coli. Cell 1990, 63, 393–404. [Google Scholar] [CrossRef]
- Idowu, T.; Schweizer, F. Ubiquitous Nature of Fluoroquinolones: The Oscillation between Antibacterial and Anticancer Activities. Antibiotics 2017, 6, 26. [Google Scholar] [CrossRef]
- Drlica, K.; Malik, M.; Kerns, R.J.; Zhao, X. Quinolone-Mediated Bacterial Death. Antimicrob. Agents Chemother. 2008, 52, 385–392. [Google Scholar] [CrossRef]
- Dwyer, D.J.; Kohanski, M.A.; Hayete, B.; Collins, J.J. Gyrase inhibitors induce an oxidative damage cellular death pathway in Escherichia coli. Mol. Syst. Biol. 2007, 3, 91. [Google Scholar] [CrossRef] [PubMed]
- Kumar, M.; Mogha, N.K.; Kumar, G.; Hussain, F.; Masram, D.T. Biological evaluation of copper(II) complex with nalidixic acid and 2,2′-bipyridine (bpy). Inorg. Chim. Acta 2019, 490, 144–154. [Google Scholar] [CrossRef]
- Tomczyk, M.D.; Walczak, K.Z. l,8-Naphthalimide based DNA intercalators and anticancer agents. A systematic review from 2007 to 2017. Eur. J. Med. Chem. 2018, 159, 393–422. [Google Scholar] [CrossRef]
- Buchtik, R.; Trávníček, Z.; Vančo, J.; Herchel, R.; Dvořák, Z. Synthesis, characterization, DNA interaction and cleavage, and in vitro cytotoxicity of copper (II) mixed-ligand complexes with 2-phenyl-3-hydroxy-4 (1H)-quinolinone. Dalt. Trans. 2011, 40, 9404–9412. [Google Scholar] [CrossRef]
- Wu, S.-S.; Yuan, W.-B.; Wang, H.-Y.; Zhang, Q.; Liu, M.; Yu, K.-B. Synthesis, crystal structure and interaction with DNA and HSA of (N,N′-dibenzylethane-1,2-diamine) transition metal complexes. J. Inorg. Biochem. 2008, 102, 2026–2034. [Google Scholar] [CrossRef]
- Li, H.; Le, X.-Y.; Pang, D.-W.; Deng, H.; Xu, Z.; Lin, Z.-H. DNA-binding and cleavage studies of novel copper(II) complex with l-phenylalaninate and 1,4,8,9-tetra-aza-triphenylene ligands. J. Inorg. Biochem. 2005, 99, 2240–2247. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Zhang, T.; Lu, T.; Qu, L.; Zhou, H.; Zhang, Q.; Ji, L. DNA-binding and cleavage studies of macrocyclic copper(II) complexes. J. Inorg. Biochem. 2002, 91, 269–276. [Google Scholar] [CrossRef]
- Kunath, S.; Marchyk, N.; Haupt, K.; Feller, K.-H. Multi-objective optimization and design of experiments as tools to tailor molecularly imprinted polymers specific for glucuronic acid. Talanta 2013, 105, 211–218. [Google Scholar] [CrossRef] [PubMed]
- Arjmand, F.; Yousuf, I.; Afzal, M.; Toupet, L. Design and synthesis of new Zn(II) nalidixic acid–DACH based Topo-II inhibiting molecular entity: Chemotherapeutic potential validated by its in vitro binding profile, pBR322 cleavage activity and molecular docking studies with DNA and RNA molecular targe. Inorg. Chim. Acta 2014, 421, 26–37. [Google Scholar] [CrossRef][Green Version]
- Chennam, K.P.; Ravi, M.; Ushaiah, B.; Srinu, V.; Eslavath, R.K.; Devi, C.S. Synthesis, characterization, DNA interactions, DNA cleavage, radical scavenging activity, antibacterial, anti-proliferative and docking studies of new transition metal complexes. J. Fluoresc. 2016, 26, 189–205. [Google Scholar] [CrossRef]
- Kozieł, S.; Lesiów, M.; Wojtala, D.; Dyguda-Kazimierowicz, E.; Bieńko, D.; Komarnicka, U. Interaction between DNA, Albumin and Apo-Transferrin and Iridium(III) Complexes with Phosphines Derived from Fluoroquinolones as a Potent Anticancer Drug. Pharmaceuticals 2021, 14, 685. [Google Scholar] [CrossRef]
- Yin, B.-T.; Yan, C.-Y.; Peng, X.-M.; Zhang, S.-L.; Rasheed, S.; Geng, R.-X.; Zhou, C.-H. Synthesis and biological evaluation of α-triazolyl chalcones as a new type of potential antimicrobial agents and their interaction with calf thymus DNA and human serum albumin. Eur. J. Med. Chem. 2014, 71, 148–159. [Google Scholar] [CrossRef]
- Du, H.; Xiang, J.; Zhang, Y.; Tang, Y. A spectroscopic and molecular modeling study of sinomenine binding to transferrin. Bioorganic Med. Chem. Lett. 2007, 17, 1701–1704. [Google Scholar] [CrossRef]
- Kratz, F.; Beyer, U.; Roth, T.; Tarasova, N.; Collery, P.; Lechenault, F.; Cazabat, A.; Schumacher, P.; Unger, C.; Falken, U. Transferrin Conjugates of Doxorubicin: Synthesis, Characterization, Cellular Uptake, and in Vitro Efficacy. J. Pharm. Sci. 1998, 87, 338–346. [Google Scholar] [CrossRef]
- Kawabata, H. Transferrin and transferrin receptors update. Free Radic. Biol. Med. 2019, 133, 46–54. [Google Scholar] [CrossRef]
- Maciuca, A.-M.; Munteanu, A.-C.; Mihaila, M.; Badea, M.; Olar, R.; Nitulescu, G.M.; Munteanu, C.V.A.; Bostan, M.; Uivarosi, V. Rare-Earth Metal Complexes of the Antibacterial Drug Oxolinic Acid: Synthesis, Characterization, DNA/Protein Binding and Cytotoxicity Studies. Molecules 2020, 25, 5418. [Google Scholar] [CrossRef] [PubMed]
- Mondal, P.; Bose, A. Spectroscopic overview of quercetin and its Cu(II) complex interaction with serum albumins. BioImpacts 2019, 9, 115–121. [Google Scholar] [CrossRef] [PubMed]
- Deepa, S.; Mishra, A.K. Fluorescence spectroscopic study of serum albumin–bromadiolone interaction: Fluorimetric determination of bromadiolone. J. Pharm. Biomed. Anal. 2005, 38, 556–563. [Google Scholar] [CrossRef]
- Munteanu, A.-C.; Badea, M.; Olar, R.; Silvestro, L.; Mihaila, M.; Brasoveanu, L.I.; Musat, M.G.; Andries, A.; Uivarosi, V. Cytotoxicity studies, DNA interaction and protein binding of new Al (III), Ga (III) and In (III) complexes with 5-hydroxyflavone. Appl. Organomet. Chem. 2018, 32, e4579. [Google Scholar] [CrossRef]
- Musiol, R. An overview of quinoline as a privileged scaffold in cancer drug discovery. Expert Opin. Drug Discov. 2017, 12, 583–597. [Google Scholar] [CrossRef] [PubMed]
- Stewart, J.J.P. Optimization of parameters for semiempirical methods I. Method. J. Comput. Chem. 1989, 10, 209–220. [Google Scholar] [CrossRef]
- Neese, F. Software update: The ORCA program system—Version 5.0. WIREs Comput. Mol. Sci. 2022, 8, e1606. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision C.01 2016; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Macrae, C.F.; Sovago, I.; Cottrell, S.J.; Galek, P.T.A.; McCabe, P.; Pidcock, E.; Platings, M.; Shields, G.P.; Stevens, J.S.; Towler, M.; et al. Mercury 4.0: From visualization to analysis, design and prediction. J. Appl. Crystallogr. 2020, 53, 226–235. [Google Scholar] [CrossRef]
- Navarro, M.; Hernández, C.; Colmenares, I.; Hernández, P.; Fernández, M.; Sierraalta, A.; Marchán, E. Synthesis and characterization of [Au(dppz)2]Cl3. DNA interaction studies and biological activity against Leishmania (L) mexicana. J. Inorg. Biochem. 2007, 101, 111–116. [Google Scholar] [CrossRef]
- Lakowicz, J.R.; Weber, G. Quenching of fluorescence by oxygen. Probe for structural fluctuations in macromolecules. Biochemistry 1973, 12, 4161–4170. [Google Scholar] [CrossRef]
- Zhang, G.; Wang, Y.; Zhang, H.; Tang, S.; Tao, W. Human serum albumin interaction with paraquat studied using spectroscopic methods. Pestic. Biochem. Physiol. 2007, 87, 23–29. [Google Scholar] [CrossRef]
- Fu, X.-B.; Lin, Z.-H.; Liu, H.-F.; Le, X.-Y. A new ternary copper (II) complex derived from 2-(2′-pyridyl) benzimidazole and glycylglycine: Synthesis, characterization, DNA binding and cleavage, antioxidation and HSA interaction. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2014, 122, 22–33. [Google Scholar] [CrossRef] [PubMed]



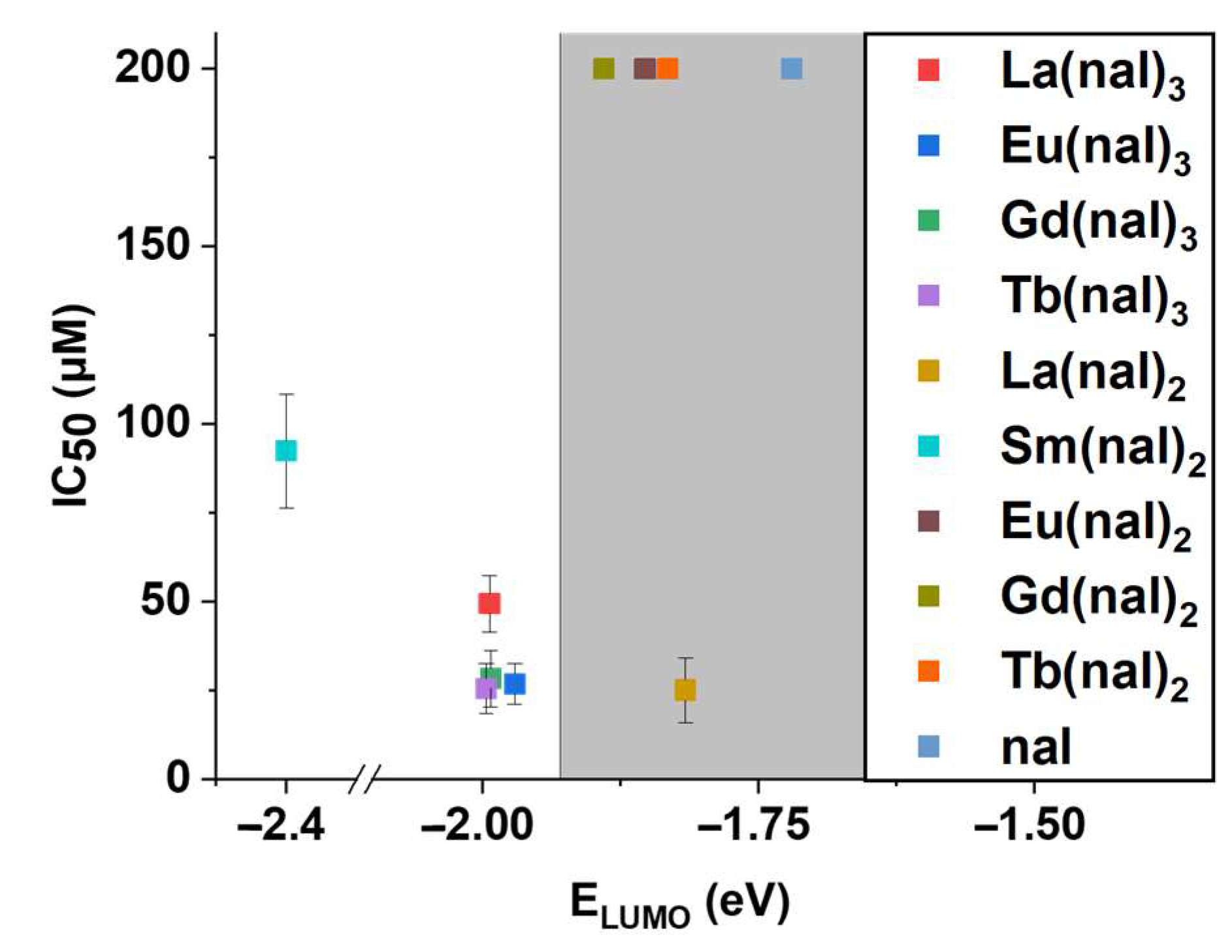

| Compound | IC50 (μM) | |
|---|---|---|
| LoVo | HUVEC | |
| Nalidixic acid | >200 | >200 |
| La(nal)2 | 25.08 ± 9.11 | 113.5 ± 46.79 |
| Sm(nal)2 | 92.39 ± 15.97 | >200 |
| Eu(nal)2 | >200 | >200 |
| Gd(nal)2 | >200 | >200 |
| Tb(nal)2 | >200 | >200 |
| La(nal)3 | 49.83 ± 7.92 | 148.8 ± 17.93 |
| Eu(nal)3 | 26.88 ± 5.72 | >200 |
| Gd(nal)3 | 28.36 ± 7.95 | 58.44 ± 29.32 |
| Tb(nal)3 | 25.55 ± 6.97 | 96.17 ± 48.80 |
| Cis-Pt | 38.55 ± 4.63 | 78.48 ± 16.03 |
| Compound | Kb (L∙mol−1) (×105) | KSV (M−1) (×103) | EB Displacement Assay | |
|---|---|---|---|---|
| K50 (µM) | n | |||
| Nalidixic acid | 4.30 ± 2.22 | 9.87 ± 0.11 | 50.41 ± 9.60 | 2.18 ± 0.30 |
| La(nal)2 | 0.61 ± 0.09 | 8.90 ± 0.17 | 31.39 ± 13.66 | 2.20 ± 0.81 |
| Sm(nal)2 | 2.18 ± 0.76 | 10.24 ± 0.22 | 31.35 ± 4.21 | 2.96 ± 0.77 |
| Eu(nal)2 | 0.49 ± 0.06 | 8.35 ± 0.13 | 34.75 ± 3.69 | 2.81 ± 0.38 |
| Gd(nal)2 | 0.80 ± 0.09 | 10.47 ± 0.13 | 34.11 ± 8.90 | 2.33 ± 0.72 |
| Tb(nal)2 | 0.37 ± 0.04 | 9.14 ± 0.16 | 34.69 ± 11.18 | 2.50 ± 0.96 |
| La(nal)3 | 0.75 ± 0.17 | 2.48 ± 0.44 | 36.45 ± 16.53 | 2.17 ± 0.99 |
| Eu(nal)3 | 3.84 ± 1.02 | 9.62 ± 0.10 | 30.96 ± 10.85 | 1.98 ± 0.95 |
| Gd(nal)3 | 4.17 ± 1.72 | 9.86 ± 0.19 | 31.88 ± 7.73 | 3.33 ± 1.43 |
| Tb(nal)3 | 2.37 ± 0.61 | 8.35 ± 0.11 | 31.96 ± 10.16 | 2.60 ± 1.21 |
| Complex | Ksv* (M−1) | Kq (M−1∙s−1) | Ka (M−1) | n1 (Number of Binding Sites) | Kd (μM) | n2 (Hill Coefficient) |
|---|---|---|---|---|---|---|
| Apo-Tf | ||||||
| Nal | (1.02 ± 0.09) × 105 (fa = 0.48 ± 0.03) | 1.02 × 1013 | (0.31 ± 0.08) × 104 | 0.79 ± 0.02 | 6.55 ± 2.11 | 1.55 ± 0.37 |
| La(nal)2 | (0.44 ± 0.23) × 105 (fa = 1.20 ± 0.49) | 0.44 × 1013 | (6.94 ± 2.32) × 104 | 1.00 ± 0.03 | 5.43 ± 1.46 | 1.82 ± 0.55 |
| Sm(nal)2 | (1.16 ± 0.06) × 105 (fa = 0.79 ± 0.02) | 1.16 × 1013 | (10.17 ± 6.14) × 104 | 1.01 ± 0.05 | 6.16 ± 1.27 | 1.71 ± 0.31 |
| Eu(nal)2 | (1.85 ± 0.06) × 105 (fa = 0.71 ± 0.01) | 1.85 × 1013 | (1.02 ± 0.13) × 104 | 0.80 ± 0.01 | 7.07 ± 6.13 | 0.82 ± 0.41 |
| Gd(nal)2 | (0.78 ± 0.09) × 105 (fa = 0.92 ± 0.07) | 0.78 × 1013 | (3.46 ± 1.34) × 104 | 0.94 ± 0.03 | 8.87 ± 6.20 | 1.20 ± 0.40 |
| Tb(nal)2 | (2.23 ± 0.15) × 105 (fa = 0.65 ± 0.02) | 2.23 × 1013 | (0.32 ± 0.08) × 104 | 0.71 ± 0.02 | 8.01 ± 4.30 | 0.84 ± 0.22 |
| La(nal)3 | (1.04 ± 0.13) × 105 (fa = 0.80 ± 0.06) | 1.04 × 1013 | (1.51 ± 0.72) × 104 | 0.86 ± 0.04 | 8.64 ± 2.22 | 1.04 ± 0.19 |
| Eu(nal)3 | (2.21 ± 0.13) × 105 (fa = 0.80 ± 0.02) | 2.21 × 1013 | (2.25 ± 1.01) × 104 | 0.84 ± 0.03 | 3.85 ± 0.29 | 1.58 ± 0.23 |
| Gd(nal)3 | (1.78 ± 0.07) × 105 (fa = 0.82 ± 0.02) | 1.78 × 1013 | (2.63 ± 0.39) × 104 | 0.87 ± 0.01 | 5.86 ± 0.72 | 1.22 ± 0.12 |
| Tb(nal)3 | (2.70 ± 0.11) × 105 (fa = 0.68 ± 0.01) | 2.70 × 1013 | (1.63 ± 0.42) × 104 | 0.83 ± 0.02 | 5.76 ± 2.60 | 0.98 ± 0.34 |
| HSA | ||||||
| Nal | (1.25 ± 0.06) × 105 (fa = 1.06 ± 0.02) | 1.25 × 1013 | (2.19 ± 0.46) × 105 | 1.03 ± 0.01 | 5.69 ± 1.44 | 1.33 ± 0.29 |
| La(nal)2 | (1.09 ± 0.02) × 105 (fa = 1.09 ± 0.02) | 1.09 × 1013 | (9.03 ± 1.04) × 105 | 1.09 ± 0.01 | 3.35 ± 0.11 | 1.32 ± 0.11 |
| Sm(nal)2 | (2.50 ± 0.12) × 105 (fa = 1.05 ± 0.02) | 2.50 × 1013 | (8.25 ± 1.62) × 105 | 1.08 ± 0.01 | 3.60 ± 0.11 | 1.34 ± 0.09 |
| Eu(nal)2 | (1.75 ± 0.11) × 105 (fa = 1.17 ± 0.04) | 1.74 × 1013 | (11.99 ± 1.38) × 105 | 1.13 ± 0.01 | 4.63 ± 0.97 | 1.14 ± 0.27 |
| Gd(nal)2 | (1.51 ± 0.07) × 105 (fa = 1.25 ± 0.03) | 1.51 × 1013 | (15.52 ± 2.93) × 105 | 1.15 ± 0.01 | 4.79 ± 0.84 | 1.20 ± 0.23 |
| Tb(nal)2 | (1.92 ± 0.08) × 105 (fa = 1.13 ± 0.02) | 1.92 × 1013 | (11.52 ± 2.87) × 105 | 1.12 ± 0.02 | 3.99 ± 0.14 | 1.52 ± 0.09 |
| La(nal)3 | (2.75 ± 0.10) × 105 (fa = 1.12 ± 0.01) | 2.75 × 1013 | (27.48 ± 2.10) × 105 | 1.16 ± 0.00 | 2.91 ± 0.07 | 1.18 ± 0.08 |
| Eu(nal)3 | (2.89 ± 0.11) × 105 (fa = 1.13 ± 0.01) | 2.89 × 1013 | (21.59 ± 2.04) × 105 | 1.13 ± 0.00 | 2.67 ± 0.21 | 1.17 ± 0.27 |
| Gd(nal)3 | (2.69 ± 0.05) × 105 (fa = 1.10 ± 0.00) | 2.69 × 1013 | (19.61 ± 1.83) × 105 | 1.14 ± 0.01 | 3.27 ± 0.11 | 1.35 ± 0.12 |
| Tb(nal)3 | (2.39 ± 0.10) × 105 (fa = 1.13 ± 0.02) | 2.39 × 1013 | (31.26 ± 9.66) × 105 | 1.18 ± 0.02 | 4.61 ± 1.67 | 0.89 ± 0.35 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maciuca, A.-M.; Munteanu, A.-C.; Mihaila, M.; Badea, M.; Olar, R.; Nitulescu, G.M.; Munteanu, C.V.A.; Uivarosi, V. A Study on Repositioning Nalidixic Acid via Lanthanide Complexation: Synthesis, Characterization, Cytotoxicity and DNA/Protein Binding Studies. Pharmaceuticals 2022, 15, 1010. https://doi.org/10.3390/ph15081010
Maciuca A-M, Munteanu A-C, Mihaila M, Badea M, Olar R, Nitulescu GM, Munteanu CVA, Uivarosi V. A Study on Repositioning Nalidixic Acid via Lanthanide Complexation: Synthesis, Characterization, Cytotoxicity and DNA/Protein Binding Studies. Pharmaceuticals. 2022; 15(8):1010. https://doi.org/10.3390/ph15081010
Chicago/Turabian StyleMaciuca, Ana-Madalina, Alexandra-Cristina Munteanu, Mirela Mihaila, Mihaela Badea, Rodica Olar, George Mihai Nitulescu, Cristian V. A. Munteanu, and Valentina Uivarosi. 2022. "A Study on Repositioning Nalidixic Acid via Lanthanide Complexation: Synthesis, Characterization, Cytotoxicity and DNA/Protein Binding Studies" Pharmaceuticals 15, no. 8: 1010. https://doi.org/10.3390/ph15081010
APA StyleMaciuca, A.-M., Munteanu, A.-C., Mihaila, M., Badea, M., Olar, R., Nitulescu, G. M., Munteanu, C. V. A., & Uivarosi, V. (2022). A Study on Repositioning Nalidixic Acid via Lanthanide Complexation: Synthesis, Characterization, Cytotoxicity and DNA/Protein Binding Studies. Pharmaceuticals, 15(8), 1010. https://doi.org/10.3390/ph15081010











