Laser‐Self‐Mixing Interferometry for Mechatronics Applications
Abstract
:1. Introduction
- -
- the x-axis is the direction of linear motion and any displacement along this axis will be indicated by Δx;
- -
- the y-axis is the direction of the straightness displacement indicated by Δy;
- -
- the z-axis is the direction of the flatness displacement indicated by Δz;
- -
- roll is the angular motion around the x-axis and any rotation around this axis will be indicated by Δϑx;
- -
- pitch is the angular motion around the y-axis and any rotation around this axis will be indicated by Δϑy;
- -
- yaw is the angular motion around the z-axis and any rotation around this axis will be indicated by Δϑz.
2. Laser-Self-Mixing Interference
2.1. Basic principle
2.2. Measurement of linear displacements
2.3. Role of the collimation condition of the laser beam
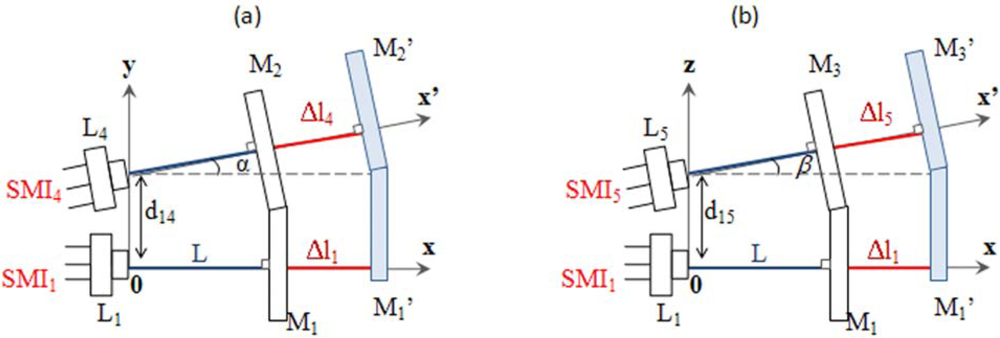
3. Measurement of yaw and pitch rotations by differential LSM
3.1. Basic principle
3.2. Experimental setup
3.3. Experimental results
3.4. Comparison with laboratory/commercial existing systems
- Operations in moderate feedback regime allow avoiding the signal instabilities typical of the coherence collapse and, above all, make possible a digital treatment of the signal in place of the analogical signal processing exploited in [25].
- In spite of a worse resolution, a larger angular measurement range (0.5° in place of the 0.06°) is achieved. On the other side, the system resolution can be improved by increasing the distance between the laser sources.
- The angular measurement can be performed simultaneously with long linear displacements.
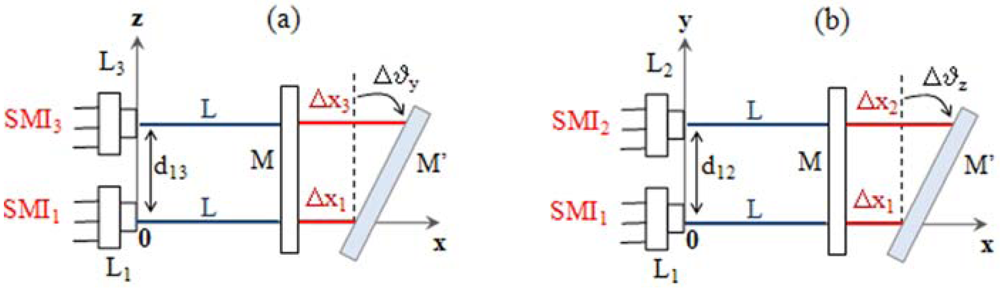
4. Measurement of transverse DOFs
4.2. Basic principle for straightness and flatness measurements by LSM
4.3. Basic principle for roll measurements
4.4 Experimental setup and results
5. System performances: measurement resolution and accuracy
- -
- the wavelengths of the six lasers are assumed comparable and equal to λ = 1.3 μm;
- -
- the distances between the laser diodes were d12 ≈ d13 = (50.0 ± 0.1) mm and d56 = (66.0 ± 0.1) mm;
- -
- the tilt angles of the mirrors Mi (i=2,3) are equal to α ≈ β = (2.1 ± 0.1)°.
- -
- the error σN about the total fringe count N = N+ - N-, due to the precision of the counting system. It is assumed σN = 2 due to the intrinsic uncertainty of ± 1 count for each electronic channel (uncompleted fringe);
- -
- the error σλ about the laser wavelength. This error has been obtained by continuously monitoring the wavelength with a wavelength meter allowing a relative accuracy σλ/λ = 10-5. However, analogous results can be realized via both the active temperature stabilization of the diode temperature to 0.01 °C or a temperature-calibrated DFB laser source [29];
- -
- the error σd about the distance between the diode lasers. By calibrating the LSM sensor against a reference angular meter, the distances dij can be evaluated as fitting parameters with an error of σd = 0.1 mm;
- -
- the error σα,β on the tilt angle between the diode lasers. After a preliminary calibrations, the tilt angle α ≈ β can be assessed as fitting parameters with an uncertainty of σα,β = 0.1°.
6. Simultaneous assessment of more DOFs
6.1. Experimental results
6.2. Mathematical algorithm
- to recover the 6 counts Ni once known the final target position t⃗\and orientation r̂j. In this case equation (16) represents a parametric system of 6 equations (i = 1..6) in 6 variables, which can be exactly solved;
- to recover the final target position t⃗ and orientation r̂j, once known the counts Ni. In this case equation (16) represents a parametric system of 6 equations (i = 1..6) in 12 scalar variables (4 variables, each having three Cartesian components x,y,z). The remaining six equations required to solve the system are provided by the 3 normalization conditions on the versors r̂j and the 3 equations describing their mutual orientation. In this way, a solvable system of 12 equations (i = 1..6) in 12 scalar variables can be obtained. The analytical complexity of such a system, often preventing it from being solved, is significantly reduced in presence of purely translational or rotational displacements.
7. Conclusions
Acknowledgments
References and Notes
- Lin, S.T. A laser interferometer for measuring straightness. Opt. Laser Technol. 2001, 33, 195–199. [Google Scholar]
- Shi, P.; Stijns, E. New optical method for measuring small-angle rotations. Appl. Opt. 1988, 27, 4342–4344. [Google Scholar]
- Zhang, J.H.; Menq, C.H. A linear/angular interferometer capable of measuring large angular motion. Meas. Sci. Technol. 1999, 10, 1247–1253. [Google Scholar]
- Wu, C.M.; Chuang, Y.T. Roll angular displacement measurement system with microradian accuracy. Sens. Actuat. A 2004, 116, 145–149. [Google Scholar]
- Fan, K.C.; Chen, M.J. A 6-degree-of-freedom measurement system for the accuracy of X-Y stage. Prec. Eng. 2000, 24, 15–23. [Google Scholar]
- Bae, E.W.; Kim, J.A.; Kim, S.H. Multi-degree-of-freedom displacement measurement system for milli-structures. Meas. Sci. Technol. 2001, 12, 1495–1502. [Google Scholar]
- Park, W.S.; Cho, H.S. Measurement of fine 6-degrees-of-freedom displacement of rigid bodies through splitting a laser beam: experimental investigation. Opt. Eng. 2002, 41, 860–871. [Google Scholar]
- Liu, C.H.; Jywe, W.Y.; Hsu, C.C.; Hsu, T.H. Development of a laser-based high-precision six-degrees-of-freedom motion errors measuring system for linear stage. Rev. Sci. Instrum. 2005, 76, 055110. [Google Scholar]
- Ottonelli, S.; De Lucia, F.; di Vietro, M.; Dabbicco, M.; Scamarcio, G.; Mezzapesa, F.P. A Compact Three Degrees – of - Freedom Motion Sensor Based on the Laser – Self - Mixing Effect. IEEE Phot. Technol. Lett. 2008, 20, 1360–1362. [Google Scholar]
- Ottonelli, S.; De Lucia, F.; Dabbicco, M.; Scamarcio, G. Simultaneous measurement of linear and transverse displacements by Laser – Self – Mixing. Appl. Opt. doc. ID 104307 (posted 9 February 2009, in press).
- Lang, R.; Kobayashi, K. External optical feedback effects on semiconductor injection laser properties. IEEE J. Quantum Electron 1980, 16, 347–355. [Google Scholar]
- de Groot, P.J.; Gallatin, G.M.; Macomber, S.H. Ranging and velocimetry signal generation in a backscatter-modulated laser diode. App. Opt. 1988, 27, 4475–4480. [Google Scholar]
- Giuliani, G.; Norgia, M.; Donati, S.; Bosch, T. Laser diode self-mixing technique for sensing applications. J. Opt. A: Pure Appl. Opt. 2002, 4, S283–S294. [Google Scholar]
- Acket, G.A.; Lenstra, D; Den Boef, J.; Verbeek, B.H. The influence of feedback intensity on longitudinal mode properties and optical noise in index-guided semiconductor lasers. IEEE J. Quantum Electron. 1984, 20, 1163–1169. [Google Scholar]
- Lenstra, D.; Verbeek, B.H.; den Boef, A.J. Coherence collapse in single-mode semiconductor lasers due to optical feedback. IEEE J. Quantum Electron. 1985, 21, 674–679. [Google Scholar]
- Donati, S.; Merlo, S. Application of diode laser feedback interferometry. J. Opt. 1998, 29, 156–161. [Google Scholar]
- Norgia, M.; Donati, S. A displacement-measuring instrument utilizing self-mixing interferometry. IEEE Trans. Instrum. Meas. 2003, 52, 1765–1770. [Google Scholar]
- Laroche, M.; Kervevan, L.; Gilles, H.; Girard, S.; Sahu, J.K. Doppler velocimetry using self-mixing effect in a short Er Yb-doped phosphate glass fiber laser. Appl. Phys. B 2005, 80, 603–607. [Google Scholar]
- Roos, P.A.; Stephens, M.; Wieman, C.E. Laser vibrometer based on optical-feedback-induced frequency modulation of a single-mode laser diode. Appl. Opt. 1996, 35, 6754–6761. [Google Scholar]
- Krehut, L.; Hast, J.; Alarousu, E. Low cost velocity sensor based on the self-mixing effect in a laser diode. Opto-Electron. Rev. 2003, 11, 313–319. [Google Scholar]
- Donati, S.; Giuliani, G.; Merlo, S. Laser diode feedback interferometer for measurement of displacements without ambiguity. IEEE J. Quantum Electron. 1995, 31, 113–119. [Google Scholar]
- Ottonelli, S.; De Lucia; di Vietro, M.; Dabbicco, M.; Scamarcio, G.; Mezzapesa, F.P.; Plantamura, M.C.; Polito, S.; Florio, C.; Ottonelli, F.P. System for laser measurement of target motion. Patent Pending, PCT/EP2008/005422, 2008. [Google Scholar]
- Ottonelli, S. Laser – Self – Mixing Interferometry for displacement sensors in mechatronics. PhD dissertation, University of Bari, Italy, 2009. [Google Scholar]
- Ottonelli, S.; De Lucia; di Vietro, M.; Dabbicco, M.; Scamarcio, G. Comparison of plane mirror vs retroreflector peformance for Laser-Self-Mixing displacement sensors. Proc. First Mediterranean Photonics Conference, Ischia, Italy; 2008; pp. 290–292. [Google Scholar]
- Giuliani, G.; Donati, D.; Passerini, M.; Bosch, T. Angle measurement by injection detection in a laser diode. Opt. Eng. 2001, 40, 95–99. [Google Scholar]
- Baldwin, W. Interferometer system for measuring straightness and roll. U.S. Patent. 3790284, 1974. [Google Scholar]
- Yamauchi, M.; Matsuda, K. Interferometric straightness measurement system using a holographic grating. Opt. Eng 1994, 33, 1078–1083. [Google Scholar]
- Zhang, J.; Cai, L. Interferometric straightness measurement system using triangular prism. Opt. Eng. 1998, 37, 1785–1789. [Google Scholar]
- Donati, S.; Falzoni, L.; Merlo, S. A PC-interfaced, compact laser-diode feedback interferometer for displacement measurements. IEEE Trans. Instrum. Meas. 1996, 45, 942–947. [Google Scholar]











© 2009 by the authors; licensee Molecular Diversity Preservation International, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Ottonelli, S.; Dabbicco, M.; De Lucia, F.; Di Vietro, M.; Scamarcio, G. Laser‐Self‐Mixing Interferometry for Mechatronics Applications. Sensors 2009, 9, 3527-3548. https://doi.org/10.3390/s90503527
Ottonelli S, Dabbicco M, De Lucia F, Di Vietro M, Scamarcio G. Laser‐Self‐Mixing Interferometry for Mechatronics Applications. Sensors. 2009; 9(5):3527-3548. https://doi.org/10.3390/s90503527
Chicago/Turabian StyleOttonelli, Simona, Maurizio Dabbicco, Francesco De Lucia, Michela Di Vietro, and Gaetano Scamarcio. 2009. "Laser‐Self‐Mixing Interferometry for Mechatronics Applications" Sensors 9, no. 5: 3527-3548. https://doi.org/10.3390/s90503527
APA StyleOttonelli, S., Dabbicco, M., De Lucia, F., Di Vietro, M., & Scamarcio, G. (2009). Laser‐Self‐Mixing Interferometry for Mechatronics Applications. Sensors, 9(5), 3527-3548. https://doi.org/10.3390/s90503527



