Synthesis Methods, Microscopy Characterization and Device Integration of Nanoscale Metal Oxide Semiconductors for Gas Sensing
Abstract
:1. Introduction
1.1. Metal Oxide Semiconductor
1.2. Advantages of Nanostructured Morphology
1.3. Crystal Structure
1.4. Nomenclature
2. Synthetic Methods
2.1. Overview
2.2. TEC for Nanowires and Nanobelts
2.3. Controlled Oxidation
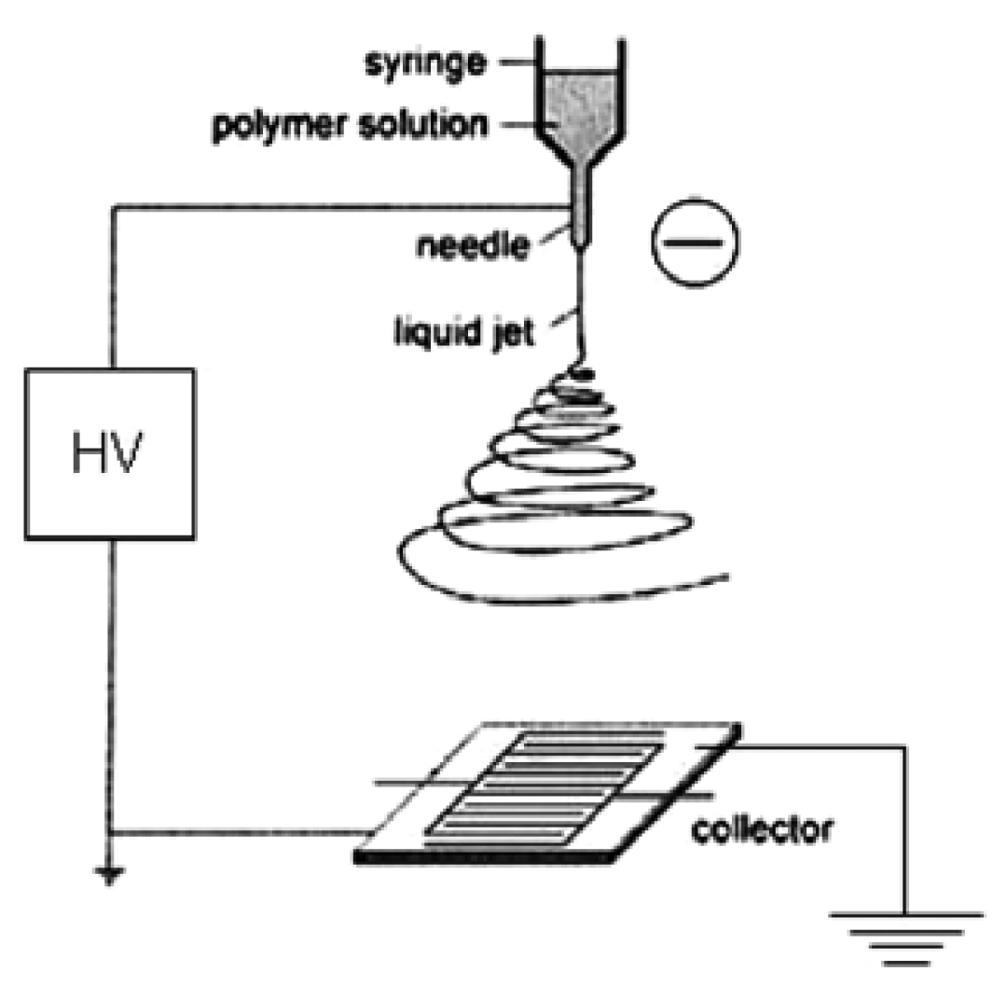
2.4. Electrospinning
3. Harvesting and Integration
3.1. Approaches
3.1.1. TEC
3.1.2. Controlled Oxidation
3.1.3. Electrospinning
3.2. Generic Dispersal and Alignment: Dielectrophoresis
3.3. Catalyst Activation of Metal Oxide Sensor Elements
4. Comparisons Between Methods
4.1. Overview
4.2. Limitations
4.2.1. TEC
4.2.2. Controlled Oxidation
4.2.3. Electrospinning
- The adherence of the nanofiber to the contact pads
- Required expertise to obtain correct viscosity of the polymer-sol gel solution as the electrospun solution
4.3. Advantages
4.3.1. TEC Nanowires
4.3.2. Controlled Oxidation
4.3.3. Electrospinning
5. Results
5.1. Synthesis
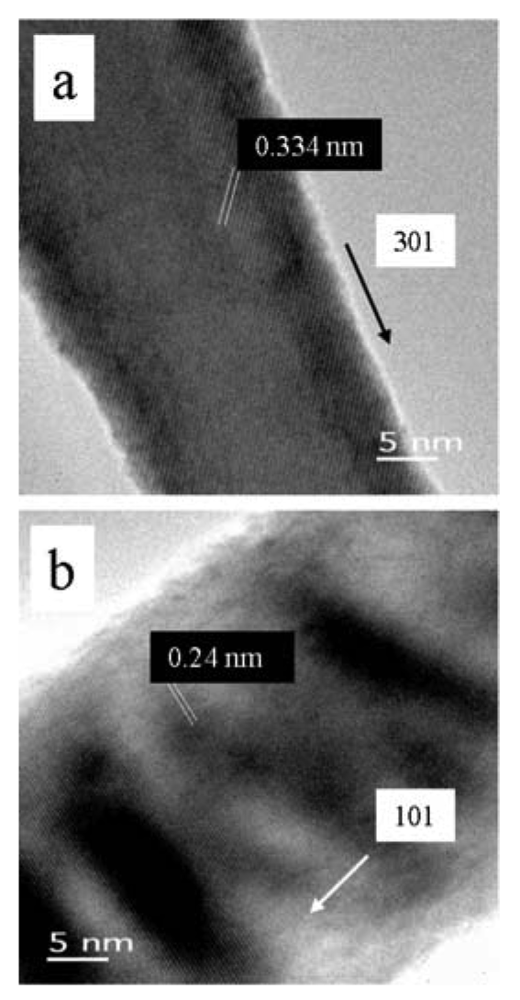
5.1.1. TEC
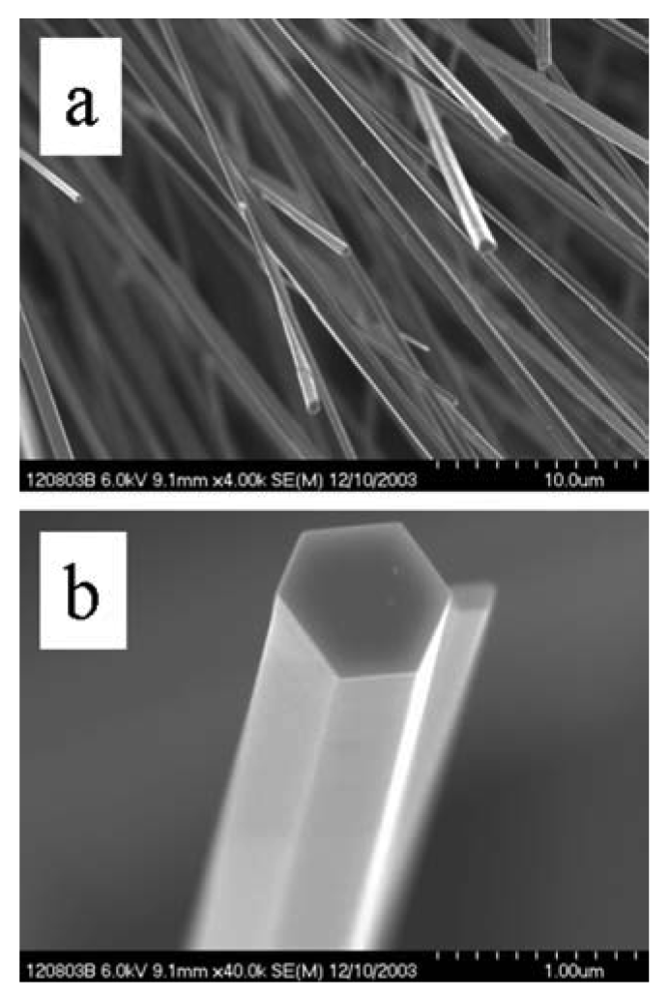
5.1.2. Controlled Oxidation
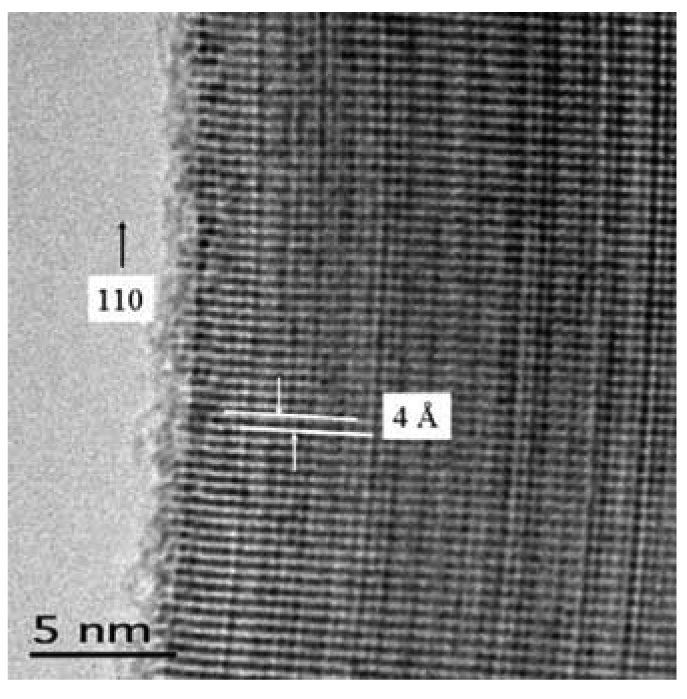
5.1.3. Electrospinning
5.2. Harvesting and Integration
5.3. Integration
5.4. Catalyst Deposition
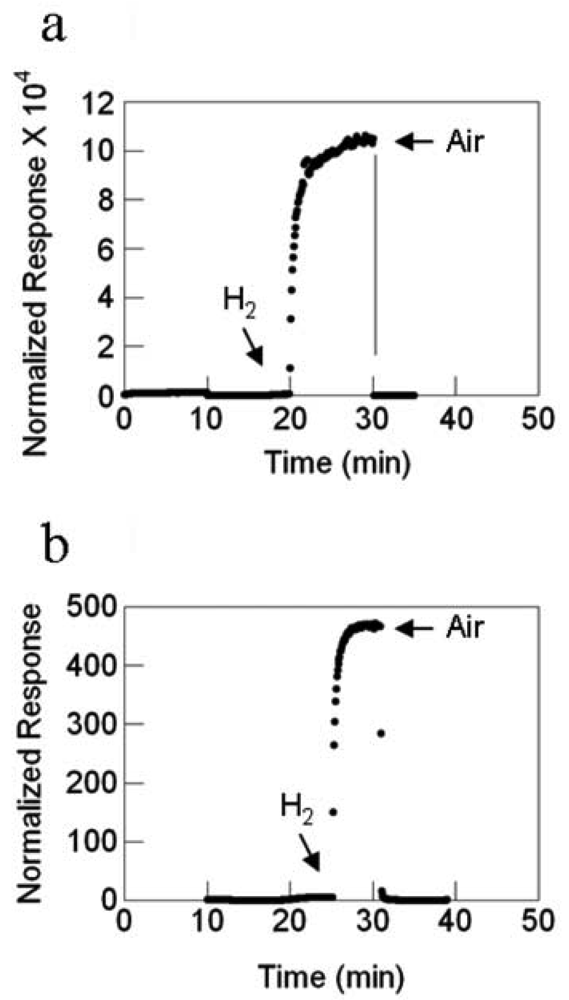
5.5. Testing Results
5.5.1. Analysis
5.5.2. TEC
5.5.3. Controlled Oxidation
5.5.4. Electrospinning
5.6. Comparative Catalyst-Oxide Systems
5.6.1. Overview
5.6.2. TiO2/Pt vs. SnO2/Pt
5.6.3. SnO2/Pt vs. SnO2/Pd
5.6.4. ZnO/Pd vs. SnO2/Pd
5.6.5. Catalyst Discussion
5.6.6. Activation Energy
6. Conclusions
Acknowledgments
References
- Hunter, G.W.; Liu, C.C.; Makel, D.D. MEMs Handbook Design and Fabrication, 2nd ed.; CRC Press LLC: Boca Raton, FL, USA, 2006; Chapter 11. [Google Scholar]
- Solid State Gas Sensing; Comini, E.; Faglia, G.; Sberveglieri, G. (Eds.) Springer-Verlag: New York, NY, USA, 2009.
- Rothschild, A.; Komem, Y. The Effect of Grain Size on the Sensitivity of Nanocrystalline Metal-Oxide Gas Sensors. J. Appl. Phys. 2004, 95, 6374–6380. [Google Scholar]
- Xia, Y.; Yang, P.; Sun, Y.; Wu, Y.; Mayers, B.; Gates, B.; Yin, Y.; Kim, F.; Yan, H. One-Dimensional Nanostructures: Synthesis, Characterization, and Applications. Adv. Mater. 2003, 15, 353–389. [Google Scholar]
- Franke, M.E.; Koplin, T.J.; Simon, U. Metal and Metal Oxide Nanoparticles in Chemiresitors: Does the Nanoscale Matter? Small. 2006, 2, 36–50. [Google Scholar]
- Kennedy, M.K.; Kruis, F.E.; Fissan, H. Tailored Nanoparticle Films from Monosized Tin Oxide Nanocrystals: Particle Synthesis, Film Formation, and Size-Dependent Gas-Sensing Properties. J. Appl. Phys. 2003, 93, 551–560. [Google Scholar]
- Klabunde, K.J. Nanoscale Materials in Chemistry; Wiley-Interscience: New York, NY, USA, 2001. [Google Scholar]
- Sysoev, V.V.; Goschnick, J.; Schneider, T.; Strelcov, E.; Kolmakov, A. A Gradient Microarray Electronic Nose Based on Percolating SnO2 Nanowire Sensing Elements. Nanoletters 2007, 7, 3182–3188. [Google Scholar]
- Li, D.; Wang, Y.; Xia, Y. Electrospinning of Polymeric and Ceramic Nanofibers as Uniaxially Aligned Arrays. Nanoletters 2003, 3, 1167–1171. [Google Scholar]
- Li, Q.H.; Wan, Q.; Liang, Y.X.; Wang, T.H. Electronic Transport through Individual ZnO Nanowires. Appl. Phys. Lett. 2004, 84, 4556–4558. [Google Scholar]
- Xu, C.X.; Sun, X.W.; Dong, Z.L.; Yu, M.B.; My, T.D.; Zhang, X.H.; Chua, S.J.; White, T.J. Zinc Oxide Nanowires and Nanorods Fabricated by Vapour-Phase Transport at Low Temperature. Nanotechnology 2004, 15, 839–842. [Google Scholar]
- Lyu, S.C.; Zhang, Y.; Ruh, H.; Lee, H.-J.; Shim, H.-W.; Suh, E.-K.; Lee, C.-J. Low Temperature Growth and Photoluminescence of Well-Aligned Zinc Oxide Nanowires. Chem. Phys. Lett. 2002, 363, 134–138. [Google Scholar]
- Cheng, G.; Wu, K.; Zhao, P.; Cheng, Y.; He, X.; Huang, K. Controlled Growth of Oxygen-Deficient Tin Oxide Nanostructures via a Solvothermal Approach in Mixed Solvents and Their Optical Properties. Nanotechnology 2007, 18, 1–7. [Google Scholar]
- Grundmann, M. The Physics of Semiconductors, An introduction including devices and nanophysics; Springer-Verlag: Berlin, Heidelberg, Germany, 2006. [Google Scholar]
- Metal Oxides: Chemistry and Applications; Fierro, J.L.G. (Ed.) CRC Press/Taylor & Francis: Boca Raton, FL, USA, 2006.
- Choi, Y.J.; Hwang, I.S.; Park, J.G.; Choi, K.J.; Park, J.H.; Lee, J.H. Novel Fabrication of an SnO2 Nanowire Sensor with High Sensitivity. Nanotechnology 2008, 19, 095508:1–095508:4. [Google Scholar]
- Barsan, N.; Koziej, D.; Weimar, U. Metal Oxide-Based Gas Sensor Research: How to? Sens. Actuat. B 2007, 121, 18–35. [Google Scholar]
- Barsan, N.; S-Berberich, M.; Gopel, W. Fundamental and Practical Aspects in the Design of Nanoscaled SnO2 Gas Sensors: A Status Report. Fresenius J. Anal. Chem. 1999, 365, 287–304. [Google Scholar]
- Comini, E. Metal oxide nano-crystals for gas sensing. Anal. Chim. Acta 2006, 568, 28–40. [Google Scholar]
- Nanowires and Nanobelts Materials, Properties and Devices, Metal and Semiconductor Nanowires; Wang, Z.L. (Ed.) Kluwer Academic Publishers: Dordrecht, The Netherlands, 2003; Vol. pp. 1–2.
- Meng, X.Q.; Shen, D.Z.; Zhang, J.Y.; Zhao, D.X.; Dong, L.; Lu, Y.M.; Liu, Y.C.; Fan, X.W. Photoluminescence Properties of Catalyst-Free Growth of Needle-like ZnO Nanowires. Nanotechnology 2005, 16, 609–612. [Google Scholar]
- Wu, R.; Xie, C. Formation of Tetrapod ZnO Nanowhiskers and Its Optical Properties. Mat. Res. Bull. 2004, 39, 637–645. [Google Scholar]
- Jiang, X.; Herricks, T.; Xia, Y. CuO Nanowires Can Be Synthesized by Heating Copper Substrates in Air. Nanoletters 2002, 2, 1333–1338. [Google Scholar]
- Peng, X.; Chen, A. Aligned TiO2 Nanorod Arrays Synthesized by Oxidizing Titanium with Acetone. J. Mat. Chem. 2004, 14, 2542–2548. [Google Scholar]
- Hong, K.; Xie, M.; Hu, R.; Wu, H. Synthesizing Tungsten Oxide Nanowires by a Thermal Evaporation Method. Appl. Phys. Lett. 2007, 90, 173121–173123. [Google Scholar]
- Qi, H.; Wang, C.; Liu, J. A Simple Method for the Synthesis of Highly Oriented Potassium-Doped Tungsten Oxide Nanowires. Adv. Mat. 2003, 15, 411–413. [Google Scholar]
- Fu, Y.Y.; Wang, R.M.; Xu, J.; Chen, J.; Yan, Y.; Narlikar, A.V.; Zhang, H. Synthesis of Large Arrays of Aligned α-Fe2O3 Nanowires. Chem. Phys. Lett. 2003, 379, 373–379. [Google Scholar]
- Wang, Y.; Aponte, M.; Leon, N.; Ramos, I.; Furlan, R.; Evoy, S.; Santiago-Aviles, J. Synthesis and Characterization of Tin Oxide Microfibres Electrospun from a Simple Precursor Solution. J. Semicond. Sci. Technol. 2004, 19, 1057–1060. [Google Scholar]
- He, H., Jr; Hsu, J.H.; Wang, C.W.; Lin, H.N.; Chen, L.J.; Wang, Z.L. Pattern and Feature Designed Growth of ZnO Nanowire Arrays for Vertical Devices. J. Phys. Chem. B 2006, 110, 50–53. [Google Scholar]
- Peng, X.; Wang, J.; Thomas, D.F.; Chen, A. Tunable Growth of TiO2 Nanostructures on Ti Substrates. Nanotechnology 2005, 16, 2389–2395. [Google Scholar]
- Vander Wal, R.L.; Berger, G.M.; Ticich, T.M.; Kulis, M.J.; Pushkarev, V. Comparison and Contrast of Synthesis and Integration of NanoScale Metal Oxide Semiconductors for Gas Sensing; NASA Contractor Report; NASA: Washington, DC, USA, 2008. [Google Scholar]
- An, L.; Cheam, D.D.; Friedrich, C.R. Controlled Dielectrophoretic Assembly of Multiwalled Carbon Nanotubes. J. Phys. Chem. C. 2009, 113, 37–39. [Google Scholar]
- Kolmakov, A.; Klenov, D.O.; Lilach, Y.; Stemmer, S.; Moskovits, M. Enhanced Gas Sensing by Individual SnO2 Nanowires and Nanobelts Functionalized with Pd Catalyst Particles. Nanoletters 2005, 5, 667–673. [Google Scholar]
- Vander Wal, R.L. Nanotechnology-Enabled Transducers for Sensing in Nanotechnology Enabled Sensing, Report of the National Nanotechnology Initiative; National Nanotechnology Initiative, Arlington, VA, USA, May 7–9, 2009; Ch. 2, p. 13.
- Liu, Y.; Liao, L; Li, J.; Pan, C. From Copper Nanocrystalline to CuO Nanoneedle Arrays: Synthesis, Growth Mechanism and Properties. Nanoletters 2007, 111, 5050–5056. [Google Scholar]
- Yang, P.; Wu, Y.; Fan, R. Inorganic Semiconductor Nanowires. Int. J. Nanosci. 2002, 1, 1–39. [Google Scholar]
- Wang, Z.L.; Pan, Z. Nanobelts of Semiconductive Oxides - A Structurally and Morphologically Controlled Nanomaterials System. Int. J. Nanosci. 2001, 1, 41–51. [Google Scholar]
- Pan, Z.W.; Dai, Z.R.; Wang, Z.L. Nanobelts of Semiconducting Oxides. Science 2001, 291, 1947–1949. [Google Scholar]
- Murphy, C.J.; Jana, N.R. Controlling the Aspect Ratio of Inorganic Nanorods and Nanowires. Adv. Mat. 2002, 14, 80–82. [Google Scholar]
- Duan, X.; Lieber, C.M. General Synthesis of Compound Semiconductor Nanowires. Adv. Mat. 2000, 12, 298–302. [Google Scholar]
- Hao, Y.; Meng, G.; Zhou, Y.; Kong, M.; Wei, Q.; Ye, M.; Zhang, L. Tuning the Architecture of MgO Nanostructures by Chemical Vapor Transport and Condensation. Nanotechnology 2006, 17, 5006–5012. [Google Scholar]
- Huo, K.; Zhang, X.; Hu, L.; Sun, X.; Fu, J.; Chu, P.K. One-Step Growth and Field Emission Properties of Quasi-Aligned TiO2 Nanowire/Carbon Nanocone Core-Shell Nanostructure Arrays on Ti Substrates. Appl. Phys. Lett. 2008, 93, 013105:1–013105:3. [Google Scholar]
- Wang, Z.L. Nanostructures of Zinc Oxide. Mat. Today 2004, 7, 26–33. [Google Scholar]
- Qazi, M.; Koley, G.; Park, S.; Vogt, T. NO2 Detection by Adsorption Induced Work Function Changes in In2O3 Thin Films. Appl. Phys. Lett. 2007, 91, 043113:1–043113:3. [Google Scholar]
- Korotcenkov, G.; Brinzari, V.; Boris, Y.; Ivanov, M.; Schwank, J.; Morante, J. Surface Pd Doping Influence on Gas Sensing Characteristics of SnO2 Thin Films Deposited by Spray Pyrolysis. Thin Solid Films 2003, 436, 119–126. [Google Scholar]
- Kolasinski, K.W. Surface Science, Foundations of Catalysis and NanoScience; John Wiley & Sons: Chichester, West Sussex, UK, 2002; Volume Chapter 3. [Google Scholar]
- Adamson, A.W.; Gast, A.P. Physical Chemistry of Surfaces, 6th ed.; John Wiley & Sons Inc.: New York, NY, USA, 1997; Volume Chapter 18. [Google Scholar]
- Sahm, T.; Gurlo, A.; Barsan, N.; Weimar, U. Basics of Oxygen and SnO2 Interaction; Work Function Change and Conductivity Measurements. Sens. Actuat. B 2006, 118, 78–83. [Google Scholar]
- Zhou, B.; Somorjai, G.A.; Hermans, S. Nanotechnology in Catalysis; Kluwer Academic Publishers: New York, NY, USA, 2004; Vol.1. [Google Scholar]




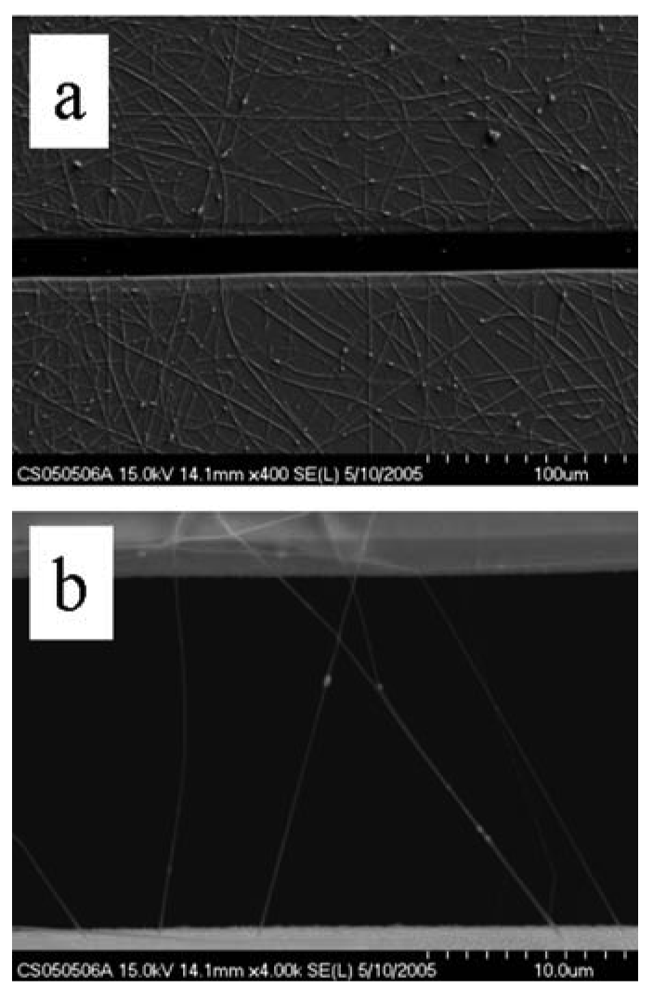





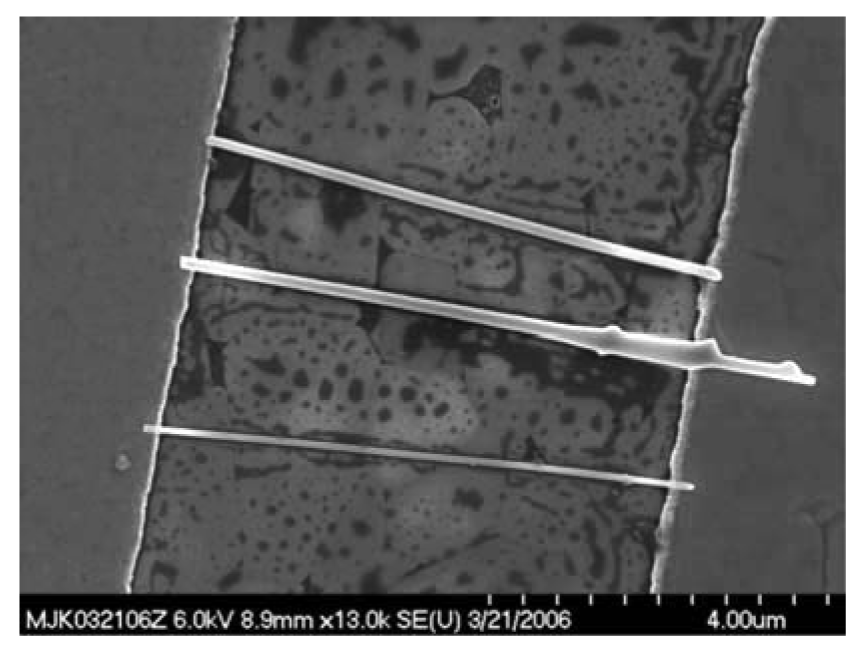
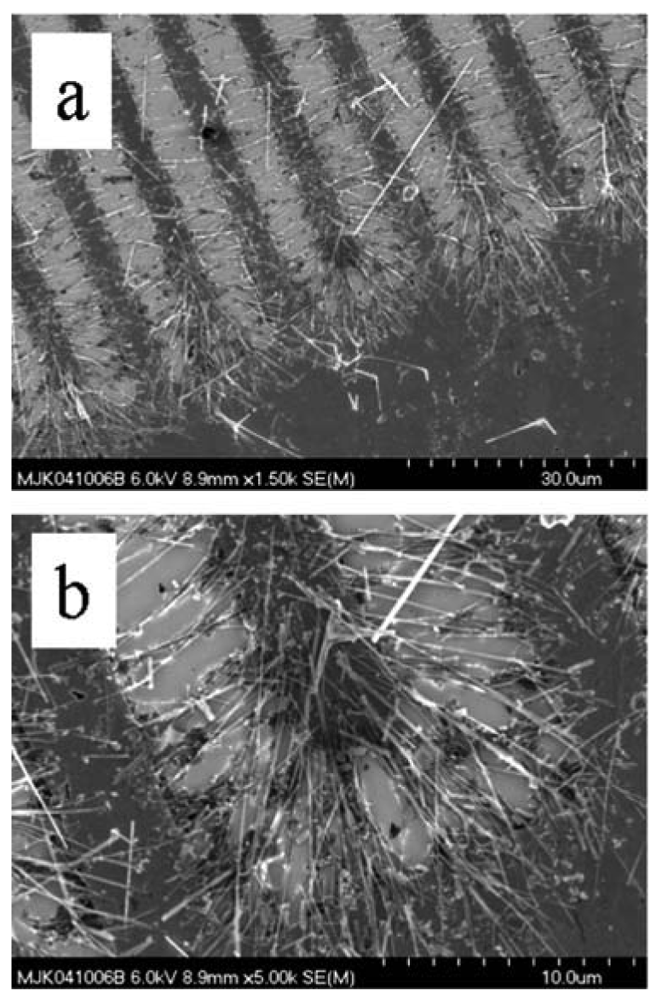










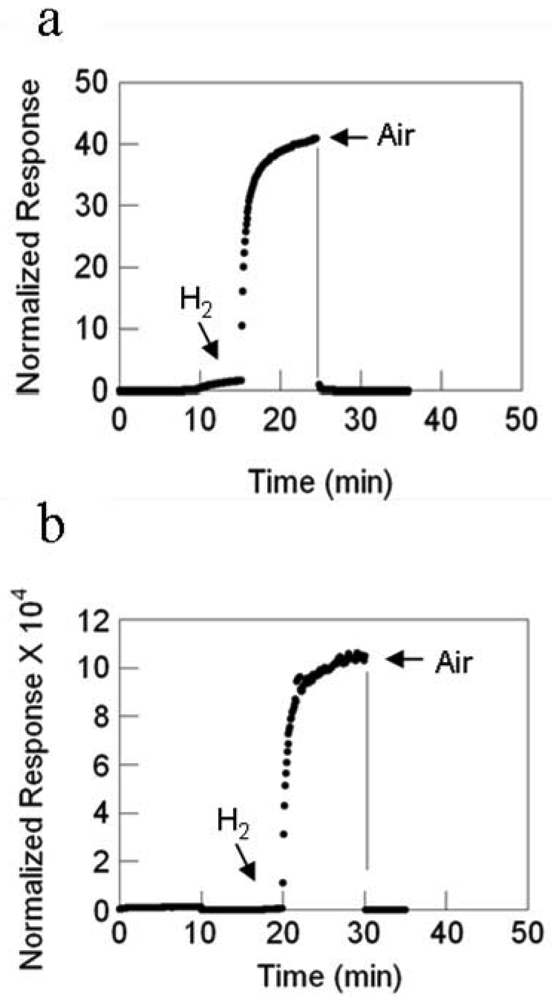

| Material | Normalized response | Rate constant, s–1 | Activation energy, kJ/mol | |
|---|---|---|---|---|
| TiO2/Pt | 4.08×101 | 2.23×10–2 | 7.1 | |
| TiO2/Pd | 1.5 | 3.13×10–2 | N/Aa | |
| SnO2/Pt | 1.04×105 | 2.27×10–2 | 4.7 | |
| SnO2/Pd | 4.68×102 | 5.10×10–2 | 17.7 | |
| ZnO/Pt | 1.90×101 | 1.80×10–2 | N/Aa | |
| ZnO/Pd | 2.21×101 | 7.00×10–3 | 3.3 | |
© 2009 by the authors; licensee Molecular Diversity Preservation International, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Vander Wal, R.L.; Berger, G.M.; Kulis, M.J.; Hunter, G.W.; Xu, J.C.; Evans, L. Synthesis Methods, Microscopy Characterization and Device Integration of Nanoscale Metal Oxide Semiconductors for Gas Sensing. Sensors 2009, 9, 7866-7902. https://doi.org/10.3390/s91007866
Vander Wal RL, Berger GM, Kulis MJ, Hunter GW, Xu JC, Evans L. Synthesis Methods, Microscopy Characterization and Device Integration of Nanoscale Metal Oxide Semiconductors for Gas Sensing. Sensors. 2009; 9(10):7866-7902. https://doi.org/10.3390/s91007866
Chicago/Turabian StyleVander Wal, Randy L., Gordon M. Berger, Michael J. Kulis, Gary W. Hunter, Jennifer C. Xu, and Laura Evans. 2009. "Synthesis Methods, Microscopy Characterization and Device Integration of Nanoscale Metal Oxide Semiconductors for Gas Sensing" Sensors 9, no. 10: 7866-7902. https://doi.org/10.3390/s91007866
APA StyleVander Wal, R. L., Berger, G. M., Kulis, M. J., Hunter, G. W., Xu, J. C., & Evans, L. (2009). Synthesis Methods, Microscopy Characterization and Device Integration of Nanoscale Metal Oxide Semiconductors for Gas Sensing. Sensors, 9(10), 7866-7902. https://doi.org/10.3390/s91007866




