An Automatic Instrument to Study the Spatial Scaling Behavior of Emissivity
Abstract
:1. Introduction
2. Methodology
- 1)
- putting the sample and the reference box in the steel plate and opening the thermal camera;
- 2)
- under the driving of horizontal rotating equipments, the cloth chamber is situated over the reference box;
- 3)
- under the drive of vertical moving equipments, the cloth chamber is stretched up and the ‘hot’ environment is made, after one second, the first measurement Mr, 8–14 μm (T1) is performed;
- 4)
- switching the measured object from reference box to the sample by horizontal moving equipments, the second measurement Mr, 8–14 μm (T2) is completed;
- 5)
- dropping the cloth chamber and rotating it off the sample by vertical moving equipments and horizontal rotating equipments as fast as possible, after one second, the third measurement Mr, 8–14 μm (T3) is obtained;
- 6)
- switching the measured object from the sample to reference box by horizontal moving equipments, the fourth measurement Mr, 8–14 μm (T4) is acquired.
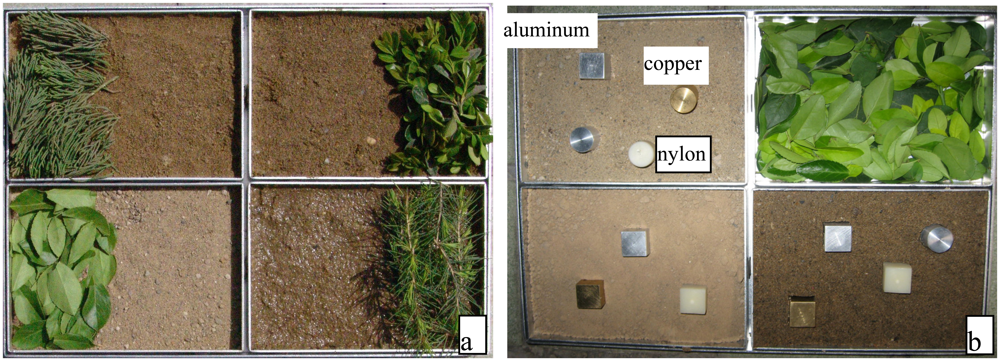
3. Emissivity Measurements
4. Analysis of Sensitivity in the Method
- (1)
- M, the measured apparent radiance. The uncertainty in M is primarily from the thermal camera itself. Using the blackbody as the sample, we analyzed the characteristics of the thermal camera. Note that, in the measurements, although an auto-adjust function for correcting the temperature drift was set, there still was 4k drift errors at most in one regulating period, moreover, we found that there was almost no error for the central part of the thermal image, while with the increase of the distance from the central, the error increased and can reach 4k, that is to say, there are large errors for the pixels around the edge of the thermal image. According to the magnitude of the errors, the thermal image can be grouped into three regions as shown in Figure.5. The percentage (I) of drift error less than Ik was about 50% and the percentage (II and III) of the other two groups was about 25%, respectively. In order to reduce this error, in terms of the view field of the camera, we installed it at height of 1.3m to make the sample locate in the region of I and II. At the same time, we also calibrated the thermal camera using the blackbody in the experiments.
- (2)
- Tenvi, environmental temperature, is calculated from Eq.(2) and Eq.(5). Obviously, Tenvi is determined by the emitting radiance of target object B8_14 μm (Tr), the radiance from the surface Mr and the emissivity of reference box εr according to Eq.(2) or Eq.(5). In terms of our simulations, it is found that ambient radiance is more sensitive to B8_14 μm (Tr) and Mr for the reference box of high emissivity than that for the reference box of low emissivity. Table 1 shows the simulated results. The left four columns represent the variation of Tenvi with Trad, for different εr and Tr = 300.71. In this case, it can be seen that 0.1k variation in the value of Trad results in variations of approximately 4k, 0.4k, 0.15k in Tenvi for different surface emissivities, respectively, calculated with Eq.(2). That is to say, for high surface emissivity, such as reference box painted black lacquer, only a little variation of Trad would induce large variation of Tenvi. The right four columns represent the variation of Tenvi with Tr, we can see that for high surface emissivity, a 0.5k increase in the value of Tr produced about 9k decrease of Tenvi, but for low surface emissivity (0.3), only a 0.2 decrease of Tenvi was generated. Above all, it can be concluded that Tenvi is more sensitive to Trad and Tr for high surface emissivity than that for low surface emissivity. For this reason, in the experiment, low surface emissivity should be adopted to calculate the ambient radiance. Because the half upper surface emissivity of our reference box is painted by black lacquer with high emissivity about 0.98, we used its undersurface to retrieve the ambient radiance, with an emissivity value of εr≈ 0.3, seen from Figure. 3.In addition, as the above section said, the radiance from the environment exhibits great heterogeneous, so only using the average ambient radiance to retrieve emissivity must produce some errors. For quantitatively evaluating and clarifying this effect on the calculation of emissivity, we specially did the following analysis based on our experimental data. Table 2 shows the average Tenvi and the standard deviation of it in ‘hot’ environment and in ‘cool’ environment, respectively, for three groups of experimental data. Here, Tenvi of every pixel and its average were obtained by the method mentioned in the above section. Obviously, the results show that Tenvi in ‘hot’ environment exhibits more homogeneous than that in ‘cool’ environment, especially for group1, the standard deviation of Tc reaches 9 degree. Hence, we conclude that using the average Tenvi to calculate emissivity other than Tenvi of every pixel will induce some errors. Table 3 quantitatively describes the absolute emissivity difference between the two methods of calculating Tenvi for aluminum surface, vegetation surface, dry agricultural soil surface, dry sandy soil surface and wet sandy soil surface, respectively. It is clear that emissivity difference of aluminum is obvious higher than the other four objects. In fact, the difference of vegetation, agricultural soil and wet sandy soil almost can be ignored in the applications because small variation of emissivity (less than 0.01) which basically has no influence on the retrieval of surface temperature. From Table 3, we can conclude that the effects of different Tenvi values on emissivity calculation are enormously larger for low emissivity objects than that for high emissivity objects, which also represents the effects of the heterogeneity of ambient radiance on the emissivity retrieval. Therefore, in the case that the sample is of low emissivity, it must adopt Tenvi of every pixel to compute emissivity of every pixel. Otherwise, for the sample with high emissivity (larger than 0.94), either method would be fine.
- (3)
- εr, the emissivity of reference box. In the calculation of emissivity, εr is mainly used to retrieve ambient radiance Tenvi, consequently, affects the retrieval of sample's emissivity. Integrating Eq.(2) and Eq.(5) into Eq.(6), we can analyze the relationship between εr, Tenvi and the emissivity of the sample. Figure.6 shows the simulated results. Clearly, under the conditions that M1, M2, M3, M4 and Tr are constant, the difference of environmental temperature (Th–Tc) increases as εr increases,accordingly, the calculated emissivities εs also increase. For the sample with higher emissivity about 0.95, the results show little change. An variation of 0.01 in εr only results in 0.00066 change of εs. Comparatively, 0.01variation in εr causes 0.0088 change of εs for the sample with lower emissivity about 0.33, which is thirteen times than the former case. Therefore, higher attention should be paid when target object of low emissivity is measured and in this case, εr should be determined precisely. While in common cases that the samples are soils or vegetations, the effects result from the small error of εr can be ignored in the applications. Using the experimental data, Table 4 was obtained, which exhibited good consistence with the above conclusion, that is to say, εr has larger effects on objects of low emissivity.
- (4)
- Ts, the true temperature of the sample. As the above said, the equation for calculating emissivity (Eq.6) is build on the assumption of T2 = T3. Although time difference between the second and the third measurement is very short about 5s, because the temperature of sample and that of the environment are different, there still exists energy exchange between them before reaching the status of energy equilibrium. Thus, Ts2 ≠ Ts3 in reality, and Eq.(6) must be modified to get more correct emissivity. Here, the method for correcting emissivity presented by Zhang (2004) was adopted. More details can be found in that paper. In terms of the method, one additional measurement was taken outside the cloth chamber before the measurement of M1. The measured radiance was called as M0, which can be expressed by
5. Ideal Temperature Difference between Hot Environment and Cool Environment
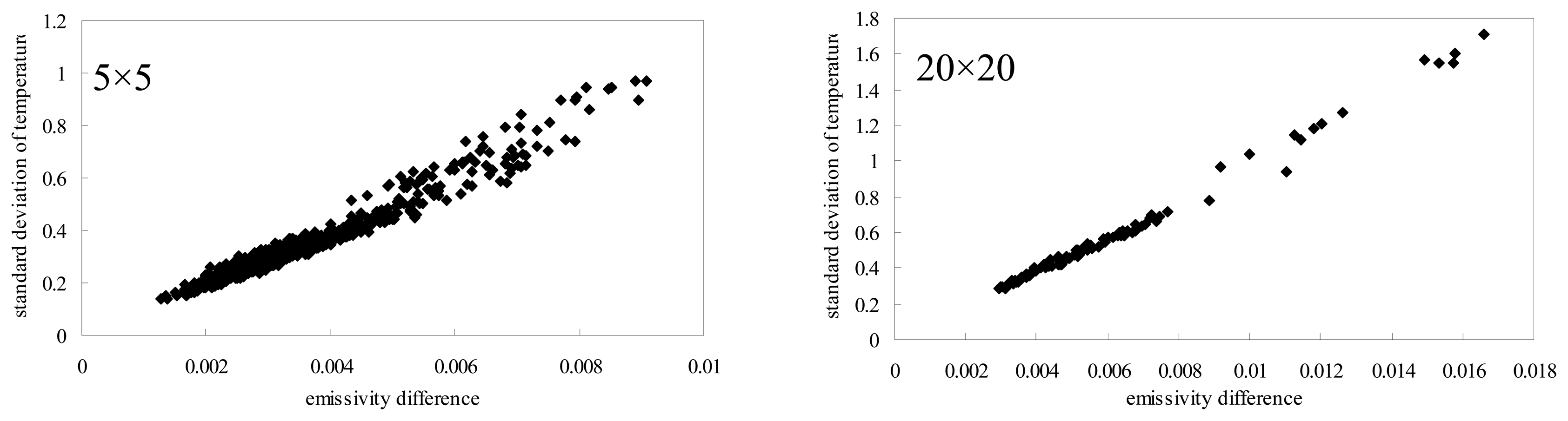
6. Scaling Effects of Emissivity Retrievals
7. Summary and Conclusions
Acknowledgments
References and Notes
- Buettner, C.D.; Kern, K.J.K. The determination of infrared of emissivities of terrestrial surface. Journal of Geophysical Research 1965, 70, 1327–1337. [Google Scholar]
- Barducci, A.; Pippi, I. Temperature and emissivity retrieval from remotely sensed images using the “grey body emissivity” method. IEEE Trans. Geosci. Remote Sens 1996, 34, 681–695. [Google Scholar]
- Becker, F. The impact of spectral emissivity on the measurement of land surface temperature from a satellite. International Journal of Remote Sensing 1987, 10, 1509–1522. [Google Scholar]
- Becker, F.; Li, Z.L. Temperature-independent spectral indices in thermal infrared bands. Remote Sensing of Environment 1990, 32, 17–33. [Google Scholar]
- Becker, F.; Li, Z.L. Surface temperature and emissivity at various scales: definition measurement and related problems. Remote Sensing Reviews 1995, 12, 225–253. [Google Scholar]
- Brunsell, N.A.; Gillies, R.R. Length scale analysis of surface energy fluxes derived from remote sensing. Journal of Hydrometeorology 2003, 4, 1212–1259. [Google Scholar]
- Coll, C.; et al. On the Atmospheric Dependence of the Split-Window Equation for Land-Surface Temperature. Int J Remote Sens 1994, 15, 105–122. [Google Scholar]
- Gillespie, A.R.; Rokugawa, S.; Matsunaga, T.; Gothern, J.S.; Hook, S.; Kahle, A.B. A temperature and emissivity separation algorithm for advanced spaceborne thermal emission and reflection radiometer (ASTER) images. IEEE Trans. Geosci. Remote Sens 1998, 36, 1113–1126. [Google Scholar]
- Li, X.W.; Strahler, A.; Fried, M. A conceptual model for effective directional emissivity for non isothermal surface. IEEE Trans. Geosci. Remote Sens 1999, 37, 2508–2517. [Google Scholar]
- Li, Z.L.; Becker, F.; Stoll, M.P.; Wan, Z.M. Evaluation of six methods for extracting relative emissivity spectral from thermal infrared images. Remote Sensing of Environment 1999, 69, 197–214. [Google Scholar]
- Liu, Y.B.; Hiyama, T.; Yamaguchi, Y. Scaling of land surface temperature using satellite data: A case examination on ASTER and MODIS products over a heterogeneous terrain area. Remote Sensing of Environment 2006, 105, 115–128. [Google Scholar]
- Moran, M.S.; Humes, K.S.; Printer, P.J., Jr. The scaling characteristics of remotely sensed variables for sparsely-vegetated heterogeneous landscapes. Journal of Hydrology 1997, 190, 337–362. [Google Scholar]
- Nerry, F.; Labed, J.; Stoll, M.P. Emissivity signatures in the thermal infrared band for remote sensing: calibration procedure and method of measurement. Applied Optics 1988, 27, 758–764. [Google Scholar]
- Nerry, F.; Labed, J.; Stoll, M.P. Spectral properties of land surfaces in the thermal infrared 1. Laborataory measurements of absolute spectral emissivity signatures. Journal of Geophysical Research 1990, 95, 7027–7043. [Google Scholar]
- Nerry, F.; Stoll, M.P.; Kologo, N. Scattering of a CO2 laser beam at 10.6um by bare soils: experimental study of the polarized bidirectional scattering coefficient; model and comparison with directional emissivity measurements. Applied Optics 1991, 30, 3984–3994. [Google Scholar]
- Norman, J.M.; Becker, F. Terminology in thermal infrared remote sensing of natural surface. Agriculture and Forest Meteorology 1995, 77, 153–176. [Google Scholar]
- Qin, Z.H.; et al. Derivation of split window algorithm and its sensitivity analysis for retrieving land surface temperature from NOAA-advanced very high resolution radiometer data. J Geophys Res-Atmos 2001, 106, 22655–22670. [Google Scholar]
- Rivard, B.; Thomas, P.J.; Giroux, J. Precise emissivity of rock samples. Remote Sensing of Environment 1995, 54, 152–160. [Google Scholar]
- Rubio, E.; Caselles, V.; Badenas, C. Emissivity Measurements of Several Soils and Vegetation Types in the 8-14μm Wave Band: Analysis of Two Field Methods. Remote Sensing of Environment 1997, 59, 490–521. [Google Scholar]
- Sobrino, J.A.; Caselles, V. A field method for estimating the thermal infrared emissivity. ISPRS Journal of Photogrammetry and Remote Sensing 1993, 48, 24–31. [Google Scholar]
- Su, H.B.; et al. Thermal model for discrete vegetation and its solution on pixel scale using computer graphics. Sci. China Ser. E 2000a, 43, 48–54. [Google Scholar]
- Su, H.B.; et al. Determination of the effective emissivity for the regular and irregular cavities using Monte-Carlo method. Int. J. Remote Sens 2000b, 21, 2313–2319. [Google Scholar]
- Su, H.B.; et al. Modeling evapotranspiration during SMACEX: Comparing two approaches for local- and regional-scale prediction. J. Hydrometeorol 2005, 6, 910–922. [Google Scholar]
- Su, H.; et al. Evaluation of remotely sensed evapotranspiration over the CEOP EOP-1 reference sites. J. Meteorol. Soc. Jpn 2007, 85A, 439–459. [Google Scholar]
- Wan, Z.M.; Li, Z.L. A physics-based algorithm for retrieving land-surface emissivity and temperature from EOS/MODIS data. IEEE Trans. Geosci. Remote Sens 1997, 35, 980–996. [Google Scholar]
- Wan, Z.M.; Zhang, Y.; Zhang, Q.; Li, Z.L. Validation of the land-surface temperature products retrieved from Terra Moderate Resolution Imaging Spectroradiometer data. Remote Sensing of Environment 2002, 83, 163–180. [Google Scholar]
- Watson, K. Two-temperature method for measuring emissivity. Remote Sensing of Environment 1992a, 42, 117–121. [Google Scholar]
- Zhang, R.H. A proposed approach to determine the infrared emissivities of terrestrial surfaces from airborne or spaceborne platforms. International Journal of Remote Sensing 1989, 3, 591–595. [Google Scholar]
- Zhang, R.H.; Li, Z.L.; Tang, X.Z.; Sun, X.M.; Su, H.B.; Zhu, Z.L. Study of Emissivity Scaling and Relativity of Homogeneity of Surface Temperature. International Journal of Remote Sensing 2004, 25, 245–259. [Google Scholar]





| Trad | Tenvi(Tr= 300.71) | Tr | Tenvi(Trad= 301.15) | ||||
|---|---|---|---|---|---|---|---|
| εr= 0.98 | εr = 0.75 | εr = 0.3 | εr = 0.98 | εr = 0.75 | εr = 0.3 | ||
| 301.15 | 320.8532 | 302.4712 | 301.3398 | 296.5 | 424.7418 | 313.9419 | 303.0786 |
| 301.25 | 324.9119 | 302.8654 | 301.4824 | 297 | 416.128 | 312.6673 | 302.8772 |
| 301.35 | 328.8277 | 303.2585 | 301.6249 | 297.5 | 406.8952 | 311.3704 | 302.6745 |
| 301.45 | 332.6122 | 303.6504 | 301.7673 | 298 | 396.9354 | 310.0504 | 302.4703 |
| 301.555 | 336.2753 | 304.0412 | 301.9097 | 298.5 | 386.1071 | 308.7065 | 302.2646 |
| 301.65 | 339.8259 | 304.4309 | 302.052 | 299 | 374.2201 | 307.3379 | 302.0575 |
| 301.75 | 343.272 | 304.8195 | 302.1943 | 299.5 | 361.0087 | 305.9438 | 301.8489 |
| 301.85 | 346.6206 | 305.207 | 302.3365 | 300 | 346.0837 | 304.5232 | 301.6388 |
| Measuring Date | Data group | average_Th | std_Th | average_Tc | std_Tc |
|---|---|---|---|---|---|
| 11/8/2007 | Group1 | 306.83 | 0.63 | 256.81 | 9.12 |
| 303.74 | 0.79 | 252.21 | 9.49 | ||
| 303.79 | 0.63 | 252.02 | 8.93 | ||
| 302.77 | 0.52 | 251.17 | 8.78 | ||
| 20/7/2007 | Group2 | 303.33 | 0.41 | 270.87 | 5.72 |
| 303.28 | 0.39 | 270.31 | 5.68 | ||
| 302.93 | 0.39 | 269.84 | 5.75 | ||
| 302.77 | 0.39 | 269.45 | 5.81 | ||
| Group3 | 300.88 | 0.44 | 268.45 | 6.11 | |
| 300.91 | 0.45 | 268.12 | 6.09 | ||
| 300.81 | 0.47 | 267.98 | 6.17 | ||
| 300.62 | 0.5 | 267.54 | 6.21 |
| Objects | Min | Max | Mean | Stdev | Average emissivity |
|---|---|---|---|---|---|
| aluminum | 0.019 | 0.17 | 0.12 | 0.029 | 0.285 |
| vegetation | 0.00001 | 0.0022 | 0.0003 | 0.0003 | 0.981 |
| dry sandy soil | 0.0049 | 0.0149 | 0.0094 | 0.0017 | 0.940 |
| dry agricultural soil | 0.0001 | 0.0077 | 0.0037 | 0.0016 | 0.945 |
| wet sandy soil | 0.000008 | 0.0072 | 0.0021 | 0.0017 | 0.964 |
| Average emissivity | |||||
|---|---|---|---|---|---|
| εr | aluminum | vegetation | dry sandy soil | dry agricultural soil | wet sandy soil |
| 0.3 | 0.285 | 0.9812 | 0.940 | 0.945 | 0.964 |
| 0.32 | 0.305 | 0.9815 | 0.942 | 0.947 | 0.965 |
| 0.34 | 0.326 | 0.9817 | 0.944 | 0.949 | 0.966 |
| 0.36 | 0.346 | 0.9819 | 0.945 | 0.95 | 0.967 |
| 0.38 | 0.366 | 0.9822 | 0.947 | 0.952 | 0.968 |
| 0.4 | 0.387 | 0.9825 | 0.949 | 0.953 | 0.969 |
| Min | Max | Mean | Std | |
|---|---|---|---|---|
| aluminum | 0.0 | 0.0063 | 0.0019 | 0.0014 |
| vegetation | 0.0 | 0.0273 | 0.0035 | 0.0031 |
| dry sandy soil | 0.0 | 0.0279 | 0.0032 | 0.0046 |
| dry agricultural soil | 0.0 | 0.0104 | 0.0021 | 0.0017 |
| wet sandy soil | 0.0 | 0.0257 | 0.0032 | 0.0030 |
| εs | Th-Tc | ||
|---|---|---|---|
| ΔT=0.5 | ΔT =1.0 | ΔT =1.5 | |
| 0.98 | 22.421 | 41.036 | 57.097 |
| 0.96 | 11.814 | 22.471 | 32.212 |
| 0.94 | 8.026 | 15.506 | 22.522 |
| 0.85 | 3.287 | 6.486 | 9.604 |
| 0.75 | 1.985 | 3.941 | 5.870 |
| 0.45 | 0.907 | 1.810 | 2.710 |
| 0.3 | 0.713 | 1.425 | 2.136 |
| 0.2 | 0.624 | 1.248 | 1.871 |
| 0.07 | 0.537 | 1.074 | 1.612 |
© 2008 by MDPI Reproduction is permitted for noncommercial purposes.
Share and Cite
Tian, J.; Zhang, R.; Su, H.; Sun, X.; Chen, S.; Xia, J. An Automatic Instrument to Study the Spatial Scaling Behavior of Emissivity. Sensors 2008, 8, 800-816. https://doi.org/10.3390/s8020800
Tian J, Zhang R, Su H, Sun X, Chen S, Xia J. An Automatic Instrument to Study the Spatial Scaling Behavior of Emissivity. Sensors. 2008; 8(2):800-816. https://doi.org/10.3390/s8020800
Chicago/Turabian StyleTian, Jing, Renhua Zhang, Hongbo Su, Xiaomin Sun, Shaohui Chen, and Jun Xia. 2008. "An Automatic Instrument to Study the Spatial Scaling Behavior of Emissivity" Sensors 8, no. 2: 800-816. https://doi.org/10.3390/s8020800
APA StyleTian, J., Zhang, R., Su, H., Sun, X., Chen, S., & Xia, J. (2008). An Automatic Instrument to Study the Spatial Scaling Behavior of Emissivity. Sensors, 8(2), 800-816. https://doi.org/10.3390/s8020800





