Acoustic Fabry–Perot Resonance Detector for Passive Acoustic Thermometry and Sound Source Localization
Abstract
1. Introduction
2. Principles of ATM and SSL
2.1. AFPRD Model and Resonance Principle
2.2. Sound Velocity and Temperature Measurement Based on AFPRD
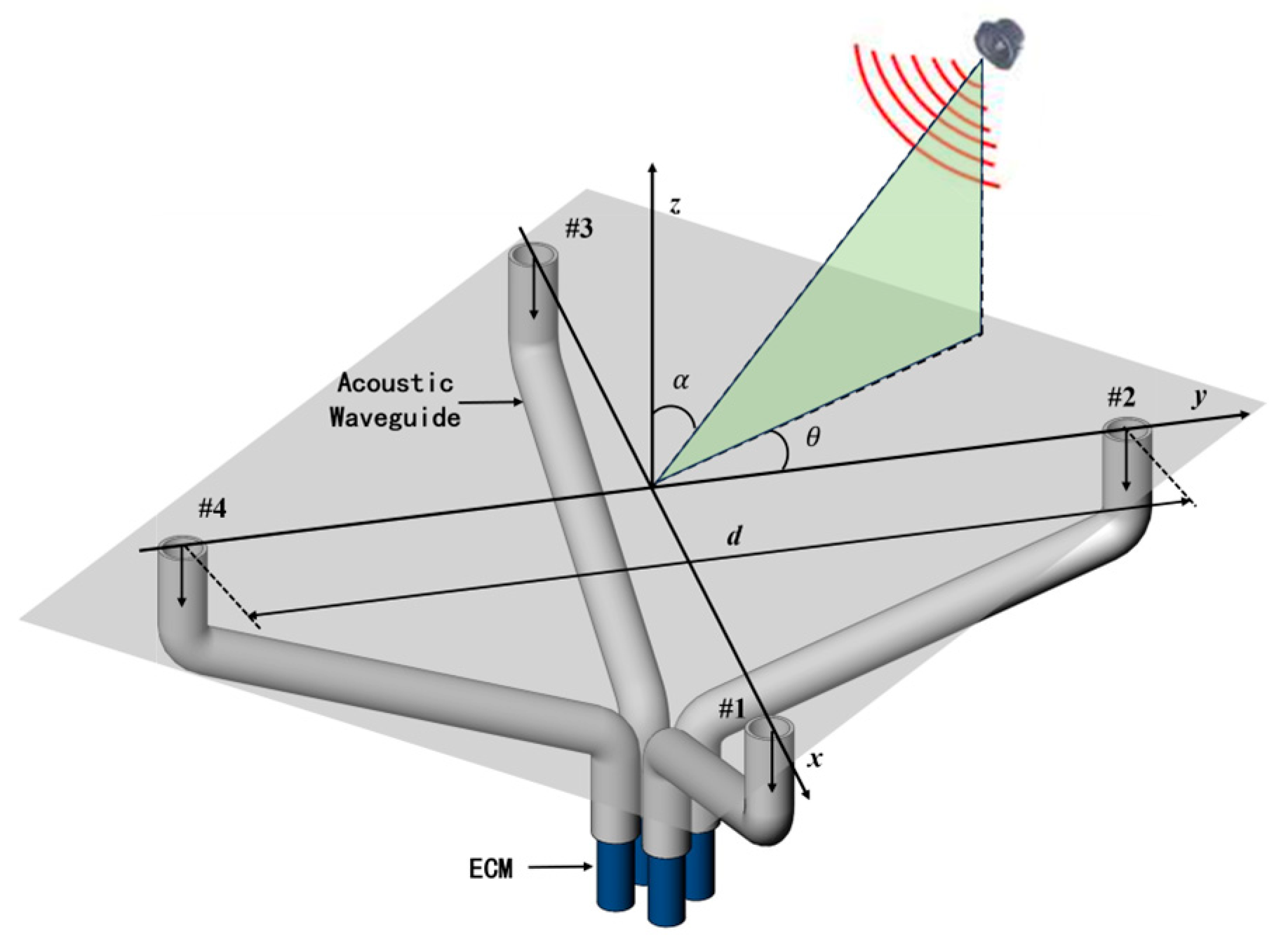
2.3. AFPRD-Based Acoustic Localization Array
3. Simulation Modeling and Principle Verification
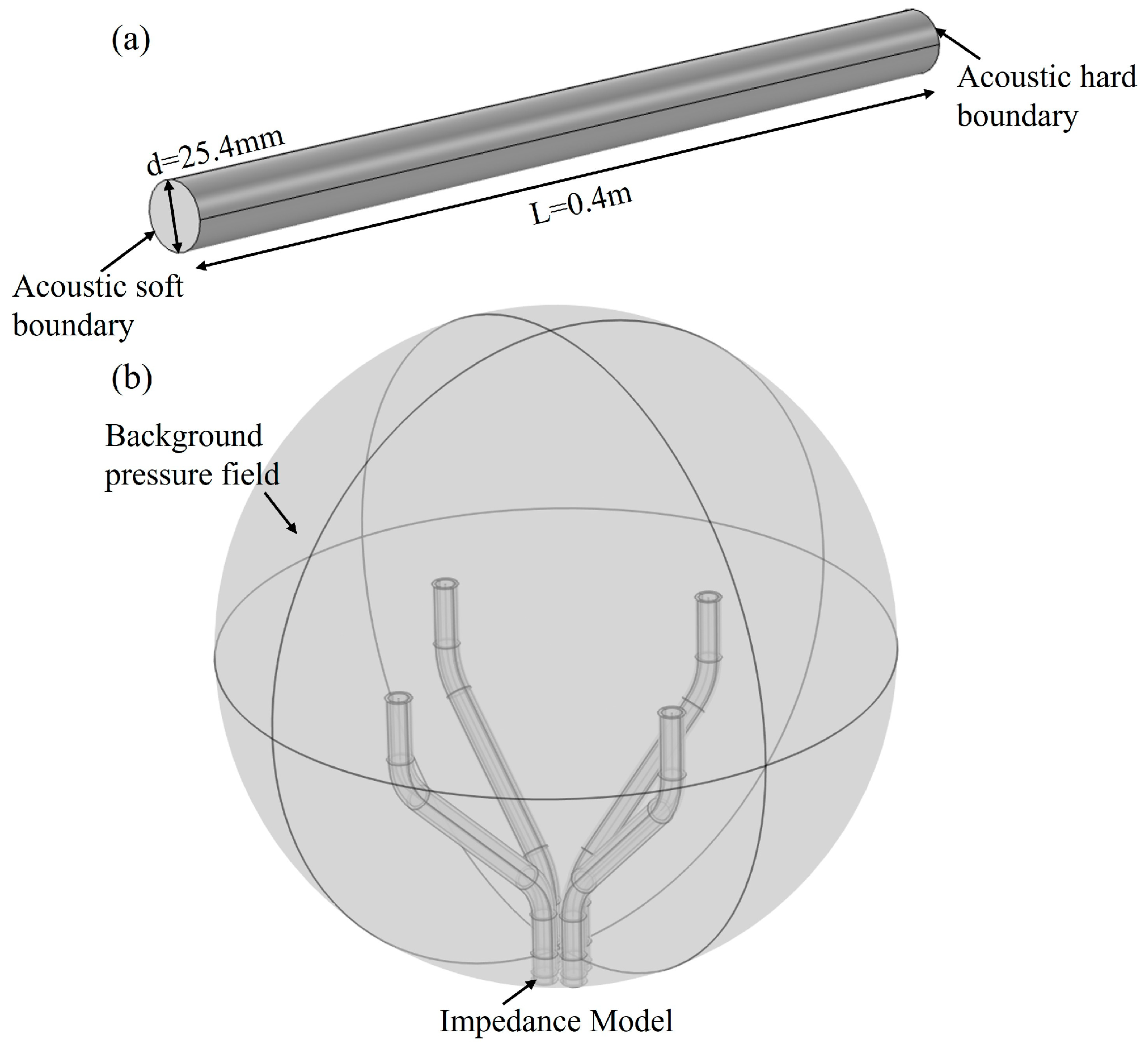
3.1. Finite Element Simulation Model
3.2. Thermoviscous Layer Effect
3.3. ATM Based on Multi-Order Resonance Frequency
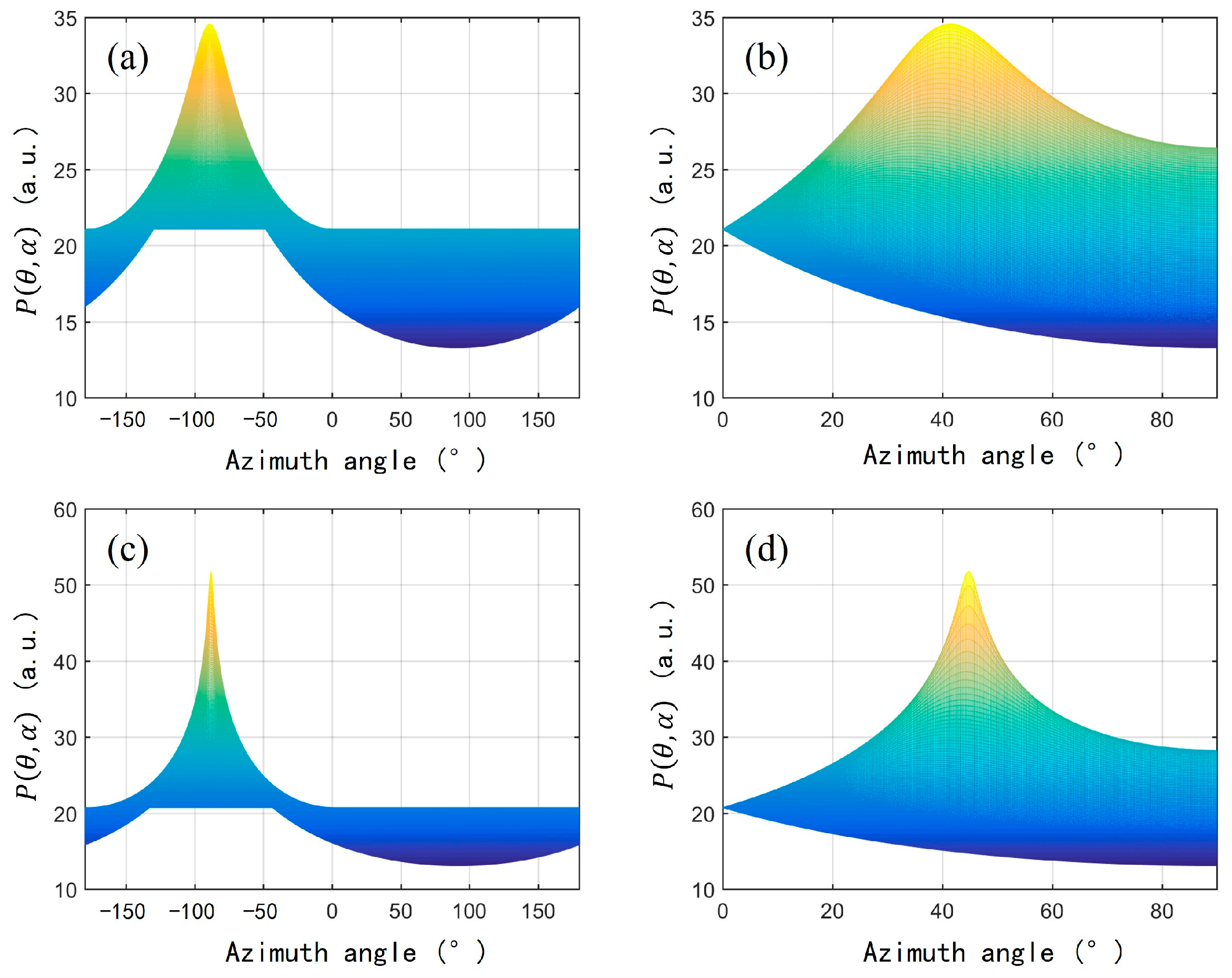
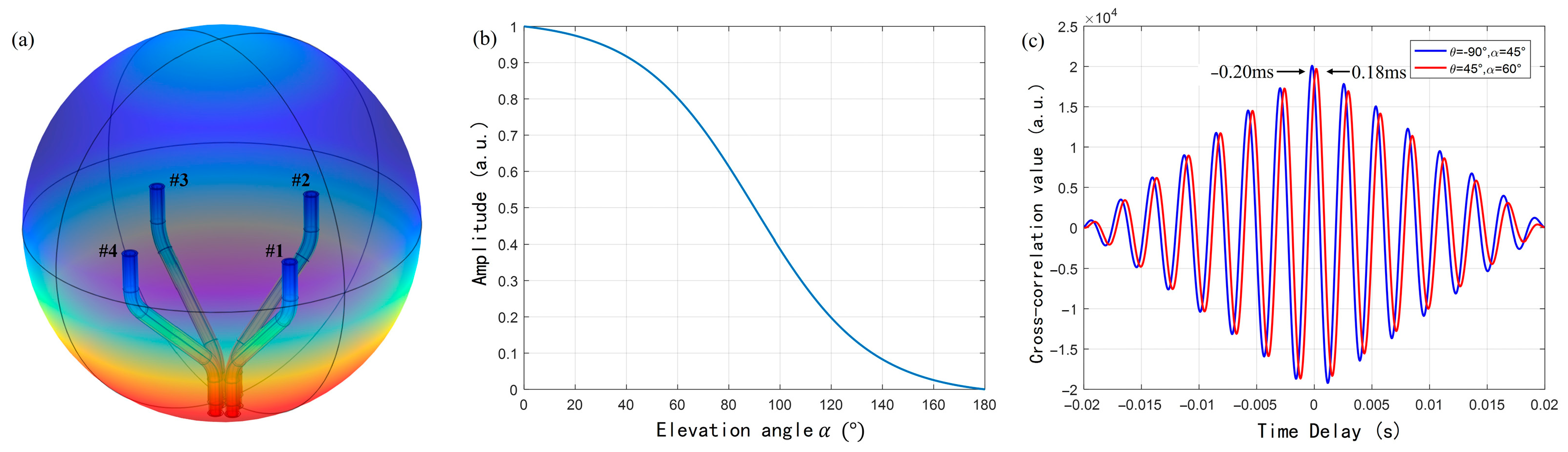
3.4. Performance Analysis of SSL Based on Bent Waveguide Array
4. Non-Ideal Factor Analysis
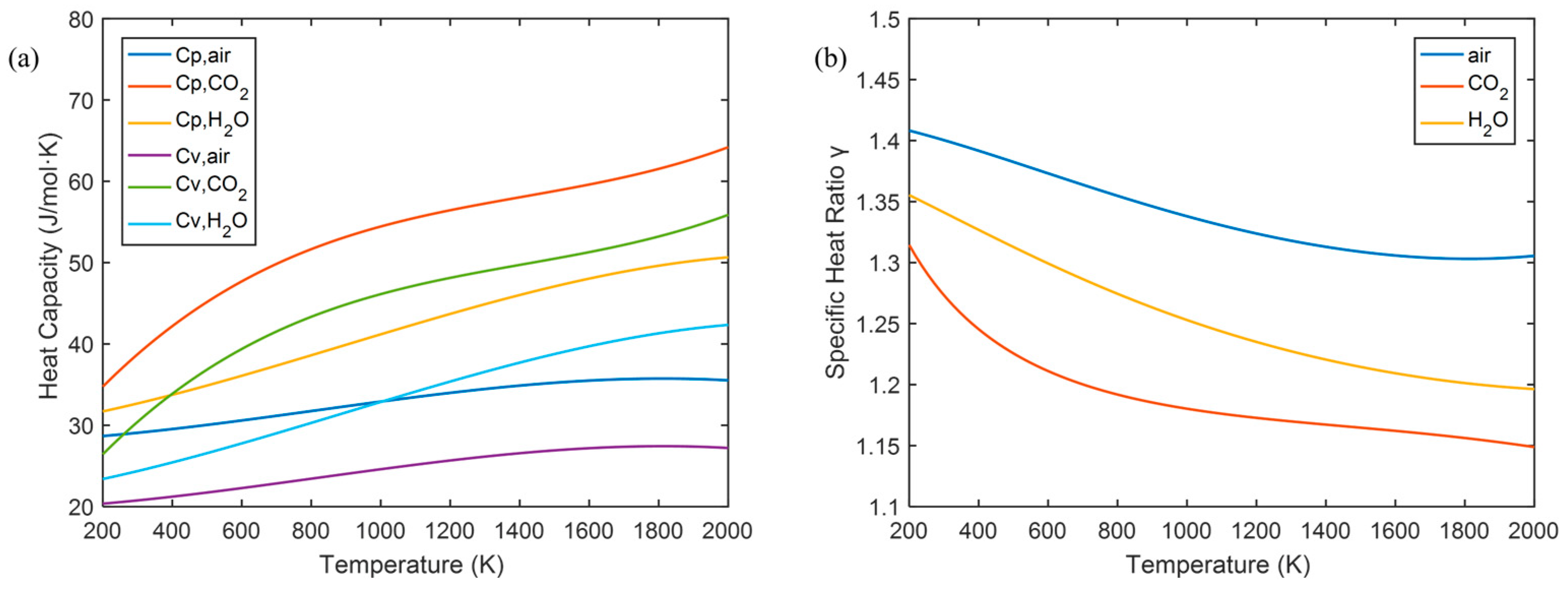
4.1. Limitation Analysis of High Temperature Gas
4.2. Effect of Acoustic Waveguide Structure Deviation
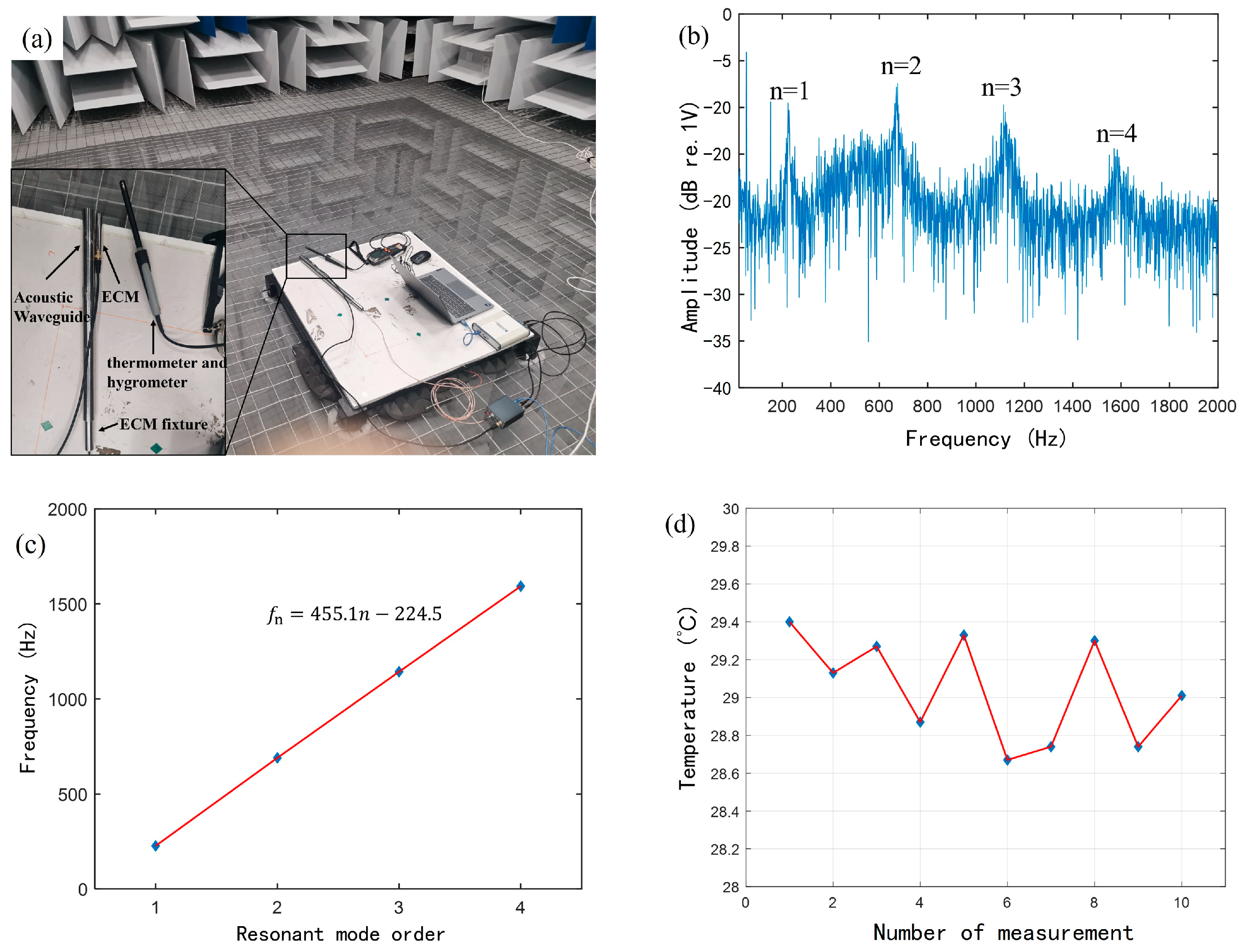
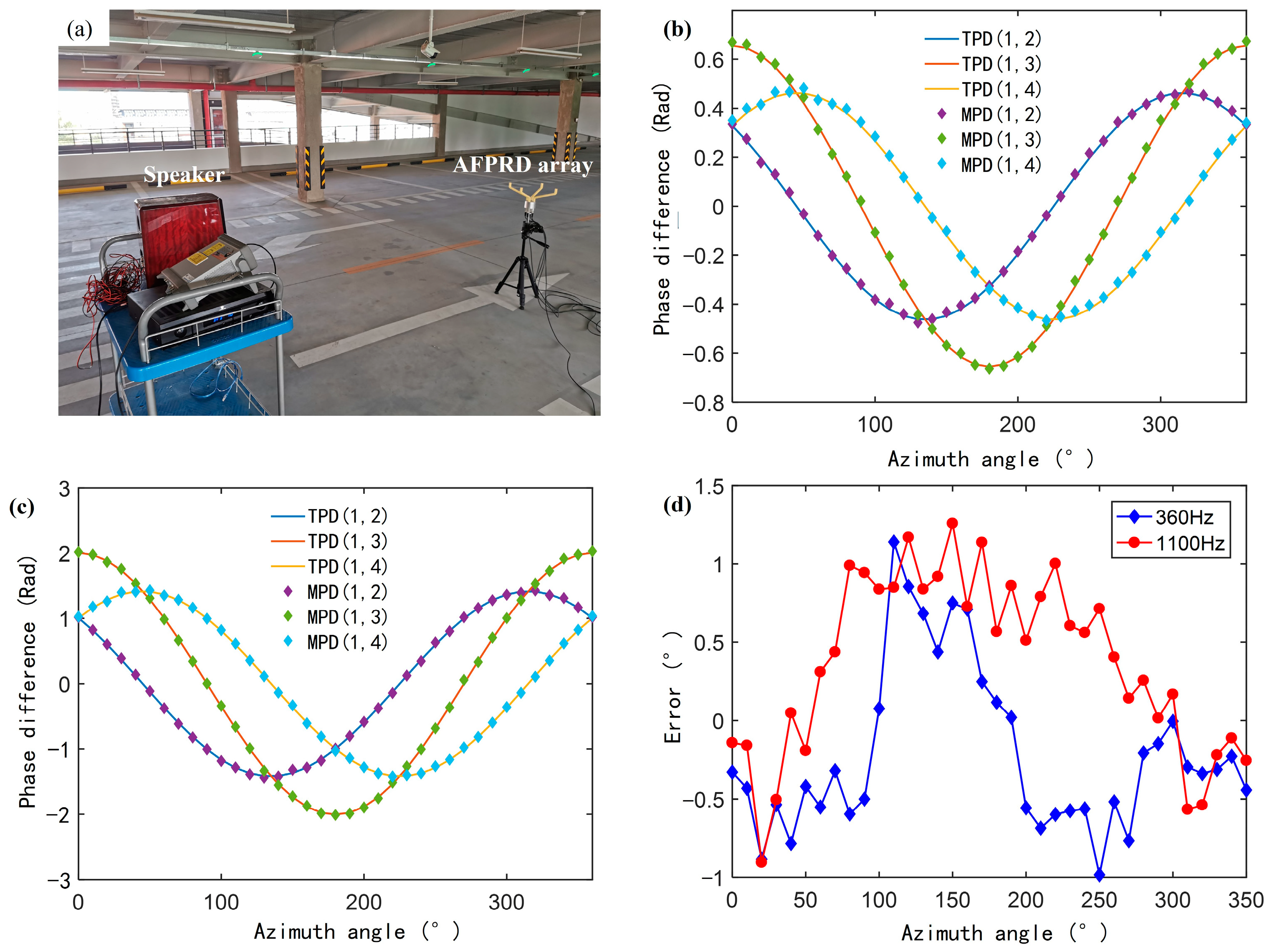
5. Experimental Verification of ATM and SSL Functions
6. Conclusions and Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jiang, C.; Zhao, J.; Huang, B.; Zhu, J.; Yu, J. A basic investigation into the optimization of cylindrical tubes used as acoustic stethoscopes for auscultation in COVID-19 diagnosis. J. Acoust. Soc. Am. 2021, 149, 66–69. [Google Scholar] [CrossRef]
- Komkin, A.I.; Mironov, M.A.; Bykov, A.I. Sound absorption by a Helmholtz resonator. Acoust. Phys. 2017, 63, 385–392. [Google Scholar] [CrossRef]
- Kolesnik, K.; Bryan, D.; Harley, W.; Segeritz, P.; Guest, M.; Rajagopal, V.; Collins, D.J. Respiration mask waveguide optimisation for maximised speech intelligibility. J. Acoust. Soc. Am. 2021, 150, 2030–2039. [Google Scholar] [CrossRef] [PubMed]
- Widiatmo, J.V.; Misawa, T.; Nakano, T.; Saito, I. Preliminary measurements of T–T90 using acoustic gas thermometer in neon gas. Meas. Sens. 2021, 18, 100191. [Google Scholar] [CrossRef]
- Pisani, M.; Astrua, M.; Zucco, M. Improved Acoustic Thermometry for Long-Distance Temperature Measurements. Sensors 2023, 23, 1638. [Google Scholar] [CrossRef] [PubMed]
- Pisani, M.; Astrua, M.; Zucco, M. An acoustic thermometer for air refractive index estimation in long distance interferometric measurements. Metrologia 2017, 55, 67–74. [Google Scholar] [CrossRef]
- Sutton, G.; Edwards, G.; Veltcheva, R.; Podesta, M. Twin-tube practical acoustic thermometry: Theory and measurements up to 1000 °C. Meas. Sci. Technol. 2015, 26, 085901. [Google Scholar] [CrossRef]
- Wang, H.; Zhou, X.; Yang, Q.; Chen, J.; Dong, C.; Zhao, L. A Reconstruction Method of Boiler Furnace Temperature Distribution Based on Acoustic Measurement. IEEE Trans. Instrum. Meas. 2021, 70, 9600413. [Google Scholar] [CrossRef]
- Zhang, S.; Shen, G.; An, L.; Li, G. Ash fouling monitoring based on acoustic pyrometry in boiler furnaces. Appl. Therm. Eng. 2015, 84, 74–81. [Google Scholar] [CrossRef]
- Didkovskyi, V.; Naida, S.; Drozdenko, O.; Drozdenko, K. Experimental researching of biological objects noninvasive passive acoustothermometry features. East. Eur. J. Enterp. Technol. 2020, 1, 6–12. [Google Scholar] [CrossRef]
- Guianvarc’h, C.; Pitre, L.; Bruneau, M.; Bruneau, A.-M. Acoustic field in a quasi-spherical resonator: Unified perturbation model. J. Acoust. Soc. Am. 2009, 125, 1416–1425. [Google Scholar] [CrossRef]
- Zhang, K.; Feng, X.J.; Zhang, J.T.; Lin, H.; Duan, Y.N.; Duan, Y.Y. Microwave measurements of the length and thermal expansion of a cylindrical resonator for primary acoustic gas thermometry. Meas. Sci. Technol. 2016, 28, 015006. [Google Scholar] [CrossRef]
- Feng, X.J.; Zhang, J.T.; Lin, H.; Gillis, K.A.; Mehl, J.B.; Moldover, M.R.; Zhang, K.; Duan, Y.N. Determination of the Boltzmann constant with cylindrical acoustic gas thermometry: New and previous results combined. Metrologia 2017, 54, 748–762. [Google Scholar] [CrossRef] [PubMed]
- Underwood, R.; Podesta, M.; Sutton, G.; Stanger, L.; Rusby, R.; Harris, P.; Morantz, P.; Machin, G. Further estimates of (T–T90) close to the triple point of water. Int. J. Thermophys. 2017, 38, 44. [Google Scholar] [CrossRef]
- Lin, M.; Kim, B. Extended Particle-Aided Unscented Kalman Filter Based on Self-Driving Car Localization. Appl. Sci. 2020, 10, 5045. [Google Scholar] [CrossRef]
- White, M.J.; Nykaza, E.T.; Hulva, A. Localization and source assignment of blast noises from a military training installation. J. Acoust. Soc. Am. 2017, 141, 3985. [Google Scholar] [CrossRef]
- Roy, P.; Chowdhury, C. A Survey of Machine Learning Techniques for Indoor Localization and Navigation Systems. J. Intell. Robot. Syst. 2021, 101, 63. [Google Scholar] [CrossRef]
- Liu, X.; Cai, C.; Ji, K.; Hu, X.; Xiong, L.; Qi, Z. Prototype Optical Bionic Microphone with a Dual-Channel Mach–Zehnder Interferometric Transducer. Sensors 2023, 23, 4416. [Google Scholar] [CrossRef]
- Shkel, A.A.; Kim, E.S. Continuous Health Monitoring with Resonant-Microphone-Array-Based Wearable Stethoscope. IEEE Sens. J. 2019, 19, 4629–4638. [Google Scholar] [CrossRef]
- Reiff, C.G. Comparison of noise levels measured on elevated acoustic sensor arrays mounted on various aerostats. J. Acoust. Soc. Am. 2010, 127, 1780. [Google Scholar] [CrossRef]
- Tang, M.; Bao, Q.; Chen, X.; Hu, T. Miniaturized Electron Optic Tracking System on Aerostat. J. Phys. Conf. Ser. 2019, 1169, 012061. [Google Scholar] [CrossRef]
- Tang, S.; Wang, R.; Han, J.; Jiang, Y. Acoustic energy transport characteristics based on amplitude and phase modulation using waveguide array. J. Appl. Phys. 2020, 128, 165103. [Google Scholar] [CrossRef]
- Rutsch, M.; Unger, A.; Allevato, G.; Hinrichs, J.; Jäger, A.; Kaindl, T.; Kupnik, M. Waveguide for air-coupled ultrasonic phased-arrays with propagation time compensation and plug-in assembly. J. Acoust. Soc. Am. 2021, 150, 3228–3237. [Google Scholar] [CrossRef] [PubMed]
- Dong, Z.; Xiong, L.; Yue, Y.; Cai, C.; Wang, J.; Qi, Z. A speakerless acoustic thermometer. Meas. Sci. Technol. 2023, 34, 055903. [Google Scholar] [CrossRef]



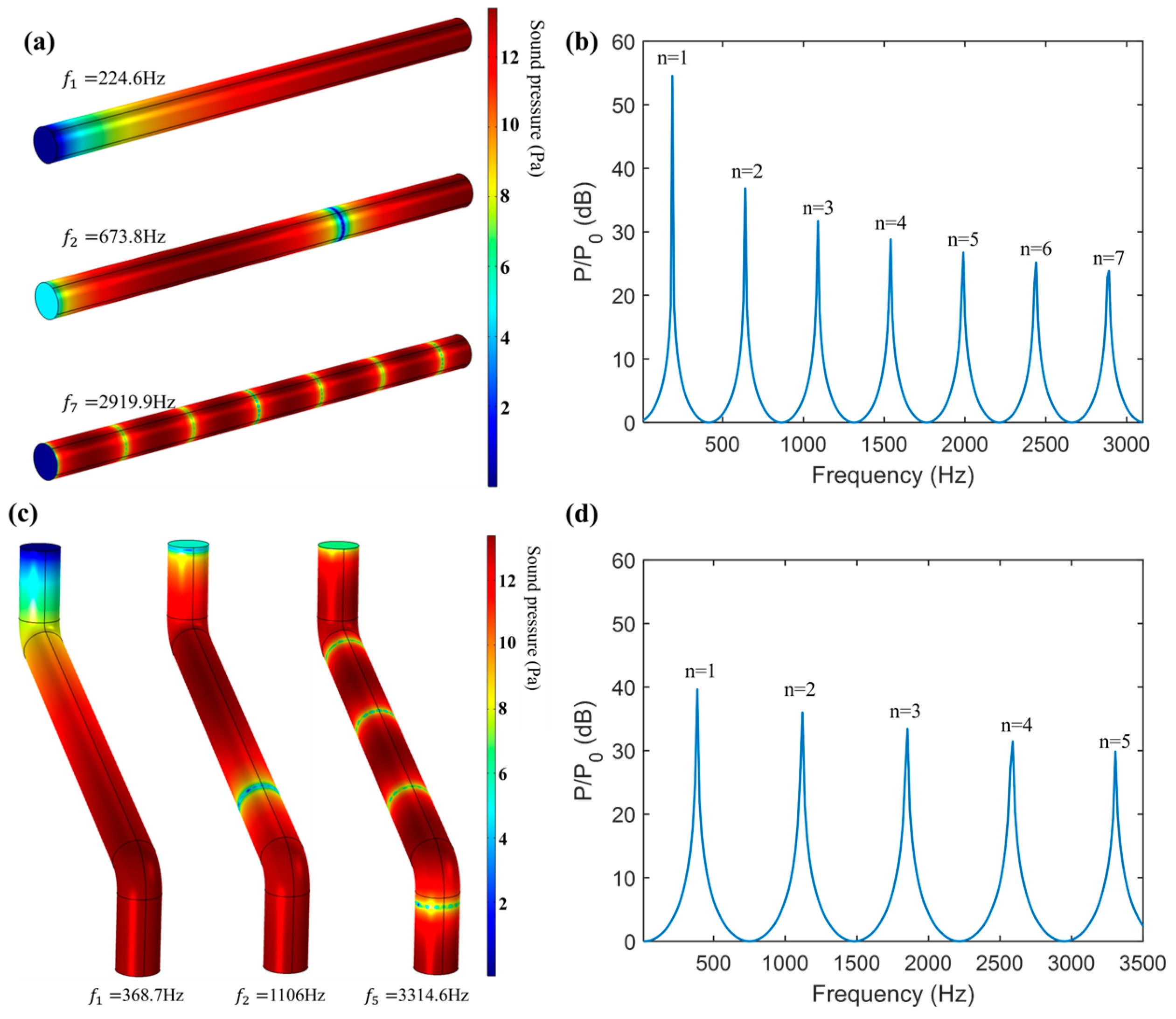


| Mode Order | L = 0.4 m Straight Waveguide | L = 0.24 m Bent Waveguide | ||
|---|---|---|---|---|
| fR (Hz) | T (°C) | fR (Hz) | T (°C) | |
| 1 | 224.61 | 49.31 | 368.71 | 48.18 |
| 2 | 678.83 | 48.36 | 1106.0 | 48.11 |
| 3 | 1123.0 | 48.17 | 1842.8 | 47.92 |
| 4 | 1572.3 | 48.09 | 2579.3 | 47.77 |
| 5 | 2021.5 | 48.36 | 3314.6 | 47.45 |
| 6 | 2470.7 | 48.27 | ||
| 7 | 2919.9 | 48.21 | ||
| Slope | 449.2 | 48.08 | 736.51 | 47.85 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yue, Y.; Dong, Z.; Qi, Z.-m. Acoustic Fabry–Perot Resonance Detector for Passive Acoustic Thermometry and Sound Source Localization. Sensors 2025, 25, 2445. https://doi.org/10.3390/s25082445
Yue Y, Dong Z, Qi Z-m. Acoustic Fabry–Perot Resonance Detector for Passive Acoustic Thermometry and Sound Source Localization. Sensors. 2025; 25(8):2445. https://doi.org/10.3390/s25082445
Chicago/Turabian StyleYue, Yan, Zhifei Dong, and Zhi-mei Qi. 2025. "Acoustic Fabry–Perot Resonance Detector for Passive Acoustic Thermometry and Sound Source Localization" Sensors 25, no. 8: 2445. https://doi.org/10.3390/s25082445
APA StyleYue, Y., Dong, Z., & Qi, Z.-m. (2025). Acoustic Fabry–Perot Resonance Detector for Passive Acoustic Thermometry and Sound Source Localization. Sensors, 25(8), 2445. https://doi.org/10.3390/s25082445





