Full Cross-Sectional Profile Measurement of a High-Aspect-Ratio Micro-Groove Using a Deflection Probe Measuring System
Abstract
:Highlights
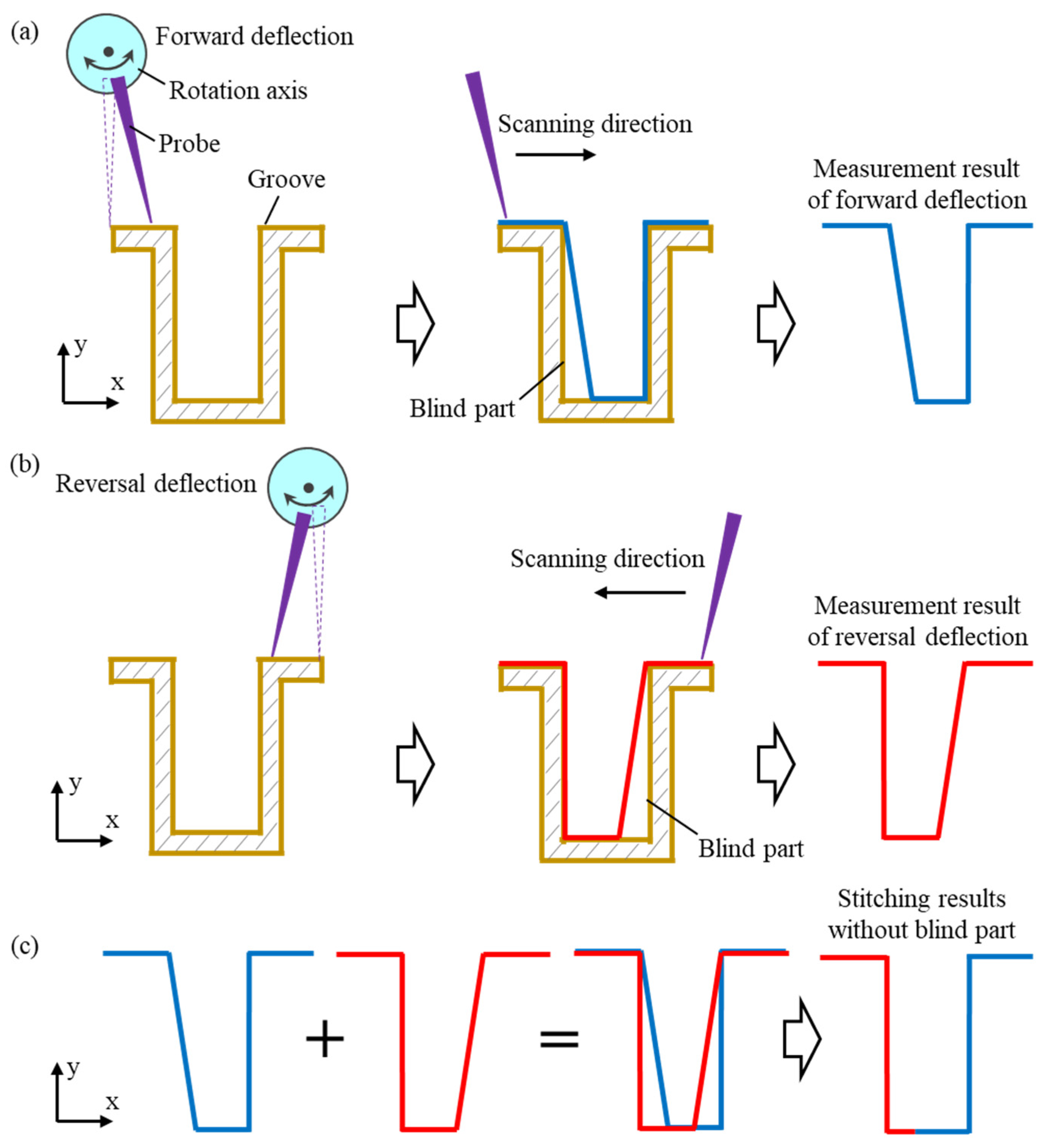
- A deflecting-probe scanning method is proposed for the measurement of a full cross-sectional profile of high-aspect-ratio grooves.
- A method that combines a large length-to-diameter ratio probe measurement and profile stitching was used to eliminate blind areas on the groove’s side walls and bottom.
- The optimal deflection angle of the probe was determined based on the probe deformation and profile stitching requirements.
- A groove-scanning measurement system was developed, and a repeated scanning method was employed to accurately separate the system’s various errors.
Abstract
1. Introduction
2. Measurement System and Method
2.1. Measurement System and Method with Deflection Probe
2.2. Measurement Procedure for High-Aspect-Ratio Micro-Groove
3. Measurement Experiments
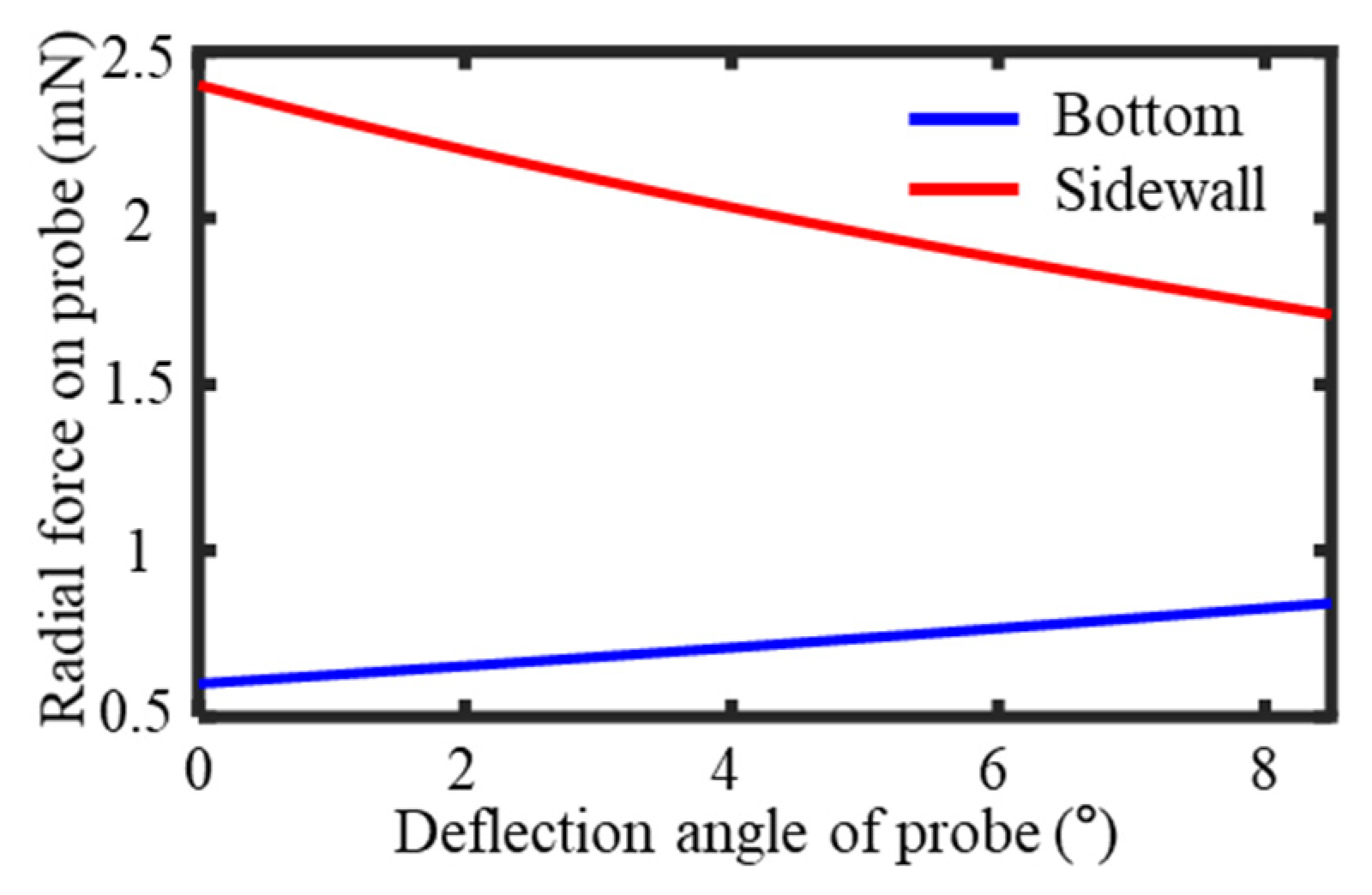
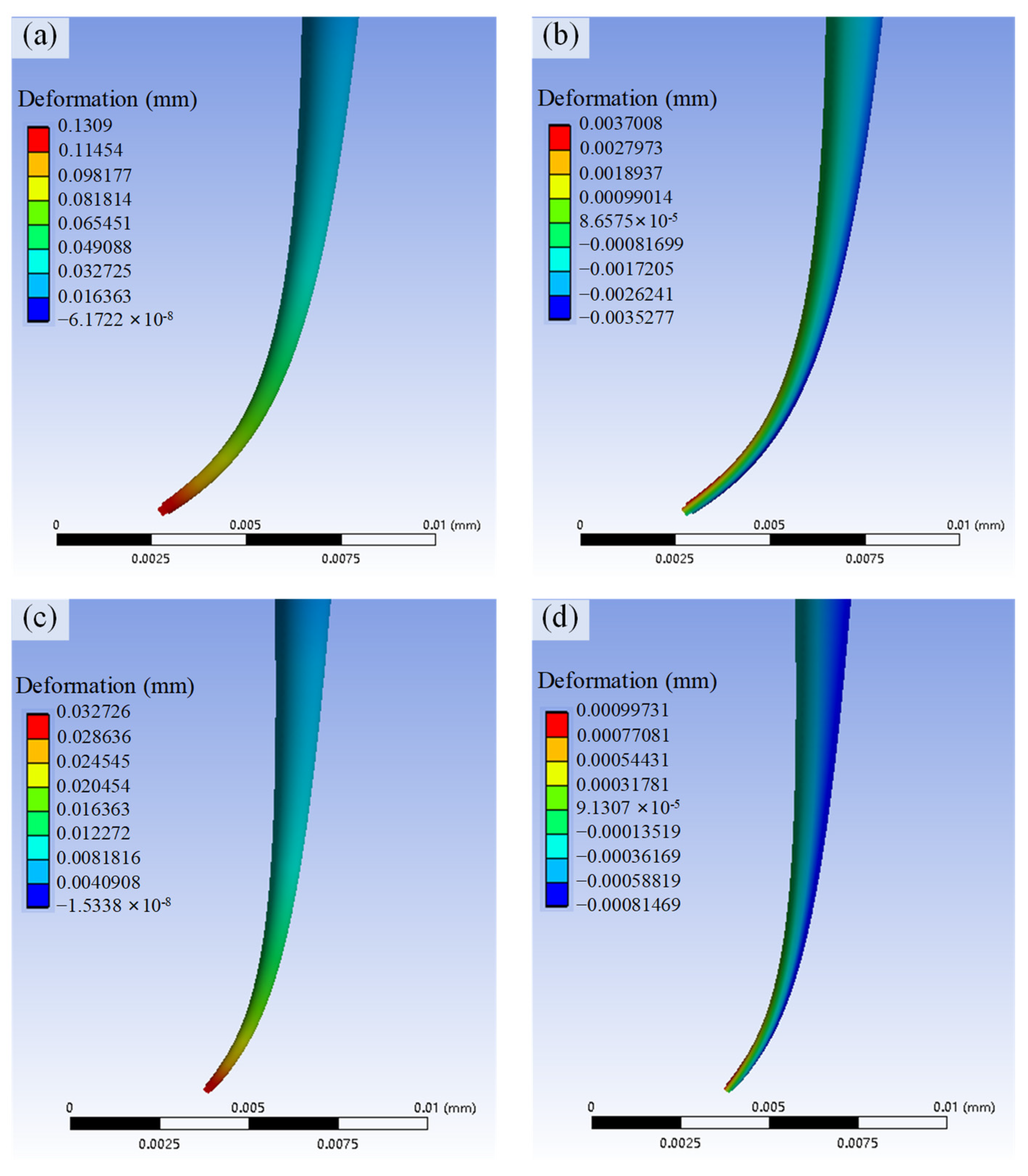
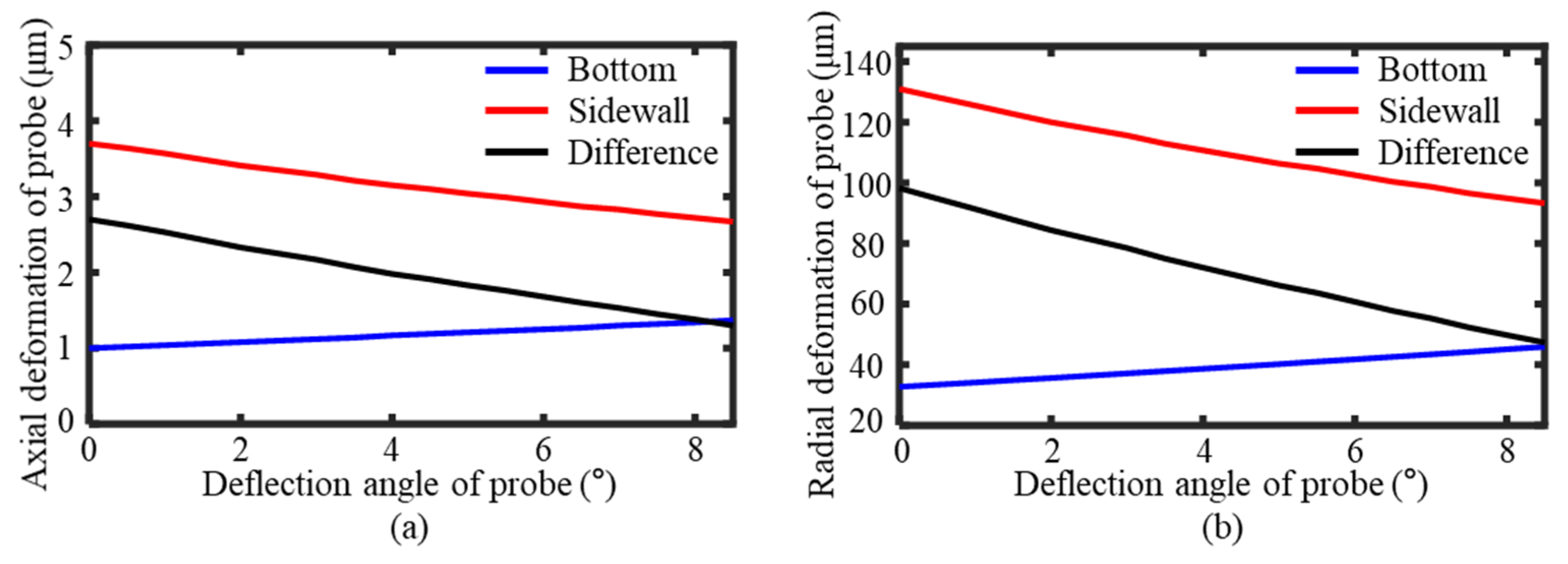
3.1. Analysis and Calculation of the Deflection Angle
3.2. Experimental Setup
3.3. Calibration of the Measuring Probe
3.4. Experimental Process of the Deflection Measurement Method
4. Discussion of the Measurement Results
4.1. Stitching of the Measured Profiles
4.2. Calculation of the Measurement Standard Deviation and Uncertainty
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| GUM | Guide to the Expression of Uncertainty in Measurement |
References
- Wang, Y.; Guo, P. One step machining of hierarchical optical structures for autostereoscopic images. CIRP Ann. 2022, 71, 441–444. [Google Scholar] [CrossRef]
- Gao, W.; Haitjema, H.; Fang, F.Z.; Leach, R.; Cheung, C.; Savio, E.; Linares, J. On-machine and in-process surface metrology for precision manufacturing. CIRP Ann. 2019, 68, 843–866. [Google Scholar] [CrossRef]
- Chang, S.; Geng, Y.; Yan, Y. Tip-based nanomachining on thin films: A mini review. Nanomanuf. Metrol. 2022, 5, 2–22. [Google Scholar] [CrossRef]
- Luo, F.; Zhou, W.; Zhong, Z.; Ren, Y.; Wang, H.; Li, J.; Chen, G. Thermal defects generation mechanism in laser precision dressing of metal-bonded micro-groove diamond wheel and their influence on grinding silicon wafer. Opt. Laser Technol. 2025, 182, 112046. [Google Scholar] [CrossRef]
- Lee, H.; Kim, H.; Jeong, H. Approaches to sustainability in chemical mechanical polishing (CMP): A review. Int. J. Precis. Eng. Manuf.-Green Technol. 2022, 9, 349–367. [Google Scholar] [CrossRef]
- Zhang, L.; Allen, Y.Y.; Yan, J. Flexible fabrication of Fresnel micro-lens array by off-spindle-axis diamond turning and precision glass molding. Precis. Eng. 2022, 74, 186–194. [Google Scholar] [CrossRef]
- Chen, C.; Li, J.; Zhan, S.; Yu, Z.; Xu, W. Study of micro groove machining by micro ECM. Procedia CIRP 2016, 42, 418–422. [Google Scholar] [CrossRef]
- Chavoshi, S.Z.; Luo, X. Hybrid micro-machining processes: A review. Precis. Eng. 2015, 41, 1–23. [Google Scholar] [CrossRef]
- Li, G.; Zhou, H.; Jing, X.; Tian, G.; Li, L. An intelligent wheel position searching algorithm for cutting tool grooves with diverse machining precision requirements. Int. J. Mach. Tools Manuf. 2017, 122, 149–160. [Google Scholar] [CrossRef]
- Fang, F.Z.; Zhang, X.D.; Gao, W.; Guo, Y.; Byrne, G.; Hansen, H. Nanomanufacturing—Perspective and applications. CIRP Ann. 2017, 66, 683–705. [Google Scholar] [CrossRef]
- Sizemore, N.E.; Nogueira, M.L.; Greis, N.P.; Davies, M.A. Application of machine learning for improved surface quality classification in ultra-precision machining of germanium. J. Manuf. Syst. 2022, 65, 296–316. [Google Scholar] [CrossRef]
- Lucca, D.A.; Klopfstein, M.J.; Riemer, O. Ultra-precision machining: Cutting with diamond tools. J. Manuf. Sci. Eng. 2020, 142, 110817. [Google Scholar] [CrossRef]
- Smith, C.L.C.; Stenger, N.; Kristensen, A.; Mortensen, N.A. Gap and channeled plasmons in tapered grooves: A review. Nanoscale 2015, 7, 9355–9386. [Google Scholar] [CrossRef] [PubMed]
- Leach, R.; Haitjema, H.; Su, R.; Thompson, A. Metrological characteristics for the calibration of surface topography measuring instruments: A review. Meas. Sci. Technol. 2020, 32, 032001. [Google Scholar] [CrossRef]
- Riebel, A.; Bauer, R.; Warkentin, A. Investigation into the effect of wheel groove depth and width on grinding performance in creep-feed grinding. Int. J. Adv. Manuf. Technol. 2020, 106, 4401–4409. [Google Scholar] [CrossRef]
- Zhang, X.; Huang, R.; Liu, K.; Kumar, A.S.; Shan, X. Rotating-tool diamond turning of Fresnel lenses on a roller mold for manufacturing of functional optical film. Precis. Eng. 2018, 51, 445–457. [Google Scholar] [CrossRef]
- Archenti, A.; Gao, W.; Donmez, A.; Savio, E.; Irino, N. Integrated metrology for advanced manufacturing. CIRP Ann. 2024, 73, 639–665. [Google Scholar] [CrossRef]
- Ito, S.; Kikuchi, H.; Chen, Y.; Shimizu, Y.; Gao, W.; Takahashi, K.; Kanayama, T.; Arakawa, K.; Hayashi, A. A micro-coordinate measurement machine (CMM) for large-scale dimensional measurement of micro-slits. Appl. Sci. 2016, 6, 156. [Google Scholar] [CrossRef]
- Fang, F.; Zeng, Z.; Zhang, X.; Jiang, L. Measurement of micro-V-groove dihedral using white light interferometry. Opt. Commun. 2016, 359, 297–303. [Google Scholar] [CrossRef]
- Möhring, H.C.; Kersting, P.; Carmignato, S.; Yagüe-Fabra, J.; Maestro, M.; Jiménez, R.; Ferraris, E.; Tunc, L.; Bleicher, F.; Wits, W.; et al. A testpart for interdisciplinary analyses in micro production engineering. Procedia CIRP 2015, 28, 106–112. [Google Scholar] [CrossRef]
- Jantzen, S.; Neugebauer, M.; Meeß, R.; Wolpert, C.; Dietzel, A.; Stein, M.; Kniel, K. Novel measurement standard for internal involute microgears with modules down to 0.1 mm. Meas. Sci. Technol. 2018, 29, 125012. [Google Scholar] [CrossRef]
- Loizou, K.; Koutroulis, E. Water level sensing: State of the art review and performance evaluation of a low-cost measurement system. Measurement 2016, 89, 204–214. [Google Scholar] [CrossRef]
- Zhao, C.; Cheung, C.F.; Xu, P. High-efficiency sub-microscale uncertainty measurement method using pattern recognition. ISA Trans. 2020, 101, 503–514. [Google Scholar] [CrossRef] [PubMed]
- Gao, W.; Kim, S.W.; Bosse, H.; Haitjema, H.; Chen, Y.; Lu, X.; Knapp, W.; Weckenmann, A.; Estler, W.; Kunzmann, H. Measurement technologies for precision positioning. CIRP Ann. 2015, 64, 773–796. [Google Scholar] [CrossRef]
- Hovell, T.; Petzing, J.; Guo, W.; Gill, C.; Justham, L.; Lohse, N.; Kinnell, P. Measurement techniques for three-dimensional metrology of high aspect ratio internal features—A review. Metrology 2023, 3, 139–168. [Google Scholar] [CrossRef]
- Cao, B.X.; Le Hoang, P.; Ahn, S.; Kim, J.-O.; Noh, J. High-precision detection of focal position on a curved surface for laser processing. Precis. Eng. 2017, 50, 204–210. [Google Scholar] [CrossRef]
- Jiménez, R.; Torralba, M.; Yagüe-Fabra, J.A.; Ontiveros, S.; Tosello, G. Experimental approach for the uncertainty assessment of 3D complex geometry dimensional measurements using computed tomography at the mm and sub-mm scales. Sensors 2017, 17, 1137. [Google Scholar] [CrossRef]
- Dai, G.; Neugebauer, M.; Stein, M.; Bütefisch, S.; Neuschaefer-Rube, U. Overview of 3D micro-and nanocoordinate metrology at PTB. Appl. Sci. 2016, 6, 257. [Google Scholar] [CrossRef]
- Zhang, J.; He, C.; Chen, G.; Luo, M.; Ren, C. Theoretical and experimental investigation on double-disc straight groove lapping: Tool contour and lapping trajectory. Int. J. Adv. Manuf. Technol. 2024, 134, 1507–1531. [Google Scholar] [CrossRef]
- Chen, Y.L.; Xu, Y.; Shimizu, Y.; Matsukuma, H.; Gao, W. High quality-factor quartz tuning fork glass probe used in tapping mode atomic force microscopy for surface profile measurement. Meas. Sci. Technol. 2018, 29, 065014. [Google Scholar] [CrossRef]
- Gao, W. Surface Metrology for Micro- and Nanofabrication; Elsevier: Amsterdam, The Netherlands, 2020. [Google Scholar]
- Miura, T.; Matsubara, A.; Yamaji, I.; Hoshide, K. Measurement and analysis of friction fluctuations in linear guideways. CIRP Ann. 2018, 67, 393–396. [Google Scholar] [CrossRef]
- Shimizu, Y.; Gao, W.; Matsukuma, H.; Szipka, K.; Archenti, A. On-machine angle measurement of a precision V-groove on a ceramic workpiece. CIRP Ann. 2020, 69, 469–472. [Google Scholar] [CrossRef]
- Yin, X.; Yin, M.; Xie, L.; Yin, G. Automatic measurement system of section geometry parameters for composite filter rods based on machine vision. IEEE Trans. Instrum. Meas. 2019, 69, 5037–5050. [Google Scholar] [CrossRef]
- Wen, B.; Shimizu, Y.; Watanabe, Y.; Matsukuma, H.; Gao, W. On-machine profile measurement of a micro cutting edge by using a contact-type compact probe unit. Precis. Eng. 2020, 65, 230–239. [Google Scholar] [CrossRef]
- Wen, B.; Sekine, S.; Osawa, S.; Shimizu, Y.; Matsukuma, H.; Archenti, A.; Gao, W. A Comparison of the Probes with a Cantilever Beam and a Double-Sided Beam in the Tool Edge Profiler for On-Machine Measurement of a Precision Cutting Tool. Machines 2021, 9, 271. [Google Scholar] [CrossRef]
- Matsukuma, H.; Wen, B.; Osawa, S.; Sekine, S.; Shimizu, Y.; Gao, W. Design and construction of a low-force stylus probe for on-machine tool cutting edge measurement. Nanomanuf. Metrol. 2020, 3, 282–291. [Google Scholar] [CrossRef]
- Li, Z.; Chen, Y.L.; Wu, L.; Ju, B.F. Development of a force controlled nanocutting system using a flexible mechanism for adaptive cutting of microstructures on non-planar surfaces. Precis. Eng. 2021, 72, 282–293. [Google Scholar] [CrossRef]
- Gauder, D.; Gölz, J.; Biehler, M.; Diener, M.; Lanza, G. Balancing the trade-off between measurement uncertainty and measurement time in optical metrology using design of experiments, meta-modelling and convex programming. CIRP J. Manuf. Sci. Technol. 2021, 35, 209–216. [Google Scholar] [CrossRef]







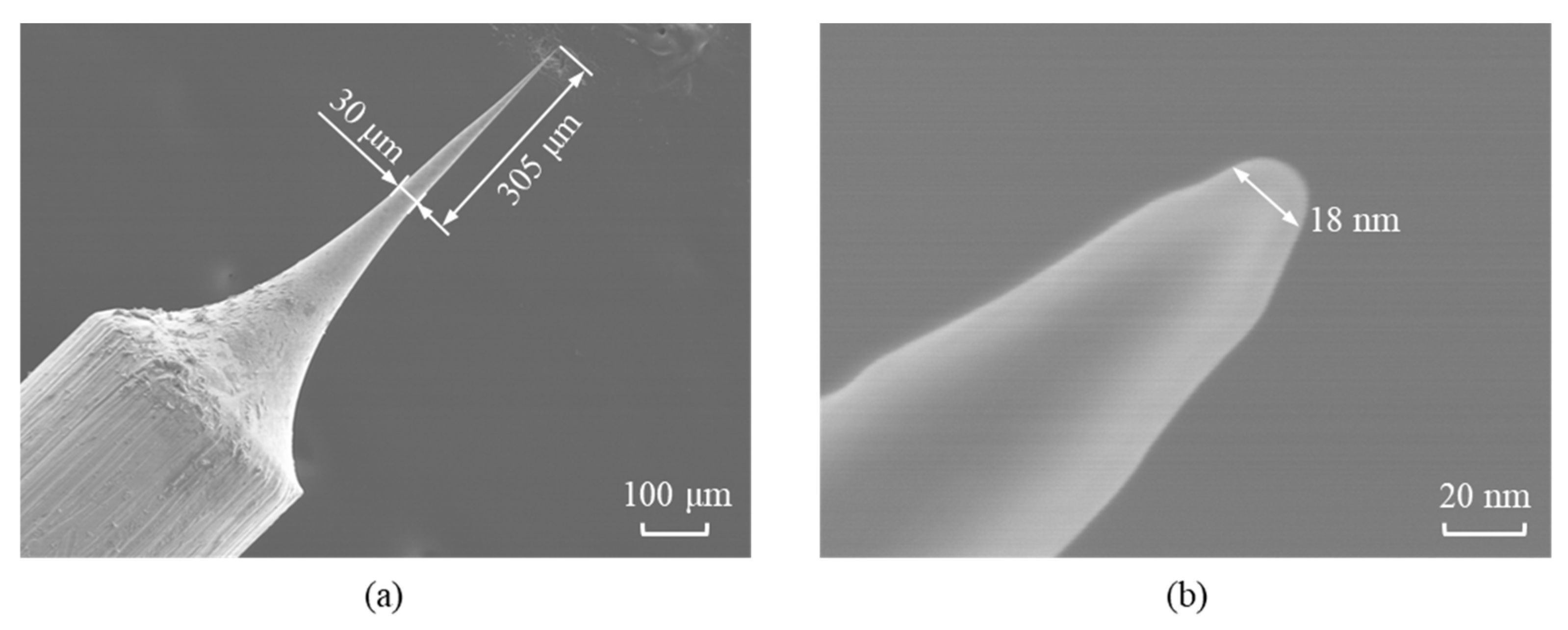


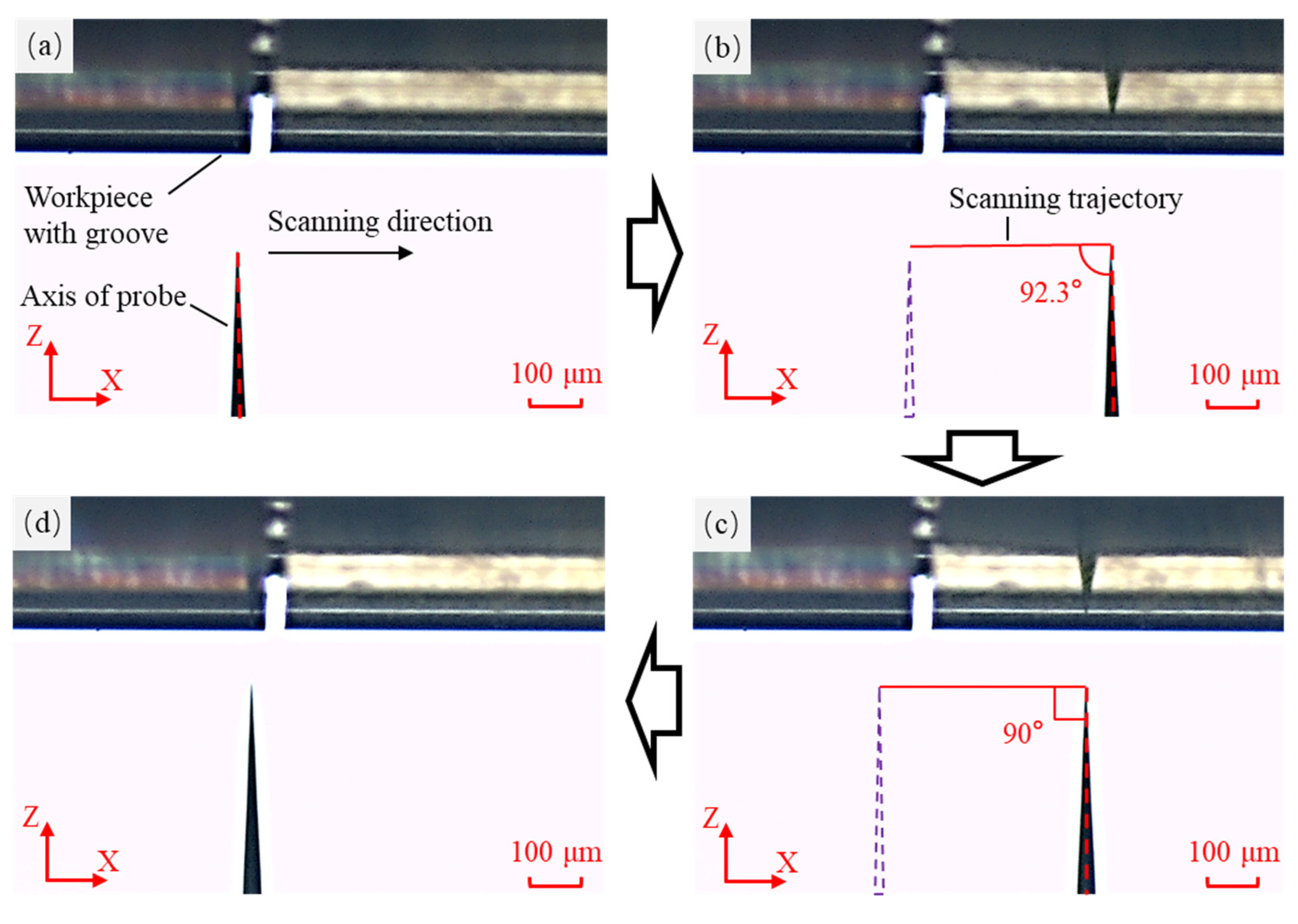
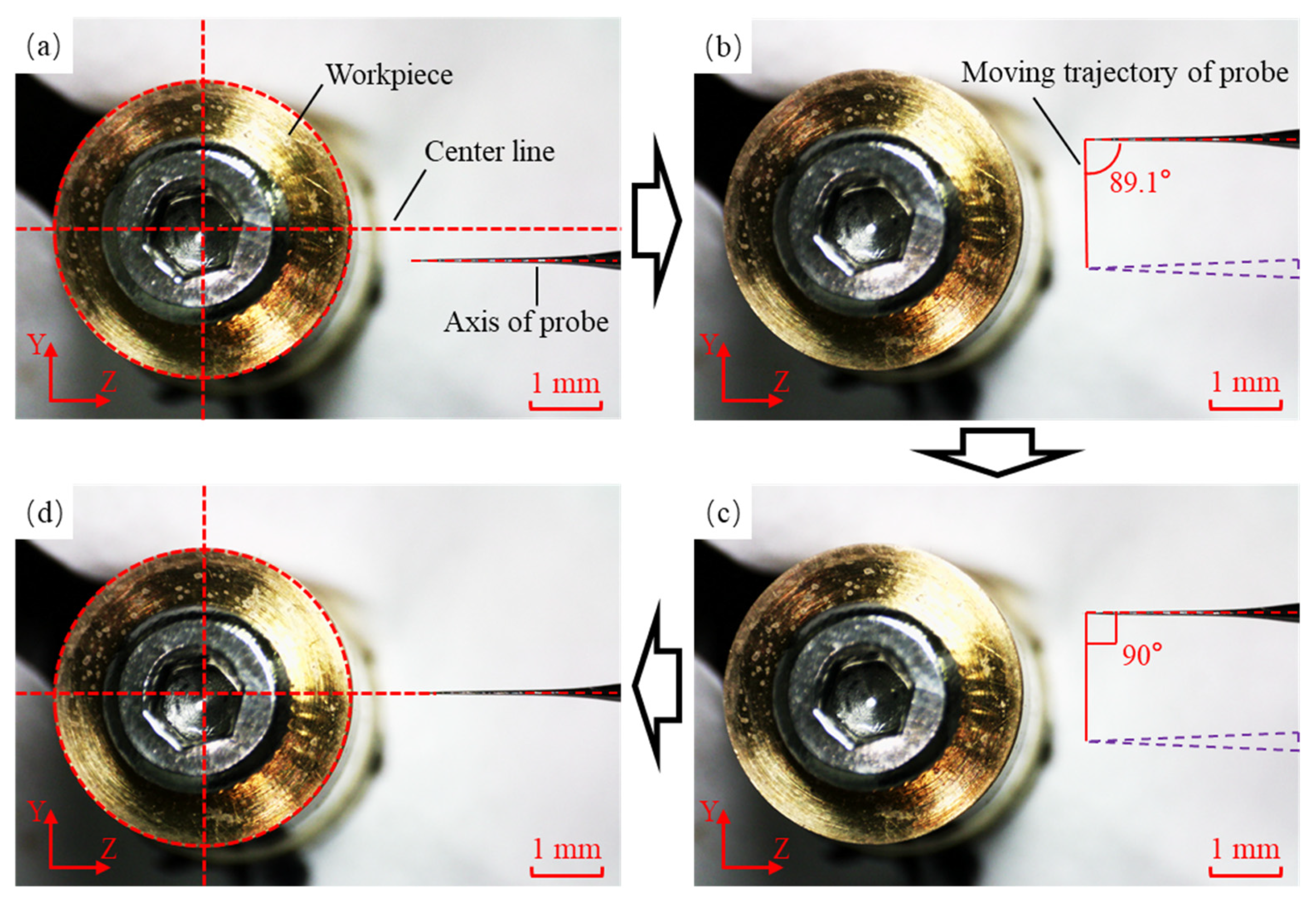
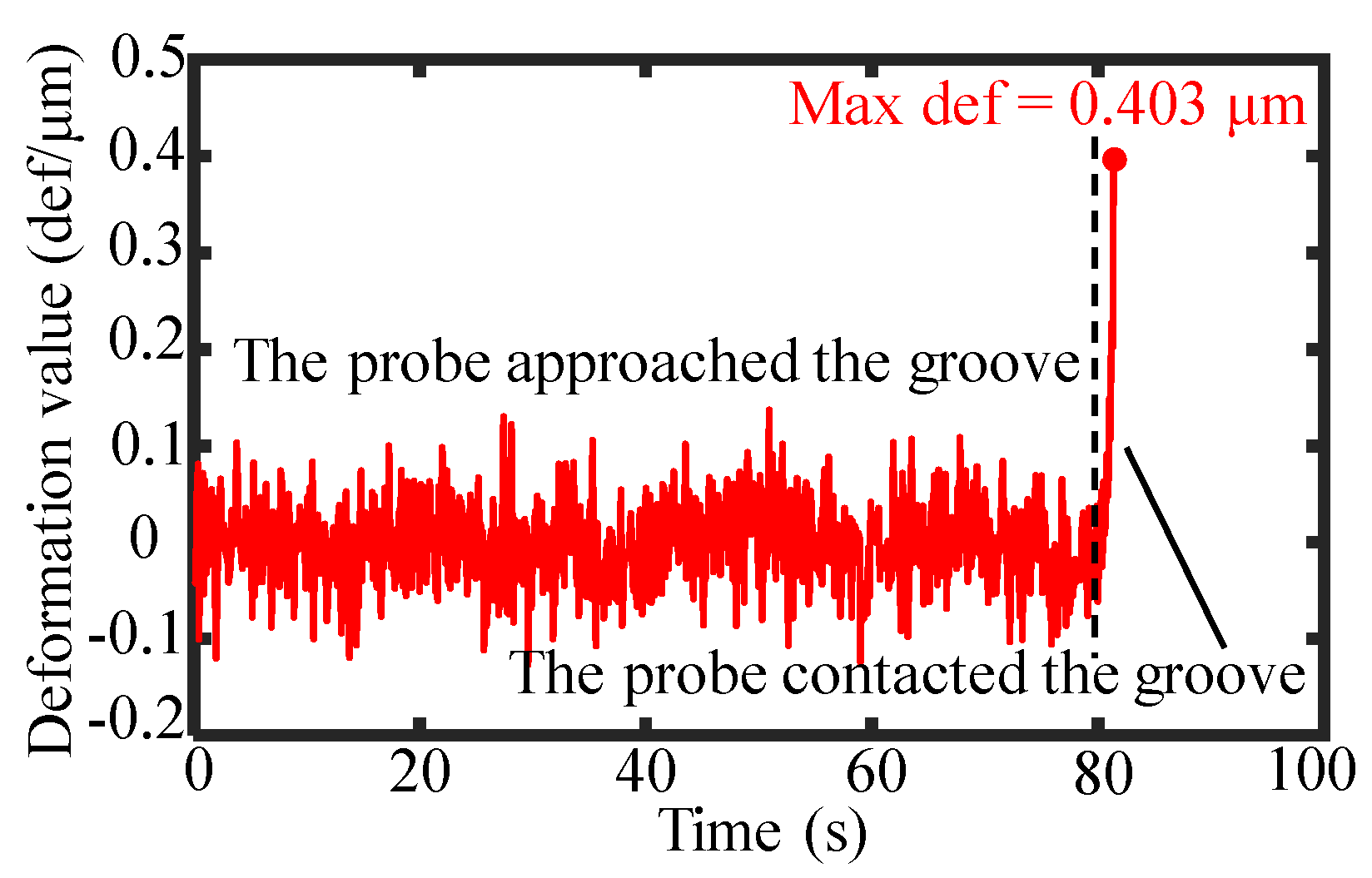
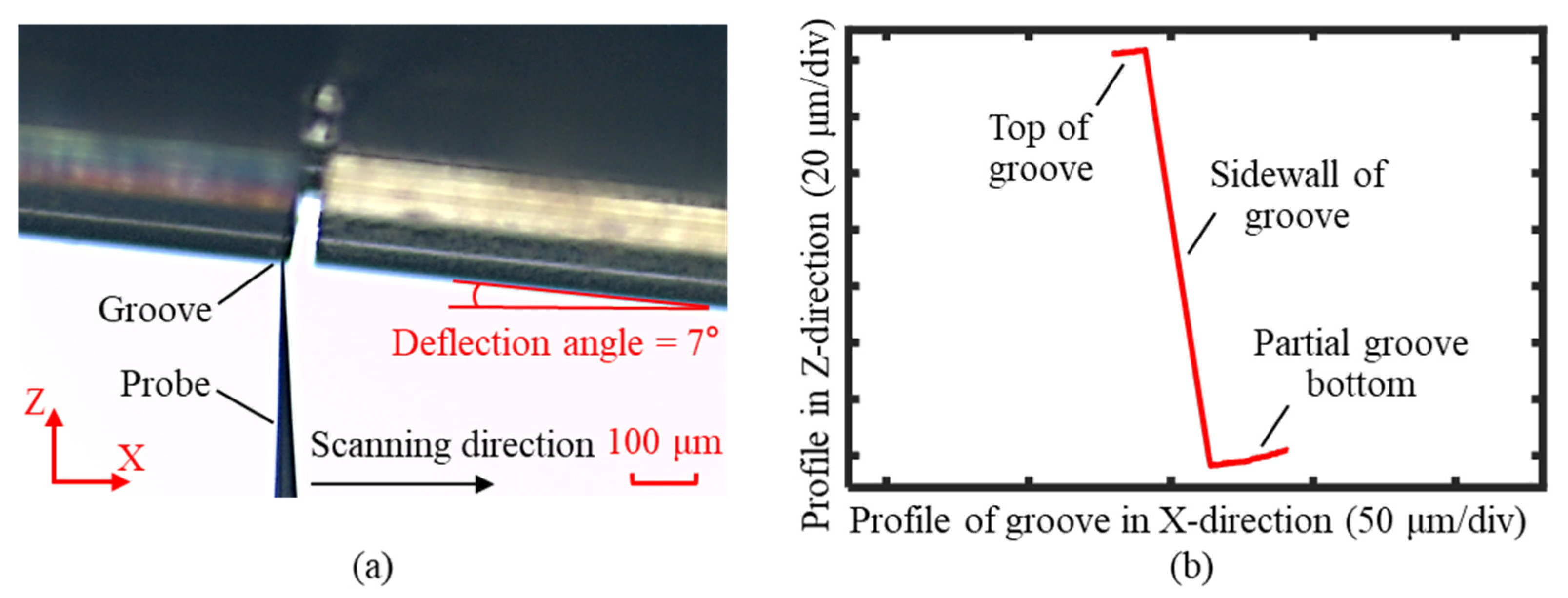
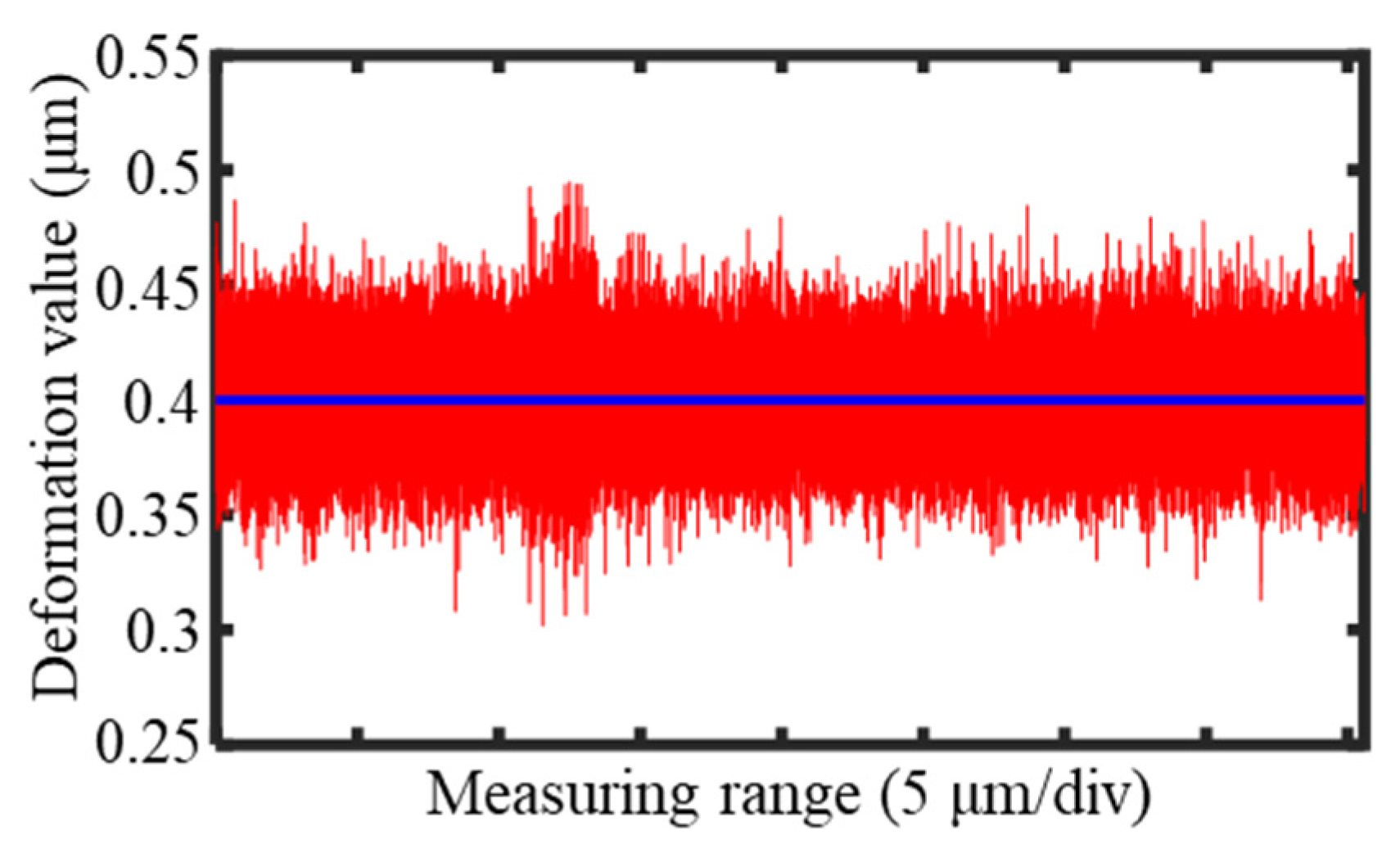
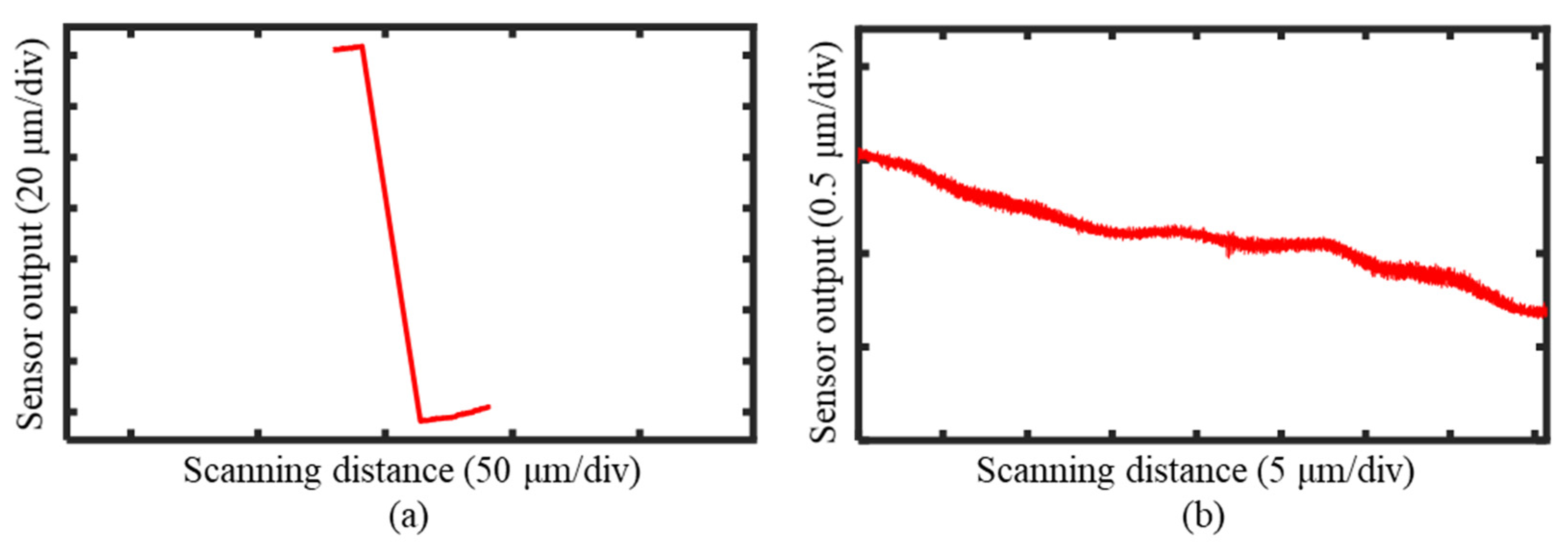


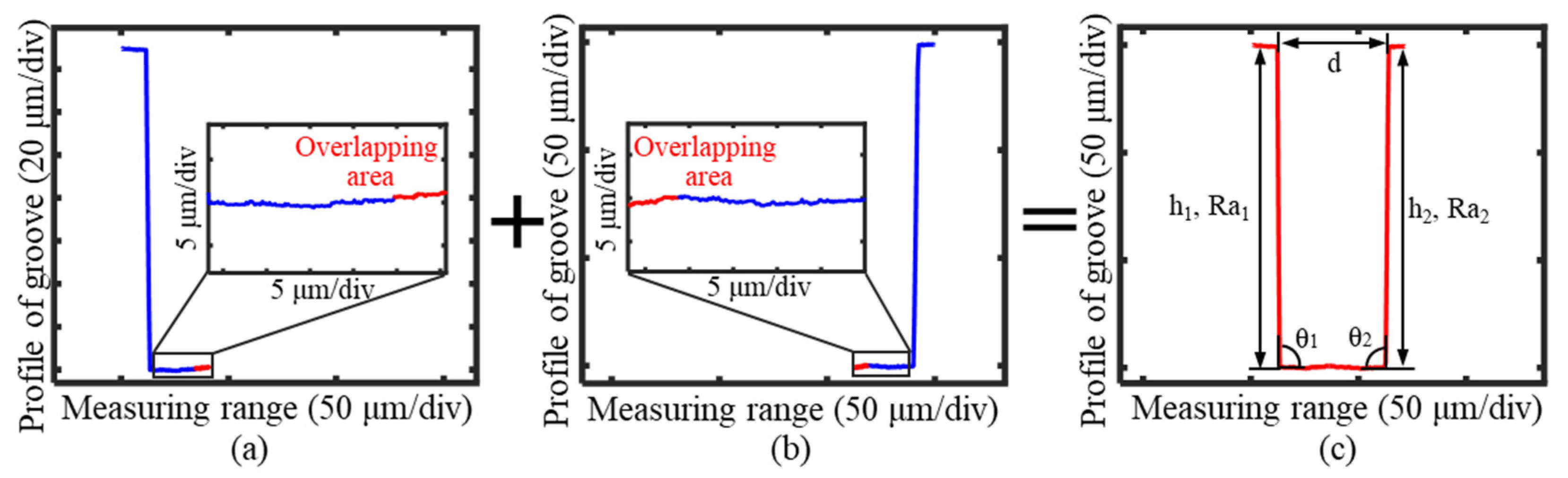

| Items | Values | |
|---|---|---|
| X/Z-axes | Measuring range | 150 mm |
| Repetitive positioning accuracy | 50 nm | |
| Capacitive sensor | Resolution | 3 nm |
| Probe | Tip diameter | 18 nm |
| Length–diameter ratio | 10:1 | |
| Laser sensor | Measuring range | 2.1 mm |
| Resolution | 1 nm |
| Symbol | Left Side | Right Side | |
|---|---|---|---|
| Width | d (μm) | 50.7 | |
| Depth | h (μm) | 149.23 | 150.43 |
| Angle | θ (°) | 90.3 | 89.7 |
| Roughness | Ra (nm) | 55 | 58 |
| Uncertainty Sources | Type | Symbol | Standard Uncertainty (nm) |
|---|---|---|---|
| Uncertainty of measuring system | B | usystem | 54 |
| Resolution of probe | B | uprobe | 20 |
| Accuracy of laser sensor | B | ulaser | 1 |
| Accuracy of capacitive sensor | B | ucapacitive | 3 |
| Repetitive positioning accuracy of Z-axis | B | uz-axis | 50 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, Z.-H.; Tang, J.; Li, Z.; Chen, Y.-L. Full Cross-Sectional Profile Measurement of a High-Aspect-Ratio Micro-Groove Using a Deflection Probe Measuring System. Sensors 2025, 25, 2335. https://doi.org/10.3390/s25072335
Cao Z-H, Tang J, Li Z, Chen Y-L. Full Cross-Sectional Profile Measurement of a High-Aspect-Ratio Micro-Groove Using a Deflection Probe Measuring System. Sensors. 2025; 25(7):2335. https://doi.org/10.3390/s25072335
Chicago/Turabian StyleCao, Zhong-Hao, Jinyan Tang, Zhongwei Li, and Yuan-Liu Chen. 2025. "Full Cross-Sectional Profile Measurement of a High-Aspect-Ratio Micro-Groove Using a Deflection Probe Measuring System" Sensors 25, no. 7: 2335. https://doi.org/10.3390/s25072335
APA StyleCao, Z.-H., Tang, J., Li, Z., & Chen, Y.-L. (2025). Full Cross-Sectional Profile Measurement of a High-Aspect-Ratio Micro-Groove Using a Deflection Probe Measuring System. Sensors, 25(7), 2335. https://doi.org/10.3390/s25072335






