Directional Torsion Sensor Based on a Paired Helical-Type Multimode Fiber
Abstract
1. Introduction
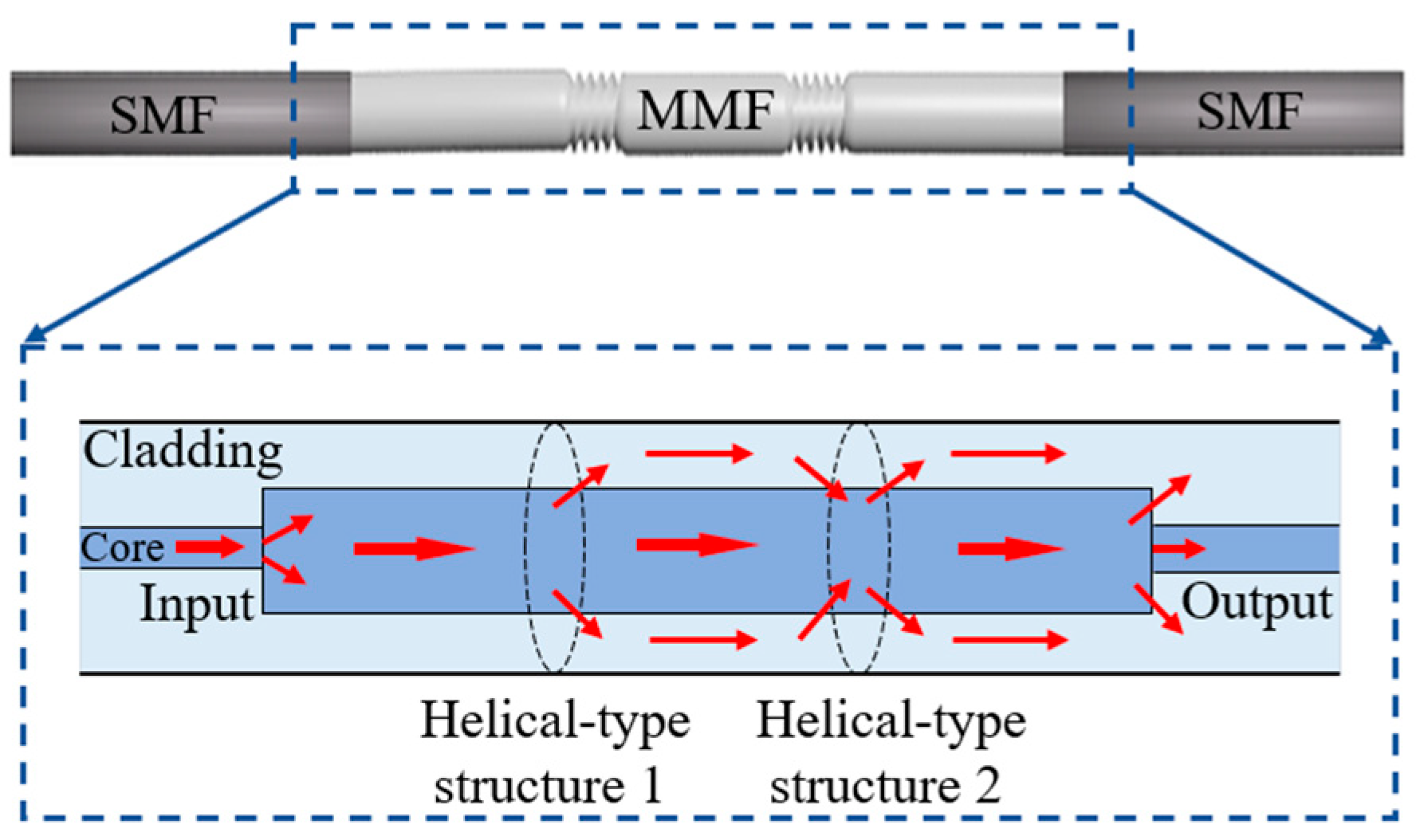
2. Analysis of Sensing Principles
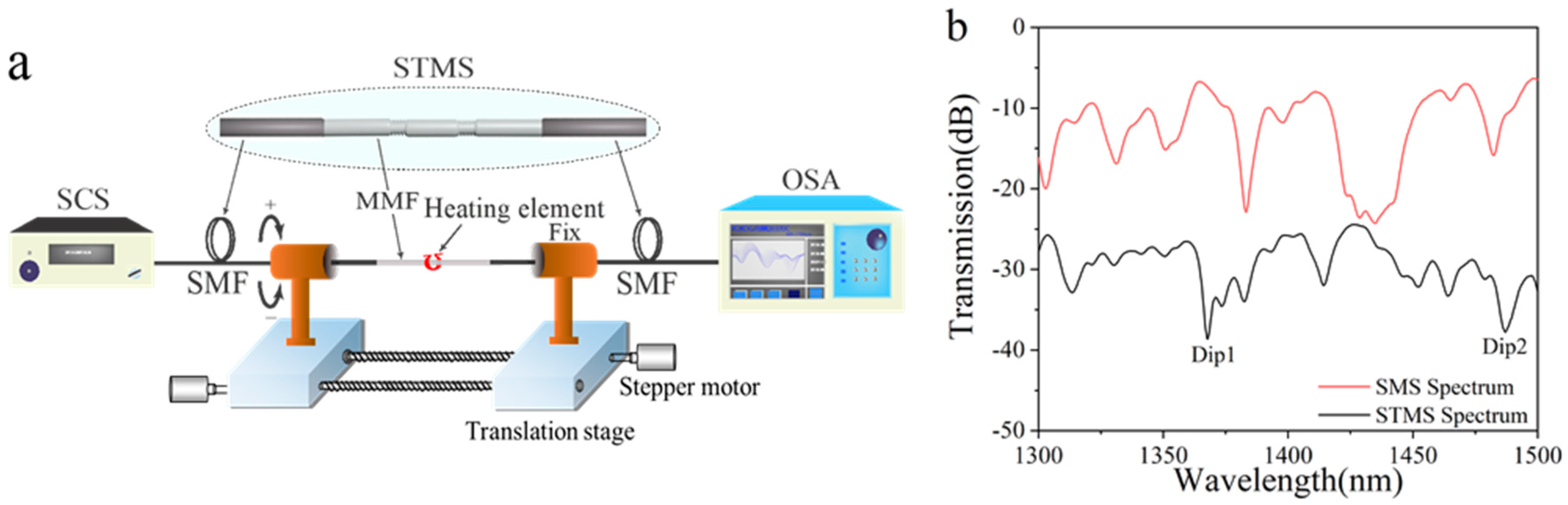
3. Fabrication of STMS Sensor
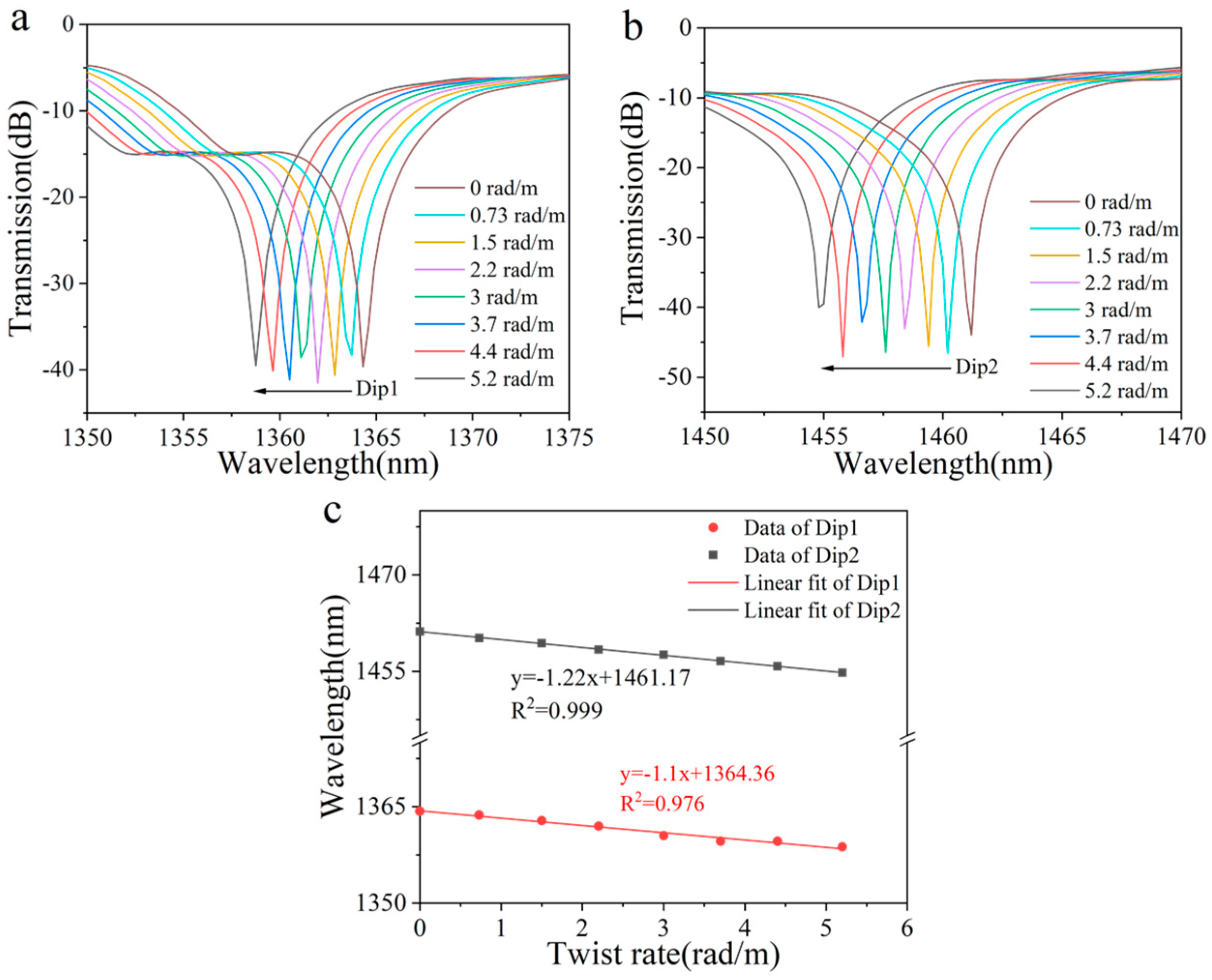
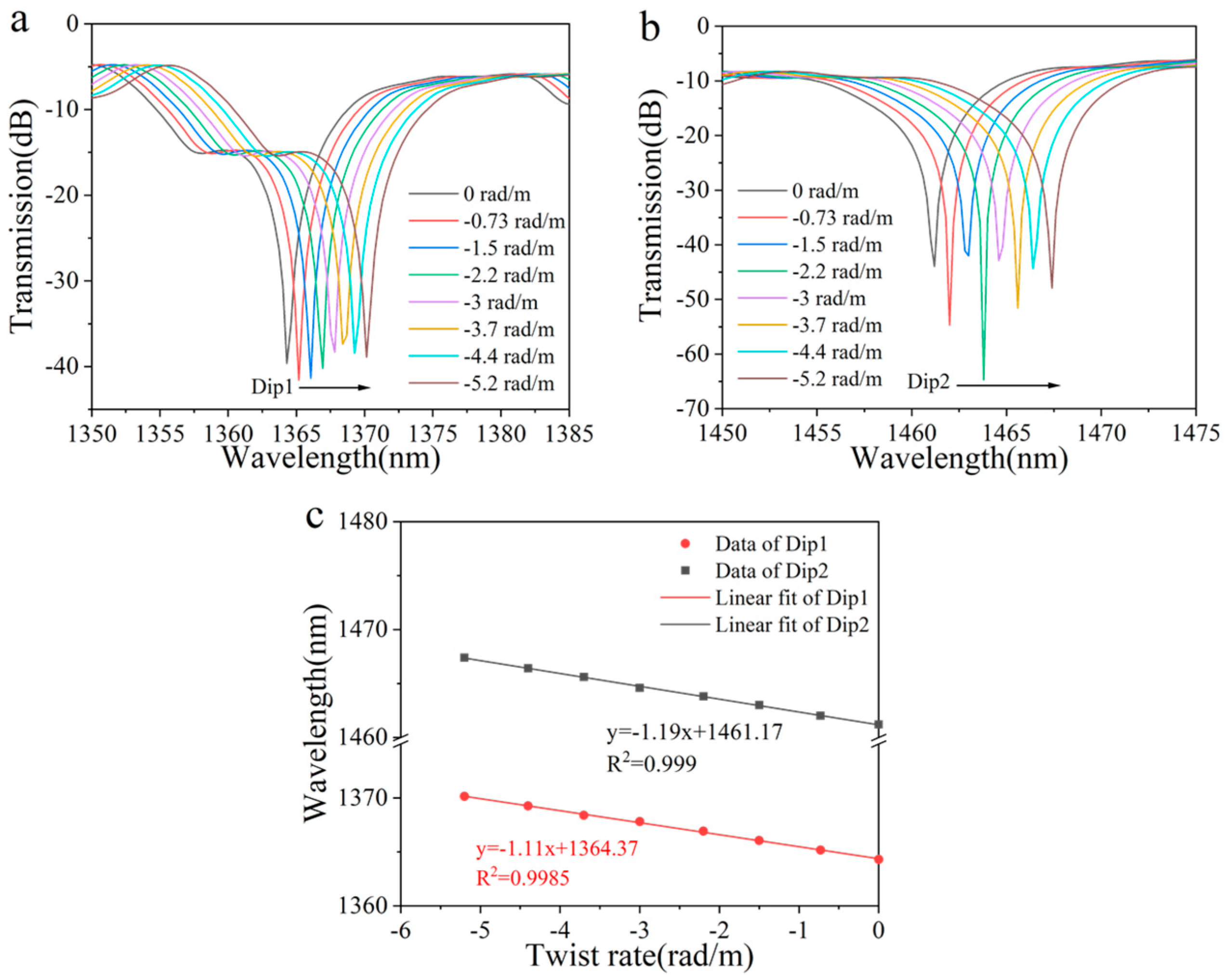
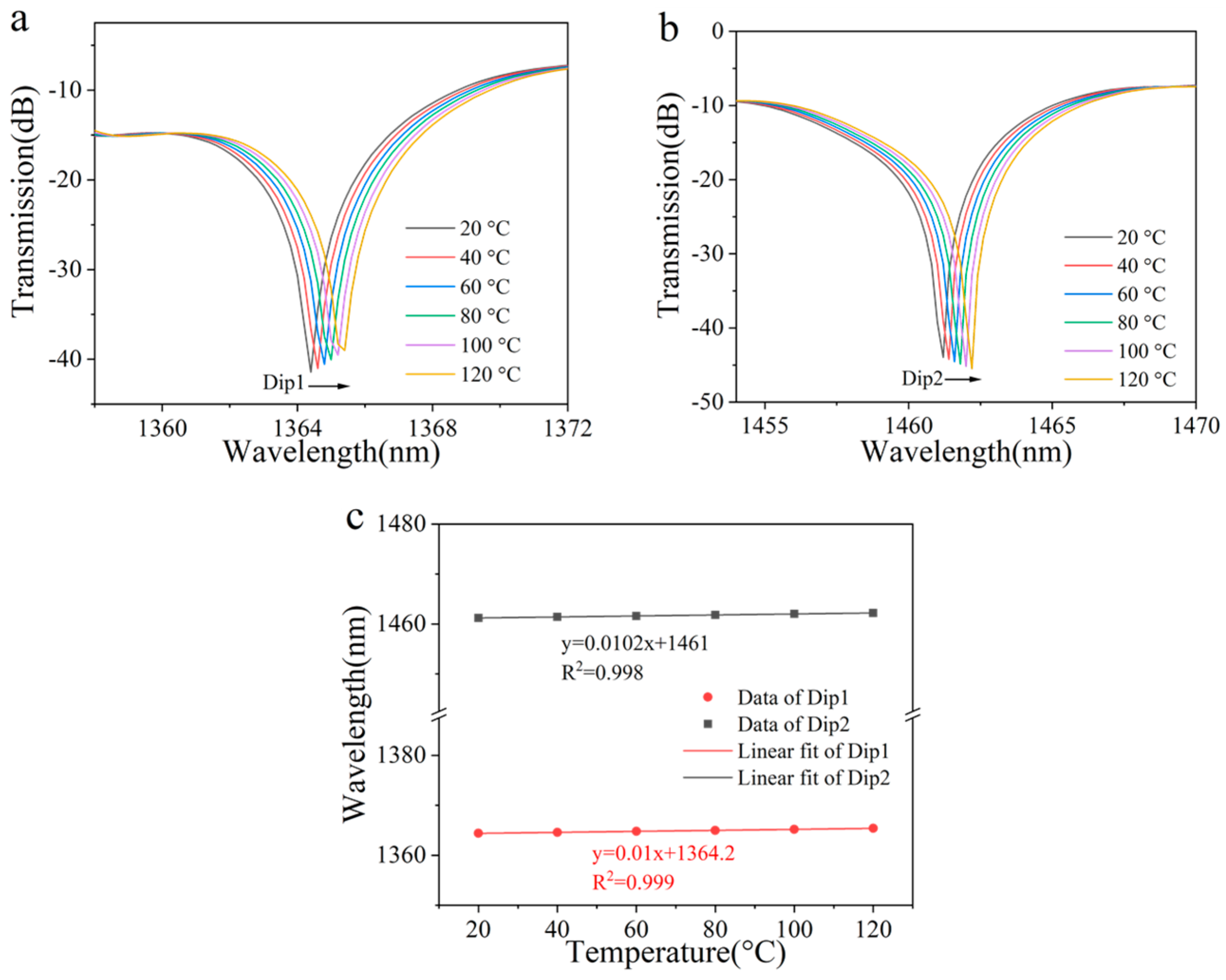
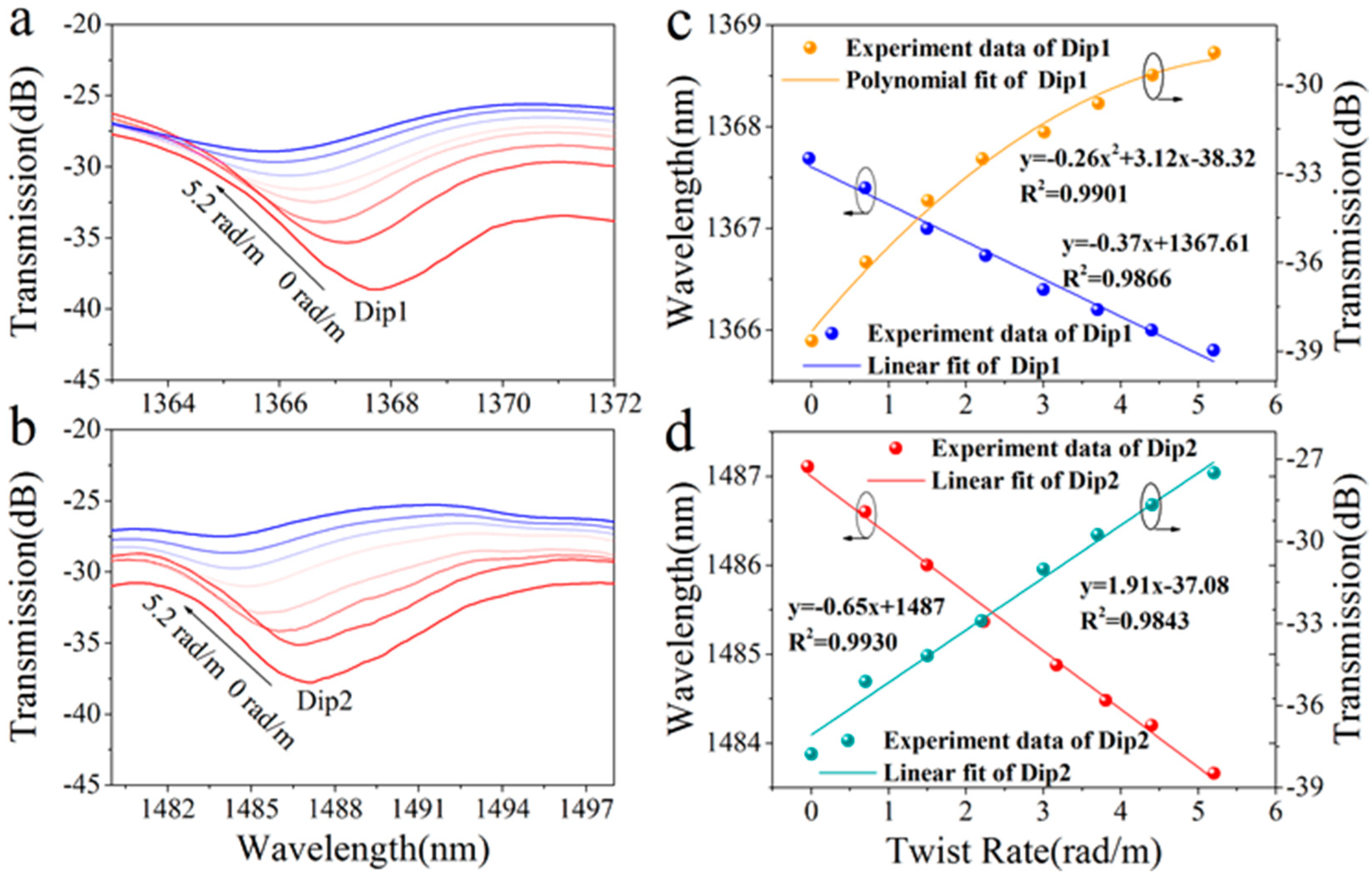
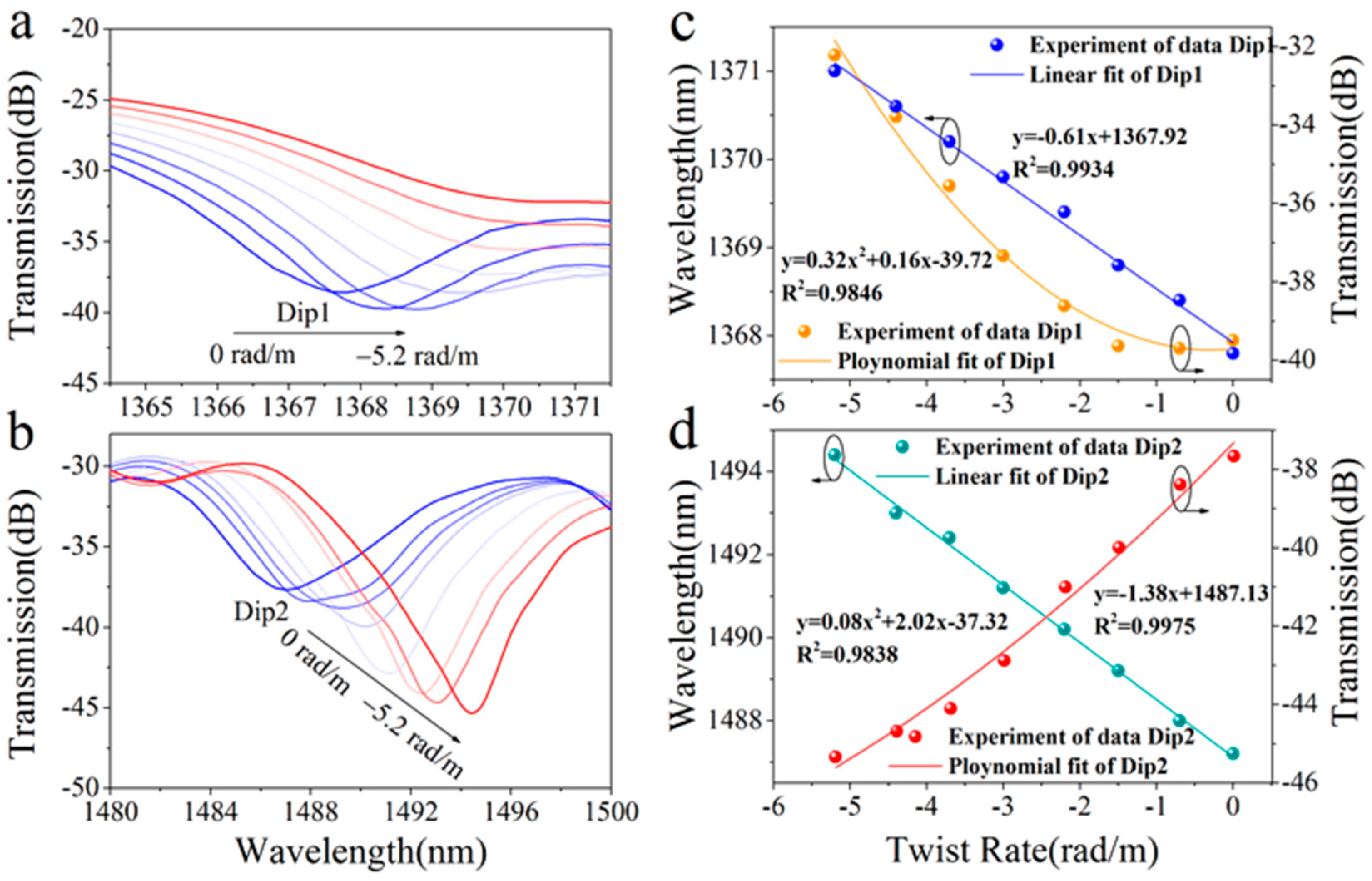
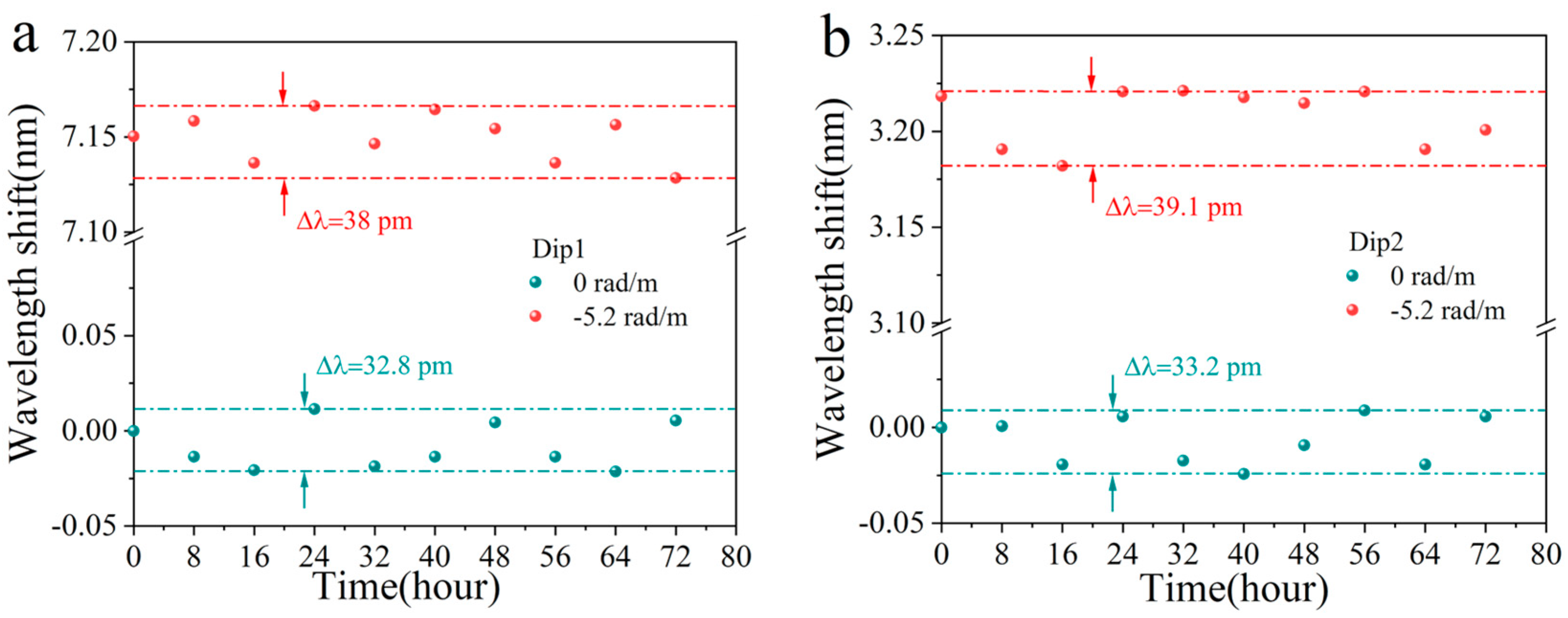
4. Experimental Measurements and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xian, L.; Wang, P.; Li, H. Power-interrogated and simultaneous measurement of temperature and torsion using paired helical long-period fiber gratings with opposite helicities. Opt. Express 2014, 22, 20260–20267. [Google Scholar] [CrossRef]
- Shen, F.; Shu, X.; Zhou, K.; Jiang, H.; Xia, H.; Xie, K.; Zhang, L. Compact vector twist sensor using a small period long period fiber grating inscribed with femtosecond laser. Chin. Opt. Lett. 2021, 19, 090601. [Google Scholar] [CrossRef]
- Zhang, L.; Liu, Y.; Cao, X. High sensitivity chiral long-period grating sensors written in the twisted fiber. IEEE Sens. J. 2016, 16, 4253–4257. [Google Scholar] [CrossRef]
- Shen, C.; Zhang, Y.; Zhou, W.; Albert, J. Au-coated tilted fiber Bragg grating twist sensor based on surface plasmon resonance. Appl. Phys. Lett. 2014, 104, 071106. [Google Scholar] [CrossRef]
- Xu, J.; Liu, T.; Dai, X.; Su, Y.; Shi, J.; Zheng, Y.; Dai, S.; Zhang, P. Multi-mode fiber Bragg grating for simultaneous detection of strain, torsion and temperature. Opt. Laser Technol. 2024, 179, 111293. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, M.; Huang, X. In fiber Bragg grating twist sensor based on analysis of polarization dependent loss. Opt. Express 2013, 21, 11913–11920. [Google Scholar]
- Sławomir, C.; Damian, H.; Piotr, K. Novel twist measurement method based on TFBG and fully optical ratiometric interrogation. Sens. Actuators A-Phys. 2018, 272, 18–22. [Google Scholar] [CrossRef]
- Wang, X.; Liu, C.; Wei, Y.; Liu, C.; Shi, C.; Yang, M.; Chen, L.; Liu, Z. Twist detection Mach-Zehnder interferometer based on optical vernier effect and its phase demodulation system. Measurement 2024, 235, 115016. [Google Scholar] [CrossRef]
- Li, J.; Zhang, M.; Li, S.; Chen, Q.; Yin, Z. An ultrasensitive twist sensor based on parallel dual HMSF-SIs with Vernier effect. Optik 2023, 286, 171012. [Google Scholar] [CrossRef]
- Fu, Q.; Zhang, J.; Liang, C.; Ikechukwu, I.; Yin, G.; Lu, L.; Shao, Y.; Liu, L.; Liu, D.; Zhu, T. Intensity-modulated directional torsion sensor based on in-line optical fiber Mach-Zehnder interferometer. Opt. Lett. 2018, 43, 2414–2417. [Google Scholar] [CrossRef]
- Zhang, Q.; Zhang, J.; Yang, S.; Zhai, R.; Xie, Y.; Li, Y. Microfiber optomechanical torsion sensor. Front. Phys. 2023, 11, 1147644. [Google Scholar] [CrossRef]
- Wo, J.; Jiang, M.; Malnou, M.; Sun, Q.; Zhang, J.; Shum, P.; Liu, D. Twist sensor based on axial strain insensitive distributed Bragg reflector fiber laser. Opt. Express 2012, 20, 2844–2850. [Google Scholar] [CrossRef] [PubMed]
- Yin, G.; Fu, Q.; Yang, P.; Zhu, T. Direction-discriminating torsion sensor based on optical fiber Mach-Zehnder interferometer. Opt. Laser Technol. 2022, 156, 108461. [Google Scholar] [CrossRef]
- Xu, Y.; Lin, H.; Zhou, A. A pre-twisted taper in dual-side hole fiber for torsion measurement with high sensitivity. IEEE Sens. J. 2020, 20, 7761–7765. [Google Scholar] [CrossRef]
- Guo, Q.; Zhu, Y.-Q.; Shan, T.-Q.; Pan, X.-P.; Liu, S.-R.; Xue, Z.-K.; Zheng, Z.-M.; Chen, C.; Yu, Y.-S. Intensity-modulated directional torsion sensor based on a helical fiber taper. Opt. Mater. Express 2021, 11, 80–88. [Google Scholar] [CrossRef]
- Wang, R.; Tian, K.; Zhang, M.; Zhang, M.; Farrell, G.; Lewis, E.; Yuan, L. Investigation on the dependence of directional torsion measurement on multimode fiber geometry. J. Lightw. Technol. 2022, 40, 3997–4002. [Google Scholar] [CrossRef]
- Kang, J.; Dang, S.; Wang, X.; Ye, K.; Bai, Y.; Wang, Y.; Xia, L.; Du, C. High-sensitivity LPFG torsion sensor based on indirect torsion slot. IEEE Sens. J. 2025, 25, 398–404. [Google Scholar] [CrossRef]
- Zhang, Y.; Fang, Z.; Zhou, G. A stable and highly sensitive directional torsion sensor based on a helical double cladding microstructured multicore fiber. IEEE Sens. J. 2024, 24, 6166–6172. [Google Scholar] [CrossRef]
- Liu, W.; Sun, C.; Ma, Y.; Bai, X.; Yu, L.; Duan, S.; Du, H.; Zhao, C.; Lu, C.; Zhao, L.; et al. A highly sensitive torsion sensor with a new fabrication method. IEEE Photon. Technol. Lett. 2019, 31, 463–466. [Google Scholar] [CrossRef]
- He, X.; Zhou, J.; Meng, L.; Zhang, K.; Wang, X.; Zhang, S.; Li, W.; Yuan, L. Directional torsion sensor based on long period fiber gratings inscribed by periodically micro taper. Opt. Fiber Technol. 2022, 71, 102908. [Google Scholar] [CrossRef]
- Jiang, C.; Liu, Y.; Huang, L.; Mou, C. Double cladding fiber chiral long-period grating-based directional torsion sensor. IEEE Photonics Technol. Lett. 2019, 31, 1522–1525. [Google Scholar] [CrossRef]
- Lu, C.; Zeng, F.; Rui, Z.; Xiang, Z.; Geng, T.; Sun, C.; Yuan, L. Helical sensor for simultaneous measurement of torsion and temperature. Opt. Laser Technol. 2023, 158, 108934. [Google Scholar] [CrossRef]
- Zhang, F.; Liu, S.; Wang, Y.; Huang, Y.; Xu, X.; Fu, C.; Wu, T.; Liao, C.; Wang, Y. Highly sensitive torsion sensor based on directional coupling in twisted photonic crystal fiber. Appl. Phys. Express 2018, 11, 042501. [Google Scholar] [CrossRef]
- Zhang, F.; Xu, R.; Wei, J.; Li, Y.; Song, Z.; Hu, J. In-line Mach-Zehnder interferometer for simultaneous measurement of temperature and directional torsion. Optik 2021, 226, 165497. [Google Scholar] [CrossRef]
- Song, Z.; Li, Y.; Hu, J. Directional torsion sensor based on a two-core fiber with a helical structure. Sensors 2023, 23, 2874. [Google Scholar] [CrossRef] [PubMed]
- Wei, Y.; Wang, X.; Jiang, T.; Liu, C.; Liu, C.; Shi, C.; Yang, M.; Chen, L.; Wang, R.; Liu, Z. High sensitivity interference torsion sensor based on dumbbell fiber. IEEE Sens. J. 2023, 23, 24552–24557. [Google Scholar] [CrossRef]
- Malka, D.; Berkovic, G.; Tischler, Y.; Zalevsky, Z. Super-resolved raman spectra of toluene and toluene-chlorobenzene mixture. Spectrosc. Lett. 2015, 48, 431–435. [Google Scholar] [CrossRef]
- Malka, D.; Berkovic, G.; Hammer, Y.; Zalevsky, Z. Super-resolved raman spectroscopy. Spectrosc. Lett. 2013, 46, 307–313. [Google Scholar] [CrossRef]
- Yang, C.; Wang, Z.; Xiao, K.; Ushakov, N.; Umar, S.K.; Li, X.; Min, R. Portable optical fiber biosensors integrated with smartphone: Technologies, applications, and challenges. Biomed. Opt. Express 2024, 15, 1630–1650. [Google Scholar] [CrossRef]
- Palmieri, L.; Galtarossa, A. Twist effects in multi-mode fibers. In Proceedings of the 2014 3rd Mediterranean Photonics Conference, Trani, Italy, 7–9 May 2014; pp. 1–3. [Google Scholar]
- Tian, K.; Xin, Y.; Yang, W.; Geng, T.; Ren, J.; Fan, Y.-X.; Farrell, G.; Lewis, E.; Wang, P. A curvature sensor based on twisted single-mode-multimode-single-mode hybrid optical fiber structure. J. Lightw. Technol. 2017, 35, 1725–1731. [Google Scholar] [CrossRef]
- Palmieri, L. Coupling mechanism in multimode fibers. In Proceedings of the SPIE—The International Society for Optical Engineering, San Francisco, CA, USA, 1 February 2014; Volume 9009, p. 90090G. [Google Scholar]
- Zhang, H.; Wu, Z.; Shum, P.P.; Shao, X.; Wang, R.; Dinh, X.Q.; Fu, S.; Tong, W.; Tang, M. Directional torsion and temperature discrimination based on a multicore fiber with a helical structure. Opt. Express 2018, 26, 544–551. [Google Scholar] [CrossRef] [PubMed]



| Sensor Structure/Type | Torsion Sensitivity | Direction Discrimination | Linearity/ R-Square | Dynamic Range | Temperature Sensitivity | Temperature Crosstalk |
|---|---|---|---|---|---|---|
| MSM fiber structure [7] | −0.361 nm/rad·m−1 | No | Good linearity/0.999 | 0~60 rad·m−1 | 1.792 nm/°C | 4.96 (rad/m)/°C |
| Twisted MCF [33] | 0.118 nm/rad·m−1 | Yes | Good linearity/0.993 | (−19.943~15.669) rad·m−1 | 101 pm/°C | 0.86 (rad/m)/°C |
| Twisted PCF [23] | 0.208 nm/rad·m−1 | Yes | Good linearity/0.99 | −76~76 rad·m−1 | — | — |
| Helical two-core fiber [25] | 0.242 nm/rad·m−1 and −0.04 dB/° | Yes | Non-linear/0.999 | −60°~60° | 32 pm/°C | 0.13 (rad/m)/°C |
| Pre-twist LPFG [22] | −0.654 nm/rad·m−1 | Yes | Good linearity/— | −12.6~12.6 rad·m−1 | 66.8 pm/°C | 0.1 (rad/m)/°C |
| MMF–SCF–MMF [24] | 1.837 dB/rad·m−1 | Yes | Good linearity/— | 0~3.5 rad·m−1 | 70 pm/°C and −0.296 dB/°C | 0.16 (rad/m)/°C |
| Twisted MMF (this work) | −1.38 nm/rad·m−1 | Yes | Good linearity/0.998 | −5.2~5.2 rad·m−1 | 10 pm/°C | 0.0072 (rad/m)/°C |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, W.; Tian, K.; Li, L. Directional Torsion Sensor Based on a Paired Helical-Type Multimode Fiber. Sensors 2025, 25, 2091. https://doi.org/10.3390/s25072091
Yang W, Tian K, Li L. Directional Torsion Sensor Based on a Paired Helical-Type Multimode Fiber. Sensors. 2025; 25(7):2091. https://doi.org/10.3390/s25072091
Chicago/Turabian StyleYang, Wenlei, Ke Tian, and Le Li. 2025. "Directional Torsion Sensor Based on a Paired Helical-Type Multimode Fiber" Sensors 25, no. 7: 2091. https://doi.org/10.3390/s25072091
APA StyleYang, W., Tian, K., & Li, L. (2025). Directional Torsion Sensor Based on a Paired Helical-Type Multimode Fiber. Sensors, 25(7), 2091. https://doi.org/10.3390/s25072091








