An Underwater Velocity-Independent DOA Estimation Based on Improved Toeplitz Matrix Reconstruction
Abstract
1. Introduction
2. Models and Methods
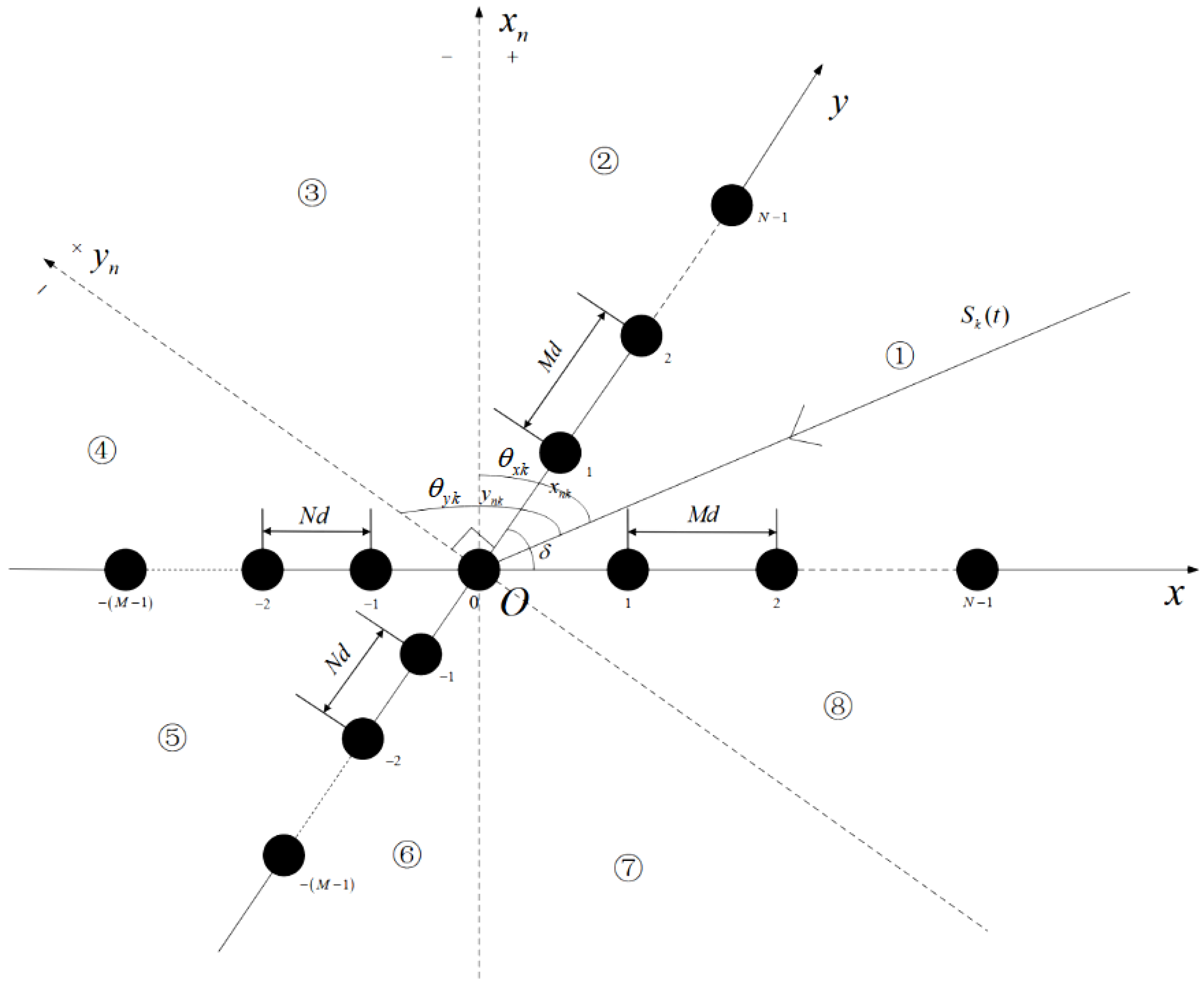
2.1. Signal Model
2.2. Low-Rank Matrix Reconstruction
2.3. Proposed Method
| Algorithm 1 Framework of ECC-TVI-ESPRIT Algorithm |
| Input: A cross-expanded coprime array with a crossing angle of , where each array consists of M elements. The received signal matrices . Output:
|
2.4. Computational Complexity Analysis
3. Results and Discussion
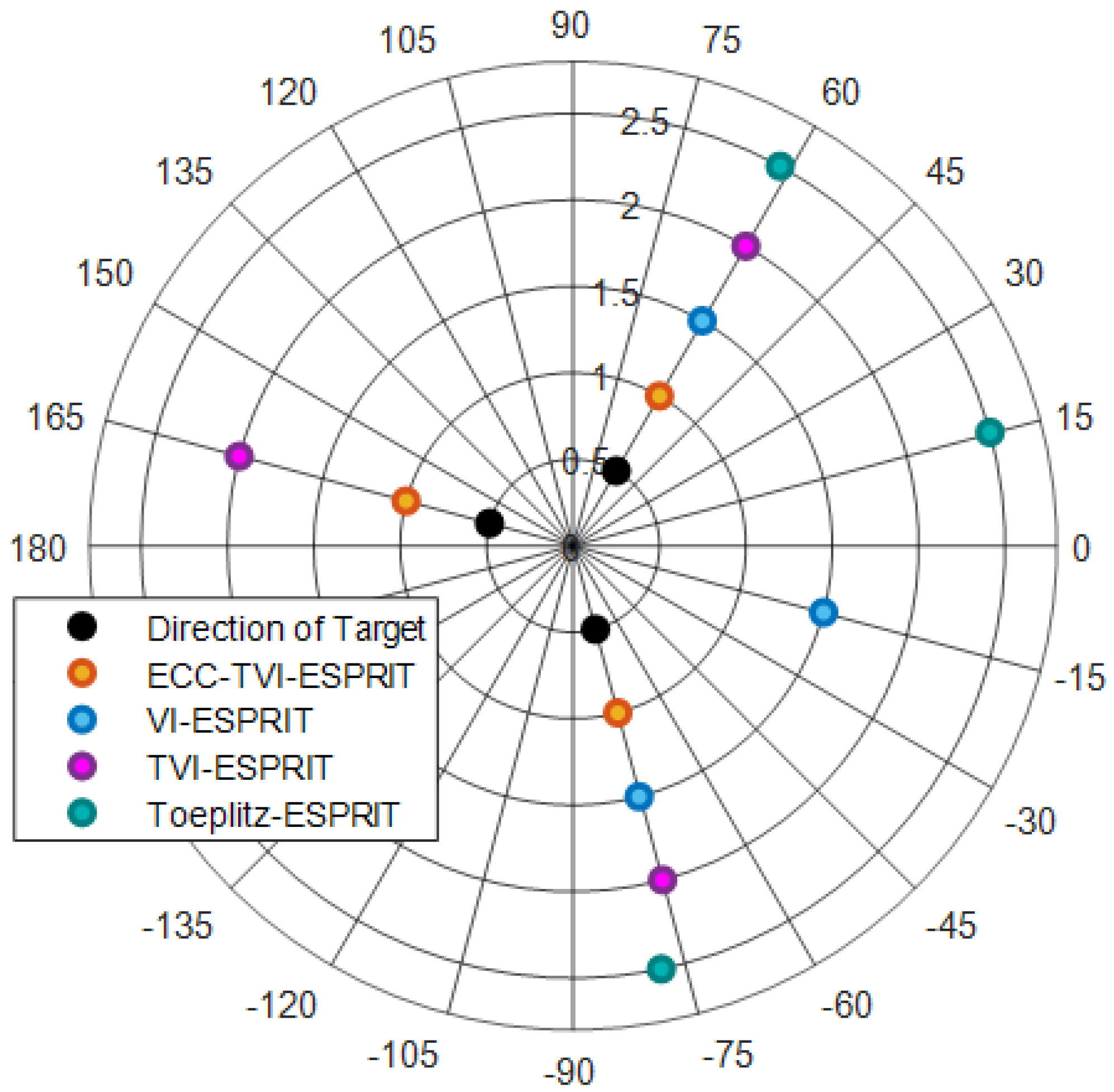
3.1. Algorithm Validity Test
3.2. Performance Comparison Under Different SNR
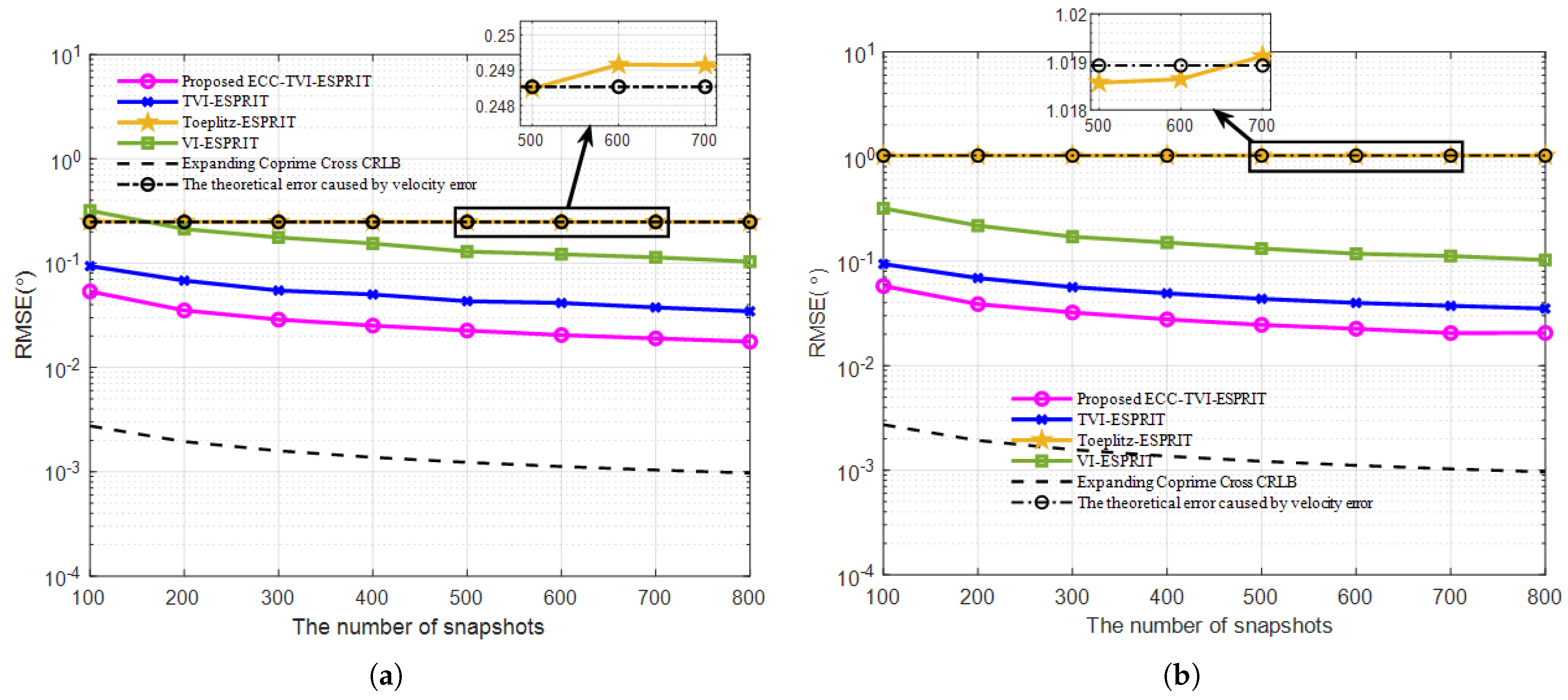
3.3. Performance Comparison Under Different Number of Snapshots
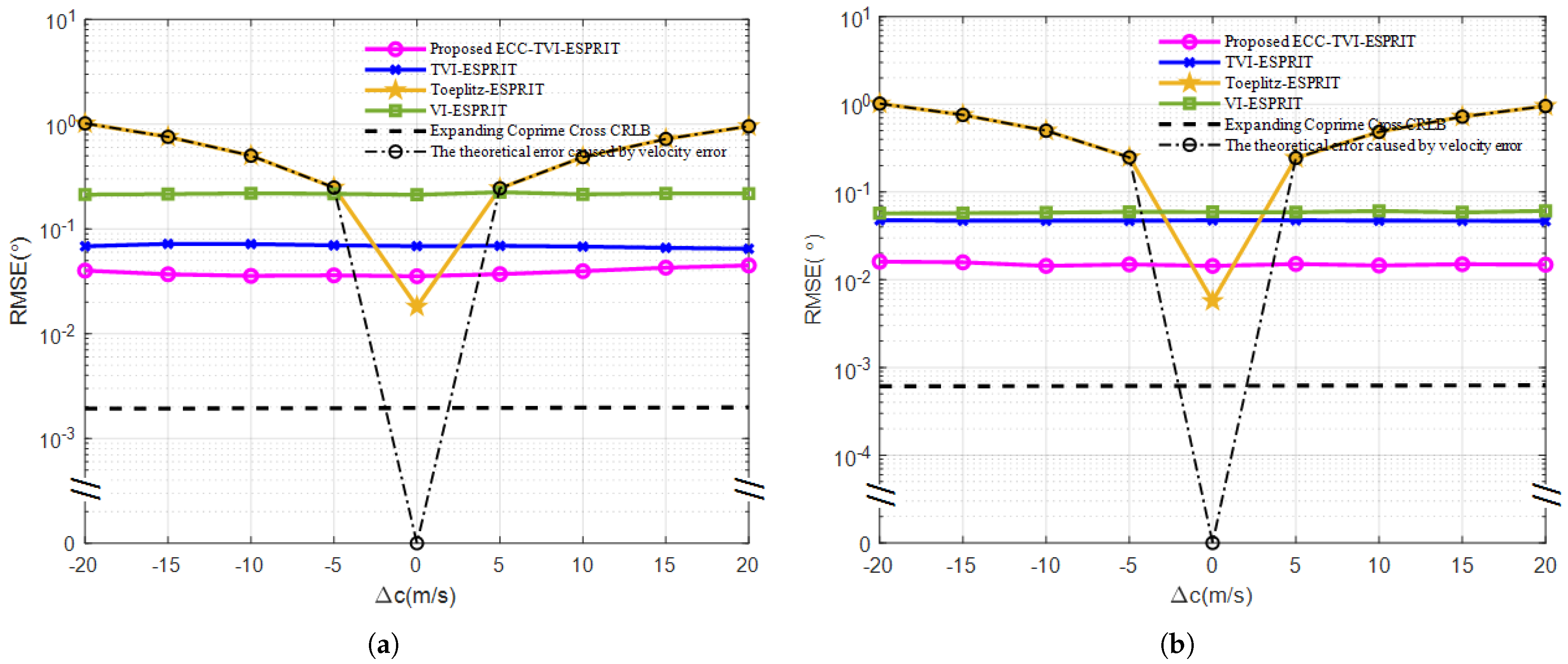
3.4. Performance Comparison Under Different Acoustic Velocity Errors
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, Z.; Shi, J.; Wen, F. Phase compensation-based 2d-doa estimation for emvs-mimo radar. IEEE Trans. Aerosp. Electron. Syst. 2024, 60, 1299–1308. [Google Scholar] [CrossRef]
- Lei, Z.; Lei, X.; Wang, N.; Zhang, Q. Present status and challenges of underwater acoustic target recognition technology: A review. Front. Phys. 2022, 10, 3389. [Google Scholar]
- Schmidt, R. Multiple emitter location and signal parameter estimation. IEEE Trans. Antennas Propag. 1986, 34, 276–280. [Google Scholar]
- Roy, R.; Paulraj, A.; Kailath, T. ESPRIT–A subspace rotation approach to estimation of parameters of cisoids in noise. IEEE Trans. Acoust. Speech Signal Process. 1986, 34, 1340–1342. [Google Scholar]
- Si, Y. Improved TLS-ESPRIT Algorithm Research for Uniform Line Array. In Proceedings of the 3rd International Conference on Computer Science and Service System, Bangkok, Thailand, 13–15 June 2014. [Google Scholar]
- Haardt, M.; Nossek, J.A. Unitary ESPRIT: How to obtain increased estimation accuracy with a reduced computational burden. IEEE Trans. Signal Process. 1995, 43, 1232–1242. [Google Scholar]
- Swindlehurst, A.L.; Ottersten, B.; Roy, R.; Kailath, T. Multiple invariance ESPRIT. IEEE Trans. Signal Process. 1992, 40, 867–881. [Google Scholar]
- Ning, G.; Wang, B.; Zhou, C.; Feng, Y. A velocity independent MUSIC algorithm for DOA estimation. In Proceedings of the 2017 IEEE International Conference on Signal Processing, Communications and Computing (ICSPCC), Xiamen, China, 22–25 October 2017. [Google Scholar]
- Ning, G.; Jing, G.; Li, X.; Zhao, X. Velocity-independent and low-complexity method for 1D DOA estimation using an arbitrary cross-linear array. EURASIP J. Adv. Signal Process. 2020, 28, 28. [Google Scholar] [CrossRef]
- Nishimura, R.; Takizawa, K. Simultaneous Estimation of Direction of Arrival and Sound Speed Using a Non-Uniform Sensor Array. In Proceedings of the 2023 IEEE International Conference on Acoustics, Speech and Signal Processing (ICASSP), Rhodes Island, Greece, 4–10 June 2023. [Google Scholar]
- Ning, G.; Wang, Y.; Jing, G.; Zhao, X. A Velocity-independent DOA Estimator of Underwater Acoustic Signals via an Arbitrary Cross-linear Nested Array. Circuits Syst. Signal Process. 2023, 42, 996–1010. [Google Scholar] [CrossRef]
- Moffet, A. Minimum-redundancy linear arrays. IEEE Trans. Antennas Propag. 1968, 16, 172–175. [Google Scholar] [CrossRef]
- Vertatschitsch, E.; Haykin, S. Nonredundant arrays. Proc. IEEE 1986, 74, 217. [Google Scholar] [CrossRef]
- Pal, P.; Vaidyanathan, P.P. Nested arrays: A novel approach to array processing with enhanced degrees of freedom. IEEE Trans. Signal Process. 2010, 58, 4167–4181. [Google Scholar] [CrossRef]
- Vaidyanathan, P.P.; Pal, P. Sparse sensing with coprime samplers and arrays. IEEE Trans. Signal Process. 2011, 59, 573–586. [Google Scholar] [CrossRef]
- Zhao, P.; Wu, Q.; Chen, Z.; Hu, G.; Wang, L.; Wan, L. Generalized nested array configuration family for direction-of-arrival estimation. IEEE Trans. Veh. Technol. 2023, 72, 10380–10392. [Google Scholar] [CrossRef]
- Zhou, L.; Qi, J.; Hong, S. Enhanced dilated nested arrays with reduced mutual coupling for DOA estimation. IEEE Sens. J. 2024, 24, 615–623. [Google Scholar] [CrossRef]
- Wang, X.; Zhao, L.; Jiang, Y. Super augmented nested arrays: A new sparse array for improved DOA estimation accuracy. IEEE Signal Process. Lett. 2024, 31, 26–30. [Google Scholar]
- Bazzi, A.; Slock, D.T.M.; Meilhac, L. Online angle of arrival estimation in the presence of mutual coupling. In Proceedings of the 2016 IEEE Statistical Signal Processing Workshop (SSP), Palma de Mallorca, Spain, 26–29 June 2016. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, X.; Lei, Z.; Wang, Y.; Ning, G. An Underwater Velocity-Independent DOA Estimation Based on Improved Toeplitz Matrix Reconstruction. Sensors 2025, 25, 1965. https://doi.org/10.3390/s25071965
Zhao X, Lei Z, Wang Y, Ning G. An Underwater Velocity-Independent DOA Estimation Based on Improved Toeplitz Matrix Reconstruction. Sensors. 2025; 25(7):1965. https://doi.org/10.3390/s25071965
Chicago/Turabian StyleZhao, Xuejin, Zihan Lei, Yu Wang, and Gengxin Ning. 2025. "An Underwater Velocity-Independent DOA Estimation Based on Improved Toeplitz Matrix Reconstruction" Sensors 25, no. 7: 1965. https://doi.org/10.3390/s25071965
APA StyleZhao, X., Lei, Z., Wang, Y., & Ning, G. (2025). An Underwater Velocity-Independent DOA Estimation Based on Improved Toeplitz Matrix Reconstruction. Sensors, 25(7), 1965. https://doi.org/10.3390/s25071965




