Detection of Lunar Regolith Acquired by Excavator Using Radiofrequency (RF) Sensors
Abstract
1. Introduction
2. Materials and Methods
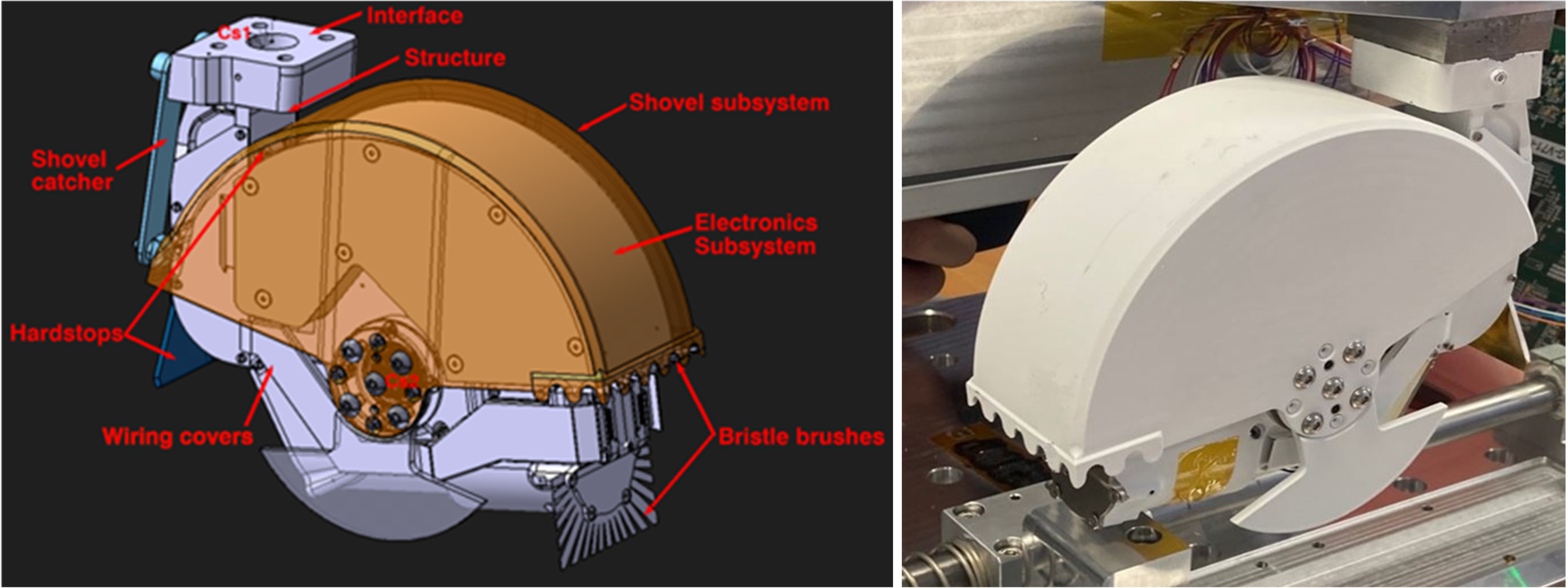
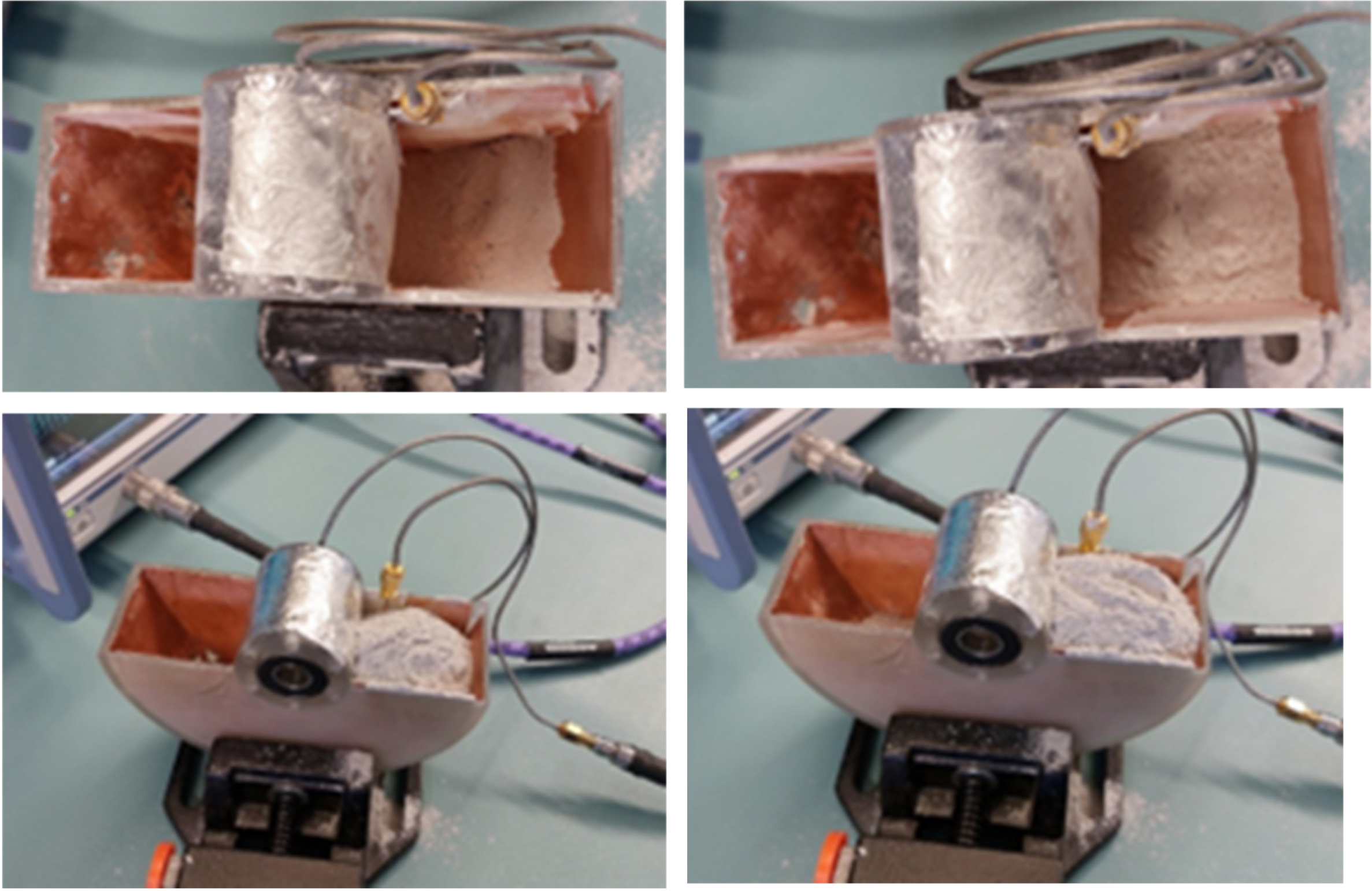
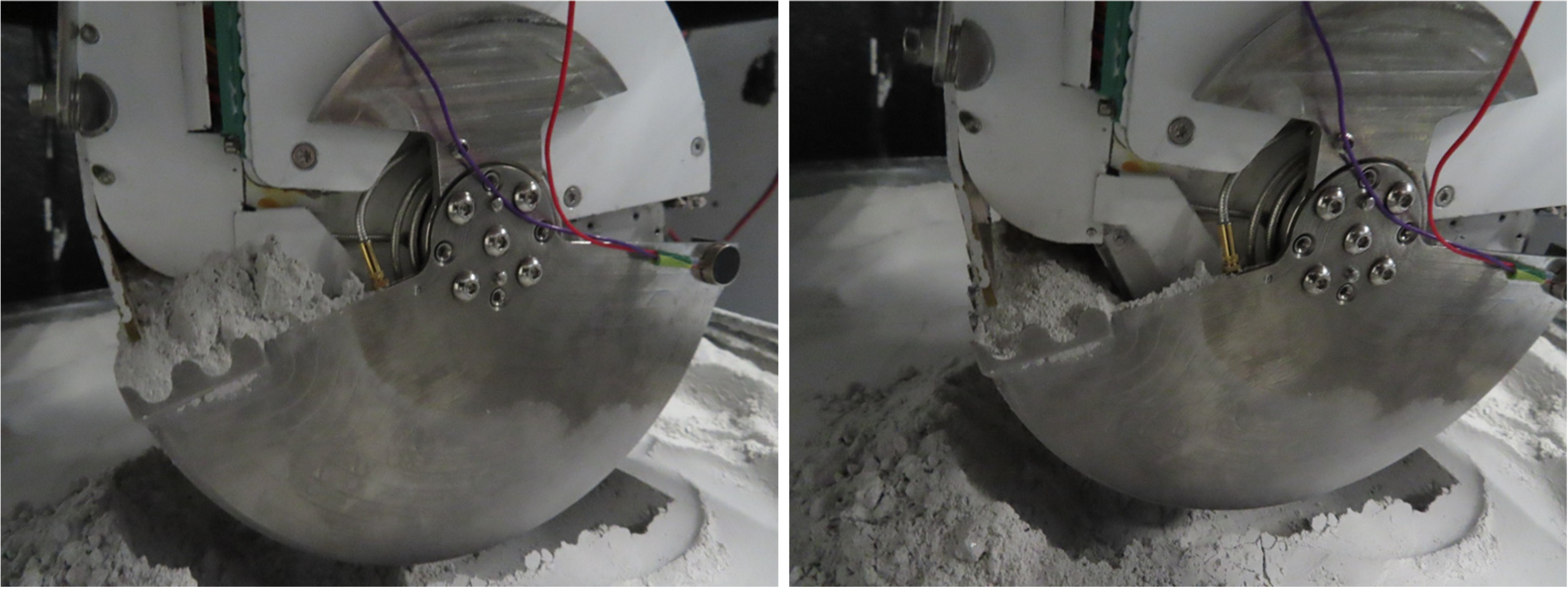
2.1. Rotary Clamshell Excavator Description
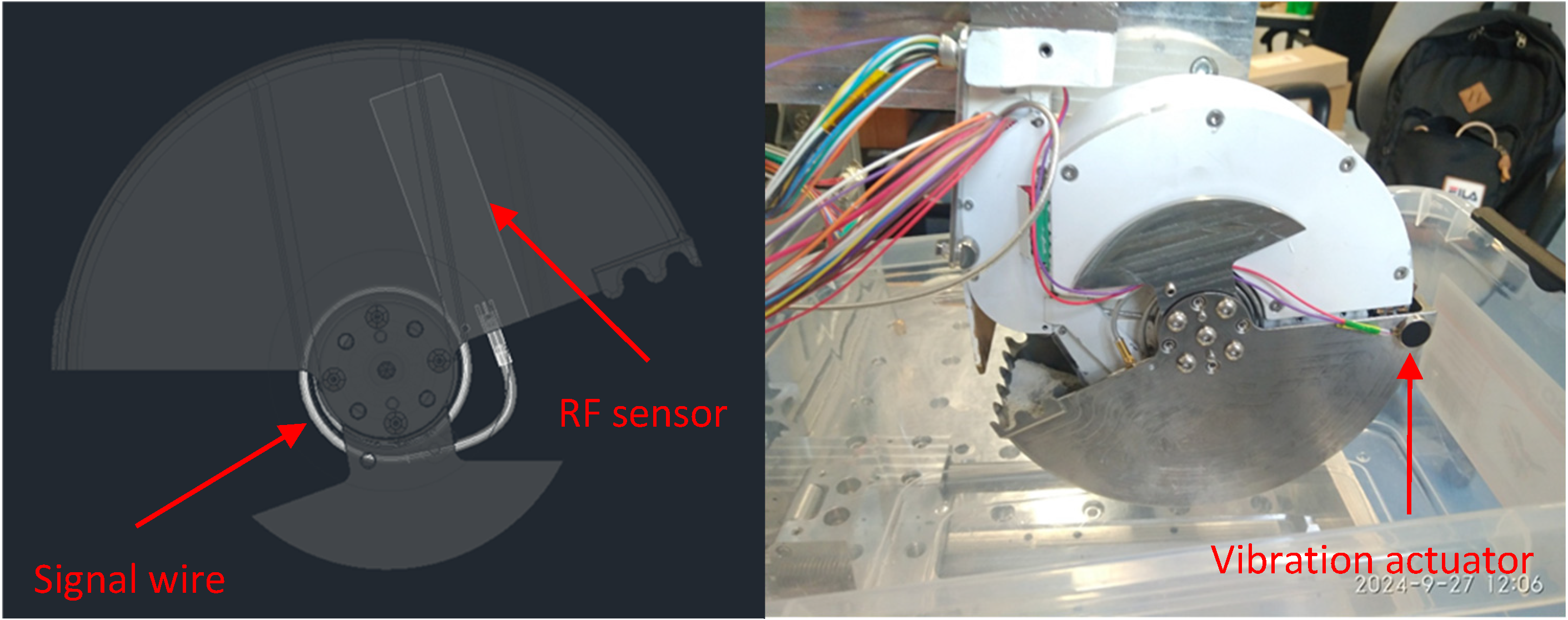
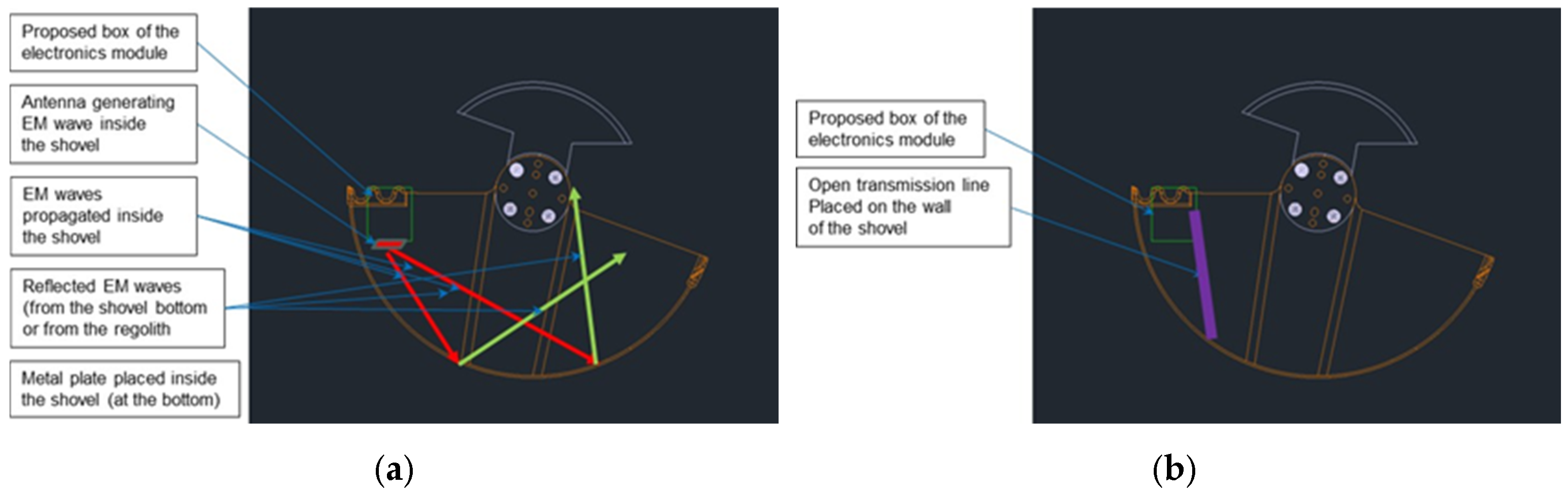
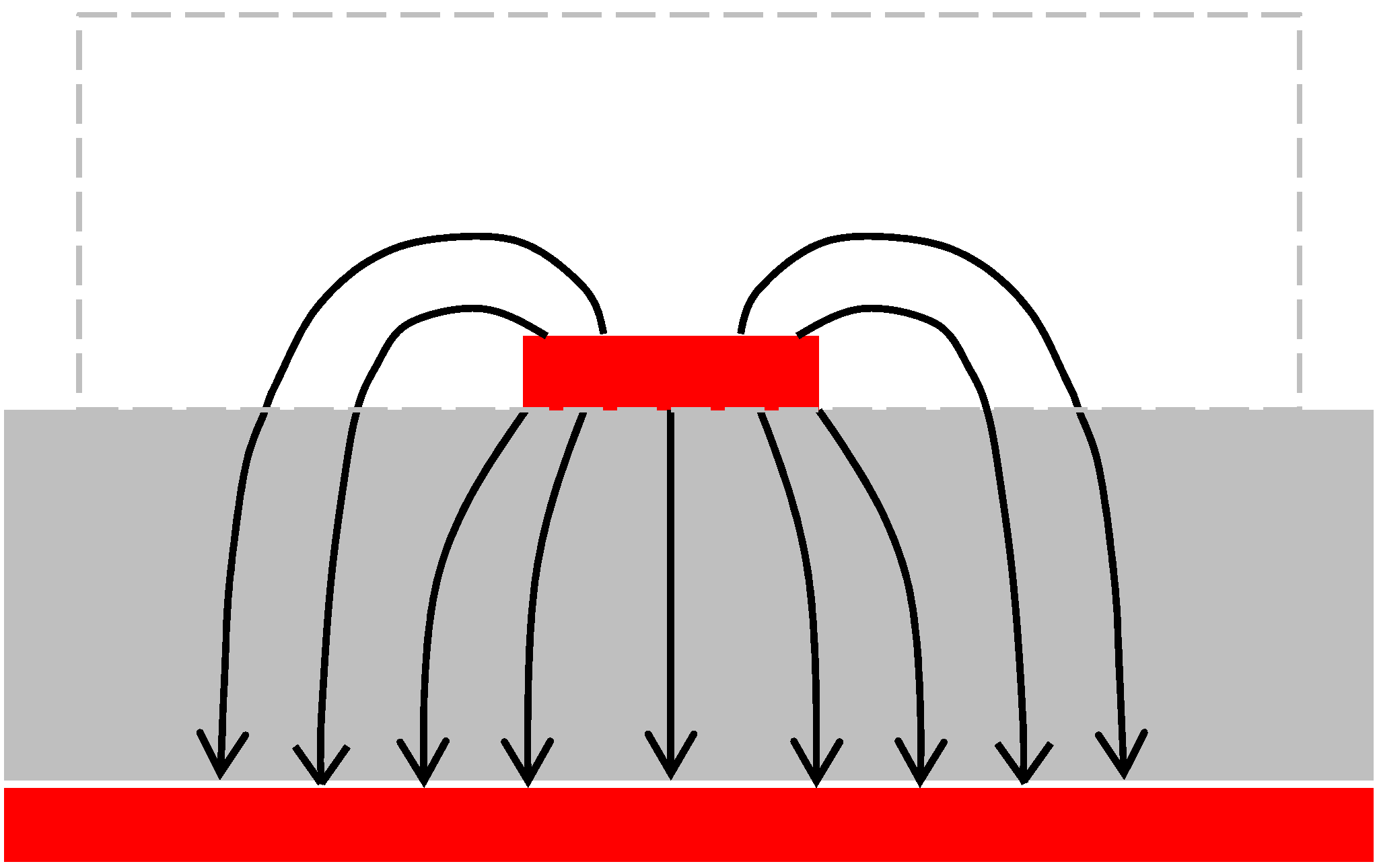
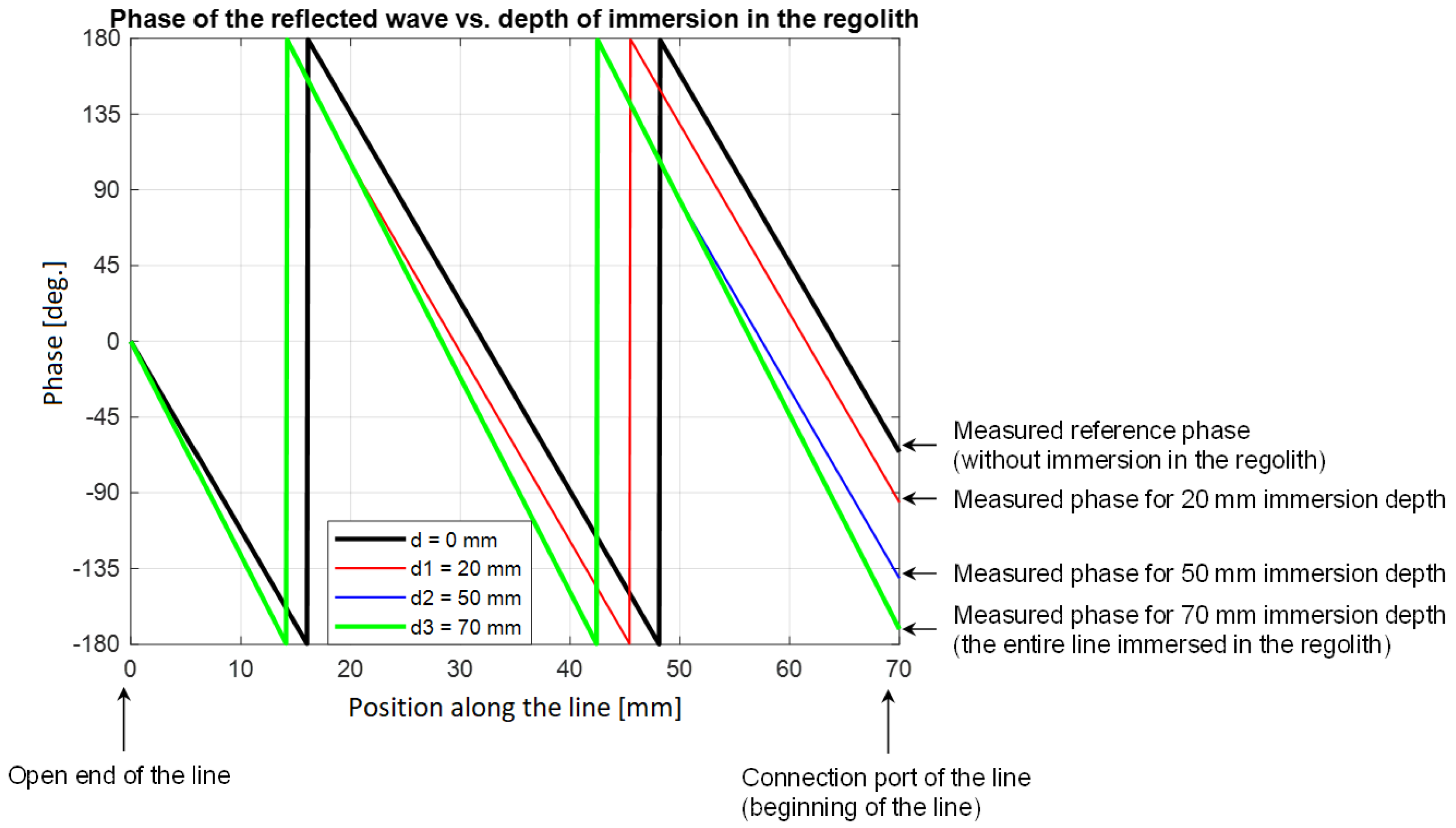
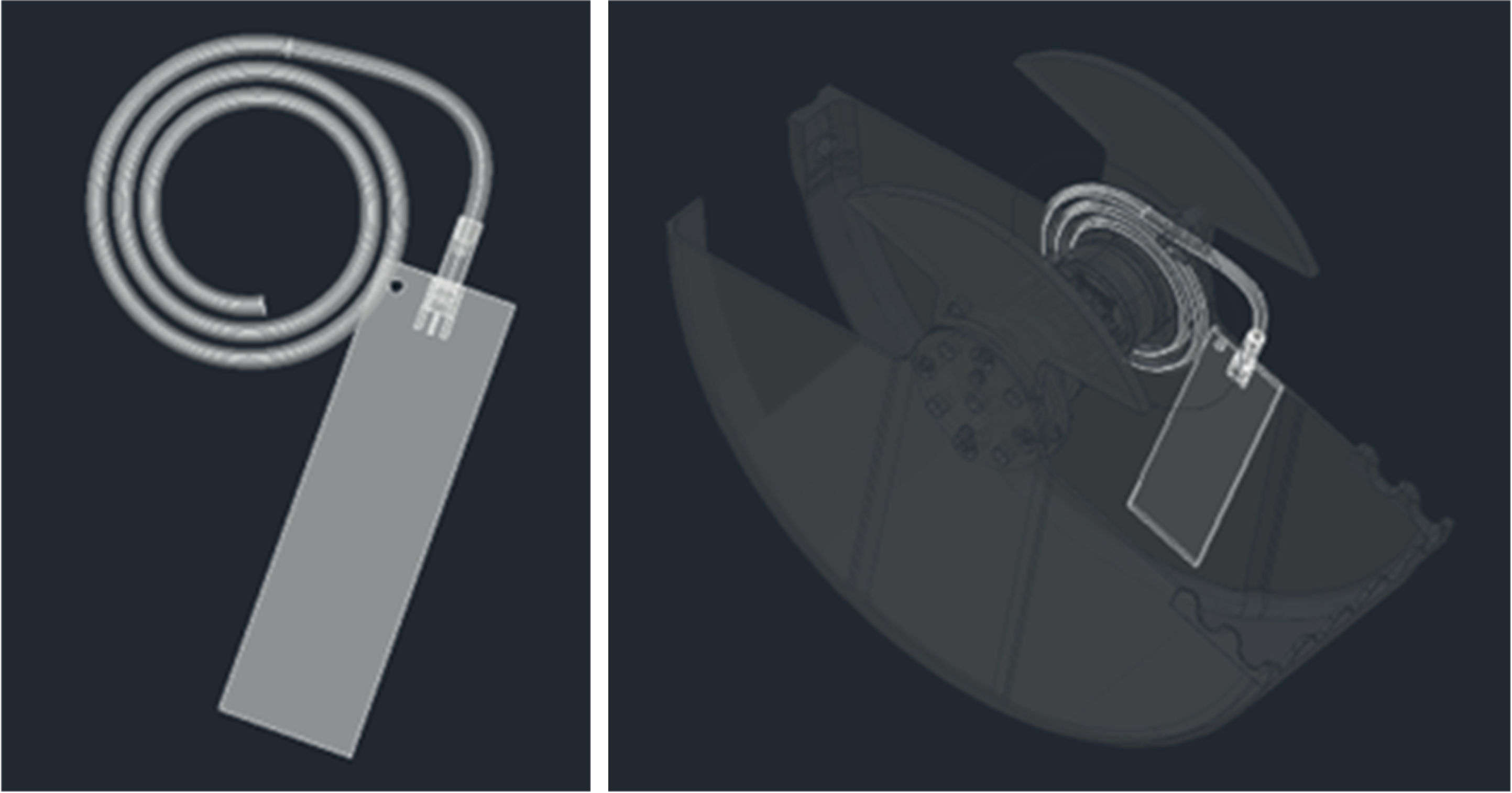
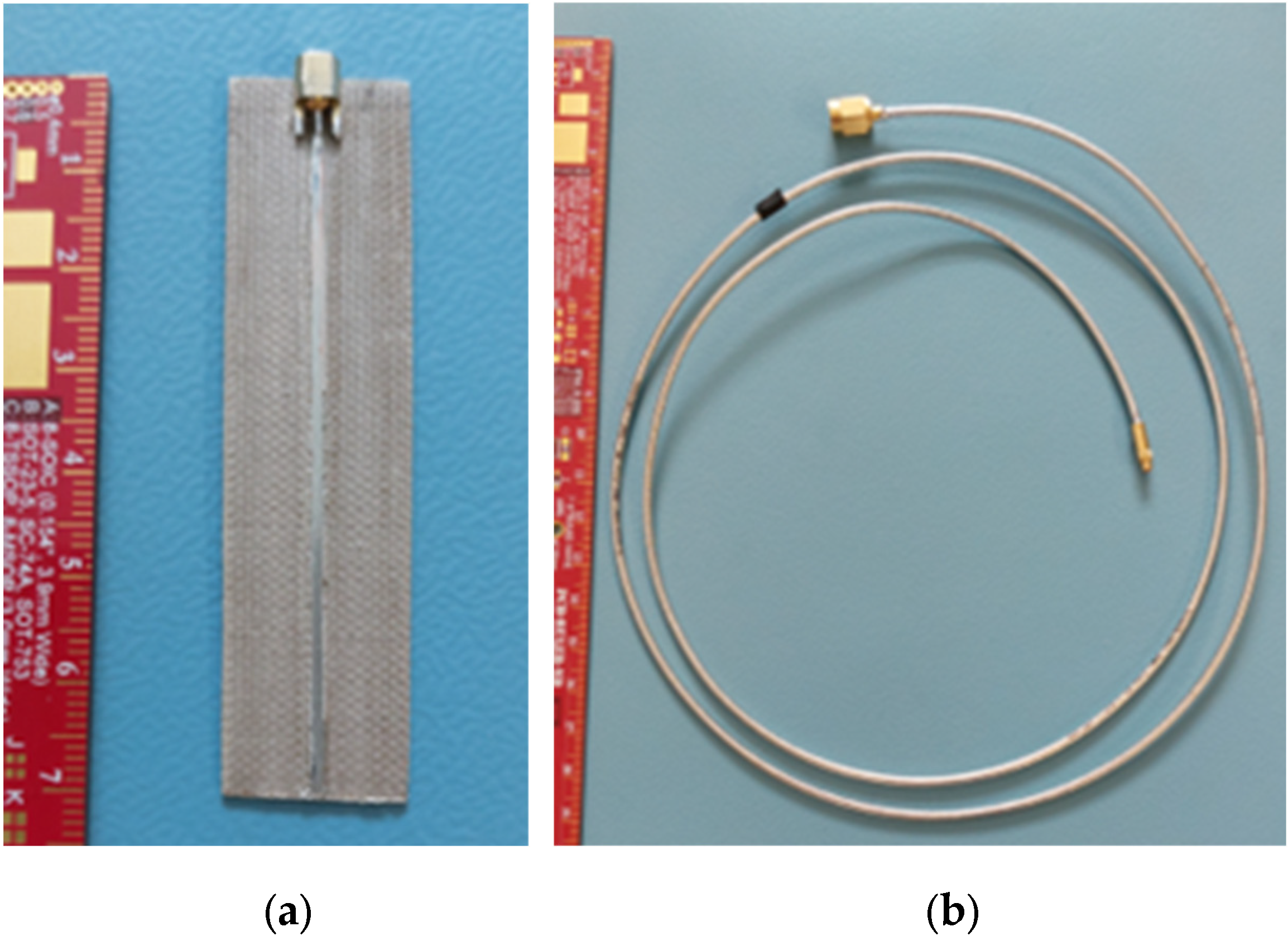
2.2. Concepts of the RF Sensor
- A patch antenna placed on top of the shovel, designed to transmit the EM wave inside the shovel and receive the reflected wave.
- A microstrip line with an open (or short) end, placed on the inner wall of the shovel and immersed in the regolith.
- A metal ground plane at the bottom.
- A dielectric layer in the middle.
- A metal strip at the top.
2.3. Proof of RF Sensor Concept Operation
- Series S—where the regolith was poured into the shovel without spreading or smoothing it inside the shovel.
- Series R—where the regolith was poured into the shovel and then smoothed by shaking or using a spoon to ensure even distribution inside the shovel.
- Determination of the amount of the regolith inside the shovel should be performed in two steps: a measurement for the empty shovel before scooping and a measurement after scooping the regolith into the shovel, considering the difference in the measured phase of S11 relative to the value for the empty shovel.
- Smoothing the regolith inside the shovel after scooping can significantly increase the accuracy of estimating the amount of the regolith in the shovel (two to three times smaller standard deviation of the measured phase difference of the S11 coefficient compared to the empty shovel).
- The accuracy of the mass estimation was evaluated using a linear approximation function, validated against a separate dataset that was not included in the calibration process. The analysis revealed an average absolute error of 8.0 g in the estimated regolith mass. Correspondingly, the average relative error was calculated to be 10.2%.
3. Results
3.1. Measurement Setup
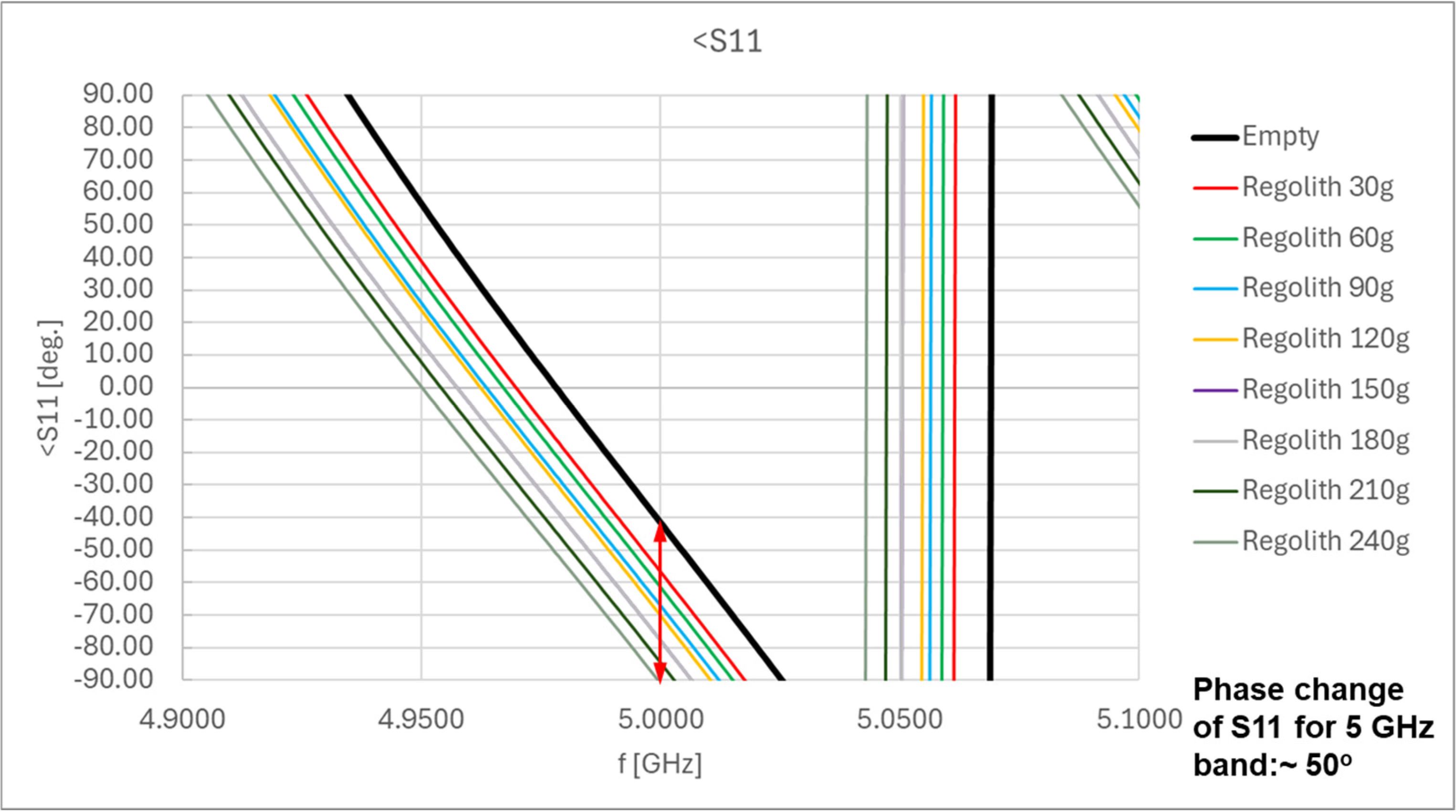
- Measurement of the S11 phase for empty shovel—used for determining the reference phase.
- Measurement of the S11 phase for the shovel with regolith inside after its acquisition.
- Regolith without shaking (before shaking).
- Regolith after shaking.
- JYC1234 (JIE YI Electronics Limited, Shenzen, China): A 3 VDC coin motor with a rotational speed of 12,000 rpm.
- VJP16-70E310 (Vybronics, Hong Kong, China): A 7.2 VDC vibration motor with a rotational speed of 8250 rpm.
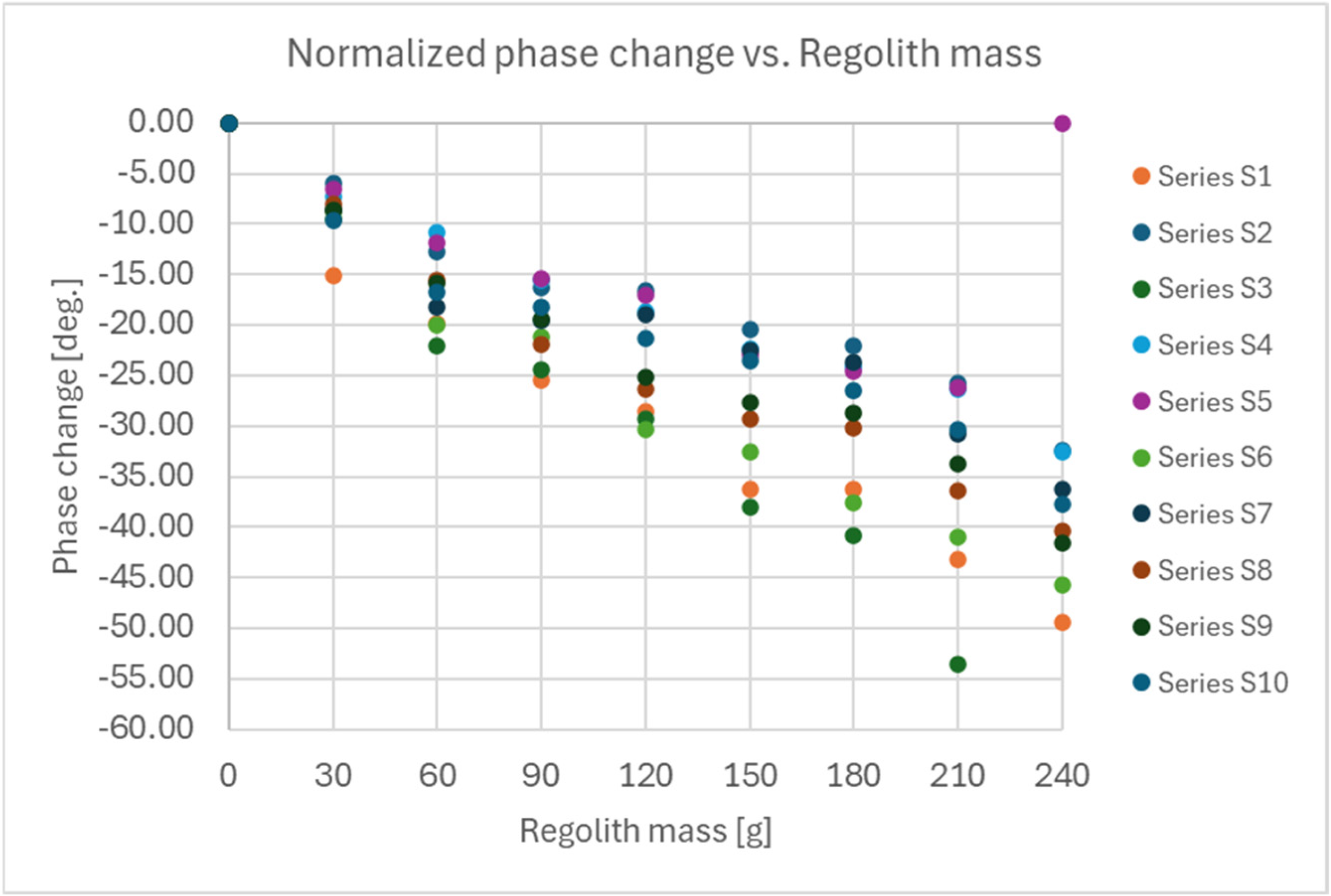
3.2. Measurement Results
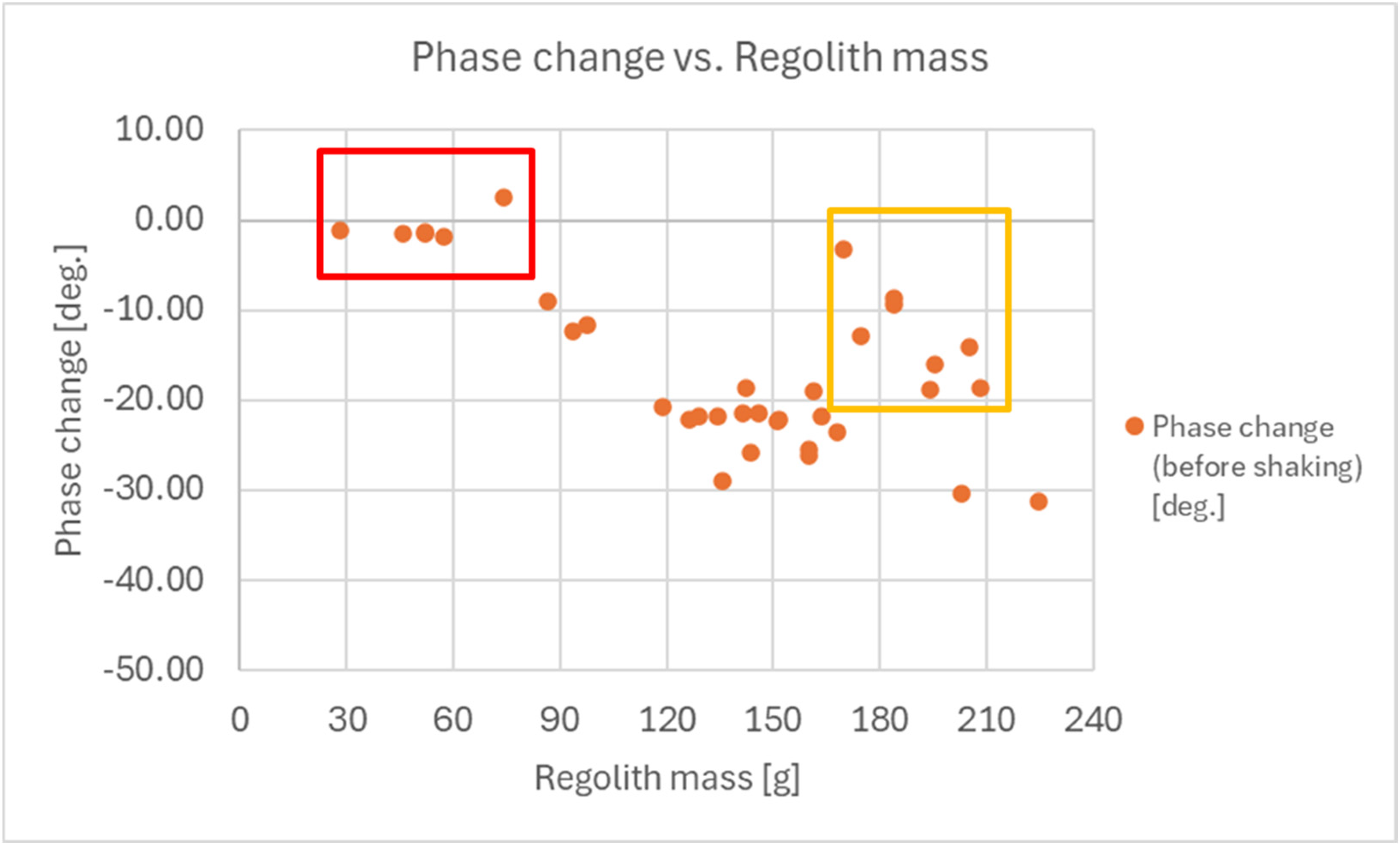
- No phase changes for a small amount of the regolith in the shovel, less than approximately 80 g—this weight range is marked using red color. Because of the few-millimeter-wide gap between the end of the RF sensor and the bottom of the shovel, a small quantity of regolith inside the shovel does not cause a significant phase change, resulting in a sensor dead zone.
- Slight phase changes for large amount of the regolith inside the shovel, exceeding approximately 170 g. This weight range is marked in brown. In this case, the regolith accumulates in the part of the shovel that was immersed during acquisition, and the RF sensor is not adequately immersed in the regolith. This causes the measured phase change to deviate from the actual amount of regolith inside the shovel.
4. Discussion
5. Conclusions
- It is possible to measure the acquired mass of regolith using RF signals with reasonable accuracy of around 15%, which is good enough for rough regolith mass estimation in sampling devices or excavators.
- The regression model underwent statistical testing, and the obtained p-values for the regression coefficients confirmed their statistical significance.
- The RF sensor can be installed on a thin cross-section shovel without impacting the digging operation and performance.
- The vibration system improves the sensor accuracy; however, its mounting on the shovel implies a need for additional cable routing and an increase in the shovel thickness.
- The use of vibrations extends the measurable mass range, enabling the estimation of both smaller and larger sample masses beyond the limits of static measurement methods.
- The proposed system is lightweight and scalable, making it suitable for integration into various planetary excavation systems where the mass constraints are critical.
- The real lunar regolith might behave differently than the lunar analogue since analogues are developed to replicate only some features of real regolith; however, as lunar regolith is a dielectric material, the key principles of the RF sensor operation, like the signal phase shift, should be applicable to real cases.
- Tests in reduced gravity conditions to validate both the RCE performance as well as the RF sensor accuracy with respect to this important difference between terrestrial and lunar environments.
- Development of dedicated RF sensor front-end electronics suitable for the RCE electronics box compartment shown on Figure 1.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- As Artemis Moves Forward, NASA Picks SpaceX to Land Next Americans on Moon, NASA. Available online: https://www.nasa.gov/news-release/as-artemis-moves-forward-nasa-picks-spacex-to-land-next-americans-on-moon/ (accessed on 6 December 2024).
- Terrae Novae 2030+ Strategy Roadmap., ESA. Available online: https://esamultimedia.esa.int/docs/HRE/Terrae_Novae_2030+strategy_roadmap.pdf (accessed on 6 December 2024).
- Zheng, Y.; Ouyang, Z.; Li, C.; Liu, J.; Zou, Y. China’s Lunar Exploration Program: Present and future. Planet. Space Sci. 2008, 56, 881–886. [Google Scholar] [CrossRef]
- Benaroya, H.; Bernold, L. Engineering of lunar bases. Acta Astronaut. 2008, 62, 277–299. [Google Scholar] [CrossRef]
- Cesaretti, G.; Dini, E.; De Kestelier, X.; Colla, V.; Pambaguian, L. Building components for an outpost on the Lunar soil by means of a novel 3D printing technology. Acta Astronaut. 2014, 93, 430–450. [Google Scholar] [CrossRef]
- International Space Exploration Coordination Group. The Global Exploration Roadmap–Supplement August 2020: Lunar Surface Exploration Scenario Update; ISECG: 2020. Available online: https://www.globalspaceexploration.org/wp-content/uploads/2020/08/GER_2020_supplement.pdf (accessed on 6 December 2024).
- Alexiadis, A.; Alberini, F.; Meyer, M.E. Geopolymers from lunar and Martian soil simulants. Adv. Space Res. 2017, 59, 490–495. [Google Scholar] [CrossRef]
- Cadogan, D.; Stein, J.; Grahne, M. Inflatable composite habitat structures for lunar and mars exploration. Acta Astronaut. 1999, 44, 399–406. [Google Scholar] [CrossRef]
- Horvath, T.; Hayne, P.O.; Paige, D.A. Thermal and Illumination Environments of Lunar Pits and Caves: Models and Observations From the Diviner Lunar Radiometer Experiment. Geophys. Res. Lett. 2022, 49, e2022GL099710. [Google Scholar] [CrossRef]
- Badescu, V. Moon: Prospective Energy and Material Resources; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar] [CrossRef]
- Crawford, I.A. Lunar resources. Prog. Phys. Geogr. Earth Environ. 2015, 39, 137–167. [Google Scholar] [CrossRef]
- Mitchell, J.K.; Houston, W.N.; Scott, R.F.; Costes, N.C.; Carrier, W.D., III; Bromwell, L.G. Mechanical Properties of Lunar Soil: Density, Porosity, Cohesion, and Angle of Internal Friction. Proc. Lunar Sci. Conf. 1972, 3, 3235–3253. [Google Scholar]
- Carrier, W.D.; Olhoeft, G.R.; Mendell, W. Physical properties of the lunar surface. In Lunar Sourcebook: A User’s Guide to the Moon; Heiken, G., Vaniman, D., French, B.M., Eds.; Cambridge University Press: Cambridge, UK, 1991; pp. 475–594. [Google Scholar]
- Cohen, B.; Zacny, K. Drilling in Extreme Environments: Penetration and Sampling on Earth and other Planets; Wiley-VCH Verlag GmbH & Co. KgaA: Weinheim, Germany, 2009. [Google Scholar]
- Li, C.; Su, Y.; Pettinelli, E.; Xing, S.; Ding, C.; Liu, J.; Ren, X.; Lauro, S.E.; Soldovieri, F.; Zeng, X.; et al. The Moon’s farside shallow subsurface structure unveiled by Chang’E-4 Lunar Penetrating Radar. Sci. Adv. 2020, 6, eaay6898. [Google Scholar] [CrossRef] [PubMed]
- Qian, Y.; Xiao, L.; Head, J.W.; Van der Bogert, C.H.; Hiesinger, H.; Wilson, L. Young lunar mare basalts in the Chang’e-5 sample return region, northern Oceanus Procellarum. Earth Planet. Sci. Lett. 2021, 555, 11670. [Google Scholar] [CrossRef]
- Taylor, L.A.; Pieters, C.M.; Britt, D. Evaluations of lunar regolith simulants. Planet. Space Sci. 2016, 126, 1–7. [Google Scholar] [CrossRef]
- Planetary Simulants Database Developed by Colorado School of Mines. Available online: https://space.mines.edu/simulants/ (accessed on 6 December 2024).
- Bednarz, S.; Rzyczniak, M.; Gonet, A.; Seweryn, K. Research of Formed Lunar Regholit Analog AGK-2010. Arch. Min. Sci. 2013, 58, 551–556. [Google Scholar] [CrossRef]
- Zhang, W.; Cheng, Q.; Li, J. Technical progress in the utilization and exploitation of small celestial body resources. Acta Astronaut. 2023, 208, 219–255. [Google Scholar] [CrossRef]
- Just, G.; Smith, K.; Joy, K.; Roy, M. Parametric review of existing regolith excavation techniques for lunar In Situ Resource Utilisation (ISRU) and recommendations for future excavation experiments. Planet. Space Sci. 2019, 180, 104746. [Google Scholar] [CrossRef]
- Skonieczny, K.; Wettergreen, D.; Whittaker, W. Advantages of continuous excavation in lightweight planetary robotic operations. Int. J. Robot. Res. 2016, 35, 1121–1139. [Google Scholar] [CrossRef]
- Rickman, H.; Słaby, E.; Gurgurewicz, J.; Śmigielski, M.; Banaszkiewicz, M.; Grygorczuk, J.; Morawski, M.; Seweryn, K.; Wawrzaszek, R. CHOMIK: A Multi-Method Approach for Studying Phobos. Sol. Syst. Res. 2014, 48, 279–286. [Google Scholar] [CrossRef]
- Seweryn, K. The new concept of a sampling device driven by rotary hammering actions. IEEE/ASME Trans. Mechatronics 2016, 21, 2477–2489. [Google Scholar] [CrossRef]
- Seweryn, K.; Bozynski, M.; Chmaj, G.; Gasowski, C.; Grassmann, K.; Osica, P.; Pietrzak, J.; Rybus, T.; Sulewski, J.; Szewczyk, P.; et al. Performance of lunar regolith sampling device RCE subject to key lunar environmental factors. In Proceedings of the International Symposium on Artificial Intelligence, Robotics and Automation in Space (i-SAIRAS) 2024, Brisbane, Australia, 19–21 November 2024. [Google Scholar]
- Bierhaus, E.B.; Clark, B.C.; Harris, J.W.; Payne, K.S.; Dubisher, R.D.; Wurts, D.W.; Hund, R.A.; Kuhns, R.M.; Linn, T.M.; Wood, J.L.; et al. The OSIRIS-REx Spacecraft and the Touch-and-Go Sample Acquisition Mechanism (TAGSAM). Space Sci. Rev. 2018, 214, 107. [Google Scholar] [CrossRef]
- Zacny, K.; Thomas, L.; Paulsen, G.; Van Dyne, D.; Lam, S.; Williams, H.; Sabahi, D.; Ng, P.; Satou, Y.; Kato, H.; et al. Pneumatic Sampler (P-Sampler) for the Martian Moons eXploration (MMX) Mission. In Proceedings of the 2020 IEEE Aerospace Conference, Big Sky, MT, USA, 7–14 March 2020; pp. 1–11. [Google Scholar] [CrossRef]
- Kawakatsu, Y.; Kuramoto, K.; Usui, T.; Ikeda, H.; Ozaki, N.; Baresi, N.; Ono, G.; Imada, T.; Shimada, T.; Kusano, H.; et al. Mission Design of Martian Moons eXploration (MMX). In Proceedings of the 70th International Astronautical Congress (IAC), Washington, DC, USA, 21–25 October 2019. [Google Scholar]
- Templeman, J.O.; Sheil, B.B.; Sun, T. Multi-axis force sensors: A state-of-the-art review. Sens. Actuators A Phys. 2020, 304, 111772. [Google Scholar] [CrossRef]
- Mukhopadhyay, S.C.; George, B.; Roy, J.K.; Islam, T. Interdigital Sensors; Springer: Cham, Switzerland, 2021. [Google Scholar] [CrossRef]
- Abbasi, Q.H.; Abbas, H.T.; Alomainy, A.; Imran, M.A. Backscattering and RF Sensing for Future Wireless Communication; Wiley: Hoboken, NJ, USA, 2021. [Google Scholar]
- Khunteta, S.; Saikrishna, P.; Agrawal, A.; Kumar, A.; Chavva, A.K.R. RF-Sensing: A New Way to Observe Surroundings. IEEE Access 2022, 10, 129653–129665. [Google Scholar] [CrossRef]
- Lubna, L.; Hameed, H.; Ansari, S.; Zahid, A.; Sharif, A.; Abbas, H.T.; Alqahtani, F.; Mufti, N.; Ullah, S.; Imran, M.A.; et al. Radio frequency sensing and its innovative applications in diverse sectors: A comprehensive study. Front. Commun. Netw. 2022, 3, 1010228. [Google Scholar] [CrossRef]
- Cui, L.; Zhang, Z.; Gao, N.; Meng, Z.; Li, Z. Radio Frequency Identification and Sensing Techniques and Their Applications—A Review of the State-of-the-Art. Sensors 2019, 19, 4012. [Google Scholar] [CrossRef] [PubMed]







| Regolith Mass [g] | Mean Value (Without Smoothing) | Standard Deviation (Without Smoothing) | Mean Value (With Smoothing) | Standard Deviation (With Smoothing) |
|---|---|---|---|---|
| 0 | −46.4 | 3.8 | −54.6 | 2.1 |
| 30 | −55.3 | 4.2 | −68.1 | 2.3 |
| 60 | −62.8 | 6.3 | −76.2 | 1.8 |
| 90 | −66.2 | 6.0 | −76.9 | 4.3 |
| 120 | −69.7 | 7.6 | −81.0 | 4.6 |
| 150 | −74.0 | 8.2 | −83.2 | 3.0 |
| 180 | −75.9 | 8.6 | −87.8 | 4.0 |
| 210 | −81.2 | 11.1 | −90.1 | 4.4 |
| 240 | −88.6 | 11.3 | −93.2 | 3.2 |
| Regolith Mass [g] | Mean Value (Without Smoothing) | Standard Deviation (Without Smoothing) | Mean Value (With Smoothing) | Standard Deviation (With Smoothing) |
|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 |
| 30 | −8.8 | 2.5 | 13.6 | 2.3 |
| 60 | −16.4 | 3.8 | 21.7 | 1.4 |
| 90 | −19.8 | 3.5 | 22.4 | 3.7 |
| 120 | −23.2 | 5.3 | 26.5 | 4.3 |
| 150 | −27.6 | 6.2 | 28.7 | 2.0 |
| 180 | −29.5 | 6.6 | 33.3 | 3.5 |
| 210 | −34.7 | 9.0 | 35.5 | 4.0 |
| 240 | −42.0 | 9.5 | 38.9 | 3.2 |
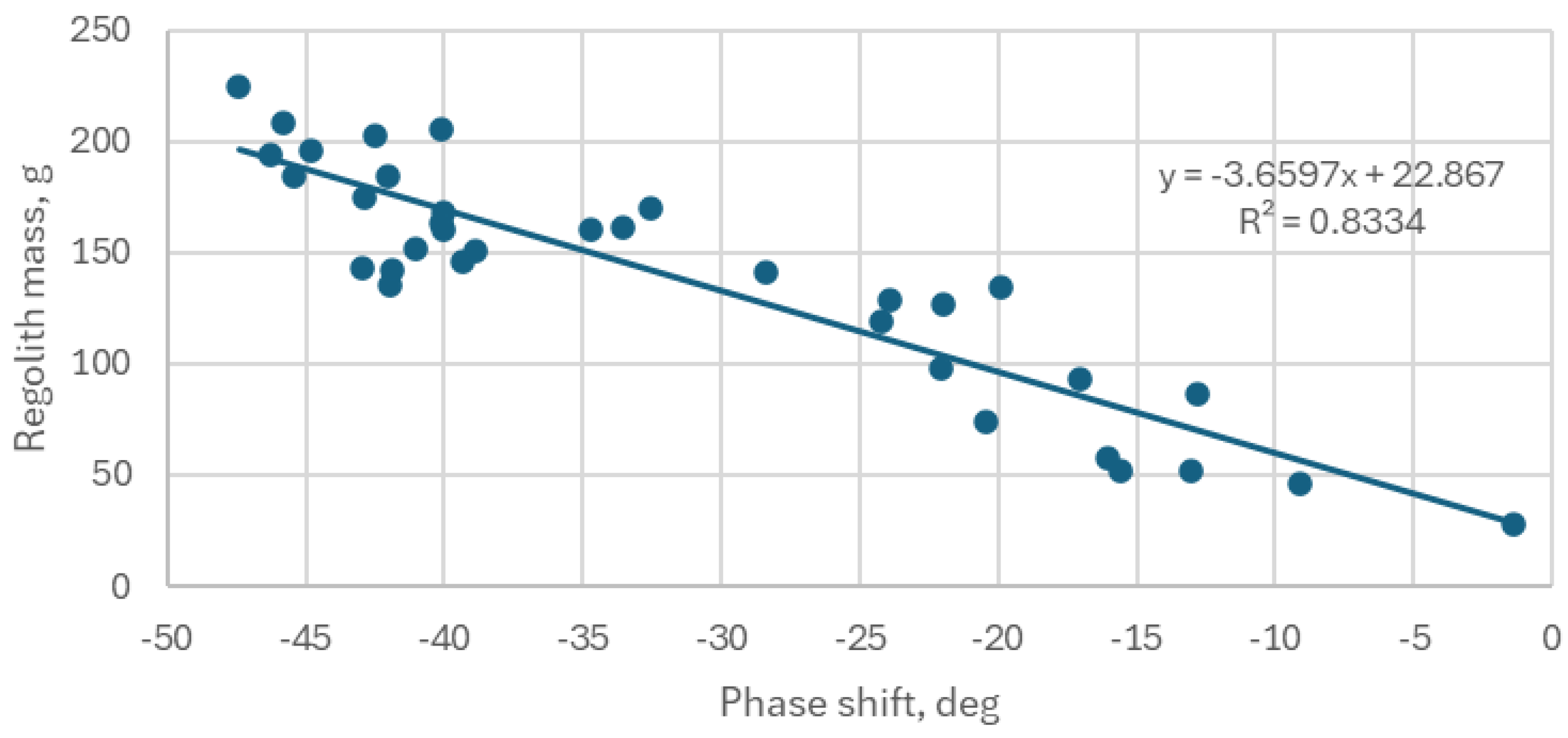
| Parameter | Value |
|---|---|
| Multiple R | 0.913 |
| R2 | 0.833 |
| Standard error, g | 21.375 |
| Observations | 35 |
| b | a | |
|---|---|---|
| Coefficient value | 22.867 | −3.660 |
| Standard error | 9.731 | 0.285 |
| T statistic | 2.350 | −12.849 |
| p-value | 0.025 | 2·10−14 |
| Lower 95% | 3.070 | −4.239 |
| Upper 95% | 42.664 | −3.080 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kurek, K.; Seweryn, K.; Tkacz, A.; Just, G. Detection of Lunar Regolith Acquired by Excavator Using Radiofrequency (RF) Sensors. Sensors 2025, 25, 751. https://doi.org/10.3390/s25030751
Kurek K, Seweryn K, Tkacz A, Just G. Detection of Lunar Regolith Acquired by Excavator Using Radiofrequency (RF) Sensors. Sensors. 2025; 25(3):751. https://doi.org/10.3390/s25030751
Chicago/Turabian StyleKurek, Krzysztof, Karol Seweryn, Arkadiusz Tkacz, and Gunter Just. 2025. "Detection of Lunar Regolith Acquired by Excavator Using Radiofrequency (RF) Sensors" Sensors 25, no. 3: 751. https://doi.org/10.3390/s25030751
APA StyleKurek, K., Seweryn, K., Tkacz, A., & Just, G. (2025). Detection of Lunar Regolith Acquired by Excavator Using Radiofrequency (RF) Sensors. Sensors, 25(3), 751. https://doi.org/10.3390/s25030751





