Design and Simulation Test of Non-Contact Voltage Sensor
Abstract
:1. Introduction
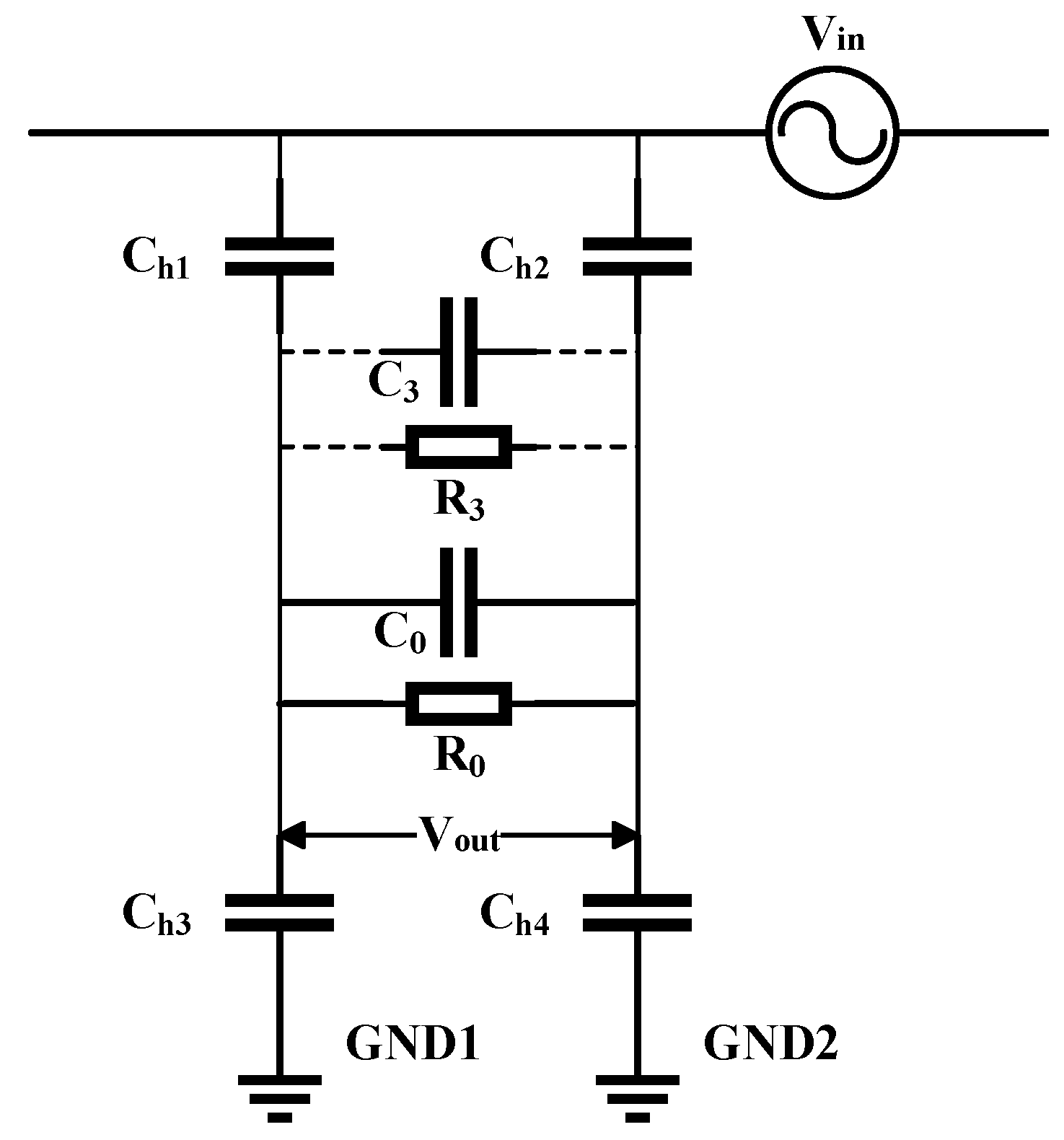
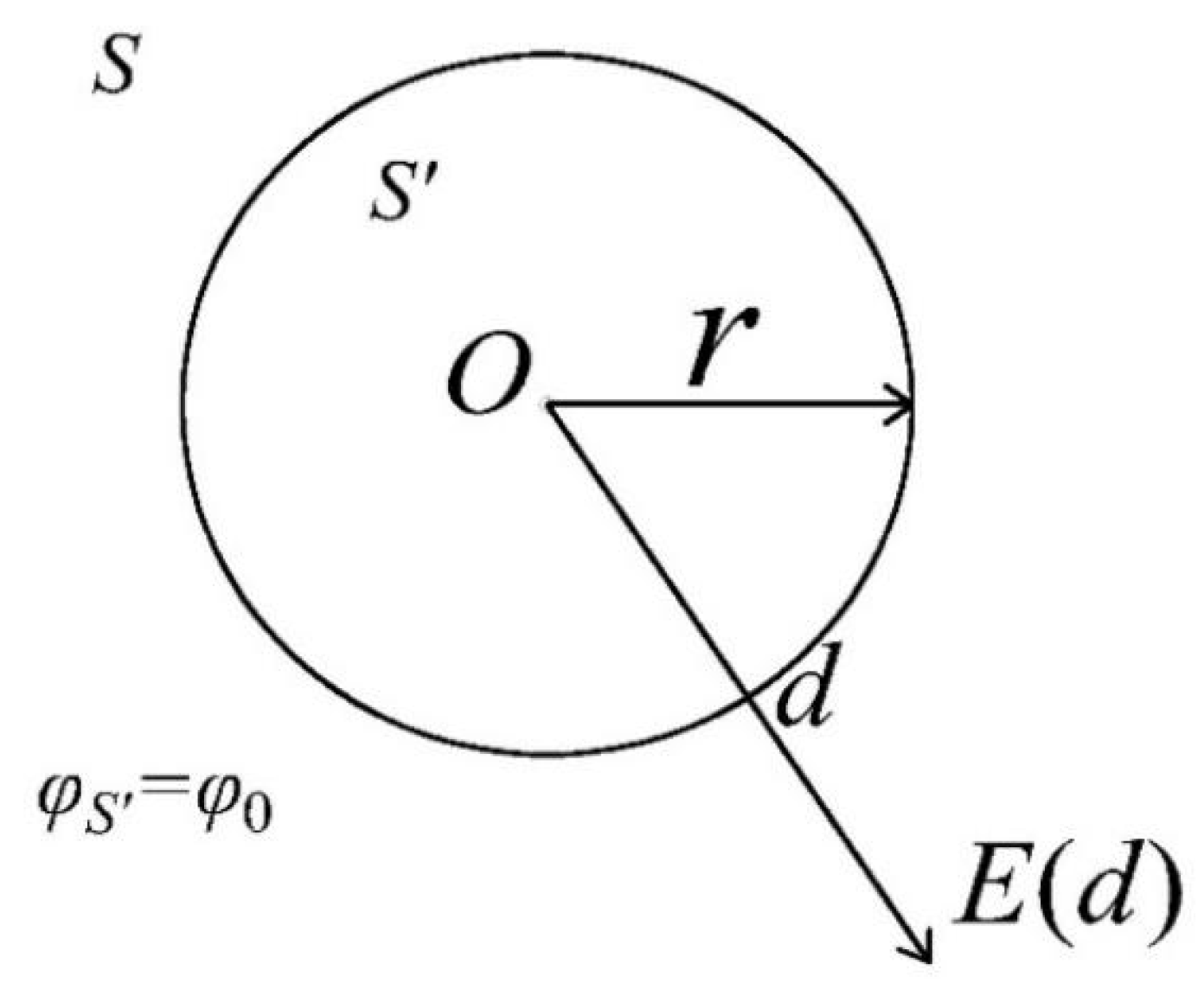
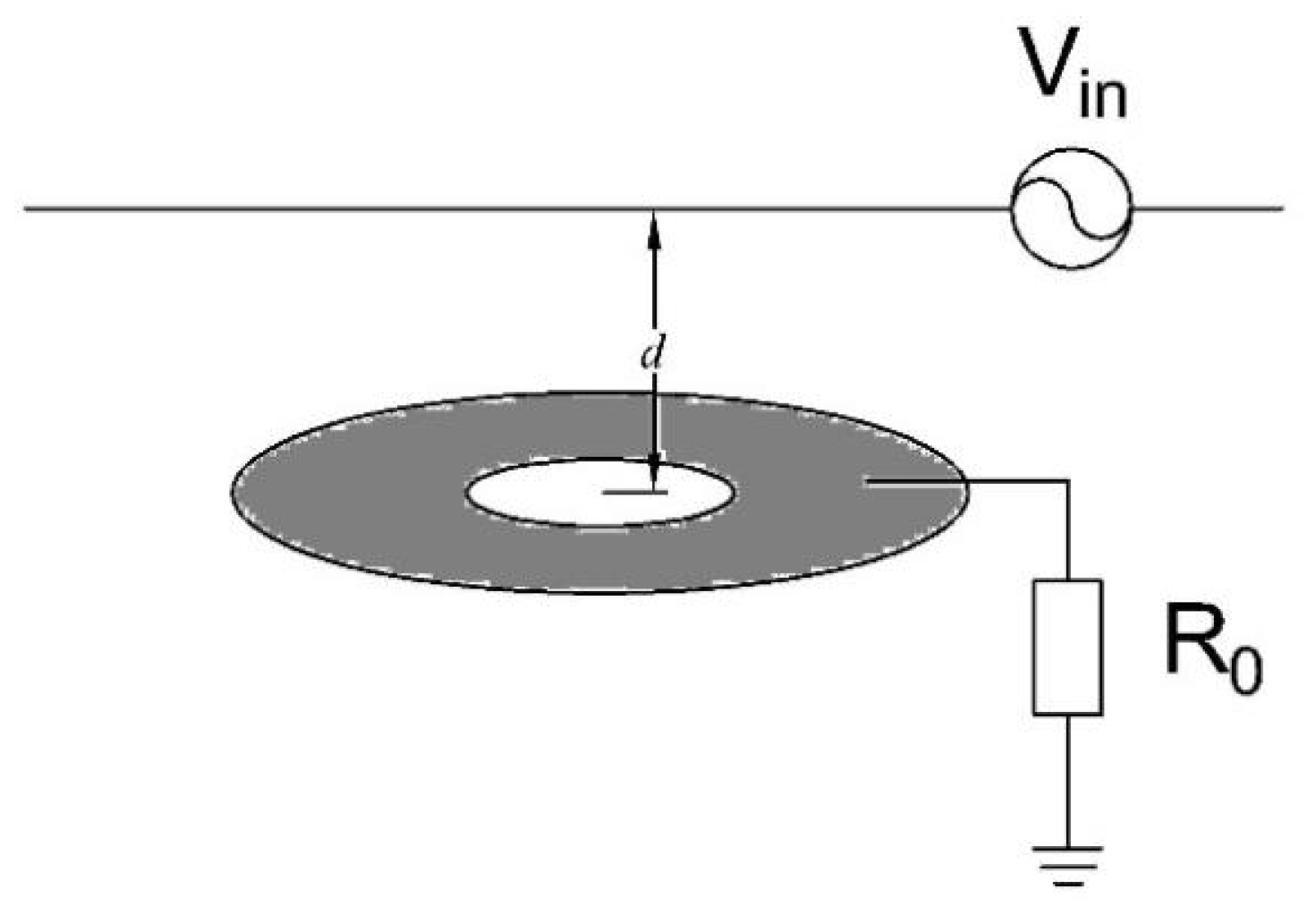
2. Principles of the Sensor
3. Sensor Design
3.1. Factors Affecting the Transformation Ratio
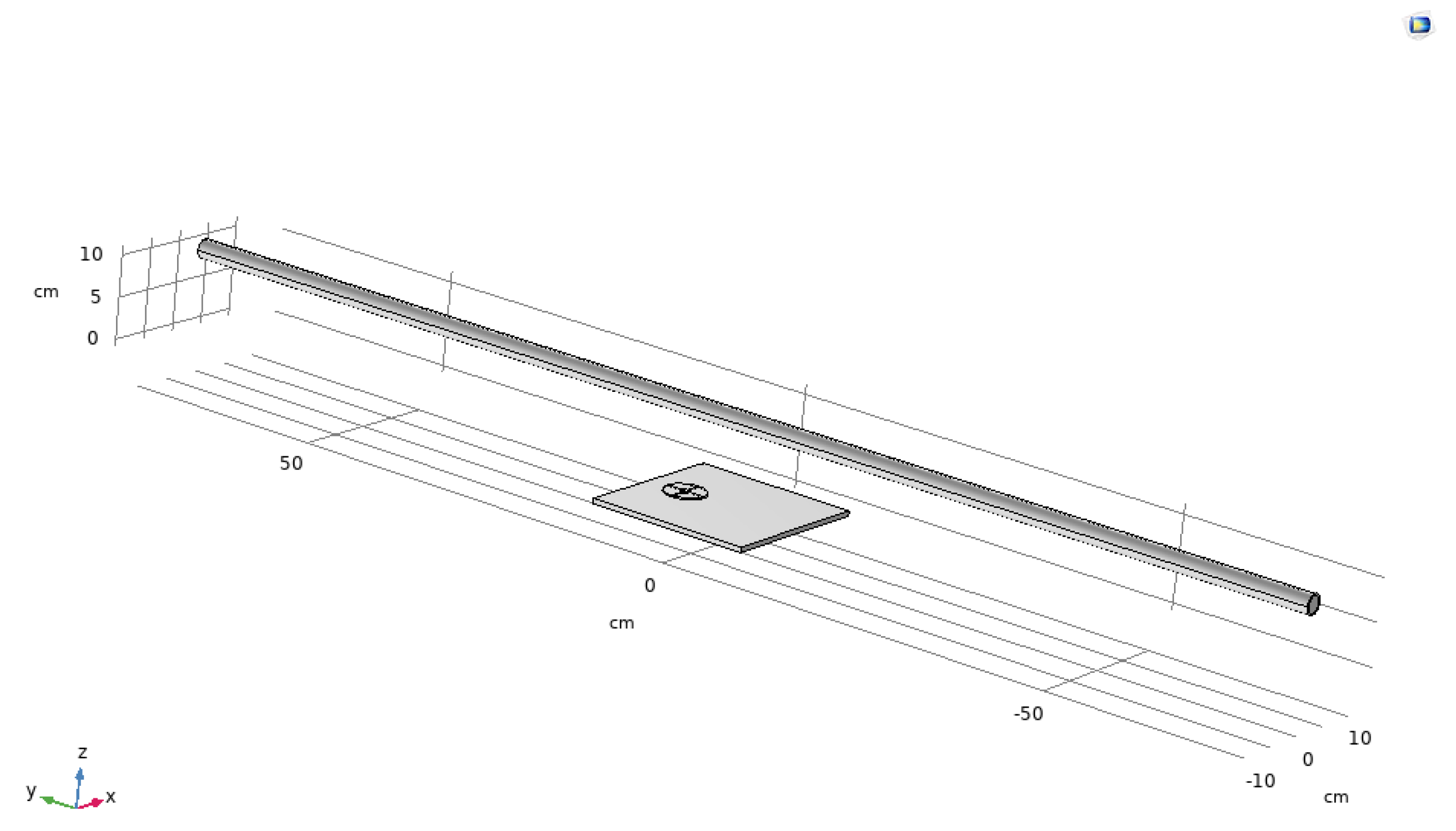
3.2. Sensor Placement
3.3. Selection of Operational Amplifier
4. Experimental Analysis
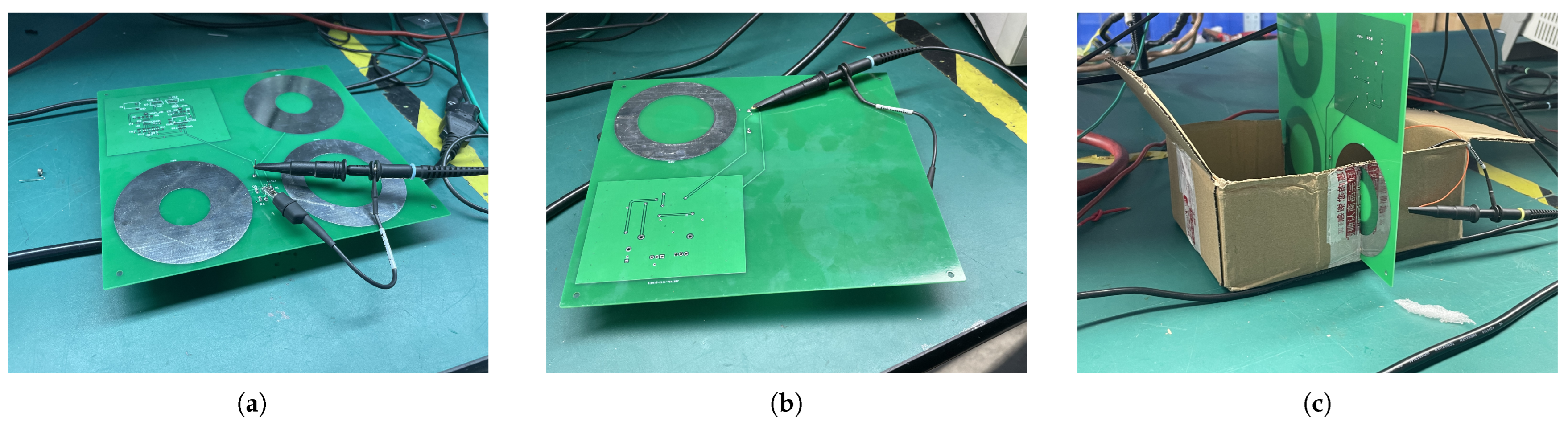
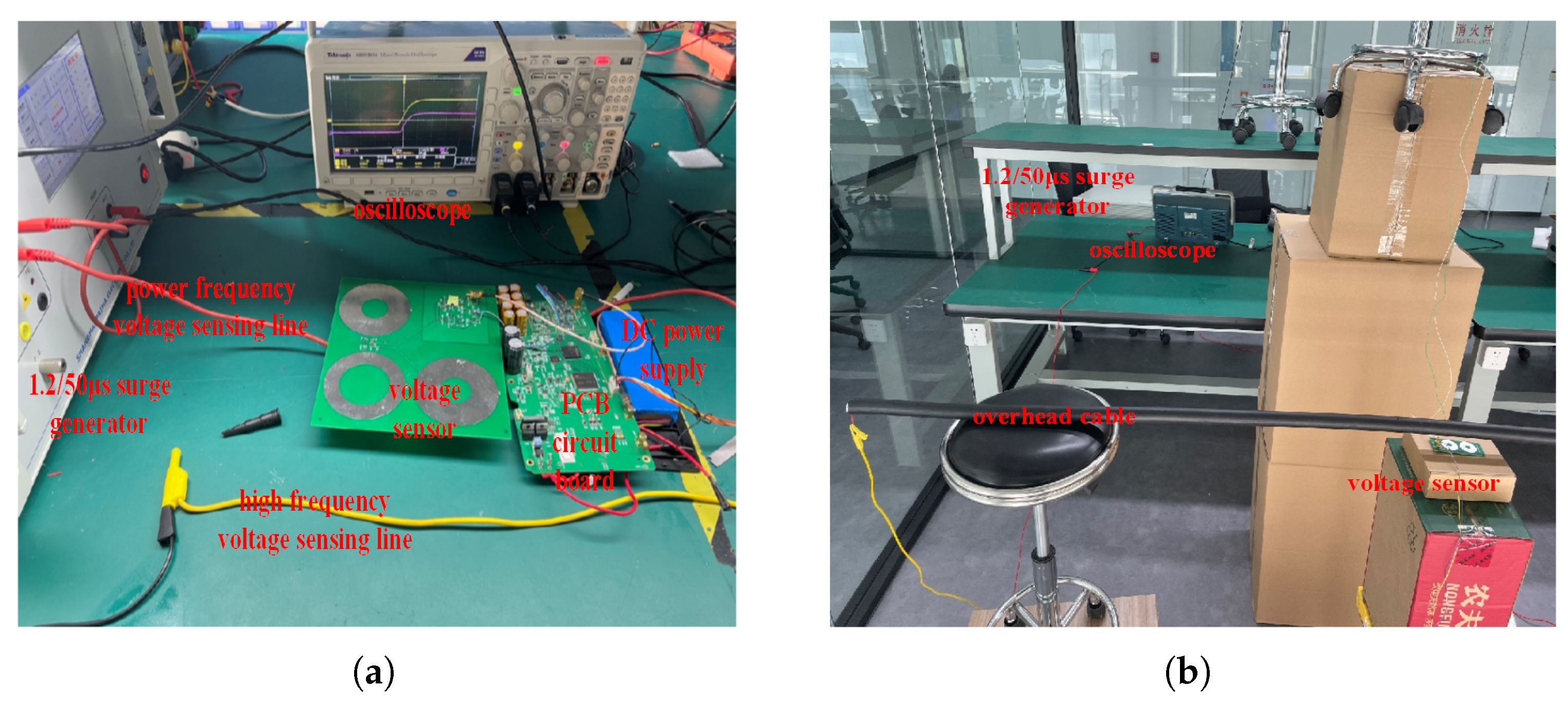
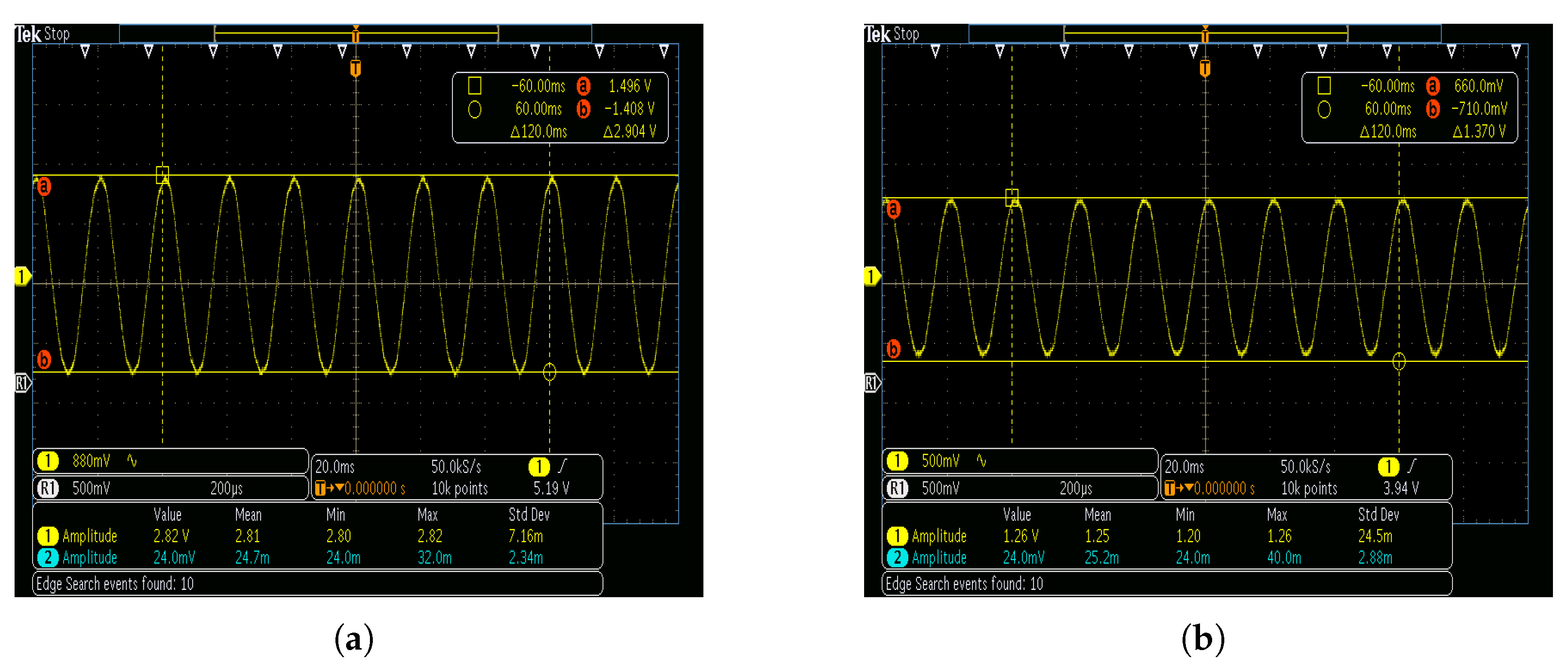
4.1. Construction of Experimental Platform
- Environment 1: a laboratory low-voltage insulated silicone wire experimental environment;
- Environment 2: a high-voltage overhead line experimental environment;
- Environment 3: a high-voltage cable experimental environment;
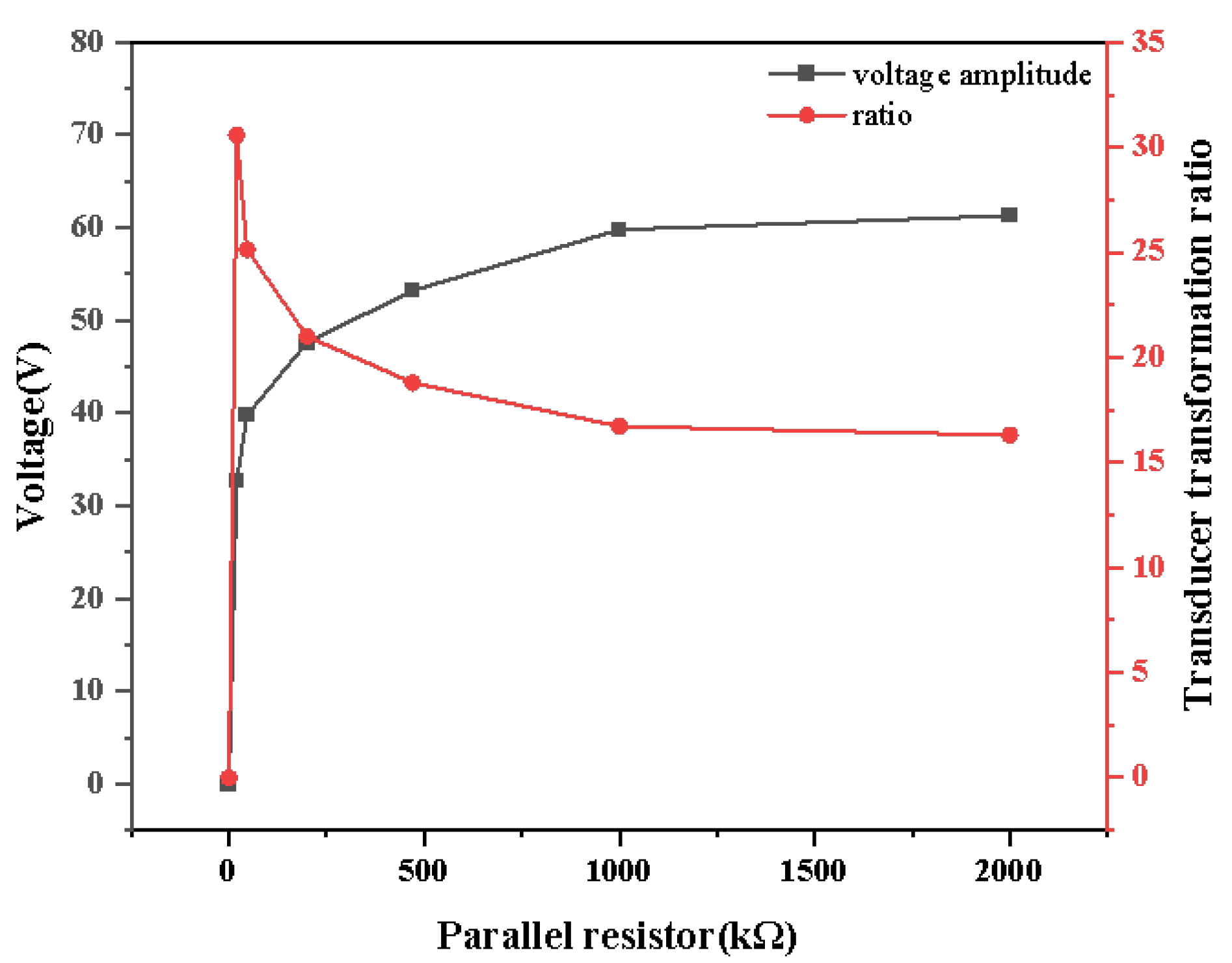
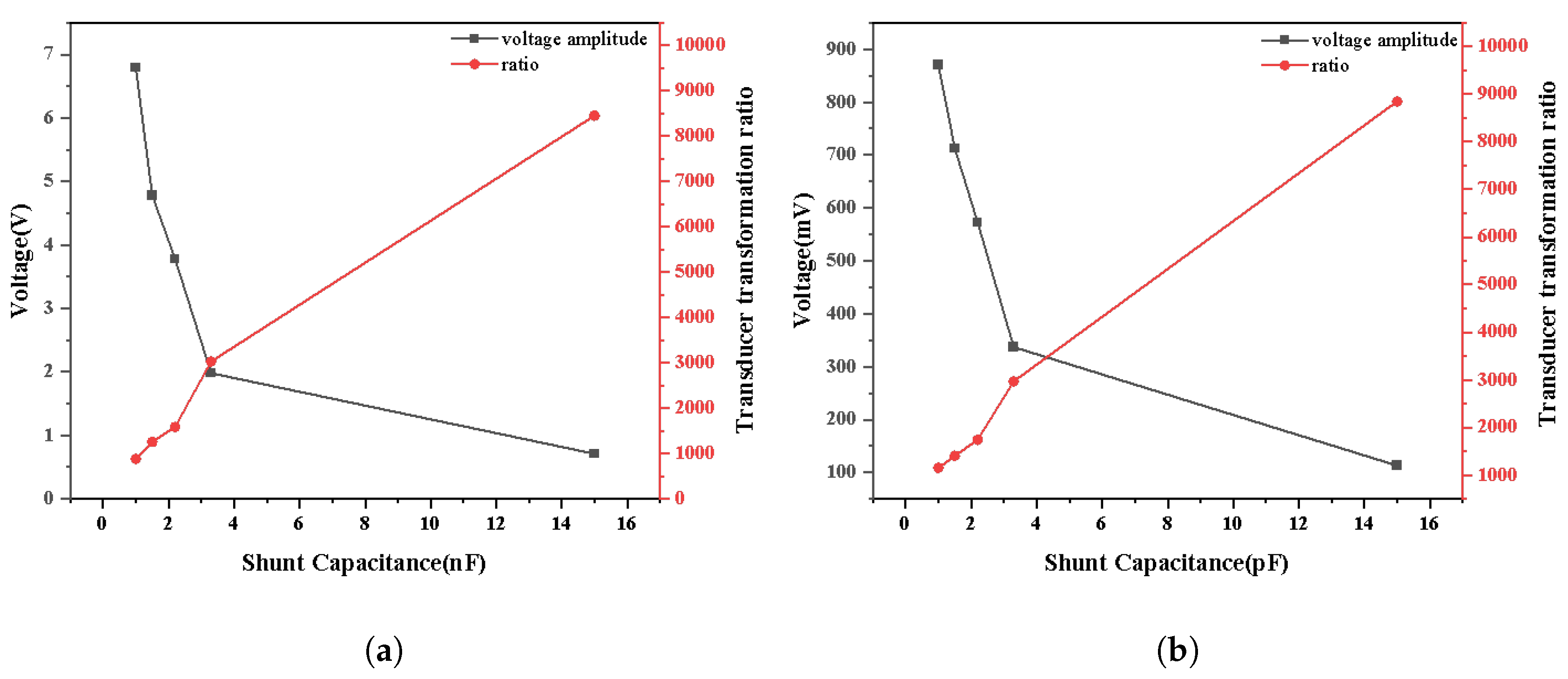
4.2. Influence of Parallel Resistance on Measurement
4.3. Influence of Parallel Capacitance on Measurement
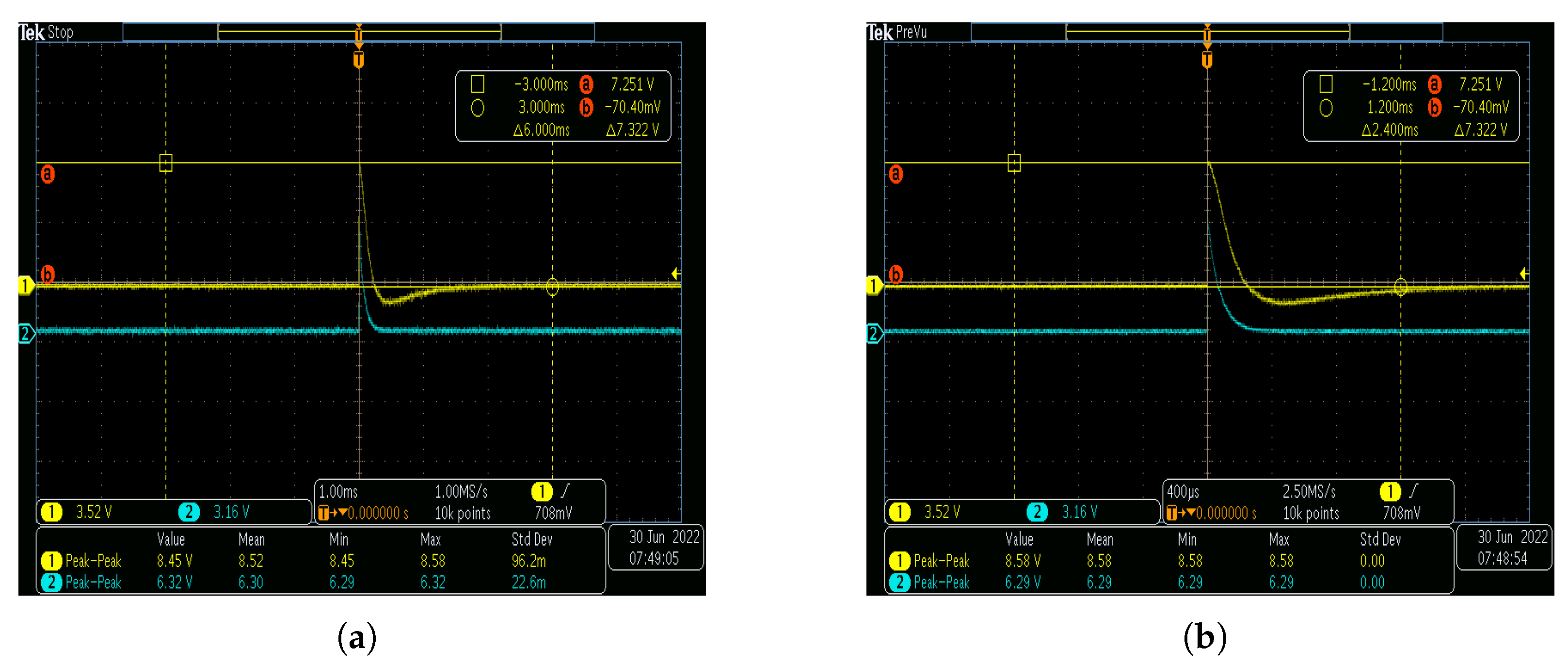
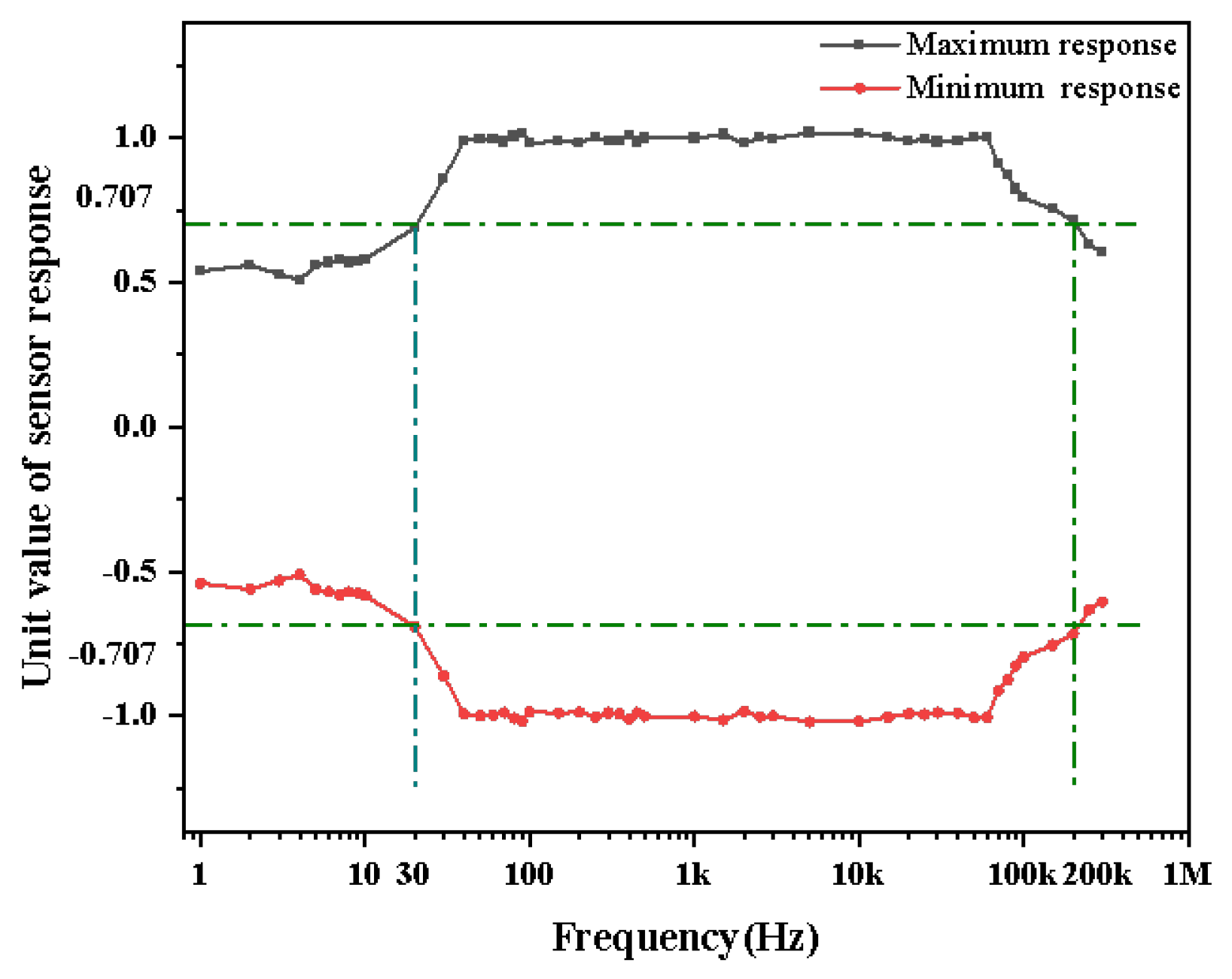
4.4. Sensor Bandwidth Test
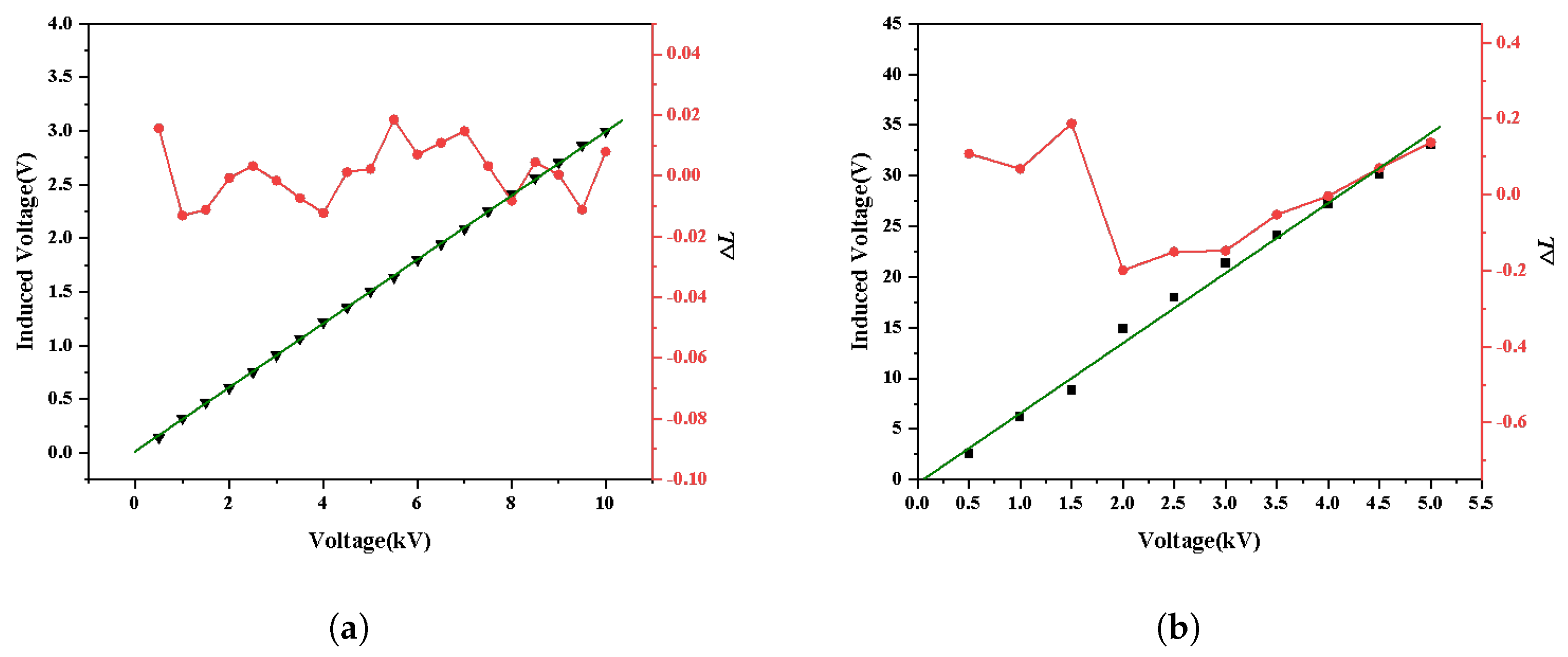
4.5. Sensor Linearity Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Alsuwian, T.; Shahid Butt, A.; Amin, A.A. Smart Grid Cyber Security Enhancement: Challenges and Solutions—A Review. Sustainability 2022, 14, 14226. [Google Scholar] [CrossRef]
- Huang, A.Q.; Crow, M.L.; Heydt, G.T.; Zheng, J.P.; Dale, S.J. The Future Renewable Electric Energy Delivery and Management (FREEDM) System: The Energy Internet. Proc. IEEE 2011, 99, 133–148. [Google Scholar] [CrossRef]
- Vashi, S.; Ram, J.; Modi, J.; Verma, S.; Prakash, C. Internet of Things (IoT): A vision, architectural elements, and security issues. In Proceedings of the 2017 International Conference on I-SMAC (IoT in Social, Mobile, Analytics and Cloud) (I-SMAC), Palladam, India, 10–11 February 2017; pp. 492–496. [Google Scholar]
- Yang, L.; Long, W.; Zhang, W.; Yan, P.; Zhou, Y.; Li, J. Transmission Line Voltage Calibration-Free Measurement Method. Electronics 2023, 12, 814. [Google Scholar] [CrossRef]
- Khazaee, M.; Rosendahl, L.A.; Rezania, A. Online Condition Monitoring of Rotating Machines by Self-Powered Piezoelectric Transducer from Real-Time Experimental Investigations. Sensors 2022, 22, 3395. [Google Scholar] [CrossRef] [PubMed]
- Deng, F.; Yan, Y.; Zhang, Y. Design of wireless strain measurement system based on vibrating wire sensor. Sens. Microsyst. 2015, 34, 103–105. [Google Scholar]
- Gao, S. Design of D-Dot Voltage Sensor and Research of Three Phase Voltage Measurement System. Master’s Thesis, Chongqing University, Chongqing, China, 2015. [Google Scholar]
- Gao, S.; Wang, J.; Yang, J.; Peng, H.; Ma, J. Design and Simulation of D-dot voltage sensor based on inverse problem of electric field. J. Electrotech. 2016, 31, 36–42. [Google Scholar]
- Haberman, M.A.; Spinelli, E.M. Noncontact AC Voltage Measurements: Error and Noise Analysis. IEEE Trans. Instrum. Meas. 2018, 67, 1946–1953. [Google Scholar] [CrossRef]
- Lawrence, D.; Donnal, J.S.; Leeb, S.; He, Y. Non-contact measurement of line voltage. IEEE Sens. J. 2016, 16, 8990–8997. [Google Scholar] [CrossRef]
- Wang, J.; Ban, S.; Yang, Y. A differential self-integration D-dot voltage sensor and experimental research. IEEE Sens. J. 2015, 15, 3846–3852. [Google Scholar] [CrossRef]
- Feng, G.; Yang, X. Study on influence of non-contact measuring circuit on micro EDM using non-contact electric feeding method. In Proceedings of the 8th International Conference Instrumentation & Measurement, Computer, Communication and Control (IMCCC), Harbin, China, 19–21 July 2018; pp. 254–258. [Google Scholar]
- Donnal, J.S.; Lindahl, P.; Lawrence, D.; Zachar, R.; Leeb, S. Untangling non-contact power monitoring puzzles. IEEE Sens. J. 2017, 17, 3542–3550. [Google Scholar] [CrossRef]
- Zhao, P.; Wang, J.; Wang, Q.; Xiao, Q.; Zhang, R.; Ou, S.; Tao, Y. Design, and Test of a Dual-Differential D-Dot Overvoltage Sensor Based on the Field-Circuit Coupling Method. Sensors 2019, 19, 3413. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Zhao, P.; Wang, Q.; Zhou, M.; Huang, H. Research on double differential D-dot overvoltage sensor based on the inverse problem of electric field. Proc. CSEE 2020, 40, 5363–5373. [Google Scholar]
- Krause, T.C.; Camenzind, K.; Green, D.H.; Moeller, A.; Huchel, L.; Leeb, S.B. A Sensor Topology for Noncontact AC Voltage Measurement of Polyphase Cables. IEEE Trans. Instrum. Meas. 2022, 71, 1–10. [Google Scholar] [CrossRef]
- Cui, Y.; Yuan, H.; Song, X.; Zhao, L.; Liu, Y.; Lin, L. Model, Design, and Testing of Field Mill Sensors for Measuring Electric Fields Under High-Voltage Direct-Current Power Lines. IEEE Trans. Ind. Electron. 2018, 65, 608–615. [Google Scholar] [CrossRef]
- Si, D.; Wang, J.; Wei, G.; Yan, X. Method and Experimental Study of Voltage Measurement Based on Electric Field Integral With Gauss–Legendre Algorithm. IEEE Trans. Instrum. Meas. 2020, 69, 2771–2778. [Google Scholar] [CrossRef]
- Wang, J.; Li, X.; Ou, S.; Zhang, R. Research on Transmission Line Voltage Measurement Method Based on Improved Gaussian Integral. IEEE Access 2019, 7, 73711–73718. [Google Scholar] [CrossRef]
- He, W.; Luo, R.; Wang, J. Principle and experimental research of self integrating D-dot voltage transformer. Proc. CSEE 2014, 34, 2445. [Google Scholar]
- Wang, J.; Bao, S.; Wei, G.; Deng, X. Research on a new type of open close D-dot voltage sensor. J. Electr. Mach. Control 2018, 22, 1–7. [Google Scholar]
- Wu, X.; Zhou, X.; Zhou, T.; Ji, S.; Tian, T.; Luo, Y.; He, N.; Liu, W. Design and experiment of voltage sensor based on electric field coupling principle and differential input structure. High Volt. Appar. 2022, 58, 172–178. [Google Scholar]
- Wang, L.; Li, Z.; Meng, X. Power supply technology of low power online monitoring sensor based on electric field induction. High Volt. 2020, 46, 538–545. [Google Scholar]




| Potential of the Tested Conductor/kV | d/cm | Output Voltage of the Sensor/V | The Transformation Ratio of the Sensor |
|---|---|---|---|
| 1 | 2 | 45.70 | 21.88:1 |
| 1 | 4 | 33.73 | 27.99:1 |
| 1 | 6 | 28.77 | 34.76:1 |
| 1 | 8 | 25.06 | 39.90:1 |
| 1 | 10 | 18.76 | 53.30:1 |
| Potential of the Tested Conductor/kV | w/cm | Output Voltage of the Sensor/V | The Transformation Ratio of the Sensor |
|---|---|---|---|
| 1 | 2 | 45.70 | 21.88:1 |
| 1 | 4 | 46.17 | 21.66:1 |
| 1 | 6 | 50.92 | 19.64:1 |
| 1 | 8 | 60.32 | 16.58:1 |
| 1 | 10 | 85.26 | 11.73:1 |
| Placement | Parallel Cap- Acitance/nF | Power-Frequency Voltage Ratio | High-Frequency Voltage Ratio | Gain Ratio of High-Frequency Voltage to Power-Frequency Voltage |
|---|---|---|---|---|
| Positive | 0 | 353:1 | 199:1 | 0.56:1 |
| Positive | 0.1 | 393:1 | 350:1 | 0.89:1 |
| Positive | 1 | 1234:1 | 1276:1 | 1.034:1 |
| Positive | 2.2 | 2287:1 | 2668:1 | 1.175:1 |
| Negative | 2.2 | 2990:1 | 4419:1 | 1.48:1 |
| Vertical | 4.7 | 33,085:1 | 22,727:1 | 0.69:1 |
| Channel | Parallel Capacitance/nF | Parallel Resistor/k | Ratio |
|---|---|---|---|
| power-frequency measurement | 3.3 | 1000 | 300:1 |
| High-frequency measurement | 1.0 | 36 | 1200:1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, H.; Liu, H.; Shang, K.; Li, G.; Zhao, L. Design and Simulation Test of Non-Contact Voltage Sensor. Sensors 2025, 25, 3118. https://doi.org/10.3390/s25103118
Peng H, Liu H, Shang K, Li G, Zhao L. Design and Simulation Test of Non-Contact Voltage Sensor. Sensors. 2025; 25(10):3118. https://doi.org/10.3390/s25103118
Chicago/Turabian StylePeng, Haojie, Hongwei Liu, Kuo Shang, Gaoyue Li, and Liping Zhao. 2025. "Design and Simulation Test of Non-Contact Voltage Sensor" Sensors 25, no. 10: 3118. https://doi.org/10.3390/s25103118
APA StylePeng, H., Liu, H., Shang, K., Li, G., & Zhao, L. (2025). Design and Simulation Test of Non-Contact Voltage Sensor. Sensors, 25(10), 3118. https://doi.org/10.3390/s25103118






