Investigating the Mechanical Properties and Temperature Compensation of a Spot-Welded Strain Sensor within an Intelligent Steel Strand Cable
Abstract
1. Introduction
2. Principle and Package of the Spot-Welding Strain Sensor
2.1. Basic Principle of the Spot-Welding Strain Sensor
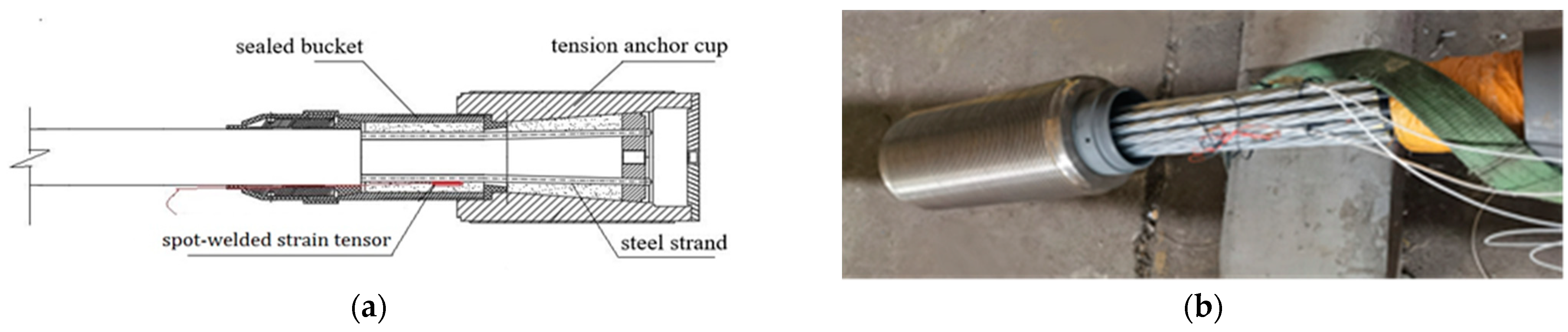
2.2. Spot-Welding Strain Sensor Packaging Solution
3. Welding Strength and Strain Transmission of the Spot-Welded Strain Sensor
3.1. Weld Strength Test of the Metal Substrate
3.2. Theoretical Analysis of Sensor Strain Transfer
3.3. Finite Element Simulation of Sensor Strain Transfer
4. Characterization of a Spot-Welded, Strain Sensor-Equipped Smart Cable
4.1. Performance Testing of Smart Steel Strand Strain Sensing
4.2. Temperature Sensitivity Performance Test and Compensation of Intelligent Steel Strands
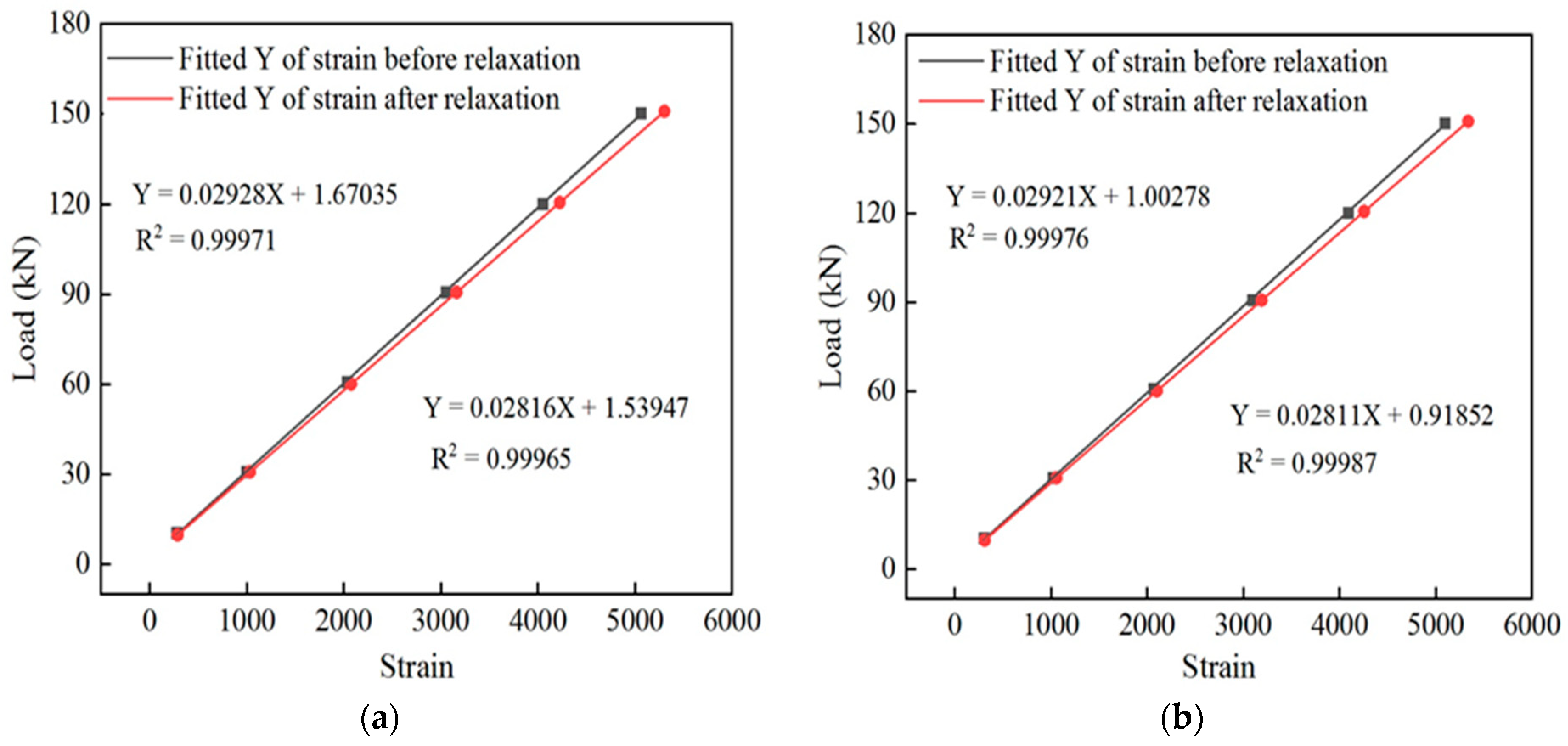
4.3. Stress Relaxation Performance Test of the Intelligent Steel Strands
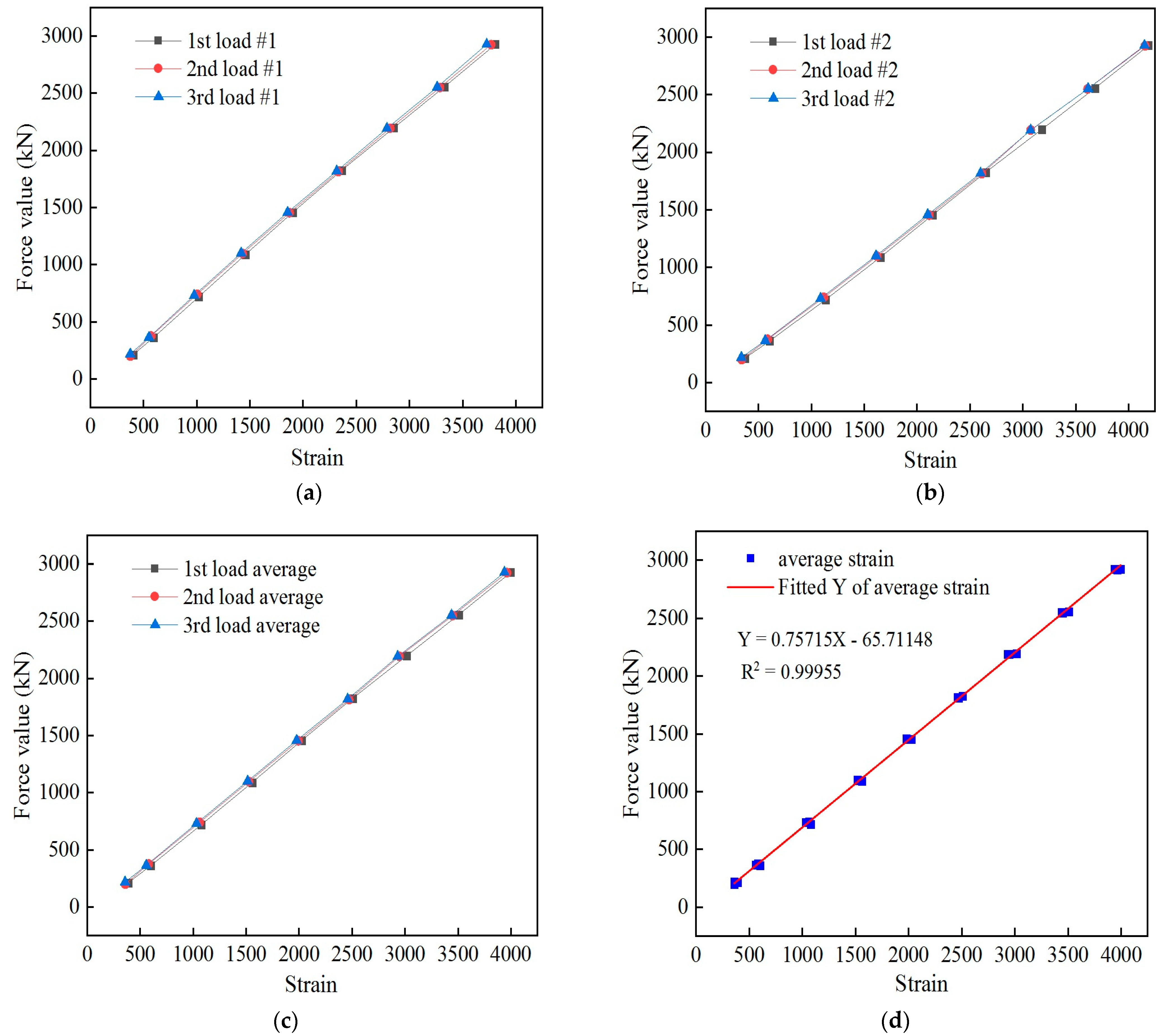
4.4. Performance Test of the Intelligent Steel Strand Cable
5. Conclusions
- (1)
- A thin steel sheet was welded onto a galvanized steel wire with a diameter of 7 mm, and the tensile test results showed that the average shear stress of the thin steel sheet and galvanized steel wire weld was 37.2 MPa.
- (2)
- The strain transfer theory of a spot-welded strain sensor was applied to the component to be measured, and a finite element model of the sensor was established. The analysis showed that the strain transfer efficiency of the spot-welded strain sensor was greater than 96%, and the strain loss was small.
- (3)
- Performance tests of the spot-welded, strain sensor-equipped intelligent steel strand demonstrated that its train-sensing performance was superior, and the combination of the spot-welded strain sensor and steel strand had favorable adaptability and transmissibility. Static load tests of the spot-welded, strain sensor-equipped intelligent steel strand cable demonstrated that the spot-welded strain sensor was functional in the cable. The maximum cable force deviation was only −1.38%, the test accuracy was high, and the sensing linearity and repeatability were favorable.
- (4)
- Under various temperatures and tensile loads, the set force, transverse temperature, and longitudinal strain coordinate methods are proposed, and linear fitting is adopted via the least squares method. The slope of the line is a coefficient, and the calibrated force value formula uses the coefficient for temperature compensation. After considering temperature compensation, the tension test of the spot-welded strain sensor compensates for the full capacity error within 1.0%. The intelligent strand exhibited favorable sensing linearity, and its tension-sensing accuracy was high.
- (5)
- The relaxation rate of the smart steel strand cable after 120 h was 3.78%, with an influence on the sensor accuracy error of 3.97%. The proposed spot-welded, strain-sensor-equipped smart steel strand cable can be applied to the long-term tension monitoring of cable-supported structures in various architectural settings, such as bridges and stadiums, from their construction to operation.
- (6)
- The proposed substrate-type sensor is mounted by spot welding. A detailed study of the stability and reliability of the measurements after mounting has been conducted, but data from long-term monitoring application tests in actual engineering are lacking. Our next step will be to carry out a long-term monitoring experimental study, and the long-term monitoring experimental data will be compared and analyzed.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, J.Q.; Li, C.C.; Zheng, X.H.; Sun, X.H. Bridge Cables And Slings, 1st ed.; People’s Communications Publishing House: Beijing, China, 2013; pp. 137–186. [Google Scholar]
- Lin, S.-W.; Yi, T.-H.; Li, H.-N.; Ren, L. Damage Detection in the Cable Structures of a Bridge Using the Virtual Distortion Method. J. Bridg. Eng. 2017, 22, 04017039. [Google Scholar] [CrossRef]
- Feng, D.; Scarangello, T.; Feng, M.Q.; Ye, Q. Cable tension force estimate using novel noncontact vision-based sensor. Measurement 2017, 99, 44–52. [Google Scholar] [CrossRef]
- Zhao, X.; Han, R.; Yu, Y.; Hu, W.; Jiao, D.; Mao, X.; Li, M.; Ou, J. Smartphone-Based Mobile Testing Technique for Quick Bridge Cable–Force Measurement. J. Bridg. Eng. 2017, 22, 06016012. [Google Scholar] [CrossRef]
- Huynh, T.-C.; Park, J.-H.; Kim, J.-T. Structural identification of cable-stayed bridge under back-to-back typhoons by wireless vibration monitoring. Measurement 2016, 88, 385–401. [Google Scholar] [CrossRef]
- Huang, Y.; Wang, Y.; Fu, J.; Liu, A.; Gao, W. Measurement of the real-time deflection of cable-stayed bridge based on cable tension variations. Measurement 2018, 119, 218–228. [Google Scholar] [CrossRef]
- Yang, S.J.; Deng, N.C.; Wang, X.L.; Dong, J.B.; Jiang, W. Comparative Analysis of Cable Force Test Methods for Steel Rod Short Suspenders. J. Guilin Univ. Technol. 2022, 42, 382–388. [Google Scholar] [CrossRef]
- He, J.; Zhou, Z.; Jinping, O. Optic fiber sensor-based smart bridge cable with functionality of self-sensing. Mech. Syst. Signal Process. 2013, 35, 84–94. [Google Scholar] [CrossRef]
- Qu, C.-X.; Yi, T.-H.; Zhou, Y.-Z.; Li, H.-N.; Zhang, Y.-F. Frequency Identification of Practical Bridges through Higher-Order Spectrum. J. Aerosp. Eng. 2018, 31, 04018018. [Google Scholar] [CrossRef]
- Dan, D.H.; Han, F.; Xu, B. Dynamic Analysis and Intelligent Monitoring of Complex Cable System; Shanghai Science and Technology Press: Shanghai, China, 2023; pp. 186–247. [Google Scholar]
- Fu, Z.; Ji, B.; Wang, Q.; Wang, Y. Cable Force Calculation Using Vibration Frequency Methods Based on Cable Geometric Parameters. J. Perform. Constr. Facil. 2017, 31, 04017021. [Google Scholar] [CrossRef]
- Chen, C.-C.; Wu, W.-H.; Leu, M.-R.; Lai, G. Tension determination of stay cable or external tendon with complicated constraints using multiple vibration measurements. Measurement 2016, 86, 182–195. [Google Scholar] [CrossRef]
- Konovalov, I.K.; Pilyuzin, A.V. Metrological reliability of vibrating-wire transducers. Power Technol. Eng. 2013, 46, 439–441. [Google Scholar] [CrossRef]
- Wang, M.L.; Wang, G. Electromagnetic sensors for assessing and monitoring civil infrastructures. Sens. Technol. Civ. Infrastruct. 2014, 1, 238–264. [Google Scholar] [CrossRef]
- Nazarian, E.; Ansari, F.; Zhang, X.; Taylor, T. Detection of Tension Loss in Cables of Cable-Stayed Bridges by Distributed Monitoring of Bridge Deck Strains. J. Struct. Eng. 2016, 142, 04016018. [Google Scholar] [CrossRef]
- Scarella, A.; Salamone, G.; Babanajad, S.K.; De Stefano, A.; Ansari, F. Dynamic Brillouin Scattering–Based Condition Assessment of Cables in Cable-Stayed Bridges. J. Bridg. Eng. 2017, 22, 04016130. [Google Scholar] [CrossRef]
- Zhu, W.X.; Jia, K.F.; Zheng, D.K.; Sang, R.H.; Deng, L.J. Development of intelligent cable coupled with large-rangefinder grating. In Proceedings of the 17th National Symposium on Modern Structural Engineering, Tianjin, China, 21 July 2017; pp. 70–75. [Google Scholar]
- Pettigrew, M.J. The behavior of weldable strain gauges under nuclear reactor core conditions. Nucl. Eng. Des. 2013, 263, 350–361. [Google Scholar] [CrossRef]
- Weng, F.J.; Zhang, Y.; Ye, W.; Zhou, Y.Y.; Teng, H. Design and application of resistance spot welded strain gaugepressure sensor elastomer. Electr. Weld. Mach. 2017, 47, 1–5. [Google Scholar]
- Zhang, K.Y.; Cui, S.S.; Li, S.; Yang, K. Research on temperature strain compensation in structural strain measurement. J. Wuhan Univ. Technol. 2021, 43, 63–67. [Google Scholar]
- Ding, W.Q.; Wang, Z.C.; Wang, L.M.; Zhang, K. Research on the Algorithm of Converting Three-wire System to Two-wire System in Strain Measurement in Static Test. Struct. Environ. 2013, 40, 57–64. [Google Scholar] [CrossRef]
- Xu, Y.J.; Tang, J.W.; Zhao, Q.Q.; Wang, X.B. Numerical analysis and experimental study of spot welded thin sheet strain sensor. China Test 2009, 35, 90–93. [Google Scholar]
- Xu, Y.J. Experimental study on the performance of TIG spot welded thin sheet strain sensor. Mech. Pract. 2008, 30, 47–49. [Google Scholar]
- Zhu, Y.L.; Kang, G.Z.; Yu, C. A finite cyclic elasto-plastic constitutive model to improve the description of cyclic stress-strain hysteresis loops. Int. J. Plasticity 2017, 95, 191–215. [Google Scholar] [CrossRef]
- Sun, L.; Li, C.; Zhang, C.; Liang, T.; Zhao, Z. The Strain Transfer Mechanism of Fiber Bragg Grating Sensor for Extra-Large Strain Monitoring. Sensors 2019, 19, 1851. [Google Scholar] [CrossRef] [PubMed]
- GB/T 5224-2014; Steel Strand for Prestressed Concrete. National standard of the People’s Republic of China: Beijing, China, 2014.
- GB/T 21839-2019; Test Method for Steel for Prestressed Concrete. National standard of the People’s Republic of China: Beijing, China, 2019.
- GB/T 10120-2013; Test Method for Tensile Stress Relaxation of Metal Materials. National standard of the People’s Republic of China: Beijing, China, 2013.

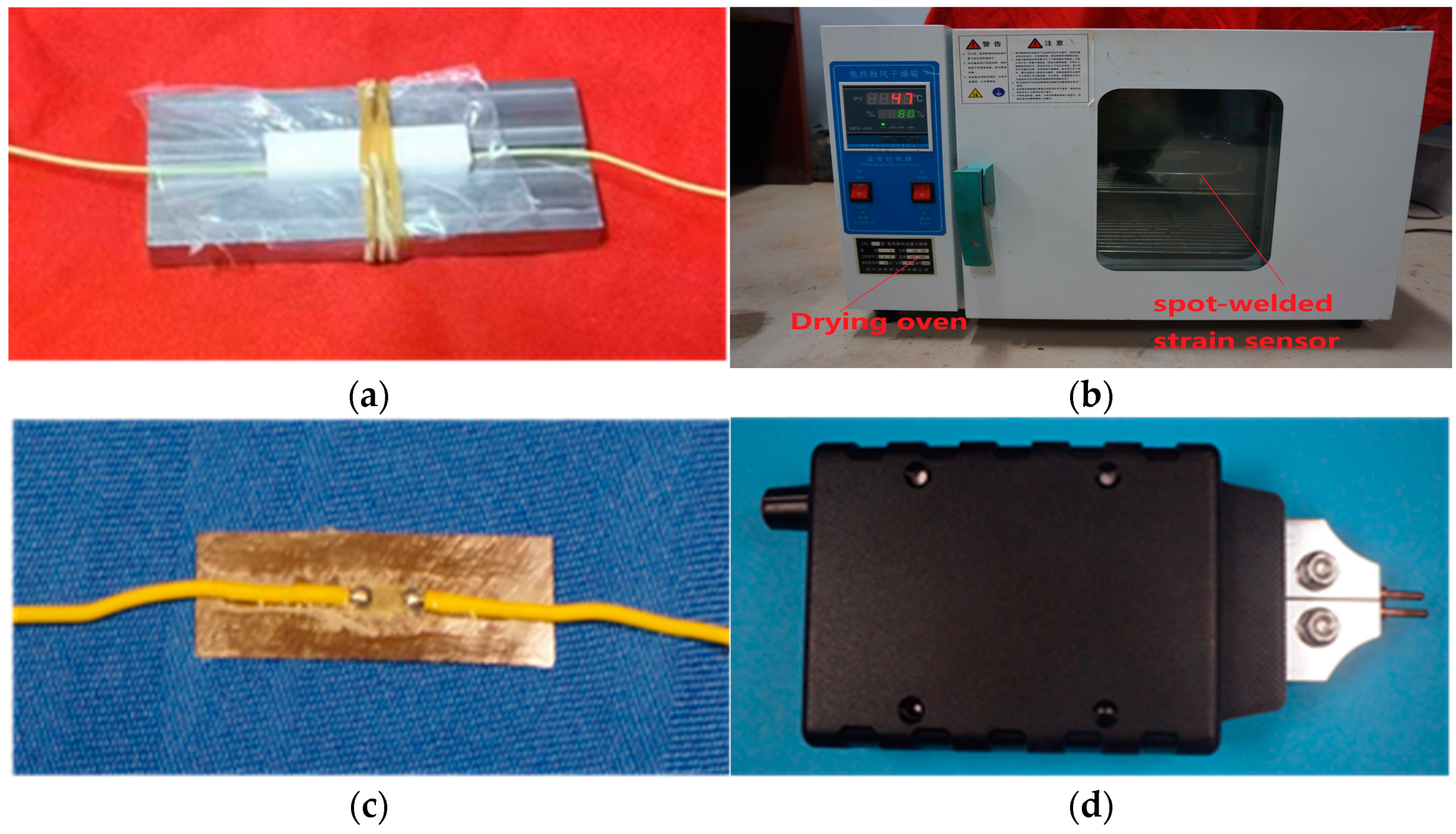
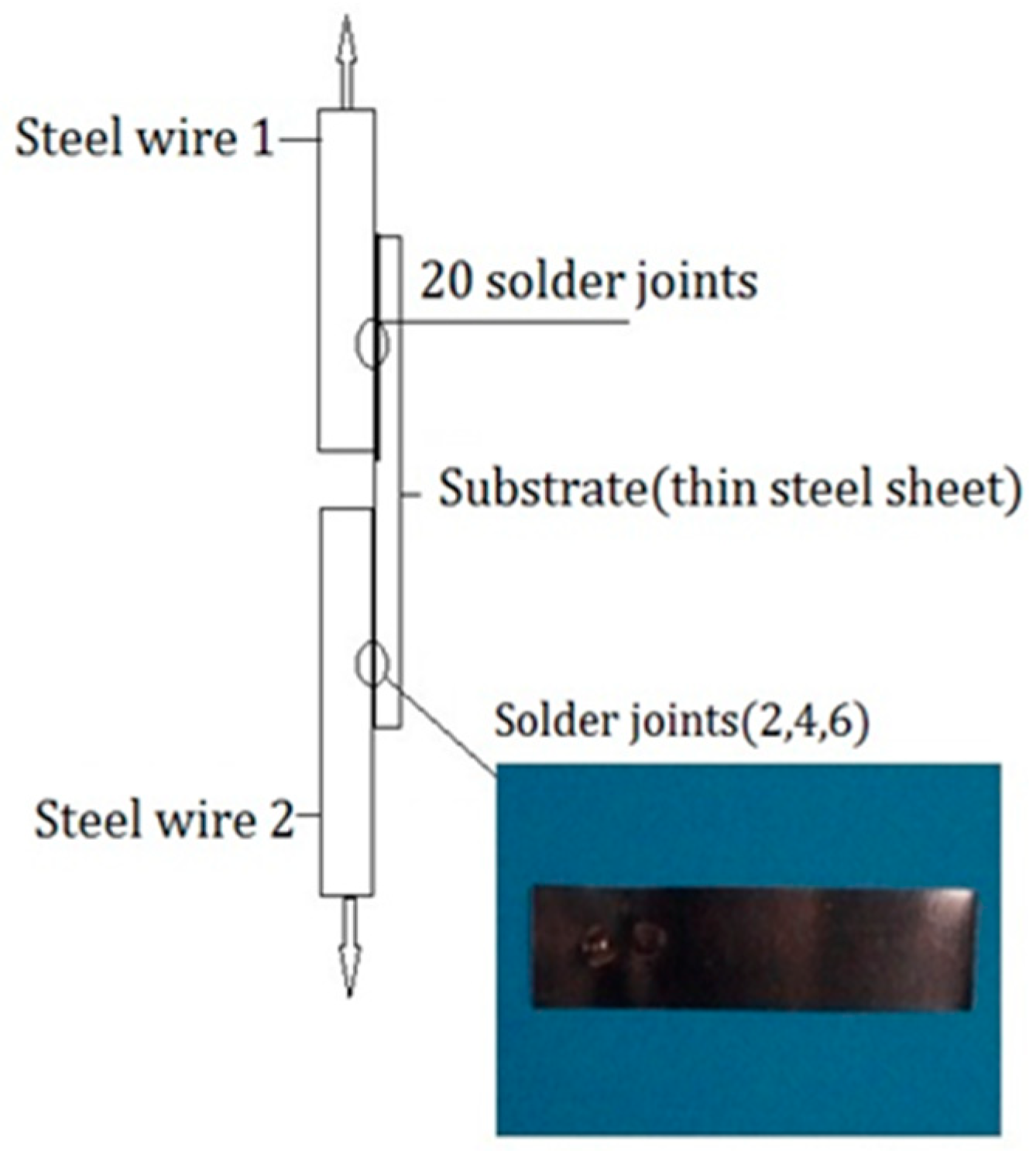

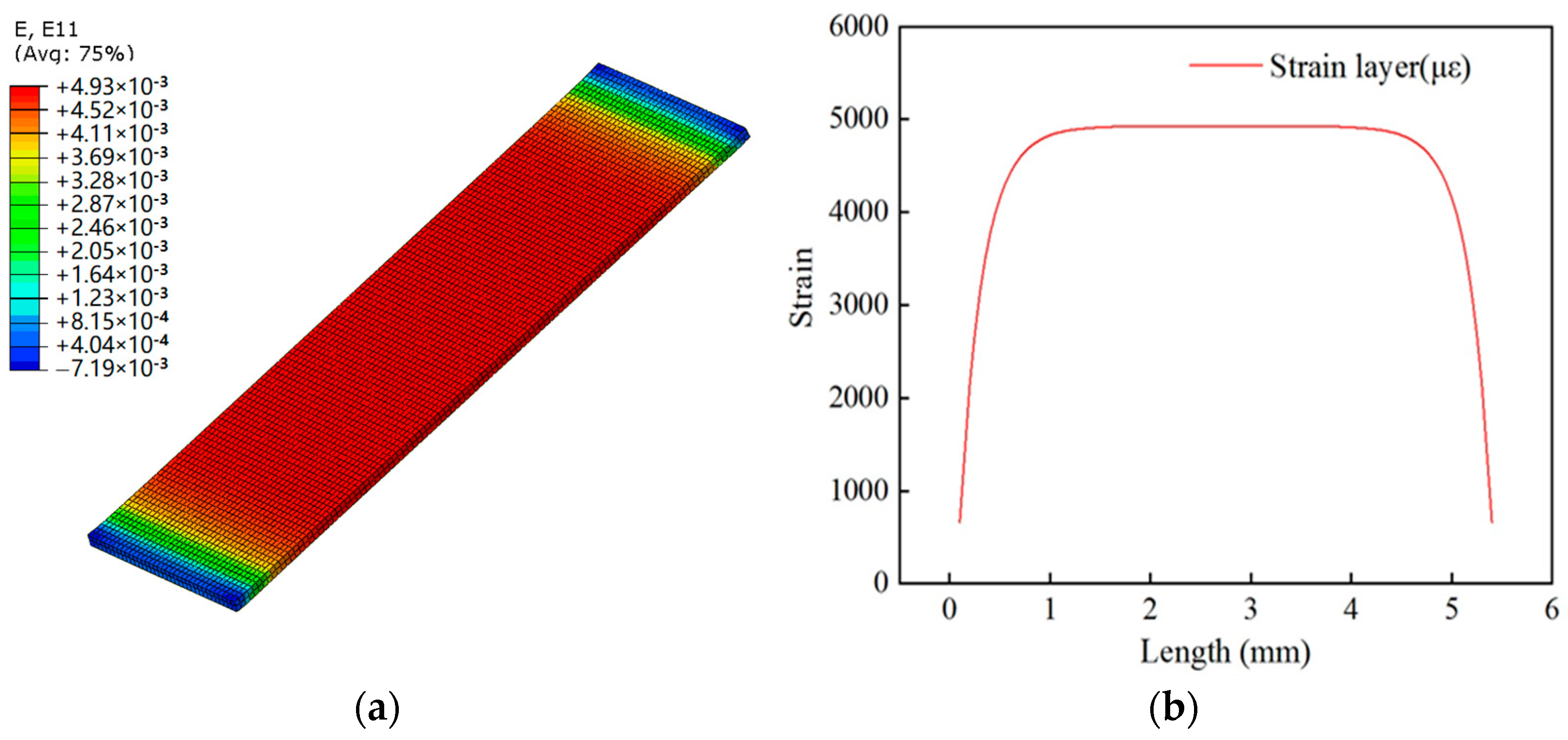
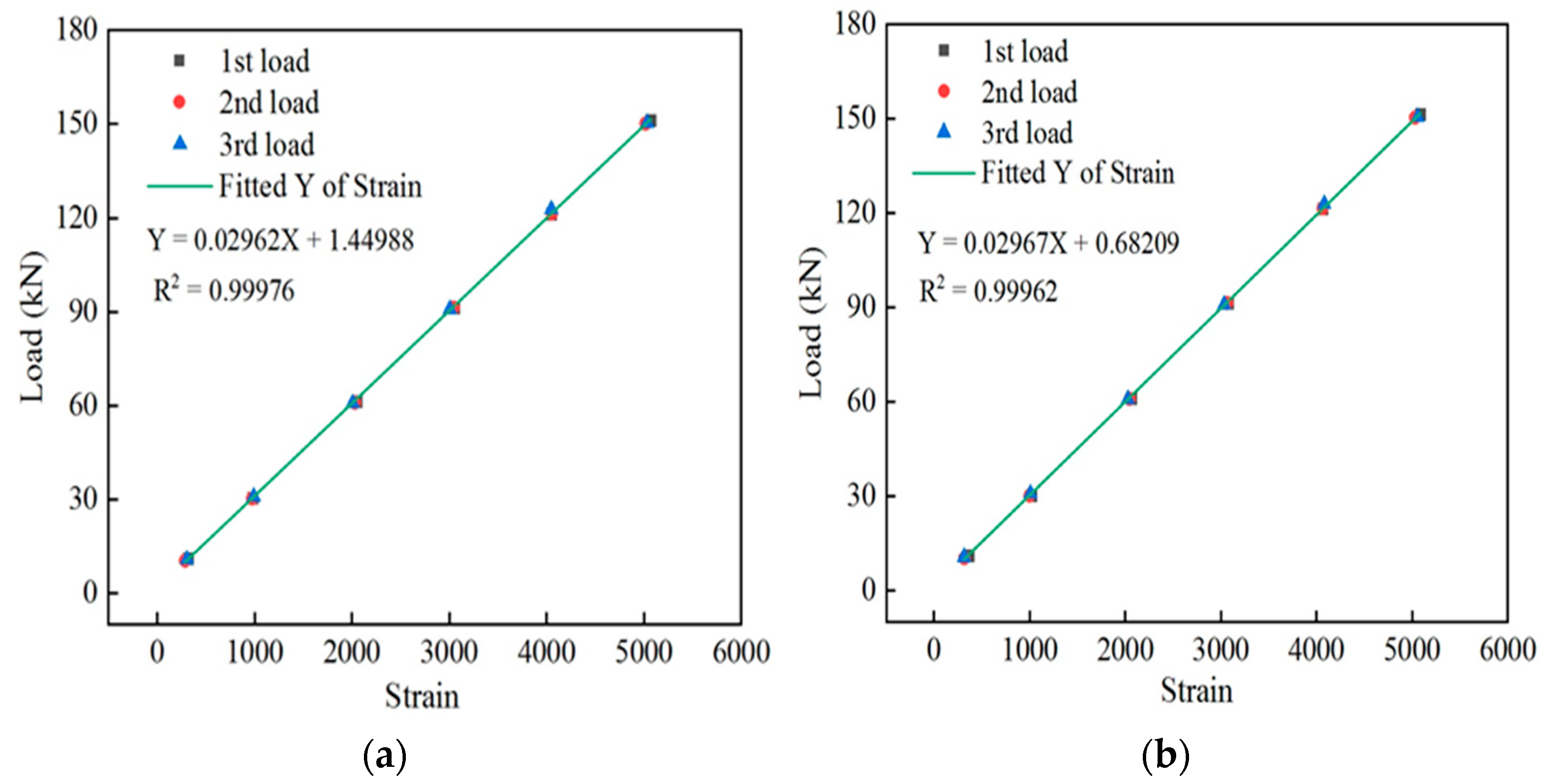



| Test | Number of Solder Joints/n | Tension/N | Shear Stress/MPa |
|---|---|---|---|
| 1 | 2 | 54.7 | 35.5 |
| 2 | 4 | 118.2 | 38.4 |
| 3 | 6 | 174.2 | 37.7 |
| Average | / | / | 37.2 |
| Part | Geometry/mm | Elastic Modulus/MPa | Poisson’s Ratio | Shear Modulus/MPa |
|---|---|---|---|---|
| Strain layer | 5.5 × 1.8 × 0.1 | 1.1 × 105 | 0.33 | 4.1 × 104 |
| Cement layer | 5.5 × 1.8 × 0.1 | 1.0 × 103 | 0.35 | 3.7 × 102 |
| Packaging substrate | 26 × 7 × 0.05 | 2.1 × 105 | 0.3 | 8.1 × 104 |
| Steel plate test piece | 200 × 50 × 5 | 2.1 × 105 | 0.3 | 8.1 × 104 |
| Number of Loads (N) | The Pressure Sensor Indicator (F)/kN | Tension Values Tested by Sensor #1 (T1)/kN | Error Value of Sensor #1 (ε1)/% | Tension Values Tested by Sensor #1 (T2)/kN | Error Value of Sensor #2 (ε2)/% | Average Tension Value (T)/kN | Average Error Value (ε)/% |
|---|---|---|---|---|---|---|---|
| 1 | 30.41 | 30.40 | −0.02 | 30.80 | 1.28 | 30.60 | 0.63 |
| 61.23 | 62.12 | 1.46 | 61.89 | 1.07 | 62.00 | 1.26 | |
| 91.39 | 91.86 | 0.51 | 91.89 | 0.54 | 91.87 | 0.53 | |
| 121.32 | 121.31 | −0.01 | 121.16 | −0.13 | 121.23 | −0.07 | |
| 151.39 | 151.52 | 0.09 | 151.63 | 0.16 | 151.58 | 0.12 | |
| 2 | 30.20 | 30.32 | 0.41 | 30.29 | 0.30 | 30.31 | 0.36 |
| 60.89 | 61.57 | 1.11 | 61.38 | 0.80 | 61.47 | 0.96 | |
| 91.33 | 91.66 | 0.36 | 91.58 | 0.27 | 91.62 | 0.32 | |
| 121.41 | 121.55 | 0.11 | 121.17 | −0.19 | 121.36 | −0.04 | |
| 150.21 | 150.06 | −0.10 | 149.92 | −0.19 | 149.99 | −0.15 | |
| 3 | 30.85 | 30.83 | −0.06 | 30.55 | −0.98 | 30.69 | −0.52 |
| 60.78 | 60.95 | 0.29 | 60.81 | 0.05 | 60.88 | 0.17 | |
| 90.83 | 90.46 | −0.41 | 90.66 | −0.18 | 90.56 | −0.29 | |
| 122.77 | 121.39 | −1.12 | 121.76 | −0.82 | 121.58 | −0.97 | |
| 150.36 | 150.49 | 0.09 | 150.74 | 0.25 | 150.62 | 0.17 |
| Temperature /°C | Test Force Value/kN | Strain Value | Temperature Computational Force/kN | Compensation Computational Force/kN | Compensation Full Capacity Error (ε)/% |
|---|---|---|---|---|---|
| 0 | 11 | −60 | 4.7 | 10.9 | −0.1 |
| 31 | 613 | 23.9 | 30.1 | −0.6 | |
| 61 | 1709 | 55.2 | 61.5 | 0.3 | |
| 91 | 2766 | 85.5 | 91.7 | 0.5 | |
| 121 | 3841 | 116.2 | 122.5 | 1.0 | |
| 151 | 4792 | 143.4 | 149.7 | −0.8 | |
| 10 | 11 | 51 | 7.8 | 11.0 | 0 |
| 31 | 736 | 27.4 | 30.5 | −0.3 | |
| 61 | 1787 | 57.5 | 60.6 | −0.3 | |
| 91 | 2899 | 89.3 | 92.4 | 0.9 | |
| 121 | 3940 | 119.1 | 122.2 | 0.8 | |
| 151 | 4921 | 147.1 | 150.2 | −0.5 | |
| 20 | 11 | 138 | 10.3 | 10.3 | −0.5 |
| 31 | 885 | 31.7 | 31.7 | 0.5 | |
| 61 | 1918 | 61.2 | 61.2 | 0.1 | |
| 91 | 2948 | 90.7 | 90.7 | −0.2 | |
| 121 | 3999 | 120.7 | 120.8 | −0.1 | |
| 151 | 5059 | 151.1 | 151.1 | 0.1 | |
| 30 | 11 | 273 | 14.2 | 11.1 | 0.1 |
| 31 | 968 | 34.1 | 31.0 | 0 | |
| 61 | 2033 | 64.5 | 61.4 | 0.3 | |
| 91 | 3085 | 94.6 | 91.5 | 0.3 | |
| 121 | 4166 | 125.5 | 122.4 | 0.9 | |
| 151 | 5171 | 154.3 | 151.2 | 0.1 | |
| 40 | 11 | 392 | 17.6 | 11.4 | 0.3 |
| 31 | 1070 | 37.0 | 30.8 | −0.1 | |
| 61 | 2111 | 66.8 | 60.6 | −0.3 | |
| 91 | 3167 | 97.0 | 90.8 | −0.1 | |
| 121 | 4241 | 127.7 | 121.5 | 0.3 | |
| 151 | 5267 | 157.0 | 150.8 | −0.1 |
| Relaxation | The Pressure Sensor Indicator (F)/kN | Tension Values Tested Using Sensor #1 (T1) /kN | Error Value of Sensor #1 (ε1)/% | Tension Values Tested Using Sensor #1 (T2)/kN | Error Value of Sensor #2 (ε2)/% | Average Tension Value (T)/kN | Average Error Value (ε)/% |
|---|---|---|---|---|---|---|---|
| Before | 30.9 | 30.61 | −0.95 | 30.83 | −0.22 | 30.72 | −0.58 |
| 60.7 | 61.23 | 0.88 | 61.26 | 0.92 | 61.25 | 0.90 | |
| 90.6 | 91.10 | 0.55 | 91.19 | 0.66 | 91.15 | 0.60 | |
| 120.2 | 120.33 | 0.10 | 120.39 | 0.16 | 120.36 | 0.13 | |
| 150.3 | 149.72 | −0.38 | 149.62 | −0.45 | 149.67 | −0.42 | |
| After | 30.7 | 31.39 | 2.25 | 30.85 | 0.50 | 31.12 | 1.38 |
| 60.0 | 60.62 | 1.04 | 60.60 | 1.00 | 60.61 | 1.02 | |
| 90.6 | 88.38 | −2.45 | 89.15 | −1.60 | 88.76 | −2.03 | |
| 120.5 | 121.36 | 0.72 | 121.43 | 0.77 | 121.40 | 0.74 | |
| 150.9 | 151.17 | 0.18 | 150.77 | −0.09 | 150.97 | 0.05 |
| Standard Load/kN | Strain in Sensor #1/με | Strain in Sensor #2/με | Average Strain/με | Cable Force/kN | Error/%F.S. (Full Scale) |
|---|---|---|---|---|---|
| 213.3 | 404.7 | 373.5 | 389.1 | 228.8 | −0.5 |
| 363.1 | 594.8 | 608.1 | 601.4 | 389.6 | −0.9 |
| 719.7 | 1019.9 | 1133.5 | 1076.7 | 749.5 | −1.0 |
| 1089.9 | 1459.8 | 1655.5 | 1557.6 | 1113.6 | −0.8 |
| 1456.1 | 1902.0 | 2148 | 2025.0 | 1467.5 | −0.4 |
| 1826.1 | 2364.5 | 2648.2 | 2506.3 | 1832.0 | −0.2 |
| 2196.4 | 2852.4 | 3177.1 | 3014.7 | 2217.0 | −0.7 |
| 2555.8 | 3329.7 | 3681.4 | 3505.5 | 2588.6 | −1.1 |
| 2925.1 | 3806.0 | 4187.2 | 3996.6 | 2960.5 | −1.2 |
| 200.1 | 377.6 | 342.5 | 360.0 | 206.8 | −0.2 |
| 376.2 | 572.6 | 588.6 | 580.6 | 373.8 | 0.1 |
| 740.2 | 1005.3 | 1118.5 | 1061.9 | 738.2 | 0.1 |
| 1097.1 | 1431.0 | 1623.2 | 1527.1 | 1090.5 | 0.2 |
| 1453.1 | 1872.9 | 2116.3 | 1994.6 | 1444.5 | 0.3 |
| 1813.2 | 2331.1 | 2613.1 | 2472.1 | 1806.1 | 0.2 |
| 2192.4 | 2818.2 | 3074.9 | 2946.5 | 2165.3 | 0.9 |
| 2547.8 | 3286.3 | 3612.8 | 3449.5 | 2546.2 | 0.1 |
| 2923.3 | 3766.7 | 4156.4 | 3961.5 | 2933.9 | −0.4 |
| 218.2 | 377.0 | 339.2 | 358.1 | 205.3 | 0.4 |
| 364.7 | 553.7 | 565.0 | 559.3 | 357.7 | 0.2 |
| 731.8 | 977.3 | 1088.5 | 1032.9 | 716.3 | 0.5 |
| 1100.9 | 1418.4 | 1612.1 | 1515.2 | 1081.5 | 0.7 |
| 1457.2 | 1855.6 | 2100.6 | 1978.1 | 1432.0 | 0.8 |
| 1817.2 | 2315.8 | 2600.9 | 2458.3 | 1795.7 | 0.7 |
| 2190.4 | 2787.4 | 3073.3 | 2930.3 | 2153.1 | 1.3 |
| 2552 | 3260.9 | 3617.6 | 3439.2 | 2538.4 | 0.5 |
| 2926.5 | 3725.9 | 4149.8 | 3937.8 | 2916.0 | 0.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, N.; Hu, L.; Liu, X.; Tang, Z. Investigating the Mechanical Properties and Temperature Compensation of a Spot-Welded Strain Sensor within an Intelligent Steel Strand Cable. Sensors 2024, 24, 745. https://doi.org/10.3390/s24030745
Deng N, Hu L, Liu X, Tang Z. Investigating the Mechanical Properties and Temperature Compensation of a Spot-Welded Strain Sensor within an Intelligent Steel Strand Cable. Sensors. 2024; 24(3):745. https://doi.org/10.3390/s24030745
Chicago/Turabian StyleDeng, Nianchun, Lehai Hu, Xin Liu, and Zhiyu Tang. 2024. "Investigating the Mechanical Properties and Temperature Compensation of a Spot-Welded Strain Sensor within an Intelligent Steel Strand Cable" Sensors 24, no. 3: 745. https://doi.org/10.3390/s24030745
APA StyleDeng, N., Hu, L., Liu, X., & Tang, Z. (2024). Investigating the Mechanical Properties and Temperature Compensation of a Spot-Welded Strain Sensor within an Intelligent Steel Strand Cable. Sensors, 24(3), 745. https://doi.org/10.3390/s24030745






