Experimental Investigation of Three-Dimensional Multi-Directional Piezoelectric Wind Energy Harvester
Abstract
1. Introduction
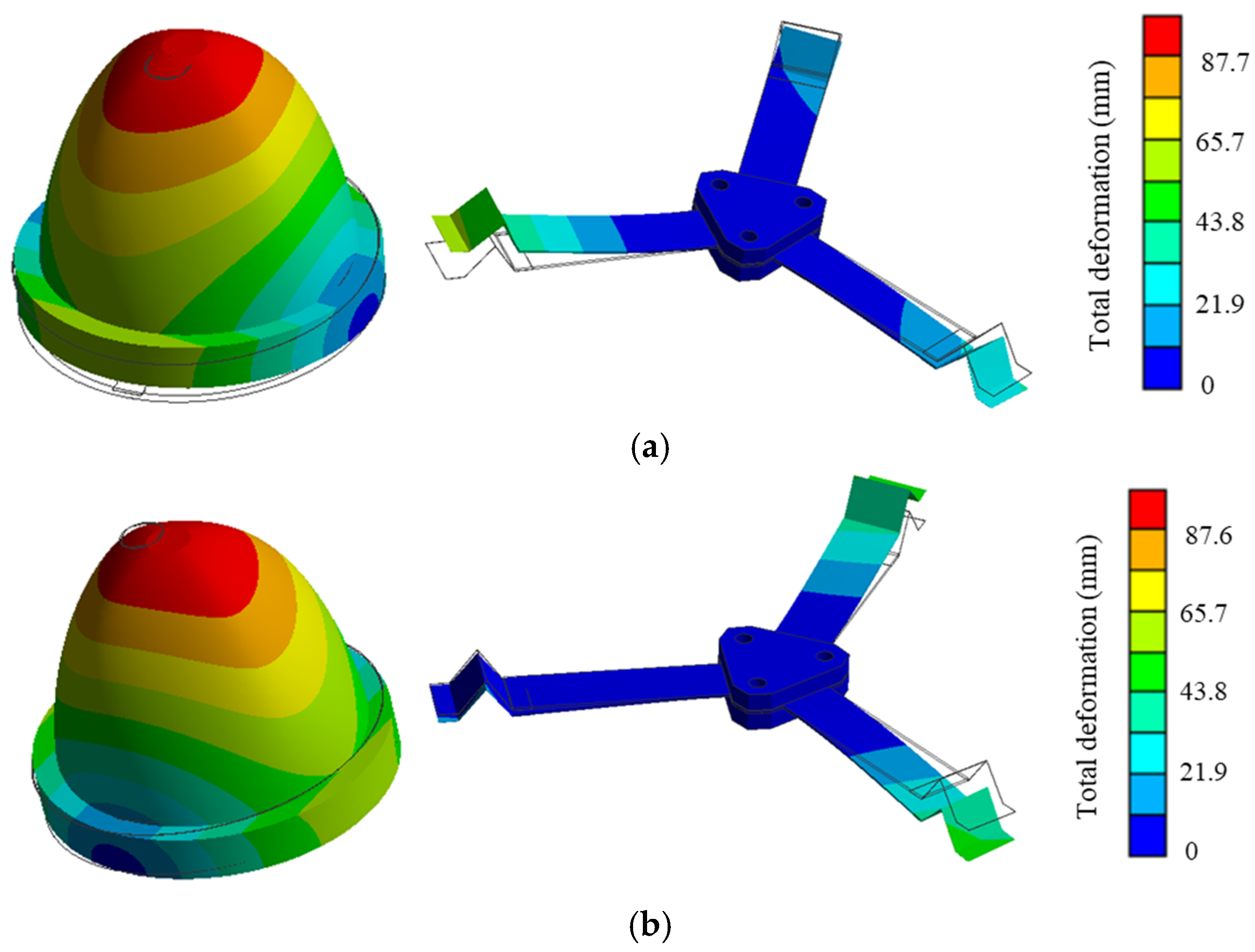
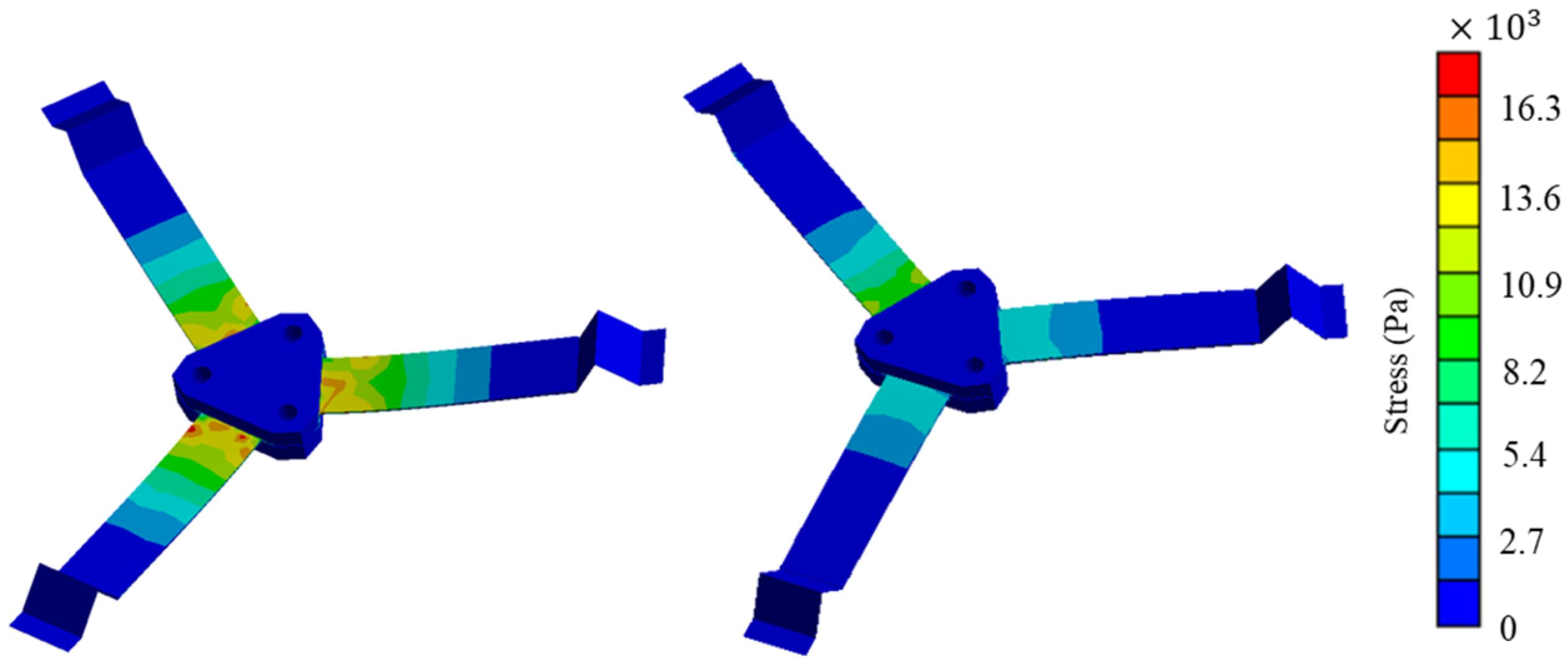
2. Harvester Structure and Simulation Analysis
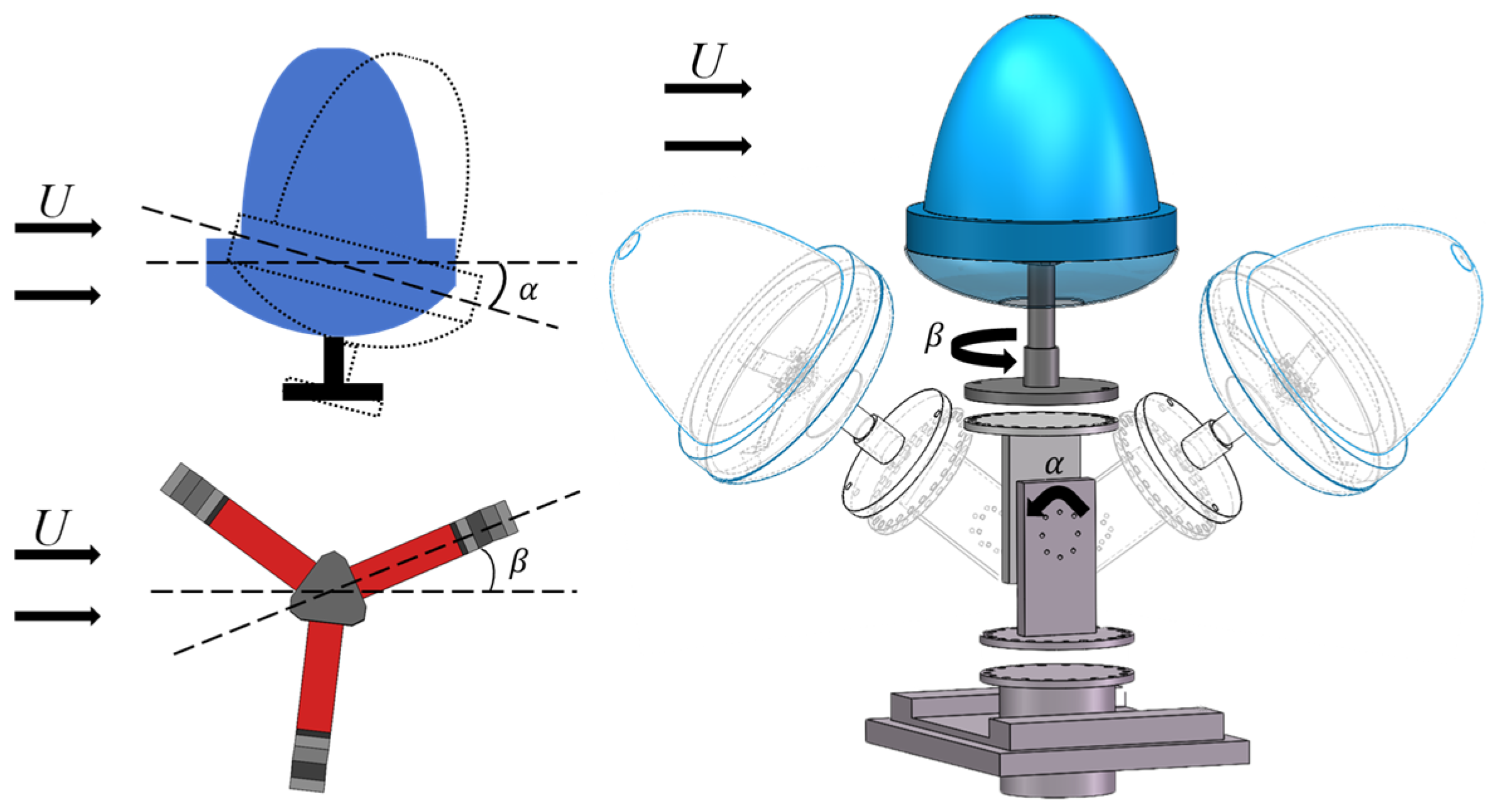
2.1. Harvester Structure
2.2. Working Principle
3. Experimental Results Analysis
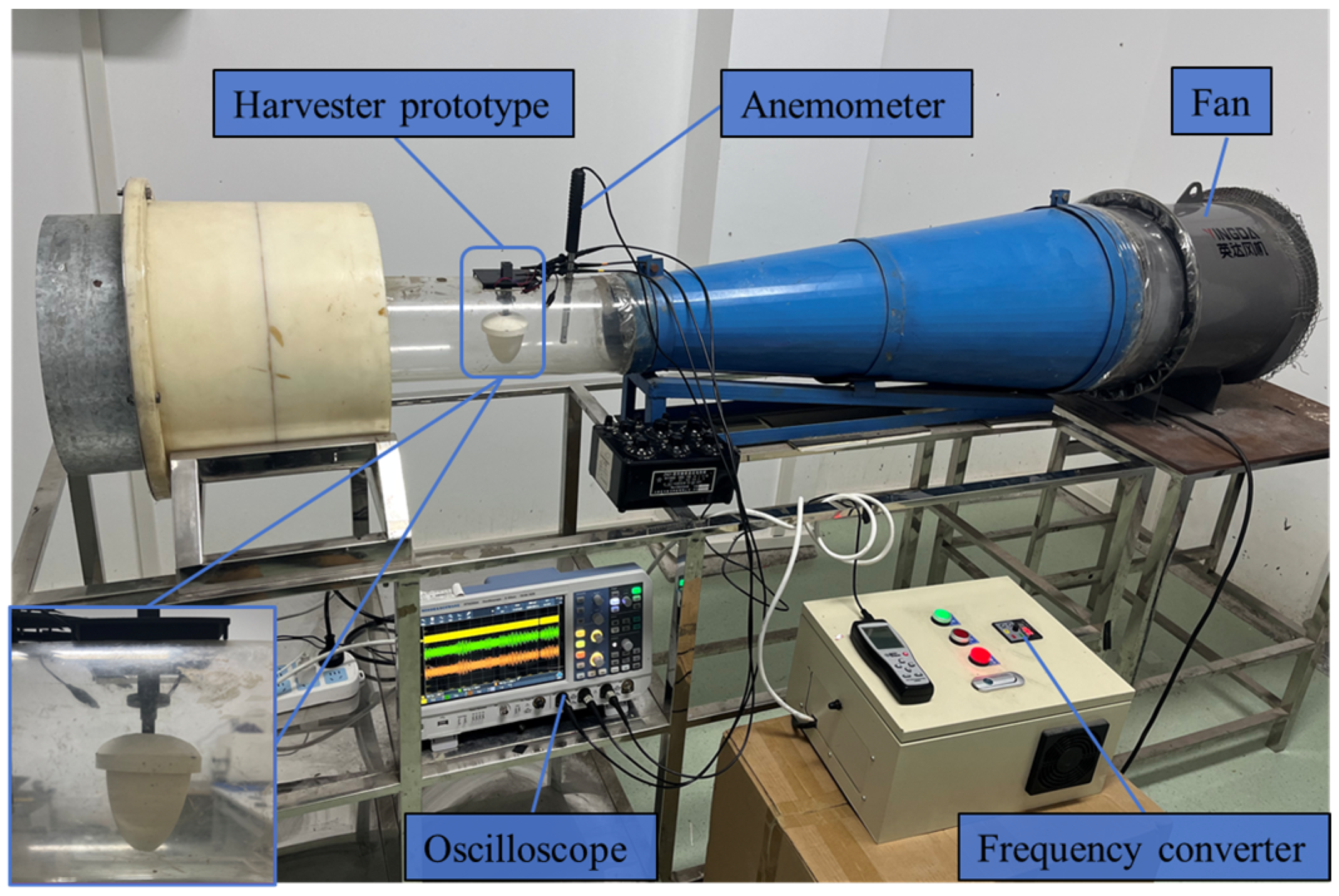
3.1. Prototype Fabrication and Experimental Setup
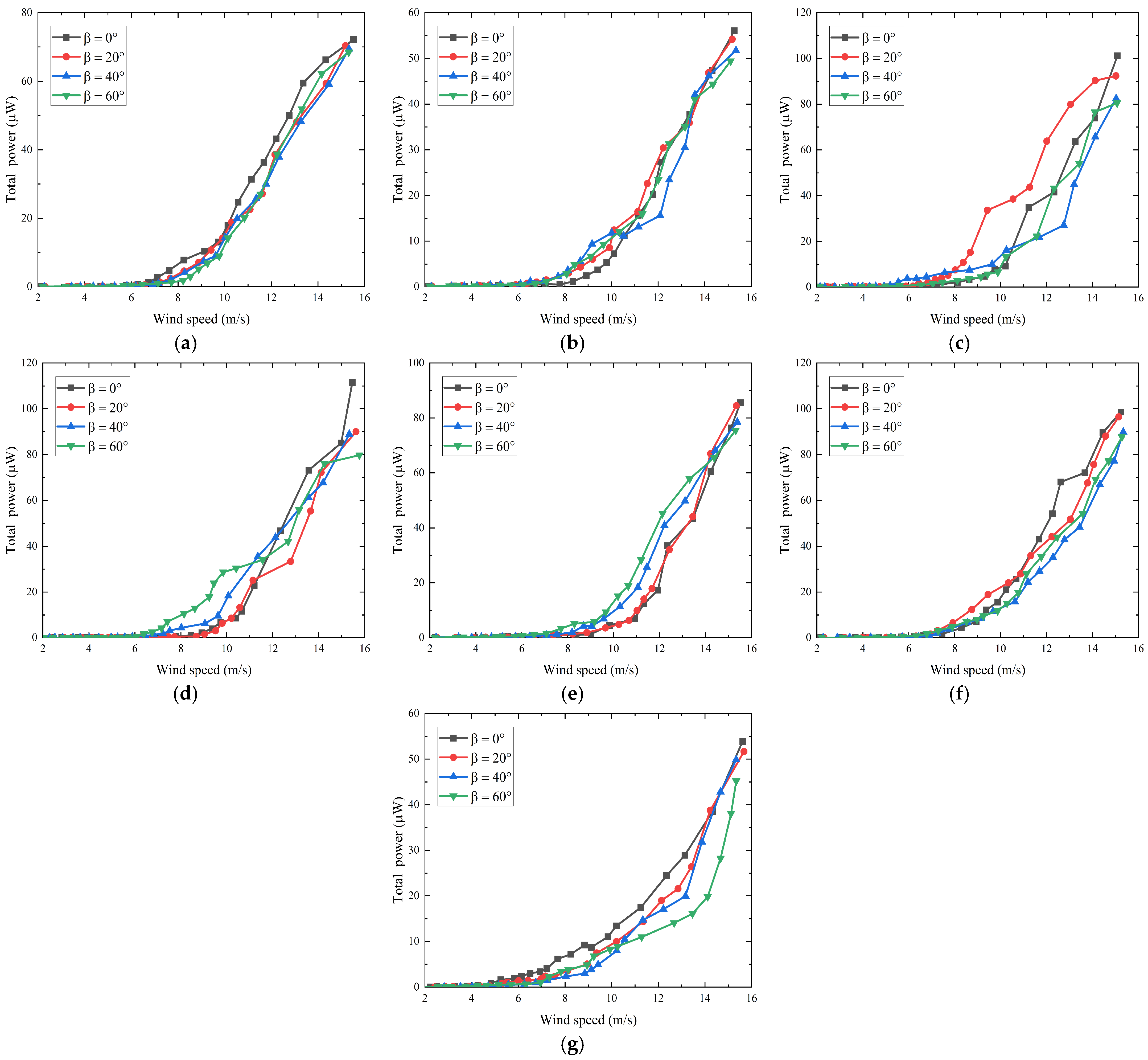
3.2. Experimental Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Roundy, S.; Wright, P.K. A piezoelectric vibration based generator for wireless electronics. Smart Mater. Struct. 2004, 13, 1131–1142. [Google Scholar] [CrossRef]
- Lu, C.; Jiang, X.; Li, L.; Zhou, H.; Yang, A.; Xin, M.; Fu, G.; Wang, X. Wind energy harvester using piezoelectric materials. Rev. Sci. Instrum. 2022, 93, 031502. [Google Scholar] [CrossRef] [PubMed]
- Zakaria, M.Y.; Pereira, D.A.; Hajj, M.R. Experimental investigation and performance modeling of centimeter-scale micro-wind turbine energy harvesters. J. Wind Eng. Ind. Aerodyn. 2015, 147, 58–65. [Google Scholar] [CrossRef]
- Palmieri, M.; Bozzella, S.; Cascella, G.L.; Bronzini, M.; Torresi, M.; Cupertino, F. Wind micro-turbine networks for urban areas: Optimal design and power scalability of permanent magnet generators. Energies 2018, 11, 2759. [Google Scholar] [CrossRef]
- Wei, C.; Jing, X. A comprehensive review on vibration energy harvesting: Modelling and realization. Renew. Sustain. Energy Rev. 2017, 74, 1–18. [Google Scholar] [CrossRef]
- Li, S.; Chen, Z.; He, X.; Ye, Y.; Wan, S.; Dong, L. High performance hybrid omnidirectional wind energy harvester based on flutter for wireless sensing and hydrogen production applications. Nano Energy 2024, 132, 110403. [Google Scholar] [CrossRef]
- Wang, S.; Liao, W.; Zhang, Z.; Liao, Y.; Yan, M.; Kan, J. Development of a novel non-contact piezoelectric wind energy harvester excited by vortex-induced vibration. Energy Convers. Manag. 2021, 235, 113980. [Google Scholar] [CrossRef]
- Wang, J.; Hu, G.; Su, Z.; Li, G.; Zhao, W.; Tang, L.; Zhao, L. A cross-coupled dual-beam for multi-directional energy harvesting from vortex induced vibrations. Smart Mater. Struct. 2019, 28, 12LT02. [Google Scholar] [CrossRef]
- Zhang, Y.; Cheng, G.; Seok, J.; Ding, J.; Sun, W. Enhancing output performance of galloping-based energy harvesting using asymmetric bluff body. Ocean. Eng. 2024, 294, 116793. [Google Scholar] [CrossRef]
- Li, Z.; Zhou, S.; Li, X. A piezoelectric-electromagnetic hybrid flutter-based wind energy harvester: Modeling and nonlinear analysis. Int. J. Non Linear Mech. 2022, 144, 104051. [Google Scholar] [CrossRef]
- Zhao, J.; Yang, J.; Lin, Z.; Zhao, N.; Liu, J.; Wen, Y.; Li, P. An arc-shaped piezoelectric generator for multi-directional wind energy harvesting. Sens. Actuators A 2015, 236, 173–179. [Google Scholar] [CrossRef]
- Tang, M.; Guan, Q.; Wu, X.; Zeng, X.; Zhang, Z.; Yuan, Y. A high-efficiency multidirectional wind energy harvester based on impact effect for self-powered wireless sensors in the grid. Smart Mater. Struct. 2019, 28, 115022. [Google Scholar] [CrossRef]
- Shi, T.; Hu, G.; Zou, L.; Song, J.; Kwok, K.C.S. Performance of an omnidirectional piezoelectric wind energy harvester. Wind Energy 2021, 24, 1167–1179. [Google Scholar] [CrossRef]
- Li, S.; He, X.; Li, J.; Feng, Z.; Yang, X.; Li, J. An in-plane omnidirectional piezoelectric wind energy harvester based on vortex-induced vibration. Appl. Phys. Lett. 2022, 120, 043901. [Google Scholar] [CrossRef]
- Li, S.; Feng, Z.; He, X.; Ye, Y.; Li, J. An in-plane omnidirectional flutter piezoelectric wind energy harvester. Mech. Syst. Signal Process. 2023, 200, 110637. [Google Scholar] [CrossRef]
- Xin, M.; Jiang, X.; Xu, C.; Yang, J.; Lu, C. Two-dimensional omnidirectional wind energy harvester with a cylindrical piezoelectric composite cantilever. Micromachines 2023, 14, 127. [Google Scholar] [CrossRef] [PubMed]





| Parameter | Value | Materials | Young’s Modulus | Density | Poisson’s Ratio |
|---|---|---|---|---|---|
| Diameter of upper curved shell (mm) | 60 | Light weight PLA | 1.94 GPa | 500 kg/m3 | 0.40 |
| Height of upper curved shell (mm) | 50 | ||||
| Diameter of lower curved shell (mm) | 70 | ||||
| Height of lower curved shell (mm) | 15 | ||||
| Diameter of cylindrical shell (mm) | 70 | ||||
| Height of cylindrical shell (mm) | 10 | ||||
| Length of corrugated spring beam (mm) | 6 | Spring steel | 178 GPa | 7850 kg/m3 | 0.28 |
| Height of corrugated spring beam (mm) | 4 | ||||
| Thickness of corrugated spring beam (μm) | 50 | ||||
| Length of piezoelectric layer (mm) | 20 | PVDF film | 2.6 GPa | 1780 kg/m3 | 0.38 |
| Thickness of piezoelectric layer (μm) | 100 | ||||
| Width of piezoelectric layer (mm) | 6 | ||||
| Thickness of PET layer (μm) | 300 | PET film | 4 GPa | 1400 kg/m3 | 0.38 |
| Length of PET layer (mm) | 30 | ||||
| Thickness of supporting bracket (mm) | 2 | PLA | 1.94 GPa | 1240 kg/m3 | 0.40 |
| Piezoelectric strain constant of PVDF (PC/N) | −33 | / | / | / | / |
| Relative permittivity (ε/ε0) | 10.5 | ||||
| Bluff body mass (g) | 3.32 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Z.; Liao, X.; Li, S.; Pu, S.; Li, P.; He, D.; Ye, Y.; He, X. Experimental Investigation of Three-Dimensional Multi-Directional Piezoelectric Wind Energy Harvester. Sensors 2024, 24, 7757. https://doi.org/10.3390/s24237757
Chen Z, Liao X, Li S, Pu S, Li P, He D, Ye Y, He X. Experimental Investigation of Three-Dimensional Multi-Directional Piezoelectric Wind Energy Harvester. Sensors. 2024; 24(23):7757. https://doi.org/10.3390/s24237757
Chicago/Turabian StyleChen, Zonghao, Xiaohan Liao, Shen Li, Shu Pu, Pengfei Li, Dingkun He, Yizhou Ye, and Xuefeng He. 2024. "Experimental Investigation of Three-Dimensional Multi-Directional Piezoelectric Wind Energy Harvester" Sensors 24, no. 23: 7757. https://doi.org/10.3390/s24237757
APA StyleChen, Z., Liao, X., Li, S., Pu, S., Li, P., He, D., Ye, Y., & He, X. (2024). Experimental Investigation of Three-Dimensional Multi-Directional Piezoelectric Wind Energy Harvester. Sensors, 24(23), 7757. https://doi.org/10.3390/s24237757







