Evaluation of Depth Size Based on Layered Magnetization by Double-Sided Scanning for Internal Defects
Abstract
1. Introduction
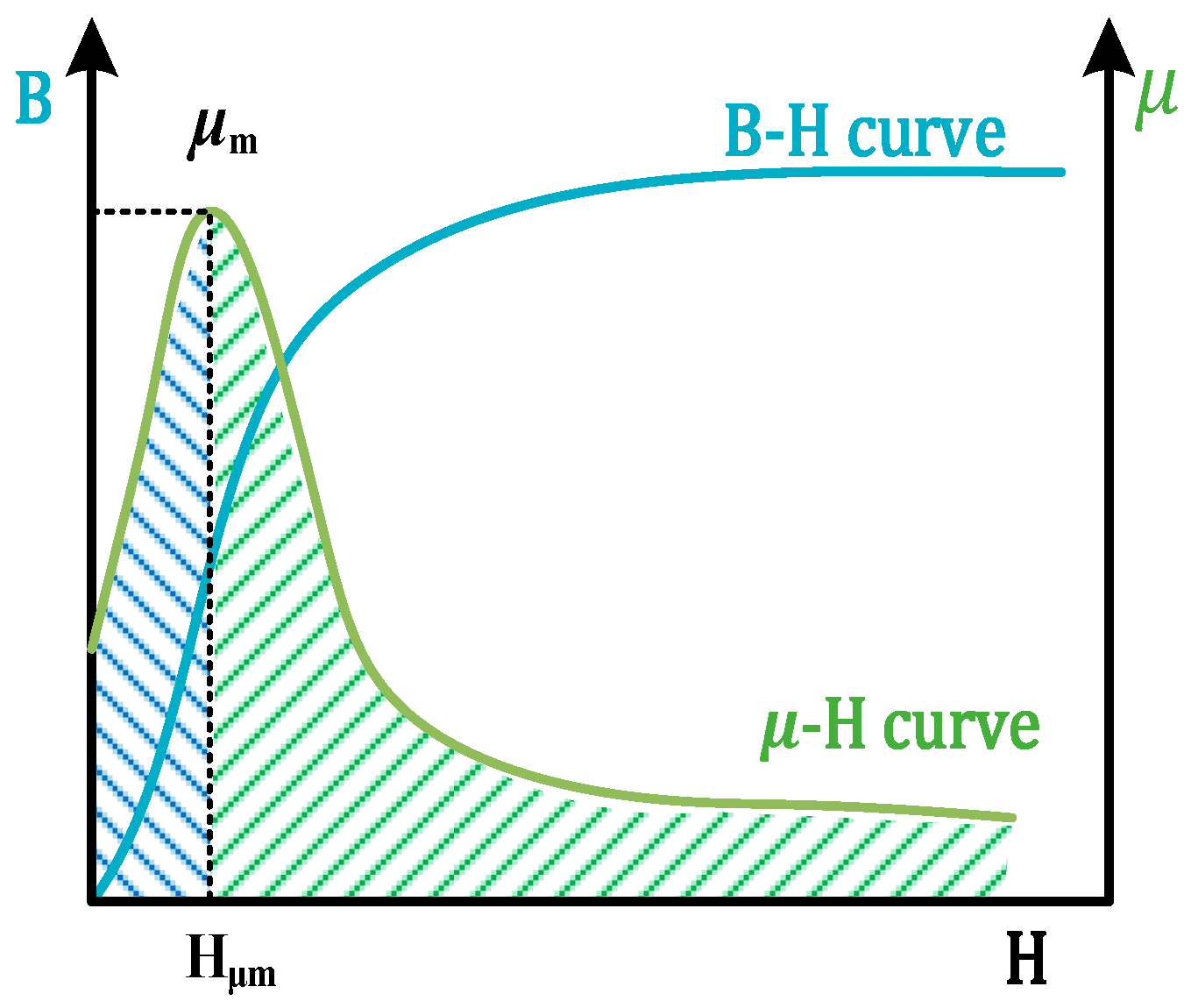
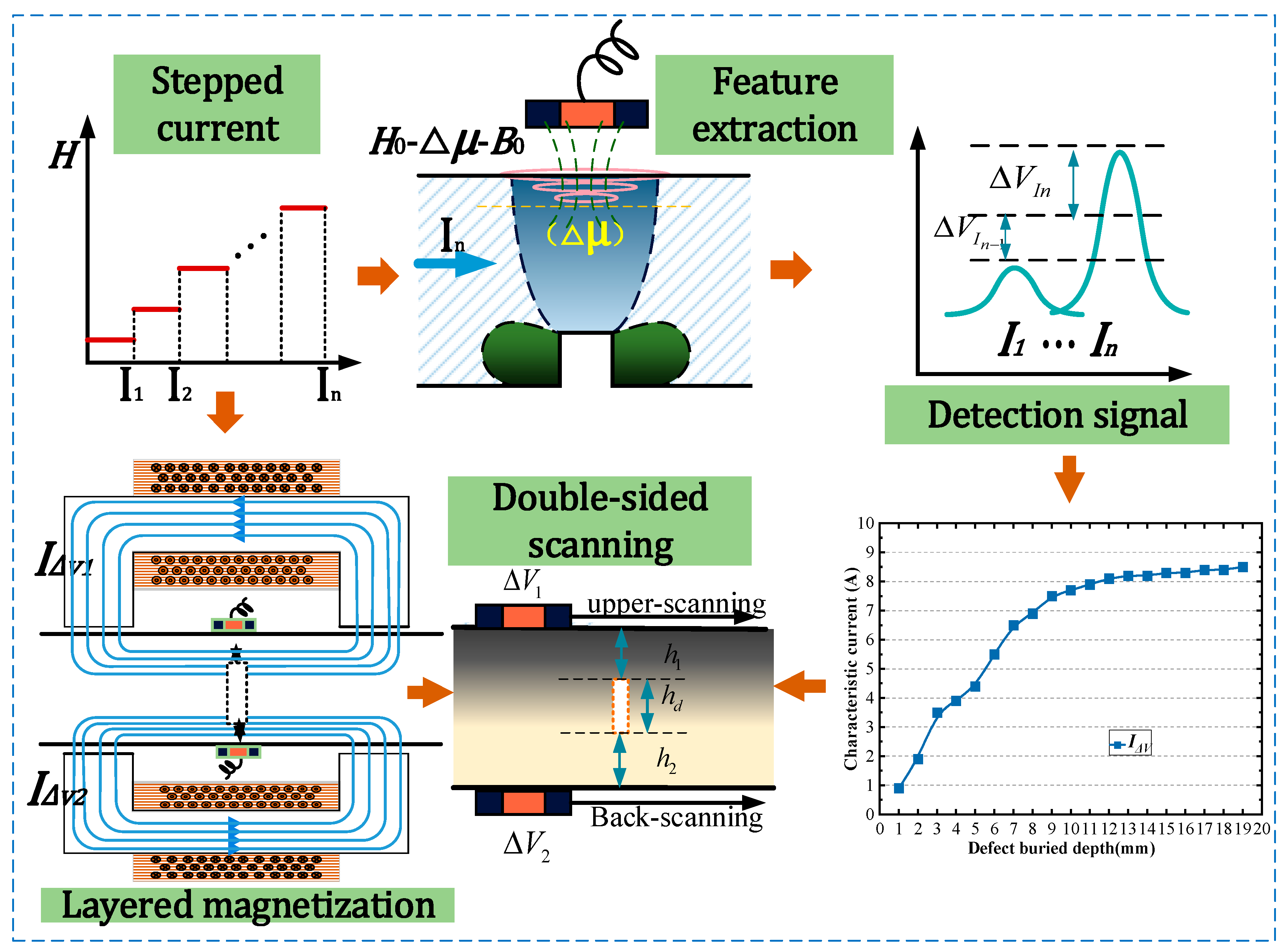
2. Layered Magnetization Mechanism
3. Simulation
3.1. Three-Dimensional (3D) FEM Model
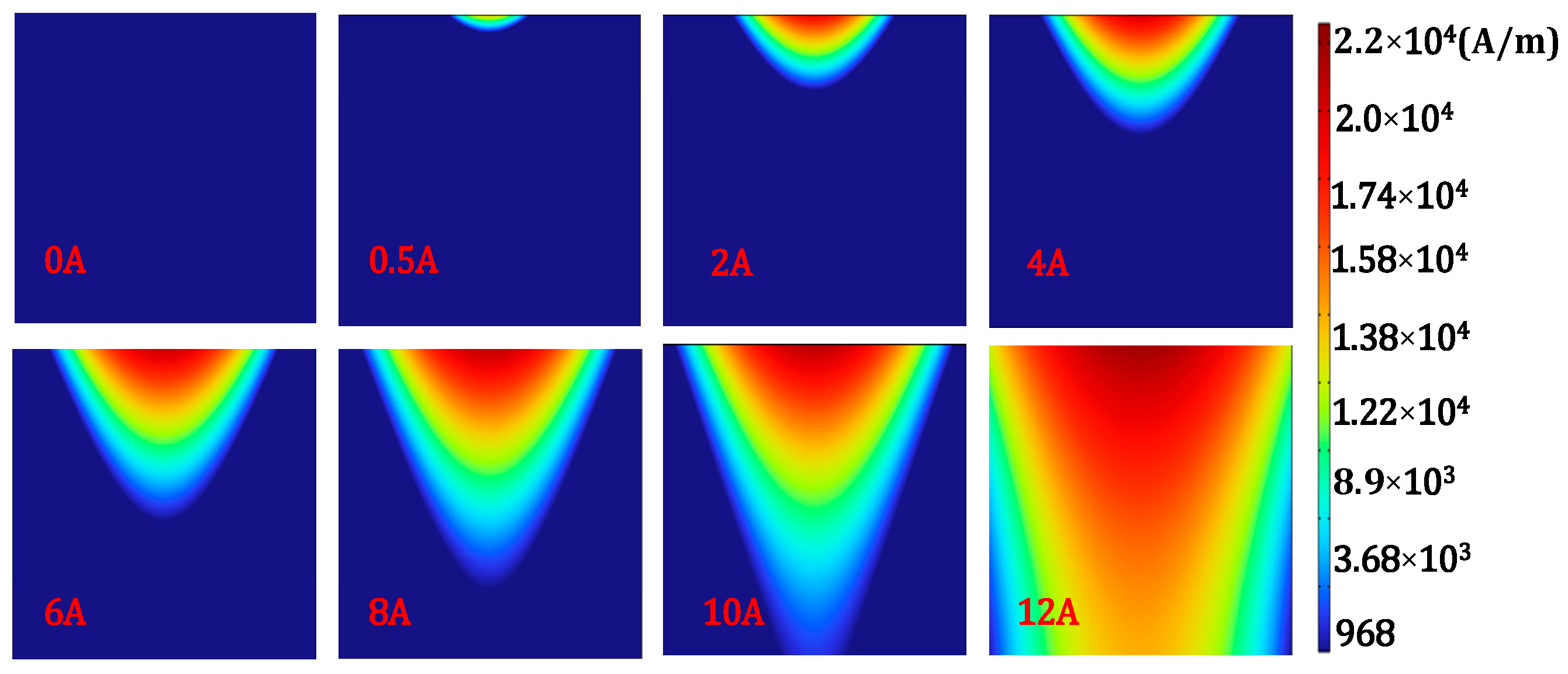
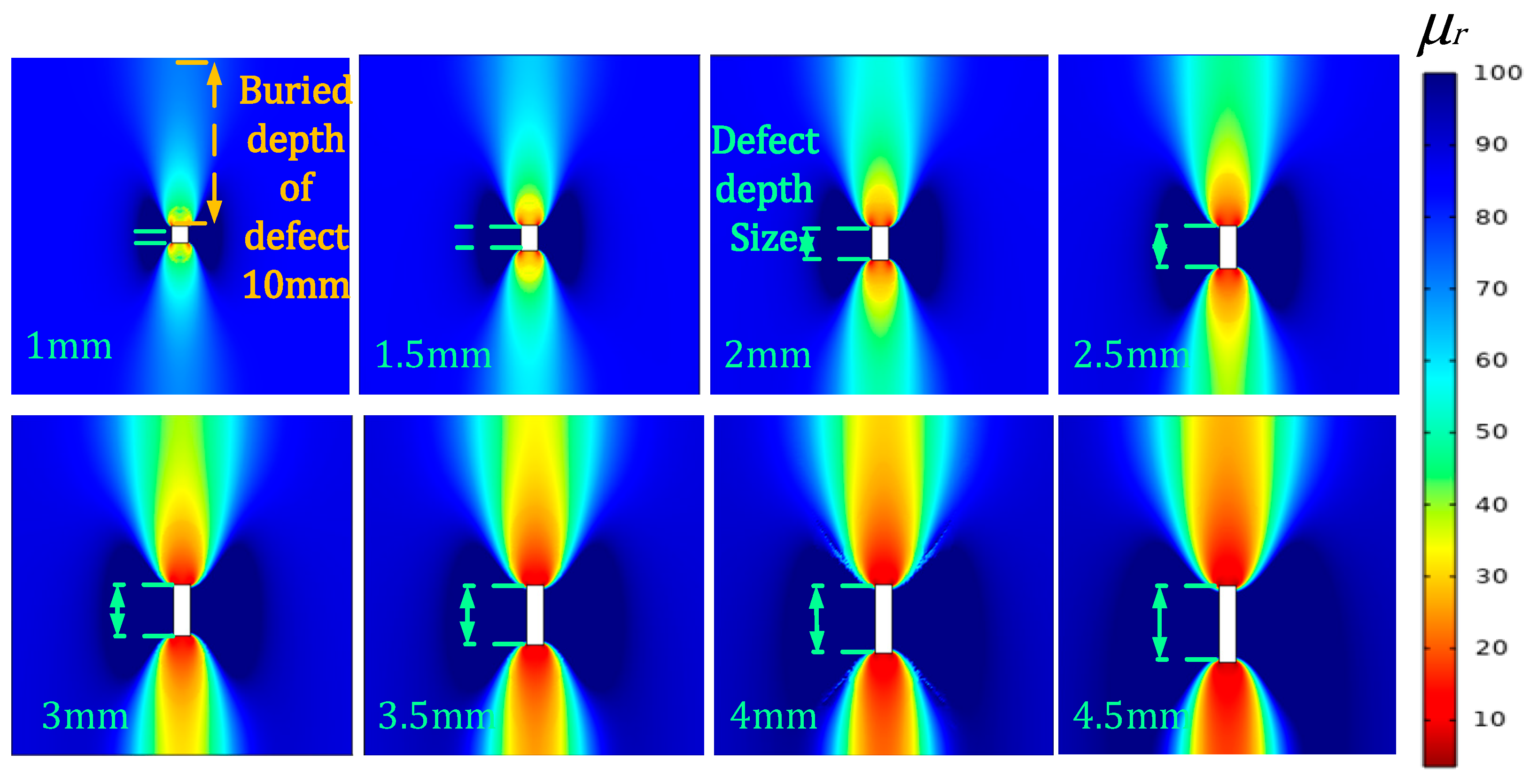
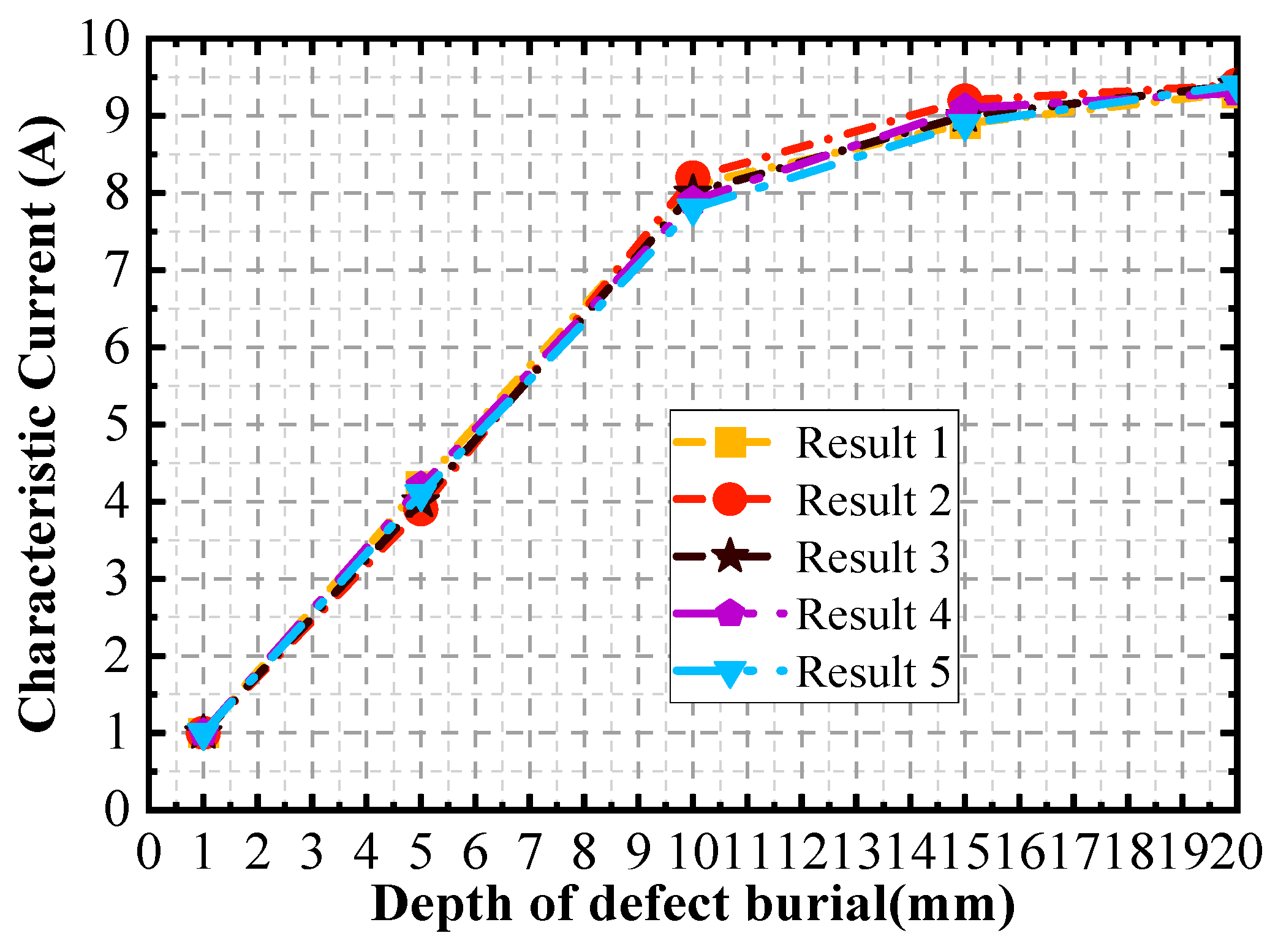
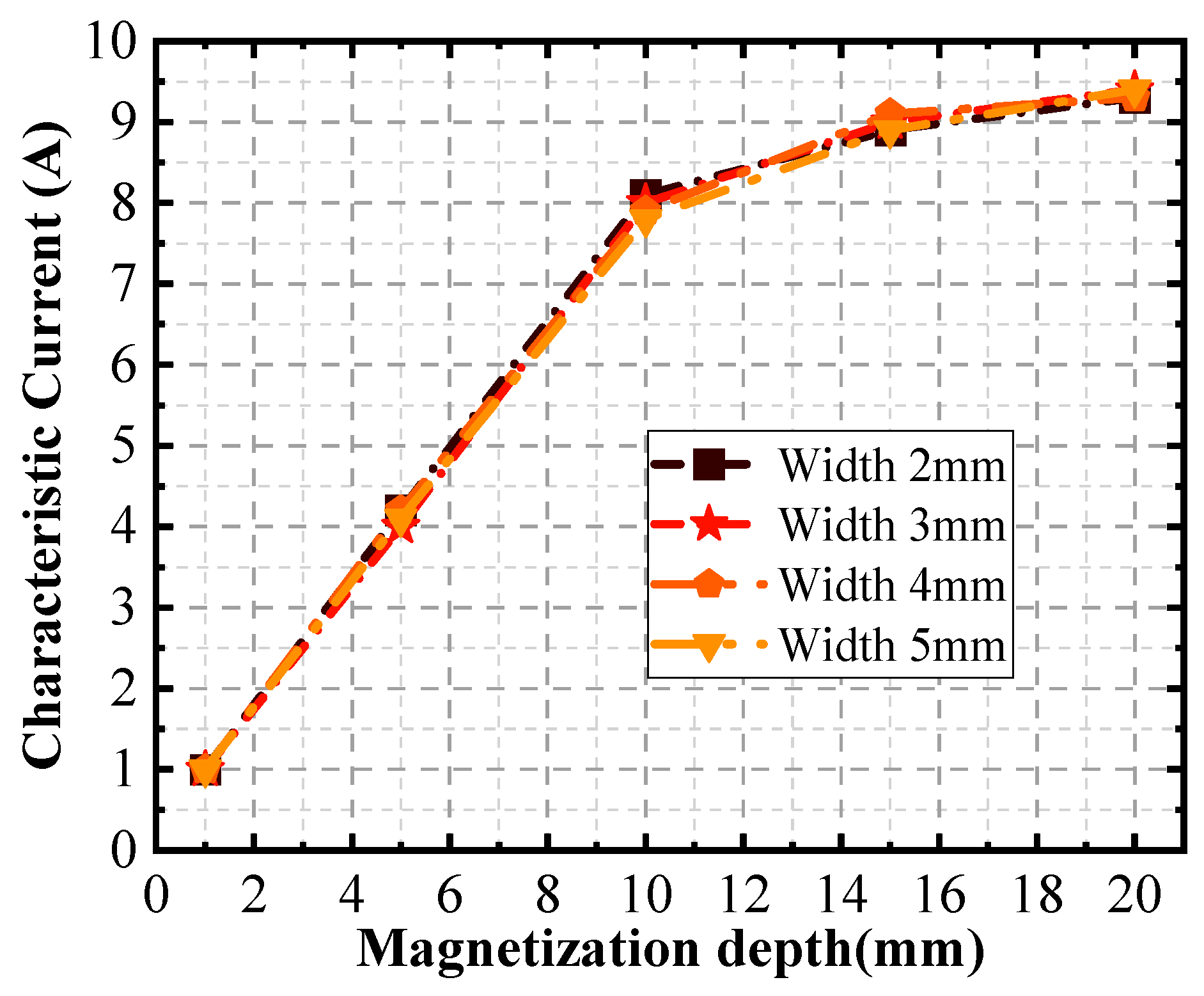
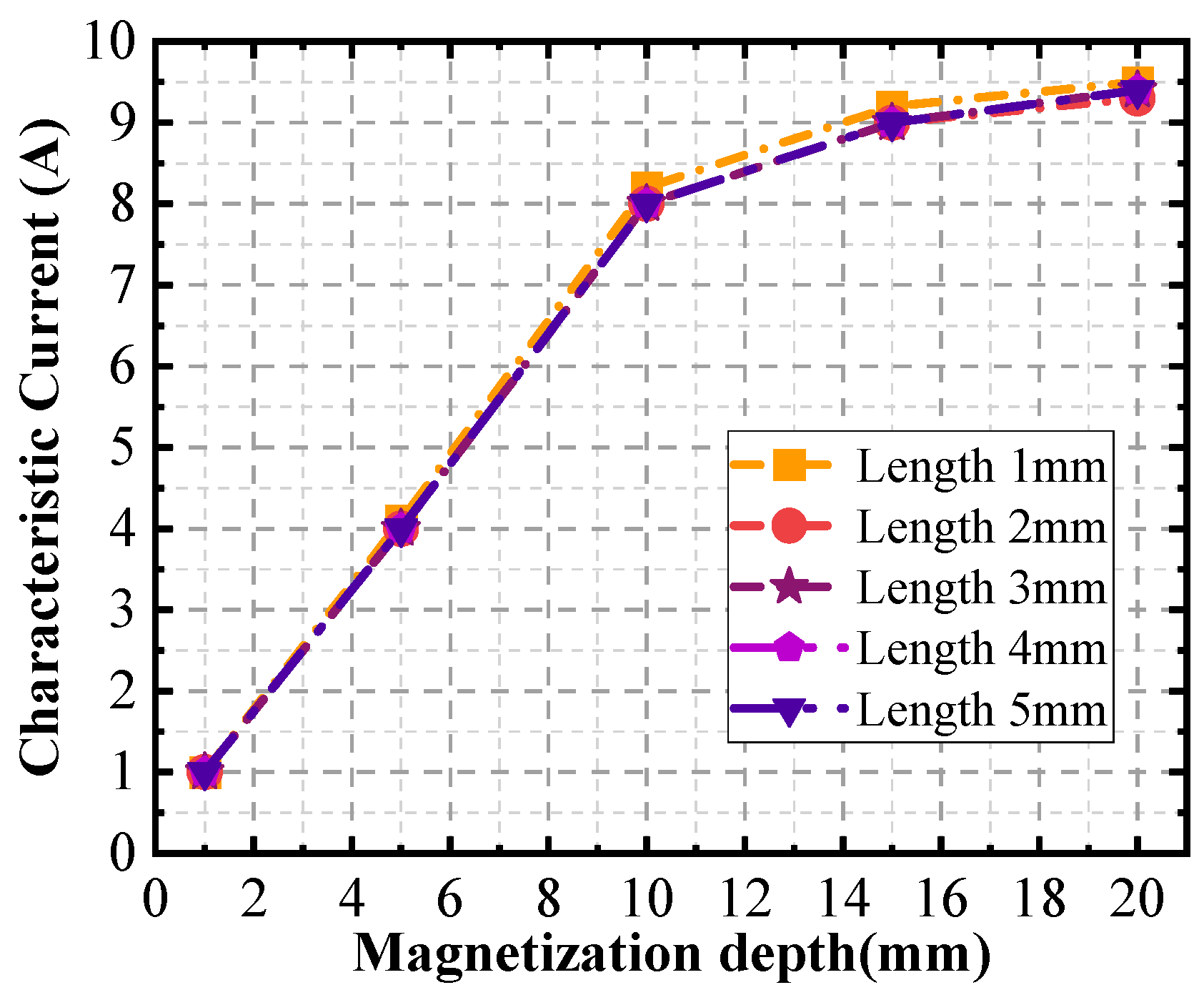
3.2. Magnetization Depth of Different Magnetizing Current
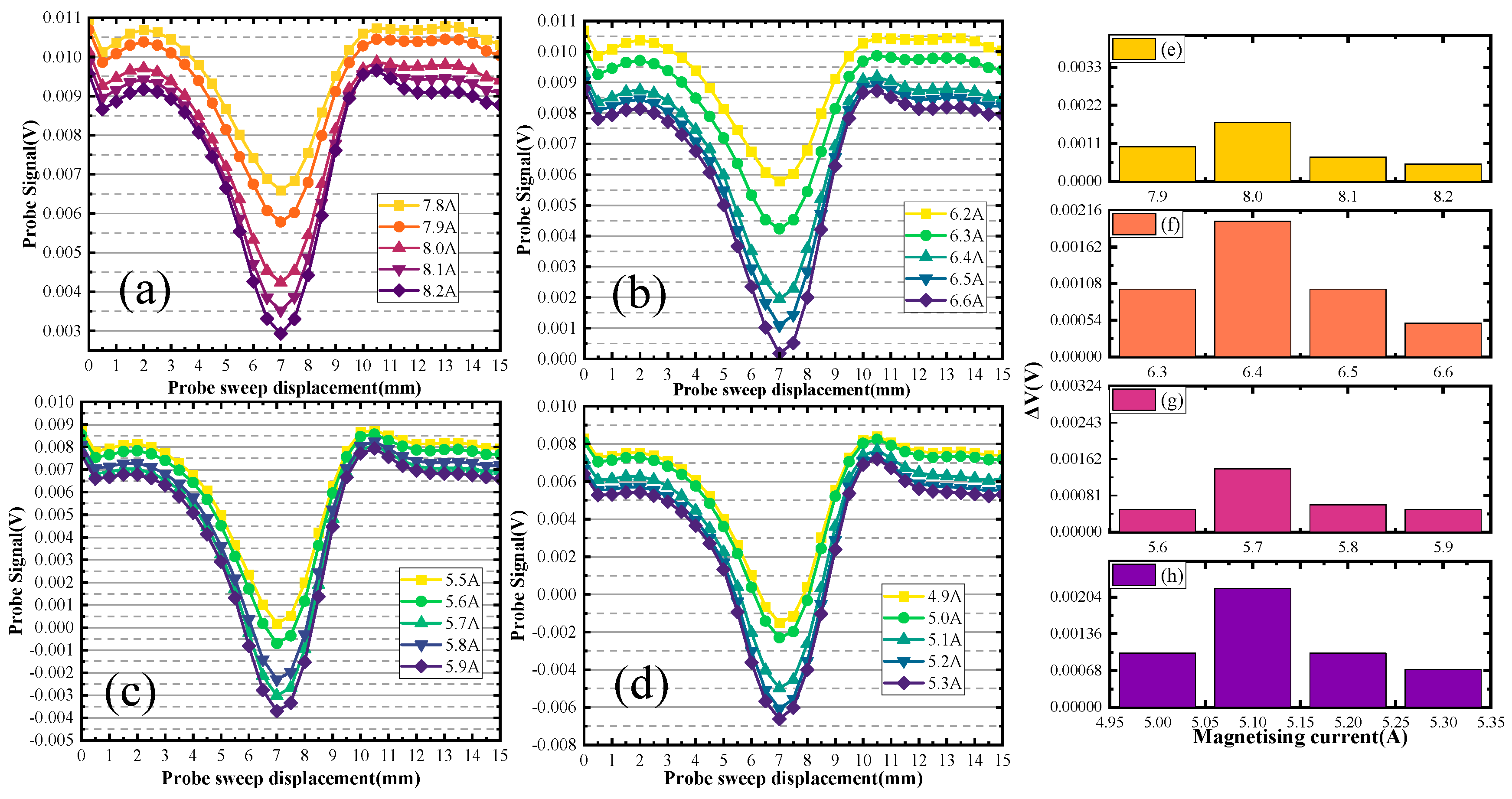
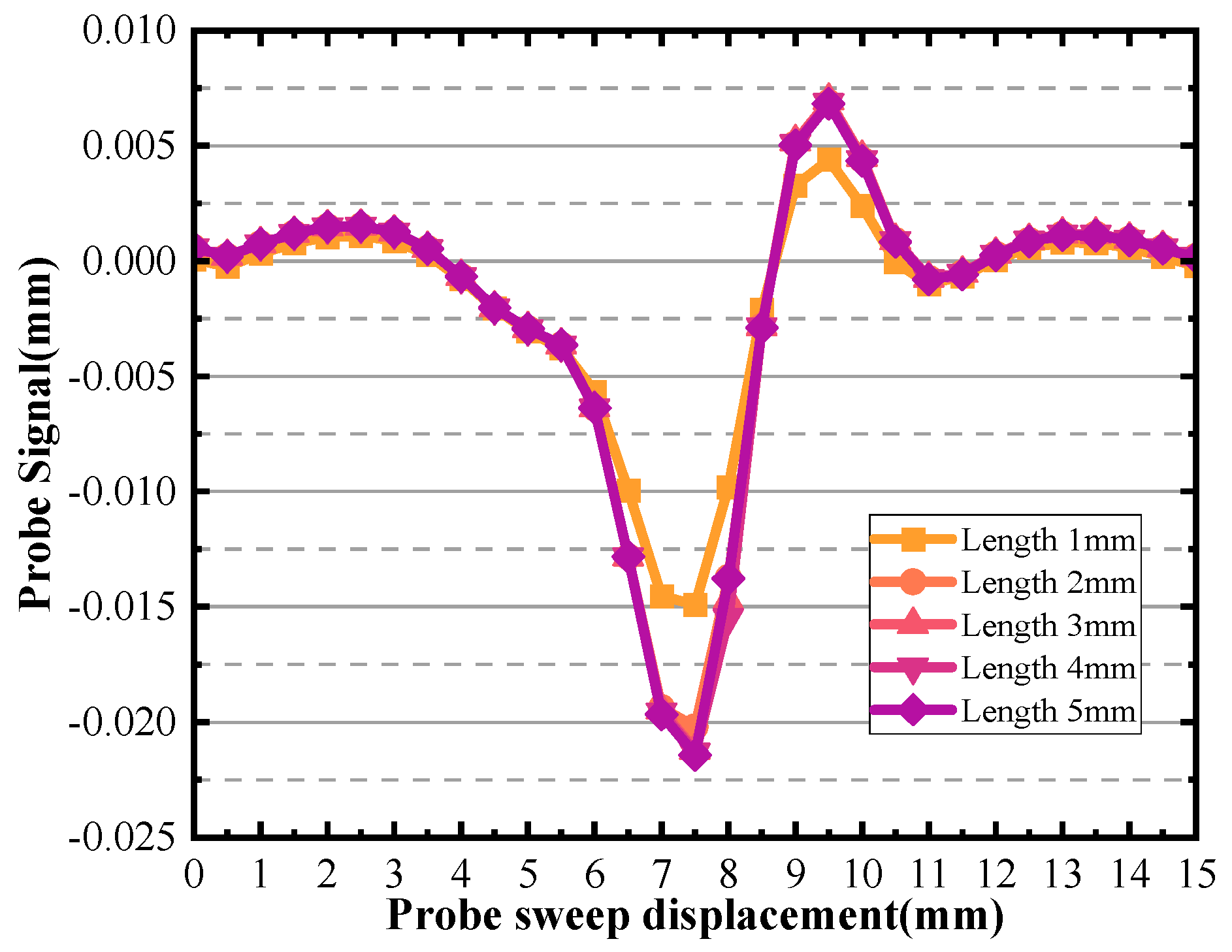
3.3. Measurement Signal and Error Analysis
4. Experimental Results and Analysis
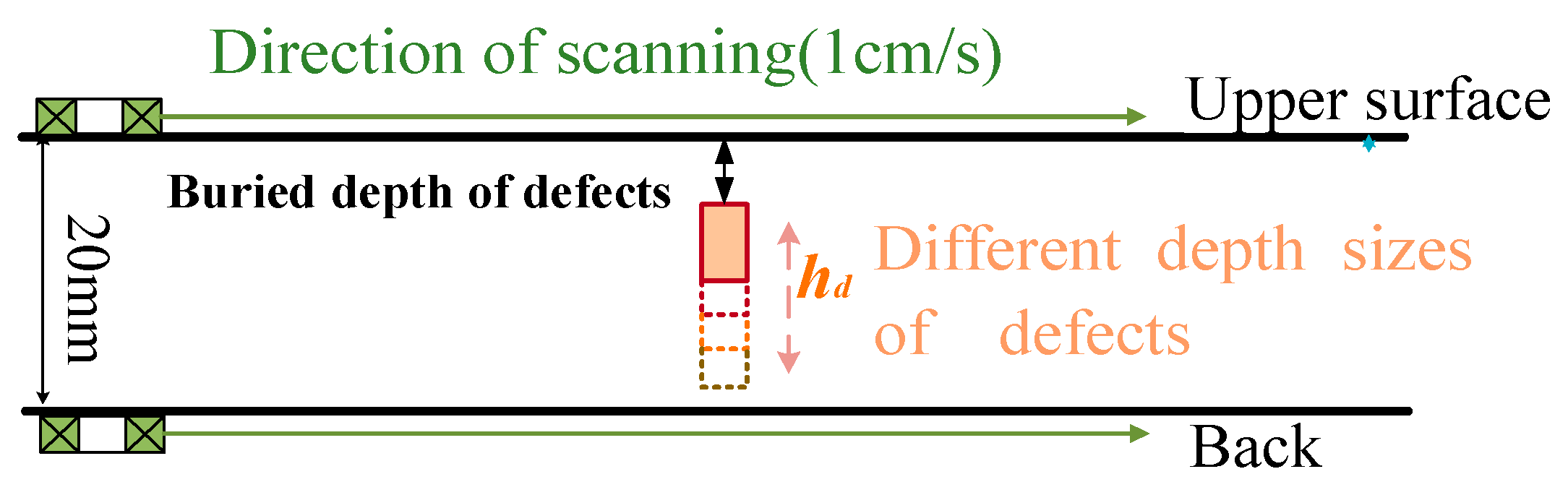
4.1. The Testing System and Specimen
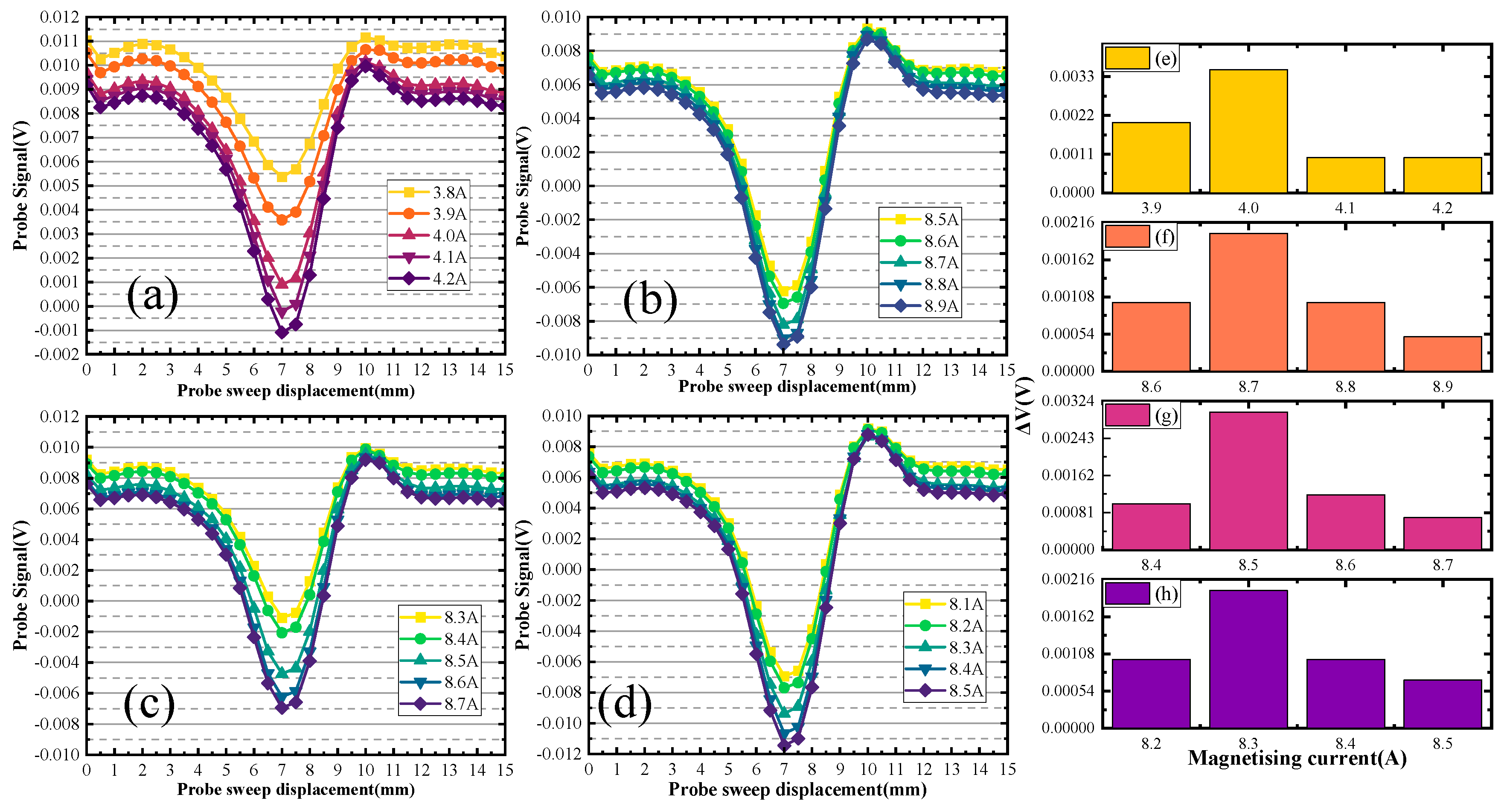
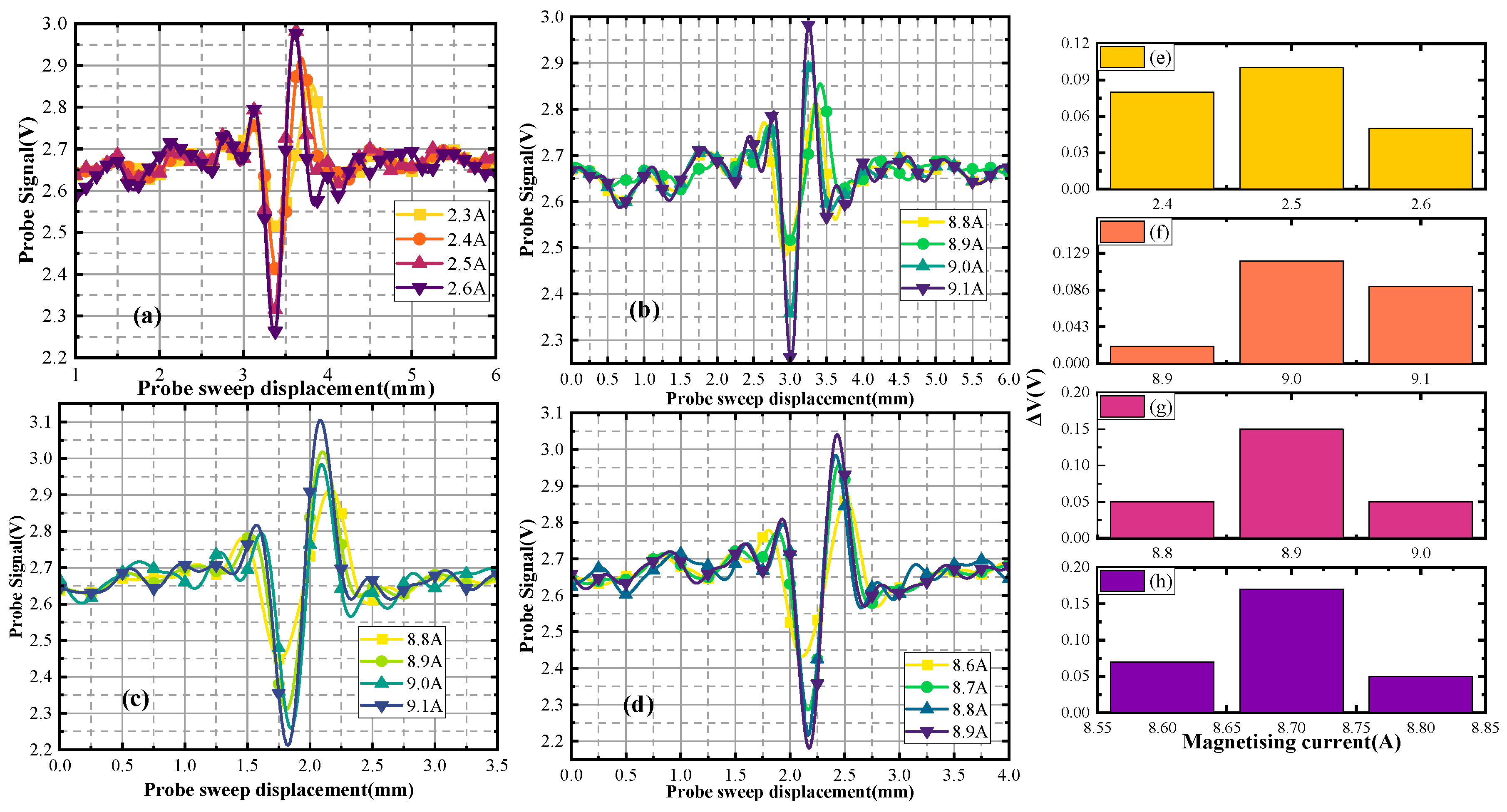
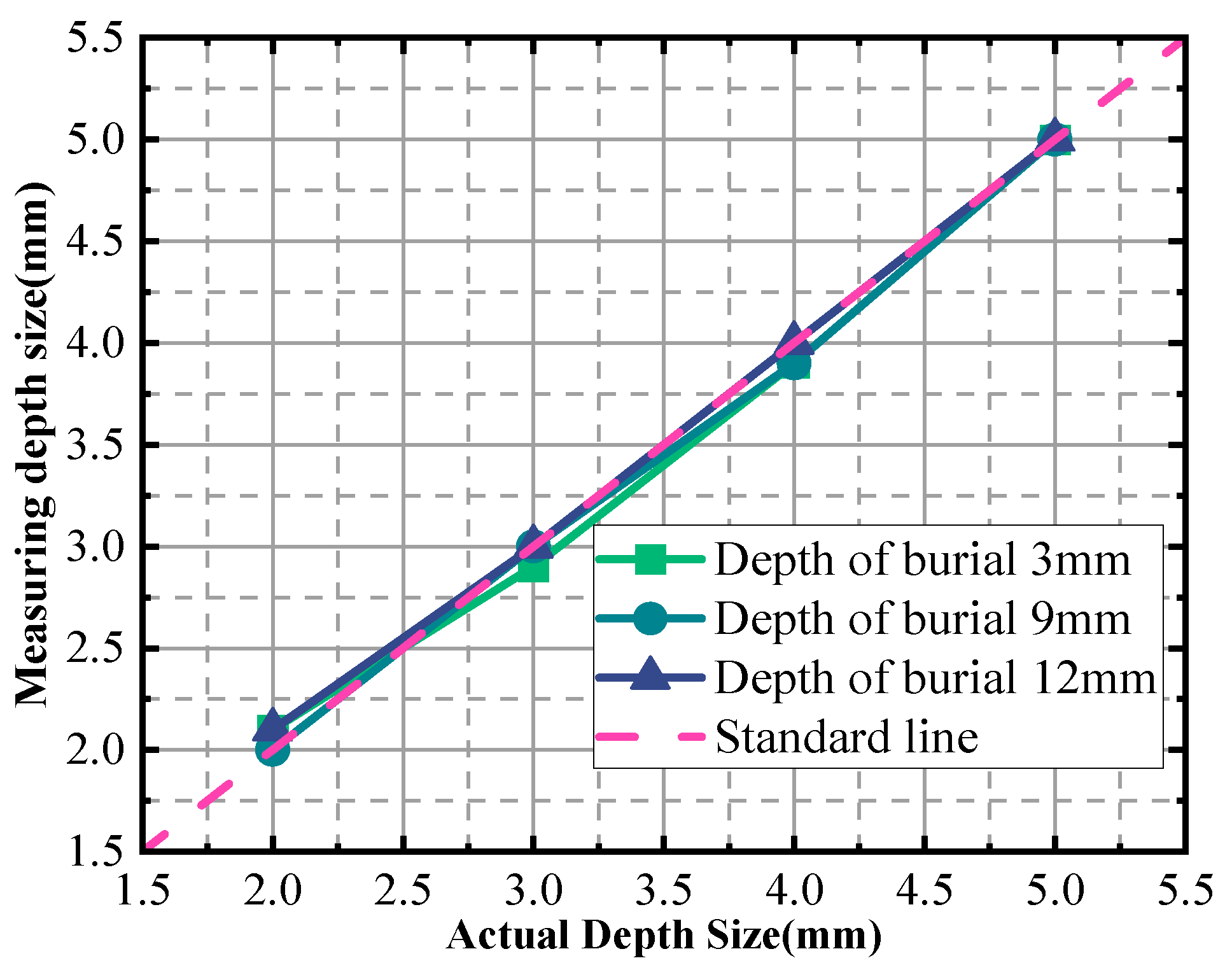
4.2. Experimental Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sun, F.; Fan, M.; Cao, B.; Ye, B.; Lu, G.; Li, W.; Tian, G. Cross-Correlation Inspired Residual Network for Pulsed Eddy Current Imaging and Detecting of Subsurface Defects. IEEE Trans. Ind. Electron. 2023, 70, 12860–12871. [Google Scholar] [CrossRef]
- Xu, Z.; Wu, J.; Liu, Z.; Xia, H.; Zhu, J. Detection, distinction, and quantification of pipeline surface and subsurface defects by DC magnetization scanning induction thermography. NDT E Int. 2023, 135, 102786. [Google Scholar] [CrossRef]
- Chen, S.; Wang, H.; Jiang, Y.; Zheng, K.; Guo, S. Wall thickness measurement and defect detection in ductile iron pipe structures using laser ultrasonic and improved variational mode decomposition. NDT E Int. 2023, 134, 102767. [Google Scholar] [CrossRef]
- Simm, A.; Theodoulidis, T.; Poulakis, N.; Tian, G.Y. Investigation of the magnetic field response from eddy current inspection of defects. Int. J. Adv. Manuf. Technol. 2011, 54, 223–230. [Google Scholar] [CrossRef]
- Luo, Q.; Shi, Y.; Wang, Z.; Zhang, W.; Li, Y. A study of applying pulsed remote field eddy current in ferromagnetic pipes testing. Sensors 2017, 17, 1038. [Google Scholar] [CrossRef] [PubMed]
- Gao, B.; Li, X.; Woo, W.L.; Yun Tian, G. Quantitative validation of Eddy current stimulated thermal features on surface crack. NDT E Int. 2017, 85, 1–12. [Google Scholar] [CrossRef]
- Sun, Y.; Liu, S.; Deng, Z.; Gu, M.; Liu, C.; He, L.; Kang, Y. New Discoveries on Electromagnetic Action and Signal Presentation in Magnetic Flux Leakage Testing. J. Nondestruct. Eval. 2019, 38, 93. [Google Scholar] [CrossRef]
- Wen, D.; Fan, M.; Cao, B.; Ye, B. Adjusting LOI for Enhancement of Pulsed Eddy Current Thickness Measurement. IEEE Trans. Instrum. Meas. 2019, 69, 521–527. [Google Scholar] [CrossRef]
- Fang, Y.; Qu, Y.; Zeng, X.; Chen, H.; Xie, S.; Wan, Q.; Uchimoto, T.; Chen, Z. Distinguishing evaluation of plastic deformation and fatigue damage using pulsed eddy current testing. NDT E Int. 2023, 140, 102972. [Google Scholar] [CrossRef]
- Xie, S.; Lu, G.; Zhang, L.; Chen, Z.; Wan, Q.; Uchimoto, T.; Takagi, T. Quantitative evaluation of deep-shallow compound defects using frequency-band-selecting pulsed eddy current testing. NDT E Int. 2023, 133, 102750. [Google Scholar] [CrossRef]
- Xie, S.; Yang, S.; Tian, M.; Zhao, R.; Chen, Z.; Zheng, Y.; Uchimoto, T.; Takagi, T. A hybrid nondestructive testing method of pulsed eddy current testing and electromagnetic acoustic transducer techniques based on wavelet analysis. NDT E Int. 2023, 138, 102900. [Google Scholar] [CrossRef]
- Li, E.; Wu, J.; Zhu, J.; Kang, Y. Quantitative Evaluation of Buried Defects in Ferromagnetic Steels Using DC Magnetization Based Eddy Current Array Testing. IEEE Trans. Magn. 2020, 56, 6200811. [Google Scholar] [CrossRef]
- Li, S.; Bai, L.; Zhang, X.; Tian, L.; Zhang, J.; Liu, Z.; Chen, C. A defect opening profile reconstruction method based on multidirectional magnetic flux leakage detection. Meas. J. Int. Meas. Confed. 2024, 233, 114701. [Google Scholar] [CrossRef]
- Sun, Y.; Feng, B.; Liu, S.; Ye, Z.; Chen, S.; Kang, Y. A Methodology for Identifying Defects in the Magnetic Flux Leakage Method and Suggestions for Standard Specimens. J. Nondestruct. Eval. 2015, 34, 20. [Google Scholar] [CrossRef]
- Ma, C.; Liu, Y.; Shen, C. Phase-Extraction-Based MFL Testing for Subsurface Defect in Ferromagnetic Steel Plate. Sensors 2022, 22, 3322. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Chen, G.; Yin, X.; Li, X.; Liu, J.; Zhao, J.; Zhao, J. Visual and intelligent identification methods for defects in underwater structure using alternating current field measurement technique. IEEE Trans. Ind. Inform. 2021, 18, 3853–3862. [Google Scholar]
- Zhao, J.; Li, W.; Yin, X.; Li, X.; Zhao, J.; Fan, M. Novel phase reversal feature for inspection of cracks using multi-frequency alternating current field measurement technique. Mech. Syst. Signal Process. 2023, 186, 109857. [Google Scholar]
- Chang, J.; Chu, Z.; Gao, X.; Soldatov, A.I.; Dong, S. A magnetoelectric-ultrasonic multimodal system for synchronous NDE of surface and internal defects in metal. Mech. Syst. Signal Process. 2022, 183, 109667. [Google Scholar] [CrossRef]
- Liu, B.; Liang, Y.; He, L.; Lian, Z.; Geng, H.; Yang, L. Quantitative study on the propagation characteristics of MFL signals of outer surface defects in long-distance oil and gas pipelines. NDT E Int. 2023, 137, 102861. [Google Scholar] [CrossRef]
- John, A.F.; Bai, L.; Cheng, Y.; Yu, H. A Heuristic Algorithm for the Reconstruction and Extraction of Defect Shape Features in Magnetic Flux Leakage Testing. IEEE Trans. Instrum. Meas. 2020, 69, 9062–9071. [Google Scholar] [CrossRef]
- Gao, P.; Geng, H.; Yang, L.; Su, Y. Research on the Forward Solving Method of Defect Leakage Signal Based on the Non-Uniform Magnetic Charge Model. Sensors 2023, 23, 6221. [Google Scholar] [CrossRef] [PubMed]
- Liu, B.; Luo, N.; Feng, G. Quantitative study on mfl signal of pipeline composite defect based on improved magnetic charge model. Sensors 2021, 21, 3412. [Google Scholar] [CrossRef] [PubMed]
- Shi, P.; Zhang, P.; Hao, S.; Wang, W.; Gou, X. Classification and evaluation for nearside/backside defect via magnetic flux leakage: A dual probe design with SVM and PSO intelligence algorithms. NDT E Int. 2024, 144, 103100. [Google Scholar] [CrossRef]
- D’Angelo, G.; Laracca, M.; Rampone, S.; Betta, G. Fast Eddy Current Testing Defect Classification Using Lissajous Figures. IEEE Trans. Instrum. Meas. 2018, 67, 821–830. [Google Scholar] [CrossRef]
- Chen, T.; Tian, G.Y.; Sophian, A.; Que, P.W. Feature extraction and selection for defect classification of pulsed eddy current NDT. NDT E Int. 2008, 41, 467–476. [Google Scholar] [CrossRef]
- He, Y.; Luo, F.; Pan, M.; Hu, X.; Gao, J.; Liu, B. Defect classification based on rectangular pulsed eddy current sensor in different directions. Sens. Actuators A Phys. 2010, 157, 26–31. [Google Scholar] [CrossRef]
- Yao, W.; Yang, Y.; Jiang, F.; Di, X.; Tao, L.; Geng, L. Quantitative evaluation of defects based on the characteristics of the induced eddy current field. Insight-Non-Destr. Test. Cond. Monit. 2022, 64, 201–205. [Google Scholar] [CrossRef]
- Li, C.; Chen, C.; Liao, K. A quantitative study of signal characteristics of non-contact pipeline magnetic testing. Insight-Non-Destr. Test. Cond. Monit. 2015, 57, 324–330. [Google Scholar] [CrossRef]
- Witek, M. Structural integrity of steel pipeline with clusters of corrosion defects. Materials 2021, 14, 852. [Google Scholar] [CrossRef]
- Yang, Y.; Liu, X.; Yang, H.; Fang, W.; Chen, P.; Li, R.; Gao, H.; Zhang, H. A Semi Empirical Regression Model for Critical Dent Depth of Externally Corroded X65 Gas Pipeline. Materials 2022, 15, 5492. [Google Scholar] [CrossRef]
- Alimey, F.J.; Yu, H.; Bai, L.; Cheng, Y.; Wang, Y. Gradient Feature Extract for the Quantification of Complex Defects Using Topographic Primal Sketch in Magnetic Flux Leakage. J. Nondestruct. Eval. Diagn. Progn. Eng. Syst. 2020, 3, 011001. [Google Scholar] [CrossRef]
- Yuan, J.; Qiao, M.; Hu, C.; Cheng, Y.; Wang, Z.; Zheng, D. A classification and quantitative assessment method for internal and external surface defects in pipelines based on ASTC-Net. Adv. Eng. Inform. 2024, 61, 102492. [Google Scholar] [CrossRef]
- Tian, G.; Yang, C.; Lu, X.; Wang, Z.; Liang, Z.; Li, X. Inductance-to-digital converters (LDC) based integrative multi-parameter eddy current testing sensors for NDT & E. NDT E Int. 2023, 138, 102888. [Google Scholar] [CrossRef]
- Li, E.; Chen, Y.; Chen, X.; Wu, J. Defect width assessment based on the near-field magnetic flux leakage method. Sensors 2021, 21, 5424. [Google Scholar] [CrossRef] [PubMed]
- Yang, Z.; Yang, J.; Cao, H.; Sun, H.; Zhao, Y.; Zhang, B.; Meng, C. Quantitative Detection of Tank Floor Defects by Pseudo-Color Imaging of Three-Dimensional Magnetic Flux Leakage Signals. Sensors 2023, 23, 2691. [Google Scholar] [CrossRef] [PubMed]
- Nafiah, F.; Sophian, A.; Khan, M.R.; Zainal Abidin, I.M. Quantitative evaluation of crack depths and angles for pulsed eddy current non-destructive testing. NDT E Int. 2019, 102, 180–188. [Google Scholar] [CrossRef]
- Xu, P.; Zhu, C.L.; Zeng, H.M.; Wang, P. Rail crack detection and evaluation at high speed based on differential ECT system. Meas. J. Int. Meas. Confed. 2020, 166, 108152. [Google Scholar] [CrossRef]
- Long, Y.; Huang, S.; Peng, L.; Wang, S.; Zhao, W. A characteristic approximation approach to defect opening profile recognition in magnetic flux leakage detection. IEEE Trans. Instrum. Meas. 2021, 70, 6003912. [Google Scholar] [CrossRef]
- Cheng, Y.; Wang, Y.; Yu, H.; Zhang, Y.; Zhang, J.; Yang, Q.; Sheng, H.; Bai, L. Solenoid model for visualizing magnetic flux leakage testing of complex defects. Ndt E Int. 2018, 100, 166–174. [Google Scholar] [CrossRef]
- Deng, Z.; Yuan, Z.; Tu, J.; Chen, L.; Song, X.; Kang, Y. A Thickness Reduction Detection Method Based on Magnetic Permeability Perturbation under DC Magnetization for Ferromagnetic Components. IEEE Trans. Instrum. Meas. 2022, 71, 6006713. [Google Scholar] [CrossRef]
- Sun, Y.; Kang, Y. The feasibility of MFL inspection for omni-directional defects under a unidirectional magnetization. Int. J. Appl. Electromagn. Mech. 2010, 33, 919–925. [Google Scholar] [CrossRef]
- Pham, H.Q.; Le, V.S.; Vu, M.H.; Doan, D.T.; Tran, Q.H. Design of a lightweight magnetizer to enable a portable circumferential magnetic flux leakage detection system. Rev. Sci. Instrum. 2019, 90, 074705. [Google Scholar] [CrossRef]
- Deng, Z.; Li, T.; Zhang, J.; Song, X.; Kang, Y. A magnetic permeability perturbation testing methodology and experimental research for deeply buried defect in ferromagnetic materials. NDT E Int. 2022, 131, 102694. [Google Scholar] [CrossRef]
- Deng, Z.; Lian, G.; Tu, J.; Feng, B.; Song, X.; Kang, Y. Magnetic Permeability Perturbation Testing Based on Unsaturated DC Magnetization for Buried Defect of Ferromagnetic Materials. J. Nondestruct. Eval. 2022, 41, 76. [Google Scholar] [CrossRef]
- Deng, Z.; Kang, Y.; Zhang, J.; Song, K. Multi-source effect in magnetizing-based eddy current testing sensor for surface crack in ferromagnetic materials. Sens. Actuators A Phys. 2018, 271, 24–36. [Google Scholar] [CrossRef]
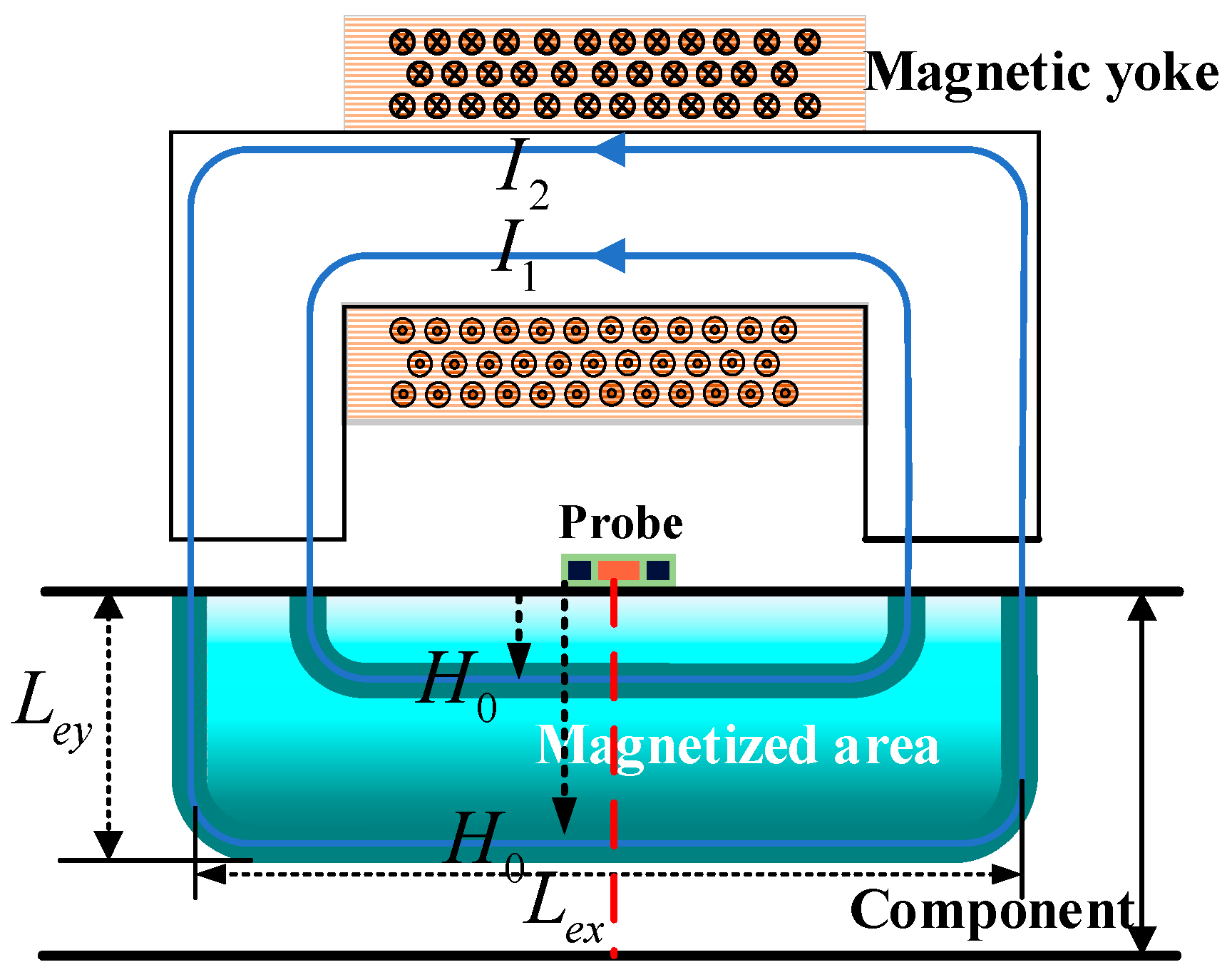











| Simulation Module | AC/DC |
|---|---|
| Magnetizing coil | 1000 turns. |
| Probe (the size parameters of the excitation coil and the detection coil are the same) | 100 turns, Frequency = 100 kHz, Voltage = 1 V, Inner diameter 2.5 mm, Outer diameter 4 mm. |
| Specimen | = 200 mm, = 100 mm, = 20 mm. |
| Magnetic yoke | = 20 mm, = 50 mm, = 80 mm, = 100 mm. |
| H (A/m) | B (T) |
|---|---|
| 0 | 0 |
| 245.8 | 0.089 |
| 414.6 | 0.185 |
| 550.5 | 0.287 |
| 673.6 | 0.402 |
| 818.6 | 0.571 |
| 996.4 | 0.748 |
| 1239.6 | 0.897 |
| 1723.8 | 1.091 |
| 2375 | 1.259 |
| 3078.8 | 1.378 |
| 4245.2 | 1.497 |
| 6495.1 | 1.632 |
| 9429.7 | 1.747 |
| 11,910.6 | 1.813 |
| 16,018.6 | 1.866 |
| 19,201.7 | 1.87 |
| Actual Size of Defect | Depth of Burial 2 mm | Depth of Burial 5 mm | Depth of Burial 10 mm | Depth of Burial 12 mm | Maximum Relative Error |
|---|---|---|---|---|---|
| 2.0 mm | 1.9 mm | 1.9 mm | 2.0 mm | 2.0 mm | 5% |
| 3.0 mm | 2.9 mm | 2.9 mm | 3.0 mm | 2.9 mm | 3.3% |
| 4.0 mm | 3.7 mm | 4.0 mm | 4.1 mm | 3.9 mm | 7.5% |
| Actual Size of Defect | Depth of Burial 3 mm | Depth of Burial 9 mm | Depth of Burial 12 mm | Maximum Relative Error |
|---|---|---|---|---|
| 2.0 mm | 2.1 mm | 2.0 mm | 2.1 mm | 5% |
| 3.0 mm | 2.9 mm | 3.0 mm | 3.0 mm | 3.3% |
| 4.0 mm | 3.9 mm | 3.9 mm | 4.0 mm | 2.5% |
| 5.0 mm | 5.0 mm | 5.0 mm | 5.0 mm | 0% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, Z.; Qian, D.; Hong, H.; Song, X.; Kang, Y. Evaluation of Depth Size Based on Layered Magnetization by Double-Sided Scanning for Internal Defects. Sensors 2024, 24, 3689. https://doi.org/10.3390/s24113689
Deng Z, Qian D, Hong H, Song X, Kang Y. Evaluation of Depth Size Based on Layered Magnetization by Double-Sided Scanning for Internal Defects. Sensors. 2024; 24(11):3689. https://doi.org/10.3390/s24113689
Chicago/Turabian StyleDeng, Zhiyang, Dingkun Qian, Haifei Hong, Xiaochun Song, and Yihua Kang. 2024. "Evaluation of Depth Size Based on Layered Magnetization by Double-Sided Scanning for Internal Defects" Sensors 24, no. 11: 3689. https://doi.org/10.3390/s24113689
APA StyleDeng, Z., Qian, D., Hong, H., Song, X., & Kang, Y. (2024). Evaluation of Depth Size Based on Layered Magnetization by Double-Sided Scanning for Internal Defects. Sensors, 24(11), 3689. https://doi.org/10.3390/s24113689






