Technique-Dependent Relationship between Local Ski Bending Curvature, Roll Angle and Radial Force in Alpine Skiing
Abstract
1. Introduction
1.1. Design and Material Properties of Alpine Skis
1.2. Skiing Techniques
1.3. The Science of Ski Turns
1.4. State of Current Knowledge and Research Gap
1.5. Aim and Research Question
2. Materials and Methods
2.1. Experimental Setup and Measurement Equipment
2.2. Data Processing and Statistical Analysis
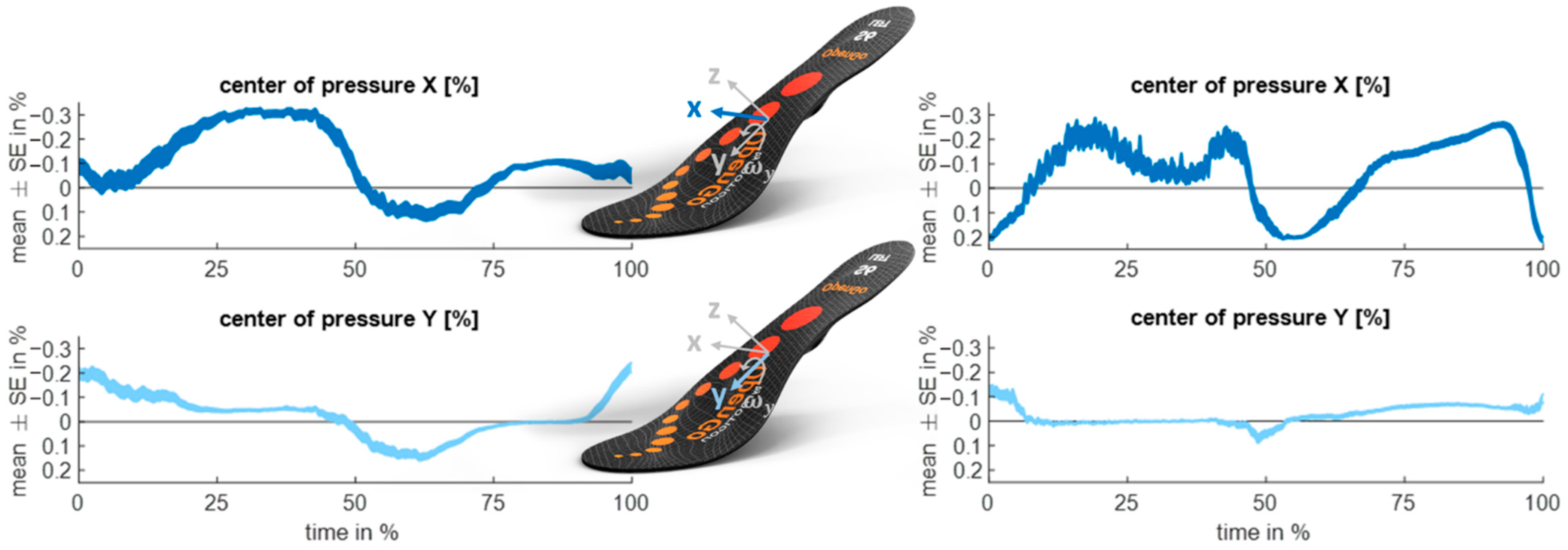
3. Results
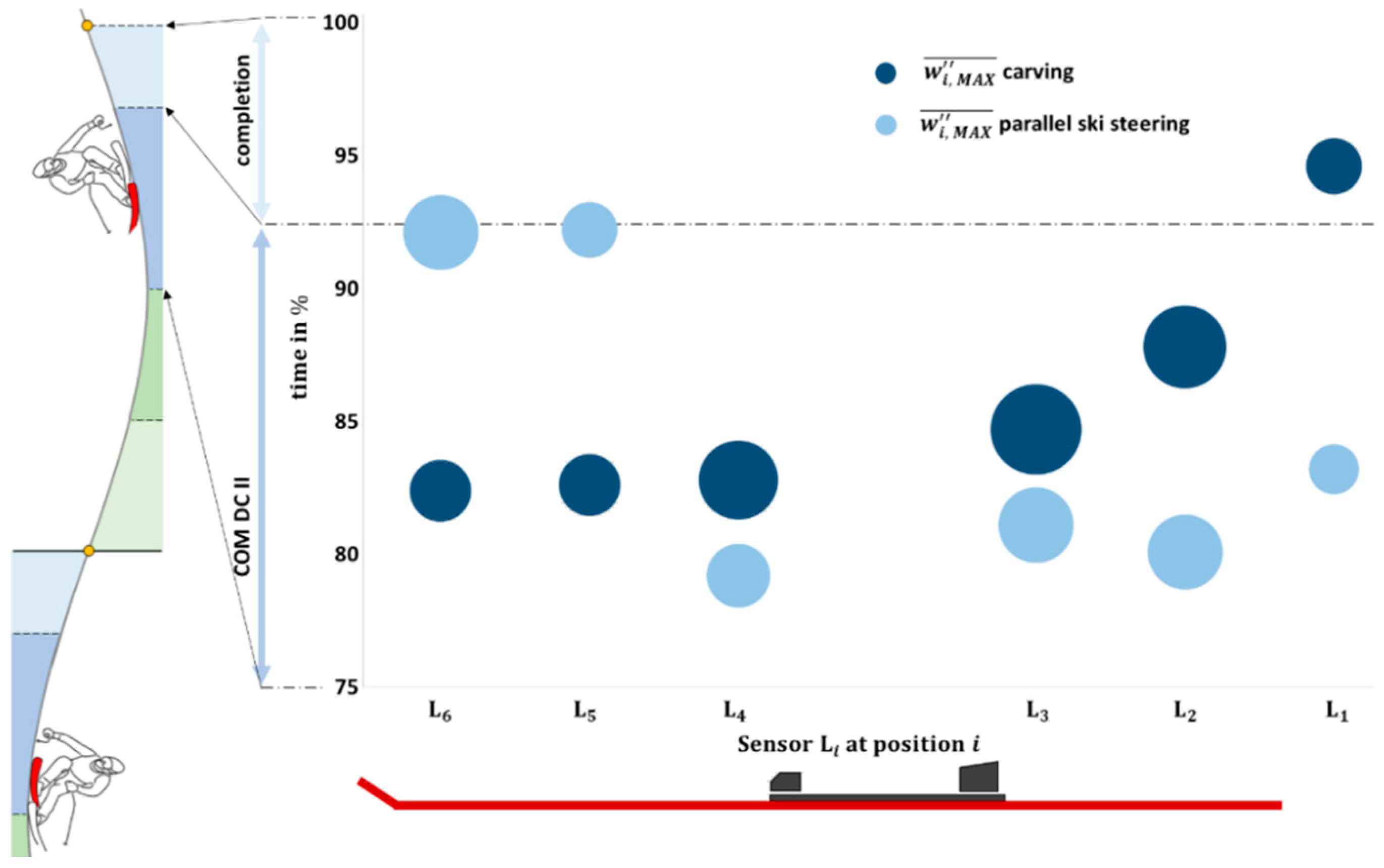
3.1. Descriptive Report
3.2. Correlation between Curvature Sensors
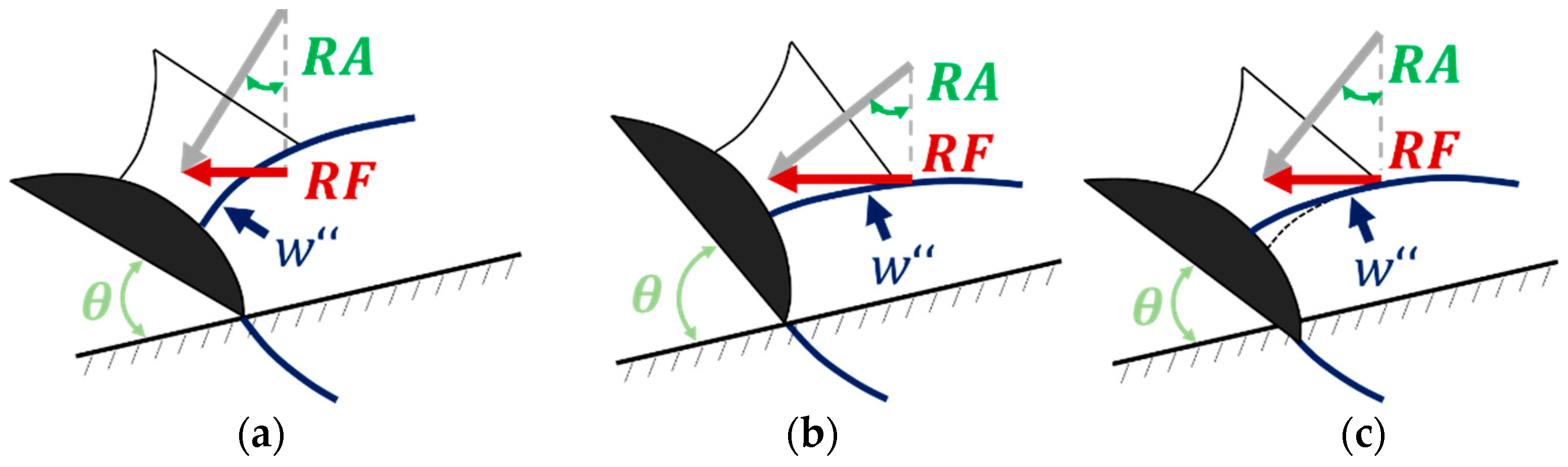
3.3. Correlation between RF, RA and Segmental w″
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Abbreviation | Definition |
| sidecut radius | |
| edge angle | |
| edge curvature radius | |
| curvature | |
| radial force | |
| roll angle | |
| sensor 1 to sensor 6 | |
| TSPs | turn switch points |
| IMU | inertial measurement unit |
| rotational velocity around y-axis | |
| time | |
| output voltage | |
| calibration values (gradient and absolute value) for sensor position i | |
| DAQ | data acquisition device |
| mean roll angle progression over time-normalized data of left-right turn combination | |
| mean radial force progression over time-normalized data of left-right turn combination | |
| mean segmental curvature at sensor position over time-normalized data of left-right turn combination | |
| maximum roll angle of | |
| minimum roll angle of | |
| maximum radial force of | |
| maximum curvature at sensor position of | |
| initiation | turn phase 1: inner ski 0–12.5%; outer ski 50–62.5% of time-normalized data |
| COM DC I | turn phase 2 (center of mass direction change I): inner ski 12.5–25%; outer ski 62.5–75% of time-normalized data |
| COM DC II | turn phase 3 (center of mass direction change II): inner ski 25–42.5%; outer ski 75–92.5% of time-normalized data |
| completion | turn phase 4: inner ski 42.5–50%; outer ski 92.5–100% of time-normalized data |
| Pearson’s correlation coefficient | |
| local translational velocity at position i | |
| local angle of attack at position i |
Appendix A
Appendix B
 | L1 | L2 | L3 | ||||||||||
| Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | ||
| L1 | Init | 1 | |||||||||||
| COM DC I | 0.64 | 1 | |||||||||||
| COM DC II | −0.21 | 0.08 | 1 | ||||||||||
| Comp | 0.49 | 0.18 | 0.37 | 1 | |||||||||
| L2 | Init | 0.24 | 0.25 | −0.17 | −0.06 | 1 | |||||||
| COM DC I | −0.66 | −0.07 | 0.25 | −0.48 | 0.37 | 1 | |||||||
| COM DC II | −0.77 | −0.35 | 0.56 | −0.20 | 0.17 | 0.81 | 1 | ||||||
| Comp | −0.40 | −0.34 | 0.39 | 0.41 | 0.23 | 0.41 | 0.67 | 1 | |||||
| L3 | Init | −0.21 | −0.11 | −0.10 | −0.19 | 0.79 | 0.62 | 0.47 | 0.47 | 1 | |||
| COM DC I | −0.67 | −0.22 | 0.28 | −0.36 | 0.31 | 0.94 | 0.81 | 0.51 | 0.68 | 1 | |||
| COM DC II | −0.74 | −0.37 | 0.48 | −0.18 | 0.20 | 0.79 | 0.98 | 0.71 | 0.53 | 0.83 | 1 | ||
| Comp | −0.57 | −0.48 | 0.31 | 0.15 | 0.14 | 0.49 | 0.72 | 0.89 | 0.44 | 0.59 | 0.79 | 1 | |
| L4 | Init | 0.10 | 0.04 | −0.36 | −0.27 | 0.01 | −0.09 | −0.34 | −0.39 | −0.09 | −0.10 | −0.33 | −0.28 |
| COM DC I | 0.20 | 0.22 | −0.07 | −0.21 | −0.01 | −0.10 | −0.17 | −0.50 | −0.08 | −0.15 | −0.18 | −0.42 | |
| COM DC II | −0.46 | −0.11 | 0.28 | 0.00 | 0.03 | 0.48 | 0.54 | 0.43 | 0.19 | 0.47 | 0.53 | 0.38 | |
| Comp | −0.07 | −0.14 | −0.02 | 0.04 | −0.31 | −0.18 | −0.17 | −0.11 | −0.25 | −0.15 | −0.16 | 0.02 | |
| L5 | Init | 0.19 | 0.14 | −0.28 | −0.18 | −0.06 | −0.17 | −0.40 | −0.43 | −0.20 | −0.20 | −0.41 | −0.35 |
| COM DC I | 0.14 | 0.20 | 0.06 | −0.22 | −0.07 | −0.07 | −0.10 | −0.47 | −0.15 | −0.14 | −0.13 | −0.36 | |
| COM DC II | −0.48 | −0.24 | 0.33 | 0.10 | −0.11 | 0.39 | 0.51 | 0.52 | 0.07 | 0.41 | 0.51 | 0.53 | |
| Comp | −0.09 | −0.14 | 0.11 | 0.16 | −0.36 | −0.18 | −0.11 | 0.02 | −0.29 | −0.13 | −0.10 | 0.13 | |
| L6 | Init | 0.20 | 0.21 | −0.24 | −0.24 | −0.07 | −0.15 | −0.39 | −0.50 | −0.21 | −0.19 | −0.40 | −0.39 |
| COM DC I | 0.06 | 0.15 | 0.12 | −0.33 | −0.16 | −0.04 | −0.08 | −0.53 | −0.23 | −0.11 | −0.12 | −0.38 | |
| COM DC II | −0.25 | −0.22 | 0.24 | 0.18 | −0.15 | 0.13 | 0.23 | 0.33 | −0.03 | 0.21 | 0.24 | 0.36 | |
| Comp | 0.07 | −0.02 | 0.11 | 0.22 | −0.37 | −0.29 | −0.25 | −0.09 | −0.37 | −0.24 | −0.25 | −0.03 | |
| L4 | L5 | L6 | |||||||||||
| Init | COM DC I | COM DC II | C | Init | COM DC I | COM DC II | C | Init | COM DC I | COM DC II | C | ||
| L4 | Init | 1 | |||||||||||
| COM DC I | −0.02 | 1 | |||||||||||
| COM DC II | −0.25 | 0.08 | 1 | ||||||||||
| Comp | 0.51 | 0.16 | 0.17 | 1 | |||||||||
| L5 | Init | 0.96 | 0.06 | −0.25 | 0.56 | 1 | |||||||
| COM DC I | 0.01 | 0.94 | 0.12 | 0.22 | 0.14 | 1 | |||||||
| COM DC II | −0.14 | −0.05 | 0.90 | 0.31 | −0.12 | 0.07 | 1 | ||||||
| Comp | 0.46 | 0.07 | 0.20 | 0.96 | 0.53 | 0.19 | 0.42 | 1 | |||||
| L6 | Init | 0.90 | 0.16 | −0.28 | 0.51 | 0.97 | 0.25 | −0.17 | 0.49 | 1 | |||
| COM DC I | 0.03 | 0.80 | 0.11 | 0.20 | 0.15 | 0.93 | 0.09 | 0.19 | 0.28 | 1 | |||
| COM DC II | 0.02 | −0.08 | 0.70 | 0.39 | 0.07 | 0.07 | 0.87 | 0.52 | 0.02 | 0.16 | 1 | ||
| Comp | 0.49 | 0.02 | 0.13 | 0.87 | 0.58 | 0.16 | 0.35 | 0.93 | 0.54 | 0.23 | 0.60 | 1 | |
 | L1 | L2 | L3 | ||||||||||
| Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | ||
| L1 | Init | 1 | |||||||||||
| COM DC I | 0.74 | 1 | |||||||||||
| COM DC II | 0.41 | 0.58 | 1 | ||||||||||
| Comp | 0.37 | 0.38 | 0.38 | 1 | |||||||||
| L2 | Init | 0.48 | 0.29 | 0.38 | −0.11 | 1 | |||||||
| COM DC I | −0.23 | 0.10 | 0.30 | −0.37 | 0.55 | 1 | |||||||
| COM DC II | −0.44 | −0.20 | 0.30 | −0.38 | 0.48 | 0.87 | 1 | ||||||
| Comp | −0.50 | −0.22 | −0.03 | 0.07 | −0.03 | 0.33 | 0.40 | 1 | |||||
| L3 | Init | 0.15 | 0.02 | 0.33 | −0.20 | 0.88 | 0.62 | 0.68 | 0.14 | 1 | |||
| COM DC I | −0.29 | −0.08 | 0.23 | −0.36 | 0.54 | 0.93 | 0.85 | 0.34 | 0.69 | 1 | |||
| COM DC II | −0.44 | −0.28 | 0.16 | −0.39 | 0.49 | 0.80 | 0.94 | 0.38 | 0.70 | 0.84 | 1 | ||
| Comp | −0.45 | −0.21 | −0.14 | −0.10 | −0.03 | 0.26 | 0.30 | 0.93 | 0.10 | 0.30 | 0.34 | 1 | |
| L4 | Init | 0.11 | 0.10 | 0.13 | 0.08 | 0.06 | −0.17 | 0.03 | −0.06 | 0.06 | −0.19 | 0.06 | −0.05 |
| COM DC I | 0.51 | 0.21 | −0.25 | −0.03 | 0.09 | −0.44 | −0.54 | −0.55 | −0.11 | −0.41 | −0.44 | −0.41 | |
| COM DC II | −0.33 | −0.15 | −0.18 | −0.37 | 0.28 | 0.53 | 0.50 | 0.55 | 0.46 | 0.53 | 0.51 | 0.55 | |
| Comp | 0.09 | 0.09 | 0.28 | 0.08 | 0.07 | −0.13 | 0.07 | 0.05 | 0.12 | −0.09 | 0.10 | 0.01 | |
| L5 | Init | 0.23 | 0.13 | 0.15 | 0.16 | 0.03 | −0.30 | −0.11 | −0.20 | −0.02 | −0.35 | −0.06 | −0.21 |
| COM DC I | 0.54 | 0.22 | −0.14 | 0.09 | 0.10 | −0.51 | −0.53 | −0.52 | −0.10 | −0.52 | −0.45 | −0.46 | |
| COM DC II | −0.27 | −0.09 | −0.10 | −0.34 | 0.35 | 0.57 | 0.54 | 0.51 | 0.51 | 0.55 | 0.57 | 0.48 | |
| Comp | 0.25 | 0.12 | 0.29 | 0.18 | 0.09 | −0.26 | −0.06 | −0.18 | 0.09 | −0.22 | 0.00 | −0.23 | |
| L6 | Init | 0.35 | 0.11 | −0.03 | 0.30 | −0.16 | −0.60 | −0.46 | −0.37 | −0.27 | −0.63 | −0.37 | −0.37 |
| COM DC I | 0.52 | 0.19 | −0.15 | 0.20 | 0.02 | −0.61 | −0.60 | −0.52 | −0.17 | −0.63 | −0.51 | −0.48 | |
| COM DC II | −0.26 | −0.09 | −0.12 | −0.32 | 0.32 | 0.54 | 0.50 | 0.46 | 0.47 | 0.55 | 0.56 | 0.44 | |
| Comp | 0.07 | −0.12 | 0.06 | −0.02 | −0.02 | −0.31 | −0.06 | −0.24 | 0.03 | −0.25 | 0.04 | −0.22 | |
| L4 | L5 | L6 | |||||||||||
| Init | COM DC I | COM DC II | C | Init | COM DC I | COM DC II | C | Init | COM DC I | COM DC II | C | ||
| L4 | Init | 1 | |||||||||||
| COM DC I | 0.05 | 1 | |||||||||||
| COM DC II | −0.11 | −0.20 | 1 | ||||||||||
| Comp | 0.50 | 0.02 | 0.19 | 1 | |||||||||
| L5 | Init | 0.90 | 0.25 | −0.27 | 0.45 | 1 | |||||||
| COM DC I | 0.11 | 0.94 | −0.21 | 0.15 | 0.36 | 1 | |||||||
| COM DC II | −0.13 | −0.11 | 0.96 | 0.23 | −0.21 | −0.09 | 1 | ||||||
| Comp | 0.46 | 0.23 | −0.03 | 0.92 | 0.53 | 0.38 | 0.08 | 1 | |||||
| L6 | Init | 0.70 | 0.44 | −0.42 | 0.31 | 0.89 | 0.55 | −0.36 | 0.47 | 1 | |||
| COM DC I | 0.11 | 0.85 | −0.24 | 0.18 | 0.35 | 0.96 | −0.12 | 0.41 | 0.60 | 1 | |||
| COM DC II | −0.18 | −0.08 | 0.92 | 0.23 | −0.24 | −0.07 | 0.98 | 0.10 | −0.34 | −0.09 | 1 | ||
| Comp | 0.34 | 0.23 | −0.04 | 0.75 | 0.42 | 0.34 | 0.06 | 0.88 | 0.40 | 0.37 | 0.10 | 1 | |
 | L1 | L2 | L3 | ||||||||||
| Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | ||
| L1 | Init | 1 | |||||||||||
| COM DC I | 0.38 | 1 | |||||||||||
| COM DC II | −0.04 | 0.62 | 1 | ||||||||||
| Comp | −0.18 | −0.03 | 0.59 | 1 | |||||||||
| L2 | Init | 0.67 | 0.12 | −0.02 | −0.20 | 1 | |||||||
| COM DC I | 0.24 | 0.95 | 0.63 | −0.06 | 0.10 | 1 | |||||||
| COM DC II | −0.15 | 0.52 | 0.93 | 0.48 | 0.03 | 0.60 | 1 | ||||||
| Comp | −0.15 | −0.22 | 0.35 | 0.89 | −0.01 | −0.22 | 0.30 | 1 | |||||
| L3 | Init | 0.35 | −0.17 | −0.05 | 0.03 | 0.77 | −0.16 | −0.02 | 0.23 | 1 | |||
| COM DC I | 0.12 | 0.73 | 0.59 | 0.12 | 0.07 | 0.81 | 0.57 | −0.02 | 0.14 | 1 | |||
| COM DC II | −0.07 | 0.38 | 0.62 | 0.28 | 0.08 | 0.43 | 0.75 | 0.19 | 0.23 | 0.69 | 1 | ||
| Comp | −0.16 | −0.31 | 0.07 | 0.68 | −0.03 | −0.30 | 0.07 | 0.83 | 0.37 | 0.07 | 0.27 | 1 | |
| L4 | Init | −0.03 | 0.39 | −0.05 | −0.36 | −0.35 | 0.43 | −0.05 | −0.50 | −0.34 | 0.42 | 0.11 | −0.24 |
| COM DC I | 0.14 | 0.66 | 0.36 | −0.13 | −0.02 | 0.67 | 0.37 | −0.33 | −0.07 | 0.70 | 0.45 | −0.16 | |
| COM DC II | −0.27 | 0.31 | 0.77 | 0.43 | −0.22 | 0.36 | 0.76 | 0.19 | −0.15 | 0.40 | 0.54 | 0.00 | |
| Comp | 0.02 | 0.04 | 0.45 | 0.71 | −0.15 | 0.00 | 0.35 | 0.60 | −0.07 | 0.05 | 0.19 | 0.44 | |
| L5 | Init | 0.12 | 0.39 | −0.12 | −0.42 | −0.24 | 0.42 | −0.11 | −0.52 | −0.31 | 0.35 | 0.05 | −0.25 |
| COM DC I | 0.18 | 0.76 | 0.44 | −0.17 | 0.06 | 0.79 | 0.46 | −0.37 | −0.07 | 0.76 | 0.49 | −0.24 | |
| COM DC II | −0.15 | 0.41 | 0.84 | 0.43 | −0.04 | 0.47 | 0.83 | 0.21 | −0.09 | 0.46 | 0.54 | −0.03 | |
| Comp | 0.07 | 0.10 | 0.59 | 0.77 | −0.03 | 0.08 | 0.49 | 0.62 | −0.04 | 0.13 | 0.27 | 0.37 | |
| L6 | Init | 0.23 | 0.35 | −0.17 | −0.44 | −0.06 | 0.38 | −0.16 | −0.50 | −0.14 | 0.34 | 0.04 | −0.17 |
| COM DC I | 0.21 | 0.82 | 0.50 | −0.20 | 0.13 | 0.85 | 0.53 | −0.38 | −0.08 | 0.75 | 0.50 | −0.31 | |
| COM DC II | −0.09 | 0.36 | 0.85 | 0.53 | 0.04 | 0.42 | 0.81 | 0.31 | 0.01 | 0.44 | 0.48 | 0.06 | |
| Comp | −0.05 | 0.05 | 0.64 | 0.84 | −0.05 | 0.05 | 0.54 | 0.66 | 0.05 | 0.18 | 0.33 | 0.45 | |
| L4 | L5 | L6 | |||||||||||
| Init | COM DC I | COM DC II | C | Init | COM DC I | COM DC II | C | Init | COM DC I | COM DC II | C | ||
| L4 | Init | 1 | |||||||||||
| COM DC I | 0.65 | 1 | |||||||||||
| COM DC II | 0.12 | 0.30 | 1 | ||||||||||
| Comp | −0.01 | 0.00 | 0.50 | 1 | |||||||||
| L5 | Init | 0.90 | 0.62 | 0.00 | −0.10 | 1 | |||||||
| COM DC I | 0.56 | 0.96 | 0.32 | −0.11 | 0.57 | 1 | |||||||
| COM DC II | −0.02 | 0.31 | 0.91 | 0.37 | −0.04 | 0.39 | 1 | ||||||
| Comp | −0.24 | −0.01 | 0.50 | 0.84 | −0.23 | −0.04 | 0.57 | 1 | |||||
| L6 | Init | 0.80 | 0.61 | −0.09 | −0.07 | 0.91 | 0.55 | −0.11 | −0.19 | 1 | |||
| COM DC I | .045 | 0.87 | 0.35 | −0.10 | 0.43 | 0.95 | 0.43 | −0.03 | 0.48 | 1 | |||
| COM DC II | −0.13 | 0.27 | 0.85 | 0.46 | −0.18 | 0.33 | 0.95 | 0.65 | −0.16 | 0.40 | 1 | ||
| Comp | −0.31 | −0.01 | 0.53 | 0.80 | −0.37 | −0.04 | 0.58 | 0.93 | −0.30 | −0.01 | 0.72 | 1 | |
 | L1 | L2 | L3 | ||||||||||
| Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | ||
| L1 | Init | 1 | |||||||||||
| COM DC I | 0.38 | 1 | |||||||||||
| COM DC II | 0.15 | 0.45 | 1 | ||||||||||
| Comp | −0.08 | −0.28 | 0.40 | 1 | |||||||||
| L2 | Init | 0.77 | 0.45 | 0.11 | −0.14 | 1 | |||||||
| COM DC I | 0.01 | 0.67 | 0.03 | −0.50 | 0.36 | 1 | |||||||
| COM DC II | −0.11 | 0.51 | 0.70 | −0.02 | 0.08 | 0.50 | 1 | ||||||
| Comp | −0.28 | −0.08 | 0.31 | 0.79 | −0.07 | −0.14 | 0.14 | 1 | |||||
| L3 | Init | 0.38 | 0.19 | 0.24 | −0.04 | 0.64 | 0.27 | 0.33 | 0.05 | 1 | |||
| COM DC I | −0.16 | 0.18 | 0.04 | −0.25 | 0.09 | 0.70 | 0.40 | −0.11 | 0.48 | 1 | |||
| COM DC II | −0.17 | 0.18 | 0.48 | −0.08 | −0.06 | 0.31 | 0.78 | −0.02 | 0.53 | 0.60 | 1 | ||
| Comp | −0.34 | −0.15 | 0.27 | 0.70 | −0.19 | −0.23 | 0.04 | 0.89 | 0.12 | −0.03 | 0.07 | 1 | |
| L4 | Init | 0.47 | 0.10 | 0.02 | −0.17 | 0.26 | −0.02 | −0.06 | −0.20 | 0.37 | 0.13 | 0.21 | −0.06 |
| COM DC I | −0.09 | 0.05 | −0.15 | −0.29 | −0.33 | 0.13 | 0.11 | −0.20 | −0.05 | 0.25 | 0.37 | −0.05 | |
| COM DC II | −0.17 | 0.37 | 0.42 | 0.05 | −0.29 | 0.18 | 0.61 | 0.16 | −0.09 | 0.06 | 0.47 | 0.13 | |
| Comp | −0.06 | −0.22 | −0.13 | 0.21 | 0.16 | −0.06 | −0.26 | 0.22 | 0.12 | −0.02 | −0.26 | 0.18 | |
| L5 | Init | 0.56 | −0.01 | −0.14 | −0.24 | 0.34 | −0.14 | −0.31 | −0.32 | 0.24 | −0.07 | −0.09 | −0.14 |
| COM DC I | 0.15 | 0.23 | −0.16 | −0.51 | 0.01 | 0.29 | 0.13 | −0.38 | 0.10 | 0.23 | 0.33 | −0.19 | |
| COM DC II | −0.07 | 0.35 | 0.49 | 0.14 | −0.24 | 0.04 | 0.53 | 0.19 | −0.16 | −0.11 | 0.35 | 0.18 | |
| Comp | −0.04 | −0.25 | −0.15 | 0.17 | 0.17 | −0.09 | −0.28 | 0.18 | 0.10 | −0.04 | −0.28 | 0.15 | |
| L6 | Init | 0.73 | 0.04 | −0.11 | −0.26 | 0.48 | −0.13 | −0.28 | −0.38 | 0.26 | −0.15 | −0.17 | −0.29 |
| COM DC I | 0.51 | 0.37 | −0.10 | −0.62 | 0.42 | 0.35 | 0.13 | −0.48 | 0.36 | 0.15 | 0.19 | −0.42 | |
| COM DC II | 0.14 | 0.13 | 0.50 | 0.29 | −0.07 | −0.25 | 0.30 | 0.22 | −0.05 | −0.32 | 0.13 | 0.16 | |
| Comp | −0.02 | −0.34 | −0.23 | 0.07 | 0.19 | −0.13 | −0.33 | 0.10 | 0.11 | −0.08 | −0.35 | 0.04 | |
| L4 | L5 | L6 | |||||||||||
| Init | COM DC I | COM DC II | C | Init | COM DC I | COM DC II | C | Init | COM DC I | COM DC II | C | ||
| L4 | Init | 1 | |||||||||||
| COM DC I | 0.51 | 1 | |||||||||||
| COM DC II | 0.08 | 0.54 | 1 | ||||||||||
| Comp | −0.15 | −0.52 | −0.57 | 1 | |||||||||
| L5 | Init | 0.76 | 0.15 | −0.24 | 0.03 | 1 | |||||||
| COM DC I | 0.39 | 0.72 | 0.26 | −0.44 | 0.40 | 1 | |||||||
| COM DC II | 0.01 | 0.33 | 0.91 | −0.49 | −0.11 | 0.24 | 1 | ||||||
| Comp | −0.15 | −0.57 | −0.63 | 0.96 | 0.14 | −0.40 | −0.51 | 1 | |||||
| L6 | Init | 0.67 | 0.05 | −0.24 | 0.06 | 0.93 | 0.32 | −0.11 | 0.16 | 1 | |||
| COM DC I | 0.30 | 0.38 | 0.09 | −0.35 | 0.34 | 0.68 | 0.04 | −0.31 | 0.47 | 1 | |||
| COM DC II | 0.02 | 0.05 | 0.65 | −0.32 | −0.04 | −0.13 | 0.80 | −0.35 | 0.05 | 0.01 | 1 | ||
| Comp | −0.15 | −0.57 | −0.63 | 0.86 | 0.11 | −0.50 | −0.55 | 0.90 | 0.21 | −0.18 | −0.24 | 1 | |
Appendix C
 | RA | RF | |||||||
| Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | ||
| RA | Init | 1 | |||||||
| COM DC I | 0.91 | 1 | |||||||
| COM DC II | 0.75 | 0.93 | 1 | ||||||
| Comp | 0.79 | 0.80 | 0.83 | 1 | |||||
| RF | Init | 0.54 | 0.32 | 0.34 | 0.41 | 1 | |||
| COM DC I | 0.87 | 0.80 | 0.71 | 0.64 | 0.67 | 1 | |||
| COM DC II | 0.75 | 0.85 | 0.83 | 0.69 | 0.41 | 0.86 | 1 | ||
| Comp | 0.64 | 0.50 | 0.48 | 0.79 | 0.40 | 0.57 | 0.55 | 1 | |
| L1 | Init | −0.57 | −0.57 | −0.42 | −0.36 | −0.26 | −0.60 | −0.54 | −0.23 |
| COM DC I | −0.50 | −0.47 | −0.26 | −0.27 | −0.07 | −0.41 | −0.36 | −0.19 | |
| COM DC II | 0.19 | 0.40 | 0.44 | 0.12 | 0.04 | 0.35 | 0.41 | −0.17 | |
| Comp | −0.20 | −0.07 | 0.06 | −0.09 | −0.17 | −0.16 | −0.11 | −0.20 | |
| L2 | Init | 0.30 | 0.20 | 0.26 | 0.35 | 0.36 | 0.23 | 0.17 | 0.32 |
| COM DC I | 0.60 | 0.55 | 0.47 | 0.43 | 0.39 | 0.67 | 0.57 | 0.41 | |
| COM DC II | 0.74 | 0.81 | 0.74 | 0.58 | 0.39 | 0.82 | 0.80 | 0.35 | |
| Comp | 0.62 | 0.67 | 0.67 | 0.61 | 0.29 | 0.61 | 0.57 | 0.41 | |
| L3 | Init | 0.64 | 0.52 | 0.47 | 0.57 | 0.42 | 0.48 | 0.40 | 0.46 |
| COM DC I | 0.72 | 0.66 | 0.52 | 0.48 | 0.36 | 0.74 | 0.62 | 0.45 | |
| COM DC II | 0.77 | 0.80 | 0.73 | 0.62 | 0.41 | 0.83 | 0.77 | 0.41 | |
| Comp | 0.67 | 0.66 | 0.59 | 0.61 | 0.28 | 0.63 | 0.51 | 0.43 | |
| L4 | Init | −0.30 | −0.51 | −0.61 | −0.53 | 0.11 | −0.24 | −0.42 | −0.26 |
| COM DC I | −0.29 | −0.28 | −0.24 | −0.26 | −0.20 | −0.28 | −0.19 | −0.21 | |
| COM DC II | 0.23 | 0.32 | 0.34 | 0.16 | 0.19 | 0.32 | 0.26 | 0.05 | |
| Comp | −0.38 | −0.36 | −0.40 | −0.48 | −0.29 | −0.41 | −0.48 | −0.54 | |
| L5 | Init | −0.39 | −0.60 | −0.69 | −0.60 | 0.06 | −0.34 | −0.50 | −0.33 |
| COM DC I | −0.29 | −0.28 | −0.25 | −0.26 | −0.12 | −0.23 | −0.19 | −0.20 | |
| COM DC II | 0.20 | 0.28 | 0.25 | 0.11 | 0.08 | 0.30 | 0.22 | 0.06 | |
| Comp | −0.35 | −0.31 | −0.36 | −0.44 | −0.28 | −0.34 | −0.42 | −0.46 | |
| L6 | Init | −0.38 | −0.59 | −0.70 | −0.59 | 0.05 | −0.35 | −0.52 | −0.33 |
| COM DC I | −0.25 | −0.25 | −0.26 | −0.27 | −0.13 | −0.20 | −0.22 | −0.23 | |
| COM DC II | 0.00 | 0.04 | −0.05 | −0.11 | −0.13 | 0.05 | −0.06 | −0.10 | |
| Comp | −0.49 | −0.45 | −0.49 | −0.56 | −0.35 | −0.46 | −0.53 | −0.54 | |
 | RA | RF | |||||||
| Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | ||
| RA | Init | 1 | |||||||
| COM DC I | 0.83 | 1 | |||||||
| COM DC II | 0.53 | 0.75 | 1 | ||||||
| Comp | 0.68 | 0.53 | 0.58 | 1 | |||||
| RF | Init | 0.64 | 0.34 | 0.14 | 0.56 | 1 | |||
| COM DC I | 0.08 | 0.39 | 0.15 | −0.23 | 0.05 | 1 | |||
| COM DC II | −0.52 | −0.24 | −0.01 | −0.65 | −0.47 | 0.42 | 1 | ||
| Comp | 0.18 | −0.20 | −0.29 | 0.31 | 0.52 | −0.26 | −0.34 | 1 | |
| L1 | Init | −0.49 | −0.39 | −0.09 | −0.26 | −0.24 | 0.05 | 0.35 | −0.17 |
| COM DC I | −0.30 | −0.38 | −0.09 | −0.10 | −0.09 | −0.08 | 0.12 | 0.08 | |
| COM DC II | −0.13 | −0.06 | 0.04 | 0.15 | −0.03 | 0.00 | −0.18 | −0.02 | |
| Comp | −0.46 | −0.37 | −0.12 | −0.19 | −0.37 | −0.08 | 0.25 | −0.15 | |
| L2 | Init | 0.07 | 0.13 | 0.11 | 0.19 | 0.22 | 0.12 | −0.24 | −0.07 |
| COM DC I | 0.60 | 0.49 | 0.34 | 0.55 | 0.50 | 0.02 | −0.60 | 0.18 | |
| COM DC II | 0.54 | 0.47 | 0.23 | 0.48 | 0.45 | 0.06 | −0.62 | 0.12 | |
| Comp | 0.19 | −0.08 | −0.31 | 0.32 | 0.30 | −0.39 | −0.62 | 0.51 | |
| L3 | Init | 0.29 | 0.32 | 0.17 | 0.33 | 0.40 | 0.23 | −0.40 | 0.02 |
| COM DC I | 0.62 | 0.58 | 0.35 | 0.54 | 0.59 | 0.17 | −0.60 | 0.18 | |
| COM DC II | 0.46 | 0.44 | 0.19 | 0.35 | 0.46 | 0.10 | −0.53 | 0.09 | |
| Comp | 0.16 | −0.15 | −0.45 | 0.21 | 0.36 | −0.41 | −0.60 | 0.57 | |
| L4 | Init | −0.43 | −0.41 | −0.33 | −0.39 | −0.24 | 0.18 | 0.29 | 0.00 |
| COM DC I | −0.37 | −0.25 | −0.01 | −0.31 | −0.16 | 0.17 | 0.54 | −0.31 | |
| COM DC II | 0.51 | 0.23 | −0.04 | 0.35 | 0.60 | −0.01 | −0.46 | 0.41 | |
| Comp | −0.26 | −0.26 | −0.19 | −0.03 | 0.06 | 0.04 | −0.02 | 0.23 | |
| L5 | Init | −0.53 | −0.47 | −0.27 | −0.41 | −0.35 | 0.09 | 0.37 | −0.20 |
| COM DC I | −0.45 | −0.30 | −0.01 | −0.34 | −0.26 | 0.13 | 0.58 | −0.38 | |
| COM DC II | 0.48 | 0.24 | 0.08 | 0.40 | 0.59 | −0.05 | −0.45 | 0.32 | |
| Comp | −0.36 | −0.25 | −0.08 | −0.10 | −0.07 | 0.05 | 0.15 | −0.04 | |
| L6 | Init | −0.65 | −0.51 | −0.25 | −0.57 | −0.50 | 0.13 | 0.63 | −0.34 |
| COM DC I | −0.50 | −0.32 | −0.03 | −0.43 | −0.33 | 0.15 | 0.68 | −0.41 | |
| COM DC II | 0.49 | 0.27 | 0.15 | 0.40 | 0.61 | −0.07 | −0.44 | 0.27 | |
| Comp | −0.24 | −0.11 | 0.00 | −0.11 | −0.08 | −0.02 | 0.14 | −0.17 | |
 | RA | RF | |||||||
| Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | ||
| RA | Init | 1 | |||||||
| COM DC I | 0.60 | 1 | |||||||
| COM DC II | 0.27 | 0.81 | 1 | ||||||
| Comp | 0.22 | 0.60 | 0.71 | 1 | |||||
| RF | Init | 0.45 | 0.36 | 0.11 | 0.19 | 1 | |||
| COM DC I | 0.49 | 0.36 | 0.02 | 0.20 | 0.72 | 1 | |||
| COM DC II | −0.07 | 0.06 | 0.13 | 0.12 | 0.35 | 0.44 | 1 | ||
| Comp | −0.36 | −0.49 | −0.51 | −0.33 | −0.12 | −0.12 | 0.55 | 1 | |
| L1 | Init | −0.05 | −0.23 | −0.20 | −0.39 | −0.05 | −0.26 | −0.27 | −0.03 |
| COM DC I | 0.15 | 0.28 | 0.16 | 0.06 | 0.34 | −0.09 | −0.21 | −0.16 | |
| COM DC II | 0.32 | 0.25 | 0.10 | 0.12 | 0.31 | 0.25 | 0.04 | −0.12 | |
| Comp | 0.05 | −0.21 | −0.36 | −0.18 | 0.11 | 0.35 | 0.37 | 0.39 | |
| L2 | Init | 0.24 | −0.20 | −0.19 | −0.37 | −0.16 | −0.19 | −0.36 | −0.19 |
| COM DC I | 0.31 | 0.47 | 0.28 | 0.18 | 0.41 | 0.04 | −0.16 | −0.26 | |
| COM DC II | 0.41 | 0.35 | 0.20 | 0.15 | 0.28 | 0.24 | 0.04 | −0.18 | |
| Comp | 0.03 | −0.32 | −0.44 | −0.31 | −0.06 | 0.25 | 0.34 | 0.46 | |
| L3 | Init | 0.26 | −0.24 | −0.24 | −0.36 | −0.15 | 0.07 | 0.02 | −0.01 |
| COM DC I | 0.45 | 0.45 | 0.18 | 0.04 | 0.53 | 0.38 | 0.09 | −0.19 | |
| COM DC II | 0.41 | 0.30 | 0.18 | −0.07 | 0.31 | 0.28 | 0.20 | −0.09 | |
| Comp | 0.08 | −0.30 | −0.37 | −0.40 | −0.07 | 0.19 | 0.47 | 0.54 | |
| L4 | Init | −0.07 | 0.40 | 0.38 | 0.08 | 0.10 | −0.17 | −0.01 | −0.15 |
| COM DC I | 0.20 | 0.39 | 0.21 | 0.01 | 0.31 | 0.00 | −0.04 | −0.11 | |
| COM DC II | 0.26 | 0.38 | 0.34 | 0.18 | 0.25 | 0.27 | 0.08 | −0.35 | |
| Comp | −0.23 | −0.21 | −0.17 | −0.28 | −0.08 | 0.07 | 0.26 | 0.23 | |
| L5 | Init | −0.09 | 0.27 | 0.28 | 0.01 | 0.00 | −0.34 | −0.09 | −0.06 |
| COM DC I | 0.35 | 0.45 | 0.24 | 0.08 | 0.37 | 0.02 | −0.11 | −0.19 | |
| COM DC II | 0.42 | 0.35 | 0.21 | 0.12 | 0.33 | 0.28 | 0.00 | −0.30 | |
| Comp | −0.02 | −0.17 | −0.29 | −0.31 | 0.13 | 0.25 | 0.25 | 0.27 | |
| L6 | Init | −0.02 | 0.27 | 0.30 | −0.06 | −0.01 | −0.32 | −0.11 | −0.13 |
| COM DC I | 0.39 | 0.54 | 0.36 | 0.13 | 0.37 | 0.02 | −0.19 | −0.32 | |
| COM DC II | 0.44 | 0.33 | 0.17 | 0.09 | 0.34 | 0.38 | 0.03 | −0.31 | |
| Comp | 0.11 | −0.12 | −0.23 | −0.17 | 0.23 | 0.40 | 0.34 | 0.20 | |
 | RA | RF | |||||||
| Init | COM DC I | COM DC II | Comp | Init | COM DC I | COM DC II | Comp | ||
| RA | Init | 1 | |||||||
| COM DC I | 0.36 | 1 | |||||||
| COM DC II | 0.31 | 0.89 | 1 | ||||||
| Comp | −0.01 | 0.34 | 0.29 | 1 | |||||
| RF | Init | 0.59 | 0.30 | 0.20 | 0.11 | 1 | |||
| COM DC I | 0.36 | 0.17 | 0.30 | −0.25 | 0.17 | 1 | |||
| COM DC II | 0.05 | 0.35 | 0.54 | −0.19 | −0.02 | 0.66 | 1 | ||
| Comp | −0.16 | 0.44 | 0.44 | 0.12 | 0.06 | 0.14 | 0.45 | 1 | |
| L1 | Init | 0.00 | −0.31 | −0.13 | −0.14 | 0.04 | 0.13 | −0.03 | −0.16 |
| COM DC I | −0.19 | −0.10 | −0.07 | 0.12 | −0.25 | −0.07 | −0.07 | −0.13 | |
| COM DC II | 0.44 | 0.26 | 0.27 | 0.29 | 0.32 | −0.01 | −0.11 | −0.12 | |
| Comp | 0.45 | −0.05 | −0.07 | 0.26 | 0.23 | −0.28 | −0.48 | −0.44 | |
| L2 | Init | −0.01 | −0.32 | −0.12 | −0.23 | −0.23 | 0.16 | −0.08 | −0.06 |
| COM DC I | −0.30 | 0.20 | 0.18 | 0.16 | −0.33 | −0.01 | 0.03 | 0.35 | |
| COM DC II | 0.22 | 0.57 | 0.61 | 0.21 | 0.02 | 0.04 | 0.21 | 0.21 | |
| Comp | 0.25 | −0.13 | −0.15 | 0.35 | −0.13 | −0.34 | −0.50 | −0.31 | |
| L3 | Init | 0.11 | 0.01 | 0.19 | −0.03 | −0.15 | 0.16 | 0.04 | 0.17 |
| COM DC I | −0.13 | 0.40 | 0.34 | 0.31 | −0.05 | −0.02 | −0.04 | 0.47 | |
| COM DC II | 0.12 | 0.59 | 0.64 | 0.27 | 0.11 | −0.03 | 0.19 | 0.34 | |
| Comp | 0.20 | −0.14 | −0.18 | 0.50 | −0.07 | −0.26 | −0.43 | −0.26 | |
| L4 | Init | −0.34 | −0.13 | −0.01 | 0.18 | −0.06 | −0.12 | −0.02 | 0.08 |
| COM DC I | −0.26 | 0.23 | 0.23 | 0.33 | −0.13 | −0.06 | 0.18 | 0.10 | |
| COM DC II | −0.08 | 0.35 | 0.35 | 0.27 | −0.22 | −0.20 | 0.11 | −0.04 | |
| Comp | 0.14 | −0.22 | −0.31 | −0.23 | 0.03 | 0.05 | −0.18 | 0.11 | |
| L5 | Init | −0.25 | −0.27 | −0.14 | 0.04 | 0.04 | −0.03 | 0.04 | 0.09 |
| COM DC I | −0.14 | 0.22 | 0.30 | 0.28 | −0.04 | 0.08 | 0.31 | 0.25 | |
| COM DC II | 0.00 | 0.29 | 0.25 | 0.25 | −0.12 | −0.30 | −0.01 | −0.08 | |
| Comp | 0.12 | −0.19 | −0.26 | −0.17 | 0.04 | 0.01 | −0.15 | 0.18 | |
| L6 | Init | −0.17 | −0.24 | −0.09 | −0.07 | 0.01 | 0.12 | 0.18 | 0.11 |
| COM DC I | −0.21 | 0.01 | 0.24 | −0.02 | −0.21 | 0.42 | 0.48 | 0.30 | |
| COM DC II | 0.09 | 0.06 | 0.04 | −0.01 | −0.03 | −0.11 | −0.10 | −0.20 | |
| Comp | 0.00 | −0.22 | −0.23 | −0.24 | −0.11 | 0.16 | 0.03 | 0.26 | |
References
- Howe, J. The New Skiing Mechanics: Including the Technology of Short Radius Carved Turn Skiing and the Claw Ski; McIntire Publishing: Waterford, ME, USA, 2001. [Google Scholar]
- Federolf, P.; Roos, M.; Lüthi, A.; Dual, J. Finite element simulation of the ski–snow interaction of an alpine ski in a carved turn. Sport. Eng. 2010, 12, 123–133. [Google Scholar] [CrossRef]
- Kaps, P.; Mössner, M.; Nachbauer, W.; Stenberg, R. Pressure Distribution Under a Ski During Carved Turns. In Science and Skiing II; Müller, E., Schwameder, H., Raschner, C., Lindinger, S., Kornexl, E., Eds.; Kovac: Hamburg, Germany, 2001; pp. 180–202. [Google Scholar]
- Reid, R.C.; Haugen, P.; Gilgien, M.; Kipp, R.W.; Smith, G.A. Alpine Ski Motion Characteristics in Slalom. Front. Sport. Act. Living 2020, 2, 25. [Google Scholar] [CrossRef]
- LeMaster, R. Ultimate Skiing; Human Kinetics: Champaign, IL, USA, 2009. [Google Scholar]
- Zorko, M.; Nemec, B.; Babič, J.; Lešnik, B.; Supej, M. The waist width of skis influences the kinematics of the knee joint in alpine skiing. J. Sport. Sci. Med. 2015, 14, 606. [Google Scholar]
- Lieu, D.; Mote, C. Mechanics of the turning snow ski. In Skiing Trauma and Safety: Fifth International Symposium; Johnson, R.J., Mote, C.D., Eds.; ASTM Internationale: Philadelphia, PA, USA, 1985; Volume 1, pp. 117–140. [Google Scholar]
- Renshaw, A.A.; Mote, C., Jr. A model for the turning snow ski. Int. J. Mech. Sci. 1989, 31, 721–736. [Google Scholar] [CrossRef]
- Brown, C.; Outwater, J.O. On the skiability of snow. In Skiing Trauma and Safety: Seventh International Symposium, ASTM STP 1022; Johnson, R., Mote, C., Binet, M., Eds.; ASTM International: Philadelphia, PA, USA, 1989; Volume 1, pp. 329–336. [Google Scholar]
- Thorwartl, C.; Kröll, J.; Tschepp, A.; Holzer, H.; Teufl, W.; Stöggl, T. Validation of a Sensor-Based Dynamic Ski Deflection Measurement in the Lab and Proof-of-Concept Field Investigation. Sensors 2022, 22, 5768. [Google Scholar] [CrossRef]
- LeMaster, R. The Skier’s Edge; Human Kinetics: Champaign, IL, USA, 1999. [Google Scholar]
- Howe, J. Skiing Mechanics; Poudre Press: Laporte, CO, USA, 1983. [Google Scholar]
- Thorwartl, C.; Kröll, J.; Tschepp, A.; Schäffner, P.; Holzer, H.; Stöggl, T. A novel sensor foil to measure ski deflections: Development and validation of a curvature model. Sensors 2021, 21, 4848. [Google Scholar] [CrossRef] [PubMed]
- Falda-Buscaiot, T.; Hintzy, F.; Coulmy, N. Ground reaction force comparison between both feet during giant slalom turns in alpine skiing. In Proceedings of the 33rd International Conference on Biomechanics in Sports, Poitiers, France, 29 June–3 July 2015; pp. 560–563. [Google Scholar]
- Lamontagne, M. Plantar pressure distribution and forces measured during slalom and giant slalom turns performed by elite skiers. In Proceedings of the 19 International Symposium on Biomechanics in Sports, San Francisco, CA, USA, 20–26 June 2001; pp. 211–214. [Google Scholar]
- Falda-Buscaiot, T.; Hintzy, F.; Rougier, P.; Lacouture, P.; Coulmy, N. Influence of slope steepness, foot position and turn phase on plantar pressure distribution during giant slalom alpine ski racing. PLoS ONE 2017, 12, e0176975. [Google Scholar] [CrossRef] [PubMed]
- Stricker, G.; Scheiber, P.; Lindenhofer, E.; Müller, E. Determination of forces in alpine skiing and snowboarding: Validation of a mobile data acquisition system. Eur. J. Sport Sci. 2010, 10, 31–41. [Google Scholar] [CrossRef]
- Martínez, A.; Nakazato, K.; Scheiber, P.; Snyder, C.; Stöggl, T. Comparison of the turn switch time points measured by portable force platforms and pressure insoles. Front. Sport. Act. Living 2020, 2, 2. [Google Scholar] [CrossRef]
- Nakazato, K.; Scheiber, P.; Müller, E. A comparison of ground reaction forces determined by portable force-plate and pressure-insole systems in alpine skiing. J. Sport. Sci. Med. 2011, 10, 754. [Google Scholar]
- Nakazato, K.; Scheiber, P.; Müller, E. Comparison between the force application point determined by portable force plate system and the center of pressure determined by pressure insole system during alpine skiing. Sport. Eng. 2013, 16, 297–307. [Google Scholar] [CrossRef]
- Cross, M.R.; Delhaye, C.; Morin, J.-B.; Bowen, M.; Coulmy, N.; Hintzy, F.; Samozino, P. Force output in giant-slalom skiing: A practical model of force application effectiveness. PLoS ONE 2021, 16, e0244698. [Google Scholar] [CrossRef] [PubMed]
- Jentschura, U.D.; Fahrbach, F. Physics of skiing: The ideal carving equation and its applications. Can. J. Phys. 2004, 82, 249–261. [Google Scholar] [CrossRef]
- Supej, M.; Kipp, R.; Holmberg, H.C. Mechanical parameters as predictors of performance in alpine World Cup slalom racing. Scand. J. Med. Sci. Sport. 2011, 21, e72–e81. [Google Scholar] [CrossRef] [PubMed]
- Spörri, J.; Kröll, J.; Schwameder, H.; Müller, E. The role of path length-and speed-related factors for the enhancement of section performance in alpine giant slalom. Eur. J. Sport Sci. 2018, 18, 911–919. [Google Scholar] [CrossRef]
- Spörri, J.; Kröll, J.; Schwameder, H.; Müller, E. Turn characteristics of a top world class athlete in giant slalom: A case study assessing current performance prediction concepts. Int. J. Sport. Sci. Coach. 2012, 7, 647–659. [Google Scholar] [CrossRef]
- Martínez, Á.A.; Brunauer, R.; Venek, V.; Snyder, C.; Jahnel, R.; Buchecker, M.; Thorwartl, C.; Stöggl, T.L. Development and validation of a gyroscope-based turn detection algorithm for alpine skiing in the field. Front. Sport. Act. Living 2019, 1, 18. [Google Scholar] [CrossRef]
- Snyder, C.; Martínez, A.; Brunauer, R.; Stöggl, T. Validation of a wearable system for edge angle estimation during alpine skiing. In Science and Skiing VIII; Karczewska-Lindinger, M., Hakkarainen, A., Linnamo, V., Lindinger, S., Eds.; University of Jyväskylä: Jyväskylä, Finland, 2020. [Google Scholar]
- Snyder, C.; Martinez, A.; Strutzenberger, G.; Stöggl, T. Connected skiing: Validation of edge angle and radial force estimation as motion quality parameters during alpine skiing. Eur. J. Sport Sci. 2021, 22, 1484–1492. [Google Scholar] [CrossRef]
- Russo, C.; Puppo, E.; Roati, S.; Somà, A. Proposal of an Alpine Skiing Kinematic Analysis with the Aid of Miniaturized Monitoring Sensors, a Pilot Study. Sensors 2022, 22, 4286. [Google Scholar] [CrossRef]
- Spitzenpfeil, P.; Hummel, C.; Huber, A.; Waibel, K.; Menhorn, F.; Senf, B. DEFLECTION, TORSION AND EDGING MEASUREMENT IN ALPINE SKIING. In Proceedings of the 9th International Congress on Science and Skiing, Saalbach-Hinterglemm, Austria, 18–22 March 2023; p. 109. [Google Scholar]
- Thorwartl, C.; Holzer, H.; Kröll, J.; Tschepp, A.; Stöggl, T. Sensor-Based Curvature Detection of Alpine Skis. In Proceedings of the 24th International Congress on Snow Sport Trauma and Safety—37th Congress of the International Society for Snowsports Medicine, Mônetier-les-Bains, France, 27 March–2 April 2022; p. 58. [Google Scholar]
- Thorwartl, C.; Kröll, J.; Holzer, H.; Tschepp, A.; Stöggl, T. Sensor-Based analysis of Ski Deflection in the Field. In Proceedings of the 2nd Conference for Smart Materials, Salzburg, Austria, 12–15 September 2022; p. 35. [Google Scholar]
- Thorwartl, C.; Kröll, J.; Lasshofer, M.; Holzer, H.; Tschepp, A.; Stöggl, T. Relationship between local ski bending curvature, lean angle and radial force in alpine skiing. In Proceedings of the 14th Symposium of the Section Sport Informatics and Engineering of the German Society of Sport Science (dvs), Chemnitz, Germany, 29–30 September 2022; pp. 63–67. [Google Scholar]
- Thorwartl, C.; Tschepp, A.; Lasshofer, M.; Holzer, H.; Stöggl, T. Analysis of Ski-Snow Interaction in Relation to Lean Angle, Radial Force and Curvature in Soft and Hard Slope Conditions. In Proceedings of the 9th International Congress on Science and Skiing, Saalbach-Hinterglemm, Austria, 18–22 March 2023; pp. 12–13. [Google Scholar]
- Spörri, J.; Kröll, J.; Schwameder, H.; Schiefermüller, C.; Müller, E. Course setting and selected biomechanical variables related to injury risk in alpine ski racing: An explorative case study. Br. J. Sport. Med. 2012, 46, 1072–1077. [Google Scholar] [CrossRef]
- Hopkins, W.; Marshall, S.; Batterham, A.; Hanin, J. Progressive statistics for studies in sports medicine and exercise science. Med. + Sci. Sport. + Exerc. 2009, 41, 3. [Google Scholar] [CrossRef] [PubMed]
- Gosselin, P.; Truong, J.; Chapdelaine, C.; Guilbert, J.-S.; St-Pierre, É.; Trahan, X.; Lussier Desbiens, A. Effect of edged snow contact on the vibration of alpine skis. Sport. Eng. 2021, 24, 1–13. [Google Scholar] [CrossRef]
- Bon, I.; Očić, M.; Cigrovski, V.; Rupčić, T.; Knjaz, D. What are kinematic and kinetic differences between short and parallel turn in Alpine skiing? Int. J. Environ. Res. Public Health 2021, 18, 3029. [Google Scholar] [CrossRef] [PubMed]
- Vaverka, F.; Vodickova, S.; Elfmark, M. Kinetic analysis of ski turns based on measured ground reaction forces. J. Appl. Biomech. 2012, 28, 41–47. [Google Scholar] [CrossRef] [PubMed]
- Yoneyama, T.; Kitade, M.; Osada, K. Investigation on the ski-snow interaction in a carved turn based on the actual measurement. Procedia Eng. 2010, 2, 2901–2906. [Google Scholar] [CrossRef]
- Tatsuno, D.; Yoneyama, T.; Kagawa, H.; Scott, N.; Osada, K. Measurement of Ski Deflection and Ski-Snow Contacting Pressure in an Actual Ski Turn on the Snow Surface; Aachen Meyer & Meyer: Aachen, Germany, 2009. [Google Scholar]
- Yoneyama, T.; Scott, N.; Kagawa, H.; Osada, K. Ski deflection measurement during skiing and estimation of ski direction and edge angle. Sport. Eng. 2008, 11, 3–13. [Google Scholar] [CrossRef]
- Adelsberger, R.; Aufdenblatten, S.; Gilgien, M.; Tröster, G. On bending characteristics of skis in use. Procedia Eng. 2014, 72, 362–367. [Google Scholar] [CrossRef]




| Carving | Parallel Ski Steering | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| L1 | L2 | L3 | L4 | L5 | L6 | L1 | L2 | L3 | L4 | L5 | L6 | |||
 | Initiation | L1 | 1 | 1 | ||||||||||
| L2 | 0.48 | 1 | 0.77 | 1 | ||||||||||
| L3 | 0.15 | 0.88 | 1 | 0.38 | 0.64 | 1 | ||||||||
| L4 | 0.11 | 0.06 | 0.06 | 1 | 0.47 | 0.26 | 0.37 | 1 | ||||||
| L5 | 0.23 | 0.03 | −0.02 | 0.90 | 1 | 0.56 | 0.34 | 0.24 | 0.76 | 1 | ||||
| L6 | 0.35 | −0.16 | −0.27 | 0.70 | 0.89 | 1 | 0.73 | 0.48 | 0.26 | 0.67 | 0.93 | 1 | ||
| COM DC I | L1 | 1 | 1 | |||||||||||
| L2 | 0.10 | 1 | 0.67 | 1 | ||||||||||
| L3 | −0.08 | 0.93 | 1 | 0.18 | 0.70 | 1 | ||||||||
| L4 | 0.21 | −0.44 | −0.41 | 1 | 0.05 | 0.13 | 0.25 | 1 | ||||||
| L5 | 0.22 | −0.51 | −0.52 | 0.94 | 1 | 0.23 | 0.29 | 0.23 | 0.72 | 1 | ||||
| L6 | 0.19 | −0.61 | −0.63 | 0.85 | 0.96 | 1 | 0.37 | 0.35 | 0.15 | 0.38 | 0.68 | 1 | ||
| COM DC II | L1 | 1 | 1 | |||||||||||
| L2 | 0.30 | 1 | 0.70 | 1 | ||||||||||
| L3 | 0.16 | 0.94 | 1 | 0.48 | 0.78 | 1 | ||||||||
| L4 | −0.18 | 0.50 | 0.51 | 1 | 0.42 | 0.61 | 0.47 | 1 | ||||||
| L5 | −0.10 | 0.54 | 0.57 | 0.96 | 1 | 0.49 | 0.53 | 0.35 | 0.91 | 1 | ||||
| L6 | −0.12 | 0.50 | 0.56 | 0.92 | 0.98 | 1 | 0.50 | 0.30 | 0.13 | 0.65 | 0.80 | 1 | ||
| Completion | L1 | 1 | 1 | |||||||||||
| L2 | 0.07 | 1 | 0.79 | 1 | ||||||||||
| L3 | −0.10 | 0.93 | 1 | 0.70 | 0.89 | 1 | ||||||||
| L4 | 0.08 | 0.05 | 0.01 | 1 | 0.21 | 0.22 | 0.18 | 1 | ||||||
| L5 | 0.18 | −0.18 | −0.23 | 0.92 | 1 | 0.17 | 0.18 | 0.15 | 0.96 | 1 | ||||
| L6 | −0.02 | −0.24 | −0.22 | 0.75 | 0.88 | 1 | 0.07 | 0.10 | 0.04 | 0.86 | 0.90 | 1 | ||
| Initiation | L1 | 1 | 1 | |||||||||||
| L2 | 0.24 | 1 | 0.67 | 1 | ||||||||||
| L3 | −0.21 | 0.79 | 1 | 0.35 | 0.77 | 1 | ||||||||
| L4 | 0.10 | 0.01 | −0.09 | 1 | −0.03 | −0.35 | −0.34 | 1 | ||||||
| L5 | 0.19 | −0.06 | −0.20 | 0.96 | 1 | 0.12 | −0.24 | −0.31 | 0.90 | 1 | ||||
| L6 | 0.20 | −0.07 | −0.21 | 0.90 | 0.97 | 1 | 0.23 | −0.06 | −0.14 | 0.80 | 0.91 | 1 | ||
| COM DC I | L1 | 1 | 1 | |||||||||||
| L2 | −0.07 | 1 | 0.95 | 1 | ||||||||||
| L3 | −0.22 | 0.94 | 1 | 0.73 | 0.81 | 1 | ||||||||
| L4 | 0.22 | −0.10 | −0.15 | 1 | 0.66 | 0.67 | 0.70 | 1 | ||||||
| L5 | 0.20 | −0.07 | −0.14 | 0.94 | 1 | 0.76 | 0.79 | 0.76 | 0.96 | 1 | ||||
| L6 | 0.15 | −0.04 | −0.11 | 0.80 | 0.93 | 1 | 0.82 | 0.85 | 0.75 | 0.87 | 0.95 | 1 | ||
| COM DC II | L1 | 1 | 1 | |||||||||||
| L2 | 0.56 | 1 | 0.93 | 1 | ||||||||||
| L3 | 0.48 | 0.98 | 1 | 0.62 | 0.75 | 1 | ||||||||
| L4 | 0.28 | 0.54 | 0.53 | 1 | 0.77 | 0.76 | 0.54 | 1 | ||||||
| L5 | 0.33 | 0.51 | 0.51 | 0.90 | 1 | 0.84 | 0.83 | 0.54 | 0.91 | 1 | ||||
| L6 | 0.24 | 0.23 | 0.24 | 0.70 | 0.87 | 1 | 0.85 | 0.81 | 0.48 | 0.85 | 0.95 | 1 | ||
| Completion | L1 | 1 | 1 | |||||||||||
| L2 | 0.41 | 1 | 0.89 | 1 | ||||||||||
| L3 | 0.15 | 0.89 | 1 | 0.68 | 0.83 | 1 | ||||||||
| L4 | 0.04 | −0.11 | 0.02 | 1 | 0.71 | 0.60 | 0.44 | 1 | ||||||
| L5 | 0.16 | 0.02 | 0.13 | 0.96 | 1 | 0.77 | 0.62 | 0.37 | 0.84 | 1 | ||||
| L6 | 0.22 | −0.09 | −0.03 | 0.87 | 0.93 | 1 | 0.84 | 0.66 | 0.45 | 0.80 | 0.93 | 1 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thorwartl, C.; Tschepp, A.; Lasshofer, M.; Holzer, H.; Zirkl, M.; Hammer, M.; Stadlober, B.; Stöggl, T. Technique-Dependent Relationship between Local Ski Bending Curvature, Roll Angle and Radial Force in Alpine Skiing. Sensors 2023, 23, 3997. https://doi.org/10.3390/s23083997
Thorwartl C, Tschepp A, Lasshofer M, Holzer H, Zirkl M, Hammer M, Stadlober B, Stöggl T. Technique-Dependent Relationship between Local Ski Bending Curvature, Roll Angle and Radial Force in Alpine Skiing. Sensors. 2023; 23(8):3997. https://doi.org/10.3390/s23083997
Chicago/Turabian StyleThorwartl, Christoph, Andreas Tschepp, Michael Lasshofer, Helmut Holzer, Martin Zirkl, Matthias Hammer, Barbara Stadlober, and Thomas Stöggl. 2023. "Technique-Dependent Relationship between Local Ski Bending Curvature, Roll Angle and Radial Force in Alpine Skiing" Sensors 23, no. 8: 3997. https://doi.org/10.3390/s23083997
APA StyleThorwartl, C., Tschepp, A., Lasshofer, M., Holzer, H., Zirkl, M., Hammer, M., Stadlober, B., & Stöggl, T. (2023). Technique-Dependent Relationship between Local Ski Bending Curvature, Roll Angle and Radial Force in Alpine Skiing. Sensors, 23(8), 3997. https://doi.org/10.3390/s23083997






