Measurements of Small Frequency Differences by Dual Mode 4 MHz Quartz Sensors
Abstract
1. Introduction
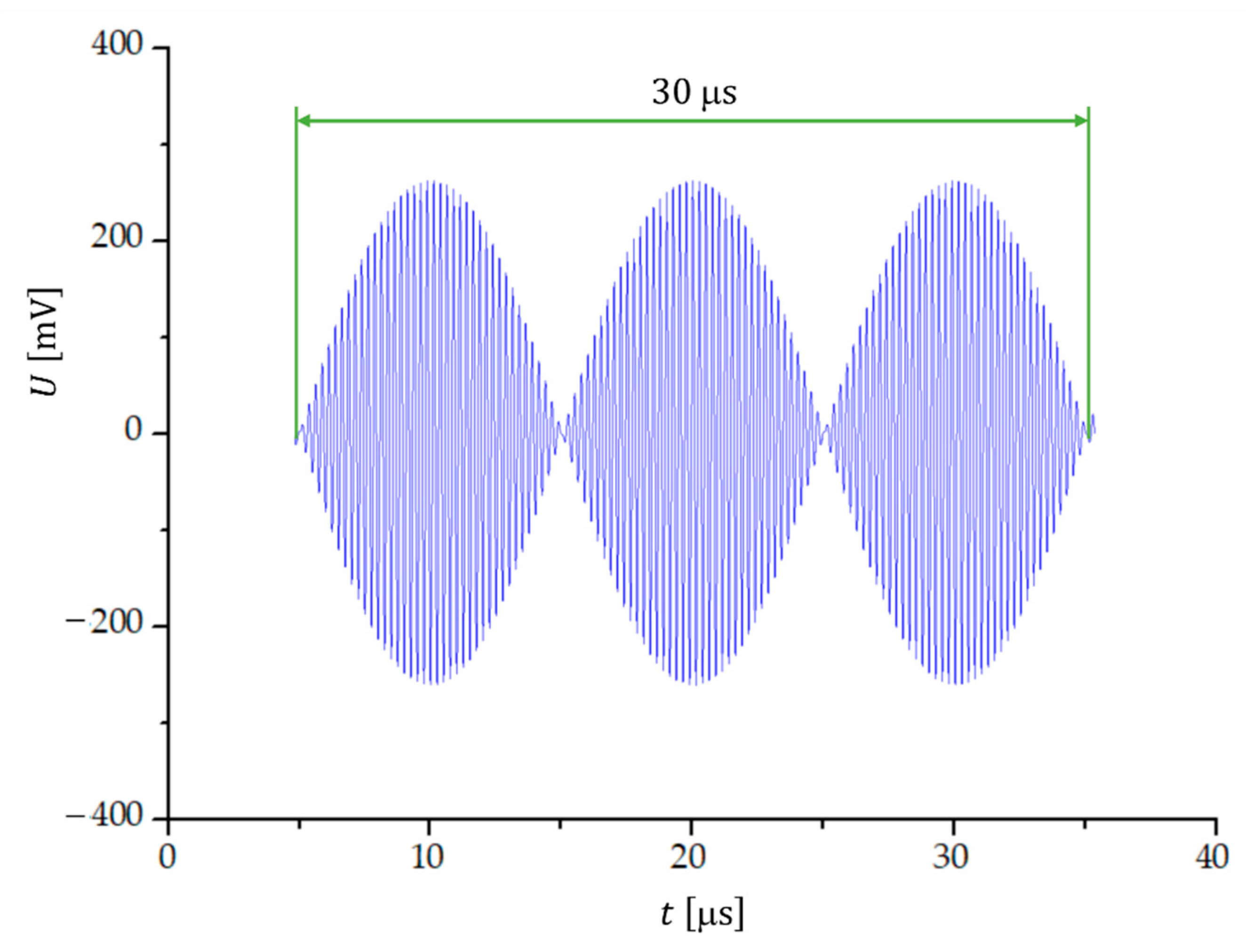
2. Obtaining the Frequency Difference from One Beat Period
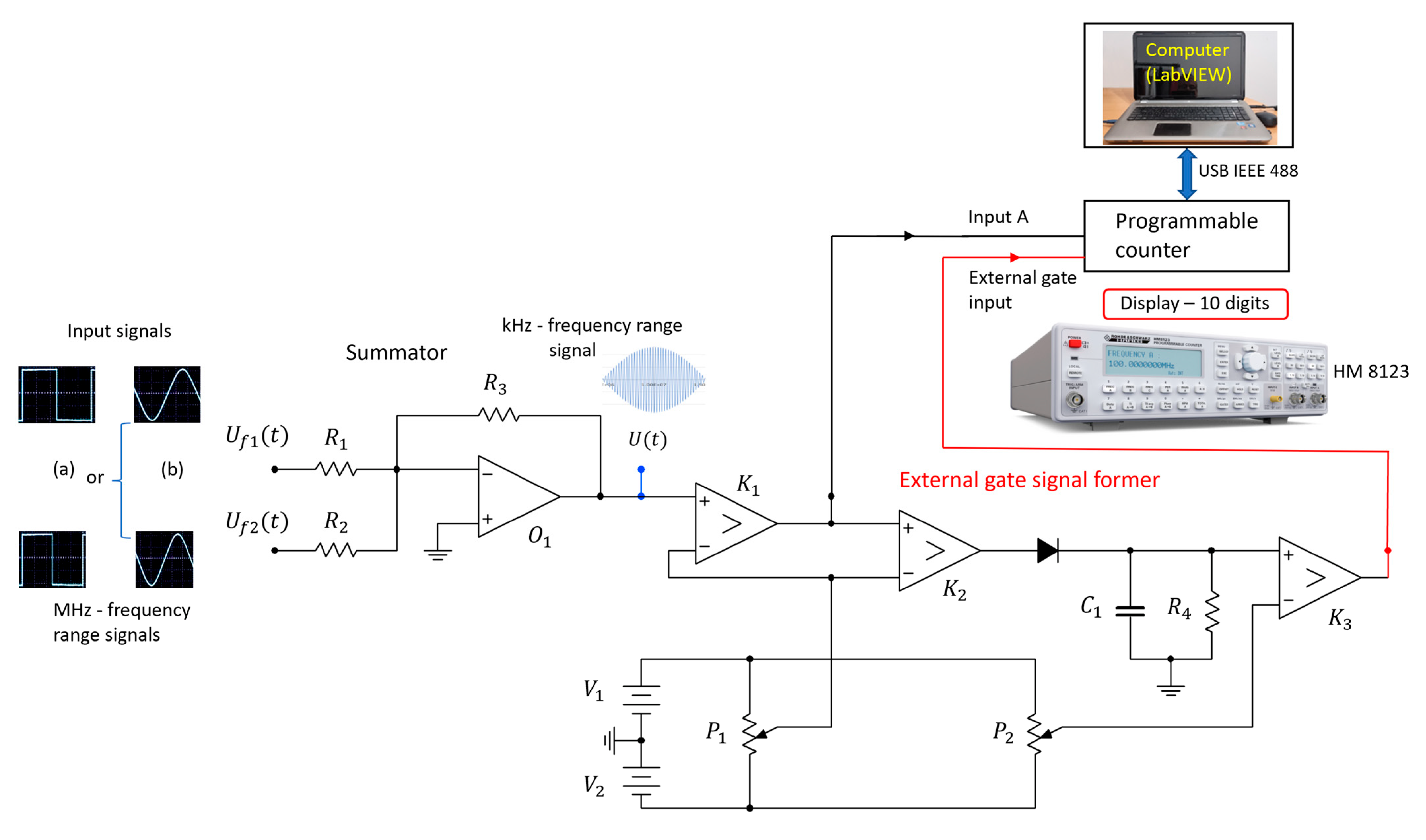
3. Experimental Setup
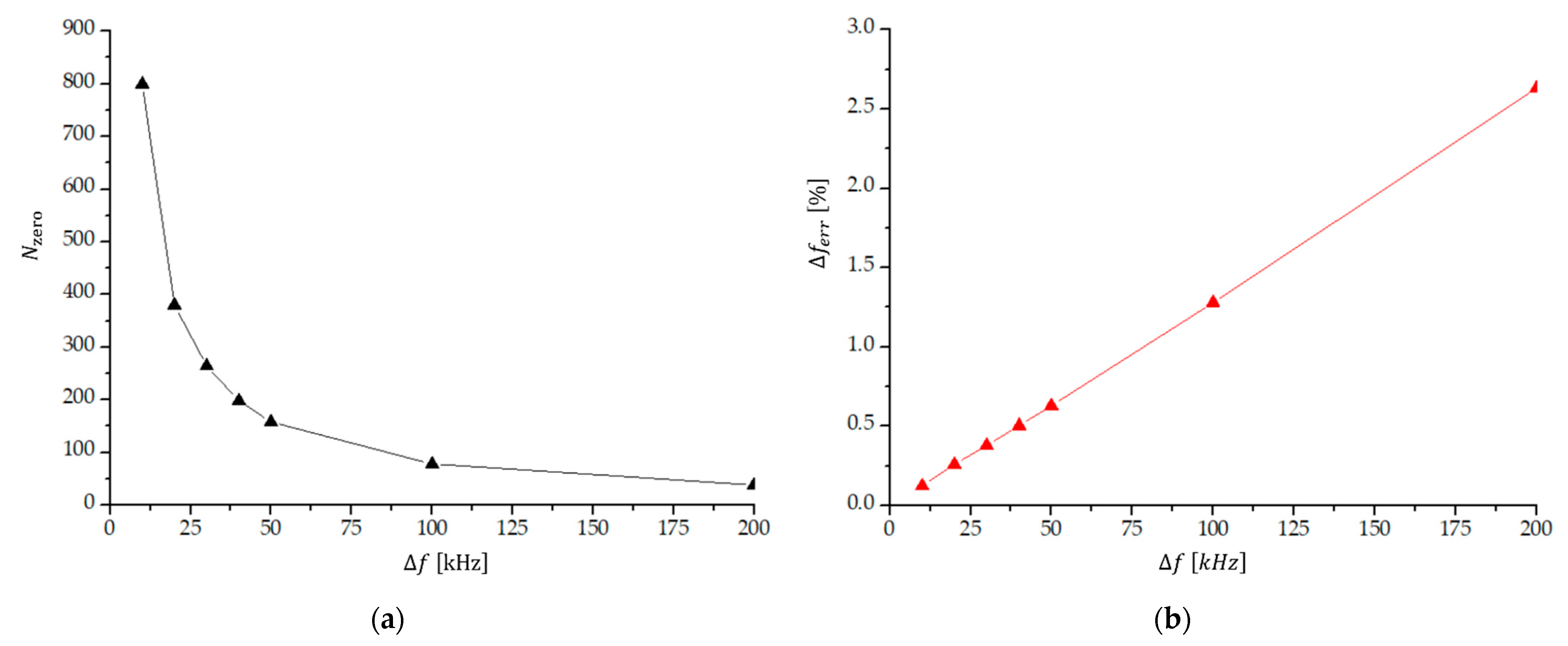
4. Results and Discussion
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Burda, I. Quartz Crystal Microbalance with Impedance Analysis Based on Virtual Instruments: Experimental Study. Sensors 2022, 22, 1506. [Google Scholar] [CrossRef] [PubMed]
- Rodriguez-Pardo, L.; Fariña, J.; Gabrielli, C.; Perrot, H.; Brendel, R. Simulation of QCM sensors based on high stability classical oscillator configurations in damping media. Sens. Actuators B Chem. 2007, 123, 560–567. [Google Scholar] [CrossRef]
- Xu, F.; Sun, L.; Huang, P.; Sun, Y.; Zheng, Q.; Zou, Y.; Chu, H.; Yan, E.; Zhang, H.; Wang, J.; et al. A pyridine vapor sensor based on metal-organic framework-modified quartz crystal microbalance. Sens. Actuators B Chem. 2018, 254, 872–877. [Google Scholar] [CrossRef]
- Matko, V.; Milanovič, M. Sensitivity and accuracy of dielectric measurements of liquids significantly improved by coupled capacitive-dependent quartz crystals. Sensors 2021, 21, 3565. [Google Scholar] [CrossRef] [PubMed]
- Fort, A.; Landi, E.; Grasso, A.L.; Mugnaini, M.; Panzardi, E.; Vaccarella, P.; Vignoli, V. QCM Measurements in Newtonian Liquids: Problems and Performance Analysis. IEEE Trans. Instrum. Meas. 2022, 71, 1–13. [Google Scholar] [CrossRef]
- Wasserman, G.S. Reliability Verification, Testing, and Analysis in Engineering Design; Marcel Dekker, Inc.: New York, NY, USA; Basel, Switzerland, 2003. [Google Scholar]
- Walls, F.L.; Vig, J.R. Fundamental limits on the frequency stabilities of crystal oscillators. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1995, 42, 576–589. [Google Scholar] [CrossRef]
- Kakalis, A.; Panayiotou, C. The temperature effect of AT-cut input quartz parameters on QCM effective properties calculated with equivalent circuit models. J. Electroceram. 2017, 40, 23–35. [Google Scholar] [CrossRef]
- Filler, R.L.; Vig, J.R. Long-term aging of oscillators. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1993, 40, 387–394. [Google Scholar] [CrossRef]
- Ferrari, V.; Marioli, D.; Taroni, A. Improving the accuracy and operating range of quartz microbalance sensors by a purposely designed oscillator circuit. IEEE Trans. Instrum. Meas. 2001, 50, 1119–1122. [Google Scholar] [CrossRef]
- Matko, V.; Milanovic, M. High Resolution Switching Mode Inductance-to-Frequency Converter with Temperature Compensation. Sensors 2014, 14, 19242–19259. [Google Scholar] [CrossRef]
- Statek. The Quartz Crystal Model and Its Frequencies. Technical Note 32. Available online: http://statek.com/wp-content/uploads/2018/03/tn32.pdf (accessed on 25 February 2023).
- Euroquartz. Crystal Theory. Available online: https://euroquartz.co.uk/media/1879/tech-notes.pdf (accessed on 26 February 2023).
- Stanford. QCM100-Quartz Microbalance Theory and Calibration. Available online: http://www.thinksrs.com/downloads/PDFs/ApplicationNotes/QCMTheoryapp.pdf (accessed on 26 February 2023).
- Matko, V. Next generation AT-cut quartz crystal sensing devices. Sensors 2011, 11, 4474–4482. [Google Scholar] [CrossRef]
- Laflamme, S.; Saleem, H.S.; Vasan, B.K.; Geiger, R.L.; Degang, C.; Kessler, M.R.; Rajan, K. Soft Elastomeric Capacitor Network for Strain Sensing Over Large Surfaces. IEEE/ASME Trans. Mechatron. 2013, 18, 1647–1654. [Google Scholar] [CrossRef]
- Kenton, B.J.; Leang, K.K. Design and Control of a Three-Axis Serial-Kinematic High-Bandwidth Nanopositioner. IEEE/ASME Trans. Mechatron. 2012, 17, 356–369. [Google Scholar] [CrossRef]
- Cheng, M.H.; Chiu, G.T.C.; Franchek, M.A. Real-Time Measurement of Eccentric Motion With Low-Cost Capacitive Sensor. IEEE/ASME Trans. Mechatron. 2013, 18, 990–997. [Google Scholar] [CrossRef]
- Camesano, T.A.; Liu, Y.; Datta, M. Measuring bacterial adhesion at environmental interfaces with single-cell and single-molecule techniques. Adv. Water Resour. 2007, 30, 1470–1491. [Google Scholar] [CrossRef]
- Yuen Kuan, Y.; Fleming, A.J.; Moheimani, S.O.R. A Novel Piezoelectric Strain Sensor for Simultaneous Damping and Tracking Control of a High-Speed Nanopositioner. IEEE/ASME Trans. Mechatron. 2013, 18, 1113–1121. [Google Scholar]
- Liu, H.; Cheng, J.; Yang, J.; Rao, Z.; Cheng, G.; Yang, S.; Huang, X.; Wang, M. Concept and evaluation of a new piezoelectric transducer for an implantable middle ear hearing device. Sensors 2017, 17, 2515. [Google Scholar] [CrossRef]
- Minin, I.V.; Minin, O.V.; Castiñeira-Ibáñez, S.; Rubio, C.; Candelas, P. Phase method for visualization of hidden dielectric objects in the millimeter waveband. Sensors 2019, 19, 3919. [Google Scholar] [CrossRef]
- Wang, W.; Thomas, P.J. Low-frequency active noise control of an underwater large-scale structure with distributed giant magnetostrictive actuators. Sens. Actuators A Phys. 2017, 263, 113–121. [Google Scholar] [CrossRef]
- Hug, T.S. Biophysical methods for monitoring cell-substrate interactions in drug discovery. Assay Drug Dev. Technol. 2003, 1, 479–488. [Google Scholar] [CrossRef]
- Ferrari, M.; Ferrari, V.; Marioli, D.; Taroni, A.; Suman, M.; Dalcanale, E. In-liquid sensing of chemical compounds by QCM sensors coupled with high-accuracy ACC oscillator. IEEE Trans. Instrum. Meas. 2006, 55, 828–834. [Google Scholar] [CrossRef]
- Kößlinger, C.; Drost, S.; Aberl, F.; Wolf, H.; Koch, S.; Woias, P. A quartz crystal biosensor for measurement in liquids. Biosens. Bioelectron. 1992, 7, 397–404. [Google Scholar] [CrossRef] [PubMed]
- Dickert, F.L.; Lieberzeit, P.; Hayden, O. Sensor strategies for microorganism detection—From physical principles to imprinting procedures. Anal. Bioanal. Chem. 2003, 377, 540–549. [Google Scholar] [CrossRef] [PubMed]
- Sönmezler, M.; Özgür, E.; Yavuz, H.; Denizli, A. Quartz crystal microbalance based histidine sensor. Artif. Cells Nanomed. Biotechnol. 2019, 47, 221–227. [Google Scholar] [CrossRef]
- Matko, V.; Jezernik, K. Greatly Improved Small Inductance Measurement Using Quartz Crystal Parasitic Capacitance Compensation. Sensors 2010, 10, 3954–3960. [Google Scholar] [CrossRef]
- Matko, V.; Milanovič, M. Detection principles of temperature compensated oscillators with reactance influence on piezoelectric resonator. Sensors 2020, 20, 802. [Google Scholar] [CrossRef]
- Bandey, H.L.; Martin, S.J.; Cernosek, R.W.; Hillman, A.R. Modeling the Responses of Thickness-Shear Mode Resonators under Various Loading Conditions. Anal. Chem. 1999, 71, 2205–2214. [Google Scholar] [CrossRef]
- Arnau, A. A review of interface electronic systems for AT-cut quartz crystal microbalance applications in liquids. Sensors 2008, 8, 370–411. [Google Scholar] [CrossRef]
- Ni, R.; Zhang, X.-B.; Liu, W.; Shen, G.-L.; Yu, R.-Q. Piezoelectric quartz crystal sensor array with optimized oscillator circuit for analysis of organic vapors mixtures. Sens. Actuators B Chem. 2003, 88, 198–204. [Google Scholar] [CrossRef]
- Rodriguez-Pardo, L.; Fariña, J.; Gabrielli, C.; Perrot, H.; Brendel, R. Resolution in quartz crystal oscillator circuits for high sensitivity microbalance sensors in damping media. Sens. Actuators B Chem. 2004, 103, 318–324. [Google Scholar] [CrossRef]
- Yipeng, L.; Horsley, D.A. Modeling, Fabrication, and Characterization of Piezoelectric Micromachined Ultrasonic Transducer Arrays Based on Cavity SOI Wafers. J. Microelectromech. Syst. 2015, 24, 1142–1149. [Google Scholar]
- Ramanathan, P.; Ramasamy, S.; Jain, P.; Nagrecha, H.; Paul, S.; Arulmozhivarman, P.; Tatavarti, R. Low Value Capacitance Measurements for Capacitive Sensors—A Review. Sens. Transducers 2013, 148, 1. [Google Scholar]
- Marioli, D.; Sardini, E.; Taroni, A. Measurement of small capacitance variations. IEEE Trans. Instrum. Meas. 1991, 40, 426–428. [Google Scholar] [CrossRef]
- Khan, F.; Younis, M.I. RF MEMS electrostatically actuated tunable capacitors and their applications: A review. J. Micromech. Microeng. 2021, 32, 13002–13021. [Google Scholar] [CrossRef]
- Ferlito, U.; Grasso, A.D.; Pennisi, S.; Vaiana, M.; Bruno, G. Sub-Femto-Farad Resolution Electronic Interfaces for Integrated Capacitive Sensors: A Review. IEEE Access 2020, 8, 153969–153980. [Google Scholar] [CrossRef]
- Matko, V. Multiple Quartz Crystals Connected in Parallel for High-Resolution Sensing of Capacitance Changes. Sensors 2022, 22, 5030. [Google Scholar] [CrossRef]
- Hameg. HM8123, Programmable Counter, Technical Data. Available online: https://www.farnell.com/datasheets/2787933.pdf (accessed on 27 February 2023).
- PIC16F877A. Available online: https://www.alldatasheet.com/datasheet-pdf/pdf/560054/MICROCHIP/PIC16F877A-E.html (accessed on 2 February 2023).
- Metzler, J. Crystal Basic. Application Note. Available online: https://www.google.com/url?sa=t&rct=j&q=&esrc=s&source=web&cd=&ved=2ahUKEwjrh4vn2rX9AhUDyqQKHXKHBnEQFnoECCsQAQ&url=https%3A%2F%2Fwww.ctscorp.com%2Fwp-content%2Fuploads%2FAppnote-Crystal-Basics.pdf&usg=AOvVaw0CppOl71v4bgFJ3E-KASPo (accessed on 27 February 2023).





| (%) | |||||
|---|---|---|---|---|---|
| 1 | 25.000000000 | 3.987500000000 | 4.012500000000 | 320 | 0.31250 |
| 2 | 12.500000000 | 3.993750000000 | 4.006250000000 | 640 | 0.15625 |
| 3 | 6.250000000 | 3.996875000000 | 4.003125000000 | 1280 | 0.07813 |
| 4 | 3.125000000 | 3.998437500000 | 4.001562500000 | 2560 | 0.03906 |
| 5 | 1.562500000 | 3.999218750000 | 4.000781250000 | 5120 | 0.01953 |
| 6 | 0.781250000 | 3.999609375000 | 4.000390625000 | 10240 | 0.00976 |
| 7 | 0.390625000 | 3.999804687500 | 4.000195312500 | 20480 | 0.00488 |
| 8 | 0.195312500 | 3.999902343750 | 4.000097656250 | 40960 | 0.00244 |
| 9 | 0.097656250 | 3.999951171875 | 4.000048828125 | 81920 | 0.00122 |
| 10 | 0.048828125 | 3.999975585937 | 4.000024414063 | 163840 | 0.00061 |
| 11 | 0.024414063 | 3.999987792969 | 4.000012207031 | 327680 | 0.00031 |
| 12 | 0.012207030 | 3.999993896485 | 4.000006103515 | 655360 | 0.00016 |
| 13 | 0.006103515 | 3.999996948243 | 4.000003051757 | 1310720 | 0.00008 |
| 14 | 0.003051756 | 3.999998474122 | 4.000001525878 | 2621440 | 0.00004 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Matko, V. Measurements of Small Frequency Differences by Dual Mode 4 MHz Quartz Sensors. Sensors 2023, 23, 3220. https://doi.org/10.3390/s23063220
Matko V. Measurements of Small Frequency Differences by Dual Mode 4 MHz Quartz Sensors. Sensors. 2023; 23(6):3220. https://doi.org/10.3390/s23063220
Chicago/Turabian StyleMatko, Vojko. 2023. "Measurements of Small Frequency Differences by Dual Mode 4 MHz Quartz Sensors" Sensors 23, no. 6: 3220. https://doi.org/10.3390/s23063220
APA StyleMatko, V. (2023). Measurements of Small Frequency Differences by Dual Mode 4 MHz Quartz Sensors. Sensors, 23(6), 3220. https://doi.org/10.3390/s23063220






